Sealability Analyses of Premium Connections Characterized by a Surface Fractal Function

Abstract

With the development of high-pressure, high-temperature wells, the sealability of premium connections is being threatened by heavy loads. For the sake of researching the sealability of premium connections at the microlevel on the sealing surface, a rough profile was characterized according to a fractal function, and the influence of the fractal parameters of contact behavior on the rough surface was analyzed. A full-size premium connection model with a fractal surface FEM was established using ABAQUS software to analyze the Von Mises stress and contact pressure, and the sealability was then analyzed using contact strength theory. It was found that utilizing fractal functions to describe the rough morphology on the sealing surface of premium connections could reveal the contact behavior of the sealing surface more realistically. The premium connection’s fractal FEM was closer to the actual situation, considering the effect of the asperities’ elastic–plastic deformation on the sealability. The fractal dimension D had a greater influence on the contact area and contact pressure than the scale coefficient G. When the fractal dimension D was less than 2.5, the maximum Von Mises stress and maximum contact pressure on the sealing surface were 8.81 × 10⁸ Pa and 1.20 × 10⁹ Pa, respectively, making the surface prone to gluing. The sealability of the premium connection was primarily affected by the axial tension. When the axial tension was 1.2 × 10⁶ N, the sealing surface experienced significant displacement along the axial direction, the effective contact length reduced from 2.72 × 10⁻³ m to 2.24 × 10⁻³ m, and the maximum contact pressure reduced from 8.10 × 10⁸ Pa to 6.39 × 10⁸ Pa, which led to a 30% decrease in sealing strength, which may pose a high risk of sealing failure. When the internal pressure reached 1 × 10⁸ Pa, the effective contact length increased from 9.08 × 10⁻³ m to 1.06 × 10⁻² m, the maximum contact pressure increased from 8.67 × 10⁸ Pa to 1.37 × 10⁹ Pa, and the sealing strength increased by 23%.

1. Introduction

In high-pressure, high-temperature (HPHT) wells with pressures greater than 7 × 10⁷ Pa and temperatures greater than 150 °C, premium connections are used to connect the tubing to several kilometers of string [1], as shown in Figure 1, forming a underground channel for oil and gas. The heavy downhole loads greatly threaten the sealability of premium connections. A premium connection, as shown in Figure 2, contains threads, sealing surfaces and a torque shoulder. The sealability of a premium connection is guaranteed by two metal sealing surfaces with an interference fit. The sealing surfaces consist of a large number of asperities with different diameters. When in contact, the actual contact area between the two sealing surfaces is significantly lower than the nominal contact area [2]. Additionally, the contact pressure on the surface also changes significantly, which affects the sealability of the premium connection.

Previous studies on the sealability of premium connections have mostly been macroscopic, assuming that the sealing surface is smooth in analyzing the stress, contact pressure, contact area or contact length. Considering the roughness of premium connections, Zhang Ying established a gas leakage rate model of a cone–cone premium connection, and investigated the impact of sealing surface roughness on the leakage rate of the premium connection [3]. Wang and Shen et al. established a two-dimensional (2D) finite element model (FEM) of premium connections with two sealing structures, and verified the accuracy of the simplified symmetry model through experiments [4,5]. Sches et al. carried out tests on a full-size premium connection and determined the optimal structural size according to the wear resistance, internal pressure strength and outer pressure resistance [6]. Man et al. established a three-dimensional (3D) FEM of premium connections [7,8] that consider the structure of the sealing surface, downhole conditions, and loads, and obtained the stress, contact pressure, and contact length. In addition, Murtatisu suggested a method to assess the sealing performance via the sealing strength [9]. Through physical tests and numerical simulation methods, the sealing strength and critical sealing strength were derived via the functional relationship between sealability and contact pressure [10,11,12,13]. Previous research has neglected the effect of changes in the real contact area and contact pressure due to elastic and plastic deformation of asperities on the sealability of the sealing surface upon contact.

The metal sealing surface is composed of a great number of asperities of different sizes. The profile of the rough surface can be described using either a statistical model or a fractal model. The statistical model mainly depends on the statistical parameters of the rough surface. Additionally, the statistical parameters are affected by the instrument’s resolution and the sampling length, so they cannot accurately characterize all the features of the rough surface. However, this problem can be solved using a fractal model. The rough surface described by the fractal model is characterized by continuity, scale independence and self-similarity; thus, it objectively represents the characteristics of the rough surface [14]. The concept of fractals was first proposed by Mandelbrot, who discovered fractal geometry, which applies to objects with natural forms, no specific proportions and sizes, and infinite detail. Since then, the Weierstrass–Mandelbrot fractal function (W-M function) has been widely used in the study of tribology [15]. Majumdar and Bhushan applied the W-M function to analyze the contact behavior of two rough surfaces, established Majumdar–Bhushan (M-B) fractal contact theory, and obtained expressions for the contact load and contact area using fractal parameters [16]. Jourani et al. established a 3D quantitative model utilizing the W-M function to examine the impact of 3D fractals on both contact area and surface roughness [17]. Due to the complex structure of premium connections, the length of the sealing surface is very short. As it is affected by the resolution of the measuring instrument and the sampling length, it cannot accurately reflect all the characteristics of the rough surface.

Compared to the traditional method of assuming a smooth sealing surface for premium connection, utilizing fractal functions to describe the rough morphology on the sealing surface of premium connections can reveal the contact behavior of the sealing surface more realistically. First, a fractal rough surface, including the geometric parameters and the material parameters of the premium connections, is constructed with the W-M function. A fractal contact model for rough surfaces is developed by taking into account the variations in actual contact area resulting from elastic, elastoplastic, and fully plastic deformation of asperities. Then, the influence law of the different values of the fractal dimension D and the scale coefficient G on the contact area and the contact pressure is analyzed. Secondly, the surface profile of the fractal roughness is created on the sealing surface, and a fractal FEM of full-size premium connection is established. The distribution of the Von Mises stress, contact pressure and effective contact length under different values of the fractal dimension, axial tension and internal pressure are obtained. Finally, based on the theory of sealing strength, an analysis of the sealability of the premium connection is conducted under different loads. The findings of this paper could provide a new method of researching the sealability of premium connections at the microscale, and may serve as a reference for the design and application of premium connections. It may also provide a reference for the sealability analyses of metal sealing parts such as bolts and flanges.

2. Analysis of Fractal Surface Contact Behavior

The key factor determining the contact behavior of a fractal surface is the interaction of the asperities on the contact surface. The asperities cause the real contact area to be smaller than the nominal contact area, and lead to greater contact pressure. As the contact pressure increases, the asperities will undergo elastic, elastoplastic and perfect plastic deformation. The W-M fractal function is utilized for characterizing the profile of a rough surface, and it can clearly describe the asperity morphology and the process of contact deformation.

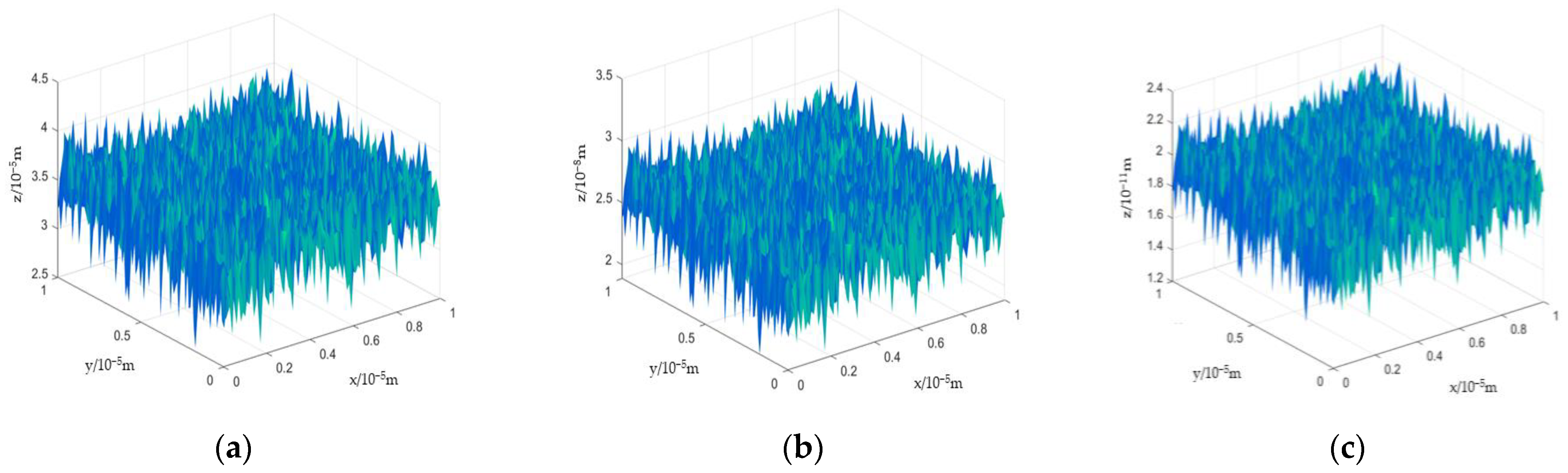
2.1. The W-M Fractal Function

The W-M fractal function, which was defined by [18], can be used to characterize the profile curve of a 3D fractal surface:

$$z(x,y)=L{\left(\frac{G}{L}\right)}^{(D-2)}{\left(\frac{In\gamma }{M}\right)}^{\frac{1}{2}}{\displaystyle \sum _{m=1}^M{\displaystyle \sum _{n=n_1}^{n\max }{\gamma }^{(D-3)}}}\left\{\cos {\varphi }_{m,n}-\cos \left[\frac{2\pi {\gamma }^{\prime }{(x^2+y^2)}^{\frac{1}{2}}}{L}\cos \left(\arctan \frac{y}{x}-\frac{\pi m}{M}\right)+{\varphi }_{m,n}\right]\right\}$$

(1)

where $z(x,y)$ is the height of the rough surface’s profile, D is the fractal dimension of a fractal surface (2D fractal function: 1 < D < 2, 3D fractal function: 2 < D < 3), and γ is a parameter related to the spectral density of the surface profiles (γ > 1). G is the scale coefficient or the fractal roughness (in m), L is the sampling length (in m), M is the number of overlaps of the surface folds, L_s the is cutoff length (in m), and m and n are the random phase (the value range is [0, 2π]). The lowest frequency order is 0, and the highest frequency order is $n_{\max }$, $n_{\max }=\mathrm{int}[\log (L/L_{\mathrm{s}})/Log\gamma ]$.

According to Equation (1), 3D fractal rough surfaces with fractal dimensions D of 2.1, 2.5 and 2.9 are shown in Figure 3, and the fractal parameters are shown in

Table 1. Fractal parameters.

G (m)	M	γ	L (m)
1 × 10−13	10	1.5	1 × 10−5

. In Figure 3, the x and y coordinate axes represent the sampling length L, while the z coordinate axis represents the height of the rough surface’s profile Z (x, y) (i.e., the height of asperities). As shown in Figure 3, the profile of the fractal rough surface is disordered, and the distribution of the asperities is random and continuous. As the fractal dimension D increases, the height of asperities decreases exponentially, the details of the fractal surface profile become more complex, and the surface becomes smoother [19]. As shown in Figure 3a–c, every 0.4 increase in the fractal dimension D will reduce the order of magnitude of the z coordinate axis (the height of asperities) by 10³.

2.2. Contact Behavior Analysis of Single Asperities

At the microscale, the contact on the fractal rough surface may be regarded as contact pairs between the plane composed of asperity and the rigid plane. Assuming that the rough surface is isotropic, it can be concluded that the asperities on the surface which have a hemispherical geometric shape, do not interact with each other. Then, the undeformed profile equation of the asperity in the rectangular plane coordinate system is [20]

$$z(x)=G^{D-1}{\displaystyle \sum _{n=n_1}^{\infty }\frac{\cos (2\pi {\gamma }^{n}x+{\phi }_n)}{{\gamma }^{(2-D)n}}}$$

(2)

The process of an asperity contacting the rigid plane and deforming is shown in Figure 4. As the external load increases, the single asperity will transform from elastic to elastoplastic to perfect plastic in succession. The corresponding radius of curvature can be obtained using the curvature calculation equation [20]:

$$R_{i,n}={\left|\frac{\left[1+{(dz(x)/d{x}^2)}^{3/2}\right]}{d^{2}z(x)/d{x}^2}\right|}_{x=L{\psi }_{i,n}/2\pi {\gamma }^n}=\frac{{(L/{\gamma }^n)}^{(D-1)}}{4{\pi }^2{G}^{(D-2)}{(In\gamma )}^{1/2}}$$

(3)

When $\omega \le {\omega }_{ec}$, part of the asperity will only undergo elastic deformation [21]. The equation for critical elastic compression is

$${\omega }_{ec}={(\pi KH/2E)}^{2}R$$

(4)

where K is the hardness coefficient that is related to Poisson’s ratio μ, K = 0.454 + 0.41μ; E is the elastic modulus, in Pa; and H is the material’s hardness, in Pa.

The corresponding critical elastic area is

$$a_{ec}=\pi R{\omega }_{ec}$$

(5)

In the elastic deformation state only, the relationship between the contact area $a_e$, the cutoff area $a_e^{\prime }$ and contact load F_e of the asperities is

$$a_e=\pi R\omega =\frac{a_e^{\prime }}{2}$$

(6)

$$F_e=\frac{8E{\pi }^{1/2}{G}^{(D-2)}{(In\gamma )}^{1/2}}{3\sqrt{2}{(L/{\gamma }^n)}^{(D-1)}}{(a_e^{\prime })}^{3/2}$$

(7)

When ω_ec ≤ ω ≤ 76.8ω_ec, some of the asperities will undergo elastoplastic deformation. The relationship between the contact area and contact load of the asperities is

$$a_{ep}=0.5{(a_{ec}^{\prime })}^{-0.1597}{(a_{ep}^{\prime })}^{1.1597}$$

(8)

$$F_{ep}=\frac{1}{3}KH{(a_{ec}^{\prime })}^{-0.3801}{(a_{ep}^{\prime })}^{1.3801}$$

(9)

where $a_{ec}^{\prime }$ is the critical cutoff area of the elastoplastic deformation stage, $a_{ec}^{\prime }=\pi R\omega$; $a_{ep}^{\prime }$ is the critical cutoff area of the plastic deformation stage, $a_{ep}^{\prime }=153.66a_{ec}^{\prime }$.

When $\omega \ge {\omega }_{\mathrm{epc}}$, perfect plastic deformation will occur. ${\omega }_{\mathrm{epc}}$ is the maximum deflection at the stage of elastoplastic deformation. The following relationships can be obtained:

$$a_p=a_p^{\prime }=2\pi R\omega$$

(10)

$$F_P=H{a}_p^{\prime }$$

(11)

where $a_p^{}$ and $a_p^{\prime }$ are the contact and cutoff areas of the perfect plastic deformation stage, respectively, and $F_p$ is contact load of the perfect plastic deformation stage.

2.3. Analysis of the Contact Behavior of the Full-Size Area

If the contact cutoff area of a single asperity is represented by $a^{\prime }$, then the distribution density function of the asperity in the fractal contact region can be expressed as [21]

$$n(a^{\prime })=\frac{D-1}{2a_1^{\prime }}{\left(\frac{a_1^{\prime }}{a^{\prime }}\right)}^{(D-2)/2}$$

(12)

In Equation (12), $a_1^{\prime }$ is the maximum cutoff area of a single asperity. The cutoff area of the contact region of the simplified model is

$$A_r^{\prime }={\displaystyle {\int }_0^{a_1^{\prime }}n(a^{\prime })}{a}^{\prime }d{a}^{\prime }$$

(13)

When $a^{\prime }\le a_{nec}^{\prime }$, the contact area has perfect elastic deformation, and the real contact area is

$$A_e={\displaystyle {\int }_0^a{n}_e(a)}ada=\frac{D-1}{1.5-0.5D}{a}_1^{0.5D-0.5}$$

(14)

The contact load is

$$F_{te}={\displaystyle {\int }_0^{a_{nec}}{n}_e(a)}{F}_e(a)da=\frac{D-1}{3(2.5-D)}{E}^{\prime }{\pi }^{0.5D-2}{2}^{6.5-1.5D}{(In\gamma )}^{0.5}{G}^{D-2}{a}_1^{0.5D-0.5}(a_1^{2.5-D}-a_c^{2.5-D})$$

(15)

When $a_{nec}^{\prime }\le a^{\prime }\le a_{nep}^{\prime }$, the contact area has elastoplastic deformation and the real contact area is

$$A_r=A_e+A_{ep}={\displaystyle {\int }_0^{a_{ec}}{n}_e}(a)ada+{\displaystyle {\int }_{a_{ec}}^{a_{}}{n}_{ep}}(a)ada=\frac{D-1}{2(1.5-0.5D)}{a}_1^{1.5D-0.5}$$

(16)

The contact load is

$$\begin{array}{l}{F}_t=F_{te}+F_{tep}={\displaystyle {\int }_0^{a_{nec}}{n}_e(a)}{F}_e(a)da+{\displaystyle {\int }_{a_{nec}}^a{n}_{ep}(a)}{F}_{ep}(a)da=\frac{D-1}{3(2.5-D)}{E}^{\prime }{\pi }^{0.5D-2}{2}^{6.5-1.5D}{(In\gamma )}^{0.5}{G}^{D-2}{a}_1^{0.5D-0.5}(a_1^{2.5-D}-a_c^{2.5-D})\\ +\frac{1.395\lambda \sigma y{a}_c^{-n}(D-1)a_1^{0.5D-0.5}}{1.5-0.5D+n}(a_c^{1.5-0.5D+n}-a_p^{1.5-0.5D+n})\end{array}$$

(17)

When $a^{\prime }\ge a_{nep}^{\prime }$, the contact area underwent complete plastic deformation, resulting in the real contact area:

$$A_r=A_e+A_{ep}+A_p={\displaystyle {\int }_0^{a_{ec}}{n}_e}(a)ada+{\displaystyle {\int }_{a_{ec}}^{a_{ep}}{n}_e}(a)ada+{\displaystyle {\int }_0^{a_{ec}}{n}_e}(a)ada=\frac{D-1}{2(1.5-0.5D)}{a}_1$$

(18)

The contact load is

$$\begin{array}{l}{F}_t=F_{te}+F_{tep}+F_{tp}={\displaystyle {\int }_0^{a_{ec}}{n}_e\left(a\right)}{F}_e\left(a\right)da+{\displaystyle {\int }_{a_{ec}}^{a_{ep}}{n}_{ep}\left(a\right)}{F}_{ep}\left(a\right)da+{\displaystyle {\int }_{a_{ep}}^{a_L}{n}_p\left(a\right)}{F}_p\left(a\right)da=\frac{D-1}{3(2.5-D)}{E}^{\prime }{\pi }^{0.5D-2}{2}^{6.5-1.5D}{(In\gamma )}^{0.5}\\ G^{D-2}{a}_1^{0.5D-0.5}(a_1^{2.5-D}-a_c^{2.5-D})+\frac{1.395\lambda \sigma y{a}_c^{-n}(D-1)a_1^{0.5D-0.5}}{1.5-0.5D+n}(a_c^{1.5-0.5D+n}-a_p^{1.5-0.5D+n})+\frac{D-1}{2(1.5-0.5D)}H{a}_1^{0.5D-0.5}{a}_p^{1.5-0.5D}\end{array}$$

(19)

Therefore, the contact pressure P in the contact area is

$$P=\frac{F}{A}=\frac{F_t}{A_r}$$

(20)

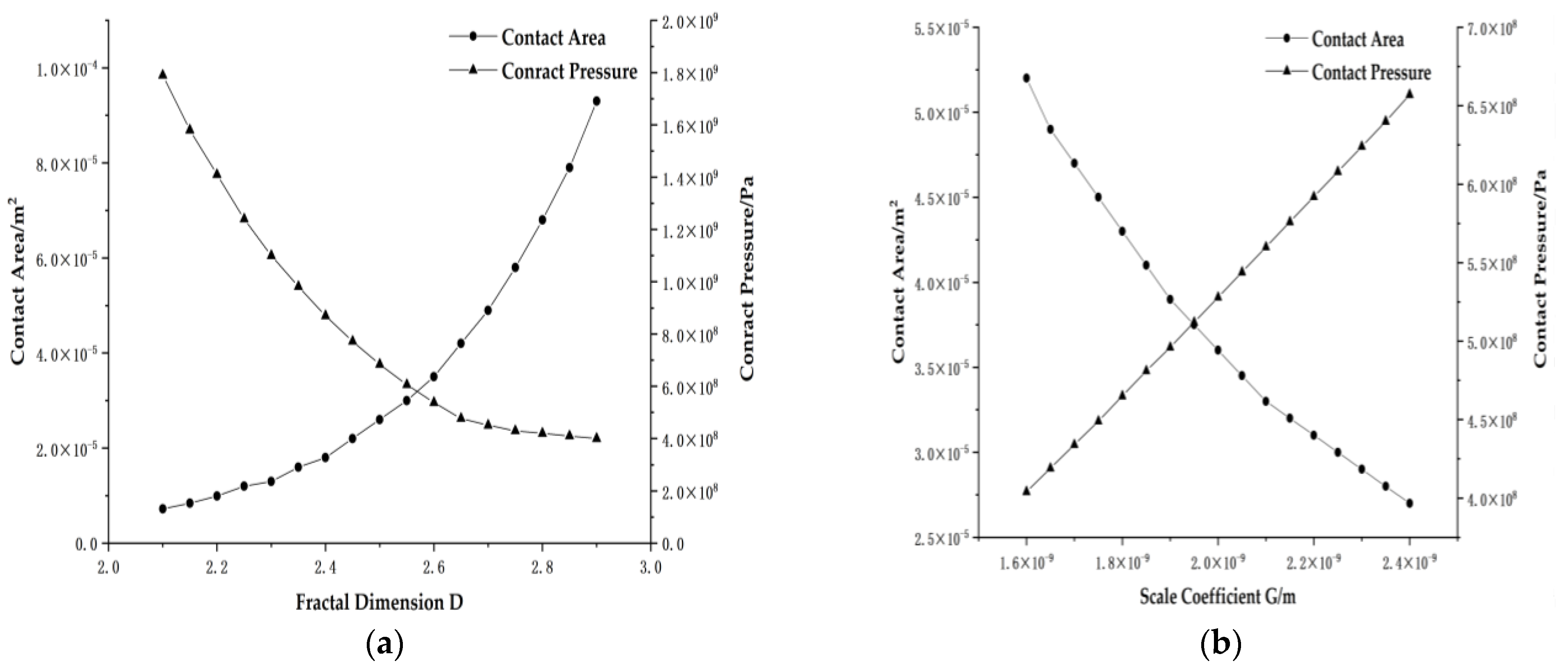
2.4. Influence of Fractal Parameters on Surface Contact Behavior

Two sets of data ((a) and (b) in

Table 2. Fractal surface and material parameters.

Parameters	D	G (m)	E (Pa)	H (Pa)	γ
(a)	2.1–2.9	2.0 × 10−9	7.58 × 108	9.85 × 108	1.5
(b)	2.5	1.6–2.4 × 10−9	7.58 × 108	9.85 × 108	1.5

) were introduced into Equations (17) and (19). The curves of contact pressure and contact area of the rough contact surface are obtained using different values of the fractal dimension D and the scale coefficient G. As shown in Figure 5a, the contact area of the rough surface increases exponentially with an increase in the fractal dimension D. This is because when the fractal dimension D is increased under the same external load, the fractal structure of the rough surface becomes finer and there is an increase in contact points, resulting in an increase in the real contact area. The contact pressure decreases exponentially with an increase in the fractal dimension D, and when the fractal dimension D exceeds 2.7, the contact pressure is maintained at around 4 × 10⁸ Pa, and the change in the contact pressure becomes stable. When the fractal dimension D is less than 2.7, the volume of a single asperity is larger and the proportion of elastic deformation of asperities is higher, so both the contact area and the contact pressure change simultaneously with the fractal dimension D. Based on Equation (20), for the same external load, the contact pressure is inversely proportional to the contact area, resulting in an increase in contact area and a decrease in contact pressure. However, when the fractal dimension D is greater than 2.7, the volume of a single asperity significantly decreases and leads to complete plastic deformation of the asperities, and as a result, the contact area continues to increase and the contact pressure remains constant.

With an increase in the scale coefficient G, the contact area decreases approximately linearly and the contact pressure increases linearly, as shown in Figure 5b. The scale coefficient G is the parameter that affects the amplitude of the fractal profile, but it does not affect the lateral spacing of the profile [22,23]. As the scale coefficient G increases, the surface becomes rougher, which leads to an increase in stiffness of the asperities and a reduction in the ability of elastic deformations of the asperities, ultimately resulting in a decrease in the real contact area. Based on Equation (20), for the same external load, the contact pressure is inversely proportional to the contact area, resulting in an increase in contact area and a decrease in contact pressure. For the same reason, based on Equation (20), the contact pressure is inversely proportional to the contact area, resulting in a decrease in contact area and an increase in contact pressure.

By comparing the changes in contact area and contact pressure between Figure 5a,b, the fractal dimension D increased from 2.1 to 2.9, and the contact area increased by 9 × 10⁻⁵ m². At the same time, the contact pressure decreased by 1.31 × 10⁹ Pa. Conversely, when the scale coefficient G increased from 1.6 × 10⁻⁹ to 2.4 × 10⁻⁹ m, the contact area decreased by 2.5 × 10⁻⁵ m², while the contact pressure increased by 2.53 × 10⁸ Pa. It can be inferred that the fractal dimension D exerts a more significant impact on the actual contact area and pressure compared to the scale coefficient G. Therefore, in the subsequent analysis, the focus is on the influence of the fractal dimension D on the contact mechanics and sealability of the premium connections.

An error analysis of the model in this study revealed that the influence of deformation behavior on the surface asperities and the interaction between them was ignored, which is main reason for the observed errors. Both of these factors can reduce the stiffness of the sealing surface, and the greater the load, the more significant the influence [23].

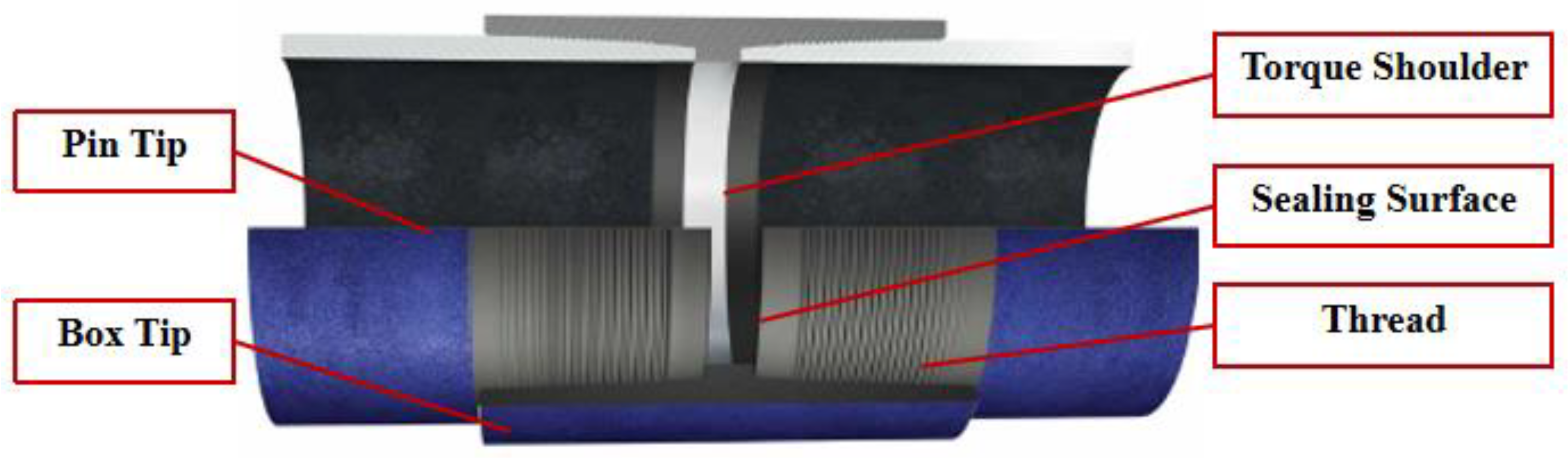
3. Contact Analysis of Premium Connections

The sealability of a premium connection is related to the contact pressure and contact length of the sealing surface. In order to simulate the downhole loads, the distribution of contact pressure on the fractal surface of the sealing surface was studied using finite element simulation.

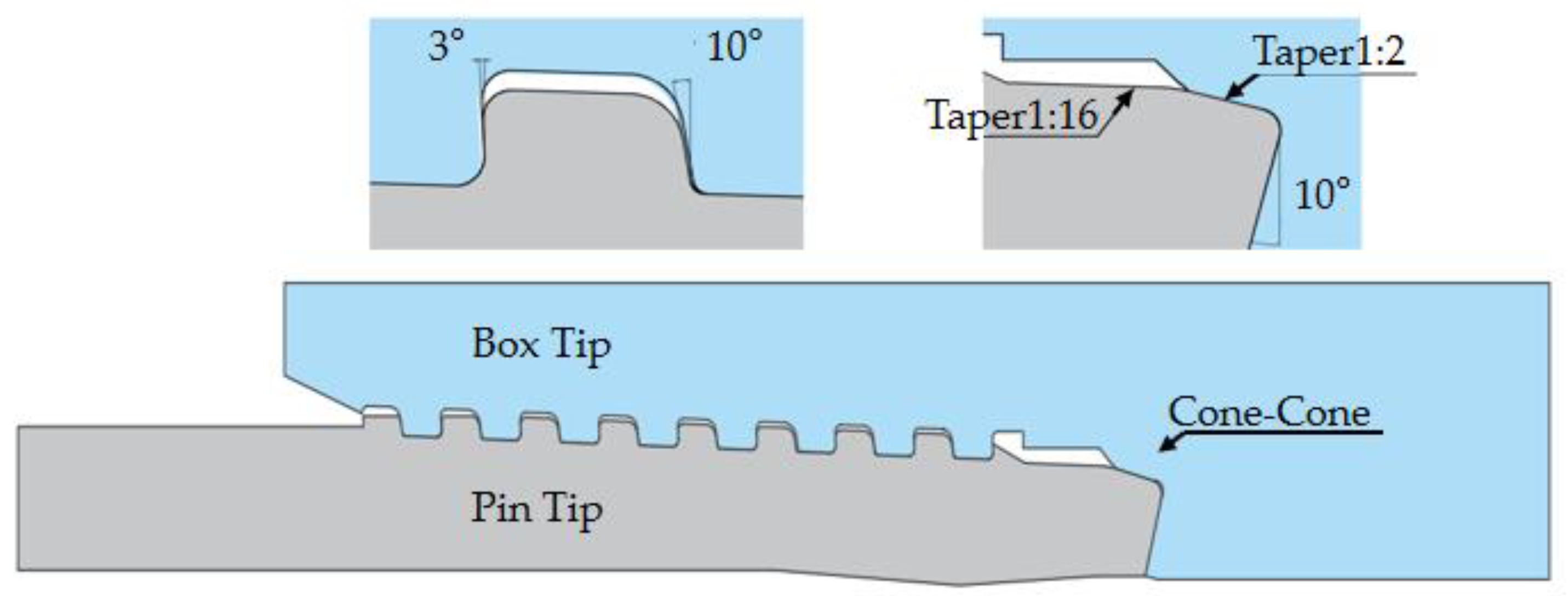
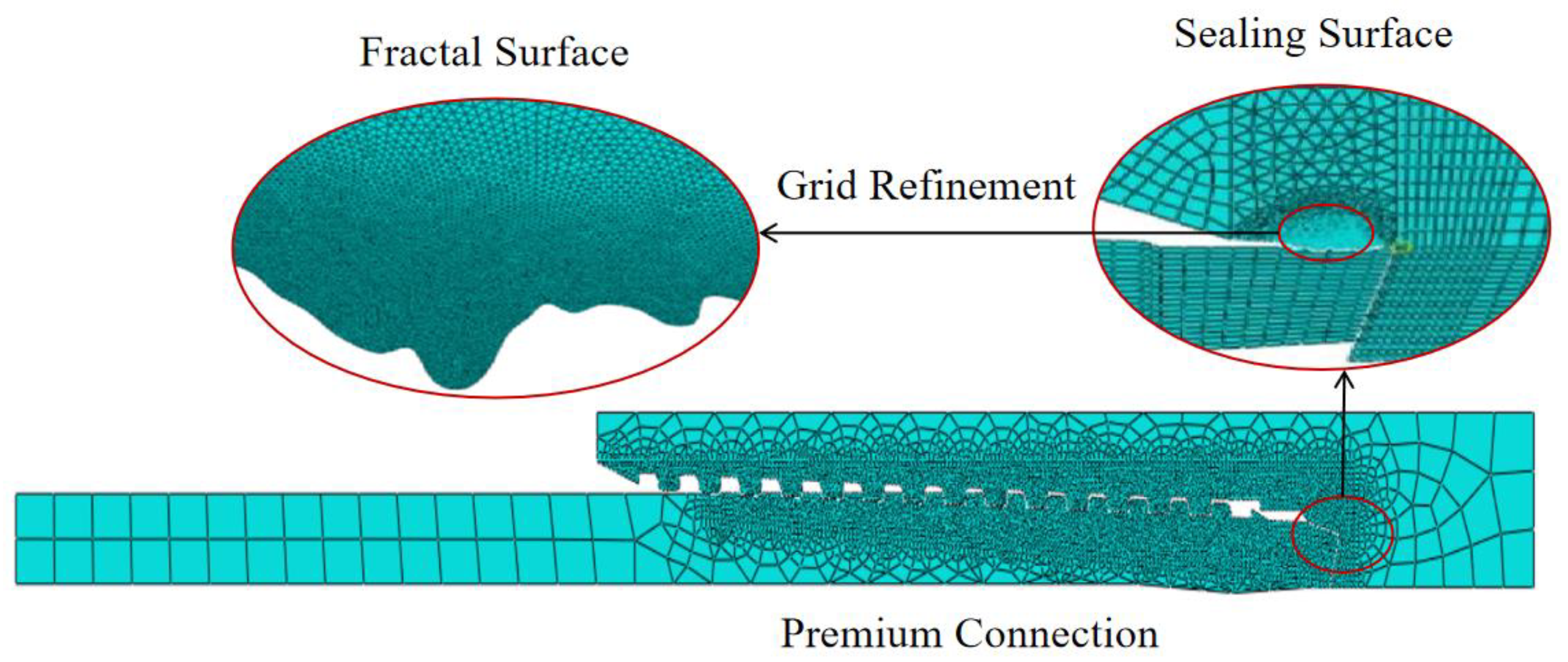
3.1. Establishment of the Fractal Model of a Premium Connection

A P110 cone–cone premium connection (Φ8.89 × 10⁻² m × 6.45 × 10⁻³ m) is taken as the example for analysis. As shown in Figure 6, the sealing surface taper is 1:2, the bearing surface angle of the thread is −3°, the guide surface angle of the thread and the torque shoulder angle are 10°, and the thread cone angle is 1:16. SolidWorks software was utilized to established a 3D fractal rough surface for the sealing surfaces.

Through use of ABAQUS software, the fractal profile of the sealing surface was imported into the full-size FEM of the premium connection for preprocessing, as shown in Figure 7. The elastic modulus E of the premium connection was 2.06 × 10¹¹ Pa, the Poisson’s ratio μ was 0.3, the yield strength was 7.58 × 10⁸ Pa, and the ultimate strength was 8.35 × 10⁸ Pa [24,25]. Due to the thread compound, the friction coefficient between the contact pairs is 0.02 [26].

In order to improve the calculation and the accuracy of calculation while controlling the convergence rate, the CAX4R element, which is the axisymmetric subtraction of an integral element, was used when meshing. Because the sealing surface and thread are involved in contact analysis, refinement of the local grid was carried out when meshing to improve the calculation accuracy. Meanwhile, because the sealing surface is a rough surface generated by the fractal function, its profile is composed of a large number of asperities. In order to avoid the calculation failing due to mesh distortion, the fractal surface of the sealing surface is again refined [27,28].

Two contact pairs were established in the FEM of the premium connection, namely the sealing surface–torque shoulder contact pair and the thread contact pair. According to the criteria of the contact pair in ABAQUS software, the box tip with the greater hardness was set as the main contact surface, and the pin tip was set as the lesser contact surface. According to the existing research, the sealing surface will have slight plastic deformation when the premium connections make up [29]. Therefore, the FEM needs to define the plastic parameters of the material. When setting the material parameters, ABAQUS software needs to convert the nominal stresses and strains obtained from tests into real stresses and strains. Therefore, the following transformation relationships should be used:

$$\epsilon =In(1+{\epsilon }_{nom})$$

(21)

$$\sigma ={\sigma }_{nom}(1+{\epsilon }_{nom})$$

(22)

where ε is the real strain, σ is the real stress (in Pa), ${\epsilon }_{nom}$ is the nominal strain and ${\sigma }_{nom}$ is the nominal stress (in Pa).

The boundary conditions and load settings of the FEM are shown in Figure 8. Because the premium connection has an axisymmetric structure, the position of the radial axis at the box tip is constrained at UX, UY, RX and RY. To simulate the downhole loads, axial tension is applied to the pin tip, and internal pressure to its inner wall.

3.2. Mesh Independence Verification

In order to eliminate the error caused by the number of mesh elements in the steady-state process, a mesh independence verification is performed by calculating the maximum contact pressure of the sealing surfaces with different mesh numbers. As shown in

Table 3. Mesh independence verification.

Mesh Size (m)	Number of Element	Maximum Contact Pressure (Pa)	Change Rate of Contact Pressure (%)
6 × 10−6	4319	9.75 × 108
5 × 10−6	5451	1.06 × 109	8.72
4 × 10−6	6902	1.15 × 109	8.49
3 × 10−6	10,608	1.20 × 109	4.35
2 × 10−6	21,584	1.14 × 109	3.33
1 × 10−6	81,812	1.21 × 109	4.31

, when the mesh size of the fractal surface on the sealing surface is 1 × 10⁻⁶ m, the maximum contact pressure tends to stabilize. Considering the accuracy and efficiency attained using finite element analysis (FEA), the mesh size of the fractal surface on the sealing surface is 3 × 10⁻⁶ m, and the mesh at other parts is 3 × 10⁻⁵ m.

3.3. Analysis of Influencing Factors

3.3.1. The Fractal Dimension

Nephograms of the premium connections’ Von Mises stress with different fractal dimensions D under the action of make-up torque are shown in Figure 9. When the fractal dimension D increases, there is a decrease in the maximum Von Mises stress at the sealing surface, which is accompanied by an increase in the area of plastic deformation. This is because the number of asperities on the fractal surface increases with the increase in the fractal dimension D. The sealing surface becomes smoother, and the distribution of stress more uniform, resulting in a reduction in Von Mises stress. When the fractal dimension D increases, the volume and stiffness of a single asperity decrease. Under the same make-up torque, there is an increase in both the proportion and area of plastic deformation of the asperities on the fractal surface.

The distribution of contact pressure with different values of the fractal dimension D on the sealing surface is shown in Figure 10. With an increase in the fractal dimension D, the effective contact length remains constant at 9.08 × 10⁻³ m. The overall contact pressure on the sealing surface decreases. The distribution of the contact pressure becomes more uniform. According to Equation (17), with an increase in the fractal dimension, there is an augmentation in the actual contact area of the sealing surface. This causes a decrease in the contact pressure at the same pressure level, and the distribution of contact pressure becomes more uniform. It can be concluded from the above analysis that the larger the fractal dimension D, the smaller the Von Mises stress on the sealing surface. The more uniform the distribution of contact pressure, the more favorable the sealing.

A premium connection should make up according to the torque and speed recommended by the manufacturer. If the recommended value is exceeded, the stress on the sealing surface will sharply increase, which can easily cause gluing, leading to sealing failure. As shown in

Table 4. The maximum Von Mises stress and contact pressure with different fractal dimensions.

Fractal Dimension D	2.1	2.5	2.9
Maximum Von Mises stress (Pa)	9.00 × 108	8.81 × 108	8.53 × 108
Maximum contact pressure (Pa)	1.39 × 109	1.20 × 109	7.45 × 108

, the maximum Von Mises stress and maximum contact pressure on the sealing surface significantly exceeded the ultimate strength of the material when the fractal dimension D was 2.1 and 2.5, and the sealing surface may have required gluing in order to avoid the sealing failing before the premium connection enters the well, which would interfere with the results in the next sections for the analysis of the load factor. Fractal dimensions D of 2.1 and 2.5 will not be considered below. Meanwhile, to reduce the computation time, according to the conclusion in Section 2.4, changes in the contact pressure tend to be stable when the fractal dimension D is greater than 2.7. In the following sections, the fractal dimension D of the fractal model of the connection is taken as 2.7.

3.3.2. The Influence of Axial Tension

The Von Mises stress nephograms when the axial tension was 4 × 10⁵, 8 × 10⁵ and 1.2 × 10⁶ N are shown in Figure 11, and the distribution of the contact pressure on the sealing surface is shown in Figure 12. As shown in Figure 11, with an increase in the axial tension, the area of concentrated stress concentration on the sealing surface decreases and there is little change in the maximum Von Mises stress. When the axial tension was less than 8 × 10⁵ N, there was a significant concentration of stress on the torque shoulder and sealing surface. When the axial tension was 1.2 × 10⁶ N, the concentration of stress on the torque shoulder disappeared, but stress concentration appeared on the thread, indicating that the thread bore all the axial tension.

As shown in Figure 12, both the contact pressure and effective contact length at the fractal surface decrease with an increase in the axial tension. This is because the box tip is fixed and fully constrained in the FEM. Under the action of axial tension, the sealing surface of the pin tip is displaced along the direction of the load, resulting in a decrease in the effective contact length (from 2.72 × 10⁻³ to 2.24 × 10⁻³ m). When the sealing surface of the pin tip is displaced in the direction of the load, the interference in the assembly decreases due to the influence of the cone angle. This causes the deformation of the asperities in the fractal surface to decrease, so the contact pressure also decreases. It can be concluded from the above analysis that when the axial tension is 1.2 × 10⁶ N, there is large displacement of the sealing surface at the pin tip along the direction of the load, and the contact pressure and the effective contact length of the fractal surface decrease sharply, finally resulting in a decrease in the sealability.

3.3.3. The Influence of Internal Pressure

The Von Mises stress nephograms of the premium connection when the internal pressure was 5 × 10⁷, 7.5 × 10⁷ and 1 × 10⁸ Pa are shown in Figure 13, and the distribution of contact pressure on the sealing surface is shown in Figure 14. As shown in Figure 13, the maximum Von Mises stress of the sealing surface increases when the internal pressure is increased. When the internal pressure exceeds 7.5 × 10⁷ Pa, the area of stress concentration is transferred from the thread to the sealing surface. This is because with the increase in the internal pressure, the plastic deformation of the asperities increases, leading to an increase in stress. Meanwhile, due to the increase in the quantity of contacts and the proportion of plastic deformation in the fractal asperities, there is an increase in the area of stress concentration on the sealing surface. When the internal pressure was 1 × 10⁸ Pa, the plastic deformation of the asperities increased; the stress concentration, then, is serious, making the sealing surface prone to strength failure.

As shown in Figure 14, the contact pressure and effective contact length on the fractal surface increase when the internal pressure is increased. This is because deformation of the contacted asperities increases under increasing internal pressure, which causes the non-contacting asperities to come into contact with the surface gradually. The increase in the quantity of contact with the asperities eventually led to an augmentation in the effective length of contact with the sealing surface (from 9.08 × 10⁻³ to 1.06 × 10⁻² m).

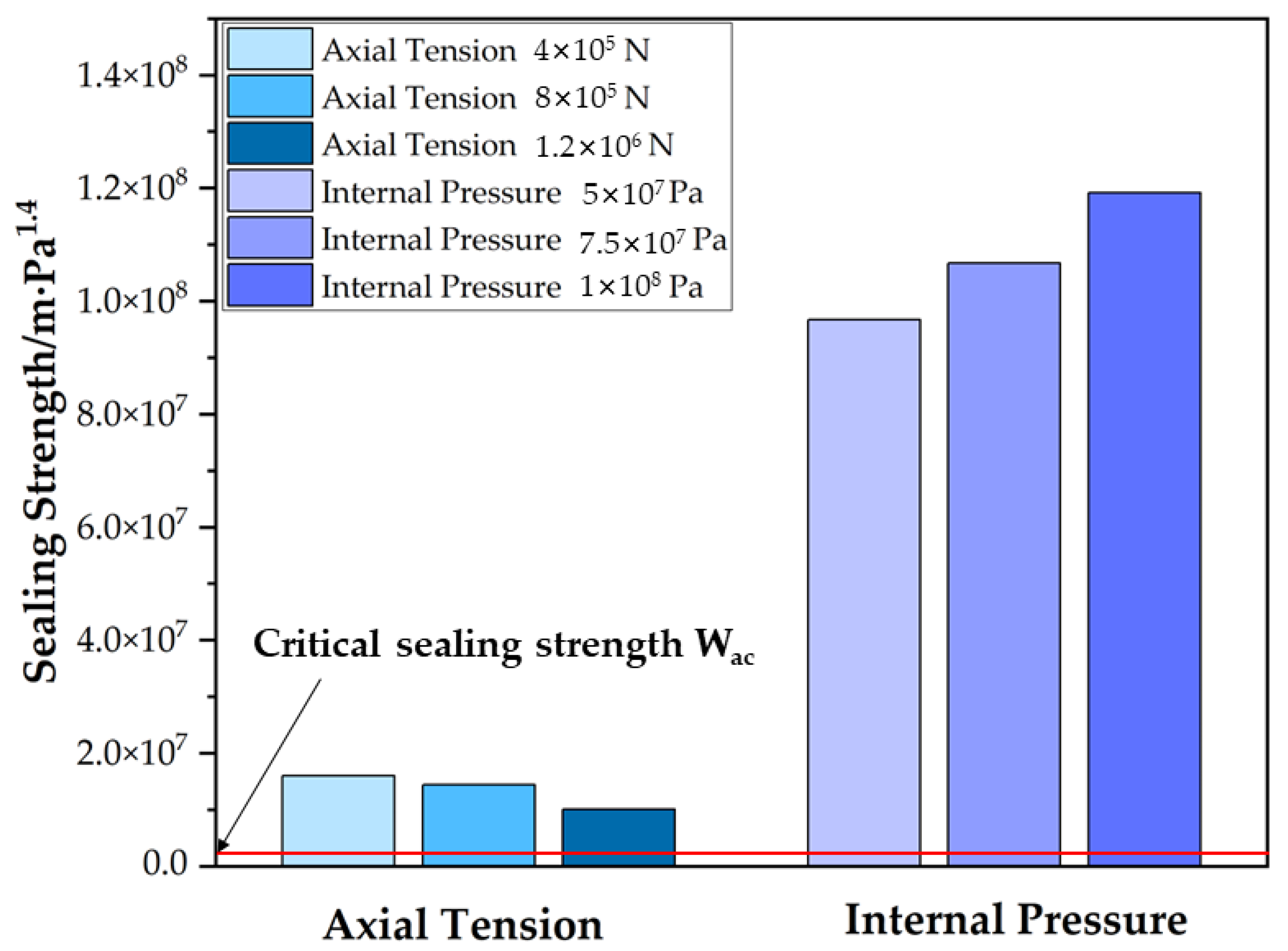
4. Analysis of the Sealability of Premium Connections

For a premium connection with a metal-to-metal sealing structure, achieving sealability relies predominantly on the distribution of contact pressure across the sealing surface. The influencing factors mainly include the geometric structure of the sealing surface, the properties of the metal materials, the properties of the sealing surface, the service environment, the load conditions, and whether a thread compound is used. The traditional design concept of sealability is that if the mean contact stress on the sealing surface surpasses the fluid pressure in the pipe, it will meet the requirements of sealability. However, sealing structures designed in line with this concept still leak [30]. Thus, this study utilized a model for sealing contact strength to assess the sealability of a premium connection.

On the basis of the theory of sealing contact energy, Murtagian established a weighted model of sealing contact strength to evaluate the sealability through experiments and a numerical simulation study [9].

$$W={\displaystyle {\int }_0^l{p}_s{}^m}dl=p_{av}{}^{m}l$$

(23)

Here, P_av is the average contact pressure (in Pa), W is the sealing strength (in m·Pa^1.2), l is the contact length of the sealing surface (in m) and m is the correlation index. If a thread compound is used, m = 1.2; if no thread compound is used, m = 1.4. In this case, we selected m = 1.4.

$$W_{ac}=\left\{\begin{array}{l}1.843\times {\left(\frac{p_g}{p_a}\right)}^{1.177}(m\cdot P{a}^{1.2})\\ 103.6\times {\left(\frac{p_g}{p_a}\right)}^{0.838}(m\cdot P{a}^{1.4})\end{array}\right.$$

(24)

Here, $W_{ac}$ is the critical sealing strength (in m·Pa^1.2), P_a is the atmospheric pressure (in Pa, the standard atmospheric pressure here was 1 × 10⁻⁵ Pa) and P_g is the pressure of gas to be sealed (in Pa).

In order to effectively assess the sealing ability of a premium connection, Xie optimized Equation (24) and established a model for the critical sealing strength of a cone–cone premium connection [31].

$$W_{ac}=10\times {\left(\frac{p_g}{p_a}\right)}^{0.838}(m\cdot P{a}^{1.4})$$

(25)

To ensure that a premium connection does not leak, it must meet the condition $W\ge W_{ac}$.

According to Equation (25), the critical sealing contact strength $W_{ac}$ of a premium connection is 3.12 × 10⁶ m·Pa^1.4. According to the results of the above FEA and Equation (23), the sealing contact strength W of a premium connection under different axial forces and internal pressures can be calculated (as shown in

Table 5. Average contact pressure and effective contact length under different loads.

	Axial Tension (N)			Internal Pressure (Pa)
	4 × 105	8 × 105	1.2 × 106	5 × 107	7.5 × 107	1 × 108
Average contact pressure (Pa)	5.13 × 108	4.74 × 108	4.26 × 108	6.75 × 108	7.83 × 108	8.70 × 108
Effective contact length (m)	2.57 × 10−3	2.57 × 10−3	2.09 × 10−3	9.08 × 10−3	9.48 × 10−3	1.05 × 10−2

). In the example shown in Figure 15, the sealing contact strength W under axial tension and internal pressure is greater than the critical sealing contact strength $W_{ac}$, but there are still potential sealing failure points. For example, when the axial force is 1.2 × 10⁶ N, the sealing contact strength W is reduced by 30%. Although the sealing contact strength W increases under the internal pressure, a large area of plastic deformation appears on the sealing surface when the internal pressure is 1 × 10⁸ Pa (as shown in Figure 13). The strength failure can also lead to sealing failure on the sealing surface. Through the above analysis, it can be concluded that the premium connection in this study has a higher risk of sealing failure when the axial tension is 1.2 × 10⁶ N or the internal pressure is 1 × 10⁸ Pa.

5. Conclusions

On the basis of fractal contact theory, the impact of fractal parameters on the contact behavior of a sealing surface was assessed. The rough surface profile and the deformation of asperities on the sealing surface of a premium connection were taken into account. Through the use of ABAQUS software and contact strength theory, the fractal FEM of a full-size premium connection was established, and the sealability of the premium connection under axial tension and internal pressure was analyzed. The following conclusions can be drawn from this study.

(1)

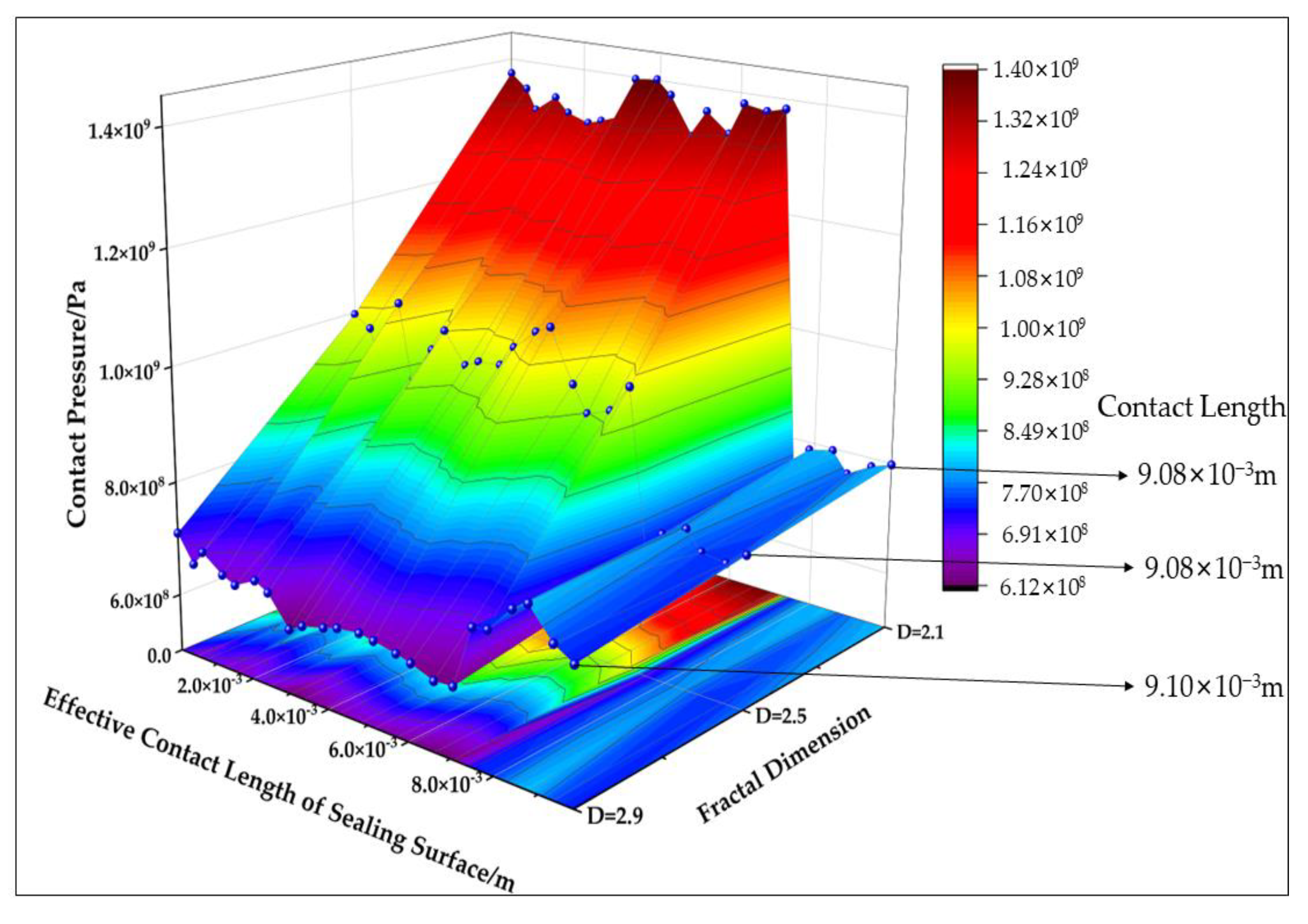
Compared with the scale coefficient G, the influence of fractal dimension D on contact area and contact pressure is more significant. With the increase in fractal dimension, the number of asperities on the fractal surface increases, and the contact area exhibits exponential growth, while the contact pressure decreases exponentially. When the fractal dimension D is less than 2.5, the maximum Von Mises stress is 8.81 × 10⁸ Pa and the maximum contact pressure on the sealing surface is 1.20 × 10⁹ Pa, making it prone to gluing and ultimately leading to sealing failure. Conversely, when the fractal dimension D is greater than 2.7, the contact pressure distribution on the sealing surface is more uniform, which improves sealability.

(2)

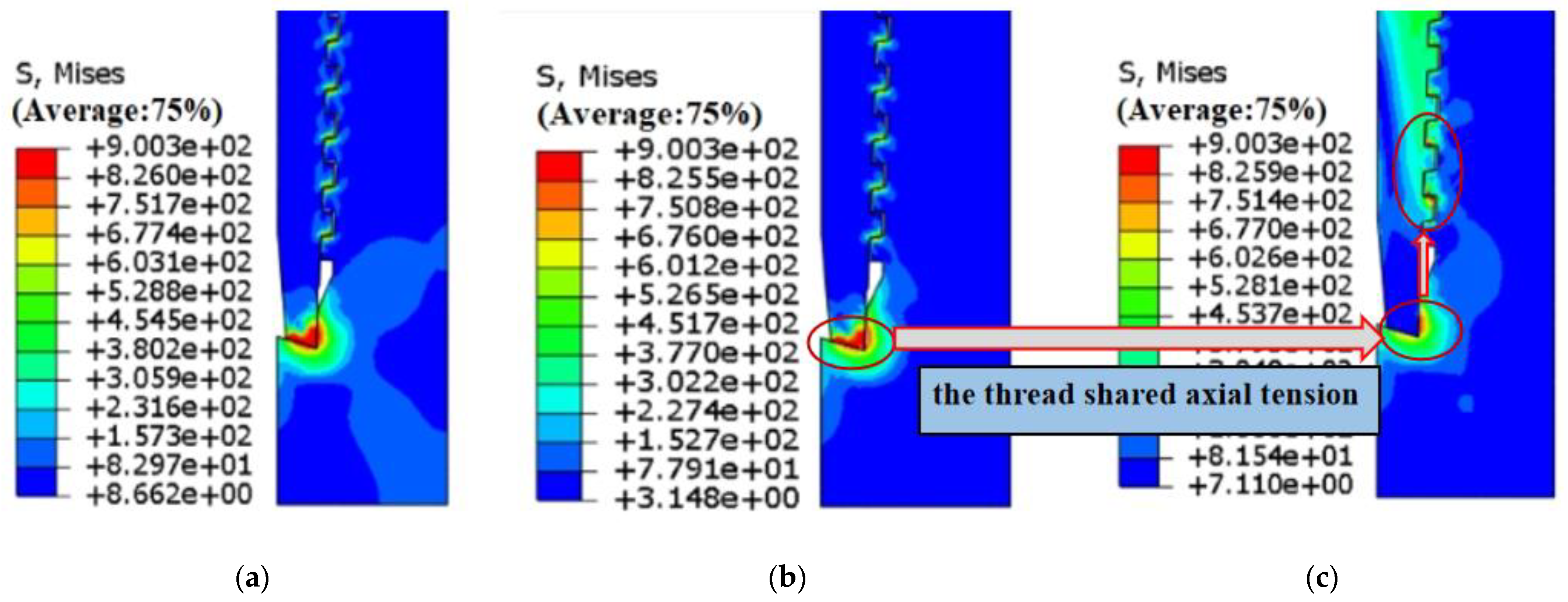
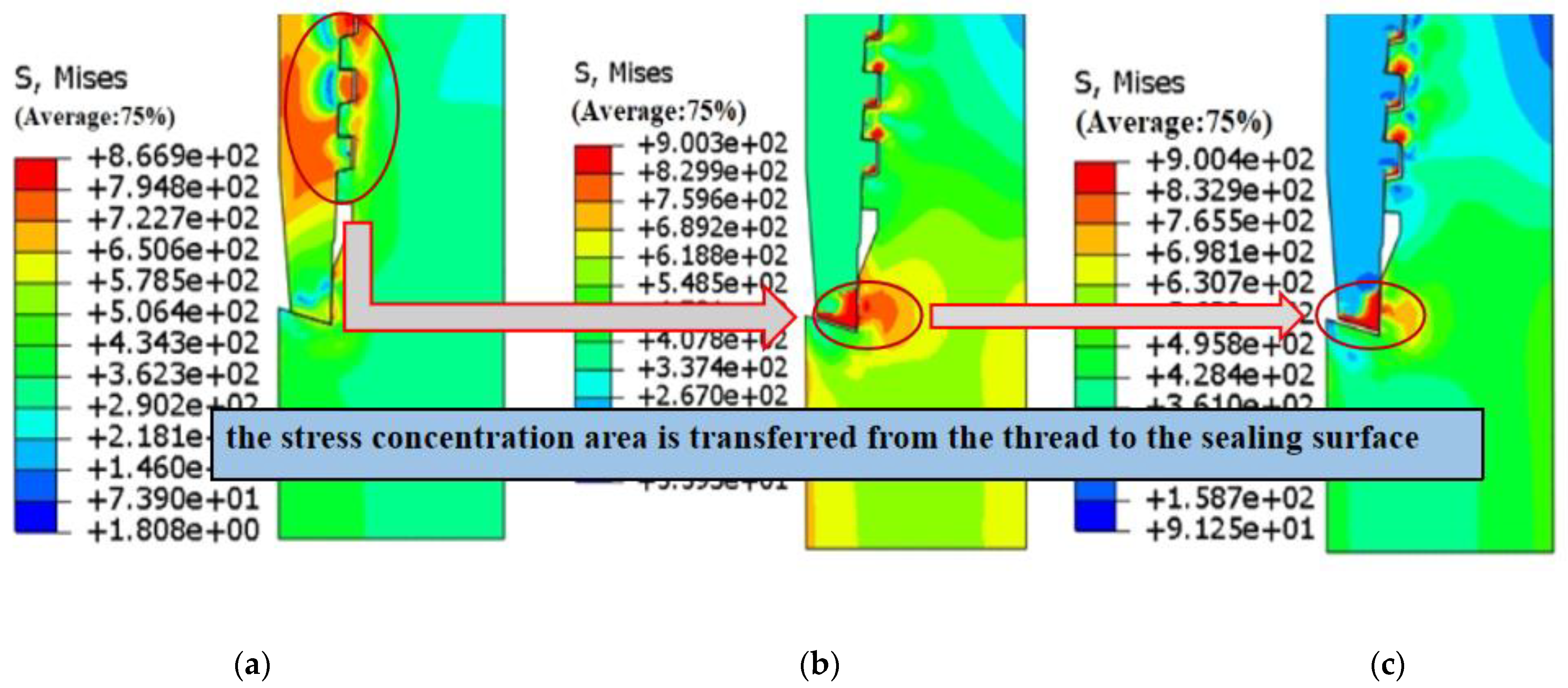
As the axial tension increases, stress concentration in the area gradually shifts from the sealing surface to the threaded portion, resulting in a reduction in the contact pressure and the effective contact length of the sealing surface. When the axial tension reaches 1.2 × 10⁶ N, the sealing surface experiences significant displacement along the axial direction, the effective contact length is reduced from 2.72 × 10⁻³ m to 2.24 × 10⁻³ m, and the maximum contact pressure is reduced from 8.10 × 10⁸ Pa to 6.39 × 10⁸ Pa, which leads to a 30% decrease in sealing strength and therefore poses a high risk of sealing failure.

(3)

As the internal pressure increases, the plastic deformation ratio of the fractal surface asperities significantly increases, leading to a proportional increase in the contact pressure and effective contact length of the sealing surface. When the internal pressure reaches 1 × 10⁸ Pa, the effective contact length is increased from 9.08 × 10⁻³ m to 1.06 × 10⁻² m, the maximum contact pressure is increased from 8.67 × 10⁸ Pa to 1.37 × 10⁹ Pa, and the sealing strength is increased by 23%. At the same time, the maximum Von Mises stress on the sealing surface reached 9 × 10⁸ Pa, resulting in a significant stress concentration on the sealing surface.

In summary, the sealability of the premium connection is primarily affected by the axial tension. Within the range in which the structural integrity is not compromised by internal pressure, increasing the internal pressure and ensuring smoother sealing surfaces can both contribute to maintaining sealability. Therefore, to ensure the sealability of the premium connections, axial tension should be kept below 1.2 × 10⁶ N, and internal pressure should be limited to below 1 × 10⁸ Pa. Moreover, to avoid the sealing surfaces gluing during assembly, surface roughness should be minimized during manufacture. Compared to the traditional method of assuming a smooth sealing surface for premium connection, utilizing fractal functions to describe the rough morphology on the sealing surface of premium connections can reveal the contact behavior of the sealing surface more realistically. The premium connections’ fractal FEM established by this method is closer to the actual situation, considering the effect of the asperities’ elastic–plastic deformation on the sealability. Consequently, this research provides a new method for researching the sealability of premium connections at the microscale, and serves as a reference for the design and application of premium connections.

However, within the model in this paper, the influence of deformation behavior on the surface asperities and the interaction between them was ignored. Both of these factors can reduce the stiffness of the sealing surface, and the greater the load, the more significant the influence [23]. Therefore, in future research, it is necessary to conduct rough surface testing experiments to reveal the relationship between fractal parameters and traditional surface roughness evaluation parameters, and to conduct rough surface loading experiments to verify and modify the fractal finite element model of the premium connection in order to improve the reliability of sealability research on premium connections.