Study of Nonlinear Aerodynamic Self-Excited Force in Flutter Bifurcation and Limit Cycle Oscillation of Long-Span Suspension Bridge
Abstract
:1. Introduction
2. Framework of Nonlinear Flutter Analysis
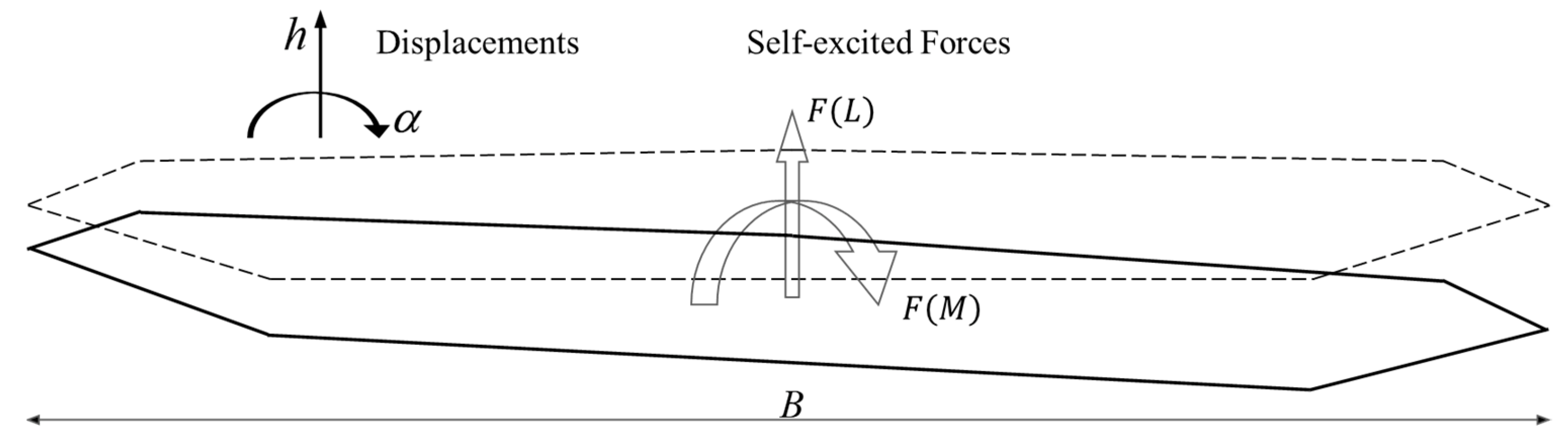
2.1. Flutter Motion Equations for Suspension Bridges
2.2. Cubic Damping and Cubic Torsional Stiffness
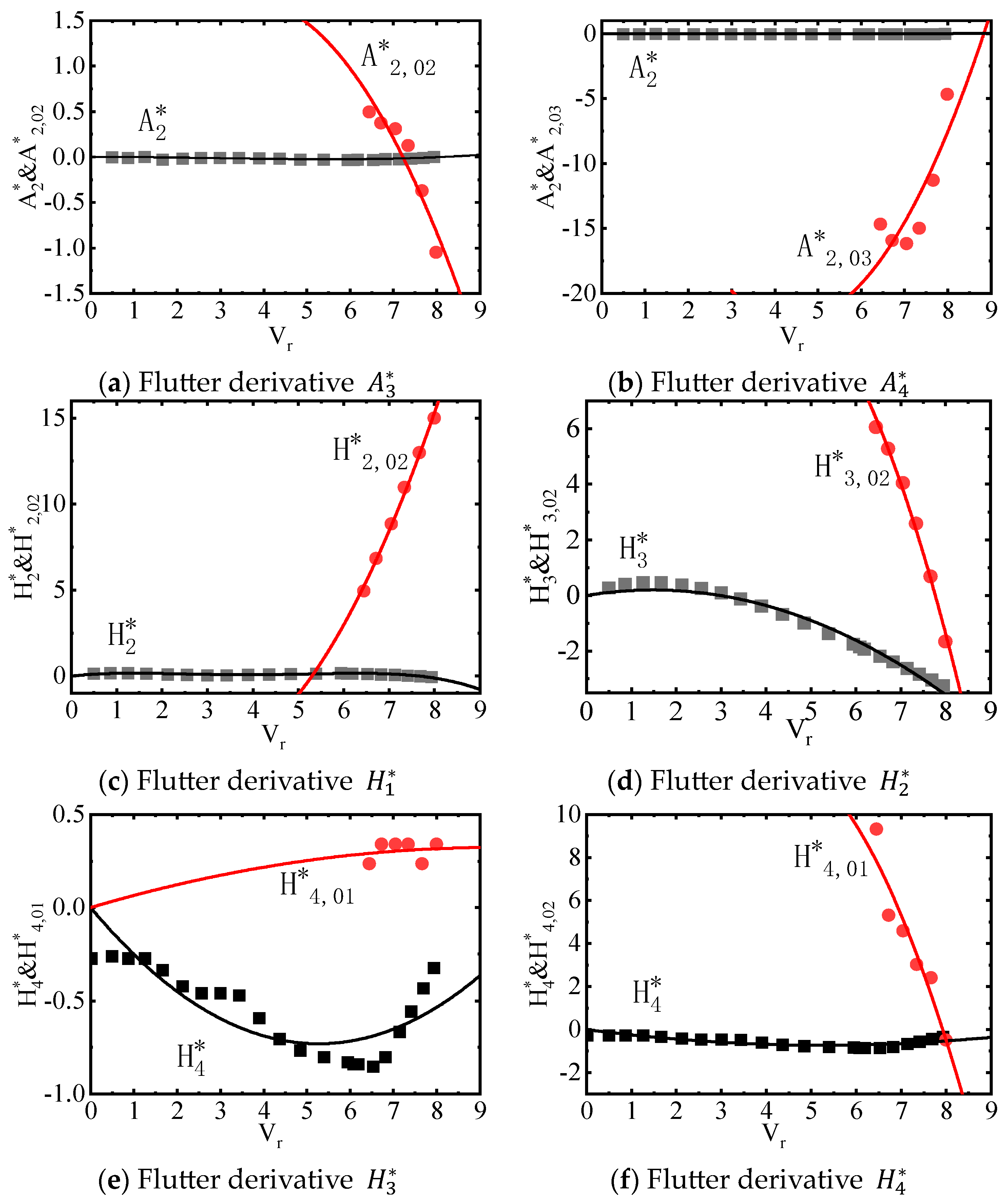
2.3. Expression of Nonlinear Aerodynamic Self-Excited Force
3. Flutter Critical State and Hopf Bifurcation
3.1. Flutter Critical Wind Speed Solution
- Assume a small value of frequency and substitute it into the matrix (15). Gradually increase the reduced wind speed until the first pair of complex conjugate eigenvalues of the matrix have zero real parts. Record the imaginary part of this eigenvalue as frequency ;
- Take as the new frequency value and substitute it into the matrix (15). Again, gradually increase the reduced wind speed until the first complex eigenvalue of the matrix has zero real parts. Record the imaginary part of this eigenvalue as the new flutter frequency .;
- Compare and . Repeat step 2 until approaches zero. At this point, the frequency value is the critical flutter frequency of the system, the reduced wind speed value is the critical reduced wind speed of the flutter, and the flutter critical wind speed can be obtained using the relationship .
3.2. Proof of Hopf Bifurcation
- Wind speed: (beyond the critical state)
- Wind Speed: Wind speed (beyond the critical state),
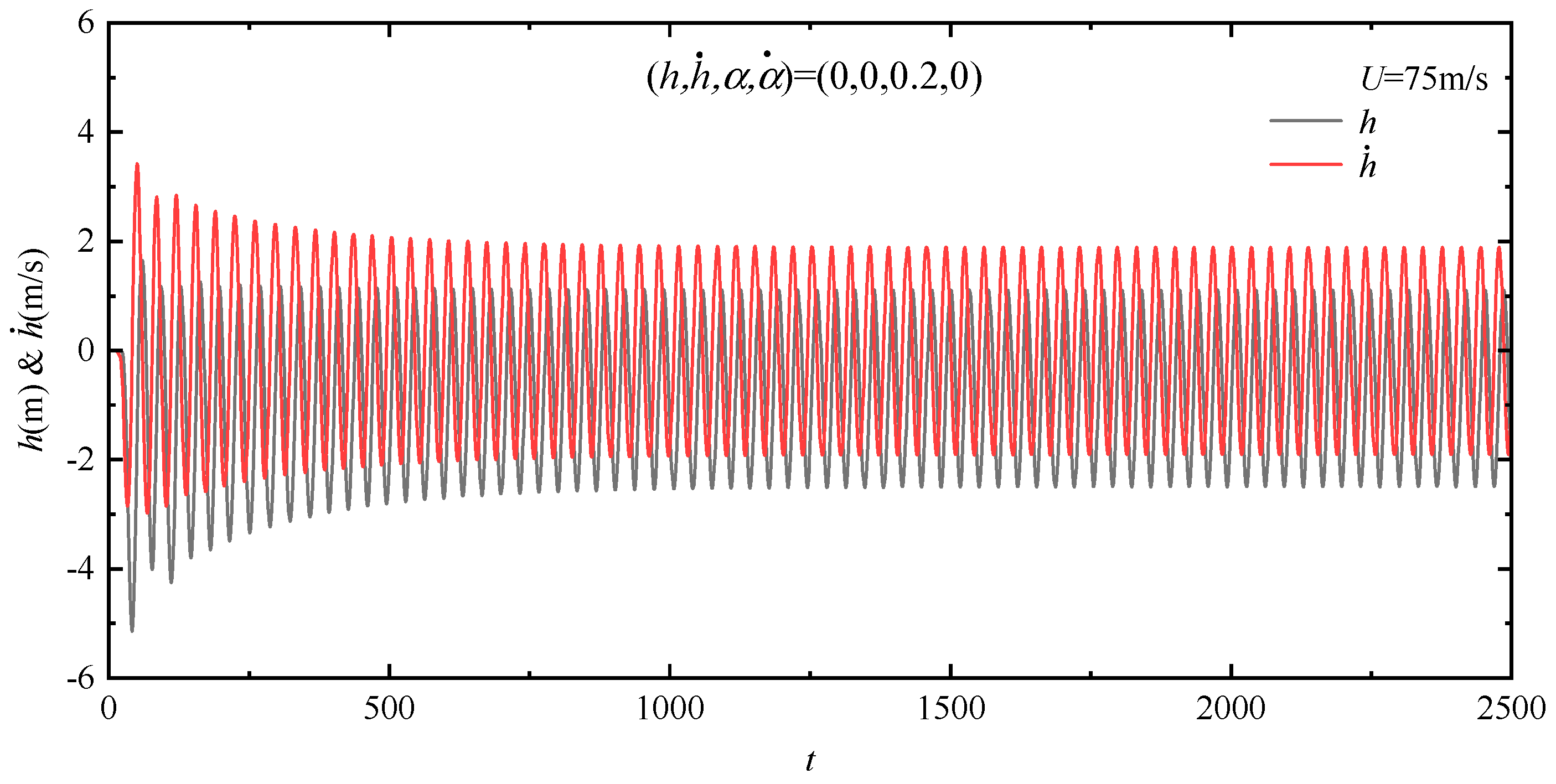
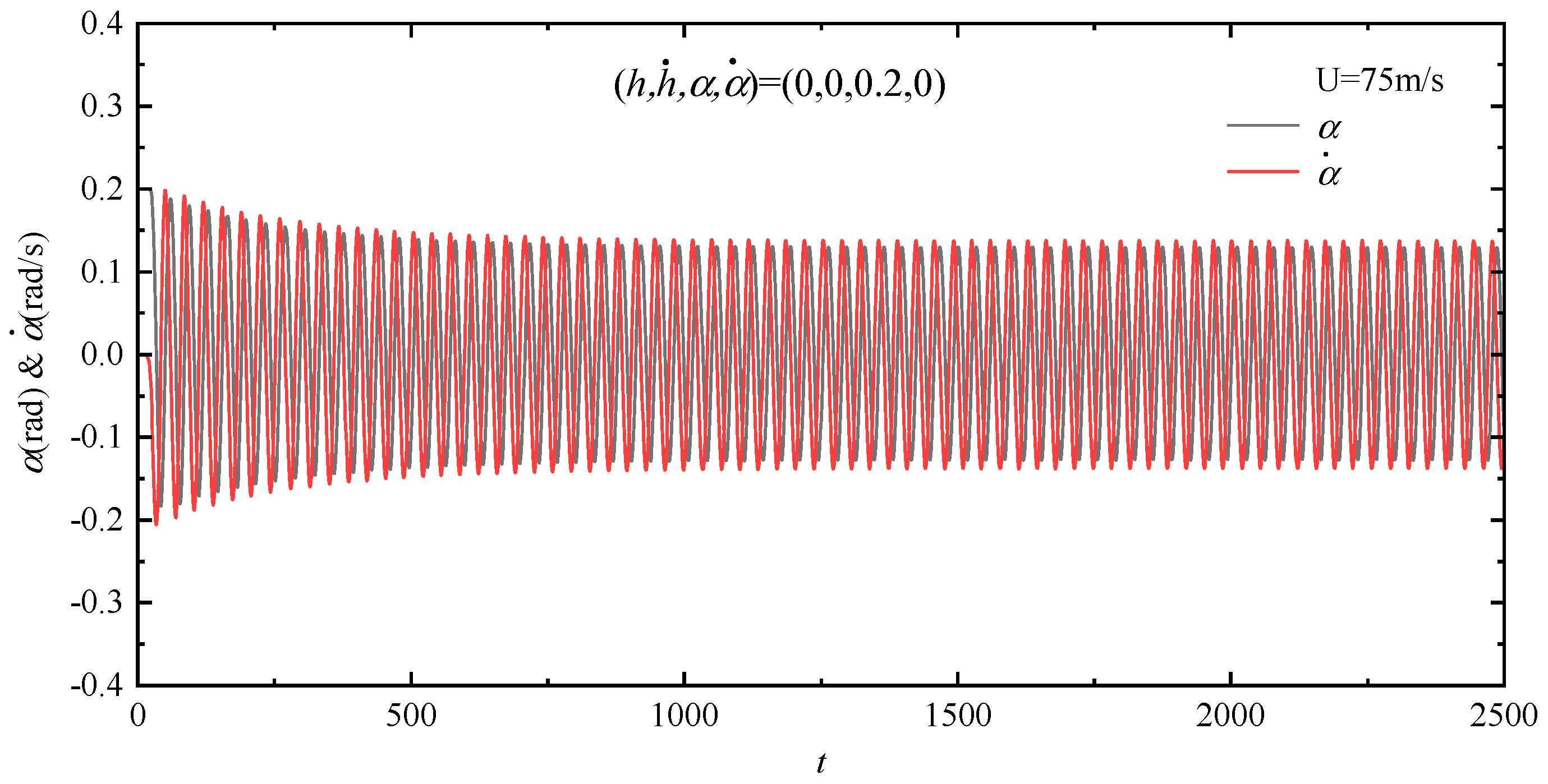
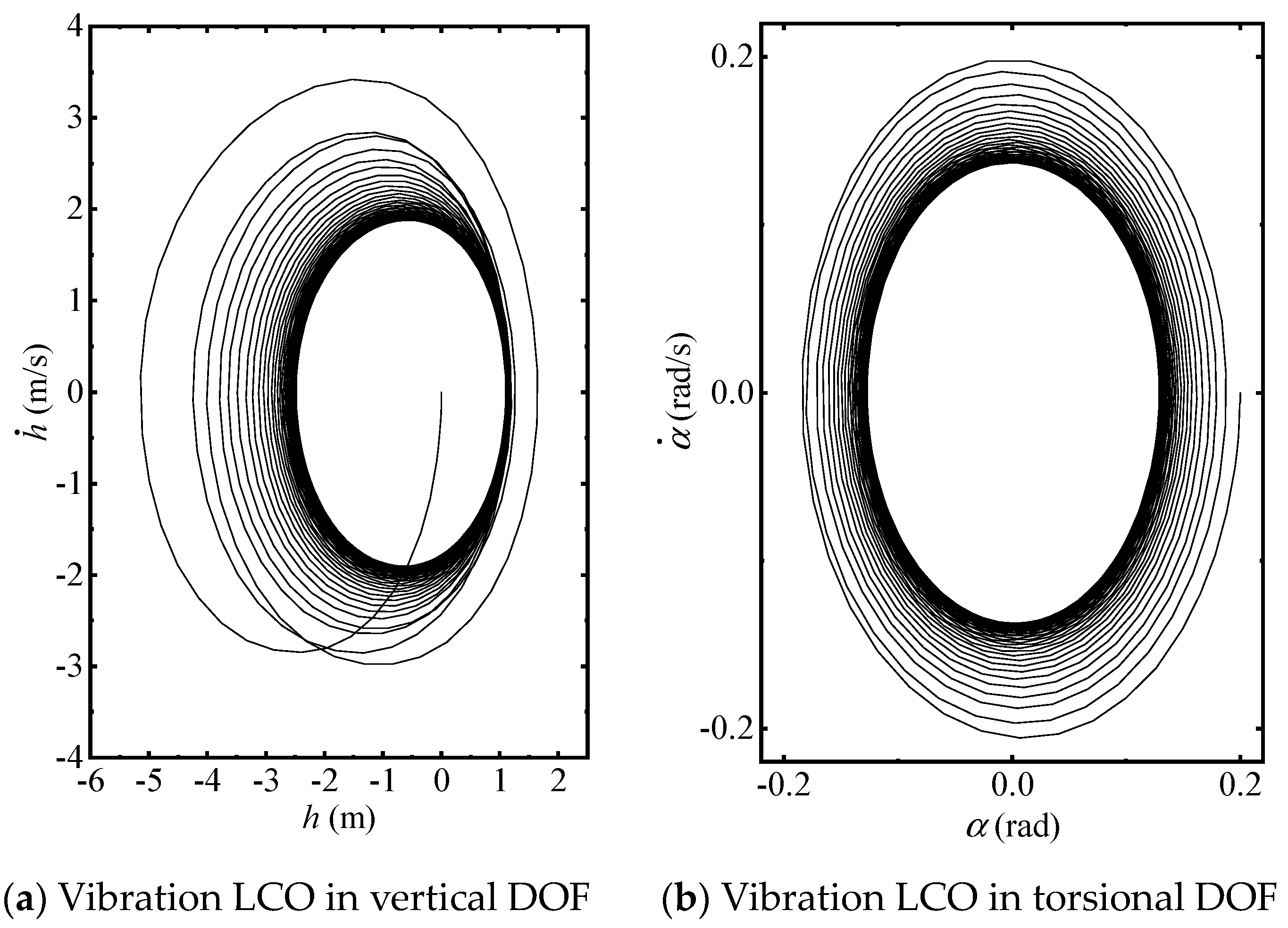
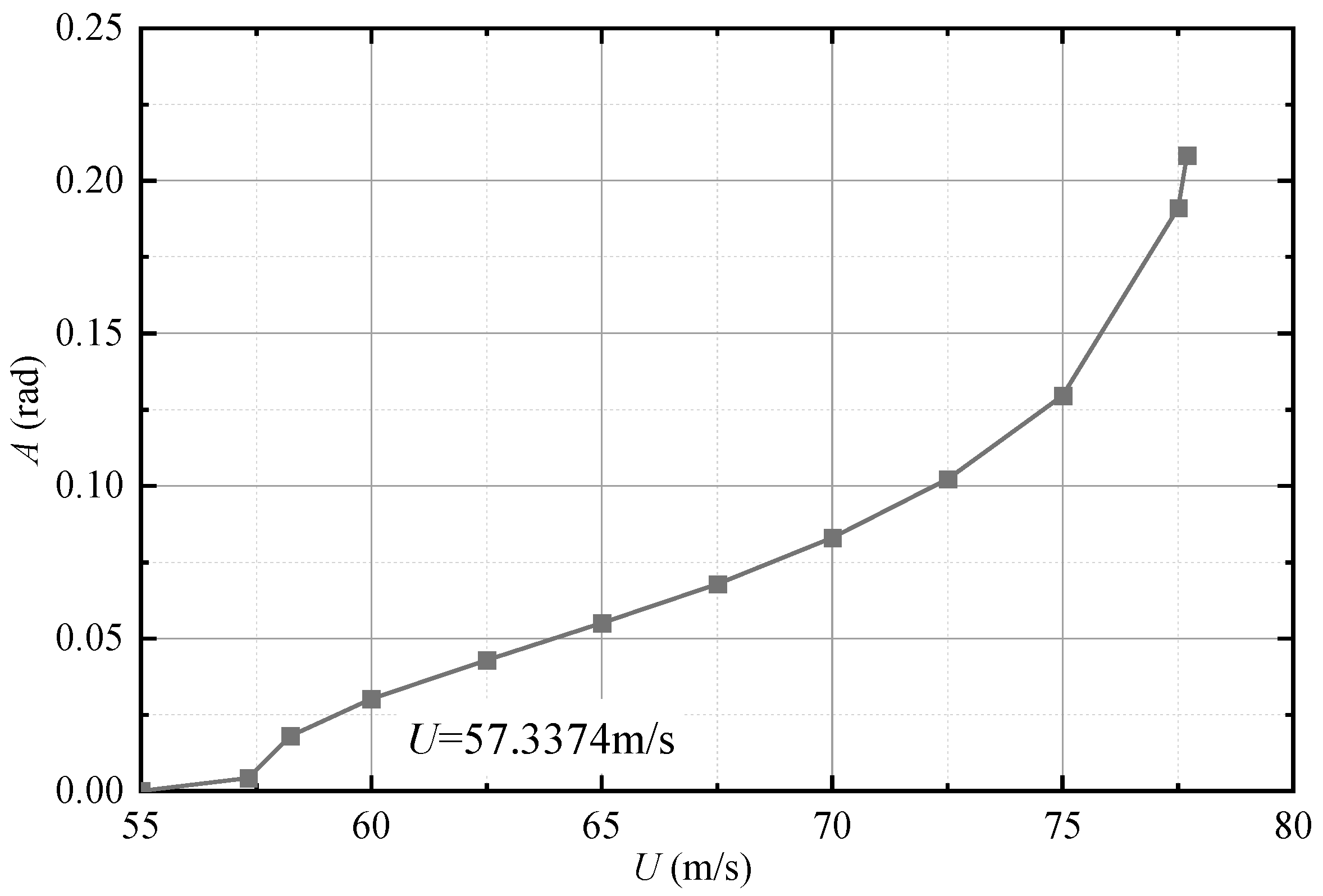
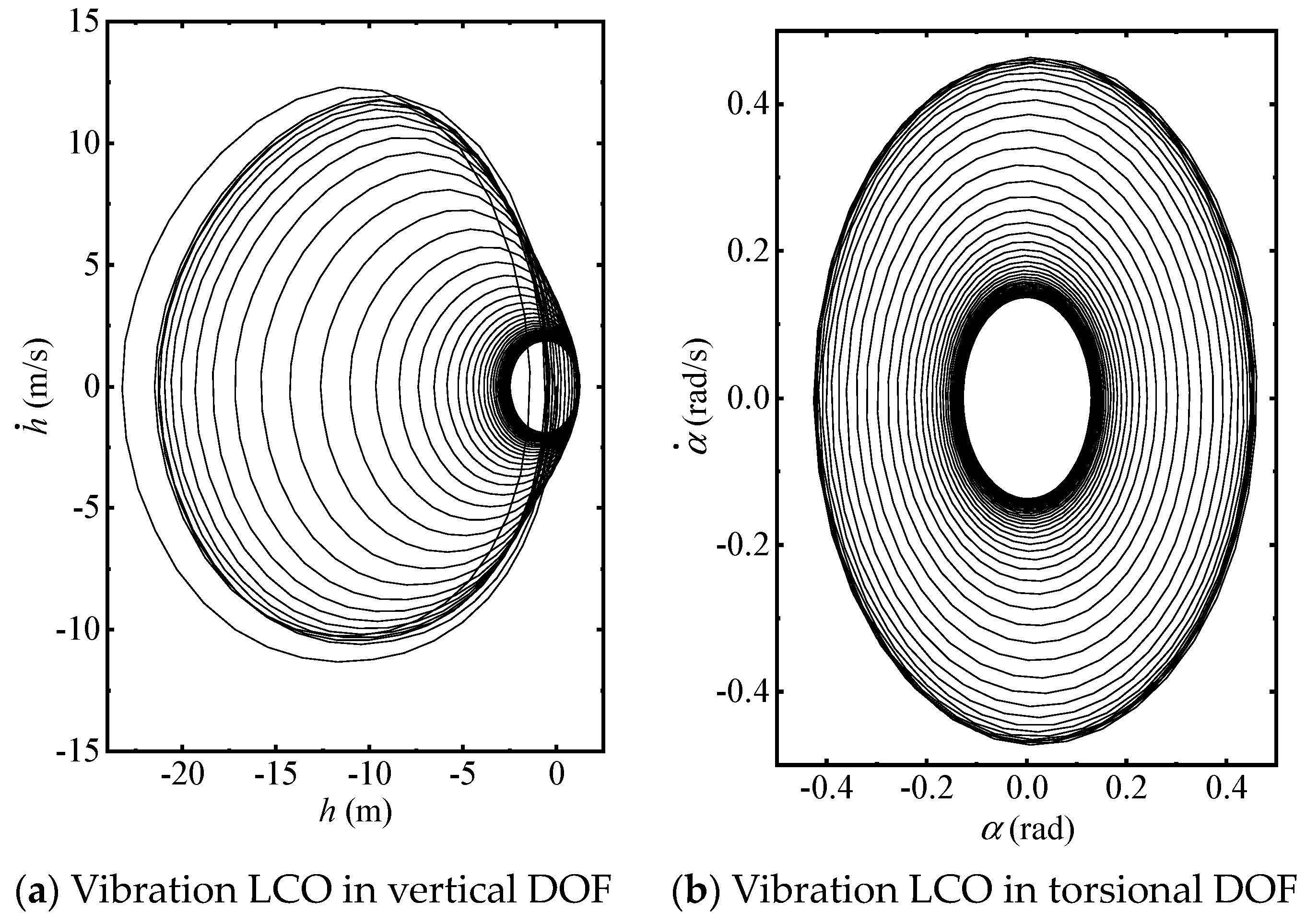
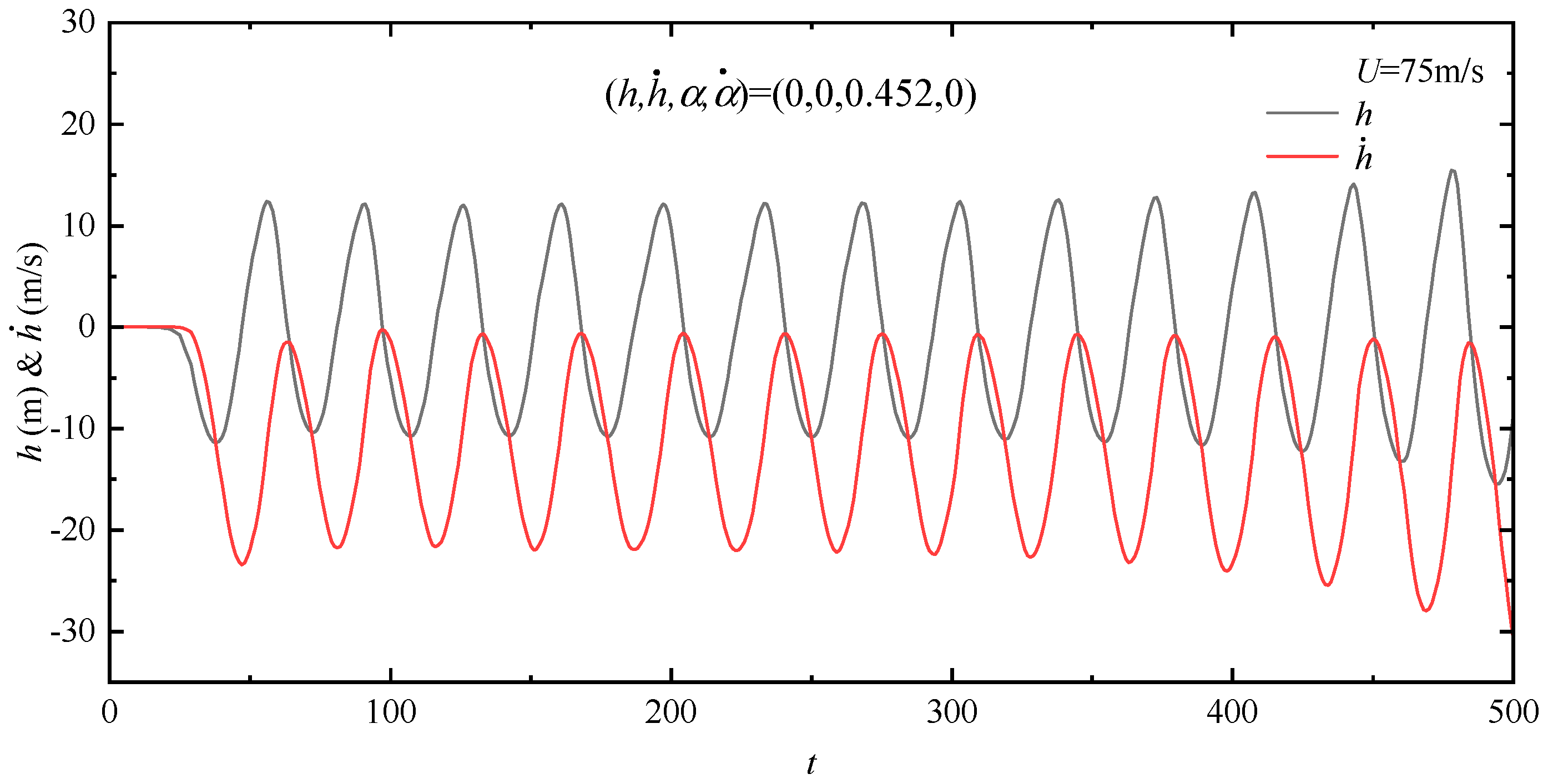
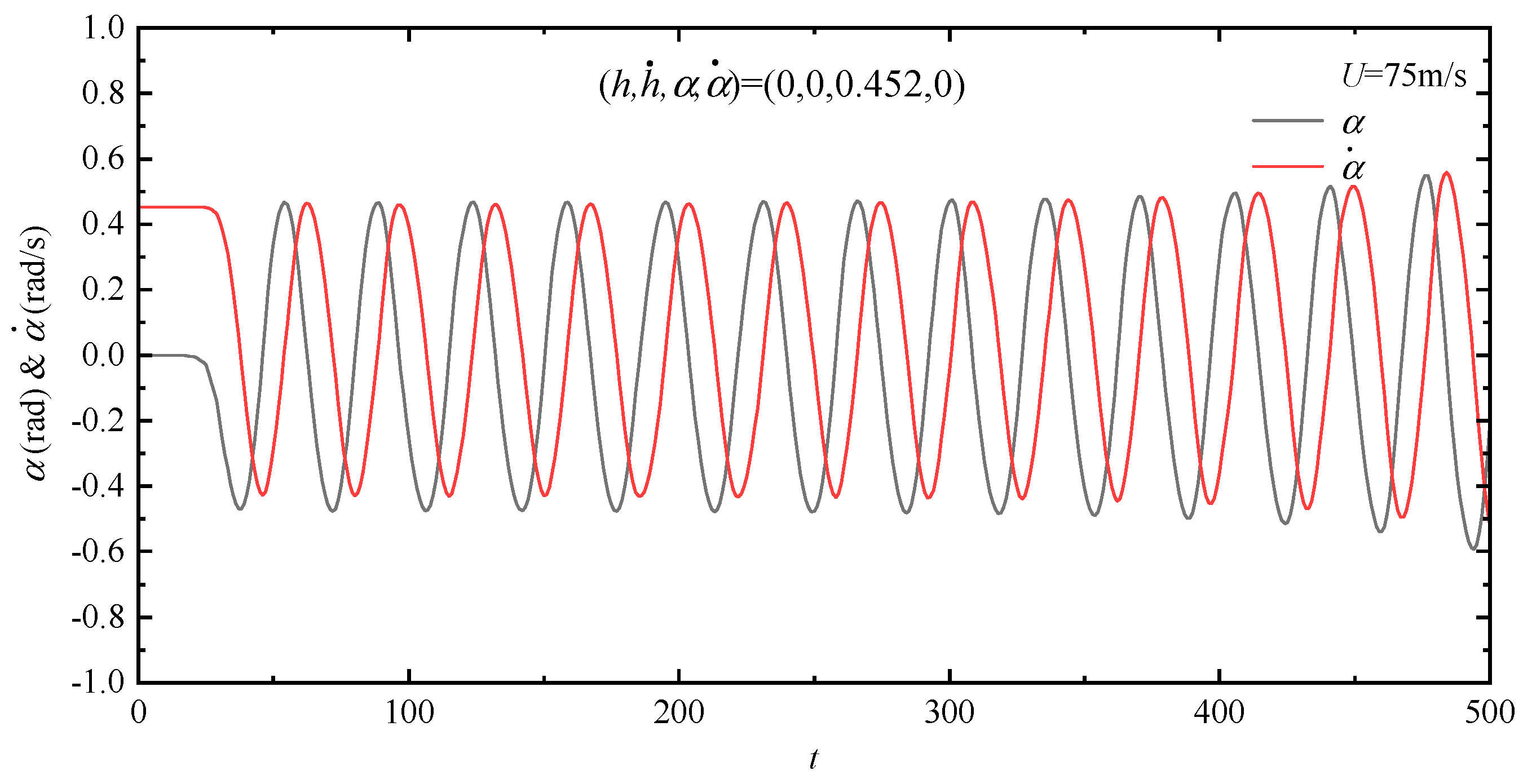
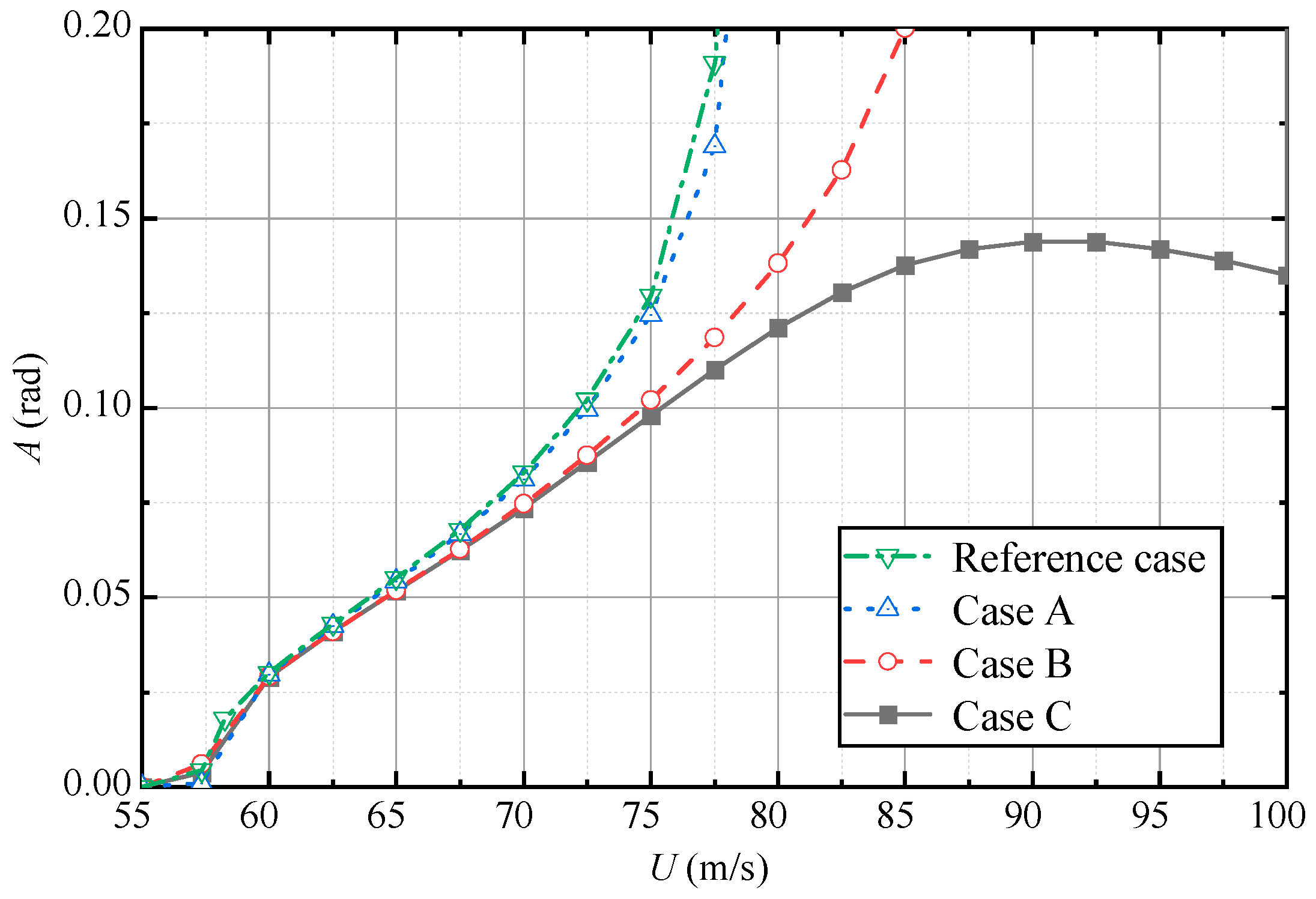
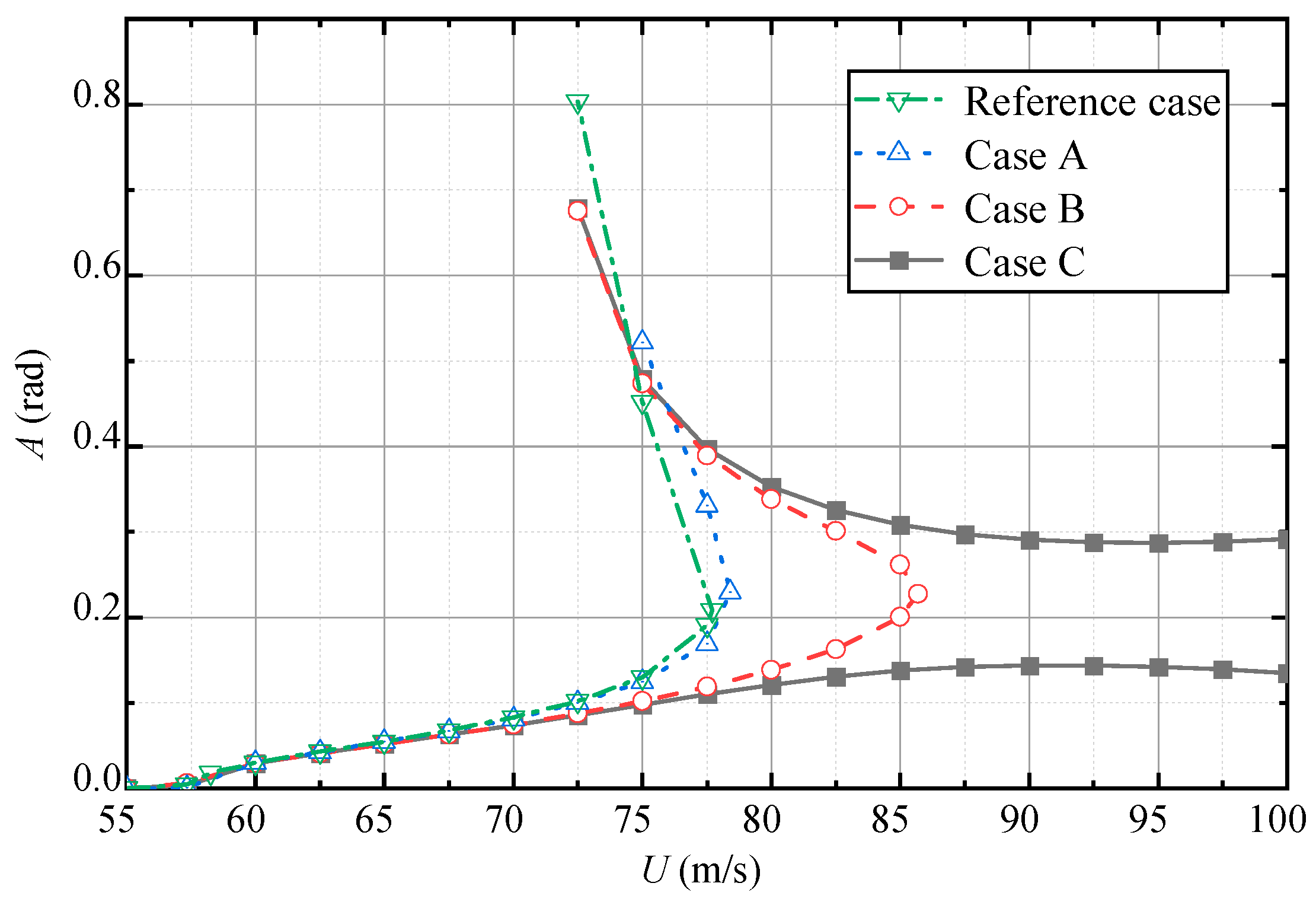
4. Analysis of Nonlinear Bifurcation and Limit Cycle Oscillation
- Wind Speed: Wind speed (beyond the critical state);
- Wind Speed: Wind speed (beyond the critical state);
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Amandolese, X.; Michelin, S.; Choquel, M. Low Speed Flutter and Limit Cycle Oscillations of a Two-Degree-of-Freedom Flat Plate in a Wind Tunnel. J. Fluids Struct. 2013, 43, 244–255. [Google Scholar] [CrossRef]
- Gao, G.; Zhu, L.; Han, W.; Li, J. Nonlinear Post-Flutter Behavior and Self-Excited Force Model of a Twin-Side-Girder Bridge Deck. J. Wind Eng. Ind. Aerodyn. 2018, 177, 227–241. [Google Scholar] [CrossRef]
- Giaccu, G.F.; Caracoglia, L. A Gyroscopic Stabilizer to Improve Flutter Performance of Long-Span Cable-Supported Bridges. Eng. Struct. 2021, 240, 112373. [Google Scholar] [CrossRef]
- Bera, K.K.; Banerjee, A. A Consistent Dynamic Stiffness Matrix for Flutter Analysis of Bridge Decks. Comput. Struct. 2023, 286, 107107. [Google Scholar] [CrossRef]
- Wu, L.; Woody Ju, J.; Zhang, J.; Zhang, M.; Li, Y. Vibration Phase Difference Analysis of Long-Span Suspension Bridge during Flutter. Eng. Struct. 2023, 276, 115351. [Google Scholar] [CrossRef]
- Náprstek, J.; Pospíšil, S.; Hračov, S. Analytical and Experimental Modelling of Non-Linear Aeroelastic Effects on Prismatic Bodies. J. Wind Eng. Ind. Aerodyn. 2007, 95, 1315–1328. [Google Scholar] [CrossRef]
- Král, R.; Pospíšil, S.; Náprstek, J. Wind Tunnel Experiments on Unstable Self-Excited Vibration of Sectional Girders. J. Fluids Struct. 2014, 44, 235–250. [Google Scholar] [CrossRef]
- Pigolotti, L.; Mannini, C.; Bartoli, G. Experimental Study on the Flutter-Induced Motion of Two-Degree-of-Freedom Plates. J. Fluids Struct. 2017, 75, 77–98. [Google Scholar] [CrossRef]
- Tang, Y.; Hua, X.G.; Chen, Z.Q.; Zhou, Y. Experimental Investigation of Flutter Characteristics of Shallow Π Section at Post-Critical Regime. J. Fluids Struct. 2019, 88, 275–291. [Google Scholar] [CrossRef]
- Wu, B.; Chen, X.; Wang, Q.; Liao, H.; Dong, J. Characterization of Vibration Amplitude of Nonlinear Bridge Flutter from Section Model Test to Full Bridge Estimation. J. Wind Eng. Ind. Aerodyn. 2020, 197, 104048. [Google Scholar] [CrossRef]
- Li, Z.; Wu, B.; Liao, H.; Li, M.; Wang, Q.; Shen, H. Influence of the Initial Amplitude on the Flutter Performance of a 2D Section and 3D Full Bridge with a Streamlined Box Girder. J. Wind Eng. Ind. Aerodyn. 2022, 222, 104916. [Google Scholar] [CrossRef]
- Liao, H.; Mei, H.; Hu, G.; Wu, B.; Wang, Q. Machine Learning Strategy for Predicting Flutter Performance of Streamlined Box Girders. J. Wind Eng. Ind. Aerodyn. 2021, 209, 104493. [Google Scholar] [CrossRef]
- Mostafa, K.; Zisis, I.; Moustafa, M.A. Machine Learning Techniques in Structural Wind Engineering: A State-of-the-Art Review. Appl. Sci. 2022, 12, 5232. [Google Scholar] [CrossRef]
- Tinmitondé, S.; He, X.; Yan, L.; Hounye, A.H. Data-Driven Prediction of Critical Flutter Velocity of Long-Span Suspension Bridges Using a Probabilistic Machine Learning Approach. Comput. Struct. 2023, 280, 107002. [Google Scholar] [CrossRef]
- Scanlan, R.H.; Tomko, J.J. Airfoil and Bridge Deck Flutter Derivatives. J. Eng. Mech. Div. 1971, 97, 1717–1737. [Google Scholar] [CrossRef]
- Náprstek, J.; Pospíšil, S. Response Types and General Stability Conditions of Linear Aero-Elastic System with Two Degrees-of-Freedom. J. Wind Eng. Ind. Aerodyn. 2012, 111, 1–13. [Google Scholar] [CrossRef]
- Náprstek, J.; Pospíšil, S.; Yau, J.-D. Stability of Two-Degrees-of-Freedom Aero-Elastic Models with Frequency and Time Variable Parametric Self-Induced Forces. J. Fluids Struct. 2015, 57, 91–107. [Google Scholar] [CrossRef]
- Gao, G.; Zhu, L.; Li, J.; Han, W.; Yao, B. A Novel Two-Degree-of-Freedom Model of Nonlinear Self-Excited Force for Coupled Flutter Instability of Bridge Decks. J. Sound Vib. 2020, 480, 115406. [Google Scholar] [CrossRef]
- Gao, G.; Zhu, L. Nonlinearity of Mechanical Damping and Stiffness of a Spring-Suspended Sectional Model System for Wind Tunnel Tests. J. Sound Vib. 2015, 355, 369–391. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F.; Ying, X. Experimental Investigations on the Nonlinear Torsional Flutter of a Bridge Deck. J. Bridge Eng. 2017, 22, 04017048. [Google Scholar] [CrossRef]
- Tesfaye, S.; Kavrakov, I.; Morgenthal, G. Numerical Investigation of the Nonlinear Interaction between the Sinusoidal Motion-Induced and Gust-Induced Forces Acting on Bridge Decks. J. Fluids Struct. 2022, 113, 103680. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F.; Zhang, Z.; Ying, X. Energy Budget Analysis and Engineering Modeling of Post-Flutter Limit Cycle Oscillation of a Bridge Deck. J. Wind Eng. Ind. Aerodyn. 2019, 188, 410–420. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F.; Han, Y. Assessment of Wind-Induced Nonlinear Post-Critical Performance of Bridge Decks. J. Wind Eng. Ind. Aerodyn. 2020, 203, 104251. [Google Scholar] [CrossRef]
- Li, K.; Han, Y.; Cai, C.S.; Hu, P.; Li, C. Experimental Investigation on Post-Flutter Characteristics of a Typical Steel-Truss Suspension Bridge Deck. J. Wind Eng. Ind. Aerodyn. 2021, 216, 104724. [Google Scholar] [CrossRef]
- Wu, B.; Shen, H.; Liao, H.; Wang, Q.; Zhang, Y.; Li, Z. Insight into the Intrinsic Time-Varying Aerodynamic Properties of a Truss Girder Undergoing a Flutter with Subcritical Hopf Bifurcation. Commun. Nonlinear Sci. Numer. Simul. 2022, 112, 106472. [Google Scholar] [CrossRef]
- Casalotti, A.; Arena, A.; Lacarbonara, W. Mitigation of Post-Flutter Oscillations in Suspension Bridges by Hysteretic Tuned Mass Dampers. Eng. Struct. 2014, 69, 62–71. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F. Tuned Mass Damper for Self-Excited Vibration Control: Optimization Involving Nonlinear Aeroelastic Effect. J. Wind Eng. Ind. Aerodyn. 2022, 220, 104836. [Google Scholar] [CrossRef]
- Scanlan, R.H. The Action of Flexible Bridges under Wind, I: Flutter Theory. J. Sound Vib. 1978, 60, 187–199. [Google Scholar] [CrossRef]
- Hong, G. Identifying Long-Span Bridge Flutter Derivatives via the Free Vibration Method Based on the Fluent Software. Master’s Thesis, Chang’an University, Xi’an, China, 2012. [Google Scholar]
- Andronov, A.A.; Leontovich, E.A.; Gordon, I.I.; Maier, A.G. Qualitative Theory of Second-Order Dynamic Systems; Halsted Press: New York, NY, USA, 1973; ISBN 0-470-03195-6. [Google Scholar]
- Theodorsen, T. General Theory of Aerodynamic Instability and the Mechanism of Flutter; NACA Technical Report; Langley: Newport News, VA, USA, 1935. [Google Scholar]
- Runge, C. Ueber die numerische Auflösung von Differentialgleichungen. Math. Ann. 1895, 46, 167–178. [Google Scholar] [CrossRef]








| Parameter | Value |
|---|---|
| 65,649 (kg/m) | |
| 7,808,150 (kg∙m) | |
| 41.7 (m) | |
| 0.005 | |
| 0.005 | |
| 0.45497 (rad/s) | |
| 1.33285 (rad/s) |
| Cases Name | |||
|---|---|---|---|
| Reference case | |||
| Case A | |||
| Case B | |||
| Case C |
| 0.04374 | −0.05154 | 0.06288 | −0.00187 | ||
| 0.26574 | −0.08902 | −0.01230 | 0.01217 | ||
| −0.27729 | 0.02632 | −0.01665 | 7.49839 × 10−4 | ||
| −3.76111 | 0.70966 | 0.95929 | −0.13286 | ||
| 5.81372 | −0.74839 | −9.99665 | 1.13183 | ||
| 0.06882 | −0.00366 | ||||
| 6.52151 | −0.82327 |
| entry 1 | 0.29832 | −0.17132 | 0.03441 | −0.00224 |
| entry 2 | 0.00214 | −0.00314 | 3.55170 × 10−4 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Wang, F.; Yang, Y. Study of Nonlinear Aerodynamic Self-Excited Force in Flutter Bifurcation and Limit Cycle Oscillation of Long-Span Suspension Bridge. Appl. Sci. 2023, 13, 10272. https://doi.org/10.3390/app131810272
Liu J, Wang F, Yang Y. Study of Nonlinear Aerodynamic Self-Excited Force in Flutter Bifurcation and Limit Cycle Oscillation of Long-Span Suspension Bridge. Applied Sciences. 2023; 13(18):10272. https://doi.org/10.3390/app131810272
Chicago/Turabian StyleLiu, Jieshan, Fan Wang, and Yang Yang. 2023. "Study of Nonlinear Aerodynamic Self-Excited Force in Flutter Bifurcation and Limit Cycle Oscillation of Long-Span Suspension Bridge" Applied Sciences 13, no. 18: 10272. https://doi.org/10.3390/app131810272
APA StyleLiu, J., Wang, F., & Yang, Y. (2023). Study of Nonlinear Aerodynamic Self-Excited Force in Flutter Bifurcation and Limit Cycle Oscillation of Long-Span Suspension Bridge. Applied Sciences, 13(18), 10272. https://doi.org/10.3390/app131810272






