Tooth Profile Construction and Experimental Verification of Non-Circular Gear Based on Double Arc Active Design
Abstract
:1. Introduction
2. Design of Double Arc Pitch Curve
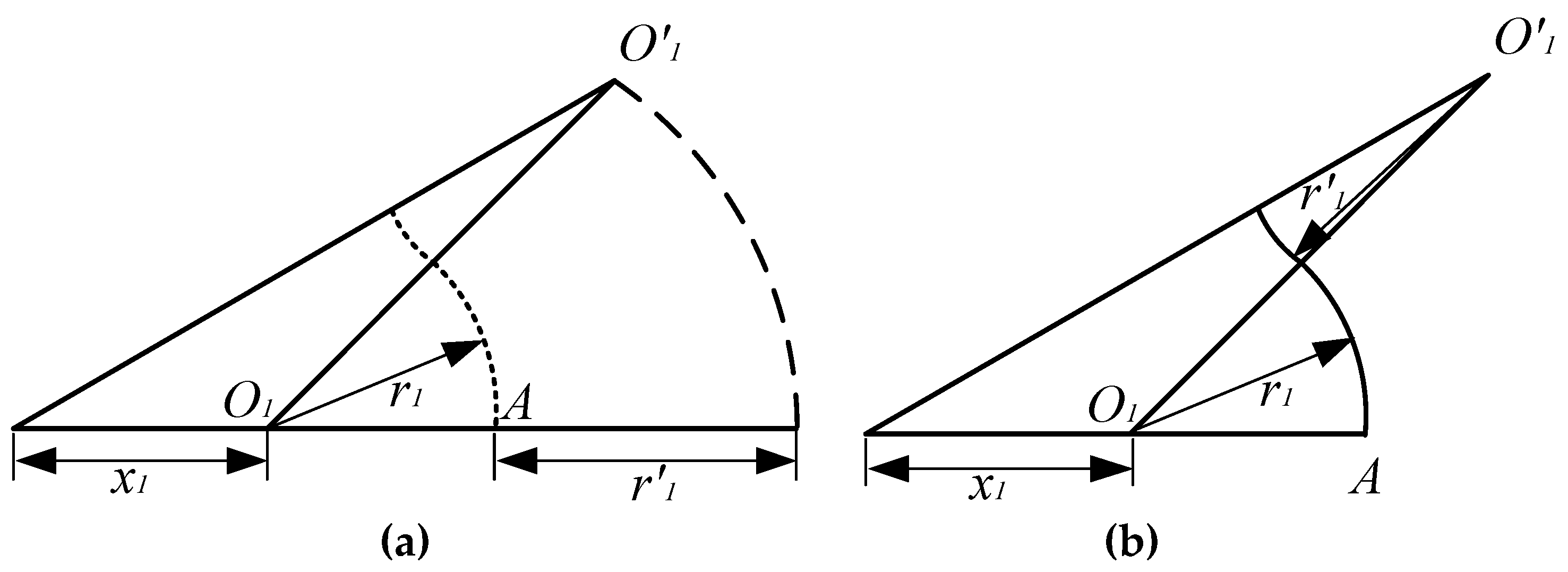
2.1. The Model for the Nonlinear Solution
2.2. Local Optimal Solution Processing for Nonlinear Solution Models
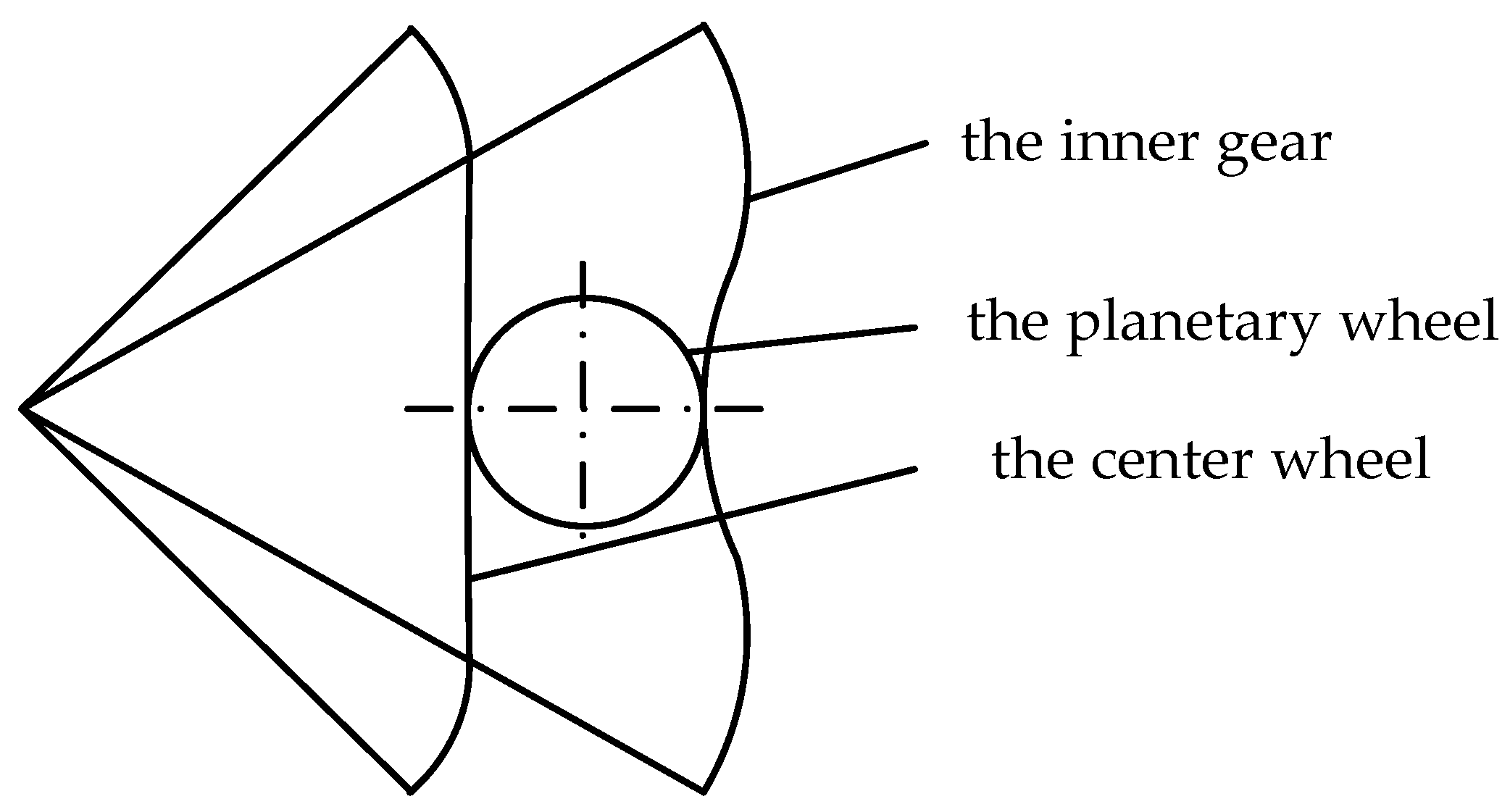
3. The Active Design of the Pitch Circle of the Integer Gear Model Building
3.1. Selection of Non-Integer and Integer Teeth
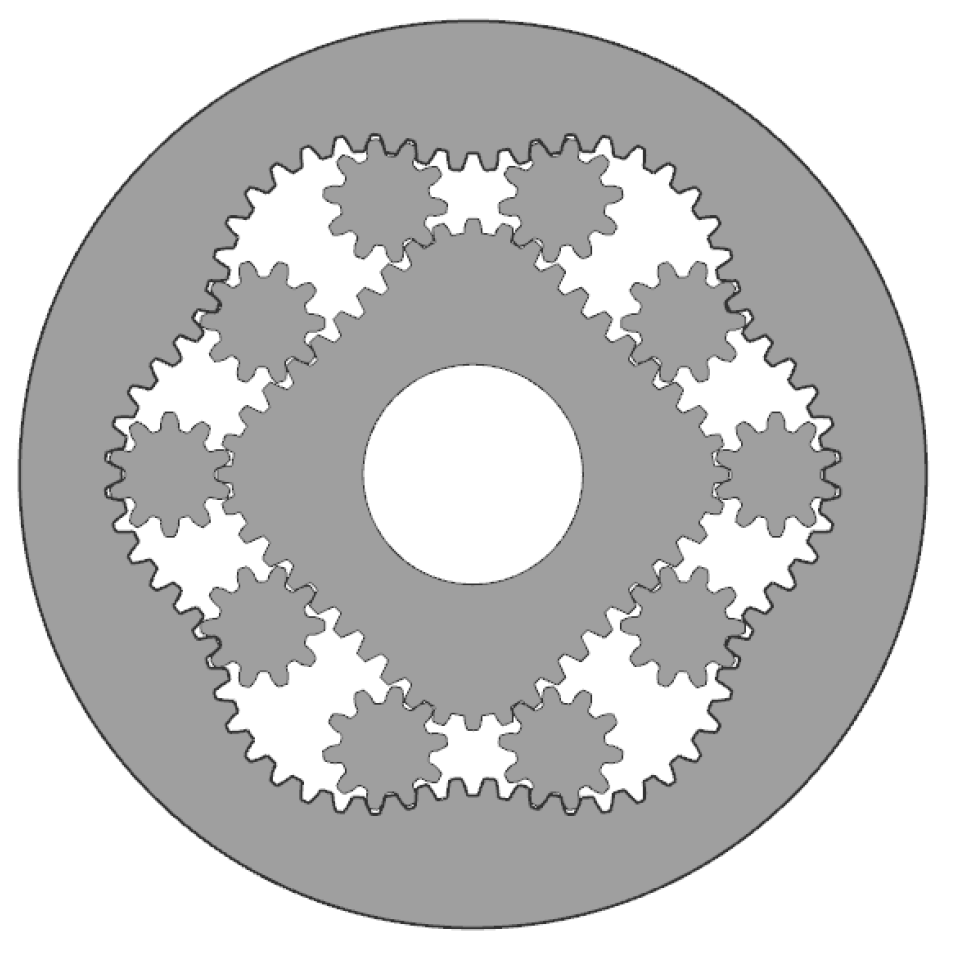
3.2. Pitch Circle Model of Double Arc Non-Circular Gears
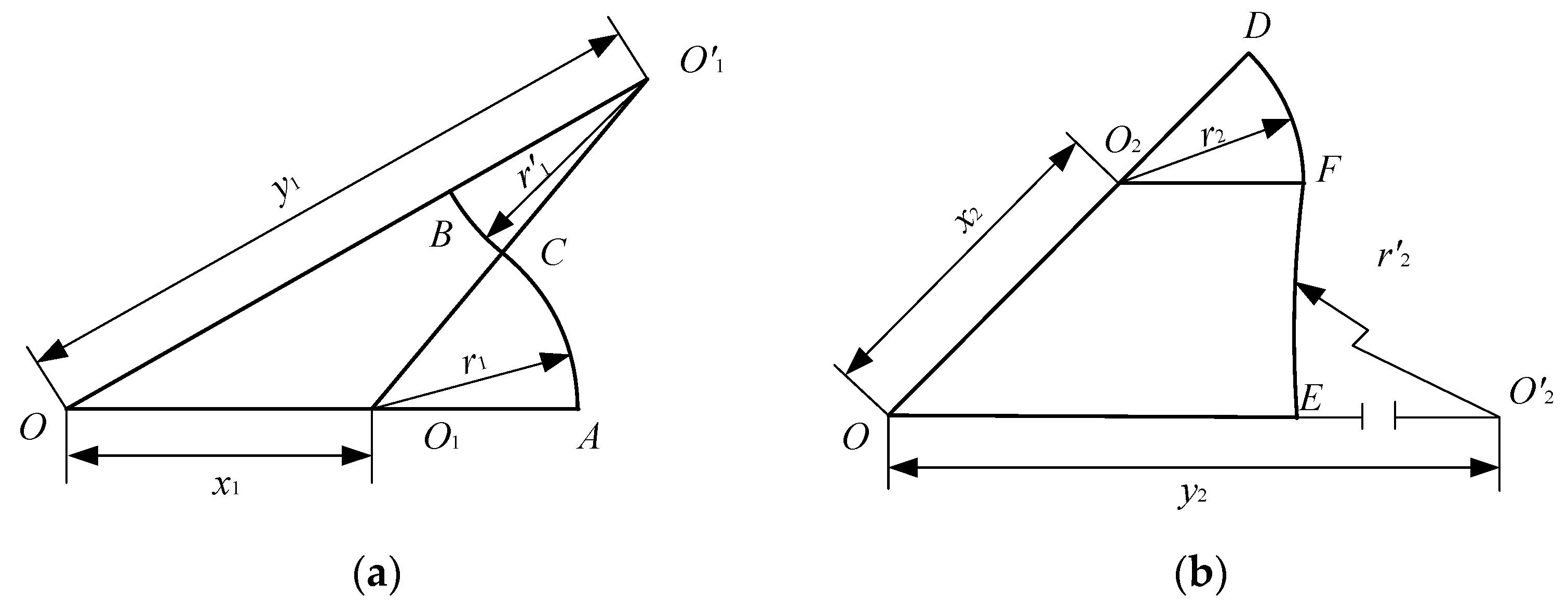
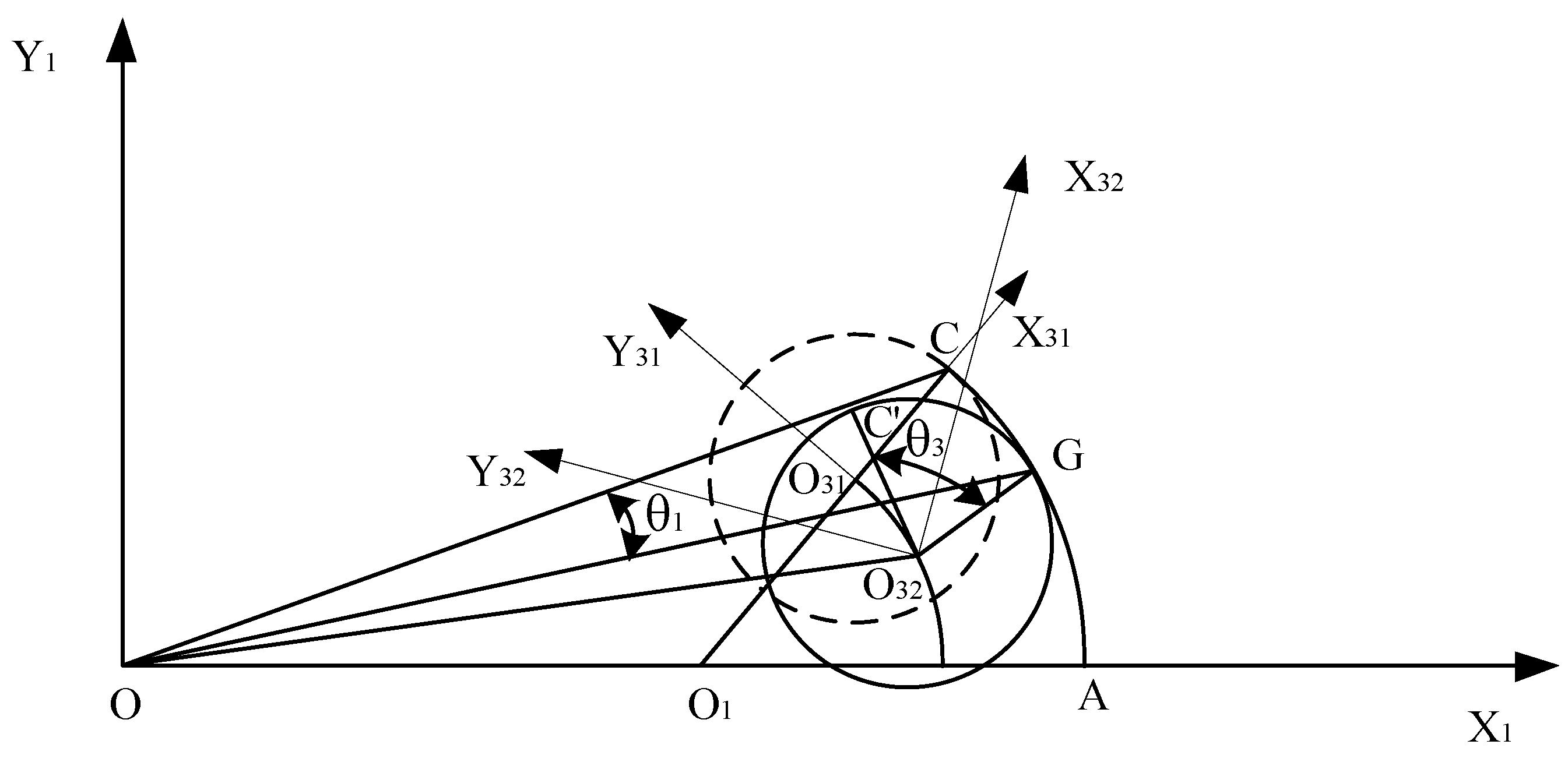
3.3. Treatment of Tooth Profile at the Point of Intersection of the Double Arc
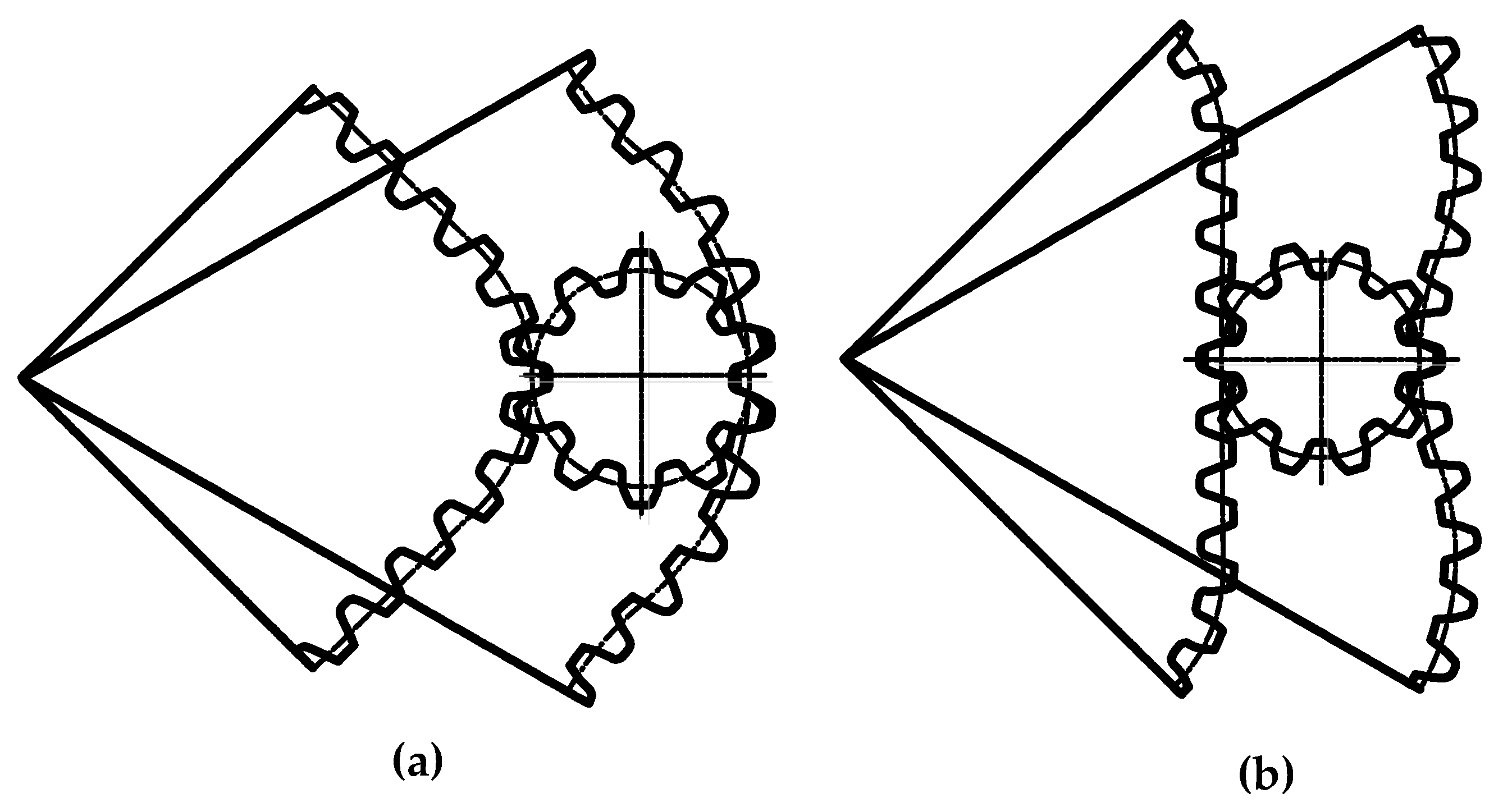
4. Modeling and Simulation of Non-Circular Gears
4.1. Determination of Basic Gear Geometric Parameters
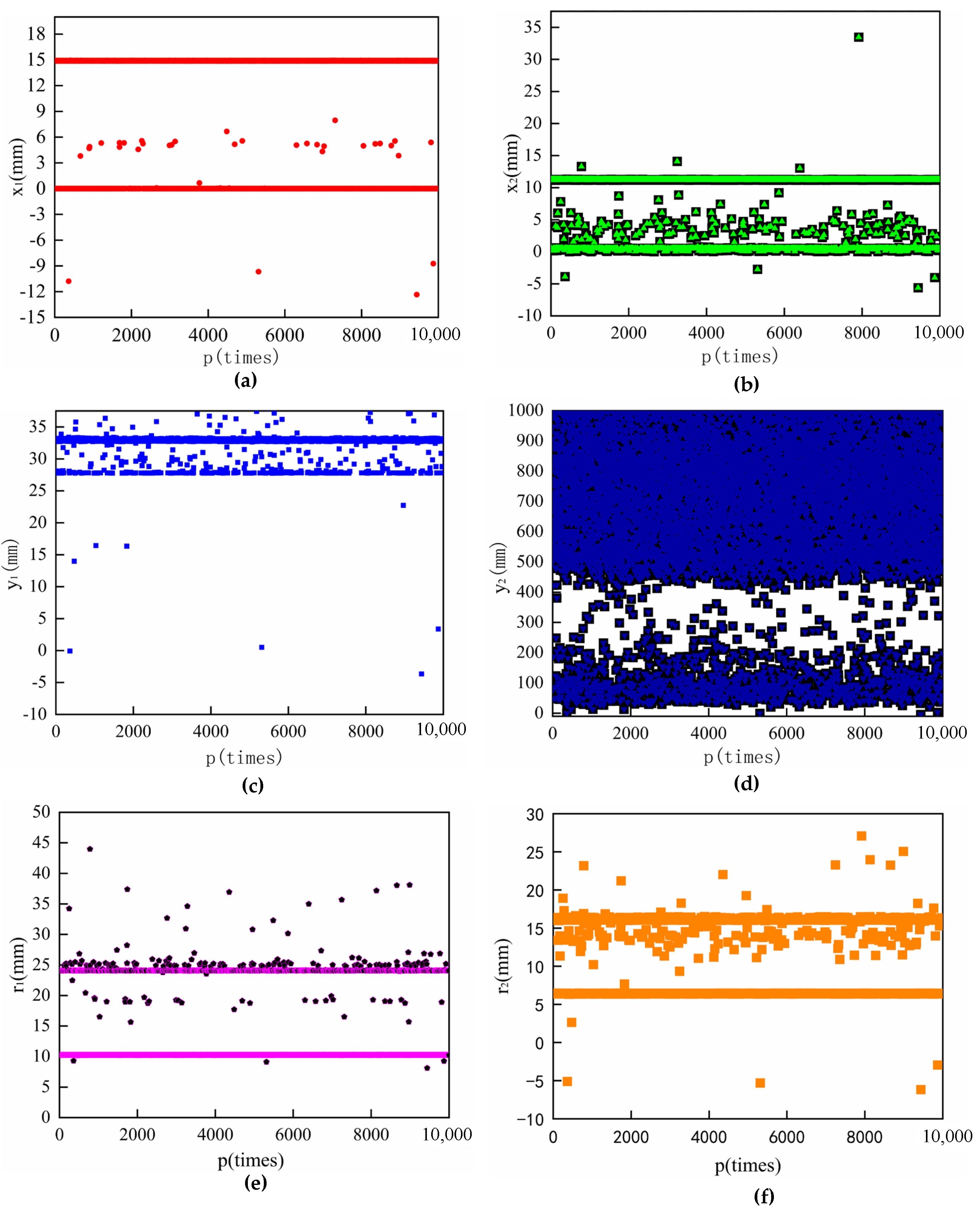
4.2. Orthogonal Experiments of Non-Circular Gears Based on the Pitch Circle Model
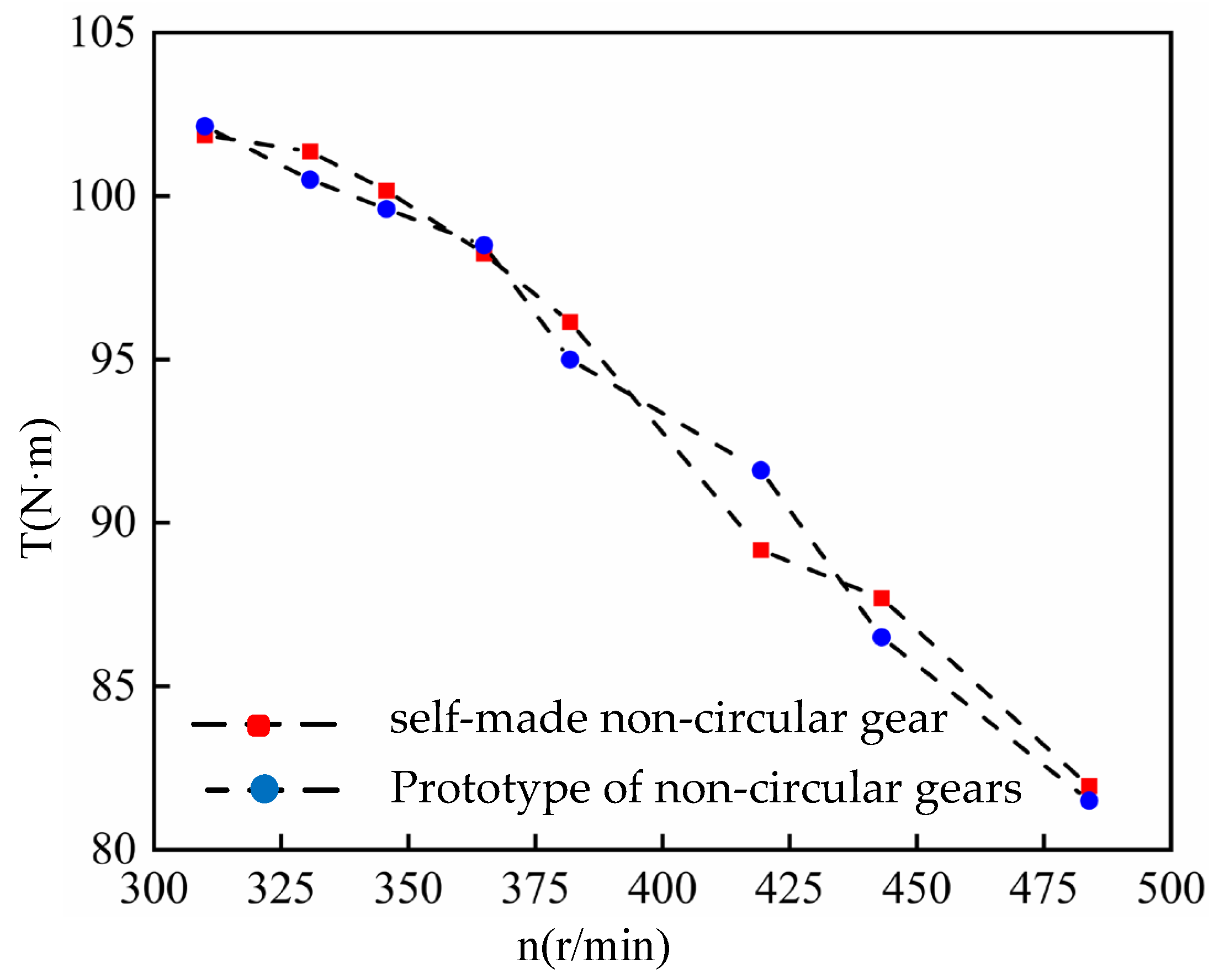
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xiong, Z.Q.; Wu, X.T.; Gao, B.H. Parametric design of non-circular planetary gear hydraulic motors. Mach. Tool Hydraul. 2004, 5, 50–52. [Google Scholar]
- Huang, Z.D. Optimization design and analysis of stamping mechanism based on noncircular gear. Manuf. Autom. 2022, 44, 85–87+92. [Google Scholar]
- Ye, J.; Chen, J.; Yu, C.; Shen, S.; Xue, M.; Ye, Z. Study of three-joint posture-constrained single-drive non-circular gear five-bar finger mechanism. Trans. Chin. Soc. Agric. Mach. 2022, 53, 430–437. [Google Scholar]
- Li, J.S.; Li, H.M. Design of pitch curve for non-circular planetary gear mechanism with variable center distance. Mech. Transm. 1993, 17, 1–3. [Google Scholar]
- Li, J.S.; Li, H.M. Study the motion law of non-circular planetary gear mechanism with variable center distance. Mech. Transm. 1994, 18, 16–19. [Google Scholar]
- Li, J.S.; Li, H.M. Performance analysis of different types of non-circular planetary gear hydraulic motors. China Mech. Eng. 1994, 5, 21–23+77–78. [Google Scholar]
- Zhang, Q.J.; Center, Z.J.; Yang, X.Y.; Wang, K.X. Study on Optimal Design Calculation Method of Tooth Profile Curve of Noncircular Gear Train. Mod. Inf. Technol. 2022, 6, 151–155. [Google Scholar]
- Tang, D.W. Study of CADCAM System and Measurement Scheme for New Non-Circular Gear Hydraulic Motor. Ph.D. Thesis, Harbin Institute of Technology, Harbin, China, 2000. [Google Scholar]
- Liao, X. Tooth Profile Calculation and Performance Analysis of Non-Circular Planetary Gear Hydraulic Motor; Beijing Institute of Machinery Industry: Beijing, China, 2007. [Google Scholar]
- Liu, Y.P.; Fu, W.X.; Wei, Y.Q.; Li, D.W. Parametric design and motion simulation analysis of non-circular gear planetary wheel system. Mech. Transm. 2021, 45, 70–75. [Google Scholar]
- Xu, H.H.; Shen, B.M. Non-circular planetary gear mechanism with double-arc pitch curve. J. China Coal Soc. 2010, 35, 691–695. [Google Scholar]
- Shen, B.M.; Xu, H.H. Arc-arc Type Non-circular Gear Mechanism for a Hydraulic Motor. Mech. Sci. Technol. Aerosp. Eng. 2010, 30, 112–115. [Google Scholar]
- Xu, H.H.; Xu, J. Determination of the number of teeth of a non-circular planetary gear system of order 4 to 6. J. China Coal Soc. 2015, 36, 37–39. [Google Scholar]
- Ogawa, K.; Yokoyama, Y.; Koshiba, T. Studies on the Noncircular Planetary Gear Mechanisms with Nonuniform Motion. Bull. JSME 1973, 16, 1433–1442. [Google Scholar] [CrossRef]
- Yokoyama, Y.; Ogawa, K.; Kurebayashi, S. Studies on the Noncircular Planetary Gear Mechanisms. Bull. JSME 1982, 25, 2046–2051. [Google Scholar] [CrossRef]
- Michal, K. Designing Elliptical Gears. Mach. Des. 1988, 60, 116–118. [Google Scholar]
- Deptuła, A.; Drewniak, J.; Partyka, M.A. Chosen Aspects of Analysis and Synthesis of Coupled and Complex Planetary Gears via Search and Contour Graphs Modelling; Mechanisms and Machine Science Vol. 107; Springer: Cham, Switzerland; Opole, Poland, 2022; Volume I, pp. 45–69. [Google Scholar]
- Deptuła, A.; Drewniak, J.; Partyka, M.A. The Method of Searching Trees in Determining of the Optimal Number of Wheel Teeth for a Compound Planetary Gear. In Advances in Mechanism and Machine Science, Proceedings of the IFToMM WC 2019, Krakow, Poland, 30 June–4 July 2019; Springer: Opole, Poland, 2019; pp. 2853–2862. [Google Scholar]
- Brown, A.R.; Johnson, L.C. Optimizing Non-Circular Gear Profiles for Enhanced Efficiency in Hydraulic Motors. Int. J. Fluid Power 2020, 42, 189–204. [Google Scholar]
- Litwin, T.; Smith, J.K. Advances in Non-Circular Gear Design for Hydraulic Motors. J. Mech. Eng. 2018, 25, 456–473. [Google Scholar]
- Wang, Z.; He, G.; Du, W.; Zhou, J.; Kou, Y. Application of parameter optimized variational mode decomposition method in fault diagnosis of the gearbox. IEEE Access 2019, 7, 44871–44882. [Google Scholar] [CrossRef]
- Wang, Y.; Qian, Q.; Chen, G.; Jin, S.; Yong, C. Multi-objective optimization design of cycloid pin gear planetary reducer. Adv. Mech. Eng. 2017, 1–10. [Google Scholar] [CrossRef]
- Liu, Z.P.; Su, J.X.; Deng, X.Z.; Xu, Z.J. Multi-objective optimization-based calculation and simulation of machining parameters for water motors with modified non-circular planetary gears. Mech. Transm. 2015, 39, 86–89. [Google Scholar]
- Huang, K.; Ma, J.Q.; Xia, G.C.; Zhou, H.Z.; Zhang, Z.F. Interval multi-objective optimization for the specific power of a helicopter’s main reducer planetary gear trains. J. Aerosp. Power 2017, 32, 2447–2455. [Google Scholar]
- Li, D.; Liu, Y.; Gong, J.; Wang, T. Design of a Noncircular Planetary Gear Mechanism for Hydraulic Motor. Math. Probl. Eng. 2021, 2021, 5510521. [Google Scholar] [CrossRef]
- Cui, S.H.; Liu, J.H.; Song, W.H.; Hu, S.L. Nonlinear solutions based on the proposed Newtonian method and applications. Aerosp. Shanghai 2013, 30, 16–18+64. [Google Scholar]
- Liu, F.; Liang, L.; Xu, G.H.; Hou, C.G.; Liu, D. Four-Point Method in the Measurement and Separation of Spindle Rotation Error. IEEE/A SME Trans. Mechatron. 2021, 26, 113–123. [Google Scholar] [CrossRef]
- Zeng, T. Spiral Bevel Gear Design and Machining; Harbin Institute of Technology Press: Hengyang, China, 1989. [Google Scholar]
- Li, J. Numerical Simulation and Experimental Research of Spur Cylindrical Gear Rolling; Shandong University: Jinan, China, 2007. [Google Scholar]

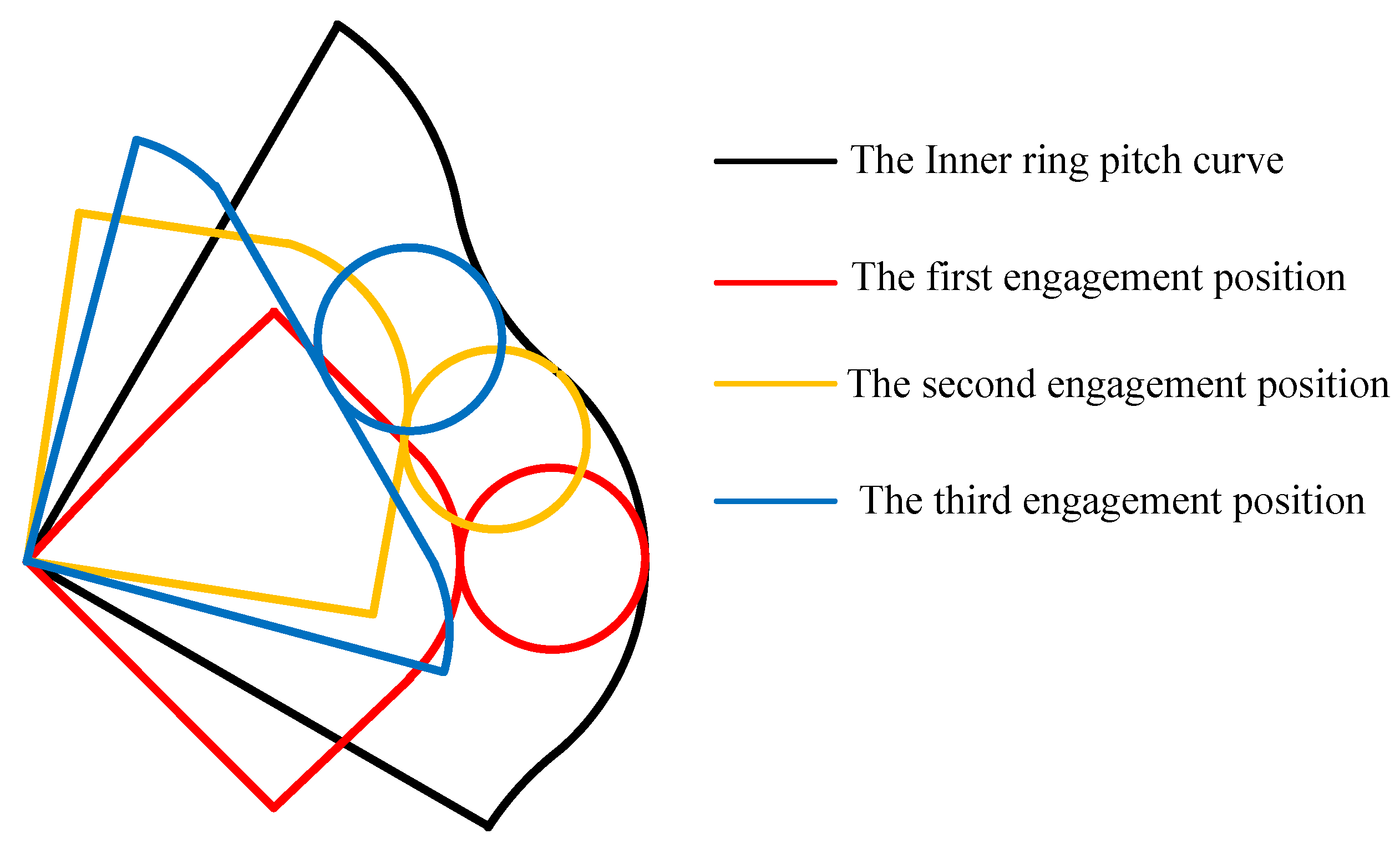
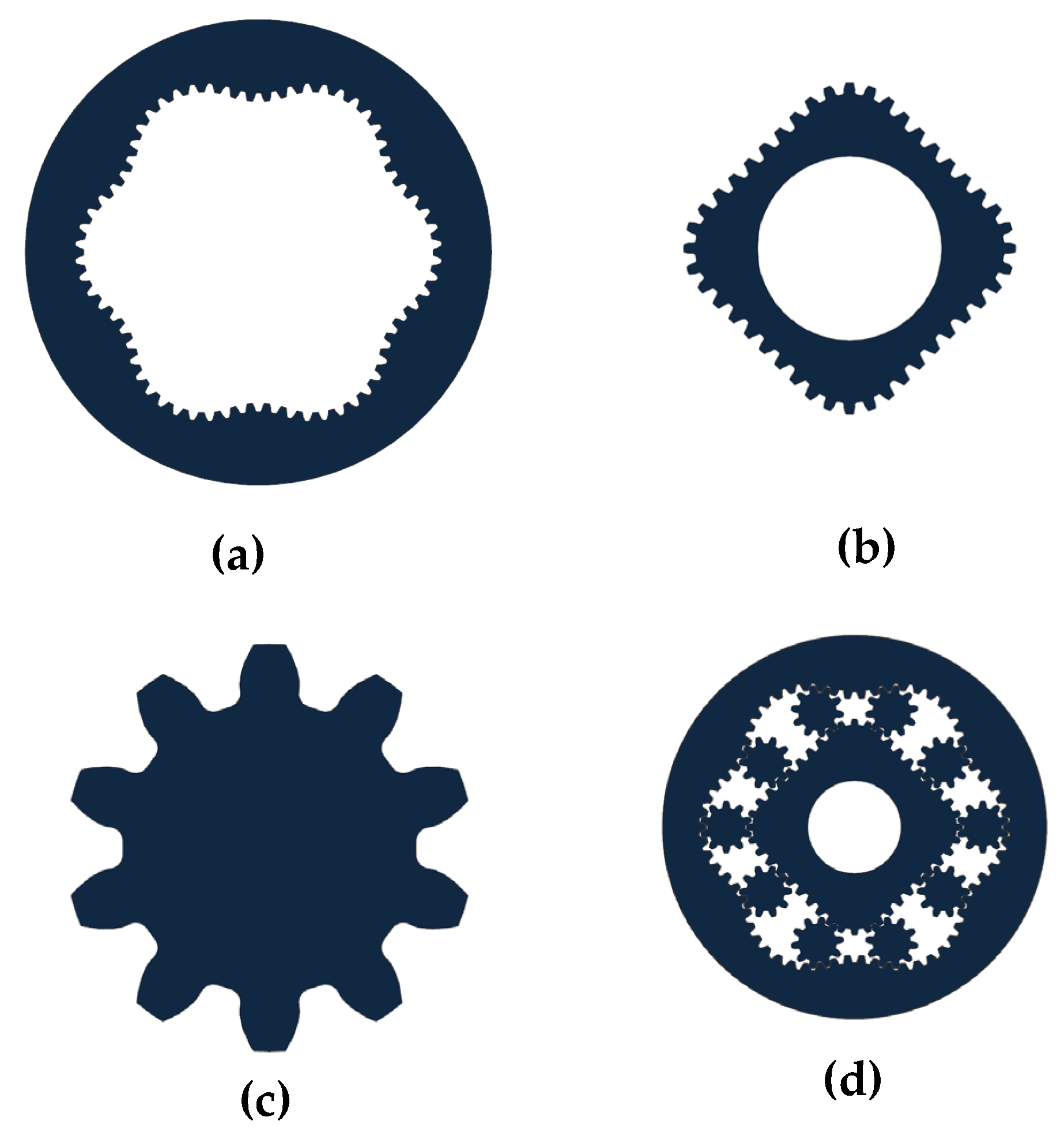
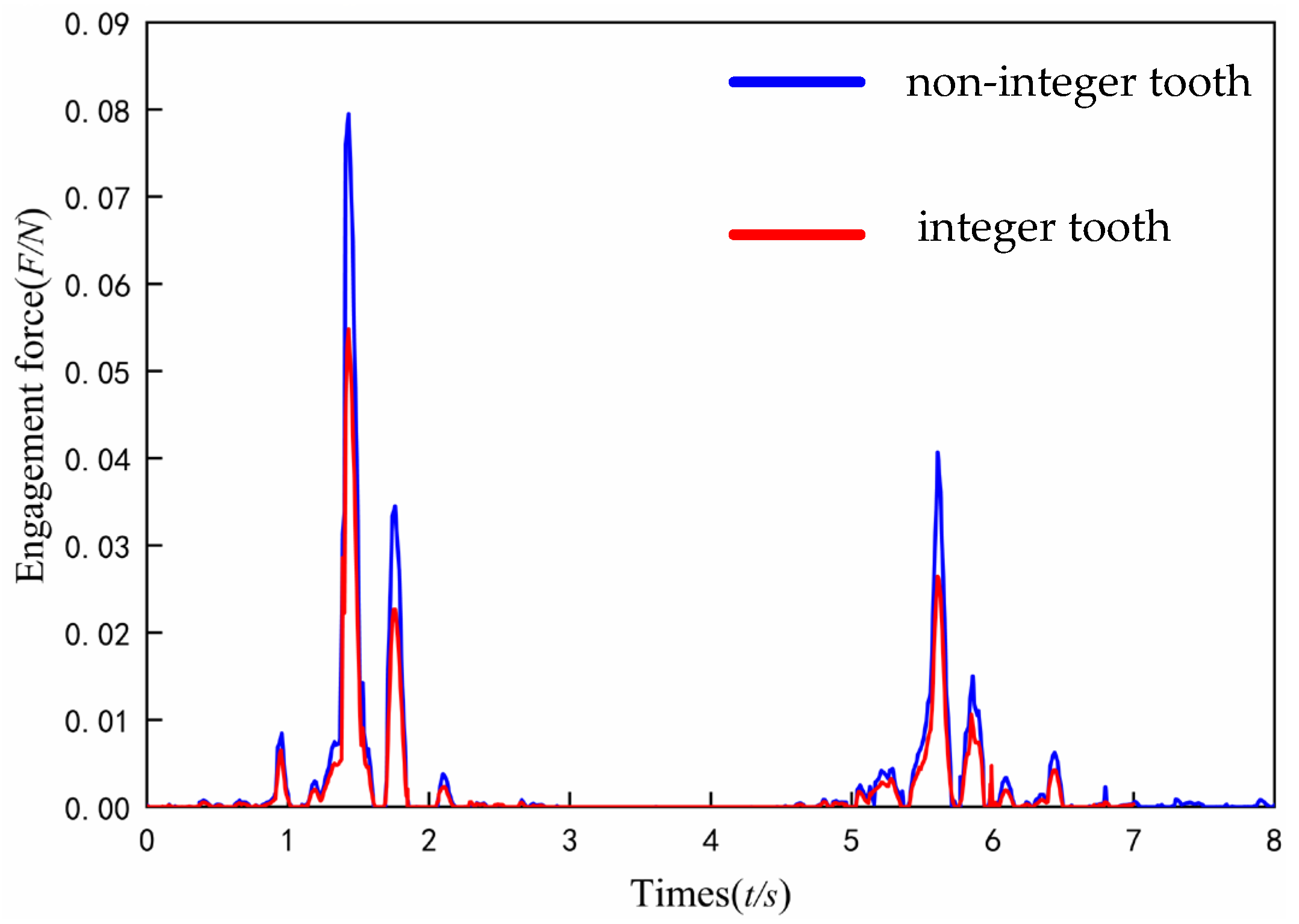

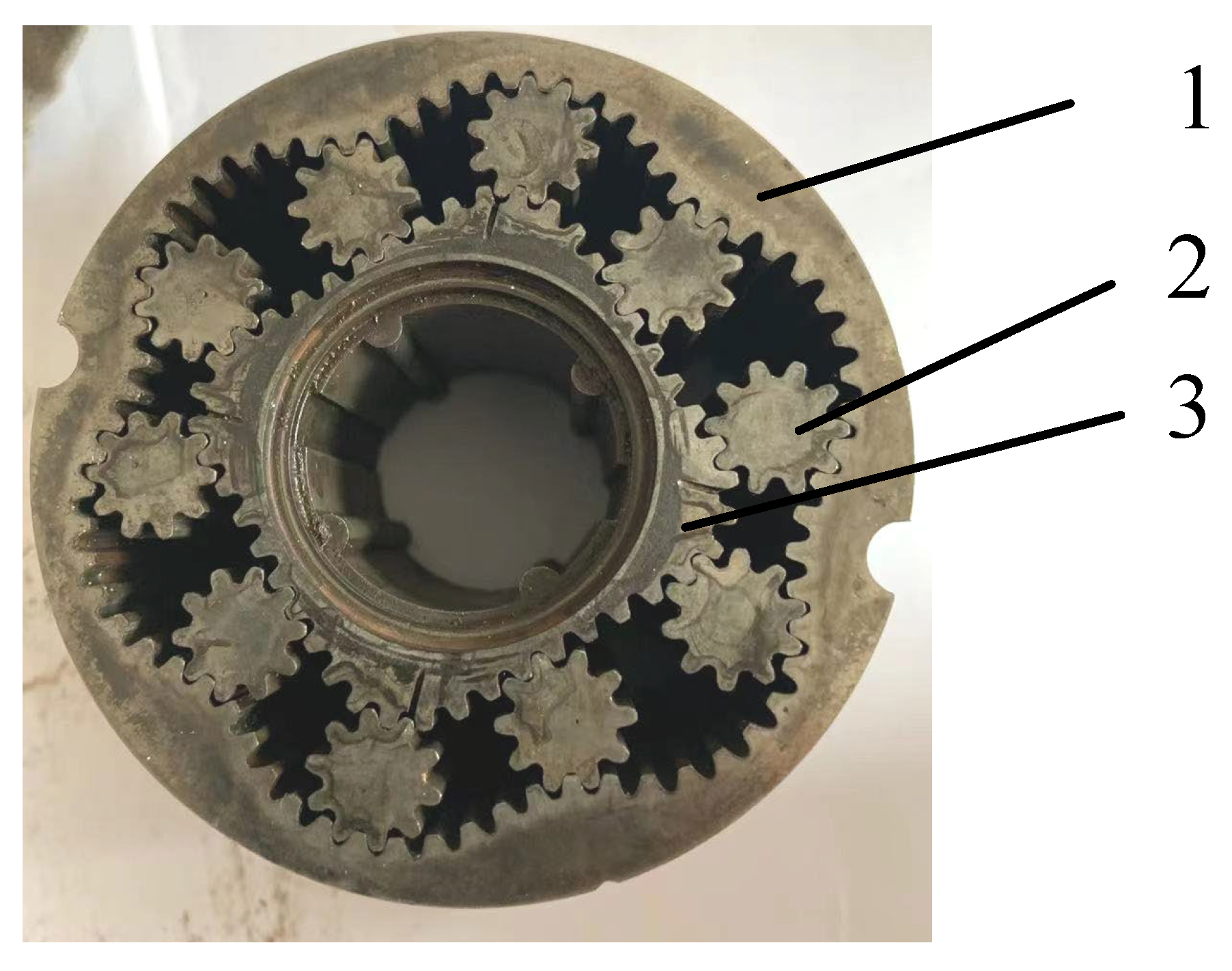
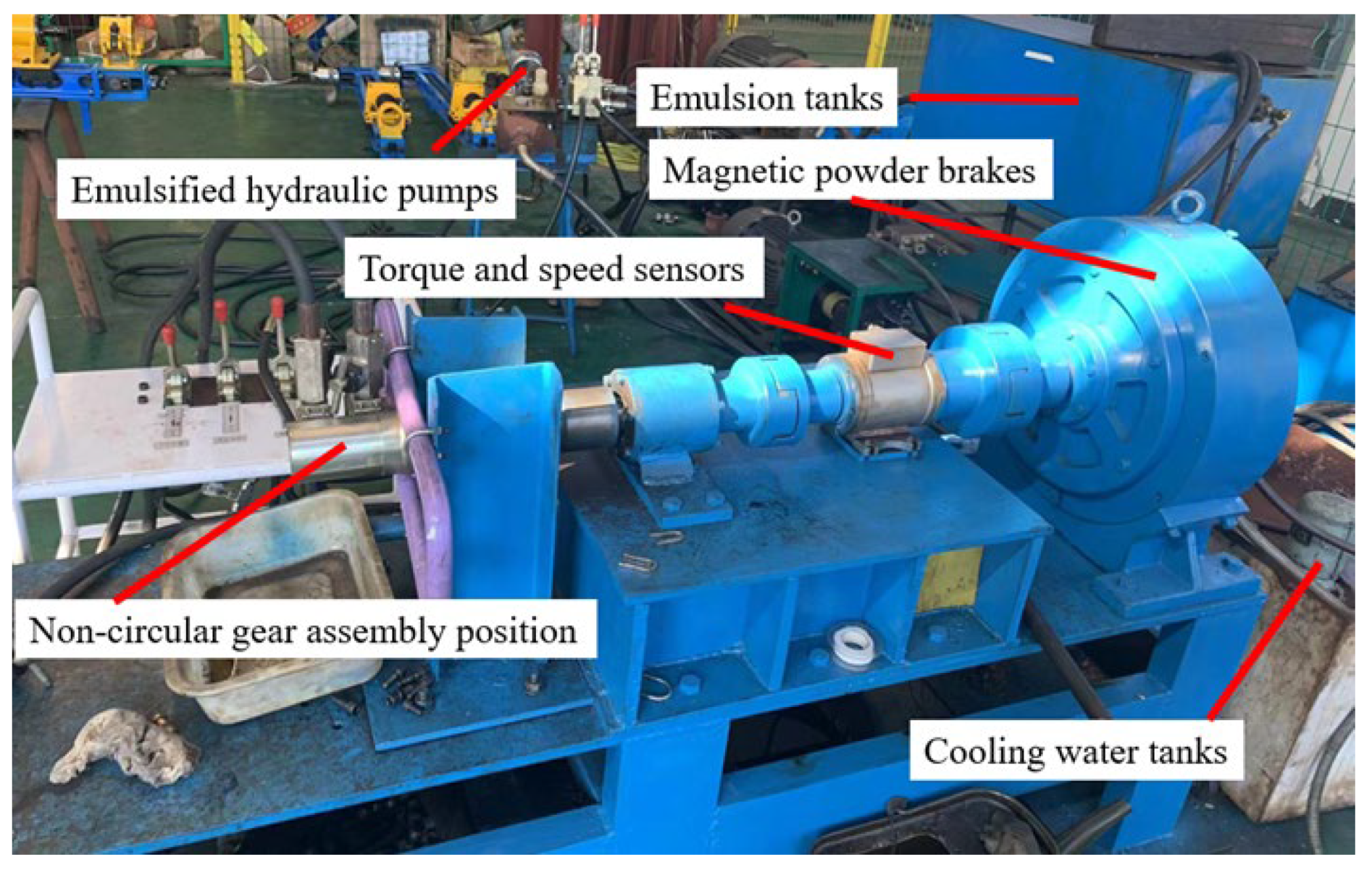
| y2/mm | Amount of Interference/mm |
|---|---|
| 979.1195 | 0.0411 |
| 972.8100 | 0.0396 |
| 854.7425 | 0.0350 |
| 724.2570 | 0.0281 |
| 698.3684 | 0.0264 |
| Gear Parameters | Values |
|---|---|
| M(mm) | 0.75 |
| Z1 | 44.00 |
| Z2 | 66.00 |
| Z3 | 10.00 |
| α° | 20.00 |
| ha* | 0.75 |
| c* | 0.25 |
| Test Levels | Test Factors | ||
|---|---|---|---|
| Z1 | z′1 | z′2 | |
| 1 | 27 | 30 | 1115 |
| 2 | 28 | 31 | 1116 |
| Number of Tests | Test Factors | P Engagement Coefficient | ||
|---|---|---|---|---|
| Z1 | Z’1 | Z’2 | ||
| 1 | 27 | 30 | 1115 | 1.294 |
| 2 | 27 | 31 | 1116 | 6.486 |
| 3 | 28 | 30 | 1116 | 1.394 |
| 4 | 28 | 31 | 1115 | 0.965 |
| Test Factors | Range | Better Level |
|---|---|---|
| Z1 | 2.216 | 28 |
| z′1 | 2.292 | 30 |
| z′2 | 2.659 | 1115 |
| Prototype | Self-Made Product | |
|---|---|---|
| Module (mm) | 0.75 | 0.75 |
| Tooth Width (mm) | 25 | 25 |
| Displacement (cm3/rev.) | 32.9 | 35.1 |
| Torque(N·m) | 100.0 | 123.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; Gao, Z.; Chen, G.; Guo, M. Tooth Profile Construction and Experimental Verification of Non-Circular Gear Based on Double Arc Active Design. Appl. Sci. 2023, 13, 10566. https://doi.org/10.3390/app131910566
Wang K, Gao Z, Chen G, Guo M. Tooth Profile Construction and Experimental Verification of Non-Circular Gear Based on Double Arc Active Design. Applied Sciences. 2023; 13(19):10566. https://doi.org/10.3390/app131910566
Chicago/Turabian StyleWang, Kang, Zhenshan Gao, Guohua Chen, and Meihua Guo. 2023. "Tooth Profile Construction and Experimental Verification of Non-Circular Gear Based on Double Arc Active Design" Applied Sciences 13, no. 19: 10566. https://doi.org/10.3390/app131910566
APA StyleWang, K., Gao, Z., Chen, G., & Guo, M. (2023). Tooth Profile Construction and Experimental Verification of Non-Circular Gear Based on Double Arc Active Design. Applied Sciences, 13(19), 10566. https://doi.org/10.3390/app131910566






