Diffusion Model of Cement Slurry in Porous Media Considering Porosity Variation and Percolation Effect
Abstract
:1. Introduction
2. Porosity Analysis of Injected Porous Media
3. Numerical Analysis
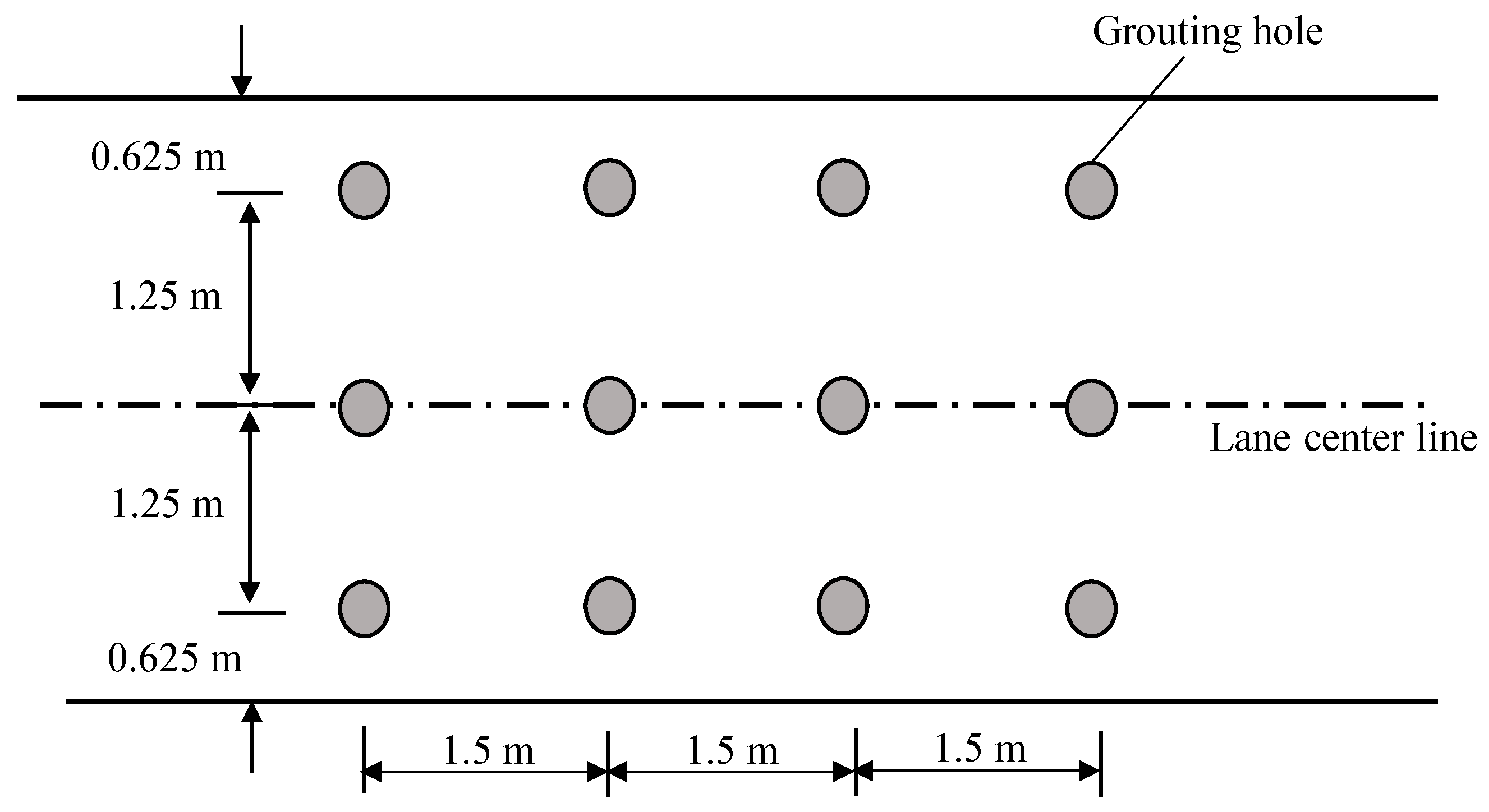
4. Engineering Application
- FWD was used to test the pavement bearing capacity before grouting, so as to evaluate the degree of pavement damage.
- The pore structure parameters of this highway section should be quantitatively analyzed by drilling and coring, and corresponding to the parameters in the model.
- According to the model deduced in this paper, the optimum spacing of grouting holes was designed according to the characteristics of the pavement pore structure and cement grout on site.
- An appropriate cement slurry ratio was selected to implement grouting on the pavement.
- After grouting was completed, the grouting holes were filled with road sealant to prevent water from penetrating the road surface.
5. Conclusions
- The percolation effect can lead to temporal and spatial variations in the injected medium’s porosity in the grouting process. The consistency between the predicted and measured results shows that the grouting diffusion law can be more accurately demonstrated by considering the real-time porosity variation.
- The change in the porosity of the injected medium affects the diffusion distance and repair effect of the cement slurry. The particles can be trapped in the grouting hole, resulting in a rapid decrease in the porosity of the media at the grouting hole. Therefore, it is difficult for the slurry to continue to diffuse, thus shortening the diffusion distance. Meanwhile, the decrease in porosity at the grouting hole will lead to the high compressive strength of the consolidated body. However, it also affects the remote repair effect of grouting, making it difficult to achieve the design’s mechanical properties.
- The diffusion model is applied to the grouting parameter design of highway repair. It was found that the pavement bearing performance is greatly improved after grouting, indicating that it is reasonable to consider the change in porosity of the injected medium.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Luo, R.; Huang, T.; Zhang, D.; Lytton, R. Water vapor diffusion in asphalt mixtures under different relative humidity differentials. Constr. Build. Mater. 2017, 136, 126–138. [Google Scholar] [CrossRef]
- Pachideh, G.; Gholhaki, M.; Moshtagh, A. Experimental study on mechanical strength of porous concrete pavement containing pozzolans. Adv. Civ. Eng. Mater. 2020, 9, 38–52. [Google Scholar] [CrossRef]
- Ma, F.; Dong, W.; Fu, Z.; Wang, R.; Huang, Y.; Liu, J. Life cycle assessment of greenhouse gas emissions from asphalt pavement maintenance: A case study in China. J. Clean. Prod. 2021, 288, 125595. [Google Scholar] [CrossRef]
- Zhang, J.; Cai, J.; Pei, J.; Li, R.; Chen, X. Formulation and performance comparison of grouting materials for semi-flexible pavement. Constr. Build. Mater. 2016, 115, 582–592. [Google Scholar] [CrossRef]
- Guo, C.; Cui, C.; Wang, F. Case study on quick treatment of voids under airport pavement by polymer grouting. J. Mater. Civ. Eng. 2020, 32, 05020006. [Google Scholar] [CrossRef]
- Wang, C.; Diao, Y.; Guo, C.; Li, P.; Du, X.; Pan, Y. Two-stage column–hemispherical penetration diffusion model considering porosity tortuosity and time-dependent viscosity behavior. Acta Geotechnica 2022, 1–20. [Google Scholar] [CrossRef]
- Qin, L.; Guo, C.; Sun, W.; Chu, X.; Ji, T.; Guan, H. Identification of damage mechanisms of polymer-concrete in direct shearing tests by acoustic emission. Constr. Build. Mater. 2022, 351, 128813. [Google Scholar] [CrossRef]
- Qin, L.; Guo, C.; Sun, W.; Guan, H.; Yan, W.; Wang, F. Experimental investigation on the interfacial shear bond performance of non-water reacting polymer and concrete. Constr. Build. Mater. 2022, 331, 127351. [Google Scholar] [CrossRef]
- Zhang, L.; Wen, P.; Wang, C.; Di, S.; Yin, W. Advances in Non-excavation Grouting Reinforcement Materials in the Road Engineering. Mater. Rep. 2017, 31, 98–105. [Google Scholar]
- Ruan, W. Spreading model of grouting in rock mass fissures based on time-dependent behavior of viscosity of cement-based grouts. Chin. J. Rock Mech. Eng. 2005, 24, 2709–2714. [Google Scholar]
- Wang, H.; Wang, Q.; Min, F.; Chen, J. Research Progress of Grouting Sealing Materials. Mater. Rep. 2013, 27, 103–106. [Google Scholar]
- Pachideh, G.; Gholhaki, M. Effect of pozzolanic materials on mechanical properties and water absorption of autoclaved aerated concrete. J. Build. Eng. 2019, 26, 100856. [Google Scholar] [CrossRef]
- Seddighi, F.; Pachideh, G.; Salimbahrami, S.B. A study of mechanical and microstructures properties of autoclaved aerated concrete containing nano-graphene. J. Build. Eng. 2021, 43, 103106. [Google Scholar] [CrossRef]
- Anagnostopoulos, C.A. Effect of different superplasticisers on the physical and mechanical properties of cement grouts. Constr. Build. Mater. 2014, 50, 162–168. [Google Scholar] [CrossRef]
- Li, S.C.; Zheng, Z.; Liu, R.T.; Feng, X.; Sun, Z.Z.; Zhang, L.Z. Analysis of diffusion of grout in porous media considering infiltration effects. Chin. J. Rock Mech. Eng. 2015, 34, 2401–2409. [Google Scholar]
- Bennacer, L.; Ahfir, N.-D.; Bouanani, A.; Alem, A.; Wang, H. Suspended Particles Transport and Deposition in Saturated Granular Porous Medium: Particle Size Effects. Transp. Porous Media 2013, 100, 377–392. [Google Scholar] [CrossRef]
- Zhang, M.Q.; Zhang, W.; Sun, G. Evaluation technique of grouting effect and its application to engineering. Chin. J. Rock Mech. Eng. 2006, 25, 3909–3918. [Google Scholar]
- Wang, X. Research on Comprehensive Evaluation Technology of Grouting Effect in Underground Engineering. Bachelor’s Thesis, Beijing Municipal Engineering Research Institute, Beijing, China, 2009. [Google Scholar]
- Li, P.; Liu, J.; Shi, L.; Li, X.; Zhu, H.; Huang, D.; Kou, X. Two-phase analytical model of seepage during grout consolidation around shield tunnel considering the temporal variation in viscosity and the infiltration effect. Eur. J. Environ. Civ. Eng. 2020, 26, 4392–4415. [Google Scholar] [CrossRef]
- Yoon, J.; El Mohtar, C.S. A filtration model for evaluating maximum penetration distance of bentonite grout through granular soils. Comput. Geotech. 2015, 65, 291–301. [Google Scholar] [CrossRef]
- Axelsson, M.; Gustafson, G.; Fransson, Å. Stop mechanism for cementitious grouts at different water-to-cement ratios. Tunn. Undergr. Space Technol. 2009, 24, 390–397. [Google Scholar] [CrossRef]
- Zhou, Z.; Du, X.; Wang, S.; Zang, H. Analysis and engineering application investigation of multiple-hole grouting injections into porous media considering filtration effects. Constr. Build. Mater. 2018, 186, 871–883. [Google Scholar] [CrossRef]
- Ye, F.; Yang, T.; Mao, J.-H.; Qin, X.-Z.; Zhao, R.-L. Half-spherical surface diffusion model of shield tunnel back-fill grouting based on infiltration effect. Tunn. Undergr. Space Technol. 2019, 83, 274–281. [Google Scholar] [CrossRef]
- Ye, F.; Qin, N.; Han, X.; Liang, X.; Gao, X.; Ying, K. Displacement infiltration diffusion model of power-law grout as backfill grouting of a shield tunnel. Eur. J. Environ. Civ. Eng. 2022, 26, 1820–1833. [Google Scholar] [CrossRef]
- Du, X.; Liu, C.; Wang, C.; Fang, H.; Xue, B.; Gao, X.; Han, Y. Diffusion characteristics and reinforcement effect of cement slurry on porous medium under dynamic water condition considering infiltration. Tunn. Undergr. Space Technol. 2022, 130, 104766. [Google Scholar] [CrossRef]
- Zheng, Z.; Fan, F.; Pan, X.; Ma, C. Study on grouting penetration rule with filtration effect and grout–water interaction. Arab. J. Geosci. 2021, 14, 1830. [Google Scholar] [CrossRef]
- Mandelbrot, B. How long is the coast of Britain? Statistical self-similarity and fractional dimension. Science 1967, 156, 636–638. [Google Scholar] [CrossRef]
- Giudicianni, C.; Di Nardo, A.; Greco, R.; Scala, A. A community-structure-based method for estimating the fractal dimension, and its application to water networks for the assessment of vulnerability to disasters. Water Resour. Manag. 2021, 35, 1197–1210. [Google Scholar] [CrossRef]
- Hoxha, E.; Vidal, Y.; Pozo, F. Damage diagnosis for offshore wind turbine foundations based on the fractal dimension. Appl. Sci. 2020, 10, 6972. [Google Scholar] [CrossRef]
- Zhao, Z.; Ni, X.; Cao, Y.; Shi, Y. Application of fractal theory to predict the coal permeability of multi-scale pores and fractures. Energy Rep. 2021, 7, 10–18. [Google Scholar] [CrossRef]
- Deng, H.; Tian, G.; Yu, S.; Jiang, Z.; Zhong, Z.; Zhang, Y. Research on strength prediction model of sand-like material based on nuclear magnetic resonance and fractal theory. Appl. Sci. 2020, 10, 6601. [Google Scholar] [CrossRef]
- Niu, D.; Huang, D.; Zheng, H.; Su, L.; Fu, Q.; Luo, D. Experimental study on mechanical properties and fractal dimension of pore structure of basalt–polypropylene fiber-reinforced concrete. Appl. Sci. 2019, 9, 1602. [Google Scholar] [CrossRef]
- Wang, J.; Guo, Q.; Chen, W.; Liu, H. Study on Multi-Scale Coral Reef Pore Structure Identification and Characterization. Appl. Sci. 2022, 12, 5280. [Google Scholar] [CrossRef]
- Zhang, M.; Sun, H.; Song, C.; Li, Y.; Hou, M. Pores Evolution of Soft Clay under Loading/Unloading Process. Appl. Sci. 2020, 10, 8468. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, C.; Ning, L.; Zhao, H.; Bi, J. Pore and fracture development in coal under stress conditions based on nuclear magnetic resonance and fractal theory. Fuel 2022, 309, 122112. [Google Scholar] [CrossRef]
- Li, H. Quantitative prediction of complex tectonic fractures in the tight sandstone reservoirs: A fractal method. Arab. J. Geosci. 2021, 14, 1986. [Google Scholar] [CrossRef]
- Shi, W.; Wang, J.; Li, X.; Xu, Q.; Jiang, X. Multi-fractal characteristics of reconstructed landform and its relationship with soil erosion at a large opencast coal-mine in the loess area of China. Geomorphology 2021, 390, 107859. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Z.; Pan, Z.; Niu, X.; Yu, Y.; Meng, S. Pore structure and its fractal dimensions of transitional shale: A cross-section from east margin of the Ordos Basin, China. Fuel 2019, 241, 417–431. [Google Scholar] [CrossRef]
- Wang, G.; Shen, J.; Liu, S.; Jiang, C.; Qin, X. Three-dimensional modeling and analysis of macro-pore structure of coal using combined X-ray CT imaging and fractal theory. Int. J. Rock Mech. Min. Sci. 2019, 123, 104082. [Google Scholar] [CrossRef]
- Nayak, S.R.; Mishra, J.; Palai, G. Analysing roughness of surface through fractal dimension: A review. Image Vis. Comput. 2019, 89, 21–34. [Google Scholar] [CrossRef]
- Ni, G.; Li, S.; Rahman, S.; Xun, M.; Wang, H.; Xu, Y.; Xie, H. Effect of nitric acid on the pore structure and fractal characteristics of coal based on the low-temperature nitrogen adsorption method. Powder Technol. 2020, 367, 506–516. [Google Scholar] [CrossRef]
- Guo, R.; Xie, Q.; Qu, X.; Chu, M.; Li, S.; Ma, D.; Ma, X. Fractal characteristics of pore-throat structure and permeability estimation of tight sandstone reservoirs: A case study of Chang 7 of the Upper Triassic Yanchang Formation in Longdong area, Ordos Basin, China. J. Pet. Sci. Eng. 2020, 184, 106555. [Google Scholar] [CrossRef]
- Miao, T.; Yu, B.; Duan, Y.; Fang, Q. A fractal model for spherical seepage in porous media. Int. Commun. Heat Mass Transf. 2014, 8, 71–78. [Google Scholar] [CrossRef]
- Yu, B.; Cheng, P. A fractal permeability model for bi-dispersed porous media. Int. J. Heat Mass Transf. 2002, 45, 2983–2993. [Google Scholar] [CrossRef]
- Zhou, Z.; Cai, X.; Du, X.; Wang, S.; Ma, D.; Zang, H. Strength and filtration stability of cement grouts in porous media. Tunn. Under-Ground Space Technol. 2019, 89, 1–9. [Google Scholar] [CrossRef]
- Bohloli, B.; Morgan, E.K.; Grøv, E.; Skjølsvold, O.; Hognestad, H.O. Strength and filtration stability of cement grouts at room and true tunneling temperatures. Tunn. Undergr. Space Technol. 2018, 71, 193–200. [Google Scholar] [CrossRef]
- Wang, C.; Guo, C.; Du, X.; Shi, M.; Liu, Q.; Xia, Y. Reinforcement of silty soil with permeable polyurethane by penetration injection. Constr. Build. Mater. 2021, 310, 124829. [Google Scholar] [CrossRef]
- Herzig, J.P.; Leclerc, D.M.; Le Goff, P. Flow of Suspensions through Porous Media—Application to Deep Filtration. Ind. Eng. Chem. 1970, 62, 8–35. [Google Scholar] [CrossRef]
- Kim, J.-S.; Lee, I.-M.; Jang, J.-H.; Choi, H. Groutability of cement-based grout with consideration of viscosity and filtration phenomenon. Int. J. Numer. Anal. Methods Geomech. 2009, 33, 1771–1797. [Google Scholar] [CrossRef]
- Gao, Z.; Wen, P.; Wang, C.; Shu, C.; Wang, X. Grouting seepage mechanism and reinforcement effect of alkali-activated materials in road base. Arab. J. Geosci. 2021, 14, 1319. [Google Scholar] [CrossRef]
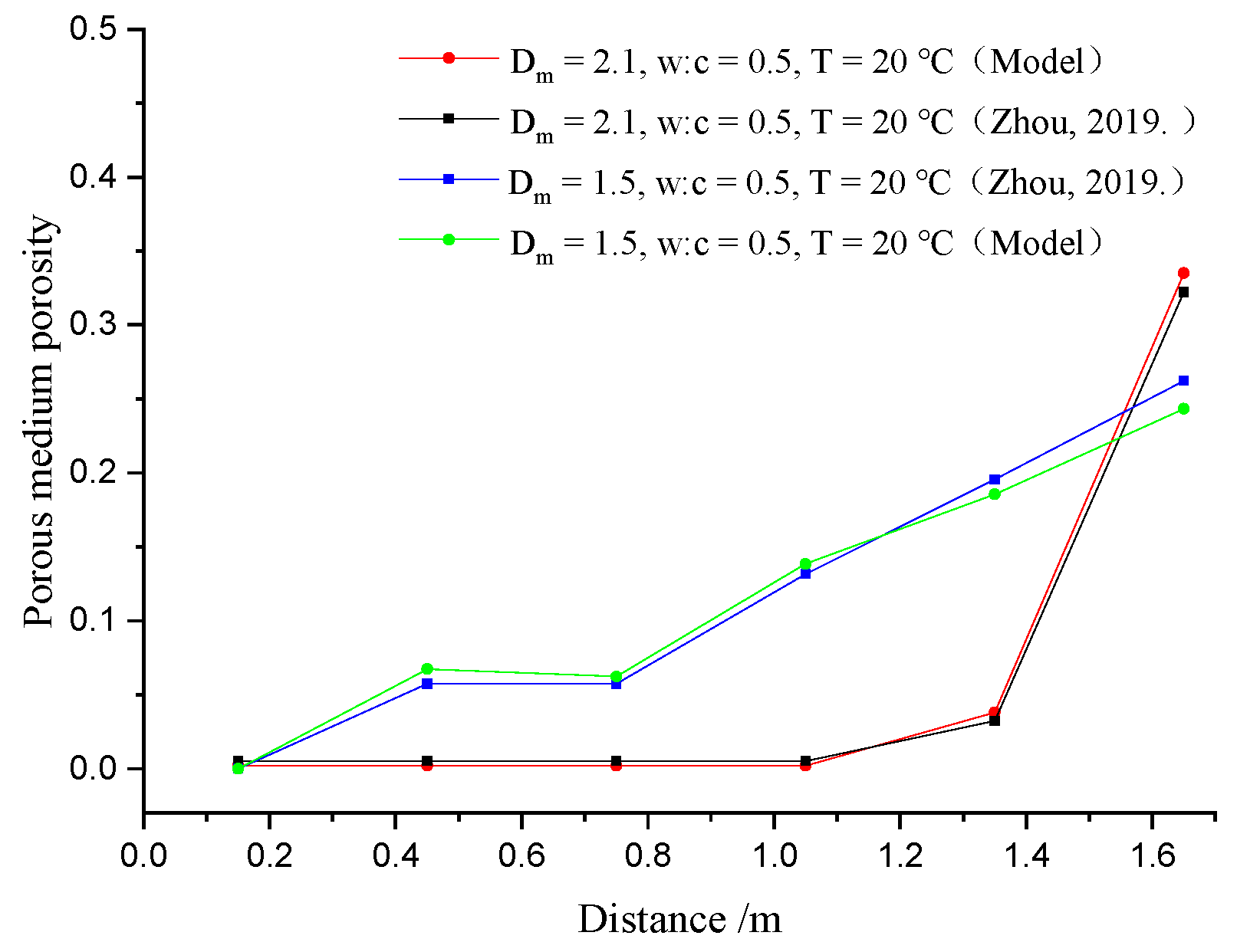
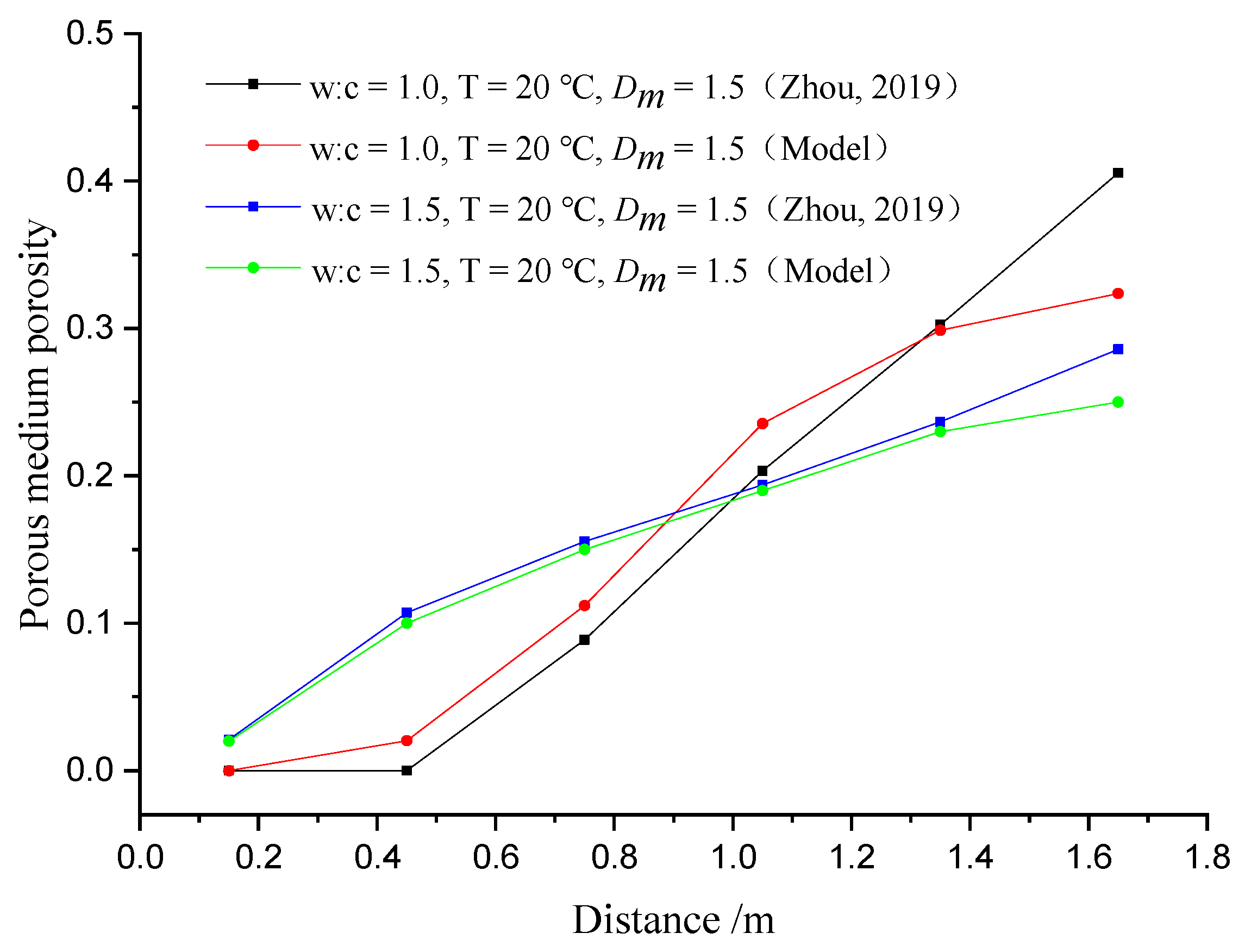
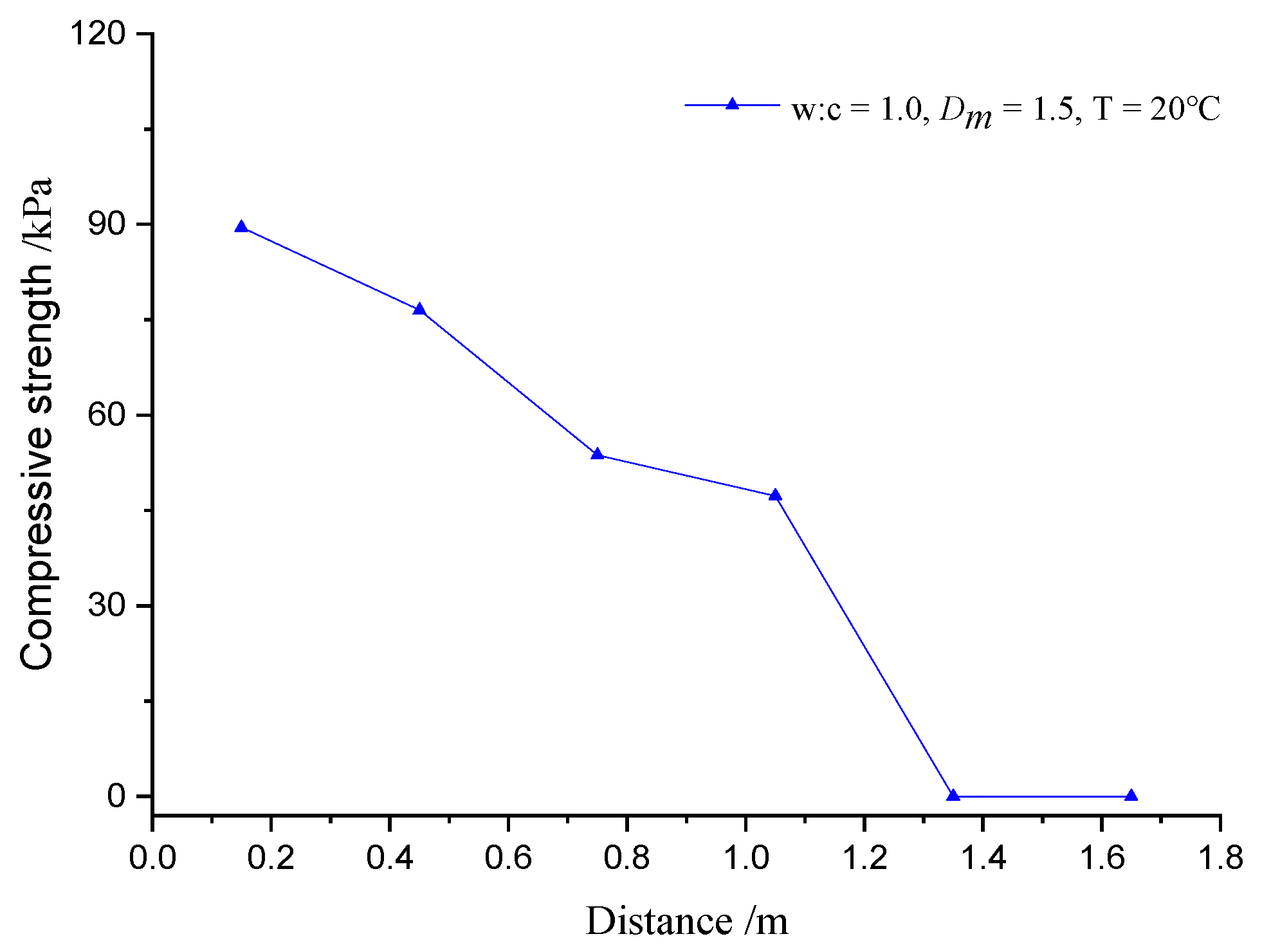







| Parameters | Water–Cement Ratio of Cement Slurry (w:c) | Fractal Dimensions of the Pore/Dm | Other Parameters |
|---|---|---|---|
| Value | 1.0 | 1.5 | T = 20 °C |
| 1.5 | 2.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, B.; Chen, X.; Pan, Y.; Wang, C.; Shi, M.; Chu, X. Diffusion Model of Cement Slurry in Porous Media Considering Porosity Variation and Percolation Effect. Appl. Sci. 2023, 13, 1919. https://doi.org/10.3390/app13031919
Han B, Chen X, Pan Y, Wang C, Shi M, Chu X. Diffusion Model of Cement Slurry in Porous Media Considering Porosity Variation and Percolation Effect. Applied Sciences. 2023; 13(3):1919. https://doi.org/10.3390/app13031919
Chicago/Turabian StyleHan, Bo, Xuemin Chen, Yanhui Pan, Chaojie Wang, Mingsheng Shi, and Xuanxuan Chu. 2023. "Diffusion Model of Cement Slurry in Porous Media Considering Porosity Variation and Percolation Effect" Applied Sciences 13, no. 3: 1919. https://doi.org/10.3390/app13031919
APA StyleHan, B., Chen, X., Pan, Y., Wang, C., Shi, M., & Chu, X. (2023). Diffusion Model of Cement Slurry in Porous Media Considering Porosity Variation and Percolation Effect. Applied Sciences, 13(3), 1919. https://doi.org/10.3390/app13031919









