Effectiveness of High-Damping Rubber (HDR) Damper and Tuned Mass—HDR Damper in Suppressing Stay-Cable Vibration
Abstract
1. Introduction
2. Formulation of the Cable—HDR Damper—TM-HDR-D System
3. Numerical Parametric Study
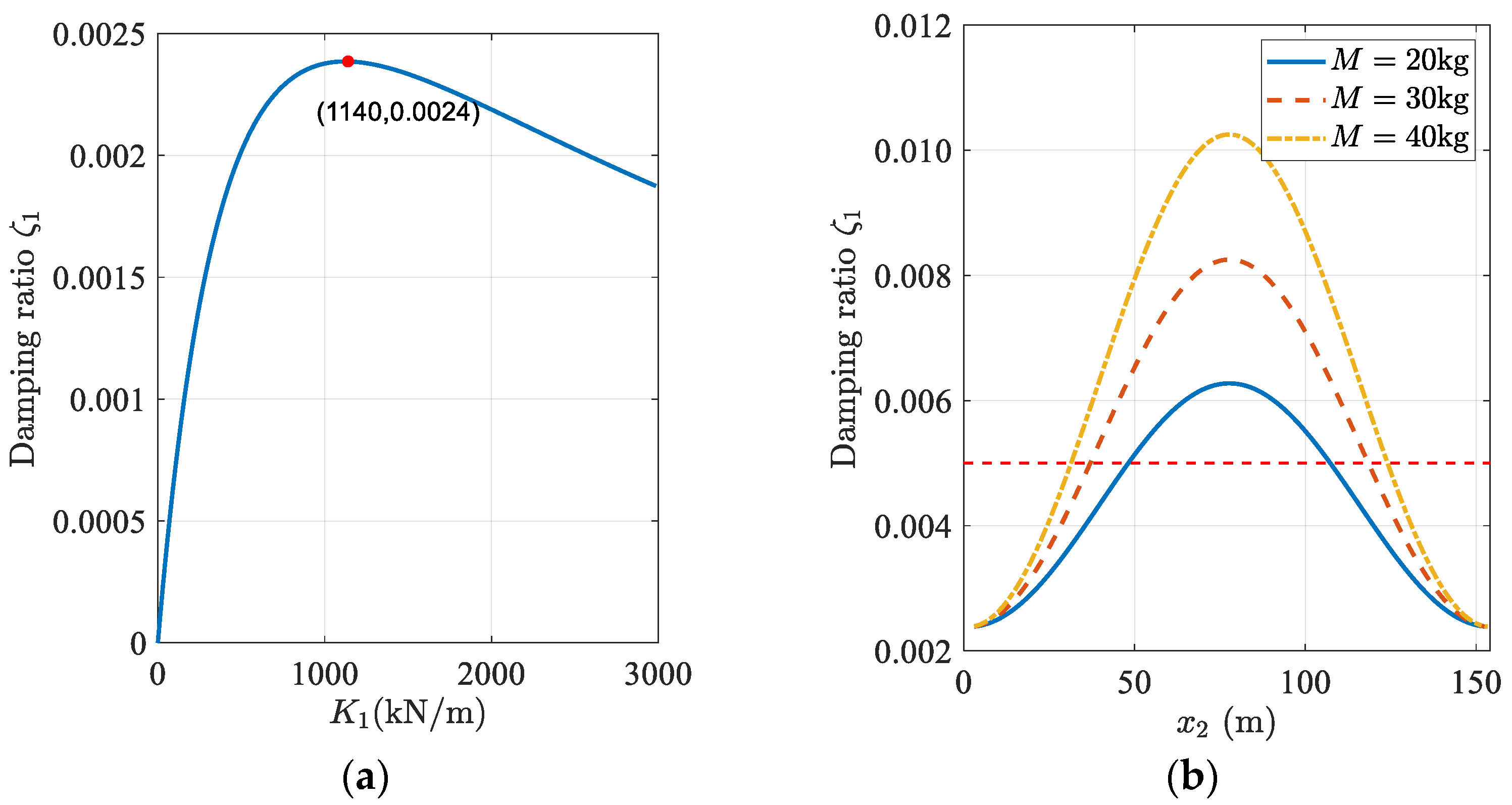
3.1. Cable with a Single HDR Damper
3.2. Cable with a Single TM-HDR-D
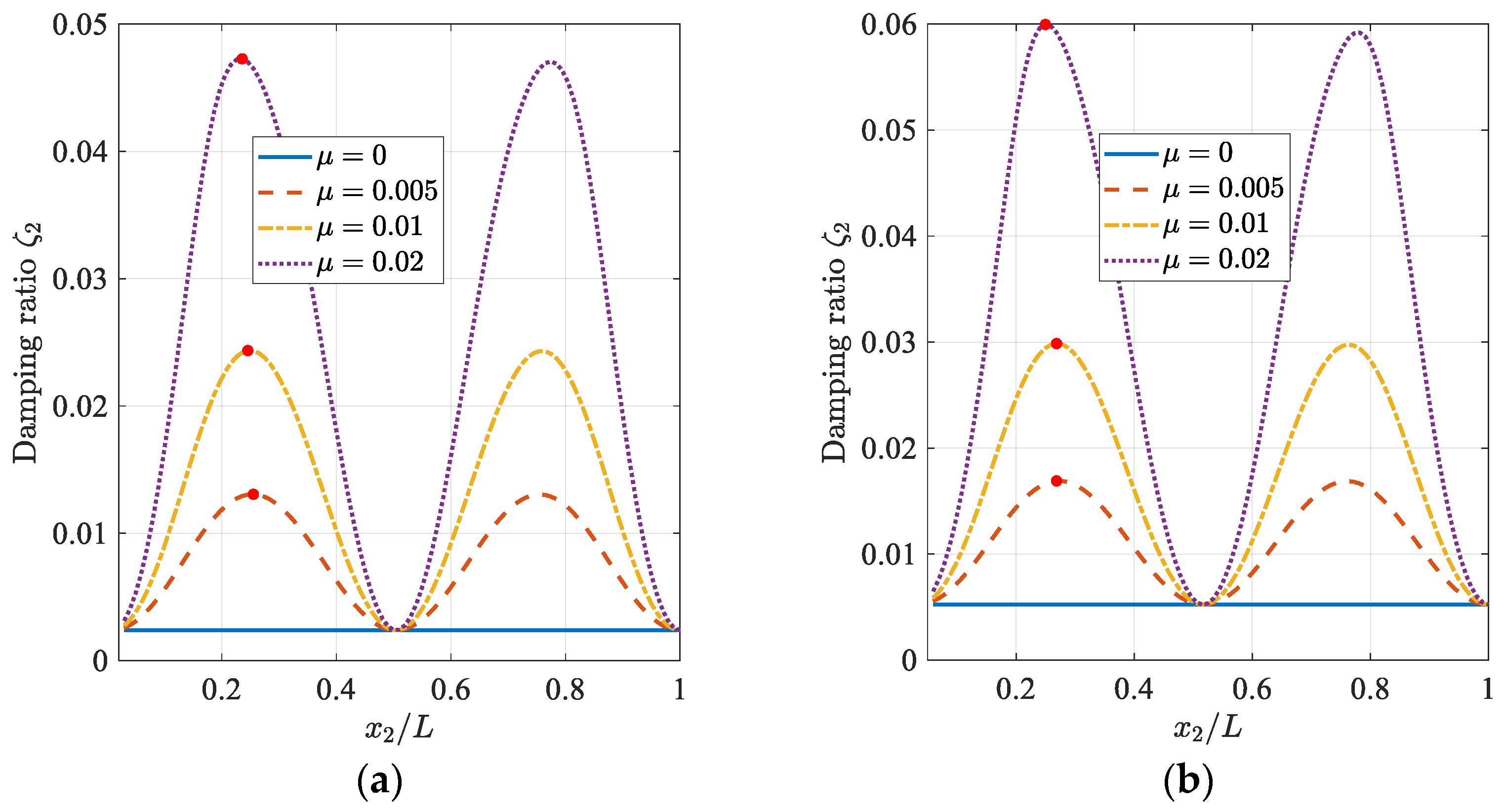
3.3. Cable with HDR Damper and TM-HDR-D
4. Example for Damper Design
4.1. Targeting for a Single Mode
4.2. Targeting for Two Modes
4.3. Remarks
5. Conclusions
- As a cable-end damper, the effectiveness of the HDR damper mainly depends on the damper’s stiffness, loss factor, and location. In practice, the damper’s stiffness needs to be optimized to achieve the best damping contribution to the cable system. The TM-HDR-D can be installed at any position of the cable, and through precise frequency tuning it can supplement the damping contribution of the cable-end HDR damper.
- When the TM-HDR-D has a small mass, the combined damping effect of the HDR damper and the TM-HDR-D approximates a linear superposition of their respective effects.
- In practice, a TM-HDR-D can be co-designed with an HDR damper to achieve multimodal damping improvement. The TM-HDR-D can make up for the deficiency of the HDR damper and achieve the multimode vibration control of the cable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Domaneschi, M.; Martinelli, L.; Po, E. Control of wind buffeting vibrations in a suspension bridge by TMD: Hybridization and robustness issues. Comput. Struct. 2015, 155, 3–17. [Google Scholar] [CrossRef]
- Martinelli, L.; Domaneschi, M.; Shi, C. Submerged floating tunnels under seismic motion: Vibration mitigation and seaquake effects. Procedia Eng. 2016, 166, 229–246. [Google Scholar] [CrossRef]
- Fujino, Y.; Kimura, K.; Tanaka, H. Cable vibrations and control methods. In Wind Resistant Design of Bridges in Japan; Springer: Tokyo, Japan, 2012; pp. 197–229. [Google Scholar]
- Zuo, D.; Jones, N.P. Interpretation of field observations of wind-and rain-wind-induced stay cable vibrations. J. Wind Eng. Ind. Aerod. 2012, 98, 73–87. [Google Scholar] [CrossRef]
- Matsumoto, M.; Shirato, H.; Yagi, T.; Goto, M.; Sakai, S.; Ohya, J. Field observation of the full-scale wind-induced cable vibration. J. Wind Eng. Ind. Aerod. 2003, 91, 13–26. [Google Scholar] [CrossRef]
- Domaneschi, M.; Martinelli, L.; Perotti, F. Wind and earthquake protection of cable-supported bridges. In Proceedings of the Institution of Civil Engineers-Bridge Engineering; Thomas Telford Ltd.: London, UK, 2016; Volume 169, pp. 157–171. [Google Scholar]
- Gu, M. On wind–rain induced vibration of cables of cable-stayed bridges based on quasi-steady assumption. J. Wind Eng. Ind. Aerod. 2009, 97, 381–391. [Google Scholar] [CrossRef]
- Kleissl, K.; Georgakis, C.T. Aerodynamic control of bridge cables through shape modification: A preliminary study. J. Fluid. Struct. 2011, 27, 1006–1020. [Google Scholar] [CrossRef]
- Gu, M.; Du, X. Experimental investigation of rain–wind-induced vibration of cables in cable-stayed bridges and its mitigation. J. Wind Eng. Ind. Aerod. 2005, 93, 79–95. [Google Scholar] [CrossRef]
- Main, J.A.; Jones, N.P. Evaluation of viscous dampers for stay-cable vibration mitigation. J. Bridge Eng. 2001, 6, 385–397. [Google Scholar] [CrossRef]
- Chen, L.; Sun, L.; Xu, Y.; Di, F.; Xu, Y.; Wang, L. A comparative study of multi-mode cable vibration control using viscous and viscoelastic dampers through field tests on the Sutong Bridge. Eng. Struct. 2020, 224, 111226. [Google Scholar] [CrossRef]
- Caracoglia, L.; Jones, N.P. In-plane dynamic behavior of cable networks. Part 1: Formulation and basic solutions. J. Sound Vib. 2005, 27, 969–991. [Google Scholar] [CrossRef]
- Caracoglia, L.; Jones, N.P. In-plane dynamic behavior of cable networks. Part 2: Prototype prediction and validation. J. Sound Vib. 2005, 279, 993–1014. [Google Scholar] [CrossRef]
- Main, J.; Jones, N. Full-scale measurements of stay cable vibration. In Proceedings of the Tenth International Conference on Wind Engineering, Copenhagen, Denmark, 21–24 June 1999; Volume 2, pp. 963–970. [Google Scholar]
- Chen, Z.Q.; Wang, X.Y.; Ko, J.M.; Ni, Y.Q.; Spencer, B.F.; Yang, G.; Hu, J.H. MR damping system for mitigating wind-rain induced vibration on Dongting Lake Cable-Stayed Bridge. Wind Struct. 2004, 7, 293–304. [Google Scholar] [CrossRef]
- Li, H.; Liu, M.; Li, J.; Guan, X.; Ou, J. Vibration control of stay cables of the shandong binzhou yellow river highway bridge using magnetorheological fluid dampers. J. Bridge Eng. 2007, 12, 401–409. [Google Scholar] [CrossRef]
- Myrvoll, F.; Kaynia, A.M.; Hjorth-Hansen, E.; Strømmen, E. Full-scale dynamic performance testing of the bridge structure and the special cable friction dampers on the cable-stayed Uddevalla bridge. In Proceedings of IMAC-XX: Structural Dynamics Vols I and II; Society for Experimental Mechanics: Orlando, FL, USA, 2002; p. 4753. [Google Scholar]
- Kim, S.; Kim, S.; Kim, H.K. High-mode vortex-induced vibration of stay cables: Monitoring, cause investigation, and mitigation. J. Sound Vib. 2022, 524, 116758. [Google Scholar] [CrossRef]
- Zhou, P.; Liu, M.; Kong, W.; Xu, Y.; Li, H. Modeling and evaluation of magnetorheological dampers with fluid leakage for cable vibration control. J. Bridge Eng. 2021, 26, 04020119. [Google Scholar] [CrossRef]
- Weber, F.; Ho-gsberg, J.; Krenk, S. Optimal tuning of amplitude proportional Coulomb friction damper for maximum cable damping. J. Struct. Eng. 2010, 136, 123. [Google Scholar] [CrossRef]
- Weber, F.; Distl, H. Amplitude and frequency independent cable damping of Sutong Bridge and Russky Bridge by magnetorheological dampers. Struct. Control. Health Monit. 2015, 22, 237–254. [Google Scholar] [CrossRef]
- Nakamura, A.; Kasuga, A.; Arai, H. The effects of mechanical dampers on stay cables with high-damping rubber. Constr. Build. Mater. 1998, 12, 115–123. [Google Scholar] [CrossRef]
- Yoneda, M.; Maeda, K. A study on practical estimation method for structural damping of stay cables with dampers. Doboku Gakkai Ronbunshu 1989, 1410, 455–458. (In Japanese) [Google Scholar] [CrossRef]
- Fujino, Y.; Hoang, N. Design formulas for damping of a stay cable with a damper. J. Struct. Eng. 2008, 134, 269–278. [Google Scholar] [CrossRef]
- Hoang, N.; Fujino, Y. Combined damping effect of two dampers on a stay cable. J. Bridge Eng. 2008, 13, 299–303. [Google Scholar] [CrossRef]
- Cu, V.H.; Han, B.; Wang, F. Damping of a taut cable with two attached high damping rubber dampers, Struct. Eng. Mech. Int. J. 2015, 55, 1261–1278. [Google Scholar]
- Di, F.; Sun, L.; Chen, L. Cable vibration control with internal and external dampers: Theoretical analysis and field test validation. Smart Struct. Syst. 2020, 26, 575–589. [Google Scholar]
- Di, F.; Sun, L.; Chen, L. Suppression of vortex-induced high-mode vibrations of a cable-damper system by an additional damper. Eng. Struct. 2021, 242, 112495. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Vo, D.H.; Haque, M. Theoretical Investigation on the Impact of Two HDR Dampers on First Modal Damping Ratio of Stay Cable. Appl. Sci. 2021, 11, 10985. [Google Scholar] [CrossRef]
- Cu, V.; Han, B. A stay cable with viscous damper and tuned mass damper. Aust. J. Struct. Eng. 2015, 16, 316–323. [Google Scholar] [CrossRef]
- Krenk, S.; Nielsen, S.R. Vibrations of a shallow cable with a viscous damper. Proc. Royal Soc. A. 2002, 458, 339–357. [Google Scholar] [CrossRef]
- Krenk, S. Vibrations of a taut cable with an external damper. J. Appl. Mech. 2000, 67, 772–776. [Google Scholar] [CrossRef]
- Chen, L.; Di, F.; Xu, Y.; Sun, L.; Xu, Y.; Wang, L. Multimode cable vibration control using a viscous-shear damper: Case studies on the Sutong Bridge. Struct. Control Health Monit. 2020, 27, e2536. [Google Scholar] [CrossRef]


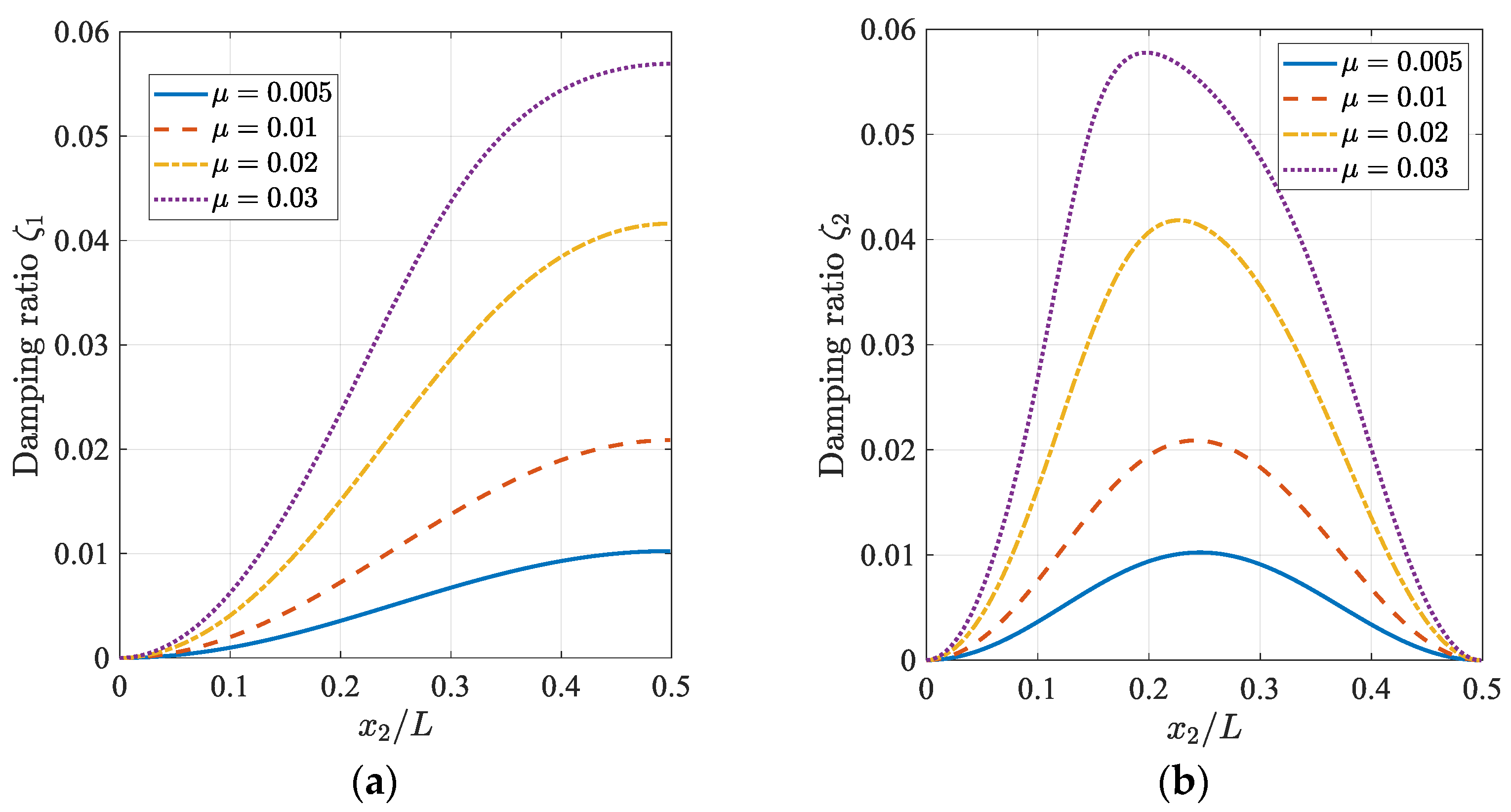
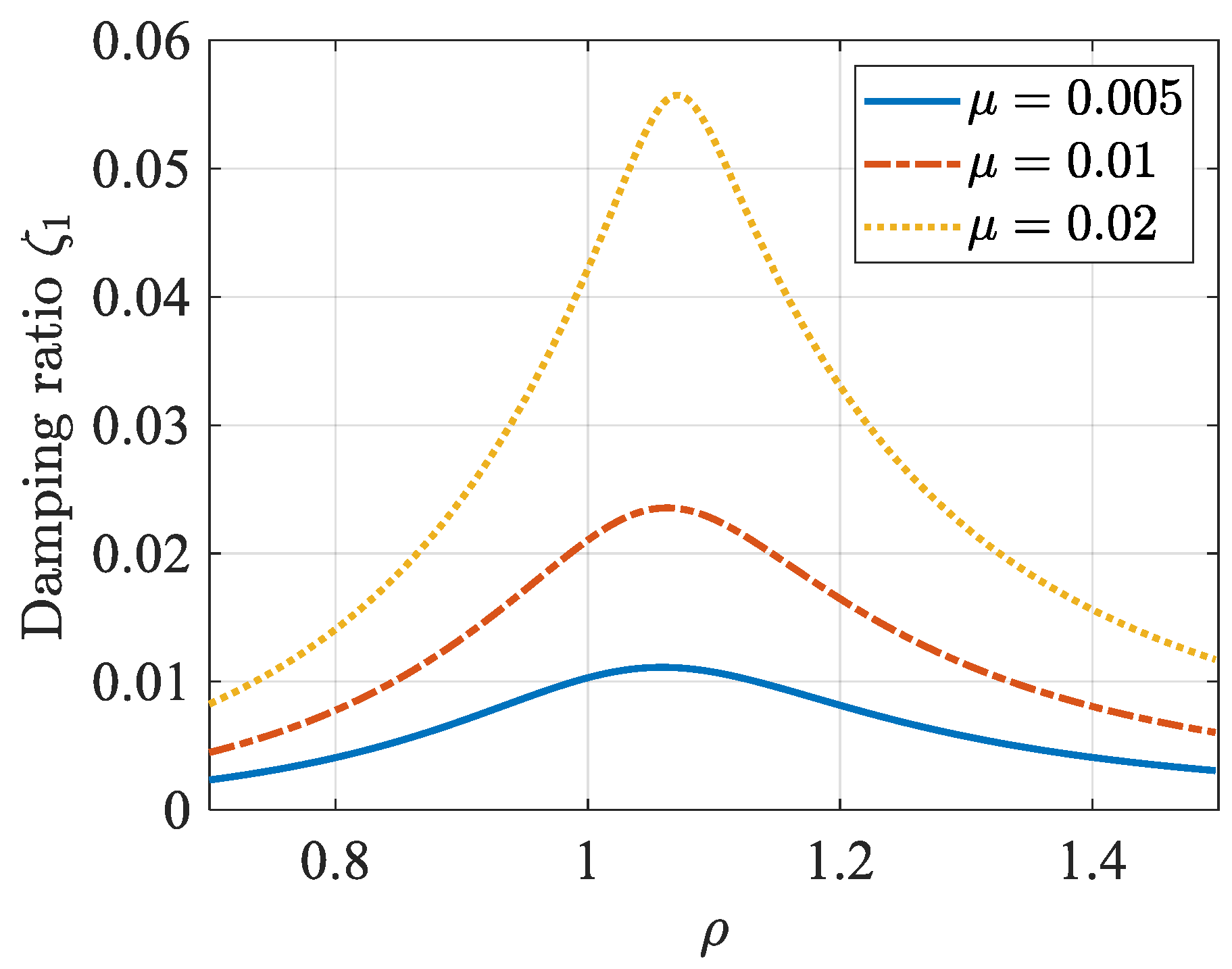


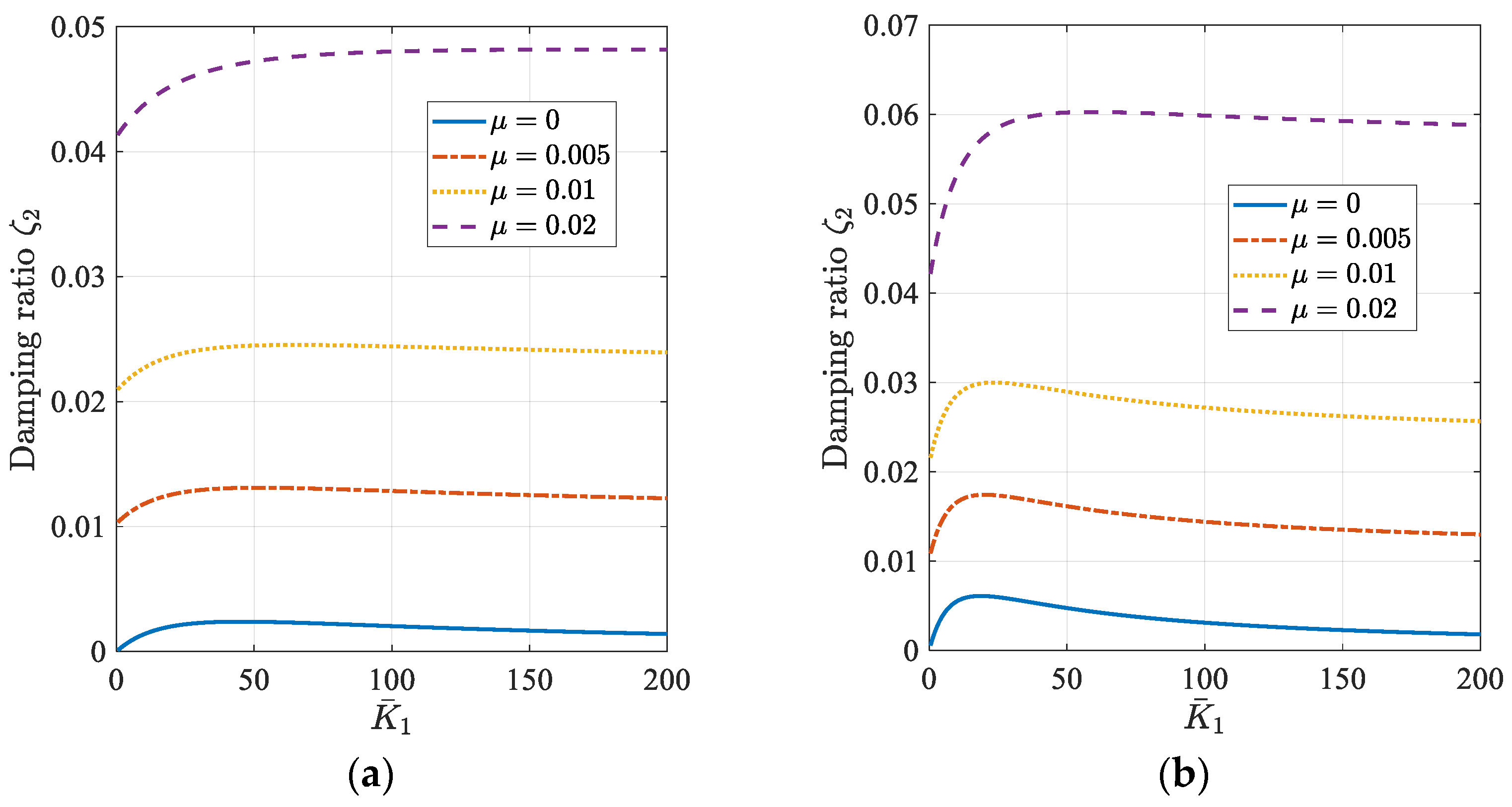
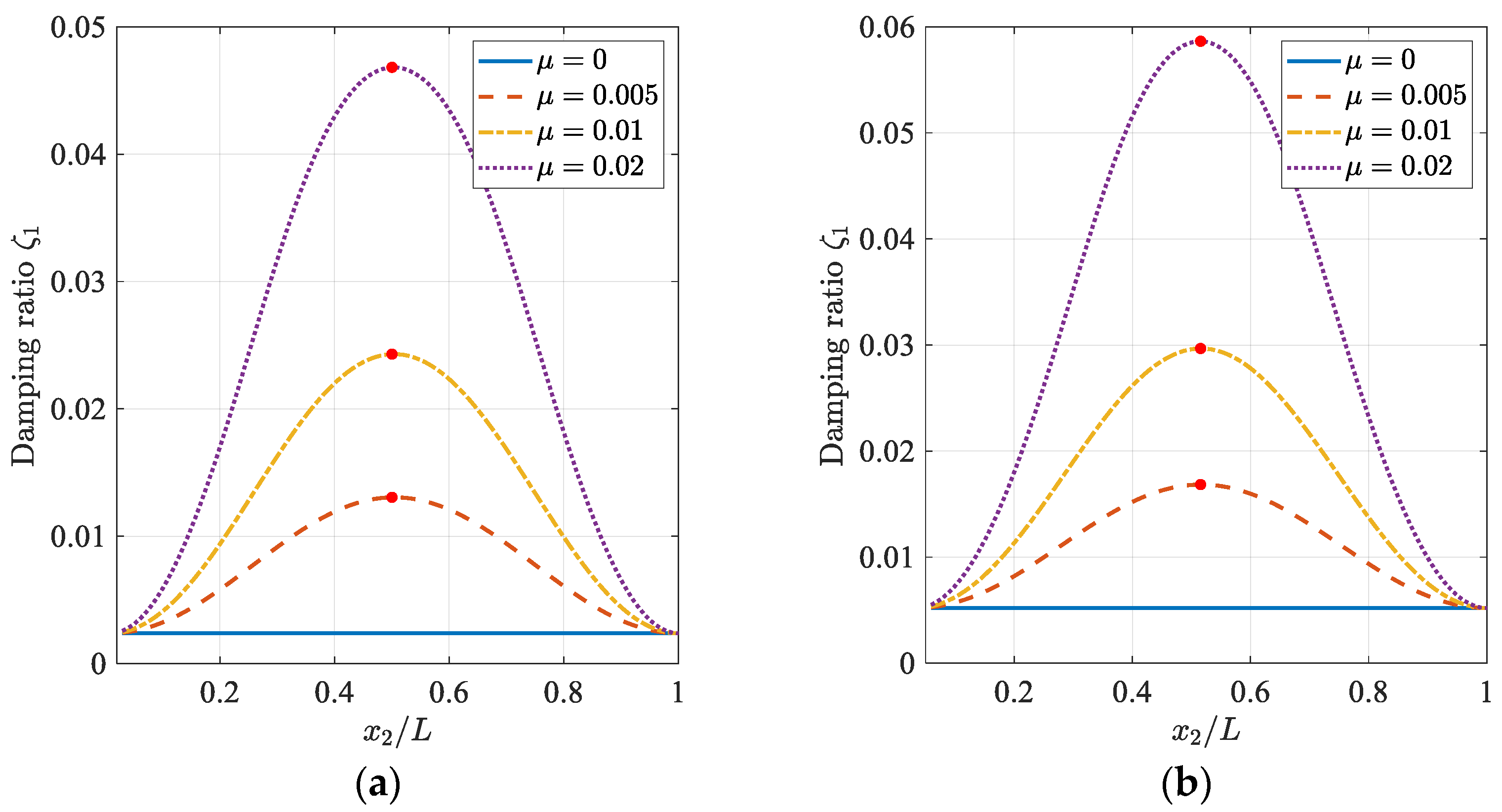

| Length L (m) | Tension T (kN) | Mass m (kg/m) | Fundamental Frequency (Hz) |
|---|---|---|---|
| 154.08 | 3831 | 70.1 | 0.759 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Li, C.; Cheng, Y.; Zhang, Y. Effectiveness of High-Damping Rubber (HDR) Damper and Tuned Mass—HDR Damper in Suppressing Stay-Cable Vibration. Appl. Sci. 2023, 13, 3356. https://doi.org/10.3390/app13053356
Xu Y, Li C, Cheng Y, Zhang Y. Effectiveness of High-Damping Rubber (HDR) Damper and Tuned Mass—HDR Damper in Suppressing Stay-Cable Vibration. Applied Sciences. 2023; 13(5):3356. https://doi.org/10.3390/app13053356
Chicago/Turabian StyleXu, Yichao, Changzhao Li, Yu Cheng, and Yufeng Zhang. 2023. "Effectiveness of High-Damping Rubber (HDR) Damper and Tuned Mass—HDR Damper in Suppressing Stay-Cable Vibration" Applied Sciences 13, no. 5: 3356. https://doi.org/10.3390/app13053356
APA StyleXu, Y., Li, C., Cheng, Y., & Zhang, Y. (2023). Effectiveness of High-Damping Rubber (HDR) Damper and Tuned Mass—HDR Damper in Suppressing Stay-Cable Vibration. Applied Sciences, 13(5), 3356. https://doi.org/10.3390/app13053356





