Minimization of the Decoupling Circuit (DC-Link) in Induction Motors
Abstract
1. Introduction
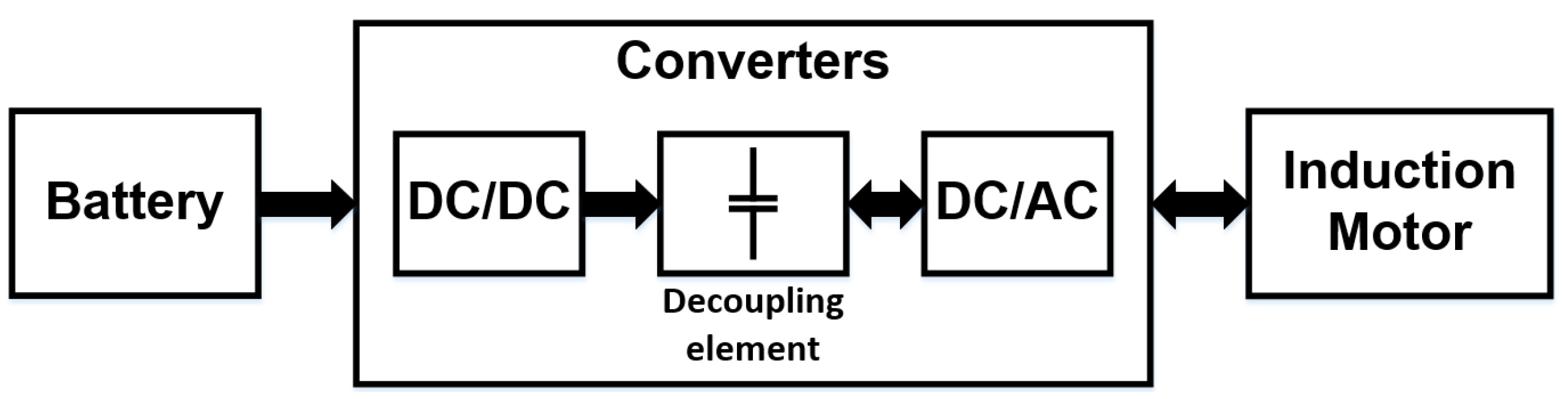
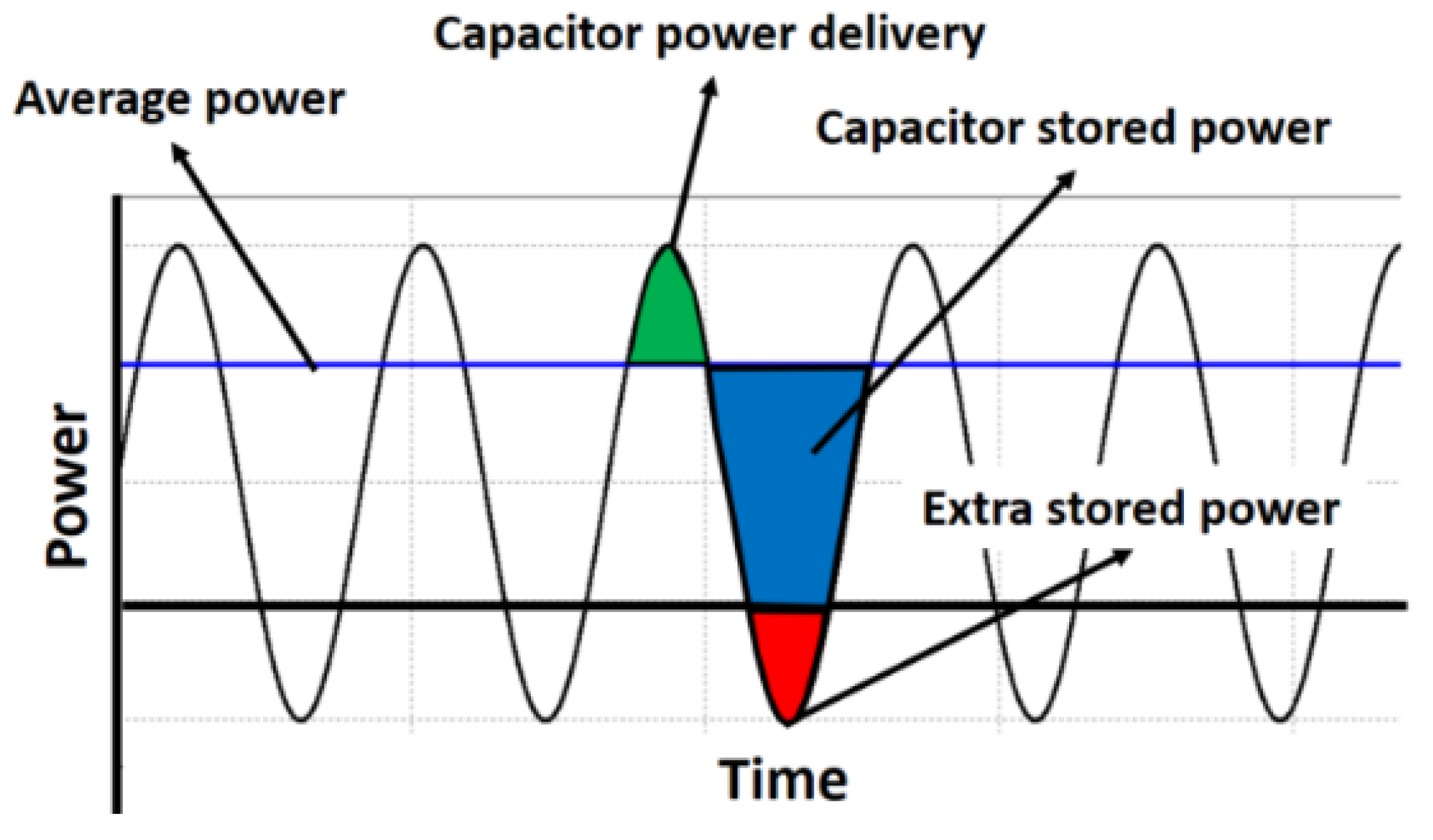
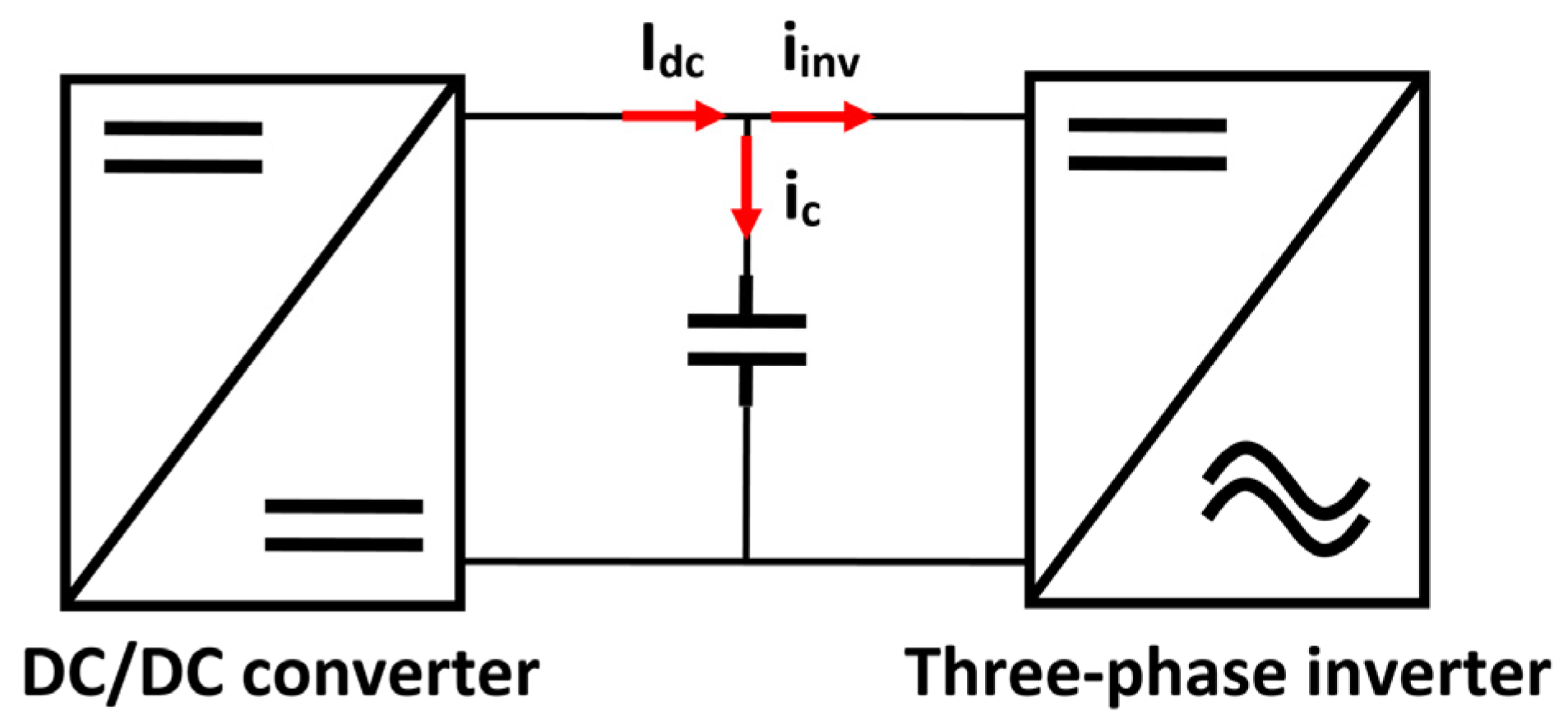
2. Energy Flow Analysis of the Study Case
2.1. Case Study Selection
- The analysis is delimited to the motor operation mode of the electric machine.
- The operation profile to be analyzed (torque variation or speed variation at constant load) is selected.
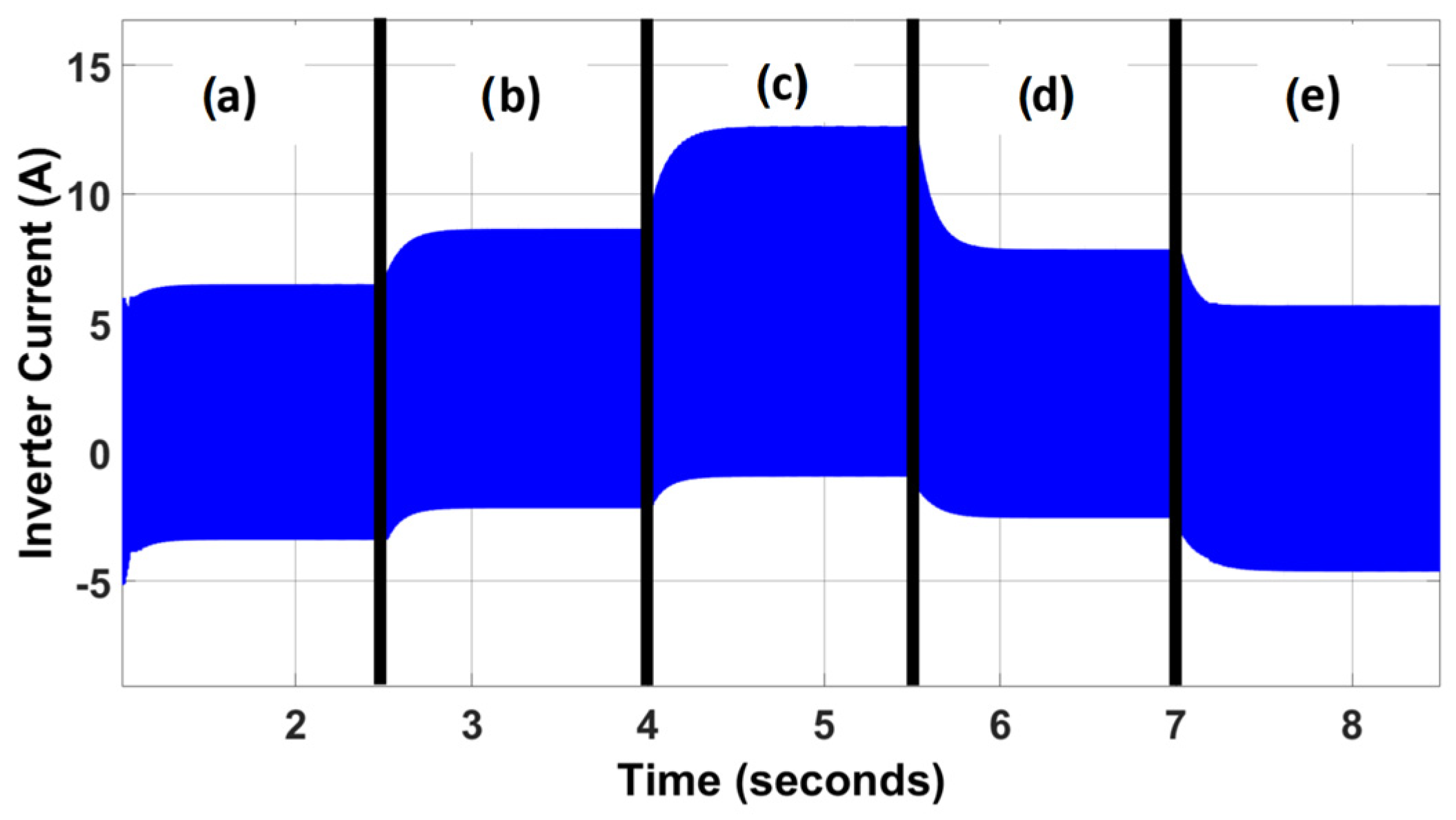
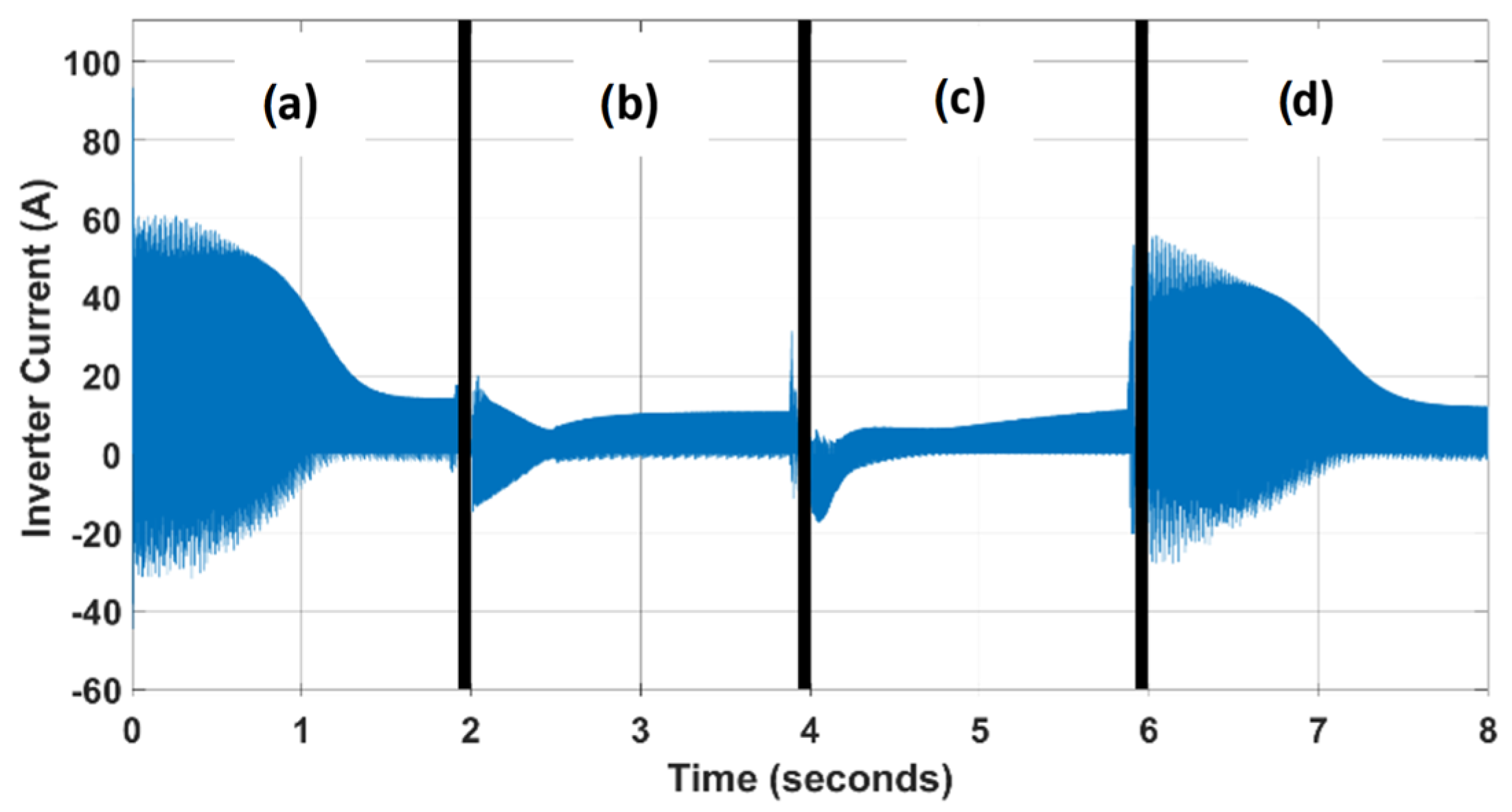
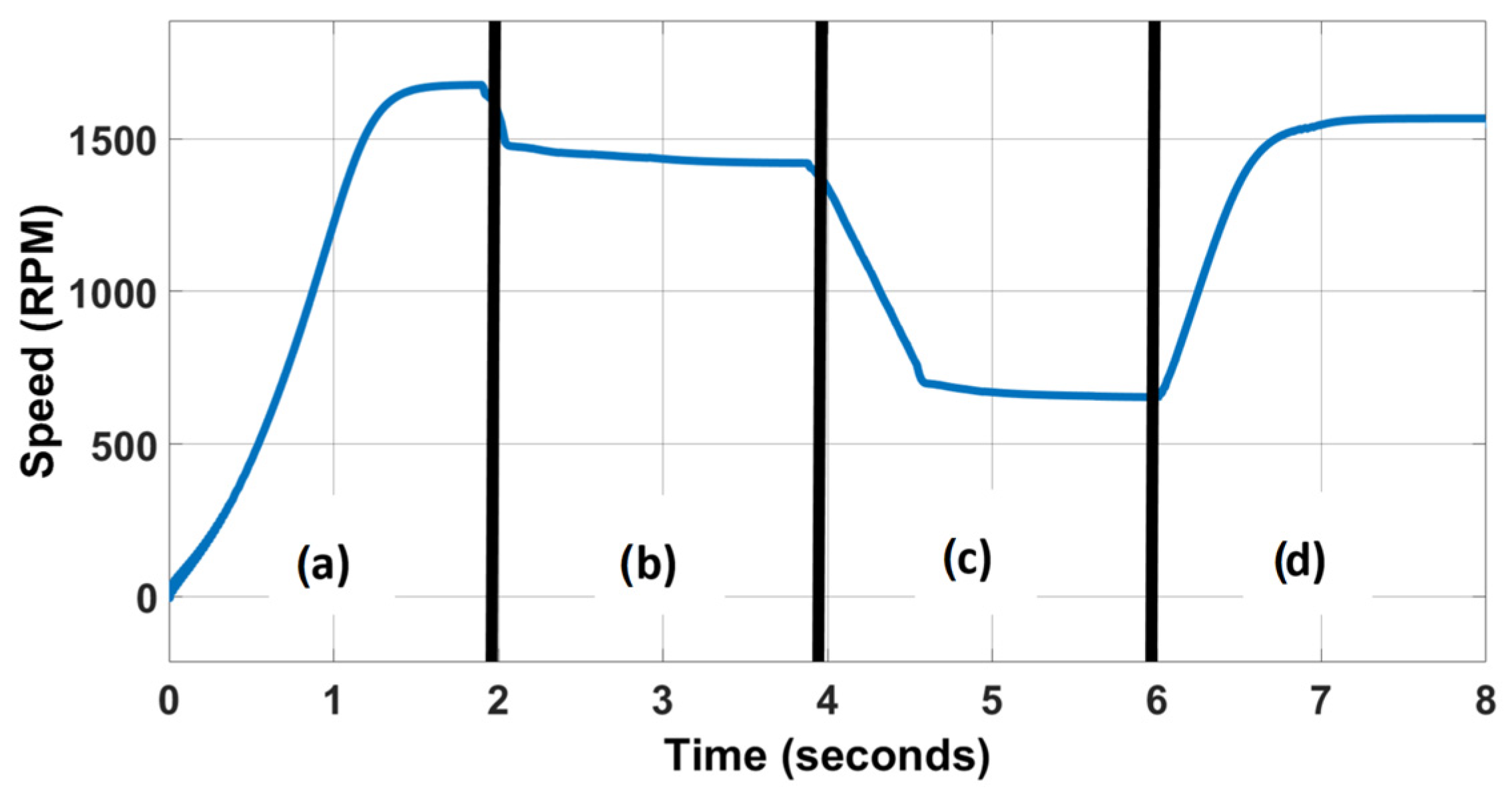
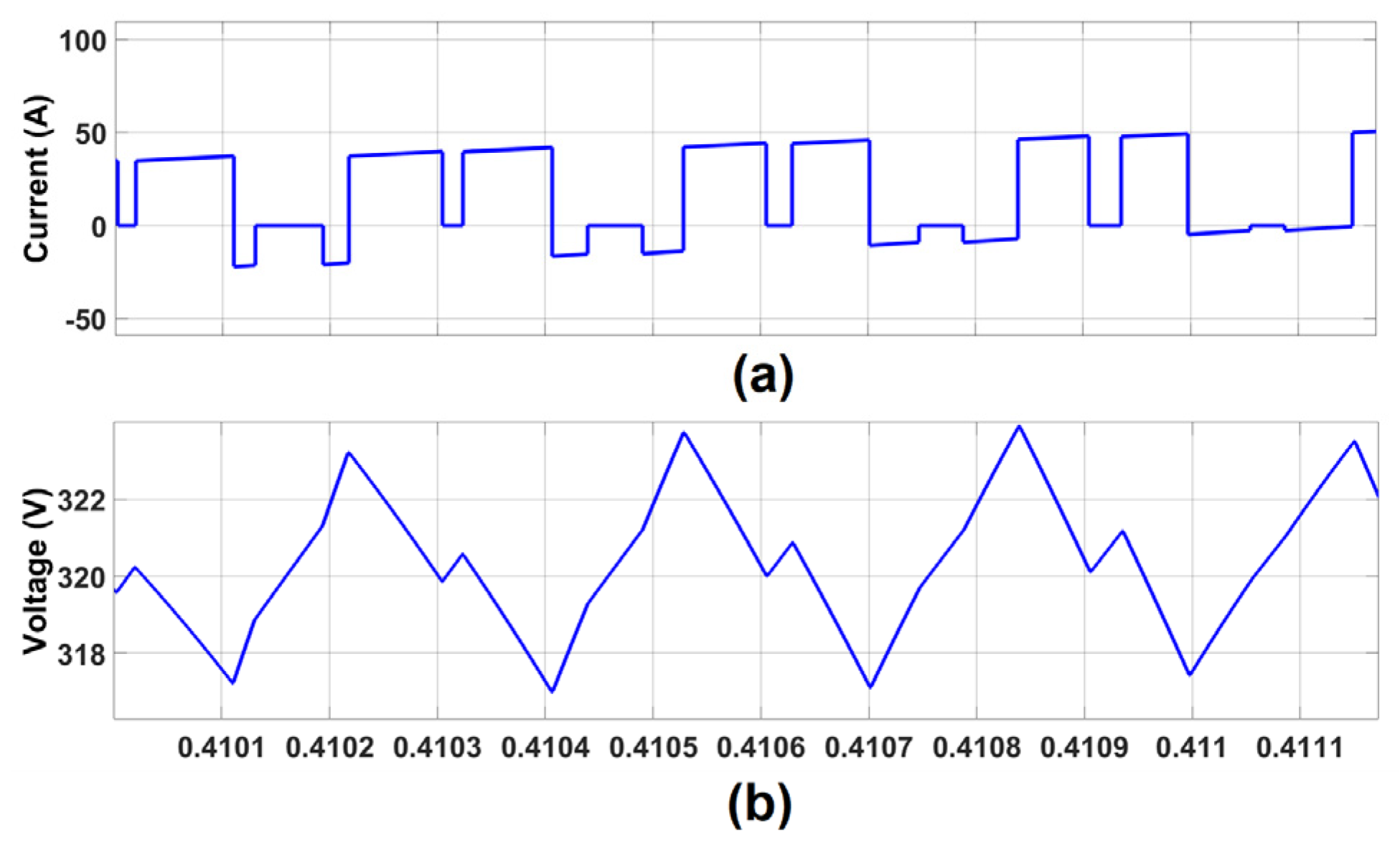
- The analysis of the power flow behavior in the selected profile is carried out, starting from the inverter current.
- The point with the highest energy return in the working profile is identified.
- The results of the previous point are obtained in both operation profiles.
- A comparison is made of the points of highest energy return obtained in both work profiles (torque variation or speed variation at constant load).
- The critical case with the highest level of energy return obtained from the previous step is selected.
- Mathematical development is performed considering the selected critical case.
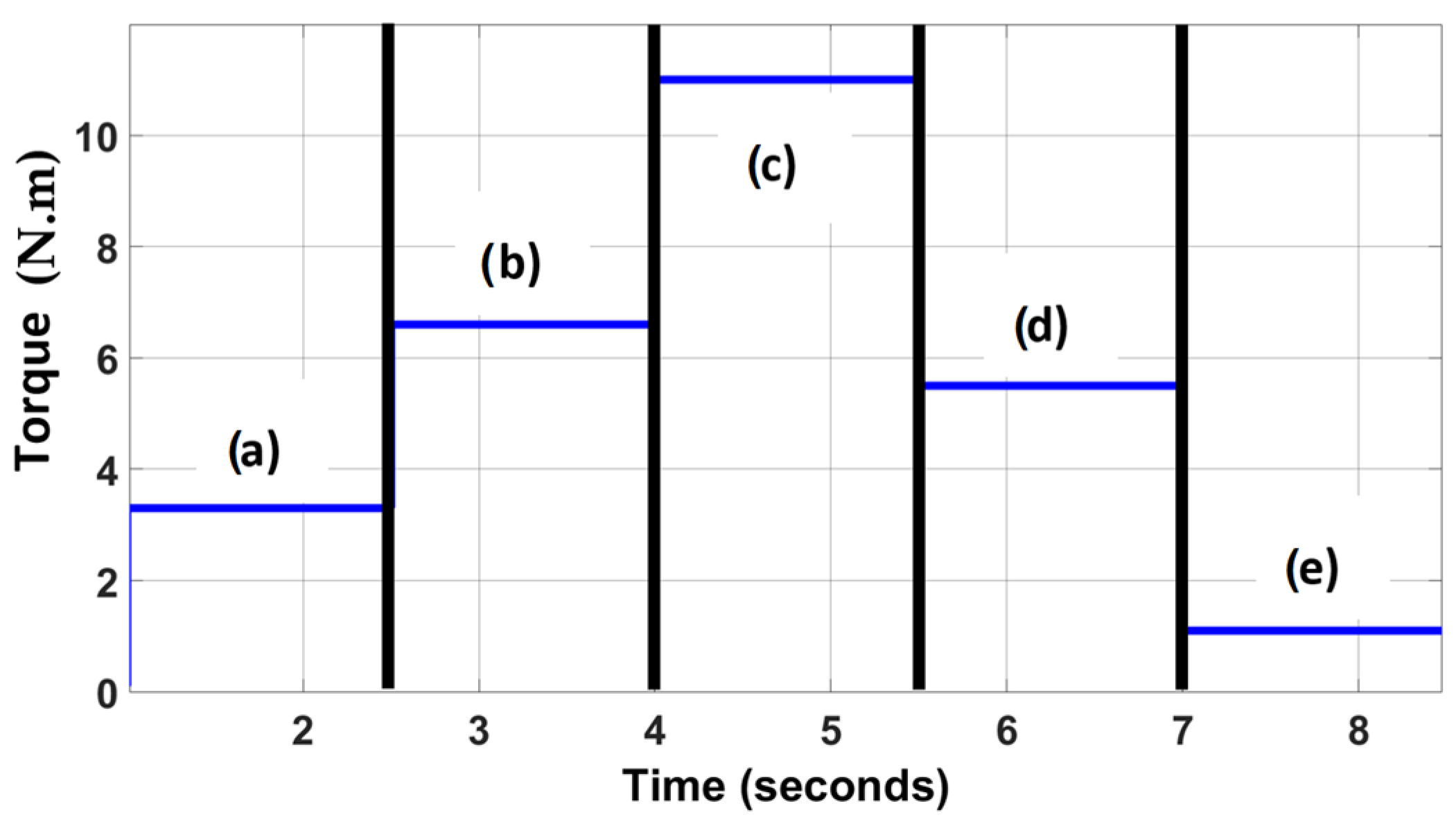
2.1.1. Torque Variation
2.1.2. Speed Variation at Constant Load
2.2. Development of a Mathematical Model
3. Results
- Average values of K-parameters are proposed to be used in (19).
- Voltage ripples are proposed and used in (19) together with the K parameters obtained in the previous step and minimum required capacitance values are obtained.
- The simulation of the complete system is carried out using the minimum required capacitance values obtained.
- The voltage ripples are obtained by simulation using the minimum capacitance value obtained previously.
- The error percentage between the values initially proposed and the values obtained using (19) is calculated.
- Select the application for which the induction motor will be used. Select the application for which the induction motor will be used to obtain the design specifications for the system.
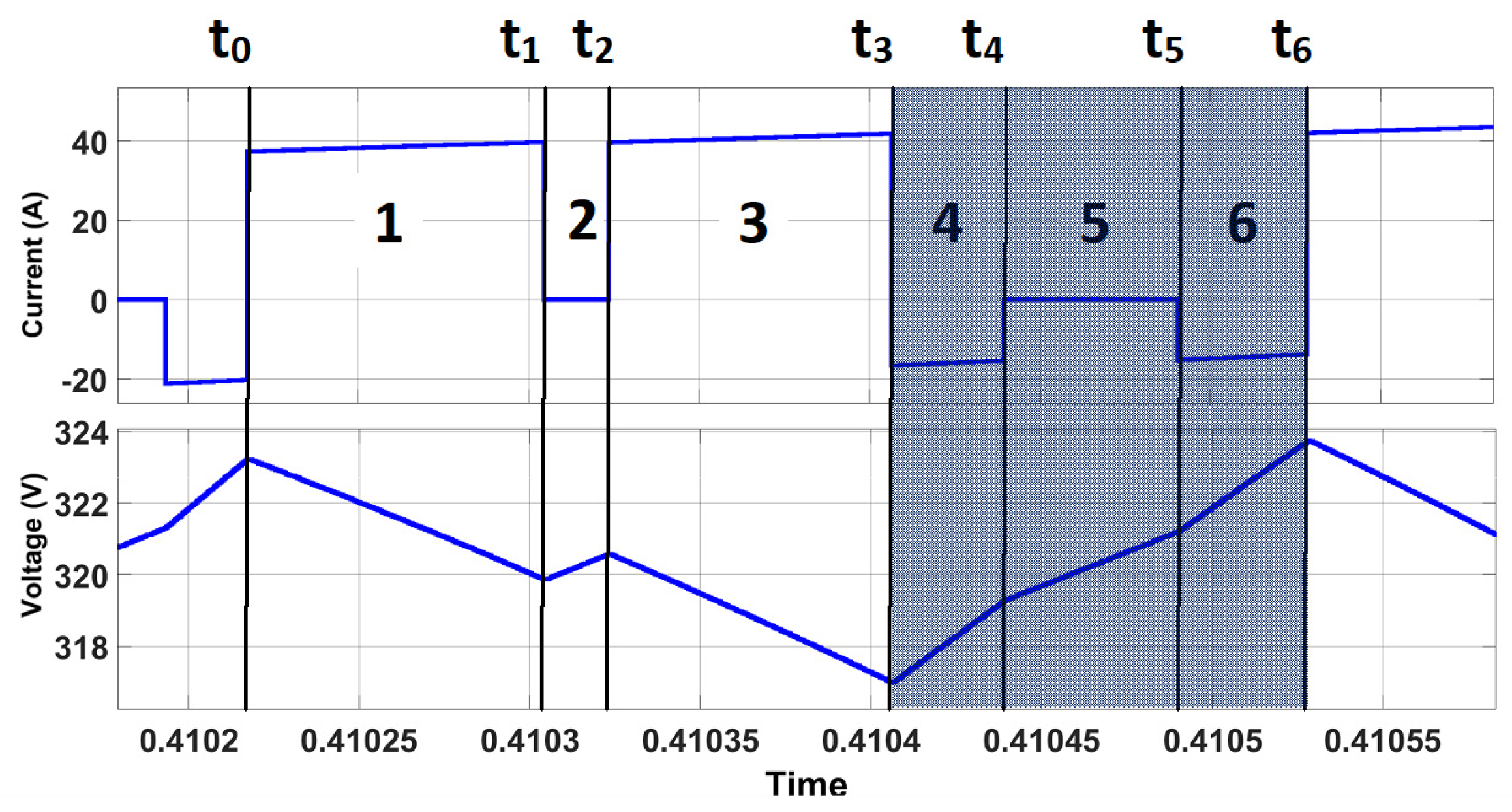
- Identify the study intervals. That is the intervals of maximum energy return to the decoupling system.
- Calculate the proportionality factors through (6), considering the intervals established in the previous point.
- Substitute the obtained values of proportionality factors and the design specifications of the selected application in the developed main equation of minimum required capacitance (19).
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Reddy, K.J.; Natarajan, S. Energy sources and multi-input DC-DC converters used in hybrid electric vehicle applications–A review. Int. J. Hydrog. Energy 2018, 43, 17387–17408. [Google Scholar] [CrossRef]
- Wang, C.; Xiong, R.; He, H.; Ding, X.; Shen, W. Efficiency analysis of a bidirectional DC/DC converter in a hybrid energy storage system for plug-in hybrid electric vehicles. Appl. Energy 2016, 183, 612–622. [Google Scholar] [CrossRef]
- Langbroek, J.H.; Cebecauer, M.; Malmsten, J.; Franklin, J.P.; Susilo, Y.O.; Georén, P. Electric vehicle rental and electric vehicle adoption. Res. Transp. Econ. 2019, 73, 72–82. [Google Scholar] [CrossRef]
- Nakamoto, Y.; Nishijima, D.; Kagawa, S. The role of vehicle lifetime extensions of countries on global CO2 emissions. J. Clean. Prod. 2019, 207, 1040–1046. [Google Scholar] [CrossRef]
- Luin, B.; Petelin, S.; Al-Mansour, F. Microsimulation of electric vehicle energy consumption. Energy 2019, 174, 24–32. [Google Scholar] [CrossRef]
- Tran, T.-V.; Edouard, N.; Mikati, K.; Pellerey, P.; Assaad, B. Optimal design and experimental prototype testing of a low-cost machine for city battery electric vehicle. In Proceedings of the 2018 IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 3886–3892. [Google Scholar]
- Szántó, A.; Kiss, J.; Mankovits, T.; Szíki, G.Á. Dynamic Test Measurements and Simulation on a Series Wound DC Motor. Appl. Sci. 2021, 11, 4542. [Google Scholar] [CrossRef]
- Szíki, G.Á.; Szántó, A.; Mankovits, T. Dynamic modelling and simulation of a prototype race car in MATLAB/Simulink applying different types of electric motors. Int. Rev. Appl. Sci. Eng. 2021, 12, 57–63. [Google Scholar] [CrossRef]
- Kapustin, A.; Terentiev, A. Rational Lifetime of a Vehicle in Terms of Ensuring Security of Its Design. Transp. Res. Procedia 2017, 20, 254–260. [Google Scholar] [CrossRef]
- Cheng, S.; Li, C.; Chai, f.; Gong, H. Research on Induction Motor for Mini Electric Vehicles. Energy Procedia 2012, 17, 249–257. [Google Scholar] [CrossRef]
- Guan, Y.; Zhu, Z.Q.; Afinowi, I.A.A.; Mipo, J.C.; Farah, P. Difference in maximum torque-speed characteristics of induction machine between motor and generator operation modes for electric vehicle application. Electr. Power Syst. Res. 2016, 136, 406–414. [Google Scholar] [CrossRef]
- Joseph, J.J.; Juliha, J.; Josh, F. Review on the recent development of the power converters for electric vehicle. In Proceedings of the 2017 2nd International Conference on Communication and Electronics Systems (ICCES), Coimbatore, India, 19–20 October 2017; pp. 641–644. [Google Scholar]
- Hu, H.; Harb, S.; Kutkut, N.H.; Shen, Z.J.; Batarseh, I. A single-stage microinverter without using eletrolytic capacitors. IEEE Trans. Power Electron. 2012, 28, 2677–2687. [Google Scholar] [CrossRef]
- Gao, Z.; LaClair, T.; Ou, S.; Huff, S.; Wu, G.; Hao, P.; Boriboonsomsin, K.; Barth, M. Evaluation of electric vehicle component performance over eco-driving cycles. Energy 2019, 172, 823–839. [Google Scholar] [CrossRef]
- Cao, J.; Emadi, A. A New Battery/UltraCapacitor Hybrid Energy Storage System for Electric, Hybrid, and Plug-In Hybrid Electric Vehicles. IEEE Trans. Power Electron. 2012, 27, 122–132. [Google Scholar] [CrossRef]
- Husain, I. Electric and hybrid vehicles: Design fundamentals; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Loganathan, M.K.; Mishra, B.; Tan, C.; Kongsvik, T.; Rai, R.N. Multi-Criteria decision making (MCDM) for the selection of Li-Ion batteries used in electric vehicles (EVs). Mater. Today Proc. 2020, 41, 1073–1077. [Google Scholar] [CrossRef]
- Loganathan, M.K.; Tan, C.M.; Sultana, S.; Hsieh, I.Y.L.; Kumaraswamidhas, L.A.; Rai, R.N. Parametric performance analysis of battery operated electric vehicle. In Proceedings of the 2021 International Conference on Sustainable Energy and Future Electric Transportation (SEFET), Hyderabad, India, 21–23 January 2021; pp. 1–6. [Google Scholar]
- Wen, H.; Xiao, W.; Wen, X.; Armstrong, P. Analysis and Evaluation of DC-Link Capacitors for High-Power-Density Electric Vehicle Drive Systems. IEEE Trans. Veh. Technol. 2012, 61, 2950–2964. [Google Scholar] [CrossRef]
- Rachev, E.; Petrov, V. DC link capacitor selection for DC-DC converters. In Proceedings of the 2020 12th Electrical Engineering Faculty Conference (BulEF), Varna Town, Bulgaria, 9–12 September 2020; pp. 1–5. [Google Scholar]
- Meral, M.E.; Çelik, D. Mitigation of DC-link voltage oscillations to reduce size of DC-side capacitor and improve lifetime of power converter. Electr. Power Syst. Res. 2021, 194, 107048. [Google Scholar] [CrossRef]
- Sher, H.A.; Addoweesh, K.E.; Khan, Y. Effect of short circuited DC link capacitor of an AC–DC–AC inverter on the performance of induction motor. J. King Saud Univ.-Eng. Sci. 2016, 28, 199–206. [Google Scholar] [CrossRef]
- Sridharan, S.; Kikuchi, J. DC-Link Capacitor Sizing in HEV/EV e-Drive Power Electronic System from Stability Viewpoint; SAE Technical Paper 2020-01-0468; SAE: Warrendale, PA, USA, 2020. [Google Scholar] [CrossRef]
- He, P.; Khaligh, A. Comprehensive analyses and comparison of 1 kW isolated DC–DC converters for bidirectional EV charging systems. IEEE Trans. Transp. Electrif. 2016, 3, 147–156. [Google Scholar] [CrossRef]
- Li, Y.; Liu, M.; Lau, J.; Zhang, B. A novel method to determine the motor efficiency under variable speed operations and partial load conditions. Appl. Energy 2015, 144, 234–240. [Google Scholar] [CrossRef]
- Moreno-Basaldúa, E.A.; Morales-Saldaña, J.A.; Loera-Palomo, R. Design methodology for quadratic step-up dc-dc converters based on non-cascading structures. In Proceedings of the 2016 13th International Conference on Power Electronics (CIEP), Guanajuato, Mexico, 20–23 June 2016; pp. 210–215. [Google Scholar]
- Heydari-Doostabad, H.; Hosseini, S.H.; Ghazi, R.; Donnell, T.O. Pseudo DC-Link EV Home Charger With a High Semiconductor Device Utilization Factor. IEEE Trans. Ind. Electron. 2022, 69, 2459–2469. [Google Scholar] [CrossRef]
- Yao, F.; Wang, B.; Li, Z.; Peng, Y. Reliability study on DC-link capacitor in fault states of VSI in elective vehicle. J. Eng. 2019, 2544–2550. [Google Scholar] [CrossRef]
- Li, Q.; Jiang, D. DC-link Current Analysis of Three-Phase Two-Level VSI Considering AC Current Ripple. IET Power Electron. 2017, 11, 202–211. [Google Scholar] [CrossRef]
- Krein, P.T.; Balog, R.S.; Mirjafari, M. Minimum Energy and Capacitance Requirements for Single-Phase Inverters and Rectifiers Using a Ripple Port. IEEE Trans. Power Electron. 2012, 27, 4690–4698. [Google Scholar] [CrossRef]
- Hussain, M.; Talha, M.; Raihan, S.; Butt, O.; Butt, T.; Ashfaq, M. Simulative Study to Reduce DC-Link Capacitor of Drive Train for Electric Vehicles. Energies 2022, 15, 4499. [Google Scholar] [CrossRef]
- Sridharan, S.; Kimmel, J.; Kikuchi, J. DC-Link Capacitor Sizing Considerations for HEV/EV e-Drive System. SAE Tech. Pap. 2017. Available online: https://www.sae.org/publications/technical-papers/content/2017-01-1234/ (accessed on 14 February 2023).
- Mnati, M.J.; Abed, J.K.; Bozalakov, D.V.; Van den Bossche, A. Analytical and calculation DC-link capacitor of a three-phase grid-tied photovoltaic inverter. In Proceedings of the 2018 IEEE 12th International Conference on Compatibility, Power Electronics and Power Engineering (CPE-POWERENG 2018), Doha, Qatar, 10–12 April 2018; pp. 1–6. [Google Scholar]
- Monteiro, V.; Afonso, J.L. A Unified Topology for the Integration of Electric Vehicle, Renewable Energy Source, and Active Filtering for the Power Quality Improvement of the Electrical Power Grid: An Experimental Validation. Electronics 2022, 11, 429. [Google Scholar] [CrossRef]
- Schäfer, J.; Kolar, J.W. Three-Port Series-Resonant DC/DC Converter for Automotive Charging Applications. Electronics 2021, 10, 2543. [Google Scholar] [CrossRef]
- Barlini, D. DC-Link Decoupling Circuit for Parallel Inverters. European Patent 12156390.2, EP 2631104A1, 2013. Available online: https://worldwide.espacenet.com/patent/search/family/045656326/publication/EP2631104A1?q=12156390.2 (accessed on 14 February 2023).
- Farhadi, M.; Mohammed, O. Energy Storage Technologies for High-Power Applications. IEEE Trans. Ind. Appl. 2016, 52, 1953–1961. [Google Scholar] [CrossRef]
- Naseri, F.; Farjah, E.; Ghanbari, T. An Efficient Regenerative Braking System Based on Battery/Supercapacitor for Electric, Hybrid, and Plug-In Hybrid Electric Vehicles With BLDC Motor. IEEE Trans. Veh. Technol. 2017, 66, 3724–3738. [Google Scholar] [CrossRef]
- Gillespie, T. Fundamentals of Vehicle Dynamics; SAE International: Warrendale, PA, USA, 2021. [Google Scholar]
- Guzzella, L.; Sciarretta, A. Vehicle Propulsion Systems; Springer: Berlin/Heidelberg, Germany, 2007; Volume 1. [Google Scholar]

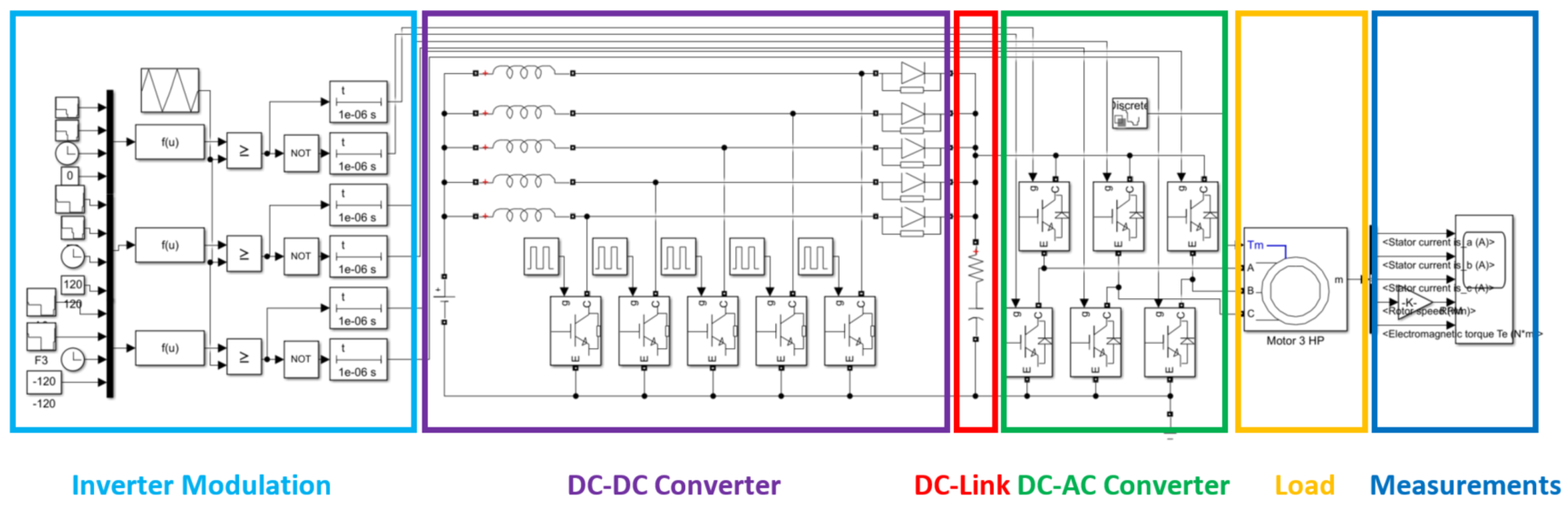

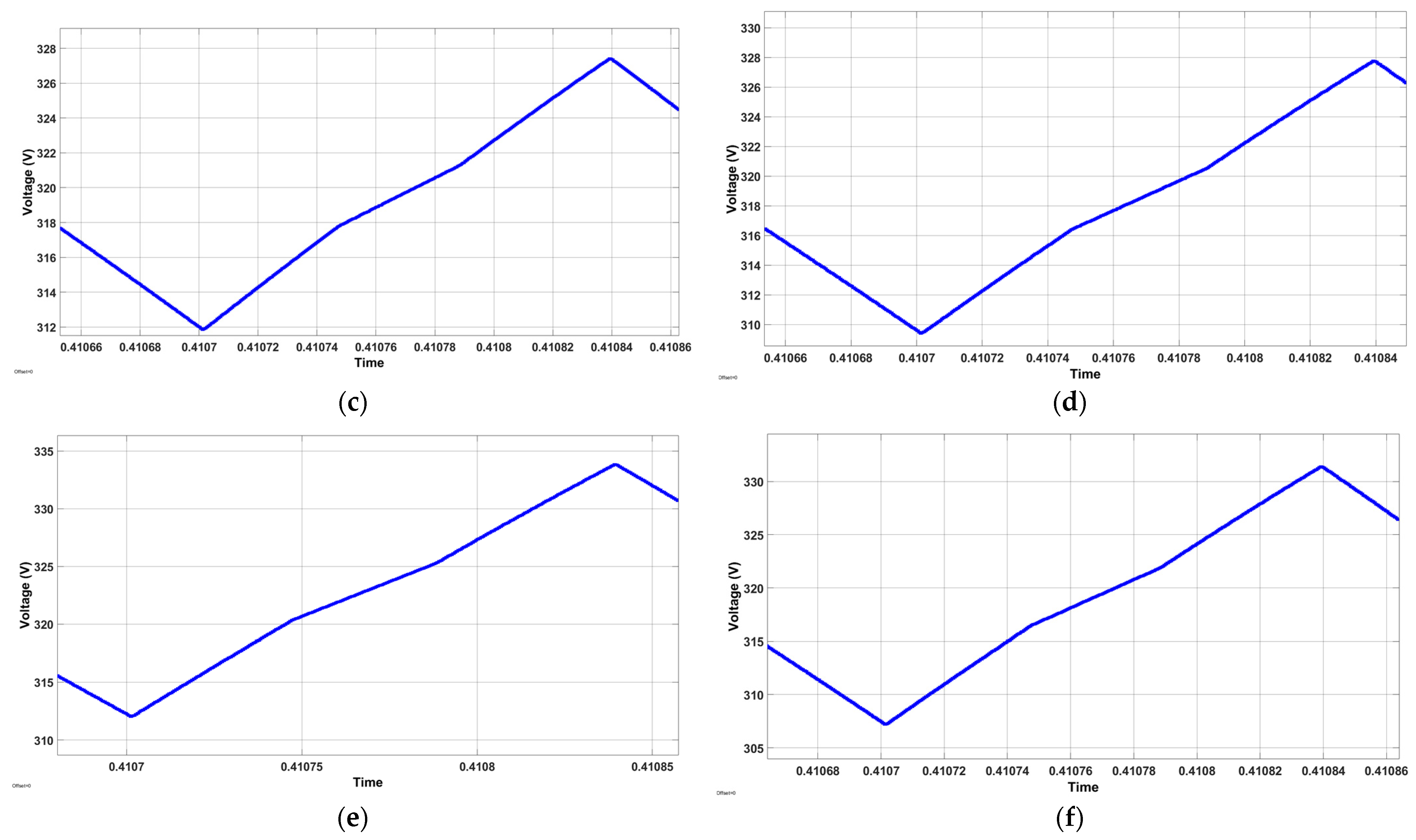

| Parameter | Value |
|---|---|
| Power load | 3 HP |
| Motor speed | 1800 RPM |
| Nominal motor current | 8 A |
| Number of poles | 4 |
| Parameter | Value |
|---|---|
| DC–AC Converter and Modulation | |
| Inverter | 33-phase H-bridge inverter |
| Modulation | SPWM |
| DC source inverter | 320 V |
| Modulating signal frequency | 60 Hz |
| Carrier signal frequency | 3300 Hz |
| Modulation index | 0.9 |
| DC-link | |
| Capacitor | 200 µ |
| ESR | 0.20 Ω |
| Section | Interval |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 |
| Parameter | Value |
|---|---|
| DC source | 320 V |
| Modulating signal frequency | 60 Hz |
| Carrier signal frequency | 3300 Hz |
| Modulation index | 0.9 |
| Power load (Motor) | 3 HP |
| 3 HP Induction Motor | Proportionality Factors | ||
|---|---|---|---|
| KDC | K4 | K6 | |
| Motor 1 | 43/14 | 10/7 | 17/14 |
| Motor 2 | 2.96 | 1.4 | 1.19 |
| Motor 3 | 2.85 | 1.48 | 1.24 |
| Motor 4 | 2.91 | 1.45 | 1.21 |
| Proposed Voltage Ripple (%) | Capacitance Obtained Using the Proposed Equation μF |
|---|---|
| 3 | 361.2 |
| 4 | 270 |
| 5 | 217 |
| 6 | 180.6 |
| 7 | 155.8 |
| 8 | 136 |
| Measurement Number | Error Percentage (%) |
|---|---|
| 1 | 4.6 |
| 2 | 3.75 |
| 3 | 4.8 |
| 4 | 5.51 |
| 5 | 5.4 |
| 6 | 6.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carrillo-Santos, L.M.; Ponce-Silva, M.; Hernández-González, L.; Calzada-Lara, G.; Reyes-Severiano, Y.; Juarez-Sandoval, O.U. Minimization of the Decoupling Circuit (DC-Link) in Induction Motors. Appl. Sci. 2023, 13, 3674. https://doi.org/10.3390/app13063674
Carrillo-Santos LM, Ponce-Silva M, Hernández-González L, Calzada-Lara G, Reyes-Severiano Y, Juarez-Sandoval OU. Minimization of the Decoupling Circuit (DC-Link) in Induction Motors. Applied Sciences. 2023; 13(6):3674. https://doi.org/10.3390/app13063674
Chicago/Turabian StyleCarrillo-Santos, Luis Mauricio, Mario Ponce-Silva, Leobardo Hernández-González, Gabriel Calzada-Lara, Yesenia Reyes-Severiano, and Oswaldo Ulises Juarez-Sandoval. 2023. "Minimization of the Decoupling Circuit (DC-Link) in Induction Motors" Applied Sciences 13, no. 6: 3674. https://doi.org/10.3390/app13063674
APA StyleCarrillo-Santos, L. M., Ponce-Silva, M., Hernández-González, L., Calzada-Lara, G., Reyes-Severiano, Y., & Juarez-Sandoval, O. U. (2023). Minimization of the Decoupling Circuit (DC-Link) in Induction Motors. Applied Sciences, 13(6), 3674. https://doi.org/10.3390/app13063674








