A 2.5D Finite Element Method Combined with Zigzag-Paraxial Boundary for Long Tunnel under Obliquely Incident Seismic Wave
Abstract
:1. Introduction
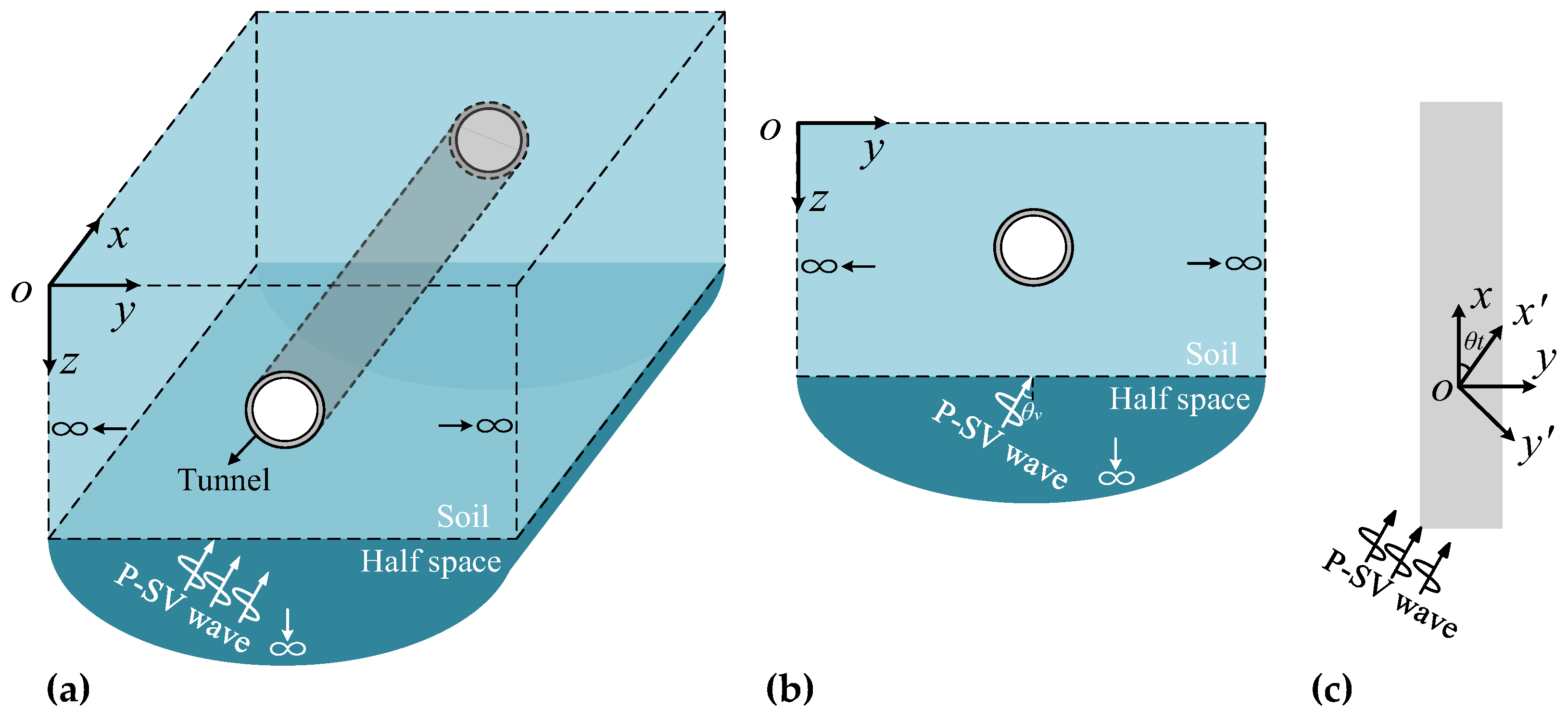
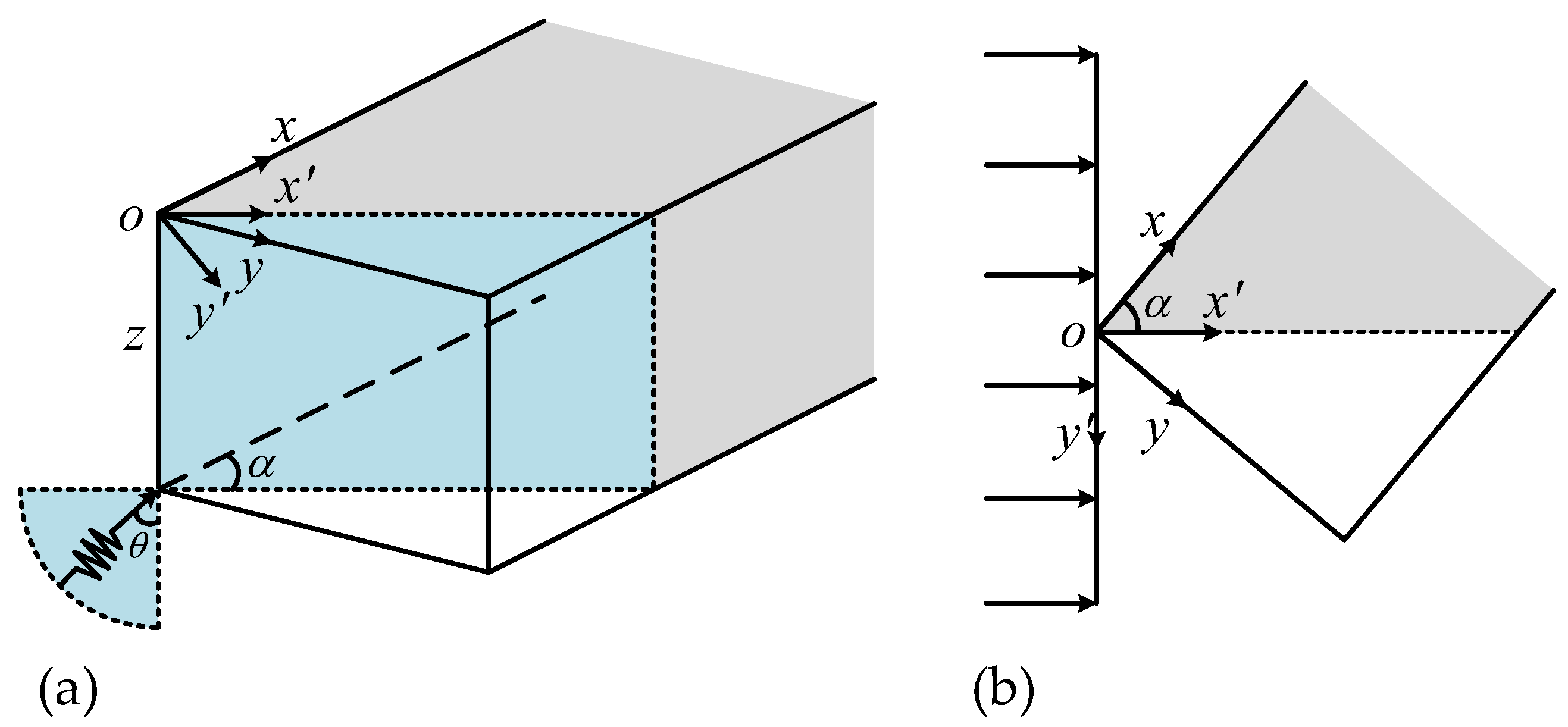
2. Wave Scattering Problem of Soil–Tunnel Interaction under Oblique Incidence of P-SV Wave
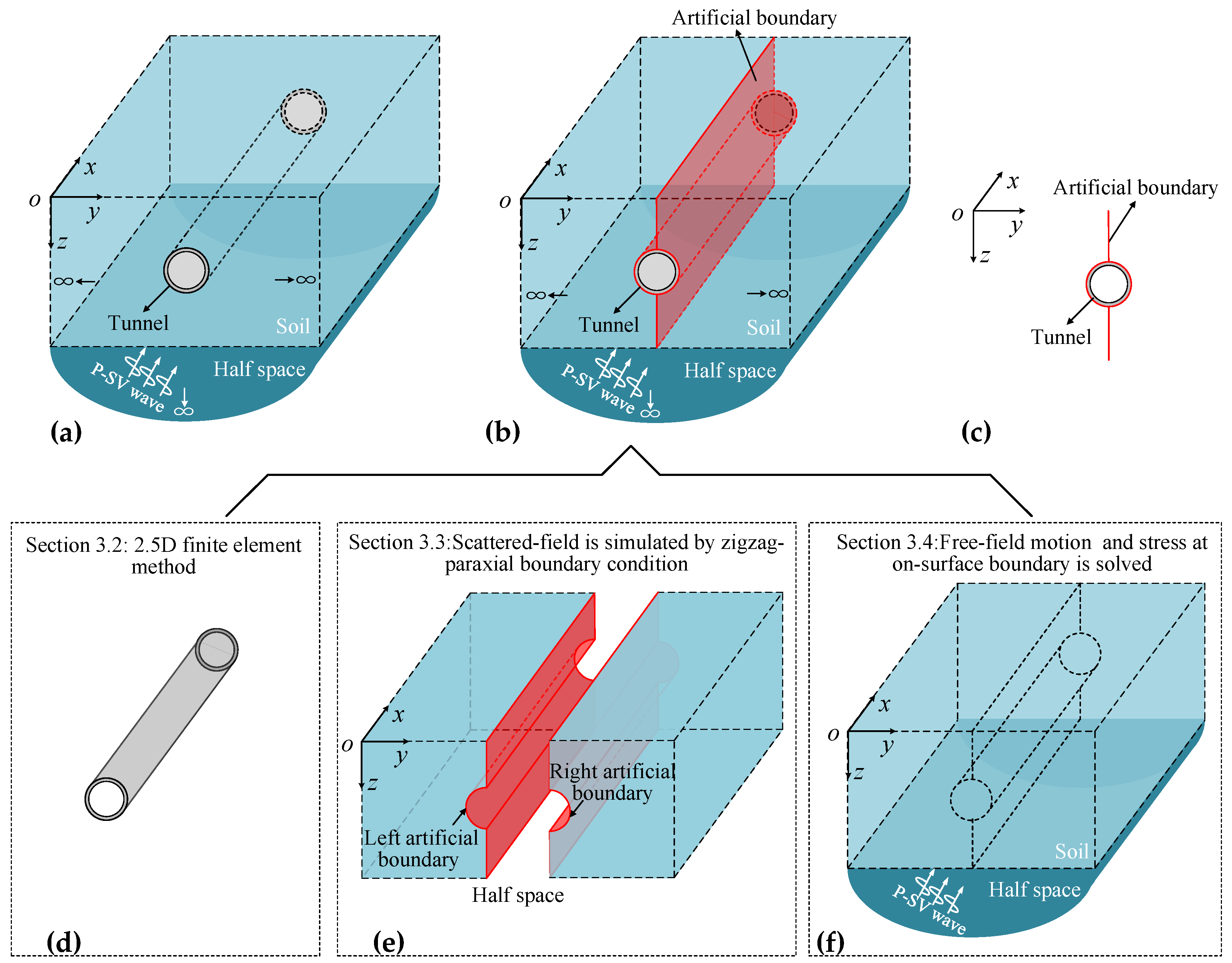
3. 2.5D Finite Element Substructure Method
3.1. The Framework of the Proposed 2.5D Finite Element Substructure Method
3.2. Description of the 2.5D Finite Element Method
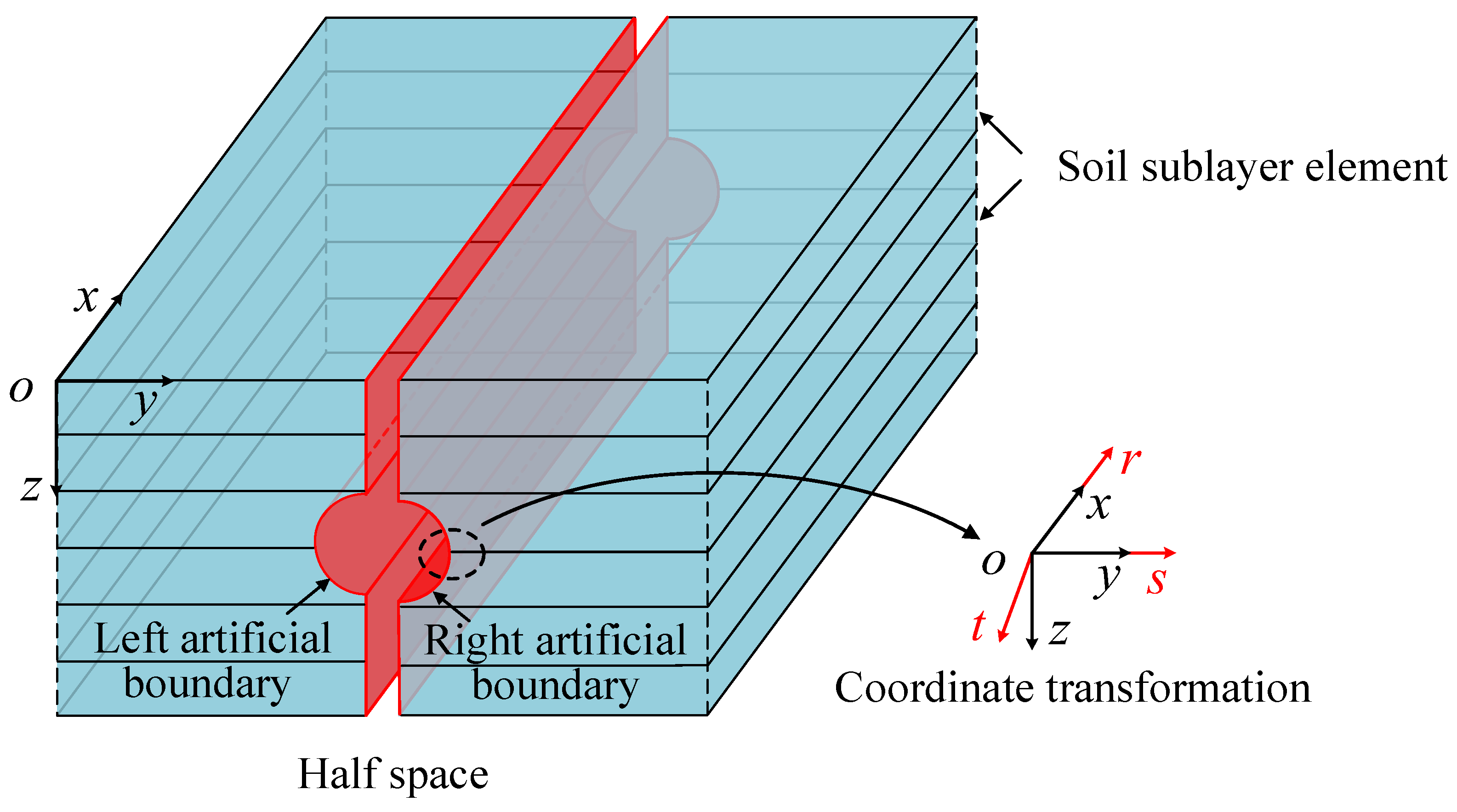
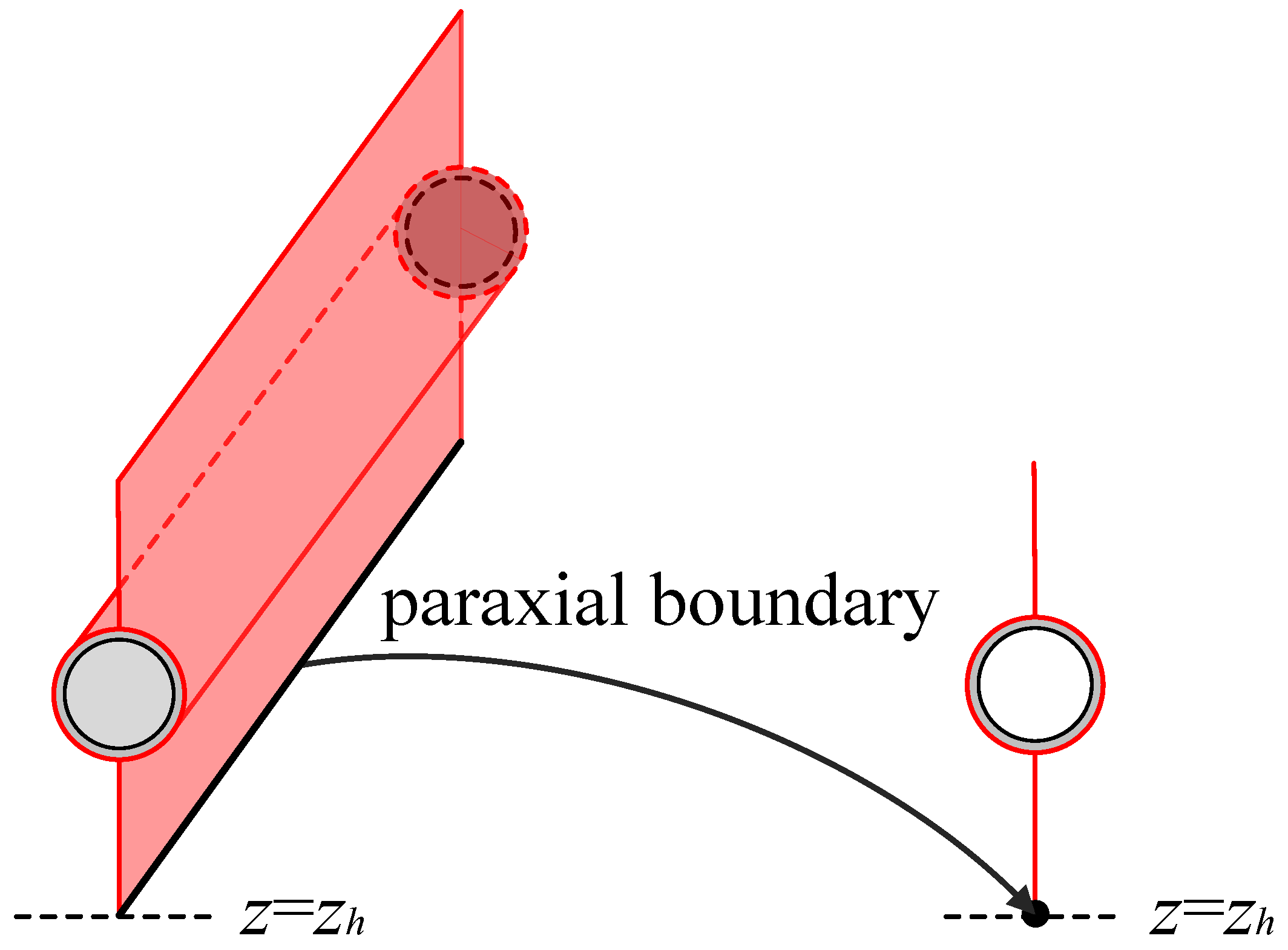
3.3. A Zigzag-Paraxial Boundary Condition Suitable for 2.5D Finite Element Method
3.4. Seismic Wave Input
3.5. System Equation
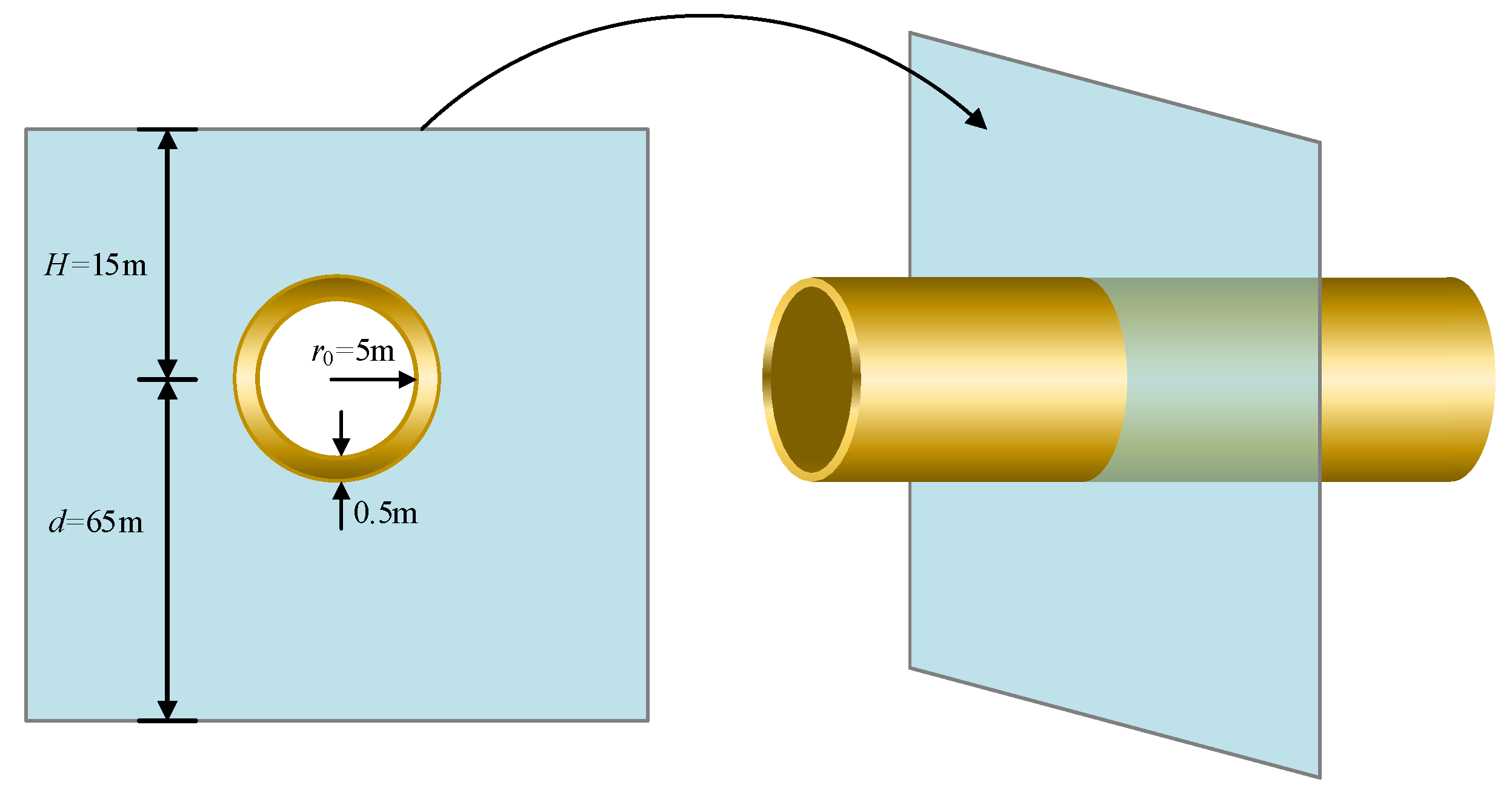
4. Method Verification
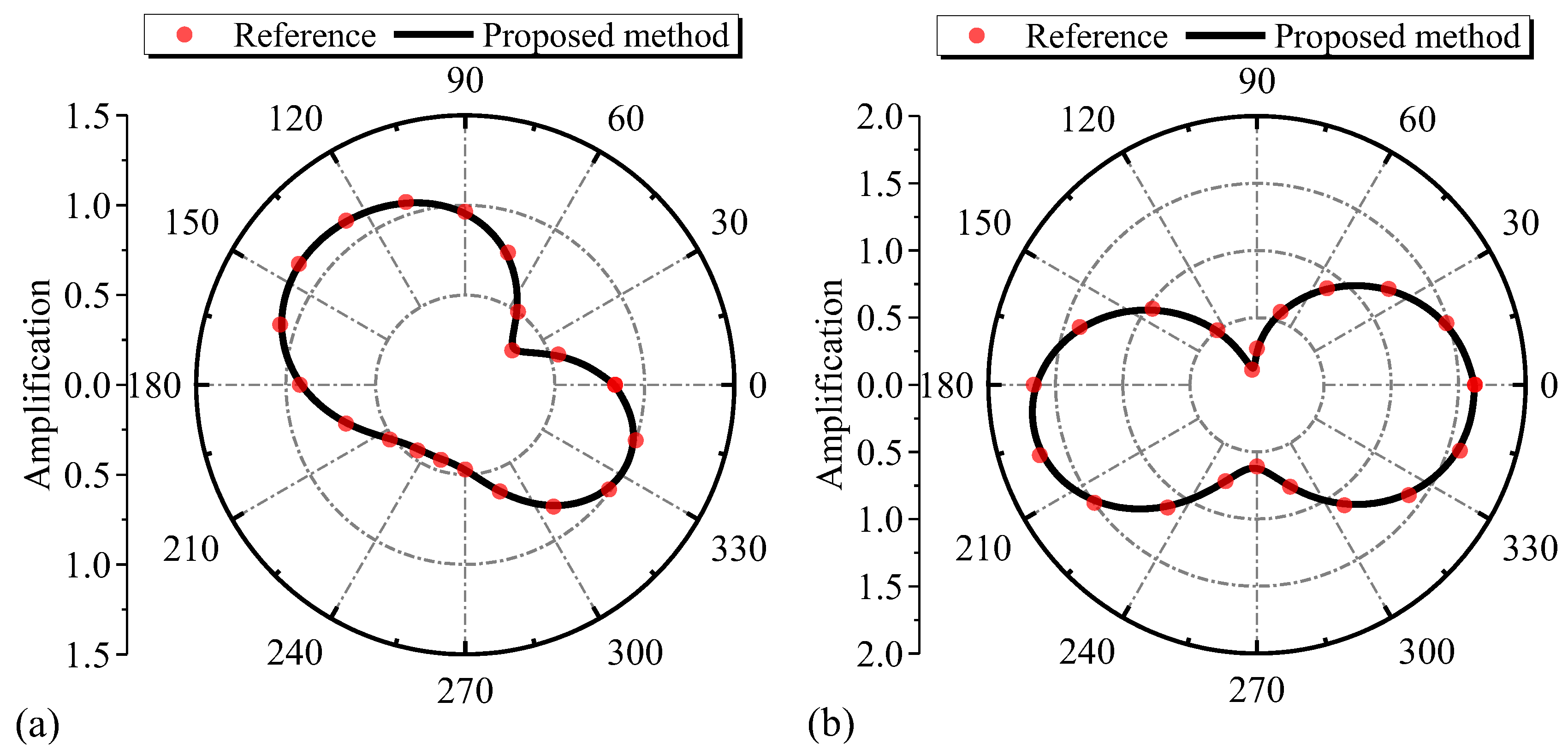
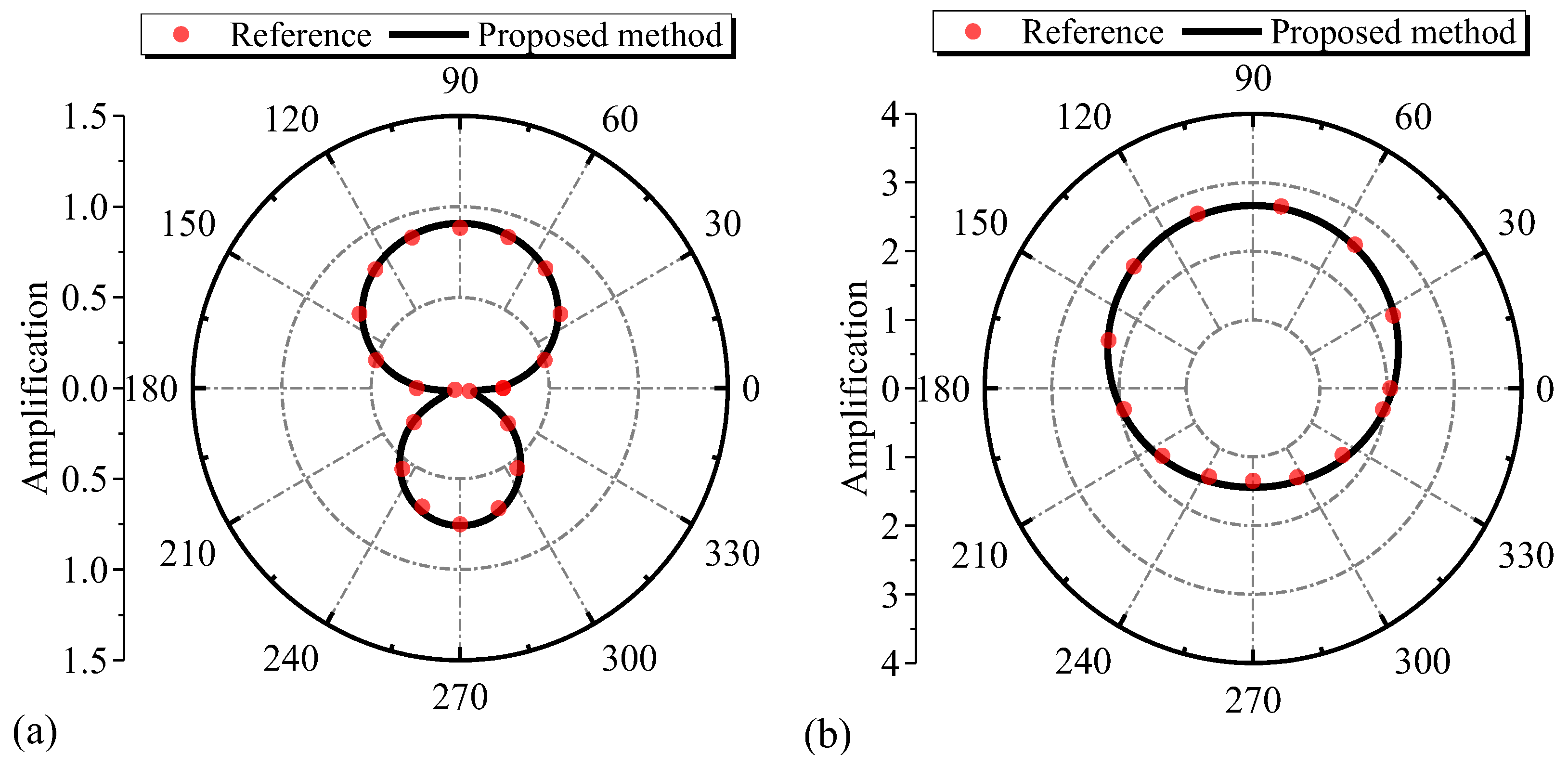
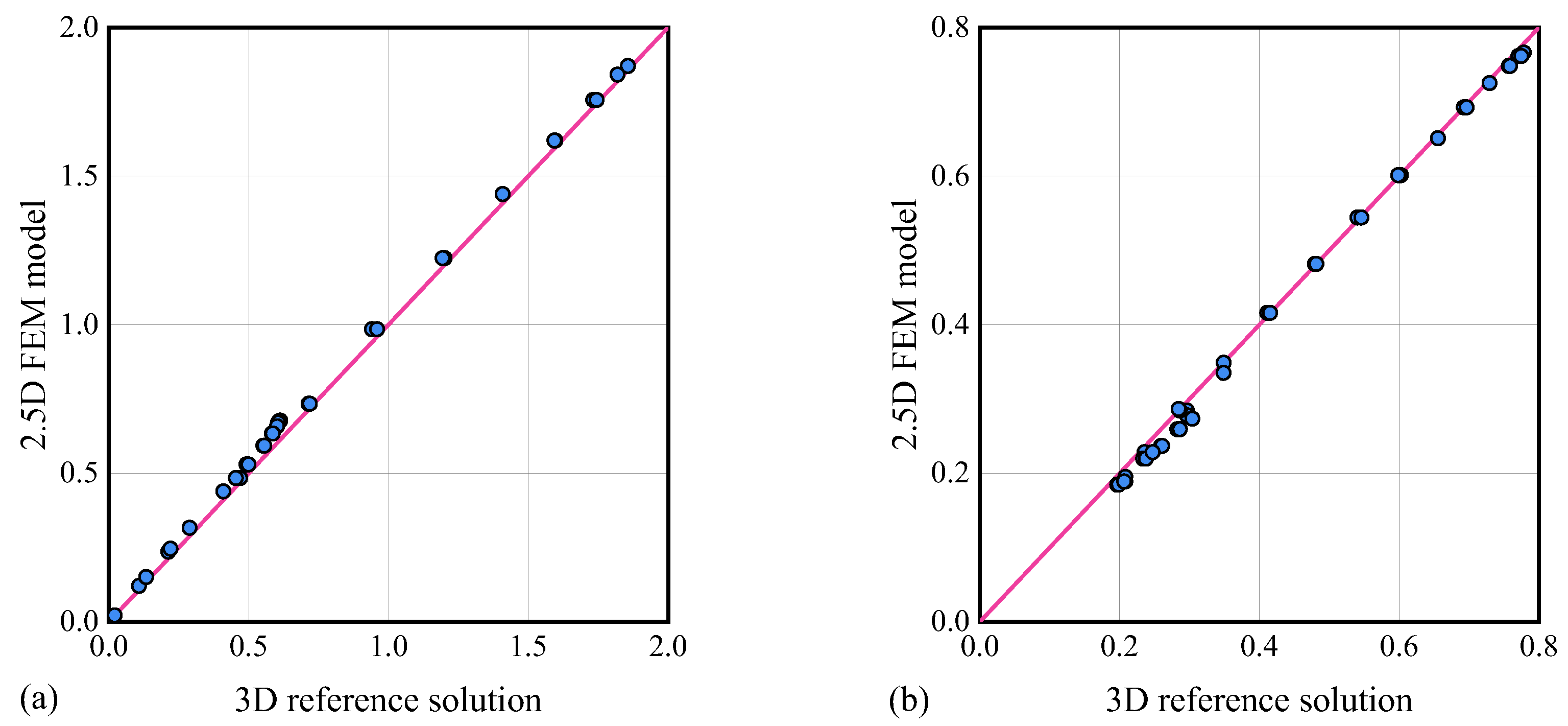
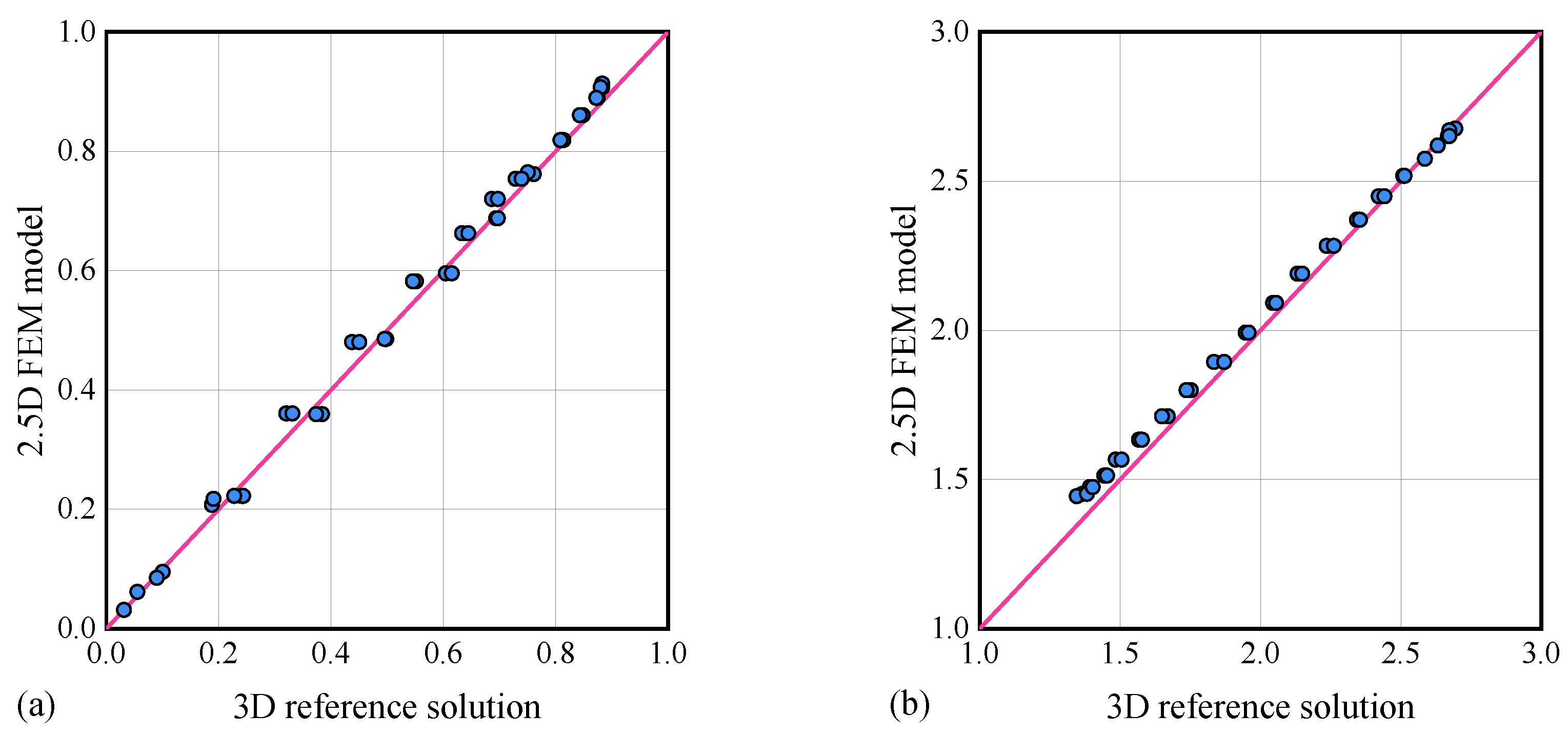
4.1. Oblique Incidence of Seismic Waves in the Plane
4.2. Incidence along the Longitudinal Direction
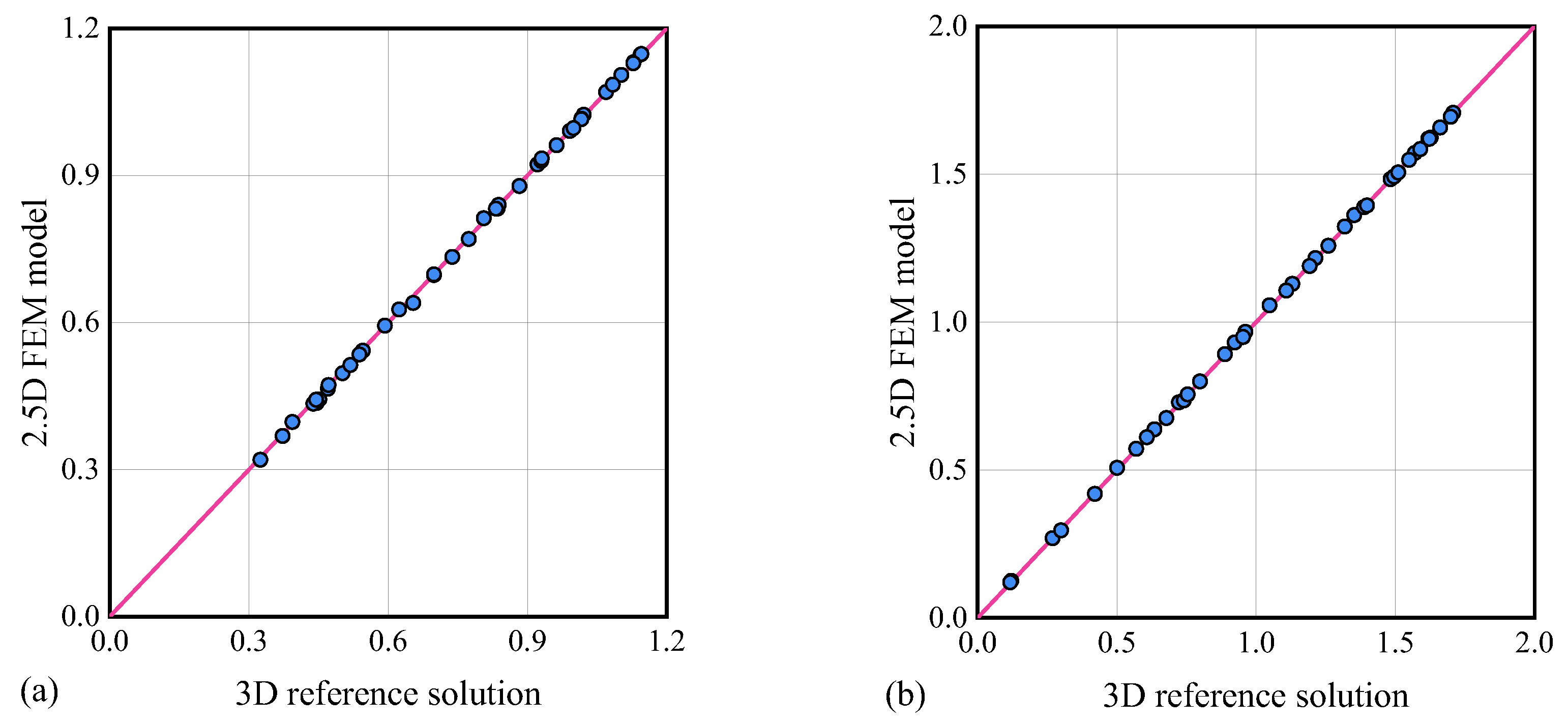
4.3. Accuracy of Longitudinal Responses
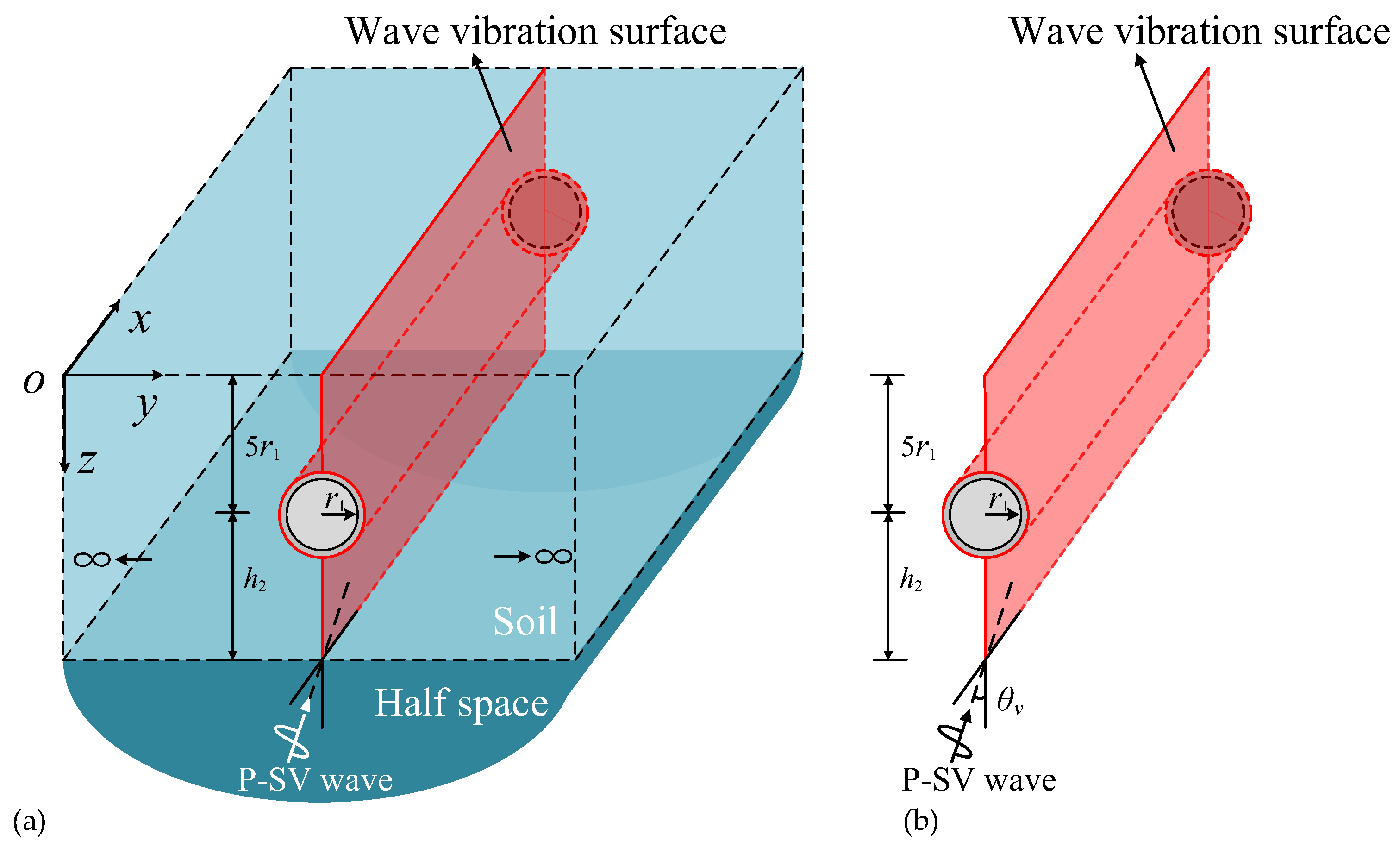
5. P-SV Wave Scattering of Long Lined Tunnel
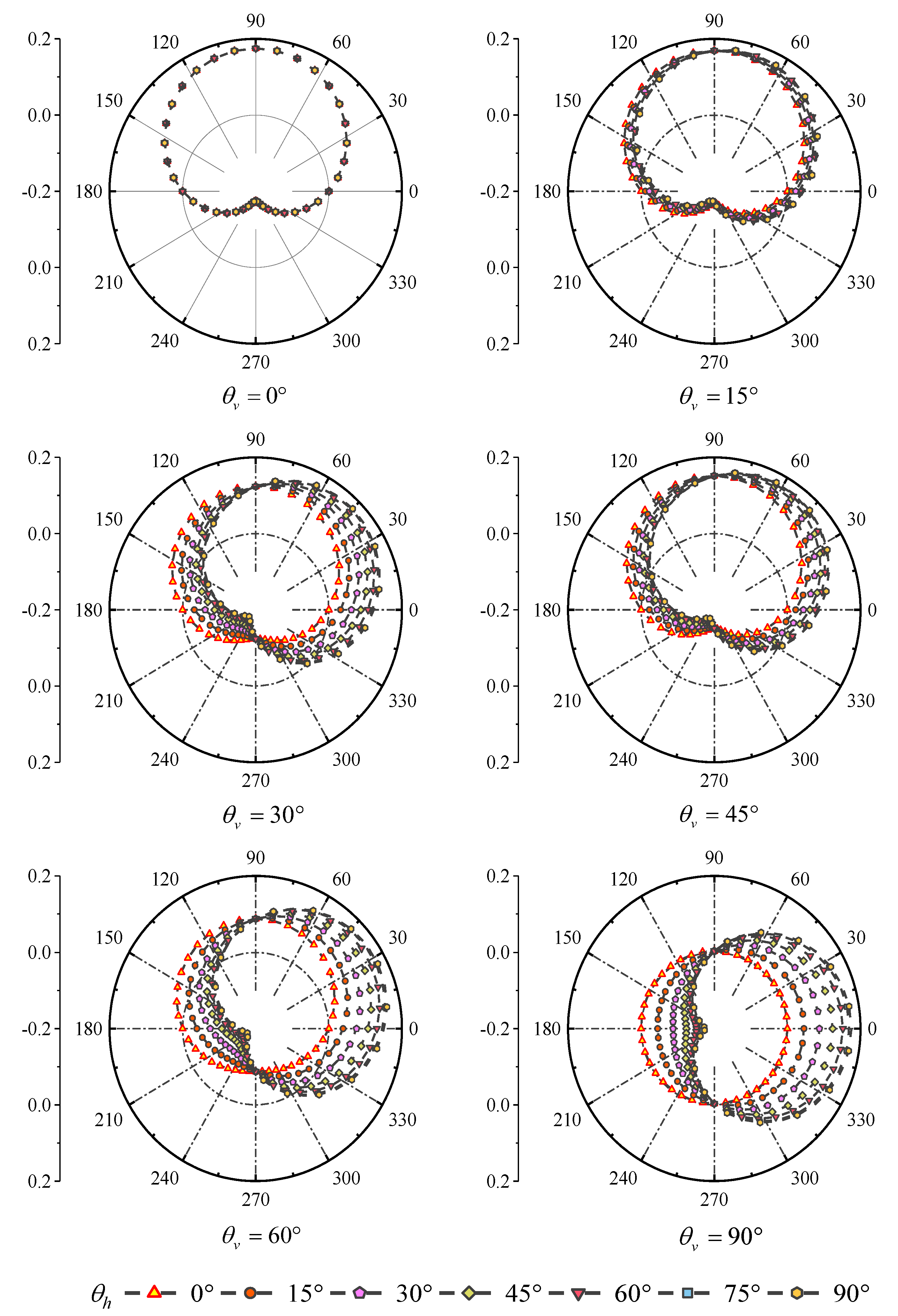
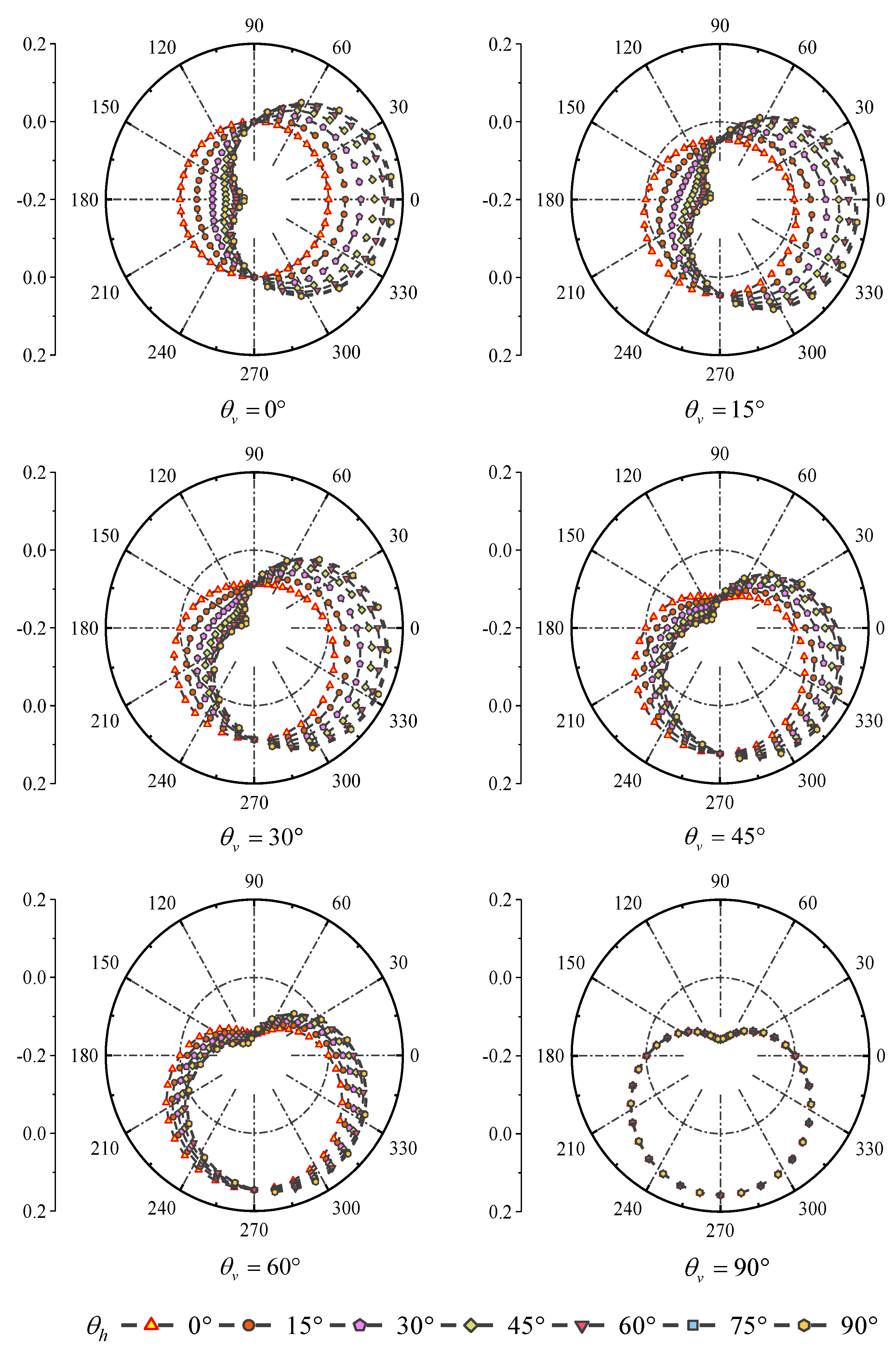
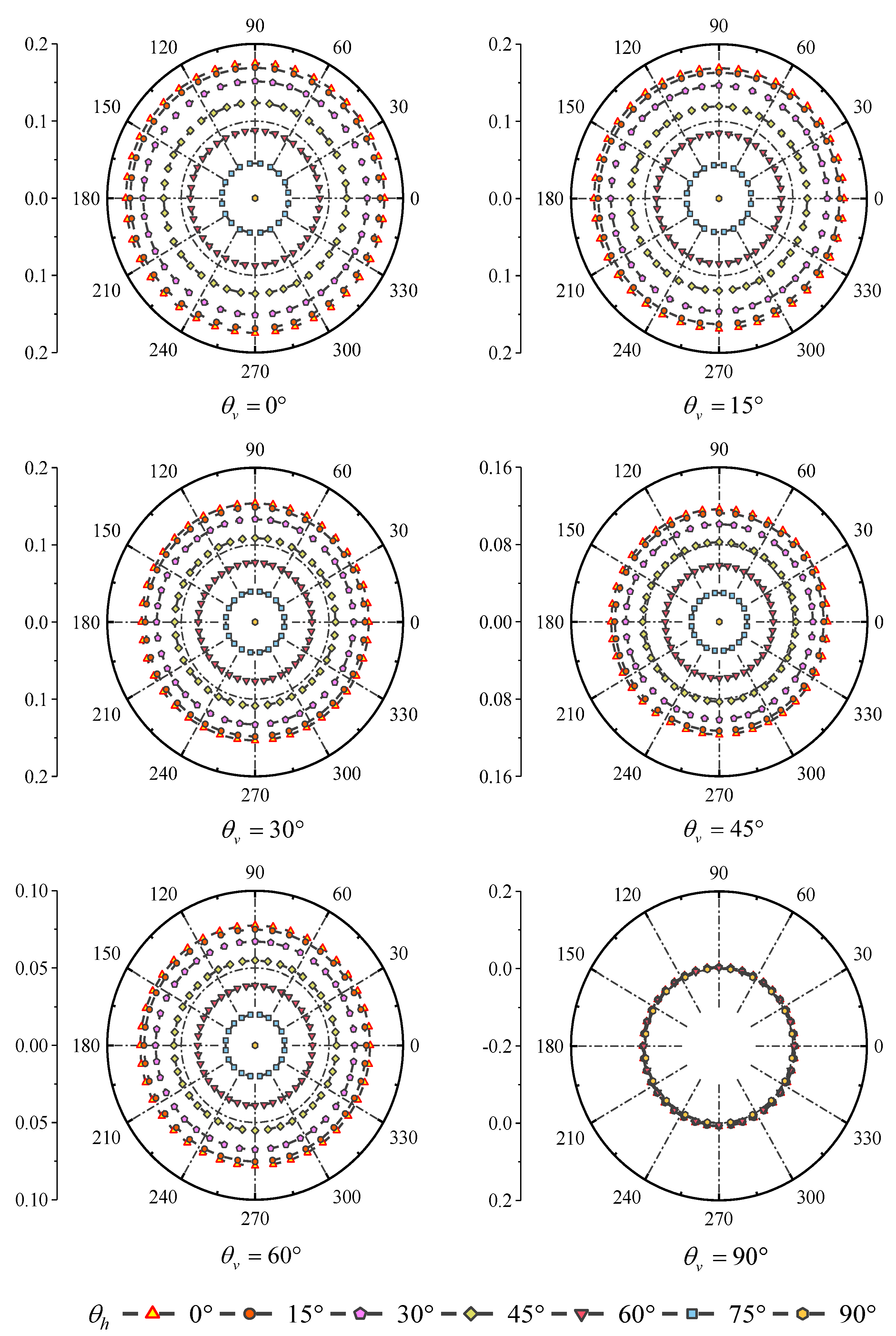
5.1. Influence of Seismic Wave Incidence Angle and Conversion Angle on Seismic Response of Tunnels
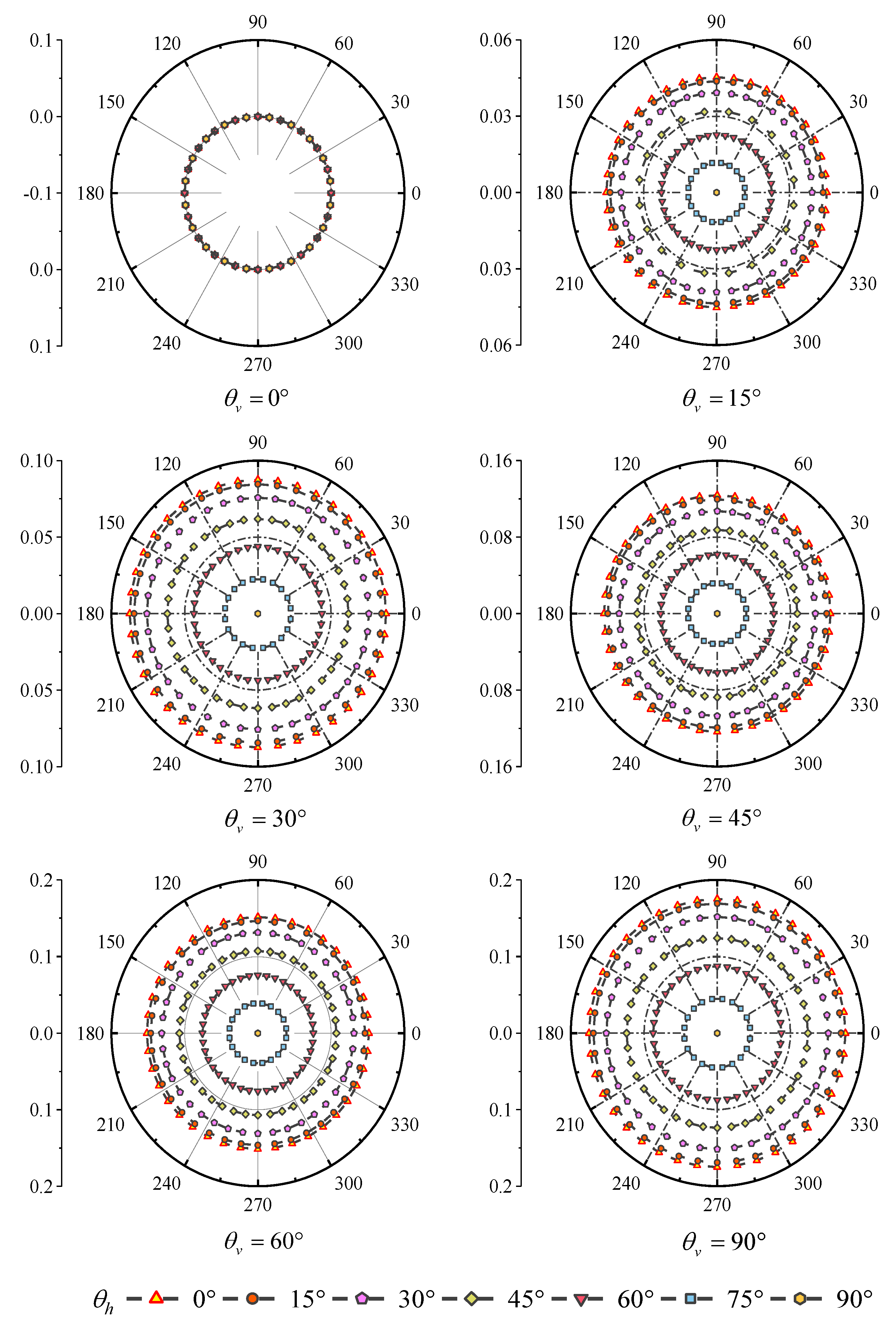
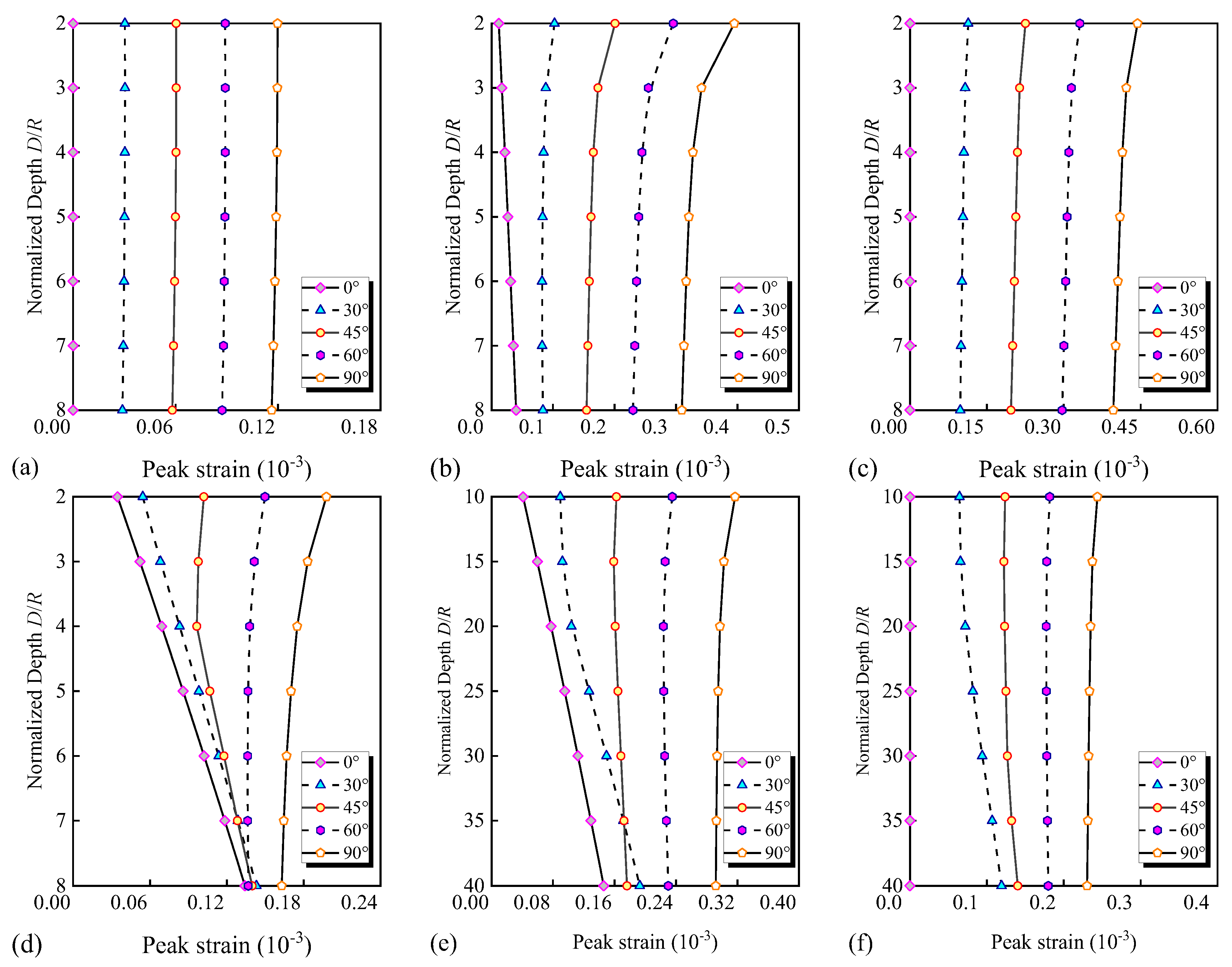
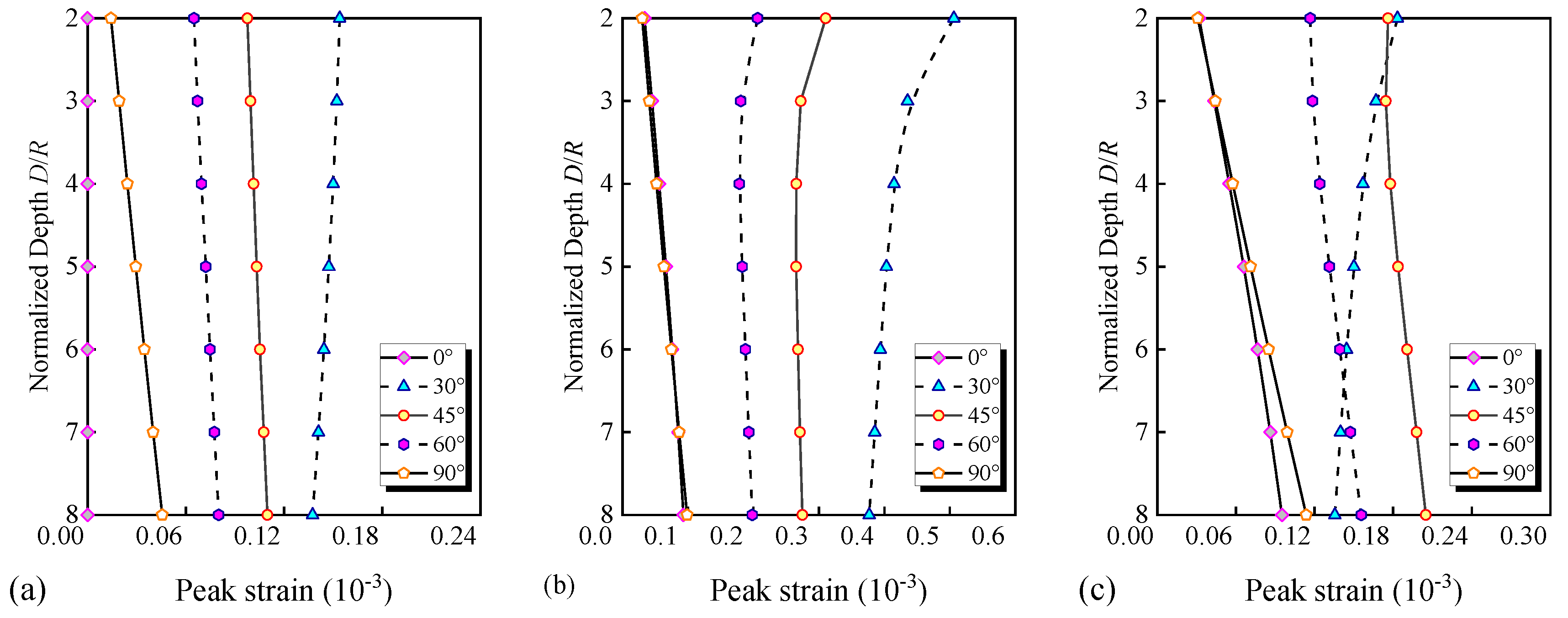
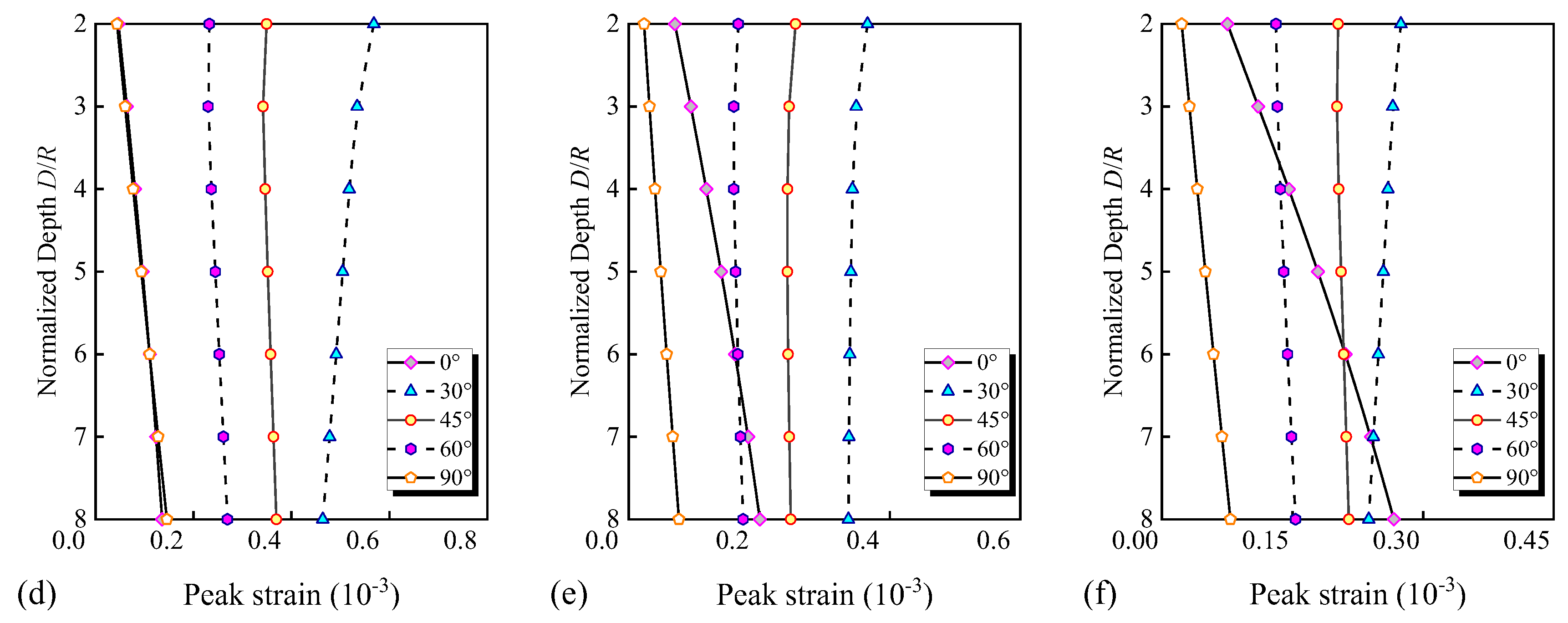
5.2. Influence of Buried Depths of Tunnels
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Iida, H.; Hiroto, T.; Yoshida, N.; Lwafuji, M. Damage to Daikai subway station. Soils Found 1996, 36, 283–300. [Google Scholar] [CrossRef] [PubMed]
- Stamo, A.A.; Beskos, D.E. Dynamic analysis of large 3-D underground structures by the BEM. Earthq. Eng. Struct. Dyn. 1995, 24, 917–934. [Google Scholar] [CrossRef]
- Stamos, A.A.; Beskos, D.E. 3-D seismic response analysis of long lined tunnels in half-space. Soil Dyn. Earthq. Eng. 1996, 15, 111–118. [Google Scholar] [CrossRef]
- Huo, H.; Bobet, A.; Fernández, G.; Fernandez, G.; Ramirez, J. Load transfer mechanisms between underground structure and surrounding ground: Evaluation of the failure of the Daikai station. J. Geotech. Geoenviron. Eng. 2005, 131, 1522–1533. [Google Scholar] [CrossRef]
- Hashash, Y.M.A.; Hook, J.J.; Schmidt, B.; Yao, J.I. Seismic design and analysis of underground structures. Tunn. Undergr. Space Technol. 2001, 16, 247–293. [Google Scholar] [CrossRef]
- Owen, G.N.; Scholl, R.E. Earthquake Engineering of Large Underground Structures; Federal Highway Administration: Washington, DC, USA, 1981. [Google Scholar]
- Yu, H.T.; Cai, C.; Yuan, Y.; Jia, M.C. Analytical solutions for Euler-Bernoulli Beam on Pasternak foundation subjected to arbitrary dynamic loads. Int. J. Numer. Anal. Methods Geomech. 2017, 41, 1125–1137. [Google Scholar] [CrossRef]
- John, C.; Zahrah, T.F. Aseismic design of underground structures. Tunn. Undergr. Space Technol. 1987, 2, 165–197. [Google Scholar] [CrossRef]
- Chen, J.; Shi, X.; Li, J. Shaking table test of utility tunnel under non-uniform earthquake wave excitation. Soil Dyn. Earthq. Eng. 2010, 30, 1400–1416. [Google Scholar] [CrossRef]
- Yu, H.T.; Yuan, Y.; Qiao, Z.Z.; Gu, Y.; Yang, Z.H.; Li, X.D. Seismic analysis of a long tunnel based on multi664 scale method. Eng. Struct. 2013, 49, 572–587. [Google Scholar] [CrossRef]
- Li, P.; Song, E.X. Three-dimensional numerical analysis for the longitudinal seismic response of tunnels under an asynchronous wave input. Comput. Geotech. 2015, 63, 229–243. [Google Scholar] [CrossRef]
- Fabozzi, S.; Bilotta, E.; Yu, H.T.; Yuan, Y. Effects of the asynchronism of ground motion on the longitudinal behaviour of a circular tunnel. Tunn. Undergr. Space Technol. 2018, 82, 529–541. [Google Scholar] [CrossRef]
- Huang, J.Q.; Du, X.L.; Jin, L.; Zhao, M. Impact of incident angles of P waves on the dynamic responses of long lined tunnels. Earthq. Eng. Struct. Dyn. 2016, 45, 2435–2454. [Google Scholar] [CrossRef]
- Gao, Z.D.; Zhao, M.; Huang, J.Q.; Du, X.L. Three-dimensional nonlinear seismic response analysis of subway station crossing longitudinally inhomogeneous geology under obliquely incident P waves. Eng. Geol. 2021, 293, 106341. [Google Scholar] [CrossRef]
- Miao, Y.; Yao, E.L.; Ruan, B.; Zhuang, H.Y. Seismic response of shield tunnel subjected to spatially varying earthquake ground motions. Tunn. Undergr. Space Technol. 2018, 77, 216–226. [Google Scholar] [CrossRef]
- Hwang, R.; Lysmer, J. Response of buried structures to traveling waves. J. Geotech. Geoenviron. 1981, 107, 183–200. [Google Scholar] [CrossRef]
- Yang, Y.B.; Hung, H.H. A 2.5D finite/infinite element approach for modelling viscoelastic bodies subjected to moving loads. Int. Int. J. Numer. Anal. Methods Geomech. 2001, 51, 1317–1336. [Google Scholar] [CrossRef]
- Alves, C.P.; Calçada, R.; Silva, C.A.; Bodare, A. Influence of soil non-linearity on the dynamic response of high-speed railway tracks. Soil Dyn. Earthq. Eng. 2010, 30, 221–235. [Google Scholar] [CrossRef]
- Sheng, X.; Jones, C.J.C.; Thompson, D.J. Prediction of ground vibration from trains using the wavenumber finite and boundary element methods. J. Sound Vibr. 2006, 293, 575–586. [Google Scholar] [CrossRef]
- François, S.; Schevenels, M.; Galvín, P.; Lombaert, G.; Degrande, G. A 2.5D coupled FE-BE methodology for the dynamic interaction between longitudinally invariant structures and a layered halfspace. Comput. Meth. Appl. Mech. Eng. 2010, 199, 1536–1548. [Google Scholar] [CrossRef]
- He, C.; Zhou, S.; Di, H.; Shan, Y. A 2.5-D coupled FE-BE model for the dynamic interaction between saturated soil and longitudinally invariant structures. Comput. Geotech. 2017, 82, 211–222. [Google Scholar] [CrossRef]
- Jin, Q.; Thompson, D.J.; Lurcock, D.; Toward, M.; Ntotsios, E. A 2.5D finite element and boundary element model for the ground vibration from trains in tunnels and validation using measurement data. J. Sound Vibr. 2018, 422, 373–389. [Google Scholar] [CrossRef]
- Lopes, P.; Alves Costa, P.; Calçada, R.; Silva Cardoso, A.; Costa, P.A.; Calçada, R.; Cardoso, A.S. Influence of soil stiffness on building vibrations due to railway traffic in tunnels: Numerical study. Comput. Geotech. 2014, 61, 277–291. [Google Scholar] [CrossRef]
- Rieckh, G.; Kreuzer, W.; Waubke, H.; Balazs, P. A 2.5D-Fourier-BEM model for vibrations in a tunnel running through layered anisotropic soil. Eng. Anal. Bound. Elem. 2012, 36, 960–967. [Google Scholar] [CrossRef]
- Liravi, H.; Arcos, R.; Clot, A.; Conto, F.; Romeu, J. A 2.5D coupled FEM–SBM methodology for soil–structure dynamic interaction problems. Eng. Struct. 2021, 250, 113371. [Google Scholar] [CrossRef]
- Lin, K.C.; Hung, H.H.; Yang, J.P.; Yang, Y.B. Seismic analysis of underground tunnels by the 2.5D finite/infinite element approach. Soil Dyn. Earthq. Eng. 2016, 85, 31–43. [Google Scholar] [CrossRef]
- Zhu, J.; Liang, J.W.; Ba, Z.N. A 2.5D equivalent linear model for longitudinal seismic analysis of tunnels in water-saturated poroelastic half-space. Comput. Geotech. 2019, 109, 166–188. [Google Scholar] [CrossRef]
- Zhu, J.; Li, X.J.; Liang, J.W. 2.5D FE-BE modelling of dynamic responses of segmented tunnels subjected to obliquely incident seismic waves. Soil Dyn. Earthq. Eng. 2022, 163, 107564. [Google Scholar] [CrossRef]
- Zhu, J.; Li, X.; Liang, J. 3D seismic responses of a long lined tunnel in layered poro-viscoelastic half-space by a hybrid FE-BE method. Eng. Anal. Bound. Elem. 2020, 114, 94–113. [Google Scholar] [CrossRef]
- Du, X.L.; Zhao, M. Stability and identiffcation for rational approximation of frequency response function of unbounded soil. Earthq. Eng. Struct. Dyn. 2010, 39, 165–186. [Google Scholar]
- Zhao, M.; Wu, L.H.; Du, X.L.; Zhong, Z.L.; Xu, C.S.; Li, L. Stable high-order absorbing boundary condition based on new continued fraction for scalar wave propagation in unbounded multilayer media. Comput. Meth. Appl. Mech. Eng. 2018, 334, 111–137. [Google Scholar] [CrossRef]
- Mossessian, T.K.; Dravinski, M. Application of a hybrid method for scattering of P, SV, and Rayleigh waves by near-surface irregularities. Bull. Seismol. Soc. Amer. 1987, 77, 1784–1803. [Google Scholar]
- Mojtabazadeh-Hasanlouei, S.; Panji, M.; Kamalian, M. Attenuated orthotropic time-domain half-space BEM for SH-wave scattering problems. Geophys. J. Int. 2022, 229, 1881–1913. [Google Scholar] [CrossRef]
- Panji, M.; Mojtabazadeh-Hasanlouei, S. On subsurface box-shaped lined tunnel under incident SH-wave propagation. Front. Struct. Civ. Eng. 2021, 15, 948–960. [Google Scholar] [CrossRef]
- Panji, M.; Mojtabazadeh Hasanlouei, S. Time-history response on the surface by regularly distributed enormous embedded cavity: Incident SH-waves. Earthq. Sci. 2018, 31, 137–153. [Google Scholar] [CrossRef]
- Shah, A.H.; Wong, K.C.; Datta, S.K. Diffraction of plane SH waves in a half-space. Earthq. Eng. Struct. Dyn. 1982, 10, 519–528. [Google Scholar] [CrossRef]
- Park, S.H.; Tassoulas, J.L. Time-harmonic analysis of wave propagation in unbounded layered strata with zigzag boundaries. J. Eng. Mech. 2002, 128, 359–368. [Google Scholar] [CrossRef]
- Lysmer, J. Lumped mass method for Rayleigh waves. Bull. Seismol. Soc. Amer. 1970, 60, 89–104. [Google Scholar] [CrossRef]
- Sun, L.; Pan, Y.; Gu, W. High-order thin layer method for viscoelastic wave propagation in stratified media. Comput. Meth. Appl. Mech. Eng. 2013, 257, 65–76. [Google Scholar] [CrossRef]
- Lin, G.; Li, Z.; Li, J. A substructure replacement technique for the numerical solution of wave scattering problem. Soil Dyn. Earthq. Eng. 2018, 111, 87–97. [Google Scholar] [CrossRef]
- Zhang, G.L.; Zhao, M.; Huang, J.Q.; Du, X.L.; Zhao, X. A substructure method for underground structure dry soil-saturated soil-bedrock interaction under obliquely incident earthquake and its application to groundwater effect on tunnel. Tunn. Undergr. Space Technol. 2021, 111, 103864. [Google Scholar] [CrossRef]
- Kausel, E.; Roesset, M.J. Stiffness matrices for layered soils. Bull. Seismol. Soc. Amer. 1981, 71, 1743–1761. [Google Scholar] [CrossRef]
- Andrade, P. Implementation of Second-Order Absorbing Boundary Conditions in Frequency Domain Computations; University of Texas at Austin: Austin, TX, USA, 1999. [Google Scholar]
- Zhang, G.L.; Zhao, M.; Du, X.L.; Zhang, X.L.; Wang, P.G. 1D finite element artificial boundary method for transient response of ocean site under obliquely incident earthquake waves. Soil Dyn. Earthq. Eng. 2019, 126, 105787. [Google Scholar] [CrossRef]
- Contento, A.; Aloisio, A.; Xue, J.; Quaranta, G.; Briseghella, B.; Gardoni, P. Probabilistic axial capacity model for concrete-filled steel tubes accounting for load eccentricity and debonding. Eng. Struct. 2022, 268, 114730. [Google Scholar] [CrossRef]
- Debarros, F.C.P.; Luco, J.E. Seismic response of a cylindrical shell embedded in a layered viscoelastic half-space. 2. Validation and numerical results. Earthq. Eng. Struct. Dyn. 1994, 23, 569–580. [Google Scholar] [CrossRef]




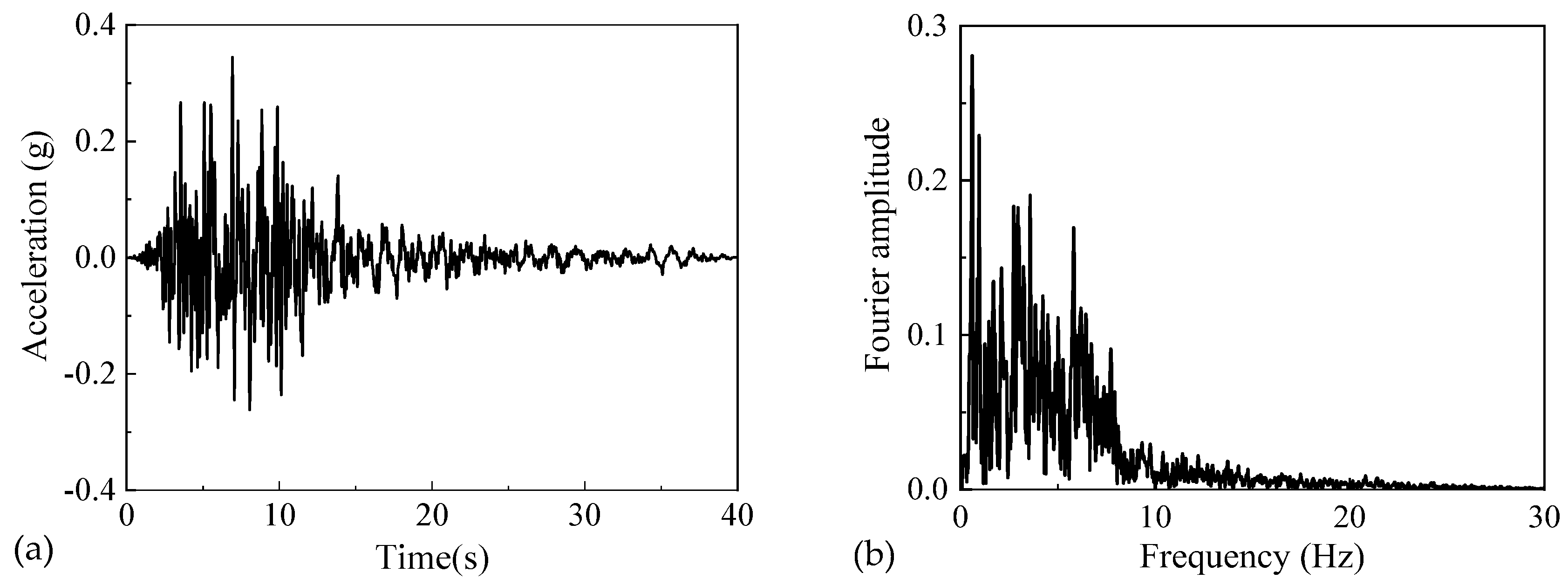


| Soil | The First Lamé Constant λ1 (GPa) | The Second Lamé Constant G1 (GPa) | Density ρ1 (kg/m3) |
|---|---|---|---|
| Lining | 4.44 | 6.67 | 2240 |
| Rock | 2.14 | 0.24 | 2665 |
| Verification | U2.5D/U3D | |||
|---|---|---|---|---|
| R2 | Maximum Error | Mean Error | Standard Deviation | |
| P wave Ur − 1 | 0.9998 | 0.0240 | 0.0057 | 0.0075 |
| SV wave Ur − 1 | 0.9999 | 0.0317 | 0.0049 | 0.0064 |
| Soil | Elastic Modulus E1 (GN/m3) | Poisson’s Ratio ν1 | Density ρ1 (kg/m3) |
|---|---|---|---|
| Lining | 16 | 0.2 | 2240 |
| Rock | 7.567 | 0.333 | 2664 |
| Verification | U2.5D/U3D | |||
|---|---|---|---|---|
| R2 | Maximum Error | Mean Error | Standard Deviation | |
| P wave Ur – 2 | 0.9990 | 0.1255 | 0.0610 | 0.0386 |
| SV wave Ur – 2 | 0.9986 | 0.1011 | 0.0347 | 0.0359 |
| P wave Ul | 0.9954 | 0.1406 | 0.0440 | 0.0360 |
| SV wave Ul | 0.9993 | 0.0730 | 0.0244 | 0.0197 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Q.; Zhao, M.; Huang, J.; Du, X.; Zhang, G. A 2.5D Finite Element Method Combined with Zigzag-Paraxial Boundary for Long Tunnel under Obliquely Incident Seismic Wave. Appl. Sci. 2023, 13, 5743. https://doi.org/10.3390/app13095743
Zhang Q, Zhao M, Huang J, Du X, Zhang G. A 2.5D Finite Element Method Combined with Zigzag-Paraxial Boundary for Long Tunnel under Obliquely Incident Seismic Wave. Applied Sciences. 2023; 13(9):5743. https://doi.org/10.3390/app13095743
Chicago/Turabian StyleZhang, Qi, Mi Zhao, Jingqi Huang, Xiuli Du, and Guoliang Zhang. 2023. "A 2.5D Finite Element Method Combined with Zigzag-Paraxial Boundary for Long Tunnel under Obliquely Incident Seismic Wave" Applied Sciences 13, no. 9: 5743. https://doi.org/10.3390/app13095743






