Evaluation of Non-Planar Tool Interaction in Milling of Shaped Surfaces Using a Copy Milling Cutter
Abstract
:1. Introduction
2. Materials and Methods
- Roughing: face cylindrical cutter D18 mm with two interchangeable cutters plates marked APXT11T3PDR-MA, axial depth of cut ap = 1 mm, radial depth of cut ae = 0.6 mm, tool path tolerance T = 0.1 mm, surface allowance P = 0.5 mm
- Semi-finishing—ball nose end mill D6 mm, cutting material solid carbide, number of teeth 2, coating AlTiN, tool geometry λ = 30°, γ = 12°, machining strategy—linear, axial depth of cut ap = 0.5 mm, radial depth of cut ae = 0.5 mm, surface allowance P = 0.2 mm
- Finishing—ball nose end mill D4 mm, cutting material solid carbide, number of teeth 2, coating AlTiN, tool geometry λ = 30°, γ = 12°, machining strategy—constant Z, radial depth of cut ae = 0.2 mm, tool path tolerance T = 0.01 mm
- Comparison of machined surfaces between CAM system and real production
- Evaluation of the effective diameter of the tool Deff with respect to the contact of the tool and the workpiece
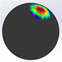
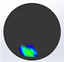
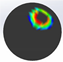
- Evaluation of tool surface area distribution using areal content and volume data extraction at the contact patch location
- Assessment of surface deviations by the 3D scanning method—scanner FARO Laser ScanArm V3 (FARO Technologies Italy S.r.l., Rezzato, Italy)
2.1. Methodology for Evaluating the Effective Diameter of the Tool with Regard to the Contact between the Tool and the Workpiece
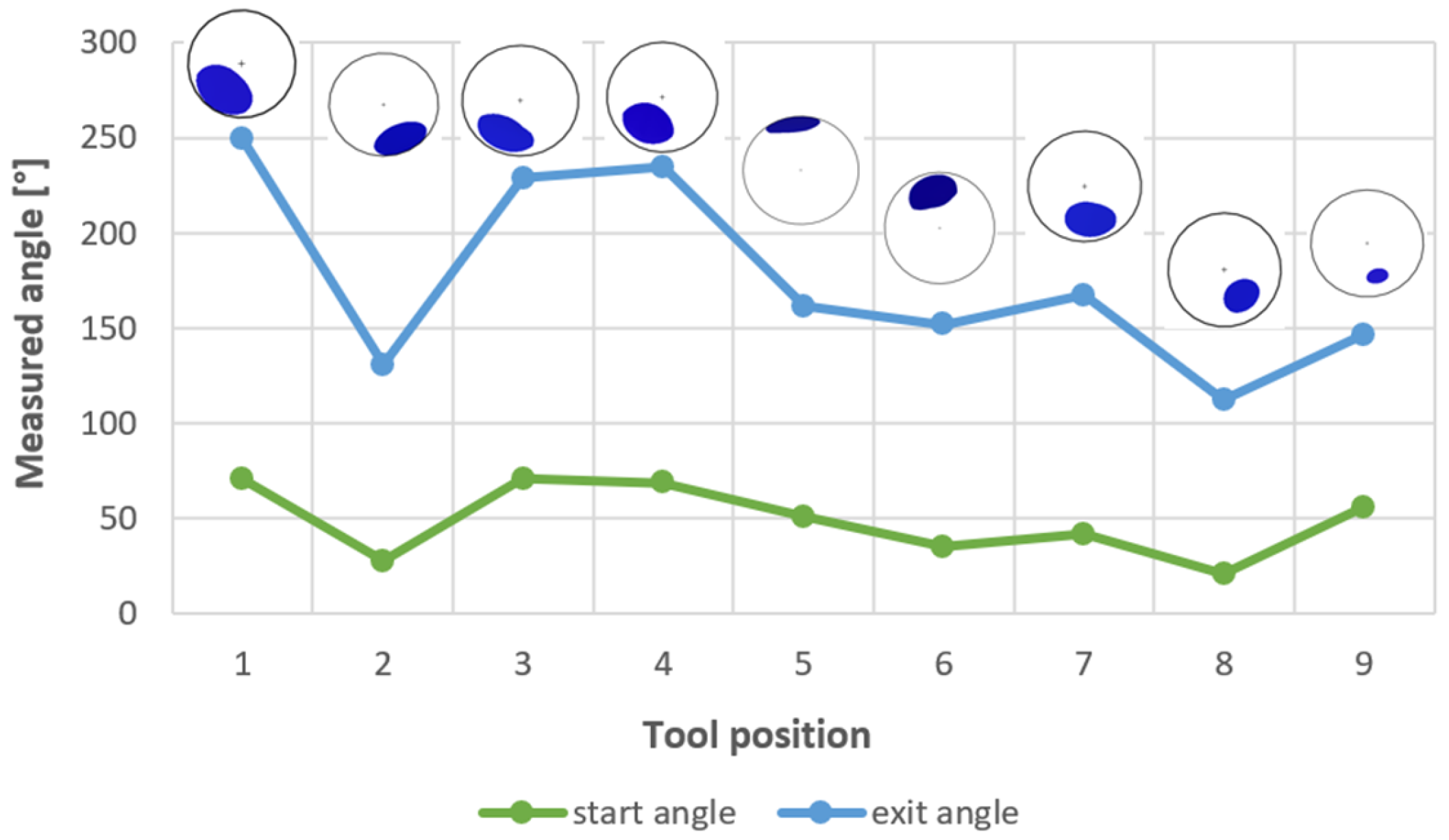
2.2. Methodology for Assessing the Distribution of the Engagement Area on the Tool Surface
2.3. Methodology for Evaluating Surface Deviations Using the Scanning Method
3. Results
3.1. Comparison of Machined Surfaces between CAM System and Real Production
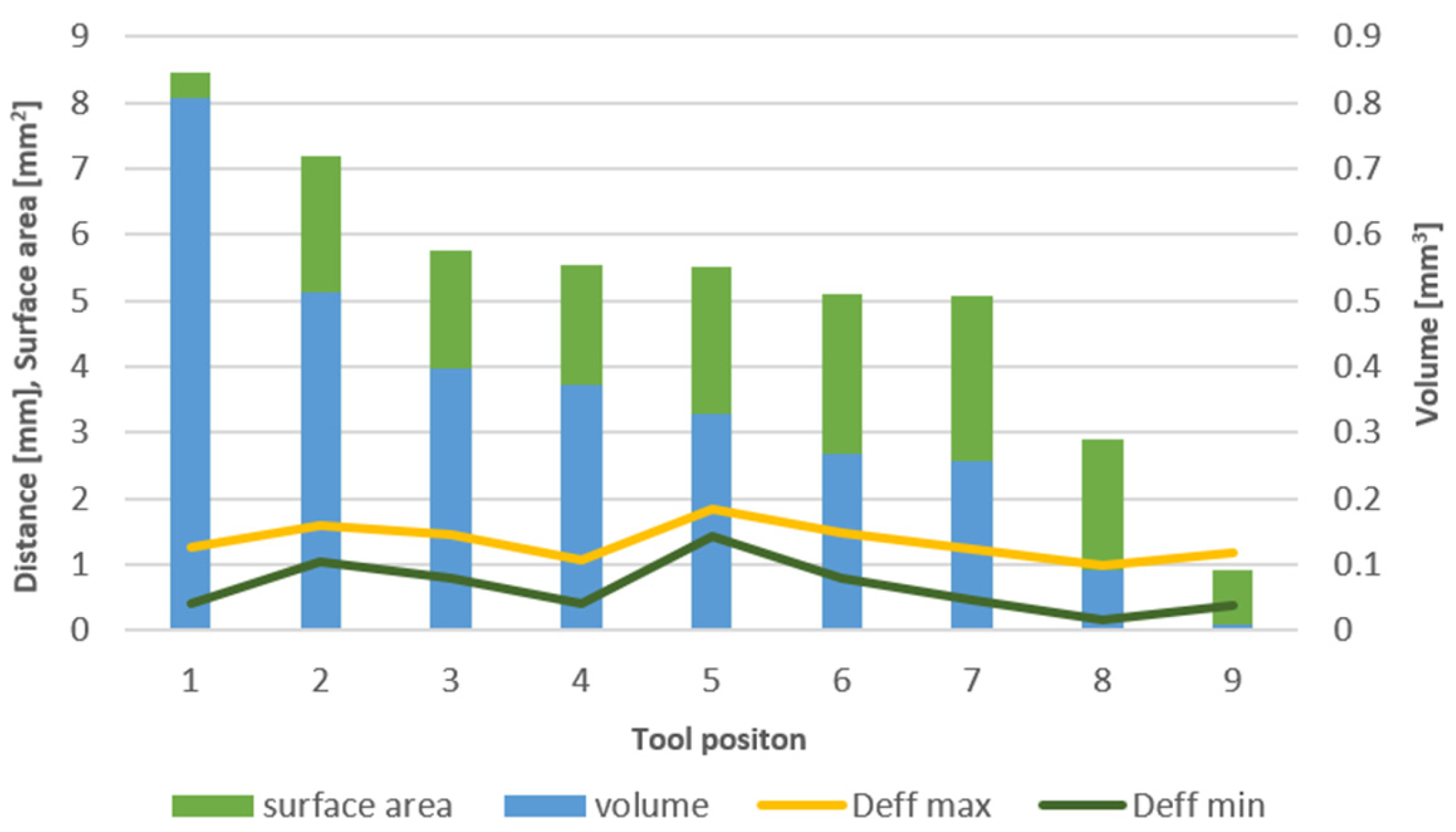
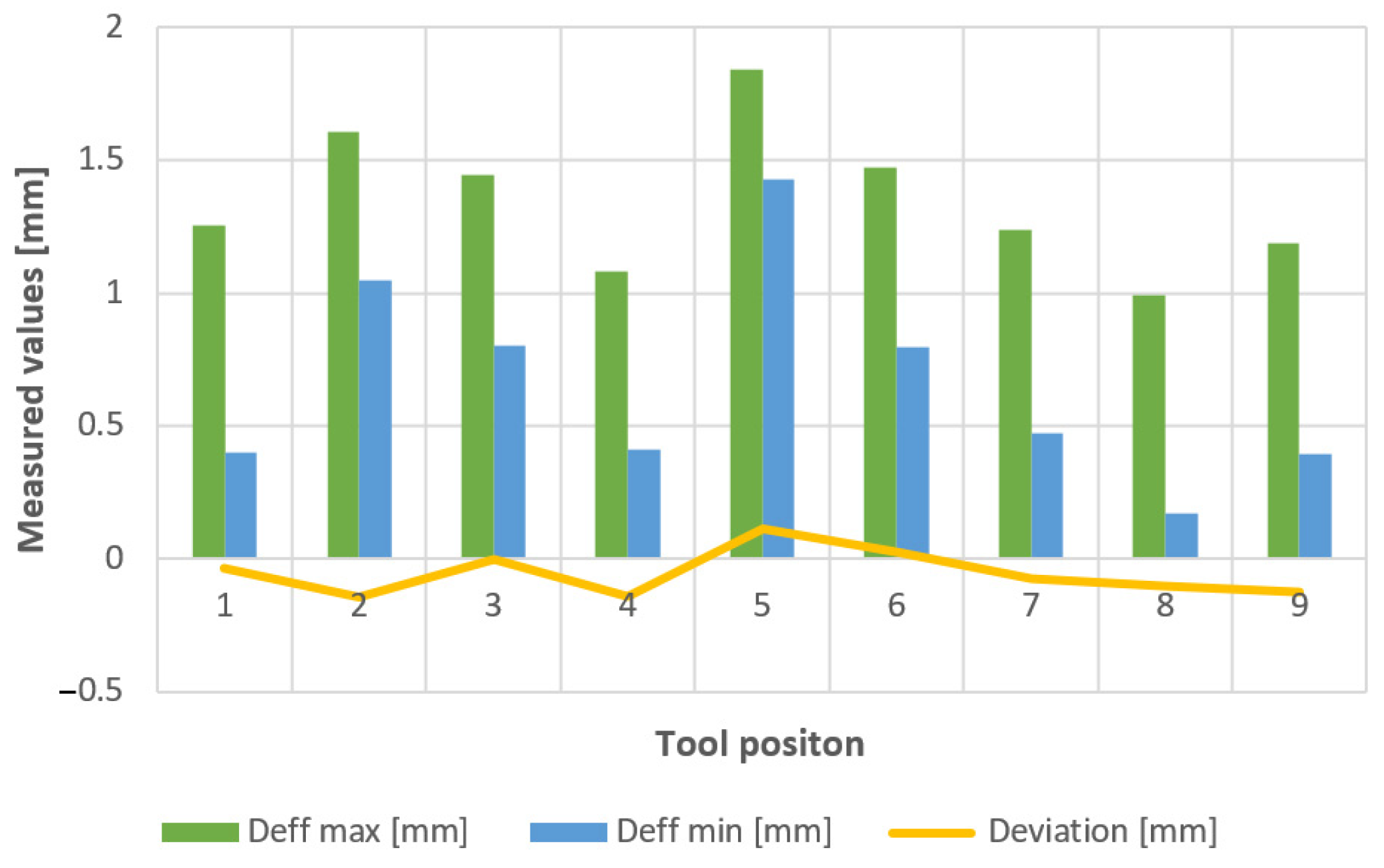
3.2. Evaluation of the Effective Diameter of the Tool Deff with Respect to the Contact of the Tool and the Workpiece
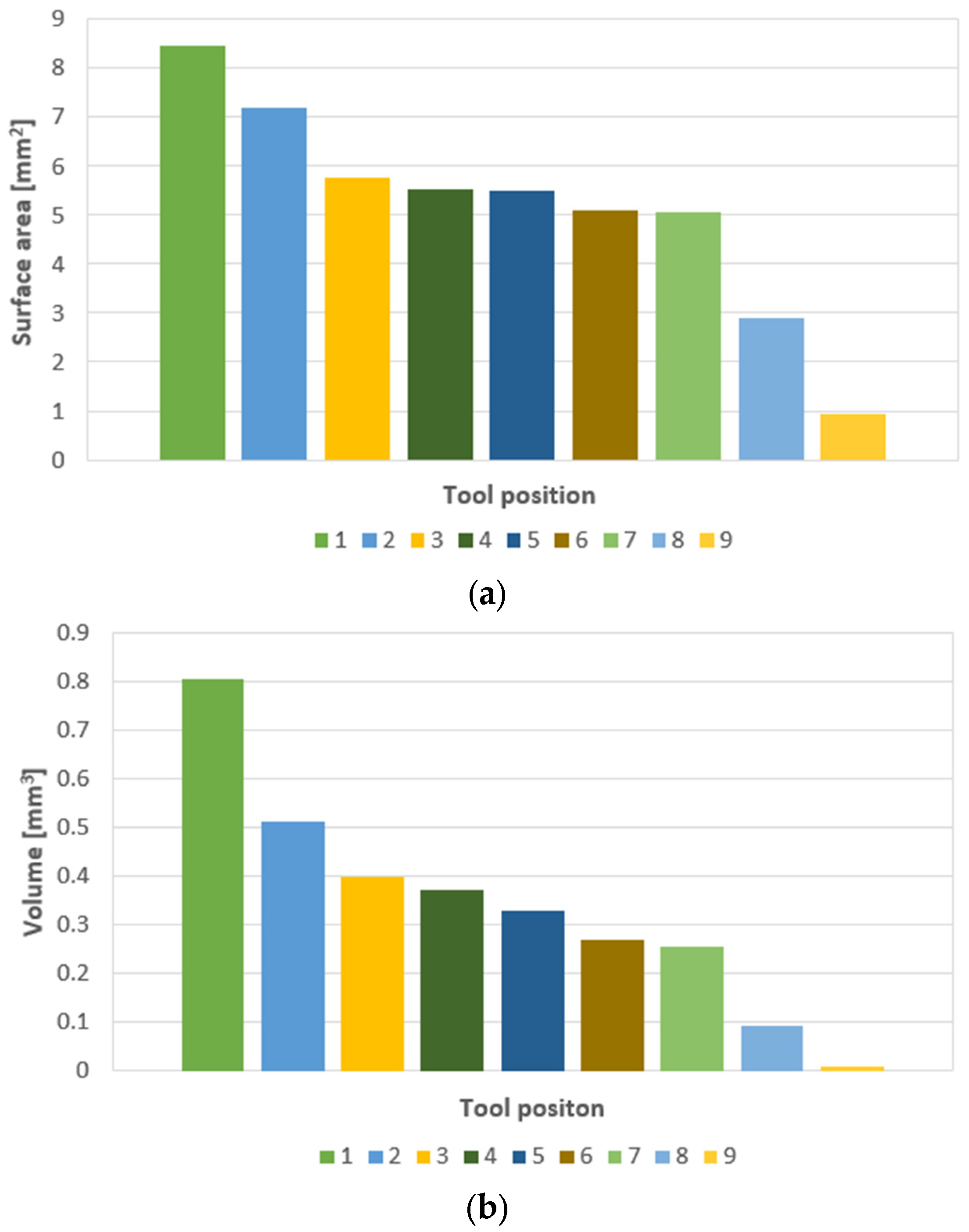
3.3. Evaluating Tool Surface Area Distribution Using Data Extraction
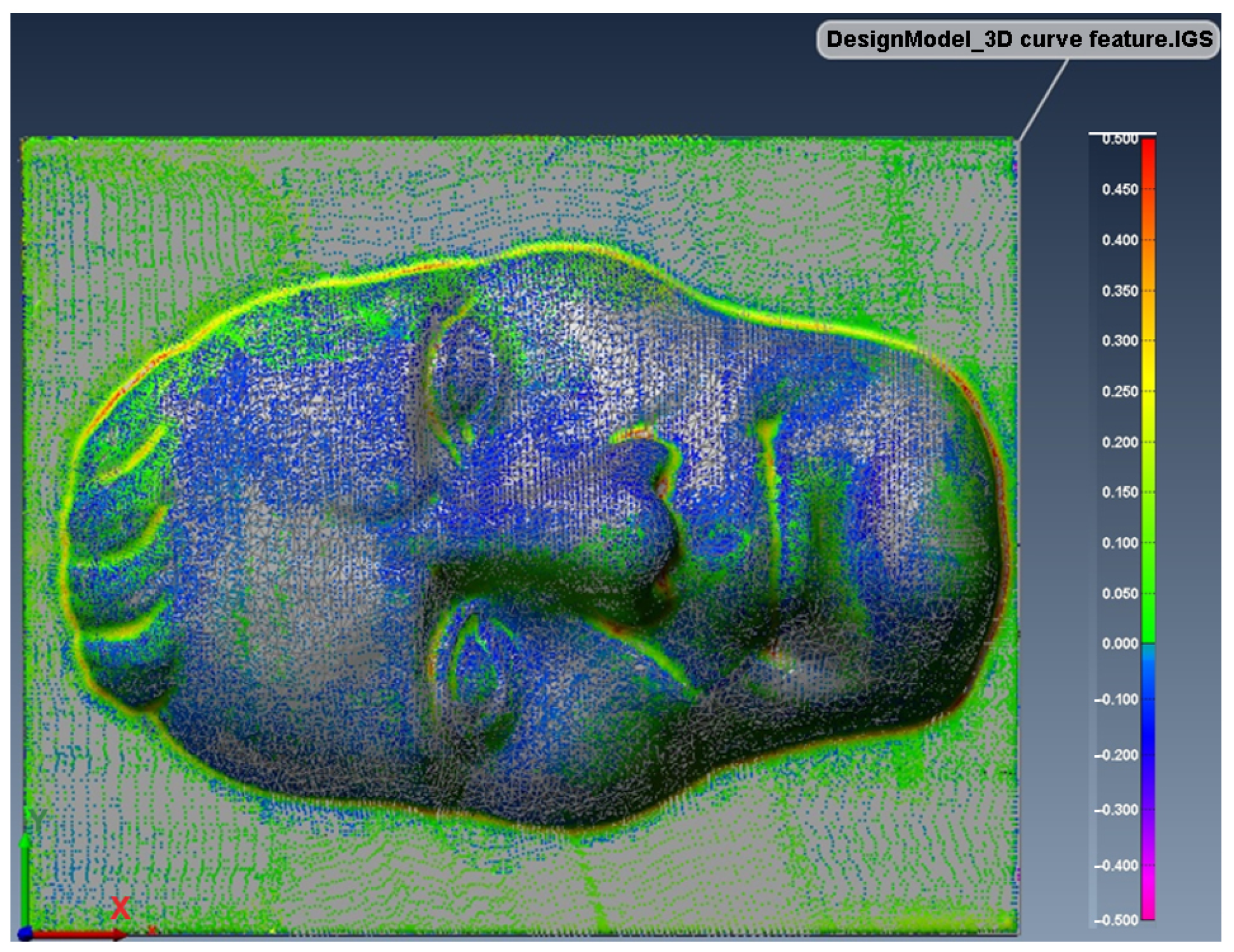
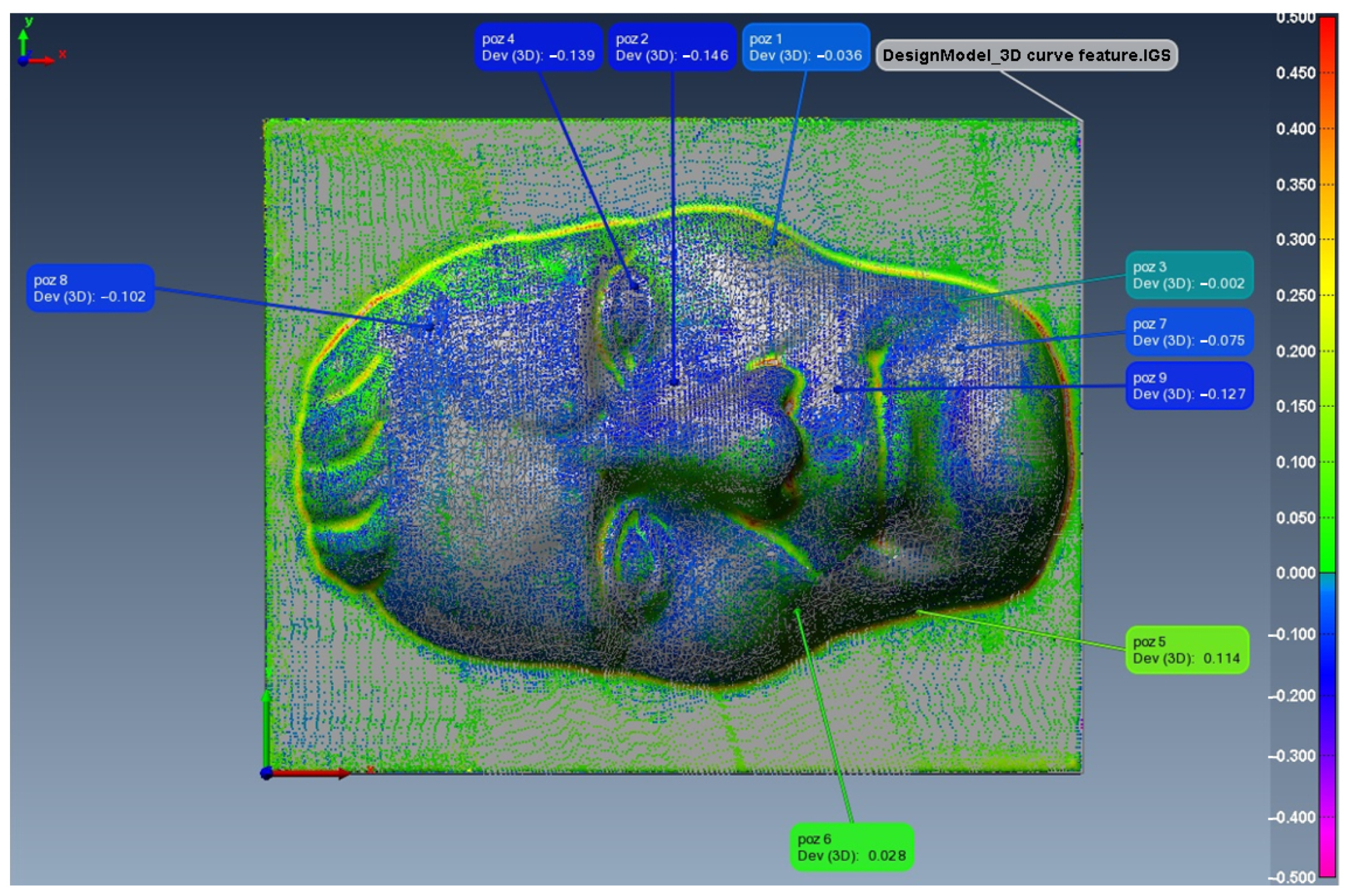
3.4. Evaluation of Surface Deviations by the 3D Scanning Method
4. Discussion
5. Conclusions
- -
- Analysis of the impact and efficiency of the machining process due to the change in the milling method (downward and upward milling).
- -
- The effect of tilting the tool on the wear of the cutting edge during the machining of shaped surfaces with the support of cutting force measurement.
- -
- Decomposition of shaped surfaces focused on deeper knowledge of the impact of milling strategies on surface topography.
- -
- Comparison of the effectiveness of the length of the tool extension in processing shaped surfaces, where the stiffness, cutting forces, and deflection of the tool in contact with the workpiece would be evaluated.
- -
- Comparison of high-speed milling strategies known as HSM with conventional milling strategies.
- -
- A certain shortcoming is the lack of comparison of 3- and 5-axis machining. For this reason, we intend to conduct further research where 5-axis milling is applied, which will provide more detailed data not only on the influence of the tilting of the tool but also on the contact zone at the cutting point between the tools and the machined surface. It will also be possible to assess the size of the maximum and minimum average diameter of the tool, i.e., according to the measurement parameters in the form of the volume of the removed material and surfaces.
- -
- There is also the possibility to examine the methodology used to assess the quality of the curved/shaped surface in the case of milling other materials, e.g., alloys that are difficult to machine, such as nickel, titanium, and stainless steel alloys.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| CNC | computer numerical control |
| NC | numerical control |
| CAM | computer-aided manufacturing |
| CL | cutter location |
| CAD | computer-aided design |
| HB | hardness Brinell |
| D | diameter of milling tool |
| RPM | revolutions per minute |
| ae | radial depth of cut |
| ap | depths of cut for given strategies |
| fz | feed per tooth |
| Deff max | maximum effective radius |
| Deff min | minimum effective radius |
| F | feed |
| T | tolerance |
| P | surface allowance |
| STL | stereolithography |
| STEP | Standard for the Exchange of Product Data |
| SQP | Sequential quadratic programming |
| Ssk | Skewness |
| Lc | cutoff |
| vc | cutting speed |
References
- Jiang, X.J.; Scott, P.J. Advanced Metrology Freeform Surfaces, 1st ed.; Academic Press: Huddersfield, England, 2020; pp. 11–34. [Google Scholar]
- Kruth, J.P.; Kiswanto, G.; Lauwers, B. Gouging elimination through tool lifting in tool path generation for five-axis milling based on faceted models. Int. J. Adv. Manuf. Technol. 2007, 32, 293–309. [Google Scholar] [CrossRef]
- Ma, J.; Hu, G.; Jia, Z.; Zhang, N.; Wang, F. Effect of geometric feature and cutting direction on variation of force and vibration in high-speed milling of TC4 curved surface. Int. J. Adv. Manuf. Technol. 2018, 95, 2207–2218. [Google Scholar] [CrossRef]
- Chen, Z.C.; Vickers, G.W.; Dong, Z. A New Principle of CNC Tool Path Planning for Three-Axis Sculptured Part Machining—A Steepest-Ascending Tool Path. J. Manuf. Sci. Eng. 2004, 126, 515–523. [Google Scholar] [CrossRef]
- Kurt, M.; Bagci, E. Feedrate optimisation/scheduling on sculptured surface machining: A comprehensive review, applications and future direction. Int. J. Adv. Manuf. Technol. 2011, 55, 1037–1067. [Google Scholar] [CrossRef]
- Kurylo, P.; Frankovský, P.; Malinowski, M.; Maciejewski, T.; Varga, J.; Kostka, J.; Adrian, L.; Szufa, S.; Rusnáková, S. Data Exchange with Support for the Neutral Processing of Formats in Computer-Aided Design/Computer-Aided Manufacturing Systems. Appl. Sci. 2023, 13, 9811. [Google Scholar] [CrossRef]
- Kascak, L.; Varga, J.; Bidulská, J.; Bidulský, R.; Grande, M.A. Simulation tool for material behaviour prediction in additive manufacturing. Acta Met. Metall. Slovaca 2023, 29, 113–118. [Google Scholar] [CrossRef]
- Kvackaj, T.; Bidulská, J.; Bidulský, R. Overview of HSS Steel Grades Development and Study of Reheating Condition Effects on Austenite Grain Size Changes. Materials 2021, 14, 1988. [Google Scholar] [CrossRef]
- Bidulsky, R.; Gobber, F.S.; Bidulska, J.; Ceroni, M.; Kvackaj, T.; Grande, M.A. Coated Metal Powders for Laser Powder Bed Fusion (L-PBF) Processing: A Review. Metals 2021, 11, 1831. [Google Scholar] [CrossRef]
- Yan, X.; Ballu, A. Review and comparison of form error simulation methods for computer-aided tolerancing. J. Comput. Inf. Sci. Eng. 2019, 19, 010802. [Google Scholar] [CrossRef]
- Bey, M.; Cherfi, A. Finishing of freeform surfaces with an optimized Z-Constant machining strategy. 8th CIRP Conference on High Performance Cutting (HPC 2018). Procedia CIRP 77 2018, 12, 271–274. [Google Scholar] [CrossRef]
- Geng, L.; Liu, P.L.; Liu, K. Optimization of cutter posture based on cutting force prediction for five-axis machining with ball-end cutters. Int. J. Adv. Manuf. Technol. 2015, 78, 1289–1303. [Google Scholar] [CrossRef]
- Souza, A.F.; Berkenbrock, E.; Diniz, A.E.; Rodrigues, A.R. Influences of the tool path strategy on the machining force when milling free form geometries with a ball-end cutting tool. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 675–687. [Google Scholar] [CrossRef]
- Souza, A.F.; Diniz, A.E.; Rodrigues, A.R.; Coelho, R.T. Investigating the cutting phenomena in freeform milling using a ball-end cutting tool for die and mold manufacturing. Int. J. Adv. Manuf. Technol. 2014, 71, 1565–1577. [Google Scholar] [CrossRef]
- Varga, J.; Tóth, T.; Kaščák, Ľ.; Spišák, E. The effect of the machining strategy on the surface accuracy when milling with a ball end cutting tool of the aluminum alloy AlCu4Mg. Appl. Sci. 2022, 12, 10638. [Google Scholar] [CrossRef]
- Varga, J.; Spišák, E.; Gajdoš, I.; Mulidrán, P. Comparison of milling strategies in the production of shaped surfaces. Adv. Sci. Technol. Res. J. 2022, 16, 267–274. [Google Scholar] [CrossRef]
- Varga, J.; Tóth, T.; Frankovský, P.; Dulebová, Ľ.; Spišák, E.; Zajačko, I.; Živčák, J. The influence of automated machining strategy on geometric deviations of machined surfaces. Appl. Sci. 2021, 11, 2353. [Google Scholar] [CrossRef]
- Kaymakci, M.; Lazoglu, I. Tool path selection strategies for complex sculptured surface machining. Mach. Sci. Technol. 2008, 12, 119–132. [Google Scholar] [CrossRef]
- Diciuc, V.; Lobontiu, M.; Nasui, V. The modeling of the ball nose end milling process by using cad methods. Acad. J. Manuf. Eng. 2011, 9, 42–47. [Google Scholar]
- Bagci, E.; Yüncüoğlu, E.U. The Effects of Milling Strategies on Forces, Material Removal Rate, Tool Deflection, and Surface Errors for the Rough Machining of Complex Surfaces. J. Mech. Eng. 2017, 63, 643–656. [Google Scholar] [CrossRef]
- Wojciechowski, S.; Wiackiewicz, G.M.; Krolczyk, G.M. Study on metrological relations between instant tool displacements and surface roughness during precise ball end milling. Measurement 2018, 129, 686–694. [Google Scholar] [CrossRef]
- Tuysuz, O.; Altintas, Y.; Feng, H.Y. Prediction of cutting forces in three and five-axis ball-end milling with tool indentation effect. Int. J. Mach. Tools Manuf. 2013, 66, 66–81. [Google Scholar] [CrossRef]
- Bas, G.; Demircioglu, P.; Durakbasa, N.; Osanna, P.H. A nanometrological management approach to assess the profile and the surface characteristics of the end milling tools by multi-measurement analysis. In Proceedings of the XX IMEKO World Congress. Metrology for Green Growth, Busan, Republic of Korea, 9–14 September 2012; pp. 1–5. [Google Scholar]
- Mizugaki, Y.; Hao, M.; Kikkawa, K. Geometric Generating Mechanism of Machined Surface by Ball-nosed End Milling. CIRP Ann. Manuf. Technol. 2001, 50, 69–72. [Google Scholar] [CrossRef]
- Capla, R.; Souza, A.F.; Brandão, L.C.; Coelho, R.T. Some effects of stock variations due to the use of 2 axes strategy on roughing. In Proceedings of the 18th International Congress of Mechanical Engineering, Ouro Preto, Brazil, 6–11 November 2005. [Google Scholar]
- Varga, J.; Ižol, P.; Vrabeľ, M.; Kaščák, Ľ.; Drbúl, M.; Brindza, J. Surface Quality Evaluation in the Milling Process Using a Ball Nose End Mill. Appl. Sci. 2023, 13, 10328. [Google Scholar] [CrossRef]
- Boujelbene, M.; Moisan, A.; Torbaty, S. Study of the tool inclination in multi-axes milling. In Proceedings of the 15th International Conference on Manufacturing Systems-ICMaS, Bucharest, Romania, 26–27 October 2006. [Google Scholar]
- Scandiffio, I.; Diniz, A.E.; Souza, A.F. The influence of tool-surface contact on tool life and surface roughness when milling free-form geometries in hardened steel. Int. J. Adv. Manuf. Technol. 2017, 92, 615–626. [Google Scholar] [CrossRef]
- Scandiffio, I.; Diniz, A.E.; Souza, A.F. Evaluating surface roughness, tool life, and machining force when milling free-form shapes on hardened AISI D6 steel. Int. J. Adv. Manuf. Technol. 2016, 82, 2075–2086. [Google Scholar] [CrossRef]
- Toh, C.K. Design, evaluation and optimisation of cutter path strategies when high speed machining hardened mould and die materials. Mater. Des. 2005, 26, 517–533. [Google Scholar] [CrossRef]
- Ramos, A.M.; Relvas, C.; Simões, J.A. The influence of finishing milling strategies on texture, roughness and dimensional deviations on the machining of complex surfaces. J. Mater. Process. Technol. 2003, 136, 209–216. [Google Scholar] [CrossRef]
- Shaghayegh, S.; Sadeghi, M.H.; Hassanpour, H. The Influence of Tool Path Strategies on Cutting Force and Surface Texture during Ball End Milling of Low Curvature Convex Surfaces. Sci. World J. 2014, 2014, 374526. [Google Scholar]
- Matras, A.; Kowalczyk, R. Analysis of machining accuracy during free form surface milling simulation for different milling strategies. In Proceedings of the SPIE—The International Society for Optical Engineering, Photonics Applications in Astronomy, Communications, Industry, and High-Energy Physics Experiments, Warszava, Poland, 26 May–1 June 2014. [Google Scholar]
- Sadeghi, M.H.; Hassanpour, H.; Shajari, S.; Rasti, A. Investigation of milling strategies effect on microhardness of a typical curved surface. Mech. Eng. 2015, 15, 34–40. [Google Scholar]
- Arruda, E.M.; Brandão, L.C.; Ribeiro Filho, S.L.M.; de Oliveira, J.A. Integrated optimization using mixture design to confirm the finishing of AISI P20 using different cutting strategies and ball nose end mills. Measurement 2014, 47, 54–63. [Google Scholar] [CrossRef]
- Ouyang, D.; Van Nest, B.A.; Feng, H.Y. Automatic Ball-End Milling Tool Selection from 3D Point Cloud Data. In Proceedings of the Flexible Automation and Intelligent Manufacturing, FAIM2004, Toronto, Canada, 12–14 July 2004; pp. 253–260. [Google Scholar]
- Choi, B.K.; Jun, C.S. Ball-end cutter interference avoidance in NC machining of sculptured surfaces. Comput. Aided Des. 1989, 21, 371–378. [Google Scholar] [CrossRef]
- Park, S.C. Tool path generation for Z-constant contour machining. Comput. Aided Des. 2003, 35, 27–36. [Google Scholar] [CrossRef]
- Lacalle, L.N.L.; Lamikiz, A.; Polvorosa, R.; Valdivielso, A.F.; Calleja, A.; Gonzales, H.; Artexte, E. Optimised methodology for aircraft engine IBRs five-axis machining process. Int. J. Mechatron. Manuf. Syst. 2016, 9, 385. [Google Scholar] [CrossRef]
- Sadílek, M.; Poruba, Z.; Čepová, L.; Šajgalík, M. Increasing the Accuracy of Free-Form Surface Multiaxis Milling. Materials 2021, 14, 25. [Google Scholar] [CrossRef] [PubMed]
- Grešová, Z.; Ižol, P.; Vrabeľ, M.; Kaščák, Ľ.; Brindza, J.; Demko, M. Influence of Ball-End Milling Strategy on the Accuracy and Roughness of Free Form Surfaces. Appl. Sci. 2022, 12, 4421. [Google Scholar] [CrossRef]
- Tran, V.H.; Truong, T.T.; Bui, K.K.; Le, H.K. Quality Assessment of Freeform Surface Manufactured by CNC Milling Using Point Cloud Data Obtained from Different Scanning Devices. In Proceedings of the International Conference on Advanced Mechanical Engineering, Automation, and Sustainable Development, Ha Long, Vietnam, 4–7 November 2021; Springer International Publishing: Cham, Switzerland, 2022; pp. 39–45. [Google Scholar] [CrossRef]
- Masood, A.; Siddiqui, R.; Pinto, M.; Rehman, H.; Khan, M.A. Tool path generation, for complex surface machining, using point cloud data. Procedia CIRP 2015, 26, 397–402. [Google Scholar] [CrossRef]
- Li, Y.; Gu, P. Inspection of free-form shaped parts. Robot. Comput. Integr. Manuf. 2005, 21, 421–430. [Google Scholar] [CrossRef]
- Deng, G.; Wang, G.; Duan, J. A new algorithm for evaluating form error: The valid characteristic point method with the rapidly contracted constraint zone. J. Mater. Process. Technol. 2003, 139, 247–252. [Google Scholar] [CrossRef]
- Yuwen, S.; Xiaoming, W.; Dongming, G.; Jian, L. Machining localization and quality evaluation of parts with sculptured surfaces using SQP method. Int. J. Adv. Manuf. Technol. 2009, 42, 1131–1139. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, Z.; Tang, K. Sweep scan path planning for five-axis inspection of free-form surfaces. Robot. Comput. Integr. Manuf. 2018, 49, 335–348. [Google Scholar] [CrossRef]
- Chen, X.X.; Zhao, J.; Zhang, W.W. Optimization analysis considering the cutting effects for high-speed five-axis down milling process by employing ball end mill. Int. J. Adv. Manuf. Technol. 2019, 105, 4989–5008. [Google Scholar] [CrossRef]
- Boz, Y.; Khavidaki, S.E.L.; Erdim, H.; Lazoglu, I. High Performance 5-Axis Milling of Complex Sculptured Surfaces. In Machining of Complex Sculptured Surfaces, 1st ed.; Springer-Verlag: London, UK, 2012; pp. 67–125. [Google Scholar]
- Szpunar, M.; Ostrowski, R.; Trzepiecinski, T.; Kaščák, Ľ. Central Composite Design Optimisation in Single Point Incremental Forming of Truncated Cones from Commercially Pure Titanium Grade 2 Sheet Metals. Materials 2021, 14, 3634. [Google Scholar] [CrossRef] [PubMed]
- Dúravčik, M.; Kender, Š. Application of reverse engineering techniques in mechanics system services. Procedia Eng. 2012, 48, 96–104. [Google Scholar] [CrossRef]
- Kender, Š.; Brezinová, J. Reverse engineering in automotive design component. Int. Sci. J. 2022, 7, 62–65. [Google Scholar]

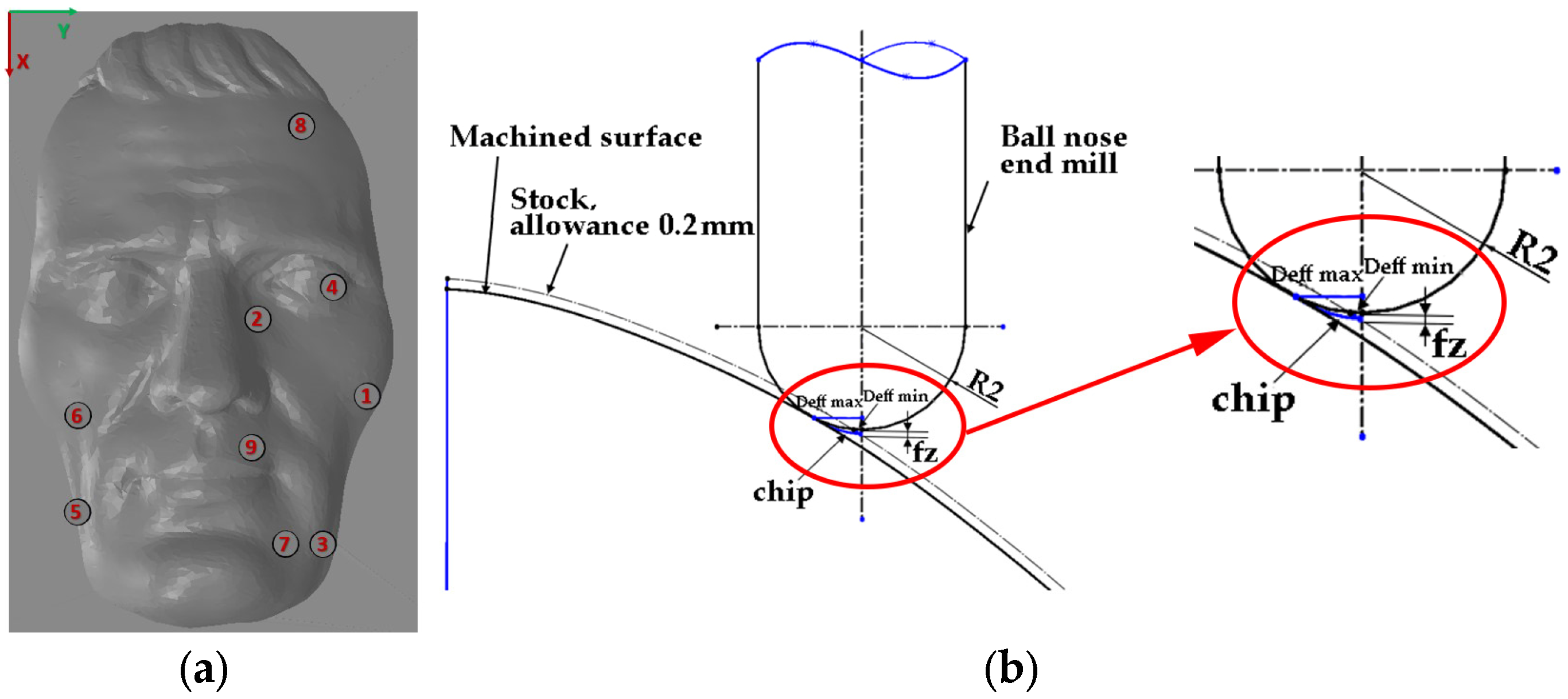
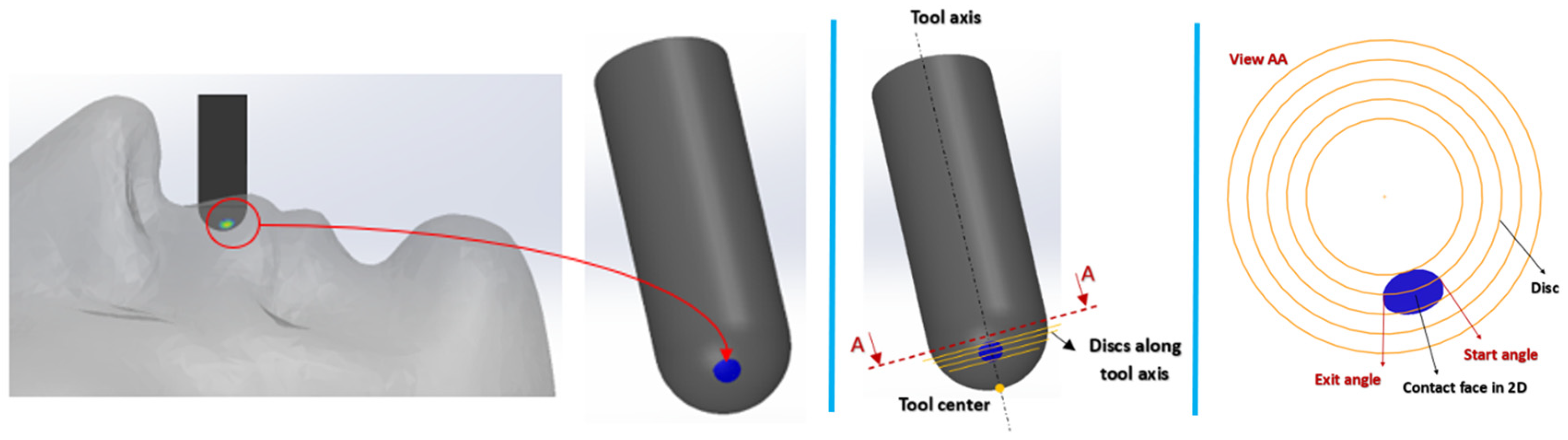

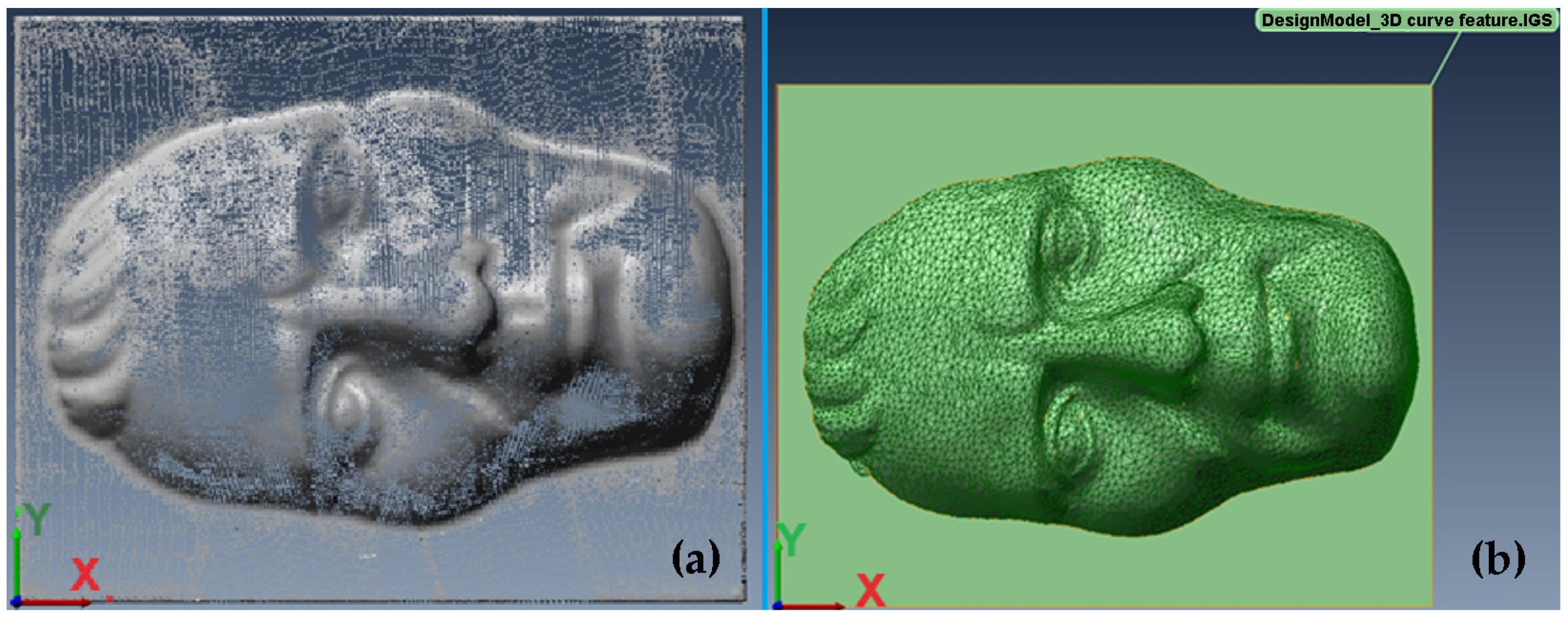
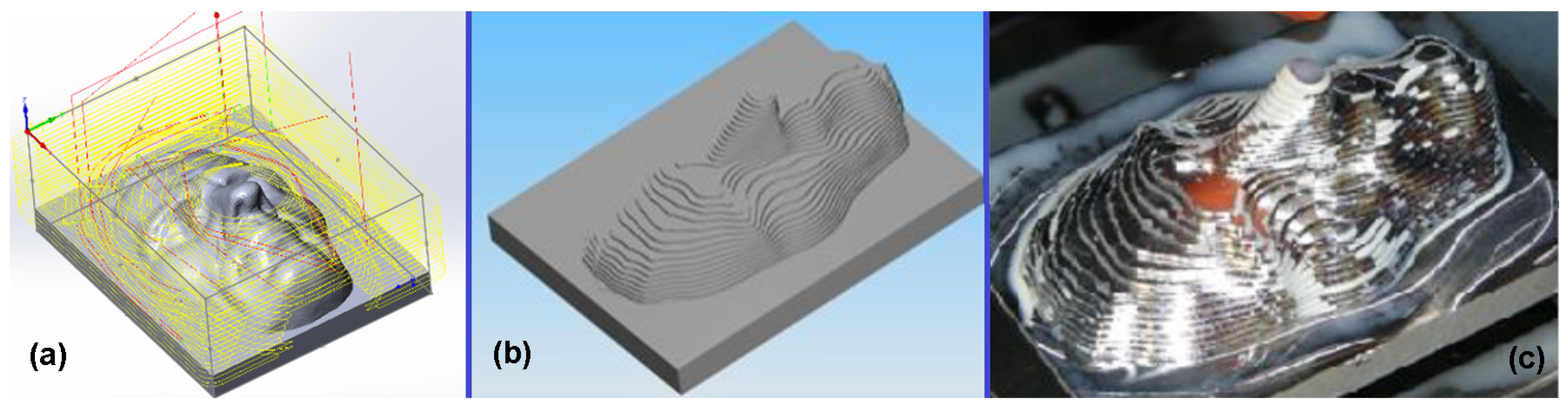
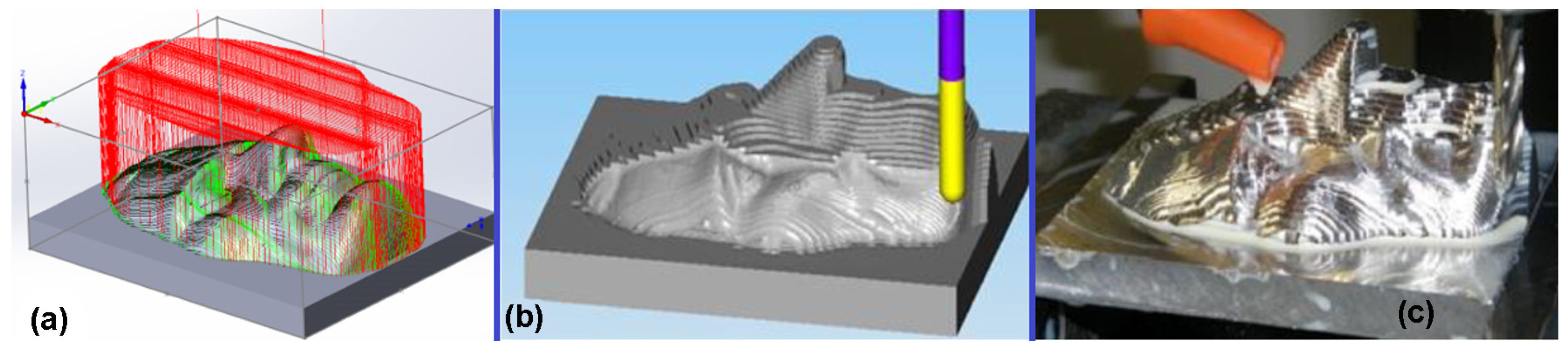
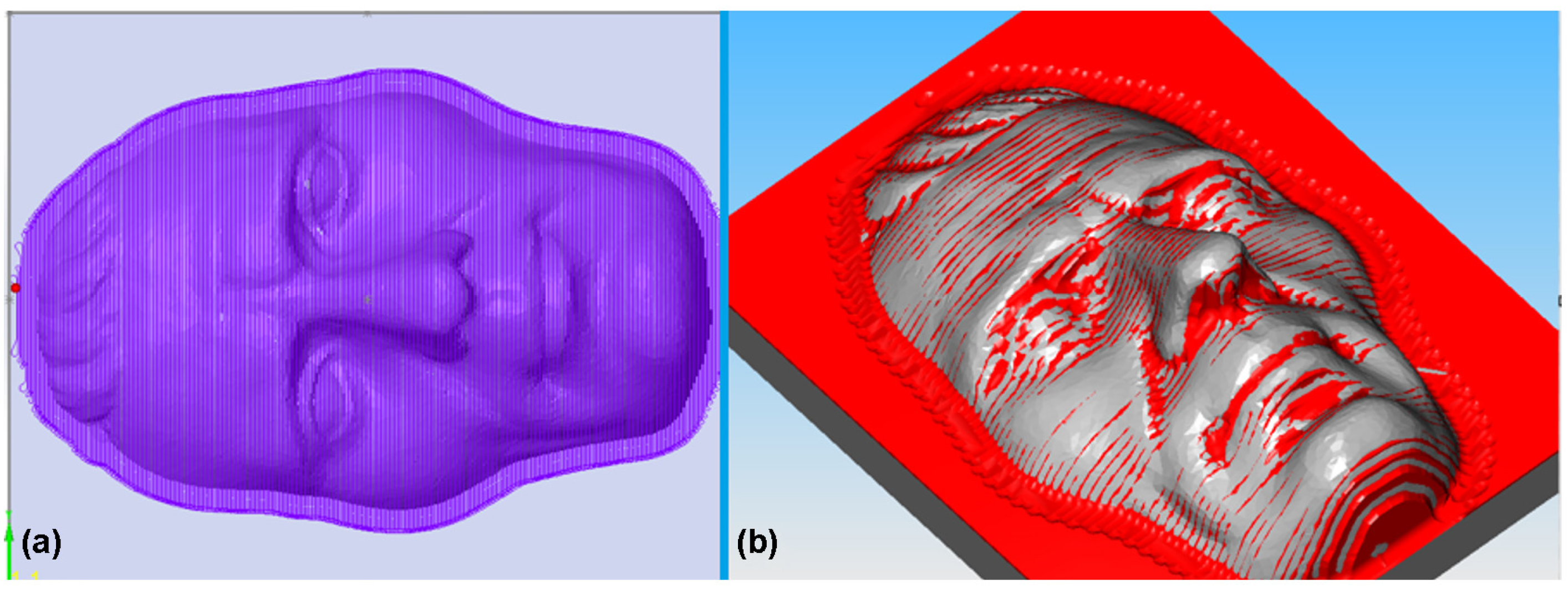
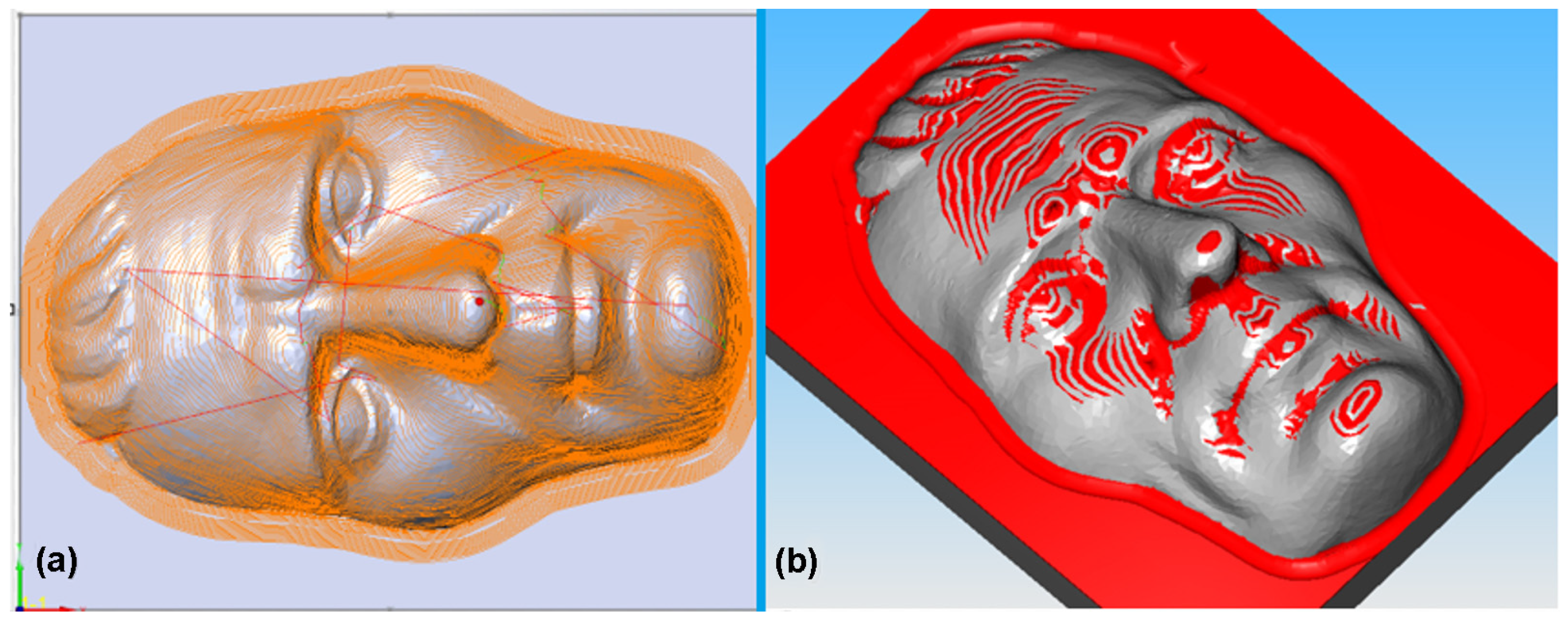

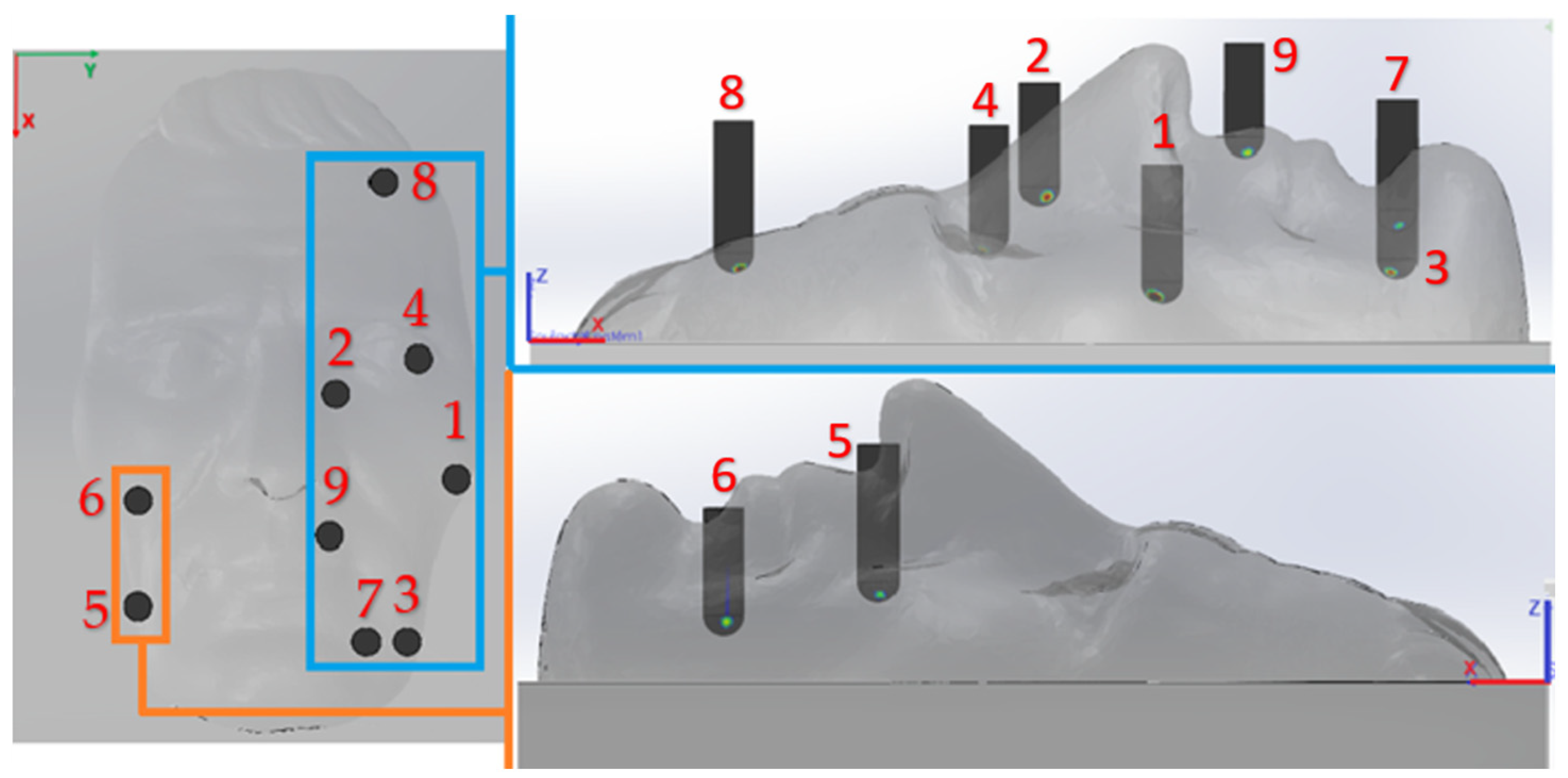



| Tool Diameter [mm] | Cutting Speed [m.min −1] | Feed per Tooth [mm] | Tooth Number | Tool Code |
|---|---|---|---|---|
| End Mill D 18 | 237 | 0.25 | 4200 | AMS2018S |
| Ball Nose End Mill D6 | 94 | 0.015 | 4900 | S510602.060 |
| Ball Nose End Mill D4 | 63 | 0.008 | 4900 | S510602.040 |
| Position | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|
  |  |  |  |  |  |  |  |  |  |
 |  |  |  |  |  |  |  |  |  |
 |  |  |  |  |  |  |  |  |  |
| Position 1 | Position 2 | Position 3 | Position 4 | Position 5 | Position 6 | Position 7 | Position 8 | Position 9 | |
|---|---|---|---|---|---|---|---|---|---|
| Deff max [mm] | 1.252 | 1.605 | 1.442 | 1.081 | 1.843 | 1.474 | 1.235 | 0.992 | 1.189 |
| Deff min [mm] | 0.398 | 1.049 | 0.802 | 0.410 | 1.425 | 0.796 | 0.471 | 0.174 | 0.393 |
| Position 1 | Position 2 | Position 3 | Position 4 | Position 5 | Position 6 | Position 7 | Position 8 | Position 9 | |
|---|---|---|---|---|---|---|---|---|---|
| Surface [mm2] | 8.467 | 7.197 | 5.751 | 5.541 | 5.513 | 5.096 | 5.080 | 2.894 | 0.921 |
| Volume [mm3] | 0.806 | 0.513 | 0.397 | 0.373 | 0.329 | 0.269 | 0.256 | 0.092 | 0.009 |
| Position 1 | Position 2 | Position 3 | Position 4 | Position 5 | Position 6 | Position 7 | Position 8 | Position 9 | |
|---|---|---|---|---|---|---|---|---|---|
| Start angle | 71.29 | 27.95 | 71.25 | 69.05 | 50.81 | 35.12 | 41.74 | 20.77 | 55.81 |
| Exit angle | 178.26 | 102.69 | 157.58 | 165.44 | 110.66 | 116.94 | 125.54 | 91.95 | 90.99 |
| Position 1 | Position 2 | Position 3 | Position 4 | Position 5 | Position 6 | Position 7 | Position 8 | Position 9 |
|---|---|---|---|---|---|---|---|---|
| −0.036 | −0.146 | −0.002 | −0.139 | 0.114 | 0.028 | −0.075 | −0.102 | −0.127 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Varga, J.; Kender, Š.; Kaščák, Ľ.; Rohaľ, V.; Spišák, E. Evaluation of Non-Planar Tool Interaction in Milling of Shaped Surfaces Using a Copy Milling Cutter. Appl. Sci. 2024, 14, 285. https://doi.org/10.3390/app14010285
Varga J, Kender Š, Kaščák Ľ, Rohaľ V, Spišák E. Evaluation of Non-Planar Tool Interaction in Milling of Shaped Surfaces Using a Copy Milling Cutter. Applied Sciences. 2024; 14(1):285. https://doi.org/10.3390/app14010285
Chicago/Turabian StyleVarga, Ján, Štefan Kender, Ľuboš Kaščák, Vladimír Rohaľ, and Emil Spišák. 2024. "Evaluation of Non-Planar Tool Interaction in Milling of Shaped Surfaces Using a Copy Milling Cutter" Applied Sciences 14, no. 1: 285. https://doi.org/10.3390/app14010285
APA StyleVarga, J., Kender, Š., Kaščák, Ľ., Rohaľ, V., & Spišák, E. (2024). Evaluation of Non-Planar Tool Interaction in Milling of Shaped Surfaces Using a Copy Milling Cutter. Applied Sciences, 14(1), 285. https://doi.org/10.3390/app14010285








