Research on Temperature Distribution and Gradient Prediction of U-Shaped Girder Bridge under Solar Radiation Effect
Abstract
1. Introduction
2. Description of U-Shaped Girder Bridges
3. Theory of Solar Radiation Intensity and Sunshine Temperature Field
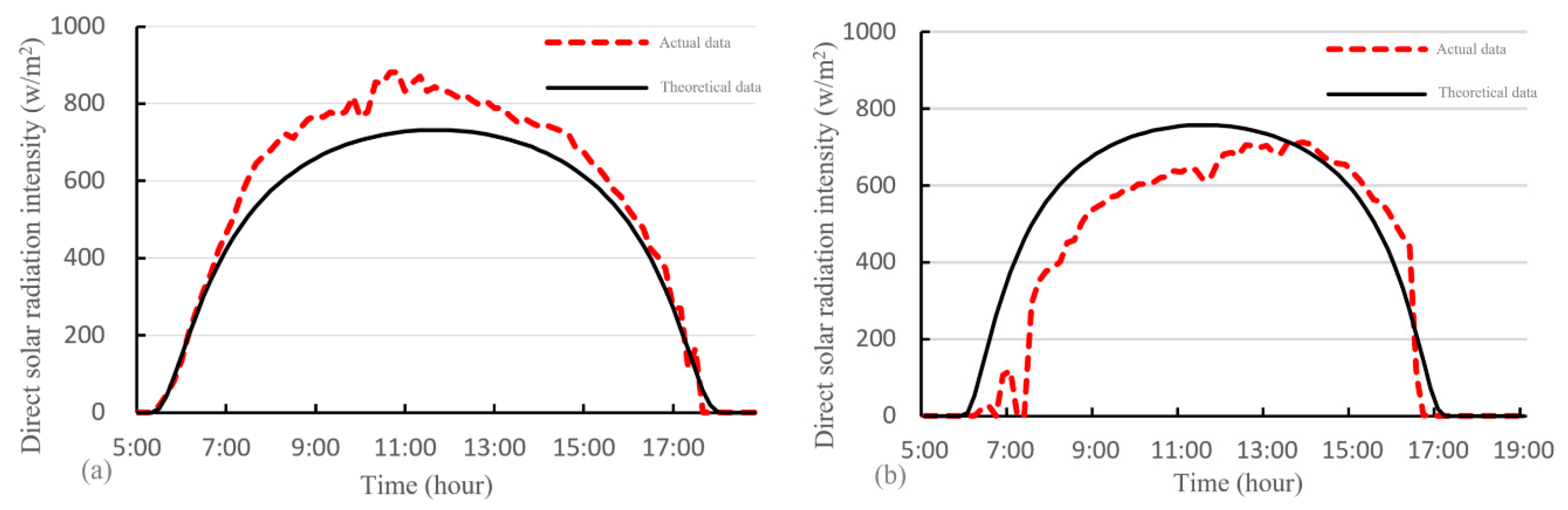
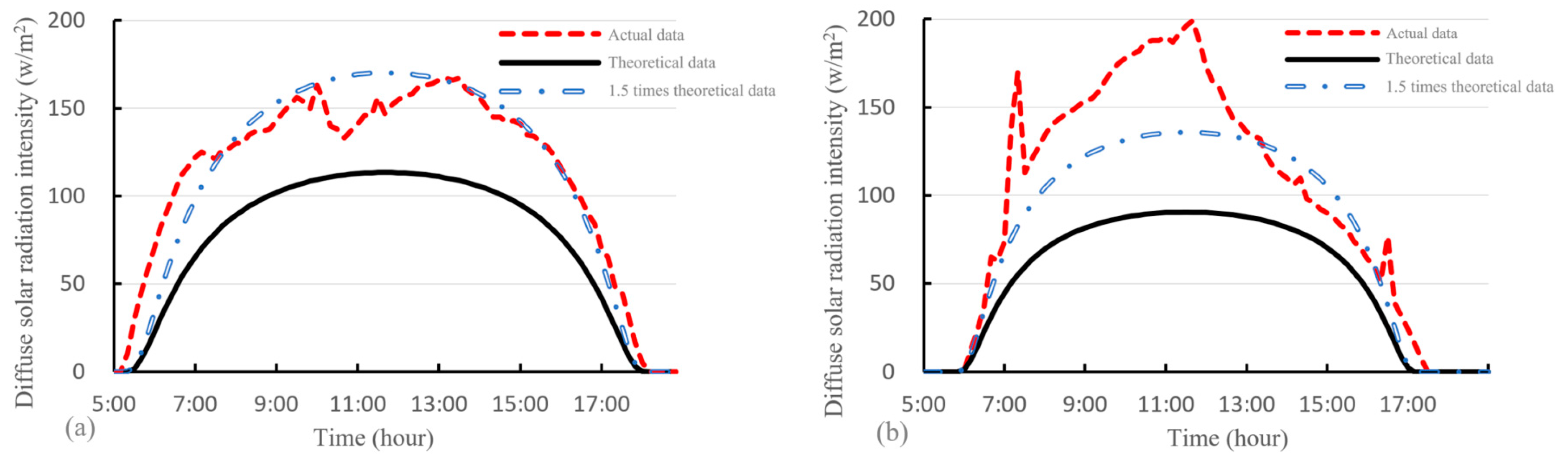
3.1. Solar Radiation Intensity Theory and Test Comparison
3.1.1. ASHRAE Clear Sky Model
3.1.2. Comparison of Actual and Theoretical Solar Radiation Intensity
3.2. Sunshine Temperature Field Theory and Test Comparison
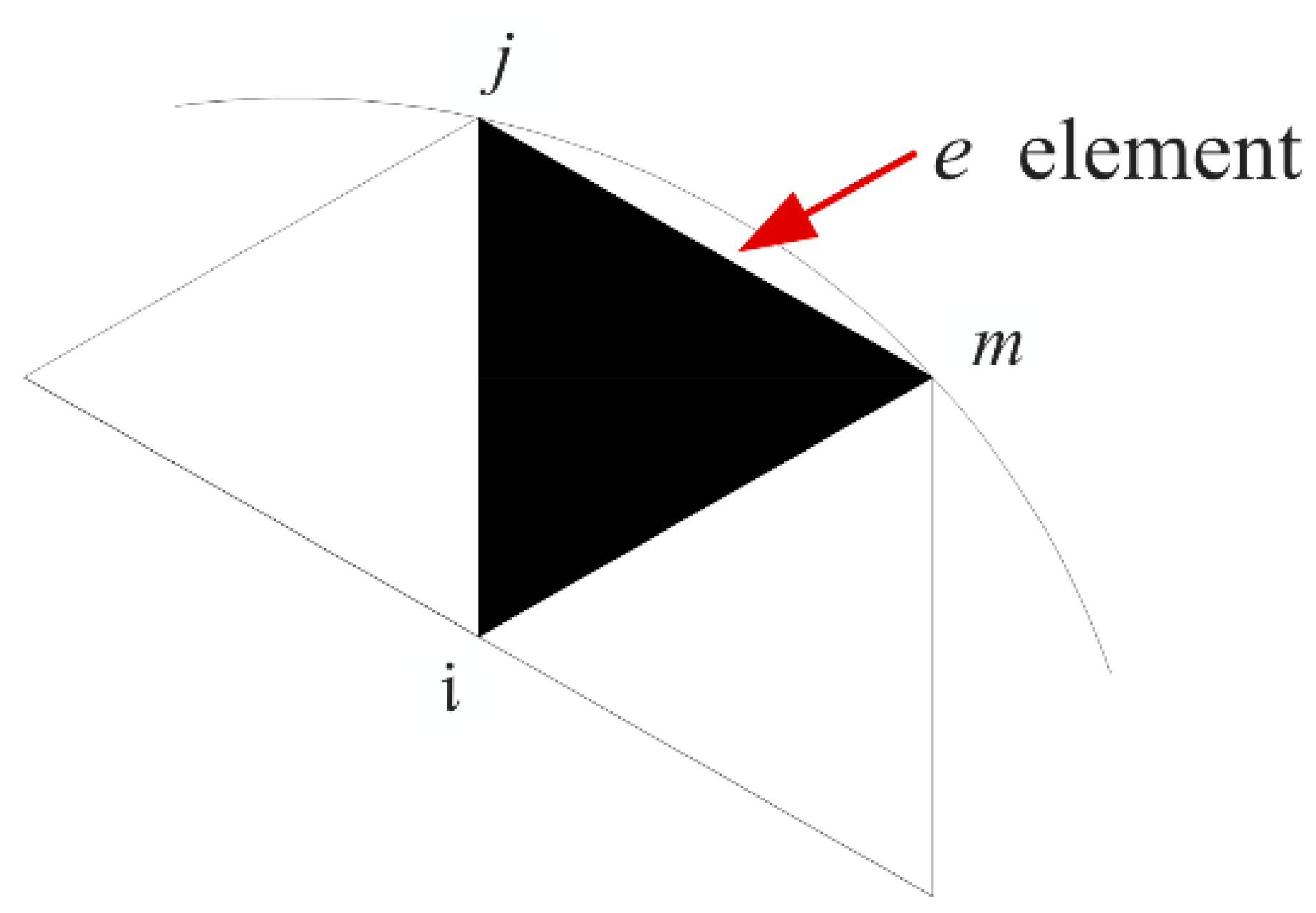
3.2.1. Finite Element Theory of Temperature Fields
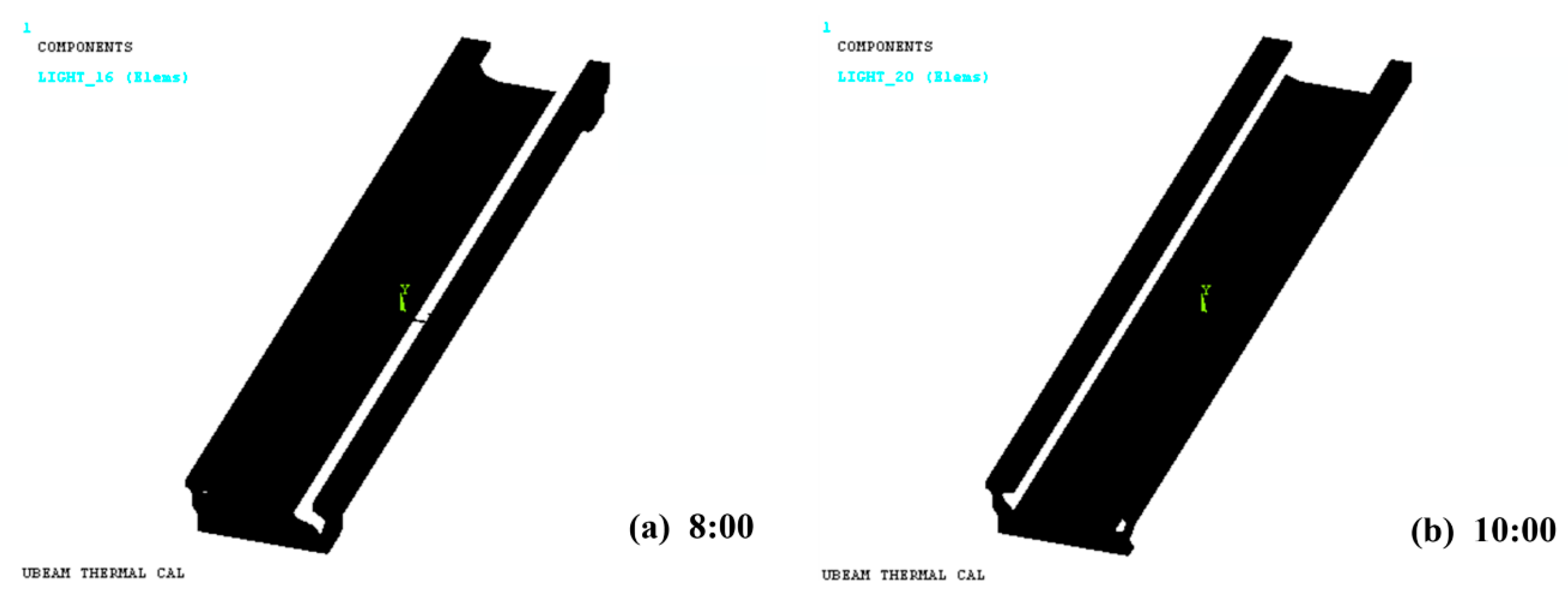
3.2.2. Comparison of Actual and Simulated Shadow Areas
4. Temperature Finite Element Model and Test Comparison
4.1. Temperature Finite Element Model
4.1.1. Temperature Field Simulation Parameters
4.1.2. Boundary and Initial Conditions for the Temperature Field
4.2. Distribution of Temperature Sensors
4.3. Test Comparison
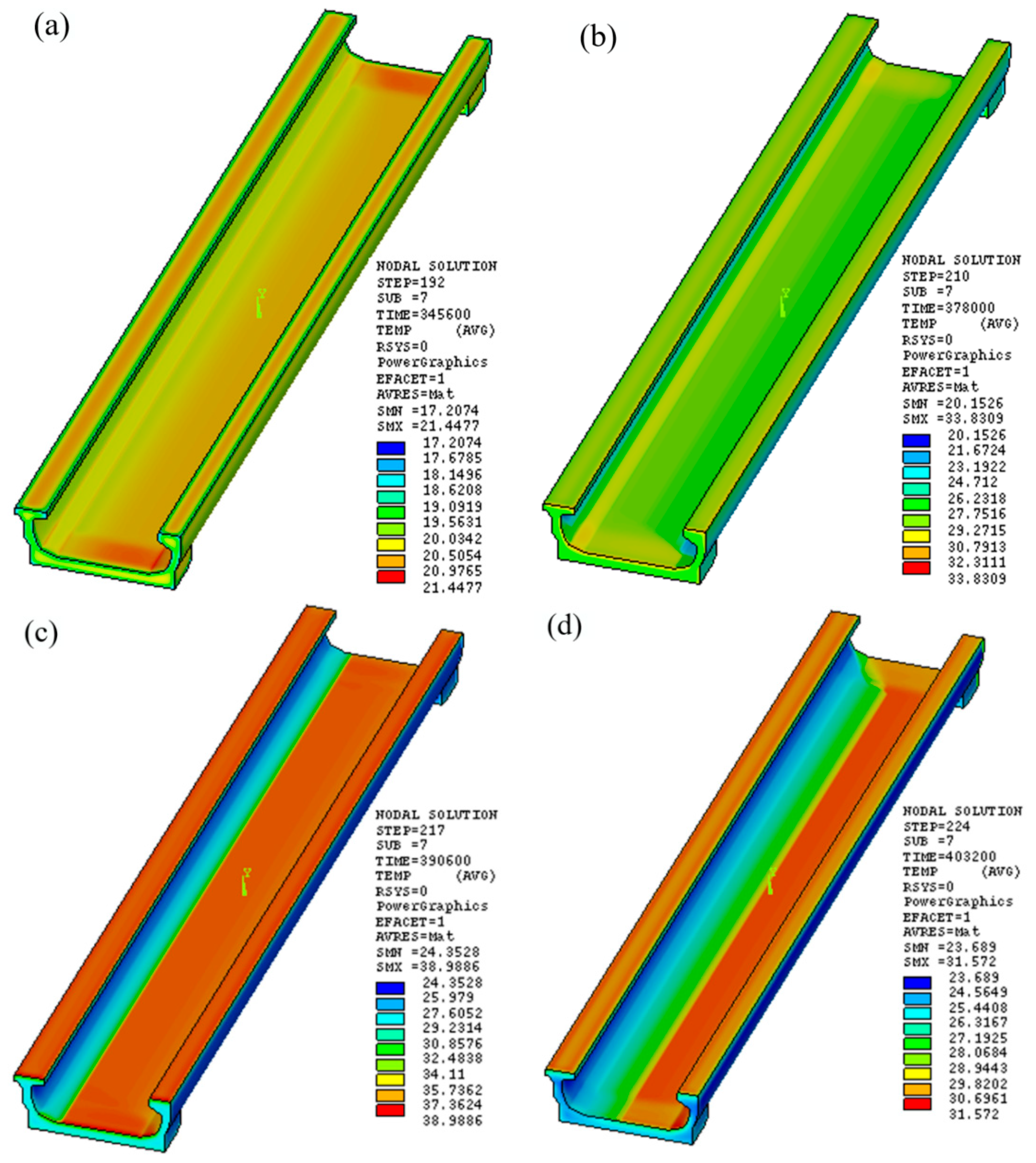
5. Temperature Distribution of U-Shaped Girder Bridge
5.1. Three-Dimensional Temperature Distribution
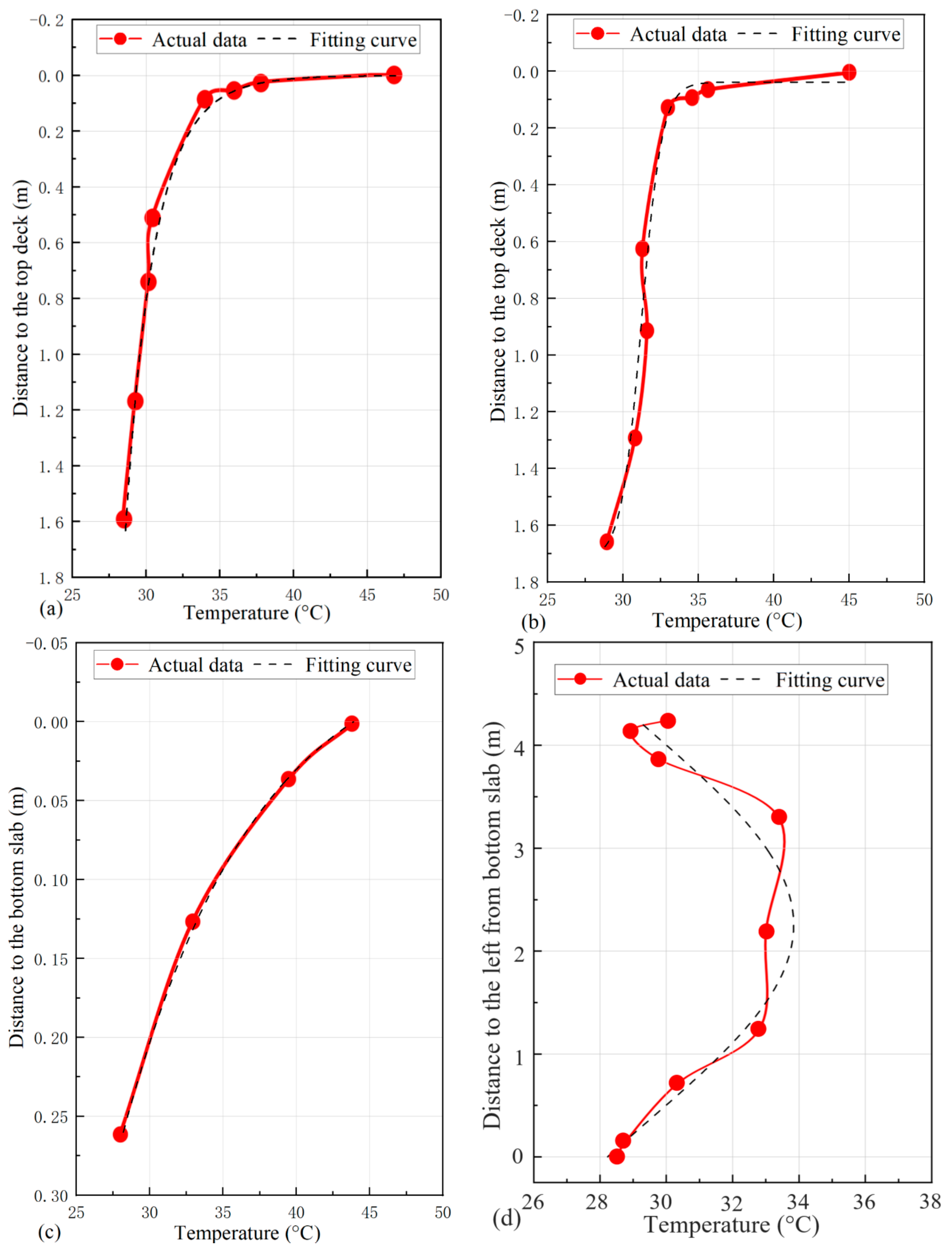
5.2. Two-Dimensional Temperature Distribution in the Mid-Span Section
5.3. Temperature Distribution of Sensors
6. Establishment of Temperature Gradient Prediction Model and Test Comparison
7. Conclusions
- (1)
- An improved ASHRAE clear sky model is proposed to simulate the shadow areas of the structure under sunlight conditions, which lays the foundation for numerical simulations of the temperature field of U-shaped girder bridges.
- (2)
- A three-dimensional transient finite element model of the U-shaped girder bridge is established based on the heat exchange theory, which was used to perform a numerical simulation of the temperature field under solar radiation, comparing the results with actual data. The finite element model results match well with the actual data, verifying the accuracy of the model.
- (3)
- The finite element model of the U-shaped girder bridge was utilized to analyze the distribution and changing patterns of the temperature field under different solar radiation conditions. The longitudinal temperature change is minimal, while the transverse temperature distribution shows significant temperature gradient changes. Particularly, there are nonlinear changes in the temperature field along the height of the webs and lateral temperature distribution of the bottom slab, with the maximum temperature difference reaching 17 °C.
- (4)
- A practical calculation method for the temperature gradient is proposed, suitable for predicting the temperature gradient of a U-shaped girder bridge. The model not only has a good fit, but also displays a correlation coefficient with actual data greater than 88%, indicating high prediction accuracy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bai, Y.; Liu, Y.; Liu, J.; Wang, Z.; Yan, X. Research on solar radiation-caused radial temperature difference and interface separation of CFST. Structures 2024, 62, 106151. [Google Scholar] [CrossRef]
- Li, B.; Nie, Y.; Zhang, J.; Jian, J.; Gao, H. Temperature field of long-span concrete box girder bridges in cold regions: Testing and analysis. Structures 2024, 61, 105969. [Google Scholar] [CrossRef]
- Li, H.; Ba, Y.K.; Zhang, N.; Liu, Y.J.; Shi, W. Temperature distribution characteristics and action pattern of concrete box girder under low-temperature and cold wave conditions. Appl. Sci. 2024, 14, 3102. [Google Scholar] [CrossRef]
- Shi, X.; Pan, H.; Liu, Z.; Hu, K.; Zhou, J. Performance of a U-shaped anchoring system for cable-stayed bridges. Structures 2022, 42, 268–283. [Google Scholar] [CrossRef]
- Junaijath, K.A.; Thomas, J. Effect of inclination of web in the behavior of through type u-girder railway bridges. Adv. Sustain. Constr. Mater. 2021, 124, 613–623. [Google Scholar]
- Song, X.; Wu, H.; Hao, J.; Cai, C.S. Noise contribution analysis of a U-shaped girder bridge with consideration of frequency dependent stiffness of rail fasteners. Appl. Acoust. 2023, 205, 109280. [Google Scholar] [CrossRef]
- Zuk, W. Summary Review of Studies Relating to Thermal Stresses in Highway Bridges; Virginia Highway Research Council: Charlottesville, VA, USA, 1965.
- Erickson, E.L.; Eenam, N.V. Application and development of AASHO specifications to bridge design. J. Struct. Div. 1957, 83, 1320–1331. [Google Scholar] [CrossRef]
- Churchward, A.; Sokal, Y.J. Prediction of temperatures in concrete bridges. J. Struct. Div. 1981, 107, 2163–2176. [Google Scholar] [CrossRef]
- Priestley, M.J.N. Thermal gradients in bridges-some design considerations. N. Z. Eng. 1972, 27, 228–233. [Google Scholar]
- Lu, Y.; Li, D.; Wang, K.; Jia, S. Study on solar radiation and the extreme thermal effect on concrete box girder bridges. Appl. Sci. 2021, 11, 6332. [Google Scholar] [CrossRef]
- Lin, C.C.; Wang, Y.Q.; Shi, Y.C. Analysis of the temperature field of steel members in sunshine. Steel Constr. 2010, 25, 38–43. [Google Scholar]
- Emerson, M. The calculation of the distribution of temperature in bridges. Transp. Res. Board Lab. Rep. 1973, 561. [Google Scholar]
- Hunt, B.; Cooke, N. Thermal calculations for bridge design. J. Struct. Div. 1975, 101, 1763–1781. [Google Scholar] [CrossRef]
- Mirambell, E.; Aguado, A. Temperature and stress distributions in concrete box girder bridges. J. Struct. Eng. 1990, 116, 2388–2409. [Google Scholar] [CrossRef]
- Elbadry, M.M.; Ghali, A. Temperature variations in concrete bridges. J. Struct. Eng. 1983, 109, 2355–2374. [Google Scholar] [CrossRef]
- Branco, F.A.; Mendes, P.A. Thermal actions for concrete bridge design. J. Struct. Eng. 1993, 119, 2313–2331. [Google Scholar] [CrossRef]
- Imbsen, R.A.; Vandershaf, D.E.; Schamber, R.A.; Nutt, R.V. Thermal Effects in Concrete Bridge Superstructures; National Cooperative Highway Research Program: Washington, DC, USA, 1985. [Google Scholar]
- Wang, Z.Q.; An, L.Q. Adaptive finite element method for temperature field analysis. J. Eng. Therm. Energy Power 2002, 17, 92–94. [Google Scholar]
- Liang, Z.; Zhou, H.; Zhao, C.; Wang, F.; Zhou, Y. Experimental and numerical study of the influence of solar radiation on the surface temperature field of low-heat concrete in a pouring block. Buildings 2023, 13, 1519. [Google Scholar] [CrossRef]
- Sun, R.H.; Ren, W.X.; Yang, X.; Wang, M.Y. Study of two-dimensional temperature gradient of multi-room concrete box girder. J. Hefei Univ. Technol. 2016, 39, 1680–1687. [Google Scholar]
- Hossain, T.; Segura, S.; Okeil, A.M. Structural effects of temperature gradient on a continuous prestressed concrete girder bridge: Analysis and field measurements. Struct. Infrastruct. Eng. 2020, 16, 1539–1550. [Google Scholar] [CrossRef]
- Lee, J.-H.; Kalkan, I. Analysis of thermal environmental effects on precast, prestressed concrete bridge girders: Temperature differentials and thermal deformations. Adv. Struct. Eng. 2012, 15, 447–459. [Google Scholar] [CrossRef]
- Xue, S.; Dai, G.L.; Yan, B. Sunshine temperature mode of prestressed concrete bridge with U-shape section. Sci. Sin. 2016, 3, 286–292. [Google Scholar]
- Giussani, F. The effects of temperature variations on the long-term behavior of composite steel-concrete beams. Eng. Struct. 2009, 31, 2392–2406. [Google Scholar] [CrossRef]
- Hagedorn, R.; Martí-Vargas, J.R.; Dang, C.N.; Hale, W.M.; Floyd, R.W. Temperature gradients in bridge concrete I-girders under heat wave. J. Bridge Eng. 2019, 24, 04019077. [Google Scholar] [CrossRef]
- Wei, X.C.; Zhang, H.S.; Liu, Y.F. Thermal effect on dynamic characteristics of concrete girder bridges. Structures 2024, 61, 105974. [Google Scholar] [CrossRef]
- Ngo, D.Q.; Nguyen, H.C. Monitoring and analysis of temperature distribution in reinforced concrete bridge box girders in Vietnam. Case Stud. Constr. Mater. 2024, 20, e02857. [Google Scholar] [CrossRef]
- Yang, X.; Zhou, Y.; Li, X.; Zhang, J. Effect of temperature changes on bearing motion of long-span steel truss continuous girder bridge. Constr. Build. Mater. 2024, 430, 136440. [Google Scholar] [CrossRef]
- Luo, Q. Temperature Field Test and Theoretical Study of Concrete U-Shaped Beams for Rail Transit; Southeast University: Nanjing, China, 2013; pp. 1–82. [Google Scholar]
- Liu, W.S.; Dai, G.L.; Rao, S.C. Numerical calculation on solar temperature field of a cable-stayed bridge with U-shaped section on high-speed railway. J. Cent. South Univ. 2014, 21, 3345–3352. [Google Scholar] [CrossRef]
- Peng, Y.; Zhao, C.; Wang, S.; Zhou, Y.; Xiang, X.; Feng, Y. Mechanical behaviors of the U-girder for urban maglev transit under temperature loads and train loads. J. Vib. Control 2023, 10, 10775463231214822. [Google Scholar] [CrossRef]
- Mendyl, A.; Mabasa, B.; Bouzghiba, H.; Weidinger, T. Calibration and validation of global horizontal irradiance clear sky models against mcclear clear sky model in morocco. Appl. Sci. 2022, 13, 320. [Google Scholar] [CrossRef]
- Li, J.P.; Song, A.G. Compare of clear day solar radiation model of Beijing and Ashare. J. Cap. Norm. Univ. Nat. Sci. Ed. 1998, 19, 37–40. [Google Scholar]
- Sene, N. Second-grade fluid with Newtonian heating under Caputo fractional derivative: Analytical investigations via Laplace transforms. Math. Model. Numer. Simul. Appl. 2022, 2, 13–25. [Google Scholar] [CrossRef]
- Sun, Y.; Du, C.; Gong, H.; Li, Y.; Chen, J. Effect of temperature field on damage initiation in asphalt pavement: A microstructure-based multiscale finite element method. Mech. Mater. 2020, 144, 103367. [Google Scholar] [CrossRef]
- Wang, G.C.; Zhang, J.; Kong, X. Study on passenger comfort based on human–bus–road coupled vibration. Appl. Sci. 2020, 10, 3254. [Google Scholar] [CrossRef]
- Schüßler, C.; Hoffmann, M.; Bräunig, J.; Ullmann, I.; Ebelt, R.; Vossiek, M. A realistic radar ray tracing simulator for large MIMO-arrays in automotive environments. IEEE J. Microw. 2021, 1, 962–974. [Google Scholar] [CrossRef]
- Kasdorf, S.; Troksa, B.; Key, C.; Harmon, J.; Notaroš, B.M. Advancing accuracy of shooting and bouncing rays method for ray-tracing propagation modeling based on novel approaches to ray cone angle calculation. IEEE Trans. Antennas Propag. 2021, 69, 4808–4815. [Google Scholar] [CrossRef]
- Soliman, A.S.; Xu, L.; Dong, J.; Cheng, P. Numerical investigation of a photovoltaic module under different weather conditions. Energy Rep. 2022, 8, 1045–1058. [Google Scholar] [CrossRef]
- Cheng, W.; Chentian, Z.; Shuo, L.; Jianhua, L. Study on Sunshine Stress Effect of Long-Span and Wide Concrete Box Girder. Int. J. Photoenergy 2022, 1, 8149765. [Google Scholar] [CrossRef]
- Liu, C.; Xiao, J.; Ma, K.; Xu, H.; Shen, R.; Zhang, H. Experimental and numerical investigation on the temperature field and effects of a large-span gymnasium under solar radiation. Appl. Therm. Eng. 2023, 225, 120169. [Google Scholar] [CrossRef]
- Xiao, J.Z.; Song, Z.W.; Zhang, C.Z. On sensitivity of thermal parameters for concrete box girder in the sunshine. Key Eng. Mater. 2008, 385, 825–828. [Google Scholar] [CrossRef]
- TB 10002-2017; Code for Design on Railway Bridge and Culvert. China Railway Publishing House: Beijing, China, 2017.
- Lu, H.; Hao, J.; Zhong, J.; Wang, Y.; Yang, H. Analysis of sunshine temperature field of steel box girder based on monitoring data. Adv. Civ. Eng. 2020, 1, 9572602. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Numerical analysis of solar energy storage within a double pipe utilizing nanoparticles for expedition of melting. Sol. Energy Mater. Sol. Cells 2022, 245, 111856. [Google Scholar] [CrossRef]




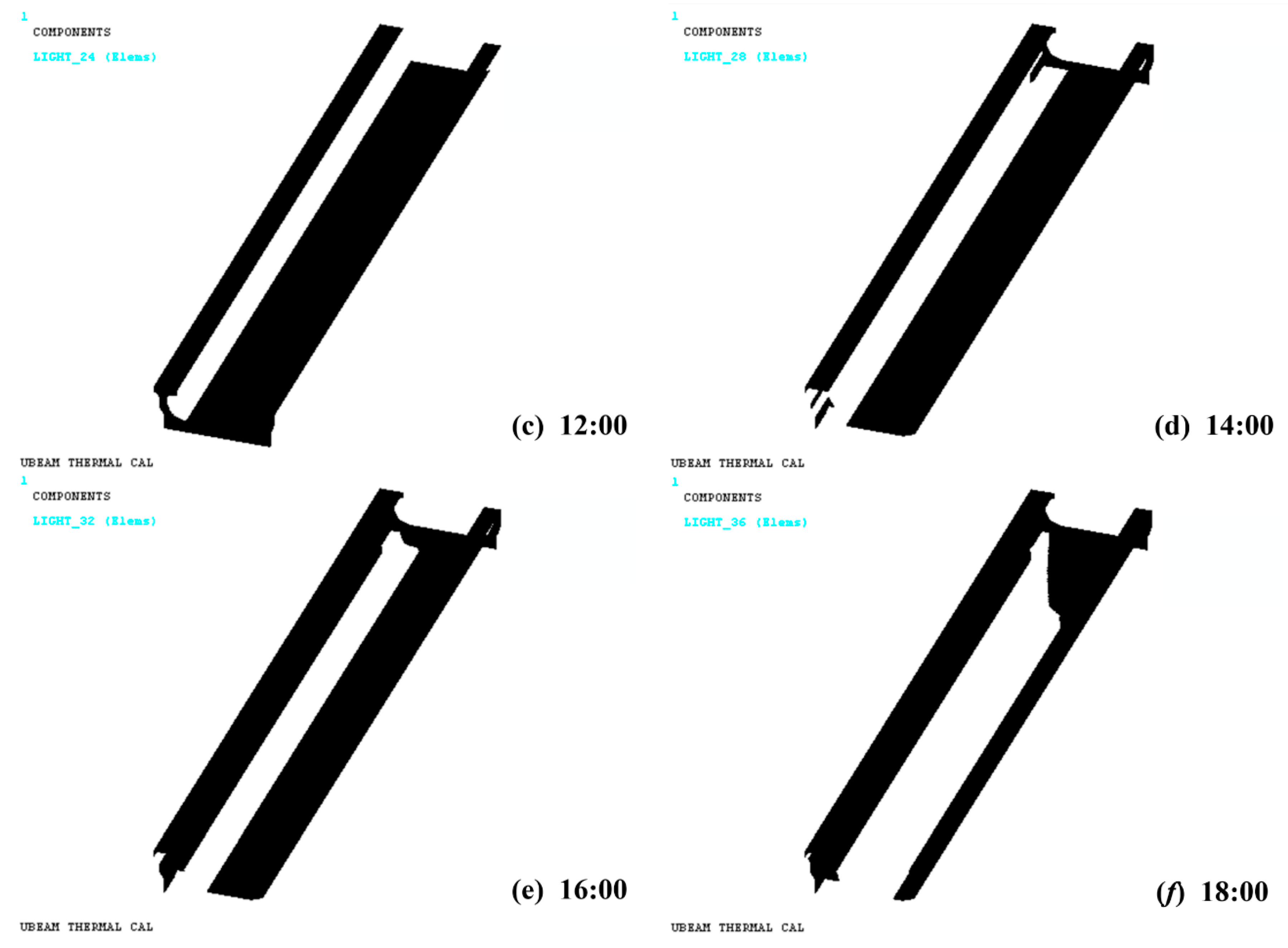
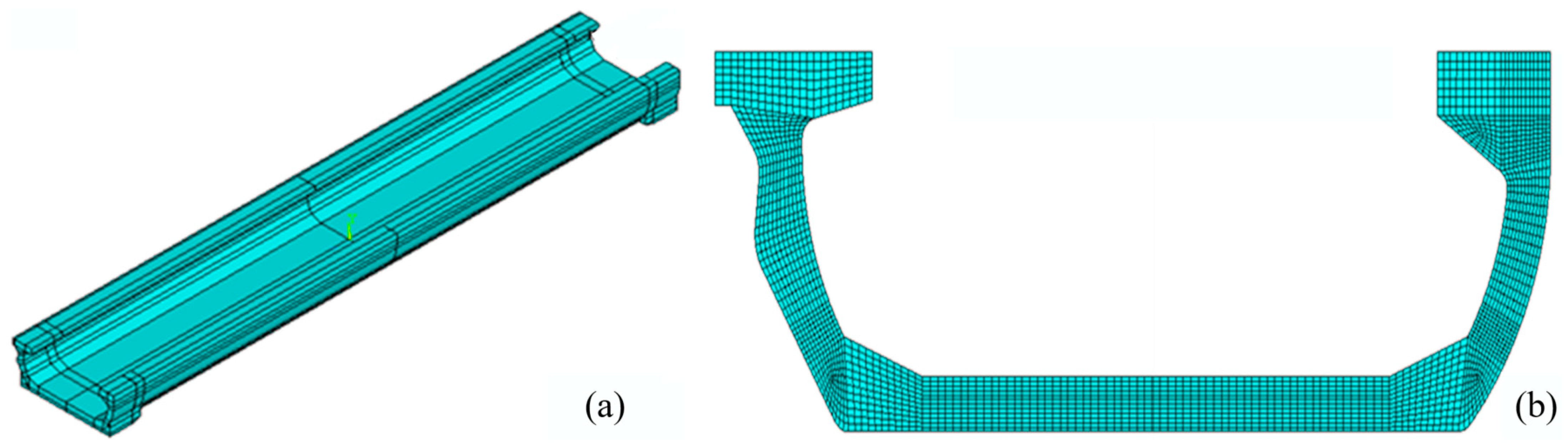
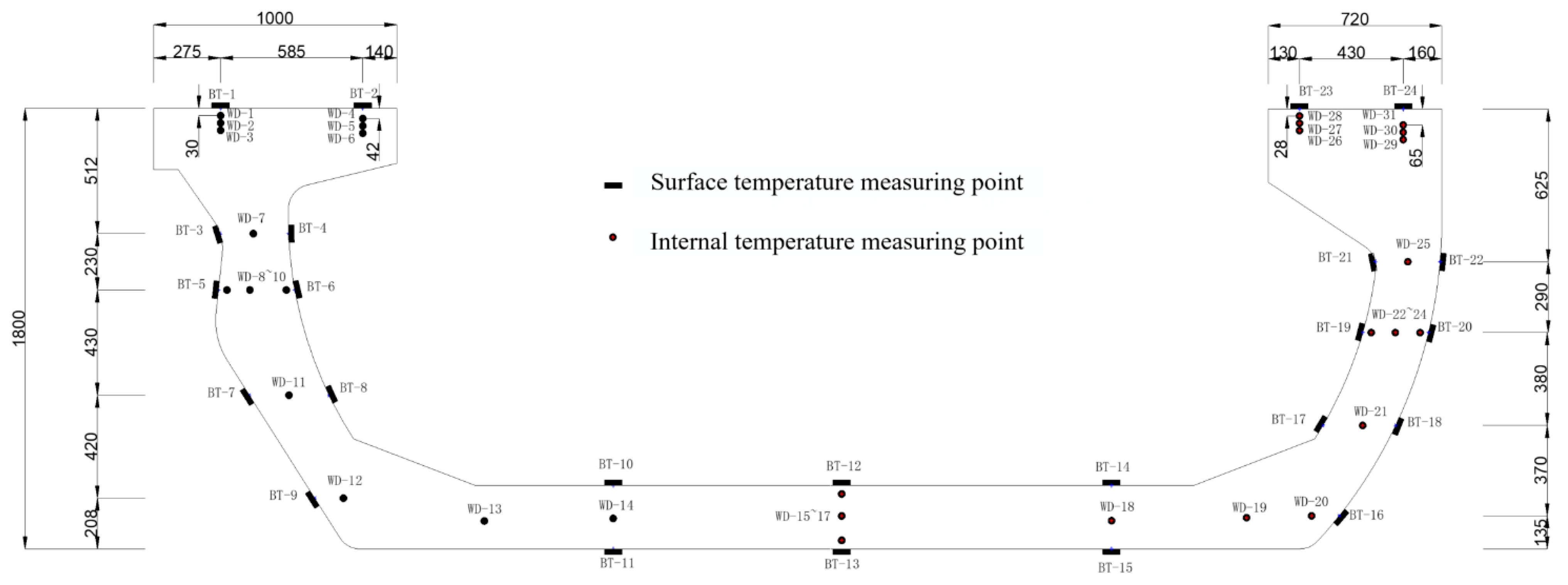
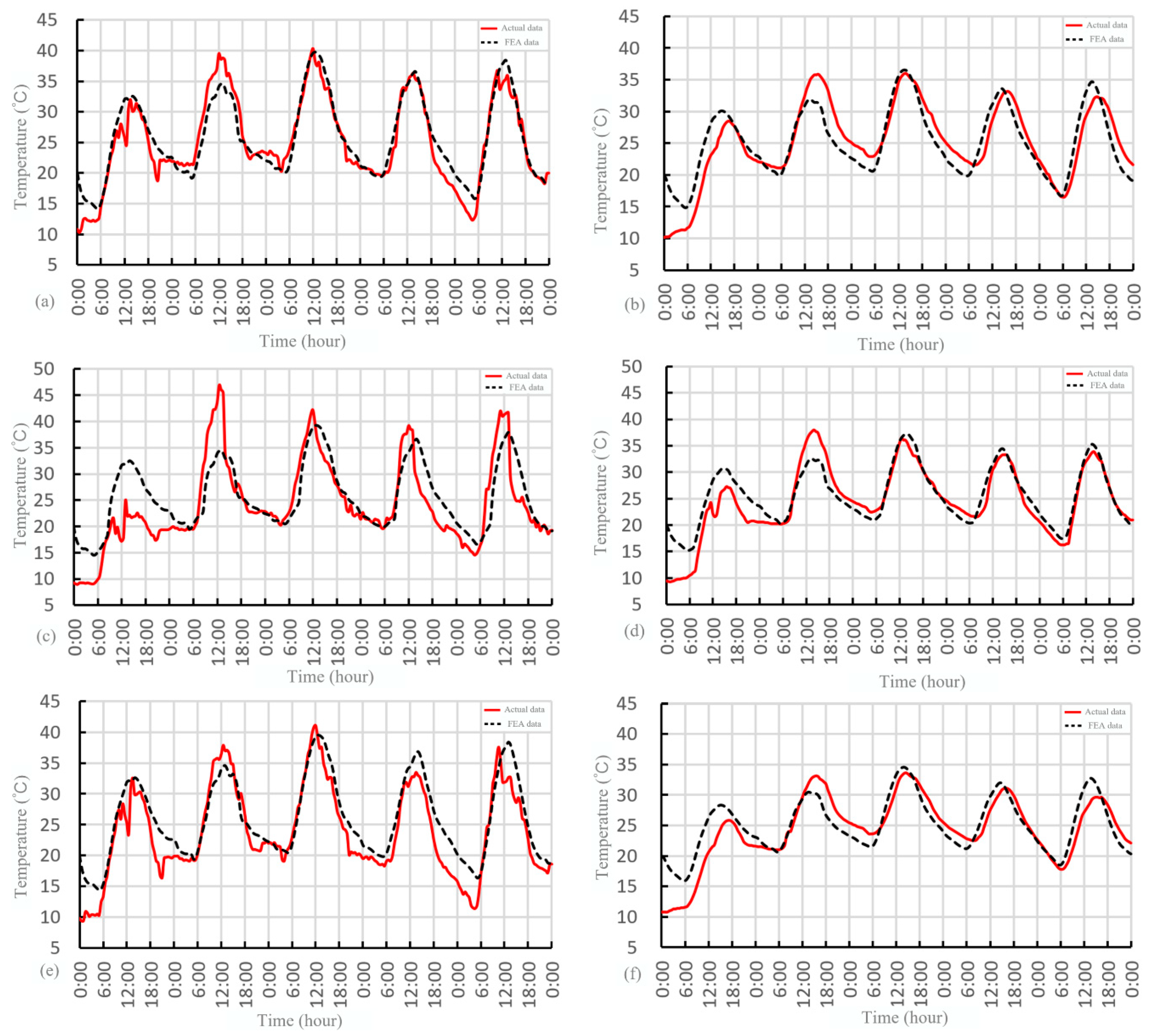


| Simulation Parameter | Density (kg/m3) | Coefficient of Heat Conductivity w/(m·k) | Specific Heat J/(kg·k) | Total Heat Exchange Coefficient h/(m2·k) |
|---|---|---|---|---|
| Parameter value | 2650.0 | 2.0 | 930.0 | 13.5 + 3.88v |
| Parameter | a11 | b11 |
|---|---|---|
| Parameter value | 4.32 × 1021 | −14.71 |
| Standard error | 1.50 | 1.02 |
| Parameter | a21 | a22 | b21 | b22 |
|---|---|---|---|---|
| Parameter value | 0.04 | 1.72 | 31.23 | 0.68 |
| Standard error | 0.11 | 0.23 | 0.28 | 0.34 |
| Parameter | a31 | a32 | b31 | b32 |
|---|---|---|---|---|
| Parameter value | 38.66 | 38.65 | −1569.50 | 461.32 |
| Standard error | 0.001 | 0.001 | 0.02 | 0.01 |
| Parameter | a41 | a42 | w | x0 |
|---|---|---|---|---|
| Parameter value | 2.25 | 53.16 | 3.81 | 22.69 |
| Standard error | 0.11 | 123.62 | 3.72 | 15.58 |
| Function | Allometricl | Boltzmann | Sine | Gauss |
|---|---|---|---|---|
| R2 | 0.99 | 0.95 | 0.99 | 0.88 |
| Iterations | 68 | 6 | 60 | 9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Zhang, J.; Meng, X.; Lin, J. Research on Temperature Distribution and Gradient Prediction of U-Shaped Girder Bridge under Solar Radiation Effect. Appl. Sci. 2024, 14, 6167. https://doi.org/10.3390/app14146167
Song Y, Zhang J, Meng X, Lin J. Research on Temperature Distribution and Gradient Prediction of U-Shaped Girder Bridge under Solar Radiation Effect. Applied Sciences. 2024; 14(14):6167. https://doi.org/10.3390/app14146167
Chicago/Turabian StyleSong, Yumin, Jie Zhang, Xiaoliang Meng, and Jiazhen Lin. 2024. "Research on Temperature Distribution and Gradient Prediction of U-Shaped Girder Bridge under Solar Radiation Effect" Applied Sciences 14, no. 14: 6167. https://doi.org/10.3390/app14146167
APA StyleSong, Y., Zhang, J., Meng, X., & Lin, J. (2024). Research on Temperature Distribution and Gradient Prediction of U-Shaped Girder Bridge under Solar Radiation Effect. Applied Sciences, 14(14), 6167. https://doi.org/10.3390/app14146167





