A Study of Friction Nonlinearity and Compensation for Turntable Servo Systems
Abstract
:1. Introduction
2. Friction Modeling and Identification
2.1. Friction Modeling
2.2. Friction Parameter Identification
2.2.1. Introduction to the Identification Algorithm
- Randomly initialize the particle population’s initial positions and velocities within the range of dynamic parameters, with a population size of M = 100 and a maximum identification number of 500.
- Evaluate the fitness of each particle, update the personal best and global best values, and retain relatively better parameter identification results.
- Update the particle positions and velocities, forming new identification parameters.
- Repeat iterations until reaching the maximum number of identifications, and select the particle corresponding to the global extremum as the best identification value.
2.2.2. Parameter Identification Experiment
3. Compensation Based on the Friction Model
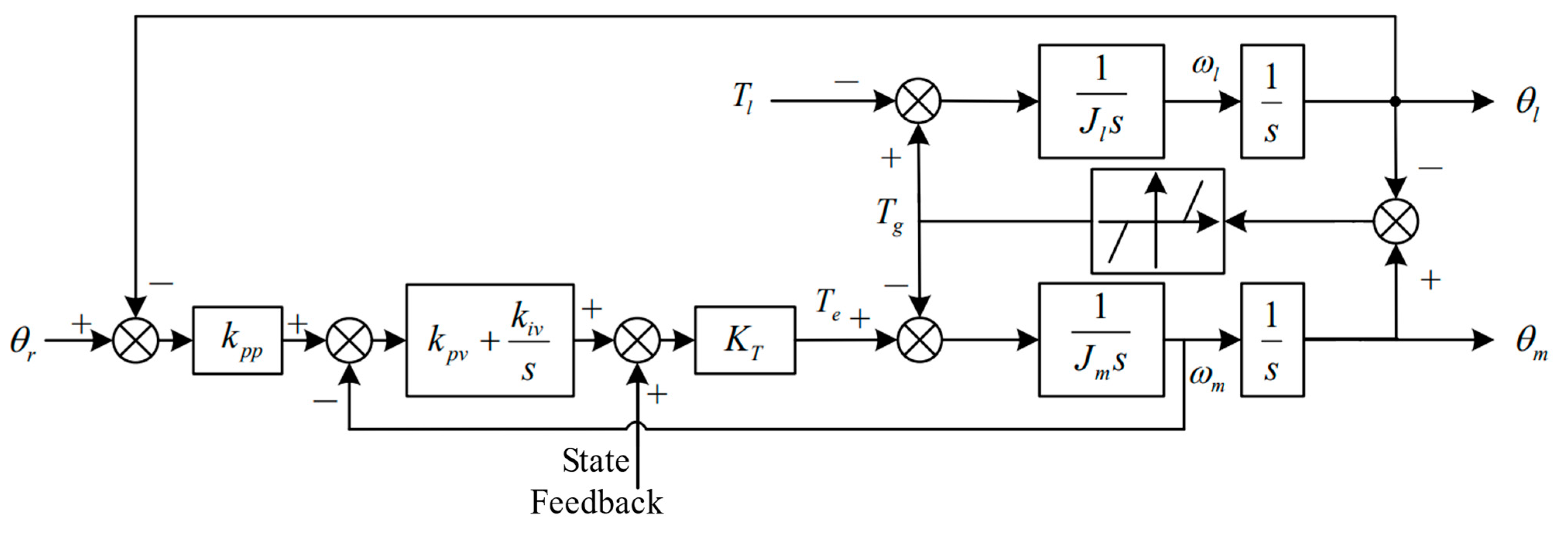
3.1. Feedforward Compensation Based on the Friction Model
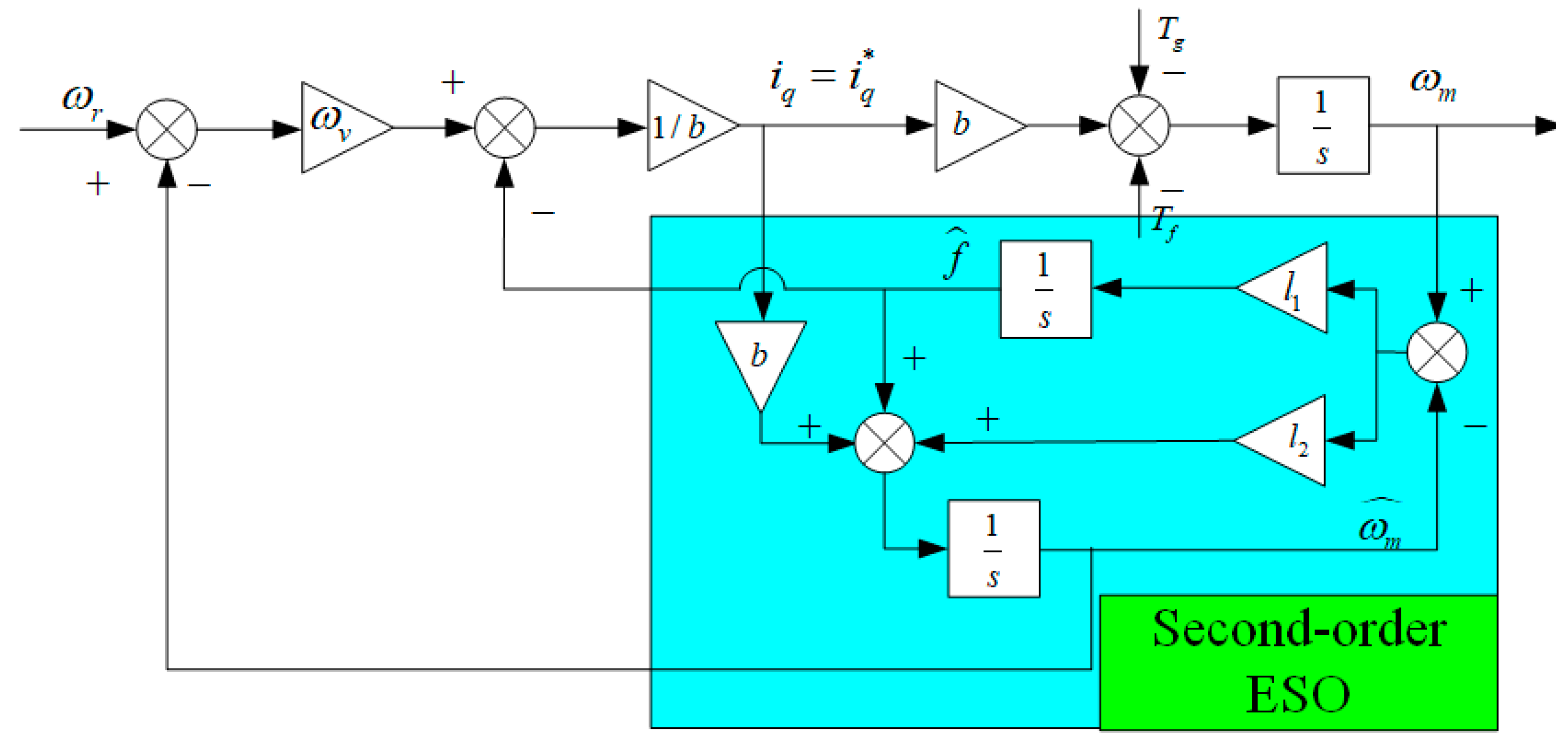
3.2. Friction Compensation Based on Extended State Observer
4. Simulation and Experimental Validation of Friction Compensation
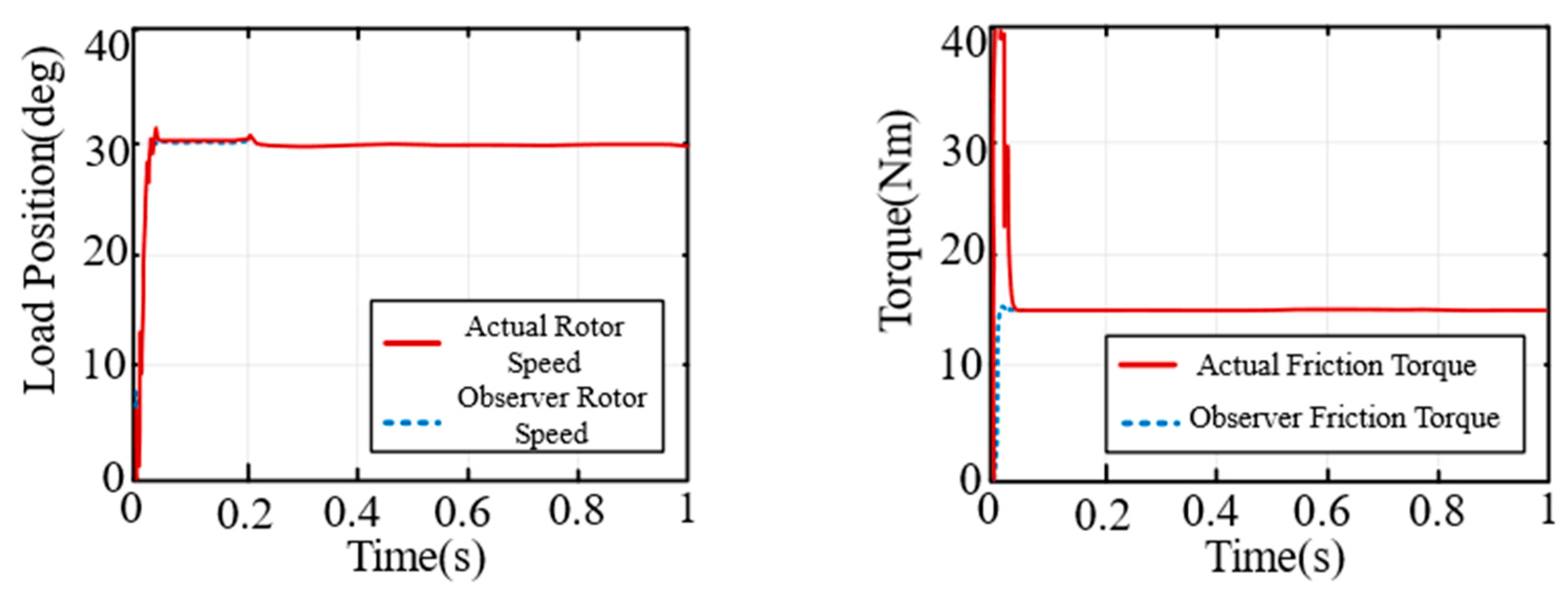
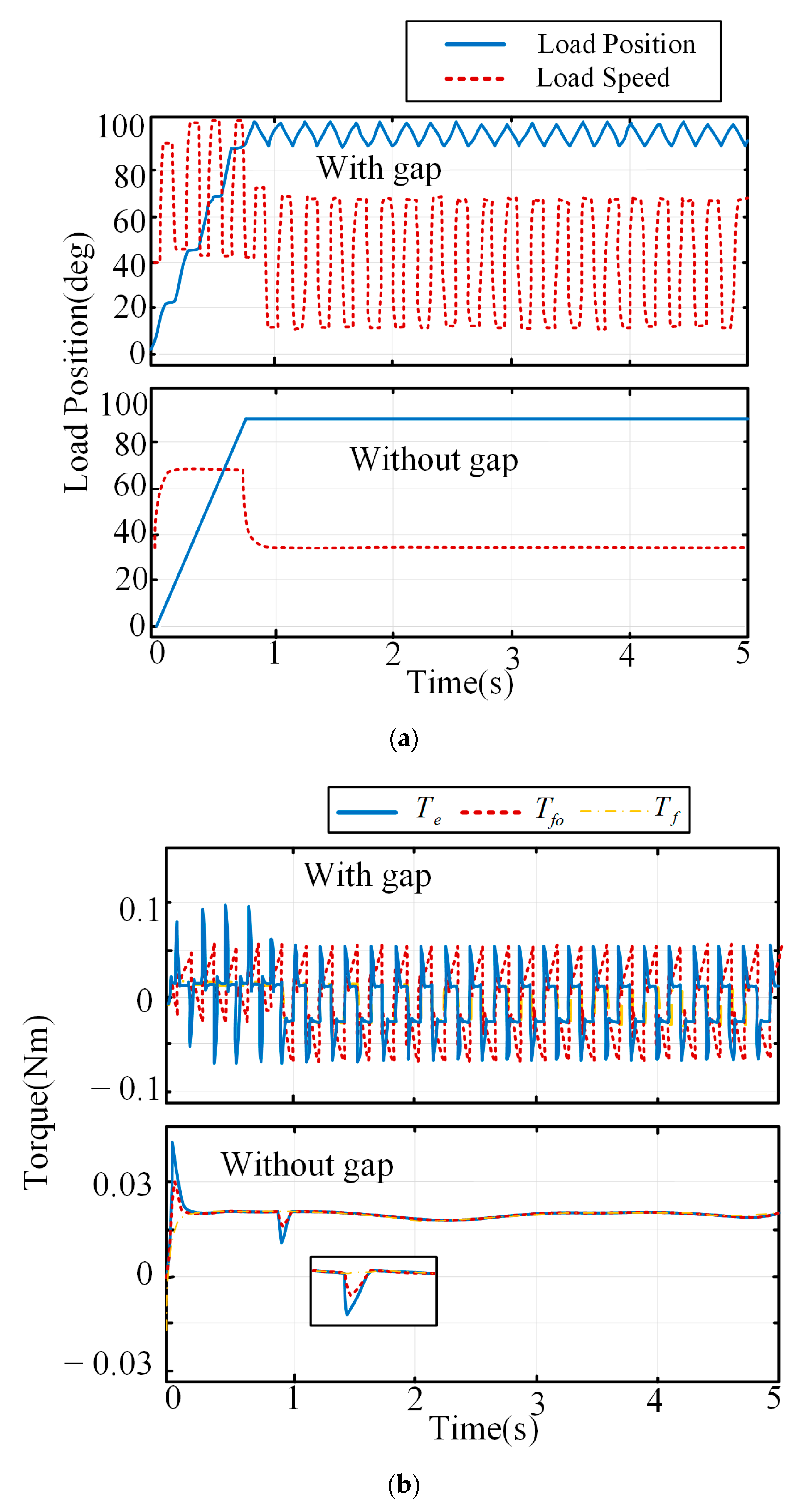
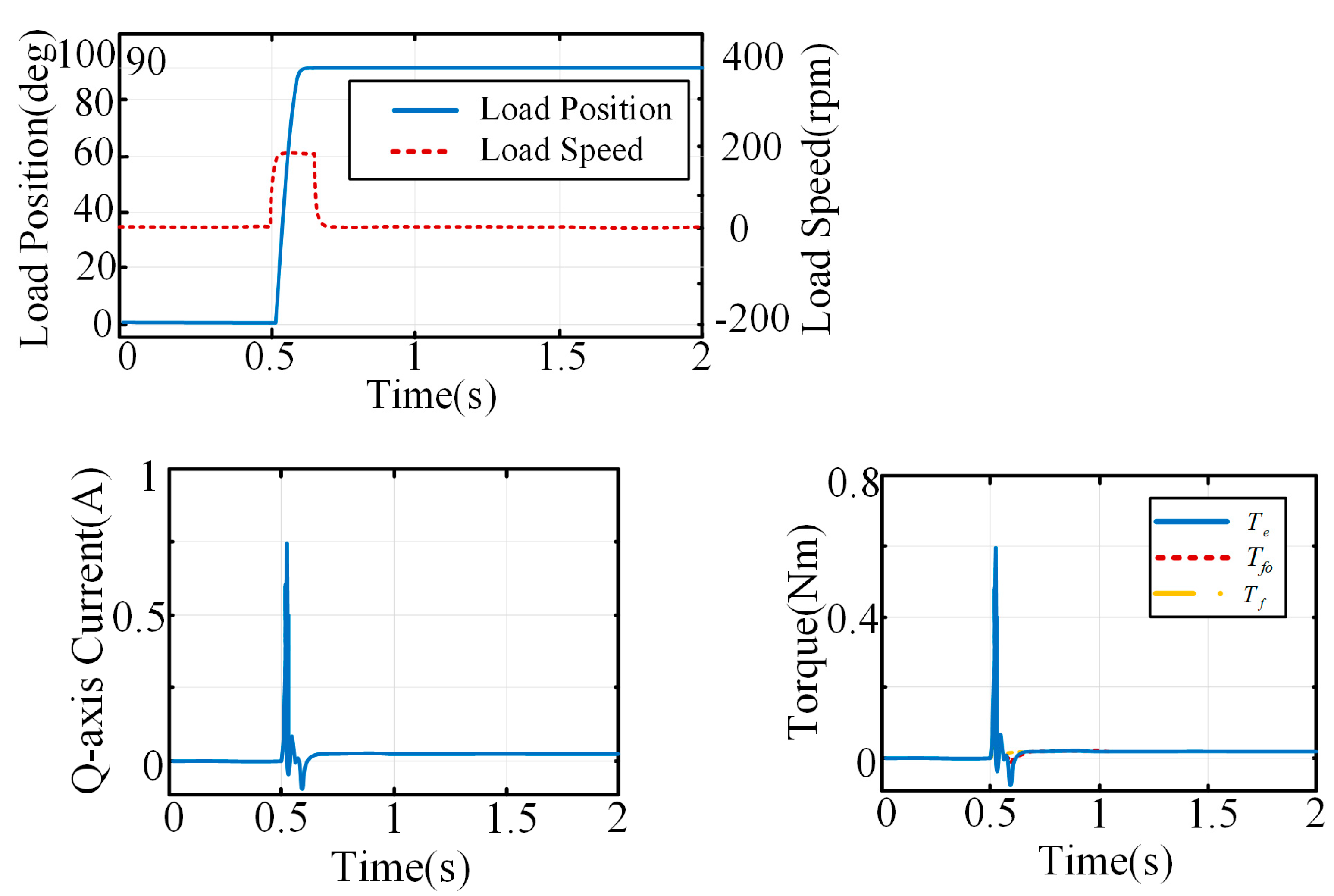
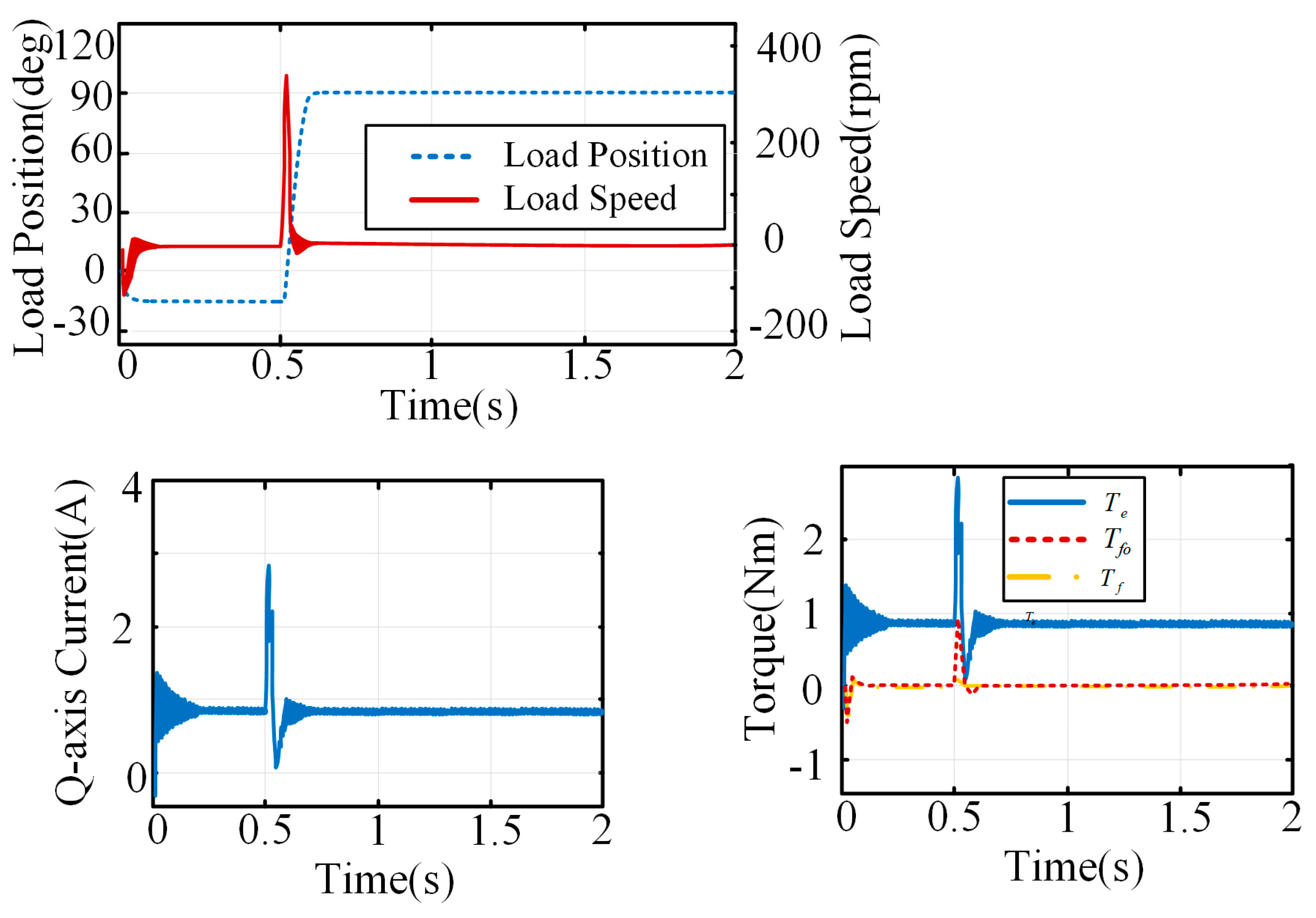
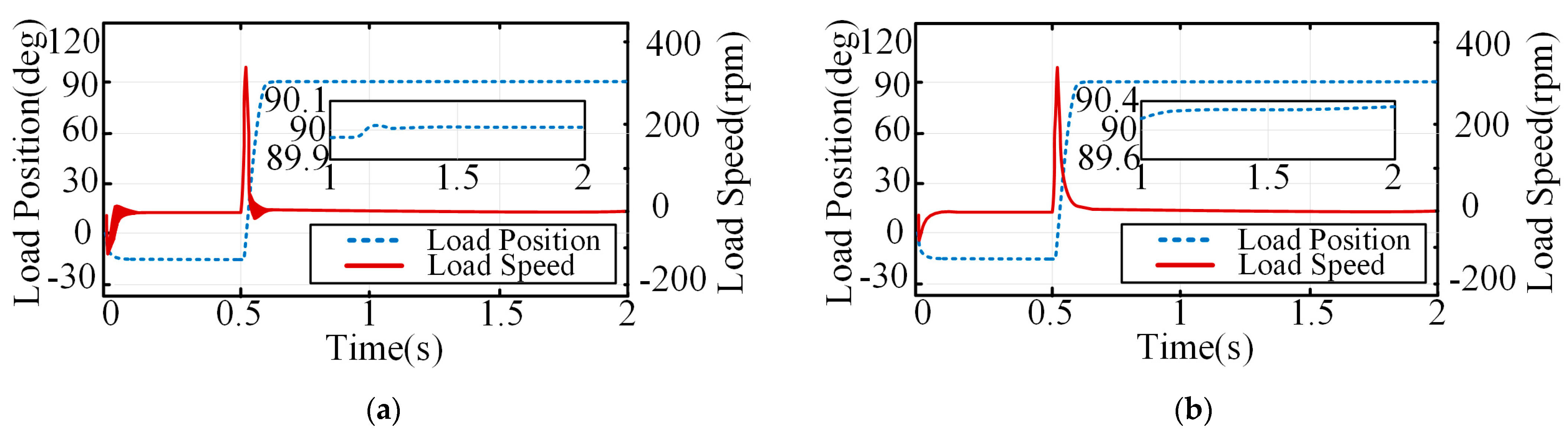
4.1. Simulation and Experimental Validation
4.2. Discussion of Simulation and Experiment
- (1).
- (2).
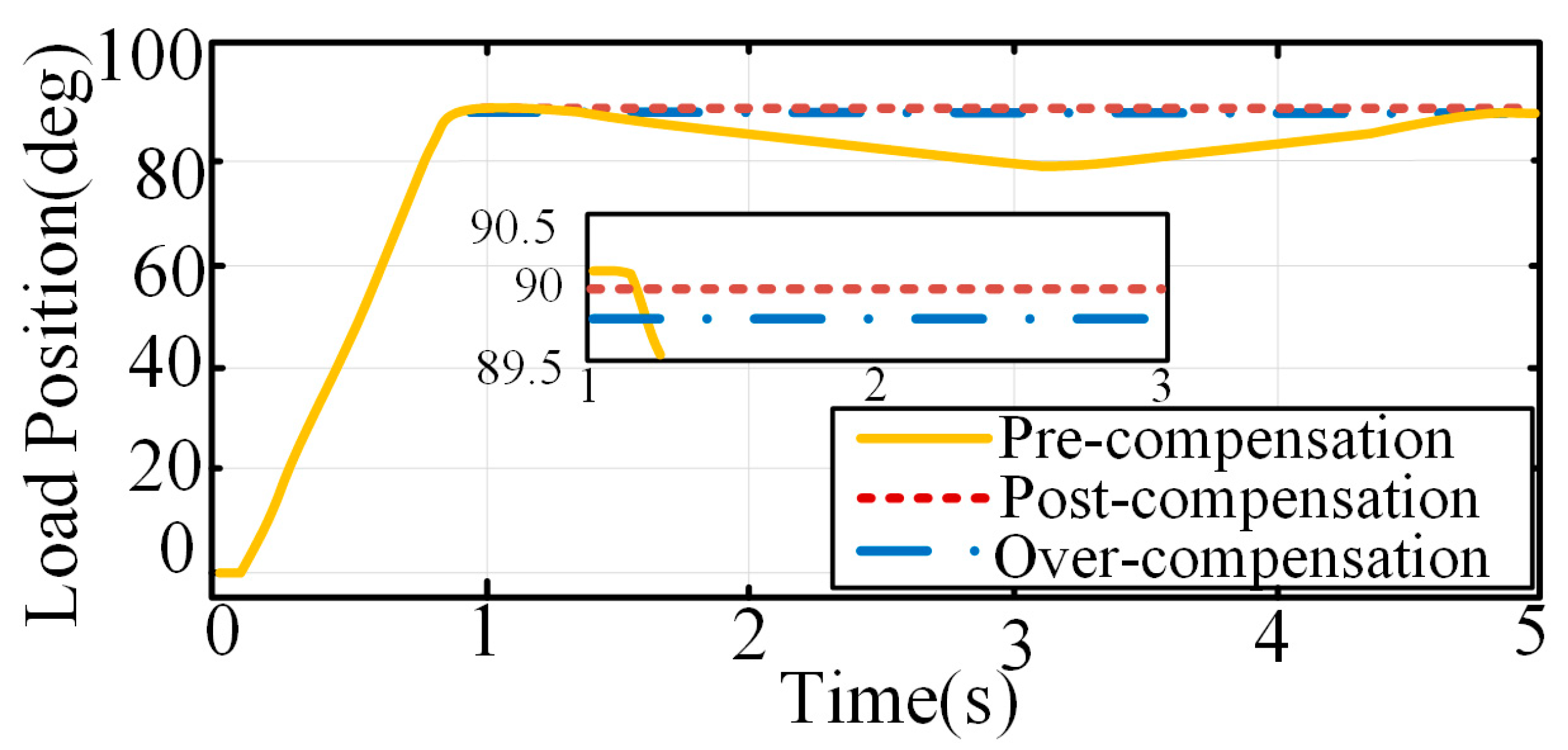
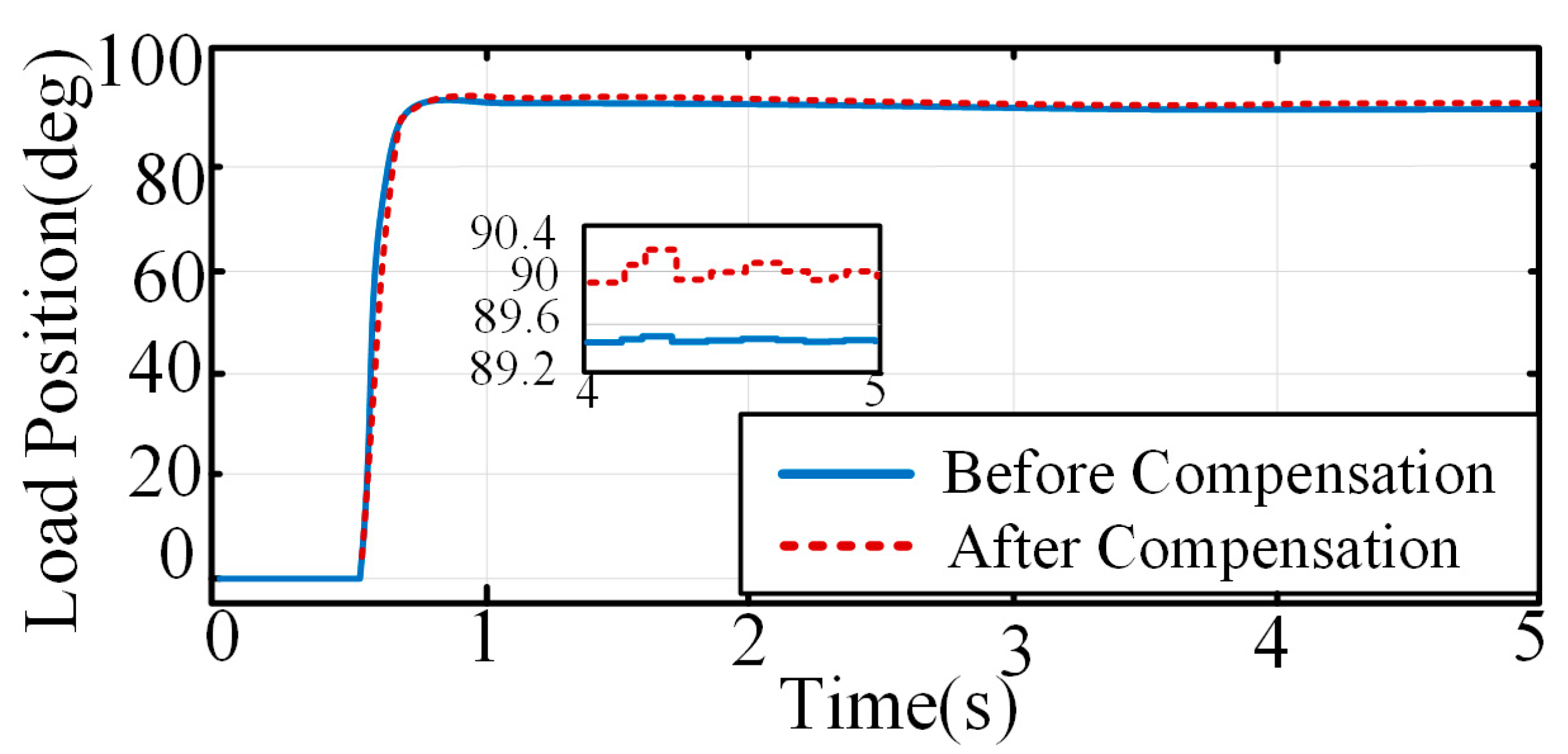
- As shown Figure 12, the friction parameters were varied and the system steady-state error was smaller, indicating that the compensation algorithm was equally effective in the case of time-varying friction.
- (3).
- As shown in Figure 13, when the friction parameters were not matched, the friction model feedforward compensation was added by the improved “LADRC + state feedback control”, and the steady-state error was much smaller than that of the “PI control + state feedback control”, which proves the effectiveness of the proposed new algorithm compared to the traditional PI control.
5. Conclusions
- (1).
- Feedforward compensation based on a friction model is highly dependent on the accuracy of parameter identification, and inaccuracies in the motor parameters trigger system oscillations.
- (2).
- With the application of the traditional ADRC algorithm in friction compensation, a small steady-state error and a rapid dynamic response can be reached. However, the compensation effect of the gap is not obvious, and the dynamic performance is seriously degraded when there is a gap in the transmission mechanism.
- (3).
- Introducing state feedback compensation on the basis of the traditional ADRC can effectively improve the positioning accuracy of the load side of the transmission system. The experimental steady state error was reduced from 0.55° to 1/3 (0.19°) after compensation. Moreover, the robustness of the improved compensation algorithm to the parameters is further improved for different load conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yu, H.; Gao, H.; Deng, H.; Yuan, S.; Zhang, L. Synchronization Control with Adaptive Friction Compensation of Treadmill-based Testing Apparatus for Wheeled Planetary Rover. IEEE Trans. Ind. Electron. 2022, 69, 592–603. [Google Scholar] [CrossRef]
- Véronneau, C.; Denis, J.; Lhommeau, P.; St-Jean, A.; Girard, A.; Plante, J.S.; Bigué, J.P.L. Modular Magnetorheological Actuator with High Torque Density and Transparency for the Collaborative Robot Industry. IEEE Robot. Autom. Lett. 2023, 8, 896–903. [Google Scholar] [CrossRef]
- Thenozhi, S.; Concha, A.; Resendiz, J.R. A Contraction Theory-based Tracking Control Design with Friction Identification and Compensation. IEEE Trans. Ind. Electron. 2022, 69, 6111–6120. [Google Scholar] [CrossRef]
- Qian, X.; Peiliang, W.; Zuxin, L. Parameter identification of permanent magnet servo system based on recursive least squares method. J. Electrotechnol. 2016, 31, 9. [Google Scholar]
- Liu, C.; Luo, G.; Duan, X.; Chen, Z.; Zhang, Z.; Qiu, C. Adaptive LADRC-Based Disturbance Rejection Method for Electromechanical Servo System. IEEE Trans. Ind. Appl. 2020, 56, 876–889. [Google Scholar] [CrossRef]
- Zhou, Z.; Guo, R. A Disturbance-Observer-Based Feedforward-Feedback Control Strategy for Driveline Launch Oscillation of Hybrid Electric Vehicles Considering Nonlinear Backlash. IEEE Trans. Veh. Technol. 2022, 71, 3727–3735. [Google Scholar] [CrossRef]
- Wang, G.; Liu, R.; Zhao, N.; Ding, D.; Xu, D. Enhanced linear ADRC strategy for HF pulse voltage signal injection based sensorless IPMSM drives. IEEE Trans. Power Electron. 2019, 34, 514–525. [Google Scholar] [CrossRef]
- Liang, L.H.; Wang, L.Y.; Wang, J.F. Energy-Saving Nonlinear Friction Compensation Method for Direct Drive Volume Control Flange-Type Rotary Vane Steering Gear. IEEE Access 2019, 7, 148351–148362. [Google Scholar] [CrossRef]
- Papadopoulos, E.G.; Chasparis, G.C. A Novel Friction Compensation Method Based on Stribeck Model With Fuzzy Filter for PMSM Servo Systems. IEEE Trans. Ind. Electron. 2023, 70, 12124–12133. [Google Scholar]
- Ahi, B.; Nobakhti, A. Hardware Implementation of an ADRC Controller on a Gimbal Mechanism. IEEE Trans. Control Syst. Technol. 2018, 26, 2268–2275. [Google Scholar] [CrossRef]
- Song, Z.; Mei, X.; Jiang, G. Inertia Identification based on Model Reference Adaptive System with Variable Gain for AC Servo Systems. In Proceedings of the International Conference on Mechatronics and Automation (ICMA), Takamatsu, Japan, 6–9 August 2017; pp. 188–192. [Google Scholar]
- Ren, C.; Li, X.; Yang, X.; Ma, S. Extended State Observer based Sliding Mode Control of an Omnidirectional Mobile Robot with Friction Compensation. IEEE Trans. Ind. Electron. 2019, 66, 9480–9489. [Google Scholar] [CrossRef]
- Ming, M.; Liang, W.; Ling, J.; Feng, Z.; Al Mamun, A.; Xiao, X. Composite Integral Sliding Mode Control with Neural Network-based Friction Compensation for a Piezoelectric Ultrasonic Motor. In Proceedings of the Annual Conference of the IEEE Industrial Electronics Society (IECON), Singapore, 18–21 October 2020; pp. 4397–4402. [Google Scholar]
- Tian, Y.-C.; Huang, S.; Liang, W.; Tan, K.K. Intelligent friction compensation: A review. IEEE/ASME Trans. Mechatron. 2019, 24, 1763–1774. [Google Scholar]
- Cui, N.; Zhang, Q.; Li, G. Servo rotary table friction modelling and compensation based on switching system theory. Micromotor 2019, 52, 6. [Google Scholar]
- Sun, L.; Li, X.; Chen, L.; Shi, H.; Jiang, Z. Dual-Motor Coordination for High-Quality Servo with Transmission Backlash. IEEE Trans. Ind. Electron. 2023, 70, 1182–1196. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, Z.; Xue, Y.; Li, D.; Li, Z. Design of linear self-immunity controller for high-order large-inertia systems. Control Decis. 2023, 38, 999–1007. [Google Scholar]
- Yang, M.; Ni, Q.; Liu, X.; Xu, D. Vibration suppression and over-quadrant error mitigation methods for a ball-screw driven servo system with dual-position feedback. IEEE Access 2020, 8, 213758–213771. [Google Scholar] [CrossRef]
- Beerens, R.; Bisoffi, A.; Zaccarian, L.; Nijmeijer, H.; Heemels, M.; van de Wouw, N. Reset PID design for motion systems with Stribeck friction. IEEE Trans. Control. Syst. Technol. 2022, 30, 294–310. [Google Scholar] [CrossRef]
- Yang, M.; Qu, W.Y.; Chen, Y.Y. Online inertia identification of servo system based on variable period recursive least squares and Kalman observer. J. Electrotechnol. 2018, 33, 367–376. [Google Scholar]
- Bu, F.; Yang, Z.; Gao, Y.; Pan, Z.; Pu, T.; Degano, M.; Gerada, C. Speed Ripple Reduction of Direct-Drive PMSM Servo System at Low-Speed Operation Using Virtual Cogging Torque Control Method. IEEE Trans. Ind. Electron. 2021, 68, 160–174. [Google Scholar] [CrossRef]






| Number of Experiments | Current Iq (A) | (Nm) | (Nm) |
|---|---|---|---|
| 1 | 0.051 | 0.041 | 0.043 |
| 2 | 0.057 | 0.046 | |
| 3 | 0.048 | 0.038 | |
| 4 | 0.069 | 0.055 | |
| 5 | 0.050 | 0.040 | |
| 6 | 0.049 | 0.039 | |
| 7 | 0.053 | 0.042 |
| Experimental Trials | Coulomb Friction Torque, Tc (Nm) | Viscous Friction Coefficient, Bv (Nm/(rad/s)) |
|---|---|---|
| 1 | ||
| 2 | ||
| Average value |
| Parameter | Numerical Value |
|---|---|
| Rated power (W) | 750 |
| Rated current (A) | 3 |
| Rated torque (Nm) | 2.39 |
| Number of pole pairs | 5 |
| ) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, M.; Liu, K.; Sohel, R.M.; Ji, R.; Ye, H. A Study of Friction Nonlinearity and Compensation for Turntable Servo Systems. Appl. Sci. 2024, 14, 8002. https://doi.org/10.3390/app14178002
Yan M, Liu K, Sohel RM, Ji R, Ye H. A Study of Friction Nonlinearity and Compensation for Turntable Servo Systems. Applied Sciences. 2024; 14(17):8002. https://doi.org/10.3390/app14178002
Chicago/Turabian StyleYan, Minjie, Kai Liu, Rana Md Sohel, Runze Ji, and Hairong Ye. 2024. "A Study of Friction Nonlinearity and Compensation for Turntable Servo Systems" Applied Sciences 14, no. 17: 8002. https://doi.org/10.3390/app14178002
APA StyleYan, M., Liu, K., Sohel, R. M., Ji, R., & Ye, H. (2024). A Study of Friction Nonlinearity and Compensation for Turntable Servo Systems. Applied Sciences, 14(17), 8002. https://doi.org/10.3390/app14178002







