Numerical and Experimental Analysis of SNCR Installation Performance in a Power Stoker Boiler
Abstract
:1. Introduction
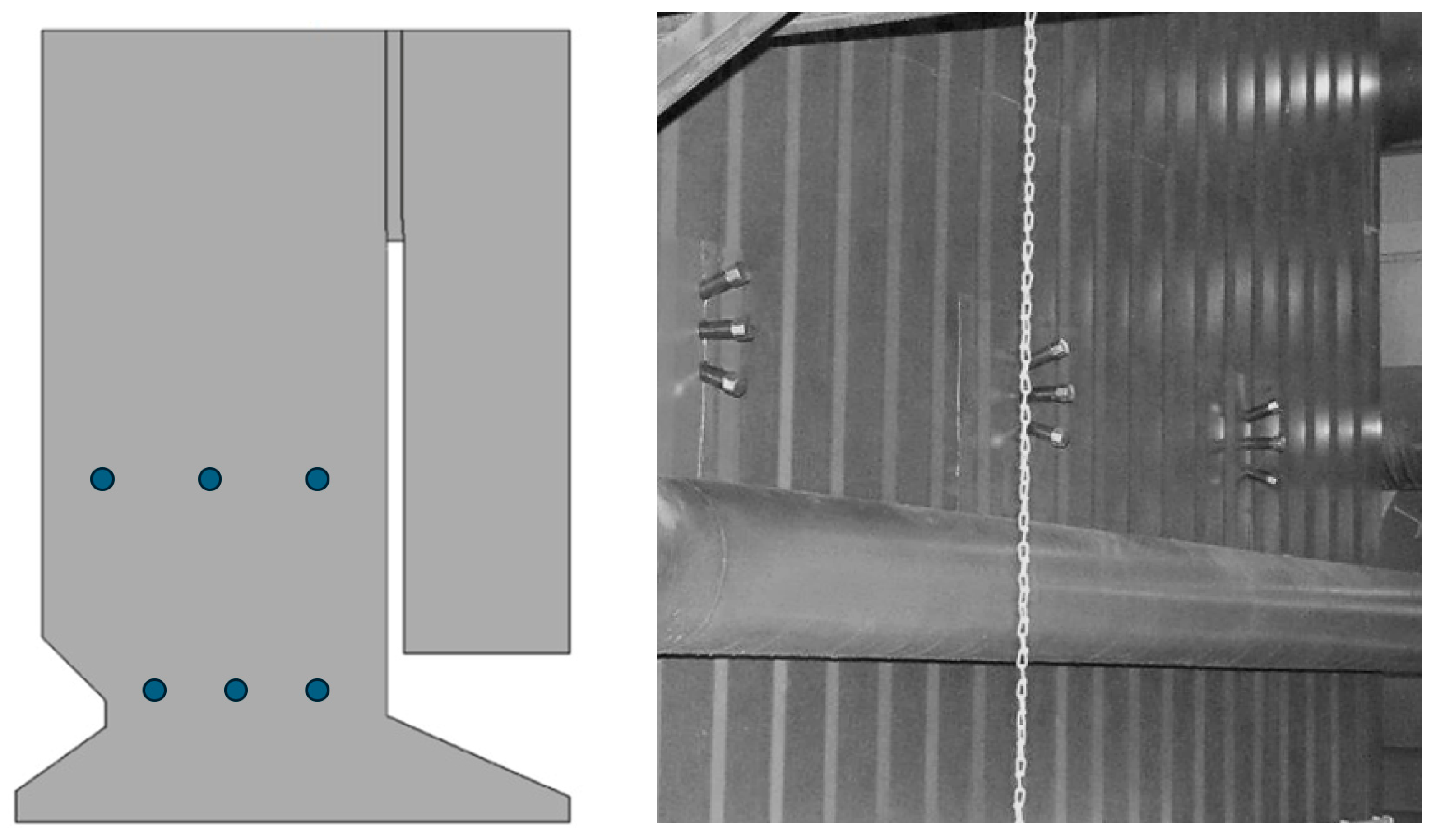
- The method of reagent distribution, specifically the design and operational parameters of the reagent injection lances;
- The levels at which the reagent is introduced into the boiler furnace chamber;
- The number of installed lances and their spacing on the boiler walls;
- The molar ratio of the reagent to the incoming NOx;
- The concentration of the reagent solution.
| Installation Type | Main Parameters | Aim of the Study | Ref. |
|---|---|---|---|
| Stoker boiler, coal, compressed urea-air injection | Injection velocity: 70 m/s | Innovative injector assesment | [6] |
| Cement industry, ammonia-based SNCR | 0.265 kg/s of ammonia water (concentration not given) | Ammonia slip assesment | [11] |
| 16 t/h waste incineration plant | Granular urea (1.5–2.2 mm), 3–6 injection points, 25 m/s | Innovative injection device testing | [12] |
| Circulating fluidized bed boiler, 140–200 MW, urea solution SNCR | Reagent concentration: 40%, mass flow rate: 310–330 kg/h | Particle swarm optimization algorithm development to model the SNCR | [13] |
| Circulating fluidized bed boiler, ammonia-based SNCR | Reduction rate: 30–75%, liquid pressure: 39.7–46.8 psi, flow rate: 0.14–0.25 gpm, air flow rate: 5.5–6.7 scfm | Spraying system optimization | [14] |
- The molar ratio of the reagent to the incoming NOx;
- The concentration of the reagent solution.
2. Methods
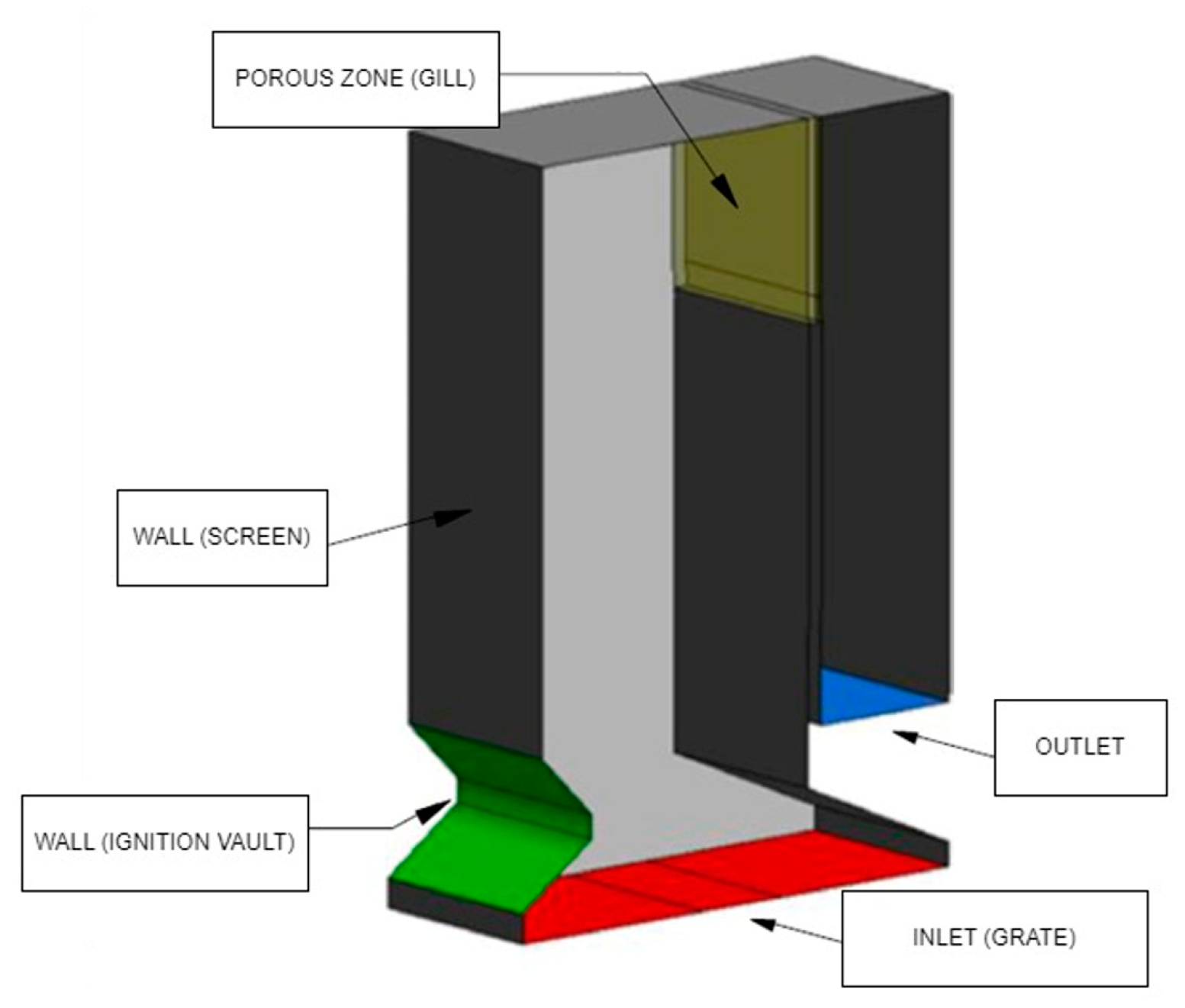
2.1. Boiler Characteristics
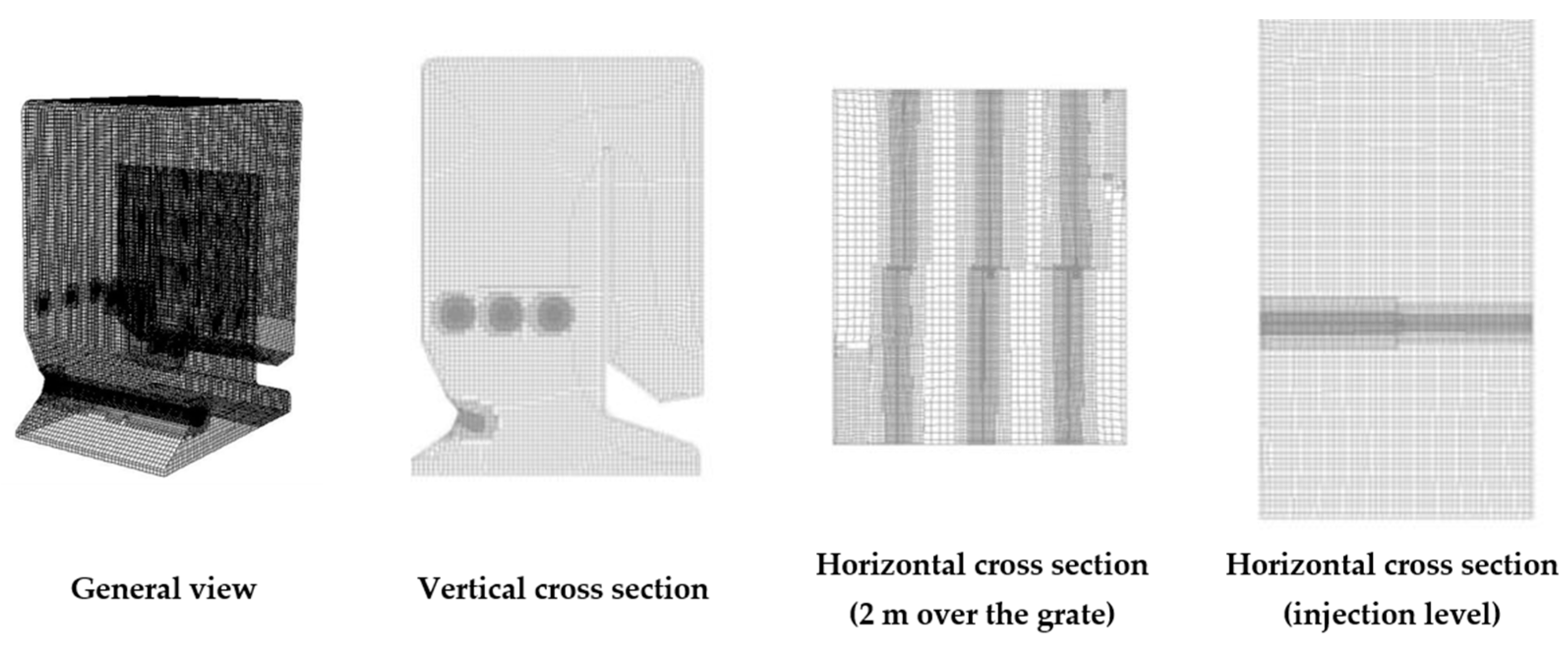
2.2. Mathematical Model
| Solver Type | Pressure-Based |
| Solver settings | Coupled, Pseudo Transient |
| Spatial Discretization Schemes | Gradient: Least Squares Cell-Based, Pressure: Standard; Density, Momentum, Turbulence: First Order Upwind, Species, Energy, DO: Second Order Upwind |
| Simulation type | Steady state |
| General models | Mass, heat, and momentum balance equations and energy equation |
| Turbulence model | RNG k-ε, Scalable Wall Functions, Differential Viscosity Model, Swirl Dominated Flow [16] |
| Radiation model | Discrete Ordinates, Theta Divisions 2, Phi Divisions 2, Theta Pixels 5, Phi Pixels 5 [18] |
| Gas model | Mixture Species: N2, O2, CO2, H2O; density: ideal gas; Cp: mixing law; thermal conductivity: ideal gas mixing law; Viscosity: Sutherland; Absorption Coefficient: WSGGM-user-specified; Scattering Coefficient: 0; Refractive Index: 1 [7,19]. |
| Droplets model | DPM, SSD breakup model [20,21], heat transfer between droplet and flue gases, evaporation |
| NOx reduction | Urea, 2-step decomposition, 7-step mechanism for SNCR [6] |
| Grate Zone | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| Emitted flue gases mass flow [kg/s] | 0.60 | 1.79 | 4.18 | 4.18 | 1.19 | 0.00 | 0.00 |
| Temperature [K] | 1620 | 1620 | 1620 | 1620 | 1620 | 773 | 773 |
| Mass fraction of O2 | 0.187 | 0.00 | 0.007 | 0.022 | 0.066 | 0.000 | 0.000 |
| Mass fraction of CO2 | 0.000 | 0.156 | 0.257 | 0.263 | 0.208 | 0.000 | 0.000 |
| Mass fraction of H2O | 0.188 | 0.180 | 0.030 | 0.000 | 0.000 | 0.000 | 0.000 |
| Mass fraction of NOx | 0.000134 | 0.00146 | 0.001415 | 0.000708 | 0.000114 | 0.000000 | 0.000000 |
| Overfire air mass flow [kg/s]; temperature [K] | OFA1/OFA2—0.75/0.75; 300 | ||||||
| Ignition vault temperature [K] | 970 | ||||||
| Ignition vault emissivity | 0.9 | ||||||
| Grate emissivity | 0.9 | ||||||
| Screen temperature [K] | 373 | ||||||
| Screen emissivity | 0.9 | ||||||
2.3. Bench Tests
2.3.1. Equipment Specification
- Reagent preparation unit;
- Feed and distribution module;
- Injection lances.
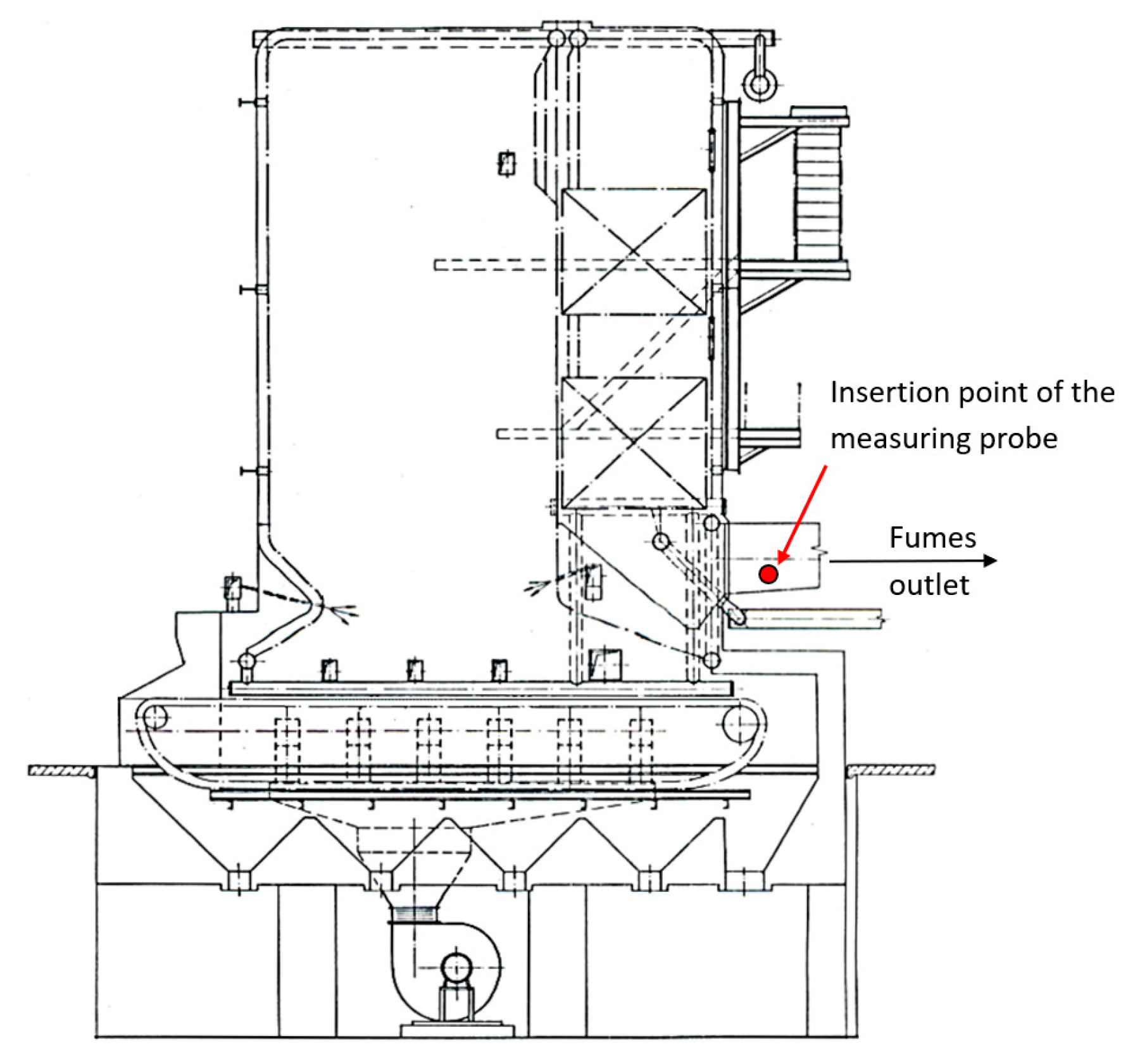
2.3.2. Measurement Procedure
- In the first step, the background was measured to determine the boiler’s steady operating conditions. The analyzer measured the concentration of NOx and other flue gas components for approximately half an hour. The measurement was averaged over time.
- In the second step, reagent injection was initiated. NOx concentration measurement started after a transition period from the reagent injection start. The plant operator observed the NOx rate of change in the flue gas in response to the reagent injection and decided whether steady-state conditions had already occurred.
- In the third step, the actual measurement period followed. The reagent injection at fixed set points (injection lance configuration, insertion points, and operating parameters) and the NOx concentration measurement in the exhaust gas took about 20–30 min.
- In the following steps, the operational parameters of the injection lances were changed (e.g., pressure, reagent output, and urea concentration). Re-measurement with the exhaust gas analyzer started each time after a transition period in which the operator had to confirm the steady-state conditions.
- In the final measurement stage, the background level of NOx concentration in the flue gas was again verified after stopping the reagent injection. This measurement was started when steady conditions were reached. The duration of the measurement was approximately 0.5 h.
3. Results
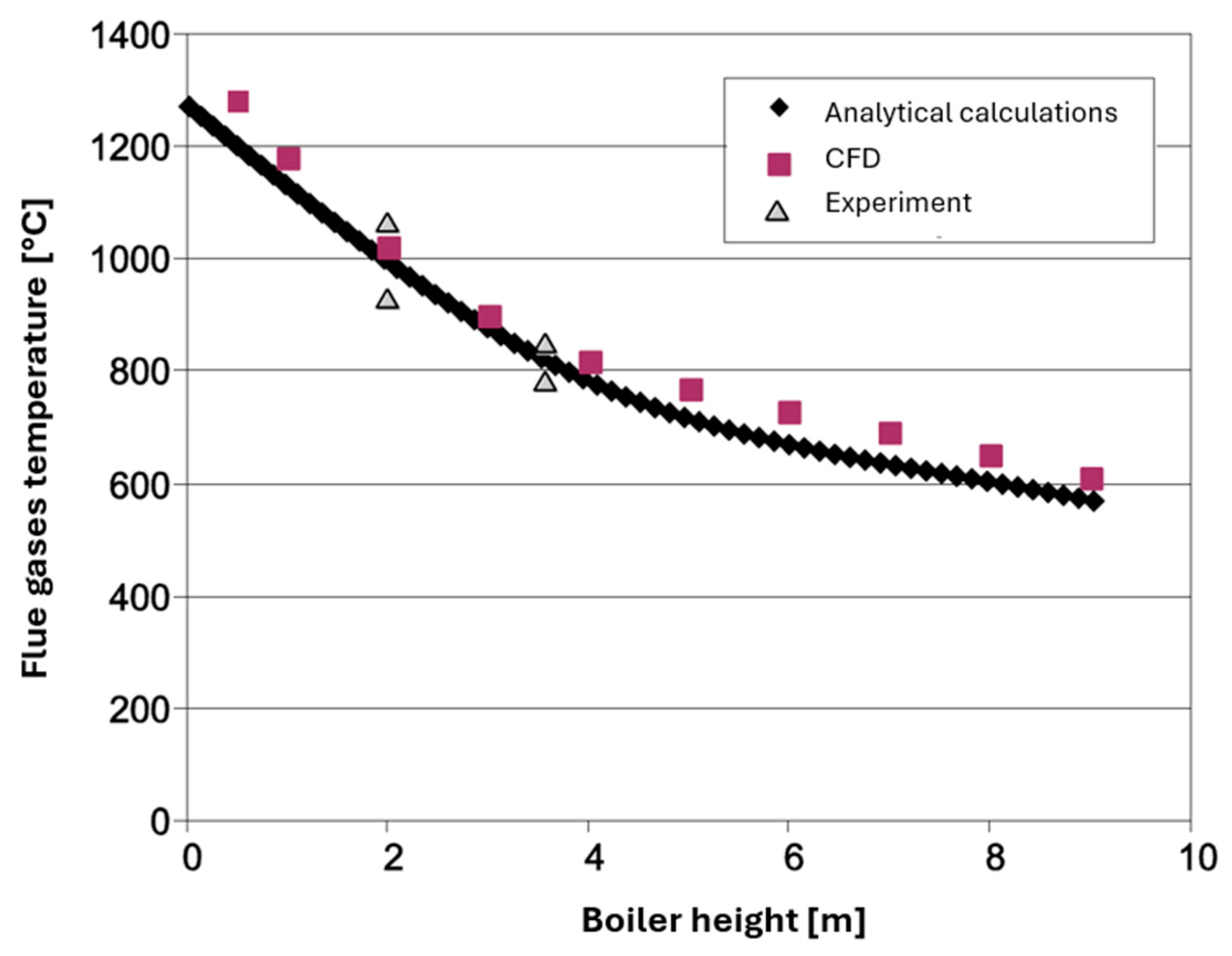
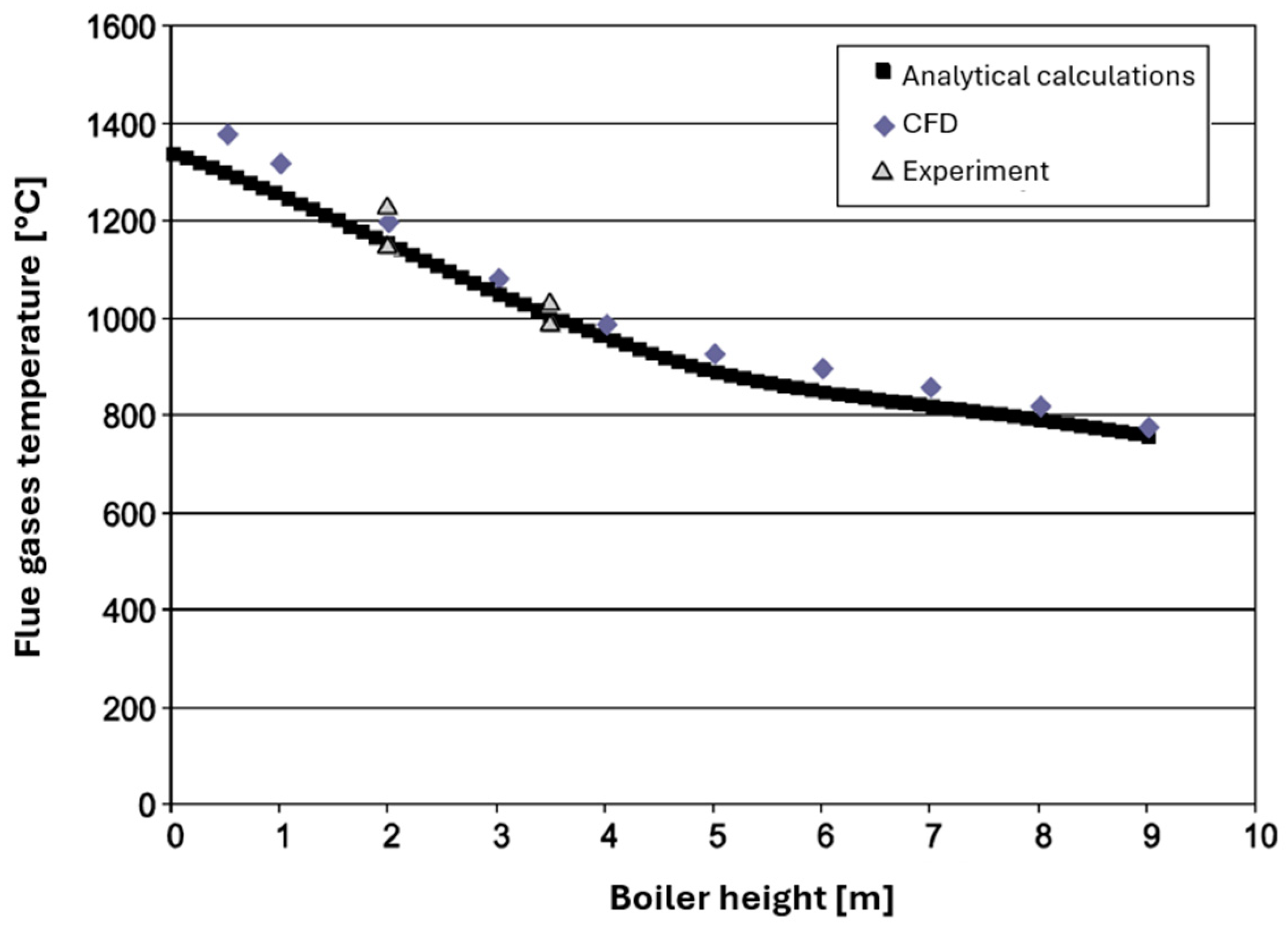
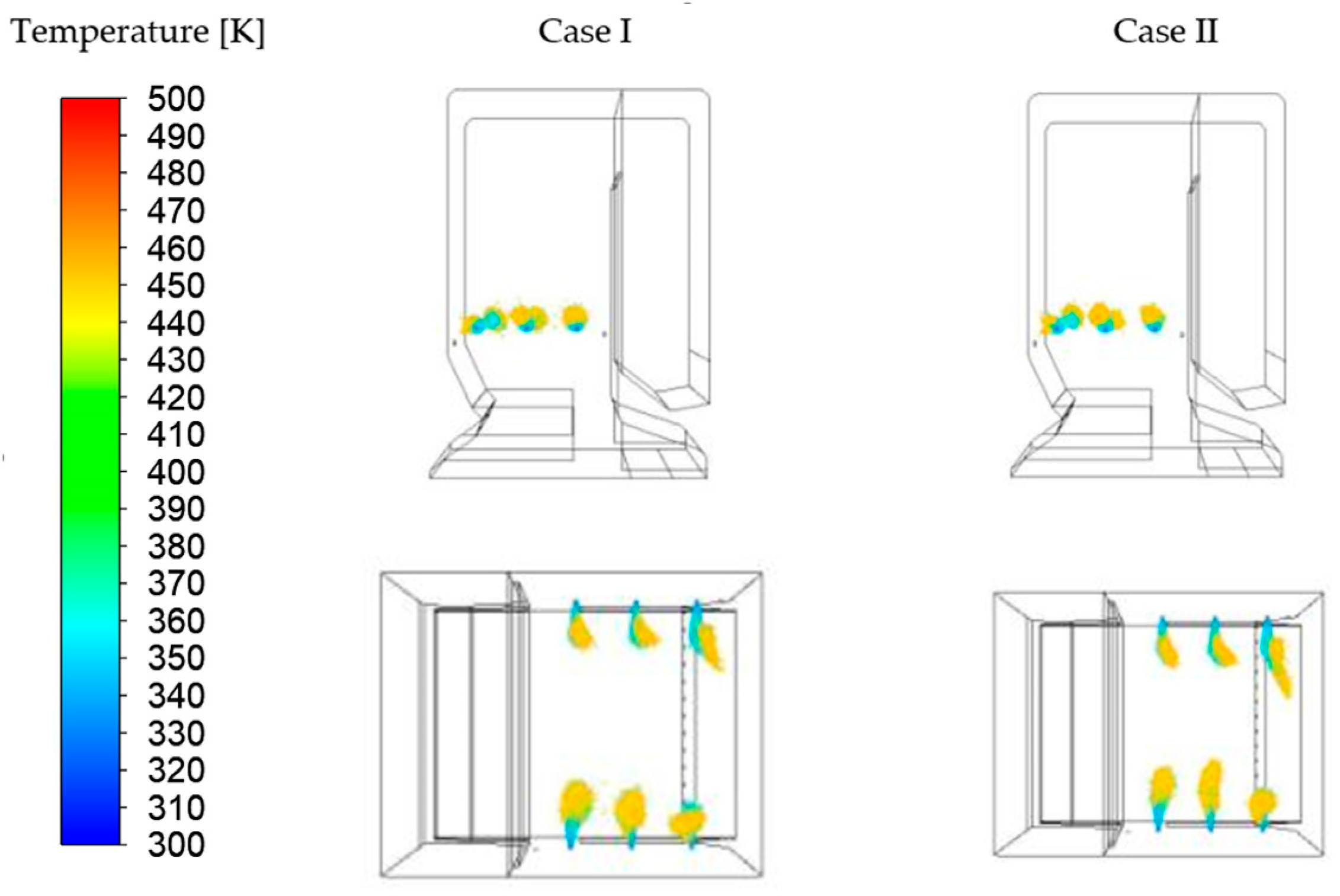
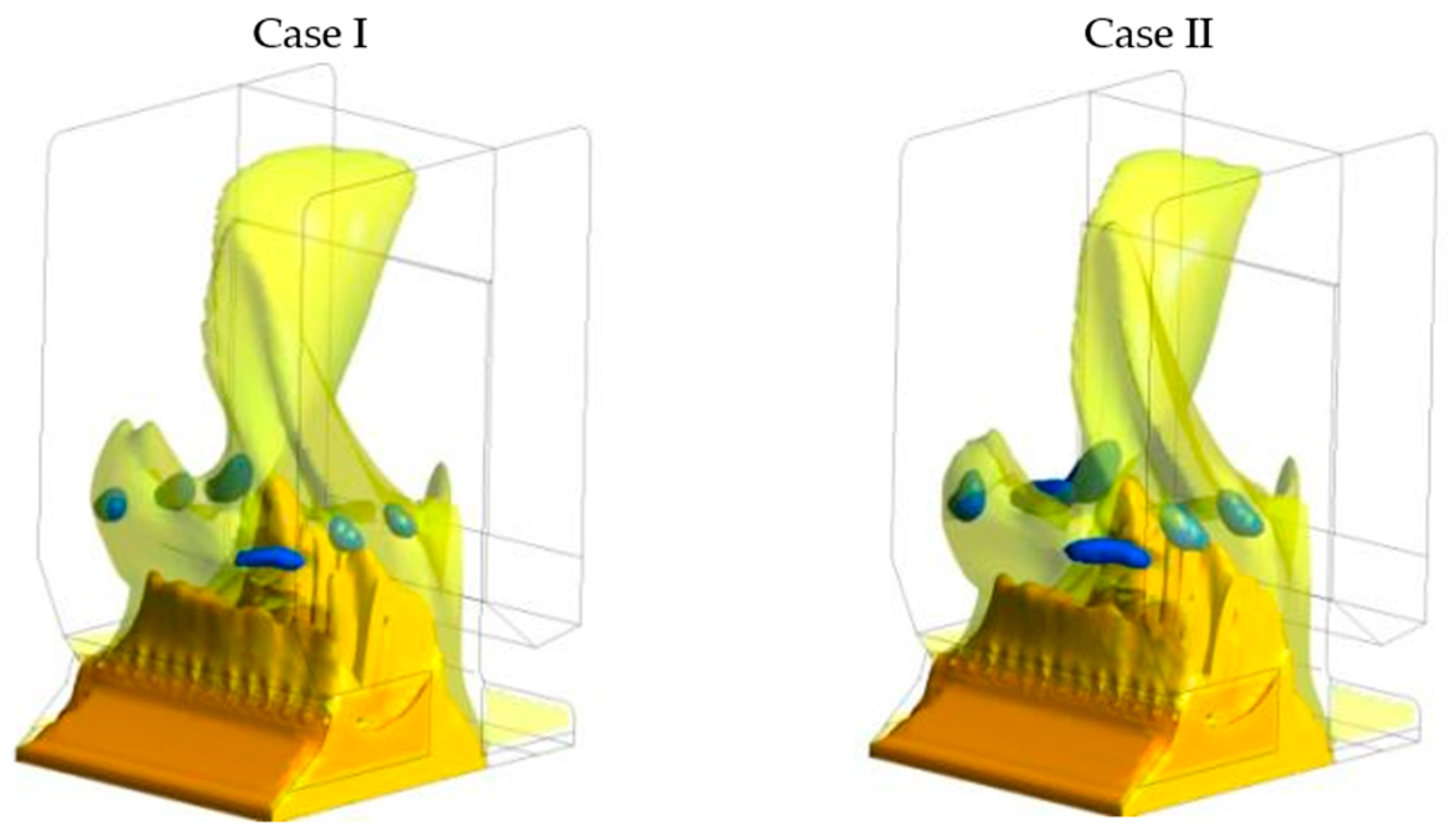
3.1. Mathematical Modeling Results
3.2. Tests Results
4. Discussion
5. Outcomes
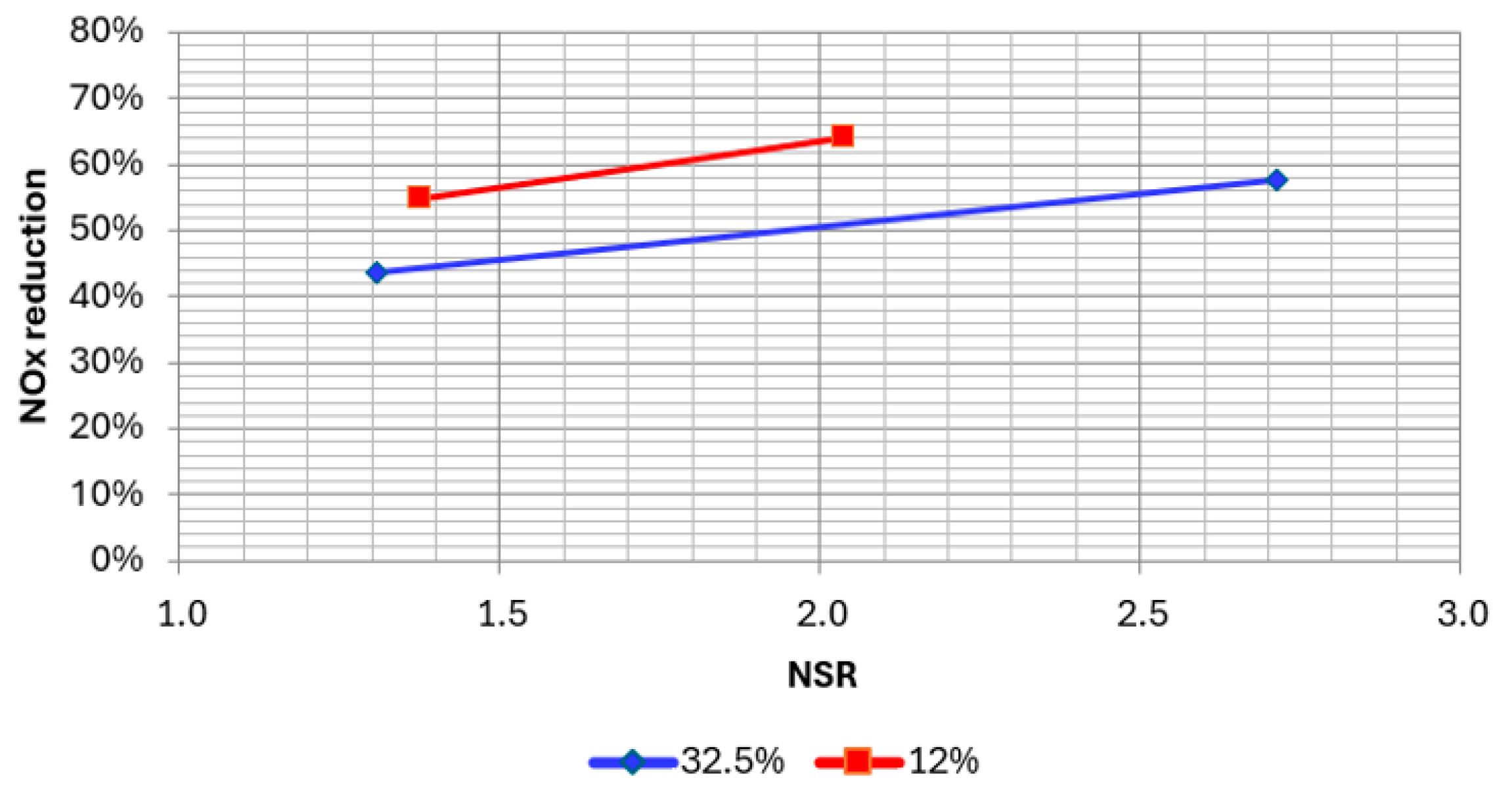
- Increasing NSR significantly enhances NOx reduction efficiency, especially between NSR 1 and 2, but further increases show diminishing returns.
- NOx reduction efficiency decreases with higher boiler power, likely due to shorter reagent residence time and difficulties maintaining optimal temperature conditions.
- Multi-hole nozzles (two- and three-holes) provide better NOx reduction compared to single-hole nozzles due to improved reagent distribution.
- Lower urea concentration (12%) in the reagent improves NOx reduction efficiency compared to higher concentrations (32.5%), as better mixing and droplet penetration are achieved.
- Optimal reagent injection should avoid the flame zone, ensuring it occurs in the correct temperature window for effective NOx reduction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wrzesińska, B.; Krzywda, R.; Wąsowski, T.; Krawczyk, P.; Badyda, K. A selective non-catalytic reduction of nitrogen oxides technology for application in industrial and municipal heating boilers. Przem. Chem. 2015, 1, 182–187. (In Polish) [Google Scholar]
- Lyon, R.K. The NH3-NO-O2 reaction. Int. J. Chem. Kinet. 1976, 8, 315–318. [Google Scholar] [CrossRef]
- Tayyeb Javed, M.; Nimmo, W.; Mahmood, A.; Irfan, N. Effect of oxygenated liquid additives on the urea based SNCR process. J. Environ. Manag. 2009, 90, 3429–3435. [Google Scholar] [CrossRef] [PubMed]
- Cai, J.; Zheng, W.; Wang, Q. Effects of hydrogen peroxide, sodium carbonate, and ethanol additives on the urea-based SNCR process. Sci. Total Environ. 2021, 772, 145551. [Google Scholar] [CrossRef] [PubMed]
- Lin, T.; Liao, Y.F.; Dai, T.H.; Ma, X.Q. Co-disposal technology for sludge and municipal solid waste based on SNCR optimization. Energy 2024, 292, 130582. [Google Scholar] [CrossRef]
- Garbacz, P.; Wejkowski, R. Numerical research on the SNCR method in a grate boiler equipped with the innovative FJBS system. Energy 2020, 207, 118240. [Google Scholar] [CrossRef]
- Krawczyk, P.; Kurkus-Gruszecka, M.; Dzido, A.; Badyda, K. A method of designing an SNCR installation for small pulverized coal-fired boilers on the example of OP-50 boiler. Appl. Therm. Eng. 2023, 235, 121321. [Google Scholar] [CrossRef]
- Krawczyk, P.; Badyda, K.; Młynarz, S. Effect of the air to water ratio on the performance of internal mixing two-fluid atomiser. Chem. Process Eng. 2016, 37, 461–471. [Google Scholar] [CrossRef]
- Nguyen, T.D.B.; Kang, T.H.; Lim, Y.I.; Eom, W.H.; Kim, S.J.; Yoo, K.S. Application of urea-based SNCR to a municipal incinerator: On-site test and CFD simulation. Chem. Eng. J. 2009, 152, 36–43. [Google Scholar] [CrossRef]
- Xia, Z.; Li, J.; Wu, T.; Chen, C.; Zhang, X. CFD simulation of MSW combustion and SNCR in a commercial incinerator. Waste Manag. 2014, 34, 1609–1618. [Google Scholar] [CrossRef]
- Guo, Y.; Mu, B.; Liu, P.; Luo, L.; Hao, L.; Li, Y.; Zhu, T. Ammonia emission estimation for the cement industry in northern China. Atmos. Pollut. Res. 2020, 11, 1738–1742. [Google Scholar] [CrossRef]
- Fraysse, G.; Venditti, D.; Durécu, S. An innovative injection device to enhance NOx abatement by SNCR in waste combustion flue-gases. High Temp. Mater. Process. 2008, 27, 383–390. [Google Scholar] [CrossRef]
- Bai, J.; Lei, X.; Wang, Q. Modeling of SNCR denitration system based on adaptive weight particle swarm optimization. IOP Conf. Ser. Earth Environ. Sci. 2020, 467, 012118. [Google Scholar] [CrossRef]
- Li, F.; Brown, K.J.; Schick, R.J.; Shen, J. SNCR Efficiency Study of Optimization of Spray Systems in CFB. In Proceedings of the ILASS Americas 27th Annual Conference on Liquid Atomization and Spray Systems, Raleigh, NC, USA, 17–20 May 2015. [Google Scholar]
- Więckowski, Ł.; Krawczyk, P.; Badyda, K. Numerical investigation of temperature distribution in the furnace of a coal fired grate boiler in part load conditions. J. Power Technol. 2018, 97, 359–365. [Google Scholar]
- Świeboda, T.; Krzyżyńska, R.; Bryszewska-Mazurek, A.; Mazurek, W.; Czapliński, T.; Przygoda, A. Advanced approach to modeling of pulverized coal boilers for SNCR process optimization—Review and recommendations. Int. J. Thermofluids 2020, 7–8, 100051. [Google Scholar] [CrossRef]
- Hu, Z.; Jiang, E.; Ma, X. Numerical simulation on operating parameters of SNCR process in a municipal solid waste incinerator. Fuel 2019, 245, 160–173. [Google Scholar] [CrossRef]
- Scharler, R.; Obernberger, I. Numerical modelling of biomass grate furnaces. In Proceedings of the 5th European Conference on Industrial Furnaces and Boilers, Porto, Portugal, 11–14 April 2000. 17p. [Google Scholar]
- Gómez, M.A.; Porteiro, J.; Patiño, D.; Míguez, J.L. CFD modelling of thermal conversion and packed bed compaction in biomass combustion. Fuel 2014, 117, 716–732. [Google Scholar] [CrossRef]
- Sonawane, U.; Agarwal, A.K. Spray Breakup Modelling for Internal Combustion Engines. In Engine Modeling and Simulation; Springer: Berlin/Heidelberg, Germany, 2022; pp. 57–85. [Google Scholar] [CrossRef]
- Krawczyk, P.; Badyda, K. Numerical analysis of the impact of parameters of urea solution injection on reagent penetration inside the combustion chamber of A WR 25 boiler. Rynek Energii 2014, 115, 139–145. [Google Scholar] [CrossRef]
- ur Rahman, Z.; Wang, X.; Zhang, J.; Baleta, J.; Vujanović, M.; Tan, H. Kinetic study and optimization on SNCR process in pressurized oxy-combustion. J. Energy Inst. 2021, 94, 263–271. [Google Scholar] [CrossRef]
- Hernik, B. Numerical Research of Flue Gas Denitrification Using the SNCR Method in an OP 650 Boiler. Energies 2022, 15, 3427. [Google Scholar] [CrossRef]
- Liu, Y.; Kao, H. Numerical simulation of urea based SNCR process in a trinal-sprayed precalciner. J. Renew. Mater. 2021, 9, 269–294. [Google Scholar] [CrossRef]
- Park, P.M.; Park, Y.K.; Dong, J.I. Reaction characteristics of NOx and N2O in selective non-catalytic reduction using various reducing agents and additives. Atmosphere 2021, 12, 1175. [Google Scholar] [CrossRef]










| Carbon (C) | 0.550 |
| Hydrogen (H) | 0.035 |
| Oxygen (O) | 0.115 |
| Nitrogen (N) | 0.020 |
| Sulphur (S) | 0.010 |
| Ash | 0.135 |
| Moisture (H2O) | 0.135 |
| Volatile matter | 0.32 |
| Fixed carbon | 0.41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krawczyk, P.; Kurkus-Gruszecka, M.; Dzido, A. Numerical and Experimental Analysis of SNCR Installation Performance in a Power Stoker Boiler. Appl. Sci. 2024, 14, 8508. https://doi.org/10.3390/app14188508
Krawczyk P, Kurkus-Gruszecka M, Dzido A. Numerical and Experimental Analysis of SNCR Installation Performance in a Power Stoker Boiler. Applied Sciences. 2024; 14(18):8508. https://doi.org/10.3390/app14188508
Chicago/Turabian StyleKrawczyk, Piotr, Michalina Kurkus-Gruszecka, and Aleksandra Dzido. 2024. "Numerical and Experimental Analysis of SNCR Installation Performance in a Power Stoker Boiler" Applied Sciences 14, no. 18: 8508. https://doi.org/10.3390/app14188508
APA StyleKrawczyk, P., Kurkus-Gruszecka, M., & Dzido, A. (2024). Numerical and Experimental Analysis of SNCR Installation Performance in a Power Stoker Boiler. Applied Sciences, 14(18), 8508. https://doi.org/10.3390/app14188508






