Characterization of Seismic Signal Patterns and Dynamic Pore Pressure Fluctuations Due to Wave-Induced Erosion on Non-Cohesive Slopes
Abstract
1. Introduction
2. Methods
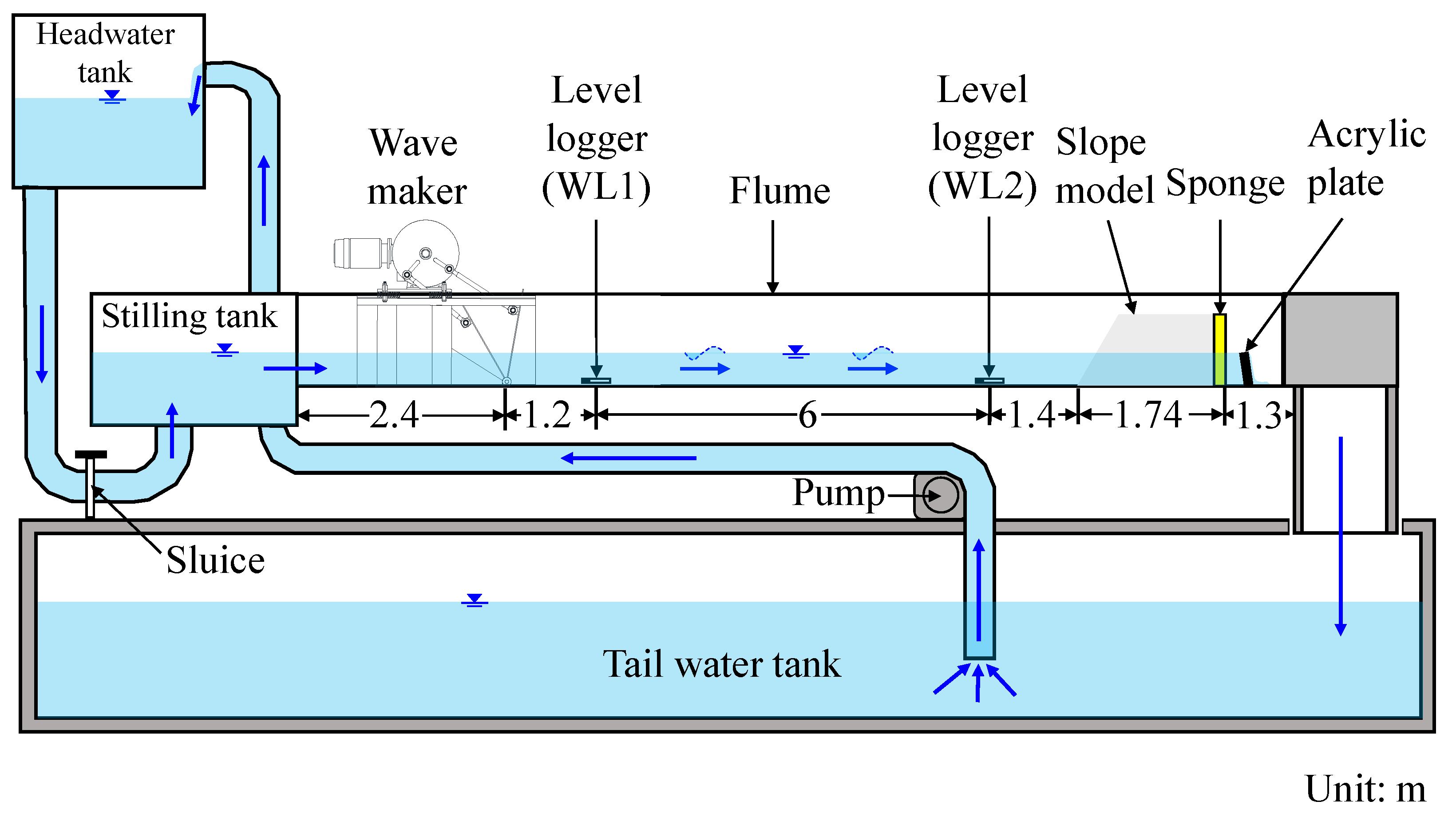
2.1. Flume Configuration
2.2. Slope Model and Layout of the Sensors
2.3. Data Analysis
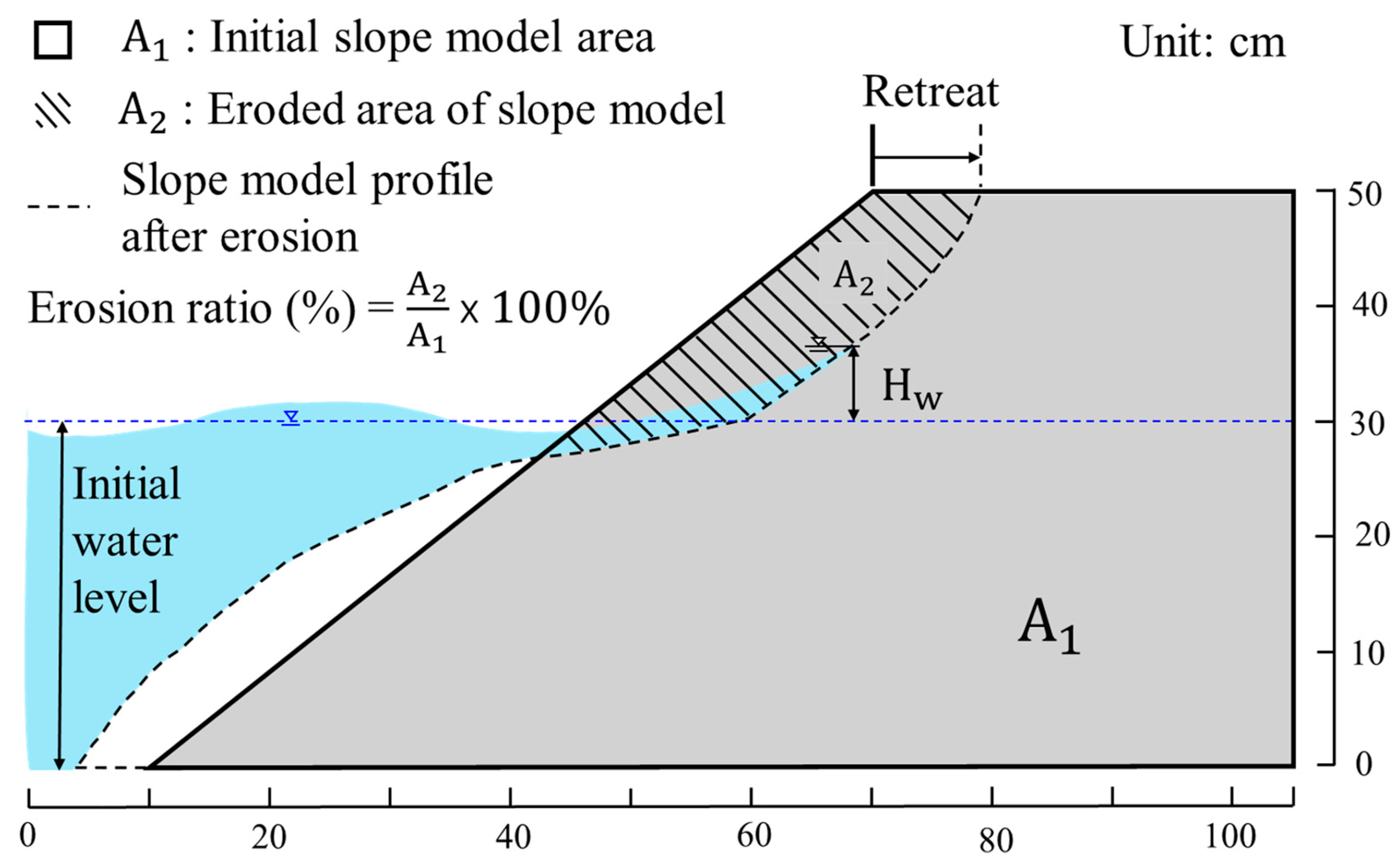
2.3.1. Erosion Ratio, the Run-Up Height of Wave and Retreat of Slope Model
2.3.2. Significant Wave Height, Hs
2.3.3. Hilbert–Huang Transform, HHT
2.3.4. Power Spectral Density
2.3.5. Arias Intensity, IA
2.4. Setup of the Model Tests
2.5. Test Procedures
- (1)
- Build the slope model, installing sensors and cameras.
- (2)
- Let the slope model sit for 5 h, allowing the water inside the slope model to naturally drain.
- (3)
- Once the slope model has settled, pump water from the reservoir into the flume’s headwater until a sufficient volume is reached.
- (4)
- Begin recording data (water level in the flume, seismic signal, acoustic signal, pore pressure, and video).
- (5)
- Open the sluice to release water into the flume until the water level reaches 0.3 m.
- (6)
- Allow the slope model to sit until the water level in the slope model also reaches 0.3 m.
- (7)
- Turn on the wave maker motor and record the seismic and acoustic signals due to the motor for analyzing its noise.
- (8)
- Start the test. Turn on the wave maker to create waves that erode the slope model.
- (9)
- When the slope profile reaches a state of equilibrium, stop the wave maker, and the test ends.
3. Results and Discussion
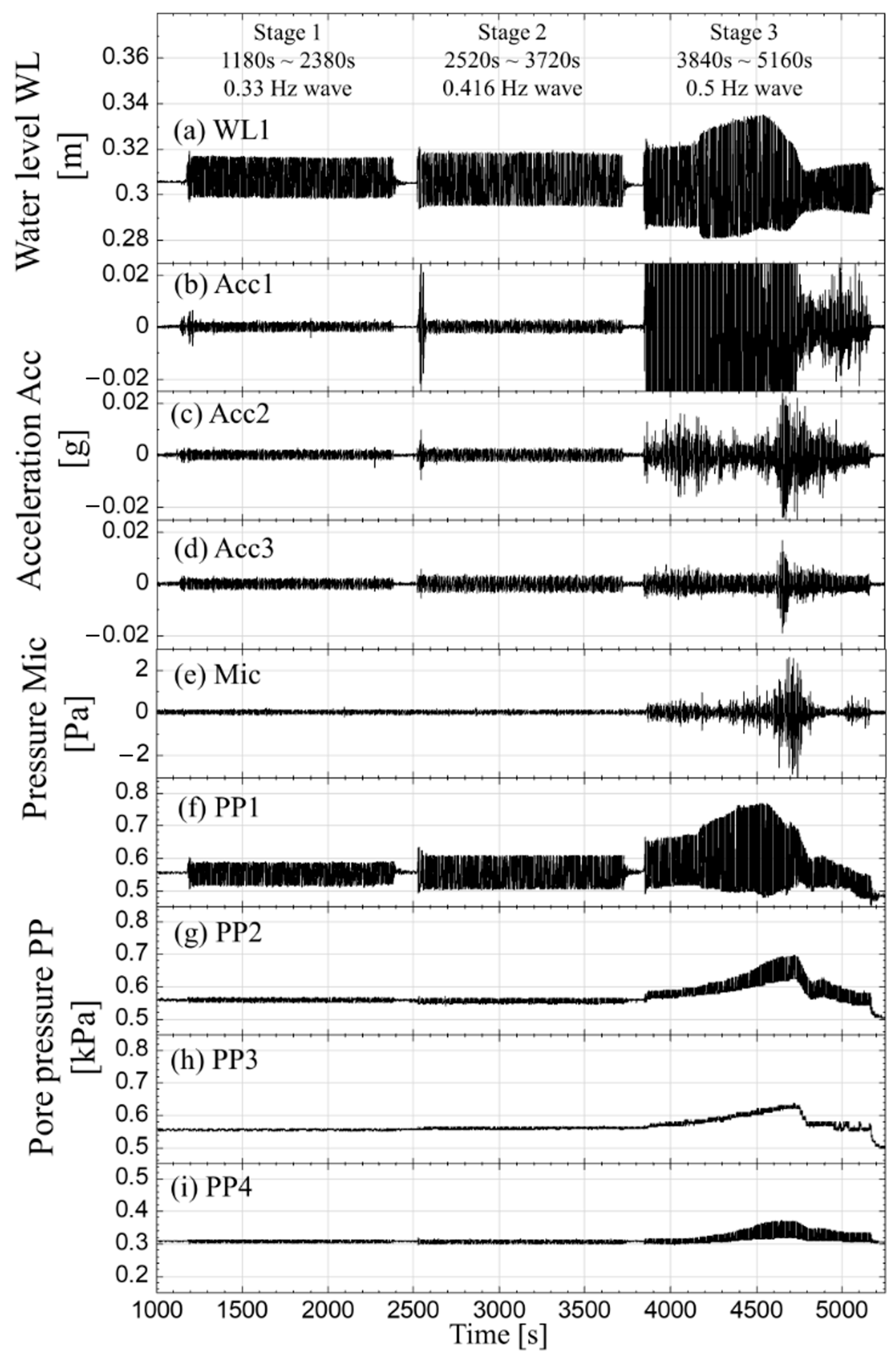
3.1. Overall Interpretations of the Results of Baseline Case
- (1)
- Water height variations: The water height is stable in Stages 1 and 2, but there are significant variations in Stage 3 due to slope profile changes affecting wave reflection.
- (2)
- Seismic signals: The amplitude of seismic signals increases with wave height, and accelerometers closer to the slope will record higher readings.
- (3)
- Acoustic signals: The acoustic signals are “quieter” in Stages 1 and 2 and are “louder” with more variations in Stage 3 due to increased wave breaking and splashing.
- (4)
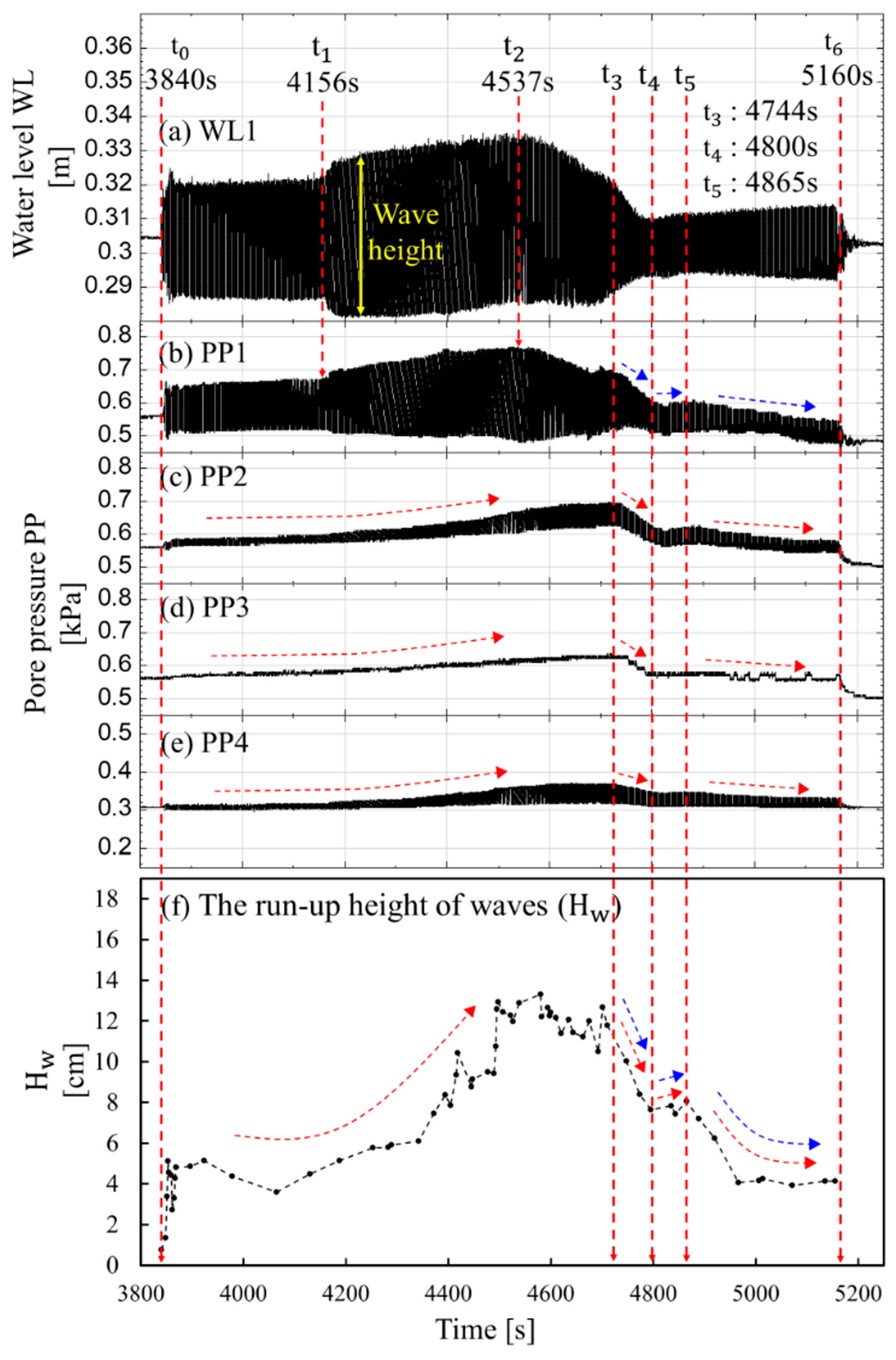
- Pore pressures: The pore pressures in the slope are stable in the early stages and have significant changes in Stage 3. Pore pressure changes reflect variations in wave height and Hw, with higher readings when the piezometer is near the eroded slope face. Higher wave height and Hw raise the water level inside the slope, increasing pore pressure.
- (5)
- Geometry changes in slope: A change in the slope’s geometry due to wave erosion affects wave energy dissipation, influencing water levels above the piezometers and altering pore pressure.
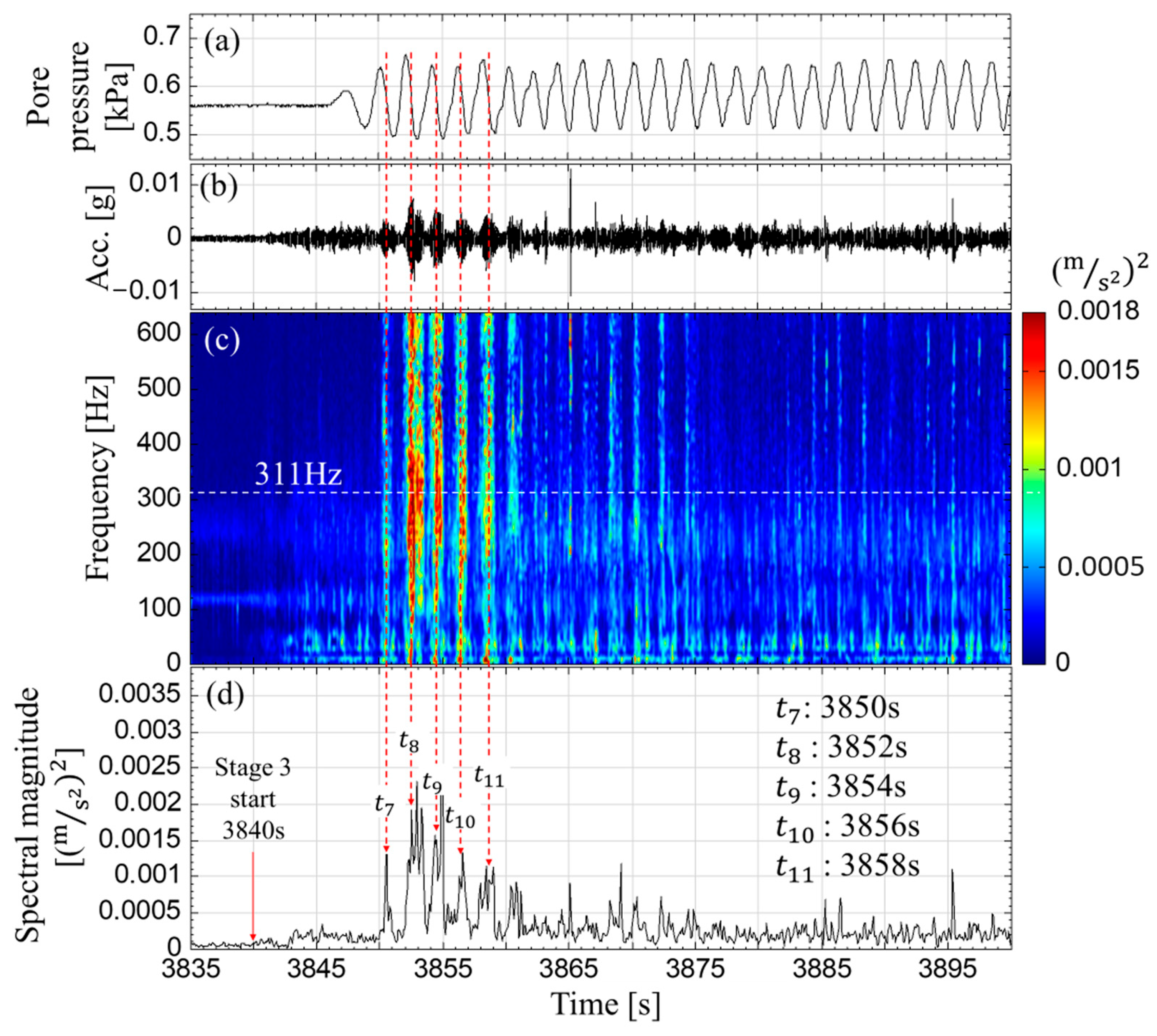
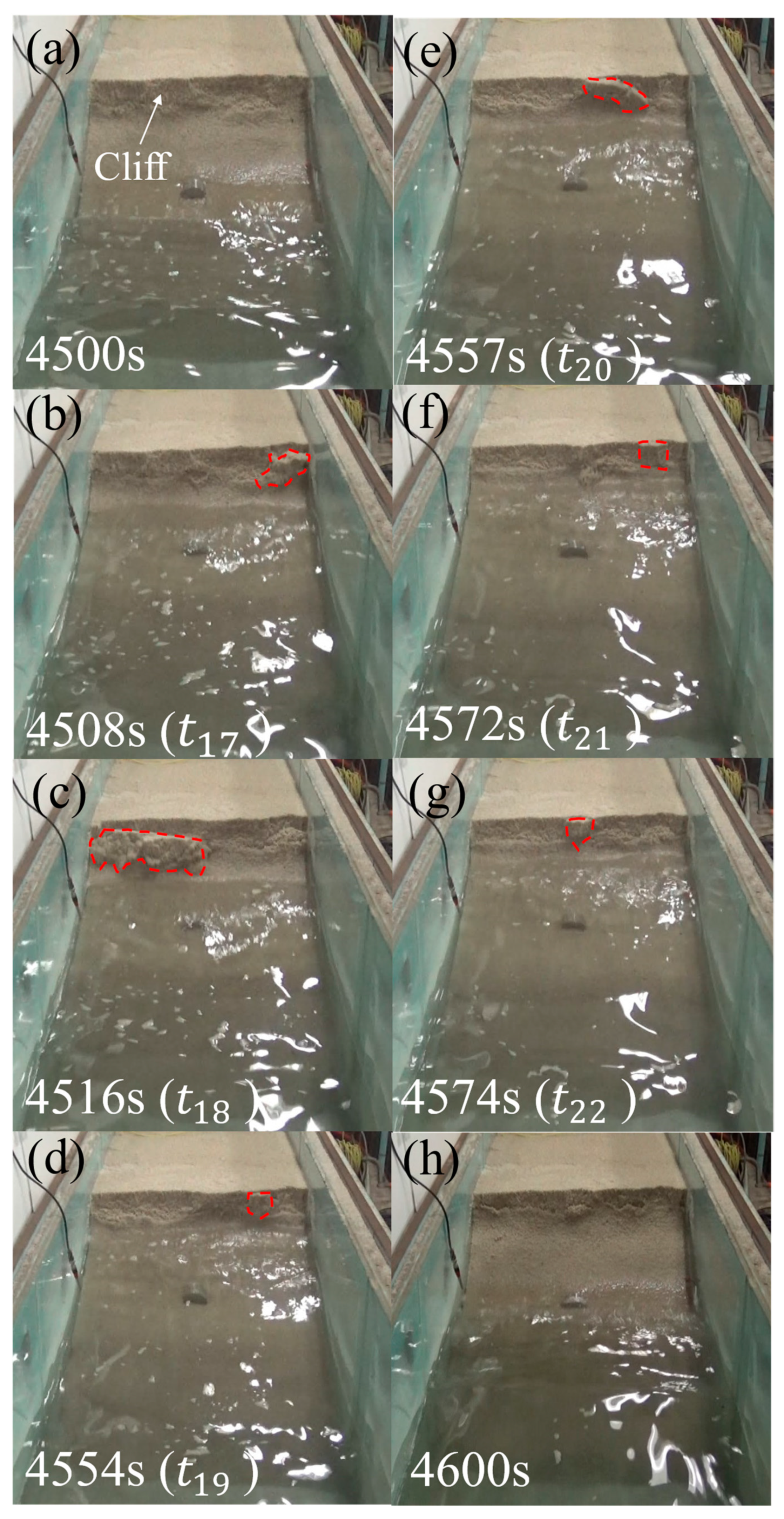
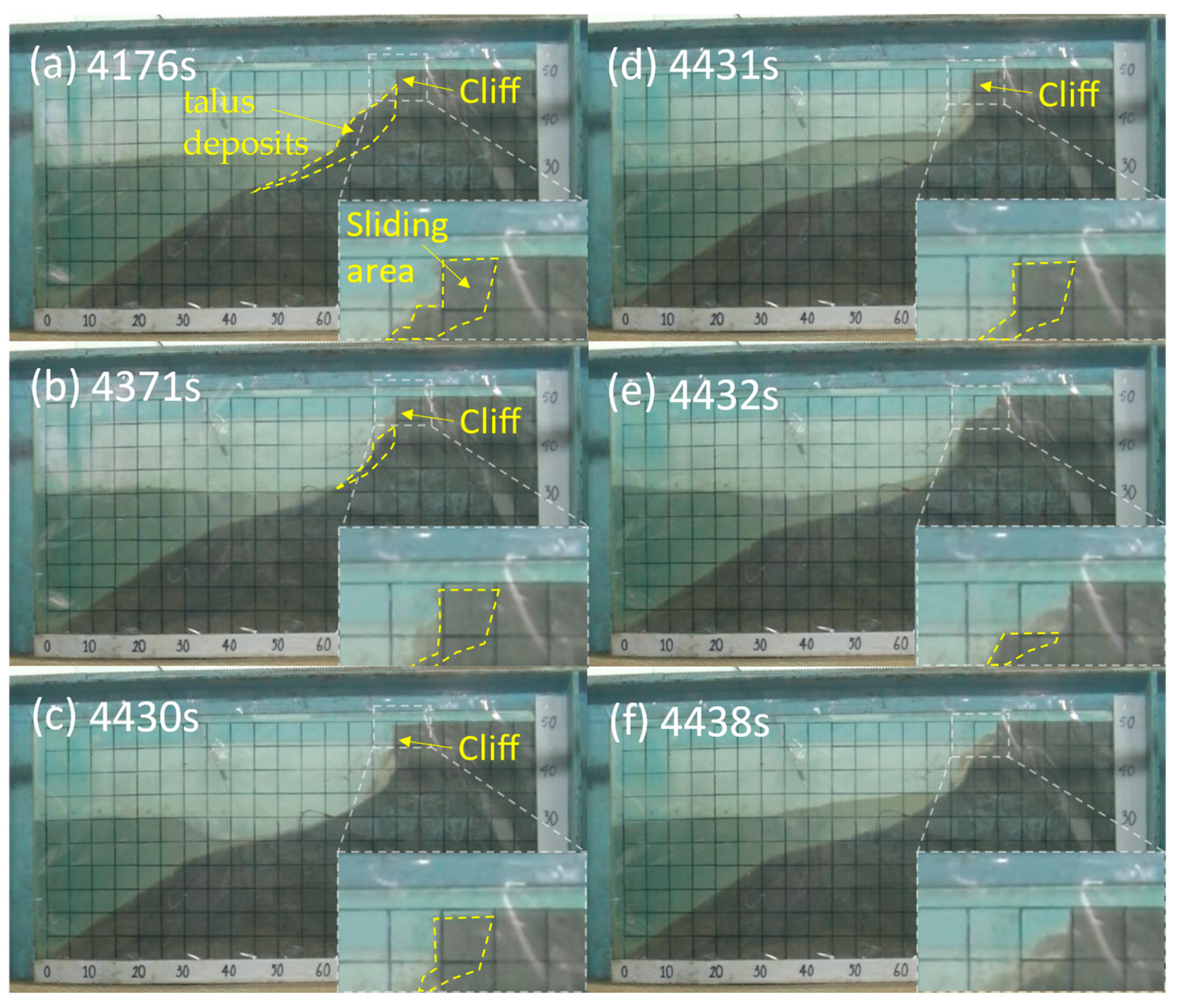
3.2. Characteristics of the Seismic Signals of the Slope Failure (Baseline Case)
- (1)
- Slope sliding induced by cyclic wave backwash: This occurs when the water retreating back into the ‘sea’ (backwash) pulls material from the slope with it, leading to sliding. The cyclic nature of the waves can cause repeated sliding events.
- (2)
- Re-sliding of the previously slid soil mass induced by wave swash: Swash refers to the water rushing up the slope after breaking. This scenario involves the reactivation and further movement of soil masses that had previously slid down but not completely detached or been washed away.
- (3)
- Slope collapse induced by wave impact: This refers to the failure and sudden movement of a portion of the slope due to the force of the waves impacting the slope, often accompanied by breaking and splashing.
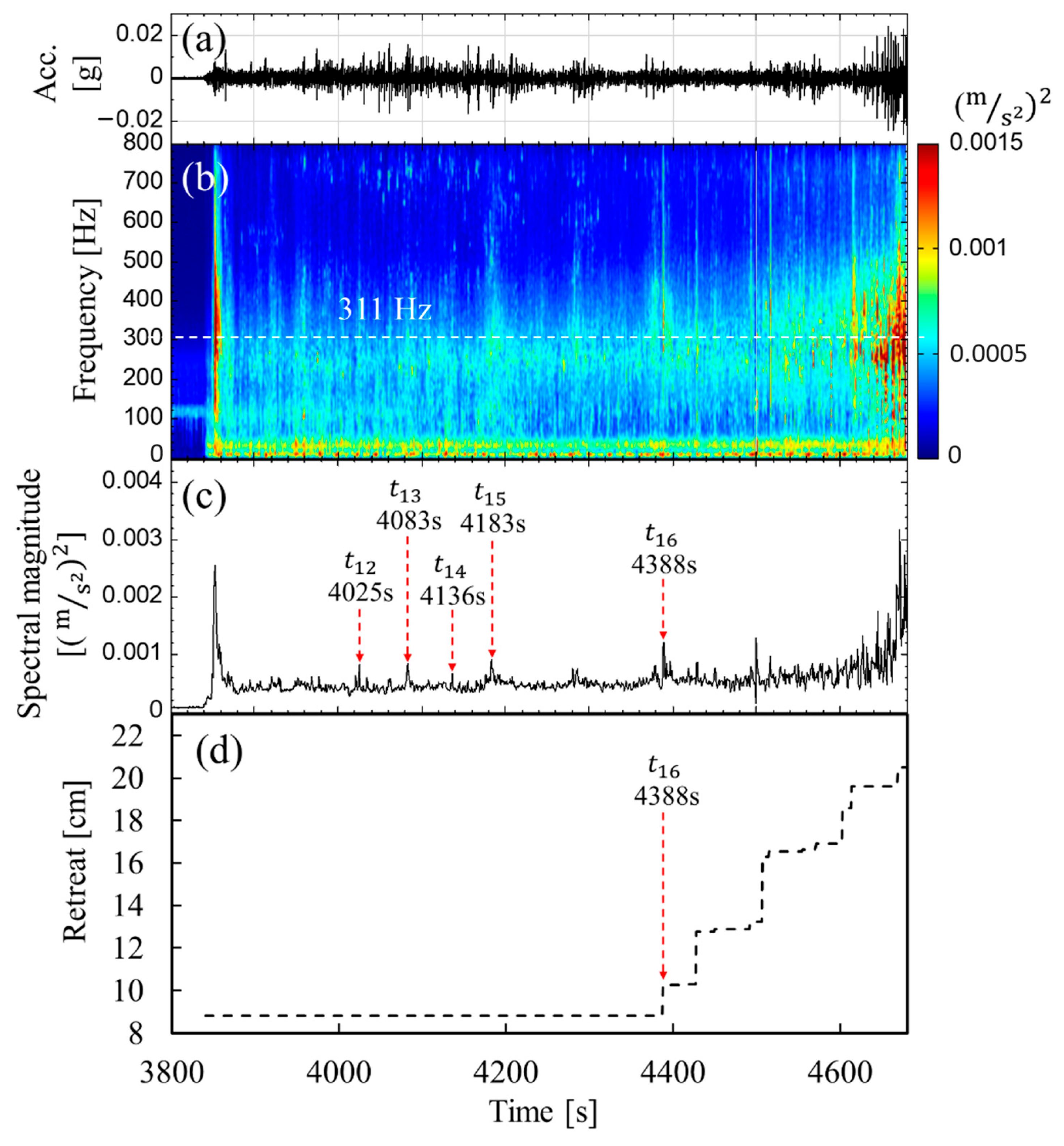
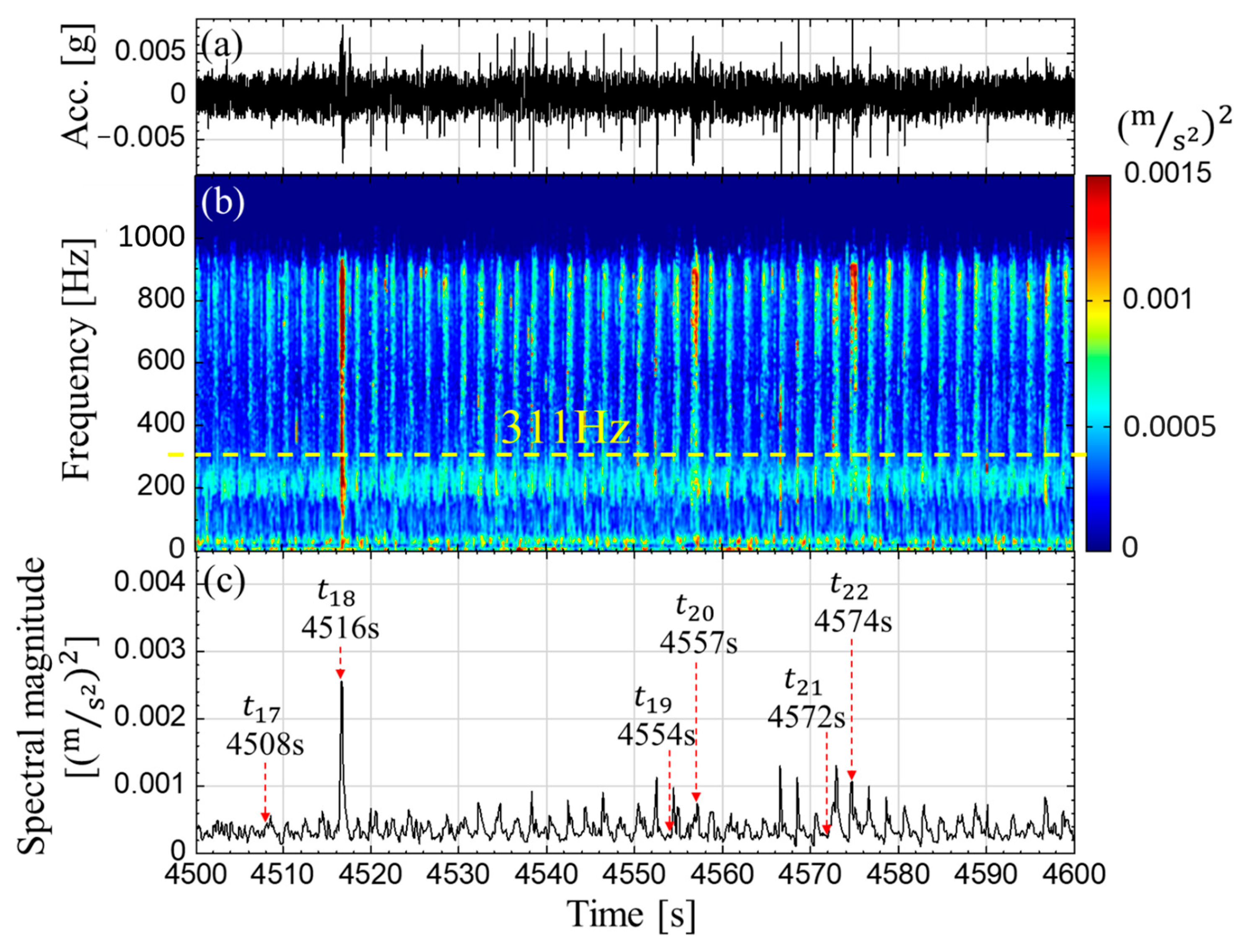
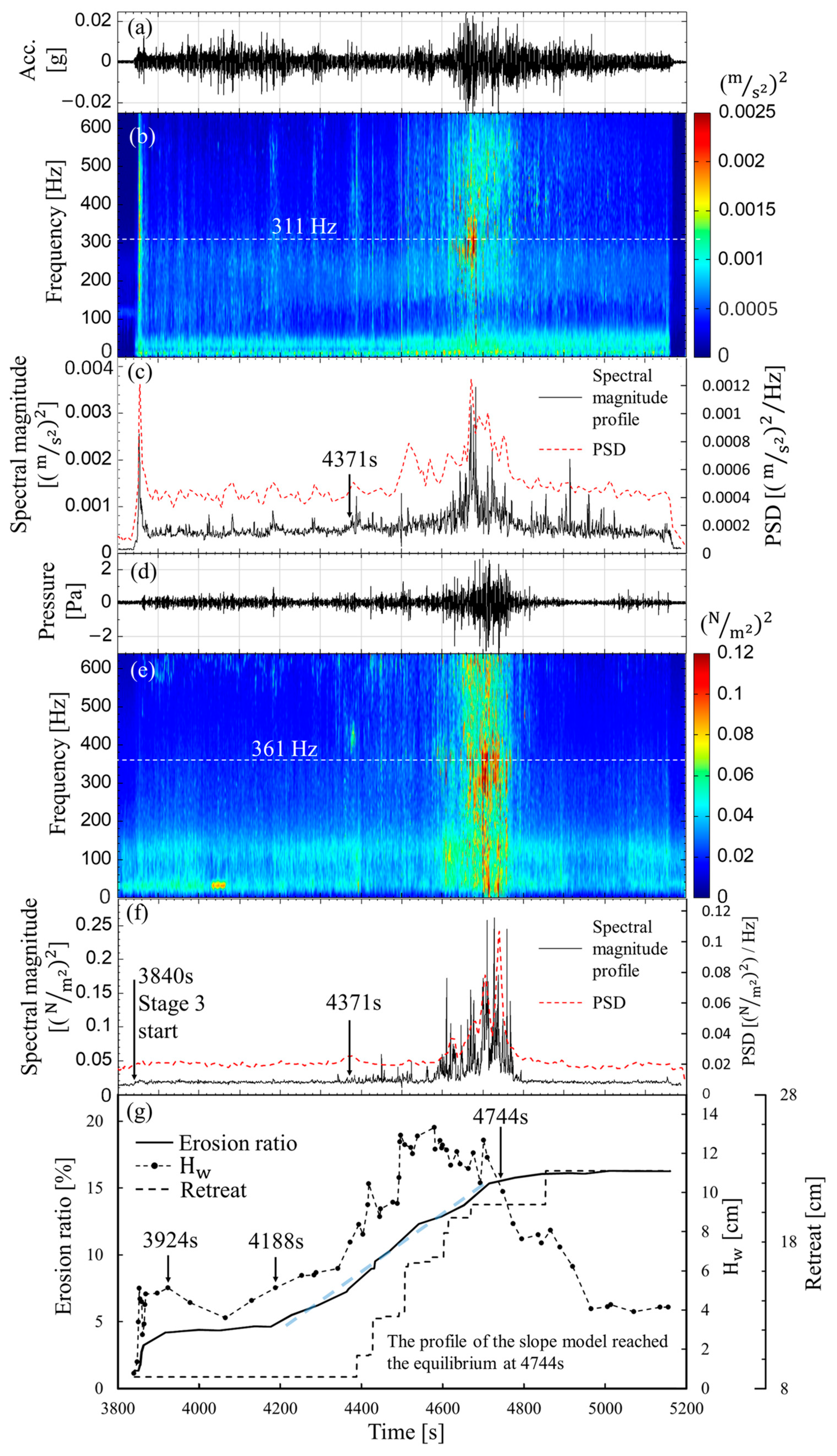
3.3. Slope Erosion Versus Seismic Signals and Acoustic Signals (Baseline Case)
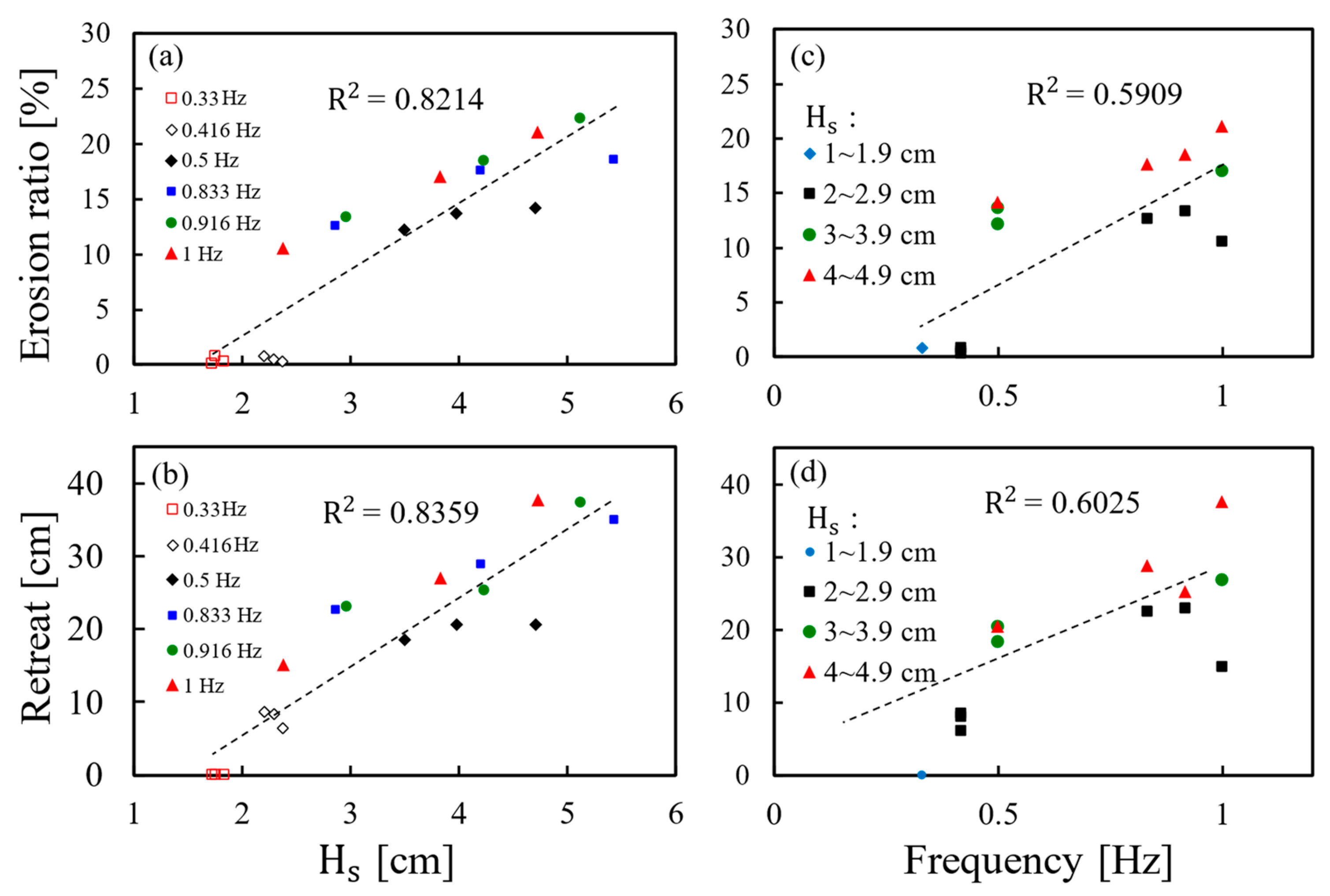
3.4. Parametric Study of Influence of Significant Wave Height Hs and Wave Frequency on the Erosion Ratio, Retreat, and Arias Intensity IA
4. Conclusions
- Piezometers and seismic sensors should be installed as close to the slope surface as possible but also positioned to avoid exposure by erosion. This placement allows for the measurement of more significant signals. The variation in wave height strongly affects the amplitude of pore pressure near the slope surface. The higher the wave height, the greater the amplitude of the pore pressure, indicating stronger wave erosion forces. Therefore, sensors should be placed as close to the slope face as possible in applications to assess changes in wave height and the condition of wave erosion.
- As the run-up height of the wave (Hw) rises, the pore pressure generally also increases, even if the piezometers are installed a bit away from the slope surface. The increase in pore pressure can be used as an indicator of the increase in the Hw, reflecting the enlargement of wave erosion on slopes. As waves gradually erode the slope surface, the amplitude variation in pore pressure increases, serving as an indicator that the slope has undergone further erosion.
- When waves backwash cyclically, the rapid drawdown phenomenon may induce sliding of the slope.
- Generally, when waves intensely swash and impact the slope surface, seismic and acoustic energy traces in the time-frequency spectrum will be very obvious. If a collapse occurs while the wave impacts the slope, the energy traces of the collapse in the time-frequency spectrum can easily be masked by the energy traces of the impacting waves.
- With the transition of erosion from swash to impact, a marked rise in both the spectral magnitude and Power Spectral Density (PSD) of seismic and acoustic signals was observed. The impact mode of wave erosion generated louder sounds and more significant seismic energy compared to that from swash erosion.
- Sometimes, even when Hw and the erosion ratio increase, the spectral values and PSD of the seismic and acoustic signals may not increase correspondingly. This is due to previously slid loose soils being swashed without being impacted. Even though the Hw gradually increased, the erosion rate remained roughly the same; that is, the erosion rate did not increase correspondingly with Hw. As the Hw decreased, the turning point where seismic and acoustic energy declined indicates that the force of wave erosion on the slope began to diminish gradually.
- Wave height has a greater influence on erosion and retreat compared to wave frequency, based on the R2 values from the linear regression.
- Arias Intensity (IA) and erosion ratio increased exponentially. Therefore, when IA due to wave action can be obtained for a slope, there is great potential for IA to be applied in estimating the erosion ratio for that slope.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bao, Y.; Tang, Q.; He, X.; Hu, Y.; Zhang, X. Soil erosion in the riparian zone of the Three Gorges Reservoir, China. Hydrol. Res. 2015, 46, 212–221. [Google Scholar] [CrossRef]
- Cleary, P.W.; Prakash, M.; Mead, S.; Lemiale, V.; Robinson, G.K.; Ye, F.; Ouyang, S.; Tang, X. A scenario-based risk framework for determining consequences of different failure modes of earth dams. Nat. Hazards 2015, 75, 1489–1530. [Google Scholar] [CrossRef]
- Lopez-Saez, J.; Corona, C.; Morel, P.; Rovéra, G.; Dewez, T.J.B.; Stoffel, M.; Berger, F. Quantification of cliff retreat in coastal Quaternary sediments using anatomical changes in exposed tree roots. Earth Surf. Process. Landf. 2018, 43, 2983–2997. [Google Scholar] [CrossRef]
- Sajinkumar, K.S.; Kannan, J.P.; Indu, G.K.; Muraleedharan, C.; Rani, V.R. A composite fall-slippage model for cliff recession in the sedimentary coastal cliffs. Geosci. Front. 2017, 8, 903–914. [Google Scholar] [CrossRef]
- Severson, J.P.; Nawrot, J.R.; Eichholz, M.W. Shoreline stabilization using riprap breakwaters on a Midwestern reservoir. Lake Reserv. Manag. 2009, 25, 208–216. [Google Scholar] [CrossRef]
- Zhu, C.; Peng, J.; Jia, Y. Marine geohazards: Past, present, and future. Eng. Geol. 2023, 323, 107230. [Google Scholar] [CrossRef]
- Vallejo, F.M. Bluff response to wave action. Eng. Geol. 1988, 26, 1–16. [Google Scholar] [CrossRef]
- Shipman, H.M. Coastal Bluffs and Sea Cliffs on Puget Sound, Washington. In Formation, Evolution, and Stability of Coastal Cliffs–Status and Trends; Hampton, M.A., Griggs, G.B., Eds.; U.S. Geological Survey Professional Paper No. 1693; U.S. Geological Survey: Reston, VA, USA, 2004. [Google Scholar]
- Hampton, M.A.; Griggs, G.B.; Edil, T.B.; Guy, D.E.; Kelley, J.T.; Komar, P.D.; Mickelson, D.M.; Shipman, H.M. Processes that Govern the Formation and Evolution of Coastal Cliffs. In Formation, Evolution, and Stability of Coastal Cliffs–Status and Trends; Hampton, M.A., Griggs, G.B., Eds.; U.S. Geological Survey Professional Paper No. 1693; U.S. Geological Survey: Reston, VA, USA, 2004. [Google Scholar]
- Kawamura, S.; Miura, S. Stability evaluation of slope with soft cliff. Int. J. Geotech. Eng. 2012, 6, 185–191. [Google Scholar] [CrossRef]
- Ji, F.; Shi, Y.; Li, R.; Zhou, H.; Wang, D.; Zhang, J. Progressive geomorphic evolution of reservoir bank in coarse-grained soil in East China—Insights from long-term observations and physical model test. Eng. Geol. 2021, 281, 105966. [Google Scholar] [CrossRef]
- Qin, Z.; Lai, Y.; Tian, Y. Study on failure mechanism of a plain irrigation reservoir soil bank slope under wind wave erosion. Nat. Hazards 2021, 109, 567–592. [Google Scholar] [CrossRef]
- Ozeren, Y.; Wren, D.G.; Reba, M.L. Wave-induced erosion of soil embankment in laboratory flume. J. Hydraul. Eng. 2021, 147, 04021011. [Google Scholar] [CrossRef]
- Morales, T.; Clemente, J.A.; Mollá, L.D.; Izagirre, E.; Uriarte, J.A. Analysis of instabilities in the Basque Coast Geopark coastal cliffs for its environmentally friendly management (Basque-Cantabrian basin, northern Spain). Eng. Geol. 2021, 283, 106023. [Google Scholar] [CrossRef]
- Alberti, S.; Olsen, M.J.; Allan, J.; Leshchinsky, B. Feedback thresholds between coastal retreat and landslide activity. Eng. Geol. 2022, 301, 106620. [Google Scholar] [CrossRef]
- Carpenter, N.E.; Dickson, M.E.; Walkden, M.J.A.; Nicholls, R.J.; Powrie, W. Effects of varied lithology on soft-cliff recession rates. Mar. Geol. 2014, 354, 40–52. [Google Scholar] [CrossRef]
- Castedo, R.; Fernández, M.; Trenhaile, A.S.; Paredes, C. Modeling cyclic recession of cohesive clay coasts: Effects of wave erosion and bluff stability. Mar. Geol. 2013, 335, 162–176. [Google Scholar] [CrossRef]
- Wang, L.; Li, Q.; Chen, Y.; Wang, S.; Li, X.; Fan, Z.; Chen, Y. Model test study on wave-induced erosion on gravelly soil bank slope. Nat. Hazards 2023, 119, 1665–1682. [Google Scholar] [CrossRef]
- Feng, Z.Y.; Hsu, C.M.; Chen, S.H. Discussion on the characteristics of seismic signals due to riverbank landslides from laboratory tests. Water 2019, 12, 83. [Google Scholar] [CrossRef]
- Feng, Z.Y.; Chen, S.H. Discussions on landslide types and seismic signals produced by the soil rupture due to seepage and retrogressive erosion. Landslides 2021, 18, 2265–2279. [Google Scholar] [CrossRef]
- Feng, Z.Y.; Huang, H.Y.; Chen, S.C. Analysis of the characteristics of seismic and acoustic signals produced by a dam failure and slope erosion test. Landslides 2020, 17, 1605–1618. [Google Scholar] [CrossRef]
- Chang, J.M.; Chao, W.A.; Chen, H.; Kuo, Y.T.; Yang, C.M. Locating rock slope failures along highways and understanding their physical processes using seismic signals. Earth Surf. Dyn. 2021, 9, 505–517. [Google Scholar] [CrossRef]
- Helmstetter, A.; Garambois, S. Seismic monitoring of Sechilienne rockslide (French Alps): Analysis of seismic signals and their correlation with rainfalls. J. Geophys. Res.—Earth Surf. 2010, 115, 03016. [Google Scholar] [CrossRef]
- Zhao, J.; Zhang, H.; Yang, C.; Lee, L.M.; Zhao, X.; Lai, Q. Experimental study of reservoir bank collapse in gravel soil under different slope gradients and water levels. Nat. Hazards 2020, 102, 249–273. [Google Scholar] [CrossRef]
- Hegge, B.J.; Masselink, G. Groundwater-table responses to wave run-up: An experimental study from Western Australia. J. Coast. Res. 1991, 7, 623–634. [Google Scholar]
- Peng, M.; Jiang, Q.; Zhang, Q.; Hong, Y.; Jiang, M.; Shi, Z.; Zhang, L. Stability analysis of landslide dams under surge action based on large-scale flume experiments. Eng. Geol. 2019, 259, 105191. [Google Scholar] [CrossRef]
- Huang, C.J.; Yeh, C.H.; Chen, C.Y.; Chang, S.T. Ground vibrations and airborne sounds generated by motion of rock in a river bed. Nat. Hazard Earth Syst. 2008, 8, 1139–1147. [Google Scholar] [CrossRef]
- Chou, H.T.; Lee, C.F.; Huang, C.H.; Chang, Y.L. The monitoring and flow dynamics of gravelly debris flows. J. Chin. Soil Water Conserv. 2013, 44, 144–157. (In Chinese) [Google Scholar]
- Liu, D.L.; Leng, X.P.; Wei, F.Q.; Zhang, S.J.; Hong, Y. Monitoring and recognition of debris flow infrasonic signals. J. Mt. Sci. 2015, 12, 797–815. [Google Scholar] [CrossRef]
- Holthuijsen, L.H. Waves in Oceanic and Coastal Waters; Cambridge University Press: Cambridge, UK, 2007; p. 28. [Google Scholar]
- Huang, N.E.; Shen, Z.; Long, S.R.; Wu, M.L.C.; Shih, H.H.; Zheng, Q.N.; Yen, N.C.; Tung, C.C.; Liu, H.H. The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. R. Soc. A Math. Phys. 1998, 454, 903–995. [Google Scholar] [CrossRef]
- Arias, A. A measure of earthquake intensity. In Seismic Design for Nuclear Power Plants; Hansen, R.J., Ed.; MIT Press: Cambridge, MA, USA, 1970; pp. 438–483. [Google Scholar]
- AnCad, Inc. Visual Signal Reference Guide. Version 1.6; AnCad, Inc.: Taipei, Taiwan, 2023; Available online: http://www.ancad.com.tw/VisualSignal/doc/1.6/RefGuide.html (accessed on 1 November 2023). (In Chinese)
- Yan, Y.; Cui, P.; Chen, S.C.; Chen, X.Q.; Chen, H.Y.; Chien, Y.L. Characteristics and interpretation of the seismic signal of a field-scale landslide dam failure experiment. J. Mt. Sci. 2017, 14, 219–236. [Google Scholar] [CrossRef]
- Morrisey, D.; Inglis, G.; Tait, L.; Woods, C.; Lewis, J.; Georgiades, E. Procedures for Evaluating in-Water Systems to Remove or Treat Vessel Biofouling; MPI Technical Paper No: 2015/39; New Zealand Government: Wellington, New Zealand, 2015; ISBN 978-1-77665-129-0.


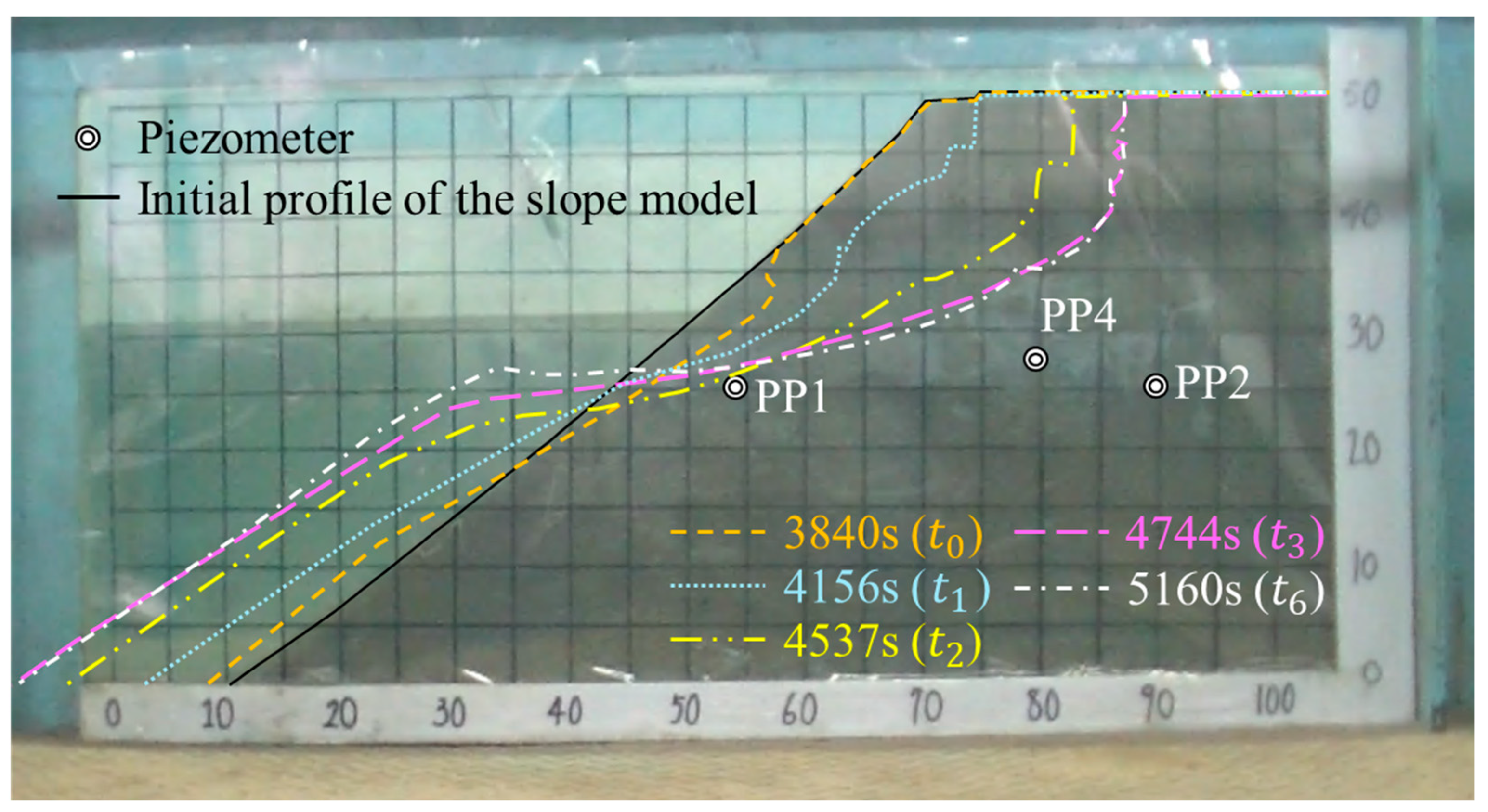



| Sloping of the Slope Model β [°] | Height of the Slope Model H [m] | Top Length of the Slope Model L1 [m] | Bottom Length of the Slope Model L2 [m] | Initial Water Level Hi [m] |
|---|---|---|---|---|
| 40 | 0.6 | 1.1 | 1.7 | 0.3 |
| Case | Initial Water Level Hi [cm] | Signifi. Wave Height Hs [cm] | Wave Frequency f [Hz] | Erosion Ratio E [%] | Retreat Re [cm] | Arias Intensity IA [m/s] |
|---|---|---|---|---|---|---|
| Baseline | 30.5 | 1.75 | Stage 1: 0.33 | 0.805 | 0 | 0.00298 |
| 2.29 | Stage 2: 0.416 | 0.530 | 8.226 | 0.00552 | ||
| 4.7 | Stage 3: 0.5 | 14.192 | 20.513 | 0.00829 | ||
| 1 | 29.2 | 1.72 | Stage 1: 0.33 | 0.150 | 0 | 0.00301 |
| 2.2 | Stage 2: 0.416 | 0.824 | 8.639 | 0.0045 | ||
| 3.49 | Stage 3: 0.5 | 12.231 | 18.508 | 0.00669 | ||
| 2 | 31.4 | 1.83 | Stage 1: 0.33 | 0.361 | 0 | 0.00338 |
| 2.37 | Stage 2: 0.416 | 0.353 | 6.298 | 0.0058 | ||
| 3.97 | Stage 3: 0.5 | 13.713 | 20.547 | 0.00605 | ||
| 3 | 29.3 | 2.85 | 0.833 | 12.671 | 22.591 | 0.01682 |
| 4 | 28.1 | 4.19 | 17.649 | 28.875 | 0.02747 | |
| 5 | 29.4 | 5.42 | 18.657 | 35.064 | 0.04605 | |
| 6 | 29.5 | 2.95 | 0.916 | 13.407 | 23.138 | 0.01319 |
| 7 | 30.3 | 4.22 | 18.535 | 25.368 | 0.0292 | |
| 8 | 30.5 | 5.11 | 22.359 | 37.327 | 0.0487 | |
| 9 | 28.6 | 2.37 | 1 | 10.561 | 15.0311 | 0.02881 |
| 10 | 28.3 | 3.82 | 10.561 | 26.917 | 0.04978 | |
| 11 | 28.5 | 4.72 | 21.131 | 37.670 | 0.0751 |
| Model Scale (Baseline Case) | Prototype Scale | Douglas Sea Scale/State of the Sea | |
|---|---|---|---|
| Significant wave height: | |||
| Stage 1 | 1.75 cm | 1.4 m | 4/Moderate |
| Stage 2 | 2.29 cm | 1.83 m | 4/Moderate |
| Stage 3 | 4.7 cm | 3.76 m | 5/Rough |
| Wave frequency (Period): | |||
| Stage 1 | 0.33 Hz (3.03 s) | 0.037 Hz (27.05 s) | 0.037 Hz (27.05 s) |
| Stage 2 | 0.416 Hz (2.4 s) | 0.047 Hz (21.43 s) | 0.047 Hz (21.43 s) |
| Stage 3 | 0.5 Hz (2 s) | 0.056 Hz (17.86 s) | 0.056 Hz (17.86 s) |
| Time [s] | Stage | Procedures/Events | Changes in the Signals/Data |
|---|---|---|---|
| 0 | - | Test started. | - |
| 250–1180 | - | Pumped water into flume until water depth reached 30 cm and waited for the water level in the slope model until steady at 30 cm. | (1) The amplitude of seismic signal increased during 250–280 s. After 280 s, the seismic signal amplitude decreased. (2) The pore pressure of PP1–PP3 and PP4 increased to 0.56 kPa and 0.31 kPa. |
| 1050 | - | Turned on the wave maker. | - |
| 1180 | Stage 1 | Stage 1 started. Wave frequency = 0.33 Hz; Significant wave height = 1.75 cm | The amplitude of the seismic signals and pore pressure increased. |
| 1188–1218 | The rapid change in the water level on the slope initiated the onset of instability in the slope model, leading to landslides. | The amplitude of seismic signals of Acc 1 significantly increased. | |
| 2380 | Stage 1 ended. | The amplitude of the seismic signals and pore pressure decreased. | |
| 2380–2520 | - | Turned off the wave maker and waited for waves, seismic and acoustic signals, and the pore pressure in the slope model to become stable before Stage 2. | - |
| 2520 | Stage 2 | Stage 2 started. Turned on the wave maker. Wave frequency = 0.416 Hz; Significant wave height = 2.29 cm | The amplitude of the seismic signals and pore pressure increased. |
| 2528–2562 | The rapid change in the water level on the slope initiated the onset of instability in the slope model, leading to landslides. | The amplitude of seismic signals of Acc 1 and Acc 2 significantly increased. | |
| 3720 | Stage 2 ended. | The amplitude of the seismic signals and pore pressure decreased. | |
| 3720–3840 | - | Turned off the wave maker and waited for waves, seismic and acoustic signals, and the pore pressure in the slope model to become stable before Stage 3. | - |
| 3840 | Stage 3 | (1) Stage 3 started. Turned on the wave maker. Wave frequency = 0.5 Hz; Significant wave height = 4.7 cm (2) The accelerometer Acc1 was exposed on the slope model. | (1) The amplitude of the seismic signal, acoustic signals, and pore pressure increased. (2) The amplitude of seismic signals of Acc1 became unreliable. |
| 3849–3862 | The rapid change in the water level on the slope initiated the onset of instability in the slope model, leading to landslides. | The amplitude of seismic signals significantly increased. | |
| 4391–4692 | The wave run-up height rose, and the force of wave impaction increased, which caused the slope model to collapse and also caused the slope to retreat rapidly. | (1) The amplitude of seismic and acoustic signals increased gradually. (2) The pore pressure increased and the amplitude of pore pressure also increased. | |
| 4693–4966 | The wave run-up height went down, and the force of wave impaction decreased. | (1) The amplitude of seismic and acoustic signals decreased. (2) The pore pressure decreased and the amplitude of pore pressure decreased slightly. | |
| 5160 | Stage 3 ended. Turned off the wave maker and the whole test ended. | The amplitude of the seismic signal, acoustic signals, and pore pressure decreased. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Z.-Y.; Wu, W.-T.; Chen, S.-C. Characterization of Seismic Signal Patterns and Dynamic Pore Pressure Fluctuations Due to Wave-Induced Erosion on Non-Cohesive Slopes. Appl. Sci. 2024, 14, 8776. https://doi.org/10.3390/app14198776
Feng Z-Y, Wu W-T, Chen S-C. Characterization of Seismic Signal Patterns and Dynamic Pore Pressure Fluctuations Due to Wave-Induced Erosion on Non-Cohesive Slopes. Applied Sciences. 2024; 14(19):8776. https://doi.org/10.3390/app14198776
Chicago/Turabian StyleFeng, Zheng-Yi, Wei-Ting Wu, and Su-Chin Chen. 2024. "Characterization of Seismic Signal Patterns and Dynamic Pore Pressure Fluctuations Due to Wave-Induced Erosion on Non-Cohesive Slopes" Applied Sciences 14, no. 19: 8776. https://doi.org/10.3390/app14198776
APA StyleFeng, Z.-Y., Wu, W.-T., & Chen, S.-C. (2024). Characterization of Seismic Signal Patterns and Dynamic Pore Pressure Fluctuations Due to Wave-Induced Erosion on Non-Cohesive Slopes. Applied Sciences, 14(19), 8776. https://doi.org/10.3390/app14198776








