Investigation of the Conservatism in Multiple Cracks Coalescence Criteria Using Finite Element-Based Crack Growth Analysis
Abstract
:1. Introduction
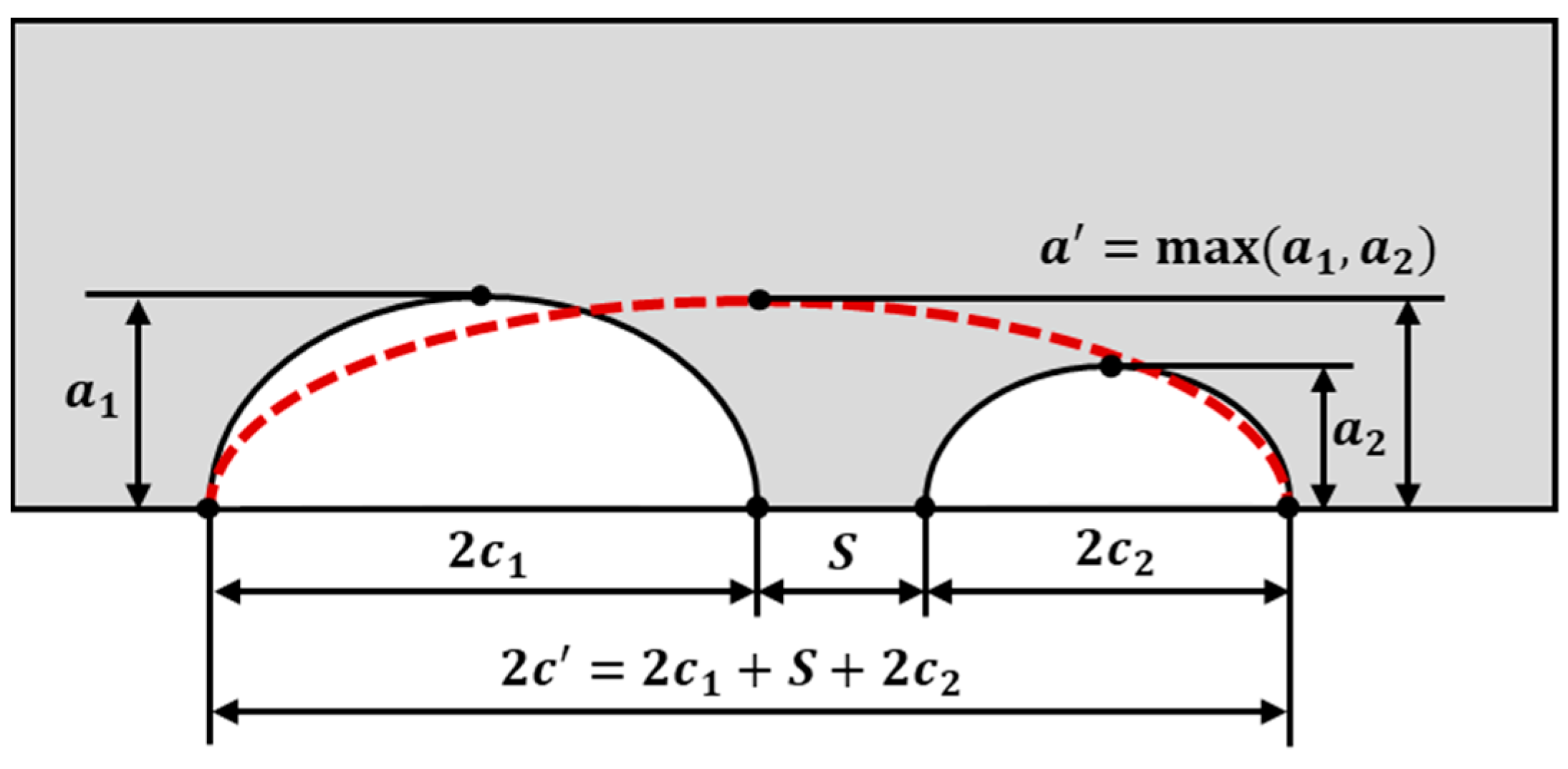
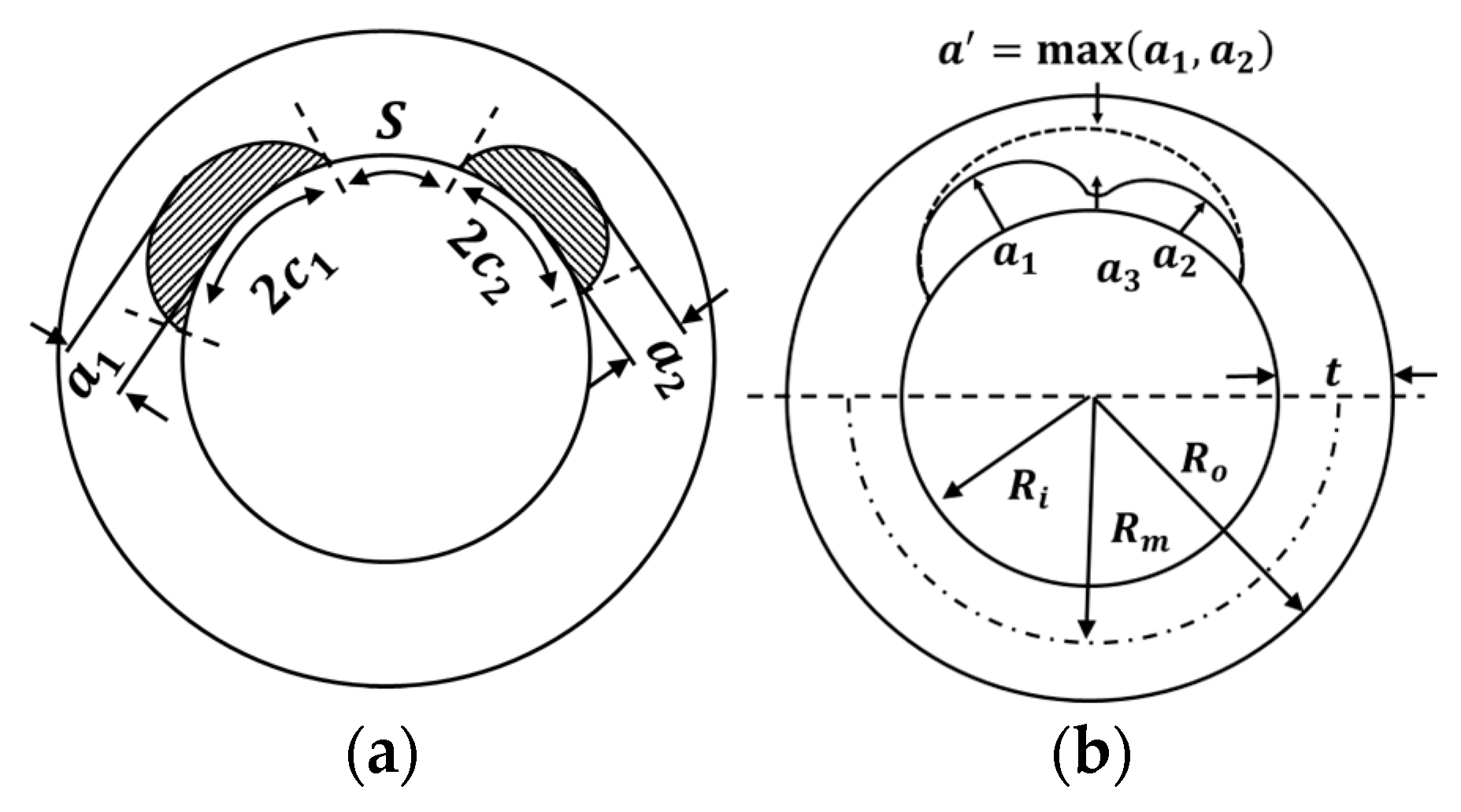
2. Review of the Multiple Cracks Coalescence Criteria in Codes
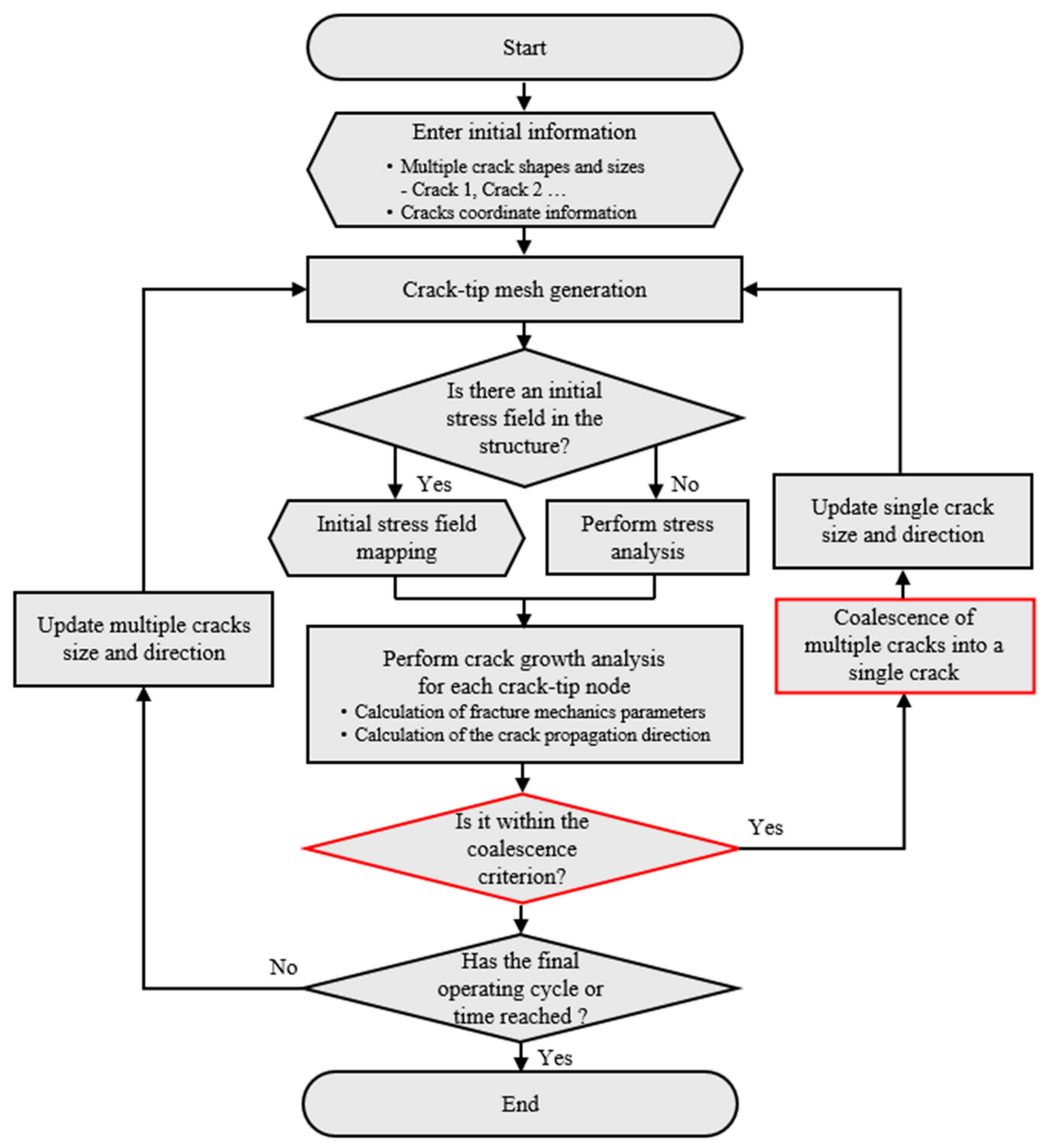
3. Development of AI-FEM Multiple Cracks Growth Algorithm
3.1. The Overall Procedure for Multiple Cracks Growth in AI-FEM
3.2. Plastic Zone Size-Based New AI-FEM Multiple Cracks Coalescence Criteria
4. Validation of New AI-FEM Multiple Cracks Coalescence Criteria
4.1. Geometry and Material
4.2. Summary of FCG Experiment
4.3. FE Model
4.4. Results
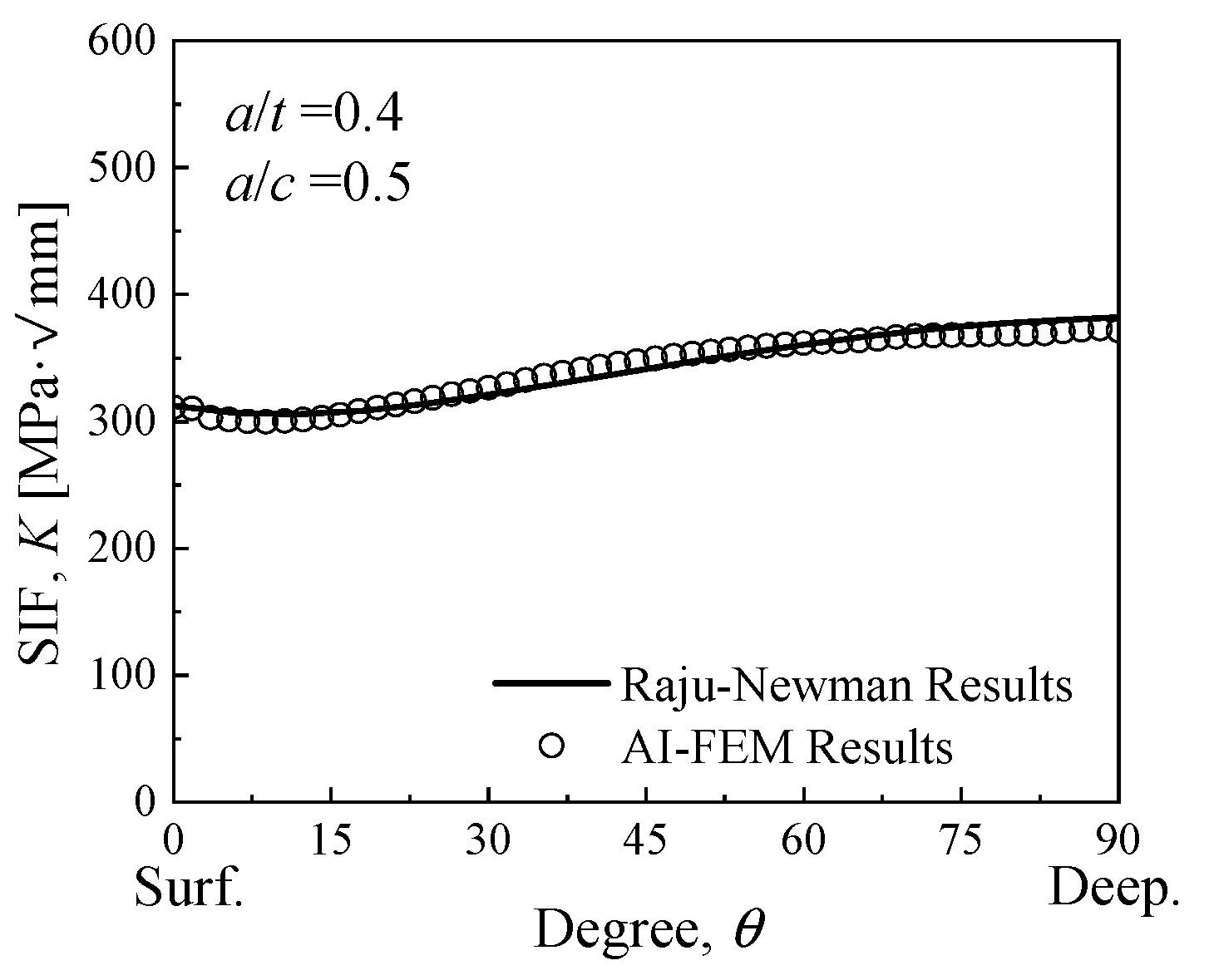
4.4.1. FE Model Verification
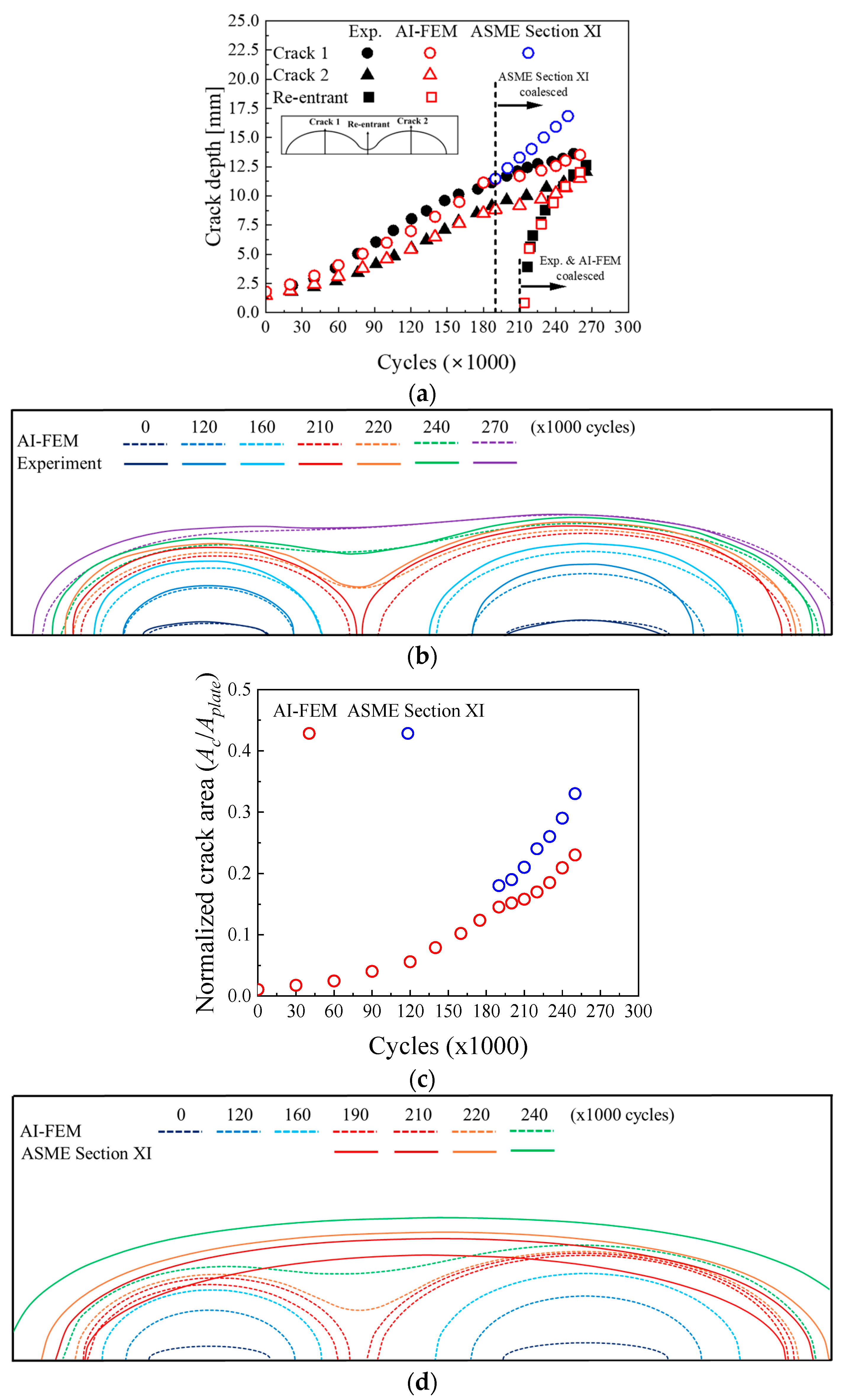
4.4.2. Comparison of FCG Results from AI-FEM, Experiment, and Codes
5. Investigation into Conservatism of Codes Using Multiple Crack Coalescence Criteria Based on Plastic Zone Size
5.1. Geometry and Material
5.2. FE Model
5.3. Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Na, M.G.; Kim, J.W.; Lim, D.H.; Kang, Y.J. Residual stress prediction of dissimilar metals welding at NPPs using support vector regression. Nucl. Eng. Des. 2008, 238, 1503–1510. [Google Scholar] [CrossRef]
- Shang, Y.B.; Shi, H.J.; Wang, Z.X.; Zhang, G.D. In-situ SEM study of short fatigue crack propagation behavior in a dissimilar metal welded joint of nuclear power plant. Mater. Des. 2015, 88, 598–609. [Google Scholar] [CrossRef]
- ASME Boiler and Pressure Vessel Code Section XI. Rules for Inservice Inspection of Nuclear Power Plant Components; ASME: New York, NY, USA, 2015. [Google Scholar]
- Rice, J.R. A Path Independent integral and the approximate analysis of strain concentration by notches and cracks. J. Appl. Mech. 1968, 35, 379–386. [Google Scholar] [CrossRef]
- Bezensek, B.; Hancock, J.W. The re-characterisation of complex defects: Part I: Fatigue and ductile tearing. Eng. Fract. Mech. 2004, 71, 981–1000. [Google Scholar] [CrossRef]
- Shibata, K.; Oba, T.; Kawamura, T.; Miyazono, S.; Yokoyama, N. Fatigue and fracture behavior of straight pipe with flaws in inner surface. Nucl. Eng. Des. 1981, 66, 33–45. [Google Scholar] [CrossRef]
- Iida, K.; Ando, K.; Hirata, T. An evaluation technique for fatigue life of multiple surface cracks (Part 1) a problem of multiple series surface cracks. J. Jpn. Soc. Nav. Archit. Ocean Eng. 1980, 1980, 284–293. [Google Scholar] [CrossRef]
- Kamaya, M. Growth evaluation of multiple interacting surface cracks. Part I: Experiments and simulation of coalesced crack. Eng. Fract. Mech. 2008, 75, 1336–1349. [Google Scholar] [CrossRef]
- Tu, S.T.; Dai, S.H. An engineering assessment of fatigue crack growth of irregularly oriented multiple cracks. Fatigue Fract. Eng. Mater. Struct. 1994, 17, 1235–1246. [Google Scholar] [CrossRef]
- API 579-1/ASME FFS-1; Fitness-for Service, 2nd ed. American Petroleum Institute and American Society of Mechanical Engineers: Houston, TX, USA, 2007.
- BS 7910:2013; Guide to Methods for Assessing the Acceptability of Flaws in Metallic Structures. British Standard Institute: London, UK, 2013.
- Milne, I.; Ainsworth, R.A.; Dowling, A.R.; Stewart, A.T. Assessment of the integrity of structures containing defects. Int. J. Press. Vessels Pip. 1988, 32, 3–104. [Google Scholar] [CrossRef]
- Hasegawa, K.; Shiratori, M.; Miyoshi, T.; Seki, N. Comparison of stress intensity factors of two flaws and a combined flaw due to combination rules. In Proceedings of the 2002 ASME Pressure Vessels and Piping Conference, Vancouver, BC, Canada, 5–9 August 2002; Volume 439, pp. 307–312. [Google Scholar]
- Kim, J.M.; Huh, N.S. On crack interaction effects of in-plane surface cracks using elastic and elastic-plastic finite element analyses. Nucl. Eng. Technol. 2010, 42, 680–689. [Google Scholar] [CrossRef]
- Kamaya, M. A criterion for combination rule in flaw assessment of parallel surface cracks. J. Press. Vessel. Technol. 2011, 42, 680–689. [Google Scholar] [CrossRef]
- Lin, X.B.; Smith, R.A. Direct simulation of fatigue crack growth for arbitrary-shaped defects in pressure vessels. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1998, 213, 175–189. [Google Scholar] [CrossRef]
- Doi, H.; Azuma, K. Simulation on propagation and coalescence of fatigue crack by automatic three-dimensional finite element crack propagation system. In Proceedings of the 2014 ASME Pressure Vessels and Piping Conference, Anaheim, CA, USA, 20–24 July 2014; Volume 50428. [Google Scholar]
- Kikuchi, M.; Wada, Y.; Suga, K.; Ohdama, C. Numerical simulation of coalescence behavior of multiple surface cracks. In Proceedings of the 2011 ASME Pressure Vessels and Piping Conference, Baltimore, MA, USA, 17–21 July 2011; Volume 44519. [Google Scholar]
- Wen, J.F.; Zhan, Y.; Tu, S.T.; Xuan, F.Z. A combination rule for multiple surface cracks based on fatigue crack growth life. AIMS Mater. Sci. 2016, 3, 1649–1664. [Google Scholar] [CrossRef]
- Branco, R.; Antunes, F.V.; Costa, J.D. A review on 3D-FE adaptive remeshing techniques for crack growth modelling. Eng. Fract. Mech. 2015, 141, 170–195. [Google Scholar] [CrossRef]
- JSME S NA1-2000; Codes for Nuclear Power Generation Facilities-Rules on Fitness-for-Service for Nuclear Power Plants. Japan Society of Mechanical Engineers: Tokyo, Japan, 2000.
- GB/T 19624; Safety Assessment for In-Service Pressure Vessels Containing Defects. Chinese Standards: Beijing, China, 2004.
- Lee, G.B.; Park, S.H.; Jang, Y.Y.; Huh, N.S.; Park, S.H.; Park, N.H.; Park, J. Development of automatic crack growth simulation program based on finite element analysis. Appl. Sci. 2022, 12, 3075. [Google Scholar] [CrossRef]
- Abaqus Version 2021, Users Manual. Available online: http://130.149.89.49:2080/v2016/index.html (accessed on 12 October 2024).
- Mohmadsalehi, M.; Soghrati, S. An automated mesh generation algorithm for simulating complex crack growth problems. Comput. Methods Appl. Mech. Eng. 2022, 398, 115015. [Google Scholar] [CrossRef]
- Gao, W.; Xiao, T.; Wang, X.; Wang, C. Theoretical study on extension of crack tip plastic zone by remote simple tensile considering crack interaction. Eur. J. Mech. A-Solid 2019, 77, 103814. [Google Scholar] [CrossRef]
- Kachanov, M. Elastic solids with many cracks: A simple method of analysis. Int. J. Solids Struct. 1987, 23, 23–43. [Google Scholar] [CrossRef]
- Nishimura, T. Strip yield analysis on coalescence of plastic zones for multiple cracks in a riveted stiffened sheet. J. Eng. Mater. Technol. 1999, 121, 352–359. [Google Scholar] [CrossRef]
- Hassani, A.; Faal, R.T. Dugdale’s model for plastic zone size of interacting cracks under anti-plane deformation. Procedia Struct. Integr. 2016, 2, 2424–2431. [Google Scholar] [CrossRef]
- Dugdale, D.S. Yielding of steel sheets containing slits. J. Mech. Phys. Solids 1960, 8, 100–104. [Google Scholar] [CrossRef]
- Irwin, G.R. Plastic zone near a crack and fracture toughness. In Proceedings of the 7th Sagamore Research Conference on Mechanics & Metals Behavior of Sheet Material, New York, NY, USA, 16–19 August 1960; Volume 4, pp. 463–478. [Google Scholar]
- Raju, I.S.; Newman, J.C., Jr. Stress-intensity factors for a wide range of semi-elliptical surface cracks in finite-thickness plates. Eng. Fract. Mech. 1979, 11, 817–829. [Google Scholar] [CrossRef]







| Document | Flaw Interaction Criteria | Flaw Interaction Criteria |
|---|---|---|
| ASME Section XI | - | |
| API 579 | - | |
| BS 7910 (and R6) |
| [mm] | [mm] | [mm] | [mm] |
|---|---|---|---|
| 150 | 25 | 230 | 200 |
| 0.4 | 0.5 |
| 0.058 | 4.5 | 0.072 | 4.9 | 1 |
| Maximum Bending Moment ] | Minimum Bending Moment ] |
|---|---|
| 1.3 106 | 1.3 105 |
| Coalesced Cycles (Cycles) | Normalized Crack Area | Normalized Crack Depth | |
|---|---|---|---|
| AI-FEM | 220,000 | 0.23 | 0.50 |
| ASME Section XI | 195,000 | 0.30 | 0.64 |
| Maximum difference | 16% | 30% | 22% |
| 0.4 | 3.5 | 0.3 | 0.2 | 3 |
| 1 |
| Coalesced Cycles (Cycles) | Normalized Crack Area | Normalized Crack Depth | |
|---|---|---|---|
| AI-FEM | 28,000 | 0.08 | 0.72 |
| ASME Section XI | 24,000 | 0.12 | 0.91 |
| Maximum difference | 16% | 33% | 20% |
| Coalesced Cycles (Cycles) | Normalized Crack Area | Normalized Crack Depth | |
|---|---|---|---|
| AI-FEM | 40,000 | 0.043 | 0.65 |
| ASME Section XI | 36,000 | 0.066 | 0.87 |
| Maximum difference | 11% | 35% | 25% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, C.-G.; Lee, G.-B.; Huh, N.-S. Investigation of the Conservatism in Multiple Cracks Coalescence Criteria Using Finite Element-Based Crack Growth Analysis. Appl. Sci. 2024, 14, 9441. https://doi.org/10.3390/app14209441
Hong C-G, Lee G-B, Huh N-S. Investigation of the Conservatism in Multiple Cracks Coalescence Criteria Using Finite Element-Based Crack Growth Analysis. Applied Sciences. 2024; 14(20):9441. https://doi.org/10.3390/app14209441
Chicago/Turabian StyleHong, Chan-Gi, Gi-Bum Lee, and Nam-Su Huh. 2024. "Investigation of the Conservatism in Multiple Cracks Coalescence Criteria Using Finite Element-Based Crack Growth Analysis" Applied Sciences 14, no. 20: 9441. https://doi.org/10.3390/app14209441
APA StyleHong, C.-G., Lee, G.-B., & Huh, N.-S. (2024). Investigation of the Conservatism in Multiple Cracks Coalescence Criteria Using Finite Element-Based Crack Growth Analysis. Applied Sciences, 14(20), 9441. https://doi.org/10.3390/app14209441






