A Train Timetable Optimization Method Considering Multi-Strategies for the Tidal Passenger Flow Phenomenon
Abstract
:1. Introduction
- Based on the tidal passenger flow phenomenon, a TUOS-ELS is proposed in this paper, consisting mainly of TUOS and ELS. In TUOS, the total number of trains running in both directions is determined. In ELS, according to passenger flow data, the stops express trains will skip are selected. Rolling stock circulation is also considered.
- The nonlinear model related to timetable and passenger flow is linearized, and the objectives of minimizing the number of stranded passengers, the total travel time of all trains, and the total number of stops are set. A multi-objective mixed integer linear programming (MILP) model is constructed to achieve an exact solution within a reasonable computational time. The optimization procedure is designed according to the actual train operation process, and the GUROBI solver is used to solve the optimization problem.
- Based on the Shanghai Suburban Railway airport link line, timetable optimization under different passenger flow scenarios is investigated, and the optimization results of the TUOS-ELS and T-TPOS are compared.
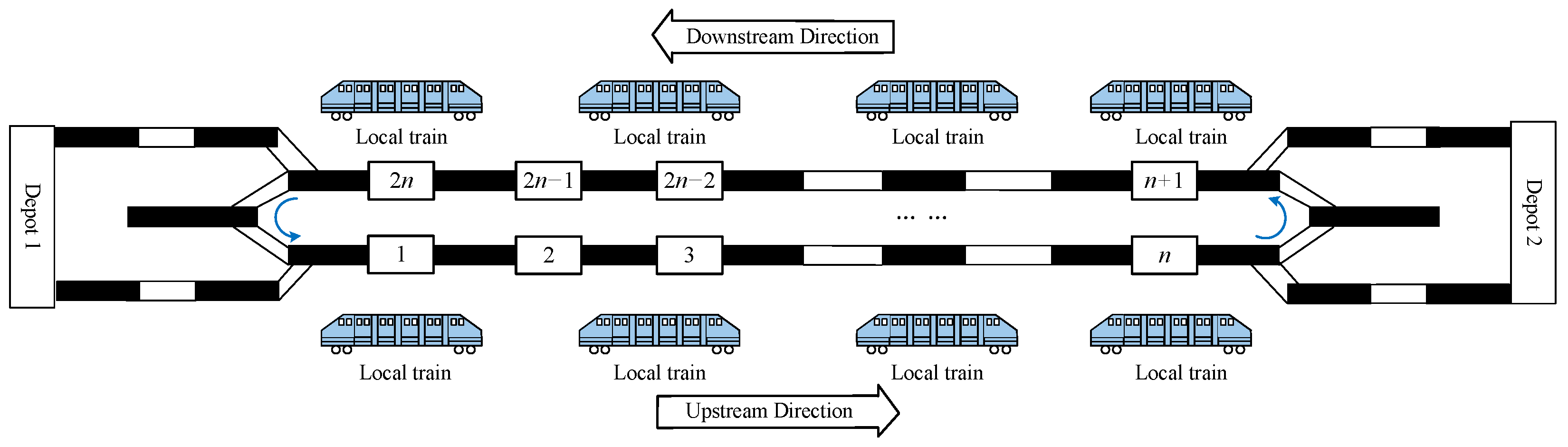
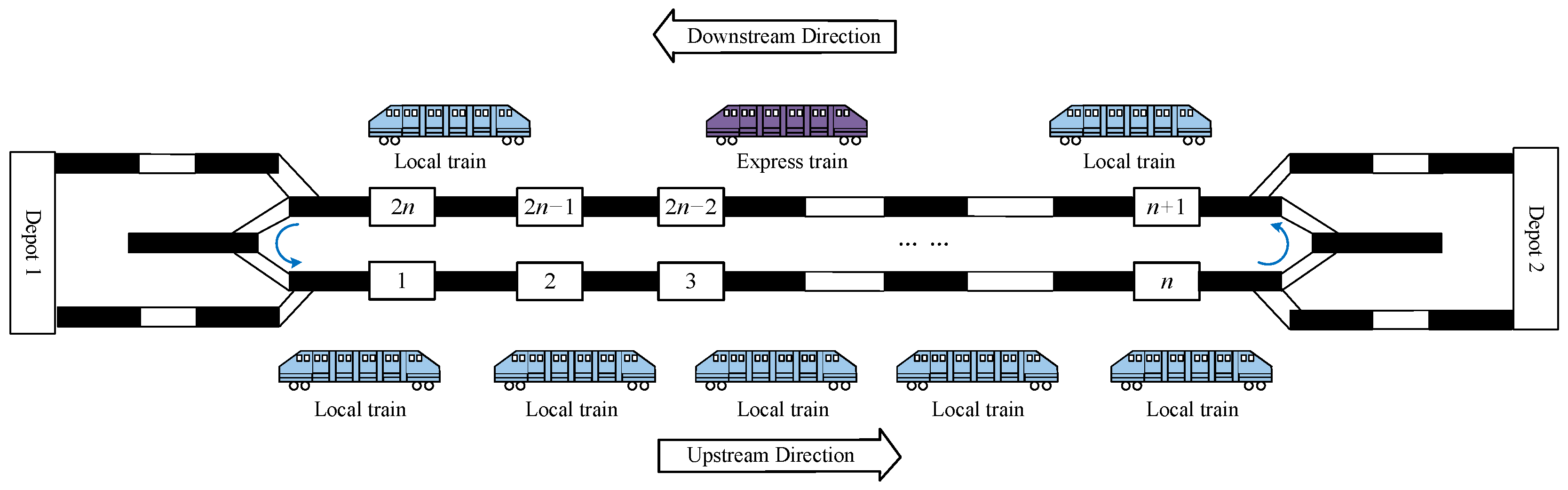
2. Problem Description
3. Mathematical Modeling
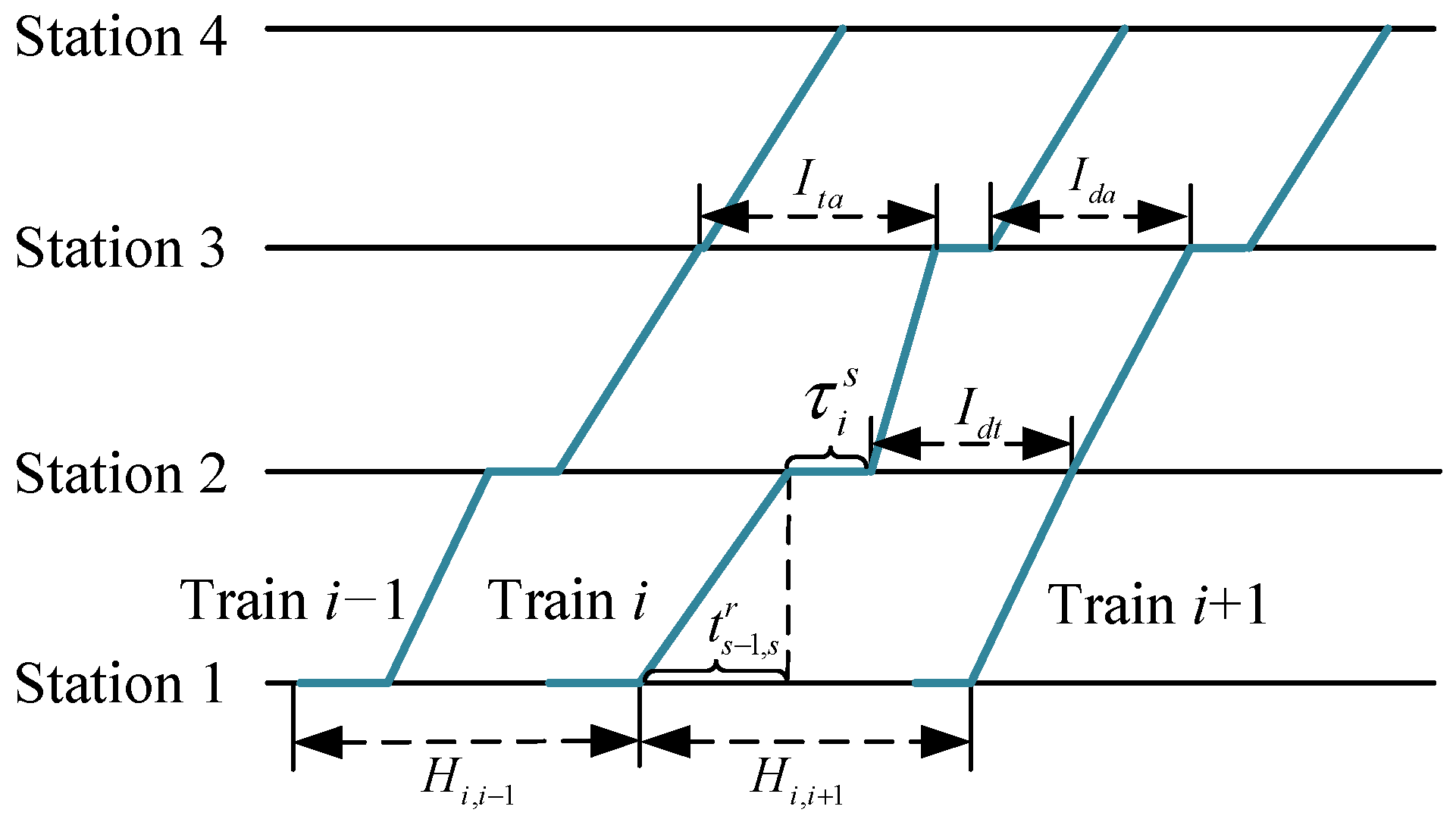
3.1. Train Traffic Dynamic Model
3.2. Passenger Flow Dynamic Model
3.3. Linearization of the Model
3.4. Problem Model
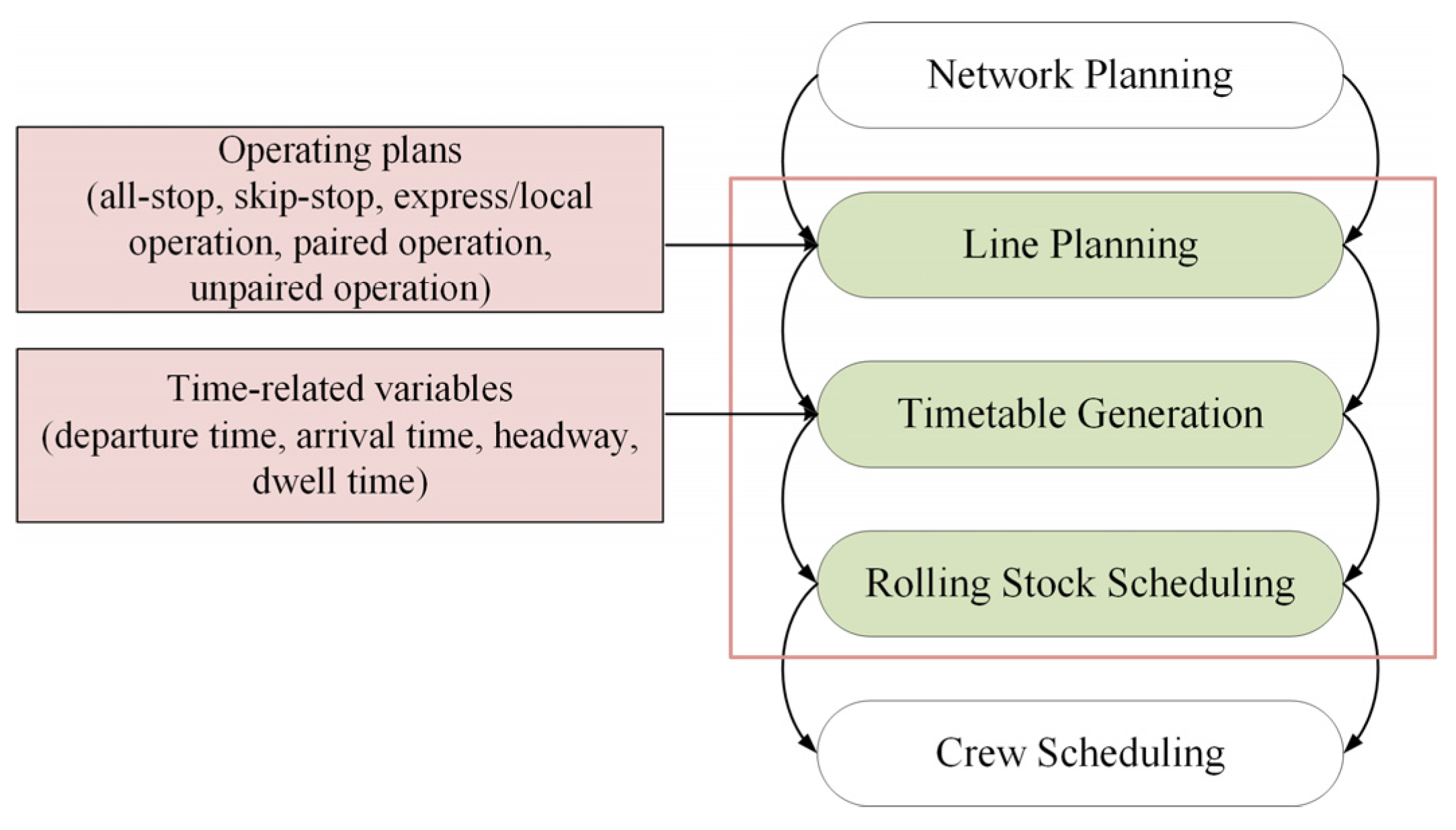
4. Method
5. Case Study
5.1. Simulation Setting
5.2. Optimization of Timetable During Trial Operation
5.3. Optimization of Timetable to Address Varying Passenger Demands
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tang, L.; Zhao, Y.; Cabrera, J.; Ma, J.; Tsui, L. Forecasting Short-Term Passenger Flow: An Empirical Study on Shenzhen Metro. IEEE Trans. Intell. Transp. Syst. 2019, 20, 3613–3622. [Google Scholar] [CrossRef]
- Yin, J.; Yang, L.; Tang, T.; Gao, Z.; Ran, B. Dynamic passenger demand oriented metro train scheduling with energy-efficiency and waiting time minimization: Mixed-integer linear programming approaches. Transp. Res. B-Meth. 2017, 97, 182–213. [Google Scholar] [CrossRef]
- Zhuo, S.; Miao, J.; Meng, L.; Yang, L.; Shang, P. Demand-driven integrated train timetabling and rolling stock scheduling on urban rail transit line. Transp. A 2024, 20, 2181024. [Google Scholar] [CrossRef]
- Chen, Z.; Li, X.; Zhou, X. Operational design for shuttle systems with modular vehicles under oversaturated traffic: Continuous modeling method. Transp. Res. B-Meth. 2020, 132, 76–100. [Google Scholar] [CrossRef]
- Sun, L.; Jin, J.; Lee, D.; Axhausen, K.; Erath, A. Demand-driven timetable design for metro services. Transp. Res. Part C Emerg. Technol. 2014, 46, 284–299. [Google Scholar] [CrossRef]
- Li, S.; Xu, R.; Han, K. Demand-oriented train services optimization for a congested urban rail line: Integrating short turning and heterogeneous headways. Transp. A 2019, 15, 1459–1486. [Google Scholar] [CrossRef]
- Yang, L.; Qi, J.; Li, S.; Gao, Y. Collaborative optimization for train scheduling and train stop planning on high-speed railways. Omega 2016, 64, 57–76. [Google Scholar] [CrossRef]
- Xu, X.; Li, C.L.; Xu, Z. Train timetabling with stop-skipping, passenger flow, and platform choice considerations. Transp. Res. B-Meth. 2021, 150, 52–74. [Google Scholar] [CrossRef]
- Zhang, F.; Li, S.; Yuan, Y.; Zhang, J.; Yang, L. Approximate dynamic programming approach to efficient metro train timetabling and passenger flow control strategy with stop-skipping. Eng. Appl. Artif. Intell. 2024, 127, 107393. [Google Scholar] [CrossRef]
- Yuan, Y.; Li, S.; Liu, R. Decomposition and approximate dynamic programming approach to optimization of train timetable and skip-stop plan for metro networks. Transp. Res. Part C Emerg. Technol. 2023, 157, 104393. [Google Scholar] [CrossRef]
- Rodolphe, Farrando; Farhi, N.; Christoforou, Z.; Urban, A. A mathematical model for a two-service skip-stop policy with demand-dependent dwell times. J. Rail Transp. Plan. Manag. 2024, 31, 100461.
- Liu, Z.; Pan, J.; Yang, Y.; Chi, X. An energy-efficient timetable optimization method for express/local train with on-board passenger number considered. IEEE Trans. Intell. Transp. Syst. 2024, 18, 2068–2088. [Google Scholar] [CrossRef]
- Zhang, R.; Yin, S.; Ye, M.; Yang, Z.; He, S. A Timetable Optimization Model for Urban Rail Transit with Express/Local Mode. J. Adv. Transport. 2021, 2021, 5589185. [Google Scholar] [CrossRef]
- Jin, B.; Guo, Y.; Wang, Q. Integrated scheduling method of timetable and rolling stock assignment scheme considering long and short routing. China Railw. Sci. 2022, 43, 173–181. [Google Scholar]
- Blanco, V.; Conde, E.; Hinojosa, Y.; Puerto, J. An optimization model for line planning and timetabling in automated urban metro subway networks. A case study. Omega 2020, 92, 102165. [Google Scholar] [CrossRef]
- Sun, P.; Yao, B.; Wang, Q.; Chen, S.; Lin, X.; Huang, Z. Optimization of unpaired timetable with express/local mode for the phenomenon of tidal passenger flow. In Proceedings of the IEEE 10th International Power Electronics and Motion Control Conference, Chengdu, China, 17–20 May 2024. [Google Scholar]
- Shi, J.; Yang, J.; Yang, L.; Tao, L. Safety-oriented train timetabling and stop planning with time-varying and elastic demand on overcrowded commuter metro lines. Transp. Res. E-Log. 2023, 175, 103136. [Google Scholar] [CrossRef]
- Zhou, H.; Qi, J.; Yang, L.; Shi, J.; Pan, H.; Gao, Y. Joint optimization of train timetabling and rolling stock circulation planning: A novel flexible train composition mode. Transp. Res. B-Meth 2022, 162, 352–385. [Google Scholar] [CrossRef]
- Zhao, S.; Yang, H.; Wu, Y. An integrated approach of train scheduling and rolling stock circulation with skip-stopping pattern for urban rail transit lines. Transp. Res. Part C Emerg. Technol. 2021, 128, 103170. [Google Scholar] [CrossRef]
- Cao, Z.; Ceder, A.; Li, D.; Zhang, S. Robust and optimized urban rail timetabling using a marshaling plan and skip-stop operation. Transp. A 2020, 16, 1217–1249. [Google Scholar] [CrossRef]
- Lusby, R.M.; Larsen, J.; Bull, S. A survey on robustness in railway planning. Eur. J. Oper. Res. 2018, 266, 1–15. [Google Scholar] [CrossRef]
- Mo, P.; Yang, L.; D’Ariano, A. Energy-Efficient Train Scheduling and Rolling Stock Circulation Planning in a Metro Line: A Linear Programming Approach. IEEE Trans. Intell. Transp. Syst. 2021, 21, 3621–3633. [Google Scholar] [CrossRef]
- Tang, L.; Xu, X. Optimization for operation scheme of express and local trains in suburban rail transit lines based on station classification and bi-level programming. J. Rail. Transport. Plan. 2022, 21, 100283. [Google Scholar] [CrossRef]
- Bucak, S.; Demirel, T. Train timetabling for a double-track urban rail transit line under dynamic passenger demand. Comput. Ind. Eng. 2022, 163, 107858. [Google Scholar] [CrossRef]
- Qi, J.; Li, S.; Gao, Y.; Yang, K.; Liu, P. Joint optimization model for train scheduling and train stop planning with passengers distribution on railway corridors. J. Oper. Res. Soc. 2018, 69, 556–570. [Google Scholar] [CrossRef]
- Cacchiani, V.; Qi, J.; Yang, L. Robust optimization models for integrated train stop planning and timetabling with passenger demand uncertainty. Transp. Res. B-Meth. 2020, 136, 1–29. [Google Scholar] [CrossRef]
- Högdahl, J.; Bohlin, M. A combined simulation-optimization approach for robust timetabling on main railway lines. Transp. Sci. 2023, 57, 52–81. [Google Scholar] [CrossRef]
- Gao, Y.; Kroon, L.; Schmidt, M.; Yang, L. Rescheduling a metro line in an over-crowded situation after disruptions. Transp. Res. B-Meth. 2016, 93, 425–449. [Google Scholar] [CrossRef]


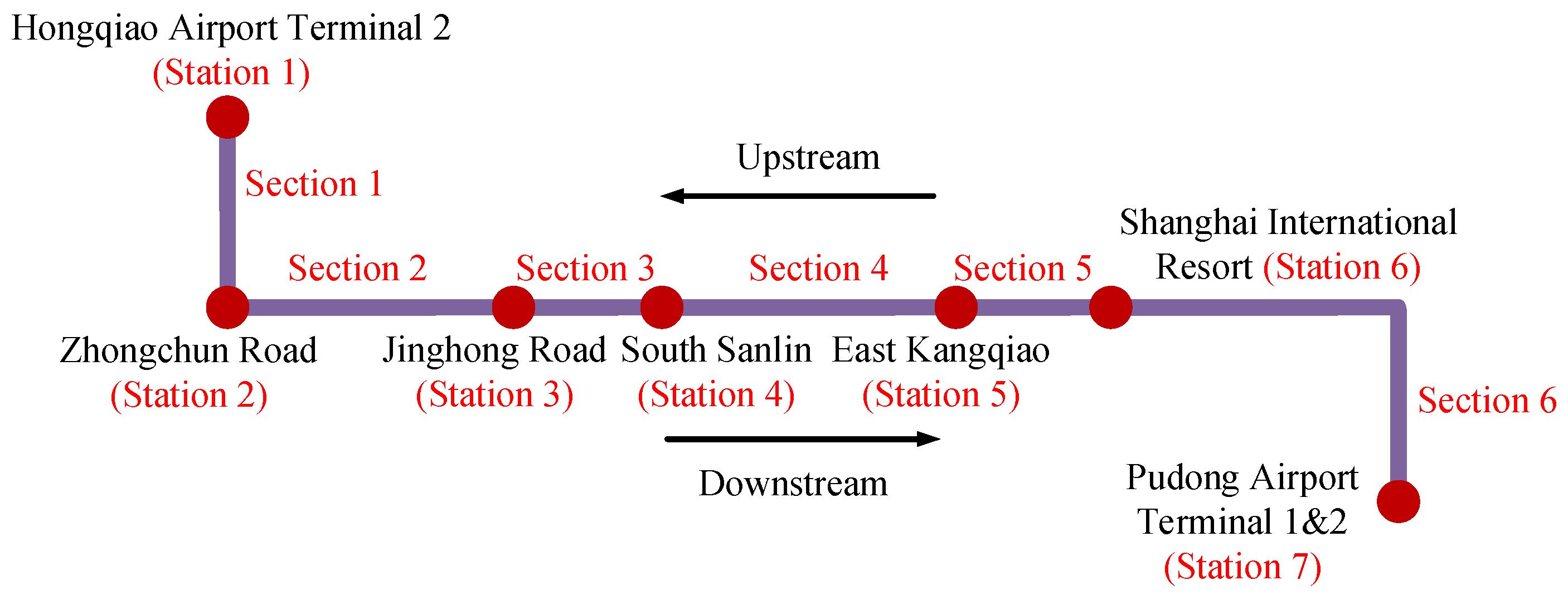
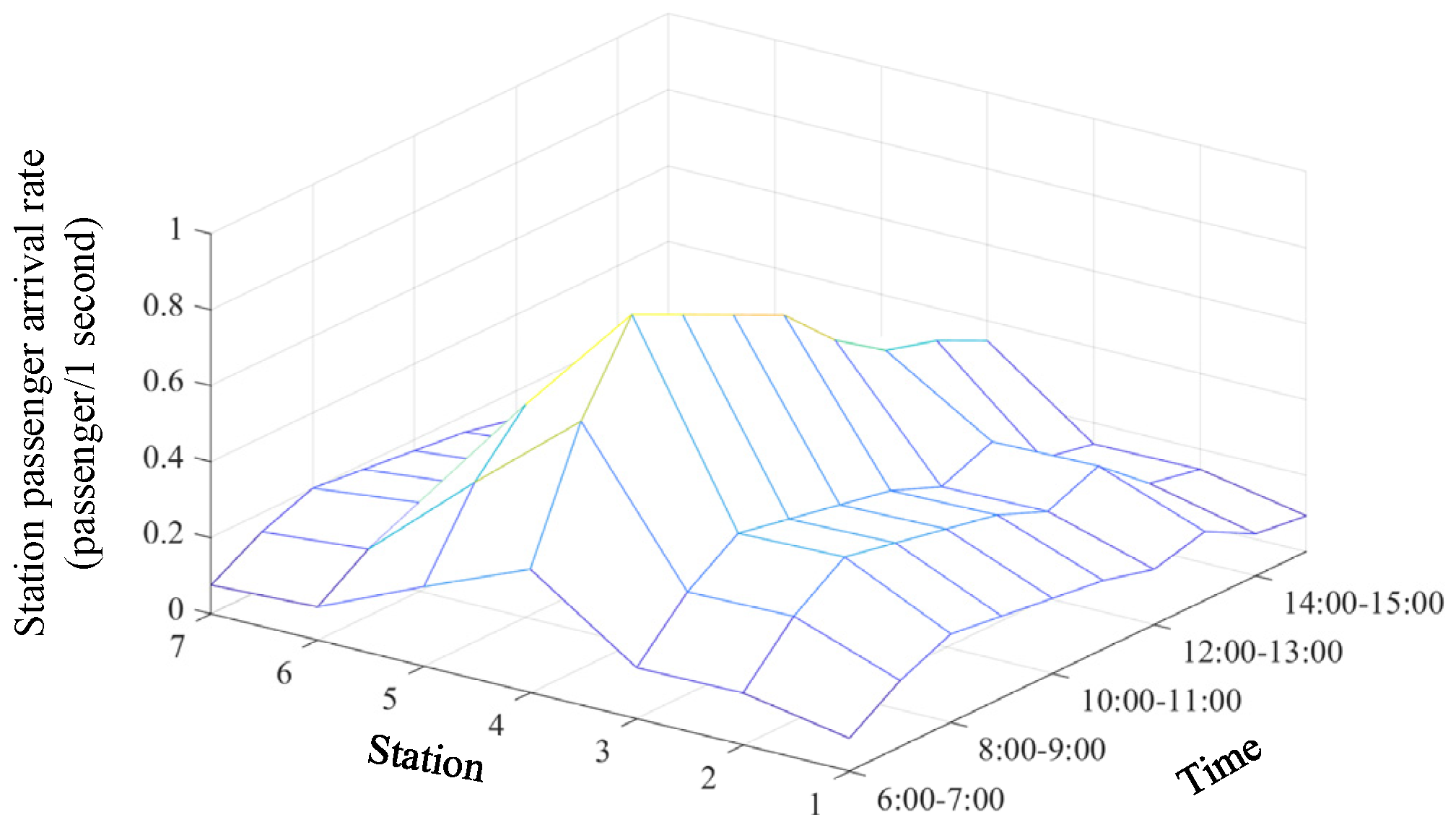
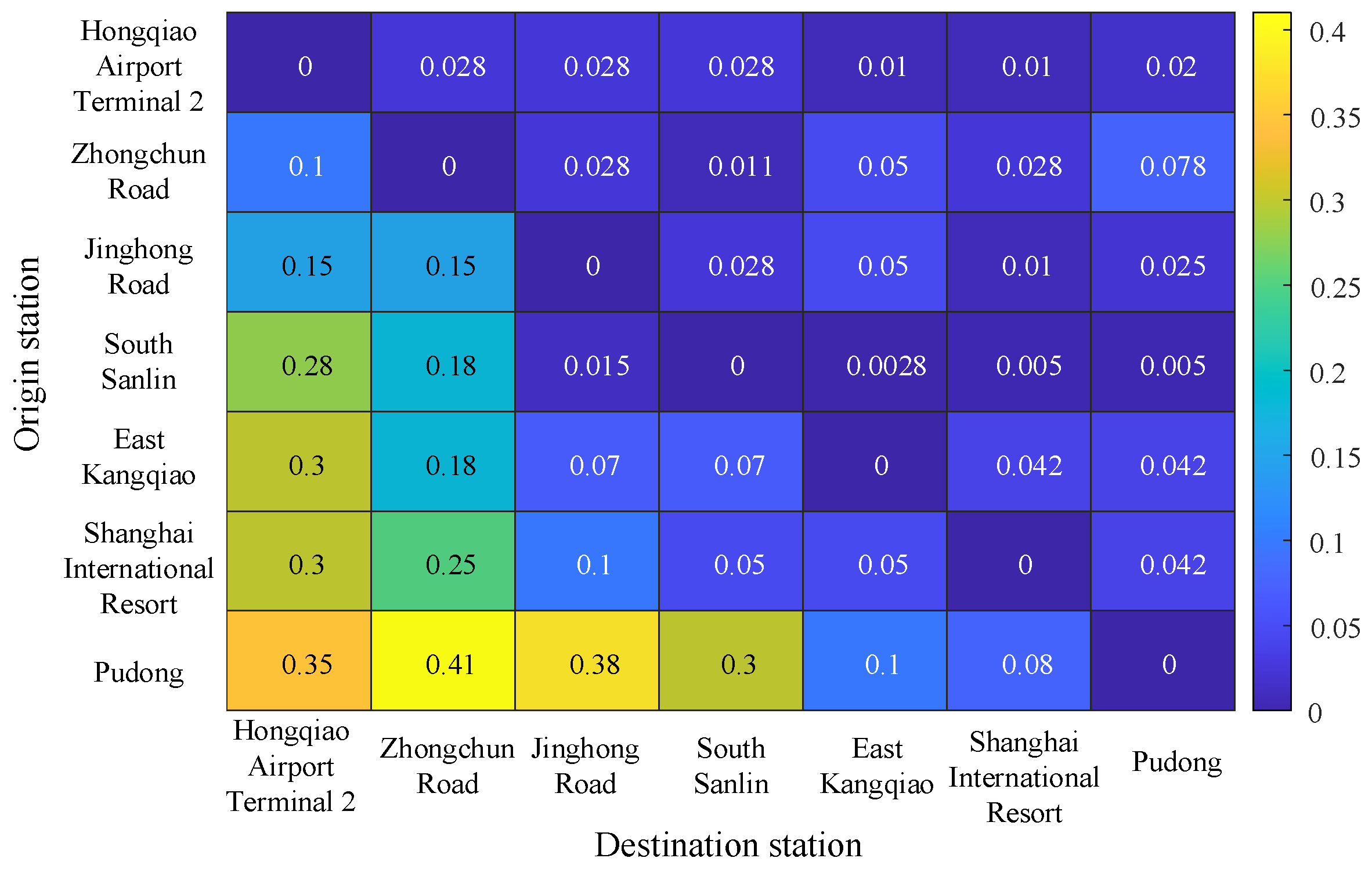
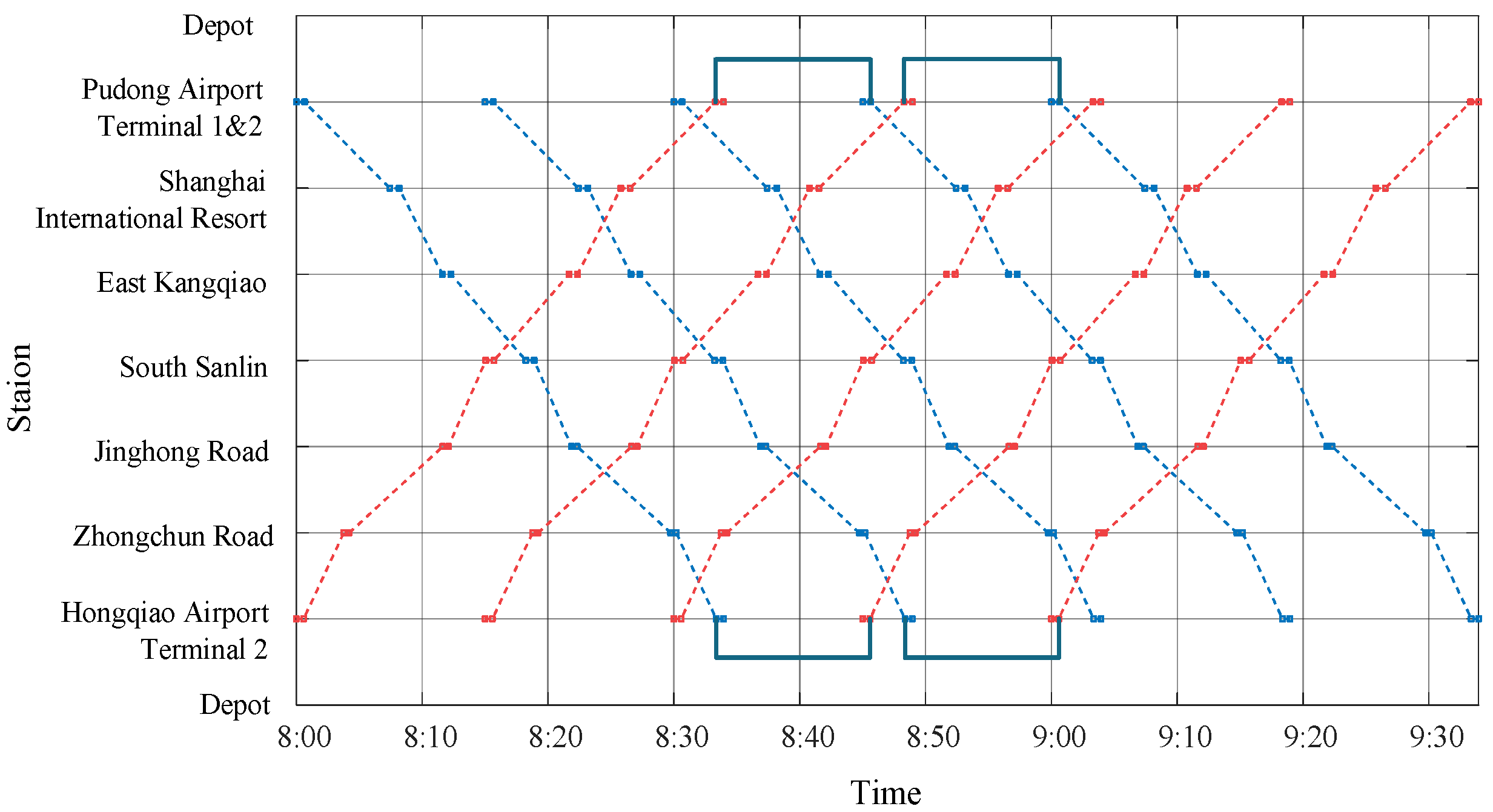
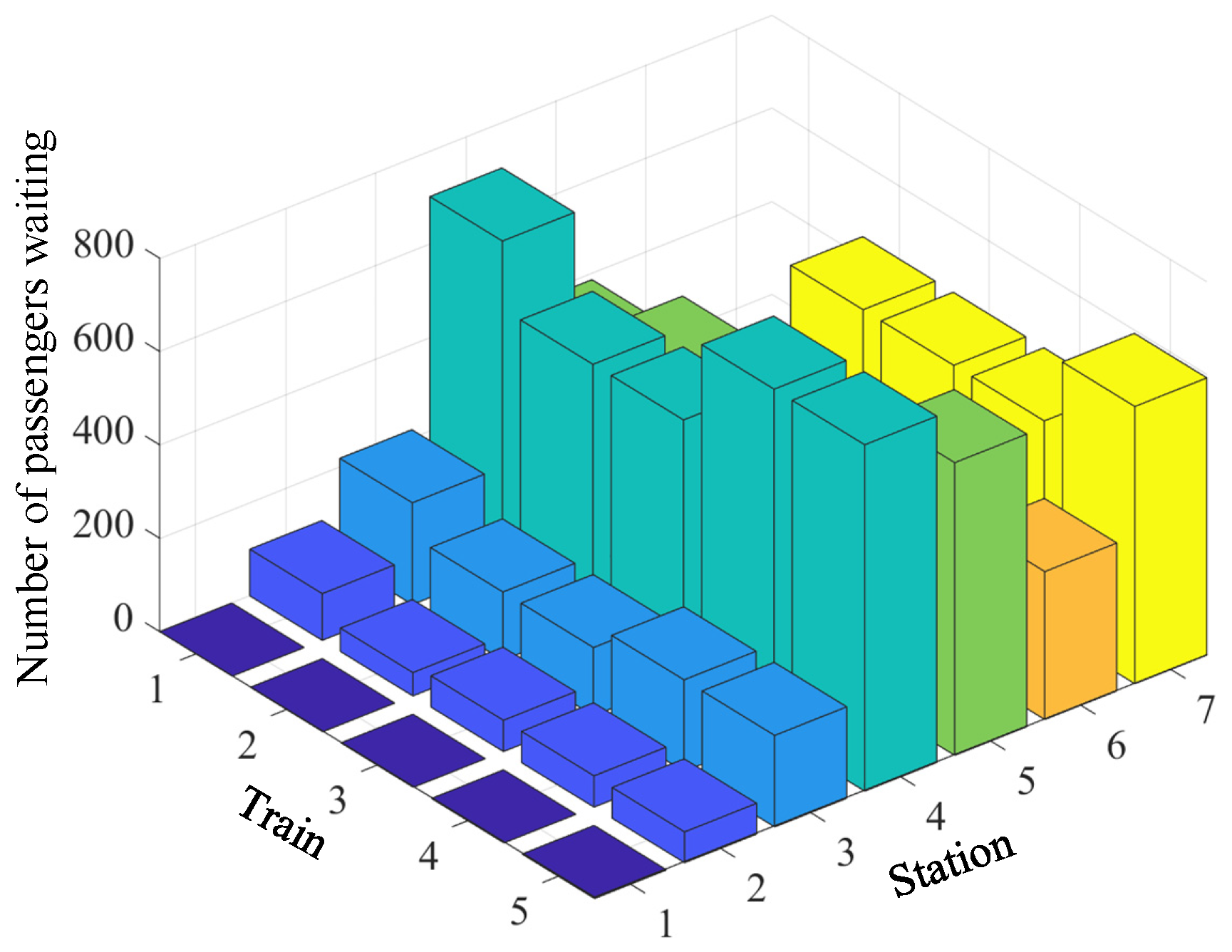

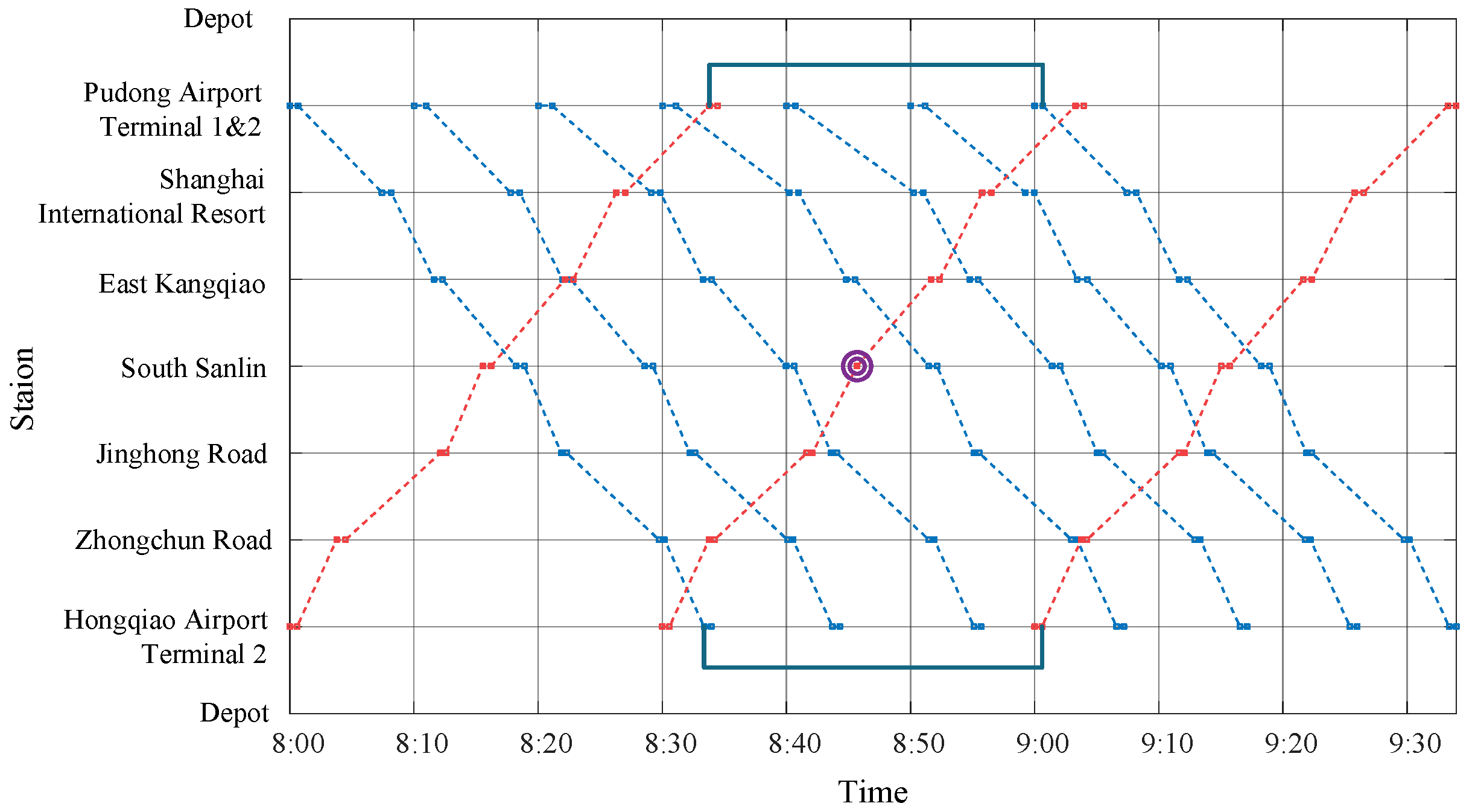
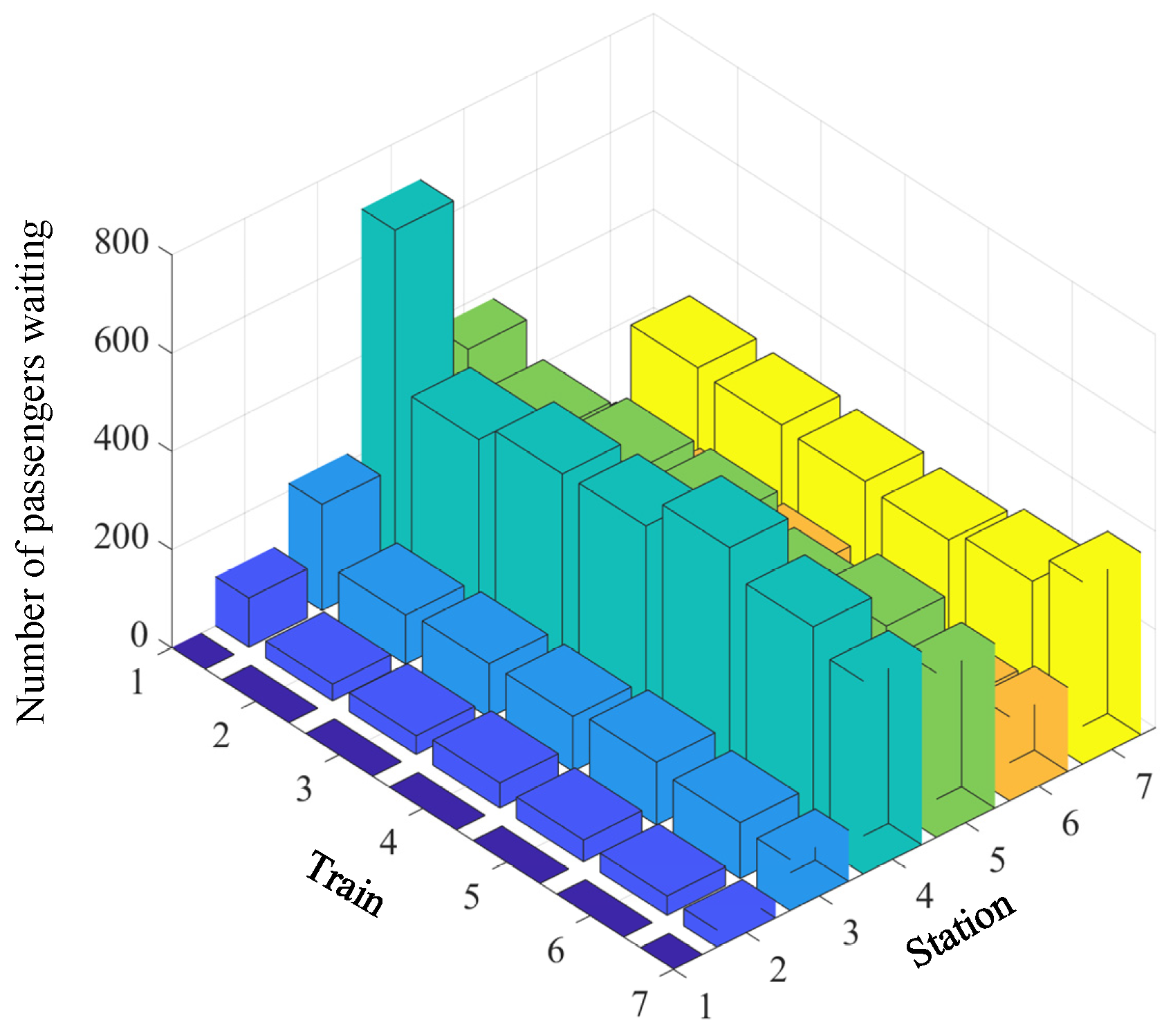

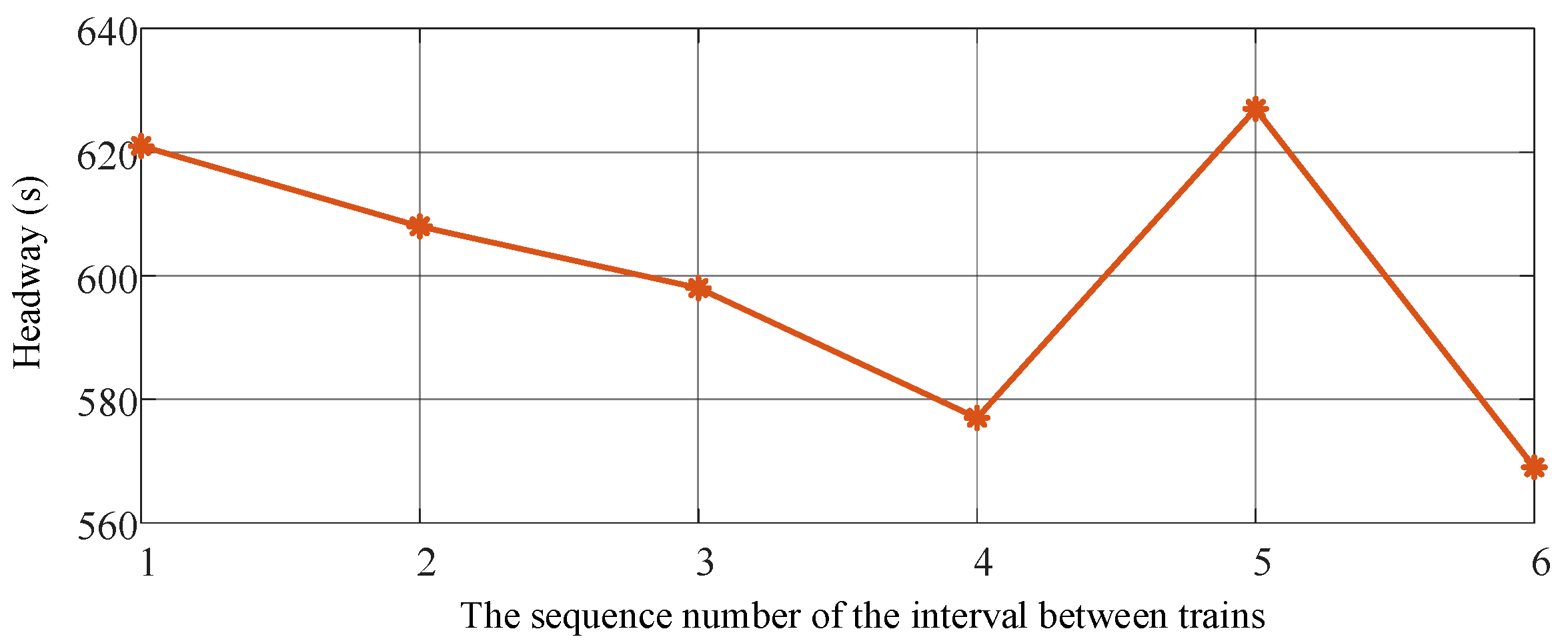
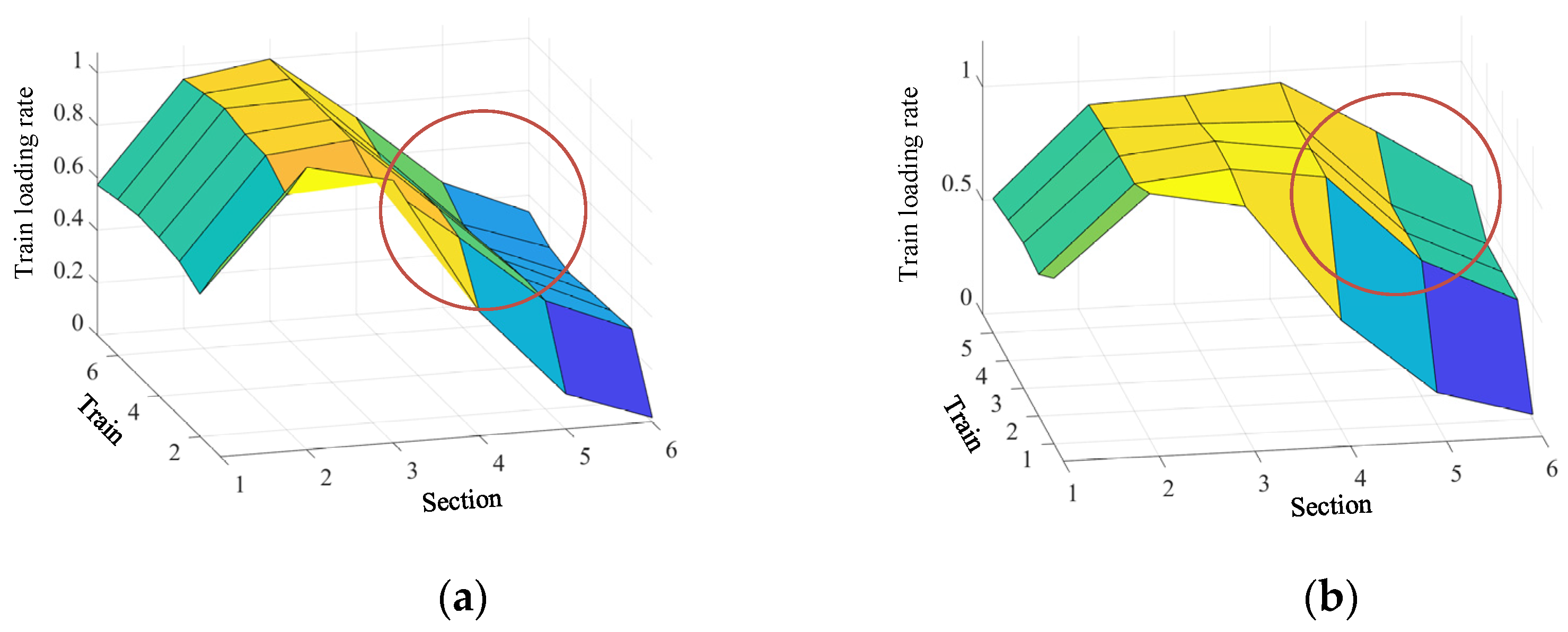

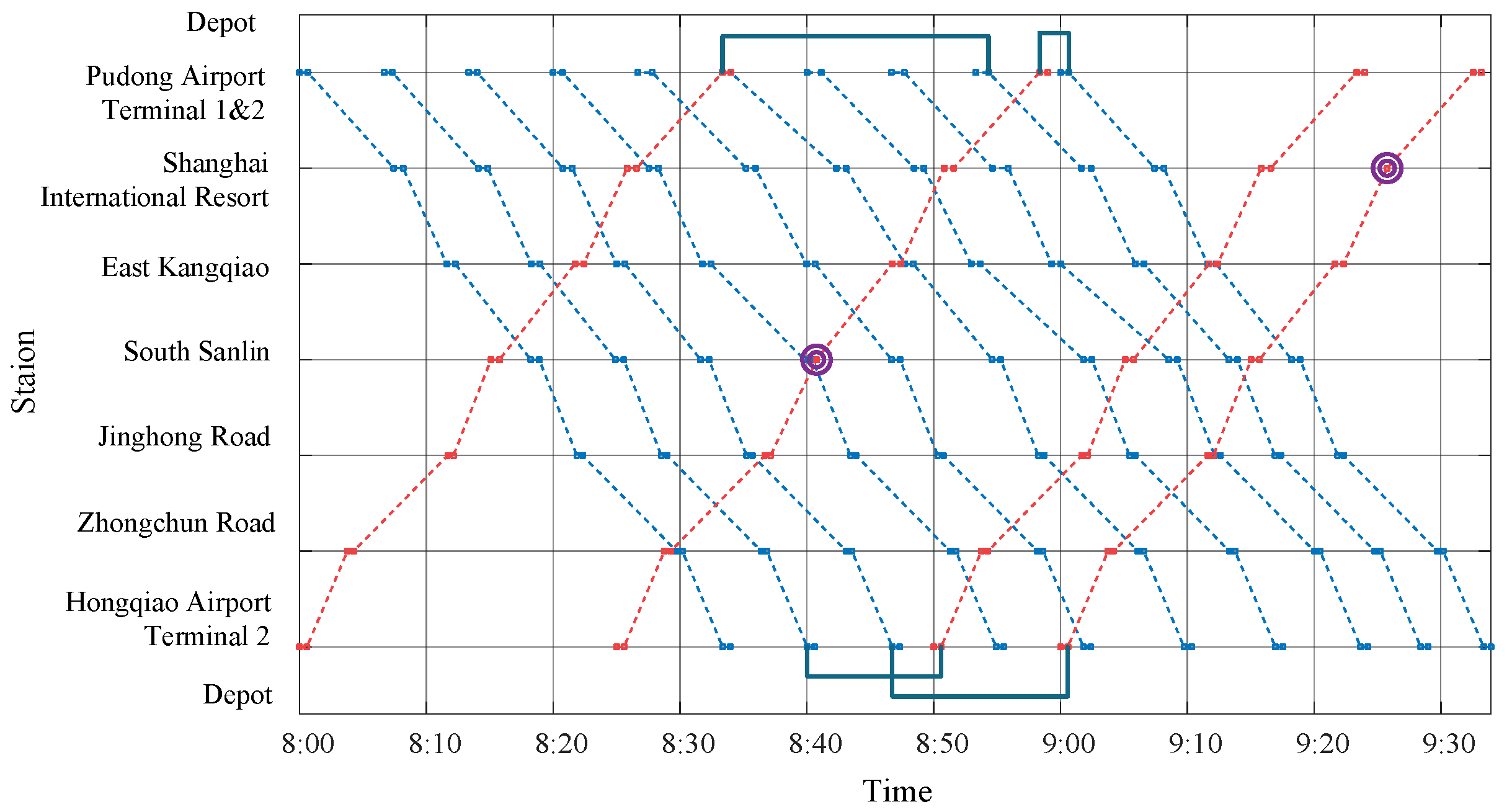
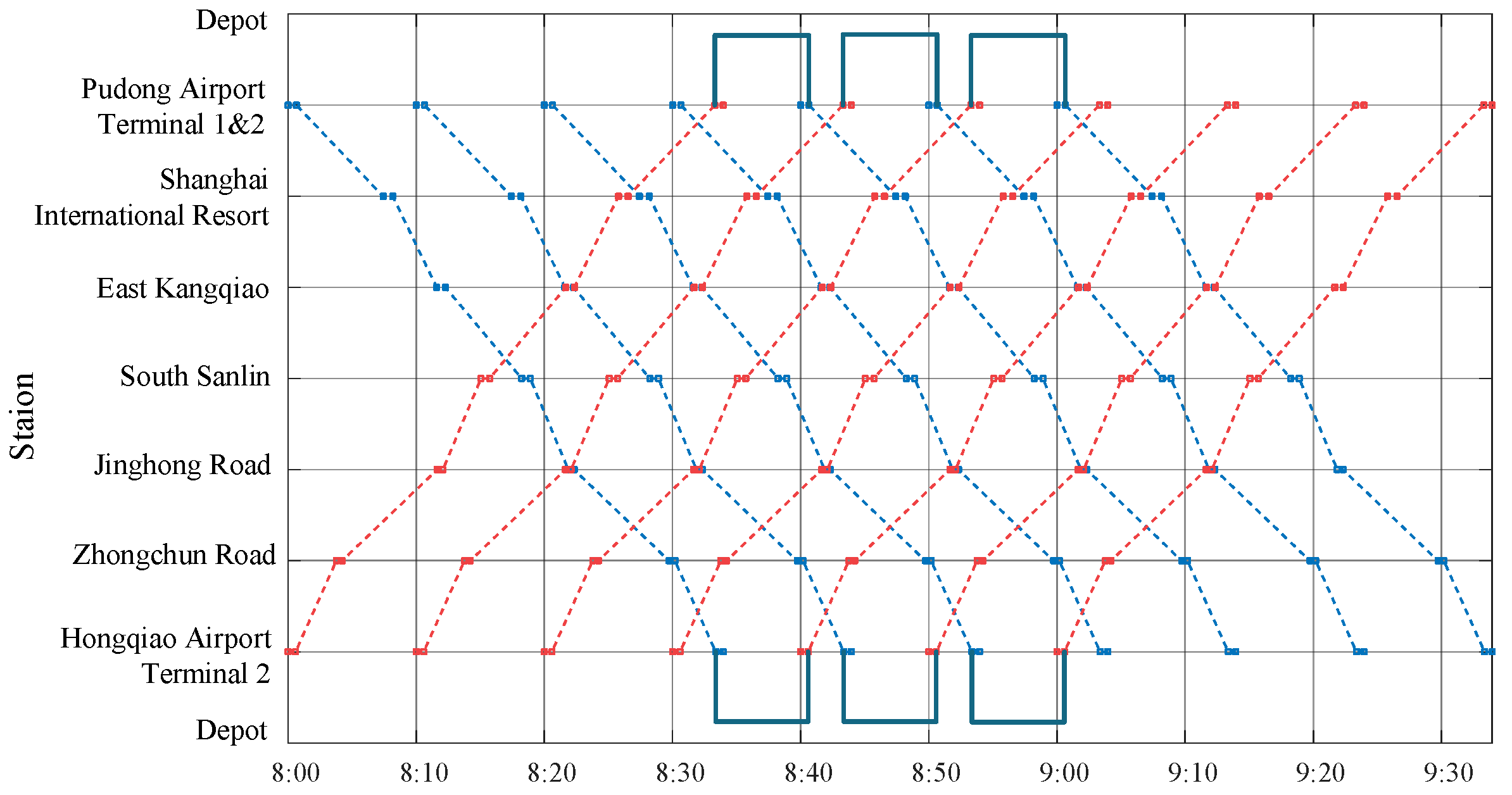

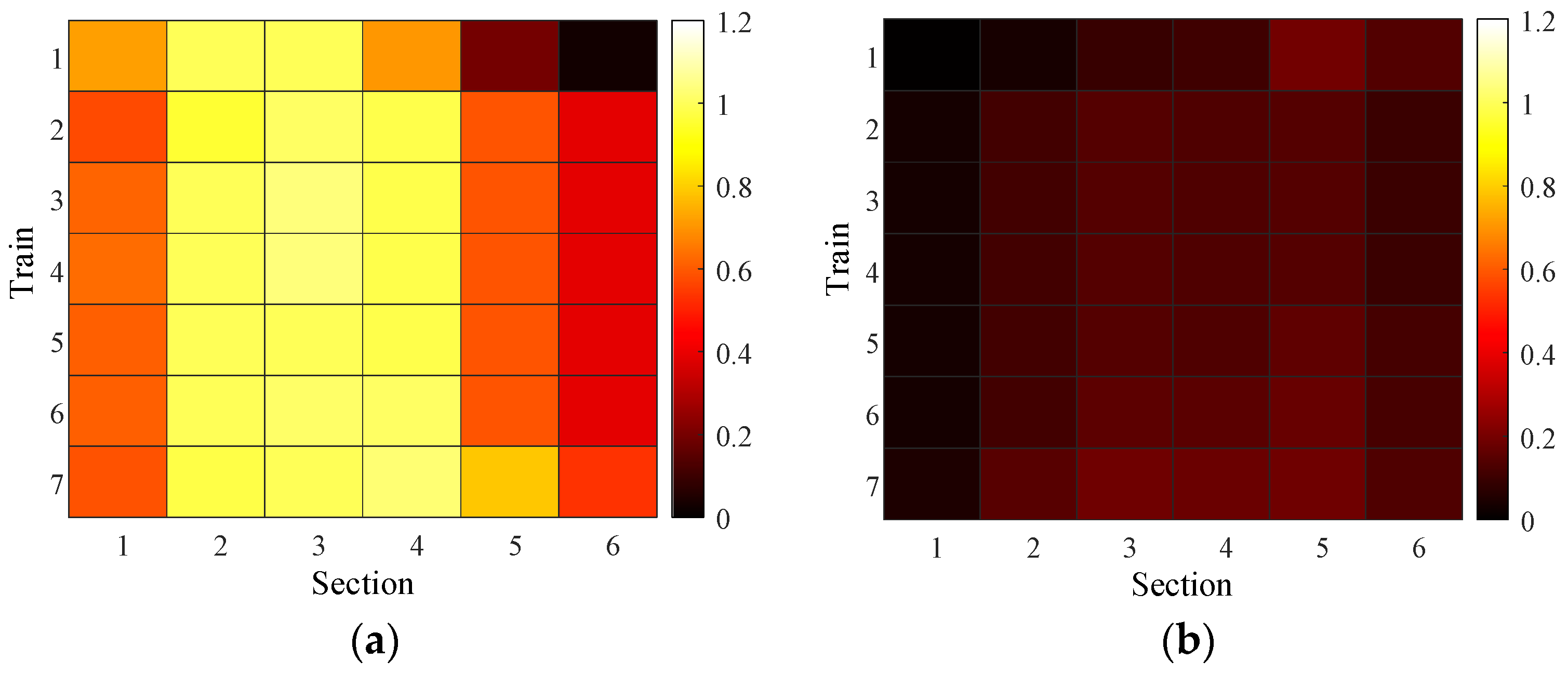
| Station | Minimum Dwell Time (s) | Maximum Dwell Time (s) | Maximum Running Time (s) | Minimum Running Time (s) |
|---|---|---|---|---|
| Hongqiao Airport Terminal 2 | 35 | 65 | - | - |
| Zhongchun Road | 27 | 57 | 382 | 191 |
| Jinghong Road | 27 | 57 | 890 | 445 |
| South Sanlin | 40 | 70 | 356 | 178 |
| East Kangqiao | 41 | 71 | 714 | 357 |
| Shanghai International Resort | 45 | 75 | 412 | 206 |
| Pudong Airport Terminal 1&2 | 39 | 69 | 812 | 406 |
| Hmax | Hmin | Ida | Idt | Ita | bmin |
|---|---|---|---|---|---|
| 1800 (s) | 400 (s) | 90 (s) | 90 (s) | 90 (s) | 90 (s) |
| Total Travel Time (s) | Number of Stops | Stranded Passengers | Objective Value (Yuan) | ||||
|---|---|---|---|---|---|---|---|
| Upstream * | Downstream * | Upstream * | Downstream * | Upstream * | Downstream * | ||
| TUOS-ELS | 14,886 | 6139 | 49 | 20 | 196 | 91 | 34,245 |
| T-TPOS | 10,185 | 10,185 | 35 | 35 | 2489 | 0 | 55,760 |
| Total Waiting Time (h) | Total On-Board Time (h) | Calculation Time (s) | Gap of GUROBI | |||
|---|---|---|---|---|---|---|
| Upstream | Downstream | Upstream | Downstream | |||
| TUOS-ELS | 1841.1 | 723.6 | 2666.5 | 330.8 | 1000 | 0.45% |
| T-TPOS | 2581.1 | 388.7 | 2189.9 | 338.8 | - | - |
| Total Travel Time (s) | Number of Stops | Stranded Passengers | Objective Value (Yuan) | ||||
|---|---|---|---|---|---|---|---|
| Upstream * | Downstream * | Upstream * | Downstream * | Upstream * | Downstream * | ||
| TUOS-ELS | 21,293 | 8111 | 70 | 26 | 866 | 210 | 54,564 |
| T-TPOS | 14,259 | 14,259 | 49 | 49 | 4109 | 0 | 84,380 |
| Total Waiting Time (h) | Total On-Board Time (h) | Calculation Time (s) | Gap of GUROBI | |||
|---|---|---|---|---|---|---|
| Upstream | Downstream | Upstream | Downstream | |||
| TUOS-ELS | 2059.4 | 804.3 | 3876.2 | 437.8 | 1000 | 0.5% |
| T-TPOS | 2770.1 | 421.1 | 3121.4 | 496.2 | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, W.; Sun, P.; Yao, B.; Ding, R. A Train Timetable Optimization Method Considering Multi-Strategies for the Tidal Passenger Flow Phenomenon. Appl. Sci. 2024, 14, 11963. https://doi.org/10.3390/app142411963
Jin W, Sun P, Yao B, Ding R. A Train Timetable Optimization Method Considering Multi-Strategies for the Tidal Passenger Flow Phenomenon. Applied Sciences. 2024; 14(24):11963. https://doi.org/10.3390/app142411963
Chicago/Turabian StyleJin, Wenbin, Pengfei Sun, Bailing Yao, and Rongjun Ding. 2024. "A Train Timetable Optimization Method Considering Multi-Strategies for the Tidal Passenger Flow Phenomenon" Applied Sciences 14, no. 24: 11963. https://doi.org/10.3390/app142411963
APA StyleJin, W., Sun, P., Yao, B., & Ding, R. (2024). A Train Timetable Optimization Method Considering Multi-Strategies for the Tidal Passenger Flow Phenomenon. Applied Sciences, 14(24), 11963. https://doi.org/10.3390/app142411963






