Optimized Design of Pipe Elbows for Erosion Wear
Abstract
:1. Introduction
2. Numerical Analysis of the Erosion Model
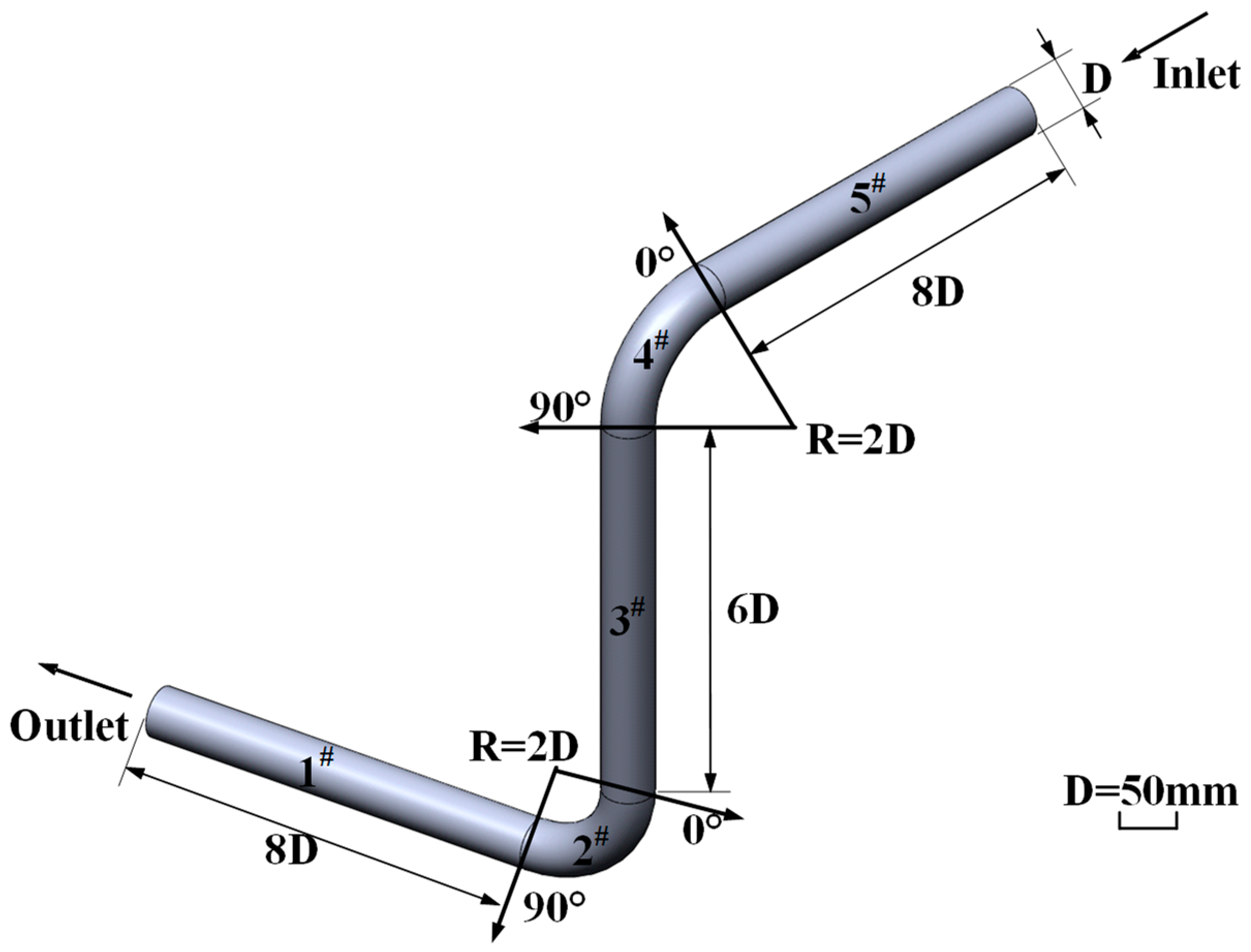
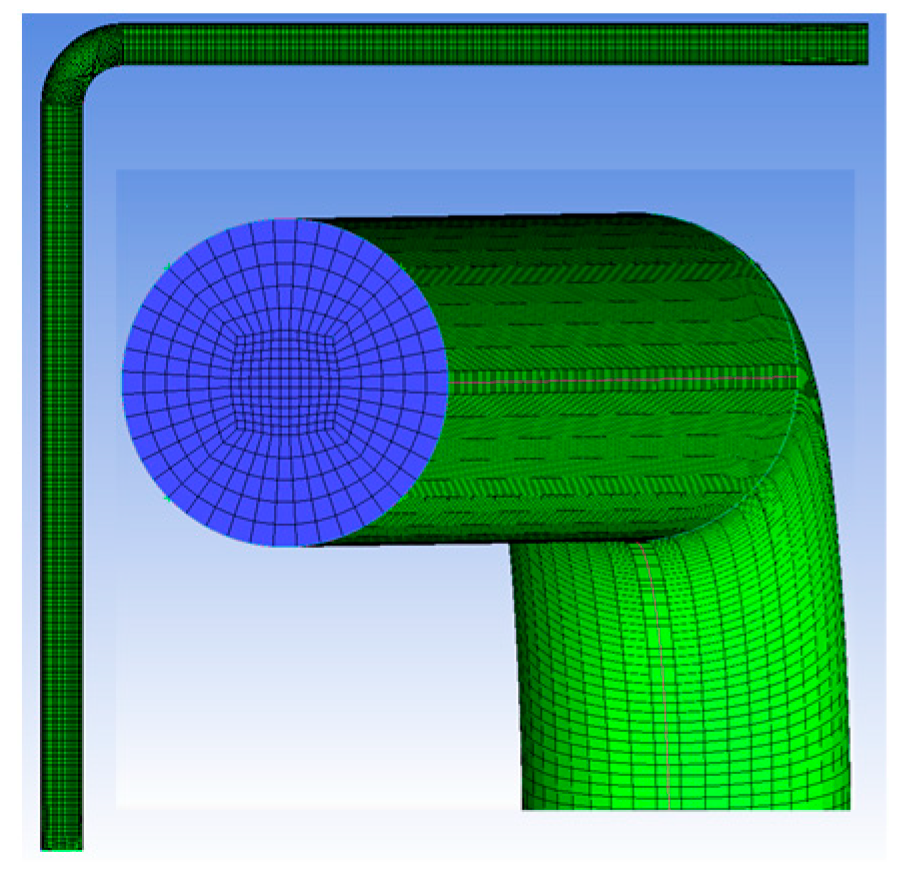
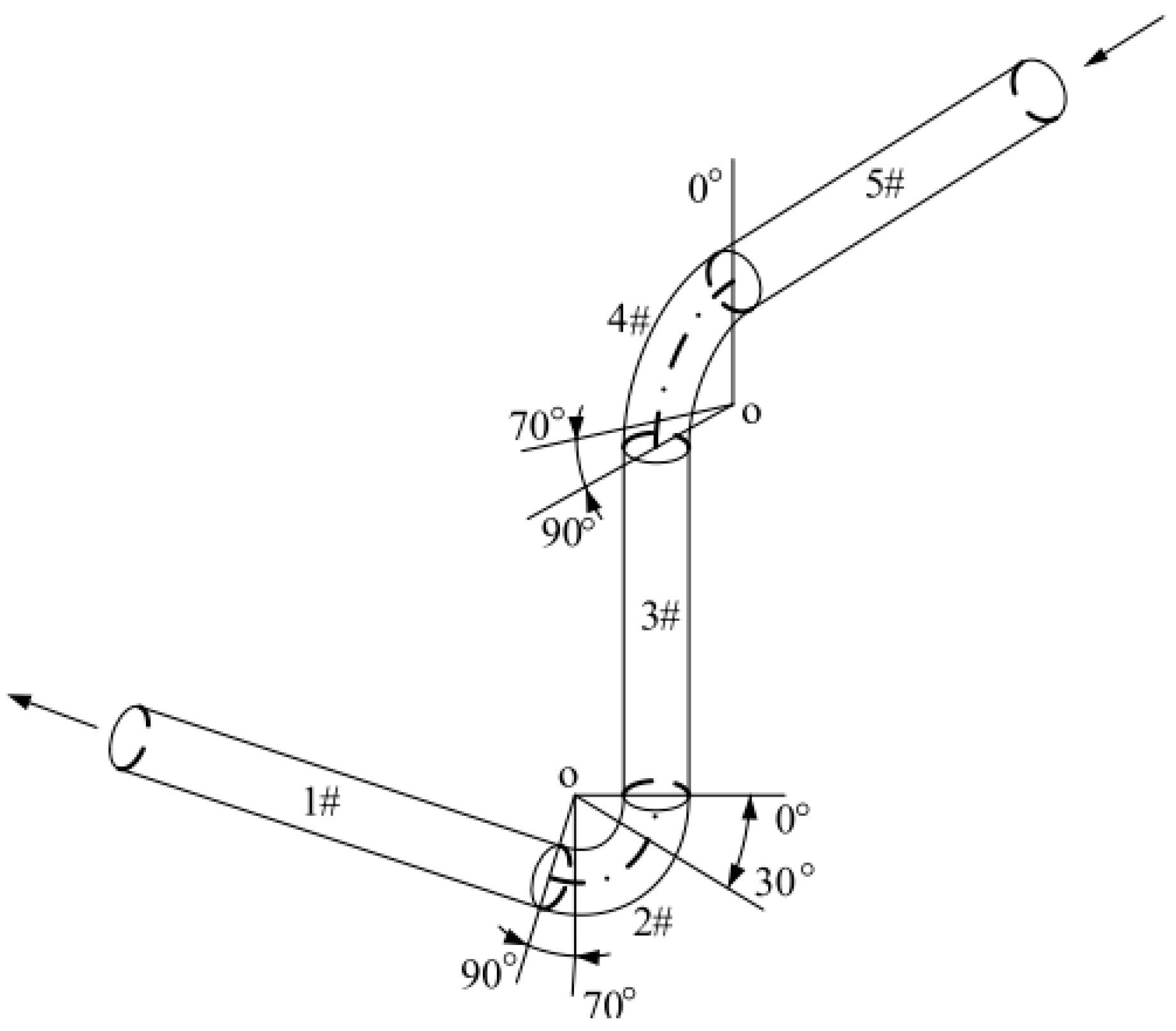
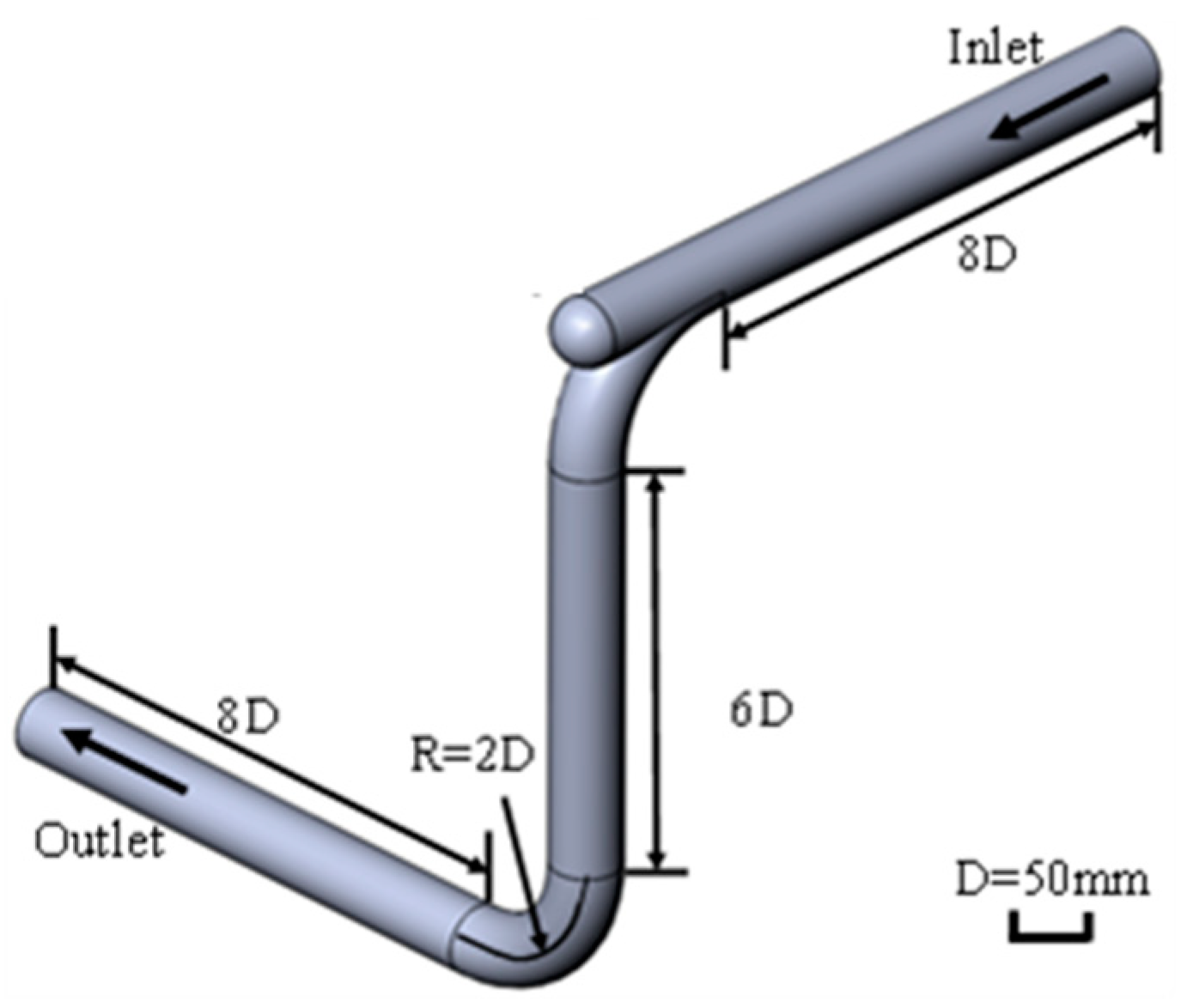
2.1. Pipe Parameters and Mesh Segmentation
2.2. Theoretical Analysis of Erosion Wear
2.2.1. Computational Model
- (1)
- Conservation of mass:
- (2)
- Momentum equation:
- (3)
- Energy equation:
2.2.2. Erosion Wear Model
2.2.3. Turbulence Model Selection
2.2.4. Wall Collision Recovery Coefficient
2.3. Boundary Condition Settings
2.4. Validation of the Numerical Model Validity
2.4.1. Calculation of Working Conditions
2.4.2. Comparison of Numerical Simulation Erosion Models
3. Analysis of the Numerical Results
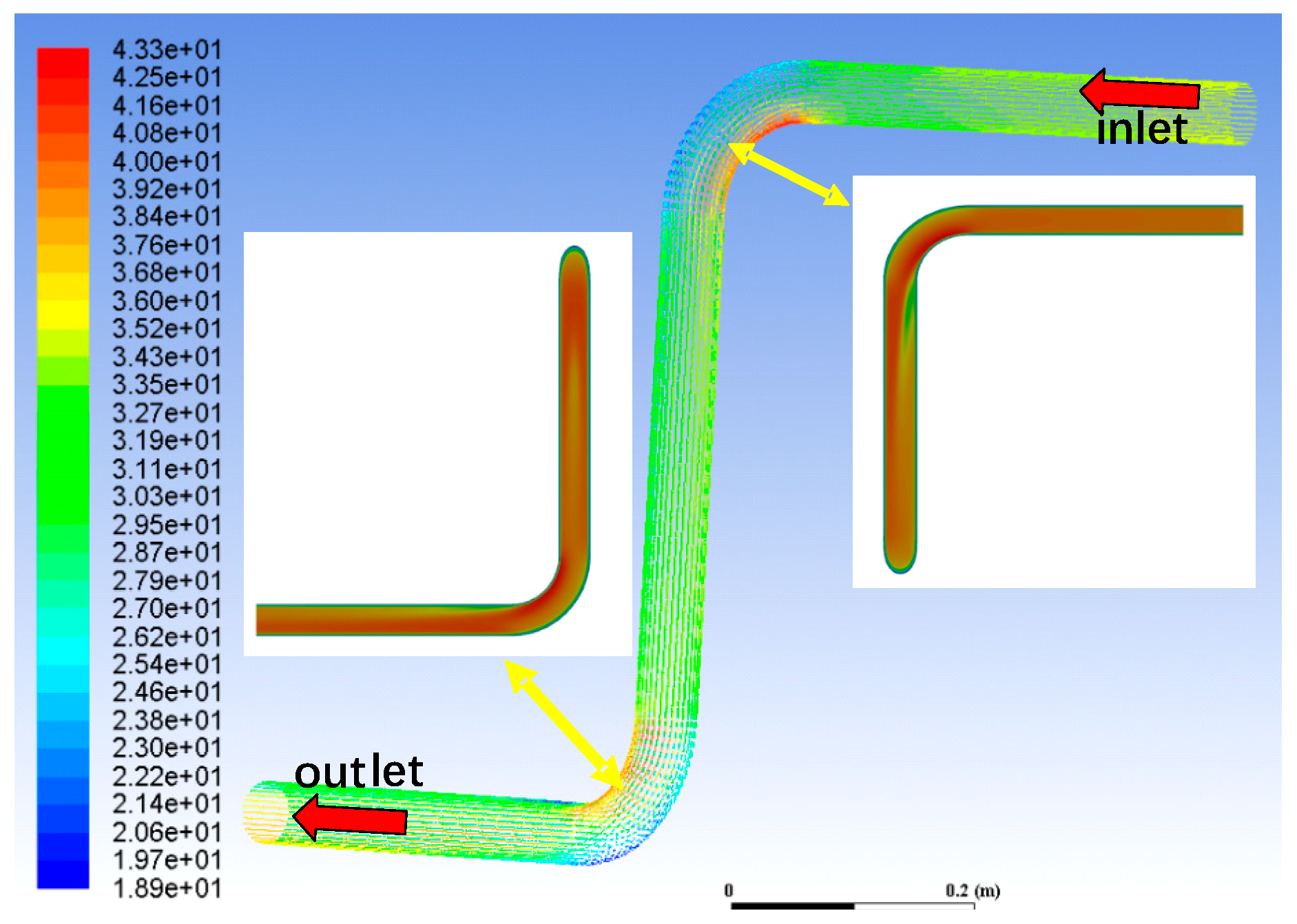
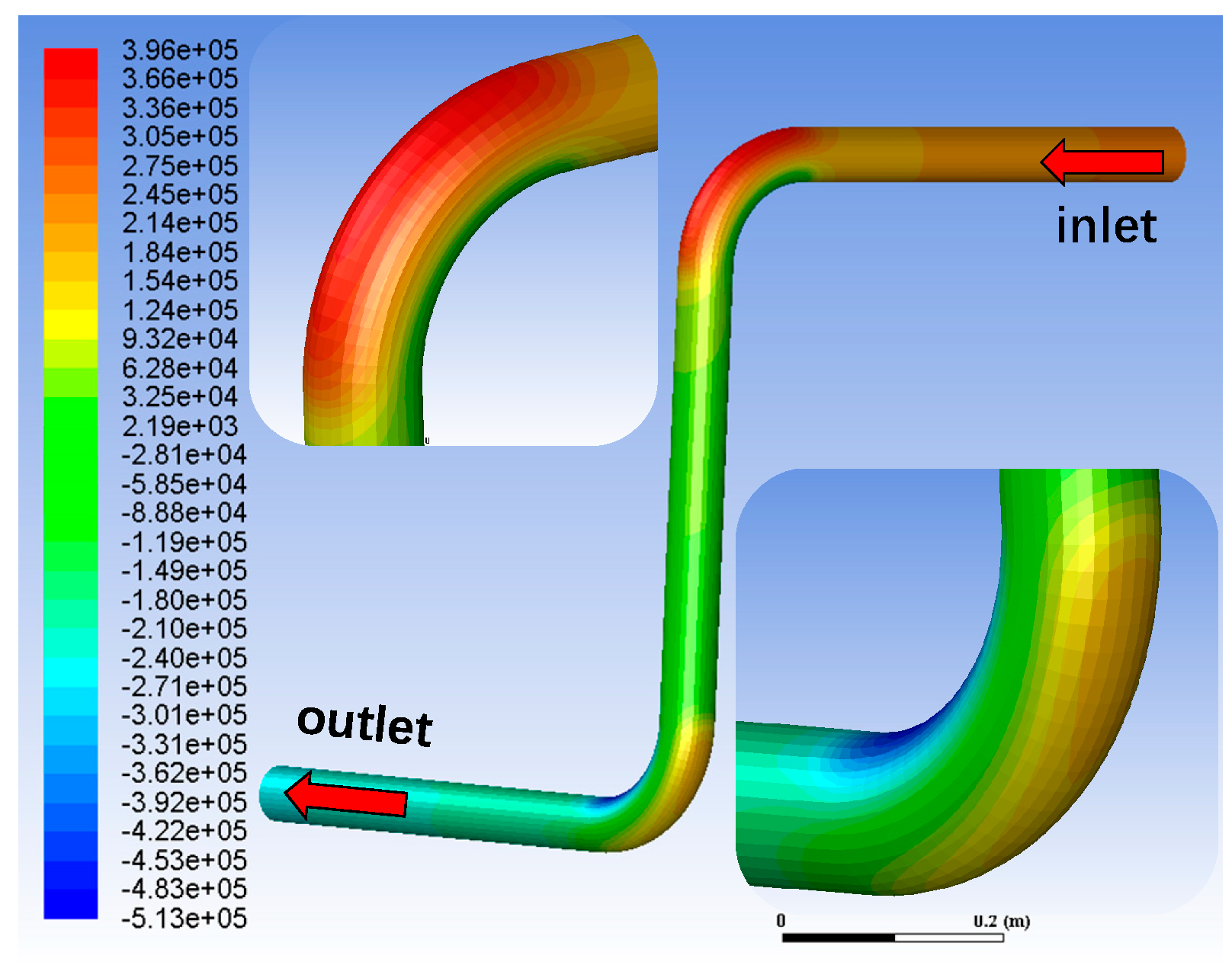
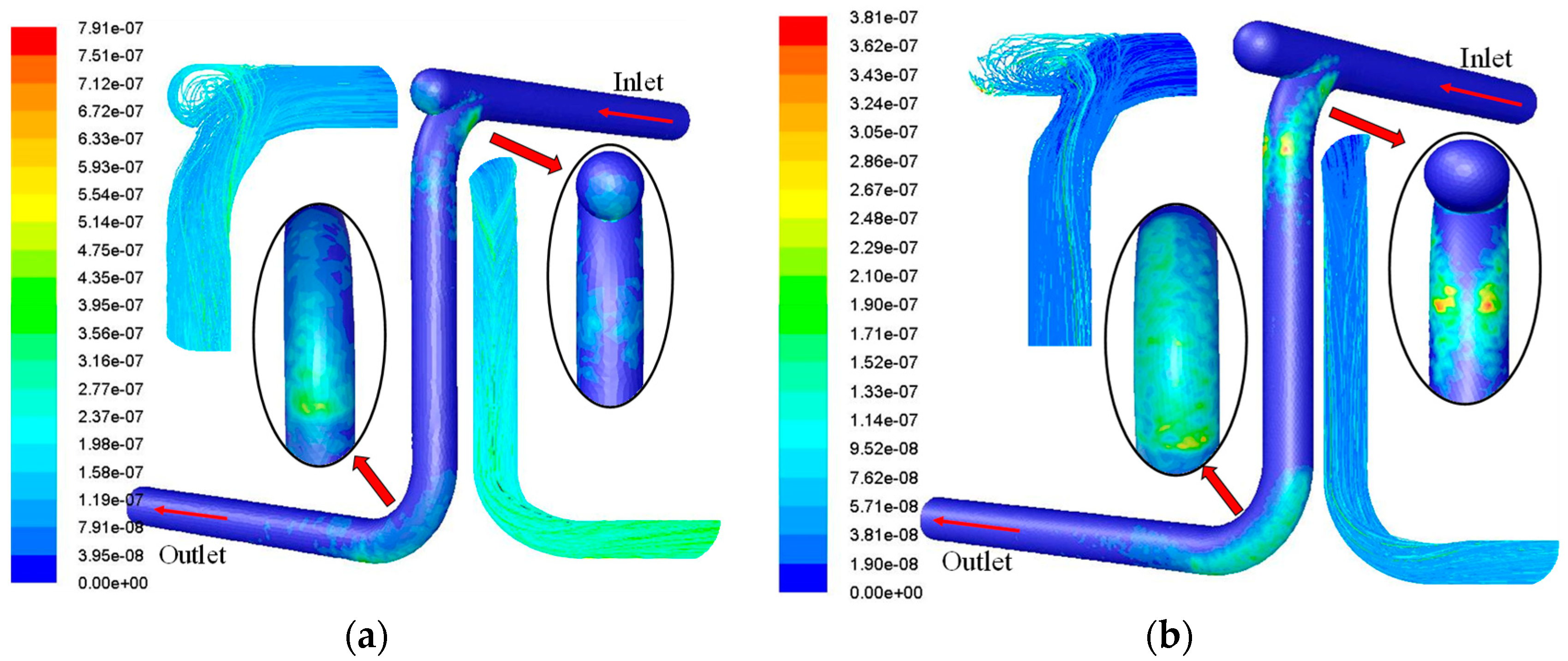
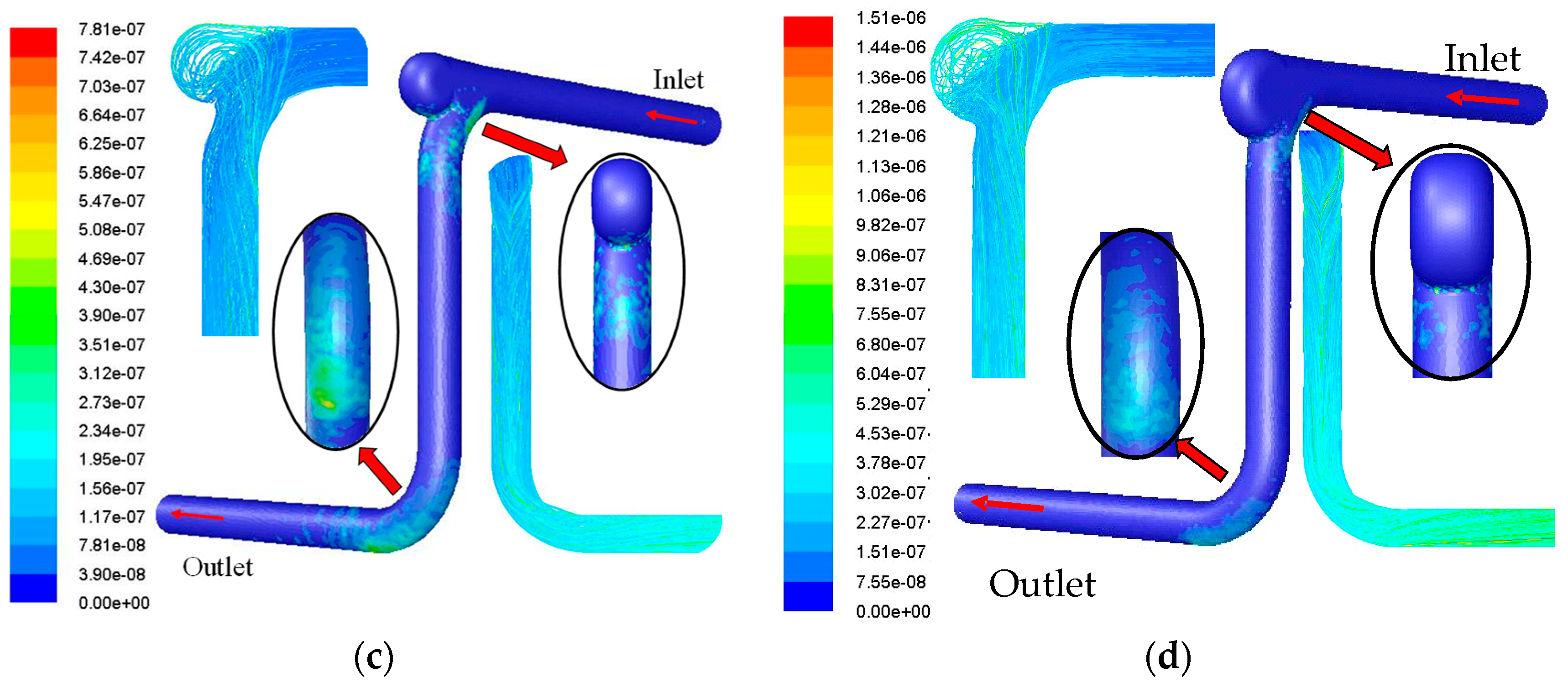
3.1. Liquid-Phase Flow Pattern
3.2. Characterization of Erosion and Wear
4. Analysis of the Influence of Structural Parameters on the Erosion and Wear of Pipe Elbows
4.1. Influence of the Bending Diameter Ratio on the Erosion Wear of Pipe Elbows
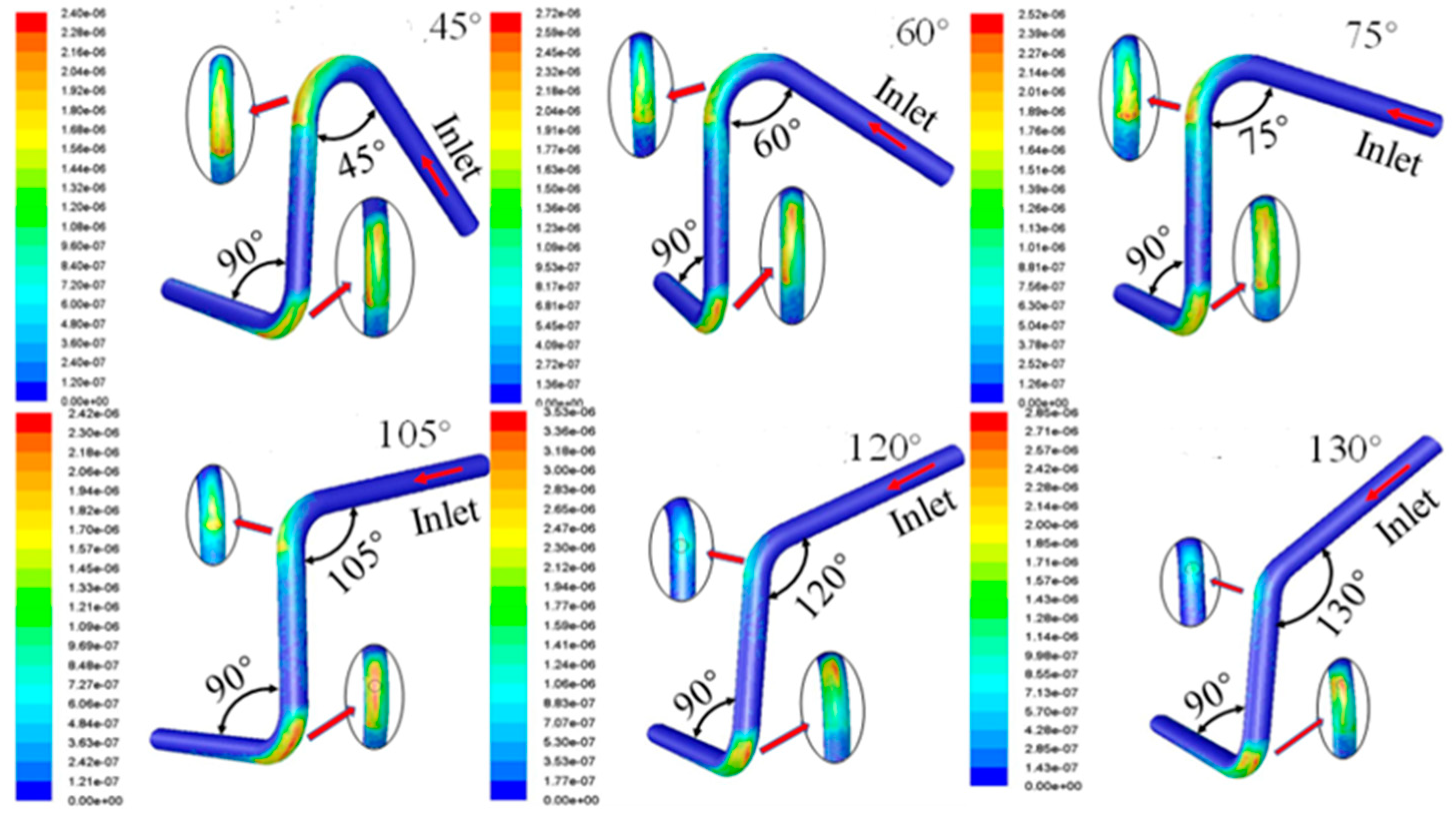
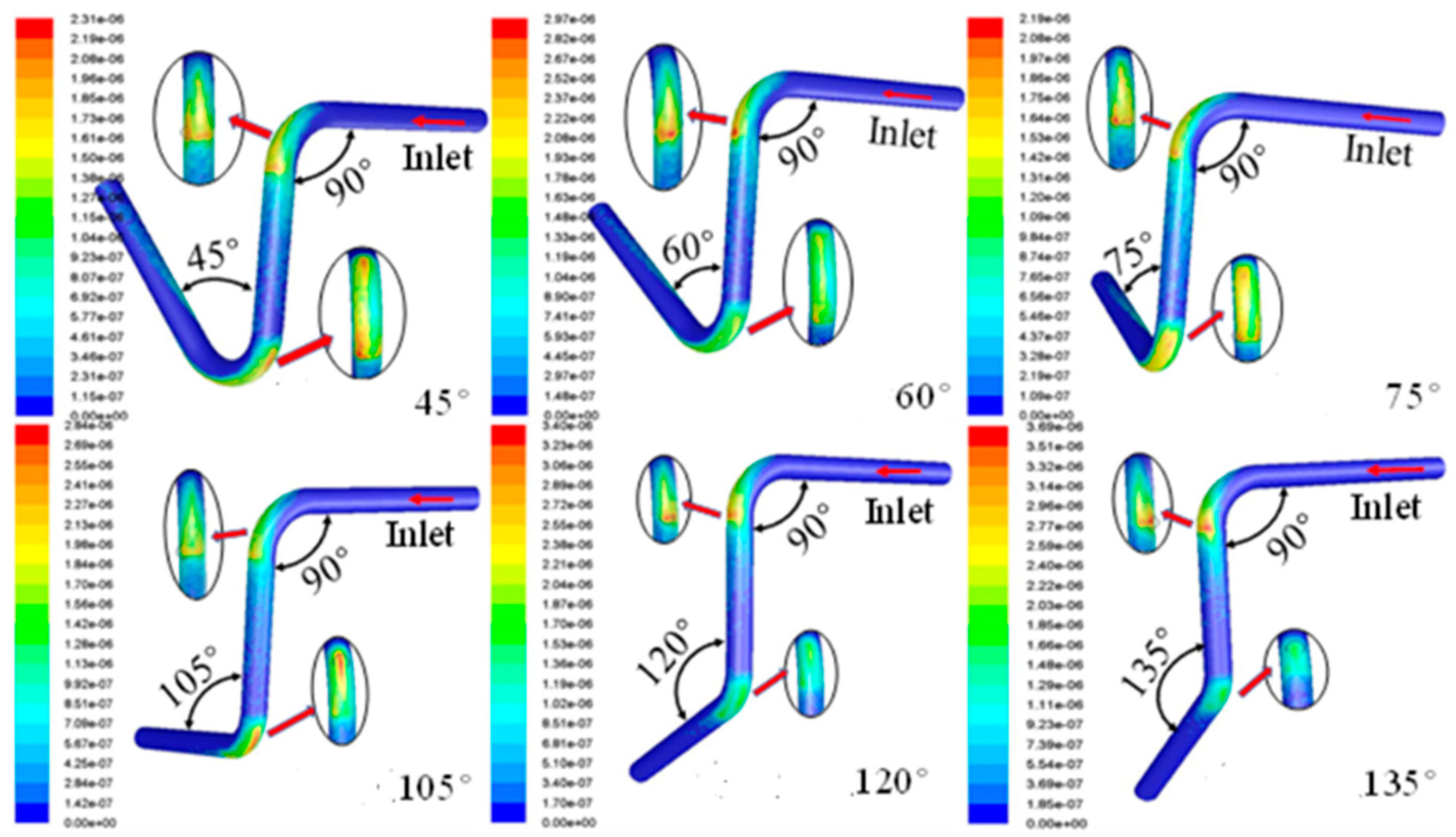
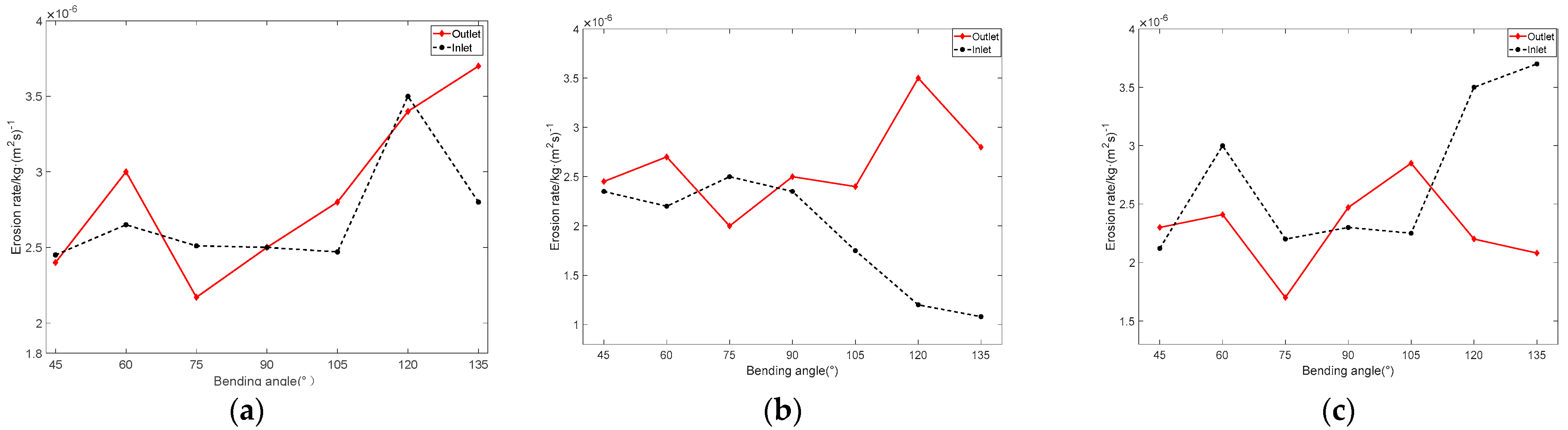
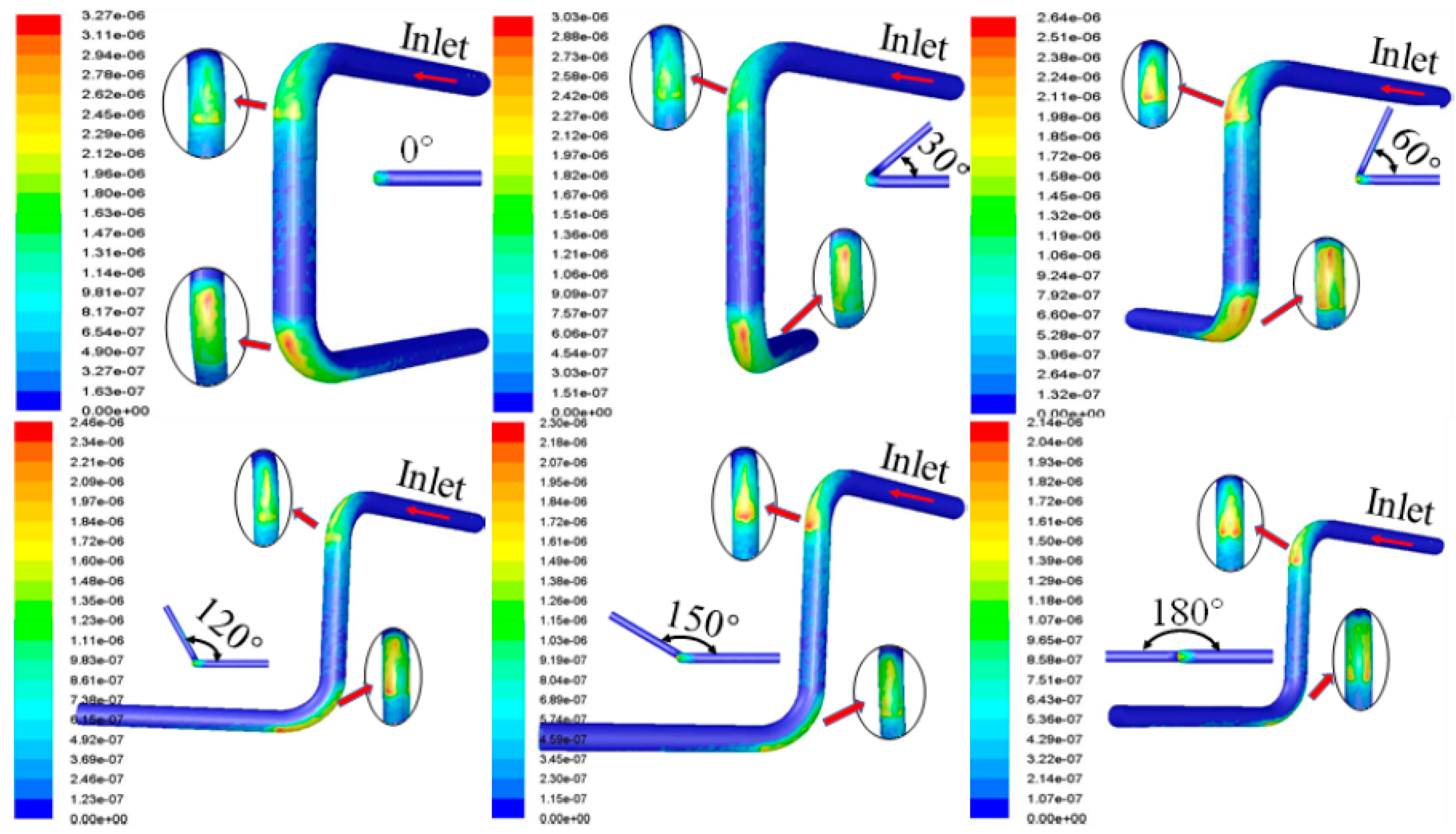
4.2. The Impact of the Bending Angle on the Pipe Elbow Erosion Wear
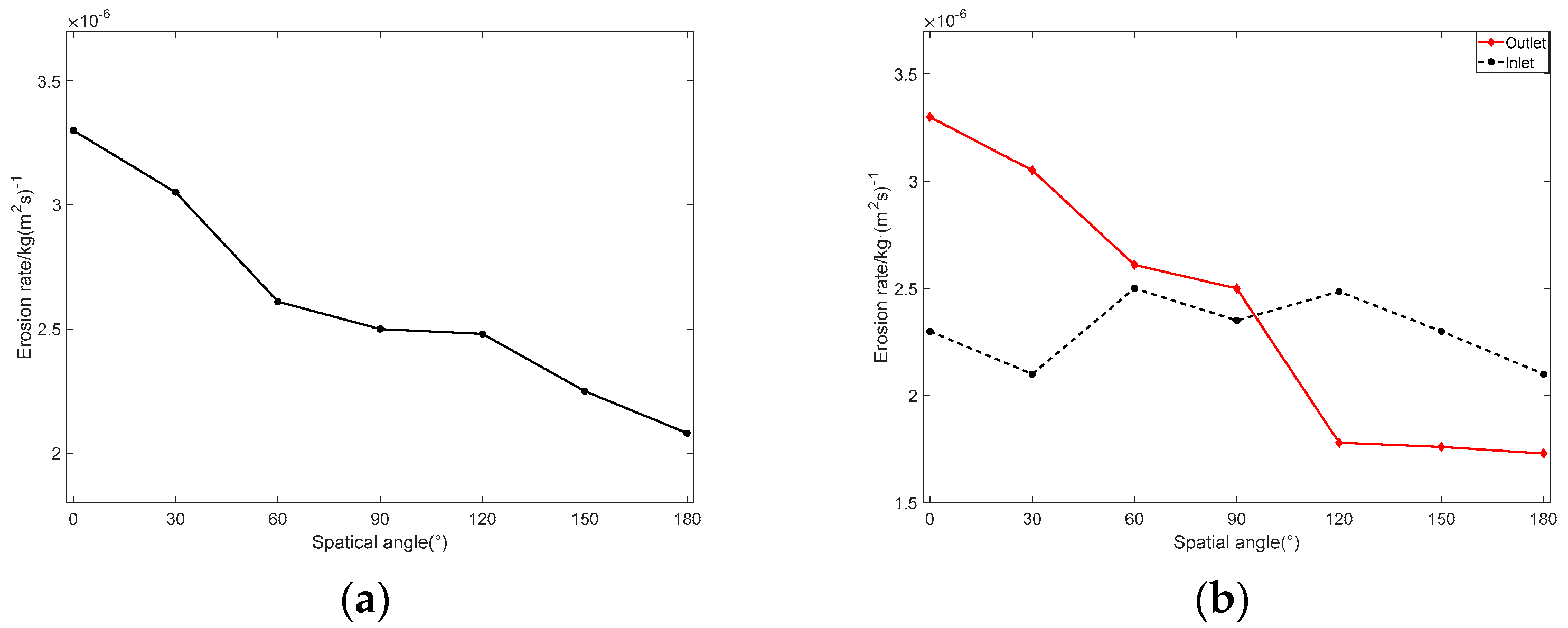
4.3. The Impact of the Spatial Angle on Pipe Elbow Erosion Wear
5. Analysis of the Erosion Resistance of Different Designs of Elbow Pipe Types
5.1. Optimization of the Erosion Resistance of Blind Bends
- (1)
- Geometric model
- (2)
- Initial boundary conditions and meshing
- (3)
- Numerical simulation results and analysis
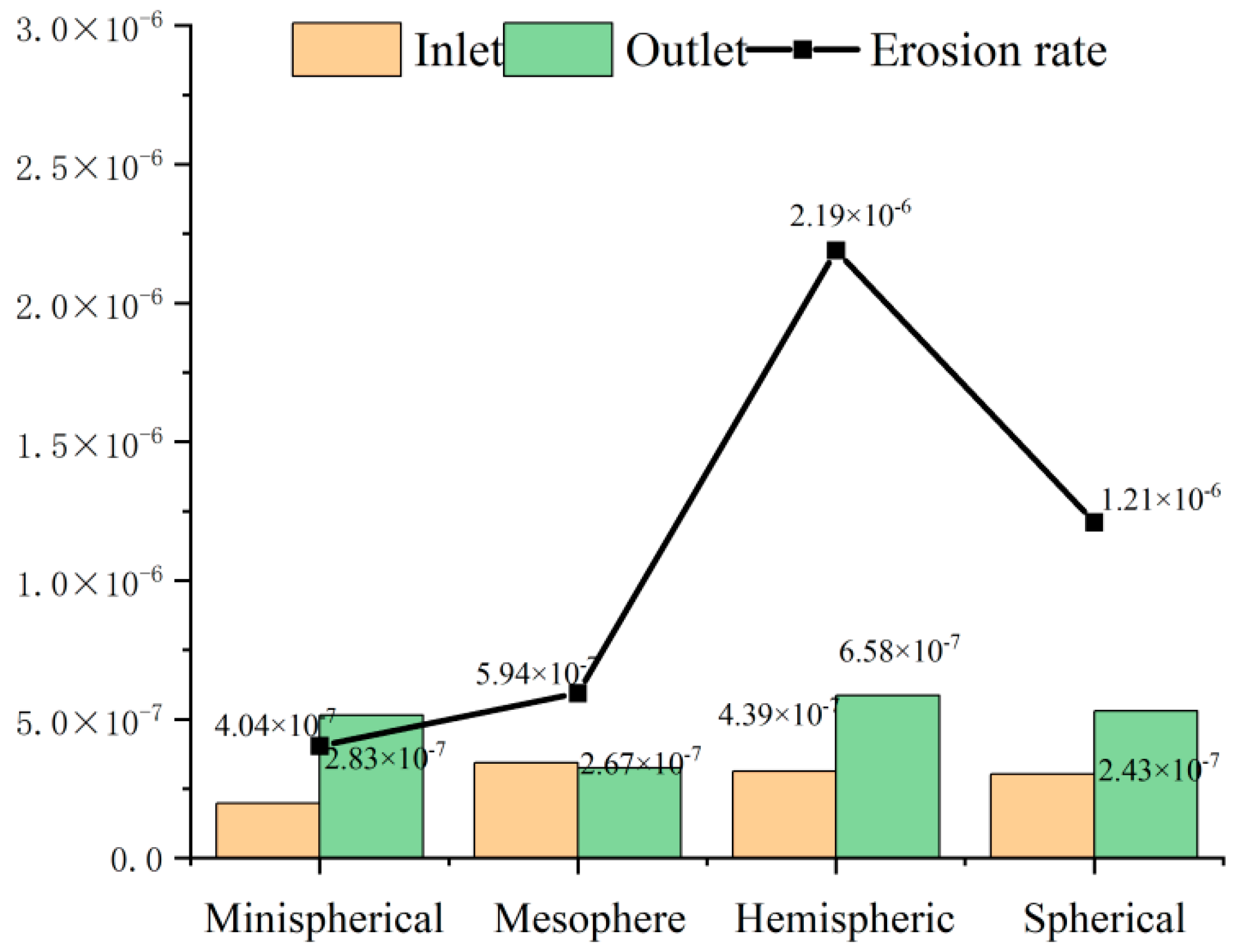
5.2. Optimization of the Erosion Resistance of a Spherical Bend
- (1)
- Geometric model
- (2)
- Numerical simulation calculation results and analysis
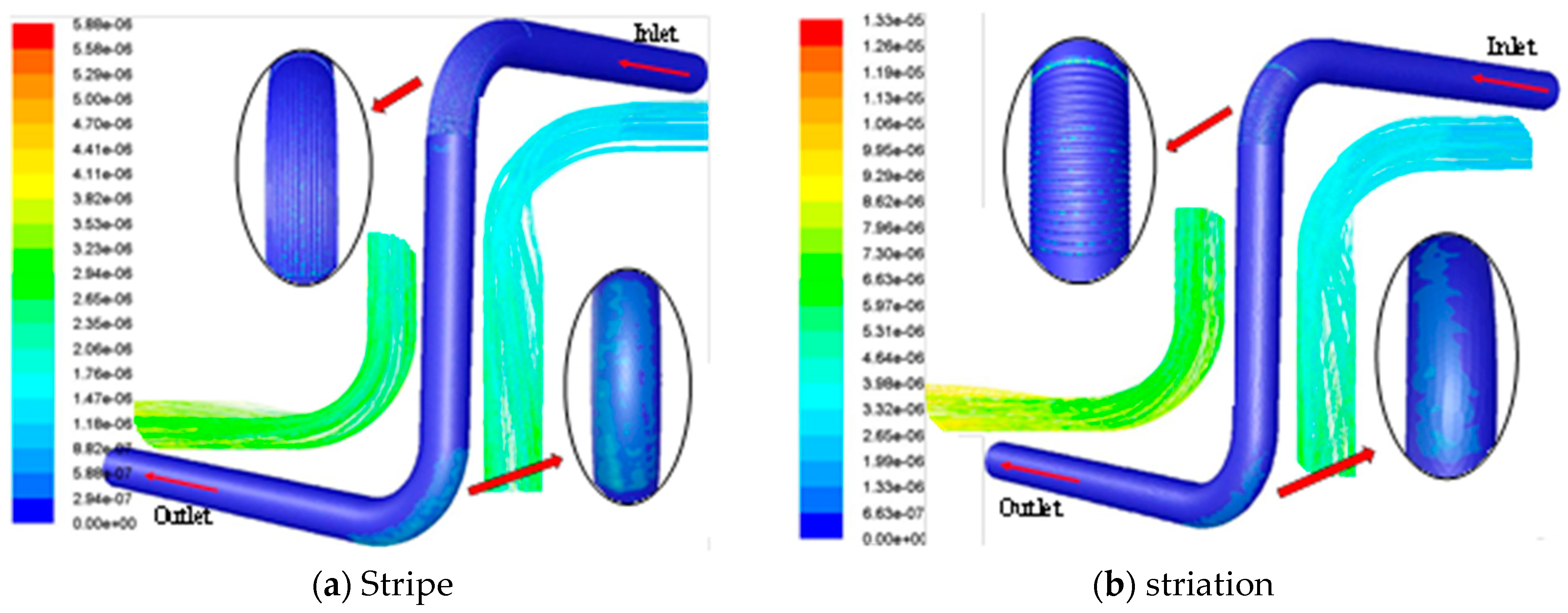
5.3. Scour Analysis of the Inner Wall Surface of Corrugated Bends
- (1)
- Geometrical model
- (2)
- Numerical simulation results and analysis
5.4. Comparative Analysis of Results
6. Conclusions
- (1)
- The degree of influence of each structural parameter on the erosion wear in descending order is as follows: bending angle, bending diameter ratio and spatial angle.
- (2)
- With the increase in the bending diameter ratio, the erosion wear rate of the pipeline structure gradually decreases. With the increase in the bending angle of the elbow, the maximum erosion rate of the pipeline elbow changes less before 90° and gradually increases beyond 90°. When the space clamping angle increases, the maximum erosion rate of the pipeline structure gradually decreases.
- (3)
- The bend to increase the blind tube can effectively reduce the erosion rate and slow down the erosion effect. An appropriate increase in the length and volume of the blind pass tube can also reduce erosion wear at the bend. Spherical bends make the particle distribution more uniform. The spherical diameter of the smaller bend has a greater anti-erosion effect than the larger diameter of the spherical bends. The maximum erosion rate of the corrugated pipe is higher than that of other structures, but its low erosion rate distribution area is wider, and the peak is more distributed in point form.
- (4)
- A new structure based on the bionic structure for optimizing the erosion resistance of pipe elbows is proposed. The influence of different structure sizes and other factors at the bends in resisting erosion is studied comparatively. It is then verified that the corrugated structure has a better erosion-resistance effect. This provides a reference for the further design and optimization of the pipeline.
- (5)
- As a result of the oil and gas extraction process, multiple factors working together have an impact on bend erosion wear. The method of controlling a single variable used in this study has caused the erosion wear numerical simulation results to deviate from the experimental, and even actual, working conditions. In subsequent studies, more situations will be considered to ensure that the numerical simulation results are more accurate. In this paper, a new optimization structure of bending pipes based on a bionic structure is proposed that has good application prospects, but more attention needs to be paid to its preparation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mills, D.; Mason, J.S. Particle size effects in bend erosion. Wear 1977, 44, 311–328. [Google Scholar] [CrossRef]
- Kosinska, A.; Balakin, B.V.; Kosinski, P. Theoretical analysis of erosion in elbows due to flows with nano- and micro-size particles. Powder Technol. 2020, 364, 484–493. [Google Scholar] [CrossRef]
- Throneberry, J.M.; Zhang, Y.; McLaury, B.S. Solid-Particle erosion in slug flow. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 20–22 September 2010. [Google Scholar]
- Kesana, N.R.; Throneberry, J.M.; Mc Laury, B.S.; Shirazi, S.A.; Rybicki, E.F. Effect of particle size and liquid viscosity on erosion in annular and slug flow. Energy Resour. Technol. 2013, 136, 012901. [Google Scholar] [CrossRef]
- Chen, X.; Mclaury, B.S.; Shirazi, S.A. Application and experimental validation of a computational fluid dynamics (CFD)-based erosion prediction model in elbows and plugged tees. Comput. Fluids 2004, 33, 1251–1272. [Google Scholar] [CrossRef]
- Grant, G.; Tabakoff, W. Erosion prediction in turbomachinery resulting from environmental solid particles. J. Aircr. 1975, 12, 471–478. [Google Scholar] [CrossRef]
- Mazumder, Q.H.; Shirazi, S.A.; McLaury, B. Experimental Investigation of the location of maximum erosive wear damage in elbows. J. Press. Technol. 2008, 130, 244–254. [Google Scholar] [CrossRef]
- Pereira, G.C.; Jos, S.F.; Alves, M.M.D. Numerical prediction of the erosion due to particles in elbows. Powder Technol. 2014, 261, 105–117. [Google Scholar] [CrossRef]
- Wood, R.; Jones, T.F.; Miles, N.J. Upstream swirl-induction for reduction of erosion damage from slurries in pipeline bends. Wear 2001, 250, 770–778. [Google Scholar] [CrossRef]
- Hashish, M. An improved model of erosion by solid particle impact. In Proceedings of the Erosion by Liquid and Solid Impact, Seventh International Conference, Cambridge, UK, 7 September 1987; p. 66. [Google Scholar]
- Solnordal, C.B.; Wong, C.Y.; Boulanger, J. An experimental and numerical analysis of erosion caused by sand pneumatically conveyed through a standard pipe elbow. Wear 2015, 336–337, 43–57. [Google Scholar] [CrossRef]
- Wood, R.J.K.; Jones, T.F.; Ganeshalingam, J. Comparison of predicted and experimental erosion estimates in slurry ducts. Wear 2004, 256, 937–947. [Google Scholar] [CrossRef]
- Zahedi, P.; Zhang, J.; Arabnejad, H. CFD simulation of multiphase flows and erosion predictions under annular flow and low liquid loading conditions. Wear 2017, 376–377, 1260–1270. [Google Scholar] [CrossRef]
- Zhang, Y.; Reuterfors, E.P.; McLaury, B.S. Comparison of computed and measured particle velocities and erosion in water and air flows. Wear 2007, 263, 330–338. [Google Scholar] [CrossRef]
- Zhang, J.; Darihaki, F.; Shirazi, S.A. A comprehensive CFD-based erosion prediction for sharp bend geometry with examination of grid effect. Wear 2019, 430–431, 191–201. [Google Scholar] [CrossRef]
- Peng, W.; Cao, X. Numerical simulation of solid particle erosion in pipe bends for liquid–solid flow. Powder Technol. 2016, 294, 266–279. [Google Scholar] [CrossRef]
- Jianlin, S.Z.; Zhang, Z.-y.; Tong, H.; Yan, S.; Peixin, G.; Tao, Y. Analysis of Erosion Characteristics of Liquid-solid Two-phase Fiow in Space Z-shape Pipeline. Chin. Hydraul. Pneum. 2022, 46, 34–43. (In Chinese) [Google Scholar]
- Zhang, Q.; Rahmatjan, I.; Memetimin, G. Design of flow characteristics and optimization of guided pneumatic. Chin. Hydraul. Pneum. 2021, 45, 44–51. (In Chinese) [Google Scholar]
- Ren, J.; Wang, J.; Min, W. Analysis of influence of valve port sealing width on flow field characteristics of piezoelectric pneumatic microvalve with nozzle baffle. Chin. Hydraul. Pneum. 2021, 45, 58–67. (In Chinese) [Google Scholar]
- Vivek, K. FLUENT 6.3 User’s Guide; Fluent Inc.: Lebanon, PA, USA, 2006. [Google Scholar]
- Yakhot, V.; Orszag, S.A. Renormalization group analysis of turbulence. I. Basic theory. J. Sci. Comput. 1986, 1, 3–51. [Google Scholar] [CrossRef]
- Morsi, S.A.; Alexander, A.J. An investigation of particle trajectories in two-phase flow systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]
- Banakermani, M.; Naderan, H.; Saffar-Avval, M.J.P.T. An investigation of erosion prediction for 15 to 90 elbows by numerical simulation of gas-solid flow. Powder Technol. 2018, 334, 9–26. [Google Scholar] [CrossRef]
- Ghosh, A. Validated Numerical Simulations Investigating the Effects of Cross-sectional Asymmetry on Fluid Flow and Heat Transfer. Ph.D. Thesis, University of Minnesota, Minneapolis, MN, USA, 2017. [Google Scholar]
- Yan, Y.; Zhao, Y.; Yao, J.; Wang, C.-H.J.P.T. Investigation of particle transport by a turbulent flow through a 90° bend pipe with electrostatic effects. Powder Technol. 2021, 394, 547–561. [Google Scholar] [CrossRef]
- Cao, L.; Tu, C.; Hu, P.; Liu, S.J.A.T.E. Influence of solid particle erosion (SPE) on safety and economy of steam turbines. Appl. Therm. Eng. 2019, 150, 552–563. [Google Scholar] [CrossRef]
- Zolfagharnasab, M.; Salimi, M.; Zolfagharnasab, H.; Alimoradi, H.; Shams, M.; Aghanajafi, C.J.P.T. A novel numerical investigation of erosion wear over various 90-degree elbow duct sections. Powder Technol. 2021, 380, 1–17. [Google Scholar] [CrossRef]
- Panda, K.; Hirokawa, T.; Huang, L.J.A.T.E. Design study of microchannel heat exchanger headers using experimentally validated multiphase flow CFD simulation. Appl. Therm. Eng. 2020, 178, 115585. [Google Scholar] [CrossRef]
- Zonglin, S.; Zhenhua, X.; Mengyun, Z.; Pengfei, D.; Yuguo, W. Numerical simulation research in erosion-corrosion of 90 elbow pipe. J. Liaoning Univ. Pet. Chem. Technol. 2018, 38, 47. [Google Scholar]
- Han, F.; Ong, M.C.; Xing, Y.; Li, W.J.O.E. Three-dimensional numerical investigation of laminar flow in blind-tee pipes. Ocean. Eng. 2020, 217, 107962. [Google Scholar] [CrossRef]
- Wang, F.; Sahana, M.; Pahlevanzadeh, B.; Pal, S.C.; Shit, P.K.; Piran, M.J.; Janizadeh, S.; Band, S.S.; Mosavi, A. Applying different resampling strategies in machine learning models to predict head-cut gully erosion susceptibility. Alex. Eng. J. 2021, 60, 5813–5829. [Google Scholar] [CrossRef]





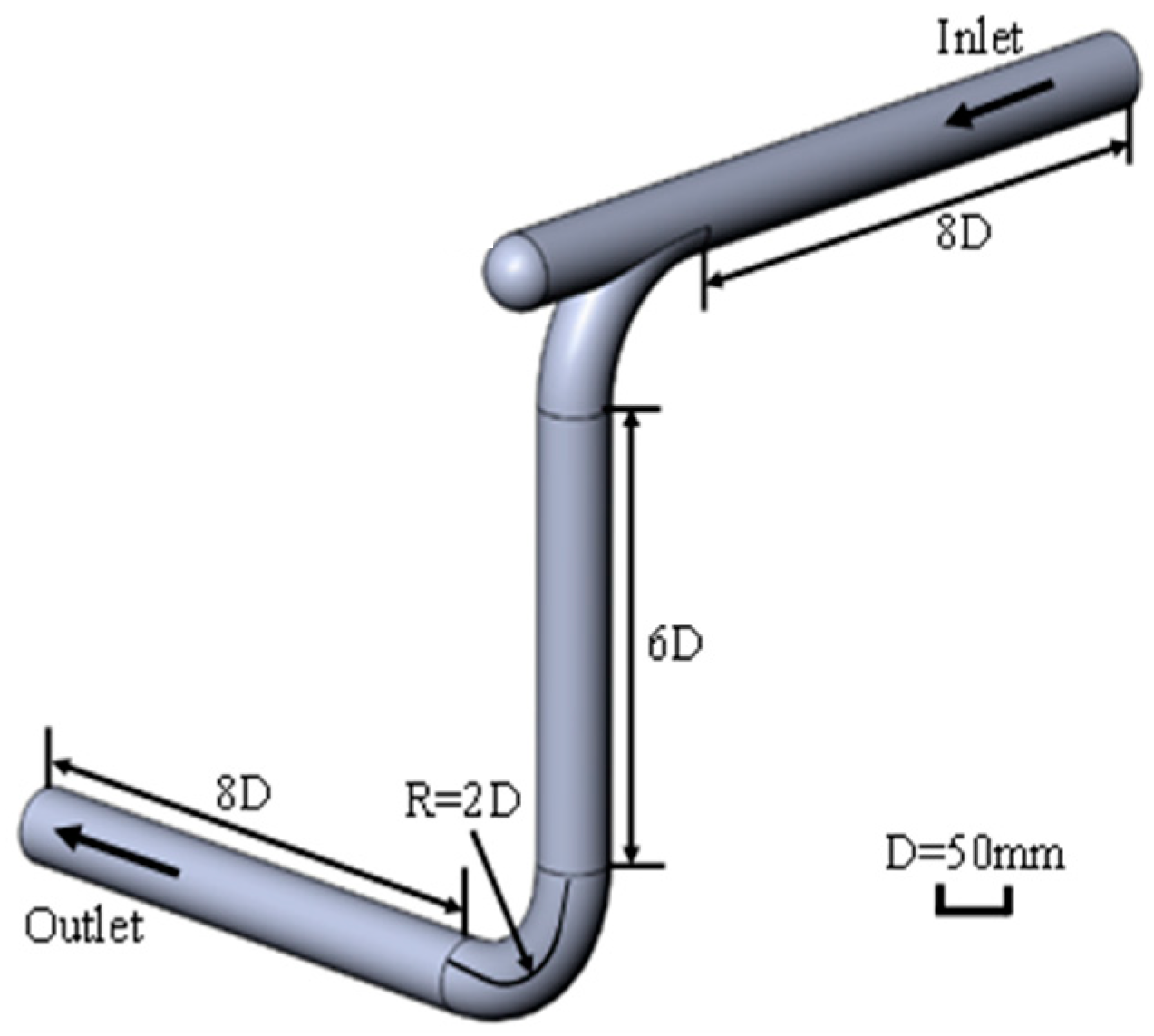
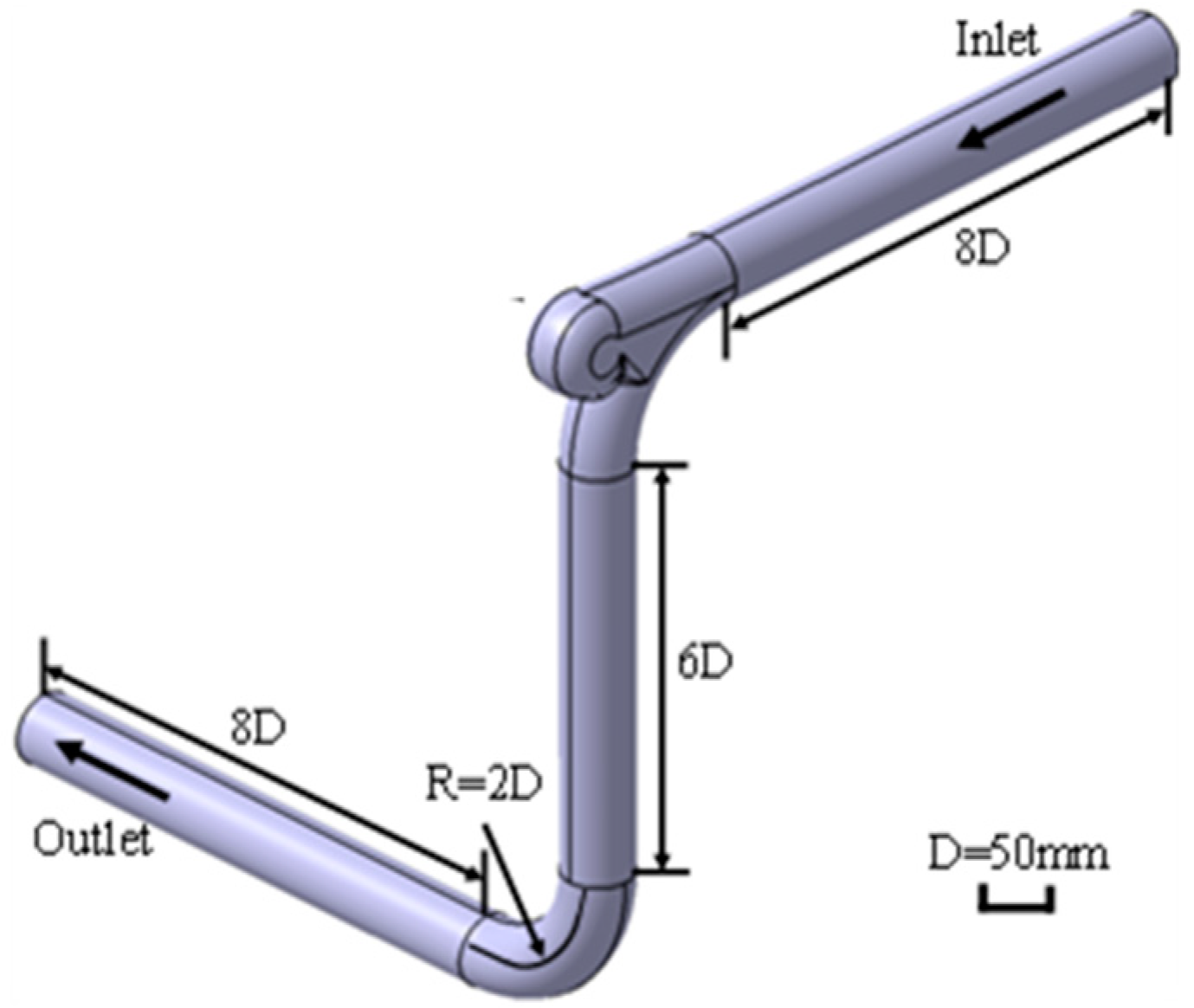







| Parameters of Fracturing Fluid | Symbol | Numerical | Units |
|---|---|---|---|
| Inner diameter | D | 50 | mm |
| Bend diameter ratio | R/D | 2 | —— |
| Velocity | v | 20 | m/s |
| Particle diameter | d | 0.00045 | m |
| Density of sand | 2650 | kg/m3 | |
| Mass flow | mn | 7 | kg/s |
| Viscosity | ɳ | 0.001 | Pa·s |
| Parameters | Symbol | Units |
|---|---|---|
| Liquid phase density | ρ | kg/m3 |
| Time | t | s |
| Thermal conductivity | λ | —— |
| Stress tensor | Pa | |
| Liquid phase velocity | v | m/s |
| Turbulent dissipation rate | E | m2/s3 |
| Volume | Vm | m3 |
| Pressure | p | MPa |
| Energy source | W/m3 |
| Numerical | Units | |
|---|---|---|
| Speed | 4.09 | m/s |
| Diameter | 300 | µm |
| Density | 2650 | kg/m3 |
| Mass flow rate | 0.1027 | kg/s |
| Density of material | 7980 | kg/m3 |
| Experimental Data from the Literature | Simulation Data from the Literature | Experimental Data from the Literature | Simulation Data from This Paper | |
|---|---|---|---|---|
| Numerical (nm/s) | 0.219 | 0.254 | 0.219 | 0.240 |
| Inaccuracy | 15.98% | 9.59% | ||
| Pipeline Type | Upper End Elbow Erosion Rate kg/(m2·s) | Lower End Elbow Erosion Rate kg/(m2·s) | Maximum Erosion Rate kg/(m2·s) | Reduction from Original Structure |
|---|---|---|---|---|
| Typical pipeline structure | —— | |||
| T-type blind through short header pipe | 70.89% | |||
| T-type blind through long header pipe | 84.57% | |||
| 70 mm round head blind pipe | 68.38% | |||
| 100 mm round head blind pipe | 53.44% | |||
| Minispherical | 83.64% | |||
| Mesopherical | 75.95% | |||
| Hemispherical | 11.34% | |||
| Spherical | 51.01% | |||
| Stripe | −138.06% | |||
| Striation | −438.46% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, R.; Tang, R.; Gao, Z.; Yu, T. Optimized Design of Pipe Elbows for Erosion Wear. Appl. Sci. 2024, 14, 984. https://doi.org/10.3390/app14030984
Ma R, Tang R, Gao Z, Yu T. Optimized Design of Pipe Elbows for Erosion Wear. Applied Sciences. 2024; 14(3):984. https://doi.org/10.3390/app14030984
Chicago/Turabian StyleMa, Rui, Rui Tang, Zhibo Gao, and Tao Yu. 2024. "Optimized Design of Pipe Elbows for Erosion Wear" Applied Sciences 14, no. 3: 984. https://doi.org/10.3390/app14030984
APA StyleMa, R., Tang, R., Gao, Z., & Yu, T. (2024). Optimized Design of Pipe Elbows for Erosion Wear. Applied Sciences, 14(3), 984. https://doi.org/10.3390/app14030984





