Tunable Ultralow-Frequency Bandgaps Based on Locally Resonant Plate with Quasi-Zero-Stiffness Resonators
Abstract
:1. Introduction
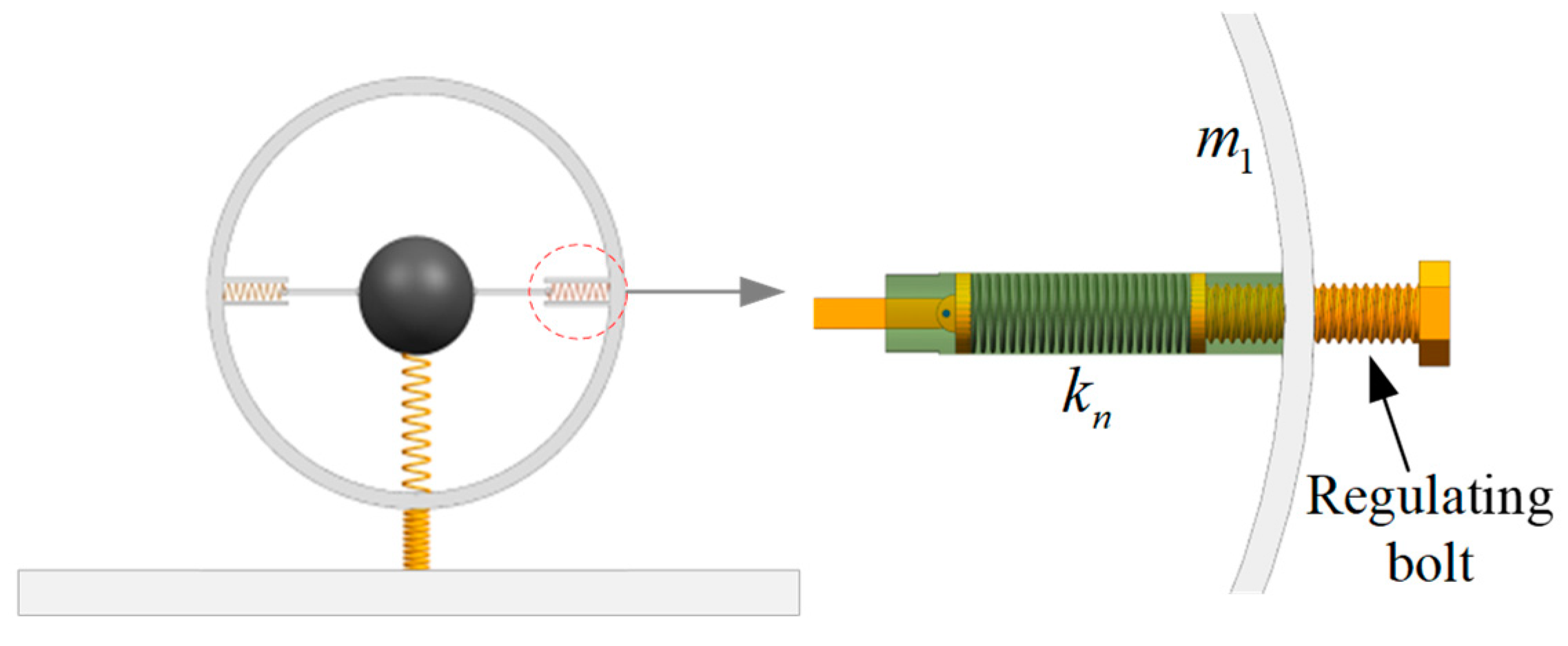
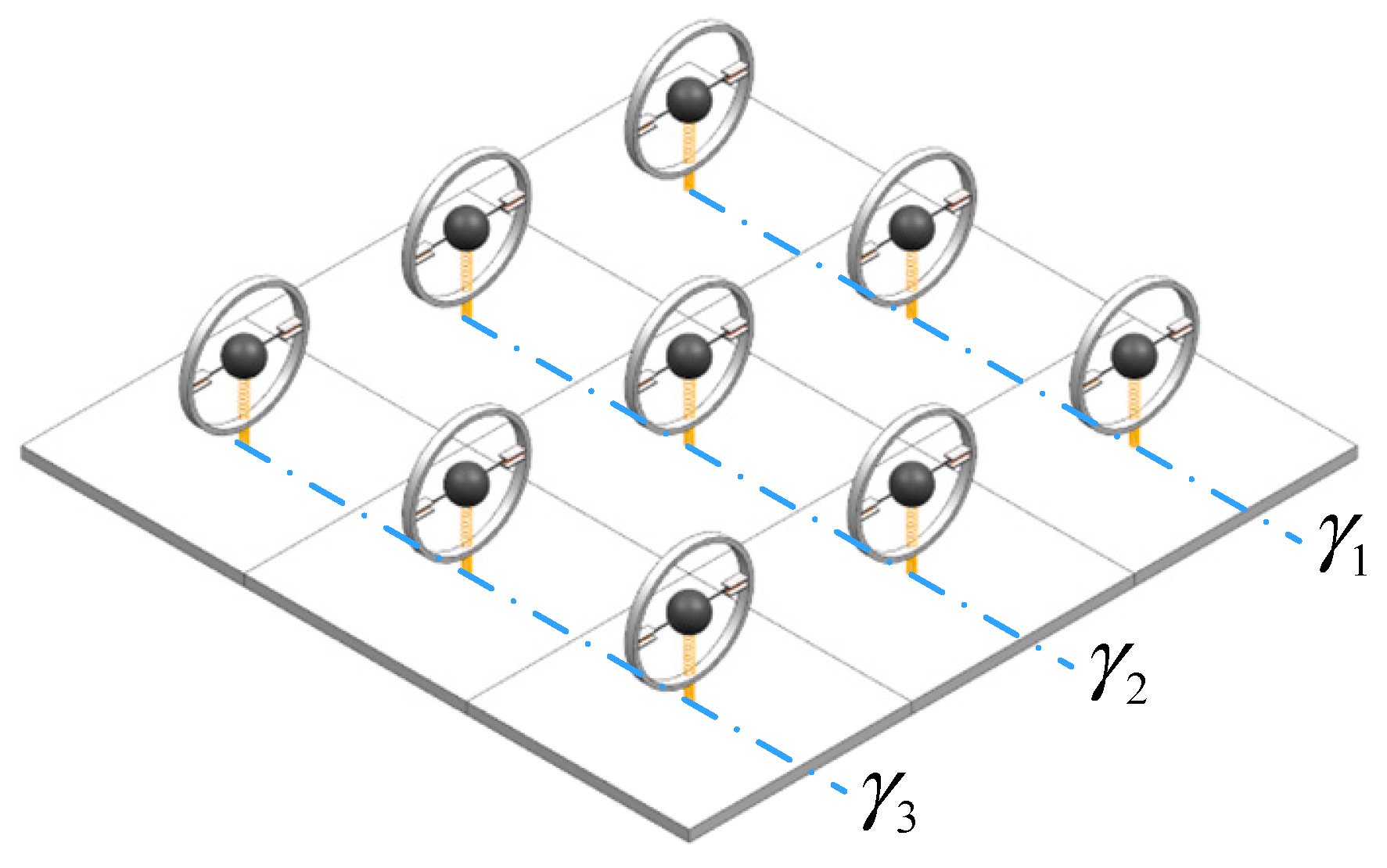
2. Model and Formulations
3. Results and Discussions
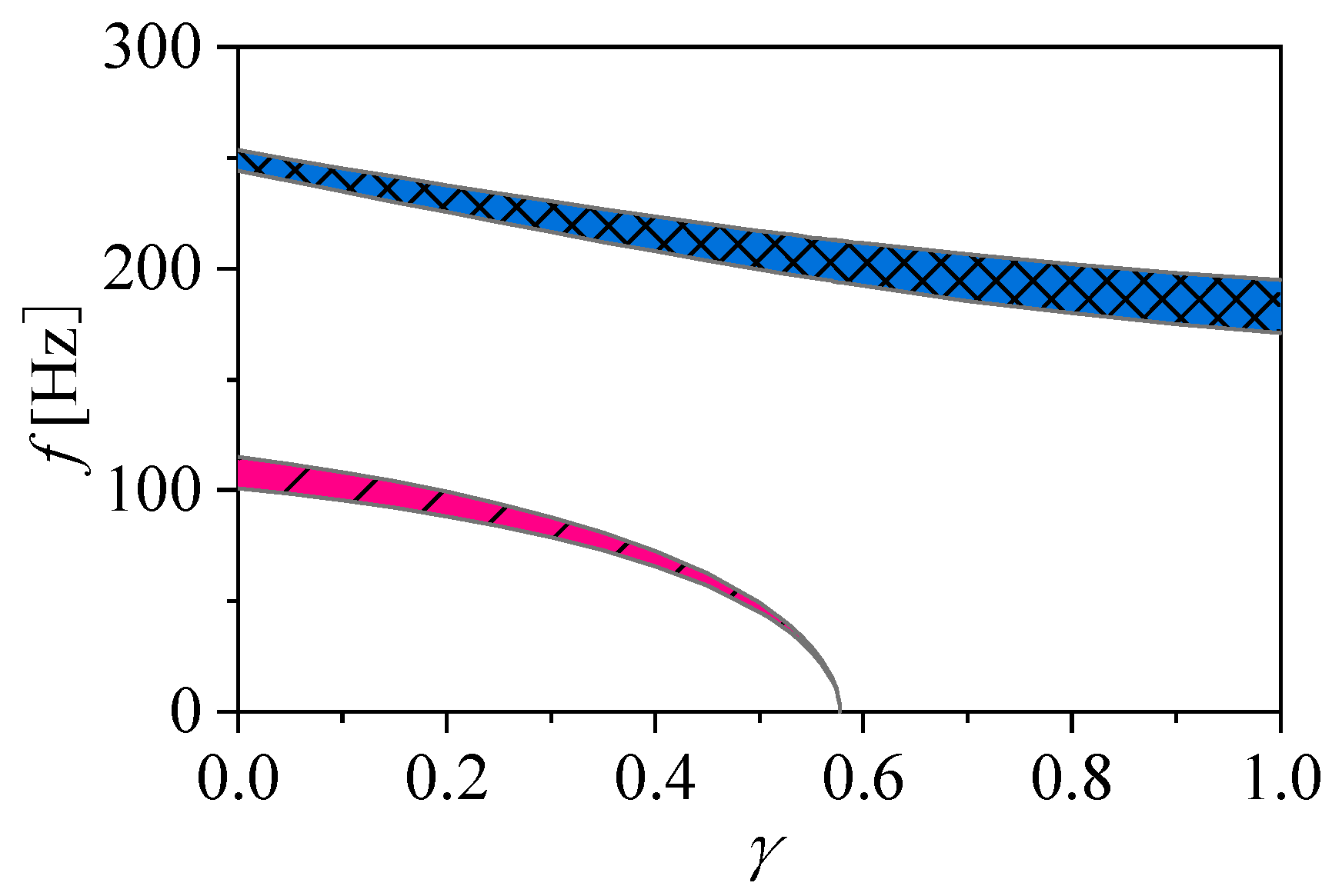
3.1. Band Structure of Infinite Systems
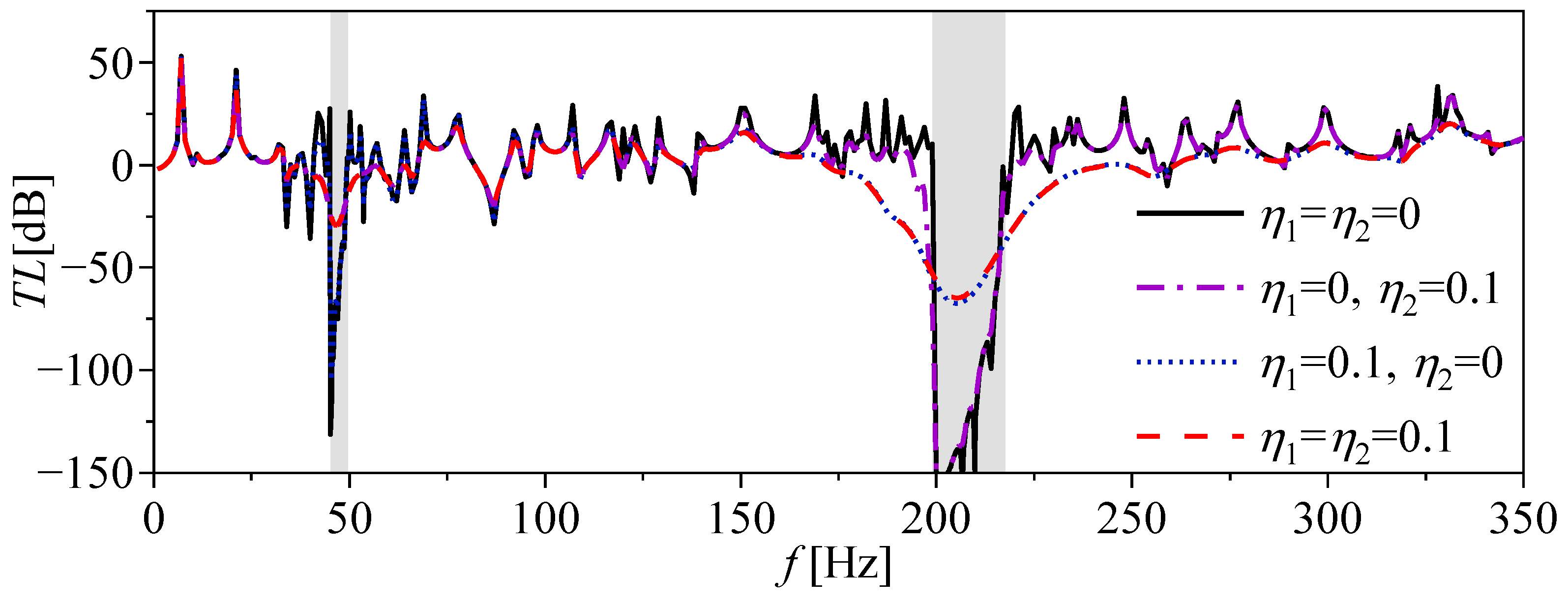
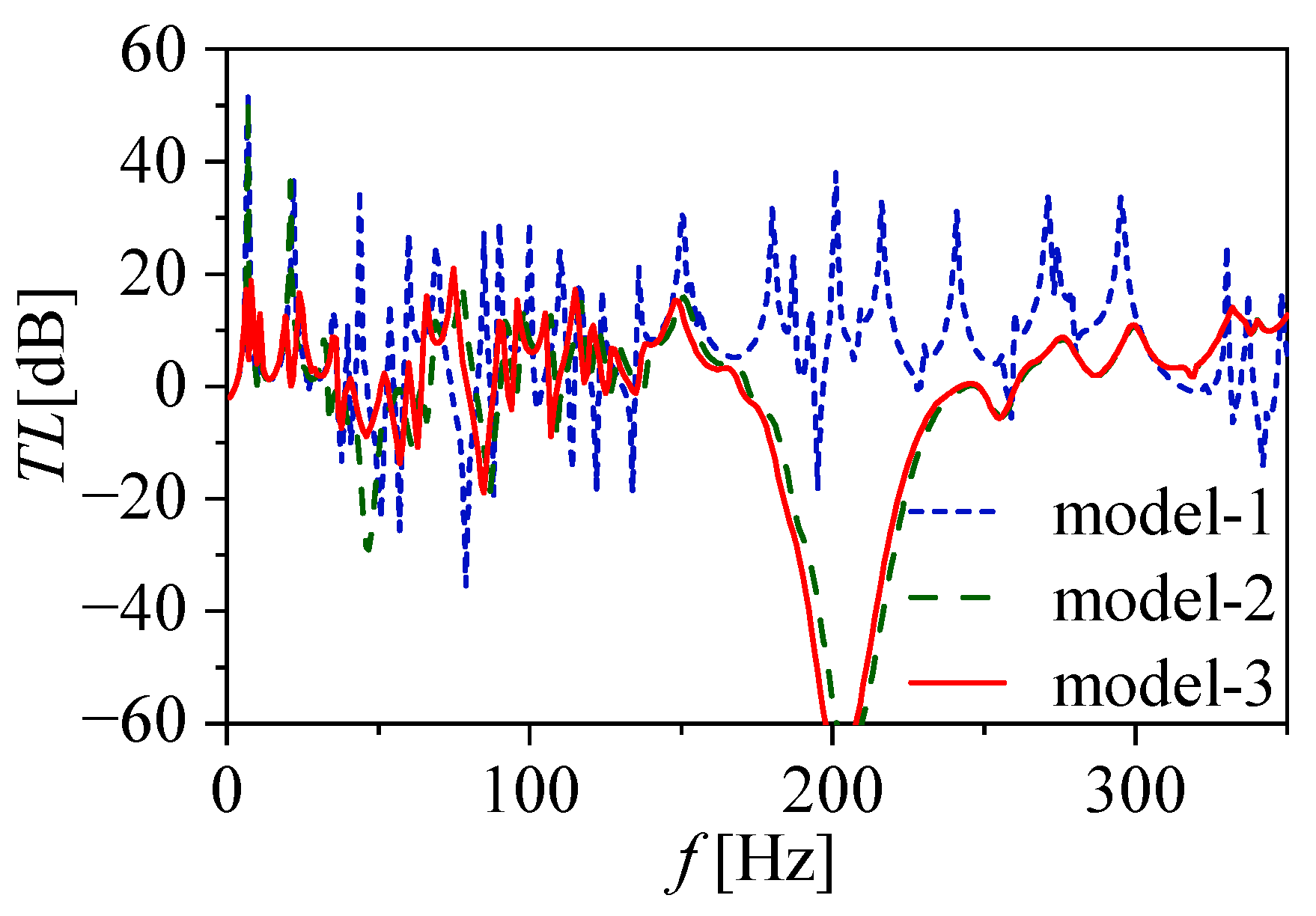
3.2. Vibration Transmittance of Finite Structures
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Putra, A.; Thompson, D.J. Sound radiation from rectangular baffled and unbaffled plates. Appl. Acoust. 2010, 71, 1113–1125. [Google Scholar] [CrossRef]
- Li, B.; Wang, N.; Tian, Y.; Kuang, W.; Wei, L.; Zheng, Z. Analysis of vibration and acoustic radiation characteristics of reinforced laminated cylindrical shell structure. Appl. Sci. 2023, 13, 9617. [Google Scholar] [CrossRef]
- Kerwin, E.M. Comparison of the vibration damping effectiveness of free and constrained viscoelastic layers. J. Acoust. Soc. Am. 2005, 31, 1852–1856. [Google Scholar] [CrossRef]
- Lei, Y.; Adhikari, S.; Friswell, M.I. Vibration of nonlocal Kelvin–Voigt viscoelastic damped Timoshenko beams. Int. J. Eng. Sci. 2013, 66–67, 1–13. [Google Scholar] [CrossRef]
- Wong, W.O.; Tang, S.L.; Cheung, Y.L.; Cheng, L. Design of a dynamic vibration absorber for vibration isolation of beams under point or distributed loading. J. Sound Vib. 2007, 301, 898–908. [Google Scholar] [CrossRef]
- Yang, C.; Li, D.; Cheng, L. Dynamic vibration absorbers for vibration control within a frequency band. J. Sound Vib. 2011, 330, 1582–1598. [Google Scholar] [CrossRef]
- Teo, Y.R.; Fleming, A.J. Optimal integral force feedback for active vibration control. J. Sound Vib. 2015, 356, 20–33. [Google Scholar] [CrossRef]
- Wang, L.; Li, J.; Yang, Y.; Wang, J.; Yuan, J. Active control of low-frequency vibrations in ultra-precision machining with blended infinite and zero stiffness. Int. J. Mach. Tools Manuf. 2019, 139, 64–74. [Google Scholar] [CrossRef]
- Xie, X.; Ren, M.; Zheng, H.; Zhang, Z. Active vibration control of a time-varying shafting system using an adaptive algorithm with online auxiliary filter estimation. J. Sound Vib. 2021, 513, 116430. [Google Scholar] [CrossRef]
- Torrent, D.; Mayou, D.; Sánchez-Dehesa, J. Elastic analog of graphene: Dirac cones and edge states for flexural waves in thin plates. Phys. Rev. B 2013, 87, 269–275. [Google Scholar] [CrossRef]
- Sun, H.; Du, X.; Pai, P.F. Theory of metamaterial beams for broadband vibration absorption. J. Intell. Mater. Syst. Struct. 2010, 21, 1085–1101. [Google Scholar] [CrossRef]
- Pai, P.F.; Peng, H.; Jiang, S. Acoustic metamaterial beams based on multi-frequency vibration absorbers. Int. J. Mech. Sci. 2014, 79, 195–205. [Google Scholar] [CrossRef]
- Peng, H.; Frank Pai, P. Acoustic metamaterial plates for elastic wave absorption and structural vibration suppression. Int. J. Mech. Sci. 2014, 89, 350–361. [Google Scholar] [CrossRef]
- Nouh, M.; Aldraihem, O.; Baz, A. Wave propagation in metamaterial plates with periodic local resonances. J. Sound Vib. 2015, 341, 53–73. [Google Scholar] [CrossRef]
- Gulia, P.; Gupta, A. Sound attenuation in triple panel using locally resonant sonic crystal and porous material. Appl. Acoust. 2019, 156, 113–119. [Google Scholar] [CrossRef]
- Qian, D.; Shi, Z. Bandgap properties in simplified model of composite locally resonant phononic crystal plate. Phys. Lett. A 2017, 381, 3505–3513. [Google Scholar] [CrossRef]
- Liu, H.; Shi, Z.; Wang, Y.; Zhai, H. A band gap optimization scheme for two-dimensional locally resonant phononic crystal with square spiral rings. Phys. Lett. A 2022, 442, 128134. [Google Scholar] [CrossRef]
- Badreddine Assouar, M.; Sun, J.H.; Lin, F.S.; Hsu, J.C. Hybrid phononic crystal plates for lowering and widening acoustic band gaps. Ultrasonics 2014, 54, 2159–2164. [Google Scholar] [CrossRef]
- Li, Y.; Chen, T.; Wang, X.; Xi, Y.; Liang, Q. Enlargement of locally resonant sonic band gap by using composite plate-type acoustic metamaterial. Phys. Lett. A 2015, 379, 412–416. [Google Scholar] [CrossRef]
- Wang, T.; Sheng, M.; Guo, Z.; Qin, Q. Flexural wave suppression by an acoustic metamaterial plate. Appl. Acoust. 2016, 114, 118–124. [Google Scholar] [CrossRef]
- Fan, X.; Li, J.; Zhang, X.; Li, F. Multi-bandgaps metamaterial plate design using complex mass-beam resonator. Int. J. Mech. Sci. 2022, 236, 107742. [Google Scholar] [CrossRef]
- Van Belle, L.; Claeys, C.; Deckers, E.; Desmet, W. The impact of damping on the sound transmission loss of locally resonant metamaterial plates. J. Sound Vib. 2019, 461, 114909. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.J.; Kovacic, I.; Waters, T.P. On the force transmissibility of a vibration isolator with quasi-zero-stiffness. J. Sound Vib. 2009, 322, 707–717. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.C.; Ye, K.; Luo, Q. Increase of quasi-zero stiffness region using two pairs of oblique springs. Mech. Syst. Signal Process. 2020, 144, 106975. [Google Scholar] [CrossRef]
- Liu, C.; Zhao, R.; Yu, K.; Liao, B. In-plane quasi-zero-stiffness vibration isolator using magnetic interaction and cables: Theoretical and experimental study. Appl. Math. Model. 2021, 96, 497–522. [Google Scholar] [CrossRef]
- Li, M.; Li, Y.; Liu, X.; Dai, F. A quasi-zero-stiffness vibration isolator using bi-stable hybrid symmetric laminate. Compos. Struct. 2022, 299, 116047. [Google Scholar] [CrossRef]
- Zhang, Y.; Cao, Q. The recent advances for an archetypal smooth and discontinuous oscillator. Int. J. Mech. Sci. 2022, 214, 106904. [Google Scholar] [CrossRef]
- Xie, B.; Sheng, M. Ultralow-frequency band gap in a quasi-zero-stiffness multi-resonator periodic hybrid structure. Wave Motion 2021, 107, 102825. [Google Scholar] [CrossRef]
- Huang, H.H.; Sun, C.T. Wave attenuation mechanism in an acoustic metamaterial with negative effective mass density. New J. Phys. 2009, 11, 013003. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, J.; Ouyang, H.; Cheng, L.; Xu, D. A semi-active metamaterial beam with electromagnetic quasi-zero-stiffness resonators for ultralow-frequency band gap tuning. Int. J. Mech. Sci. 2020, 176, 105548. [Google Scholar] [CrossRef]
- Brillouin, L. Wave Propagation in Periodic Structures; Dover Publication: New York, NY, USA, 1953; pp. 17–18. [Google Scholar]
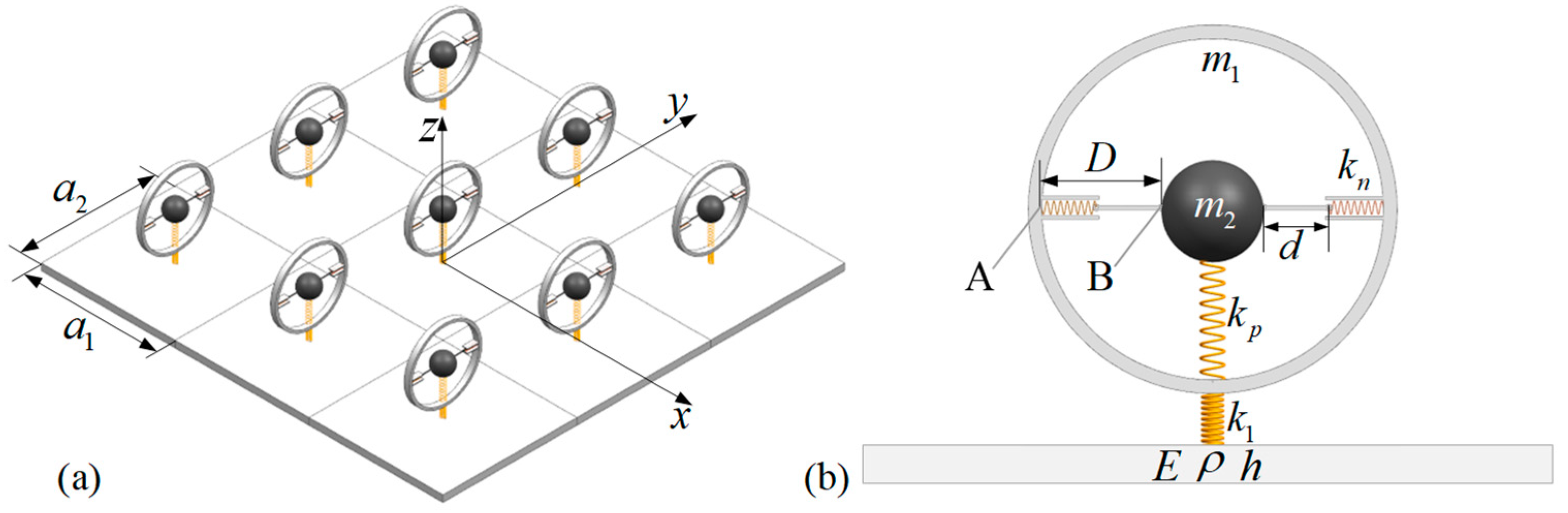

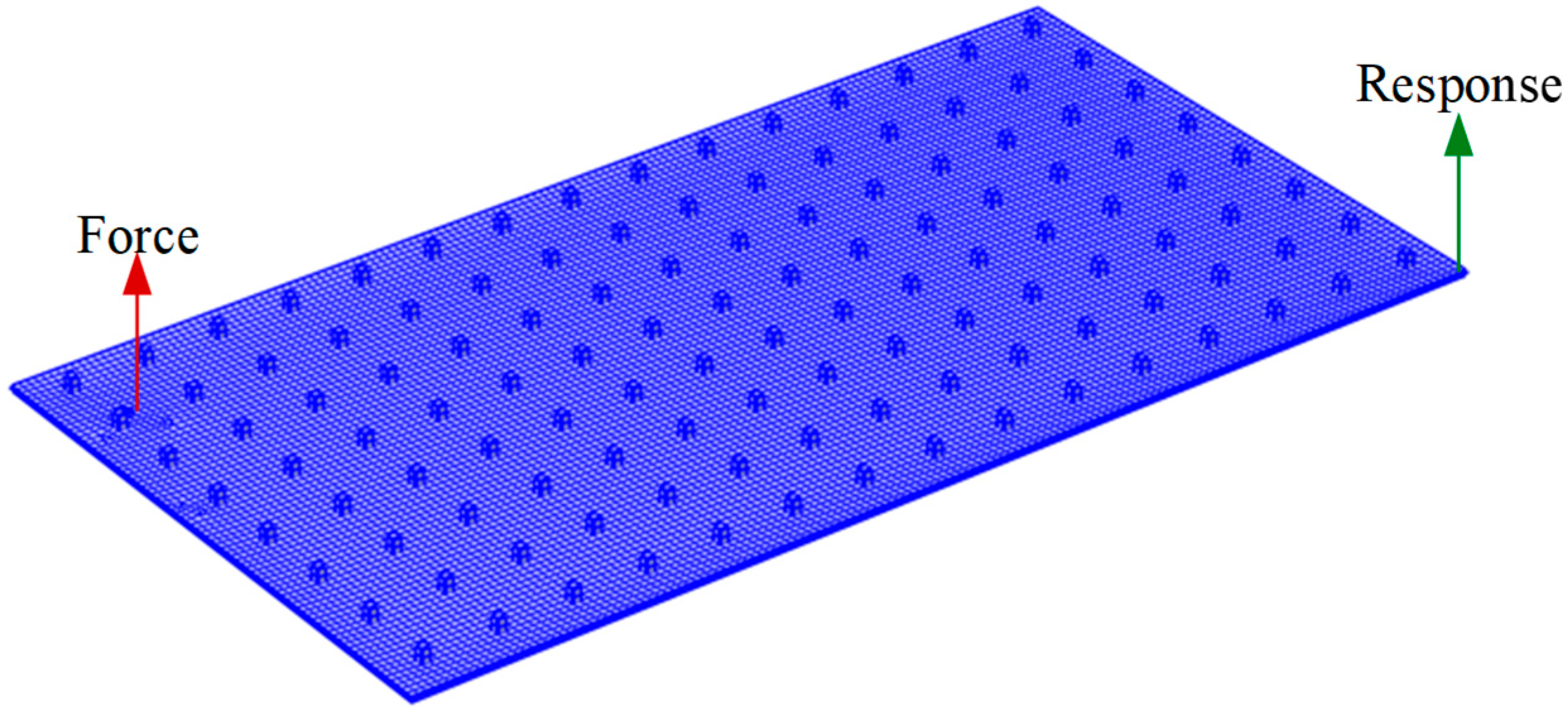
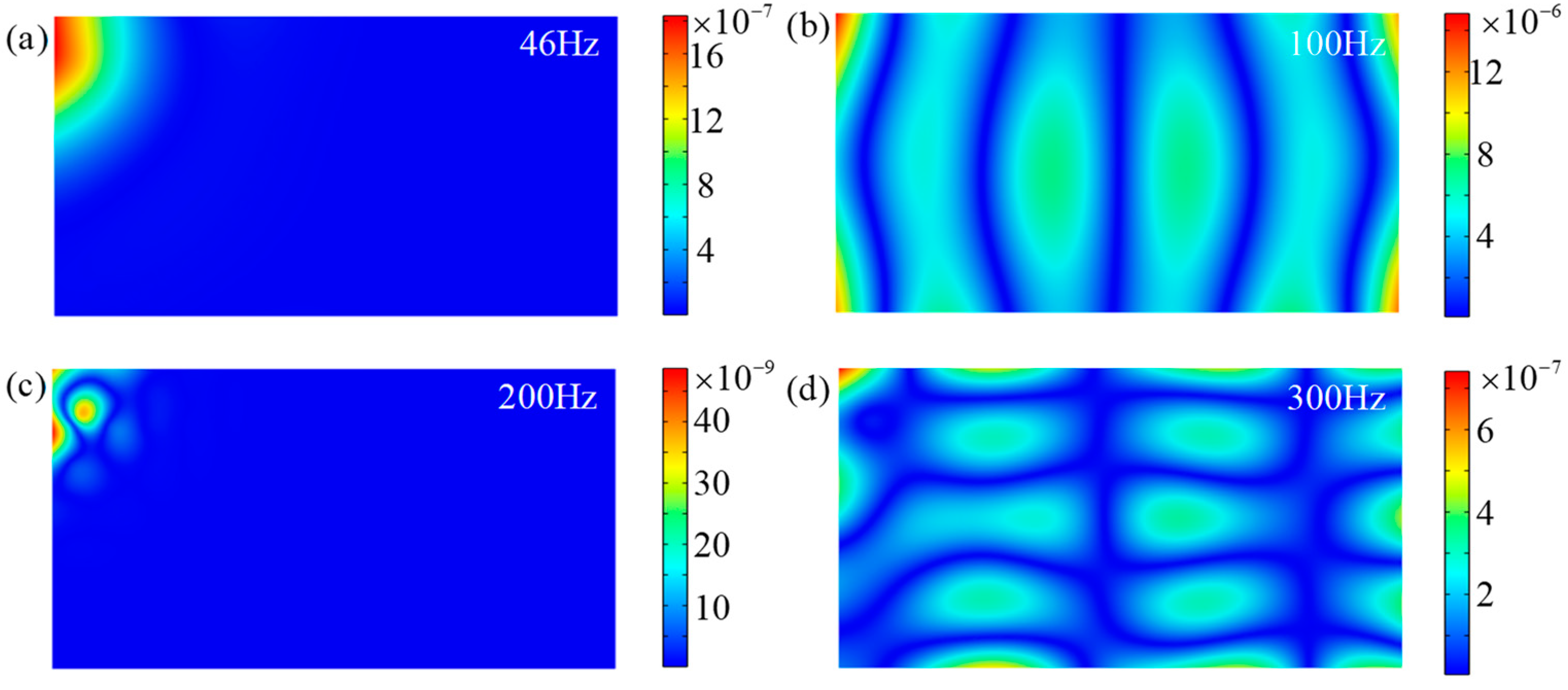
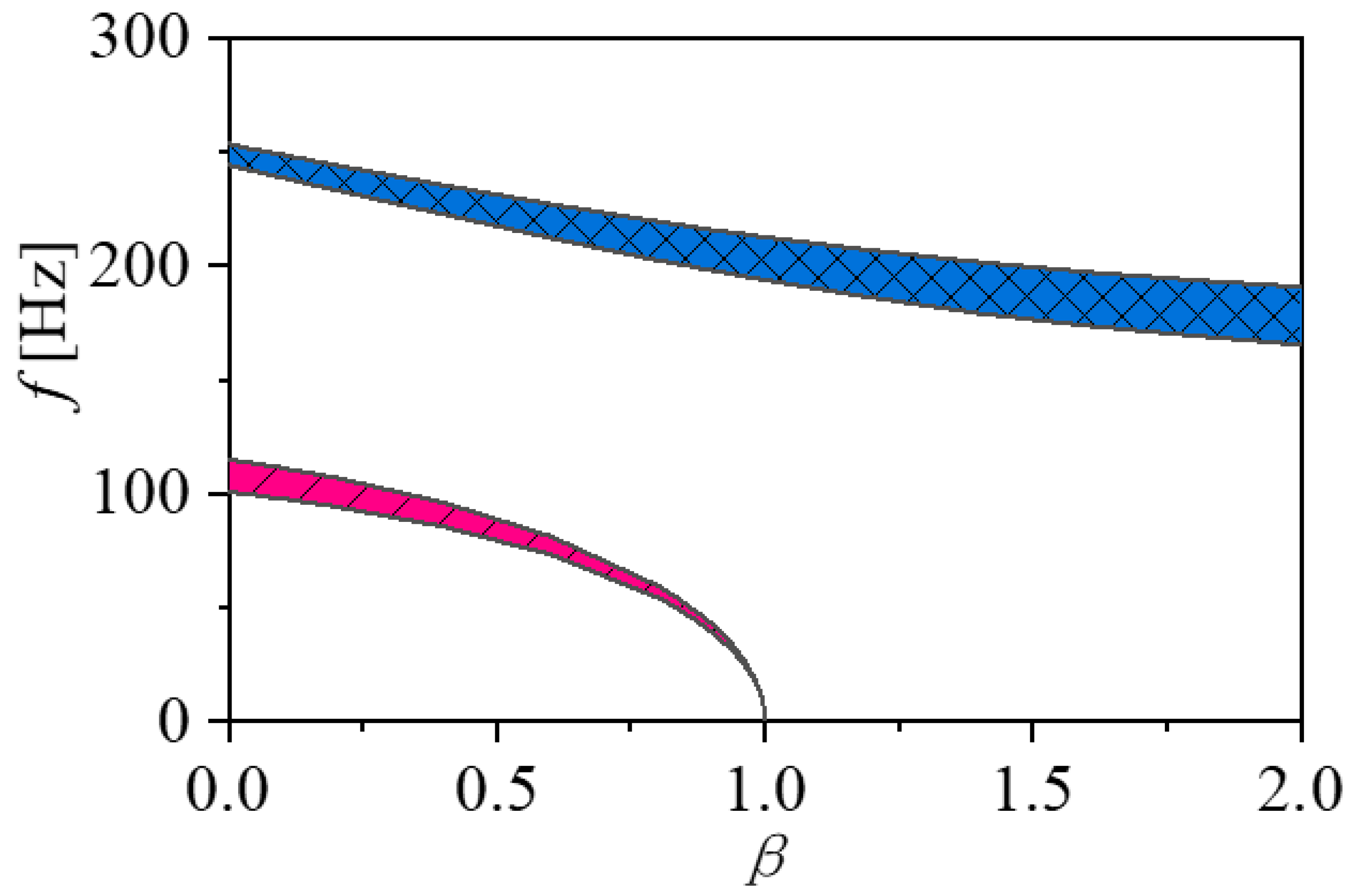

| Plate Parameters | QZS Resonator Parameters | ||
|---|---|---|---|
| Density | Mass 1 | ||
| Young’s modulus | Mass 2 | ||
| Poisson’s ratio | Spring stiffness | ||
| Plate thickness | Spring stiffness | ||
| Lattice constant | Spring stiffness | ||
| Compression ratio | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Z.; Xie, B.; Sheng, M.; Zeng, H. Tunable Ultralow-Frequency Bandgaps Based on Locally Resonant Plate with Quasi-Zero-Stiffness Resonators. Appl. Sci. 2024, 14, 1467. https://doi.org/10.3390/app14041467
Guo Z, Xie B, Sheng M, Zeng H. Tunable Ultralow-Frequency Bandgaps Based on Locally Resonant Plate with Quasi-Zero-Stiffness Resonators. Applied Sciences. 2024; 14(4):1467. https://doi.org/10.3390/app14041467
Chicago/Turabian StyleGuo, Zhiwei, Buliang Xie, Meiping Sheng, and Hao Zeng. 2024. "Tunable Ultralow-Frequency Bandgaps Based on Locally Resonant Plate with Quasi-Zero-Stiffness Resonators" Applied Sciences 14, no. 4: 1467. https://doi.org/10.3390/app14041467





