Eccentric Wear Mechanism and Centralizer Layout Design in 3D Curved Wellbores
Abstract
1. Introduction
2. Materials and Methods
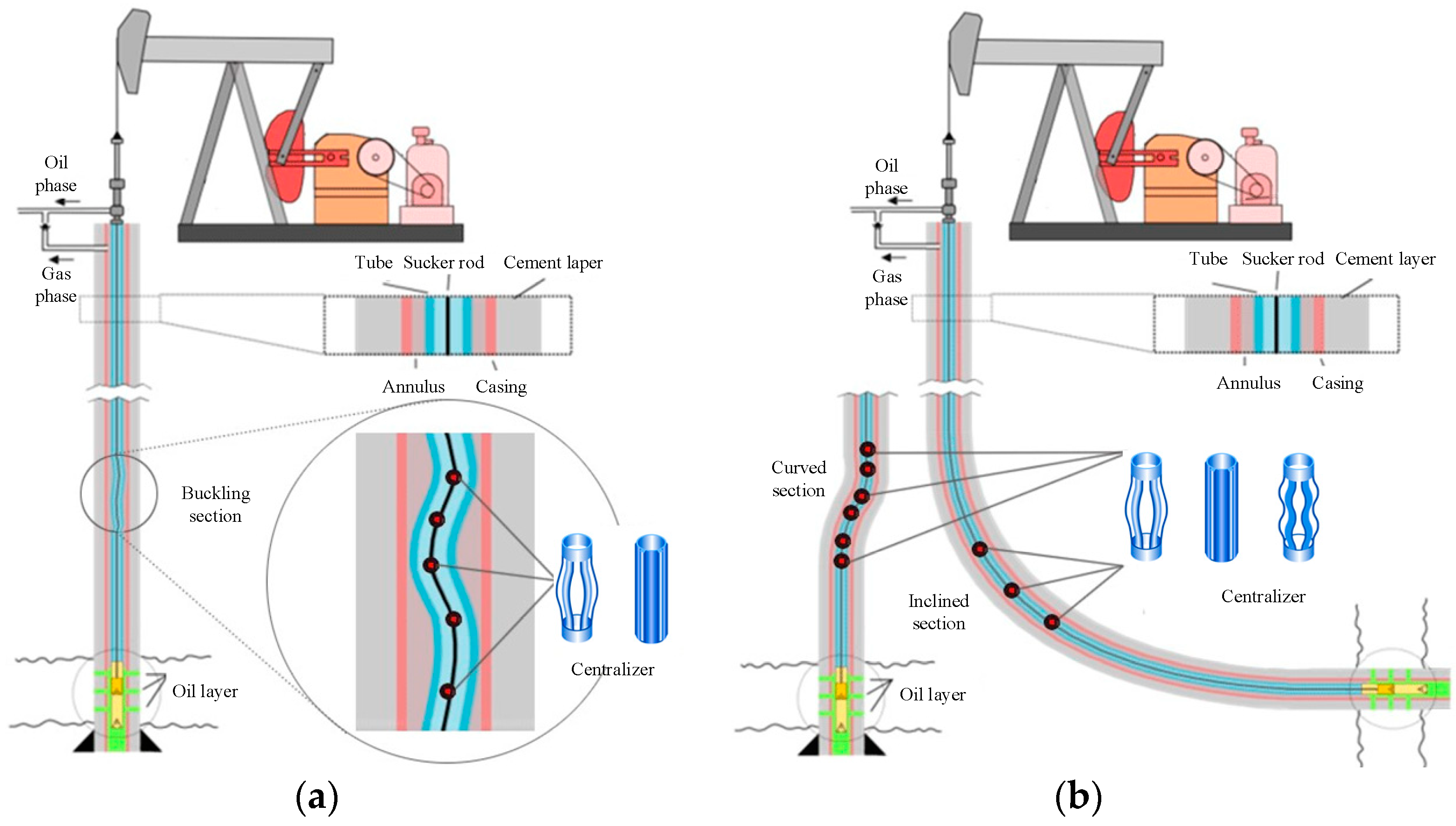
2.1. 3D Curved Wells
2.2. Dynamics Model for SRS
2.2.1. Mechanical Assumptions
- (1)
- The material of SRS is anisotropic.
- (2)
- SRS is treated as a complete elastomer.
- (3)
- SRS is circular in cross-section.
- (4)
- The deformation of the element is a small deformation.
- (5)
- The deformation processing is perpendicular to the neutral axis.
- (6)
- The axis of the SRS coincides with the axis of the wellbore.
- (7)
- The movement direction of SRS is axial and reciprocating.
- (8)
- The tube is anchored.
- (9)
- No shear stress in the cross-section of SRS.
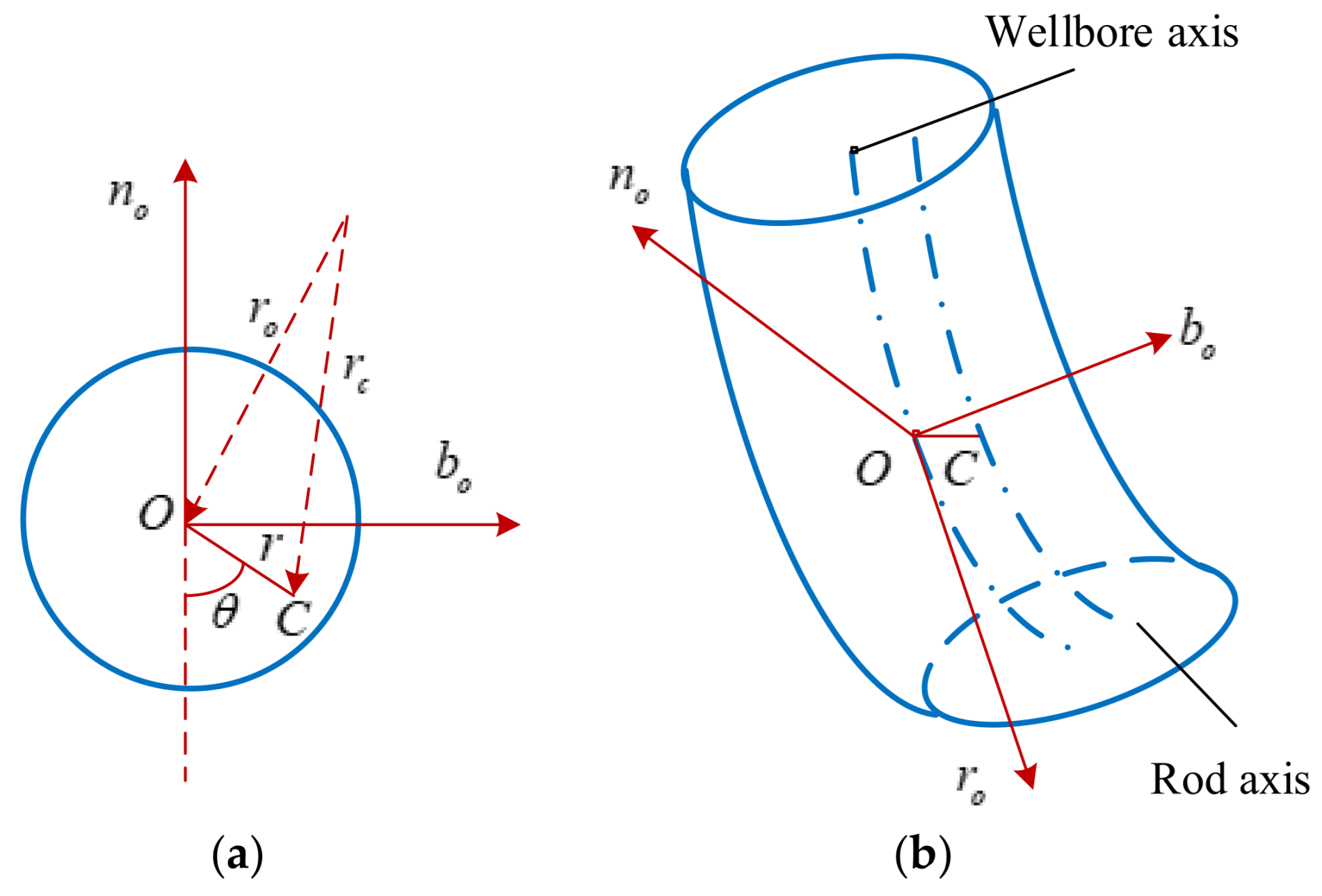
2.2.2. Geometric Description of 3D Curved Well Trajectory
2.2.3. Description of the Shape of SRS Axis
2.2.4. Force Analysis of the Micro-Element of SRS
2.2.5. Dynamic Differential Equation of SRS
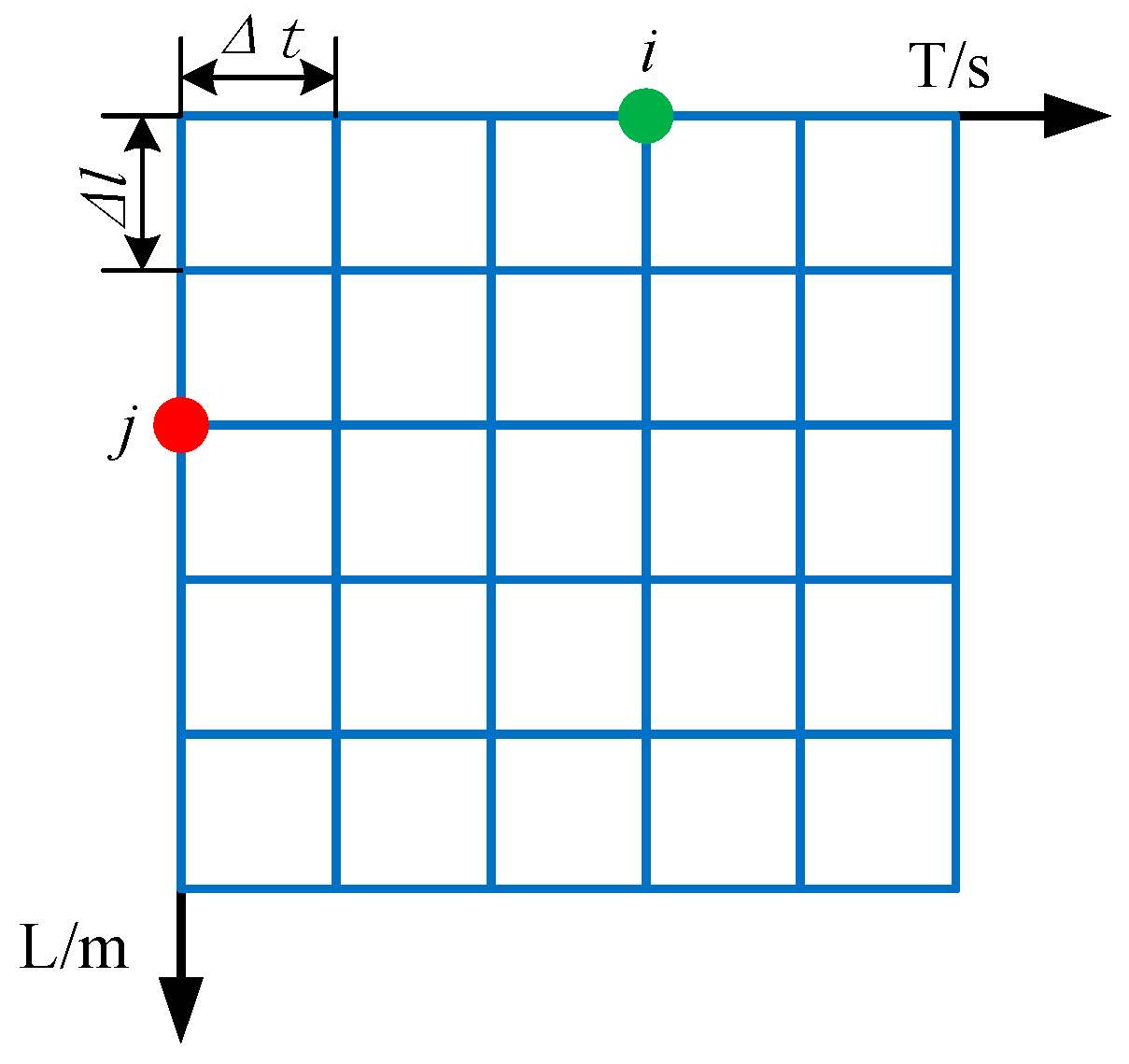
2.2.6. Solution of Dynamic Model of Sucker Rod String
2.2.7. Continuity and Initial Conditions of Discrete Form of Dynamics Model
- (1)
- Continuity conditions
- (2)
- Initial conditions
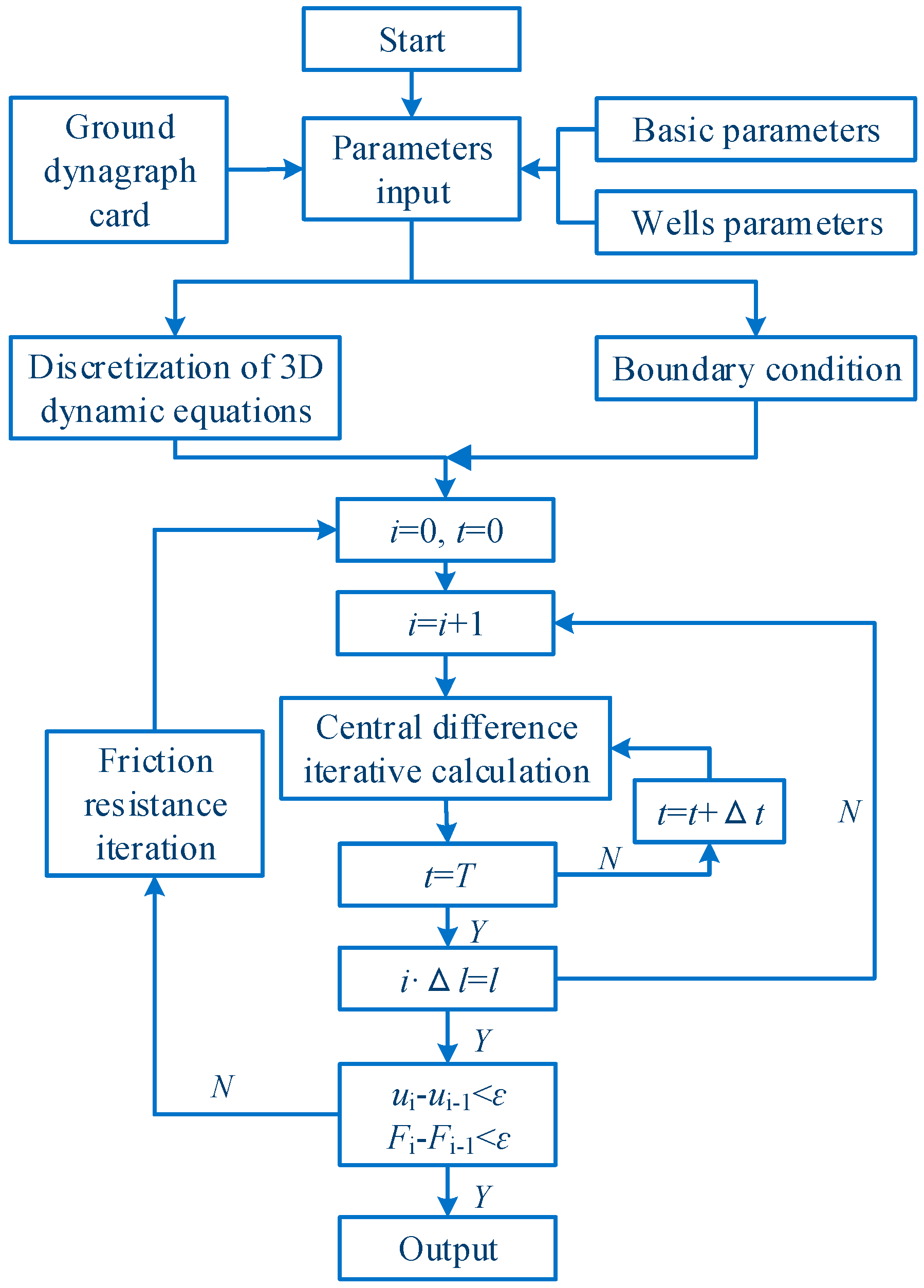
2.2.8. Calculation Process
3. Optimisation Design Method for the Arrangement of Centralisers
- (1)
- Collecting well conditions and design parameters: Inputting data such as wellbore trajectory, rod string parameters, and fluid column characteristics to provide a foundation for model calculations.
- (2)
- Establishing a three-dimensional mechanical coupling mathematical model: Taking into account the axial load, lateral forces, torque effects on the sucker rod string, and the three-dimensional curvature of the wellbore trajectory.
- (3)
- Calculating the stress state of the rod string: Using the model to calculate the axial load, lateral force distribution, and dynamic stress conditions of the rod string.
- (4)
- Analysing the buckling behaviour of the rod string: Studying the buckling forms of the rod string under different load conditions (e.g., two-dimensional buckling, three-dimensional helical buckling).
- (5)
- Determining the contact points between the rod string and the tubing: Calculating the positions of contact points between the rod string and the tubing based on the stress and buckling analysis results.
- (6)
- Optimising the placement of centralisers: Prioritising placing centralisers in areas with contact points and high lateral forces.
- (7)
- Dynamically adjusting the spacing of centraliser placement: Adjusting the spacing of centralisers dynamically based on the wellbore curvature and the stress distribution of the rod string, avoiding the limitations of fixed-spacing designs.
- (8)
- Validating the design: Checking whether the centraliser placement effectively reduces wear and buckling, ensuring the design meets the requirements of actual well conditions.
- (9)
- Adjusting model parameters and re-optimising: If the design does not meet the requirements, model parameters must be adjusted (e.g., rod string diameter, number of centralisers), and the placement re-optimised.
- (10)
- Finalising the design: Outputting the placement positions and spacing of the centralisers, along with the stress and wear analysis results of the rod string.
4. Comparative Analysis of Application Cases
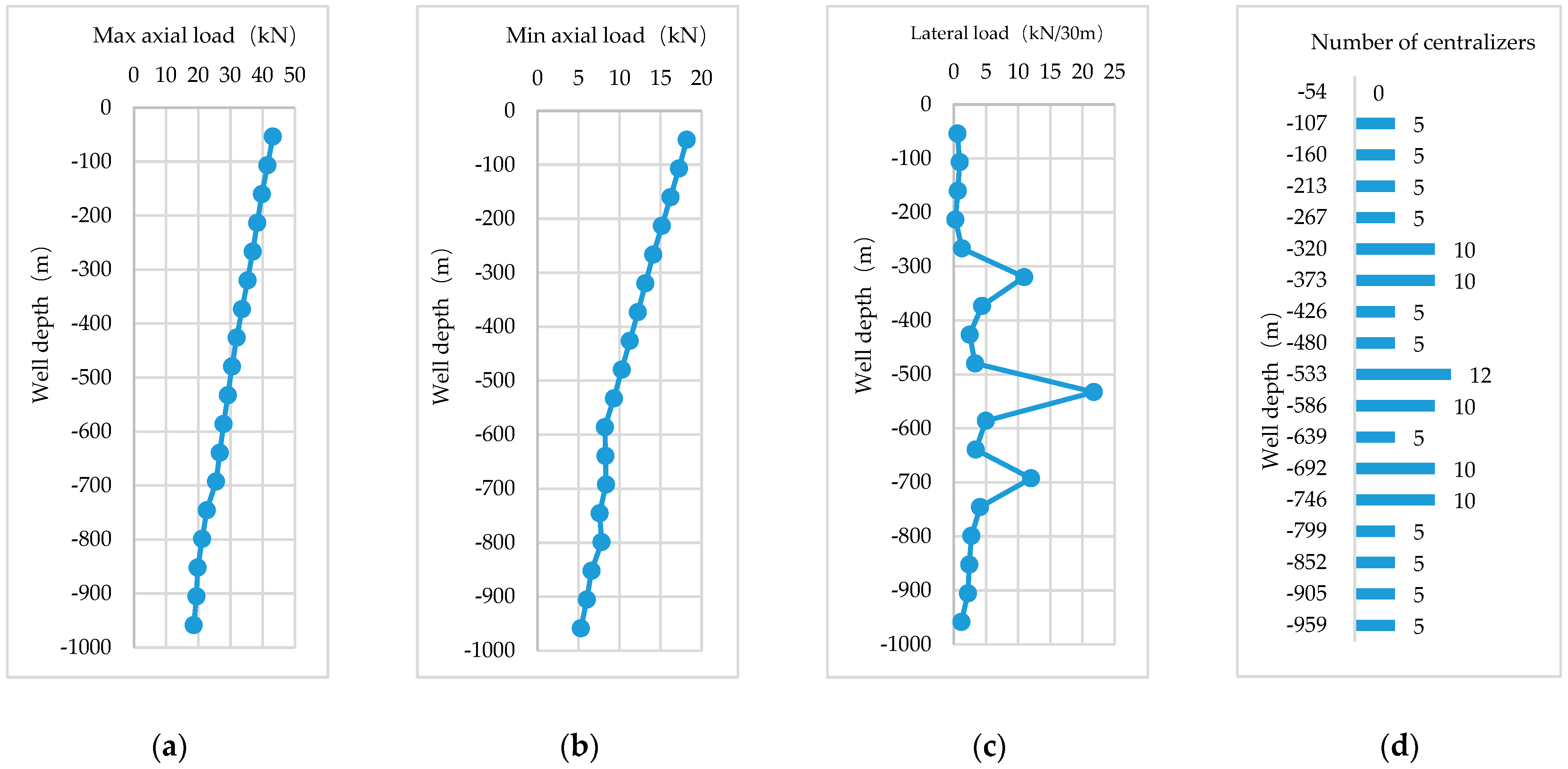
4.1. Experiment Case 1. A1# Oil Well Engineering Design
4.2. Experiment Case 2. A2# Oil Well Engineering Design
5. Conclusions
- (1)
- To solve the problems of SRS being prone to breakage and deflection in 3D curved wells, the mechanically coupled mathematical model of SRS in 3D curved wells is established. The central difference method is applied to solve the problem. In the solution procedure, the micro SRS element is taken as the force unit, while the influence of the elastic force, inertia force, and friction are included. The well trajectory, rod string structure, and tubing are treated as the external conditions.
- (2)
- The motion state and 3D stress–strain distribution of each point on SRS, as well as the location of the centraliser arrangement, are obtained by the proposed new dynamics model.
- (3)
- In the DaQing oilfield, the mechanically coupled mathematical model was applied to design the centraliser arrangement for two oil wells, achieving significant improvements in production performance. For the A1# oil well, the rod diameter was reduced from 22 mm to 19 mm, the maximum load decreased by 11.4% (from 27.67 kN to 24.52 kN), and the minimum load decreased by 9.3% (from 15.15 kN to 13.74 kN). The system efficiency increased by 25% (from 16% to 20%), and the pumping efficiency remained stable. For the A2# oil well, the pumping efficiency increased by 47.9% (from 39.8% to 58.9%), the system efficiency improved by 4.8% (from 33.4% to 35%), and the submergence depth increased by 860% (from 30.45 m to 292.33 m). This method, proposed in the study, overcomes the limitations of traditional approaches under complex well conditions through precise mathematical modelling and optimised design, significantly enhancing system efficiency, oil extraction efficiency, and production stability.
- (4)
- The practical oil well application shows that the arrangement of centralisers in the well section where the rod and tube deviation wear can improve the stability of the sucker rod pumping system, reduce the load of the suspension point, and improve the system efficiency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gibbs, S.G. Predicting the behavior of sucker rod pumping systems. J. Pet. Technol. 1963, 15, 769–778. [Google Scholar] [CrossRef]
- Svinos, J.G. Rodstar—An expert rod pumping system predictive simulator. In Proceedings of the Annual Technical Meeting, Calgary, AB, Canada, 10–13 June 1990; pp. 1–18. [Google Scholar] [CrossRef]
- Lea, J.F. Boundary Conditions Used with Dynamic Models of Beam Pump Performance; Chicago, United States, Amoco Production Company, Southwestern Petroleum Short Course: Chicago, IL, USA, 1988; pp. 251–263. [Google Scholar]
- Yin, J.J.; Sun, D.; Yang, Y.S. Predicting multi-tapered sucker-rod pumping systems with the analytical solution. J. Pet. Sci. Eng. 2021, 197, 108115. [Google Scholar] [CrossRef]
- Wang, X.B.; Lv, L.Q.; Li, S.; Pu, H.; Liu, Y.; Bian, B.; Li, D. Longitudinal vibration analysis of sucker rod based on a simplified thermo-solid model. J. Pet. Sci. Eng. 2021, 196, 107951. [Google Scholar] [CrossRef]
- Doty, D.R.; Schmidt, Z. An Improved Model for Sucker Rod Pumping. Soc. Pet. Eng. J. 1983, 23, 33–41. [Google Scholar] [CrossRef]
- Lelia, S.D.L.; Evans, R.D. A coupled rod and fluid dynamic model for predicting the behavior of sucker-rod pumping systems. SPE Prod. Facil. 1995, 10, 26–33. [Google Scholar] [CrossRef]
- Lelia, S.D.L.; Evans, R.D. A coupled rod and fluid dynamic model for prediction the behavior of sucker-rod pumping systems-Part 2: Parametric study and demonstration of model capabilities. SPE Prod. Facil. 1995, 10, 34–40. [Google Scholar] [CrossRef]
- Yu, G.A.; Wu, Y.J.; Wang, G.Y. Three dimensional vibration in a sucker rod beam pumping system. Acta Pet. Sin. 1989, 10, 76–82. [Google Scholar]
- Lollback, P.A.; Wang, G.Y.; Rahman, S.S. An alternative approach to the analysis of sucker-rod dynamics in vertical and deviated wells. J. Pet. Sci. Eng. 1997, 17, 313–320. [Google Scholar] [CrossRef]
- Wang, D.; Liu, H. Dynamic modeling and analysis of sucker rod pumping system in a directional well. In Mechanism and Machine Science; Springer: Singapore, 2016; pp. 1115–1127. [Google Scholar] [CrossRef]
- Feng, Z.M.; Ma, Q.Y.; Liu, X.L.; Cui, W.; Tan, C.D.; Liu, Y. Dynamic coupling modelling and application case analysis of high-slip motors and pumping units. PLoS ONE 2020, 15, e0227827. [Google Scholar] [CrossRef]
- Huang, W.J.; Gao, D.L.; Liu, Y.H. A study of tubular string buckling in vertical wells. Int. J. Mech. Sci. 2016, 118, 231–253. [Google Scholar] [CrossRef]
- Sun, X.R.; Jiang, S.B.; Huang, Y.Q.; Yao, H.T.; Li, S.F.; Dong, S.M. Simulation research of transverse vibration of sucker rod string under buckling deformation excitation in vertical wells. J. Vib. Eng. 2018, 31, 854–861. [Google Scholar] [CrossRef]
- Sun, X.R.; Dong, S.M.; Wang, H.B.; Li, W.C.; Sun, L. Comparison of multistage simulation models of entire sucker rod with spatial buckling in tubing. J. Jilin Univ. (Eng. Technol. Ed.) 2018, 48, 153–161. [Google Scholar] [CrossRef]
- Zhang, Q.; Jiang, B.; Huang, W.J.; Cui, W.; Liu, J.B. Effect of wellhead tension on buckling load of tubular strings in vertical wells. J. Pet. Sci. Eng. 2018, 164, 351–361. [Google Scholar] [CrossRef]
- Li, Z.F.; Li, J.Y. Fundamental equations for dynamic analysis of rod and pipe string in oil-gas wells and application in static buckling analysis. J. Can. Pet. Technol. 2002, 41, 44–53. [Google Scholar] [CrossRef]
- Lukasiewicz, S.A.; Knight, C. On lateral and helical buckling of a rod in a tubing. J. Can. Pet. Technol. 2006, 44, 17–21. [Google Scholar] [CrossRef]
- Gao, G.H.; Di, Q.F.; Miska, S.; Wang, W.H. Stability analysis of pipe with connectors in horizontal wells. SPE J. 2012, 17, 931–941. [Google Scholar] [CrossRef]
- Gao, D.L.; Huang, W.J. A review of down-hole tubular string buckling in well engineering. Pet. Sci. 2015, 12, 443–457. [Google Scholar] [CrossRef]
- Huang, W.J.; Gao, D.L. Helical buckling of a thin rod with connectors constrained in a cylinder. Int. J. Mech. Sci. 2014, 84, 89–198. [Google Scholar] [CrossRef]
- Moreno, G.A.; Garriz, A.E. Sucker rod string dynamics in deviated wells. J. Pet. Sci. Eng. 2020, 184, 106534. [Google Scholar] [CrossRef]
- Wang, H.B.; Dong, S.M. Research on the coupled axial-transverse nonlinear vibration of sucker rod string in deviated wells. J. Vib. Eng. Technol. 2021, 9, 115–129. [Google Scholar] [CrossRef]
- Wang, W.C. Research and Application of Dynamic Characteristics Analysis Method for Sucker Rod String in Three-Dimensional Curved Wells. Ph.D. Thesis, Shanghai University, Shanghai, China, 2011. [Google Scholar]
- Wang, W.C.; Bai, N.; Di, Q.F.; Wang, M.J.; Sheng, L.M.; Yang, X.K. A new method in designing position of centralizers of sucker rod strings in 3D directional wellbores. Drill. Prod. Technol. 2015, 38, 49–52+9. [Google Scholar] [CrossRef]
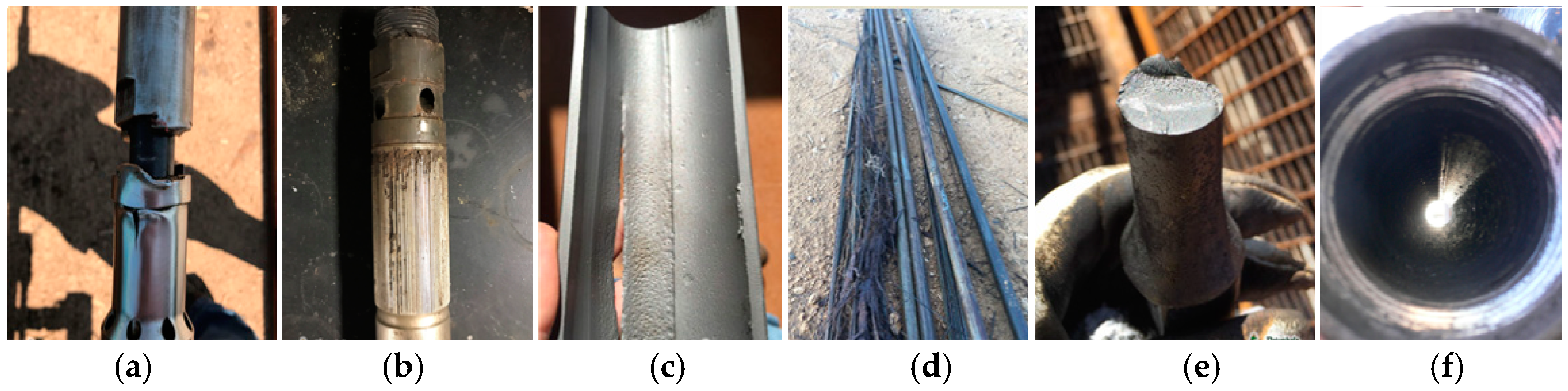



| Specific Elements | Unit | Data |
|---|---|---|
| Medium-deep oil layer | m | 990.6 |
| Relative density of crude oil | % | 0.85 |
| Production gas oil ratio | m3/m3 | 10.00 |
| Casing inner diameter | mm | 124.00 |
| Formation pressure | MPa | 10.53 |
| Relative density of formation water | % | 1.00 |
| Water cut | % | 0.99 |
| Inner diameter of oil pipe | mm | 76.00 |
| Reservoir temperature | °C | 54.62 |
| Relative density of natural gas | % | 0.70 |
| Specific Elements | Unit | Data |
|---|---|---|
| Stroke | m | 4.20 |
| Pump diameter | mm | 38.00 |
| Pump efficiency | % | 47.78 |
| Rod diameter | mm | 19 |
| Frequency of stroke | Hz | 6.00 |
| Pump depth | m | 900.05 |
| System efficiency | % | 15 |
| Liquid production | m3 | 19.65 |
| Dynamic liquid level depth | m | 650 |
| Submergence | m | 250.05 |
| Maximum load | KN | 28.07 |
| Specific Elements | Unit | Data |
|---|---|---|
| Stroke | m | 4.20 |
| Pump diameter | mm | 38.00 |
| Pump efficiency | % | 47.78 |
| Rod diameter | mm | 19 |
| Frequency of stroke | Hz | 6.00 |
| Pump depth | m | 900.05 |
| System efficiency | % | 20 |
| Liquid production | m3 | 19.65 |
| Dynamic liquid level depth | m | 600 |
| Submergence | m | 300.05 |
| Maximum load | KN | 24.52 |
| Specific Elements | Unit | Data |
|---|---|---|
| Medium-deep oil layer | m | 1057 |
| Relative density of crude oil | % | 85 |
| Production gas oil ratio | m3/m3 | 10.0 |
| Casing inner diameter | mm | 124.0 |
| Formation pressure | MPa | 10 |
| Relative density of formation water | % | 100 |
| Water cut | % | 89 |
| Inner diameter of oil pipe | mm | 76.0 |
| Reservoir temperature | °C | 53.35 |
| Relative density of natural gas | % | 70 |
| Specific Elements | Unit | Data |
|---|---|---|
| Stroke | m | 4.20 |
| Pump diameter | mm | 70.00 |
| Pump efficiency | % | 39.8 |
| Rod diameter | mm | 25 |
| Frequency of stroke | Hz | 4.00 |
| Pump depth | m | 992.3 |
| System efficiency | % | 33.4 |
| Liquid production | m3 | 36.39 |
| Dynamic liquid level depth | m | 961.88 |
| Submergence | m | 30.45 |
| Maximum load | KN | 56.45 |
| Specific Elements | Unit | Data |
|---|---|---|
| Stroke | m | 4.20 |
| Pump diameter | mm | 57.00 |
| Pump efficiency | % | 58.9 |
| Rod diameter | mm | 22 |
| Frequency of stroke | Hz | 4.00 |
| Pump depth | m | 992.3 |
| System efficiency | % | 35 |
| Liquid production | m3 | 36.39 |
| Dynamic liquid level depth | m | 700 |
| Submergence | m | 292.33 |
| Maximum load | KN | 51.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Z.; Guo, B.; Cai, Z.; Yuan, H. Eccentric Wear Mechanism and Centralizer Layout Design in 3D Curved Wellbores. Appl. Sci. 2025, 15, 1494. https://doi.org/10.3390/app15031494
Feng Z, Guo B, Cai Z, Yuan H. Eccentric Wear Mechanism and Centralizer Layout Design in 3D Curved Wellbores. Applied Sciences. 2025; 15(3):1494. https://doi.org/10.3390/app15031494
Chicago/Turabian StyleFeng, Ziming, Botao Guo, Zhihui Cai, and Heng Yuan. 2025. "Eccentric Wear Mechanism and Centralizer Layout Design in 3D Curved Wellbores" Applied Sciences 15, no. 3: 1494. https://doi.org/10.3390/app15031494
APA StyleFeng, Z., Guo, B., Cai, Z., & Yuan, H. (2025). Eccentric Wear Mechanism and Centralizer Layout Design in 3D Curved Wellbores. Applied Sciences, 15(3), 1494. https://doi.org/10.3390/app15031494





