Risk Identification Method and Application of Roof Water Inrush Under Multi-Working Face Mining
Abstract
1. Introduction
2. The Theory of Key Strata and Full Mining Disturbance
2.1. Identification of Key Strata
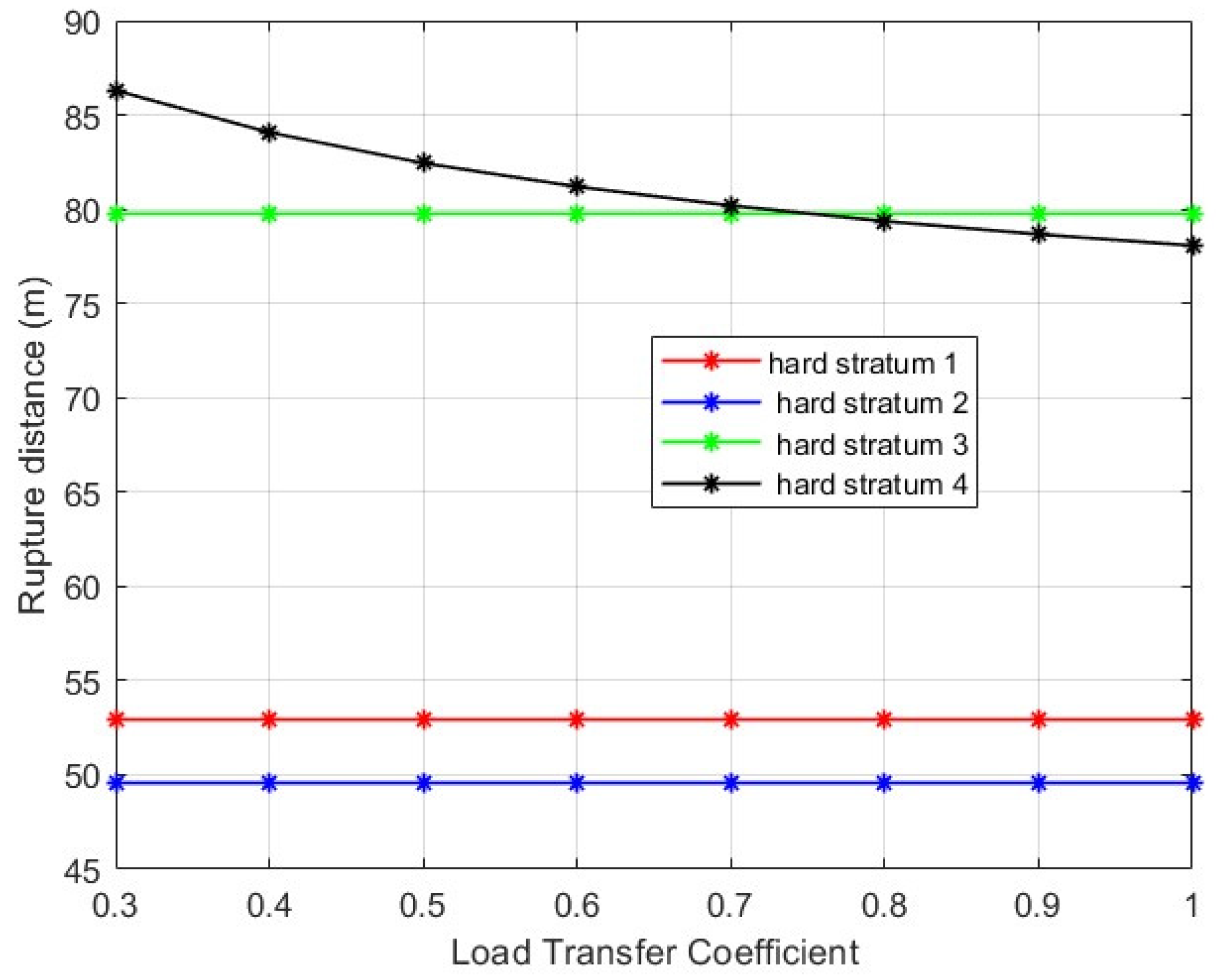
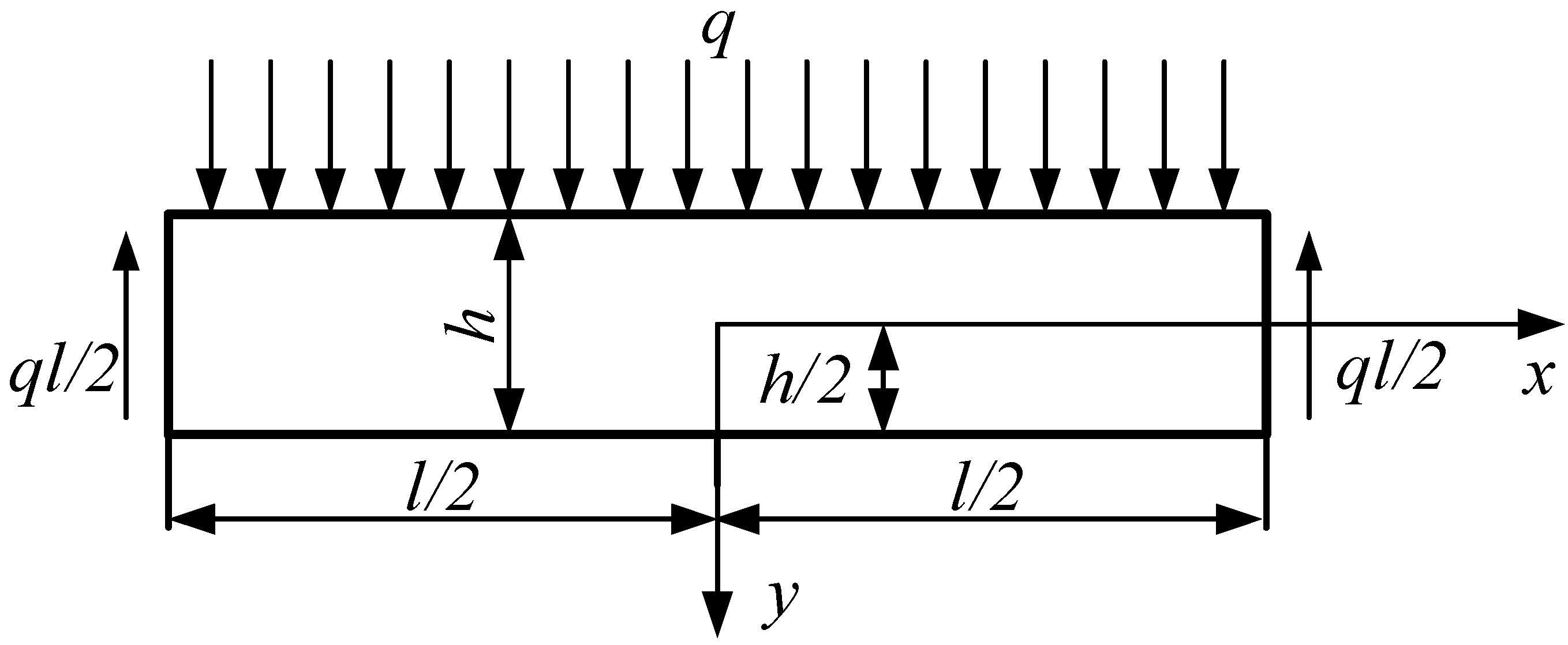
2.2. Critical Dimension Calculation
2.3. Prediction of the Development Height of Water-Conducting Fracture Zones
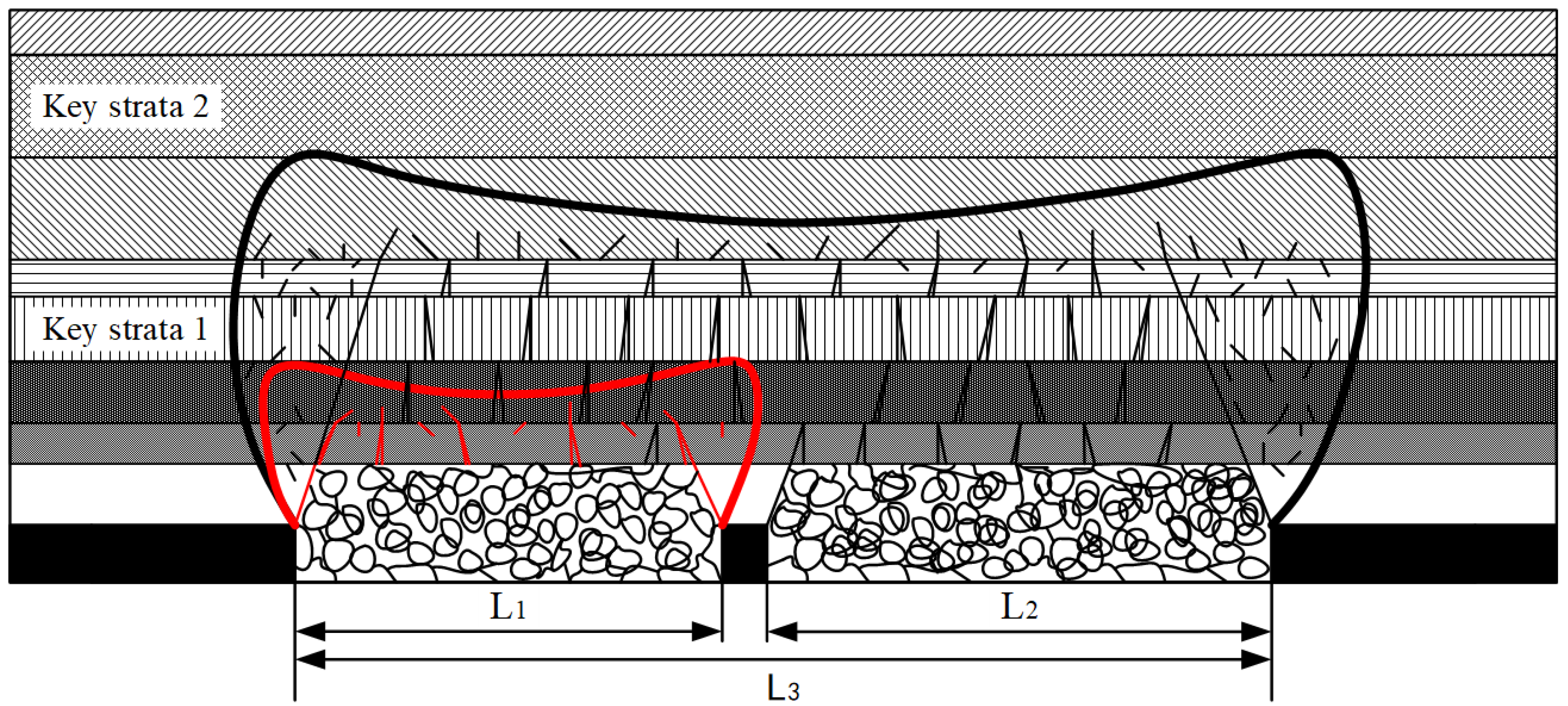
- When the critical dimension of the working face required for the fracture of the key stratum exceeds the combined width of the two working faces, the key stratum will remain intact, and the water-conducting fracture zone will not propagate upwards. Consequently, the maximum development height of the water-conducting fracture zone will be constrained to the height of the key stratum.
- Conversely, when the critical dimension of the working face for the fracture of the key stratum is less than the combined width of the two working faces, the key stratum will fracture, allowing the water-conducting fracture zone to propagate upwards. In this scenario, the maximum development height of the water-conducting fracture zone will be limited to the height of the upper key stratum that has not yet fractured.
- In cases where the critical dimension of the working face for the fracture of the key stratum is smaller than the combined width of the two working faces but greater than the width of the preceding working face, the mining-induced disturbance from the subsequent working face will result in an increase in the maximum development height of the water-conducting fracture zone in the preceding working face.
- Finally, when the main key stratum fractures, the maximum development height of the water-conducting fracture zone will be equal to or greater than the thickness of the bedrock.

2.4. Identification of the Risk of Roof Water Inrush
3. Engineering Application
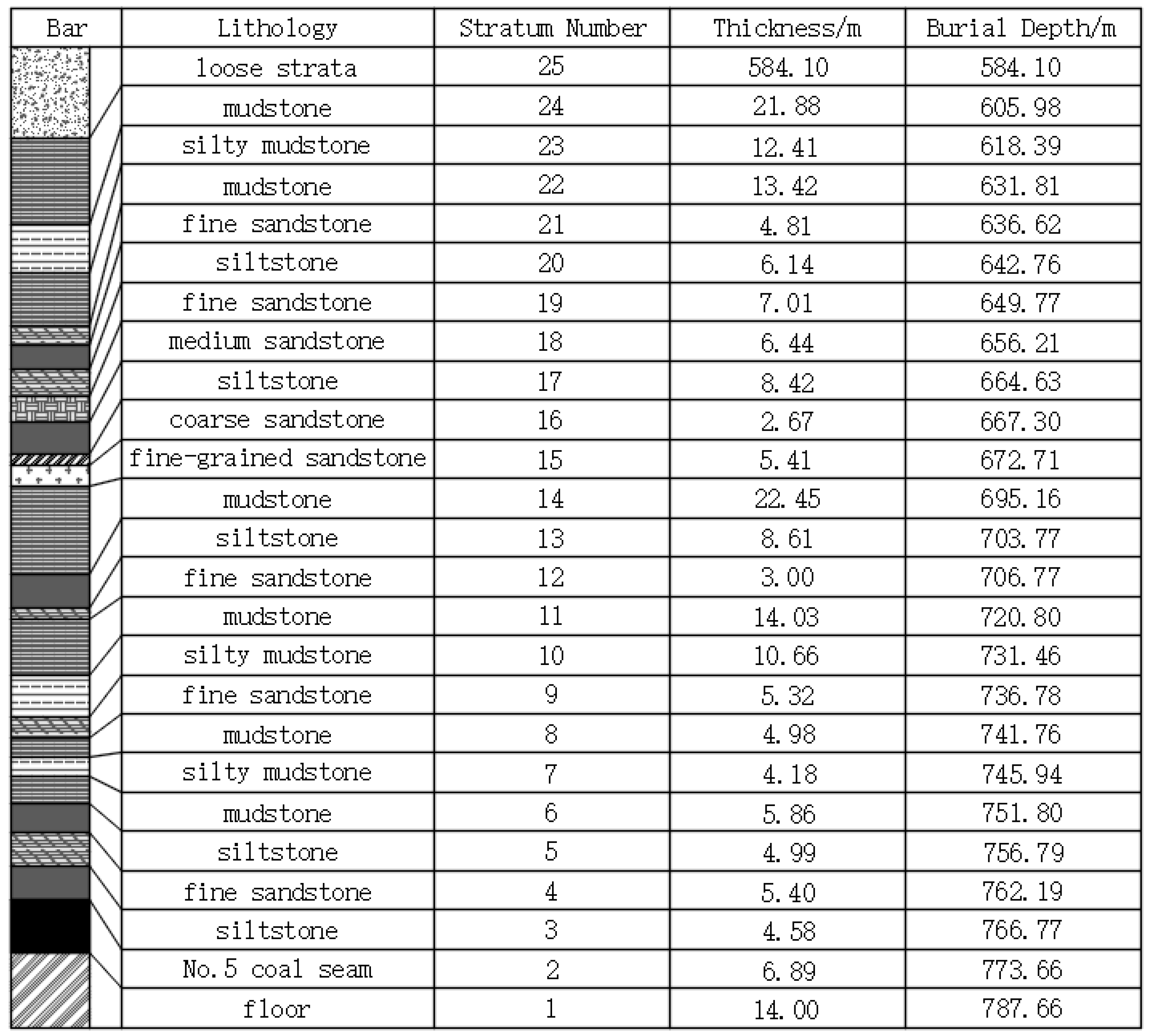
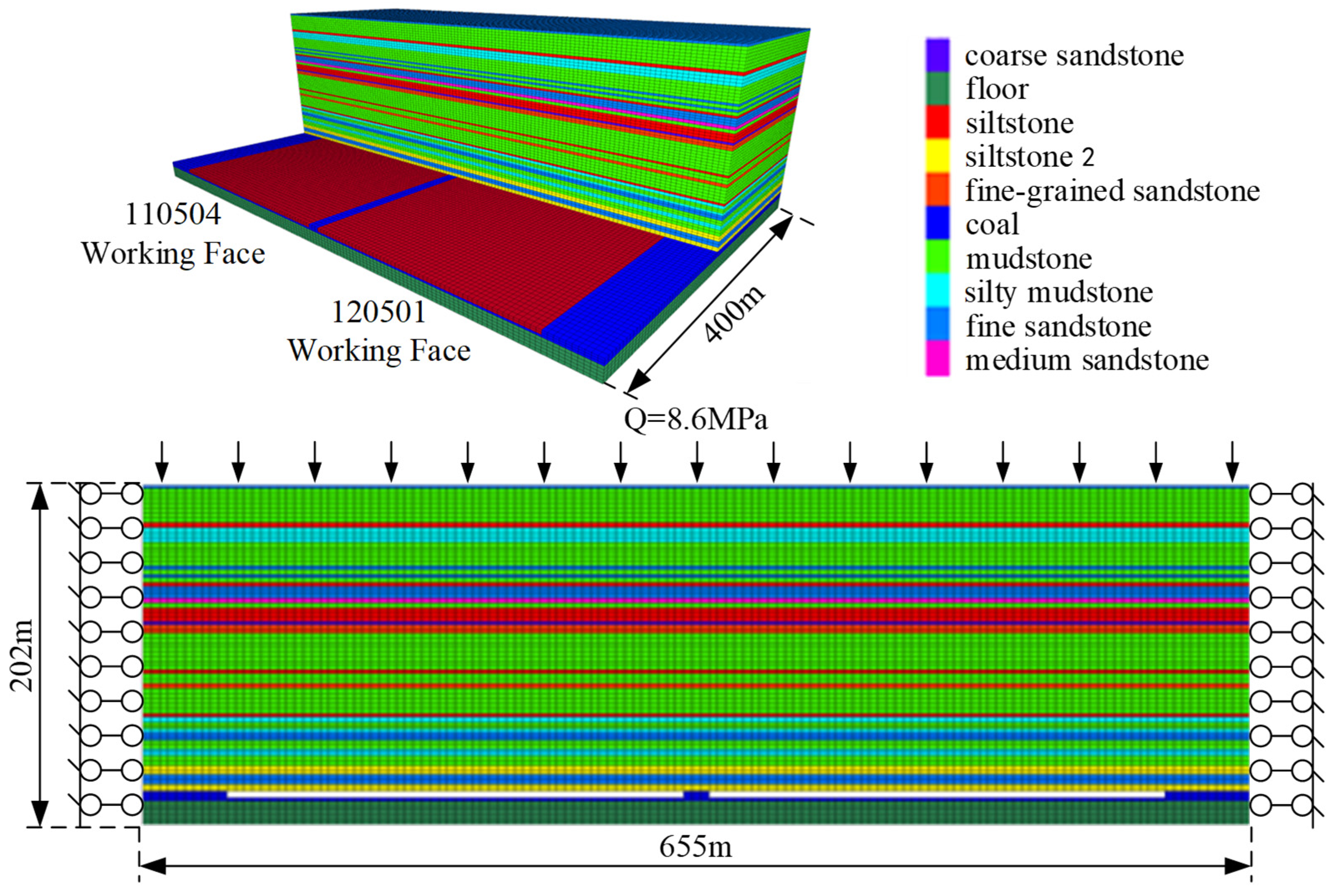
3.1. Engineering Overview
3.2. Engineering Theoretical Identification
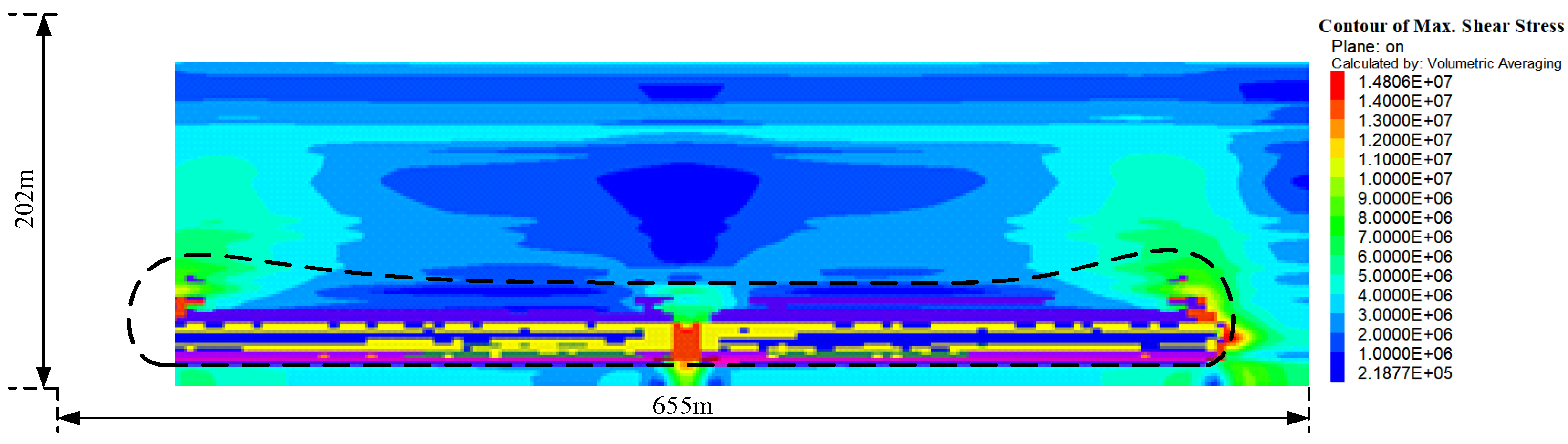
4. Numerical Simulation
5. Field Monitoring Verification
6. Discussion
- Overview: The research conducted in the domain of water-conducting fracture zone development and water inrush risk within mining engineering has significantly enhanced our understanding of the interactions between mining activities and geological formations. Key contributions to this field include the development of empirical formulas and predictive models for assessing the height of water-conducting fracture zones, employing various methodologies such as statistical analysis, numerical simulations, and machine learning techniques. Noteworthy studies have demonstrated the correlation between mining operations, stress distribution, and the likelihood of delayed water inrush incidents. Furthermore, innovative monitoring techniques, including distributed optical fiber systems, have been employed to track deformation in the overlying strata, providing valuable insights into the dynamic processes associated with fracture zone development. In summary, the findings not only contribute to the theoretical framework of mining engineering, but also offer practical implications for enhancing safety and environmental sustainability in mining practices.
- Novelty: This study introduces a novel risk identification method for roof water inrush under multi-working face mining conditions, grounded in the theory of Key Strata and Full Mining Disturbance. The proposed method incorporates the cumulative effects of repeated disturbances from multiple working faces and involves the derivation of a critical dimension formula for key strata failure. This formula is then applied to assess the delayed risk of water inrush from the roof of previously mined working faces. The predictive results obtained using this method provide a more accurate representation of the overall developmental characteristics of the water-conducting fracture zone.
- Limitations: The method for identifying the risk of roof water inrush under multi-working face mining conditions, based on the Key Strata Theory and Full Mining Disturbance Theory, relies on certain idealized assumptions. However, the actual geological conditions encountered in coal mining are often more complex, exhibiting anisotropy in the strata and spatial variability in rock properties, which may affect the general applicability and accuracy of the critical dimension calculation formula. Moreover, the analysis combining the Key Strata Theory and the Full Mining Disturbance Theory does not fully account for some dynamic factors that arise during the mining process. For instance, variations in mining speed can influence stress distribution and fracture development in the roof; however, the impact of this factor on the risk assessment of roof water inrush is not comprehensively addressed in the theoretical framework of the study. Additionally, the accuracy of the microseismic event location is significantly influenced by geological conditions. The underground environment in mining areas is often characterized by complex geological structures, such as fracture zones, bedding variations, and ore body distributions, all of which may lead to irregular refraction, reflection, or attenuation of seismic waves during propagation, thereby affecting the precision of the monitoring data. Background noise interference during the monitoring process may also reduce the accuracy of event localization to some extent [49,50].
- Outlook: Future work should focus on further considering the non-uniformity of geological structures and rock mechanical properties to refine the critical dimension calculation formula for the failure of the key strata. The introduction of probabilistic analysis methods could be beneficial in accounting for the variability and uncertainty of parameters such as rock properties, thus making the formula more representative of actual geological conditions. Moreover, comprehensive research into the mechanisms by which dynamic factors, such as mining speed, influence the risk of roof water inrush is warranted. These dynamic factors should be incorporated into existing theoretical models to enhance their predictive accuracy and applicability in real-world mining scenarios.
7. Conclusions
- A method of risk identification for roof water inrush under multi-working face mining conditions, based on the theory of Key Strata and Full Mining Disturbance, has been proposed. This method comprehensively accounts for the effects of repeated disturbances from multiple working faces and involves the derivation of a critical dimension formula for the key strata at the working face. When the critical dimension of the working face of the key stratum exceeds the combined width of the two working faces, the maximum development height of the water-conducting fracture zone is determined to be equal to the height of the key stratum. In contrast, if this dimension is less than the combined width, the maximum development height of the water-conducting fracture zone corresponds to the height of the unfractured portion of the upper key stratum. Furthermore, if the critical dimension exceeds the width of the preceding working face but remains less than the combined width of the working faces, there exists a risk of delayed water inrush from the roof of the preceding working face. Additionally, when the main key stratum experiences fracturing, the maximum development height of the water-conducting fracture zone is either equal to or greater than the thickness of the bedrock.
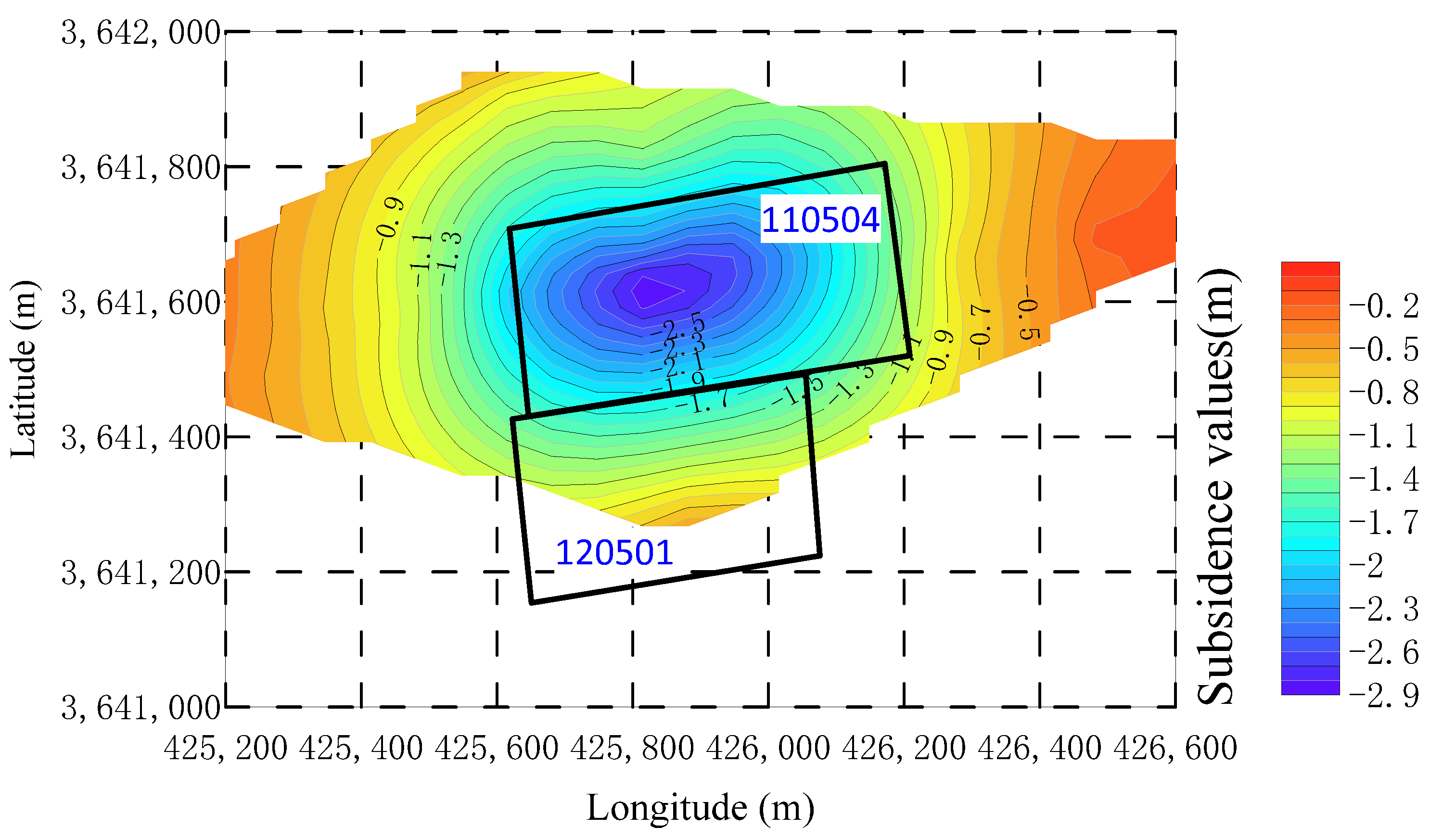
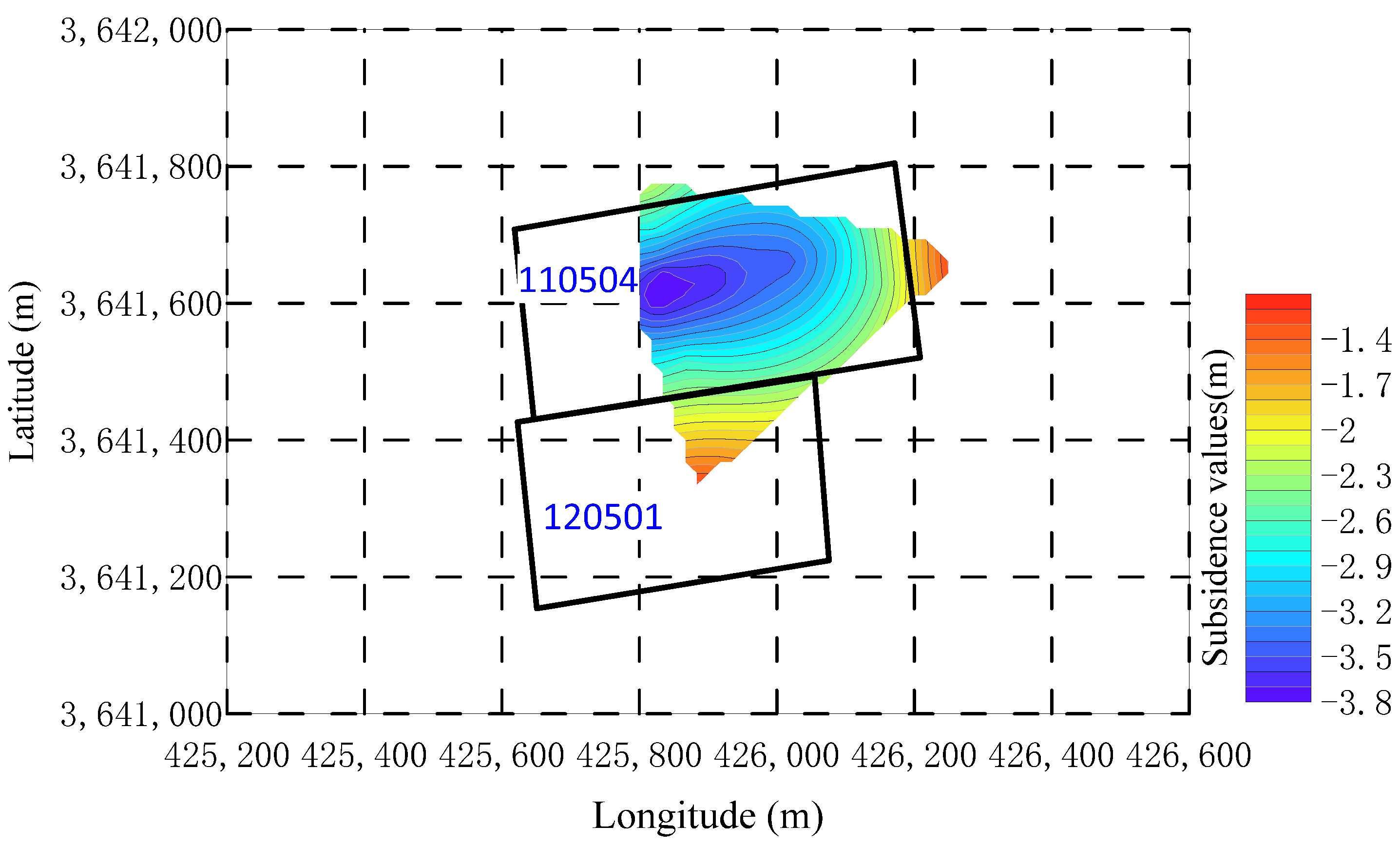
- Based on the theory of Key Strata and Full Mining Disturbance, in conjunction with numerical simulation calculations, it was determined that, for a mining height of 4 m, the development height of the water-conducting fracture zone in the 120501 working face is approximately 60 m, with the protective strata thickness ranging from 100 to 140 m. Under these conditions, there is no risk of roof water inrush, and the adjacent 110504 working face is not at risk for delayed roof water inrush.
- Microseismic monitoring was employed to measure the development height of the water-conducting fracture zone in the 120501 working face, which reached a maximum of 64 m. The development height of the water-conducting fracture zone in the 110504 working face remained unchanged, and no delayed roof water inrush was observed. The results align closely with the theoretical predictions, further validating the proposed method.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, Y.F. “Four-zone” model of rockmass movement and back analysis of dynamic displacement. J. China Coal Soc. 1996, 21, 51–56. [Google Scholar] [CrossRef]
- Qi, T.Y.; Zhang, F.; Pei, X.M.; Feng, G.R.; Wei, H.R. Simulation research and application on response characteristics of detecting water-filled goaf by transient electromagnetic method. Int. J. Coal Sci. Technol. 2022, 9, 167–192. [Google Scholar] [CrossRef]
- Wu, F.F.; Gao, Z.Q.; Liu, H.D.; Yu, X.; Gu, H.Y. Theoretical discrimination method of water-flowing fractured zone development height based on thin plate theory. Appl. Sci. 2024, 14, 6284. [Google Scholar] [CrossRef]
- Gao, Z.J.; Ding, Z.Q.; Liu, J.T.; Wang, Z.Y.; Wang, S.; Liu, W.Y. Study on water distribution and migration characteristics of inverted saturated zone and unsaturated zone beneath rive. J. Shandong Univ. Sci. Technol. (Nat. Sci.) 2023, 42, 1–10. [Google Scholar] [CrossRef]
- Xu, J.L. Research and progress of coal mine green mining in 20 years. Coal Sci. Technol. 2020, 48, 1–15. [Google Scholar] [CrossRef]
- Ma, D.; Duan, H.Y.; Zhang, J.X.; Bai, H.B. A state-of-the-art review on rock seepage mechanism of water inrush disaster in coal mines. Int. J. Coal Sci. Technol. 2022, 9, 50. [Google Scholar] [CrossRef]
- Liu, X.S.; Song, S.L.; Tan, Y.L.; Fan, D.Y.; Ning, J.G.; Li, X.B.; Yin, Y.C. Similar simulation study on the deformation and failure of surrounding rock of a large section chamber group under dynamic loading. Int. J. Min. Sci. Technol. 2021, 31, 495–505. [Google Scholar] [CrossRef]
- Liu, W.T.; Li, H.; Zhao, J.Y.; Shen, J.J. Deformation and stability in coal seam mining under fluid–solid coupling. Geotech. Geol. Eng. 2024, 42, 4333–4348. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, J.K.; Zhang, J.X.; Sun, X.M.; Chen, C.; Zheng, X.Y.; Xu, H.C. Modeling the spatial and temporal evolution of stress during multiworking face mining in close distance coal seams. Adv. Civ. Eng. 2021, 2021, 5624972. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, D.S.; Feng, G.R. Quantitative evaluation and planning method of shallow surface water response in multi-face mining—Case study regarding Zhuanlongwan coal mine. J. Clean. Prod. 2022, 373, 133830. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Cui, B.Y.; Wang, Y.N.; Zhang, S.; Feng, G.R.; Zhang, Z.J. Evolution law of shallow water in multi-face mining based on partition characteristics of catastrophe theory. Fractal Fract. 2023, 7, 779. [Google Scholar] [CrossRef]
- Zheng, L.L.; Wang, X.K.; Lan, H.; Ren, W.D.; Tian, Y.W.; Xu, J.; Tian, S.Y. Study of the development patterns of water-conducting fracture zones under karst aquifers and the mechanism of water inrush. Sci. Rep. 2024, 14, 20790. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.B.; Yang, D.M.; Zhang, Z.Q.; Sun, Y.; Zhao, L.S. Study on fracture evolution and water-conducting fracture zone height beneath the sandstone fissure confined aquifer. Sustainability 2024, 16, 6006. [Google Scholar] [CrossRef]
- Wu, Z.Y.; Chen, Y.; Luo, D.Y. Comparative study of five machine learning algorithms on prediction of the height of the water-conducting fractured zone in undersea mining. Sci. Rep. 2024, 14, 21047. [Google Scholar] [CrossRef]
- Chang, X.K.; Wang, M.G.; Zhu, W.; Fan, J.M.; Liu, M.Y. Study on height development characteristics of water-conducting fracture zone in fully mechanized mining of shallow thick coal seam under water. Sustainability 2023, 15, 7370. [Google Scholar] [CrossRef]
- Chai, J.; Ma, Z.; Du, W.G.; Gao, J.B.; Huang, Z.M.; Zhang, D.D.; Yang, J.F. Dynamic evolution law analysis of overburden separation and water flowing fracture under mining based on distributed optical fiber and information entropy theory. Opt. Fiber Technol. 2023, 80, 103408. [Google Scholar] [CrossRef]
- Xie, J.L.; Wang, X.Z.; Qiao, W. In situ monitoring and analysis of the development characteristics of separation in internal overburden. Appl. Sci. 2024, 14, 6935. [Google Scholar] [CrossRef]
- Hisz, D.B.; Ebenhack, J.; Burbey, T.J.; Germanovich, L.N.; Murdoch, L.C. MultiComponent Deformation of a Dipping Fracture Zone during a Well Test. In Proceedings of the AGU Fall Meeting Abstracts, San Francisco, CA, USA, 14–18 December 2009. [Google Scholar]
- Hisz, D.B.; Ebenhack, J.; Germanovich, L.N.; Murdoch, L.C. Characterization of Fractured Rock during Well Tests using Tilt-X, a Portable Tiltmeter and Extensometer for MultiComponent Deformation measurements. In Proceedings of the AGU Fall Meeting Abstracts, San Francisco, CA, USA, 13–17 December 2010. [Google Scholar] [CrossRef]
- Gusev, V.; Maliukhina, E. Prediction of Water Conducting Fracture Zone. Int. J. Appl. Eng. Res. 2016, 11, 11. [Google Scholar]
- Poulsen, B.A.; Adhikary, D.; Guo, H. Simulating mining-induced strata permeability changes. Eng. Geol. 2018, 237, 208–216. [Google Scholar] [CrossRef]
- Bezruchko, K.A.; Prykchodchenko, V.F.; Prykchodchenko, O.V.; Hladka, M.O. Predicting zones of increased water inflows in local folded structures. Sci. Bull. Natl. Min. Univ. 2023, 4, 5–10. [Google Scholar] [CrossRef]
- Vlastos, S.; Liu, E.; Main, I.G.; Li, X.Y. Numerical simulation of wave propagation in media with discrete distributions of fractures: Effects of fracture sizes and spatial distributions. Geophys. J. Int. 2003, 152, 649–668. [Google Scholar] [CrossRef]
- Barlow, P.M.; Leake, S.A. Streamflow Depletion by Wells—Understanding and Managing the Effects of Groundwater Pumping on Streamflow; Circular 1376; U.S. Geological Survey Circular: Reston, VI, USA, 2012.
- Taucare, M.; Viguier, B.; Daniele, L.; Heuser, G.; Leonardi, V. Connectivity of fractures and groundwater flows analyses into the Western Andean Front by means of a topological approach (Aconcagua Basin, Central Chile). Hydrogeol. J. 2020, 28, 2429–2438. [Google Scholar] [CrossRef]
- Li, J.; He, Z.; Piao, C.; Chi, W.; Lu, Y. Research on Subsidence Prediction Method of Water-Conducting Fracture Zone of Overlying Strata in Coal Mine Based on Grey Theory Model. Water 2023, 15, 4177. [Google Scholar] [CrossRef]
- Ding, Z.W.; Wang, S.Y.; Liao, J.L.; Li, L.; Jia, J.D.; Tang, Q.B.; Li, X.F.; Gao, C.D. Reasonable Working-Face Size Based on Full Mining of Overburden Failure. Sustainability 2023, 15, 3351. [Google Scholar] [CrossRef]
- Guo, W.B.; Lou, G.Z. Definition and distinguishing method of critical mining degree of overburden failure. J. China Coal Soc. 2019, 44, 755–766. [Google Scholar] [CrossRef]
- Qian, M.G.; Miao, X.X.; Xu, J.L. Theoretical study of key stratum in ground control. J. China Coal Soc. 1996, 21, 2–7. [Google Scholar]
- Qian, M.G.; Miao, X.X.; He, F.L. Analysis of key block in the structure of voussoir beam in longwall mining. J. China Coal Soc. 1994, 19, 557–563. [Google Scholar] [CrossRef]
- Liu, X.S.; Fan, D.Y.; Tan, Y.L.; Ning, J.G.; Song, S.L.; Wang, H.L.; Li, X.B. New detecting method on the connecting fractured zone above the coal face and a case study. Rock Mech. Rock Eng. 2021, 54, 4379–4391. [Google Scholar] [CrossRef]
- Liu, X.S.; Tan, Y.L.; Wu, Y.H.; Li, X.B. Microstructure, Characterization and Mechanical Properties of Coal and Coal-Like Materials. Materials 2023, 16, 1913. [Google Scholar] [CrossRef]
- He, Y.F.; Zhang, J.; Yang, T.; Wu, J.J.; Gao, S.S.; Sun, J.P. Study on the bearing structure of key strata and the linkage evolution mechanism of surface subsidence in shallow coal seam mining. Appl. Sci. 2024, 14, 9608. [Google Scholar] [CrossRef]
- Liu, J.X.; Zhang, S.C.; Shen, B.T.; Xiao, Y.; Li, Y.Y.; Zhang, Y.C. Study on fractal characteristics of fracture network in overlying strata mining under different breaking distances. J. Shandong Univ. Sci. Technol. (Nat. Sci.) 2024, 43, 11–21. [Google Scholar] [CrossRef]
- Zhang, B.; Cao, S.G. Study on first caving fracture mechanism of overlying roof rock in steep thick coal seam. Int. J. Min. Sci. Technol. 2015, 25, 133–138. [Google Scholar] [CrossRef]
- Zhu, D.F.; Yu, B.B.; Wang, D.Y.; Zhang, Y.J. Fusion of finite element and machine learning methods to predict rock shear strength parameters. J. Geophys. Eng. 2024, 21, 1183–1193. [Google Scholar] [CrossRef]
- Xu, J.L.; Zhu, W.B.; Wang, X.Z. New method to predict the height of fractured water-conducting zone by location of key strata. J. China Coal Soc. 2012, 37, 762–769. [Google Scholar] [CrossRef]
- Vu, T.T.; Do, S.A. Determination of the rock mass displacement zone by numerical modeling method when exploiting the longwall at the Nui Beo Coal Mine, Vietnam. Min. Miner. Depos. 2023, 17, 59–66. [Google Scholar] [CrossRef]
- Wu, W.B. Coupled numerical model of hydraulic fracturing and seepage of soft coal based on elastoplastic damage. J. Shandong Univ. Sci. Technol. (Nat. Sci.) 2021, 40, 69–76. [Google Scholar] [CrossRef]
- Smoliński, A.; Malashkevych, D.; Petlovanyi, M.; Rysbekov, K.; Lozynskyi, V.; Sai, K. Research into Impact of Leaving Waste Rocks in the Mined-Out Space on the Geomechanical State of the Rock Mass Surrounding the Longwall Face. Energies 2022, 15, 9522. [Google Scholar] [CrossRef]
- Fan, D.Y.; Liu, X.S.; Tan, Y.L.; Yang, S.L. Energy mechanism of bolt supporting effect to fissured rock under static and dynamic loads in deep coal mines. Int. J. Min. Sci. Technol. 2024, 34, 371–384. [Google Scholar] [CrossRef]
- Babets, D.; Sdvyzhkova, O.; Hapieiev, S.; Shashenko, O.; Prykhodchenko, V. Multifactorial analysis of a gateroad stability at goaf interface during longwall coal mining—A case study. Min. Miner. Depos. 2023, 17, 9–19. [Google Scholar] [CrossRef]
- Bai, E.H.; Guo, W.B.; Tan, Y.; Li, X.Y.; Shen, C.B.; Ma, Z.B. Green coal mining under buildings by overburden grout injection for coalmine sustainable development of central China. Heliyon 2023, 9, e18965. [Google Scholar] [CrossRef]
- Mohammad, N.; Reddish, D.J.; Stace, L.R. The relation between in situ, and laboratory rock properties used in numerical modelling. Int. J. Rock Mech. Min. Sci. 1997, 34, 289–297. [Google Scholar] [CrossRef]
- Cai, M.F.; He, M.C.; Liu, D.Y. Rock Mechanics and Engineering; Science Press: Beijing, China, 2022. [Google Scholar]
- Gong, Y.Q.; Guo, G.L. A Data-Intensive FLAC3D Computation Model: Application of Geospatial Big Data to Predict Mining Induced Subsidence. Comput. Model. Eng. Sci. 2019, 119, 395–408. [Google Scholar] [CrossRef]
- Zhang, Y. Based on Flac3D Research and Analysis of the Mining Pressure Development Law in the Top Coal Section of the Roadway Support of the Working Face Transport. Int. J. Energy 2024, 5, 14–18. [Google Scholar] [CrossRef]
- Wei, C.Y.; Shu, B.B. Research on Stability of Mining Roadway of “Three Soft” Coal Seam based on FLAC3D. Int. J. Energy 2024, 5, 42–46. [Google Scholar] [CrossRef]
- Baziw, E.; Weir-Jones, I. Application of Kalman Filtering Techniques for Microseismic Event Detection. Pure Appl. Geophys. 2002, 159, 449–471. [Google Scholar] [CrossRef]
- Calvez, J.L.; Ay, E. Introduction to this special section: Microseismic monitoring. Lead. Edge 2024, 43, 5. [Google Scholar] [CrossRef]






| Lithology | Elastic Modulus /GPa | Bulk Density /(KN·m−3) | Tensile Strength /MPa | Compressive Strength /MPa |
|---|---|---|---|---|
| coal | 1.56 | 14.10 | 0.23 | 12 |
| mudstone | 6.00 | 25.35 | 1.64 | 15 |
| silty mudstone | 10.00 | 24.96 | 2.20 | 42 |
| siltstone | 18.00 | 26.30 | 3.86 | 46 |
| medium sandstone | 26.00 | 25.28 | 4.95 | 43 |
| fine sandstone | 22.00 | 26.74 | 6.22 | 44 |
| fine-grained sandstone | 18.00 | 25.48 | 3.56 | 37 |
| siltstone 2 | 7.20 | 24.95 | 2.08 | 35 |
| coarse sandstone | 12.98 | 23.89 | 2.24 | 41 |
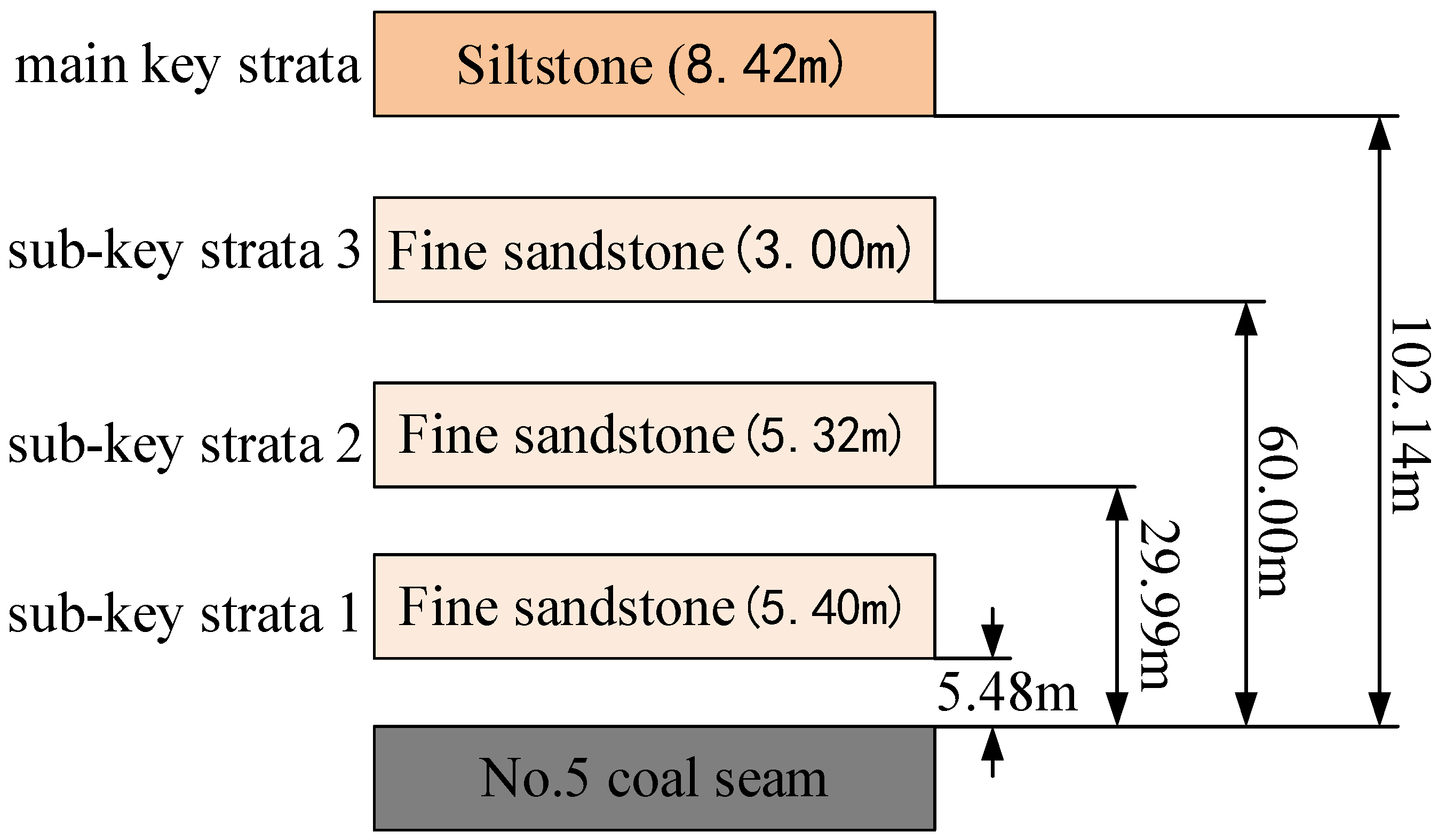
| Stratum Number | Lithology | Distance to the Roof of the No. 5 Coal Seam/m | Critical Dimension |
|---|---|---|---|
| 4 | fine sandstone | 4.58 | 110 |
| 9 | fine sandstone | 29.99 | 125 |
| 12 | fine sandstone | 60.00 | ∞ |
| 17 | siltstone | 102.14 | ∞ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Z.; Wang, K.; Liu, X.; Zhao, Y.; Li, X.; Fu, B.; Zhou, Y. Risk Identification Method and Application of Roof Water Inrush Under Multi-Working Face Mining. Appl. Sci. 2025, 15, 3511. https://doi.org/10.3390/app15073511
Huang Z, Wang K, Liu X, Zhao Y, Li X, Fu B, Zhou Y. Risk Identification Method and Application of Roof Water Inrush Under Multi-Working Face Mining. Applied Sciences. 2025; 15(7):3511. https://doi.org/10.3390/app15073511
Chicago/Turabian StyleHuang, Zhendi, Kun Wang, Xuesheng Liu, Yongqiang Zhao, Xuebin Li, Biao Fu, and Yu Zhou. 2025. "Risk Identification Method and Application of Roof Water Inrush Under Multi-Working Face Mining" Applied Sciences 15, no. 7: 3511. https://doi.org/10.3390/app15073511
APA StyleHuang, Z., Wang, K., Liu, X., Zhao, Y., Li, X., Fu, B., & Zhou, Y. (2025). Risk Identification Method and Application of Roof Water Inrush Under Multi-Working Face Mining. Applied Sciences, 15(7), 3511. https://doi.org/10.3390/app15073511






