The Influence of Lateral Wind Velocity on Spray Drift Dynamics of Liquid Droplets Sprayed by Agricultural Robot
Abstract
:1. Introduction
2. Materials and Methods
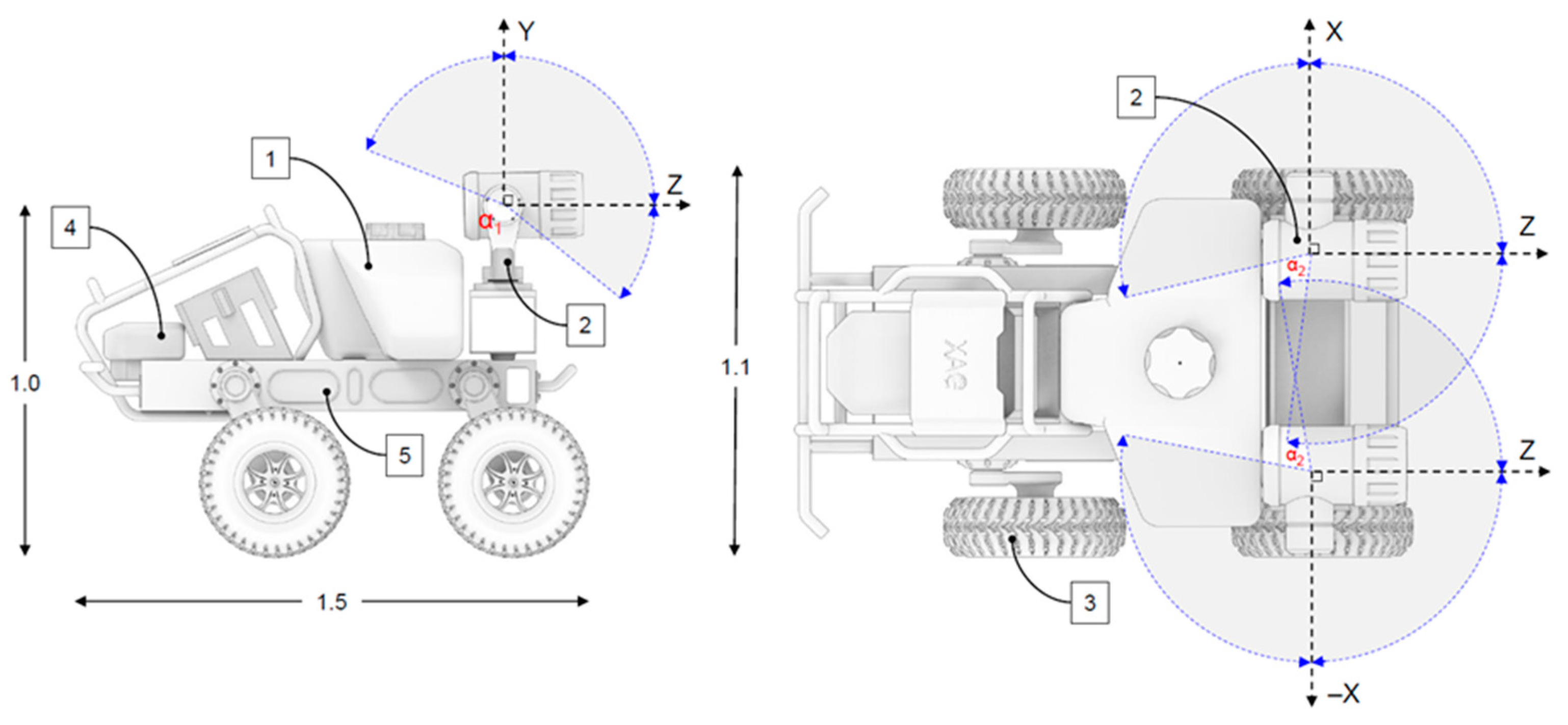
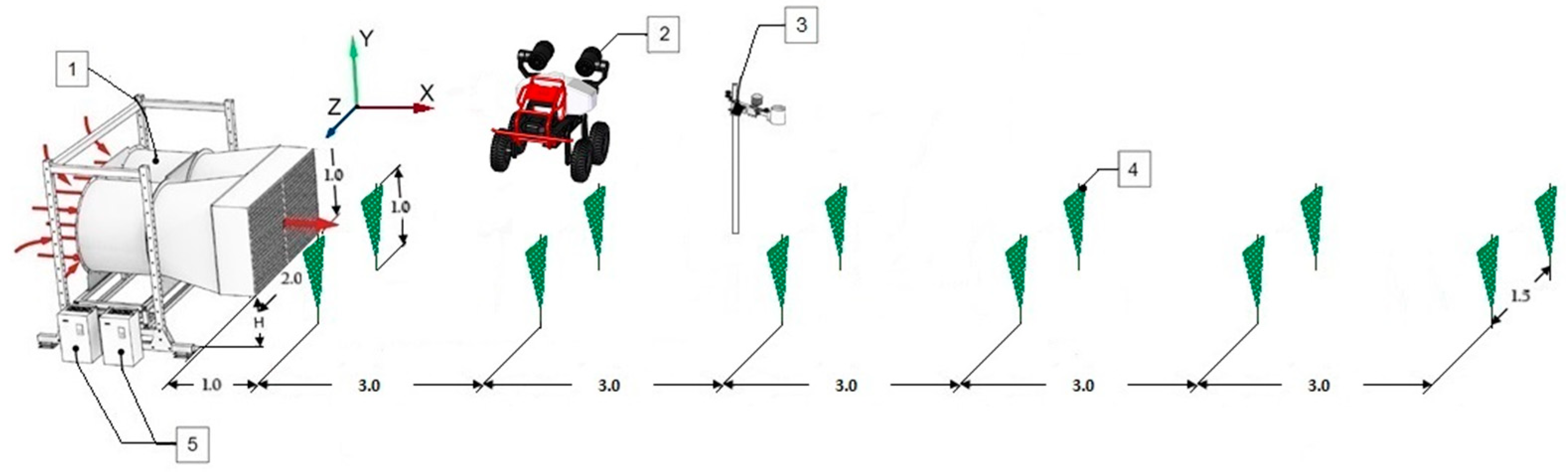
2.1. Tools and Equipment Used
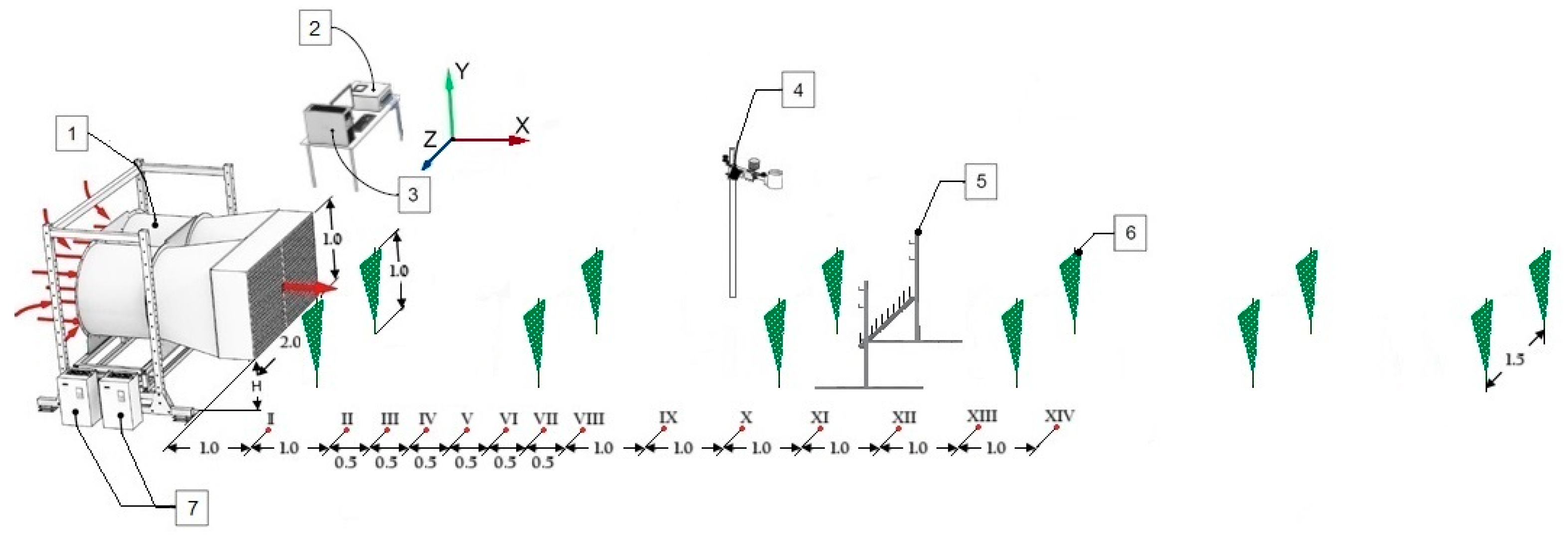
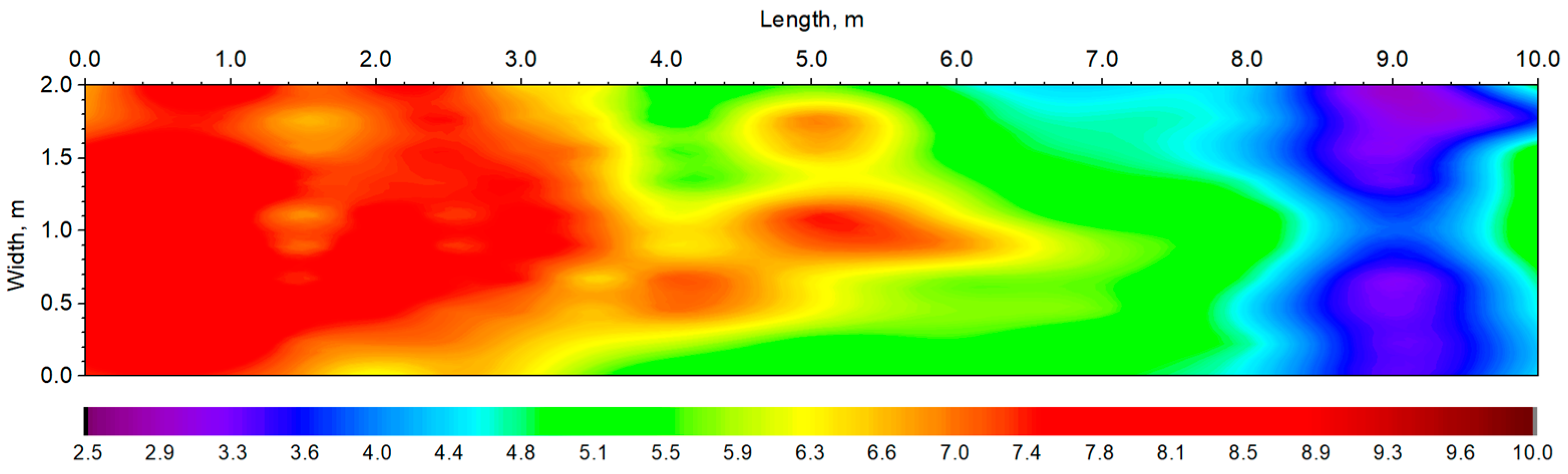
2.2. Determination of the Uniformity of Lateral Wind Generated by the Air Flow Generator
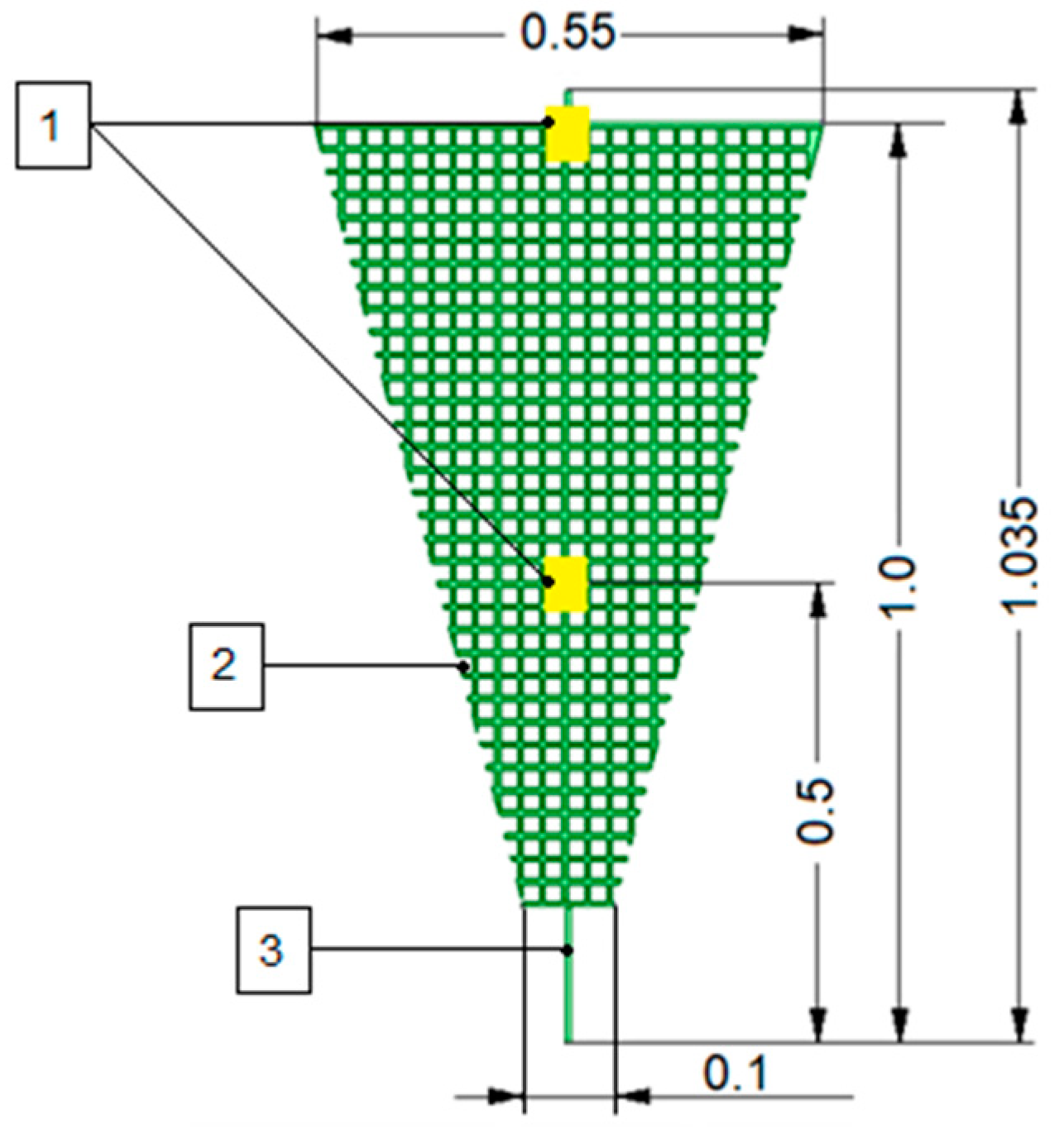
2.3. Experimental Spray Studies and Determination of Spray Drift
2.4. Drift Reduction Agents
2.5. Droplet Analysis and Statistical Analysis of the Resulting Data
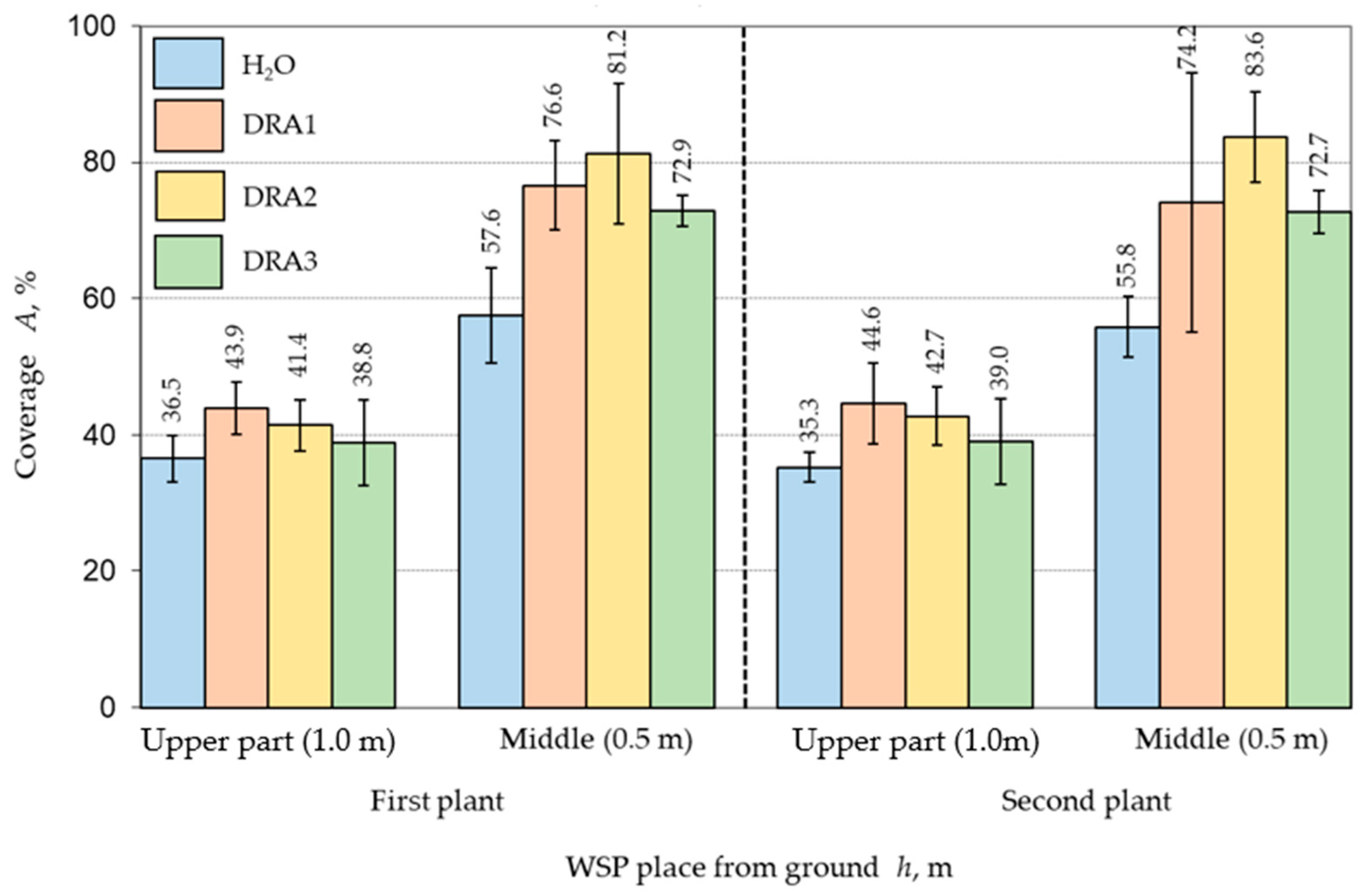
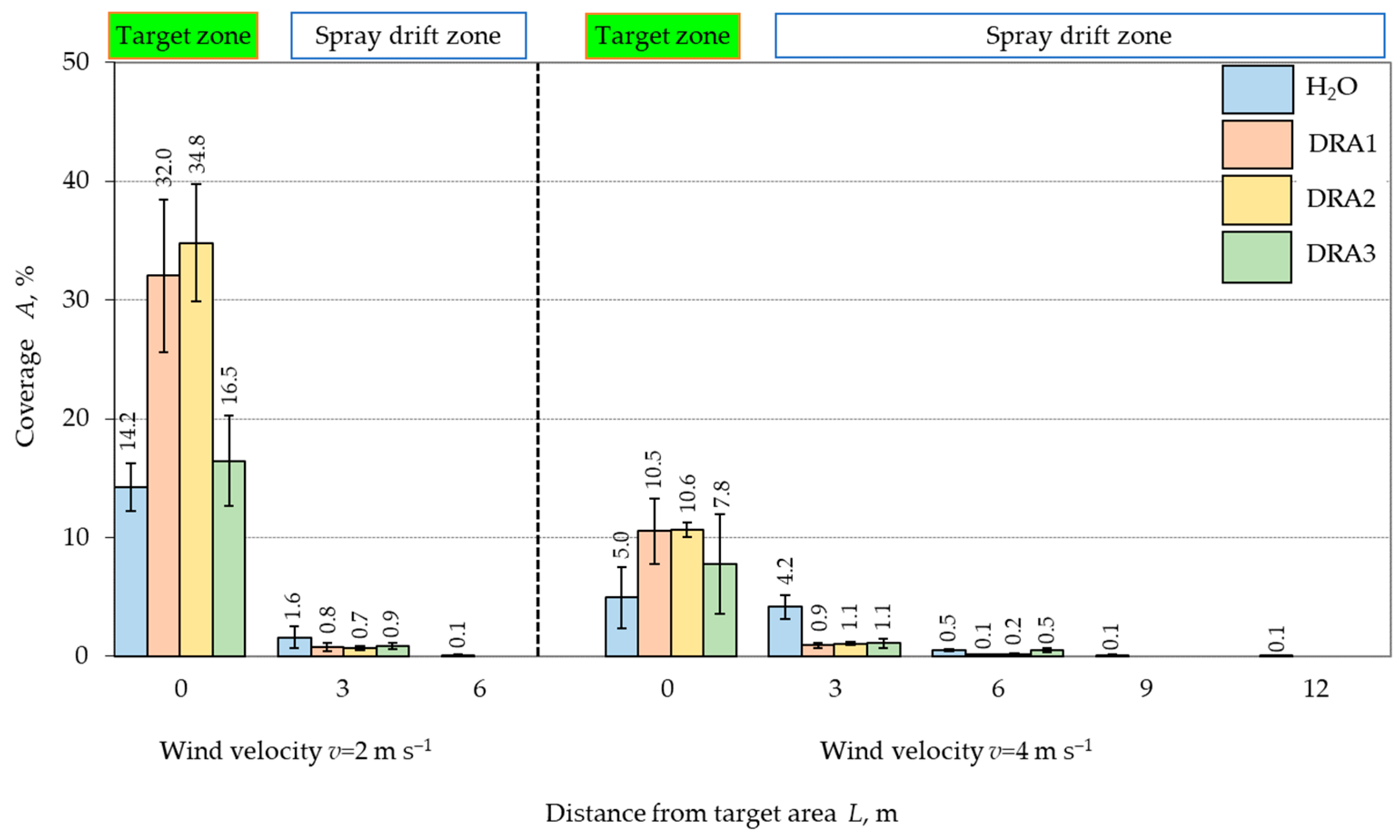
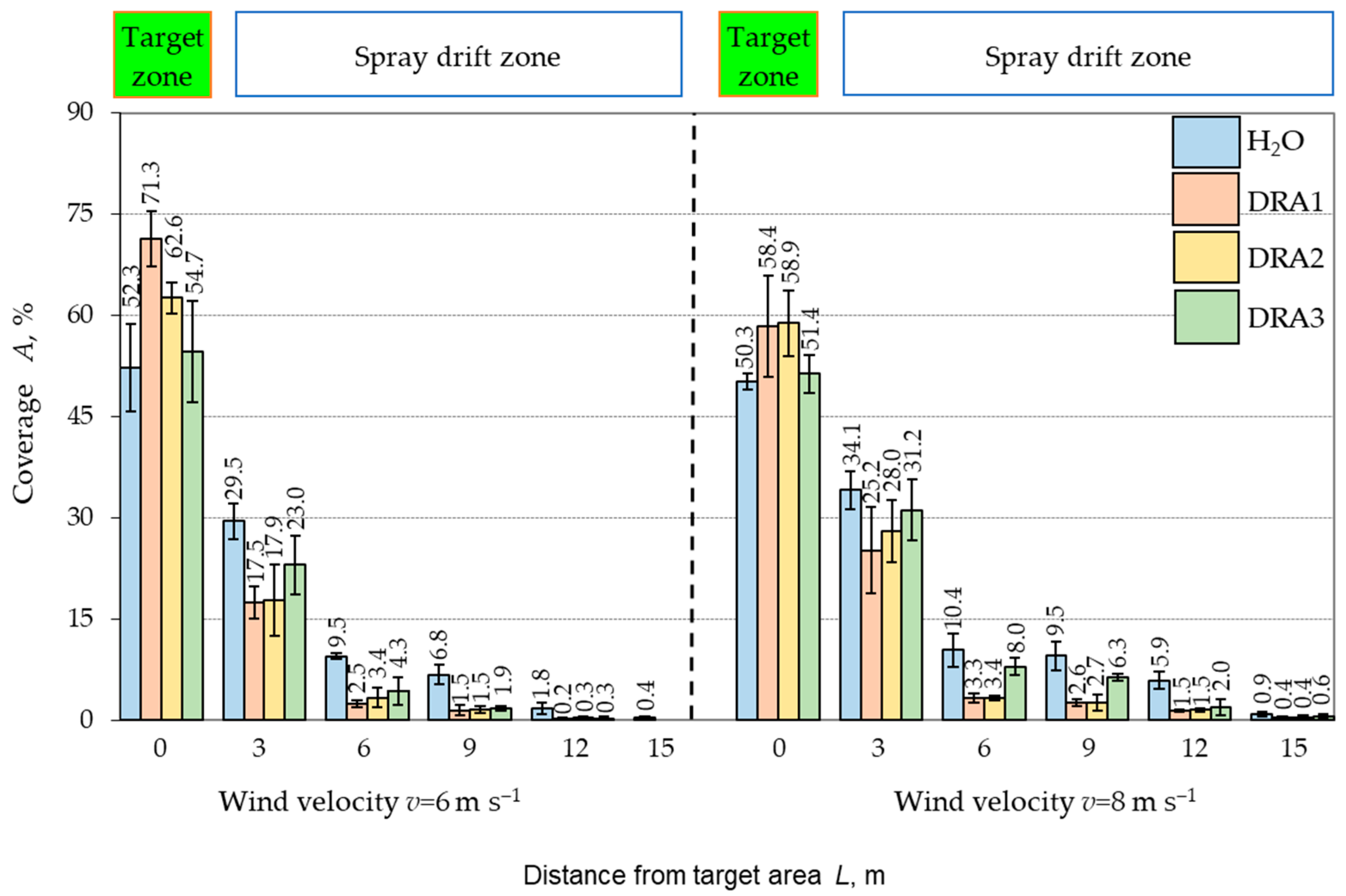
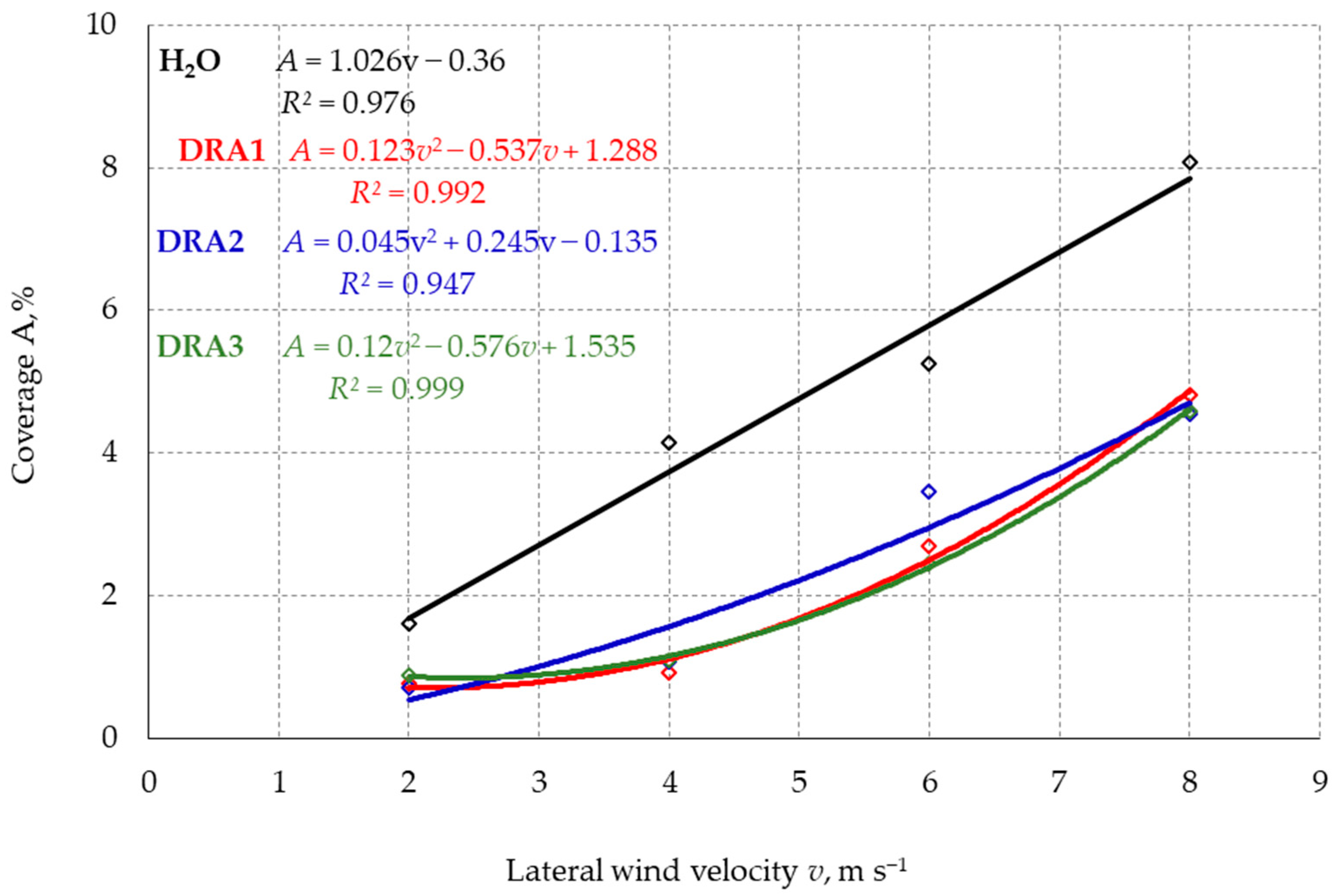
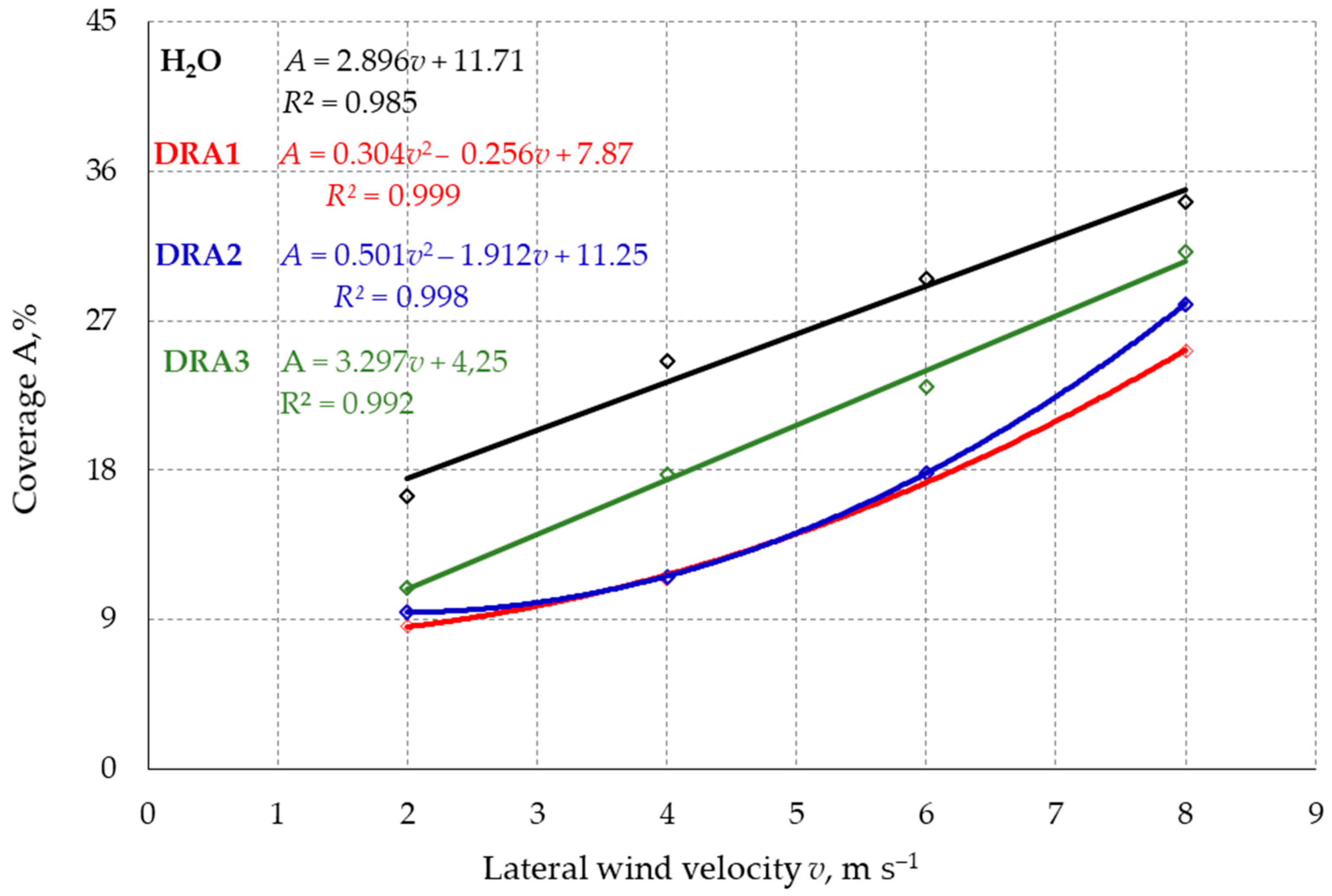
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Abbreviation | Definition |
| DRA | drift reduction agent |
| DV10 | the 10th percentile of cumulative volume distribution, µm |
| DV50 | the median for volume distribution, µm |
| DV90 | signifies the point in the size distribution, up to and including which, 90% of the total volume of material in the sample is contained, µm |
| RTK | real-time kinematic |
| VMD | volume median diameter, µm |
| WSP | water-sensitive paper |
References
- Milošević, T.; Milošević, N. Vegetative growth, productivity, berry quality attributes and leaf macronutrients content of currants as affected by species and cultivars. Erwerbs-Obstbau 2018, 60, 53–65. [Google Scholar] [CrossRef]
- Lanauskas, J.; Uselis, N.; Valiuskaite, A.; Viskelis, P. Effect of foliar and soil applied fertilizers on strawberry healthiness, yield and berry quality. Agron. Res. 2006, 4, 247–250. [Google Scholar]
- Wei, Z.; Xue, X.; Salcedo, R.; Zhang, Z.; Gil, E.; Sun, Y.; Li, Q.; Shen, J.; He, Q.; Dou, Q.; et al. Key technologies for an orchard variable-rate sprayer: Current status and future prospects. Agronomy 2022, 13, 59. [Google Scholar] [CrossRef]
- Berk, P.; Hocevar, M.; Stajnko, D.; Belsak, A. Development of alternative plant protection product application techniques in orchards, based on measurement sensing systems: A review. Comput. Electron. Agric. 2016, 124, 273–288. [Google Scholar] [CrossRef]
- Simon, S.; Bouvier, J.C.; Debras, J.F.; Sauphanor, B. Biodiversity and pest management in orchard systems. Sustain. Agric. 2011, 2, 693–709. [Google Scholar] [CrossRef]
- Ciucu Paraschiv, M.; Hoza, D. Impact of foliar fertilization on the quality parameters of blueberry fruits. Sci. Pap. Ser. B Hortic. 2022, 66, 48–57. [Google Scholar]
- Carvalho, F.P. Pesticides, environment, and food safety. Food Energy Secur. 2017, 6, 48–60. [Google Scholar] [CrossRef]
- Pathak, V.M.; Verma, V.K.; Rawat, B.S.; Kaur, B.; Babu, N.; Sharma, A.; Dewali, S.; Yadav, M.; Kumari, R.; Singh, S.; et al. Current status of pesticide effects on environment, human health and it’s eco-friendly management as bioremediation: A comprehensive review. Front. Microbiol. 2022, 13, 962619. [Google Scholar] [CrossRef]
- Tudi, M.; Daniel Ruan, H.; Wang, L.; Lyu, J.; Sadler, R.; Connell, D.; Chu, C.; Phung, D.T. Agriculture development, pesticide application and its impact on the environment. Int. J. Environ. Res. Public Health 2021, 18, 1112. [Google Scholar] [CrossRef]
- Nicolopoulou-Stamati, P.; Maipas, S.; Kotampasi, C.; Stamatis, P.; Hens, L. Chemical pesticides and human health: The urgent need for a new concept in agriculture. Front. Public Health 2016, 4, 148. [Google Scholar] [CrossRef]
- Zhang, G.; Olsson, R.L.; Hopkins, B.K. Strategies and techniques to mitigate the negative impacts of pesticide exposure to honey bees. Environ. Pollut. 2023, 318, 120915. [Google Scholar] [CrossRef] [PubMed]
- Yarpuz-Bozdogan, N. The importance of personal protective equipment in pesticide applications in agriculture. Curr. Opin. Environ. Sci. Health 2018, 4, 1–4. [Google Scholar] [CrossRef]
- Indu; Baghel, A.S.; Bhardwaj, A.; Ibrahim, W. Optimization of pesticides spray on crops in agriculture using machine learning. Comput. Intell. Neurosci. 2022, 2022, 9408535. [Google Scholar] [CrossRef] [PubMed]
- Dhananjayan, V.; Jayakumar, S.; Ravichandran, B. Conventional methods of pesticide application in agricultural field and fate of the pesticides in the environment and human health. In Controlled Release of Pesticides for Sustainable Agriculture; Springer: Cham, Switzerland, 2020; pp. 1–39. [Google Scholar] [CrossRef]
- Wołejko, E.; Łozowicka, B.; Kaczyński, P. Pesticide residues in berries fruits and juices and the potential risk for consumers. Desalination Water Treat. 2014, 52, 3804–3818. [Google Scholar] [CrossRef]
- González- Núñez, M.; Sandín-España, P.; Mateos-Miranda, M.; Cobos, G.; De Cal, A.; Sánchez-Ramos, I.; Alonso-Prados, J.L.; Larena, I. Development of a disease and pest management program to reduce the use of pesticides in sweet-cherry orchards. Agronomy 2022, 12, 1986. [Google Scholar] [CrossRef]
- Giles, D.K.; Klassen, P.; Niederholzer, F.J.; Downey, D. “Smart” sprayer technology provides environmental and economic benefits in California orchards. Calif. Agric. 2011, 65, 85–89. [Google Scholar] [CrossRef]
- Arvidsson, T.; Bergström, L.; Kreuger, J. Spray drift as influenced by meteorological and technical factors. Pest Manag. Sci. 2011, 67, 586–598. [Google Scholar] [CrossRef]
- Alves, G.S.; Vieira, B.C.; Butts, T.R.; Silva, S.M.; da Cunha, J.P.A.; Kruger, G.R. Drift potential from glyphosate and 2,4-D applications as influenced by nozzle type, adjuvant, and airspeed. Appl. Eng. Agric. 2020, 36, 687–696. [Google Scholar] [CrossRef]
- De Schampheleire, M.; Nuyttens, D.; Baetens, K.; Cornelis, W.; Gabriels, D.; Spanoghe, P. Effects on pesticide spray drift of the physicochemical properties of the spray liquid. Precis. Agric. 2009, 10, 409–420. [Google Scholar] [CrossRef]
- Bretthauer, S. Spray drift management. Outlooks Pest Manag. 2011, 22, 262–267. [Google Scholar] [CrossRef]
- Cunha, M.; Carvalho, C.; Marcal, A.R. Assessing the ability of image processing software to analyse spray quality on water-sensitive papers used as artificial targets. Biosyst. Eng. 2012, 111, 11–23. [Google Scholar] [CrossRef]
- Jensen, P.K.; Jørgensen, L.N.; Kirknel, E. Biological efficacy of herbicides and fungicides applied with low-drift and twin-fluid nozzles. Crop Prot. 2001, 20, 57–64. [Google Scholar] [CrossRef]
- Makhnenko, I.; Alonzi, E.R.; Fredericks, S.A.; Colby, C.M.; Dutcher, C.S. A review of liquid sheet breakup: Perspectives from agricultural sprays. J. Aerosol Sci. 2021, 157, 105805. [Google Scholar] [CrossRef]
- Ravier, I.; Haouisee, E.; Clément, M.; Seux, R.; Briand, O. Field experiments for the evaluation of pesticide spray-drift on arable crops. Pest Manag. Sci. Former. Pestic. Sci. 2005, 61, 728–736. [Google Scholar] [CrossRef]
- Felsot, A.S.; Unsworth, J.B.; Linders, J.B.; Roberts, G.; Rautman, D.; Harris, C.; Carazo, E. Agrochemical spray drift; assessment and mitigation—A review. J. Environ. Sci. Health Part B 2010, 46, 1–23. [Google Scholar] [CrossRef]
- Katzman, D.; Zivan, O.; Dubowski, Y. Assessing the Influence of Polymer-Based Anti-Drift Adjuvants on the Photolysis, Volatilization, and Secondary Drift of Pesticides after Application. Atmosphere 2023, 14, 1627. [Google Scholar] [CrossRef]
- Jomantas, T.; Lekavičienė, K.; Steponavičius, D.; Andriušis, A.; Zaleckas, E.; Zinkevičius, R.; Popescu, C.V.; Salceanu, C.; Ignatavičius, J.; Kemzūraitė, A. The influence of newly developed spray drift reduction agents on drift mitigation by means of wind tunnel and field evaluation methods. Agriculture 2023, 13, 349. [Google Scholar] [CrossRef]
- Mullin, A.; Fine, J.D.; Reynolds, R.D.; Frazier, M.T. Toxicological risks of agrochemical spray adjuvants: Organosilicone surfactants may not be safe. Front. Public Health 2016, 4, 92. [Google Scholar] [CrossRef]
- Mesnage, R.; Antoniou, M.N. Ignoring adjuvant toxicity falsifies the safety profile of commercial pesticides. Front. Public Health 2018, 5, 361. [Google Scholar] [CrossRef]
- Green, J.M.; Beestman, G.B. Recently patented and commercialized formulation and adjuvant technology. Crop Prot. 2007, 26, 320–327. [Google Scholar] [CrossRef]
- Baseeth, S.S.; Sebree, B.R. Renewable surfactants in spray adjuvants. Lipid Technol. 2010, 22, 79–82. [Google Scholar] [CrossRef]
- İtmeç, M.; Bayat, A.; Bolat, A.; Toraman, M.C.; Soysal, A. Assessment of spray drift with various adjuvants in a wind tunnel. Agronomy 2022, 12, 2377. [Google Scholar] [CrossRef]
- Sustainable Use of Pesticides. Directive 2009/128/EC of the European Parliament and of the Council of 21 October 2009 Establishing a Framework for Community Action to Achieve the Sustainable Use of Pesticides. 2009. Available online: https://eur-lex.europa.eu/LexUriServ/LexUriServ.do?uri=OJ:L:2009:309:0071:0086:en:PDF (accessed on 4 January 2025).
- Directive 2009/127/EC of the European Parliament and of the Council of 21 October 2009 Amending Directive 2006/42/EC with Regard to Machinery for Pesticide Application. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/PDF/?uri=CELEX:32009L0127 (accessed on 8 January 2025).
- ISO 16119-3:2013(E); Agricultural and Forestry Machinery—Environmental Requirements for Sprayers—Part 3: Sprayers for Bush and Tree Crops. ISO: Geneva, Switzerland, 2013. Available online: https://www.iso.org/standard/55707.html (accessed on 10 January 2025).
- ISO 22866:2005(E); Equipment for Crop Protection—Methods for Field Measurement of Spray Drift. International Standards Organization: Geneva, Switzerland, 2005. Available online: https://www.iso.org/standard/35161.html (accessed on 14 January 2025).
- Torrent, X.; Gregorio, E.; Rosell-Polo, J.R.; Arnó, J.; Peris, M.; van de Zande, J.C.; Planas, S. Determination of spray drift and buffer zones in 3D crops using the ISO standard and new LiDAR methodologies. Sci. Total Environ. 2020, 714, 136666. [Google Scholar] [CrossRef]
- Reyes, J.F.; Correa, C.; Esquivel, W.; Ortega, R. Development and field testing of a data acquisition system to assess the quality of spraying in fruit orchards. Comput. Electron. Agric. 2012, 84, 62–67. [Google Scholar] [CrossRef]
- Doruchowski, G.; Holownicki, R.; Godyn, A.; Swiechowski, W. Calibration of orchard sprayers–the parameters and methods. In Proceedings of the Fourth European Workshop on Standardised Procedure for the Inspection of Sprayers—SPISE, Lana, Italy, 27–29 March 2012; Volume 4, pp. 140–144. [Google Scholar] [CrossRef]
- Duga, A.T.; Dekeyser, D.; Ruysen, K.; Bylemans, D.; Nuyttens, D.; Nicolai, B.M.; Verboven, P. Numerical analysis of the effects of wind and sprayer type on spray distribution in different orchard training systems. Bound.-Layer Meteorol. 2015, 157, 517–535. [Google Scholar] [CrossRef]
- Panneton, B.; Philion, V.; Chouinard, G. Spray deposition with conventional nozzles, low-drift nozzles, or permanent sprinklers for controlling apple orchard pests. Trans. ASABE 2015, 58, 607–619. [Google Scholar] [CrossRef]
- Perine, J.; Anderson, J.C.; Kruger, G.R.; Abi-Akar, F.; Overmyer, J. Effect of nozzle selection on deposition of thiamethoxam in Actara® spray drift and implications for off-field risk assessment. Sci. Total Environ. 2021, 772, 144808. [Google Scholar] [CrossRef]
- Biocca, M.; Cutini, M.; Romano, E.; Pallottino, F.; Gallo, P. Evaluation of drift-reducing nozzles for pesticide application in hazelnut (Corylus avellana L.). AgriEngineering 2021, 3, 230–239. [Google Scholar] [CrossRef]
- Boatwright, H.; Zhu, H.; Clark, A.; Schnabel, G. Evaluation of the intelligent sprayer system in peach production. Plant Dis. 2020, 104, 3207–3212. [Google Scholar] [CrossRef]
- Song, Y.; Sun, H.; Li, M.; Zhang, Q. Technology application of smart spray in agriculture: A review. Intell. Autom. Soft Comput. 2015, 21, 319–333. [Google Scholar] [CrossRef]
- Pergher, G.; Gubiani, R.; Cividino, S.R.; Dell’Antonia, D.; Lagazio, C. Assessment of spray deposition and recycling rate in the vineyard from a new type of air-assisted tunnel sprayer. Crop Prot. 2013, 45, 6–14. [Google Scholar] [CrossRef]
- Szymczyk, S.; Legutko, S.; Szulc, T.; Zawada, M.; Nijak, M.; Szychta, M. Innovative Solutions in Crop Protection Technology. J. Res. Appl. Agric. Eng. 2022, 67, 15–20. [Google Scholar] [CrossRef]
- Seol, J.; Kim, J.; Son, H.I. Field evaluations of a deep learning-based intelligent spraying robot with flow control for pear orchards. Precis. Agric. 2022, 23, 712–732. [Google Scholar] [CrossRef]
- Chang, C.-L.; Chen, H.-W. Robust Guidance and Precise Spraying of a Four-wheeled Agricultural Robot based on Deep Learning Approach. Preprints 2023, 2023101427. [Google Scholar] [CrossRef]
- Barbosa Júnior, M.R.; Santos, R.G.D.; Sales, L.D.A.; Oliveira, L.P.D. Advancements in Agricultural Ground Robots for Specialty Crops: An Overview of Innovations, Challenges, and Prospects. Plants 2024, 13, 3372. [Google Scholar] [CrossRef]
- Kang, C.; He, L.; Zhu, H. Assessment of spray patterns and efficiency of an unmanned sprayer used in planar growing systems. Precis. Agric. 2024, 25, 2271–2291. [Google Scholar] [CrossRef]
- Semenišin, M.; Steponavičius, D.; Kemzūraitė, A.; Savickas, D. Optimizing UAV Spraying for Sustainability: Different System Spray Drift Control and Adjuvants Performance. Sustainability 2025, 17, 2083. [Google Scholar] [CrossRef]
- Jomantas, T.; Kemzūraitė, A.; Steponavičius, D.; Andriušis, A.; Dorelis, M.; Balčiūnas, J. Management measures for the mitigation of spray drift of very fine droplets sprayed by a spraying robot. Sci. Rep. 2025, in press. [Google Scholar]
- Wang, C.; Herbst, A.; Zeng, A.; Wongsuk, S.; Qiao, B.; Qi, P.; Bonds, J.; Overbeck, V.; Yang, Y.; Gao, W.; et al. Assessment of spray deposition, drift and mass balance from unmanned aerial vehicle sprayer using an artificial vineyard. Sci. Total Environ. 2021, 777, 146181. [Google Scholar] [CrossRef]
- Mamatkulova, I.E.; Boyqabulova, K.I.; Tokhtasinov, A.A. Black currant (Ribes nigrum) growing technology. J. Acad. Res. Trends Educ. Sci. 2022, 39–41. [Google Scholar]
- Zhu, H.; Salyani, M.; Fox, R.D. A portable scanning system for evaluation of spray deposit distribution. Comput. Electron. Agric. 2011, 76, 38–43. [Google Scholar] [CrossRef]
- Liu, H.; Du, Z.; Shen, Y.; Du, W.; Zhang, X. Development and evaluation of an intelligent multivariable spraying robot for orchards and nurseries. Comput. Electron. Agric. 2024, 222, 109056. [Google Scholar] [CrossRef]
- Wang, G.; Han, Y.; Li, X.; Andaloro, J.; Chen, P.; Hoffmann, W.C.; Han, X.; Chen, S.; Lan, Y. Field evaluation of spray drift and environmental impact using an agricultural unmanned aerial vehicle (UAV) sprayer. Sci. Total Environ. 2020, 737, 139793. [Google Scholar] [CrossRef]





| Measurement Points | Sensor Number and Place on the Frame | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 (0.1 m) | 2 (0.23 m) | 3 (0.45 m) | 4 (0.67 m) | 5 (0.89 m) | 6 (1.11 m) | 7 (1.33 m) | 8 (1.55 m) | 9 (1.77 m) | 10 (1.99 m) | |
| I point (1 m from fan) | 2.0 ± 0.1 | 1.9 ± 0.1 | 1.6 ± 0.1 | 2.0 ± 0.1 | 2.3 ± 0.1 | 2.6 ± 0.1 | 2.9 ± 0.1 | 2.1 ± 0.1 | 2.2 ± 0.1 | 1.9 ± 0.1 |
| II point (2 m) | 1.8 ± 0.1 | 1.8 ± 0.1 | 1.9 ± 0.1 | 1.6 ± 0.1 | 1.3 ± 0.1 | 1.3 ± 0.1 | 1.1 ± 0.1 | 1.1 ± 0.1 | 1.5 ± 0.1 | 1.6 ± 0.1 |
| III point (2.5 m) | 1.0 ± 0.2 | 1.0 ± 0.2 | 1.7 ± 0.1 | 3.0 ± 0.1 | 2.6 ± 0.1 | 2.4 ± 0.1 | 1.6 ± 0.1 | 1.1 ± 0.1 | 1.6 ± 0.1 | 1.5 ± 0.1 |
| IV point (3 m) | 2.6 ± 0.1 | 2.4 ± 0.2 | 2.5 ± 0.1 | 2.0 ± 0.1 | 2.4 ± 0.1 | 2.4 ± 0.1 | 1.9 ± 0.3 | 1.3 ± 0.1 | 1.7 ± 0.1 | 1.7 ± 0.1 |
| V point (3.5 m) | 2.5 ± 0.1 | 1.8 ± 0.1 | 2.0 ± 0.1 | 1.4 ± 0.1 | 1.7 ± 0.1 | 2.1 ± 0.1 | 2.2 ± 0.1 | 1.7 ± 0.1 | 2.2 ± 0.1 | 2.2 ± 0.1 |
| VI point (4 m) | 2.2 ± 0.1 | 2.2 ± 0.1 | 1.6 ± 0.1 | 1.5 ± 0.1 | 1.7 ± 0.1 | 1.5 ± 0.1 | 1.7 ± 0.1 | 1.4 ± 0.1 | 1.6 ± 0.1 | 1.7 ± 0.1 |
| VII point (4.5 m) | 1.7 ± 0.2 | 1.5 ± 0.5 | 1.3 ± 0.2 | 1.4 ± 0.2 | 1.6 ± 0.1 | 1.9 ± 0.1 | 1.6 ± 0.1 | 2.0 ± 0.1 | 1.7 ± 0.1 | 2.0 ± 0.1 |
| VIII point (5 m) | 1.8 ± 0.1 | 1.5 ± 0.1 | 1.7 ± 0.1 | 1.2 ± 0.1 | 1.4 ± 0.1 | 1.8 ± 0.1 | 1.2 ± 0.1 | 1.5 ± 0.1 | 1.5 ± 0.1 | 1.3 ± 0.1 |
| IX point (6 m) | 1.8 ± 0.1 | 1.7 ± 0.1 | 1.9 ± 0.1 | 1.6 ± 0.1 | 1.3 ± 0.1 | 1.3 ± 0.1 | 1.1 ± 0.1 | 1.1 ± 0.1 | 1.4 ± 0.1 | 1.6 ± 0.1 |
| X point (7 m) | 1.4 ± 0.1 | 1.5 ± 0.1 | 1.2 ± 0.1 | 1.6 ± 0.2 | 1.0 ± 0.1 | 1.1 ± 0.1 | 1.2 ± 0.3 | 1.0 ± 0.1 | 1.1 ± 0.1 | 1.1 ± 0.1 |
| XI point (8 m) | 1.7 ± 0.1 | 0.9 ± 0.1 | 1.1 ± 0.2 | 1.0 ± 0.1 | 1.5 ± 0.2 | 1.8 ± 0.1 | 1.8 ± 0.1 | 1.2 ± 0.1 | 1.9 ± 0.1 | 1.9 ± 0.1 |
| XII point (9 m) | 1.3 ± 0.1 | 1.2 ± 0.1 | 1.3 ± 0.1 | 1.1 ± 0.1 | 1.2 ± 0.1 | 1.4 ± 0.1 | 1.7 ± 0.1 | 1.6 ± 0.1 | 1.8 ± 0.1 | 1.9 ± 0.1 |
| XIII point (10 m) | 1.2 ± 0.1 | 1.1 ± 0.1 | 1.2 ± 0.1 | 1.2 ± 0.1 | 1.3 ± 0.1 | 1.4 ± 0.1 | 1.5 ± 0.1 | 1.3 ± 0.1 | 1.9 ± 0.1 | 2.1 ± 0.1 |
| XIV point (11 m) | 1.5 ± 0.1 | 1.3 ± 0.1 | 1.4 ± 0.1 | 1.4 ± 0.1 | 1.6 ± 0.1 | 1.6 ± 0.1 | 1.7 ± 0.1 | 1.6 ± 0.1 | 1.6 ± 0.1 | 1.6 ± 0.1 |
| Measurement Points | Sensor Number and Place on the Frame | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 (0.1 m) | 2 (0.23 m) | 3 (0.45 m) | 4 (0.67 m) | 5 (0.89 m) | 6 (1.11 m) | 7 (1.33 m) | 8 (1.55 m) | 9 (1.77 m) | 10 (1.99 m) | |
| I point (1 m from fan) | 7.3 ± 0.1 | 7.5 ± 0.1 | 7.8 ± 0.1 | 8.1 ± 0.1 | 7.8 ± 0.1 | 7.4 ± 0.1 | 7.7 ± 0.4 | 7.5 ± 0.2 | 6.9 ± 0.2 | 6.8 ± 0.3 |
| II point (2 m) | 7.3 ± 0.2 | 8.0 ± 0.3 | 8.0 ± 0.3 | 7.9 ± 0.2 | 8.1 ± 0.3 | 7.8 ± 0.3 | 8.3 ± 0.2 | 7.7 ± 0.2 | 7.3 ± 0.2 | 8.0 ± 0.1 |
| III point (2.5 m) | 6.8 ± 0.5 | 7.1 ± 0.1 | 7.5 ± 0.1 | 7.2 ± 0.1 | 7.0 ± 0.1 | 6.7 ± 0.1 | 7.3 ± 0.3 | 6.9 ± 0.3 | 6.6 ± 0.2 | 7.0 ± 0.2 |
| IV point (3 m) | 6.1 ± 0.1 | 7.0 ± 0.1 | 7.6 ± 0.1 | 8.1 ± 0.1 | 8.1 ± 0.1 | 7.8 ± 0.2 | 7.3 ± 0.2 | 7.2 ± 0.4 | 7.1 ± 0.3 | 7.4 ± 0.1 |
| V point (3.5 m) | 6.7 ± 0.1 | 7.0 ± 0.1 | 7.1 ± 0.1 | 7.4 ± 0.1 | 7.2 ± 0.1 | 7.2 ± 0.1 | 7.3 ± 0.1 | 7.4 ± 0.3 | 7.5 ± 0.2 | 7.4 ± 0.2 |
| VI point (4 m) | 6.3 ± 0.3 | 6.6 ± 0.2 | 7.0 ± 0.1 | 7.5 ± 0.1 | 7.6 ± 0.1 | 7.6 ± 0.1 | 7.5 ± 0.1 | 7.1 ± 0.2 | 6.7 ± 0.5 | 6.5 ± 0.1 |
| VII point (4.5 m) | 5.6 ± 0.1 | 6.2 ± 0.1 | 6.5 ± 0.1 | 6.4 ± 0.3 | 7.2 ± 0.2 | 7.1 ± 0.1 | 6.8 ± 0.1 | 6.9 ± 0.1 | 6.5 ± 0.1 | 6.3 ± 0.1 |
| VIII point (5 m) | 5.2 ± 0.2 | 6.0 ± 0.2 | 7.0 ± 0.2 | 7.2 ± 0.2 | 6.3 ± 0.1 | 6.1 ± 0.1 | 5.6 ± 0.2 | 5.5 ± 0.2 | 5.3 ± 0.1 | 5.2 ± 0.1 |
| IX point (6 m) | 5.3 ± 0.2 | 5.4 ± 0.2 | 6.2 ± 0.1 | 6.3 ± 0.1 | 7.1 ± 0.2 | 7.4 ± 0.2 | 6.2 ± 0.1 | 6.7 ± 0.2 | 7.0 ± 0.2 | 5.6 ± 0.2 |
| X point (7 m) | 5.3 ± 0.5 | 5.5 ± 0.2 | 5.8 ± 0.1 | 5.7 ± 0.3 | 6.9 ± 0.3 | 6.2 ± 0.3 | 5.7 ± 0.4 | 5.2 ± 0.4 | 5.1 ± 0.3 | 4.7 ± 0.2 |
| XI point (8 m) | 4.9 ± 0.1 | 5.0 ± 0.2 | 5.7 ± 0.2 | 5.6 ± 0.2 | 5.8 ± 0.2 | 5.2 ± 0.3 | 4.9 ± 0.1 | 4.7 ± 0.2 | 4.6 ± 0.1 | 4.4 ± 0.1 |
| XII point (9 m) | 4.5 ± 0.2 | 4.7 ± 0.2 | 4.4 ± 0.1 | 4.8 ± 0.2 | 5.2 ± 0.1 | 5.2 ± 0.3 | 4.7 ± 0.1 | 4.2 ± 0.1 | 4.4 ± 0.1 | 4.3 ± 0.1 |
| XIII point (10 m) | 3.3 ± 0.1 | 3.3 ± 0.1 | 3.3 ± 0.1 | 3.2 ± 0.1 | 3.6 ± 0.2 | 3.7 ± 0.1 | 3.3 ± 0.1 | 3.2 ± 0.2 | 3.1 ± 0.1 | 2.9 ± 0.1 |
| XIV point (11 m) | 4.4 ± 0.3 | 4.3 ± 0.3 | 4.6 ± 0.2 | 4.8 ± 0.3 | 5.3 ± 0.6 | 5.3 ± 0.5 | 5.3 ± 0.4 | 5.2 ± 0.3 | 3.7 ± 0.4 | 4.9 ± 0.6 |
| Main Parameters to Be Used and Analyzed | Description |
|---|---|
| Types of sprayed liquid | Water, DRA1, DRA2, DRA3 |
| Artificial plants | Six rows of two artificial plants each (1.2 m heights) |
| Lateral wind velocity, m s−1 | 0, 2, 4, 6, and 8 |
| Spray drift coverage, % | On WSP at different distance from the spray zone (3, 6, 9, 12, and 15 m) and two heights on the artificial plant (0.5 m and 1 m) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jomantas, T.; Kemzūraitė, A.; Savickas, D.; Grigas, A.; Steponavičius, D. The Influence of Lateral Wind Velocity on Spray Drift Dynamics of Liquid Droplets Sprayed by Agricultural Robot. Appl. Sci. 2025, 15, 4860. https://doi.org/10.3390/app15094860
Jomantas T, Kemzūraitė A, Savickas D, Grigas A, Steponavičius D. The Influence of Lateral Wind Velocity on Spray Drift Dynamics of Liquid Droplets Sprayed by Agricultural Robot. Applied Sciences. 2025; 15(9):4860. https://doi.org/10.3390/app15094860
Chicago/Turabian StyleJomantas, Tadas, Aurelija Kemzūraitė, Dainius Savickas, Andrius Grigas, and Dainius Steponavičius. 2025. "The Influence of Lateral Wind Velocity on Spray Drift Dynamics of Liquid Droplets Sprayed by Agricultural Robot" Applied Sciences 15, no. 9: 4860. https://doi.org/10.3390/app15094860
APA StyleJomantas, T., Kemzūraitė, A., Savickas, D., Grigas, A., & Steponavičius, D. (2025). The Influence of Lateral Wind Velocity on Spray Drift Dynamics of Liquid Droplets Sprayed by Agricultural Robot. Applied Sciences, 15(9), 4860. https://doi.org/10.3390/app15094860








