Modeling Power Generation and Energy Efficiencies in Air-Cathode Microbial Fuel Cells Based on Freter Equations
Abstract
:Featured Application
Abstract
1. Introduction
2. Materials and Methods
2.1. MFC Design and Operation
Data Acquisition
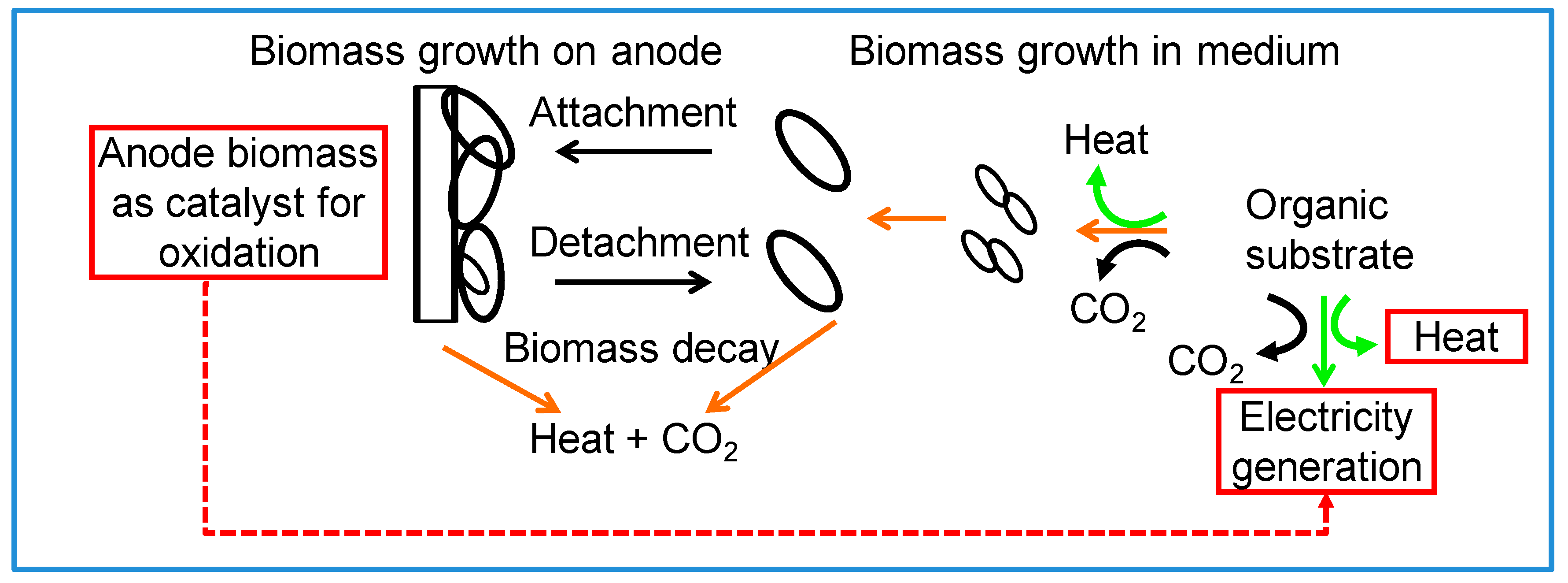
2.2. Model Description
2.2.1. Electrode Potentials
2.2.2. Exchange Current and Overpotential
2.2.3. Mass Balances
2.2.4. Ohm’s Law and Kirchhoff’s Voltage Law
2.2.5. Power and Energy Efficiencies
2.2.6. Model Parameters
3. Results and Discussion
3.1. Parameter Estimation
3.2. Simulation of Batch Mode MFC
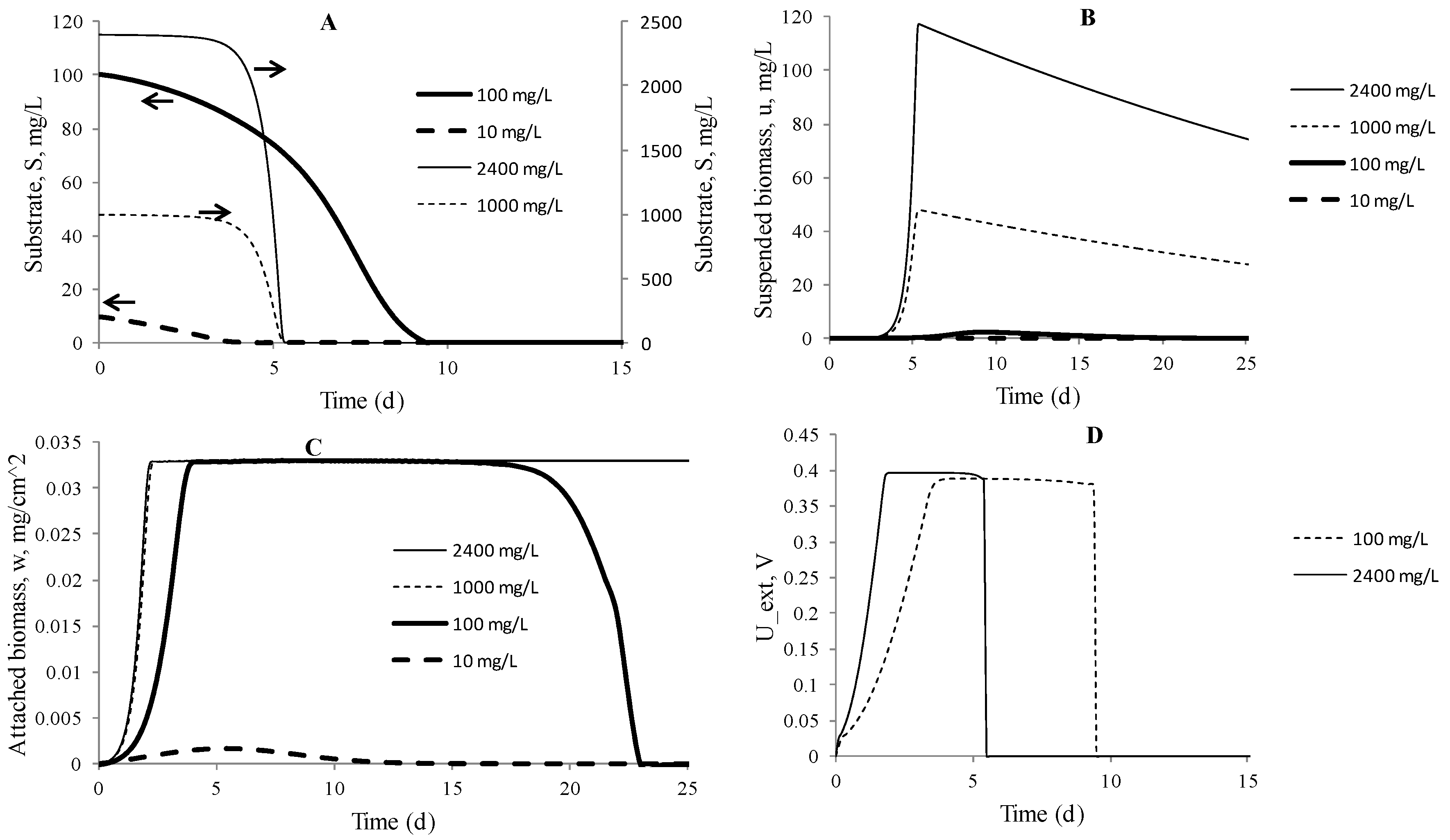
3.2.1. Effect of Initial Substrate Concentration
3.2.2. Effect of External Resistor
3.3. Simulation of Continuous Mode MFC
3.3.1. Effect of Influent Substrate Concentration and Dilution Rate
3.3.2. Effect of External Resistor
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Logan, B.E. Exoelectrogenic bacteria that power microbial fuel cells. Nat. Rev. Microbiol. 2009, 7, 375–381. [Google Scholar] [CrossRef] [PubMed]
- Ren, Z.; Ward, T.E.; Regan, J.M. Electricity production from cellulose in a microbial fuel cell using a defined binary culture. Environ. Sci. Technol. 2007, 41, 4781–4786. [Google Scholar] [CrossRef] [PubMed]
- Rezaei, F.; Xing, D.; Wagner, R.; Regan, J.M.; Richard, T.L.; Logan, B.E. Simultaneous cellulose degradation and electricity production by Enterobacter cloacae in a microbial fuel cell. Appl. Environ. Microb. 2009, 75, 3673–3678. [Google Scholar] [CrossRef] [PubMed]
- Picioreanu, C.; Head, I.M.; Katuri, K.P.; van Loosdrecht, M.C.M.; Scott, K. A computational model for biofilm-based microbial fuel cells. Water Res. 2007, 41, 2921–2940. [Google Scholar] [CrossRef] [PubMed]
- Hamelers, H.V.M.; Ter Heijne, A.; Stein, N.; Rozendal, R.A.; Buisman, C.J.N. Butler-Volmer-Monod model for describing bio-anode polarization curves. Bioresour. Technol. 2011, 102, 381–387. [Google Scholar] [CrossRef] [PubMed]
- Ren, Z.; Ramasamy, R.P.; Cloud-Owen, S.R.; Yan, H.; Mench, M.M.; Regan, J.M. Time-course correlation of biofilm properties and electrochemical performance in single-chamber microbial fuel cells. Bioresour. Technol. 2011, 102, 416–421. [Google Scholar] [CrossRef] [PubMed]
- Bond, D.R.; Lovley, D.R. Electricity production by Geobacter sulfurreducens attached to electrodes. Appl. Environ. Microb. 2003, 69, 1548–1555. [Google Scholar] [CrossRef]
- Freter, R.; Brickner, H.; Fekete, J.; Vickerman, M.M.; Carey, K.E. Survival and implantation of Escherichia coli in the intestinal tract. Infect. Immun. 1983, 39, 686–703. [Google Scholar] [PubMed]
- Mašić, A.; Eberl, H.J. Persistence in a Single Species CSTR Model with Suspended Flocs and Wall Attached Biofilms. Bull. Math. Boil. 2012, 74, 1001–1026. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Logan, B.E. Electricity generation using an air-cathode single chamber microbial fuel cell in the presence and absence of a proton exchange membrane. Environ. Sci. Technol. 2004, 38, 4040–4046. [Google Scholar] [CrossRef] [PubMed]
- Schecher, W.; McAvoy, D. MINEQL+ Version 4.5; Environmental Research Software: Hallowell, ME, USA, 1998. [Google Scholar]
- Logan, B.E. Microbial Fuel Cells, 1st ed.; Wiley-Interscience: Hoboken, NJ, USA, 2008. [Google Scholar]
- Schröder, U. Anodic electron transfer mechanisms in microbial fuel cells and their energy efficiency. Phys. Chem. Chem. Phys. 2007, 9, 2619–2629. [Google Scholar] [CrossRef] [PubMed]
- Zeng, Y.; Choo, Y.F.; Kim, B.H.; Wu, P. Modelling and simulation of two-chamber microbial fuel cell. J. Power Sources 2010, 195, 79–89. [Google Scholar] [CrossRef]
- Neyerlin, K.; Gu, W.; Jorne, J.; Gasteiger, H.A. Study of the exchange current density for the hydrogen oxidation and evolution reactions. J. Electrochem. Soc. 2007, 154, B631–B635. [Google Scholar] [CrossRef]
- Batstone, D.J.; Keller, J.; Angelidaki, I.; Kalyuzhnyi, S.; Pavlostathis, S.; Rozzi, A.; Sanders, W.; Siegrist, H.; Vavilin, V. The IWA Anaerobic Digestion Model No 1(ADM 1). Water Sci. Technol. 2002, 45, 65–73. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Lowy, D.; Baumann, R.; Tender, L. Influence of anode pretreatment on its microbial colonization. J. Appl. Microbiol. 2007, 102, 177–183. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Freguia, S.; Rabaey, K.; Yuan, Z.; Keller, J. Non-catalyzed cathodic oxygen reduction at graphite granules in microbial fuel cells. Electrochim. Acta 2007, 53, 598–603. [Google Scholar] [CrossRef]
- Freguia, S.; Rabaey, K.; Yuan, Z.; Keller, J. Sequential anode-cathode configuration improves cathodic oxygen reduction and effluent quality of microbial fuel cells. Water Res. 2008, 42, 1387–1396. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, B.; Han, J.-I. A single chamber stackable microbial fuel cell with air cathode. Biotechnol. Lett. 2009, 31, 387–393. [Google Scholar] [CrossRef] [PubMed]
- Fan, Y.; Sharbrough, E.; Liu, H. Quantification of the internal resistance distribution of microbial fuel cells. Environ. Sci. Technol. 2008, 42, 8101–8107. [Google Scholar] [CrossRef] [PubMed]
- Jia, Y.H.; Tran, H.T.; Kim, D.H.; Oh, S.J.; Park, D.H.; Zhang, R.H.; Ahn, D.H. Simultaneous organics removal and bio-electrochemical denitrification in microbial fuel cells. Bioprocess Biosyst. Eng. 2008, 31, 315–321. [Google Scholar] [CrossRef] [PubMed]
- Katuri, K.P.; Scott, K.; Head, I.M.; Picioreanu, C.; Curtis, T.P. Microbial fuel cells meet with external resistance. Bioresour. Technol. 2011, 102, 2758–2766. [Google Scholar] [CrossRef] [PubMed]
- Mench, M.M. Fuel Cell Engines; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Wu, X.; Yao, W.; Zhu, J. Effect of pH on continuous biohydrogen production from liquid swine manure with glucose supplement using an anaerobic sequencing batch reactor. Int. J. Hydrogen Energy 2010, 35, 6592–6599. [Google Scholar] [CrossRef]
- Jung, S.; Regan, J.M. Comparison of anode bacterial communities and performance in microbial fuel cells with different electron donors. Appl. Microbiol. Biotechnol. 2007, 77, 393–402. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Cheng, S.; Logan, B.E. Power generation in fed-batch microbial fuel cells as a function of ionic strength, temperature, and reactor configuration. Environ. Sci. Technol. 2005, 39, 5488–5493. [Google Scholar] [CrossRef] [PubMed]
- He, Z.; Wagner, N.; Minteer, S.D.; Angenent, L.T. An upflow microbial fuel cell with an interior cathode: Assessment of the internal resistance by impedance spectroscopy. Environ. Sci. Technol. 2006, 40, 5212–5217. [Google Scholar] [CrossRef] [PubMed]





| Parameter Symbol | Description | Value | Units |
|---|---|---|---|
| Physical | |||
| F | Faraday’s constant | 96,485 | C/mol |
| R | Ideal gas constant | 8.314 | J/mol/K |
| T | Room temperature | 298 | K |
| ΔHc | Heat of combustion of acetic acid | −875,000 | J/mol |
| M | Relative molecular weight of acetic acid | 60 | g/mol |
| Electrochemical | |||
| EA0’ | Formal reduction potential of anode | −0.335 | V vs. NHE |
| EC0’ | Formal reduction potential of cathode | 0.51 | V vs. NHE |
| Reactor configuration | |||
| A | Projected surface area of electrode | 0.002 | m2 |
| d | Distance between anode and cathode | 0.075 | m |
| V | Volume of MFC reactor | 1.55 × 10−4 | m3 |
| δ | Ratio of anode surface area to MFC volume | 13 | m2/m3 |
| Rc | Contact resistance of MFC | 20 | Ω |
| Operating | |||
| D | Dilution rate | 0–2 | d−1 |
| Sin | Substrate concentration | 10–2400 | g/m3 |
| Q | Flow rate | 0–7.8 × 10−5 | m3/d |
| σ | Conductivity of MFC medium | 1.1 | S/m |
| Rext | External resistor | 2200 | Ω |
| [HCO3−] | Bicarbonate concentration | 1 × 10−4.5 | kmol/m3 |
| PO2 | Partial oxygen pressure at cathode | 0.2 | atm |
| pH | −log10 of proton concentration | 7 | Dimensionless |
| Microbial | |||
| γ | Biomass yield from substrate | 0.05 | g/g |
| ku | Death rate of suspended bacteria | 0.02 | d−1 |
| kw | Death rate of attached bacteria | 0.02 | d−1 |
| α | Bacterial attachment rate | 0.05 | d−1 |
| β | Bacterial detachment rate | 0.05 | d−1 |
| mu | Maximum specific growth rate of suspended bacteria | 2.4 | d−1 |
| mu | Maximum specific growth rate of attached bacteria | 2.4 | d−1 |
| au | Half saturation coefficient of suspended bacteria | 100 | g/m3 |
| aw | Half saturation coefficient of attached bacteria | 100 | g/m3 |
| wmax | Maximum bacterial attachment | 0.33 a | g/m2 |
| Estimated Parameters | Description | Value | Units |
|---|---|---|---|
| αA | Charge transfer coefficient, anode | 0.318 | Dimensionless |
| αC | Charge transfer coefficient, cathode | 0.694 | Dimensionless |
| joA | Exchange current density, anode | 62.5 | mA/m2 |
| joAU | Unit exchange current, anode | 0.189 | mA/mg |
| joC | Exchange current density, cathode | 0.975 | mA/m2 |
| External Resistor | Peak Power | Energy Recovered | ηoverall |
|---|---|---|---|
| 10 Ω | 0.0142 mW | 1.96 J | 0.87% |
| 300 Ω | 0.0998 mW | 37.1 J | 16.4% |
| 1000 Ω | 0.0882 mW | 47.3 J | 20.7% |
| 2200 Ω | 0.0685 mW | 40.0 J | 17.7% |
| Sin | D | Steady State S | S Removal | Pext, max | ηoverall, max | Rina | Rext, Pb | Rext, ηc |
|---|---|---|---|---|---|---|---|---|
| mg/L | d−1 | mg/L | % | mW | Ω | Ω | Ω | |
| 100 | 0.1 | 0–5.26 | >94.7 | 0.091 | 0.322 | 471 | 1007 | 928 |
| 1 | 57.6–73.5 | 26.5–42.4 | 0.106 | 0.105 | 352 | 405 | ||
| 2 | 79–100 | <21.0 | 0.106 | 0.156 | 352 | 1017 | ||
| 2400 | 0.1 | 5.17–5.26 | 100 | 0.106 | 0.0167 | 352 | 352 | |
| 1 | 73.9 | 96.9 | 0.106 | 0.0017 | 352 | 352 | ||
| 2 | 531 | 77.9 | 0.106 | 0.0011 | 352 | 352 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.; Wu, S.; Zhu, J. Modeling Power Generation and Energy Efficiencies in Air-Cathode Microbial Fuel Cells Based on Freter Equations. Appl. Sci. 2018, 8, 1983. https://doi.org/10.3390/app8101983
Lin H, Wu S, Zhu J. Modeling Power Generation and Energy Efficiencies in Air-Cathode Microbial Fuel Cells Based on Freter Equations. Applied Sciences. 2018; 8(10):1983. https://doi.org/10.3390/app8101983
Chicago/Turabian StyleLin, Hongjian, Sarah (Xiao) Wu, and Jun Zhu. 2018. "Modeling Power Generation and Energy Efficiencies in Air-Cathode Microbial Fuel Cells Based on Freter Equations" Applied Sciences 8, no. 10: 1983. https://doi.org/10.3390/app8101983
APA StyleLin, H., Wu, S., & Zhu, J. (2018). Modeling Power Generation and Energy Efficiencies in Air-Cathode Microbial Fuel Cells Based on Freter Equations. Applied Sciences, 8(10), 1983. https://doi.org/10.3390/app8101983





