Experimental Study of the Behavior of Rectangular Excavations Supported by a Pipe Roof
Abstract
1. Introduction
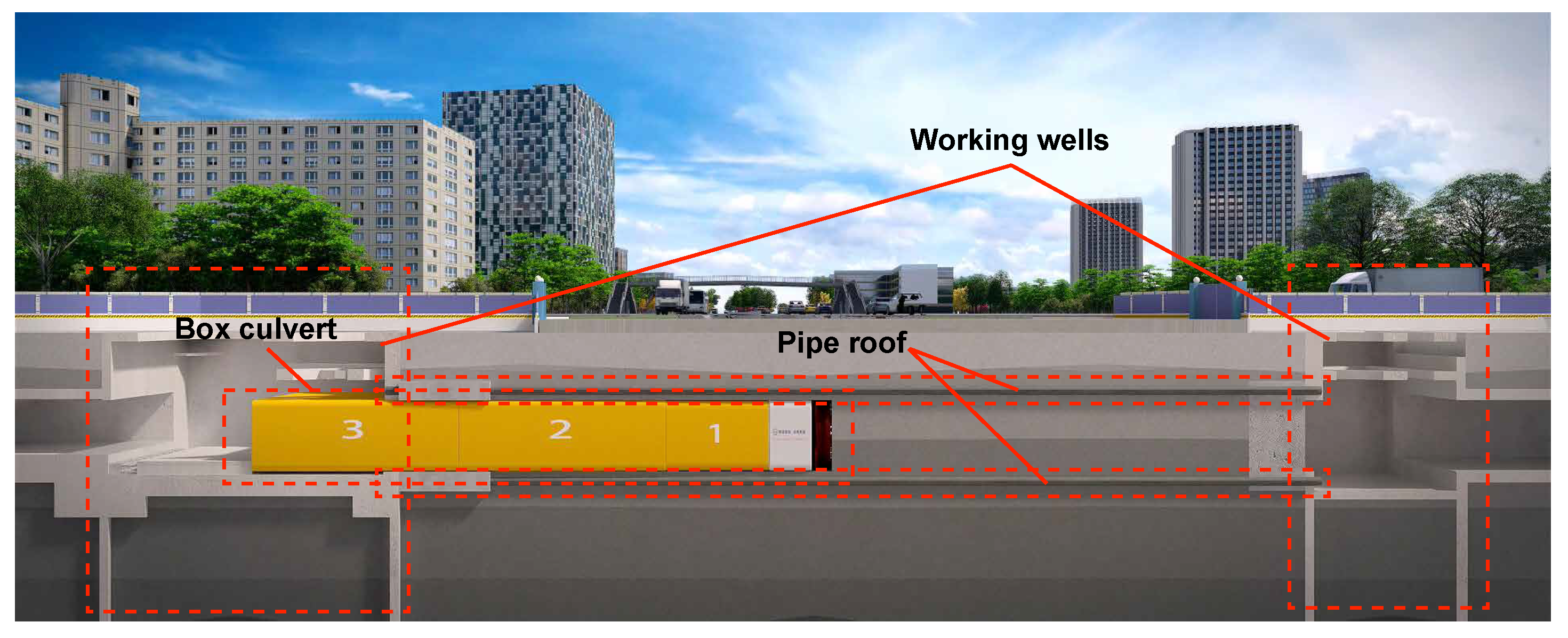
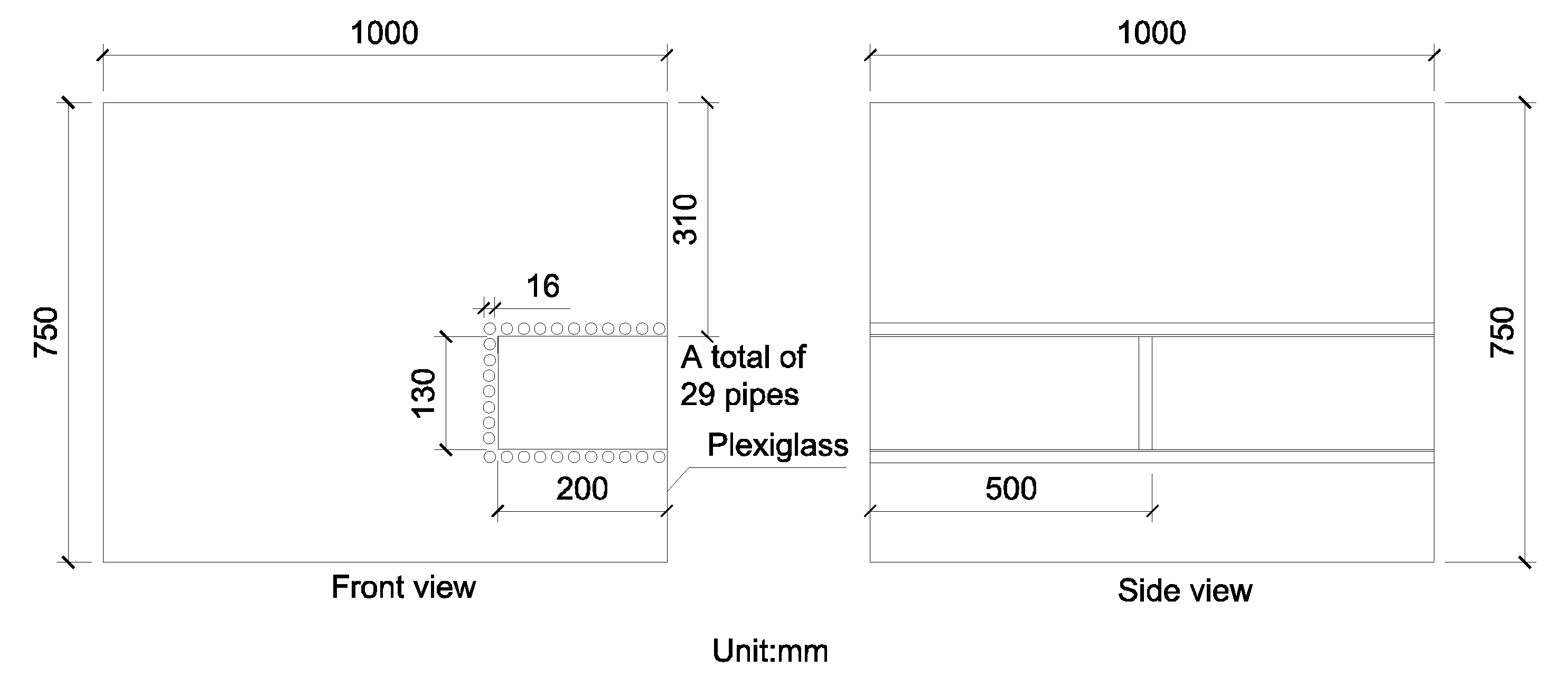
2. Experimental
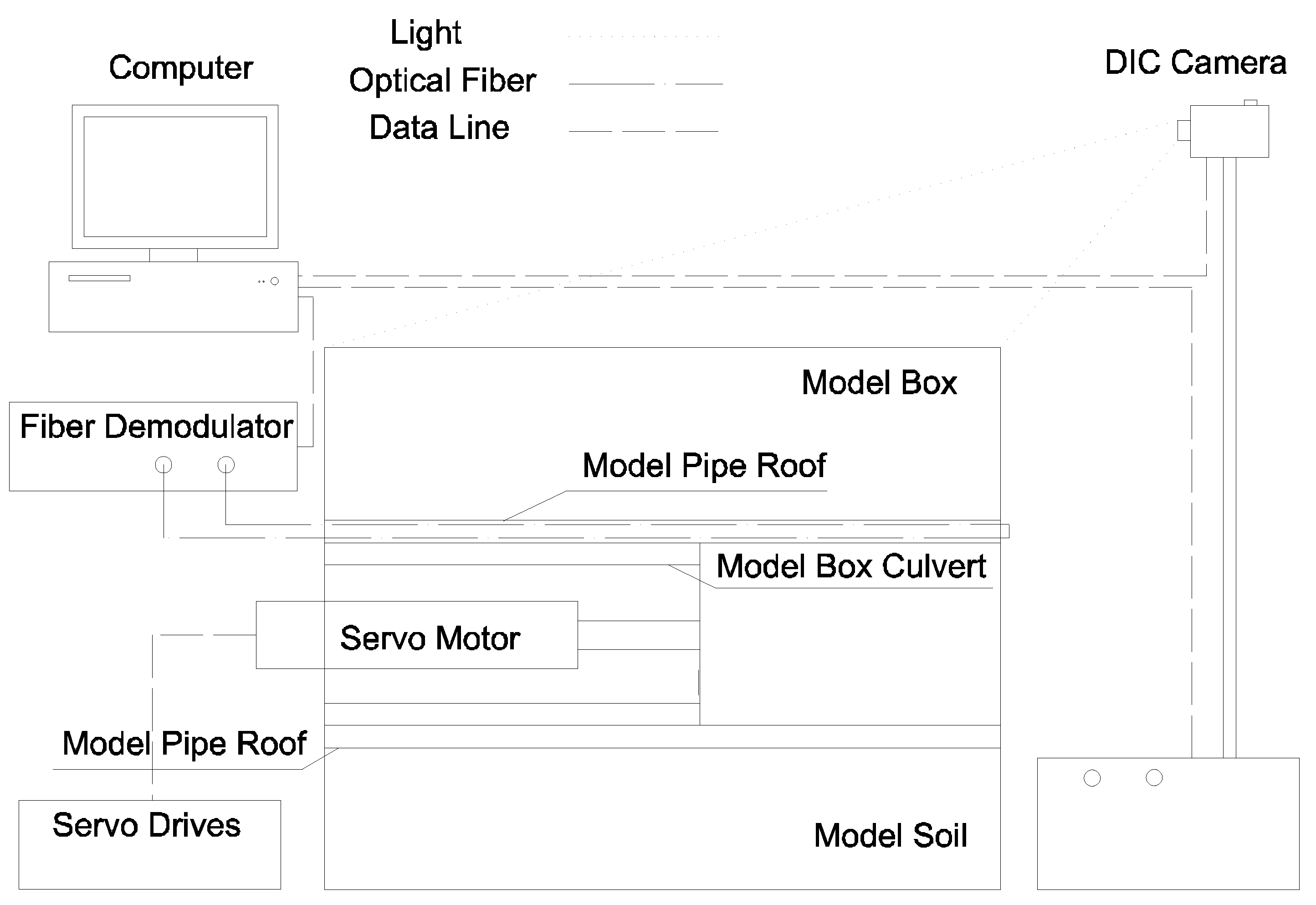
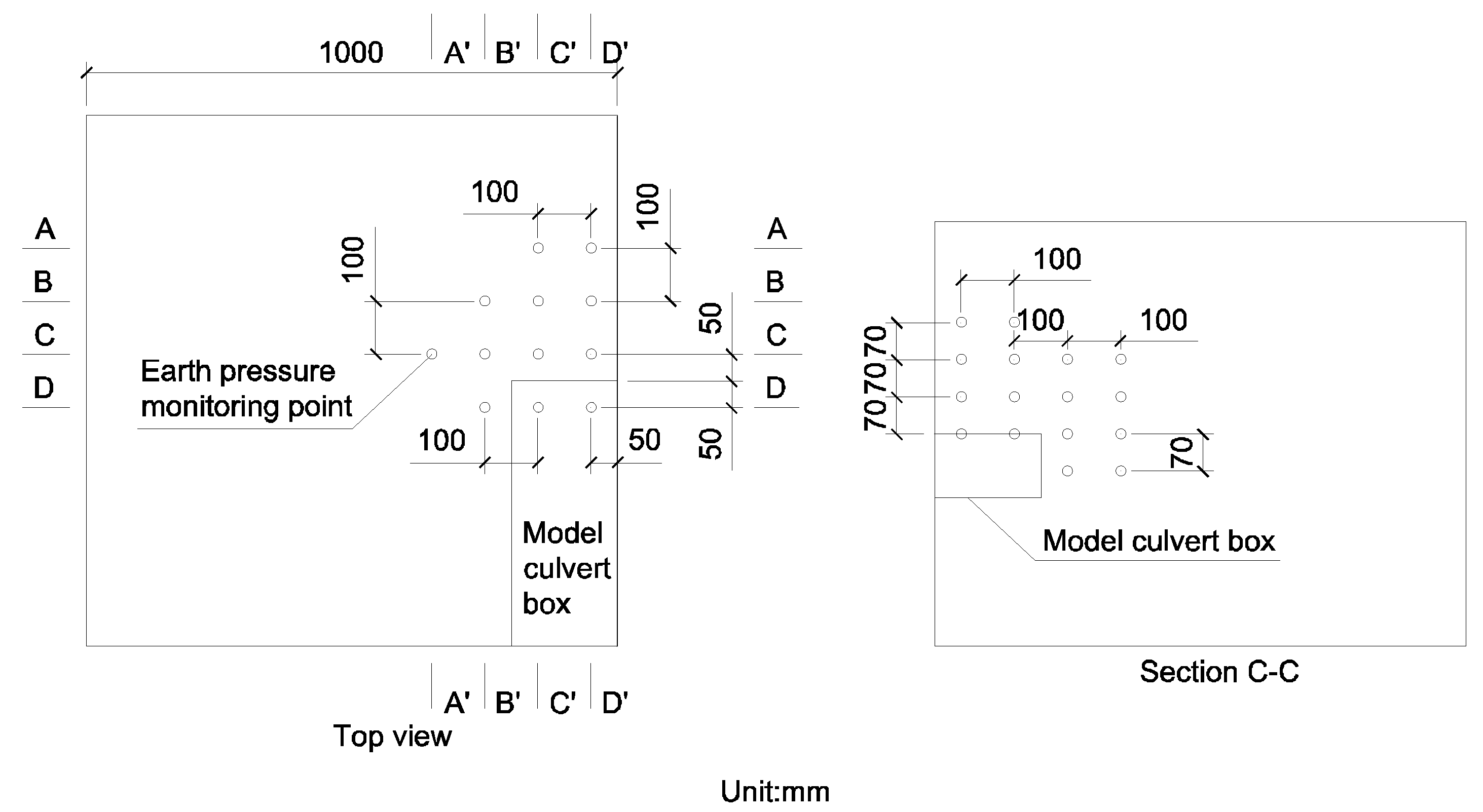
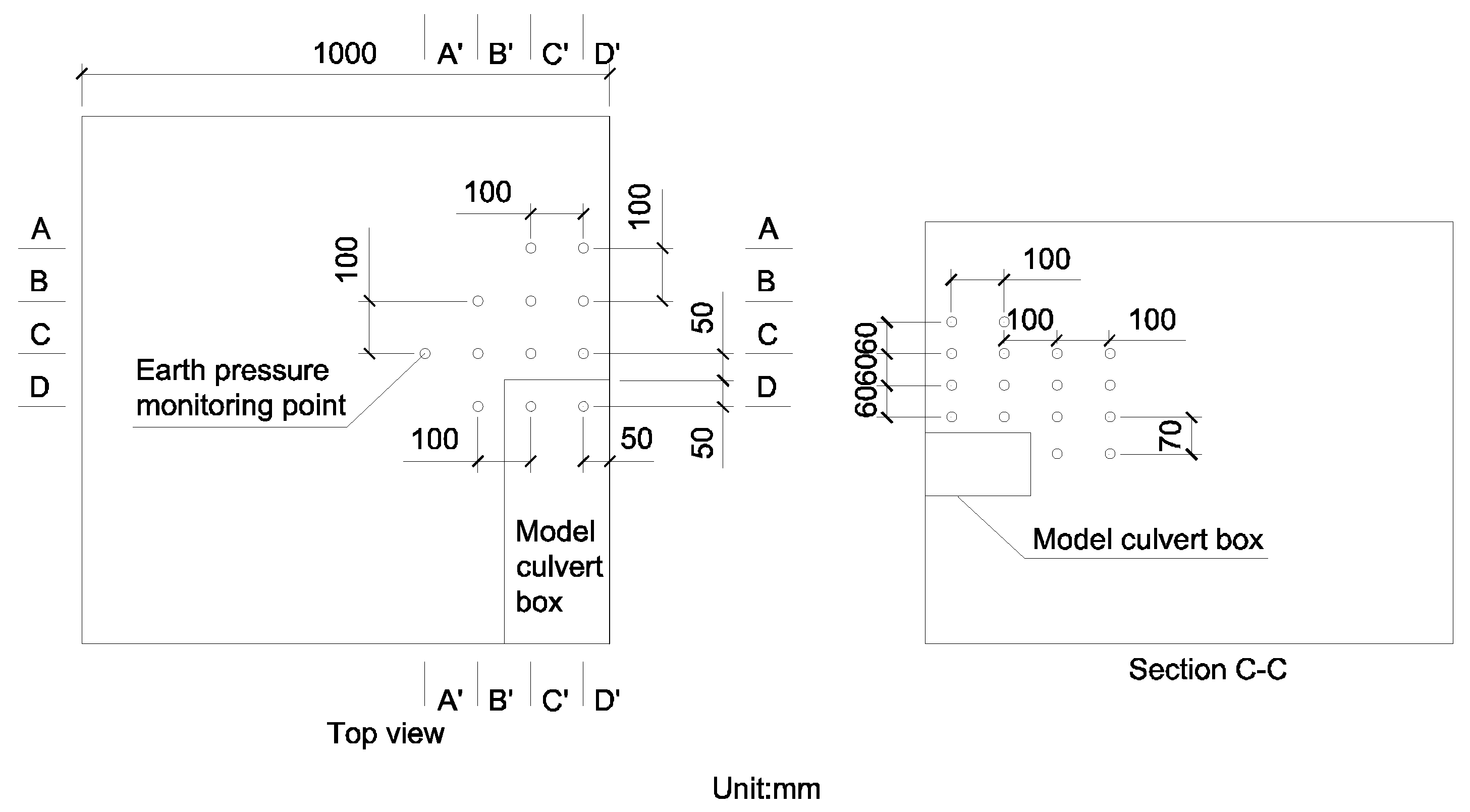
2.1. Monitoring System
2.2. Experiment Program
3. Results and Analysis
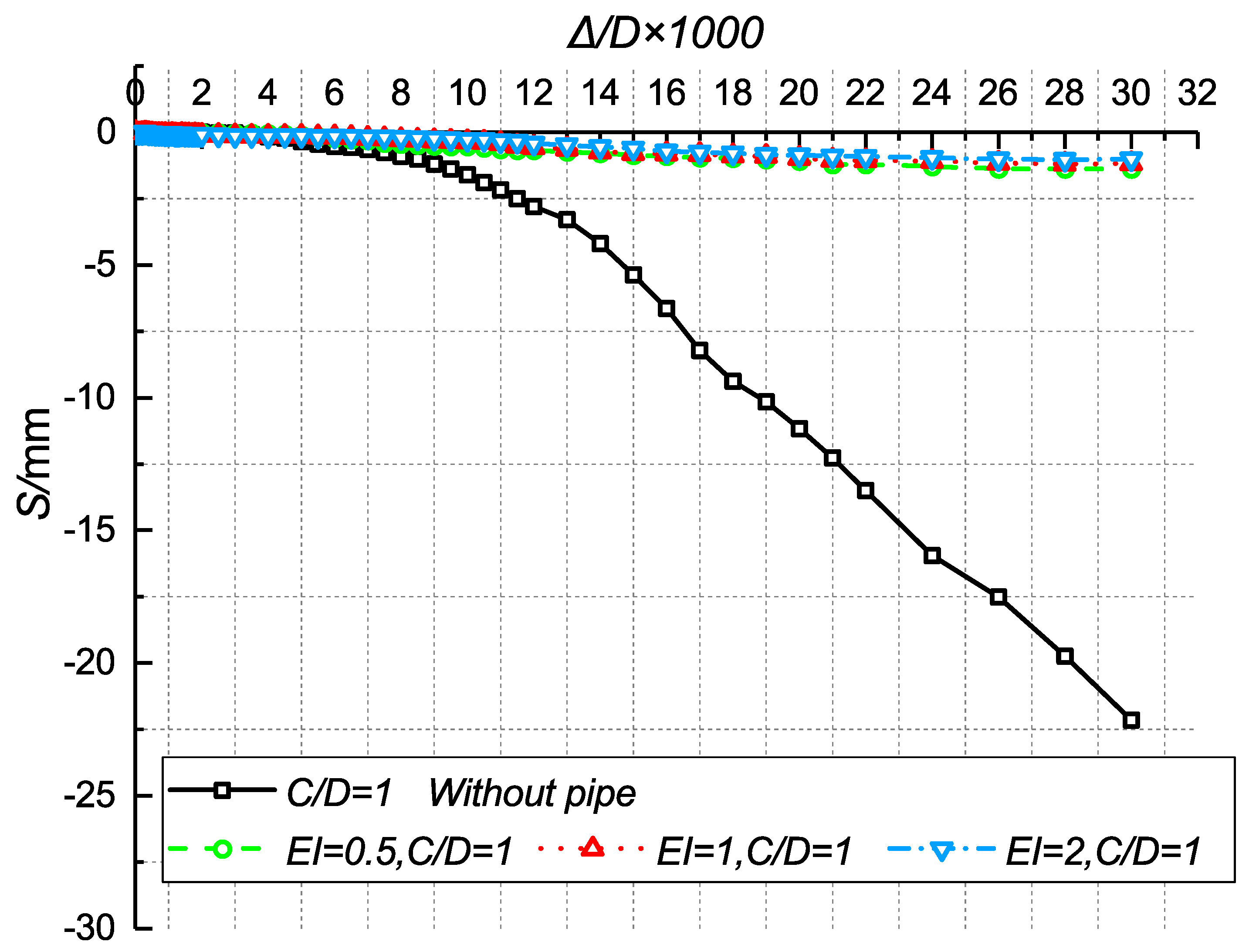
3.1. Excavation Face Support Pressure
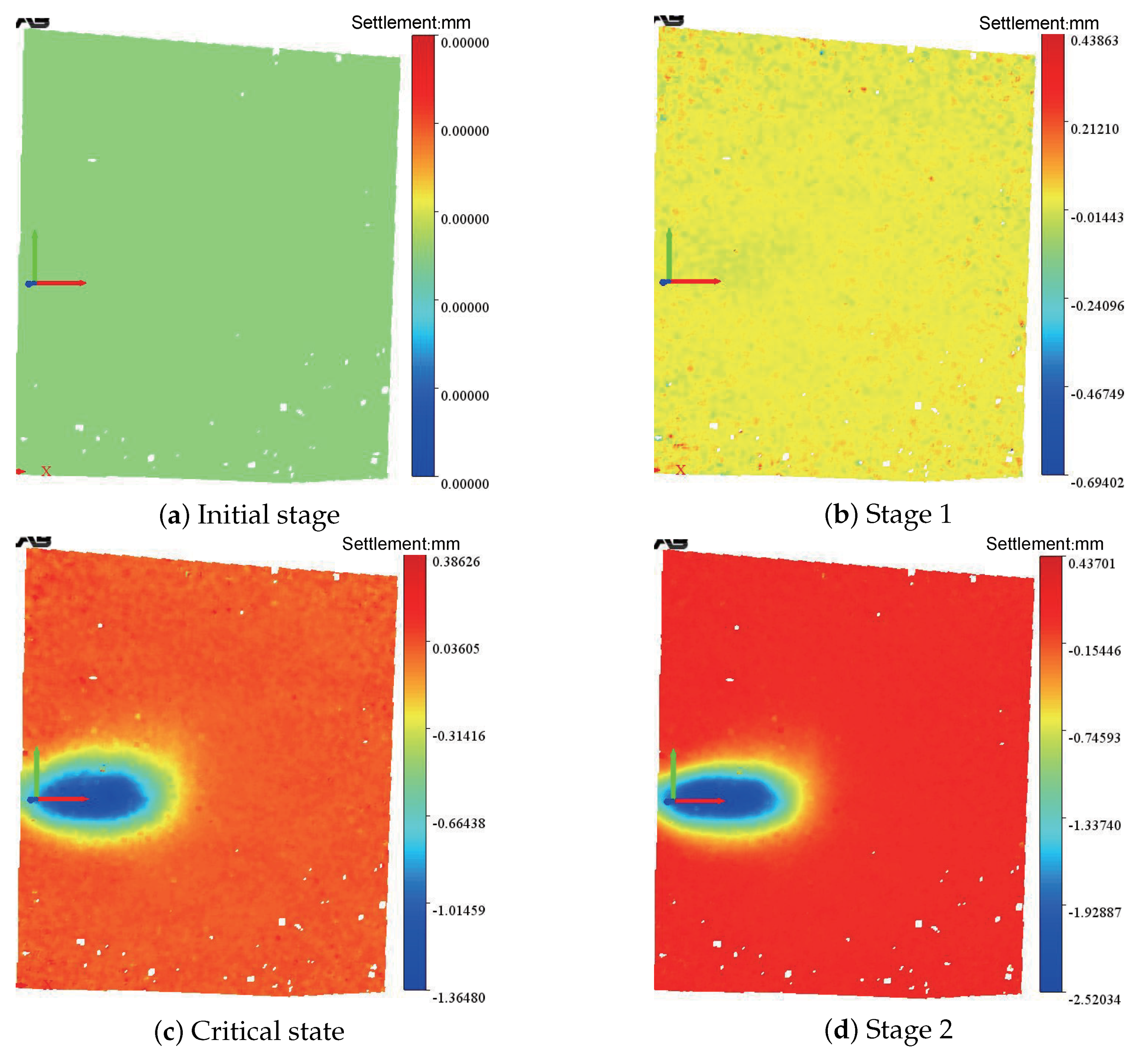
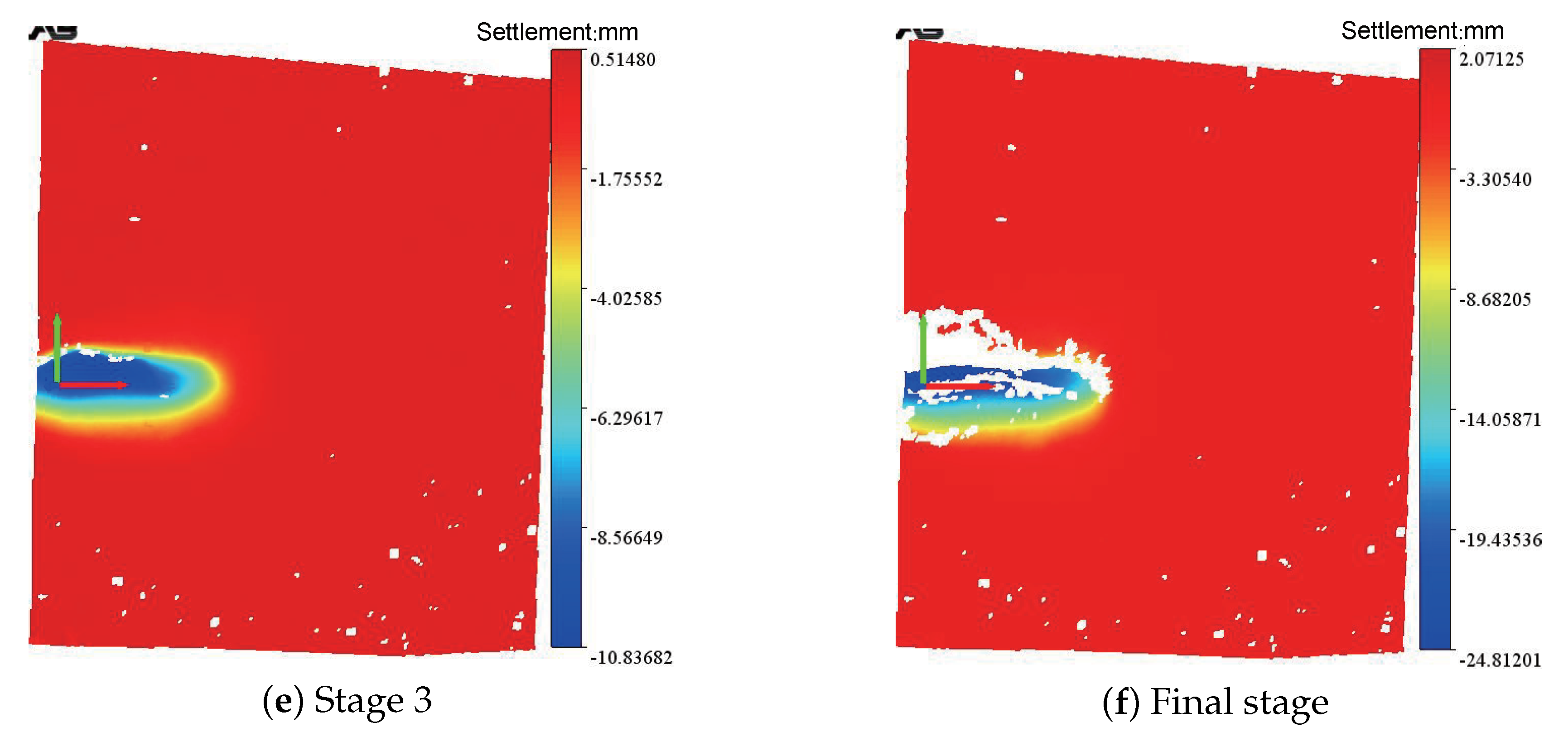
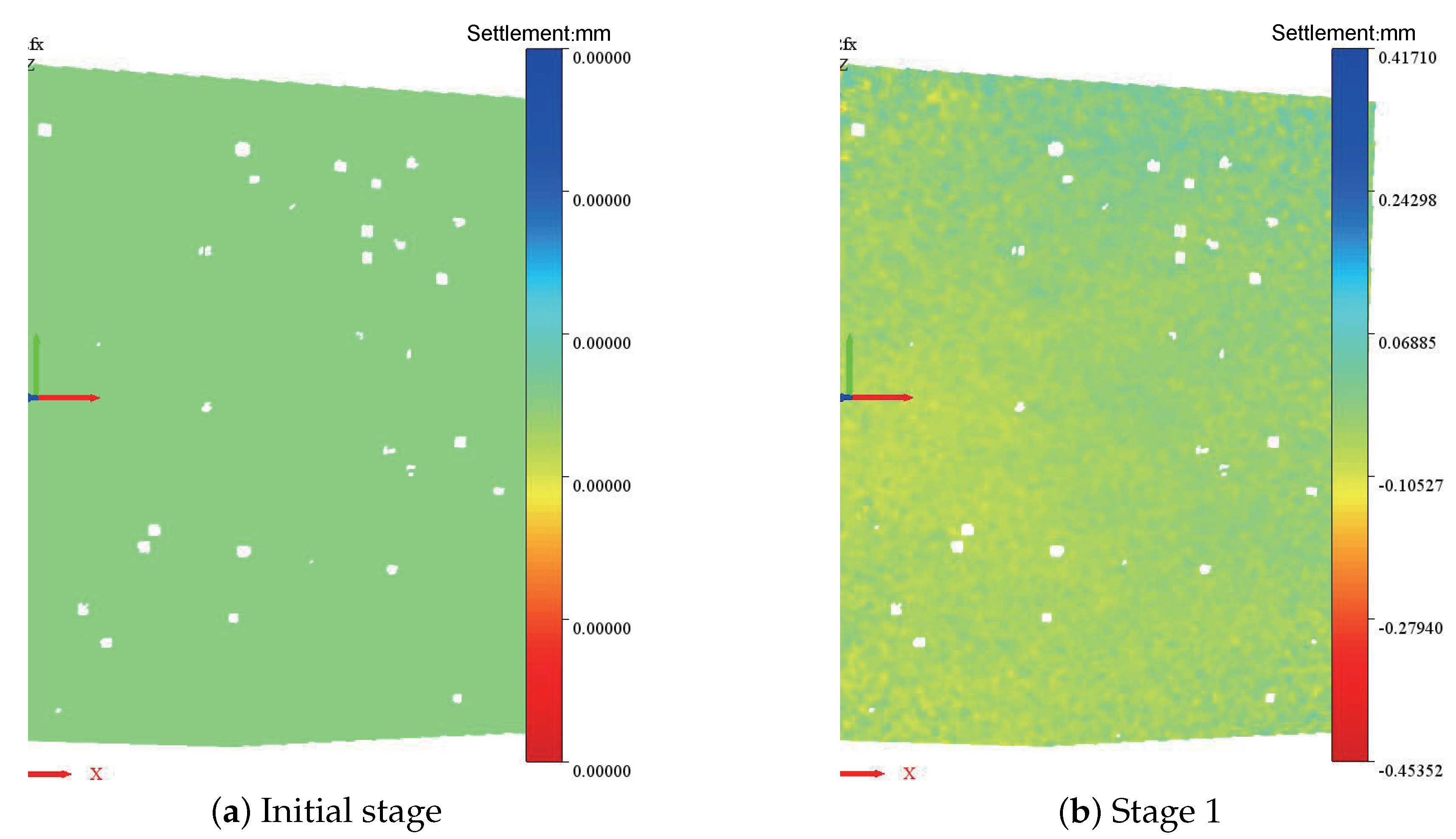
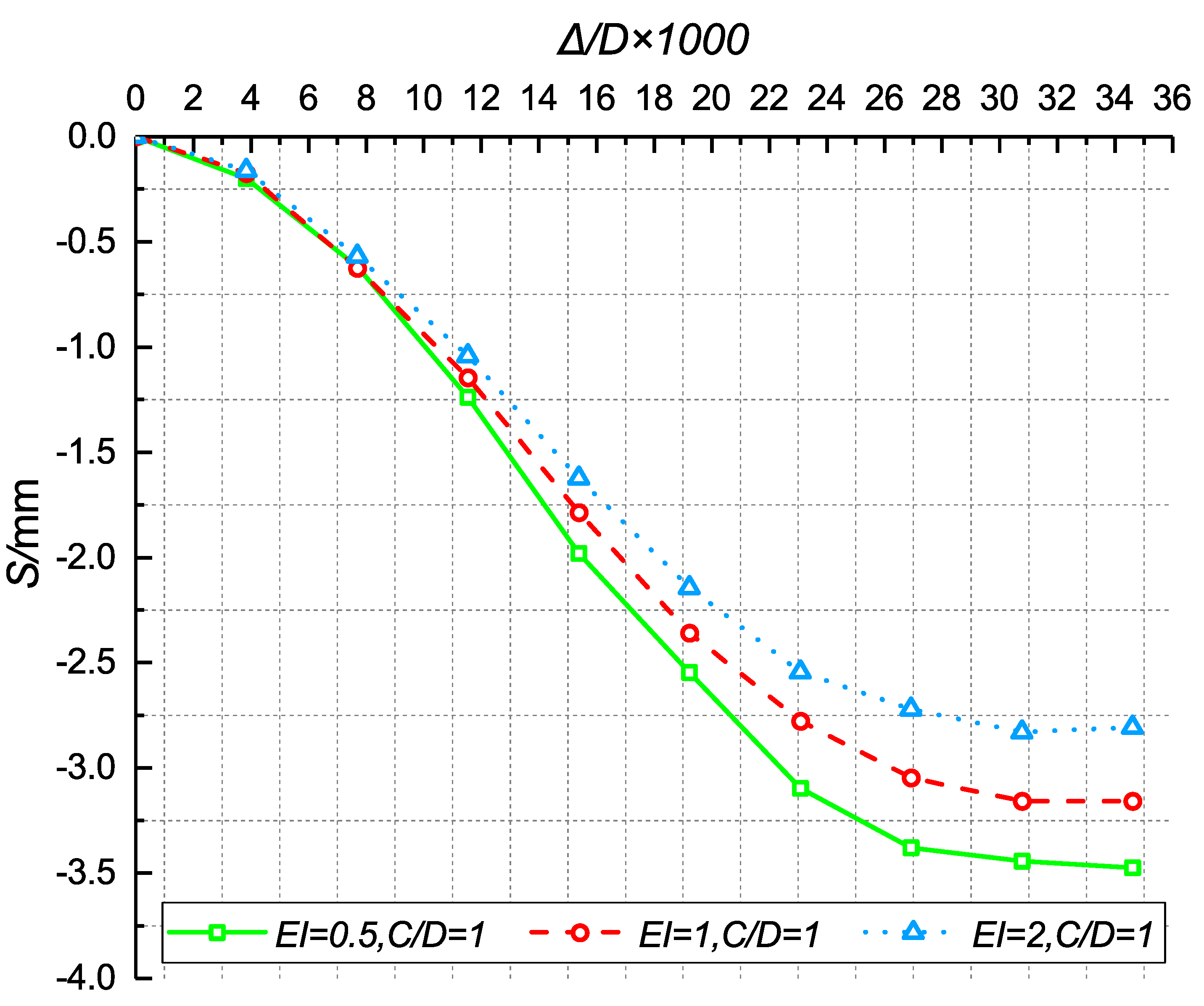
3.2. Ground Settlement
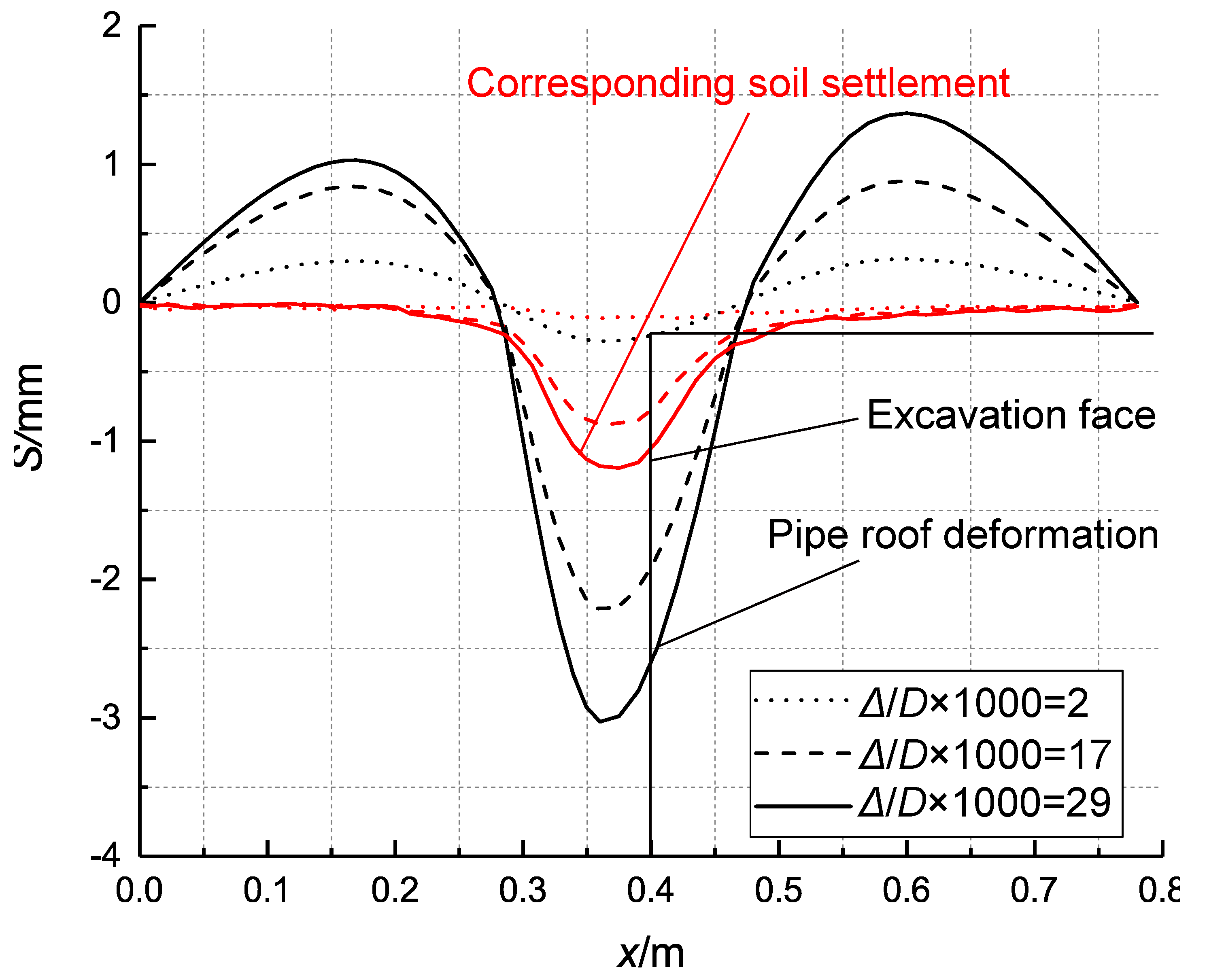
3.3. Pipe Roof Deformation
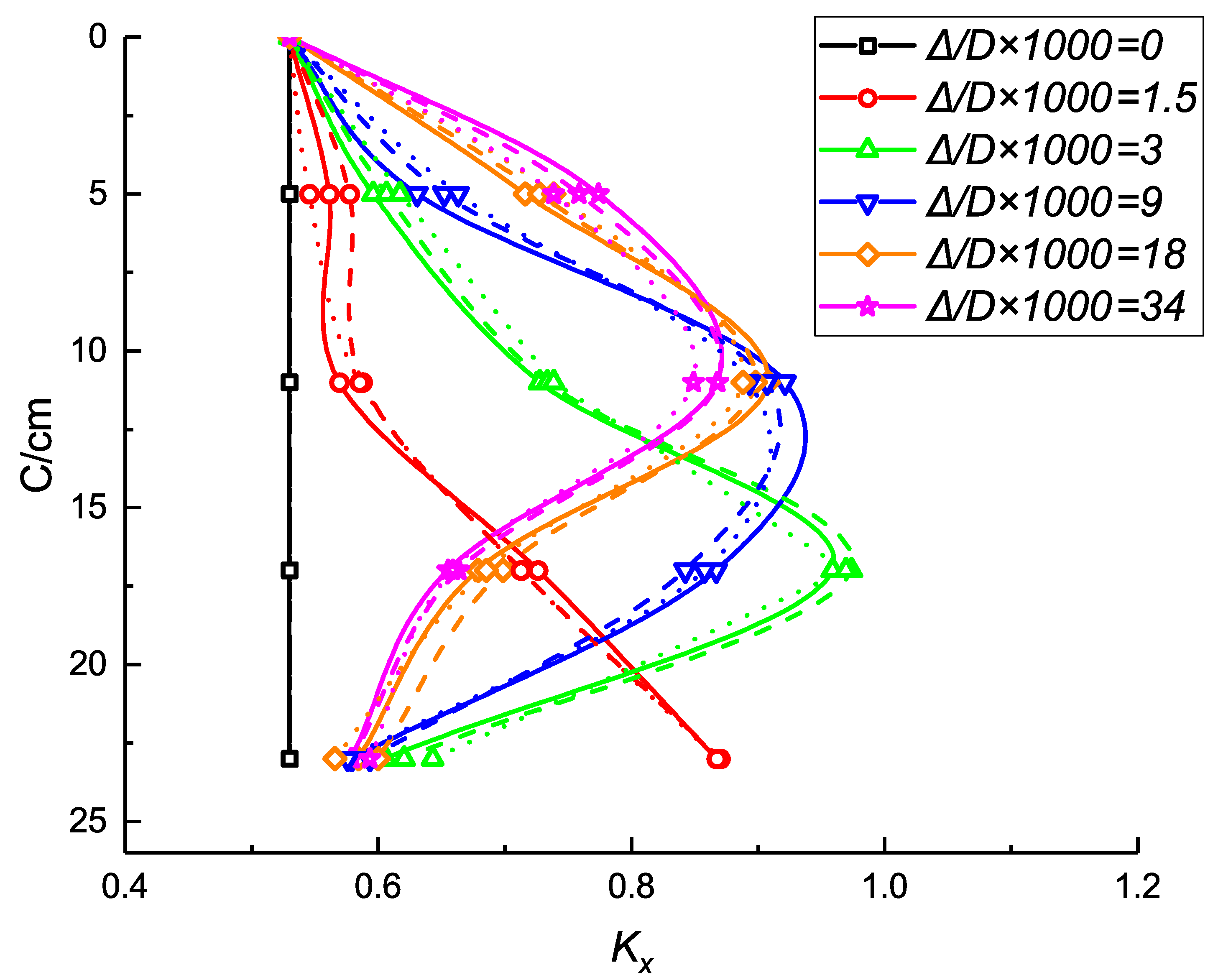
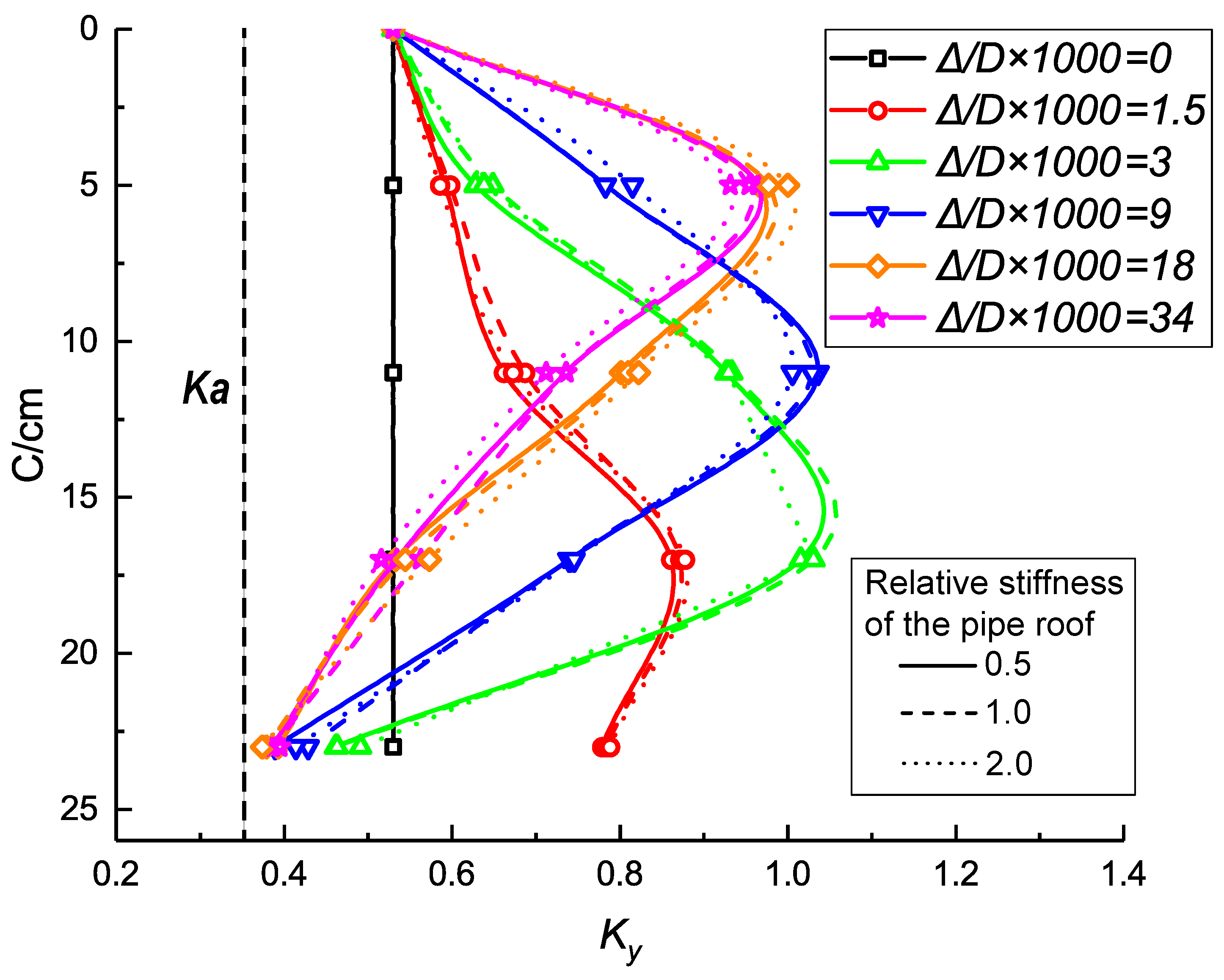
3.4. Soil Pressure
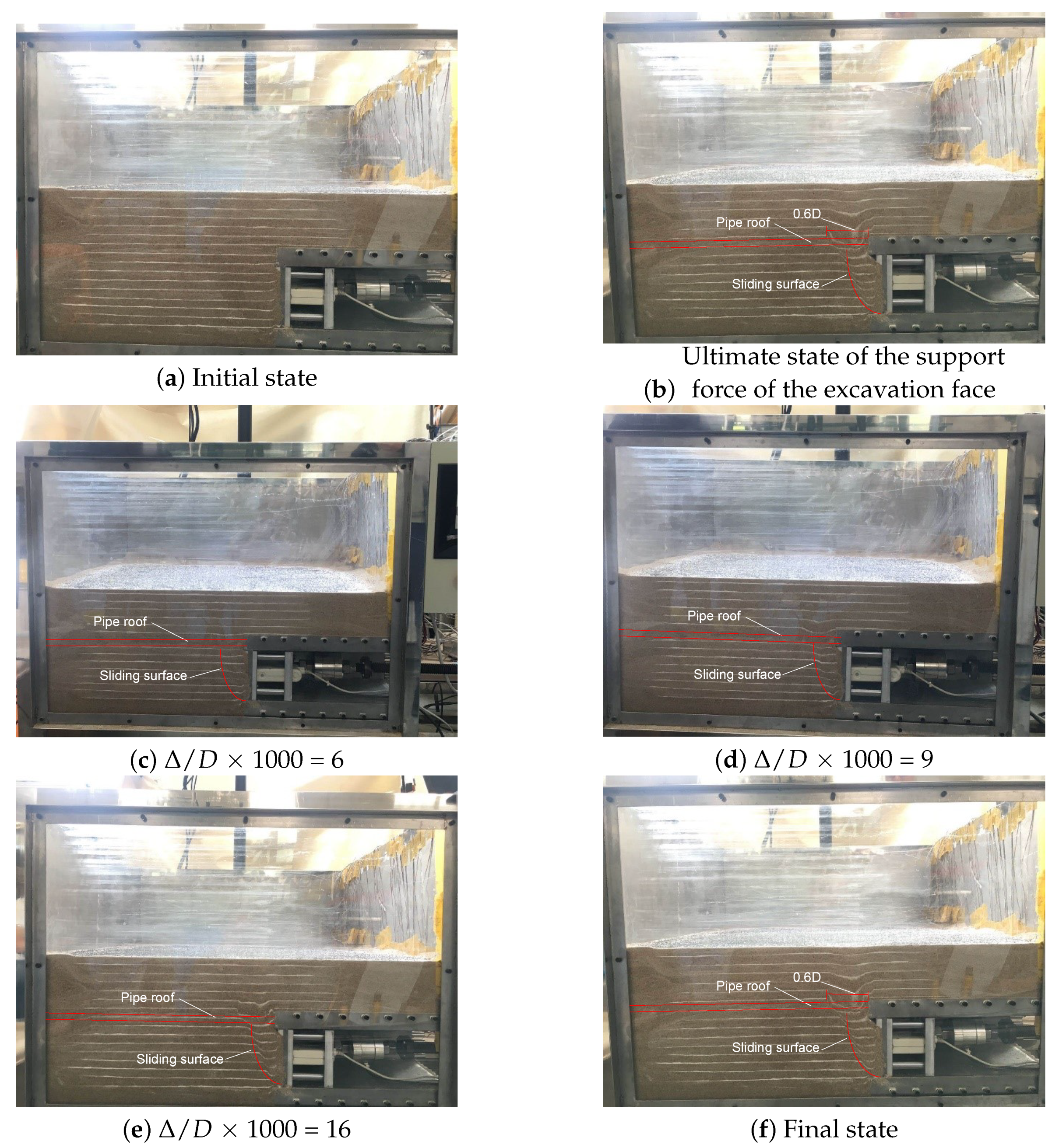
3.5. Excavation Face Instability Mode
4. Conclusions
- The pipe roof can reduce the load on the excavation face and suppress the load fluctuation. The excavation stability increase with increasing pipe roof stiffness.
- The presence of the pipe roof induces an important decrease in the ground settlement. Increasing pipe stiffness reduces the soil settlement, but the influence is weak. The pipe roof increases the range of the longitudinal settling tank and transmits the overburden pressure above the sliding block to the soil on both sides of the sliding block, which effectively reduces the surface settlement.
- Under the same working conditions, the vertical deformation of the pipe roof is greater than the corresponding ground surface settlement and the range of ground settlement is larger than that of the pipe roof deformation.
- The change in lateral pressure coefficient in the two directions reflects the formation of the soil arch in the overburden soil of the pipe roof and the pipe roof stiffness does not influence the distribution of the earth pressure coefficients.
- Compared with the condition without the pipe roof, the pipe roof prevent the failure zone from extending to the ground surface and causes an increase of approximately 50% of the longitudinal width of the failure zone.
Author Contributions
Funding
Conflicts of Interest
References
- Broere, W. Urban underground space: Solving the problems of today’s cities. Tunn. Undergr. Space Technol. 2016, 55, 245–248. [Google Scholar] [CrossRef]
- Huang, F.; Yang, X.L. Upper bound limit analysis of collapse shape for circular tunnel subjected to pore pressure based on the Hoek–Brown failure criterion. Tunn. Undergr. Space Technol. 2011, 26, 614–618. [Google Scholar] [CrossRef]
- Senent, S.; Jimenez, R. A tunnel face failure mechanism for layered ground, considering the possibility of partial collapse. Tunn. Undergr. Space Technol. 2015, 47, 182–192. [Google Scholar] [CrossRef]
- Zingg, S.; Anagnostou, G. An investigation into efficient drainage layouts for the stabilization of tunnel faces in homogeneous ground. Tunn. Undergr. Space Technol. 2016, 58, 49–73. [Google Scholar] [CrossRef]
- Chen, R.P.; Li, J.; Kong, L.G.; Tang, L.J. Experimental study on face instability of shield tunnel in sand. Tunn. Undergr. Space Technol. 2013, 13, 12–21. [Google Scholar] [CrossRef]
- Lee, C.J.; Wu, B.R.; Chen, H.T.; Chiang, K.H. Tunnel stability and arching effects during tunneling in soft clayey soil. Tunn. Undergr. Space Technol. 2006, 21, 119–132. [Google Scholar] [CrossRef]
- Idinger, G.; Aklik, P.; Wu, W.; Borja, R.I. Centrifuge model test on the face stability of shallow tunnel. Acta Geotech. 2011, 6, 105–117. [Google Scholar] [CrossRef]
- Sun, J.; Liu, J. Visualization of tunnelling-induced ground movement in transparent sand. Tunn. Undergr. Space Technol. 2014, 40, 236–240. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, D.; Fang, Q.; Yu, Q.; Xia, L. A physical and numerical investigation of the failure mechanism of weak rocks surrounding tunnels. Comput. Geotech. 2014, 61, 292–307. [Google Scholar] [CrossRef]
- Lin, P.; Liu, H.; Zhou, W. Experimental study on failure behaviour of deep tunnels under high in-situ stresses. Tunn. Undergr. Space Technol. 2015, 46, 28–45. [Google Scholar] [CrossRef]
- Zhu, Z.; Li, Y.; Xie, J.; Liu, B. The effect of principal stress orientation on tunnel stability. Tunn. Undergr. Space Technol. 2015, 49, 279–286. [Google Scholar] [CrossRef]
- Qiu, Z.; Li, H.; Hu, W.; Wang, C.; Liu, J.; Sun, Q. Real-Time Tunnel Deformation Monitoring Technology Based on Laser and Machine Vision. Appl. Sci. 2018, 8, 2579. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Q.; Lin, Z.; Wang, X. Spatiotemporal evolution rule of rocks fracture surrounding gob-side roadway with model experiments. Int. J. Min. Sci. Technol. 2016, 26, 895–902. [Google Scholar] [CrossRef]
- Sun, X.; Song, P.; Zhao, C.; Zhang, Y.; Li, G.; Miao, C. Physical modeling experimental study on failure mechanism of surrounding rock of deep-buried soft tunnel based on digital image correlation technology. Arab. J. Geosci. 2018, 11, 624. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Z.; Zhu, Y.; Huang, X.; Zhuang, Q. Capturing the cracking characteristics of concrete lining during prototype tests of a special-shaped tunnel using 3D DIC photogrammetry. Eur. J. Environ. Civ. Eng. 2018, 22, 179–199. [Google Scholar] [CrossRef]
- Minardo, A.; Catalano, E.; Coscetta, A.; Zeni, G.; Zhang, L.; Di Maio, C.; Vassallo, R.; Coviello, R.; Macchia, G.; Picarelli, L.; et al. Distributed Fiber Optic Sensors for the Monitoring of a Tunnel Crossing a Landslide. Remote Sens. 2018, 10, 1291. [Google Scholar] [CrossRef]
- Ge, J.K. The Study on Construction Method of Pipe-roofing Tunnel in Saturated Soft Soil. Shanghai Highw. 2004, 1, 38–43. [Google Scholar]









| Dry Density (g/cm) | Proportion | / | Compressive Strength (kPa) | Modulus of Compression (MPa) | |
|---|---|---|---|---|---|
| 1.856 | 2.56 | 0.21 | 37 | 3.876 | 3.034 |
| Monitoring Equipment | Monitoring Object | Precision |
|---|---|---|
| DIC binocular camera | The settlement of the ground surface | 0.01 mm |
| Distributed optical fiber | The deformation of pipes | ≤1.0% FS |
| Earth pressure cell | Earth pressure in soil stratum | ≤0.5% FS |
| Pull pressure sensor | The axial force of excavation face | ≤0.5% FS |
| Displacement sensor | The displacement of excavation face | ≤0.5% FS |
| Load | The Relative Stiffness | Load | Load Deviation | (-)/% | |||
|---|---|---|---|---|---|---|---|
| / | / | of the Pipe Roof | / | / | /% | /% | |
| 0.5 | 0.128 | 0.109 | 15.23 | 27.85 | 12.62 | ||
| 0.151 | 0.253 | 1.0 | 0.118 | 0.102 | 21.85 | 32.45 | 10.60 |
| 2.0 | 0.110 | 0.097 | 27.15 | 35.76 | 8.61 | ||
| Without the Pipe Roof | With the Pipe Roof | |||||
|---|---|---|---|---|---|---|
| / | The Relative Stiffness of the Pipe Roof | / | ||||
| 0.5 | 12.13 | 5.06 | 239.72% | |||
| 3.04 | 8.38 | 36.28% | 1.0 | 12.32 | 5.48 | 224.82% |
| 2.0 | 12.51 | 6.12 | 204.41% | |||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, X.; Zhao, M.; Shahrour, I. Experimental Study of the Behavior of Rectangular Excavations Supported by a Pipe Roof. Appl. Sci. 2019, 9, 2082. https://doi.org/10.3390/app9102082
Xie X, Zhao M, Shahrour I. Experimental Study of the Behavior of Rectangular Excavations Supported by a Pipe Roof. Applied Sciences. 2019; 9(10):2082. https://doi.org/10.3390/app9102082
Chicago/Turabian StyleXie, Xiongyao, Mingrui Zhao, and Isam Shahrour. 2019. "Experimental Study of the Behavior of Rectangular Excavations Supported by a Pipe Roof" Applied Sciences 9, no. 10: 2082. https://doi.org/10.3390/app9102082
APA StyleXie, X., Zhao, M., & Shahrour, I. (2019). Experimental Study of the Behavior of Rectangular Excavations Supported by a Pipe Roof. Applied Sciences, 9(10), 2082. https://doi.org/10.3390/app9102082







