Effects of Laser Beam Parameters on Bendability and Microstructure of Stainless Steel in Three-Dimensional Laser Forming
Abstract
:1. Introduction
2. Methods
2.1. Laser Forming Experiments
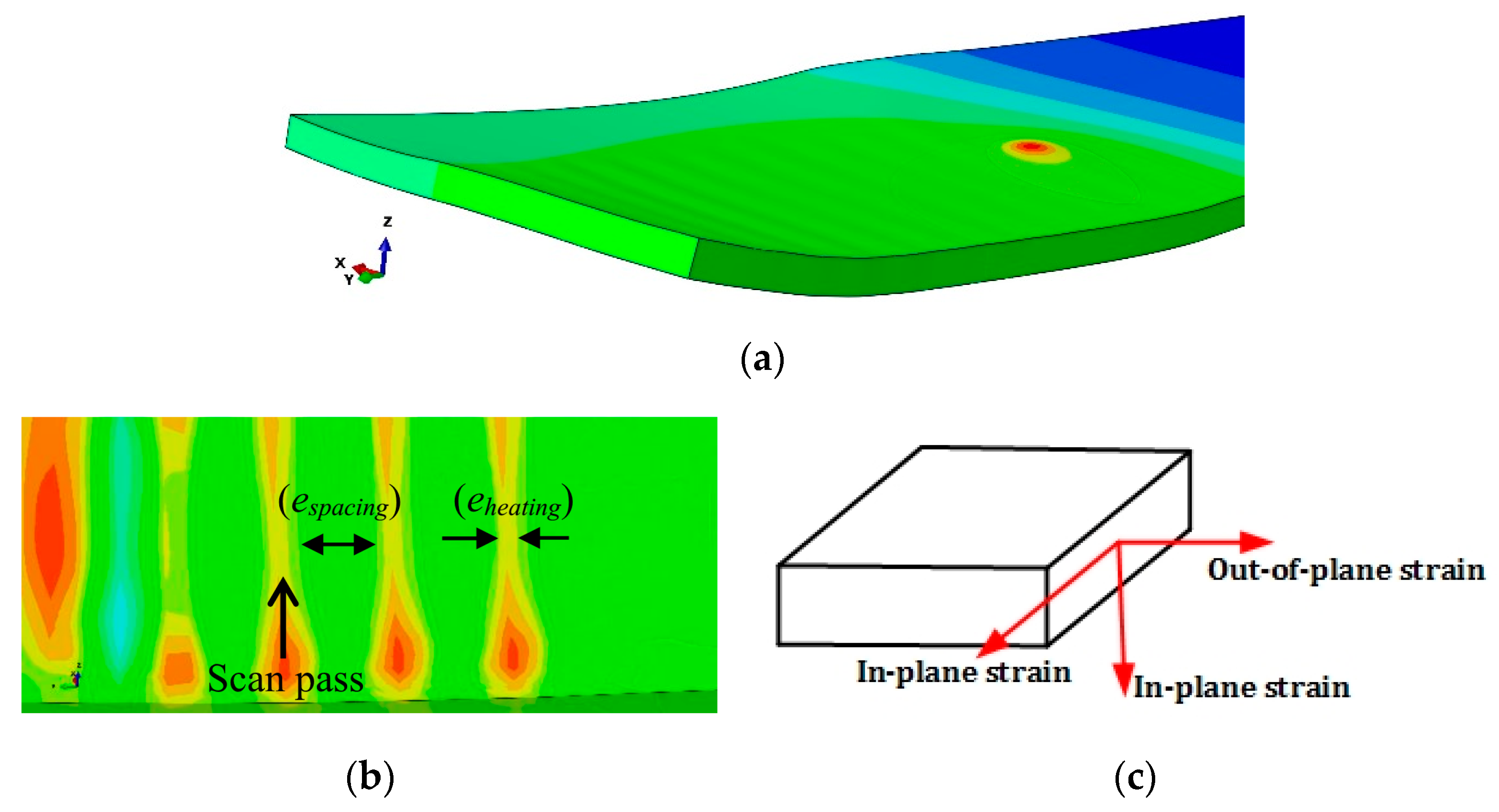
2.2. Determination of Strains and Temperatures
3. Results and Discussion
3.1. Effects of the Beam Diameter and Hatch Spacing
3.2. Effects of the Temperature and Microstructure
3.3. Overlap Region
3.4. Microhardness
4. Conclusions
- (1).
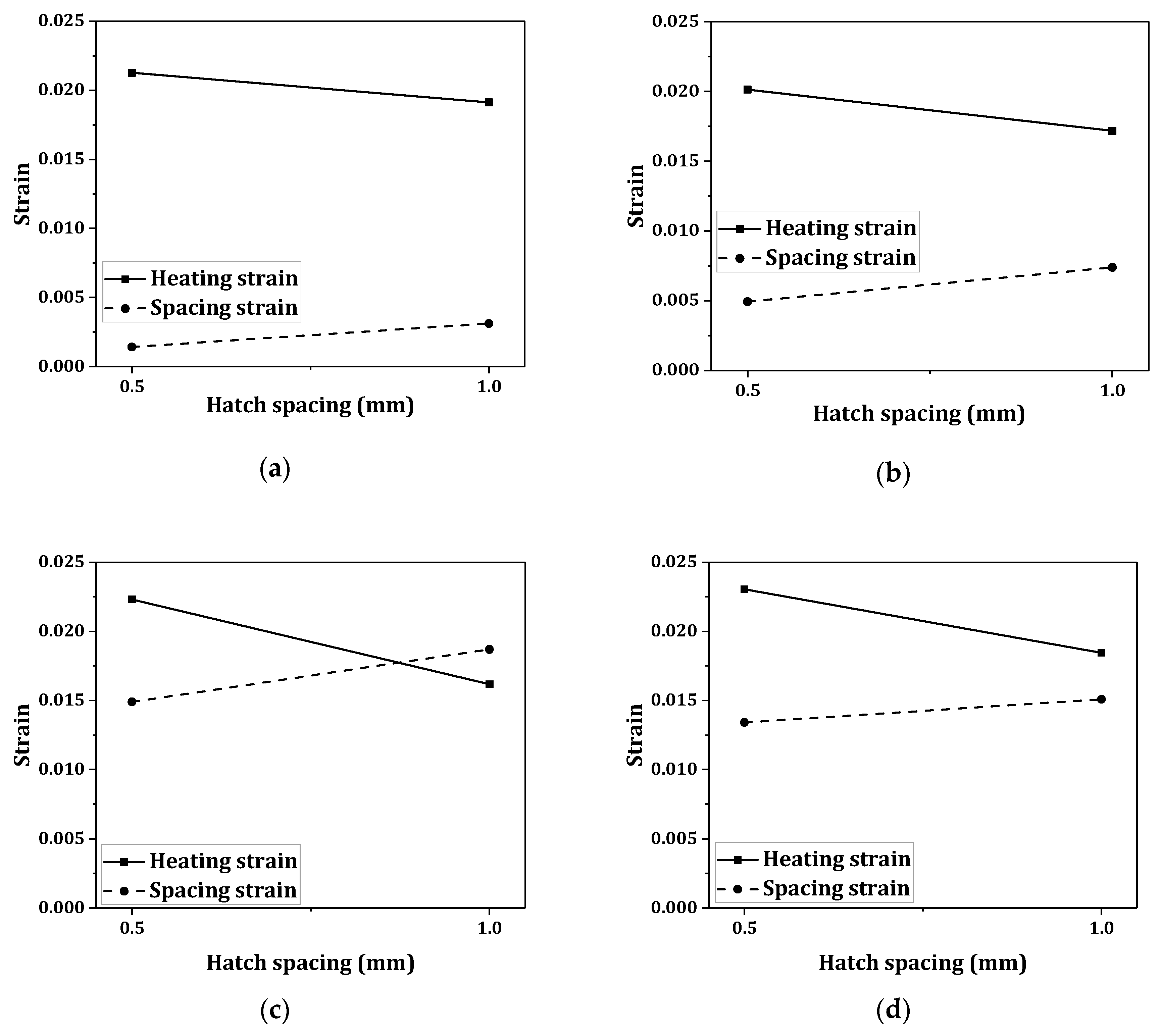
- At a constant beam diameter, with an increase in hatch spacing, the strain on the spacing between the scan tracks increased, while the strain on the heating line decreased. The increase in strain of the spacing between the scan tracks was responsible for the lower bendability of the material, which demonstrated the counter bending between the scan paths.
- (2).
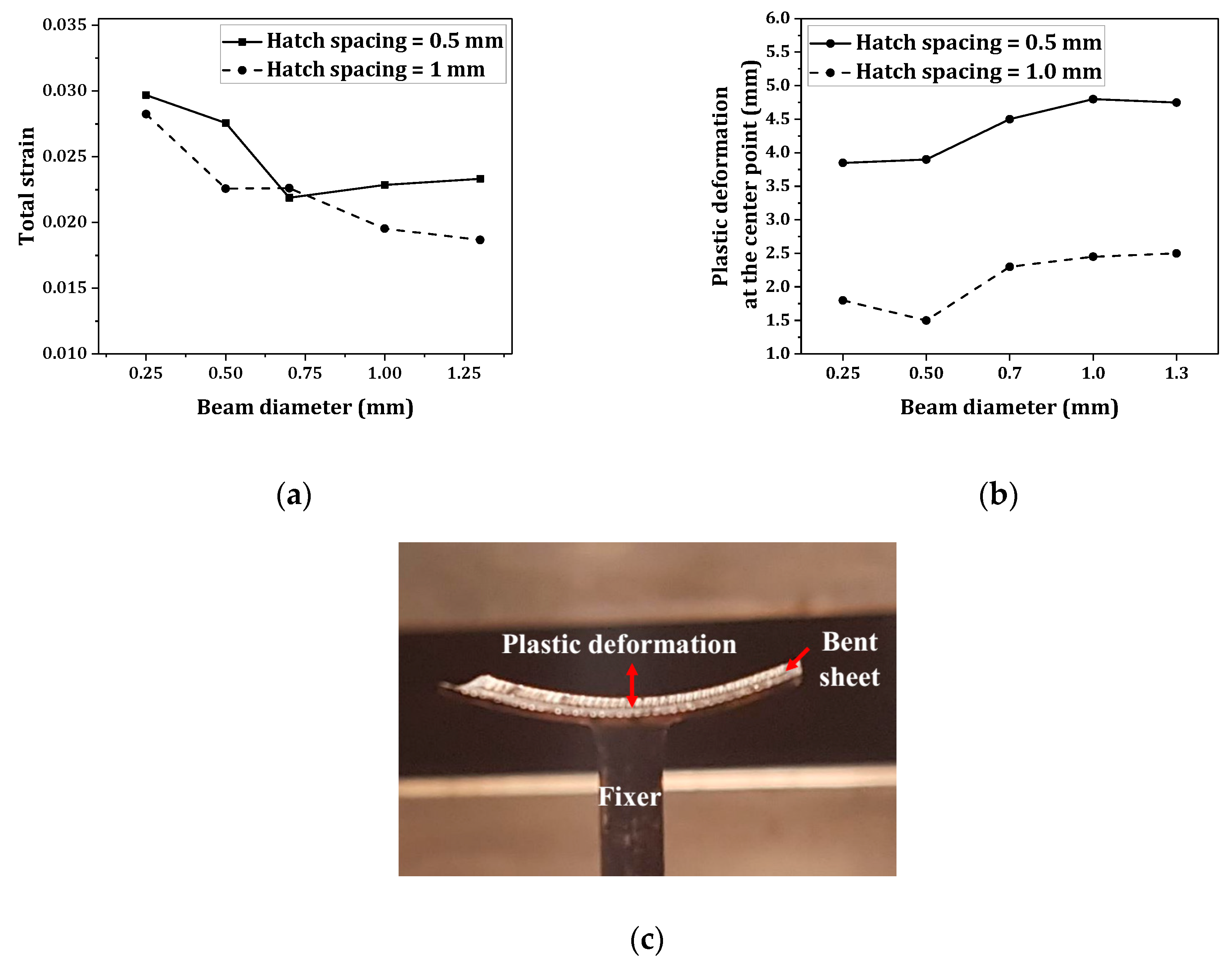
- The total plastic strain increased with a decrease in beam diameter and increase in hatch spacing. This was attributed to the increase in temperature and implied a correlation between the melting and an increase in the total strain.
- (3).
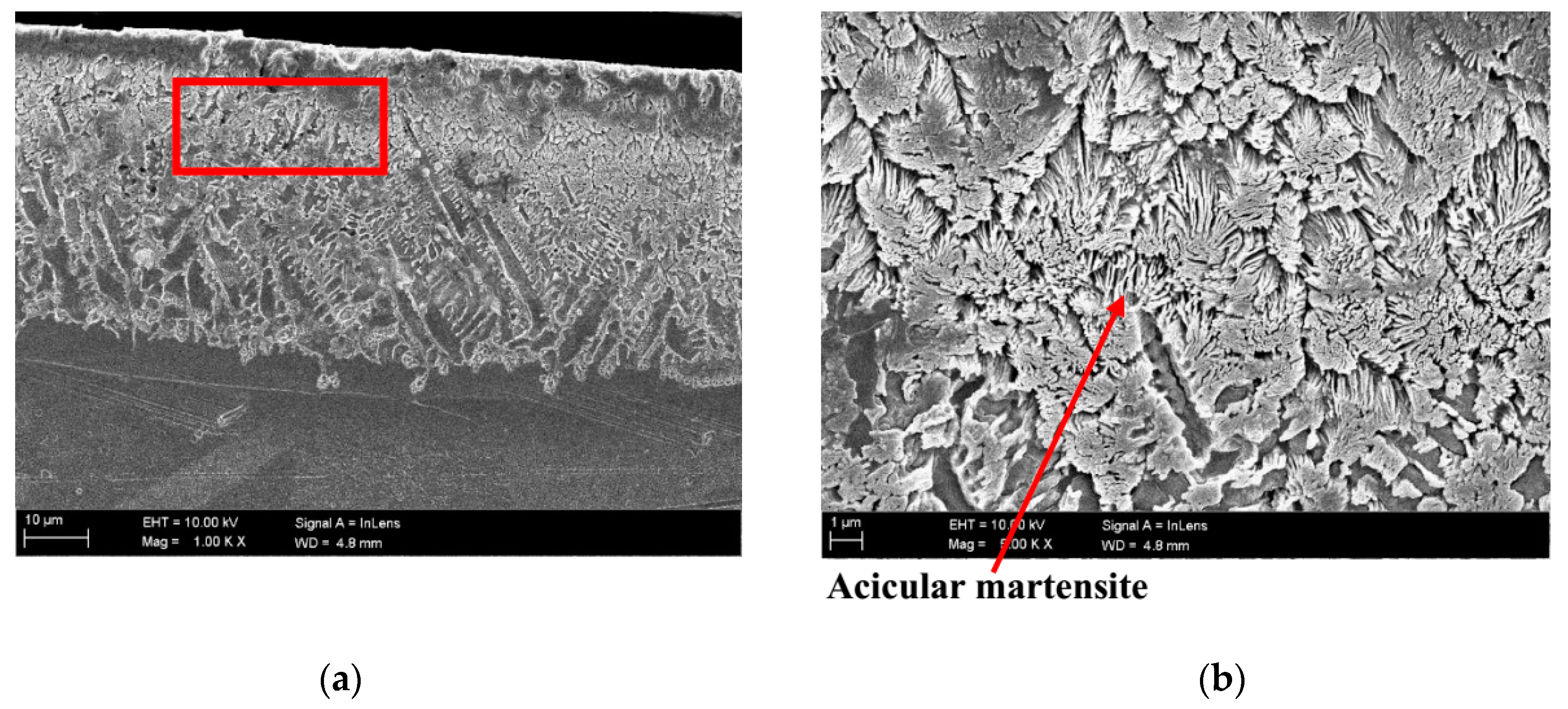
- When the beam diameter was increased and the hatch spacing was decreased, the formation of the small equiaxed dendrite grains instead of coarse and inhomogeneous austenite grains increased the bendability of the material. This could be attributed to the decrease in anisotropy in the microstructure, which increased the work hardening capacity.
- (4).
- The grain sizes were increased in the reheated overlap regions of the samples. The reduction in the yield stress of the material in the overlap region owing to the grain growth led to a larger deformation.
- (5).
- The grain boundary strengthening effect with the larger strain hardening of the material were responsible for the increase in microhardness with an increase in beam diameter and decrease in hatch spacing. However, at the small beam diameter and large hatch spacing, the increase in the cooling rate was responsible for the microhardness improvement.
Author Contributions
Funding
Conflicts of Interest
References
- Chen, J.Y.; Conlon, K.; Xue, L.; Rogge, R. Experimental study of residual stresses in laser clad AISI P20 tool steel on pre-hardened wrought P20 substrate. Mater. Sci. Eng. A 2010, 527, 7265–7273. [Google Scholar] [CrossRef] [Green Version]
- Woo, Y.Y.; Hwang, T.; Oh, I.; Seo, D.; Moon, Y.H. Analysis on selective laser melting of WC-reinforced H13 steel composite powder by finite element method. Adv. Mech. Eng. 2019, 11, 1687814018822200. [Google Scholar] [CrossRef]
- Seyedkashi, S.M.H.; Abazari, H.D.; Hoseinpour Gollo, M.; Woo, Y.Y.; Moon, Y.H. Characterization of laser bending of SUS304/C11000clad sheets. J. Mech. Sci. Technol. 2019, 33, 3223–3230. [Google Scholar] [CrossRef]
- Imhan, K.I.; Baharudin, B.T.H.T.; Zakaria, A.; Shah, B.; Ismail, M.I.; Alsabti, N.M.H.; Ahmad, A.K. Investigation of material specifications changes during laser tube bending and its influence on the modification and optimization of analytical modeling. Opt. Laser Technol. 2017, 95, 151–156. [Google Scholar] [CrossRef]
- Hwang, T.; Woo, Y.Y.; Han, S.W.; Moon, Y.H. Functionally graded properties in directed-energy-deposition titanium parts. Opt. Laser Technol. 2018, 105, 80–88. [Google Scholar] [CrossRef]
- Seyedkashi, S.H.; Hosseinpour Gollo, M.; Biao, J.; Moon, Y.H. Laser bendability of SUS430/C11000/SUS430 laminated composite and its constituent layers. Met. Mater. Int. 2016, 22, 527–534. [Google Scholar] [CrossRef]
- Yang, L.; Tang, J.; Wang, M.; Wang, Y.; Chen, Y. Surface characteristic of stainless steel sheet after pulsed laser forming. Appl. Surf. Sci. 2010, 256, 7018–7026. [Google Scholar] [CrossRef]
- Zhang, X.R.; Xu, X. High precision microscale bending by pulsed and CW lasers. J. Manuf. Sci. Eng. 2003, 125, 512–518. [Google Scholar] [CrossRef]
- Birnbaum, A.J.; Yao, Y.L. The effects of laser forming on NiTi superelastic shape memory alloys. J. Manuf. Sci. Eng. 2010, 132, 041002. [Google Scholar] [CrossRef]
- Abazari, H.D.; Seyedkashi, S.M.H.; Hosseinpour Gollo, M.; Moon, Y.H. Evolution of microstructure and mechanical properties of SUS430/C11000/SUS430 composites during the laser-forming process. Met. Mater. Int. 2017, 23, 865–876. [Google Scholar] [CrossRef]
- Seyedkashi, S.H.; Cho, J.; Lee, S.; Moon, Y.H. Feasibility of underwater laser forming of laminated metal composites. Mater. Manuf. Process. 2018, 33, 546–551. [Google Scholar] [CrossRef]
- Abolhasani, D.; Seyedkashi, S.M.H.; Kim, Y.T.; Hoseinpour Gollo, M.; Moon, Y.H. A double raster laser scanning strategy for rapid die-less bending of 3D shape. J. Mater. Res. Technol. 2019, 8, 4741–4756. [Google Scholar] [CrossRef]
- Joo, B.D.; Jang, J.H.; Lee, J.H.; Son, Y.M.; Moon, Y.H. Selective laser melting of Fe-Ni-Cr layer on AISI H13 tool steel. Trans. Nonferr. Met. Soc. China 2009, 19, 921–924. [Google Scholar] [CrossRef]
- Abolhasani, D.; Seyedkashi, S.M.H.; Kang, N.; Kim, Y.J.; Woo, Y.Y.; Moon, Y.H. Analysis of melt-pool behaviors during selective laser melting of AISI 304 stainless-steel composites. Metals 2019, 9, 876. [Google Scholar] [CrossRef]
- Davis, J.R.; Mills, K.M.; Lampman, S.R. Properties and Selection: Irons, Steels, and High Performance Alloys; Metals Handbook; ASM: Materials Park, OH, USA, 2005. [Google Scholar]
- Moon, Y.H.; Kim, D.W.; Van Tyne, C.J. Analytical model for prediction of sidewall curl during stretch-bend sheet metal forming. Int. J. Mech. Sci. 2008, 50, 666–675. [Google Scholar] [CrossRef]
- Jeon, C.H.; Han, S.W.; Joo, B.D.; Van Tyne, C.J.; Moon, Y.H. Deformation analysis for cold rolling of Al-Cu double layered sheet by the physical modeling and finite element method. Met. Mater. Int. 2013, 19, 1069–1076. [Google Scholar] [CrossRef]
- Cheng, J.; Yao, Y.L. Process design of laser forming for three-dimensional thin plates. J. Manuf. Sci. Eng. 2004, 126, 217–225. [Google Scholar] [CrossRef]
- Kim, S.Y.; Joo, B.D.; Shin, S.; Van Tyne, C.J.; Moon, Y.H. Discrete layer hydroforming of three-layered tubes. Int. J. Mach. Tool Manuf. 2013, 68, 56–62. [Google Scholar] [CrossRef]
- Park, J.Y.; Oh, I.Y.; Van Tyne, C.J.; Moon, Y.H. Characterization of deformation processing maps of 304L stainless steel based on compressive and tensile flow curves. Proc. Inst. Mech. Eng. 2018, 233, 203–210. [Google Scholar] [CrossRef]
- Solomon, N.; Solomon, I. Deformation induced martensite in AISI 316 stainless steel. Rev. Metal. 2010, 46, 121–128. [Google Scholar] [CrossRef]
- Shen, N.; Ding, H.; Wang, Q.; Ding, H. Effect of confinement on surface modification for laser peen forming without protective coating. Surf. Coat. Tech. 2016, 289, 194–205. [Google Scholar] [CrossRef] [Green Version]
- Walczak, M.; Ramos-Grez, J.; Celentano, D.; Lima, E. Sensitization of AISI 302 stainless steel during low-power laser forming. Opt. Laser Eng. 2010, 48, 906–914. [Google Scholar] [CrossRef]
- Abolhasani, D.; Ezatpour, H.R.; Sajjadi, S.A.; Abolhasani, Q. Microstructure and mechanical properties evolution of 6061 aluminum alloy formed by forward thixoextrusion process. Mater. Des. 2013, 49, 784–790. [Google Scholar] [CrossRef]
- Jang, J.H.; Lee, J.H.; Joo, B.D.; Moon, Y.H. Flow characteristics of aluminum coated boron steel in hot press forming. Trans. Nonferr. Met. Soc. China 2009, 19, 913–916. [Google Scholar] [CrossRef]
- Everhart, W.; Newkirk, J. Grain Size Effects in Selective Laser Melted Fe-Co-2V. Appl. Sci. 2019, 9, 3701. [Google Scholar] [CrossRef]
- Abolhasani, D.; Seyedkashi, S.M.H.; Hwang, T.W.; Moon, Y.H. Selective laser melting of AISI 304 stainless steel composites reinforced by Al2O3 and eutectic mixture of Al2O3–ZrO2 powders. Mater. Sci. Eng. A 2019, 763, 138161. [Google Scholar] [CrossRef]
- Dieter, G.E.; Bacon, D.J. Mechanical Metallurgy; McGraw-hill: New York, NY, USA, 1986. [Google Scholar]
- Blecher, J.; Palmer, T.; DebRoy, T. Solidification map of a nickel-base alloy. Metall. Mater. Trans. 2014, 45, 2142–2151. [Google Scholar] [CrossRef]
- Park, Z.S.; Kim, J.; Woo, Y.Y.; Lee, H.; Kim, J.H.; Moon, Y.H. Forced circulation of nitrogen gas for accelerated and eco-friendly cooling of metallic parts. Appl. Sci. 2019, 9, 3679. [Google Scholar] [CrossRef]
- Jang, J.H.; Joo, B.D.; Van Tyne, C.J.; Moon, Y.H. Characterization of deposited layer fabricated by direct laser melting process. Met. Mater. Int. 2013, 19, 497–506. [Google Scholar] [CrossRef]








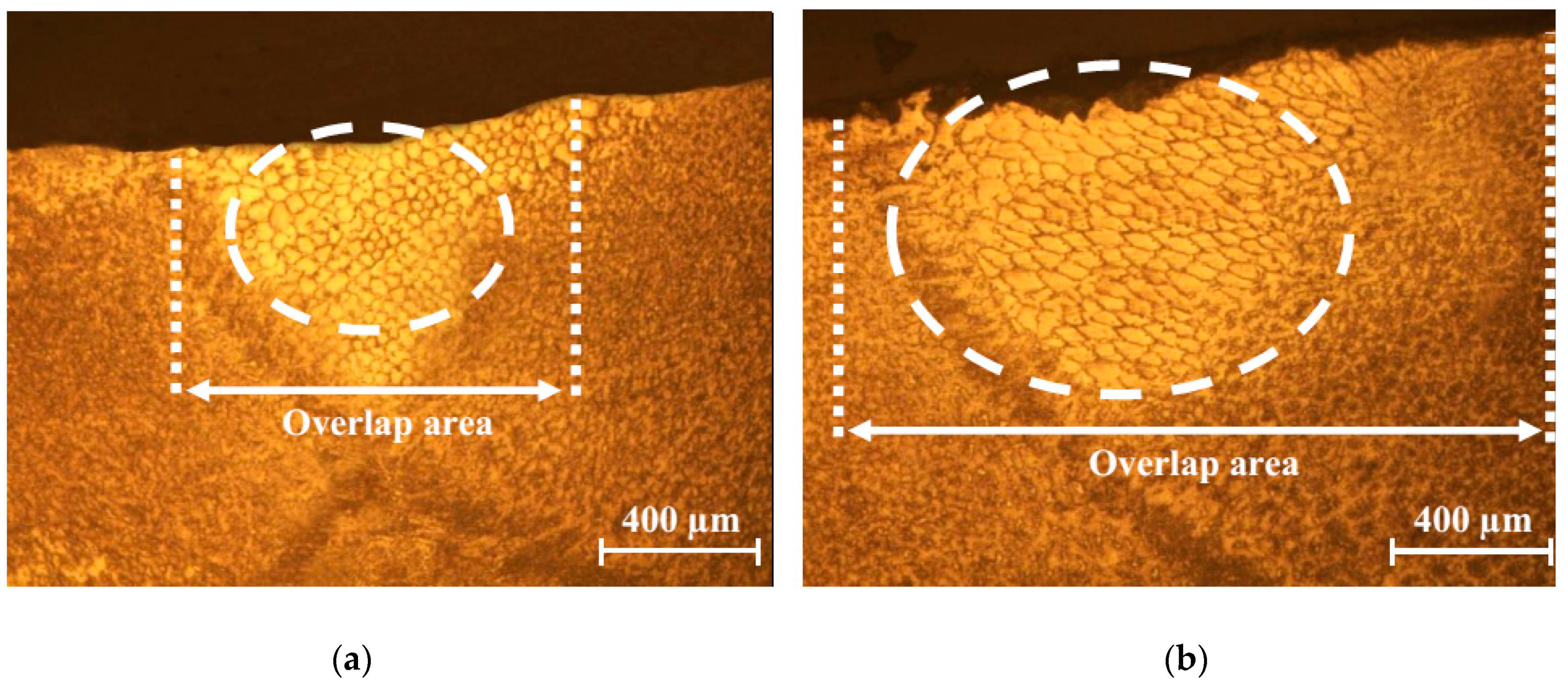

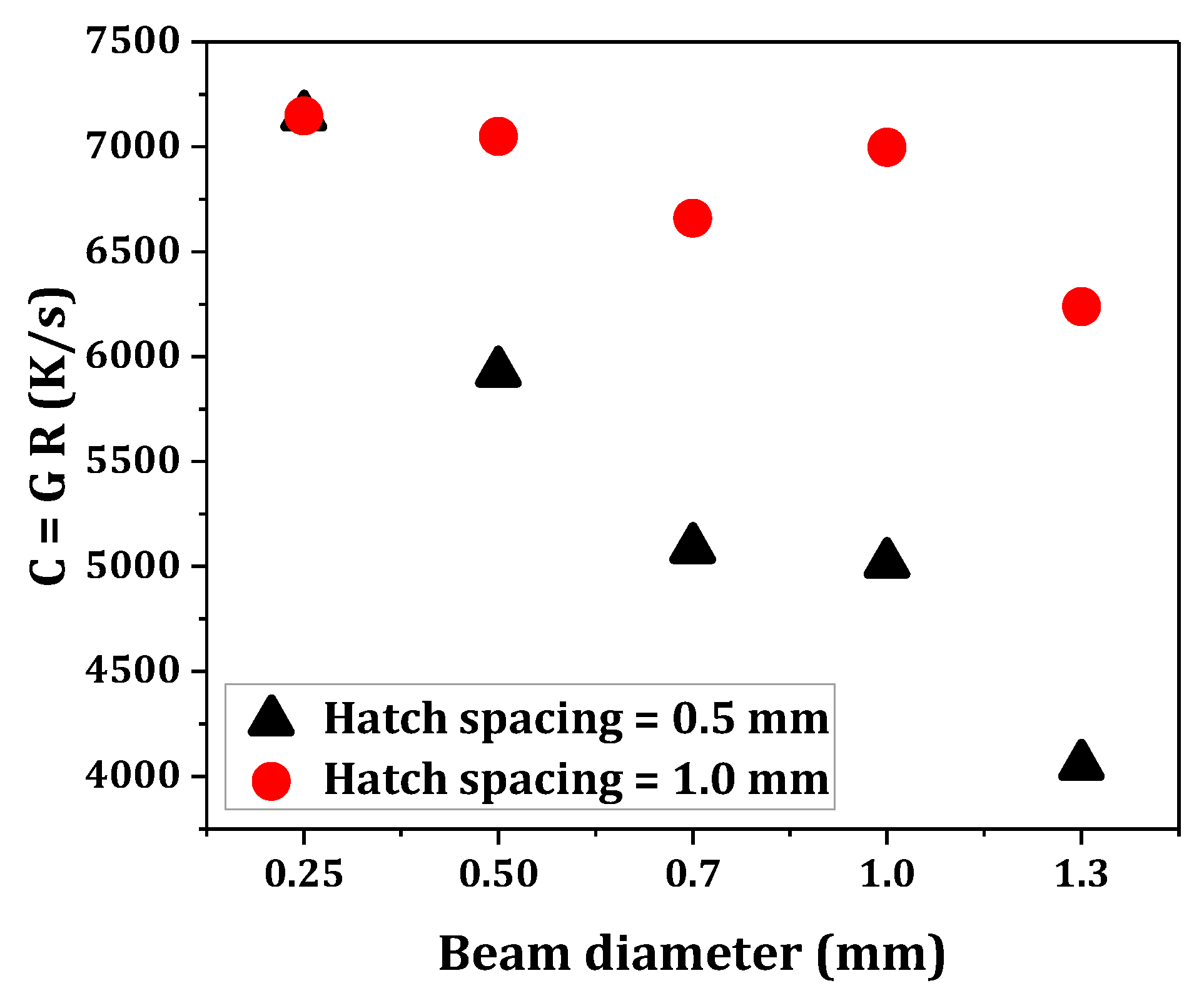
| Case | A | B | C | D | E | a | b | c | d | e |
|---|---|---|---|---|---|---|---|---|---|---|
| hs (mm) | 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.5 | 0.5 | 0.5 | 0.5 | 0.5 |
| Db (mm) | 0.25 | 0.5 | 0.7 | 1.0 | 1.3 | 0.25 | 0.5 | 0.7 | 1.0 | 1.3 |
| OR | −3.0 | −1.0 | −0.43 | 0.0 | 0.23 | −1.0 | 0.0 | 0.30 | 0.50 | 0.62 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abolhasani, D.; Seyedkashi, S.M.H.; Hoseinpour Gollo, M.; Moon, Y.H. Effects of Laser Beam Parameters on Bendability and Microstructure of Stainless Steel in Three-Dimensional Laser Forming. Appl. Sci. 2019, 9, 4463. https://doi.org/10.3390/app9204463
Abolhasani D, Seyedkashi SMH, Hoseinpour Gollo M, Moon YH. Effects of Laser Beam Parameters on Bendability and Microstructure of Stainless Steel in Three-Dimensional Laser Forming. Applied Sciences. 2019; 9(20):4463. https://doi.org/10.3390/app9204463
Chicago/Turabian StyleAbolhasani, Daniyal, Seyed Mohammad Hossein Seyedkashi, Mohammad Hoseinpour Gollo, and Young Hoon Moon. 2019. "Effects of Laser Beam Parameters on Bendability and Microstructure of Stainless Steel in Three-Dimensional Laser Forming" Applied Sciences 9, no. 20: 4463. https://doi.org/10.3390/app9204463
APA StyleAbolhasani, D., Seyedkashi, S. M. H., Hoseinpour Gollo, M., & Moon, Y. H. (2019). Effects of Laser Beam Parameters on Bendability and Microstructure of Stainless Steel in Three-Dimensional Laser Forming. Applied Sciences, 9(20), 4463. https://doi.org/10.3390/app9204463






