Wind-Induced Response Control of High-Rise Buildings Using Inerter-Based Vibration Absorbers

Abstract

The beneficial mass-amplification effect induced by the inerter can be conveniently used in enhanced variants of the traditional Tuned Mass Damper (TMD), namely the Tuned Mass-Damper-Inerter (TMDI) and its special case of Tuned Inerter Damper (TID). In this paper, these inerter-based vibration absorbers are studied for mitigating the wind-induced response of high-rise buildings, with particular emphasis on a 340 m tall building analyzed as case study. To adopt a realistic wind-excitation model, the analysis is based on aerodynamic forces computed through experimental wind tunnel tests for a scaled prototype of the benchmark building, which accounts for the actual cross-section of the structure and the existing surrounding conditions. Mass and stiffness parameters are extracted from the finite element model of the primary structure. Performance-based optimization of the TMDI and the TID is carried out to find a good trade-off between displacement- and acceleration-response mitigation, with the installation floor being an explicit design variable in addition to frequency and damping ratio. The results corresponding to 24 different wind directions indicate that the best vibration mitigation is achieved with a lower installation floor of the TMDI/TID scheme than the topmost floor. The effects of different parameters of TMD, TMDI and TID on wind-induced displacement and acceleration responses and on the equivalent static wind loads (ESWLs) are comparatively evaluated. It is shown that the optimally designed TMDI/TID can achieve better wind-induced vibration mitigation than the TMD while allocating lower or null attached mass, especially in terms of acceleration response.

1. Introduction

In 2018 alone, 143 tall buildings having height of more than 200 m have been constructed, which set up a new record for the annual completion of high-rise buildings around the world. The total number of such buildings reached 1497 up to now based on the statistics from the Council on Tall Buildings and Urban Habitat [1]. These high-rise buildings are very sensitive to wind loads especially in hurricane prone regions. Wind loads may induce large displacement and acceleration responses, which may cause higher stresses in the structural members and discomfort to building occupants. Shape optimization was put forward to improve aerodynamic performance of tall buildings and suppress wind-induced responses [2,3,4,5,6]. However, methods of structural modification sometimes limit the usage of building space (setback of cross-section) [7]. As an alternative, installation of passive vibration control devices, e.g., fluid-viscous dampers [8,9,10,11,12,13], Tuned Mass Damper (TMD) [14,15], Multi Tuned Mass Damper (MTMD) [16] distributed TMD [17,18], and Tuned Liquid Column Damper (TLCD) [19,20,21], was widely used to suppress wind-induced responses. Other attractive and effective implementations of un-conventional TMD schemes were also recently proposed that take advantage from claddings and facades in buildings [22,23]. Further researches based on the wind tunnel test [14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31] and full-scale monitoring [27,32] were performed to evaluate the mitigation effect of TMD on wind-induced responses.

It is widely recognized that the effectiveness of TMD to mitigate vibrations depends heavily on its mass. In general, the larger the attached TMD mass that can be accommodated, the more effective and robust the TMD becomes for vibration control [33,34,35]. However, the attached mass of TMD in high-rise buildings rarely exceeds 0.5% of the total primary mass because of structural and architectural constraints in practical projects [36]. For example, TMD systems of Ping-An Finance Center in Shenzhen City, China, have a weight of 1000 t including mass blocks and supporting-frame structures [37]. The mass of TMD installed in Taipei 101 Tower reaches 660 t. It has the diameter of 6 m and occupies the space from the 87th to 91st floor, including the supporting cables [38]. The TMD not only occupies valuable space of top floors of high-rise buildings but also increases construction cost because of its enormous mass. Motivated by these practical aspects, inerter-based vibration absorbers, e.g., TID and TMDI, were recently proposed to mitigate the vibrations of structure. Lazar et al. [39,40] presented a novel inerter-based vibration absorber system termed as tuned inerter damper (TID). The TID takes advantage of the “mass amplification effect” of the inerter, a two-terminal device of negligible mass/weight whose internal force can reasonably be assumed proportional to the relative acceleration of its two terminals [41]. Acting as an additional, apparent mass, the inerter can modify the inertial properties of the system. Therefore, the TID represents a lower-mass and more effective alternative to the TMD, as it can achieve comparable or even higher vibration suppression level by significantly reducing the attached mass. Marian and Giaralis [42] unified both TMD and TID scheme by proposing an effective passive control system termed Tuned Mass-Damper-Inerter (TMDI). The TMDI scheme will degenerate into TMD and TID by decreasing the inertance ratio and mass ratio to zero, respectively. Most of the recent research has been directed towards optimal design and performance evaluation of inerter-based systems for seismic protection of building structures [39,43,44,45,46,47,48,49,50,51], wind turbine towers [52] and storage tanks [53], for vibration suppression of cables [40], and for mitigation of vortex-induced vibration in long-span bridges [54]. A few earlier studies also suggested the use of TID [55] and TMDI [36,56] to suppress wind-induced vibration in high-rise buildings. Giaralis and Petrini [36] investigated wind-induced vibration mitigation of a 74-story benchmark building equipped with TMDI using a frequency-domain stochastic approach, based on empirical power spectral density (PSD) matrix of across-wind aerodynamic force [57]. Their results indicated that the TMDI reduced the peak top-floor acceleration more effectively than the TMD but employing smaller attached-mass values, especially for some selected topologies of installation. Additionally, they showed that the inclusion of the inerter dramatically reduced the TMD stroke. In this regard, it is worth noting that across-wind aerodynamic forces in high-rise buildings are induced by vortex shedding which highly rely on the actual cross section of the building [57]. Moreover, the empirical PSD of across-wind aerodynamic force adopted in [36] is not applicable when surrounding buildings exist, such that wind directions are not consistent with coordinates of structures.

This research work falls into the same research line as the previous papers, but it uses a different wind-excitation model underlying an alternative time-domain analysis perspective. More specifically, in this paper synchronous multi-point pressure measurements from wind tunnel tests of a scaled high-rise building of height 340 m are carried out. This allows the definition of a more appropriate set of aerodynamic forces that are consistent with the actual cross-section of the benchmark building and with the existing surrounding conditions. The time histories of aerodynamic forces at each story are determined along 24 different wind directions from 0° to 345° at an interval of 15°. Performance-oriented optimization of parameters of both the TMDI and the TID are carried out to find out the optimal parameters of corresponding vibration mitigation device to suppress wind-induced responses with an eye for practical aspects like frequency ratio, damping ratio and placement of TID/TMDI. Combining aerodynamic forces from wind tunnel tests, structural dynamic characteristics (mass and stiffness of the building) extracted from the finite element model of the primary structure and parameters of optimally-designed TMDI and TID, a time-domain mathematical model of the benchmark building is used in this study for analyzing the wind-induced responses under the assumption of linear elastic behaviors. The effects of TMDI and TID on wind-induced displacement and acceleration responses and on ESWLs are studied and compared with results of the benchmark building equipped with classical TMD that shares the same physical mass as the former two inerter-based vibration absorbers (the physical mass denotes the attached mass in the TMD case, and the sum of mass of inerter devices and attached mass in the inerter-based vibration absorbers).

2. Equations of Motion and Preliminary Concepts

According to the sketch in Figure 1, let us consider a high-rise building modeled as a lumped-mass system, equipped with a linear TMDI comprising an attached mass $m_t$ that is connected to the primary structure via linear spring and dashpot elements, $k_t$ and $c_t$, respectively. The TMDI mass is placed in series with one or several linear inerters (of inertance $b$ in sum) whose two terminals are denoted as 1 and 2, respectively. The first terminal 1 is connected to the attached mass $m_t$, while the second terminal 2 can be attached to another floor along the building height. The building is subject to a wind-induced excitation field represented by a set of forces acting at each floor of the discretized system. Adopting a standard matrix-vector notation, the equations of motion of this n-story lumped-mass system can be written in the following form

$$M\ddot{u}(t)+C\dot{u}(t)+Ku(t)=p(t)$$

(1)

where $\ddot{u}(t),\dot{u}(t),u(t)$ represent the acceleration, velocity, and displacement vectors, respectively, and $p(t)$ is the vector of the corresponding aerodynamic forces applied at the center of mass of each floor slab. All these vectors are (n + 1)-dimensional, as this is the total number of degrees of freedom (DOFs) of the n-story building equipped with TMDI. We assume all these vectors are augmented by one last (bottom) row containing the kinematic terms related to the TMDI DOF for $\ddot{u}(t),\dot{u}(t),u(t)$ and containing a zero entry for the aerodynamic force vector $p(t)$. Aerodynamic loading can be obtained from wind tunnel test measurements by synchronous multi-point scanning of pressures on the high-rise building model. In Equation (1), $M,C,K$ are the mass, damping and stiffness matrices of the TMDI-equipped structure, respectively. When the TMDI is installed at the $t^{\mathrm{th}}$ floor and has a so-called “$-p$” topology (meaning that the second terminal of the inerter is attached to a floor $t-p$), these matrices can be expressed as:

$$\begin{array}{l}M=M_s^{n+1}+\left(m_t+b\right)1_{n+1}{1}_{n+1}^T+b\hspace{0.17em}{1}_{t-p}{1}_{t-p}^T-b\left(1_{n+1}{1}_{t-p}^T+1_{t-p}{1}_{n+1}^T\right)\\ C=C_s^{n+1}+c_t\left(1_{n+1}{1}_{n+1}^T+1_t{1}_t^T-1_{n+1}{1}_t^T-1_t{1}_{n+1}^T\right)\\ K=K_s^{n+1}+k_t\left(1_{n+1}{1}_{n+1}^T+1_t{1}_t^T-1_{n+1}{1}_t^T-1_t{1}_{n+1}^T\right)\end{array}$$

(2)

where $M_s^{n+1},C_s^{n+1},K_s^{n+1}\in {ℝ}^{\left(n+1\right)\times \left(n+1\right)}$ represent the augmented mass, damping and stiffness matrices of the primary structure, respectively, constructed by adding one last (bottom) row with zero entries and one last (rightmost) column of zero entries in the original matrices $M_s,C_s,K_s\in {ℝ}^{n\times n}$. All the vectors $1_j\in {ℝ}^{\left(n+1\right)\times 1}$ are constructed such that only the $j^{\mathrm{th}}$ entry is equal to one while all the remaining entries are equal to zero (the superscript $T$ indicates transpose operator). The above equations also apply to a TMD-equipped structure (without inerter), which is retrieved by setting $b=0$ in the mass matrix. In the same way, a TID scheme as series-Parallel Layout 1 Inerter system proposed in [50] can be obtained by setting $m_t=0$ (without attached mass). Besides the ${(t-p)}^{\mathrm{th}}$ entry in the diagonal of the augmented mass matrix, the presence of the inerter modifies the mass matrix by introducing certain non-diagonal inertial coupling terms between the ${(n+1)}^{\mathrm{th}}$ DOF of the attached mass and the DOF of the ${(t-p)}^{\mathrm{th}}$ floor. Inerter topologies in which the inerter spans more than one story ($p>1$) may be realized with pendulum-like implementations like in the Taipei 101 skyscraper ($p=4$ for 87th floor to 91st floor). Such inerter topologies or installation configurations, earlier studied in [36], were found to achieve higher vibration control effectiveness. This was intuitively motivated by the fact that the motion of two non-consecutive floors is seemingly less correlated: therefore, the inerter is likely to undergo higher relative accelerations at its two terminals than if it were installed between two consecutive floors, thus experiencing higher engagement (larger forces for equal inertance value $b$).

By inspection of Equations (1) and (2) it is worth pointing out the following distinctive aspects in comparison with the earlier study by Giaralis and Petrini [36]: (i) The analysis is here conducted in the time domain since the aerodynamic forces are identified by wind tunnel tests of the benchmark building, whereas Giaralis and Petrini operated on a frequency-domain stochastic approach based on an empirical PSD for the across-wind force field; (ii) The formulation in Equation (2) slightly extends that developed by Giaralis and Petrini since the TMDI should not necessarily be placed at the topmost floor, but it can installed at a generic $t^{\mathrm{th}}$ floor. Although it is customary to attach the TMD at the top floor because of its widely recognized effectiveness to control the fundamental mode in multistory buildings [58], this installation configuration might be not feasible in some practical projects because of potential structural or architectural constraints. The installation of traditional TMD at different floors, not just at the topmost floor, was recently investigated by Elias and Matsagar [29]. For TMDI, Ruiz et al. [59] and Giaralis and Taflandis [48] also assessed the influence of installation floor on its performance, although they did not explicitly consider the installation floor as one of the variables to be optimized. Additionally, the inerter might be unable to exert its due performance because the relative peak acceleration does not always occur between the top floor and the ${(n-p)}^{\mathrm{th}}$ floor. This is why in this study we have directly set the installation floor as one of the design variables of the inerter-based vibration absorber within parametric optimizations, which represents another novel aspect in comparison with definitions of optimization problem in previous literature studies.

The TMDI-equipped structure may have non-proportional damping. Therefore, it is convenient to transform Equation (1) into state space variable form that is more suitable for complex modal analysis

$$A\dot{z}\left(t\right)+Bz\left(t\right)=f\left(t\right)$$

(3)

where $z\left(t\right)={[u(t),\dot{u}(t)]}^T$ is the state space variable vector and the matrices $A,B$ and the vector $f(t)$ are expressed as

$$A=\left[\begin{array}{cc}C& M\\ M& 0\end{array}\right],\hspace{0.17em}B=\left[\begin{array}{cc}K& 0\\ 0& -M\end{array}\right],\hspace{0.17em}f\left(t\right)=\left[\begin{array}{c}p\left(t\right)\\ 0\end{array}\right].$$

(4)

From Equations (3) and (4), after performing complex modal analysis it is possible to determine the transfer function of the system response to assess wind-induced response mitigation induced by the TMD\TMDI\TID. In particular, the transfer function of the displacement and acceleration response at the $p^{\mathrm{th}}$ DOF induced by forces at the $q^{\mathrm{th}}$ DOF can be expressed in the following forms, respectively

$$\begin{array}{l}{H}_D^{{}_{pq}}\left(j\omega \right)={\displaystyle \sum _{k=1}^{n+1}\left(\frac{{\phi }_{pk}{\phi }_{qk}}{a_k\left(j\omega -s_k\right)}+\frac{{\phi }_{pk}^{*}{\phi }_{qk}^{*}}{a_k^{*}\left(j\omega -s_k^{*}\right)}\right)}\\ H_A^{{}_{pq}}\left(j\omega \right)={\displaystyle \sum _{k=1}^{n+1}\left(\frac{-{\omega }^2{\phi }_{pk}{\phi }_{qk}}{a_k\left(j\omega -s_k\right)}+\frac{-{\omega }^2{\phi }_{pk}^{*}{\phi }_{qk}^{*}}{a_k^{*}\left(j\omega -s_k^{*}\right)}\right)}\end{array}$$

(5)

where ${\phi }_{pk}$, ${\phi }_{qk}$ are the values of the $p^{th}$ and $q^{th}$ degree of freedom, respectively, in the $k^{\mathrm{th}}$ complex mode shape, while ${\phi }_{pk}^{*}$ and ${\phi }_{qk}^{*}$ represent the conjugate values of ${\phi }_{pk}$ and ${\phi }_{qk}$, respectively. In Equation (5) $s_k$ and $s_k^{*}$ denote the complex eigenvalue and its conjugate value, respectively, while $a_k$ and $a_k^{*}$ are coefficients determined as follows

$${\Phi }^{T}A\Phi =\mathrm{diag}\left\{\begin{array}{cccccc}{a}_1& \cdots & a_k\begin{array}{cc}\cdots & a_{n+1}\end{array}& a_1^{*}& \cdots & a_k^{*}\begin{array}{cc}\cdots & a_{n+1}^{*}\end{array}\end{array}\right\}$$

(6)

where $\Phi$ is the modal shape matrix collecting the eigenvectors ${\mathbf{\varphi }}_i$. According to the complex mode superposition approach, the state space vector response $z\left(t\right)$ (under the assumption of zero initial conditions) can be expressed as

$$\begin{array}{c}z\left(t\right)={\displaystyle \sum _{i=1}^{2n+2}{\mathbf{\varphi }}_i\hspace{0.17em}{q}_i\left(t\right)}\\ z\left(t\right)={\displaystyle \sum _{i=1}^{n+1}\left(\frac{{\mathbf{\varphi }}_i}{a_i}{\displaystyle \underset{0}{\overset{t}{\int }}{F}_i\left(\tau \right)e^{s_i\left(t-\tau \right)}}\mathrm{d}\tau +\frac{{\mathbf{\varphi }}_i^{*}}{a_i^{*}}{\displaystyle \underset{0}{\overset{t}{\int }}{F}_i^{*}\left(\tau \right)e^{s_i^{*}\left(t-\tau \right)}}\mathrm{d}\tau \right)}\end{array}$$

(7)

where ${\mathbf{\varphi }}_{\hspace{0.17em}i}$ and ${\mathbf{\varphi }}_{\hspace{0.17em}i}^{*}$ represent the $i^{\mathrm{th}}$ mode shape and its conjugate mode, respectively. The $i^{\mathrm{th}}$ generalized force and its conjugate are expressed as

$$\begin{array}{l}{F}_i\left(t\right)={\mathbf{\varphi }}_i{}^{T}f\left(t\right)\\ F_i^{*}\left(t\right)={\mathbf{\varphi }}_i^{*}{}^{T}f\left(t\right)\end{array}$$

(8)

Once the wind-induced displacement responses are calculated, they are utilized to predict equivalent static wind loads by the method of Displacement Gust Loads Factor (DGLF) [60]:

$$F_{eswl}(z)=G(z)\overline{P}(z)$$

(9)

where $\overline{P}(z)$ is the mean wind load, which can be obtained by pressure measurements from wind tunnel test, and $G(z)$ is the DGLF, which considers effects of structural dynamic characteristics on response. In the DGLF method, $G(z)$ is evaluated in terms of the expected extreme and mean displacement:

$$G(z)=\frac{\widehat{D}(z)}{\overline{D}(z)}$$

(10)

where $\overline{D}(z)$ and $\widehat{D}(z)$ are the mean and expected extreme displacement, respectively, at the structural height $z$. The expected values of extreme displacement and acceleration used are $\widehat{D}(z)={\mu }_{dis}+g{\sigma }_{dis}$ and ${\widehat{D}}_{acc}(z)=g{\sigma }_{acc}$, respectively, where $g=3.5$ is the peak factor estimated from the widely used empirical formula given by Davenport [61].

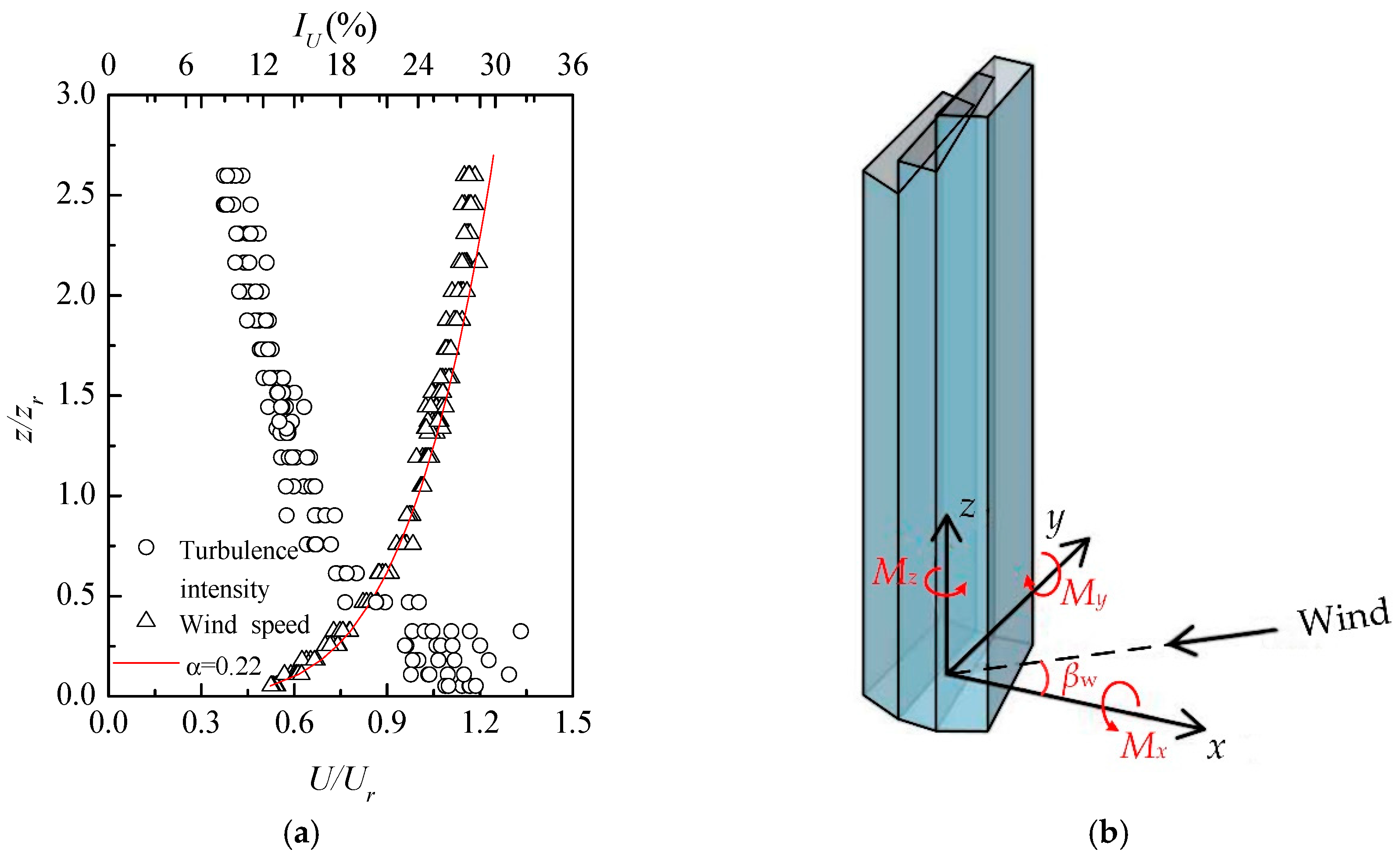
3. Description of the 340 m Tall Building and Wind Tunnel Testing

The primary structure has 69 stories and total height of 340 m. This benchmark building represents the Qiaokou tower, located in Wuhan City, China, built in 2012. The photograph of the building and the main peculiarities of its structural configuration are presented in Figure 2.

The mass distribution of the primary structure, including dead load and live load, has been extracted from the finite element model of the building and is reported in Figure 3a. Similarly, the distribution of the lateral stiffness along the x-axis, which is much smaller than that along the y-axis, has been extracted from the finite element model as well, and is presented in Figure 3b. Mass and stiffness matrices can be established in terms of mass and lateral stiffness distributions. It is worth noting that the developed model of the case study building accounts for the primary structural elements only. The presence of secondary structural components (here not considered for simplicity) could slightly modify the results in terms of interstory displacements and could add some stiffening contributions in the overall building model.

The full damping matrix $C_{\mathrm{s}}$ of the original structure (without vibration absorber) has been calculated from the modal damping matrix $C_{\mathrm{mod}}$ [62]:

$$C_s={\left({\Phi }^T\right)}^{-1}{C}_{\mathrm{mod}}{\left(\Phi \right)}^{-1}$$

(11)

where $\Phi$ is the modal shape matrix of the original structure. The modal damping matrix $C_{\mathrm{mod}}\in {ℝ}^{n\times n}$ is a diagonal matrix collecting the modal damping ratios and can be calculated as follows

$$C_{\mathrm{mod}}\left(k,k\right)=2{\xi }_k{\omega }_k\left({\phi }_k^T{M}_s{\phi }_k\right);\hspace{1em}k=1,2,\dots ,69$$

(12)

where ${\omega }_k$,${\phi }_k$ are the kth natural frequency and vibration mode, respectively. The kth modal damping ratio of the system ${\xi }_k$ is taken equal to 1% for k = 1, 2, 3; 4% for k = 4, 5, 6; 6% for k = 7, 8, 9, 10; 9% for k = 11, 12, …, 20; 12% for k = 21, 22, …, 40; 15% for k = 41, 42, …, 60; 18% for k = 61, 62, …, 69. These values were selected based on available field-recorded of high-rise steel framed buildings in the [0–7] Hz frequency range [63]. The main dynamic parameters of the primary structure are listed in

Table 1. Main dynamic parameters of the primary structure.

Total Mass $\mathit{M}$	First-Order Natural Frequency along x-axis ${\mathit{\omega }}_1$	First-Order Generalized Mass	First-Order Damping Ratio (Assumed)
231,659 t	0.176 Hz	61,287 t	1%

.

The synchronous multi-point pressure tests of the building with existing surrounding conditions were performed in boundary layer wind tunnel tests (shown in Figure 4) under a simulated C type wind field corresponding to China load code for the design of building structures [64], which reflects the characteristics of the wind field in urban areas. The profiles of mean wind speed and turbulence intensity are shown in Figure 5a. The reference coordinates of the wind tunnel test and wind-induced response analysis are shown in Figure 5b. 24 wind directions are considered in this study, which are identified by a ${\beta }_{\mathrm{w}}$ angle (between wind axis and x-axis) ranging from 0° to 345° at an interval of 15°.

When the wind direction ${\beta }_{\mathrm{w}}$ is 0° and 90°, the wind is blowing from the positive direction of the x and y axes, respectively. The parameters of wind tunnel tests are listed in

Table 2. Wind tunnel test parameters.

Geometric Scale	Wind Speed	Sampling Frequency	Sampling Length	Incremental Step	Measuring Taps
1:350	12 m/s	312.5 Hz	20,480	15°	471

. By properly scaling wind tunnel test results, the wind pressure coefficients firstly were transferred into wind aerodynamic pressure on the full-scale building, and then aerodynamic pressure in the prototype building was integrated at the base of the tributary area of each pressure tap to obtain the aerodynamic force component of each floor along the x-axis.

In Figure 5a, $\alpha$ (the exponent of power law formulation for vertical mean wind profile) corresponding to C type wind field is 0.22 in China load code for the design of building structures [64], $I_U$ represents the turbulence intensity, $U$ is the wind speed, and $U_r$ is the wind speed at the reference height.

Samples of aerodynamic force time histories acting on two stories (30th and 50th) along the x-axis are depicted in Figure 6 corresponding to 90° wind direction and wind velocity equal to 42.02 m/s. Figure 6 indicates that mean wind loads approach to zero, which is expected for across-wind aerodynamic forces mainly induced by vortex shedding.

4. Wind-Induced Response Mitigation Using Inerter-Based Vibration Absorbers

4.1. Optimization of the Parameters of the TMDI/TID Scheme Applied to the Benchmark Building

To compare the vibration mitigation effect of optimal TMD, TMDI and TID, performance-based optimizations for parameters of TMDI and TID were conducted to obtain the best TMDI/TID scheme. At the same time, the influence of variations of parameters in preset intervals on the vibration mitigation effect is also investigated.

In the mathematical model of TMD/TMDI/TID-equipped structure as described in Equations (1) and (2), vibration absorbers are introduced to control the wind-induced response along the x-axis, which is the most critical direction due to the lower lateral stiffness of the building (higher oscillations are expected). As the largest displacement and acceleration responses at the top floor of primary structure occurs at wind direction of 90°, the peak displacement and acceleration at the top floor induced by aerodynamic forces at this wind direction are selected to be the two individual objective functions.

From Equation (2), there are totally six parameters of TMDI, i.e., $\mu$, $\beta$, $\upsilon$, $\zeta$, $p$ and $t$, that need to be fixed to calculate the wind-induced responses of TMDI-equipped structure (5 parameters for TID). The two mass related notation, i.e., mass ratio and inertance ratio, are defined as $\mu =m_t/M$ and $\beta =b/M$, respectively. The frequency ratio is defined as:

$$\upsilon =\frac{{\omega }_t}{{\omega }_1}=\sqrt{\frac{k_t}{\left(m_t+b\right)}}/{\omega }_1$$

(13)

where ${\omega }_t$ is the circular frequency of TMD, TMDI or TID, and ${\omega }_1$ is the first order circular frequency of the primary structure. The damping ratio is defined as:

$$\zeta =\frac{c_t}{2\sqrt{\left(m_t+b\right)k_t}}$$

(14)

The other two discrete parameters are the topologies of inerter and the installation floor of vibration absorber as denoted in Equation (2).

To shed light on the better vibration mitigation effect of inerter-based vibration absorbers, the physical mass ratio ${\mu }_{phy}$ of TMD, TMDI and TID are defined below and fixed to the same value equal to 0.5%:

$${\mu }_{phy}=\mu +\beta /200=m_{\mathrm{TMD}, \mathrm{TMDI} \mathrm{or} \mathrm{TID}}/M=0.5\%$$

(15)

In the above-introduced physical mass ratio, not only the physical mass of the TMD (named as the attached mass), but also the physical mass of the inerter device is taken into consideration in the TMDI and TID schemes. Indeed, when dealing with large values of inertance (apparent mass of the inerter) in the order of tons, which might be the case for high-rise buildings, the physical mass of the inerter turns out to be non-negligible, whereas the majority of the literature studies ignored this term. In Equation (15), the physical mass ratio is constrained to be 0.5% based on the same threshold of TMD mass ratio proposed in [36], and the ratio of the inertance coefficient and physical mass of inertance devices is assumed to be 200 following the previous research about the “mass-amplification” effect of inerter [65]. Therefore, the mass-related parameter of the three vibration absorbers can be determined, i.e., TMD (μ = 0.5%), TMDI (μ = 0.25%, β = 50%) and TID (β = 100%). This makes it possible to compare three different configuration schemes sharing a common physical mass ratio for wind-induced response mitigation of the benchmark building. According to the conclusion from previous research that inerter devices spanning more stories lead to a better mitigation effect of the TMDI, the value of the topologies is determined to be p = 4 in the optimization procedure, based on practical considerations like in the pendulum-like TMD scheme implemented in the Taipei 101 (spanning from 87th floor to 91st floor).

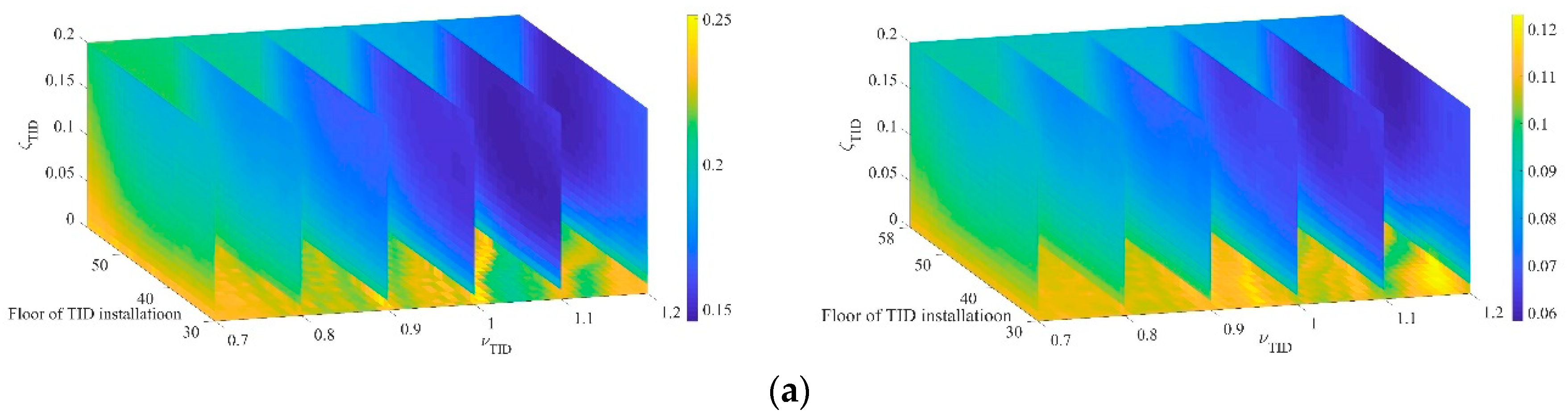
4.1.1. Optimization of Parameters of TMDI

As stated above, the displacement- and acceleration-based optimization of the three parameters, i.e., frequency ratio, damping ratio and floor of installation, can be expressed as Equations (16) and (17), respectively. The preset intervals of three parameters are determined based on practical considerations and results of previous researches [35,50]

$$\{\begin{array}{l}\mathrm{minimize}\hspace{1em}{f}_1\left(\upsilon ,\zeta ,t\right)={\widehat{D}}_{dis}\hfill \\ \begin{array}{l}s.t.\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\mu =0.25\%,\beta =50\%,-p=-4,\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\upsilon \in \left[0.7,1.2\right],\zeta \in \left[0,20\%\right],\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}t\in \left[30,58\right]\end{array}\hfill \end{array}$$

(16)

$$\{\begin{array}{l}\mathrm{minimize}\hspace{1em}{f}_2\left(\upsilon ,\zeta ,t\right)={\widehat{D}}_{acc}\hfill \\ \begin{array}{l}s.t.\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\mu =0.25\%,\beta =50\%,-p=-4,\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}\upsilon \in \left[0.7,1.2\right],\zeta \in \left[0,20\%\right],\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}t\in \left[30,58\right]\end{array}\hfill \end{array}$$

(17)

where ${\widehat{D}}_{dis}$ and ${\widehat{D}}_{acc}$ are the peak displacement and acceleration at the top floor at wind direction of 90°. It is worth noting that the installation floor t represents an explicit design variable of the constrained optimization problem stated in Equations (16) and (17).

For such an optimization of three variables, i.e., $\upsilon$, $\zeta$ and $t$, a three-dimensional space representation is proposed where the three variables are set to be the orthogonal axes and the value of corresponding object (peak responses) is expressed by different colors. To present a clear vision of the distributions of the colors in a 3D space, sliced contours from three aspects were plotted as shown in Figure 7.

From the sliced contour shown in Figure 7, the cool-color area represents the most efficient combination of parameters, leading to the minimum value of the two specific response indicators. The following conclusions can be drawn:

• For TMDIs which efficiently mitigate the wind-induced displacement responses, the optimal frequency ratio lies around 1.1, which indicates that the frequency of optimal TMDI is close to the first order frequency of the primary structure;
• The minimal peak displacement and acceleration are achieved when the damping ratios are 7% and 10%, respectively;
• As for the optimal floor of installation of TMDI, it can be seen that the best vibration mitigation effect is achieved when the TMDI is installed at the middle-upper portion of the benchmark building (around 44th floor), and not in the conventional configuration of TMD, i.e., at the topmost floor;
• For acceleration mitigation purpose, the optimal frequency ratio and installation floor of TMDI is slightly larger than that of displacement-oriented optimization. Such differences may be justified in view of the fact that the transfer function of acceleration is $-{\omega }^2$ times that of displacement, which means that a better mitigation effect of acceleration can be realized by decreasing the value of transfer function at higher frequency around the first peak under the same fluctuating wind excitations.

Through the optimization results of TMDI at wind direction of 90° (the most adverse conditions), a set of optimal tuning parameters of TMDI with good trade-off between displacement mitigation and acceleration mitigation was selected by approximately averaging the two corresponding parameters due to the smooth gradient between two optimal schemes of TMDI (as shown in Figure 8). The notations ${\mathrm{Dis}}_{\min }$ and ${\mathrm{Acc}}_{\min }$ represent two configurations of TMDI that achieve the best displacement and acceleration mitigation effect, respectively.

4.1.2. Optimization of Parameters of TID

Similar to the configurations of the optimization in Section 4.1.1, the optimization of parameters of TID for mitigating peak displacement and acceleration at top floor can be expressed as Equations (18) and (19), respectively.

$$\{\begin{array}{l}\mathrm{minimize}\hspace{1em}{f}_1\left(\upsilon ,\zeta ,t\right)={\widehat{D}}_{dis}\hfill \\ \begin{array}{l}s.t.\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\beta =100\%,-p=-4,\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}\upsilon \in \left[0.7,1.2\right],\zeta \in \left[0,20\%\right],\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}t\in \left[30,58\right]\end{array}\hfill \end{array}$$

(18)

$$\{\begin{array}{l}\mathrm{minimize}\hspace{1em}{f}_2\left(\upsilon ,\zeta ,t\right)={\widehat{D}}_{acc}\hfill \\ \begin{array}{l}s.t.\hspace{1em}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\beta =100\%,-p=-4,\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}\upsilon \in \left[0.7,1.2\right],\zeta \in \left[0,20\%\right],\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{0.17em}t\in \left[30,58\right]\end{array}\hfill \end{array}$$

(19)

The results of optimization of TID in $\upsilon -\zeta -t$ space are displayed in Figure 9:

The similar trends of the distribution of the parameters of TID as that of TMDI can be observed by comparing the Figure 7 and Figure 9. For TID having inertance ratio of 100%, which is twice that of TMDI, the highest reduction of displacement and acceleration responses is achieved when the damping ratios are 12% and 19%, respectively, which are almost twice those of TMDI. The inerter devices in TID scheme produce larger inertia, which corresponds to a better ability to store energy, thus the corresponding requirement for dissipating rate of energy stored in both inerter and attached mass increases at the same time. Based on the same considerations of determining the optimal parameters of TMDI as shown in Figure 10, the configuration of the optimally-designed TID is determined and listed in

Table 3. Design parameters of Tuned Mass Damper (TMD), Tuned Mass-Damper-Inerter (TMDI) and Tuned Inerter Damper (TID) used for the comparative study.

Parameters	TMD	TMDI	TID
TMD installation floor	58th floor	45th floor	45th floor
Effective mass ratio ${\mu }_{eff}=\mu +\beta$	0.5%	50.5%	100%
Physical mass ratio ${\mu }_{phy}=\mu +\beta /200$	0.5%	0.5%	0.5%
Mass ratio $\mu$	0.5%	0.25%	\
Inertance ratio $\beta$	\	50%	100%
Frequency ratio $\upsilon$	0.99	1.07	1.15
Damping ratio $\zeta$	7%	9%	16%
TMDI topology −p	\	−4	−4

. Slightly different from the result of that of TMDI, the point inside the orange square, which represents the selected configuration of TID, lies close to the dashed line due to the limitation of discrete parameter, i.e., floor of TMDI installation.

Apart from the two inerter-based vibration absorbers, the optimal parameters of TMD with fixed mass ratio equal to 0.5% (and, thus, equal physical mass ratio to that of the TMDI and TID) are determined by performing the same optimization procedure.

As stated above, the optimal parameters of TMD, TMDI and TID in a comparison group are listed in Table 3. It can be seen that the TMDI and TID scheme benefit a lot from the inerter system on the effective mass.

Once the optimal parameters of the TMD/TMDI/TMD are selected, the corresponding mass, damping and stiffness matrices can be determined according to Equation (2). Therefore, the transfer function of displacement and acceleration response as per Equation (5) can be computed to assess the effects of TMD/TMDI/TID in mitigating the wind-induced response. Figure 11 and Figure 12 present the modulus of the transfer function of displacement and acceleration responses, respectively, at the 69th story (top floor) of the benchmark 340 m tall building subject to the aerodynamic forces consistent with the wind tunnel test measurements.

In Figure 11, the first peaks of displacement transfer function of the original structure, structure with TMD, TMDI and TID appear around the first natural frequency (1.10 rad/s, consistent with 0.176 Hz reported in Table 1). The peak of the original structure (in dark cyan) is much higher than that of three other vibration absorber equipped structures, i.e., TMD-equipped structure (in blue), TMDI-equipped structure (in orange) and the TID-equipped structure (in red). Overall, Figure 11 indicates that TMD, TMDI and TID mitigate the displacement response corresponding to the first vibration mode, and the mitigation effects of the TID are better than that of TMD and TMDI whose physical mass ratios are the same as that of TID. Around the second and third natural frequencies, the transfer function of the original structure overlaps with that of structure with TMD, while the transfer function of the structure with TMDI is slightly lower, and the TID achieves the best mitigation effect. For higher natural frequencies, transfer functions of the four cases are almost identical.

In Figure 12, the highest peak of the acceleration transfer function is observed around the third natural frequency. All three vibration absorbers efficiently suppress (in a comparable manner) wind-induced acceleration response corresponding to the first natural frequency with the optimal frequency ratio between 0.99 and 1.15. These graphs demonstrate the advantages of the inerter-based vibration absorbers in achieving a considerable wind-induced vibration mitigation in comparison to the TMD by employing the same physical mass ratio.

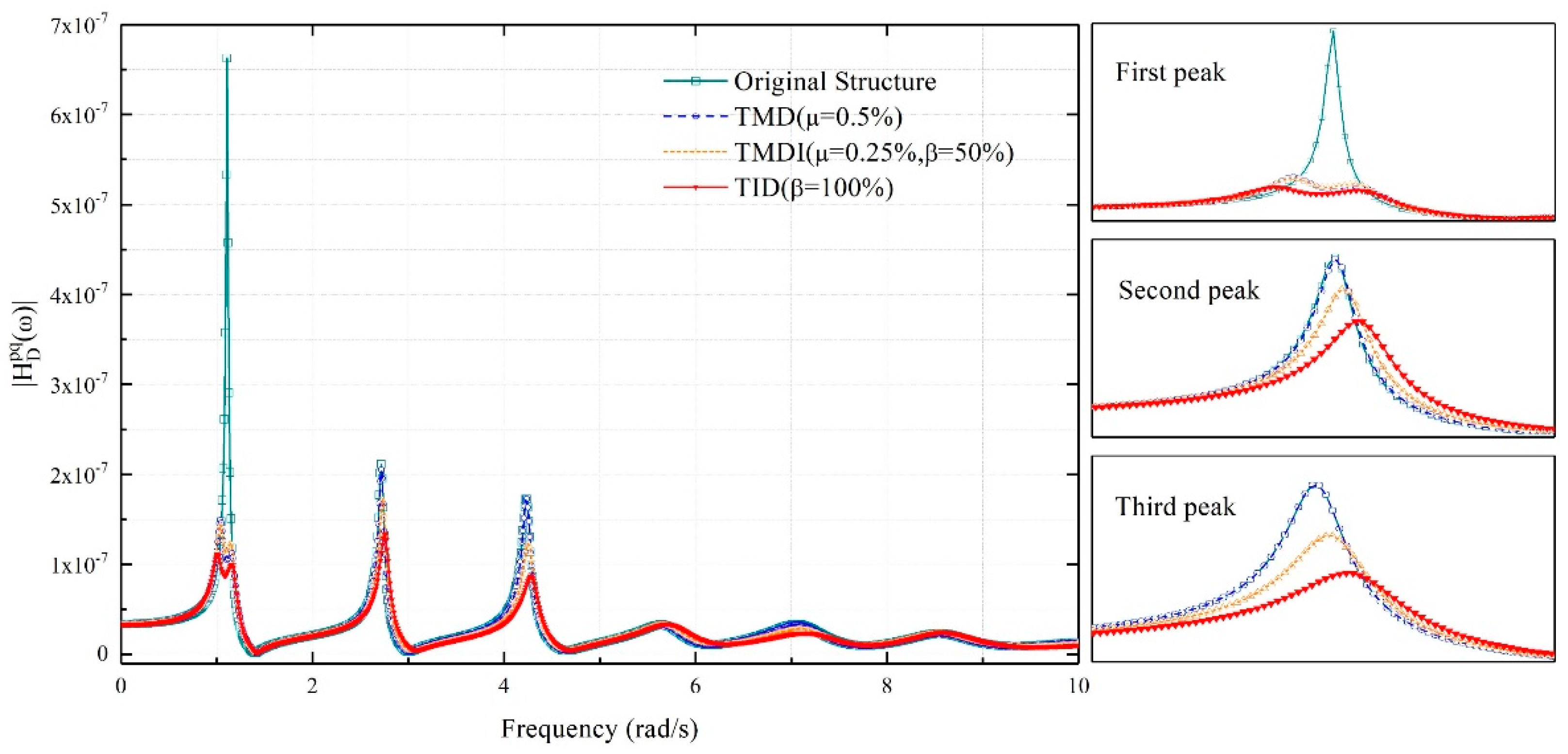
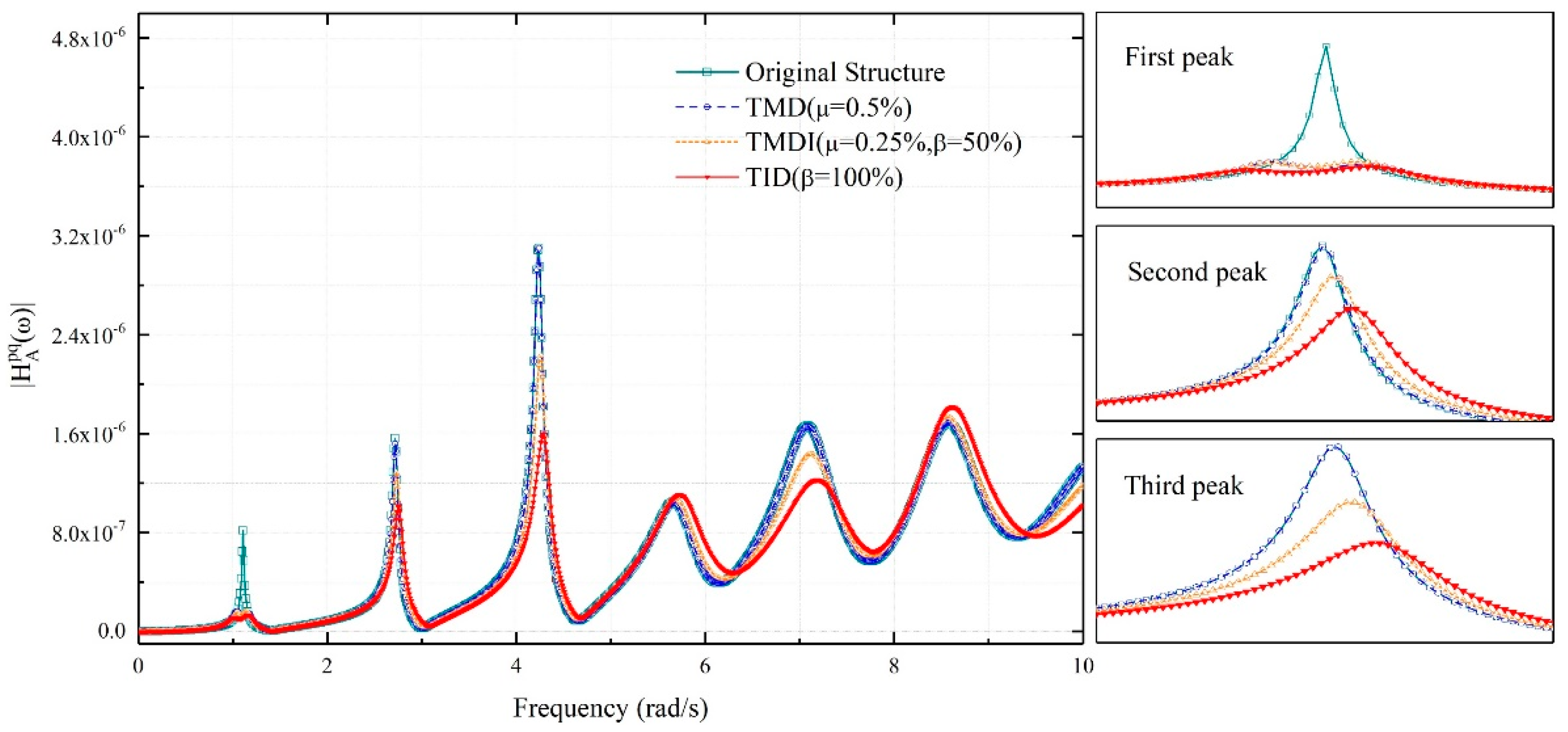
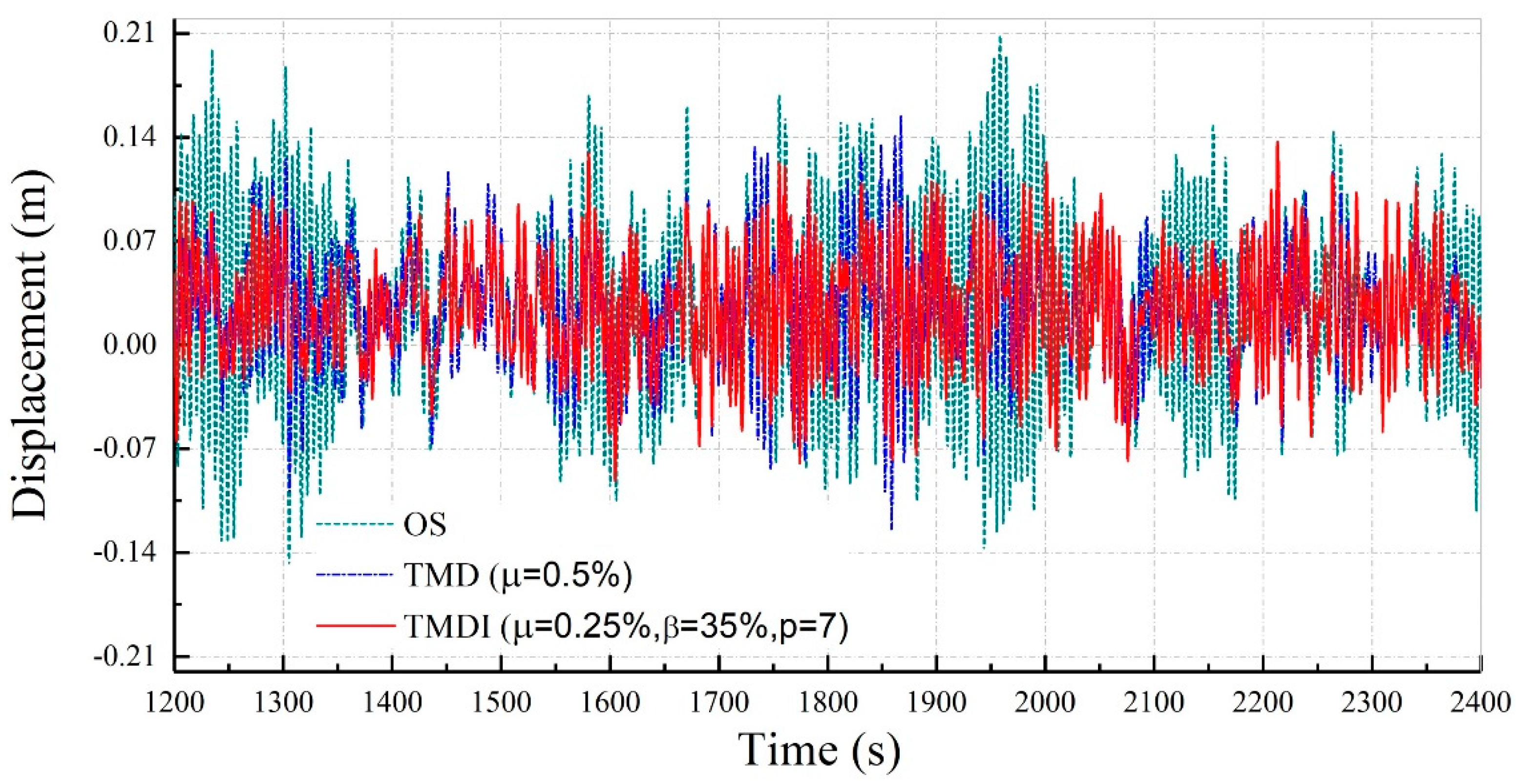
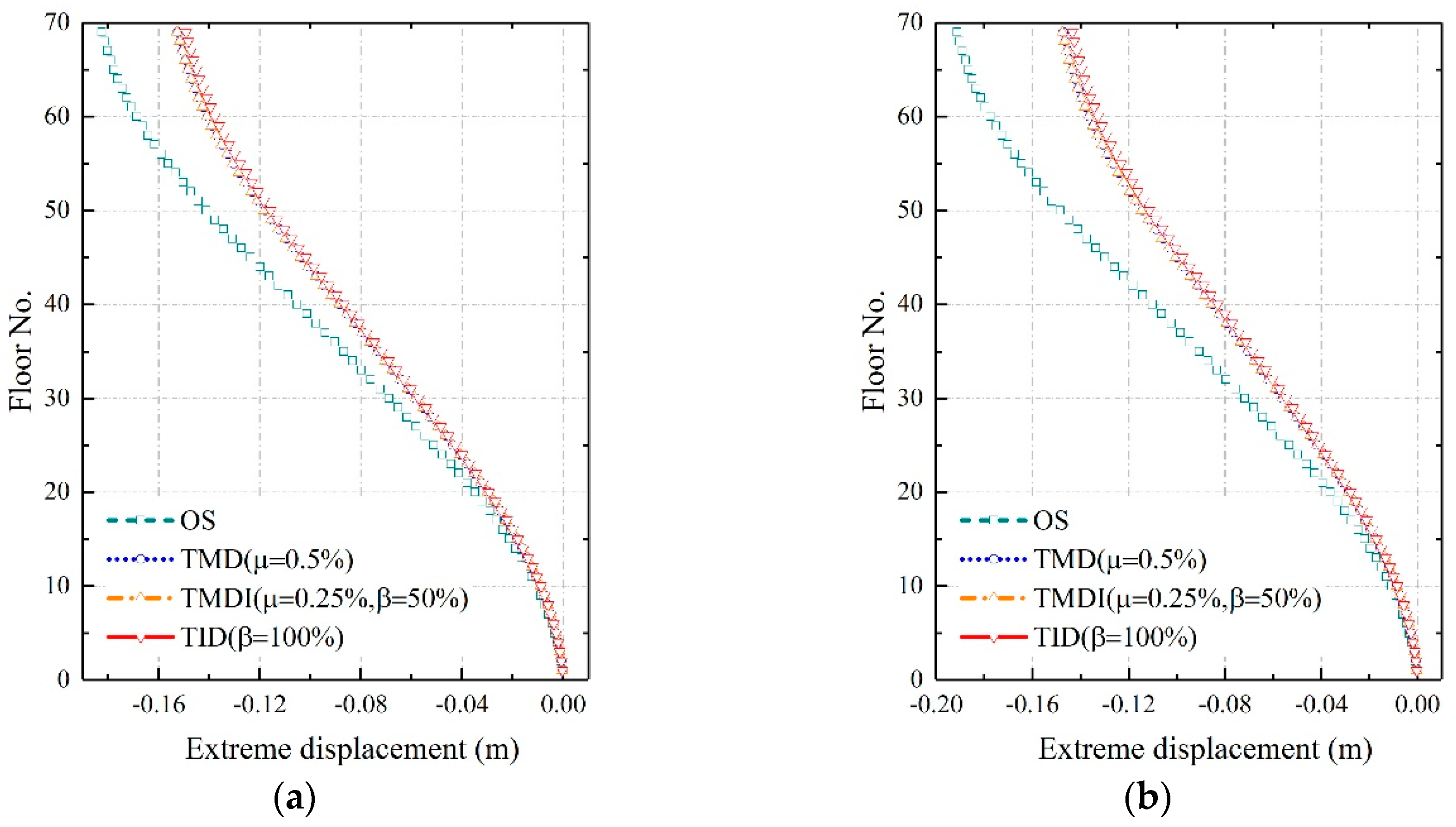
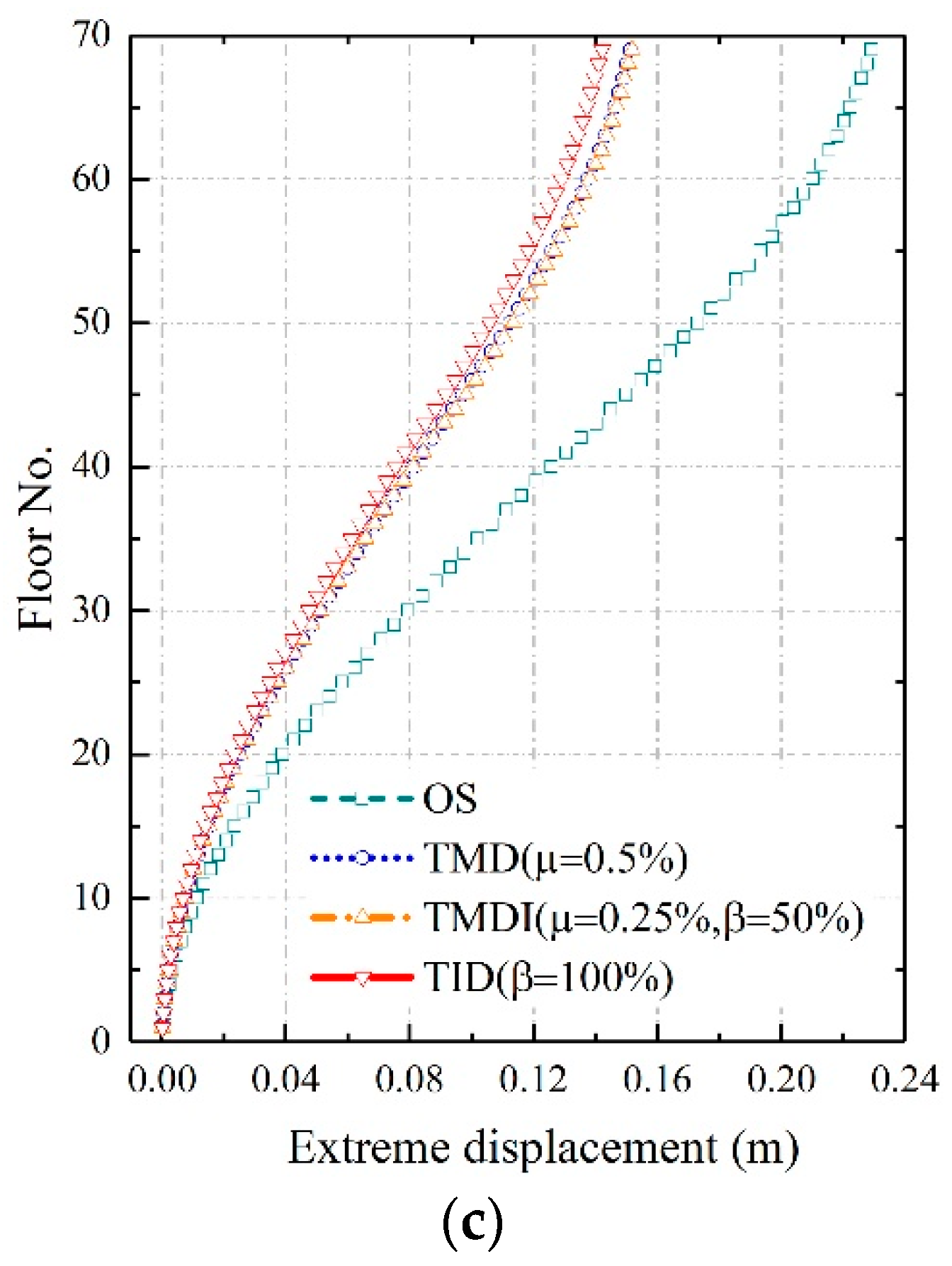
4.2. Effects of the Inerter-Based Vibration Absorbers on Wind-Induced Displacements

After setting the parameters of the TMDI and TID systems, the ${\rm M},C,K$ matrices can be calculated by using Equation (2), respectively, hence the time histories of wind-induced displacements of the TMDI- and TID-equipped benchmark buildings can be analyzed corresponding to wind speed of 40.07 m/s (50-years return period stipulated by survivability limit state design) for each of the 24 wind directions from 0° to 345° according to Equation (8). Figure 13 represents a segment of the displacement time-history response (for an overall duration of 20 min) at the 69th story corresponding to 90° wind direction.

Extreme wind-induced displacement response can be evaluated in terms of mean and Root Mean Square (RMS) value for each of the 24 wind directions considered in this study. Figure 14 presents variation of extreme displacement responses (as per Equation (10)) along the building height corresponding to three typical wind directions (0°, 45° and 90°). By inspection of Figure 14, it appears clear that TMD, TMDI and TID significantly decrease the wind-induced extreme displacement responses. The mitigation effects of TID are slightly better than that of TMD and TMDI. This demonstrates that the inerter plays a significant role in the vibration mitigation of the structure.

Figure 15 shows the variation of mean and extreme top-floor displacements corresponding to a variety of wind directions ranging from 0° to 345°. The maximum absolute displacement is smaller than 1/1500 of the height of the benchmark building, which justifies the linear elastic behavior assumption made in this paper for the building dynamic model. Figure 15 indicates that three vibration absorbers have no effects on mean displacement responses, which in fact coincide with those of the OS. The mean displacement responses approach zero for wind direction corresponding to 90° and 270° wind-direction, because this corresponds to the across-wind response induced by the vortices shedding at both edges of the windward side. For any other wind direction, TMD, TMDI and TID significantly suppress wind-induced top-floor displacement responses along the x-axis. Based on Figure 13, Figure 14 and Figure 15, it can be concluded that TID with same physical mass ratio achieved a slightly better vibration mitigation effect to the TMD and TMDI in terms of displacement response.

To quantify the mitigation effect of TMD, TMDI and TID, a factor of vibration-absorbing $F_{va}$ is defined as follows

$$F_{va}(\%)=\left|\frac{R_{\mathrm{OS}}-\hspace{0.17em}{R}_{\mathrm{T}}}{R_{\mathrm{OS}}}\right|\times 100$$

(20)

where $R_{\mathrm{OS}}$ represents the response of the original structure, while $R_{\mathrm{T}}$ denotes the corresponding response indicator for the structure with TMD\TMDI\TID. Some relevant results for a few emblematic wind directions and loading conditions are listed in

Table 4. Vibration-mitigation effects in terms of top-floor displacement response for some loading configurations.

Selected Condition	Wind Direction (°)	${\mathit{u}}_{\mathbf{OS}}^{\mathit{t}\mathit{o}\mathit{p}}\left(\mathbf{m}\right)$	${\mathit{u}}_{\mathbf{TMD}}^{\mathit{t}\mathit{o}\mathit{p}}\left(\mathbf{m}\right)$	${\mathit{u}}_{\mathbf{TMDI}}^{\mathit{t}\mathit{o}\mathit{p}}\left(\mathbf{m}\right)$	${\mathit{u}}_{\mathbf{TID}}^{\mathit{t}\mathit{o}\mathit{p}}\left(\mathbf{m}\right)$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TMD}\right)}$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TMDI}\right)}$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TID}\right)}$
Minimum $F_{va}$ (TMDI)	330	−0.1608	−0.1438	−0.1438	−0.1416	10.56	10.52	11.94
Maximum $F_{va}$ (TMDI)	90	0.2293	0.1513	0.1519	0.1421	34.01	33.74	38.02
Minimum $F_{va}$ (TID)	330	−0.1608	−0.1438	−0.1438	−0.1416	10.56	10.52	11.94
Maximum $F_{va}$ (TID)	90	0.2293	0.1513	0.1519	0.1421	34.01	33.74	38.02
Max positive displacement OS	90	0.2293	0.1560	0.1519	0.1421	34.01	33.74	38.02
Max negative displacement OS	75	−0.2130	−0.1476	−0.1482	−0.1404	30.71	30.44	34.07

. In particular, Table 4 presents the vibration-absorbing factor for some typical conditions. For example, for wind direction of 90° the TID has shown the best vibration mitigation effect among the three vibration absorbers: the $F_{va}$ is equal to 38.02% for the TID, 34.01% for the TMD and 33.74% for the TMDI. The worst vibration-absorbing effect of TID takes place for 330° wind direction, where the $F_{va}$ with TID is only 11.94% and $F_{va}$ with TMD and TMDI are only 10.56% and 10.52%, respectively. The two inerter-based vibration absorbers share a similar variation (vary synchronously) against wind excitations at different directions as it can be observed from the curvilinear shapes of both TMDI and TID in Figure 15. This leads to the result that the 1st and the 3rd, the 2nd and the 4th rows are identical. Generally, structural engineers are mainly concerned about the maximum absolute value of extreme displacement. For example, in this study, the maximum positive extreme top-floor displacement of the original structure is 0.23 m corresponding to 90° wind direction. The positive extreme displacement decreases from 0.23 m to around 0.14 m when the TID is installed on the original structure. The negative extreme top-floor displacement occurs for 75° wind direction. The displacement drops from −0.21 m to −0.14 m when the TID is used to mitigate the wind-induced vibration of the primary structure. The corresponding factor of vibration-absorbing is 34.07%.

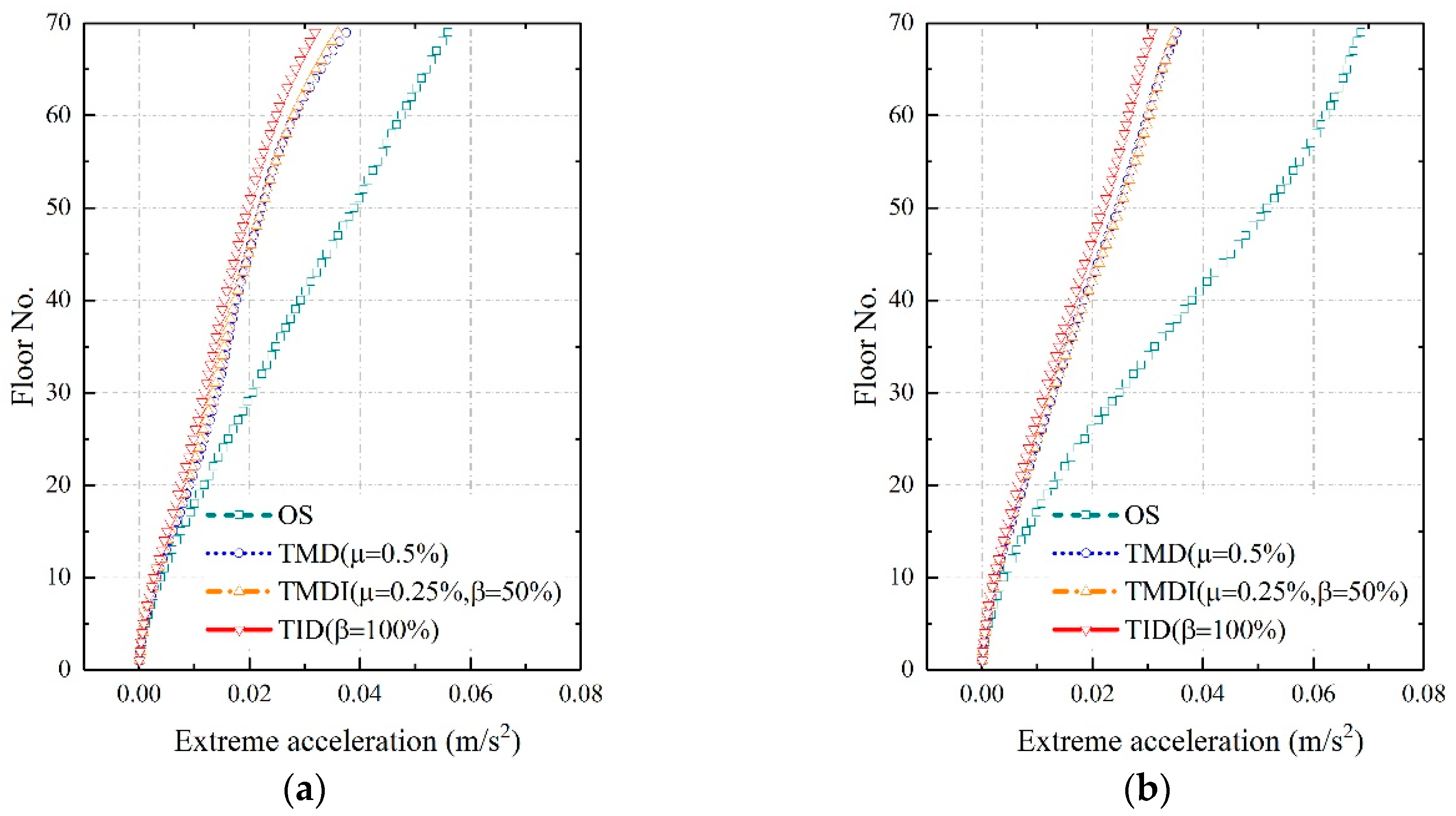
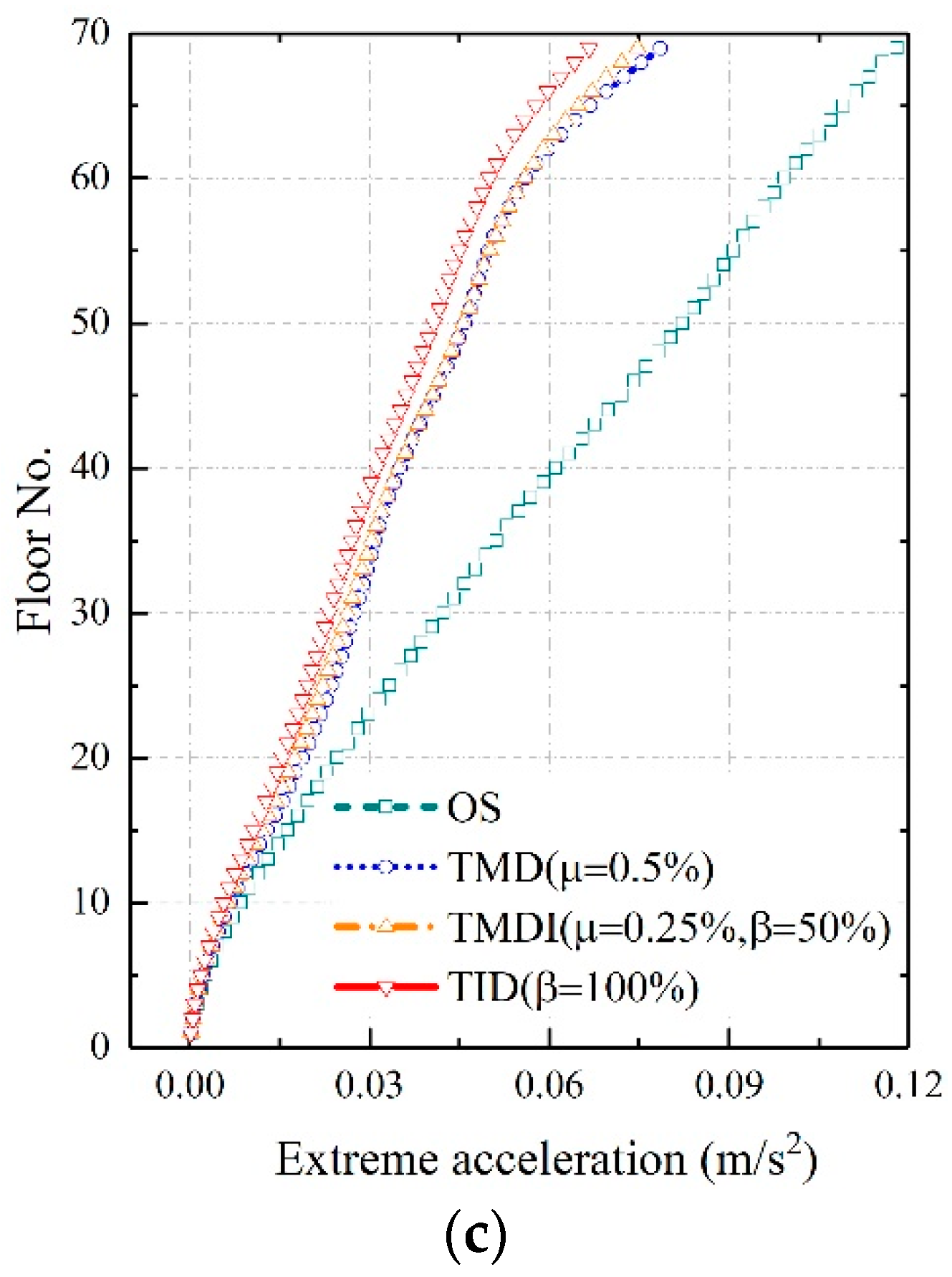
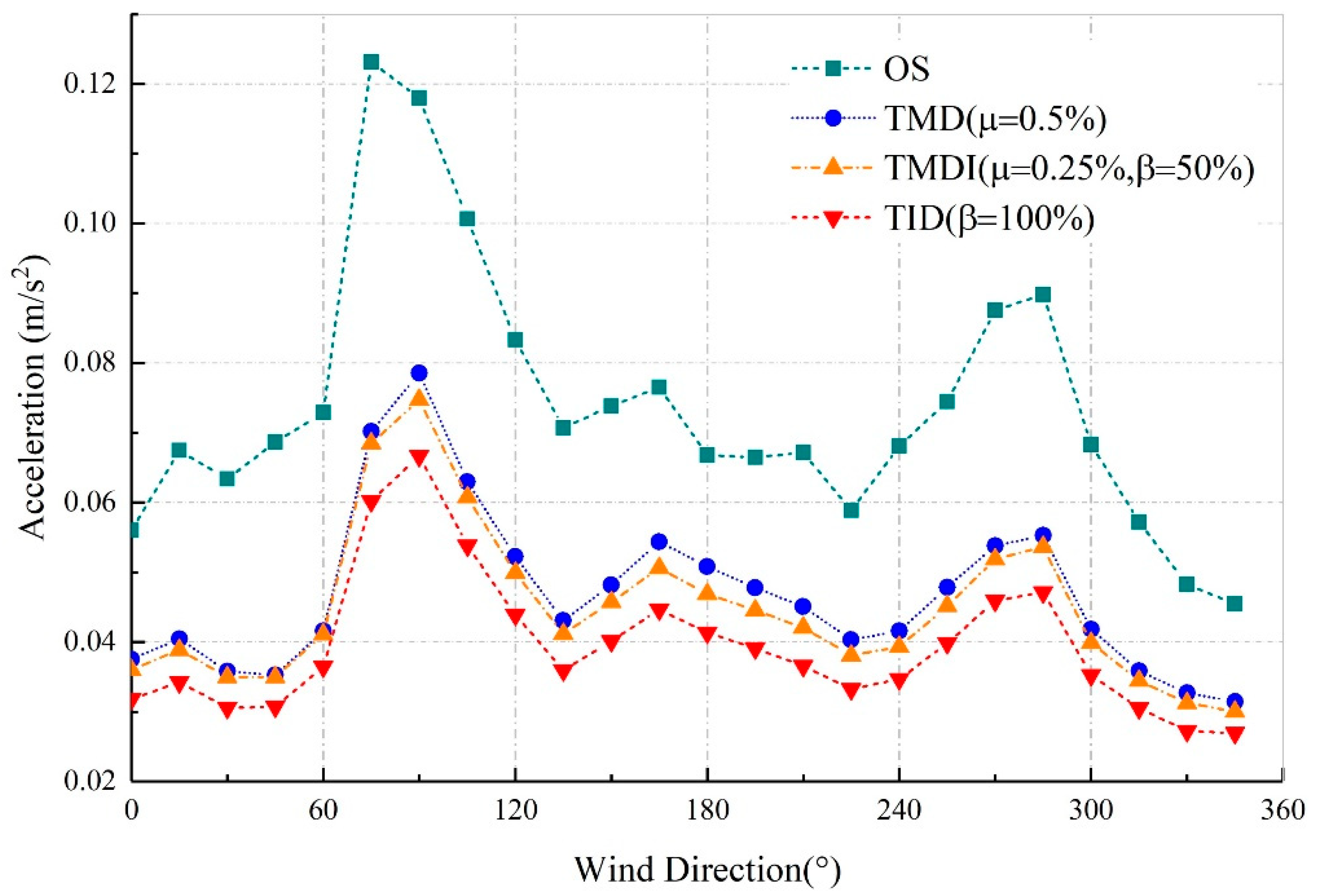
4.3. Effects of the Inerter-Based Vibration Absorbers on Wind-Induced Accelerations

Excessive wind-induced acceleration response may cause discomfort to building occupants and poses serious serviceability issues [66]. Wind-induced accelerations of the benchmark 340 m tall building, together with the TMD-, TMDI- and the TID-equipped building, are analyzed for a 33.86 m/s wind speed (10-years return period related to serviceability limit state) for each of the 24 wind directions. Figure 16 illustrates a segment of the top-floor time-history acceleration response (for an overall duration of 20 min) corresponding to 90° wind direction.

Following time histories of acceleration response, extreme acceleration responses can be obtained in terms of RMS value. Figure 17 presents variation of extreme acceleration responses along the building height corresponding to three typical wind directions, namely 0°, 45° and 90° wind directions. Figure 17 demonstrates that TMD, TMDI and TID have significant vibration absorbing effects on wind-induced acceleration response. In general, the vibration-mitigation effects of the TID are better than those of the TMD and TMDI, especially for wind direction of 45°. As stated previously, the three vibration absorbers share the same physical mass ratio. The vibration-mitigation effects are not only related to the parameters of TMD, TMDI or TID, but also depend on the predominant frequency components of aerodynamic forces. At 0° and 90° wind direction (cf. Figure 17a,c) the acceleration response at the x-axis are mainly induced by incoming turbulence flow and vortex shedding effects, respectively. At 45° wind direction, the acceleration response at the x-axis is affected by a combination of incoming turbulence flow and vortex shedding. Hence, it can be concluded that the vibration mitigation effects of TID is the best among three vibration absorbers, and the performance of TMDI is better than that of TMD for specific wind direction of 45°.

Figure 18 shows the variation of extreme top-floor accelerations corresponding to a variety of wind directions ranging from 0° to 345°. From Figure 18 we can see that TMD, TMDI and TID significantly control extreme acceleration responses, especially at 45° wind direction. For this wind direction, the response decreased from 0.0687 m/s² (original structure) to 0.0307 m/s² (TID-equipped structure).

Based on Equation (20), the factor of vibration-absorbing $F_{va}$ related to the top-floor acceleration response is evaluated and listed in

Table 5. Vibration-mitigation effects in terms of top-floor acceleration response for some loading configurations.

Selected Condition	Wind Direction (°)	$\begin{array}{c}{\ddot{\mathit{u}}}_{\mathbf{OS}}^{\mathit{t}\mathit{o}\mathit{p}}\\ ({\mathbf{m}/\mathbf{s}}^2)\end{array}$	$\begin{array}{c}{\ddot{\mathit{u}}}_{\mathbf{TMD}}^{\mathit{t}\mathit{o}\mathit{p}}\\ ({\mathbf{m}/\mathbf{s}}^2)\end{array}$	$\begin{array}{c}{\ddot{\mathit{u}}}_{\mathbf{TMDI}}^{\mathit{t}\mathit{o}\mathit{p}}\\ ({\mathbf{m}/\mathbf{s}}^2)\end{array}$	$\begin{array}{c}{\ddot{\mathit{u}}}_{\mathbf{TID}}^{\mathit{t}\mathit{o}\mathit{p}}\\ ({\mathbf{m}/\mathbf{s}}^2)\end{array}$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TMD}\right)}$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TMDI}\right)}$	${\mathit{F}}_{\mathit{v}\mathit{a}}^{\left(\mathbf{TID}\right)}$
Minimum $F_{va}$ (TMDI)	180	0.0667	0.0508	0.0469	0.0413	23.88	29.78	38.09
Maximum $F_{va}$ (TMDI)	45	0.0687	0.0353	0.0350	0.0307	48.63	49.06	55.26
Minimum $F_{va}$ (TID)	180	0.0667	0.0508	0.0469	0.0413	23.88	29.78	38.09
Maximum $F_{va}$ (TID)	45	0.0687	0.0353	0.0350	0.0307	48.63	49.06	55.26
Maximum acceleration OS	75	0.1232	0.0701	0.0685	0.0602	43.04	44.40	51.12

for a few emblematic wind directions and loading conditions.

In all wind directions, the TID dramatically reduces wind-induced top-floor accelerations. The worst vibration-absorbing effect of TMDI takes place for 180° wind direction, where the corresponding $F_{va}$ is 38.09% and is 1.6 times than that of the TMD having same physical mass. Generally, structural engineers are mainly concerned about the maximum extreme acceleration response of top floor, which may cause discomfort to residents. The maximum value of the acceleration of the original structure is 0.1232 m/s² corresponding to a wind direction of 75°. The TMD, TMDI and TID can reduce such extreme acceleration value of more than 40%, namely from 0.1232 m/s² to 0.0701 m/s², 0.0685 m/s² and 0.0602 m/s², respectively. These results show that the TID has a significant acceleration-reduction effect due to the enormous inertia benefitting from the inerter device, despite employing the same physical mass of the TMD. An important aspect for practical implementation of TMDI/TID systems is the force generated by the inerter, as bigger inertances bring also higher forces that are difficult to handle in a conventional structure [59]. In the present example, the maximum resistance force produced by inerter is 5319 kN at wind direction of 330°. Such requirement for inerter force can be practically implemented by installing several parallel inerter devices as shown in Figure 1.

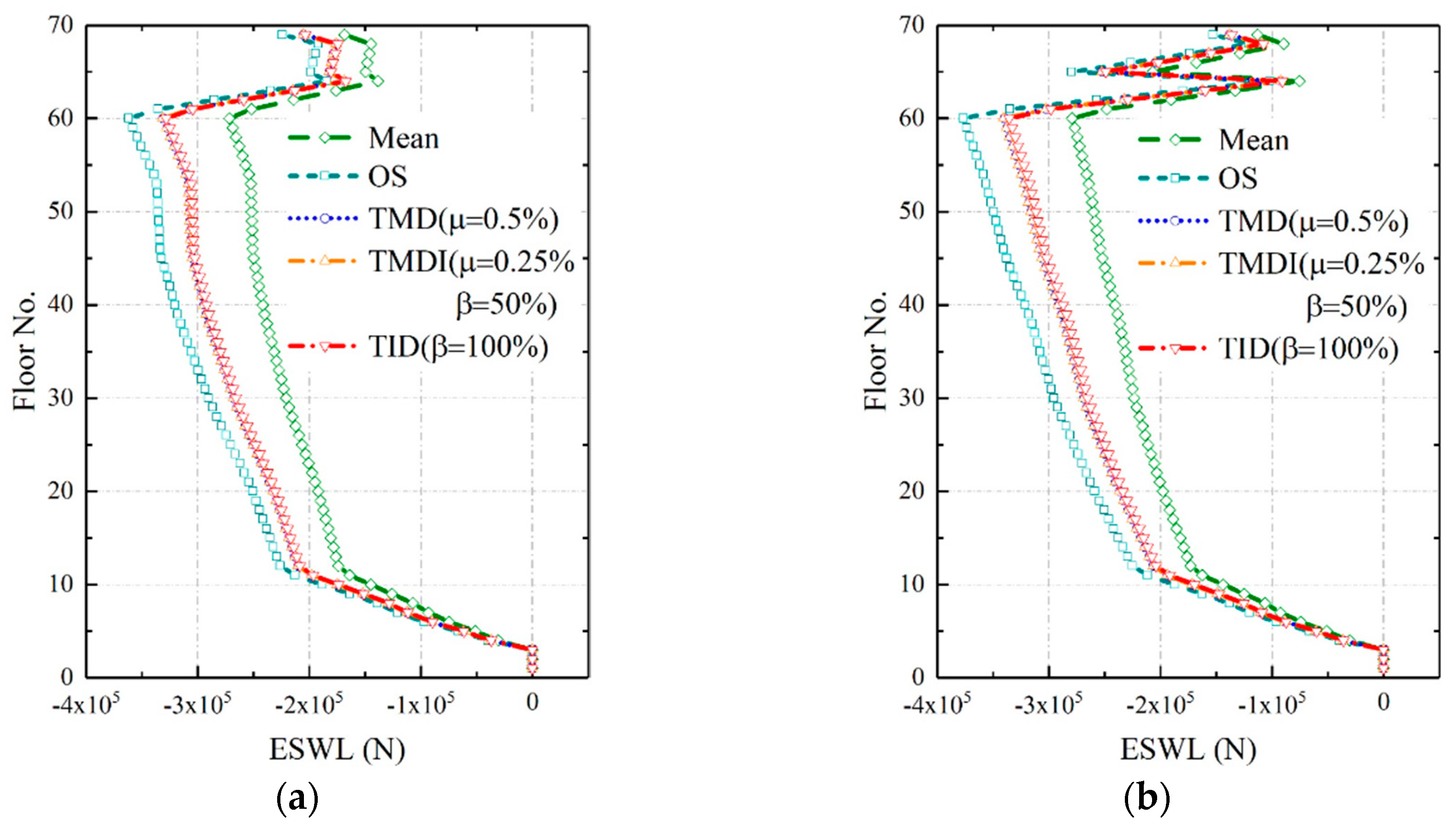
4.4. Effects of the Inerter-Based Vibration Absorbers on ESWLs

ESWLs are important parameters used by structural engineers for limit-state design as well as for assessing the bearing capacity of structures. Displacement Gust Loads Factor (DGLF) method [59], Moment-Based Gust Loads Factor (MGLF) method [66,67], Load-Response Correlation (LRC) method [68], and Weighted Combination of Modal Inertial Load Component (WCMILC) method [69] have been proposed to calculate ESWLs of high-rise buildings. Among them, the DGLF method is widely used in practical projects owing to its simplicity and for this reason it is adopted in this paper.

After the calculation of mean and extreme values of wind-induced profiles of displacements along the building height corresponding to 24 wind directions, ESWLs can be obtained from Equations (9) and (10). We here describe variation of ESWLs along the building height at a wind speed of 42.02 m/s (100-years return period).

Figure 19a,b show the profiles of ESWLs of original structure, structure with TMD, TMDI and TID along the building height, corresponding to wind directions of 0° and 45°, respectively. It is noted that the ESWLs of the original structure are larger than those of structure with vibration absorbers for every story. In this case, the performance of the three vibration absorbers is more or less comparable. As the ESWL is calculated by Equations (9) and (10) based on the extreme displacement, the mitigation effects of three vibration absorbers on ESWL are similar to that on extreme displacement. Above the 60th floor, the mean wind loads become smaller because floors above 60th floor gradually draw back in plane as already illustrated in Figure 2.

5. Conclusions

The wind-induced response of a benchmark 340 m tall building equipped with inerter-based vibration absorber, i.e., TMDI and TID, has been investigated. The analysis has been carried out in the time-domain, by considering the time histories of aerodynamic forces computed from synchronous multi-point pressure measurements in wind tunnel tests, which accounts for the actual cross section of the building and the existing surrounding conditions. The results have been analyzed in terms of wind-induced displacement and acceleration response as well as ESWLs on the original structure, and comparatively on the building equipped with the TMD, TMDI and TID corresponding to 24 different wind directions (from 0° to 345° at an interval of 15°).

The main contents and findings of the present work are summarized as follows:

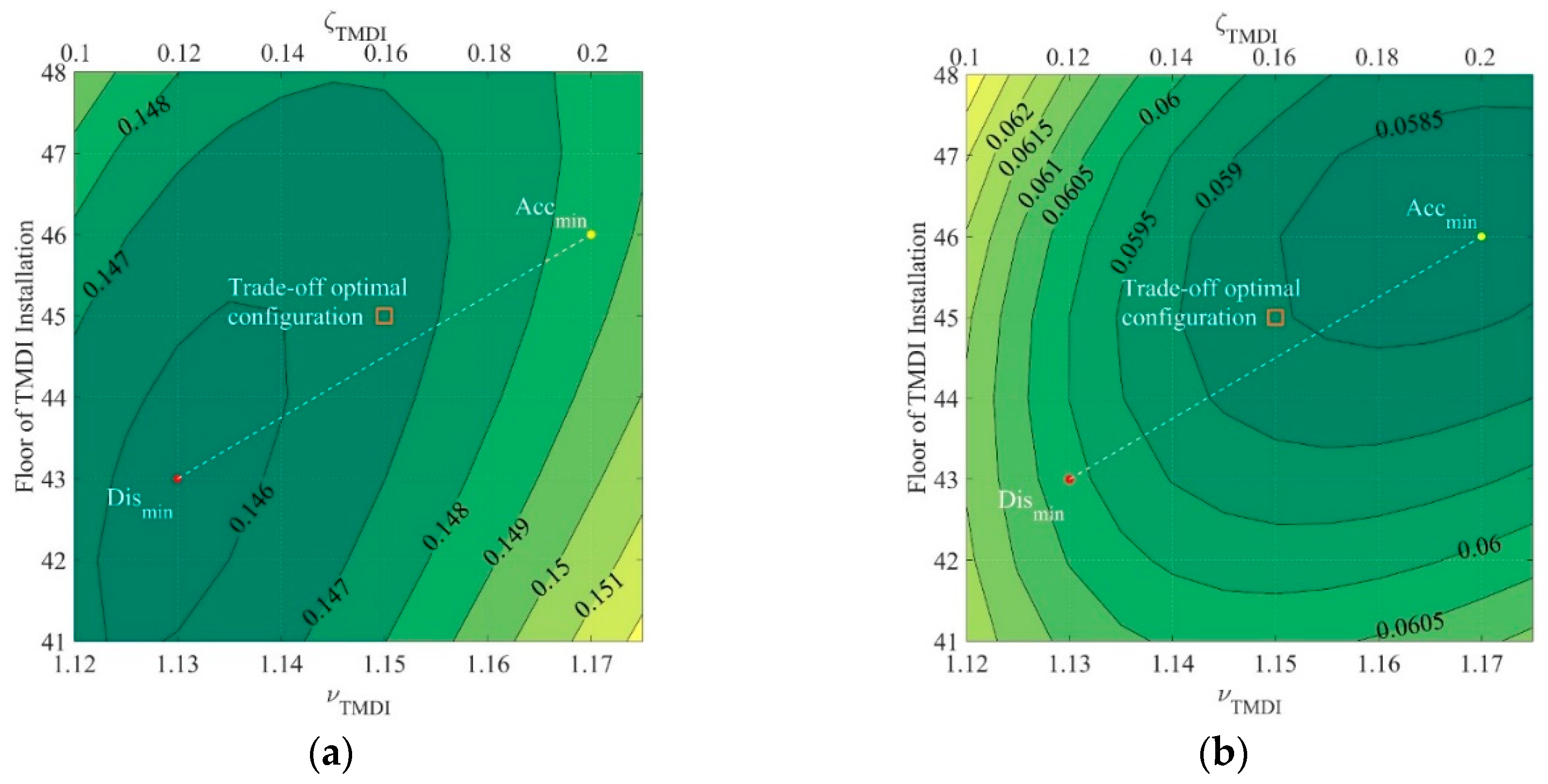
• Displacement- and acceleration-based optimizations have been performed to obtain the best parameters of the TMD, TMDI and TID in a 3D design space, including the installation floor, the frequency ratio and the damping ratio as explicit design variables. The proposed procedure attempts to find a good trade-off between displacement mitigation and acceleration mitigation, considering results from a constrained optimization problem in which the installation floor represents a design variable being incorporated in the optimization procedure;
• Both wind-induced extreme top-floor displacement and acceleration responses of the benchmark building can be effectively mitigated by the TMDI and TID. Among the three vibration absorbers, the TID outperforms the TMDI and the TMD, and the acceleration mitigation effect of the TMDI is better than that of the TMD. The extreme displacement and acceleration response of the original structure are 0.2293 m and 0.1232 m/s², respectively. The installation of the TMDI has reduced these response values to 0.1519 m and 0.0685 m/s², respectively, with a resulting factor of vibration absorbing $F_{va}$ equal to 33.74% and 44.40%, respectively. The best vibration mitigation effect is achieved by the TID, which reduces the extreme displacement and acceleration to 0.1421 m and 0.0602 m/s², respectively, corresponding to $F_{va}$ of 38.02% and 51.12%, respectively;
• Comparison among the three different vibration absorbers has shown that the TID with same physical mass ratio as the TMD and TMDI can achieve better vibration mitigation effects in terms of displacement and acceleration responses. In particular, the factors of vibration absorbing $F_{va}$ of TMD, TMDI and TID for extreme displacement have been 34.01%, 33.74% and 38.02%, respectively, and the analogous factors for extreme acceleration have been 43.04%, 44.40% and 51.12%, respectively. The performance of TID slightly outperforms the other two vibration absorbers in terms of mitigating ESWLs, which is consistent with the results of displacement mitigation effect;
• Optimizations of parameters have demonstrated that the TMDI and TID achieve the best vibration mitigation effects when the first terminal is not installed at the top floor, but at the mid-upper place of the primary structure with TMDI/TID topologies such that the inerter spans four stories. In this configuration, the TID can achieve better wind-induced vibration mitigation than the TMD employing the same physical mass ratio as that of the corresponding TMD (thus implying a significant reduction in terms of physical mass actually allocated due to the mass-amplification effect of the inerter when the TID scheme is designed to achieve the same vibration mitigation effect as that of TMD);
• The TID having the same physical mass as the TMD (meaning that the inertia is entirely provided by the inerter, with ideally null attached mass) can achieve much better vibration mitigation effects than the TMD in terms of acceleration response when the frequency ratio $\upsilon$ and damping ratio $\zeta$ of the TID are tuned to be around 1.15 and 16%, respectively, and the TID is installed at the 45th floor. A slightly better displacement mitigation effect can be achieved by adopting a relative smaller frequency ratio, damping ratio and lower installation floor, e.g., 1.13, 12% and 43rd floor, respectively.

The present study has focused on the design and optimization of the vibration absorbers based on the expected wind pressure, thus emphasizing the effects of wind loading on the high-rise building. Future investigations concerning the analysis of the proposed structural control systems against other types of dynamic loads, such as earthquake excitations, are currently underway.