Silicon Waveguide Optical Isolator with Directly Bonded Magneto-Optical Garnet
Abstract
:Featured Application
Abstract
1. Introduction
2. Device Structure and Operation Principle
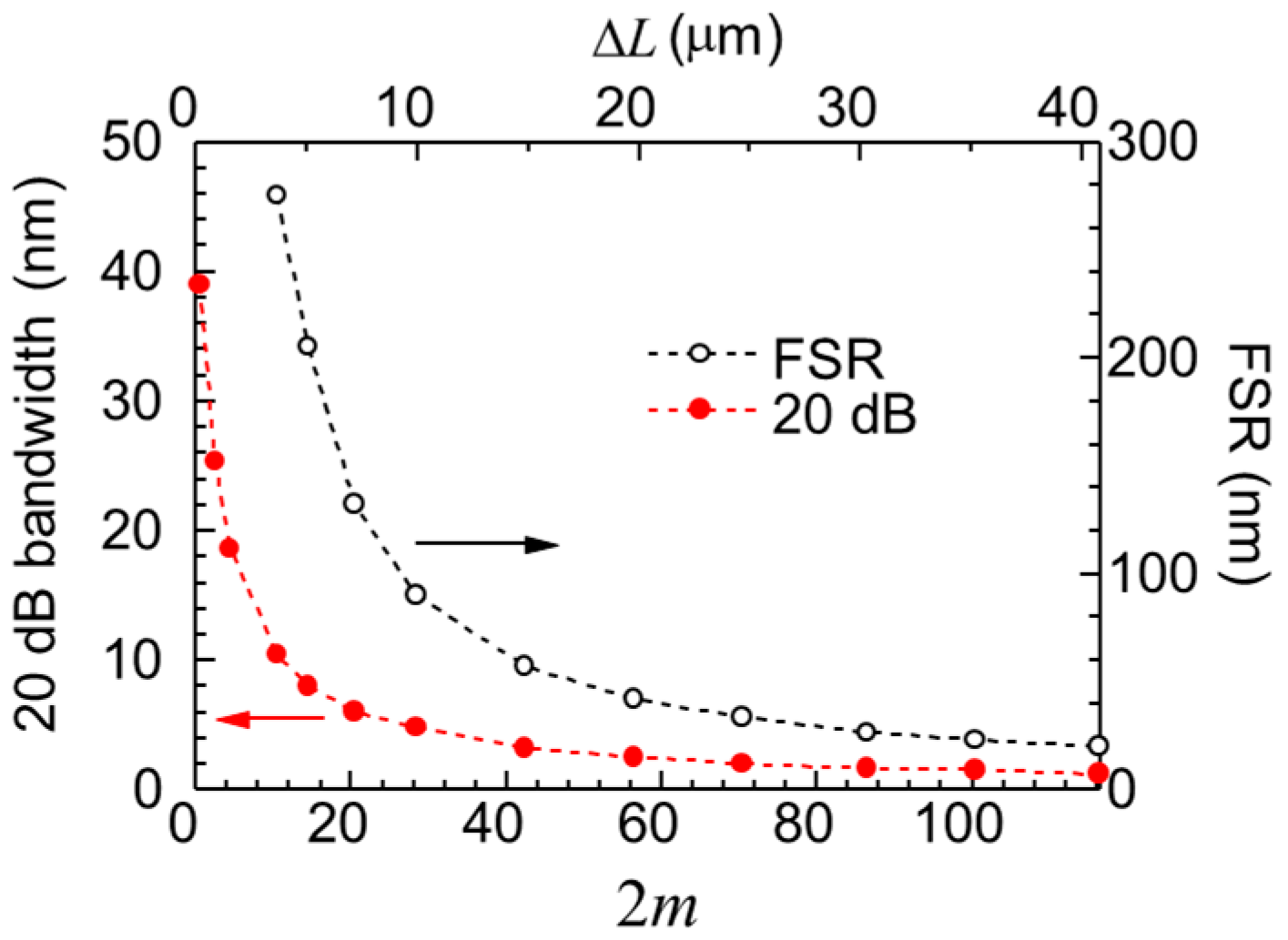
3. Operation Bandwidth
4. Temperature-Insensitive Operation
5. Integration of TE–TM Mode Converter
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Krause, M.; Renner, H.; Brinkmeyer, E. Optical isolation in silicon waveguide based on nonreciprocal Raman amplification. Electron. Lett. 2008, 44, 691–693. [Google Scholar] [CrossRef]
- Shi, Y.; Fan, S. Limitation of nonlinear optical isolators due to dynamic reciprocity. Nat. Photonics 2015, 9, 388–392. [Google Scholar] [CrossRef]
- Dong, C.; Shen, Z.; Zou, C.; Zhang, Y.; Fu, W.; Guo, G. Brillouin-scattering-induced transparency and non-reciprocal light storage. Nat. Commun. 2015, 6, 6193. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, K.; Gao, S.; Wang, Y.; Nirmalathas, A.; Lim, C.; Almeh, K.; Skafidas, E. Four-wave-mixing-based silicon integrated optical isolator with dynamic non-reciprocity. IEEE Photonics Technol. Lett. 2016, 28, 1739–1742. [Google Scholar] [CrossRef]
- Yu, Z.; Fan, S. Complete optical isolation created by indirect interband photonic transitions. Nat. Photonics 2009, 3, 91–94. [Google Scholar] [CrossRef]
- Lira, H.; Yu, Z.; Fan, S.; Lipson, M. Electrically driven nonreciprocity induced by interband photonic transition on a silicon chip. Phys. Rev. Lett. 2012, 109, 033901-1-5. [Google Scholar] [CrossRef]
- Doerr, C.; Chen, L.; Vermeulen, D. Silicon photonics broadband modulation-based isolator. Opt. Express 2014, 22, 4493–4498. [Google Scholar] [CrossRef]
- Gomi, M.; Satoh, K.; Abe, M. Giant Faraday rotation of Ce-substituted YIG films epitaxially grown by RF sputtering. Jpn. J. Appl. Phys. 1988, 27, L1536–L1538. [Google Scholar] [CrossRef]
- Shintaku, T.; Tate, A.; Mino, S. Ce-substituted yttrium iron garnet films prepared on Gd3Sc2Ga3O12 garnet substrates by sputter epitaxy. Appl. Phys. Lett. 1997, 71, 1640–1642. [Google Scholar] [CrossRef]
- Sun, X.; Du, Q.; Goto, T.; Onbasli, C.; Kim, D.; Aimon, N.; Hu, J.; Ross, C. Single-step deposition of cerium-substituted yttrium iron garnet for monolithic on-chip optical isolation. ACS Photonics 2015, 2, 856–863. [Google Scholar] [CrossRef]
- Dulal, P.; Block, A.; Gage, T.; Haldren, H.; Sung, S.; Hutching, D.; Stadler, B. Optimized magneto-optical isolator designs inspired by seedlayer-free terbium iron garnet with opposite chirality. ACS Photonics 2016, 3, 1818–1825. [Google Scholar] [CrossRef]
- Huang, D.; Pintus, P.; Zhang, C.; Shoji, Y.; Mizumoto, T.; Bowers, J. Electrically driven and thermally tunable integrated optical isolators for silicon photonics. IEEE J. Sel. Top. Quantum Electron. 2016, 22, 4403408. [Google Scholar] [CrossRef]
- Pintus, P.; Huang, D.; Zhang, C.; Shoji, Y.; Mizumoto, T.; Bowers, J. Microring-based optical isolator and circulator with integrated electromagnet for silicon photonics. J. Lightw. Technol. 2017, 35, 1429–1437. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T.; Yokoi, H.; Hsieh, I.-W.; Osgood, R.M., Jr. Magneto-optical isolator with silicon waveguides fabricated by direct bonding. Appl. Phys. Lett. 2008, 92, 071117. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T. Magneto-optical non-reciprocal devices in silicon photonics. Sci. Technol. Adv. Mater. 2014, 15, 014602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shoji, Y.; Shirato, Y.; Mizumoto, T. Silicon Mach-Zehnder interferometer optical isolator having 8 nm bandwidth for over 20 dB isolation. Jpn. J. Appl. Phys. 2014, 53, 022202. [Google Scholar] [CrossRef]
- Furuya, K.; Nemoto, T.; Kato, K.; Shoji, Y.; Mizumoto, T. Athermal operation of a waveguide optical isolator based on canceling phase deviations in a Mach-Zehnder interferometer. J. Lightw. Technol. 2016, 34, 1699–1705. [Google Scholar] [CrossRef]
- Shoji, Y.; Fujie, A.; Mizumoto, T. Silicon waveguide optical isolator operating for TE mode input light. J. Sel. Top. Quantum Electron. 2016, 22, 4403307. [Google Scholar] [CrossRef]
- Shoji, Y.; Ito, M.; Shirato, Y.; Mizumoto, T. MZI optical isolator with Si-wire waveguides by surface-activated direct bonding. Opt. Express 2012, 20, 18440–18448. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T. Wideband design of nonreciprocal phase shift magneto-optical isolators using phase adjustment in Mach-Zehnder interferometer. Appl. Opt. 2006, 45, 7144–7150. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T. Ultra-wideband design of waveguide magneto-optical isolators operating in 1.31 µm and 1.55 µm. Opt. Express 2007, 15, 639–645. [Google Scholar] [CrossRef] [PubMed]
- Popkov, A.F.; Fehndrich, M.; Lohmeyer, M.; Dötsch, H. Nonreciprocal TE-mode phase shift by domain walls in magnetooptic rib waveguides. Appl. Phys. Lett. 1998, 72, 2508–2510. [Google Scholar] [CrossRef]
- Fujita, J.; Levy, M.; Osgood, R.M., Jr.; Wilkens, L.; Dötsch, H. Polarization-independent waveguide optical isolator based on nonreciprocal phase shift. IEEE Photonics Technol. Lett. 2000, 12, 1510–1512. [Google Scholar] [CrossRef]
- Pintus, P.; Pasquale, F.D.; Bowers, J.E. Integrated TE and TM optical circulators on ultra-low-loss silicon nitride platform. Opt. Express 2013, 21, 5041–5052. [Google Scholar] [CrossRef] [PubMed]
- Ishida, E.; Miura, K.; Shoji, Y.; Yokoi, H.; Mizumoto, T.; Nishiyama, N.; Arai, S. Amorphous-Si waveguide on a garnet magneto-optical isolator with a TE mode nonreciprocal phase shift. Opt. Express 2017, 25, 452–462. [Google Scholar] [CrossRef]
- Ghosh, S.; Keyvaninia, S.; Shirato, Y.; Mizumoto, T.; Roelkens, G.; Baets, R. Optical isolator for TE polarized light realized by adhesive bonding of Ce:YIG on silicon-on-insulator waveguide circuits. IEEE Photonics J. 2013, 5, 6601108. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T. Waveguide magneto-optical devices for photonics integrated circuits. Opt. Mater. Express 2018, 8, 2387–2394. [Google Scholar] [CrossRef]
- Yamaguchi, R.; Shoji, Y.; Mizumoto, T. Low-loss waveguide optical isolator with tapered mode converter and magneto-optical phase shifter for TE mode input. Opt. Express 2018, 26, 21271–21278. [Google Scholar] [CrossRef]







© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shoji, Y.; Mizumoto, T. Silicon Waveguide Optical Isolator with Directly Bonded Magneto-Optical Garnet. Appl. Sci. 2019, 9, 609. https://doi.org/10.3390/app9030609
Shoji Y, Mizumoto T. Silicon Waveguide Optical Isolator with Directly Bonded Magneto-Optical Garnet. Applied Sciences. 2019; 9(3):609. https://doi.org/10.3390/app9030609
Chicago/Turabian StyleShoji, Yuya, and Tetsuya Mizumoto. 2019. "Silicon Waveguide Optical Isolator with Directly Bonded Magneto-Optical Garnet" Applied Sciences 9, no. 3: 609. https://doi.org/10.3390/app9030609
APA StyleShoji, Y., & Mizumoto, T. (2019). Silicon Waveguide Optical Isolator with Directly Bonded Magneto-Optical Garnet. Applied Sciences, 9(3), 609. https://doi.org/10.3390/app9030609




