Pullout Response of Ultra-High-Performance Concrete with Twisted Steel Fibers
Abstract
Featured Application
Abstract
1. Introduction
2. Analytical Model
2.1. Model Assumption
2.2. Model Derivation
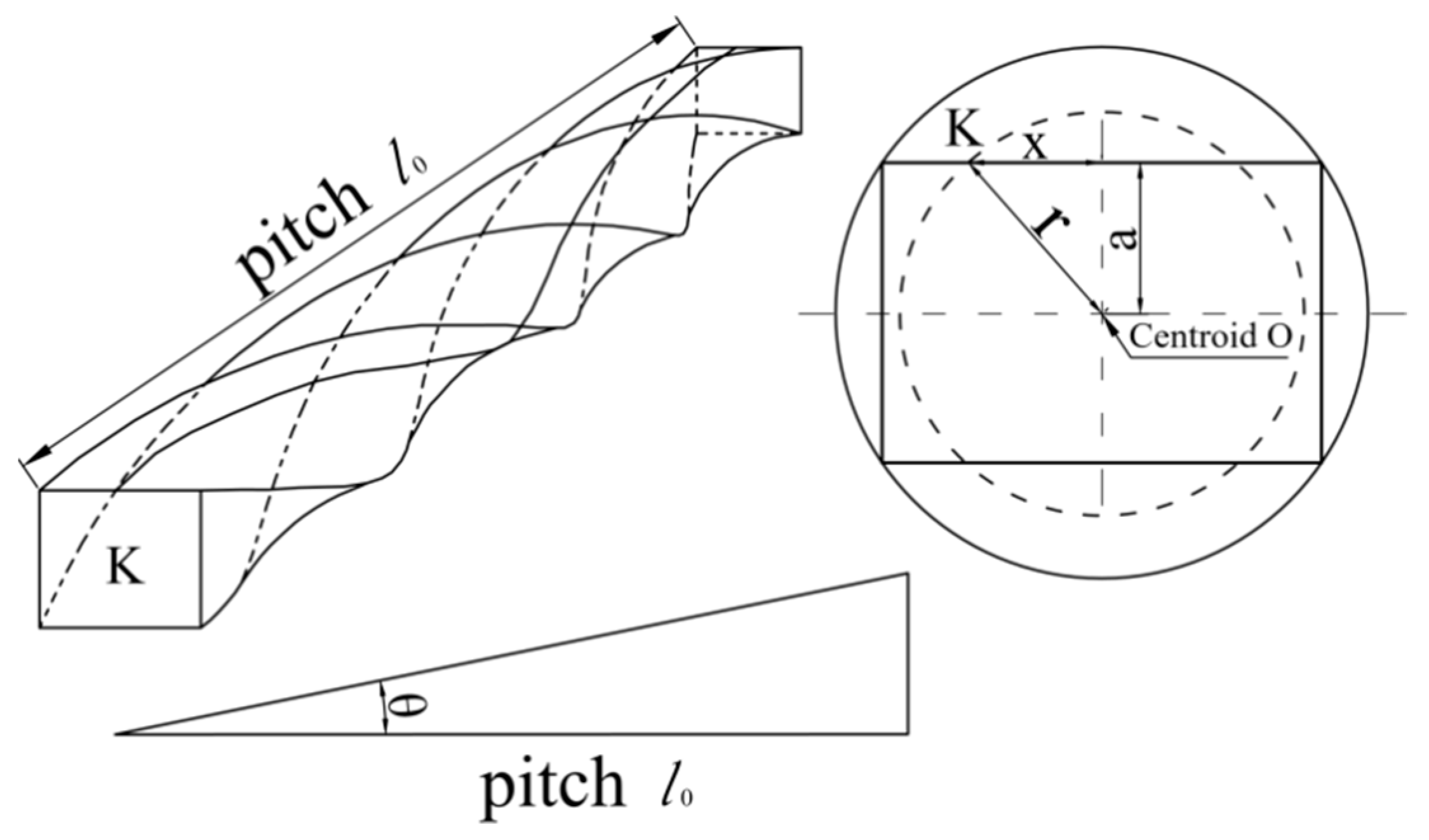
2.2.1. Fiber with a Rectangular Section
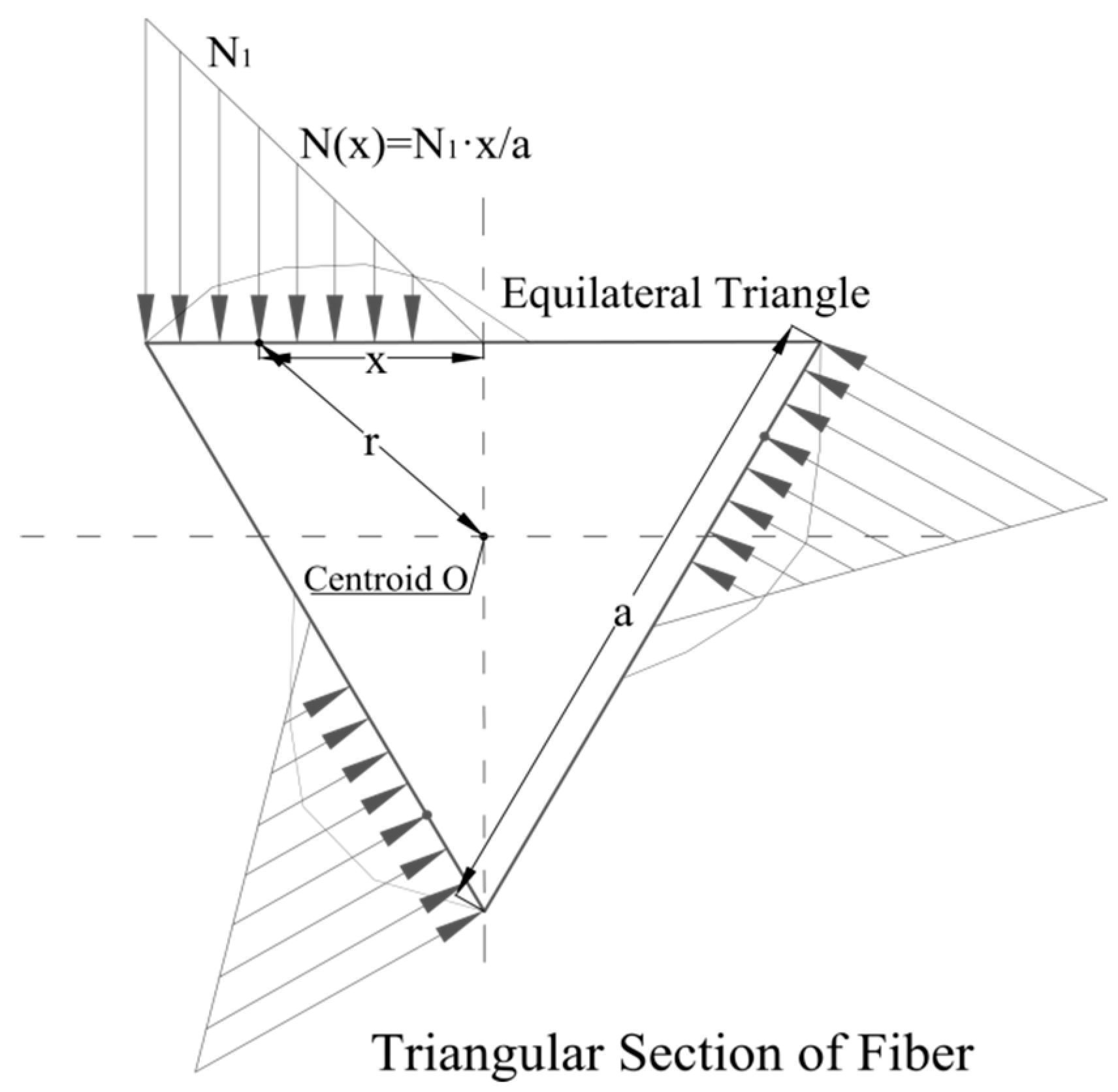
2.2.2. Fiber with a Triangular Section
3. Experimental and Verification
3.1. Concrete Samples
3.2. Concrete Samples with a Single Twisted Steel Fiber
3.3. Experimental Results
3.3.1. Image Analysis
3.3.2. Untwisting Mechanism
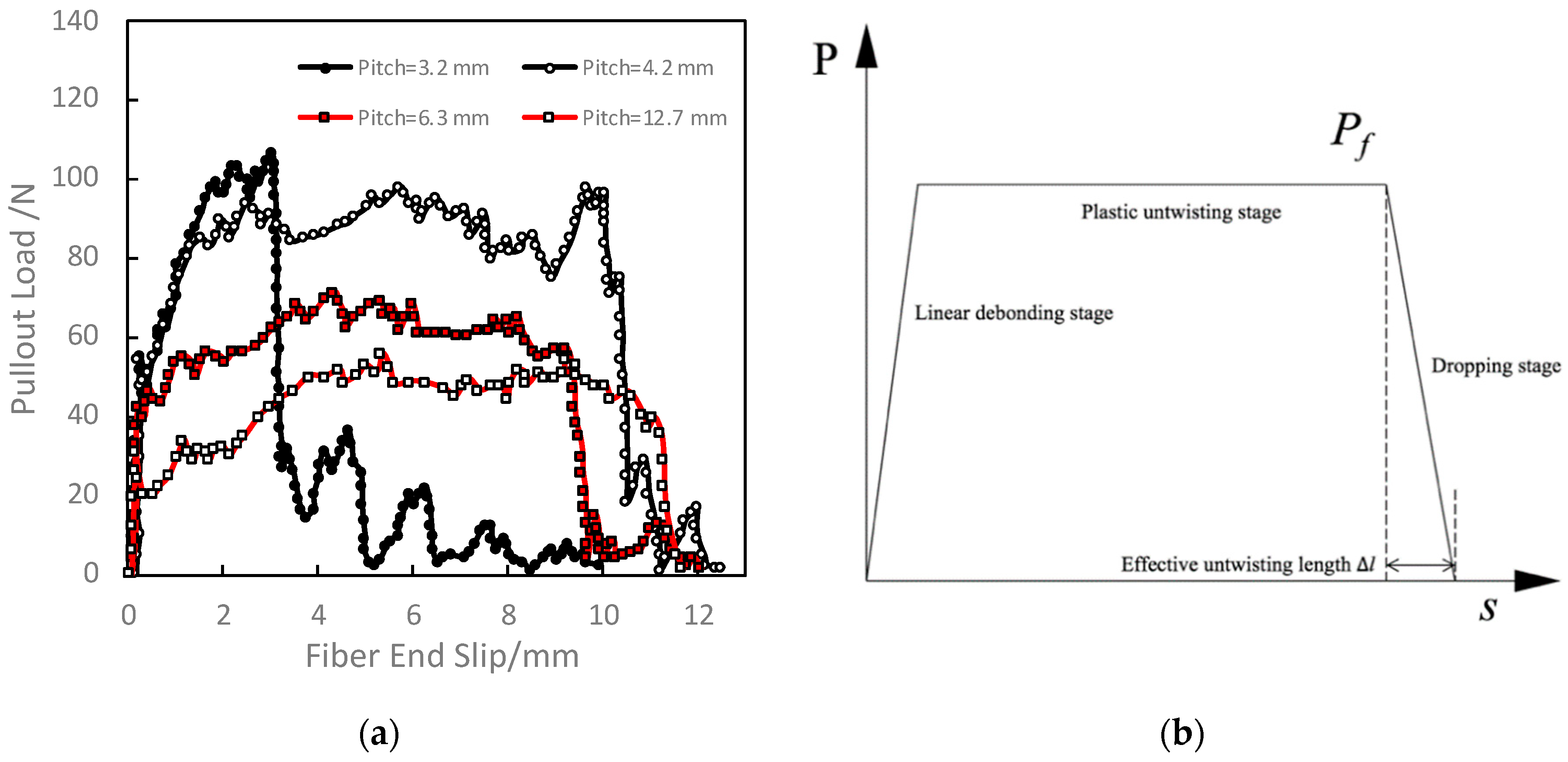
- Linear debonding stage: The pullout force increased linearly with the fiber end displacement, similar to the elastic stage for the normal concrete [25]. This process takes around 1–3 min. There is no relative displacement between matrix and TSF, indicating the matrix-fiber interface remains intact with no debonding occurs. Thus, the strong bonding between fiber and its surrounding matrix produces a united resistance to the external pulling force from both TSF and matrix. By the end of this linear process, debonding initiates at the matrix-fiber interface where the pullout force-loading displacement relationship becomes weakly nonlinear.
- Plastic untwisting stage: After the debonding occurs, debonding slip rotation of TSF is believed to start. This movement is a kind of screw-like movements at the embedded fiber part after debonding. As there is no relative rotation at the sample ends due to the constraint from the fixture, a torque is generated. This torque leads to the plastic ‘untwisting’ deformation in the fiber part near the outlet and thus the stable untwisting pull-out force. This pull-out force hardening stage was also reported by Wille et al. [17]. As shown in Figure 7, there are slight fluctuations in the untwisting pullout force. The fluctuations may result from the inhomogeneity of the matrix-fiber interface and the stress concentration on the rectangular corner of TSF. This stress concentration may be difficult to penetration by the matrix [1]. The super-high strength of matrix prevents the TSF from damaging the matrix due to the undesirable sites of stress concentration.
- Dropping stage: When the remaining embedment length of the fiber is less than the effective untwisting length Δl, the pull-out force rapidly decreases to zero when the torque is released.
3.3.3. Pullout Force Calculation
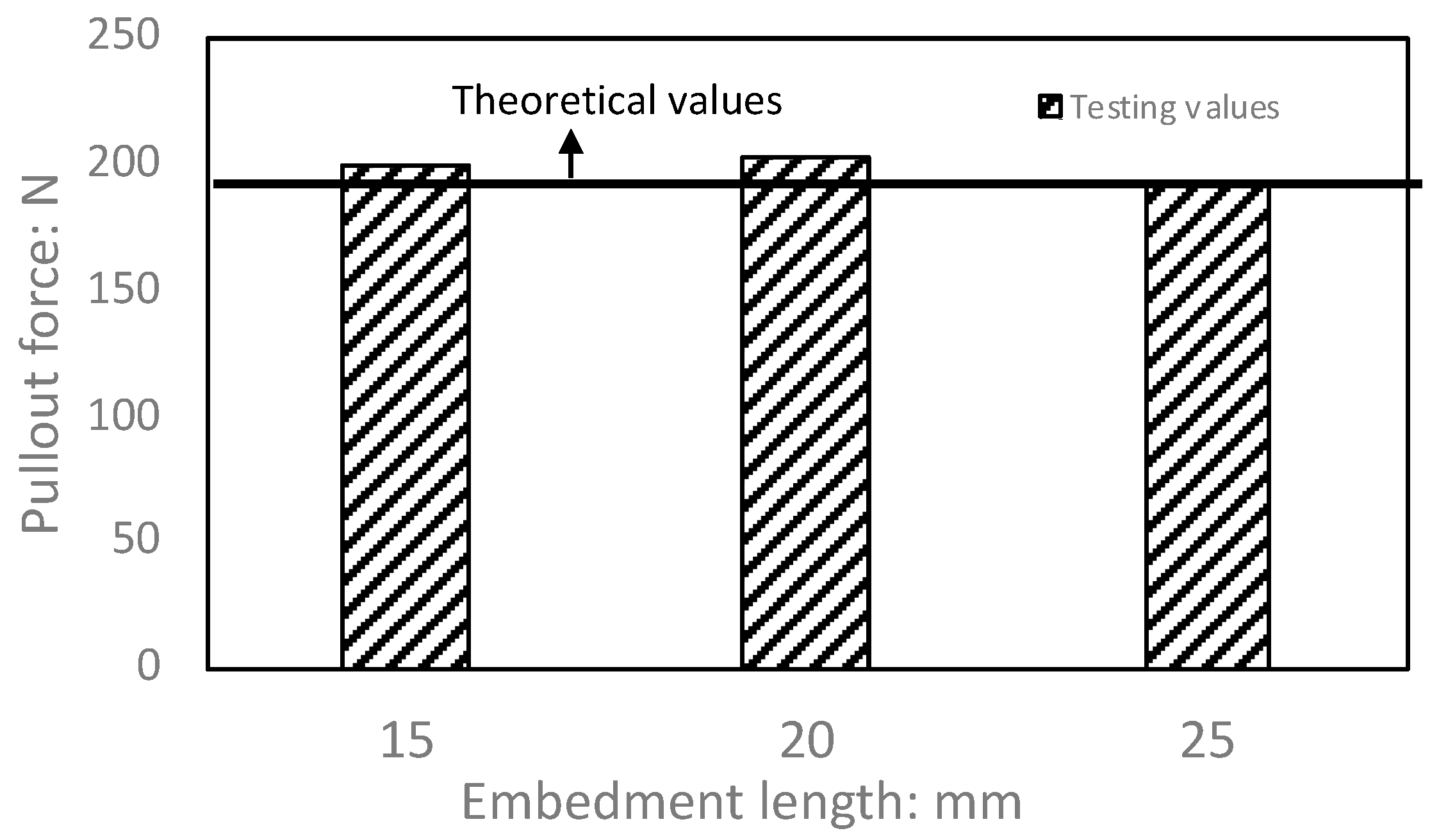
3.4. Comparison between Test and Analytical Solution
4. Numerical Analysis
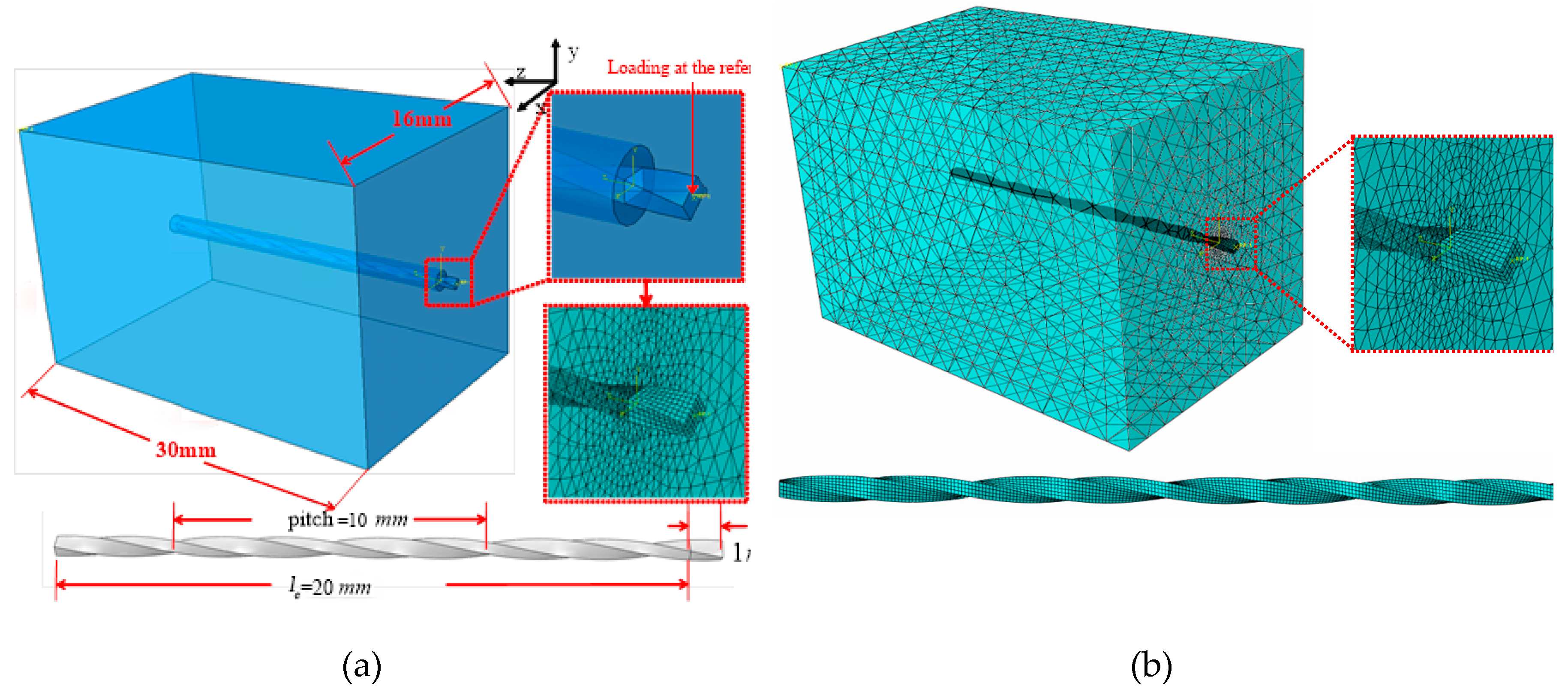
4.1. Structure Model
4.2. Material Model
4.3. Loading and Boundary Condition
4.4. Numerical Results
5. Conclusions
- The pullout process of a single TSF in UHPFRC can be divided into a linear debonding stage, plastic untwisting stage and a dropping stage, so the pullout force versus displacement curve can be simplified as a trilinear curve.
- A significant strain hardening characteristic occurred due to the plastic deformation of TSF and its untwisting deformation during the pullout process. This untwisting deformation occurred on the fiber (within the effective untwisting fiber length) close to the exterior concrete surface.
- The fiber channel inside the matrix was not damaged after pullout, indicating a strong pullout resistance of matrix-fiber interface due to the high mechanical strength of matrix and strong bonding between matrix and TSF.
- The pullout force is independent of the embedment length of TSF and dependent of the geometry of fiber cross-section as well as the friction at matrix-fiber interface and its adhesive bond.
- The developed analytical formula was verified by the experimental data and thus can be used to gain insights into the mechanism of pullout process and to understand the parameters that influence the mechanical property of UHPFRC with TSFs.
Author Contributions
Funding
References
- Naaman, A.E. Engineered steel fibers with optimal properties for reinforcementof cement composites. J. Adv. Concr. Technol. 2003, 1, 241–251. [Google Scholar] [CrossRef]
- Wille, K.; Naaman, A.E.; El-Tawil, S.; Parra-Montesinos, G.J. Ultra-high performance concrete and fiber reinforced concrete: Achieving strength and ductility without heat curing. Mater. Struct. 2012, 45, 309–324. [Google Scholar] [CrossRef]
- Wille, K.; El-Tawil, S.; Naaman, A.E. Properties of strain hardening ultra high performance fiber reinforced concrete (UHP-FRC) under direct tensile loading. Cement Concr. Compos. 2014, 48, 53–66. [Google Scholar] [CrossRef]
- Yu, R.; Spiesz, P.; Brouwers, H.J. Energy absorption capacity of a sustainable Ultra-High Performance Fibre Reinforced Concrete (UHPFRC) in quasi-static mode and under high velocity projectile impact. Cement Concr. Compos. 2016, 68, 109–122. [Google Scholar] [CrossRef]
- Venkateshwaran, A.; Tan, K.H.; Li, Y. Residual flexural strengths of steel fiber reinforced concrete with multiple hooked-end fibers. Struct. Concr. 2018, 19, 352–365. [Google Scholar] [CrossRef]
- Zhao, M.; Zhao, M.; Chen, M.; Li, J.; Law, D. An experimental study on strength and toughness of steel fiber reinforced expanded-shale lightweight concrete. Constr. Build. Mater. 2018, 183, 493–501. [Google Scholar] [CrossRef]
- Qu, D.; Cai, X.; Chang, W. Evaluating the Effects of Steel Fibers on Mechanical Properties of Ultra-High Performance Concrete Using Artificial Neural Networks. Appl. Sci. 2018, 8, 1120. [Google Scholar] [CrossRef]
- Kang, S.T.; Kim, J.; Lee, B. Effects of Water Reducing Admixture on Rheological Properties, Fiber Distribution and Mechanical Behavior of UHPFRC. Appl. Sci. 2019, 9, 29. [Google Scholar] [CrossRef]
- Wang, D.; Ju, Y.; Zheng, W.; Shen, H. Seismic Behavior and Shear Bearing Capacity of Ultra-High Performance, Fiber-Reinforced Concrete (UHPFRC) Beam-Column Joints. Appl. Sci. 2018, 8, 810. [Google Scholar] [CrossRef]
- Ferreira, S.R.; Pepe, M.; Martinelli, E.; de Andrade Silva, F.; Toledo Filho, R.D. Influence of natural fibers characteristics on the interface mechanics with cement based matrices. Compos. Part B Eng. 2018, 140, 183–196. [Google Scholar] [CrossRef]
- Kim, M.J.; Kim, S.; Yoo, D.Y. Hybrid Effect of Twisted Steel and Polyethylene Fibers on the Tensile Performance of Ultra-High-Performance Cementitious Composites. Appl. Sci. 2018, 10, 879. [Google Scholar] [CrossRef]
- De Andrade Silva, F.; Mobasher, B.; Soranakom, C.; Toledo Filho, R.D. Effect of fiber shape and morphology on interfacial bond and cracking behaviors of sisal fiber cement based composites. Cement Concr. Compos. 2011, 33, 814–823. [Google Scholar] [CrossRef]
- Kim, D.J.; El-Tawil, S.; Naaman, A.E. Loading rate effect on pullout behavior of deformed steel fibers. ACI Mater. J. 2008, 105, 576. [Google Scholar] [CrossRef]
- Ellis, B.D.; McDowell, D.L.; Zhou, M. Simulation of single fiber pullout response with account of fiber morphology. Cement Concr. Compos. 2014, 48, 42–52. [Google Scholar] [CrossRef]
- Lee, Y.; Kang, S.T.; Kim, J.K. Pullout behavior of inclined steel fiber in an ultra-high strength cementitious matrix. Constr. Build. Mater. 2010, 24, 2030–2041. [Google Scholar] [CrossRef]
- Alkaysi, M. Strength and Durability of Ultra-High Performance Concrete Materials and Structures. Ph.D. Thesis, University of Michigan, Ann Arbor, MI, USA, 2016. [Google Scholar]
- Wille, K.; Naaman, A.E. Effect of ultra-high-performance concrete on pullout behavior of high-strength brass-coated straight steel fibers. ACI Mater. J. 2013, 110, 451. [Google Scholar] [CrossRef]
- Qsymah, A.; Sharma, R.; Yang, Z.; Margetts, L.; Mummery, P. Micro X-ray Computed Tomography Image-based Two-scale Homogenisation of Ultra High Performance Fibre Reinforced Concrete. Constr. Build. Mater. 2017, 130, 230–240. [Google Scholar] [CrossRef]
- Baltay, P.; Gjelsvik, A. Coefficient of friction for steel on concrete at high normal stress. J. Mater. Civ. Eng. 1990, 2, 46–49. [Google Scholar] [CrossRef]
- Hassan, A.M.; Jones, S.W.; Mahmud, G.H. Experimental test methods to determine the uniaxial tensile and compressive behaviour of ultra high performance fibre reinforced concrete (UHPFRC). Constr. Build. Mater. 2012, 37, 874–882. [Google Scholar] [CrossRef]
- China Association for Engineering Construction Standardization. Test Methods Used for Steel Fiber Reinforced Concrete; CECS13:89; China Association for Engineering Construction Standardization: Beijing, China, 1989; pp. 30–35. (In Chinese) [Google Scholar]
- Volume Graphics Gmbh. VGStudio MAX 3.0; Volume Graphics Gmbh: Heidelberg, Germany, 2014. [Google Scholar]
- Wu, Z.; Wong, H.S.; Buenfeld, N.R. Effect of confining pressure and microcracks on mass transport properties of concrete. Adv. Appl. Ceram. 2014, 113, 485–495. [Google Scholar] [CrossRef]
- Wu, Z.; Wong, H.S.; Buenfeld, N.R. Influence of drying-induced microcracking and related size effects on mass transport properties of concrete. Cement Concr. Res. 2015, 68, 35–48. [Google Scholar] [CrossRef]
- Popovics, S. A numerical approach to the complete stress-strain curve of concrete. Cement Concr. Res. 1973, 3, 583–599. [Google Scholar] [CrossRef]




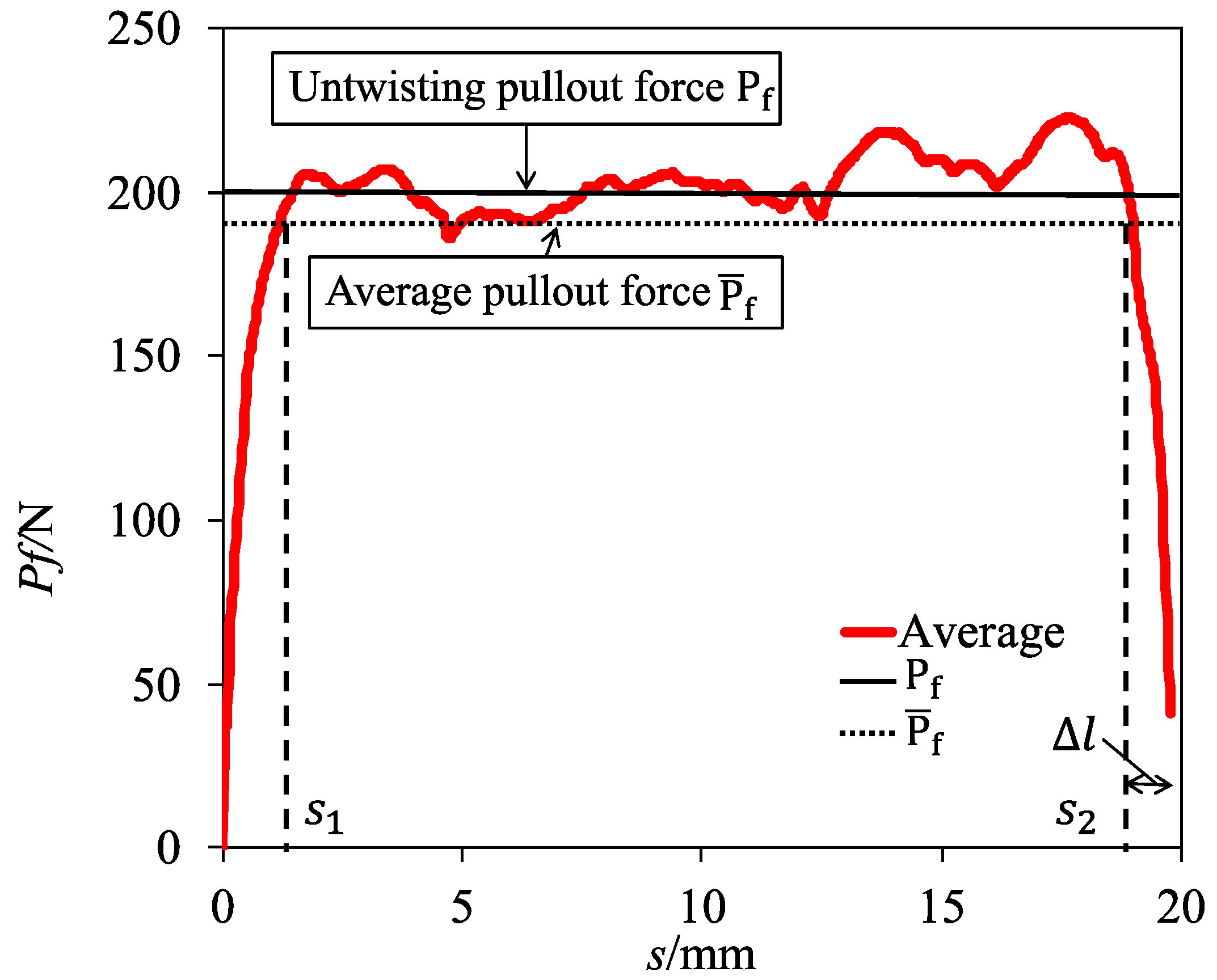



| le/mm | ID | Ep (N.mm) | Average Pullout Force (N) | s1 (mm) | s2 (mm) | △l (mm) | Untwisting Pullout Force (N) |
|---|---|---|---|---|---|---|---|
| 15 | Average line | 2873 | 191.5 | 1.26 | 13.24 | 1.76 | 199.1 |
| T15-1 | 3295 | 219.7 | 1.11 | 13.94 | 1.06 | 229.5 | |
| T15-2 | 2980 | 198.7 | 1.75 | 13.04 | 1.96 | 210.2 | |
| T15-3 | 2461 | 164.1 | 1.41 | 13.63 | 1.37 | 179.7 | |
| T15-4 | 2895 | 193.0 | 1.21 | 13.31 | 1.69 | 201.3 | |
| T15-5 | 2810 | 187.3 | 1.21 | 13.75 | 1.25 | 195.8 | |
| T15-6 | 2539 | 169.3 | 1.37 | 13.51 | 1.49 | 182.1 | |
| 20 | Average line | 3905 | 193.3 | 1.27 | 18.28 | 1.72 | 203.3 |
| T20-1 | 3476 | 173.8 | 1.54 | 18.65 | 1.35 | 180.1 | |
| T20-2 | 4355 | 217.8 | 1.51 | 18.68 | 1.32 | 223.9 | |
| T20-3 | 3993 | 199.7 | 2.17 | 18.32 | 1.68 | 207.5 | |
| T20-4 | 3763 | 188.2 | 1.21 | 18.25 | 1.75 | 197.7 | |
| T20-5 | 3873 | 193.7 | 1.01 | 18.13 | 1.87 | 201 | |
| T20-6 | 3965 | 198.3 | 1.12 | 18.2 | 1.8 | 207.8 | |
| 25 | Average line | 4582 | 184.4 | 1.22 | 23.35 | 1.65 | 193.0 |
| T25-1 | 4720 | 188.8 | 1.28 | 23.44 | 1.56 | 198.3 | |
| T25-2 | 4678 | 187.1 | 1.31 | 23.12 | 1.88 | 194.8 | |
| T25-3 | 4494 | 179.8 | 1.33 | 22.61 | 2.39 | 192.1 | |
| T25-4 | 4427 | 177.1 | 1.29 | 23.61 | 1.39 | 184.0 | |
| T25-5 | 4445 | 177.8 | 1.23 | 23.70 | 1.30 | 184.3 | |
| T25-6 | 4738 | 189.1 | 1.25 | 22.47 | 2.53 | 196.6 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, J.; Liu, G. Pullout Response of Ultra-High-Performance Concrete with Twisted Steel Fibers. Appl. Sci. 2019, 9, 658. https://doi.org/10.3390/app9040658
Ye J, Liu G. Pullout Response of Ultra-High-Performance Concrete with Twisted Steel Fibers. Applied Sciences. 2019; 9(4):658. https://doi.org/10.3390/app9040658
Chicago/Turabian StyleYe, Judong, and Guohua Liu. 2019. "Pullout Response of Ultra-High-Performance Concrete with Twisted Steel Fibers" Applied Sciences 9, no. 4: 658. https://doi.org/10.3390/app9040658
APA StyleYe, J., & Liu, G. (2019). Pullout Response of Ultra-High-Performance Concrete with Twisted Steel Fibers. Applied Sciences, 9(4), 658. https://doi.org/10.3390/app9040658





