Nondestructive Ultrasonic Inspection of Composite Materials: A Comparative Advantage of Phased Array Ultrasonic
Abstract
:Featured Application
Abstract
1. Introduction
2. Materials and Sample Preparation
2.1. SEUT Versus PAUT Methods
2.2. PAUT Guided Wave Method
3. Experimental Setup
3.1. SEUT Versus PAUT Methods
3.2. PAUT Guided Wave Method
4. Results and Discussions
4.1. SEUT Versus PAUT Methods
4.1.1. Focusing Depth Comparison
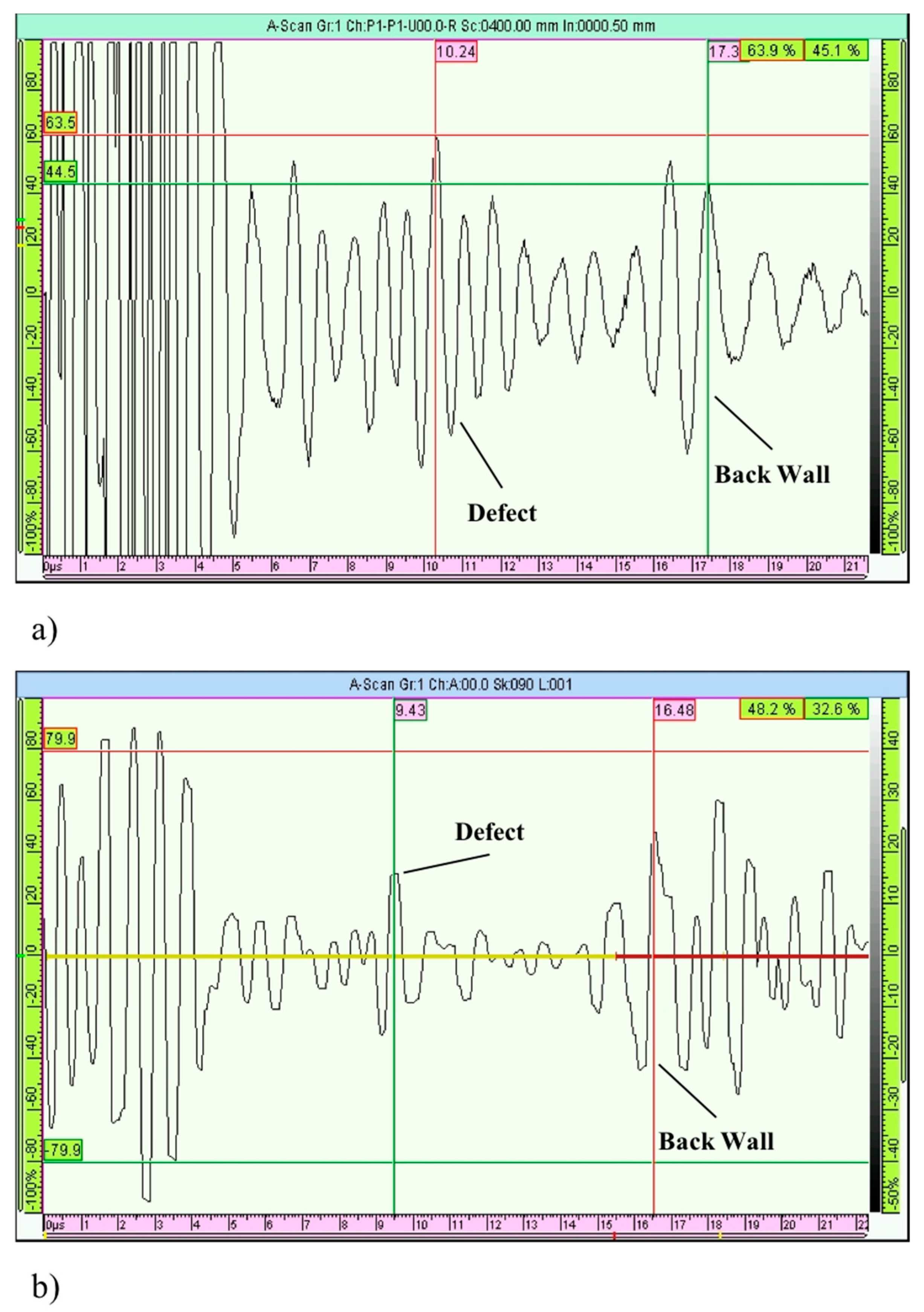
4.1.2. Sensitivity Comparison and Defect Detection
4.2. PAUT Guided Wave Method
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Poudel, A.; Shrestha, S.S.; Sandhu, J.S.; Chu, T.P.; Pergantis, C.G. Comparison and Analysis of Acoustography with Other NDE Techniques for Foreign Object Inclusion Detection in Graphite Epoxy Composites. Compos. Part B Eng. 2015, 78, 86–94. [Google Scholar] [CrossRef]
- Raišutis, R.; Jasiuniene, E.; Sliteris, R.; Vladišauskas, A. The review of non-destructive testing techniques suitable for inspection of the wind turbine blades. Ultrasound 2008, 63, 26–30. [Google Scholar]
- Amenabar, I.; Mendikute, A.; López-Arraiza, A.; Lizaranzu, M.; Aurrekoetxea, J. Comparison and analysis of non-destructive testing techniques suitable for delamination inspection in wind turbine blades. Compos. Part B Eng. 2011, 42, 1298–1305. [Google Scholar] [CrossRef]
- Adem, E.; Reddy, G.M.; Koricho, E.G.; Science, A.; Science, A.; Science, A.; Vehicle, C. Experimental Analysis of E-Glass/Epoxy & E-Glass/polyester Composites for Auto Body Panel. Am. Int. J. Res. Sci. Technol. Eng. Math. 2015, 10, 377–383. [Google Scholar]
- Ensminger, D.; Bond, L.J. Ultrasonics: Fundamentals, Technologies, and Applications, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Hübschen, G.; Altpeter, I.; Tschuncky, R.; Herrmann, H.-G. (Eds.) Materials Characterization Using Nondestructive Evaluation (NDE) Methods; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Taheri, H. Classification of Nondestructive Inspection Techniques with Principal Component Analysis (PCA) for Aerospace Application. In Proceedings of the ASNT 26th Research Symposium, Jacksonville, FL, USA, 13–16 March 2017; pp. 219–227. [Google Scholar]
- Taheri, H. Utilization of Non-Destructive Testing (NDT) Methods for Composite Material Inspection (Phased array Ultrasonic). Master’s Thesis, South Dakota State University, Brookings, South Dakota, 2014. [Google Scholar]
- Wertz, J.; Homa, L.; Welter, J.; Sparkman, D.; Aldrin, J.C. Case Study of Model-Based Inversion of the Angle Beam Ultrasonic Response from Composite Impact Damage. J. Nondestr. Eval. Diagn. Progn. Eng. Syst. 2018, 1, 41001–41010. [Google Scholar] [CrossRef]
- Taheri, H.; Delfanian, F.; Du, J. Ultrasonic phased array techniques for composite material evaluation. J. Acoust. Soc. Am. 2013, 134, 4013. [Google Scholar] [CrossRef]
- Taheri, H.; Ladd, K.M.; Delfanian, F.; Du, J. Phased array ultrasonic technique parametric evaluation for composite materials. In ASME International Mechanical Engineering Congress and Exposition, Proceedings (IMECE); American Society of Mechanical Engineers: New York, NY, USA, 2014; Volume 13, p. V013T16A028. [Google Scholar]
- Caminero, M.A.; García-Moreno, I.; Rodríguez, G.P.; Chacón, J.M. Internal damage evaluation of composite structures using phased array ultrasonic technique: Impact damage assessment in CFRP and 3D printed reinforced composites. Compos. Part B Eng. 2019, 165, 131–142. [Google Scholar] [CrossRef]
- Hassen, A.A.; Taheri, H.; Vaidya, U.K. Non-destructive investigation of thermoplastic reinforced composites. Compos. Part B Eng. 2016, 97, 244–254. [Google Scholar] [CrossRef] [Green Version]
- Aldrin, J.C.; Wertz, J.N.; Welter, J.T.; Wallentine, S.; Lindgren, E.A.; Kramb, V.; Zainey, D. Fundamentals of angled-beam ultrasonic NDE for potential characterization of hidden regions of impact damage in composites. AIP Conf. Proc. 2018, 1949, 120005. [Google Scholar]
- Toyama, N.; Ye, J.; Kokuyama, W.; Yashiro, S. Non-Contact Ultrasonic Inspection of Impact Damage in Composite Laminates by Visualization of Lamb wave Propagation. Appl. Sci. 2018, 9, 46. [Google Scholar] [CrossRef]
- Castellano, A.; Fraddosio, A.; Piccioni, M.D. Quantitative analysis of QSI and LVI damage in GFRP unidirectional composite laminates by a new ultrasonic approach. Compos. Part B Eng. 2018, 151, 106–117. [Google Scholar] [CrossRef]
- Taheri, H.; Delfanian, F.; Du, J. Acoustic Emission and Ultrasound Phased Array Technique for Composite Material Evaluation. In ASME International Mechanical Engineering Congress and Exposition, Proceedings (IMECE): Advances in Aerodynamics; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 1, p. V001T01A015. [Google Scholar]
- Bai, Z.; Chen, S.; Xiao, Q.; Jia, L.; Zhao, Y.; Zeng, Z. Compressive sensing of phased array ultrasonic signal in defect detection: Simulation study and experimental verification. Struct. Health Monit. 2017, 17, 434–449. [Google Scholar] [CrossRef]
- Taheri, H.; Du, J.; Delfanian, F. Experimental Observation of Phased Array Guided Wave Application in Composite Materials. Mater. Eval. 2017, 75, 1308–1316. [Google Scholar]
- Bolotina, I.; Borikov, V.; Ivanova, V.; Mertins, K.; Uchaikin, S. Application of phased antenna arrays for pipeline leak detection. J. Pet. Sci. Eng. 2018, 161, 497–505. [Google Scholar] [CrossRef]
- Taheri, H.; Koester, L.; Bigelow, T.; Bond, L.J.; Braconnier, D.; Carcreff, E.; Dao, A.; Caulder, L.; Hassen, A.A. Fast Ultrasonic Imaging with Total Focusing Method (TFM) for Inspection of Additively Manufactured Polymer Composite Component. In Proceedings of the 27th ASNT Research Symposium, São Paulo, Brazil, 27–29 August 2018; pp. 212–220. [Google Scholar]
- Drinkwater, B.W.; Wilcox, P.D. Ultrasonic arrays for non-destructive evaluation: A review. NDT&E Int. 2006, 39, 525–541. [Google Scholar]
- Fromme, P.; Wilcox, P.D.; Lowe, M.J.S.; Cawley, P. On the development and testing of a guided ultrasonic wave array for structural integrity monitoring. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 777–785. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leleux, A.; Micheau, P.; Castaings, M. Long Range Detection of Defects in Composite Plates Using Lamb Waves Generated and Detected by Ultrasonic Phased Array Probes. J. Nondestr. Eval. 2013, 32, 200–214. [Google Scholar] [CrossRef]
- Philtron, J.H.; Rose, J.L. Guided wave phased array sensor tuning for improved defect detection and characterization. In Proc. SPIE 9063, Nondestructive Characterization for Composite Materials, Aerospace Engineering, Civil Infrastructure, and Homeland Security; International Society for Optics and Photonics: San Diego, CA, USA, 2014; p. 906306. [Google Scholar]
- Wang, W.; Zhang, H.; Lynch, J.P.; Cesnik, C.E.S.; Li, H. Experimental and numerical validation of guided wave phased arrays integrated within standard data acquisition systems for structural health monitoring. Struct. Control Health Monit. 2018, 25, e2171. [Google Scholar] [CrossRef]
- Rose, J.L. Ultrasonic guided waves in structural health monitoring. Key Eng. Mater. 2004, 270, 14–21. [Google Scholar] [CrossRef]
- Rose, J.L. Ultrasonic Guided Waves in Solid Media; Ultrasonic Guided Waves in Solid Media; Cambridge University Press: Cambridge, UK, 2014; Volume 9781107048959, pp. 1–512. [Google Scholar]
- Wilcox, P.; Lowe, M.; Cawley, P. Effect of dispersion on long-range inspection using ultrasonic guided waves. NDT E Int. 2001, 34, 1–9. [Google Scholar] [CrossRef]
- Chimenti, D.E. Guided waves in plates and their use in materials characterization. Appl. Mech. Rev. 1997, 50, 247–284. [Google Scholar] [CrossRef]
- Rose, J.L. Successes and Challenges in Ultrasonic Guided Waves for NDT and SHM. In Proceedings of the National Seminar & Exhibition on Non-Destructive Evaluation, Pune, India, 4–6 December 2009. [Google Scholar]










| Sample Name | Material | Thickness (mm) |
|---|---|---|
| Al-1 | Aluminum 6063 (Plate) | 2.2 |
| Al-2 | Aluminum 6063 (Plate) | 0.635 |
| CFRP | Unidirectional Carbon Fiber Composite (5 layers of carbon fiber fabric) | 1.0 |
| Frequency | Method | Sample | Thickness (mm) | Gain (dB) | Time | Signal-to-Noise Ratio |
|---|---|---|---|---|---|---|
| 0.5 MHz | SEUT | GFRP | 4 | 42 | 5.01 | 7.95 |
| 10 | 55.8 | 9.87 | 3.99 | |||
| 12 | 60.5 | 11.01 | 3.07 | |||
| 18 | 61 | 14.73 | 2.98 | |||
| 25 | 64.7 | 18.92 | 2.53 | |||
| 1 MHz | SEUT | GFRP | 4 | 18.5 | 4.76 | 5.31 |
| 10 | 36 | 8.56 | 11.93 | |||
| 12 | 39.6 | 9.80 | 11.75 | |||
| 18 | 41.2 | 13.60 | 11.10 | |||
| 25 | 45.9 | 18.35 | 9.57 | |||
| 1.5 MHz | SEUT | GFRP | 4 | 15.5 | 4.67 | 5.14 |
| 10 | 37 | 8.48 | 11.75 | |||
| 12 | 40.8 | 9.76 | 11.10 | |||
| 18 | 43 | 13.55 | 9.51 | |||
| 25 | 46.7 | 18.25 | 9.43 | |||
| 1.5 MHz | PAUT | GFRP | 4 | 13 | 3.64 | 6.10 |
| 10 | 27 | 7.56 | 5.44 | |||
| 12 | 28.5 | 8.93 | 5.81 | |||
| 18 | 36 | 12.49 | 3.39 | |||
| 25 | 40 | 17.41 | 3.22 |
| Experimental Setup Parameters for PAUT Guided Wave Inspection of Al-1 Sample | ||||||||
| Frequency (MHz) | Thickness (mm) | Gain (dB) | Element Qty.1 | fd2 | Element Step3 | |||
| 1.5 | 2.2 | 30 | 4 | 3.3 | 1 | |||
| 1 Number of active elements at each sequence in phased array ultrasound transducer 2 frequency plate thickness 3 Incremental steps in terms of number of elements at each sequence | ||||||||
| Defect Detection Signal Characteristics | ||||||||
| Experimental Trials # | Hole Diameter (mm) | Signal | Distance of Transducer from The Edge (mm) | Arrival Time (us) | DTime (us) | Amplitude (%) | ||
| Edge | Hole | Edge | Hole | |||||
| 1 | 1 | Edge/Hole | 50 | 58.07 | 47.61 | 10.5 | 53.9 | 46.6 |
| 2 | Edge/Hole | 100 | 87.99 | 70.27 | 17.7 | 33.1 | 24.3 | |
| 3 | Edge/Hole | 150 | 109.77 | 92.34 | 17.4 | 10.3 | 21.1 | |
| Experimental Setup Parameters for PAUT Guided Wave Inspection of Al-2 Sample | ||||||||
| Frequency (MHz) | Thickness (mm) | Gain (dB) | Element Qty.1 | fd2 | Element Step3 | |||
| 1.5 | 0.635 | 30 | 4 | 0.96 | 1 | |||
| 1 Number of active elements at each sequence in phased array ultrasound transducer 2 frequency plate thickness 3 Incremental steps in terms of number of elements at each sequence | ||||||||
| Defect Detection Signal Characteristics | ||||||||
| Experimental Trial # | Hole Diameter(mm) | Signal | Distance of Transducer from The Edge (mm) | Arrival Time (us) | DTime (us) | Amplitude (%) | ||
| Edge | Hole | Edge | Hole | |||||
| 1 | 1 | Edge/Hole | 50 | 39.19 | 28.15 | 11.0 | 100 | 15.5 |
| 2 | Edge/Hole | 100 | 57.78 | 46.45 | 11.3 | 100 | 11.5 | |
| 3 | Edge/Hole | 175 | 85.08 | 74.34 | 10.7 | 82.7 | 7.8 | |
| 4 | Edge/Hole | 200 | 94.09 | 83.05 | 11.0 | 77.7 | 4.5 | |
| Experimental Setup Parameters for PAUT Guided Wave Inspection of CFRP | |||||||
| Frequency (MHz) | Thickness(mm) | Gain (dB) | Element Qty.1 | fd2 | Element Step3 | ||
| 1.5 | 1 | 35 | 4 | 1.5 | 1 | ||
| 1 Number of active elements at each sequence in phased array ultrasound transducer 2 frequency plate thickness 3 Incremental steps in terms of number of elements at each sequence | |||||||
| Defect Detection Signal Characteristics | |||||||
| Experimental Trial # | Hole Diameter(mm) | Signal | Distance of Transducer from The Edge(mm) | Arrival Time (us) (Edge/Hole) | DTime (us) | Ddist (mm) | |
| Edge | Hole | ||||||
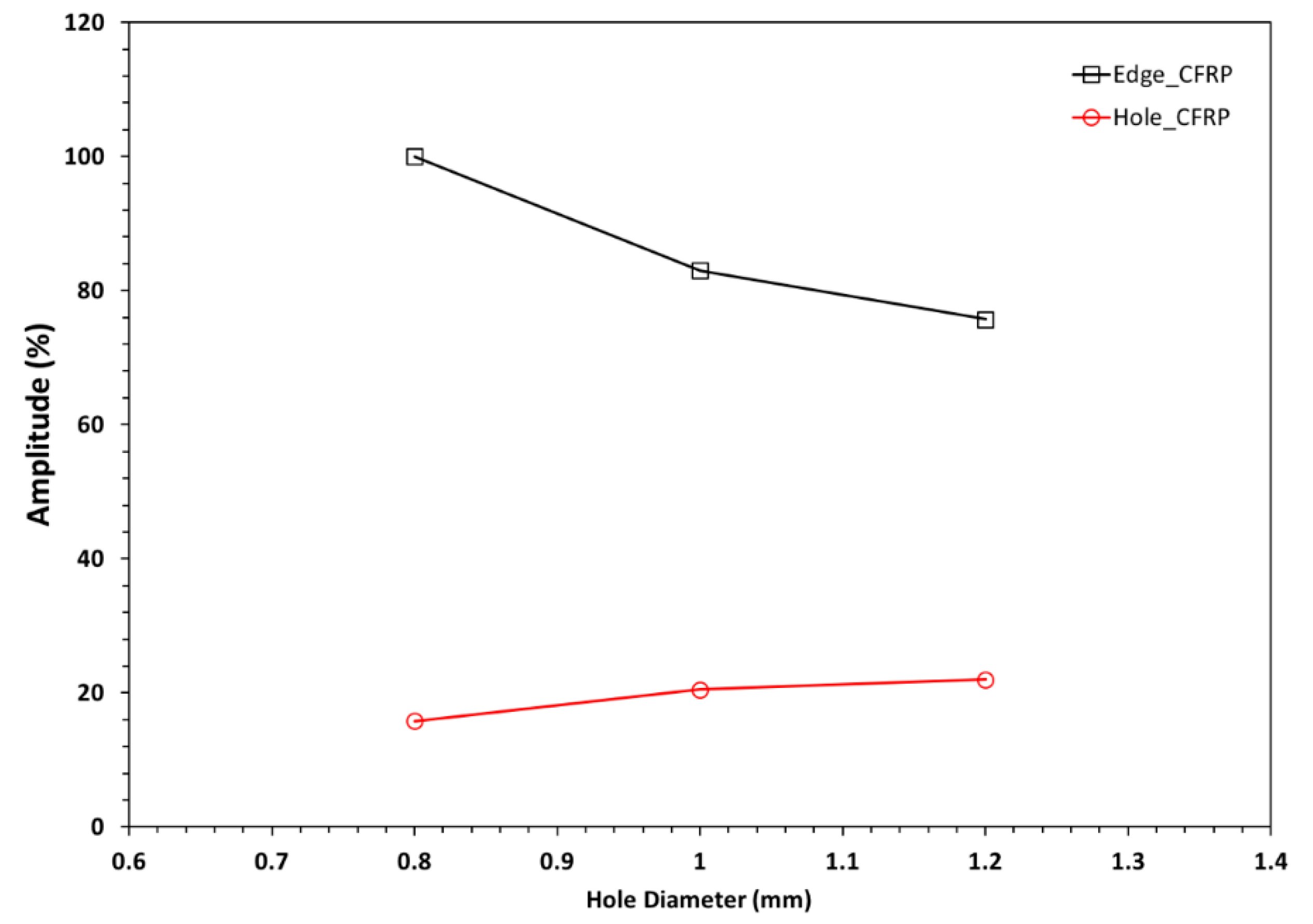
| 1 | 0.8 | Edge/Hole | 50 | 25.64 | 13.81 | 11.83 | 48.9 |
| 2 | Edge/Hole | 100 | 35.6 | 24.54 | 11.06 | 45.7 | |
| 3 | Edge/Hole | 150 | 46.11 | 37.08 | 9.03 | 37.3 | |
| 1 | 1 | Edge/Hole | 50 | 26.76 | 14.07 | 12.69 | 52.5 |
| 2 | Edge/Hole | 100 | 35.62 | 26.11 | 9.51 | 39.3 | |
| 3 | Edge/Hole | 150 | 45.97 | 37.28 | 8.69 | 35.9 | |
| 1 | 1.2 | Edge/Hole | 50 | 24.52 | 14.11 | 10.41 | 43.0 |
| 2 | Edge/Hole | 100 | 36.24 | 26.89 | 9.35 | 38.6 | |
| 3 | Edge/Hole | 150 | 46.25 | 37.36 | 8.89 | 36.7 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taheri, H.; Hassen, A.A. Nondestructive Ultrasonic Inspection of Composite Materials: A Comparative Advantage of Phased Array Ultrasonic. Appl. Sci. 2019, 9, 1628. https://doi.org/10.3390/app9081628
Taheri H, Hassen AA. Nondestructive Ultrasonic Inspection of Composite Materials: A Comparative Advantage of Phased Array Ultrasonic. Applied Sciences. 2019; 9(8):1628. https://doi.org/10.3390/app9081628
Chicago/Turabian StyleTaheri, Hossein, and Ahmed Arabi Hassen. 2019. "Nondestructive Ultrasonic Inspection of Composite Materials: A Comparative Advantage of Phased Array Ultrasonic" Applied Sciences 9, no. 8: 1628. https://doi.org/10.3390/app9081628
APA StyleTaheri, H., & Hassen, A. A. (2019). Nondestructive Ultrasonic Inspection of Composite Materials: A Comparative Advantage of Phased Array Ultrasonic. Applied Sciences, 9(8), 1628. https://doi.org/10.3390/app9081628






