Effect of Stabilized nZVI Nanoparticles on the Reduction and Immobilization of Cr in Contaminated Soil: Column Experiment and Transport Modeling
Abstract
1. Introduction
2. Materials and Methods
2.1. Soil Sampling and Analysis
2.2. Synthesis and Characterization of Stabilized nZVI Nanoparticles
2.3. Batch Experiments of Cr(VI) Reduction in the Soil
2.4. Column Experiments
2.5. Simulation of Cr Removal and Transport
2.6. Statistical Analysis and Quality Control
3. Results and Discussions
3.1. Chemical and Physical Properties of the Collected Soil
3.2. Characterization of the Synthesized nZVI Nanoparticles
3.2.1. BET Surface Area and Microporosity
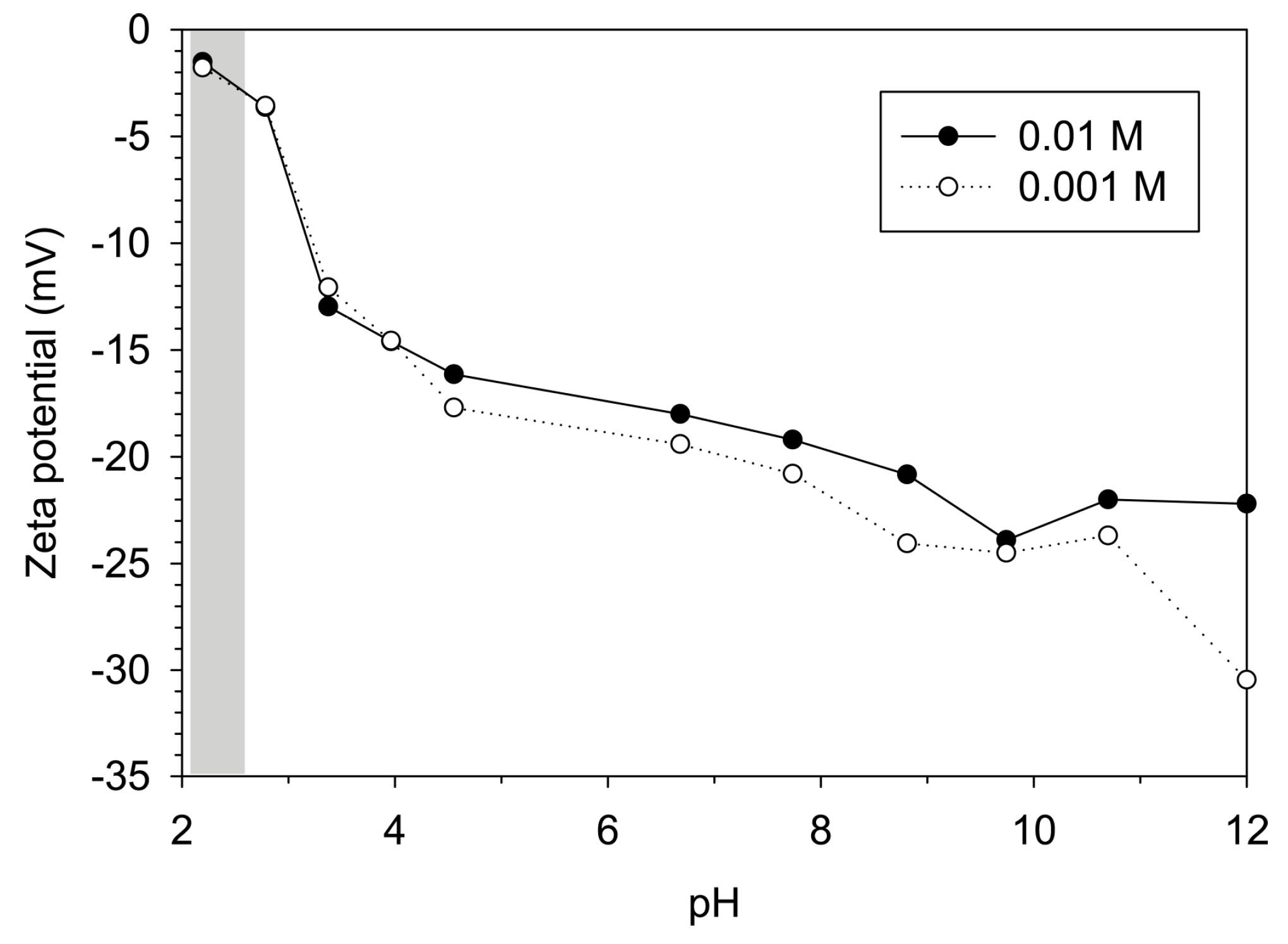
3.2.2. Hydrodynamic Size and Zeta Potential
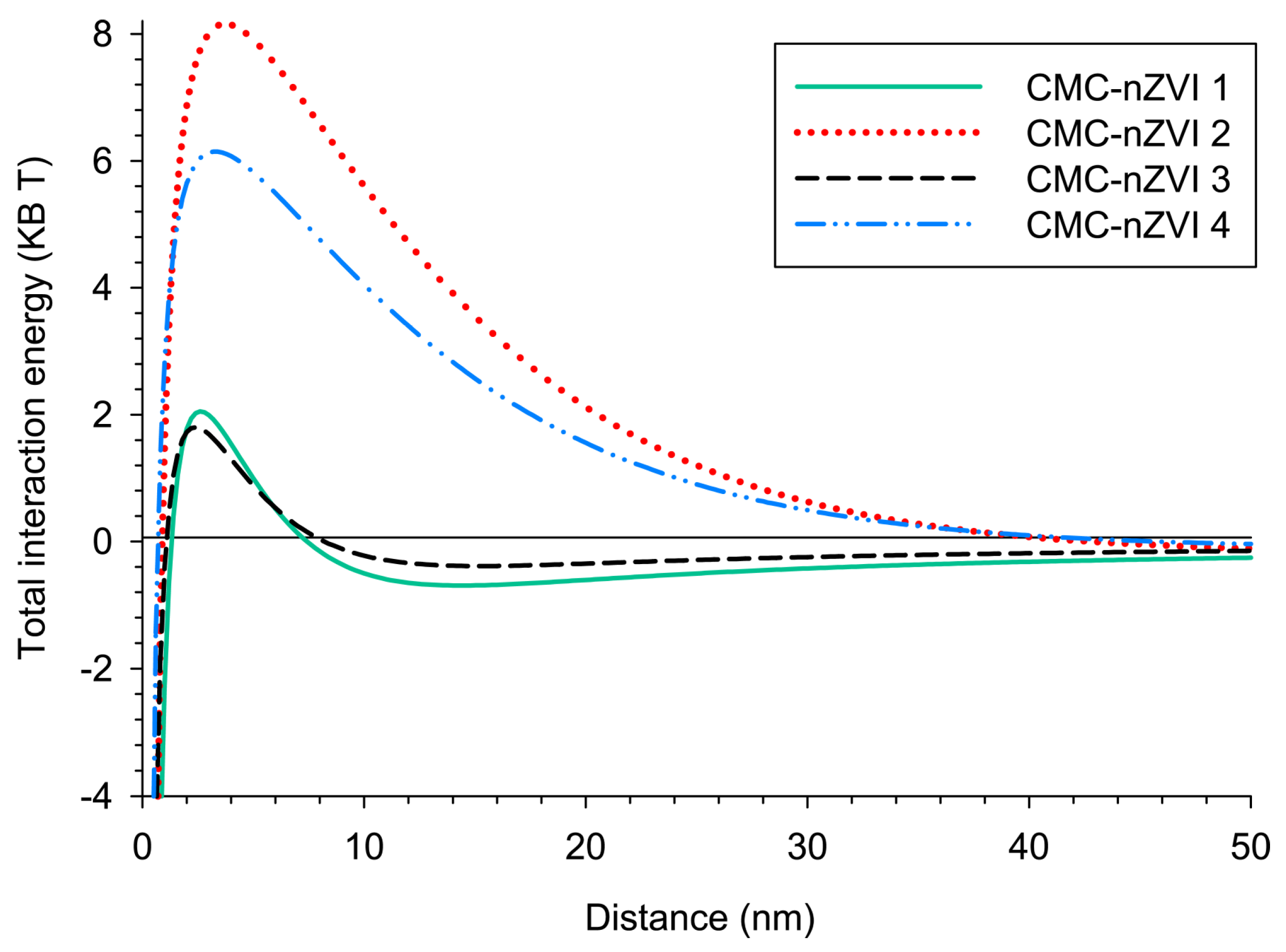
3.2.3. Total Interaction Energy (DLVO)
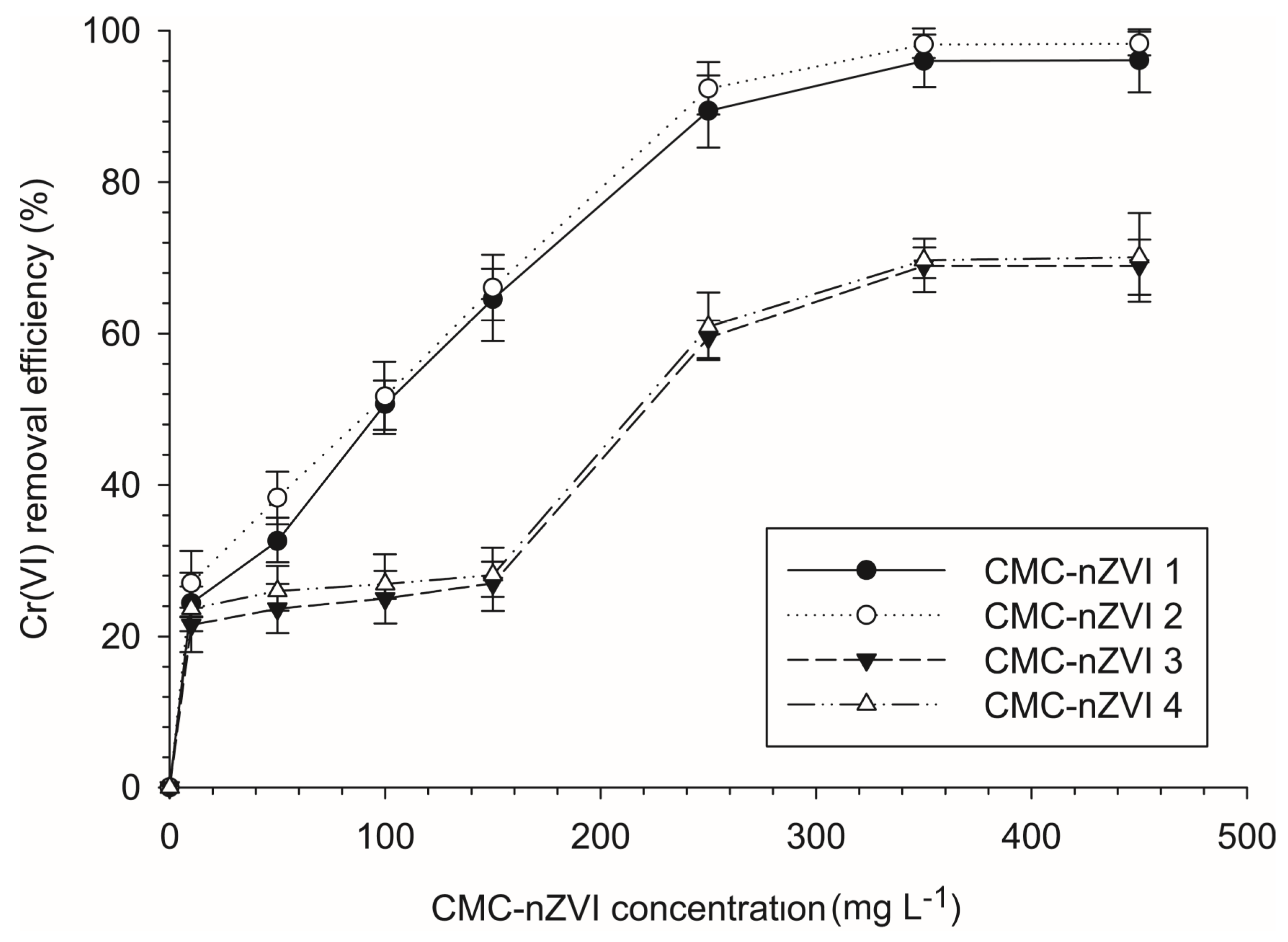
3.3. Effect of the Concentration of CMC-nZVI on the Reduction Efficiency of Cr(VI)
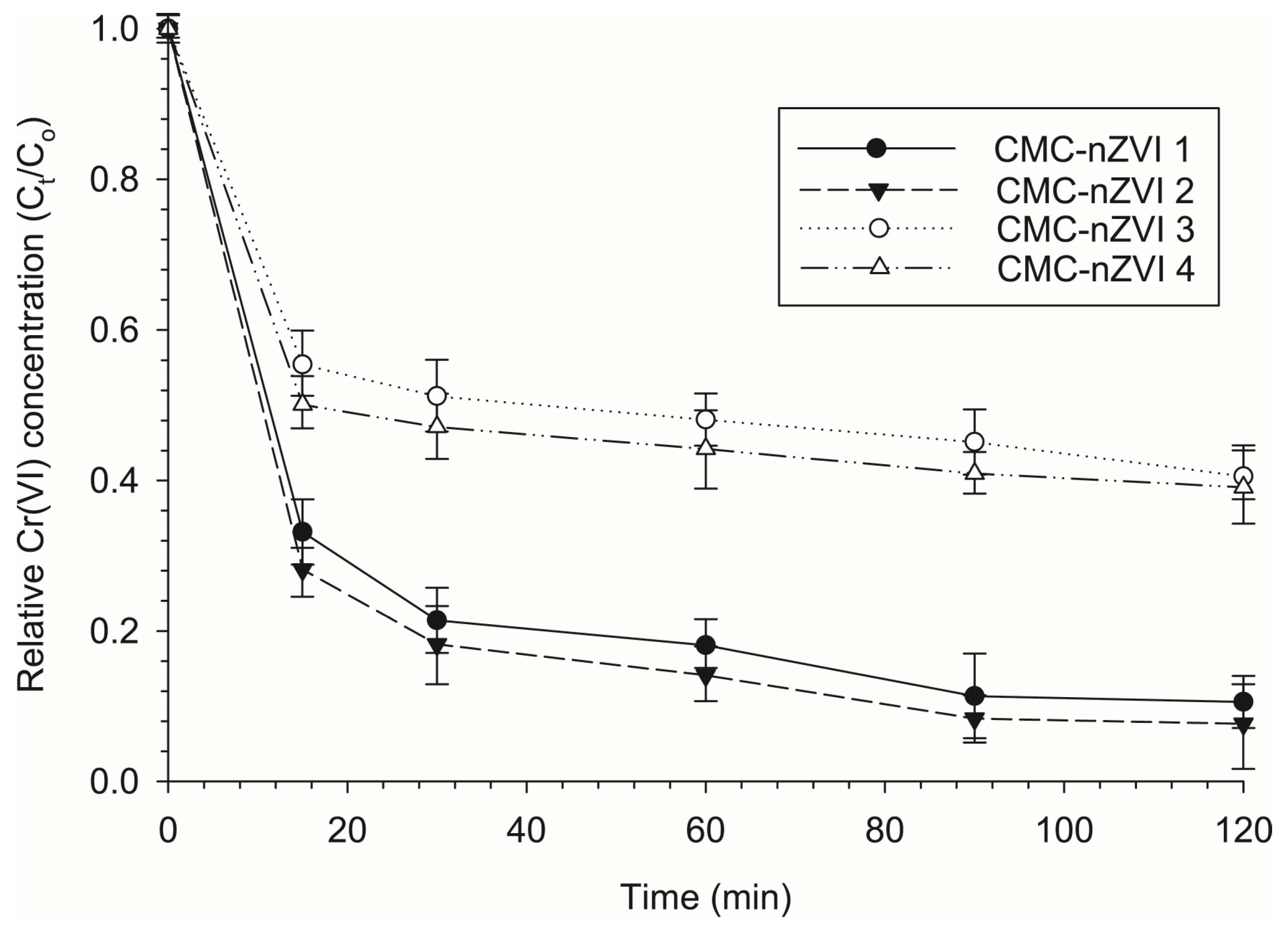
3.4. Effect of Contact Time on the Reduction Efficiency of Cr(VI)
3.5. Effect of pH and Is on the Reduction Efficiency of Cr(VI)
3.6. Adsorption Kinetics of Cr(VI)
3.7. Column Transport of Cr(VI)
3.8. Modeling the Transport of Cr(VI) (Uniform Model)
3.9. Modeling the Transport of Cr(VI) (Two-Site Sorption Model)
3.10. Effect of Flow Rate on the Transport of Cr(VI)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Calculations of the of Ionic Strength of the Background Electrolyte Solution, the Debye–Huckel Length, and the DLVO Interactions of the CMC-nZVI Nanoparticles
| Symbol | Definition | Units/Comments |
|---|---|---|
| Cj | Molar concentration | Mole∙L−1 |
| Zj | Valence of ion | - |
| e0 | Permittivity of free space | F m−1 |
| ɛ | Dielectric Constant | 80 for water |
| kB | Boltzmann constant | J K−1 |
| T | Absolute temperature | °K |
| NA | Avogadro’s Number | 1 mole−1 |
| e | Electron charge | Coulomb |
| I | Ionic strength | Mole∙m−3 |
| The radius of the first interacting CMC-nZVI | nm | |
| The radius of the second interacting CMC-nZVI | nm | |
| The bulk number density of ions | - | |
| The inverse Debye–Huckel length | m | |
| The surface potentials of interacting CMC-nZVI | mV, Zeta potential value is used | |
| The separation distance between two particles | nm | |
| The Hamaker constant for: CMC-nZVI-water-CMC-nZVI | 3.5 × 10−20 J | |
| The characteristics wavelength of the interaction | 100 nm (Gregory, 1981 [55]) |
References
- Su, M.; Yin, W.Z.; Liu, L.; Li, P.; Fang, Z.Q.; Chiang, P.C.; Wu, J.H. Enhanced Cr(VI) stabilization in soil by carboxymethyl cellulose-stabilized nanosized Fe0 (CMCnFe0) and mixed anaerobic microorganisms. J. Environ. Manag. 2020, 257, 109951. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Yang, L.; Zhao, H.; Wang, W. Pyrolytic production of zerovalent iron nanoparticles supported on rice husk-derived biochar: Simple, in situ synthesis and use for remediation of Cr(VI)-polluted soils. Sci. Total Environ. 2020, 708, 134479. [Google Scholar] [CrossRef] [PubMed]
- Latif, A.; Sheng, D.; Sun, K.; Si, Y.; Azeem, M.; Abbas, A.; Bilal, M. Remediation of heavy metals polluted environment using Fe-based nanoparticles: Mechanisms, influencing factors, and environmental implications. Environ. Pollut. 2020, 264, 114728. [Google Scholar] [CrossRef] [PubMed]
- Sousa, A.; Pereira, R.; Antunes, S.; Cachada, A.; Pereira, E.; Duarte, A.; Gonçalves, F. Validation of avoidance assays for the screening assessment of soils under different anthropogenic disturbances. Ecotoxicol. Environ. Saf. 2008, 71, 661–670. [Google Scholar] [CrossRef] [PubMed]
- Van der Perk, M. Soil and Water Contamination 2nd Edition (Special Sale Only): From Molecular to Catchment Scale, 1st ed.; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Jovanović, V.S.; Mitić, V.; Mandić, S.N.; Ilić, M.; Simonović, S. Heavy metals in the post-catastrophic soils. Soil Biol. 2015, 44, 3–21. [Google Scholar]
- Aboutorabi, L.; Morsali, A.; Tahmasebi, E.; Büyükgüngor, O. Metal–Organic Framework Based on Isonicotinate N-Oxide for Fast and Highly Efficient Aqueous Phase Cr(VI) Adsorption. Inorg. Chem. 2016, 55, 5507–5513. [Google Scholar] [CrossRef] [PubMed]
- Chrysochoou, M.; Johnston, C.P.; Dahal, G. A comparative evaluation of hexavalent chromium treatment in contaminated soil by calcium polysulfide and green-tea nanoscale zero-valent iron. J. Hazard. Mater. 2012, 201, 33–42. [Google Scholar] [CrossRef] [PubMed]
- Du, J.; Lu, J.; Wu, Q.; Jing, C. Reduction and immobilization of chromate in chromite ore processing residue with nanoscale zero-valent iron. J. Hazard. Mater. 2012, 215, 152–158. [Google Scholar] [CrossRef] [PubMed]
- Fu, F.; Dionysiou, D.D.; Liu, H. The use of zero-valent iron for groundwater remediation and wastewater treatment: A review. J. Hazard. Mater. 2014, 267, 194–205. [Google Scholar] [CrossRef]
- Mueller, N.C.; Braun, J.; Bruns, J.; ČErník, M.; Rissing, P.; Rickerby, D.; Nowack, B. Application of nanoscale zero valent iron (NZVI) for groundwater remediation in Europe. Environ. Sci. Pollut. Res. 2011, 19, 550–558. [Google Scholar] [CrossRef]
- Motzer, W.E. Chemistry, Geochemistry, and Geology of Chromium and Chromium Compounds. In Chromium (VI) Handbook; Guertin, J., Avakian, C.P., Jacobs, J.A., Eds.; CRC Press: Boca Raton, FL, USA, 2004; Chapter 2. [Google Scholar]
- Xu, Y.; Zhao, D. Reductive immobilization of chromate in water and soil using stabilized iron nanoparticles. Water Res. 2007, 41, 2101–2108. [Google Scholar] [CrossRef] [PubMed]
- Sallam, A.; Al-Zahrani, M.; Al-Wabel, M.; Al-Farraj, A.; Usman, A. Removal of Cr(VI) and Toxic Ions from Aqueous Solutions and Tannery Wastewater Using Polymer-Clay Composites. Sustainability 2017, 9, 1993. [Google Scholar] [CrossRef]
- Almasoud, F.I.; Usman, A.R.; Al-Farraj, A.S. Heavy metals in the soils of the Arabian Gulf coast affected by industrial activities: Analysis and assessment using enrichment factor and multivariate analysis. Arab. J. Geosci. 2014, 8, 1691–1703. [Google Scholar] [CrossRef]
- Al-Hammad, B.A.; Abd El-Salam, M.M. Evaluation of heavy metal pollution in water wells and soil using common leafy green plant indicators in the Al-Kharj region, Saudi Arabia. Environ. Monit. Assess. 2016, 188, 324. [Google Scholar] [CrossRef] [PubMed]
- Al-Farraj, A.S.; Al-Wabel, M.I.; El-Saeid, M.H.; El-Naggar, A.H.; Ahmed, Z. Evaluation of Groundwater for Arsenic Contamination Using Hydrogeochemical Properties and Multivariate Statistical Methods in Saudi Arabia. J. Chem. 2013, 2013, 812365. [Google Scholar] [CrossRef]
- Sallam, A.S.; Usman, A.R.A.; Al-Makrami, H.A.; Al-Wabel, M.I.; Al-Omran, A. Environmental assessment of tannery wastes in relation to dumpsite soil: A case study from Riyadh, Saudi Arabia. Arab. J. Geosci. 2015, 8, 11019–11029. [Google Scholar] [CrossRef]
- Shi, L.N.; Lin, Y.M.; Zhang, X.; Chen, Z.L. Synthesis, characterization and kinetics of bentonite supported nZVI for the removal of Cr(VI) from aqueous solution. Chem. Eng. J. 2011, 171, 612–617. [Google Scholar] [CrossRef]
- Singh, R.; Misra, V.; Singh, R.P. Removal of Cr(VI) by Nanoscale Zero-valent Iron (nZVI) From Soil Contaminated with Tannery Wastes. Bull. Environ. Contam. Toxicol. 2012, 88, 210–214. [Google Scholar] [CrossRef]
- Zhang, S.; Lyu, H.H.; Tang, J.C.; Song, B.R.; Zhen, M.N.; Liu, X.M. A novel biochar supported CMC stabilized nano zero-valent iron composite for hexavalent chromium removal from water. Chemosphere 2019, 217, 686–694. [Google Scholar] [CrossRef]
- Chang, M.C.; Kang, H.Y. Remediation of pyrene-contaminated soil by synthesized nanoscale zero-valent iron particles. J. Environ. Sci. Health Part A 2009, 44, 576–582. [Google Scholar] [CrossRef]
- Kocur, C.M.; O’Carroll, D.M.; Sleep, B.E. Impact of nZVI stability on mobility in porous media. J. Contam. Hydrol. 2013, 145, 17–25. [Google Scholar] [CrossRef] [PubMed]
- Yang, G.; TU, H.; Hung, C. Stability of nanoiron slurries and their transport in the subsurface environment. Sep. Purif. Technol. 2007, 58, 166–172. [Google Scholar] [CrossRef]
- He, F.; Zhao, D. Manipulating the Size and Dispersibility of Zerovalent Iron Nanoparticles by Use of Carboxymethyl Cellulose Stabilizers. Environ. Sci. Technol. 2007, 41, 6216–6221. [Google Scholar] [CrossRef] [PubMed]
- Chowdhury, A.I.; Krol, M.M.; Kocur, C.M.; Boparai, H.K.; Weber, K.P.; Sleep, B.E.; O’Carroll, D.M. nZVI injection into variably saturated soils: Field and modeling study. J. Contam. Hydrol. 2015, 183, 16–28. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Dave, R.N.; Pfeffer, R. Polymer coating/encapsulation of nanoparticles using a supercritical anti-solvent process. J. Supercrit. Fluids 2004, 28, 85–99. [Google Scholar] [CrossRef]
- Sparks, D.L.; Page, A.L.; Helmke, P.A.; Loeppert, R.H. Methods of Soil Analysis Part 3: Chemical Methods; SSSA Book Series; John Wiley & Sons: Hoboken, NJ, USA, 1996. [Google Scholar]
- Gee, W.G.; Or, D. Particle-size analysis. In Methods of Soil Analysis; Book Series: 5; Dane, J., Topp, G.C., Eds.; Soil Science Society of America: Madison, WI, USA, 2002; Part 4; pp. 255–293. [Google Scholar]
- Dane, J.H.; Hopmans, J.W. Hanging water column. In Methods of Soil Analysis; Part 4. Physical Methods; Soil Science Society of America: Madison, WI, USA, 2002; Volume 5. [Google Scholar]
- Reynolds, W.D.; Elrick, D.E.; Youngs, E.G.; Amoozegar, A.; Booltink, H.W.G.; Bouma, J. Saturated and field-saturated water flow parameters. In Methods of Soil Analysis; Part 4. Physical Methods; Soil Science Society of America, Inc.: Madison, WI, USA, 2002; Volume 5, pp. 797–878. [Google Scholar]
- Hossner, L.R. Dissolution for Total Elemental Analysis. In SSSA Book Series; Soil Science Society of America: Madison, WI, USA, 1996; pp. 49–64. [Google Scholar]
- Johnson, R.L.; Nurmi, J.T.; Johnson, G.S.O.; Fan, D.M.; Johnson, R.L.O.; Shi, Z.Q.; Salter-Blanc, A.J.; Tratnyek, P.G.; Lowry, G.V. Field-scale transport and transformation of carboxymethylcellulose-stabilized nano zero-valent iron. Environ. Sci. Technol 2013, 47, 1573–1580. [Google Scholar] [CrossRef] [PubMed]
- Balamurugan, S.; Ashna, L.; Parthiban, P. Synthesis of Nanocrystalline MgO Particles by Combustion Followed by Annealing Method Using Hexamine as a Fuel. J. Nanotechnol. 2014, 2014, 841803. [Google Scholar] [CrossRef]
- Zhu, S.; Huang, X.; Wang, D.; Wang, L.; Ma, F. Enhanced hexavalent chromium removal performance and stabilization by magnetic iron nanoparticles assisted biochar in aqueous solution: Mechanisms and application potential. Chemosphere 2018, 207, 50–59. [Google Scholar] [CrossRef] [PubMed]
- Rudzinski, W.; Pluzinski, W. Kinetics of solute adsorption at solid/ solution interfaces: A theoretical development of the empirical pseudo-first and pseudo-second order kinetic rate equations, based on applying the statistical rate theory of interfacial transport. J. Phys. Chem. B 2006, 110, 16514–16525. [Google Scholar] [CrossRef]
- Ho, Y.S.; McKay, G. Pseudo-second order model for sorption processes. Process Biochem. 1999, 34, 451–465. [Google Scholar] [CrossRef]
- Toride, N.; Leij, F.J.; van Genuchten, M.T. The CXTFIT code for estimating transport parameters from laboratory or field tracer experiments. In Research Report Vol. 137 of US Salinity Laboratory, Agricultural Research Service, Version 2.1; US Department of Agriculture: Riverside, CA, USA, 1999. [Google Scholar]
- Šimůnek, J.; Genuchten, M.T.; Šejna, M. Development and Applications of the HYDRUS and STANMOD Software Packages and Related Codes. Vadose Zone J. 2008, 7, 587–600. [Google Scholar] [CrossRef]
- van Genuchten, M.T.; Leij, F.J.; Yates, S.R. The RETC Code for Quantifying the Hydraulic Functions of Unsaturated Soils; EPA/600/2-91/065; U.S. Salinity Laboratory (USDA, ARS): Riverside, CA, USA, 1991. [Google Scholar]
- Phenrat, T.; Long, T.C.; Lowry, G.V.; Veronesi, B. Partial oxidation (“aging”) and surface modification decrease the toxicity of nanosized zerovalent iron. Environ. Sci. Technol. 2009, 43, 195–200. [Google Scholar] [CrossRef] [PubMed]
- Gueye, M.T.; Petrucci, E.; Palma, L.D. Chemical reduction of hexavalent chromium (VI) in soil slurry by nano zero valent iron. Chem. Eng. Trans. 2015, 43, 655–660. [Google Scholar]
- Shu, Y.R.; Bin, J.I.; Baihui, C.; Yuting, S.; Wang, J.; Hu, M.; Luo, S.; Guo, D. Almond shell-derived, biochar-supported, nano-zero-valent iron composite for aqueous hexavalent chromium removal: Performance and mechanisms. Nanomaterials 2020, 10, 2. [Google Scholar] [CrossRef]
- Xilu, C.; Xiaomin, L.; Dandan, X.; Weichun, Y.; Shaoyuan, B. Application of nanoscale zero-valent iron in hexavalent chromium-contaminated soil: A Review. Nanotechnol. Rev. 2020, 9, 736–750. [Google Scholar]
- Haitao, W.; Yue, W.; Zhenglong, L.; Shuangjiang, L.; Valentin, R.; Xianqiang, H.; Bożena, C.; Hongwen, S.; Tielong, L. Rational construction of micron-sized zero-valent iron/graphene composite for enhanced Cr(VI) removal from aqueous solution. J. Environ. Chem. Eng. 2022, 10, 109004. [Google Scholar]
- Xu, T.; Nan, F.; Jiang, X.; Tang, Y.; Zeng, Y.; Zhang, W.; Shi, B. Effect of soil pH on the transport, fractionation, and oxidation of chromium(III). Ecotoxicol. Environ. Saf. 2020, 195, 110459. [Google Scholar] [CrossRef]
- Phenrat, T.; Saleh, N.; Sirk, K.; Kim, H.J.; Tilton, R.D.; Lowry, G.V. Stabilization of aqueous nanoscale zerovalent iron dispersions by anionic polyelectrolytes: Adsorbed anionic polyelectrolyte layer properties and their effect on aggregation and sedimentation. J. Nanopart. Res. 2008, 10, 795–814. [Google Scholar] [CrossRef]
- Di Palma, L.; Verdone, N.; Vilardi, G. Kinetic modeling of Cr(VI) reduction by nZVI in soil: The influence of organic matter and manganese oxide. Bull. Environ. Contam. Toxicol. 2018, 101, 692–697. [Google Scholar] [CrossRef]
- Hao, B.; Jiang, W.; Tariq, M.; Gehui, W.; Li, S.; Lingling, J.; Huadong, W.; Yinjie, Z.; Cheng, P.; Wei, Z.; et al. Computational study and optimization experiment of nZVI modified by anionic and cationic polymer for Cr(VI) stabilization in soil: Kinetics and response surface methodology (RSM). Environ. Pollut. 2021, 276, 116745. [Google Scholar]
- Zhu, F.; Li, L.; Ren, W.; Deng, X.; Liu, T. Effect of pH, temperature, humic acid and coexisting anions on reduction of Cr(Ⅵ) in the soil leachate by nZVI/Ni bimetal material. Environ. Pollut. 2017, 227, 444–450. [Google Scholar] [CrossRef] [PubMed]
- Pantsar-Kallio, M.; Reinikainen, S.P.; Oksanen, M. Interactions of soil components and their effects on speciation of chromium in soils. Anal. Chim. Acta 2001, 439, 9–17. [Google Scholar] [CrossRef]
- Fonseca, B.; Teixeira, H.; Figueiredo, H.; Tavares, T. Modelling of the Cr(VI) transport in typical soils of the North of Portugal. J. Hazard. Mater. 2009, 167, 756–762. [Google Scholar] [CrossRef] [PubMed]
- Huang, D.; Ren, Z.; Li, X.; Jing, Q. Mechanism of Stability and Transport of Chitosan-Stabilized Nano Zero-Valent Iron in Saturated Porous Media. Int. J. Environ. Res. Public Health 2021, 18, 5115. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharjee, S.; Elimelech, M. Surface element integration: A novel technique for evaluation of DLVO interaction between a particle and a flat plate. J. Colloid Interface Sci. 1997, 193, 273–285. [Google Scholar] [CrossRef]
- Gregory, J. Approximate expressions for retarded van der Waals interaction. J. Colloid Interface Sci. 1981, 51, 44–51. [Google Scholar] [CrossRef]


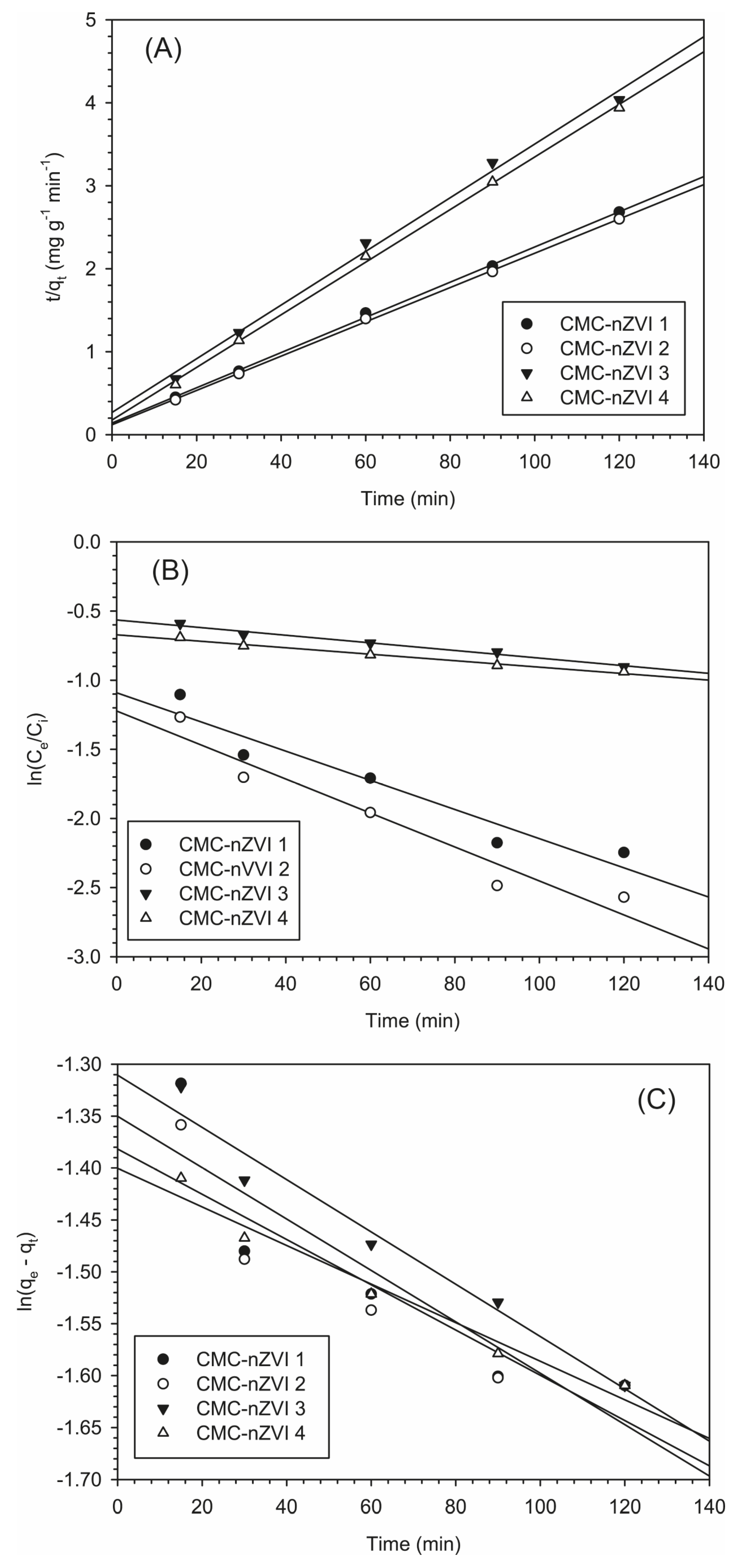
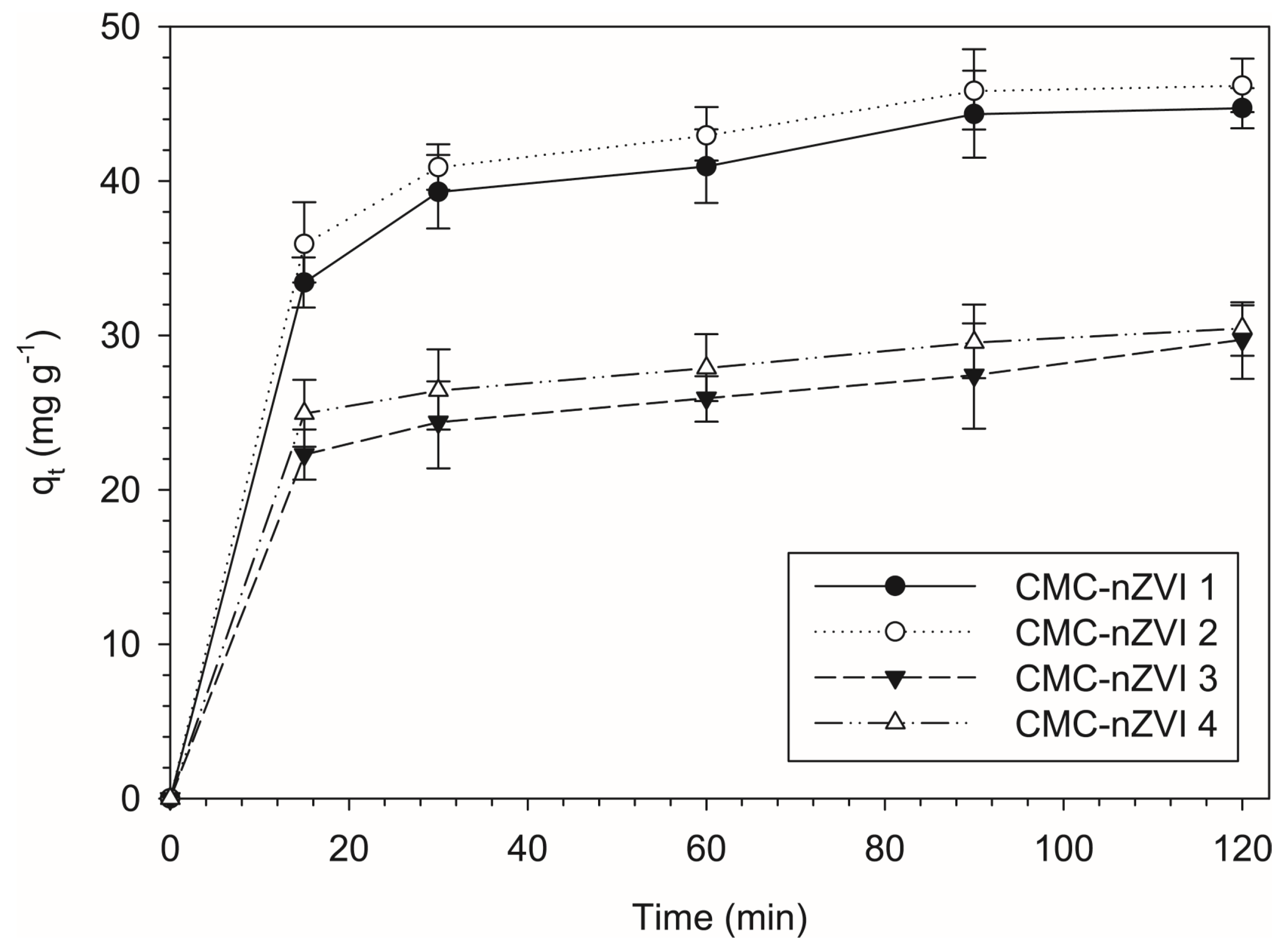

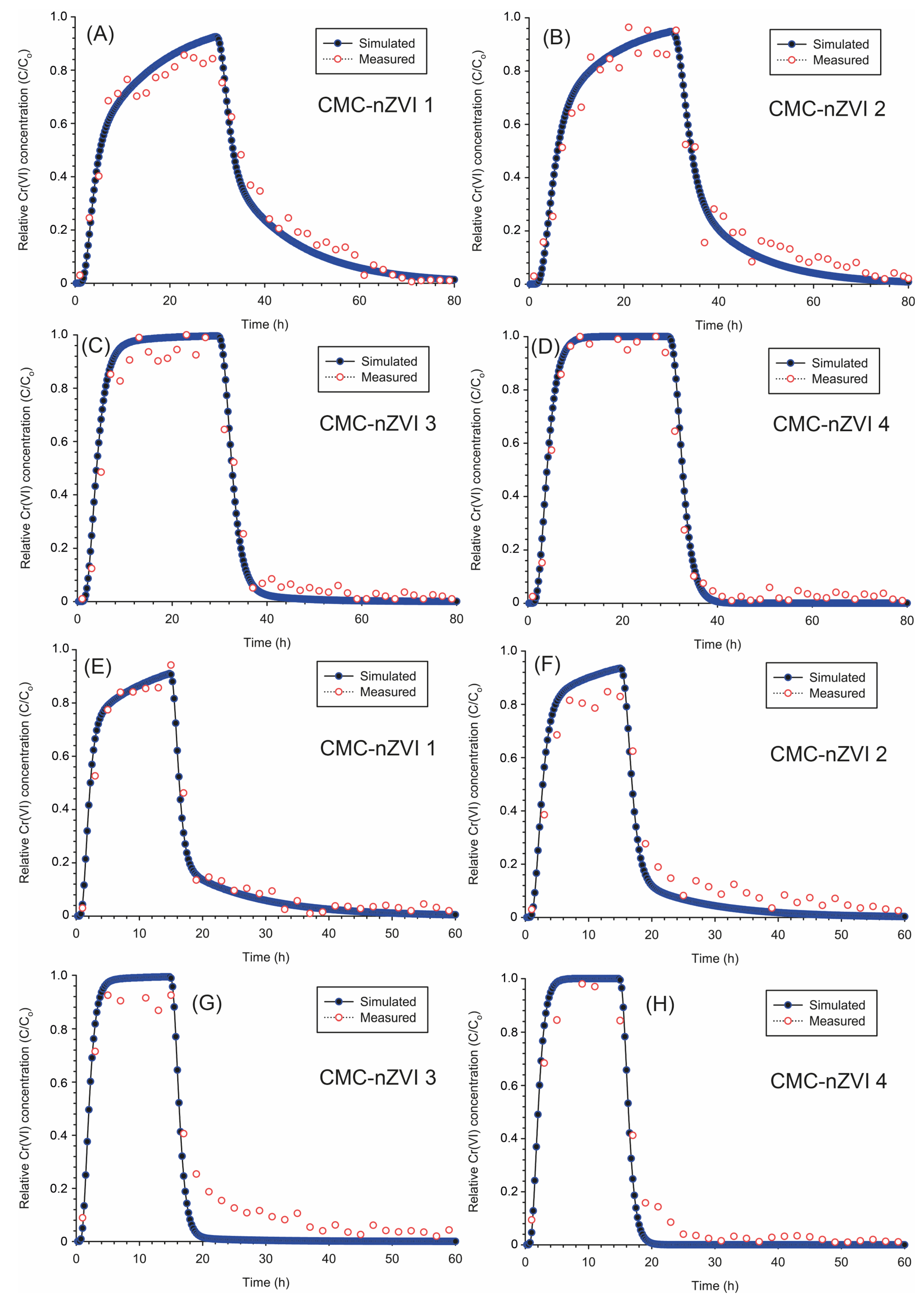
| Measurement | Unit | Value |
|---|---|---|
| Sand | % | 81.5 |
| Silt | % | 11.4 |
| Clay | % | 7.1 |
| Texture | - | Loamy sand |
| SHC | cm s−1 | 11.4 × 10−4 |
| pH | - | 7.85 |
| EC | dS m−1 | 1.92 |
| OM | % | 0.18 |
| CEC | cmolc∙kg−1 | 6.2 |
| Calcium | meq L−1 | 4.17 |
| Magnesium | meq L−1 | 1.33 |
| Sodium | meq L−1 | 12.54 |
| Potassium | meq L−1 | 1.05 |
| Bicarbonate | meq L−1 | 1.17 |
| Chloride | meq L−1 | 10.60 |
| Sulphate | meq L−1 | 7.32 |
| Cr(VI) | mg∙kg−1 | 7.4 |
| CMC-nZVI Suspensions | pH | IS (M) | Hydrodynamic Size (nm) | Zeta Potential (mV) |
|---|---|---|---|---|
| CMC-nZVI 1 | 5 | 0.01 | 131.2 ± 12.5 | −16.8 ± 1.2 |
| CMC-nZVI 2 | 5 | 0.001 | 129.8 ± 14.2 | −18.1 ± 2.3 |
| CMC-nZVI 3 | 7 | 0.01 | 76.1 ± 9.4 | −18.0 ± 1.1 |
| CMC-nZVI 4 | 7 | 0.001 | 71.3 ± 8.5 | −20.3 ± 0.9 |
| Kinetic Model | Parameters | CMC-nZVI Nanoparticles | |||
|---|---|---|---|---|---|
| CMC-nZVI 1 | CMC-nZVI 2 | CMC-nZVI 3 | CMC-nZVI 4 | ||
| Pseudo-first-order | qe | 0.259 | 0.251 | 0.269 | 0.246 |
| K1 | 2.08 × 10−5 | 1.83 × 10−5 | 2.08 × 10−5 | 1.58 × 10−5 | |
| r2 | 0.810 | 0.831 | 0.967 | 0.966 | |
| Pseudo-second-order | qe | 47.169 | 48.309 | 30.864 | 30.345 |
| K2 | 1.26 × 10−4 | 1.01 × 10−4 | 5.61 × 10−4 | 3.55 × 10−4 | |
| Vo | 0.282 | 0.237 | 0.535 | 0.354 | |
| r2 | 0.999 | 0.999 | 0.994 | 0.998 | |
| Langmuir–Hinshelwood first-order | K3 | 10.6 × 10−3 | 12.3 × 10−3 | 2.8 × 10−3 | 2.3 × 10−3 |
| t1/2 | 65.3 | 56.4 | 247.5 | 301.4 | |
| r2 | 0.922 | 0.939 | 0.981 | 0.984 | |
| CMC-nZVI Suspension | Water Flow Rate | |||||||
|---|---|---|---|---|---|---|---|---|
| Low (2.0 cm h−1) | High (4.0 cm h−1) | |||||||
| D | λ | Kd | r2 | D | λ | Kd | r2 | |
| CMC-nZVI 1 | 8.16 | 1.56 | 0.67 | 0.81 | 16.33 | 1.56 | 0.67 | 0.85 |
| CMC-nZVI 2 | 8.11 | 1.55 | 0.61 | 0.88 | 16.23 | 1.55 | 0.60 | 0.95 |
| CMC-nZVI 3 | 8.42 | 1.61 | 0.17 | 0.97 | 16.86 | 1.61 | 0.16 | 0.97 |
| CMC-nZVI 4 | 8.26 | 1.58 | 0.14 | 0.98 | 16.54 | 1.58 | 0.14 | 0.97 |
| CMC-nZVI Suspension | Water Flow Rate | |||||||
|---|---|---|---|---|---|---|---|---|
| Low (2.0 cm h−1) | High (4.0 cm h−1) | |||||||
| R | β | ω | r2 | R | β | ω | r2 | |
| CMC-nZVI 1 | 3.62 | 0.42 | 6.02 | 0.97 | 3.62 | 0.42 | 3.00 | 0.98 |
| CMC-nZVI 2 | 3.38 | 0.58 | 4.07 | 0.97 | 3.34 | 0.58 | 2.01 | 0.96 |
| CMC-nZVI 3 | 1.67 | 0.92 | 0.38 | 0.97 | 1.62 | 0.92 | 0.19 | 0.97 |
| CMC-nZVI 4 | 1.54 | 0.99 | 0.04 | 0.98 | 1.54 | 0.99 | 0.02 | 0.97 |
| Simulation Outputs | Flow Rate (cm h−1) | CMC-nZVI Suspensions | |||
|---|---|---|---|---|---|
| CMC-nZVI 1 | CMC-nZVI 2 | CMC-nZVI 3 | CMC-nZVI 4 | ||
| Time for solute arrival (h) | 2.0 | 3.75 | 3.50 | 1.75 | 1.75 |
| Time for maximum RC (h) | 2.0 | 29.75 | 27.75 | 16.25 | 15.25 |
| Time for RC < 0.01 (h) | 2.0 | 56.0 | 48.0 | 40.0 | 40.0 |
| Max. Cr(VI) concentration (mg L−1) | 2.0 | 99.8 | 99.8 | 99.9 | 99.9 |
| Amount of Cr(VI) removed (mg) | 2.0 | 27.4 | 27.4 | 27.5 | 27.6 |
| Removal efficiency of Cr(VI) (%) | 2.0 | 70.1 | 70.1 | 70.3 | 70.6 |
| Time for solute arrival (h) | 4.0 | 2.00 | 1.75 | 1.00 | 1.00 |
| Time for maximum RC (h) | 4.0 | 15.00 | 14.50 | 8.00 | 7.50 |
| Time for RC < 0.01 (h) | 4.0 | 28.0 | 26.0 | 21.0 | 20.0 |
| Max. Cr(VI) concentration (mg L−1) | 4.0 | 99.8 | 99.9 | 100.0 | 100.0 |
| Amount of Cr(VI) removed (mg) | 4.0 | 28.3 | 28.3 | 28.4 | 28.5 |
| Removal efficiency of Cr(VI) (%) | 4.0 | 72.4 | 72.4 | 72.6 | 72.9 |
| Simulation Outputs | Flow Rate (cm h−1) | CMC-nZVI Suspensions | |||
|---|---|---|---|---|---|
| CMC-nZVI 1 | CMC-nZVI 2 | CMC-nZVI 3 | CMC-nZVI 4 | ||
| Time for solute arrival (h) | 2.0 | 1.75 | 2.25 | 1.75 | 1.75 |
| Time for maximum RC (h) | 2.0 | 29.75 | 29.75 | 28.00 | 15.25 |
| Time for RC < 0.01 (h) | 2.0 | 80.0 | 80.0 | 48.00 | 44.0 |
| Max. Cr(VI) concentration (mg L−1) | 2.0 | 92.5 | 94.8 | 99.6 | 100.0 |
| Amount of Cr(VI) removed (mg) | 2.0 | 27.2 | 27.4 | 27.4 | 27.5 |
| Removal efficiency of Cr(VI) (%) | 2.0 | 69.5 | 70.1 | 70.1 | 70.3 |
| Time for solute arrival (h) | 4.0 | 1.00 | 1.00 | 1.00 | 1.00 |
| Time for maximum RC (h) | 4.0 | 15.00 | 15.00 | 13.50 | 7.50 |
| Time for RC < 0.01 (h) | 4.0 | 52.0 | 46.0 | 22.0 | 21.0 |
| Max. Cr(VI) concentration (mg L−1) | 4.0 | 91.0 | 93.5 | 99.4 | 100.0 |
| Amount of Cr(VI) removed (mg) | 4.0 | 28.2 | 28.3 | 28.3 | 28.4 |
| Removal efficiency of Cr(VI) (%) | 4.0 | 72.1 | 72.4 | 72.4 | 72.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ibrahim, H.M.; Al-Issa, A.A.; Al-Farraj, A.S.; Alghamdi, A.G.; Al-Turki, A.M. Effect of Stabilized nZVI Nanoparticles on the Reduction and Immobilization of Cr in Contaminated Soil: Column Experiment and Transport Modeling. Nanomaterials 2024, 14, 862. https://doi.org/10.3390/nano14100862
Ibrahim HM, Al-Issa AA, Al-Farraj AS, Alghamdi AG, Al-Turki AM. Effect of Stabilized nZVI Nanoparticles on the Reduction and Immobilization of Cr in Contaminated Soil: Column Experiment and Transport Modeling. Nanomaterials. 2024; 14(10):862. https://doi.org/10.3390/nano14100862
Chicago/Turabian StyleIbrahim, Hesham M., Abdallah A. Al-Issa, Abdullah S. Al-Farraj, Abdulaziz G. Alghamdi, and Ali M. Al-Turki. 2024. "Effect of Stabilized nZVI Nanoparticles on the Reduction and Immobilization of Cr in Contaminated Soil: Column Experiment and Transport Modeling" Nanomaterials 14, no. 10: 862. https://doi.org/10.3390/nano14100862
APA StyleIbrahim, H. M., Al-Issa, A. A., Al-Farraj, A. S., Alghamdi, A. G., & Al-Turki, A. M. (2024). Effect of Stabilized nZVI Nanoparticles on the Reduction and Immobilization of Cr in Contaminated Soil: Column Experiment and Transport Modeling. Nanomaterials, 14(10), 862. https://doi.org/10.3390/nano14100862









