Interfacial Damage Mechanisms and Performance Prediction in Recycled Aggregate Concrete
Abstract
1. Introduction
2. Materials and Methods
2.1. Introduction to the Test
2.1.1. Constituents and Properties
2.1.2. Test Methods
2.2. Test Results
2.2.1. Destructive Form
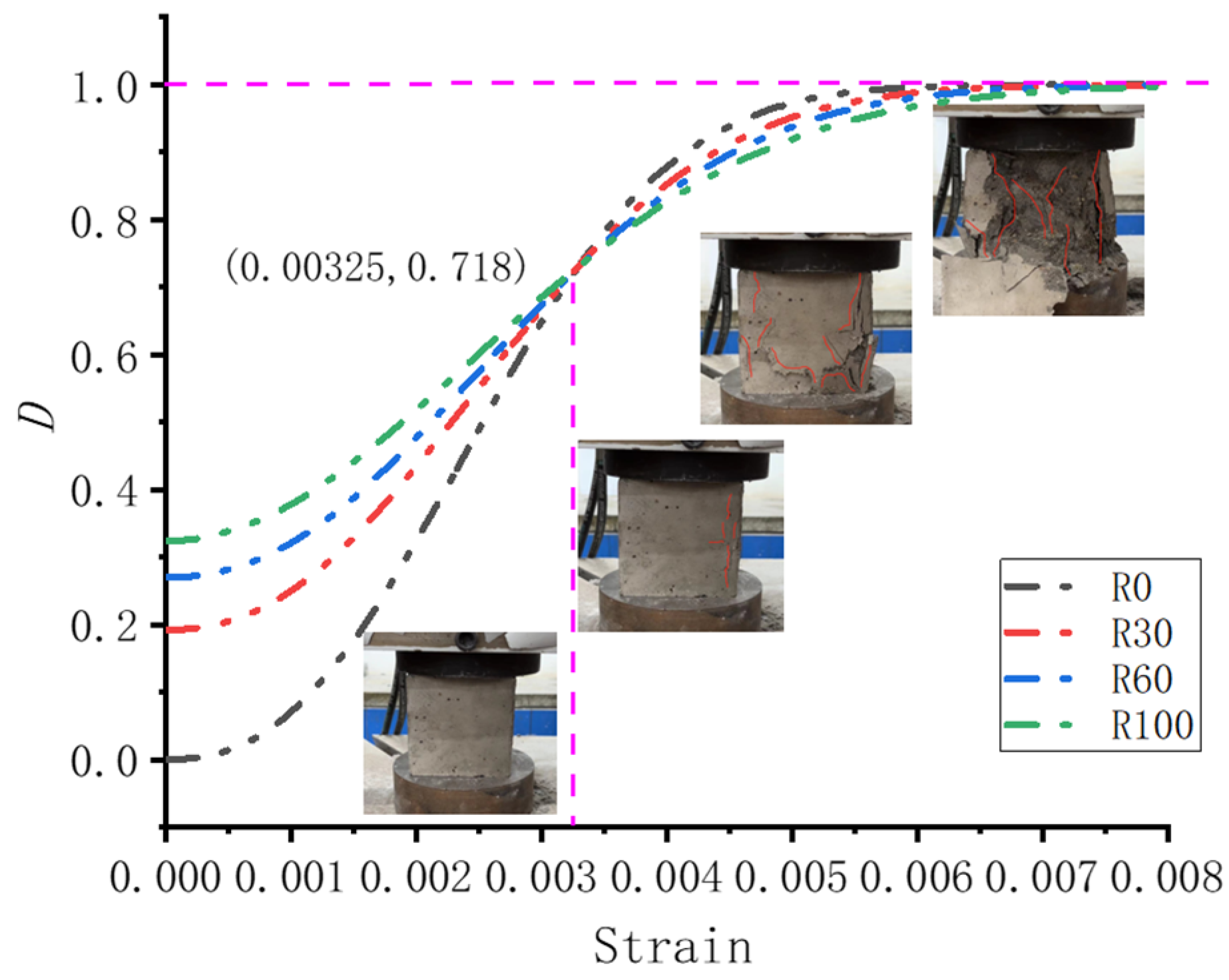
2.2.2. Mechanical Property Analysis
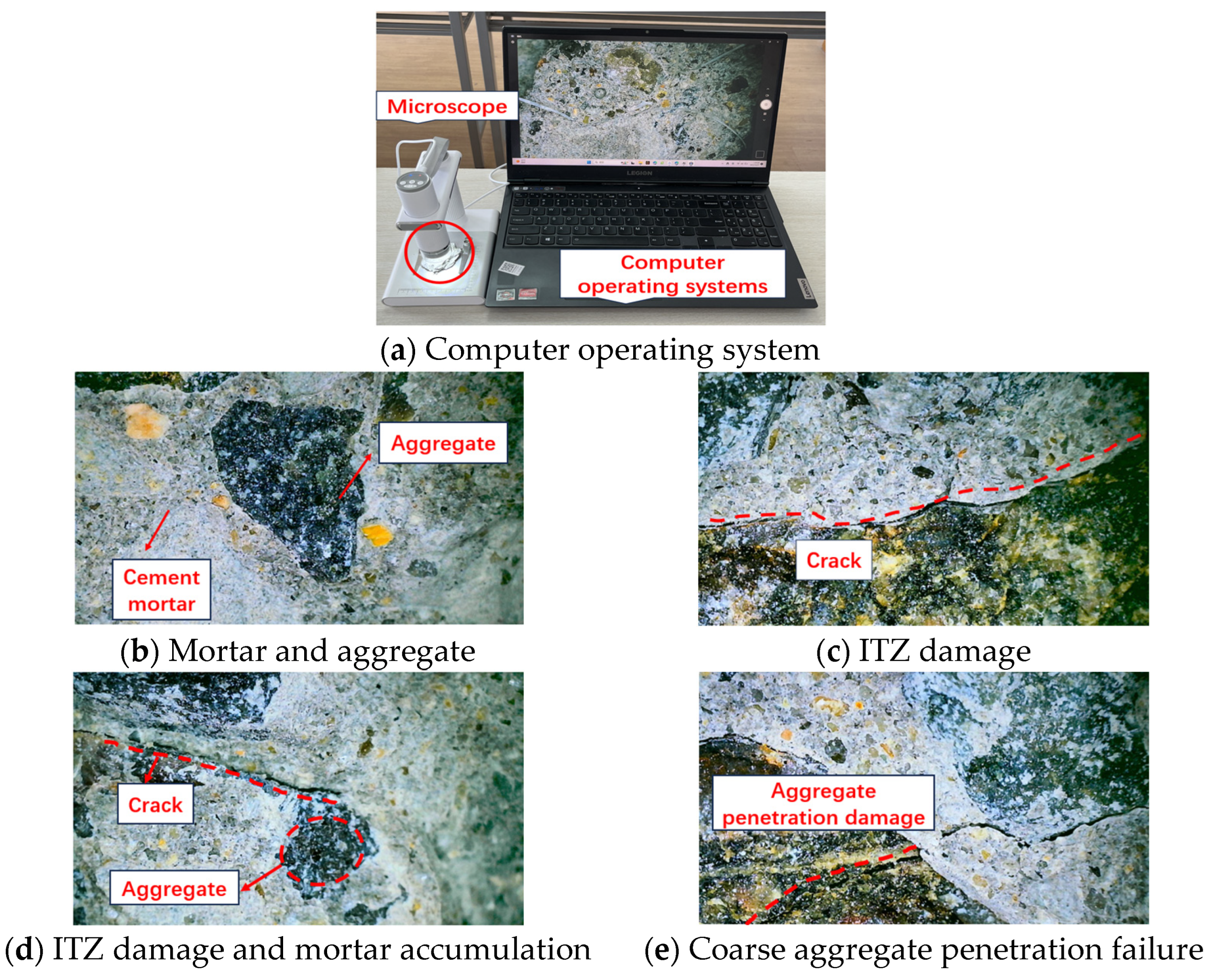
2.2.3. Analysis of Internal Structure of Concrete
3. Damage Ontology Model
3.1. Applied Theory
3.2. Parameter Fitting
4. Stochastic Aggregate Distribution Model
4.1. Parameter Setting
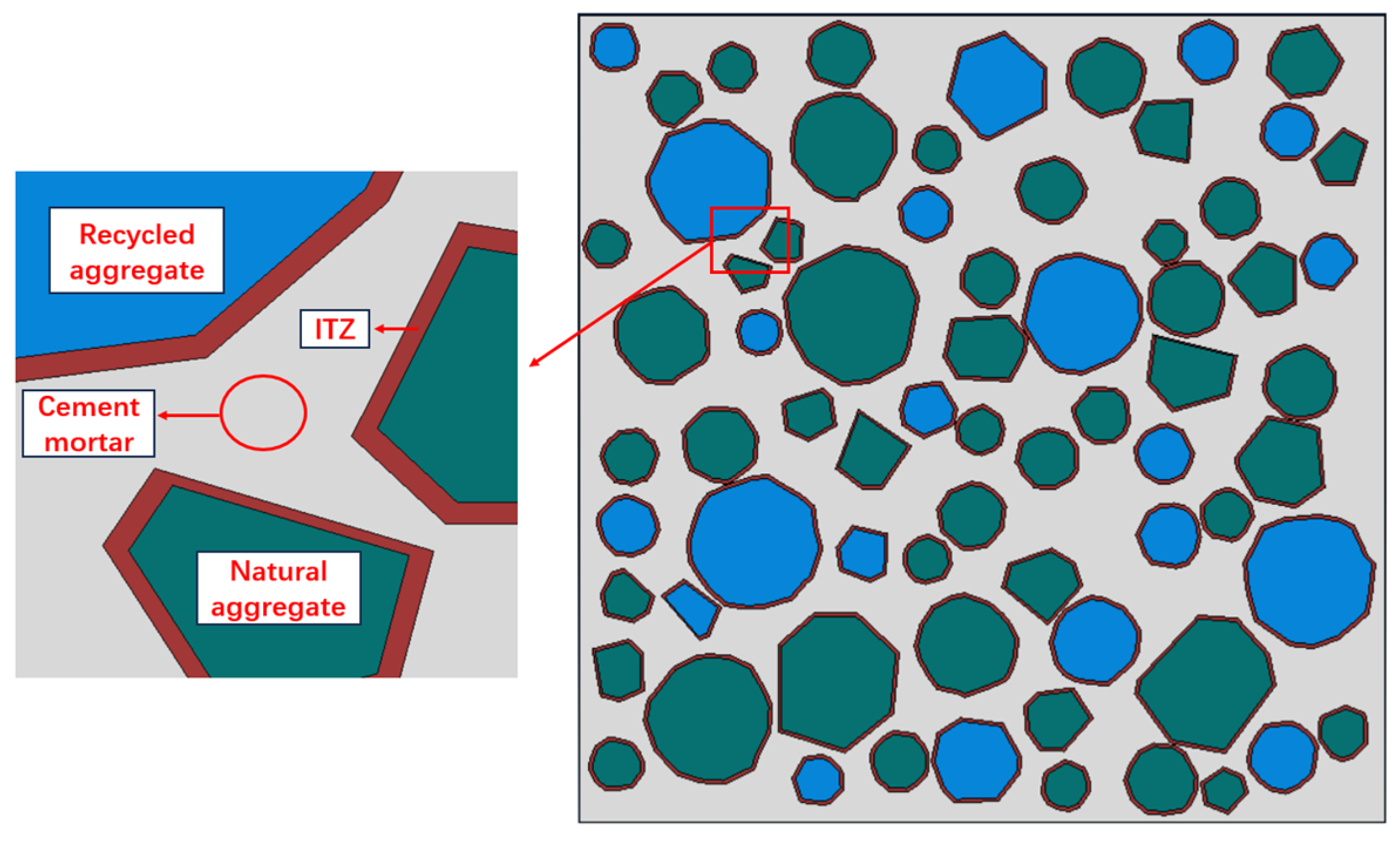
4.2. Building the Model
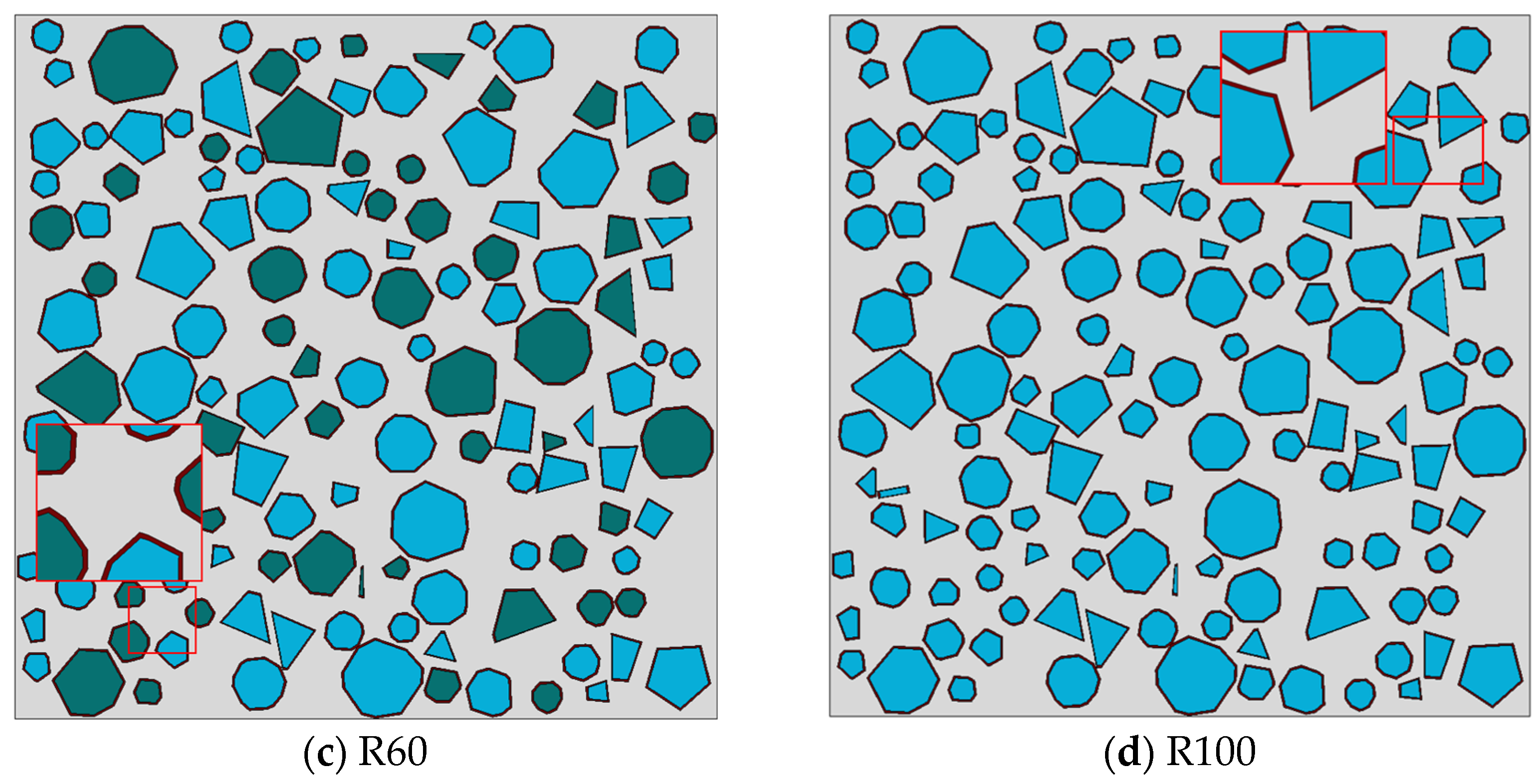
4.3. Computational Results and Analyses
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Akça, K.R.; Çakır, Ö.; İpek, M. Properties of polypropylene fiber reinforced concrete using recycled aggregates. Constr. Build. Mater. 2015, 98, 620–630. [Google Scholar] [CrossRef]
- Blazy, J.; Blazy, R. Polypropylene fiber reinforced concrete and its application in creating architectural forms of public spaces. Case Stud. Constr. Mater. 2021, 14, e00549. [Google Scholar] [CrossRef]
- Yoo, D.-Y.; Banthia, N. Impact resistance of fiber-reinforced concrete—A review. Cem. Concr. Compos. 2019, 104, 103389. [Google Scholar] [CrossRef]
- Bai, G.; Zhu, C.; Liu, C.; Liu, B. An evaluation of the recycled aggregate characteristics and the recycled aggregate concrete mechanical properties. Constr. Build. Mater. 2020, 240, 117978. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhang, Y.; Zhuo, J.; Zhang, P.; Kong, W. Mechanical properties and microstructure of nano-strengthened recycled aggregate concrete. Nanotechnol. Reviews. 2022, 11, 1499–1510. [Google Scholar] [CrossRef]
- Shi, D.; Shi, Q. Study on Mechanical Properties and Mesoscopic Numerical Simulation of Recycled Concrete. Sustainability 2022, 14, 12125. [Google Scholar] [CrossRef]
- Moradi, M.; Bagherieh, A.R.; Esfahani, M.R. Constitutive modeling of steel fiber-reinforced concrete. Int. J. Damage Mech. 2020, 29, 388–412. [Google Scholar] [CrossRef]
- Guo, S.; Zheng, D.; Zhao, L.; Lu, Q.; Liu, X. Mechanical test and constitutive model of recycled plastic fiber reinforced recycled concrete. Constr. Build. Mater. 2022, 348, 128578. [Google Scholar] [CrossRef]
- Ma, K.; Huang, X.Y.; Hu, M. Damage constitutive relationship of brick-concrete regenerated coarse aggregate. J. Build. Mater. 2022, 25, 131–141. [Google Scholar]
- Belén, G.-F.; Fernando, M.-A.; Diego, C.L.; Sindy, S.-P. Stress–strain relationship in axial compression for concrete using recycled saturated coarse aggregate. Constr. Build. Mater. 2011, 25, 2335–2342. [Google Scholar] [CrossRef]
- Ji, Y.; Wang, D.; Wang, L. Numerical and Experimental Investigation of Recycled Brick Coarse Aggregate Concrete. Appl. Sci. 2022, 12, 9035. [Google Scholar] [CrossRef]
- Li, Y.; Guo, H.; Zhou, H.; Li, Y.; Chen, J. Damage characteristics and constitutive model of concrete under uniaxial compression after Freeze-Thaw damage. Constr. Build. Mater. 2022, 345, 128171. [Google Scholar]
- Wan, Z.; Ma, K.L.; Long, G. Fatigue damage constitutive model of SCC based on Weibull distribution and residual strain. Mater. Rev. 2019, 33, 634–638. [Google Scholar]
- Zhang, T.; Huang, W.; Rong, C. Research of polypropylene fiber recycled concrete damage constitutive model. Mater. Rev. 2015, 29, 150–155. [Google Scholar]
- Zheng, Y.; Zhuo, J.; Zhang, P.; Ma, M. Mechanical properties and meso-microscopic mechanism of basalt fiber-reinforced recycled aggregate concrete. J. Clean. Prod. 2022, 370, 133555. [Google Scholar] [CrossRef]
- Jalilifar, H.; Sajedi, F. Micro-structural analysis of recycled concretes made with recycled coarse concrete aggregates. Constr. Build. Mater. 2021, 267, 121041. [Google Scholar]
- Lu, B.; Shi, C.; Cao, Z.; Guo, M.; Zheng, J. Effect of carbonated coarse recycled concrete aggregate on the properties and microstructure of recycled concrete. J. Clean. Prod. 2019, 233, 421–428. [Google Scholar]
- Wang, J.; Zhang, J.; Cao, D.; Dang, H.; Ding, B. Comparison of recycled aggregate treatment methods on the performance for recycled concrete. Constr. Build. Mater. 2020, 234, 117366. [Google Scholar]
- Mahmood, W.; Khan, A.-U.-R.; Ayub, T. Mechanical and Durability Properties of Concrete Containing Recycled Concrete Aggregates. Iran. J. Sci. Technol. Trans. Civ. Eng. 2022, 46, 2111–2130. [Google Scholar] [CrossRef]
- Gao, S.; Guo, X.; Ban, S.; Ma, Y.; Yu, Q.; Sui, S. Influence of supplementary cementitious materials on ITZ characteristics of recycled concrete. Constr. Build. Mater. 2023, 363, 129736. [Google Scholar]
- Zheng, Y.; Xi, X.; Zhang, Y.; Zhang, P.; Du, C. Review of mechanical properties and strengthening mechanism of fully recycled aggregate concrete under high temperature. Constr. Build. Mater. 2023, 394, 132221. [Google Scholar]
- Zheng, Y.; Zhang, Y.; Zhang, P. Methods for improving the durability of recycled aggregate concrete: A review. J. Mater. Res. Technol. 2021, 15, 6367–6386. [Google Scholar]
- Zhang, P.; Sun, X.; Wang, F.; Wang, J. Mechanical Properties and Durability of Geopolymer Recycled Aggregate Concrete: A Review. Polymers 2023, 15, 615. [Google Scholar] [CrossRef]
- JGJ63-2006; Standard for Concrete Water Use. Ministry of Construction of the People’s Republic of China: Beijing, China, 2006.
- GB/T 25177-2010; Recycled Coarse Aggregate for Concrete. China Building Industry Press: Beijing, China, 2010.
- GB/T 14685-2011; Pebble and Crushed Stone for Construction. China Standards Press: Beijing, China, 2022.
- GB 50204-2002; Concrete Structure Engineering Construction Quality Acceptance Code. China Building and Construction Press: Beijing, China, 2022.
- Ji, Y.; Wang, D.; Jia, Y. Numerical simulation and size effect of PVA fiber reinforced brick aggregate recycled concrete. Mater. Rev. 2024, 39, 23100214-11. [Google Scholar]
- GB/T 50080-2016; Standard Building Code for Performance Test Methods of Ordinary Concrete Mixes. General Administration of Quality Supervision: Beijing, China, 2017.
- GB/T50081-2002; Standard Test Methods for Mechanical Properties of Ordinary Concrete. China Building and Construction Press: Beijing, China, 2019.
- Deresa, S.T.; Xu, J.; Shan, B.; Ren, H.; Xiao, Y. Experimental investigation on flexural behavior of full-scale glued laminated bamboo (glubam)-concrete composite beams: A case study of using recycled concrete aggregates. Eng. Struct. 2021, 233, 111896. [Google Scholar]
- Ollivier, J.P.; Maso, J.C.; Bourdette, B. Interfacial transition zone in concrete. Adv. Cem. Based Mater. 1995, 2, 30–38. [Google Scholar]
- Djerbi, A. Effect of recycled coarse aggregate on the new interfacial transition zone concrete. Constr. Build. Mater. 2018, 190, 1023–1033. [Google Scholar]
- Krishnya, S.; Elakneswaran, Y.; Yoda, Y. Proposing a three-phase model for predicting the mechanical properties of mortar and concrete. Mater. Today Commun. 2021, 29, 102858. [Google Scholar]
- Chen, Q.; Zhang, J.; Wang, Z.; Zhao, T.; Wang, Z. A review of the interfacial transition zones in concrete: Identification, physical characteristics, and mechanical properties. Eng. Fract. Mech. 2024, 300, 109979. [Google Scholar] [CrossRef]
- Hu, J.; Stroeven, P. Properties of the Interfacial Transition Zone in Model Concrete. Interface Sci. 2004, 12, 389–397. [Google Scholar] [CrossRef]
- Soltanabadi, R.; Behfarnia, K. Evaluation of Mechanical Properties of Concrete Containing Recycled Concrete Aggregate and Recycled Asphalt Pavement. J. Mater. Civ. Eng. 2022, 34, 04022348. [Google Scholar] [CrossRef]
- Liao, K.-Y.; Chang, P.; Peng, Y.; Yang, C. A study on characteristics of interfacial transition zone in concrete. Cem. Concr. Res. 2004, 34, 977–989. [Google Scholar] [CrossRef]
- Gao, S.; Guo, J.; Zhu, Y.; Jin, Z. Study on the influence of the properties of interfacial transition zones on the performance of recycled aggregate concrete. Constr. Build. Mater. 2023, 408, 133592. [Google Scholar] [CrossRef]
- Qiu, J.; Li, L.; Li, L.; Luan, X.; Guan, X.; Niu, G. Study on the deterioration characteristics and mechanisms of recycled brick-concrete aggregate concrete under load-freeze-thaw coupling. Constr. Build. Mater. 2024, 413, 134817. [Google Scholar] [CrossRef]
- Yang, L.; Li, K.; Hu, X.; Peng, Z.; Liu, Q.; Shi, C. Mesoscopic discrete modeling of compression and fracture behavior of concrete: Effects of aggregate size distribution and interface transition zone. Cem. Concr. Compos. 2024, 147, 105411. [Google Scholar] [CrossRef]
- Vu, C.-C.; Ho, N.-K.; Pham, T.-A. Weibull statistical analysis and experimental investigation of size effects on the compressive strength of concrete-building materials. Case Stud. Constr. Mater. 2022, 17, e01231. [Google Scholar] [CrossRef]
- Liu, Q.; Xiao, J.; Sun, Z. Experimental study on the failure mechanism of recycled concrete. Cem. Concr. Res. 2011, 41, 1050–1057. [Google Scholar]
- Xu, J.; Chen, W.; Yu, Y.; Xu, J.; Zhao, X. Data-driven analysis on compressive behavior of unconfined and confined recycled aggregate concretes. Constr. Build. Mater. 2022, 356, 129282. [Google Scholar] [CrossRef]
- Dong, X.; Yu, T.; Zhang, Q.; Bui, T. Multiscale freezing-thaw in concrete: A numerical study. Compos. Struct. 2023, 309, 116758. [Google Scholar] [CrossRef]
- Xie, T.; Yang, G.; Zhao, X.; Xu, J.; Fang, C. A unified model for predicting the compressive strength of recycled aggregate concrete containing supplementary cementitious materials. J. Clean. Prod. 2020, 251, 119752. [Google Scholar] [CrossRef]
- Chen, J.; Wang, Y. Finite element analysis of steel-concrete-steel sandwich beams with novel interlocked angle connectors subjected to impact loading. J. Constr. Steel Res. 2023, 207, 107977. [Google Scholar] [CrossRef]
- Peng, R.-X.; Qiu, W.-L.; Jiang, M. Application of a micro-model for concrete to the simulation of crack propagation. Theor. Appl. Fract. Mech. 2021, 116, 103081. [Google Scholar]
- Najafi Koopas, R.; Rauter, N.; Lammering, R. Two-Dimensional Mesoscale Finite Element Modeling of Concrete Damage and Failure. Appl. Sci. 2023, 13, 8971. [Google Scholar] [CrossRef]
- Hu Dalin, Z.L.; Chen, D. The establishment of three-dimensional concrete mesoscopic stochastic model and finite element subdivision. J. Transp. Eng. 2018, 18, 1–11. [Google Scholar]
- Wang, J.; Jivkov, A.; Li, Q.; Engelberg, D. Experimental and numerical investigation of mortar and ITZ parameters in meso-scale models of concrete. Theor. Appl. Fract. Mech. 2020, 109, 102722. [Google Scholar]
- Xu, J.; Zhang, X. Research on modeling method of porous air bearing materials based on random particles. Mater. Today Commun. 2022, 31, 103831. [Google Scholar]
- Grondin, F.; Matallah, M. How to consider the Interfacial Transition Zones in the finite element modelling of concrete? Cem. Concr. Res. 2014, 58, 67–75. [Google Scholar] [CrossRef]
- Shuguang, L.; Qingbin, L. Method of meshing ITZ structure in 3D meso-level finite element analysis for concrete. Finite Elem. Anal. Des. 2015, 93, 96–106. [Google Scholar] [CrossRef]
- Maleki, M.; Rasoolan, I.; Khajehdezfuly, A.; Jivkov, A. On the effect of ITZ thickness in mesoscale models of concrete. Constr. Build. Mater. 2020, 258, 119639. [Google Scholar] [CrossRef]
- Ji, Y.; Wang, D. Constitutive model of waste brick concrete based on Weibull strength theory. Case Stud. Constr. Mater. 2023, 18, e01738. [Google Scholar]
- Jayasuriya, A.; Adams, M.P.; Bandelt, M.J. Generation and Numerical Analysis of Random Aggregate Structures in Recycled Concrete Aggregate Systems. J. Mater. Civ. Eng. 2020, 32, 04020044. [Google Scholar] [CrossRef]
- Ji, Y.; Pei, Z.; Xu, W.; Li, Z.; Li, Y.; Jia, Y. Deterioration performance analysis of recycled brick concrete subjected to freezing and thawing effect. Case Stud. Constr. Mater. 2024, 20, e02722. [Google Scholar] [CrossRef]
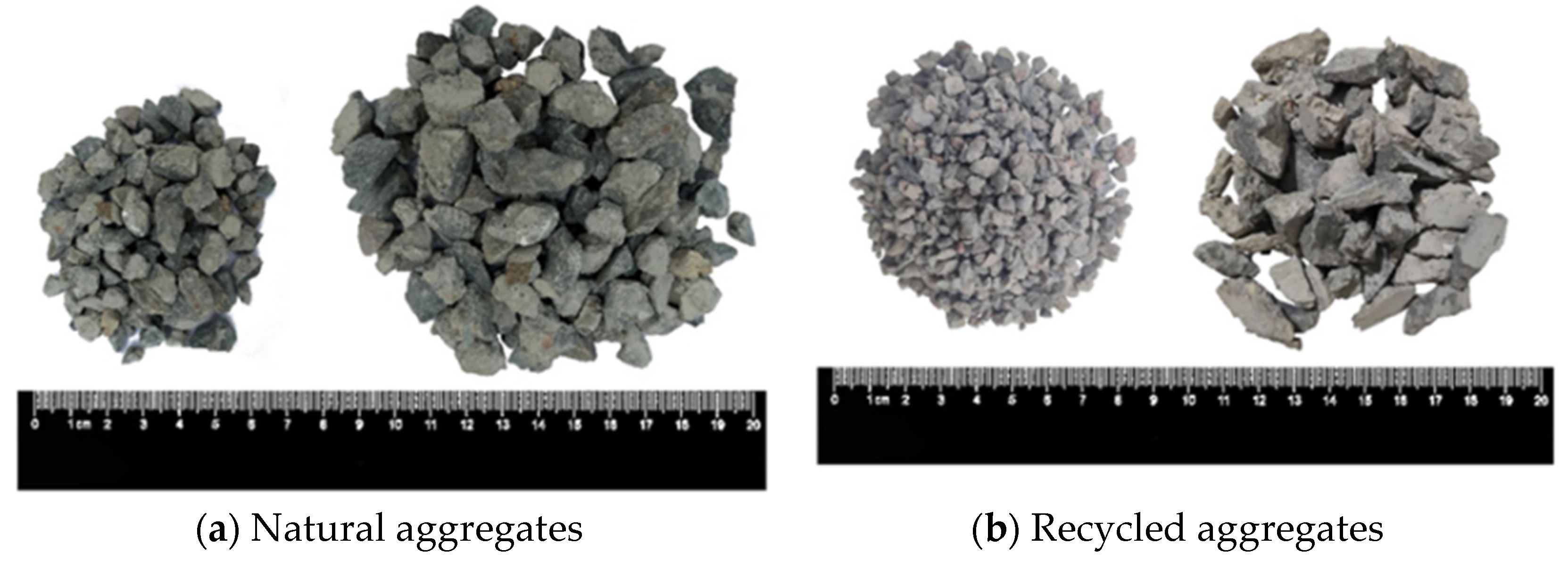



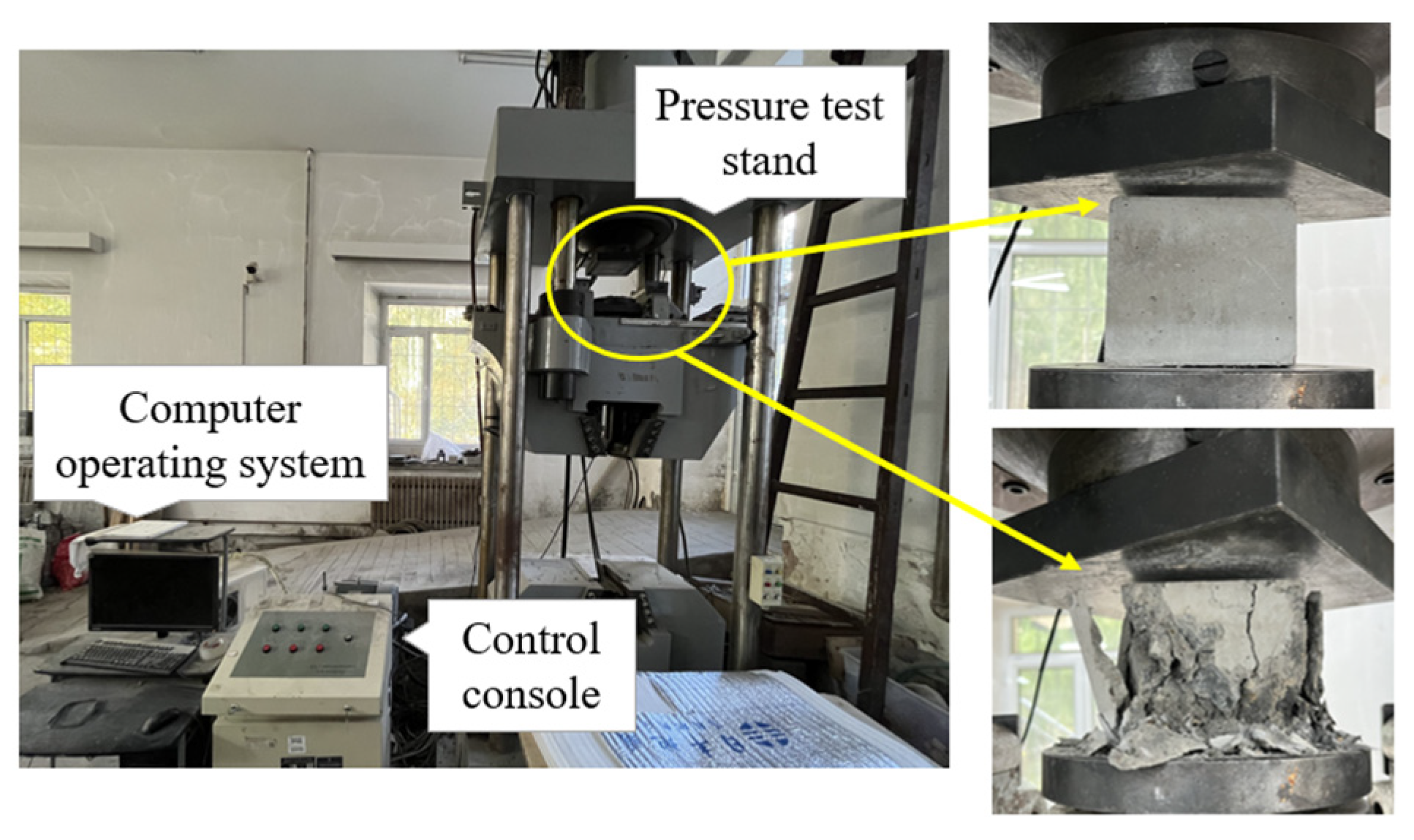


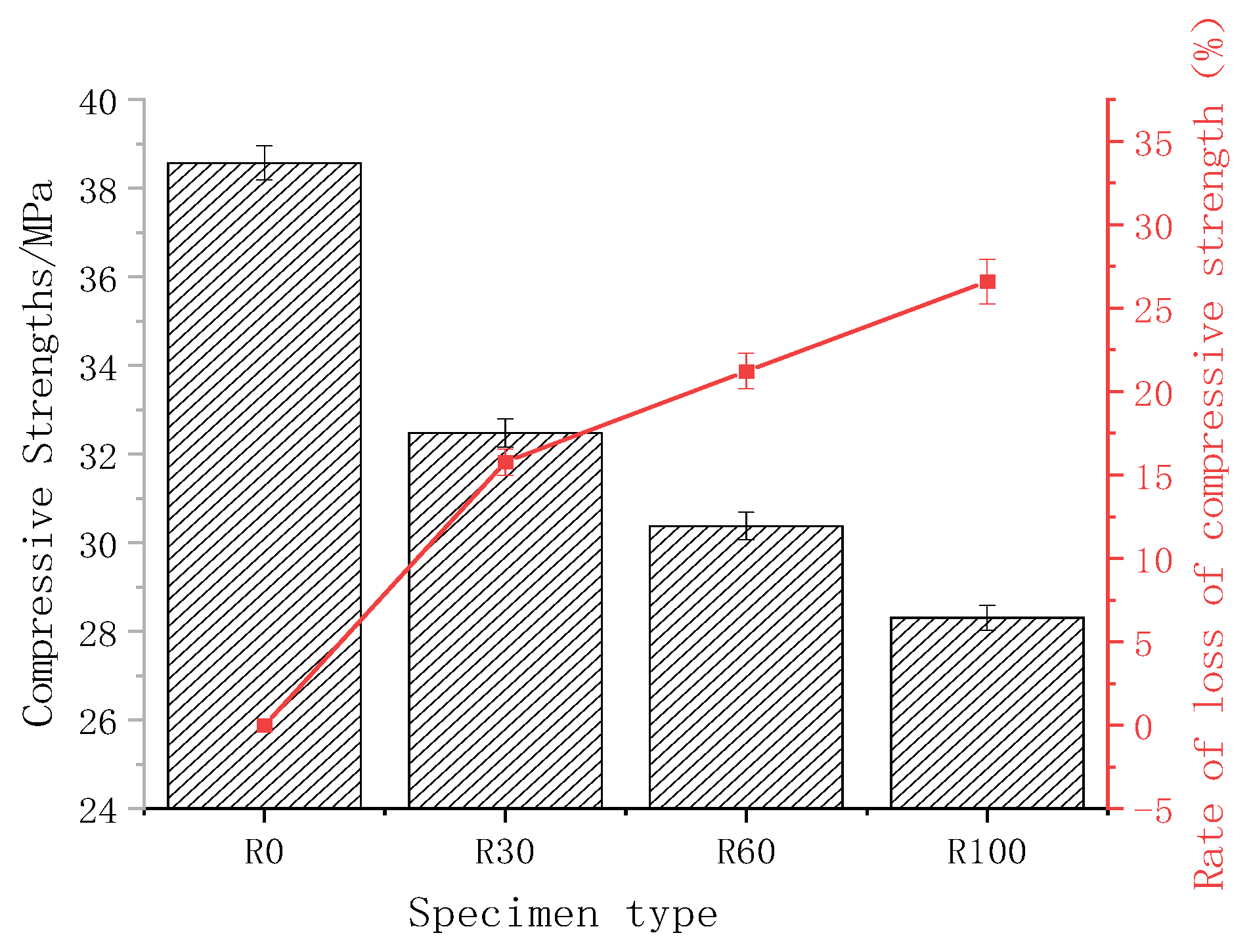
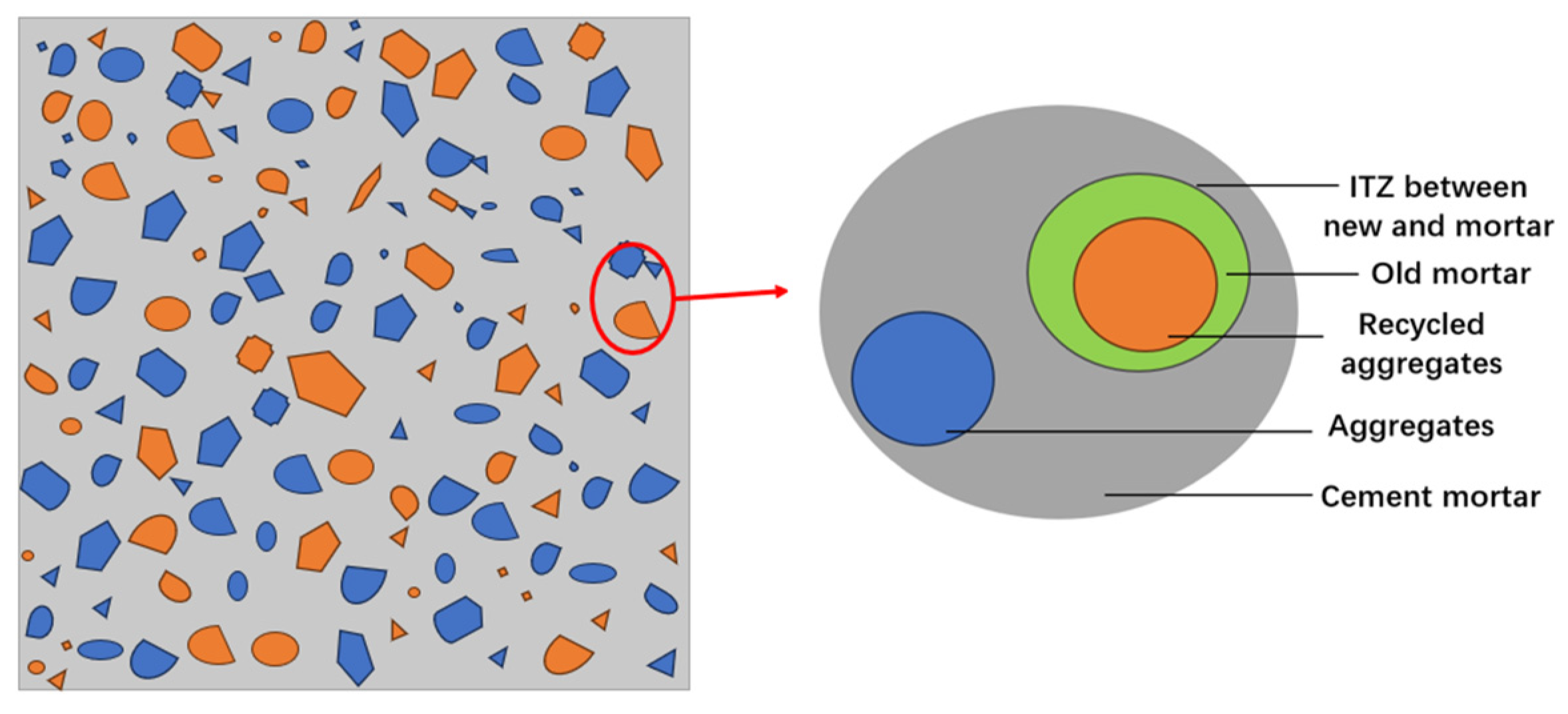



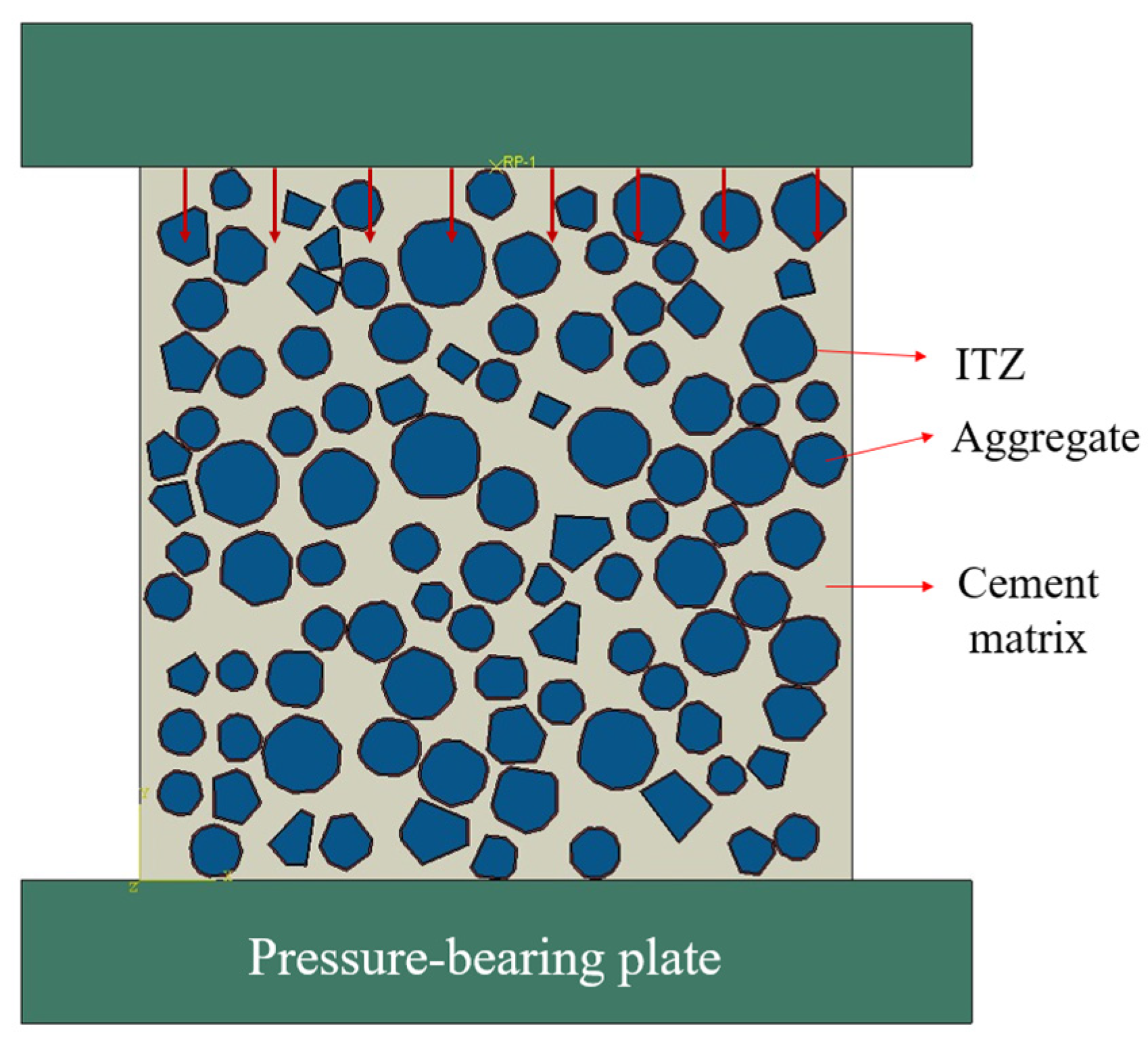
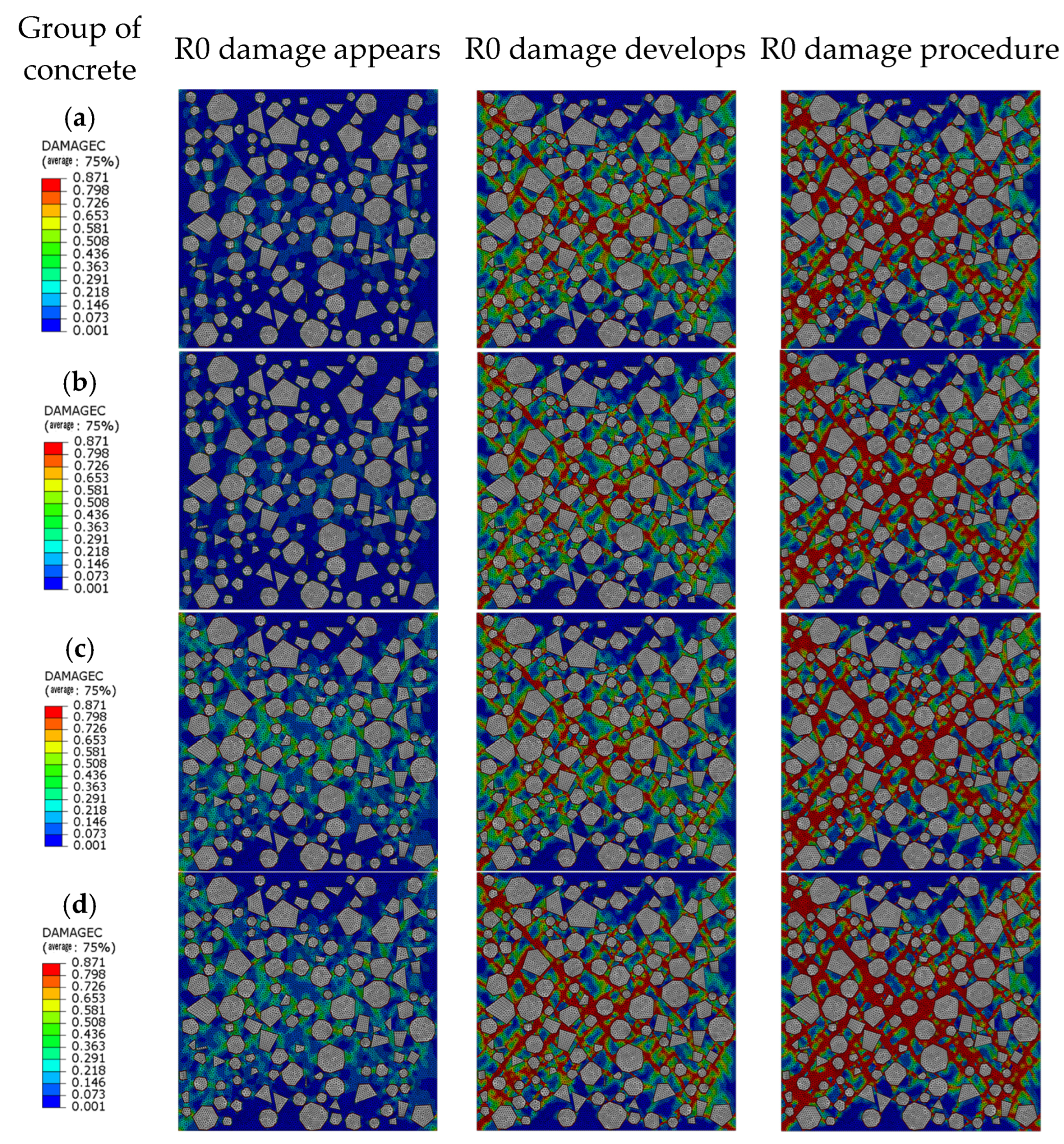
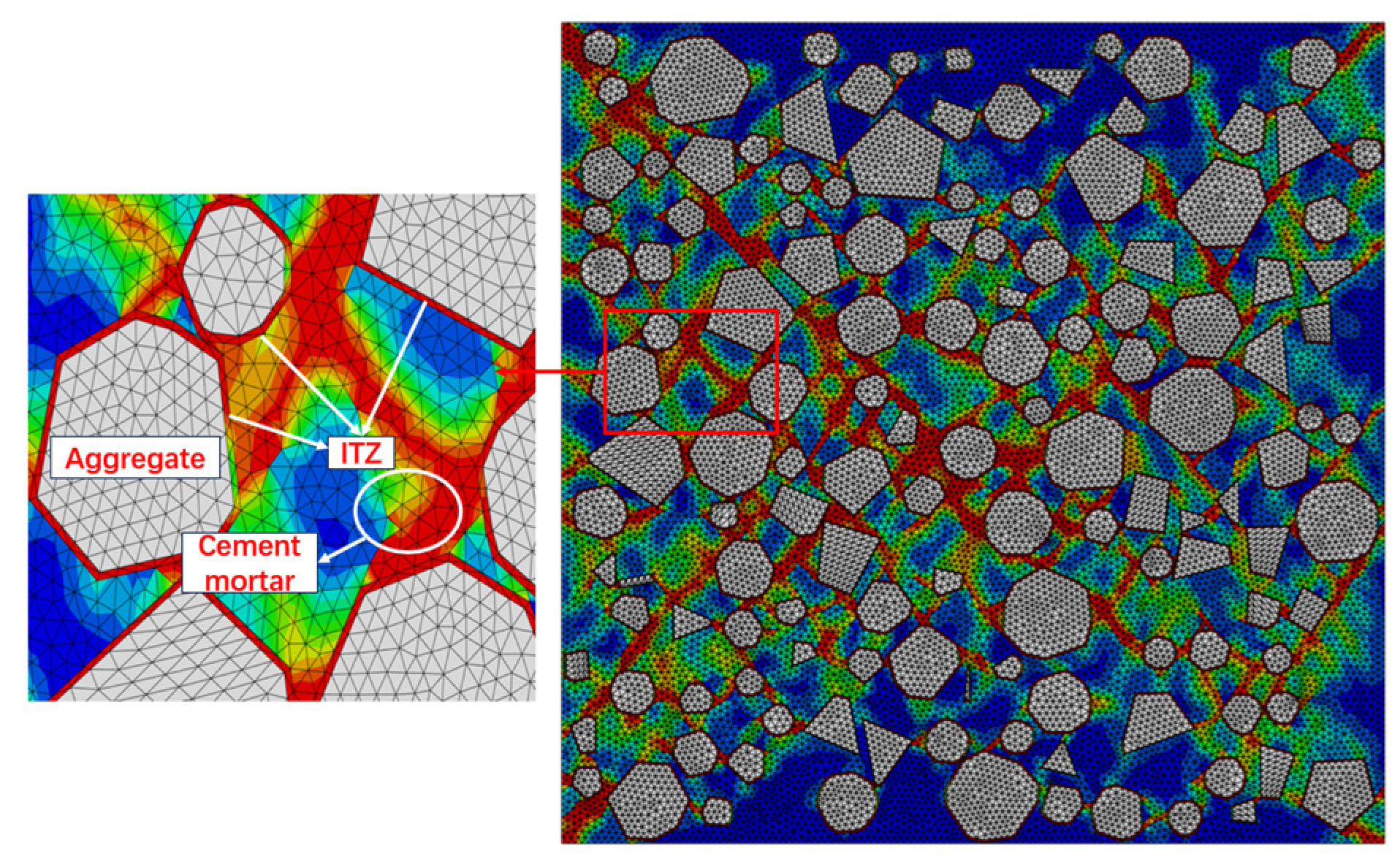

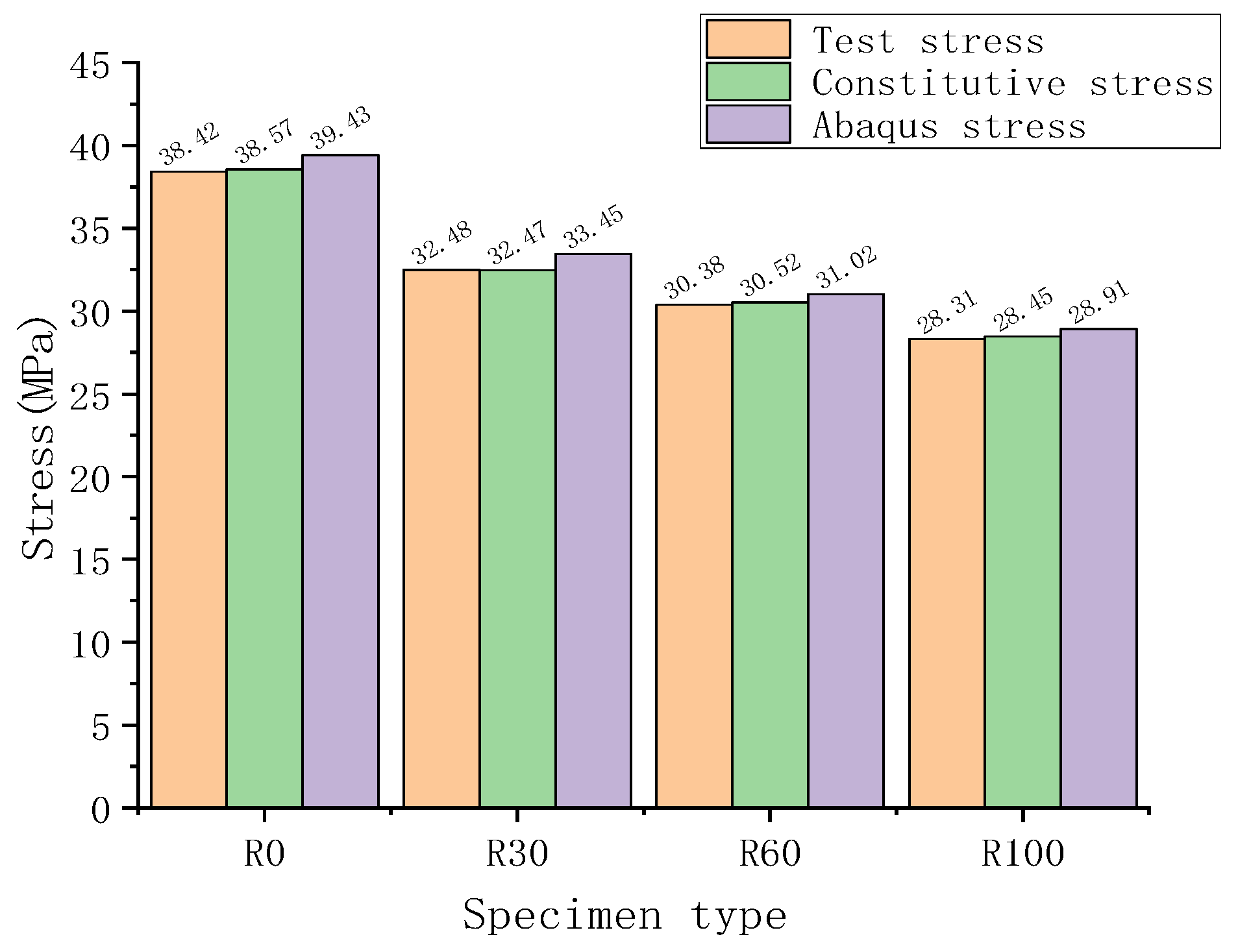
| Type | Particle Size | Water Absorption | Apparent Density | Crush Value |
|---|---|---|---|---|
| NA | 5 mm–20 mm | 2.50% | 2.66 g/cm3 | 6.70% |
| RA | 5 mm–20 mm | 7.14% | 2.41 g/cm3 | 15.3% |
| Group of Concrete | Water/kg·m−3 | Cement/kg·m−3 | Fly Ash/kg·m−3 | Sand/kg·m−3 | RA/kg·m−3 | NA/kg·m−3 | Water-Reducing Agent/kg·m−3 |
|---|---|---|---|---|---|---|---|
| R0 | 195 | 462.65 | 24.35 | 613 | 0 | 1138 | 0.7305 |
| R30 | 195 | 462.65 | 24.35 | 613 | 341.4 | 796.6 | 0.7305 |
| R60 | 195 | 462.65 | 24.35 | 613 | 682.8 | 455.2 | 0.7305 |
| R100 | 195 | 462.65 | 24.35 | 613 | 1138 | 0 | 0.7305 |
| Difference Source | Degree of Freedom (df) | Mean Square | F | P |
|---|---|---|---|---|
| Interclass | 3 | 58.8789 | 495.093 | 2 × 10−9 |
| Intraclass | 8 | 0.11893 | - | - |
| Sum total | 11 | - | - | - |
| Group of Concrete | |||||
|---|---|---|---|---|---|
| R0 | 0.00204148 | 38.42 | 28,422.5 | 0.002942 | 2.4262 |
| R30 | 0.00220229 | 32.48 | 22,964.78 | 0.003159 | 2.2579 |
| R60 | 0.0023119 | 30.38 | 20,759.65 | 0.003306 | 2.1870 |
| R100 | 0.00242504 | 28.31 | 19,221.81 | 0.003431 | 2.0057 |
| Component | Young’s Modulus (MPa) | Poisson’s Ratio | Expansion Angle | Eccentricity | fb0/fc0 | K | Viscosity Coefficient |
|---|---|---|---|---|---|---|---|
| Cement mortar | 30,000 | 0.22 | 32 | 0.1 | 1.16 | 0.66667 | 1 × 10−5 |
| RA | 33,000 | 0.20 | 35 | 0.1 | 1.16 | 0.66667 | 1 × 10−5 |
| NA | 36,000 | 0.18 | 38 | 0.1 | 1.16 | 0.66667 | 1 × 10−5 |
| ITZ | 28,000 | 0.22 | 20 | 0.1 | 1.16 | 0.66667 | 1 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Ji, Y.; Hao, X. Interfacial Damage Mechanisms and Performance Prediction in Recycled Aggregate Concrete. Coatings 2025, 15, 441. https://doi.org/10.3390/coatings15040441
Zhang S, Ji Y, Hao X. Interfacial Damage Mechanisms and Performance Prediction in Recycled Aggregate Concrete. Coatings. 2025; 15(4):441. https://doi.org/10.3390/coatings15040441
Chicago/Turabian StyleZhang, Siyu, Yongcheng Ji, and Xiangwei Hao. 2025. "Interfacial Damage Mechanisms and Performance Prediction in Recycled Aggregate Concrete" Coatings 15, no. 4: 441. https://doi.org/10.3390/coatings15040441
APA StyleZhang, S., Ji, Y., & Hao, X. (2025). Interfacial Damage Mechanisms and Performance Prediction in Recycled Aggregate Concrete. Coatings, 15(4), 441. https://doi.org/10.3390/coatings15040441






