Highly Sensitive and Tunable Graphene Metamaterial Perfect Absorber in the Near-Terahertz Band

Abstract

This paper presents a highly sensitive and tunable graphene-based metamaterial perfect absorber (MPA) operating in the near-terahertz band. The structure features a unique flower-like graphene pattern, consisting of a Au substrate, a SiO₂ dielectric layer, and the patterned graphene. Multiple reflections of incident light between the gold and graphene layers increase the duration and intensity of the interaction, resulting in efficient absorption at specific frequencies. The design utilizes surface plasmon resonance (SPR) to achieve near-perfect absorption of 99.9947% and 99.6079% at 11.7475 THz and 15.8196 THz, respectively. By tuning the Fermi level and relaxation time of graphene, it is possible to precisely control the frequency and absorptivity of the absorption peak, thereby demonstrating the dynamic tunability of the absorber. The high symmetry and periodic arrangement of the structure ensures insensitivity to the polarization angle of the incident light in the range of 0° to 90°, making it extremely valuable in practical applications. In addition, the absorber exhibits very high sensitivity to changes in ambient refractive index with a maximum sensitivity of 3.205 THz/RIU, a quality factor (FOM) of 11.3011 RIU⁻¹, and a Q-Factor of 48.61. It has broad application prospects in the fields of sensors, optoelectronic devices, and terahertz imaging.

1. Introduction

There is a new type of absorber that has appeared in the realm of artificial absorbers—the metamaterial-based perfect absorber (MPA)—and it has become a key subject of our research [1,2,3]. The classic MPA is composed of a three-layer structure: it is a composite material consisting of a metal substrate, dielectric support, and a metasurface layer [4,5,6]. Among them, the metal substrate is usually used to block the propagation of waves [7,8,9]. The dielectric spacer layer acts as a support layer. Finally, the metasurface layer is typically a metal pattern or other new material with unique physical characteristics. The graphene used in this study is a two-dimensional material with outstanding optical and electrical properties, making it widely applicable in various fields [10,11]. Graphene is an allotrope of carbon. It is a bidimensional sheet hexagonal honeycomb lattice structure with high-mobility carriers, and its Fermi level can be adjusted through chemical doping or by adjusting the external potential difference [12,13]. Differently from traditional metals such as Au and Al, graphene supports SPR through the within-band transition of electrons [14]. When graphene interacts with light within the range between the mid-infrared band and the terahertz band, it can effectively capture radiance and enhance its absorptivity. Moreover, it shows a comprehensive spectral response that covers the range from ultraviolet to terahertz. When graphene is irradiated by ordinary light, the absorption rate is extremely low, and only 2.3% of the light can be absorbed [15]; however, this problem can be well solved by using plasma resonance. Combined with past research, it is known that special patterns will be helpful for improving the absorption rate. Since Landy first proposed the metamaterial perfect absorber (MPA), it has promoted many researchers to improve devices in various fields to make them have better performance [16]. At present, MPA is still a popular research field. The principle of MPA is to utilize surface plasmon resonance (SPR). A large number of plasmons on the surface will absorb incident photons, so that the energy of reflected light will be reduced. The incident wave can couple along with the electromagnetic wave, and when it breaks through the optical diffraction limit, the electromagnetic field is intensified in a certain area, ultimately achieving perfect absorption [17]. Under the unremitting research of scholars from different countries, a variety of absorbers with different functions have been designed, such as multi-band absorbers, broadband absorbers, and narrow-band absorbers [18,19,20]. For example, in 2016, Chen et al. suggested a unimodal absorber with an absorptivity of 99.5% at 2.71 THz [21]. By superimposing graphene layers of different patterns, dual-frequency and broadband conversion are realized. In 2019, Yan et al. studied a single-mode absorber. They achieved single-peak tuning via adjusting the Fermi level to modify the location of the absorptive peak [22]. In 2021, an absorber with single and dual bands was designed by Zhu et al. [23]. These are all milestones in the development history of graphene metamaterial absorbers. MPA has very extensive practical applications. For example, the graphene-based metasurface sensor proposed by Hamouleh-Alipour can be used as a nanosensor to excite surface plasmons and has potential application value in the field of nanosensing [24]. The dual-band narrowband graphene metamaterial absorber proposed by X. Du can be used for the detection of microorganisms, cancer cells, and nutrients [25]. The ultrathin dual-band bidirectional metasurface absorber with a ring-disk (RD) resonator structure studied by B. Li et al. can be applied in the imaging field due to its ability to efficiently absorb electromagnetic waves at specific frequencies [26]. In 2025, Chao’s team designed a model that can be applied to a variety of nonlinear optics and terahertz integrated circuit fields, including terahertz switches and modulators [27].

A metamaterial perfect absorber with two perfect absorptive peaks is proposed by us in this paper. Au is used for the bottom layer of the absorber, SiO₂ is utilized as the middle dielectric layer, and our upper layer is graphene with a flower-like structure composed of a ring, four triangles, and eight rectangles. Using FDTD Solution for simulation, a model with two absorptive peaks with absorption rates both above 99% was obtained. At the same time, in this paper, the impedance matching principle is used to verify whether the calculation results conform to classical physical theories. Furthermore, owing to unique properties of graphene, with changing chemical potential and relaxation time, the characteristics of the absorption peaks, such as frequency position and absorptivity, can be well controlled By varying the polarization angle of incident light, we demonstrated that the proposed absorber exhibits polarization-insensitive characteristics, leading to the development of a polarization-angle-independent absorption model. Finally, by changing the environmental refractive index of the absorber and the refractive index of the dielectric layer, a detailed study on the sensitivity, figure of merit (FOM), and Q value was conducted according to the formula to explore the value of the absorber in practice [28].

The novelties of this work are briefly described below:

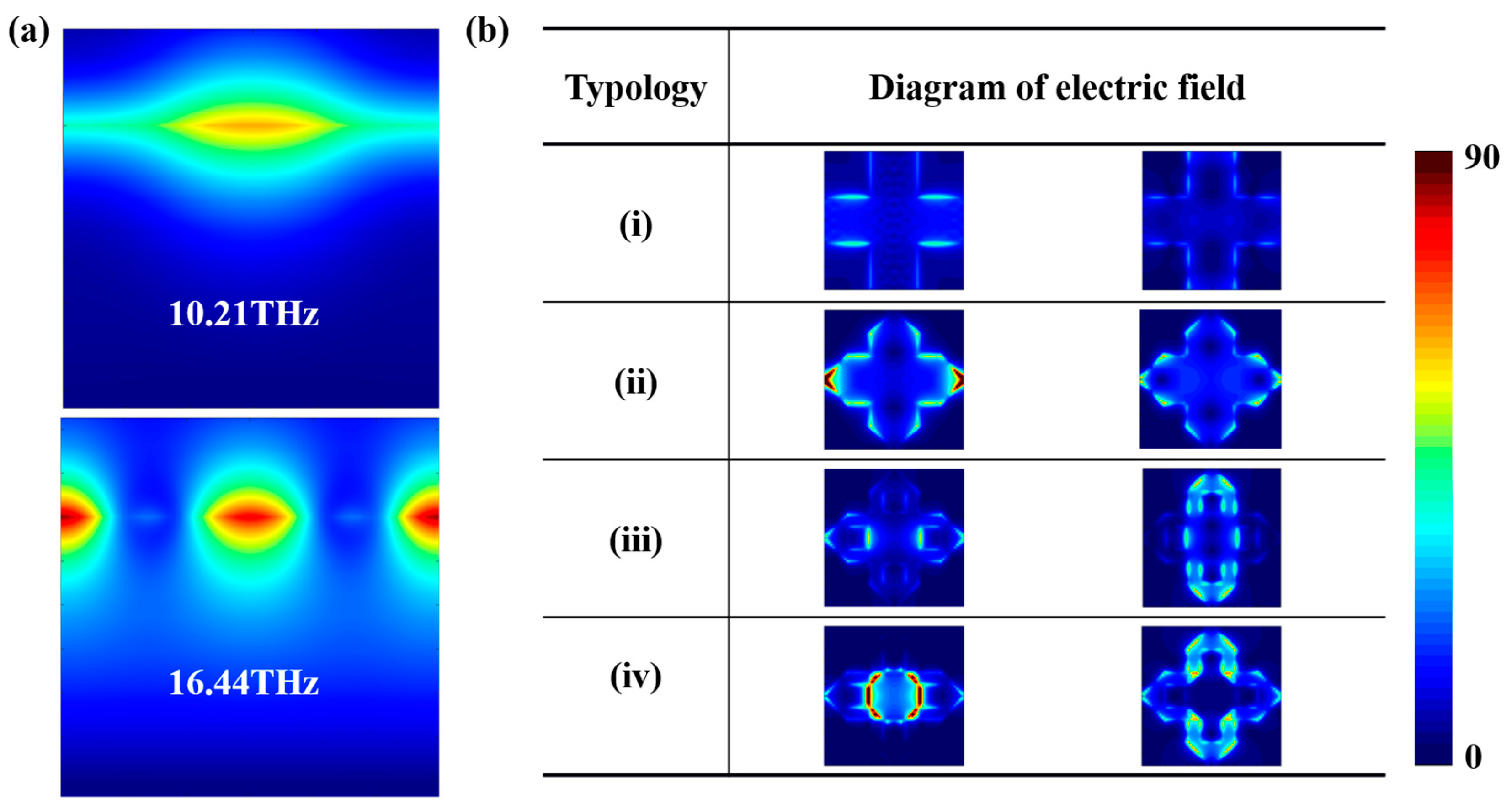
(i)

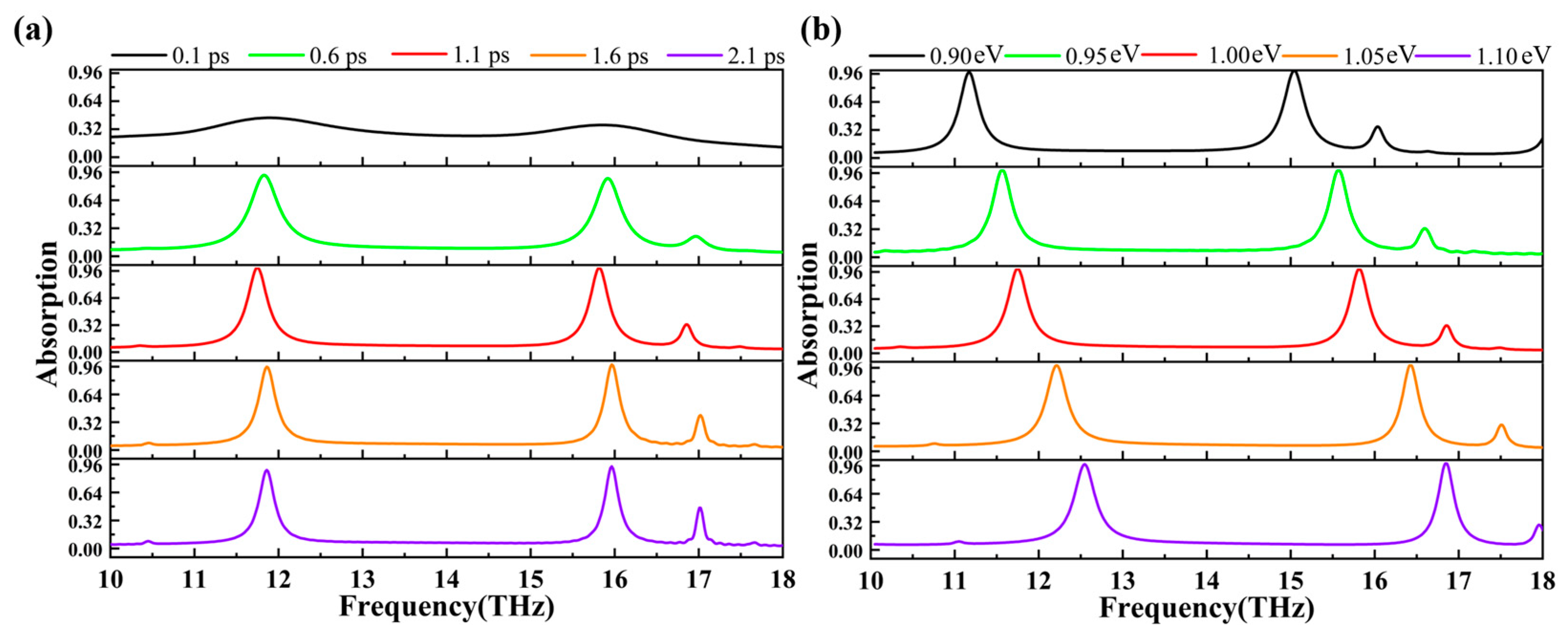
The absorption characteristics of this design are dynamically adjustable. By skillfully adjusting the parameters of the metamaterial, the absorption efficiency of the whole absorber can be effectively affected, which greatly improves the flexibility of the absorber at the design level and the real-time adjustability in actual use.

(ii)

The absorber is insensitive to the angle of polarization of the incident light (0–90°) and is therefore very practical.

(iii)

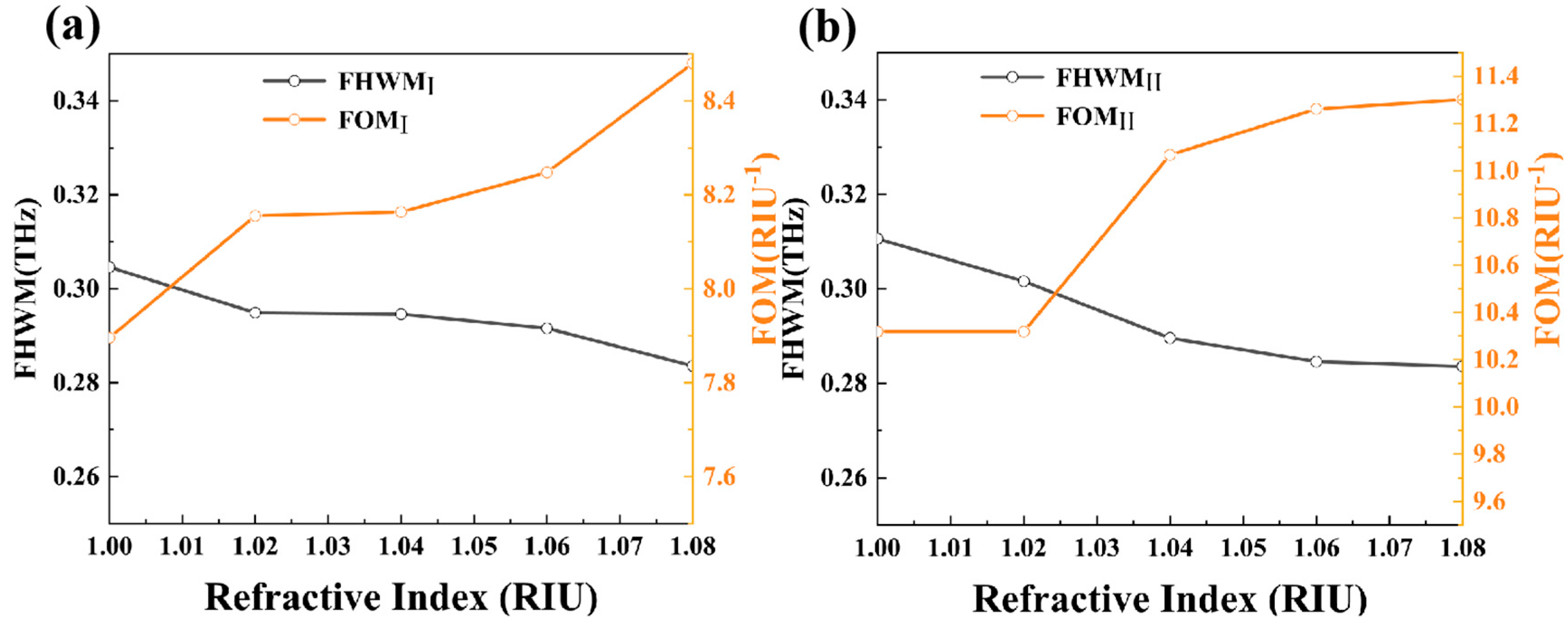
To comprehensively assess the performance of the design, key parameters such as sensitivity (S), figure of merit (FOM), and quality factor (Q-Factor) are introduced. The design demonstrates exceptional sensing capabilities, supported by its high sensitivity, superior FOM, and high Q-Factor.

(iv)

The design achieves two perfect absorption peaks within the near-terahertz spectrum.

(v)

The absorber’s structure is composed of just three stacked layers, with only the top layer being a metamaterial. This simple configuration facilitates easier and more cost-effective fabrication.

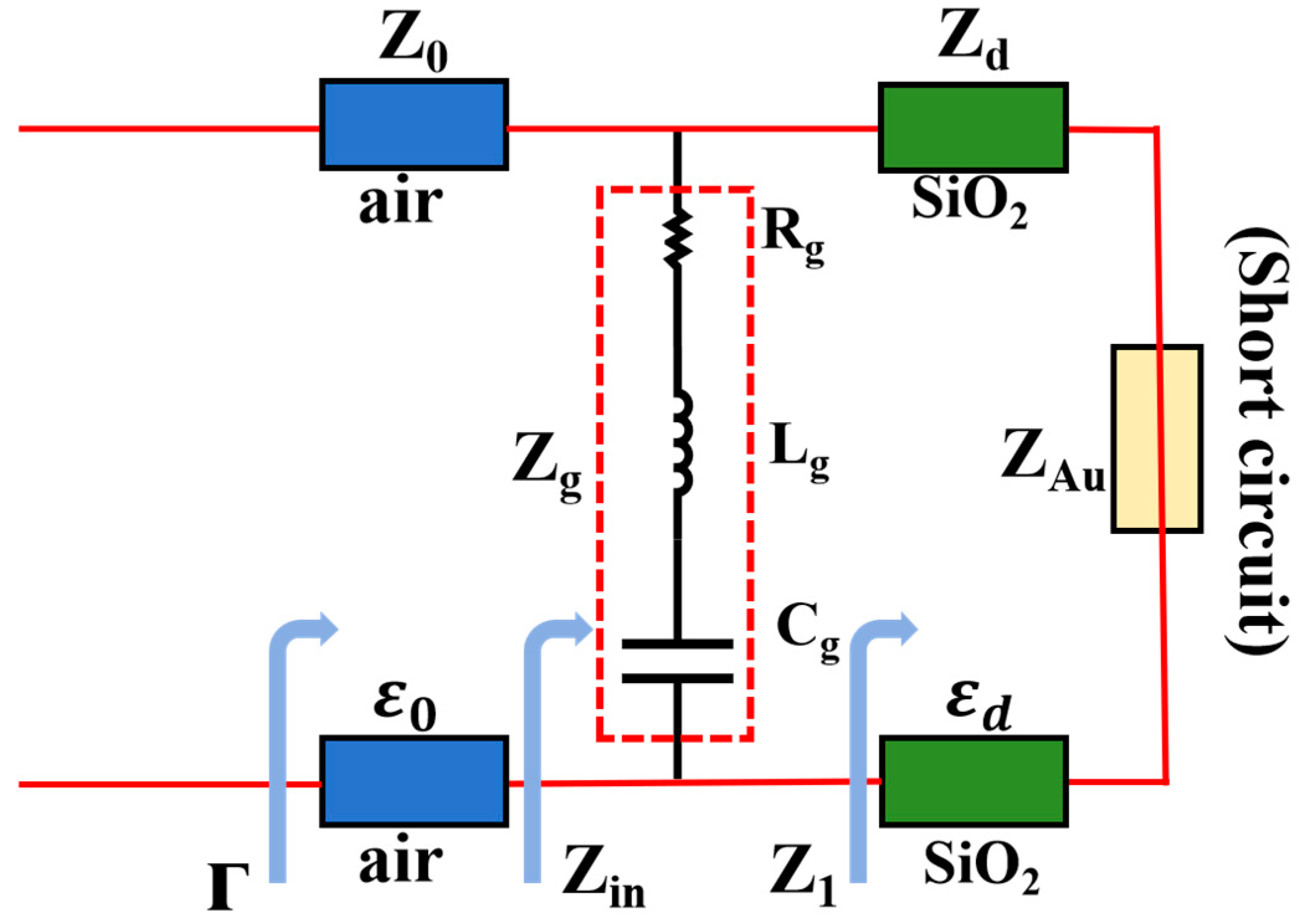
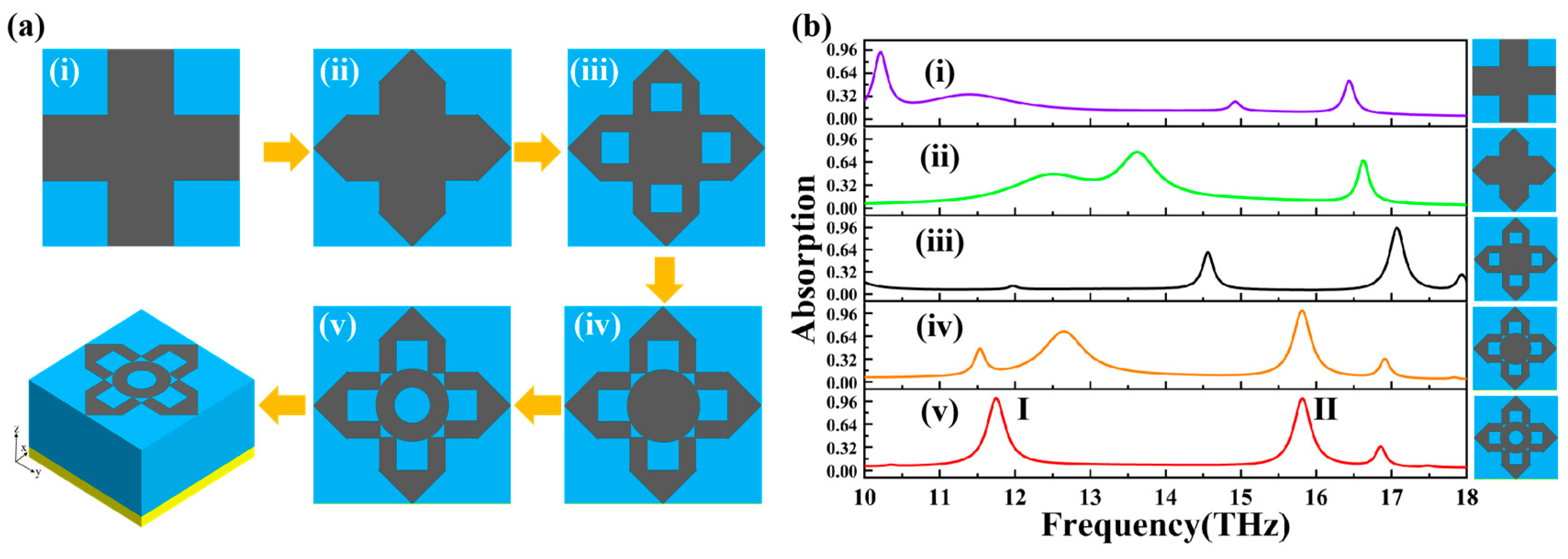
2. Materials and Structure

Rooted in the surface plasmon resonance (SPR) of top graphene, this paper presents an adjustable two-band super-perfect absorber. The 3-D repeating array configuration of this model is shown in Figure 1a, while the basic unit structure is shown in Figure 1b, and the top configuration of the absorber model is shown in Figure 1c. The fundamental unit structure of the absorber is composed of a Au reflective bottom layer, a middle layer of SiO₂, and an upper layer of interconnected flower-like graphene. There is also a ring in the center. Simulation is carried out by using FDTD Solution [29,30,31]. The absorber model’s optimal parameters are obtained. The cycle of the fundamental structural element is P = 6 μm. Metal Au functions as the planar reflective layer at the bottom, which has a thickness of 0.25 μm. The Drude model with a plasma oscillation frequency ${\mathsf{\omega }}_{\mathrm{p}}$ = 1.37 × 10¹⁶ s⁻¹ and damping coefficient $\mathsf{\gamma }$ = 1.23 × 10¹⁴ s⁻¹ is employed [32,33].

The absorber absorption rates obtained from our simulations for different SiO₂ thicknesses are shown in Figure 2. It can be seen that when the thickness of SiO₂ is 1 μm, a resonance peak with an absorption rate of 56.9% appears; when the thickness of SiO₂ is 2 μm, two resonance peaks with absorption rates of 77.2% and 98.8% appear; and when the thickness of SiO₂ is 3 μm, two resonance peaks with absorption rates of 99.9947% and 99.6079%. When the thickness of SiO₂ is 4 μm, there is a resonance peak with 97.2% absorption, but the absorption of the other resonance peak is only 36.1%. When the thickness of SiO₂ is 5 μm, there is only one resonance peak with 48% absorption. Therefore, the SiO₂ thickness of 3 μm is selected in this paper. Setting the initial refractive index of SiO₂ = 2, the top graphene has a depth of Δ = 1 nm; specific data in the planar direction are L₁ = 0.5 μm, L₂ = 1 μm, L₃ = 2.45 μm, L₄ = 1 μm, and D₅ = 1 μm. The proposed absorber model can be manufactured by cutting-edge manufacturing processes. The fabrication process begins with a silicon wafer substrate. A gold (Au) film is first deposited onto the substrate. Subsequently, a silicon dioxide (SiO₂) supporting layer is deposited on the Au film using either magnetron sputtering or plasma-enhanced chemical vapor deposition (PECVD). Finally, two-dimensional graphene is transferred onto the SiO₂ dielectric layer and patterned through a combination of electron-beam lithography (EBL) and reactive ion etching (RIE). It should be noted that, currently, electron beam lithography is capable of achieving precise operation at a scale of 10 nm, and this provides technical support for the formation of such graphene patterns [34,35]. The workflow using electron beam lithography is roughly as follows: first, graphene is transferred to silicon dioxide, and electron beam photoresist is spin-coated on the surface of the graphene. Next, a predetermined pattern is drawn on the photoresist by the electron beam, and a developer is used to remove the photoresist from the exposed area to form a patterned mask. Finally, the pattern is transferred to graphene by removing areas of graphene that are not covered by the mask by means of oxygen plasma (O₂ plasma). The desired patterned graphene is obtained after cleaning the remaining photoresist with a solvent (e.g., acetone) [36].

The skinning depth of Au is calculated as follows [37]:

$$\mathsf{\delta }=\sqrt{{\displaystyle \frac{2}{\mathsf{\sigma }\mathsf{\mu }\mathsf{\omega }}}}$$

(1)

where the electrical conductivity of gold $\mathsf{\sigma }=4.52\times {10}^7\mathrm{S}/\mathrm{m}.$ Under room temperature conditions, the relative permeability of the magnetic medium gold is ${\mathsf{\mu }}_{\mathrm{r}}=1$; in vacuum, the permeability of gold is ${\mathsf{\mu }}_0=4\mathsf{\pi }\times {10}^{-7}$ H/m. According to the formula ${\mathsf{\mu }}_{\mathrm{r}}={\displaystyle \frac{\mathsf{\mu }}{{\mathsf{\mu }}_0}}$, the permeability μ of gold is calculated to be $\mathsf{\mu }=4\pi \times {10}^{-7}\mathrm{H}/\mathrm{m}$, where ω represents the angular frequency and can be derived from the formula $\mathsf{\omega }=2\mathsf{\pi }\mathrm{f}$. It was calculated that the thickness of the Au layer should be more than 24 nm. The model in this paper uses 0.25 μm, which satisfies the requirements.

The following formula can be used to express the surface conductivity of top-layer graphene [38,39]:

$$\mathsf{\sigma }(\mathsf{\omega })={\displaystyle \frac{{\mathrm{e}}^2{\mathrm{E}}_{\mathrm{F}}}{\mathsf{\pi }{\mathrm{\hslash }}^2}}{\displaystyle \frac{\mathrm{i}}{\mathsf{\omega }+\mathrm{i}{\mathsf{\tau }}^{-1}}}$$

(2)

In this formula, elementary charge is represented by e, with a value of e = 1.6 × 10⁻¹⁹ C and ${\mathrm{E}}_{\mathrm{F}}$ stands for the Fermi level. Here, ℏ represents the reduced Planck constant and τ denotes the relaxation time, i represents the unit of imaginary numbers, and ω stands for the angular frequency of the incoming light. In this model, 1 eV is selected as the Fermi level of graphene. This can be achieved by applying an external voltage or controlling the doping concentration. As depicted in Figure 1d, to achieve a tunable Fermi level in graphene, a thin layer of ionic gel is applied to the patterned top layer of graphene, with gold electrodes in contact with the ionic gel. The ionic gel has low absorption and high capacitance density, which results in minimal influence on the overall absorption spectrum [40]. The relaxation time τ = 1.1 ps.

Using an equivalent circuit diagram such as the one in Figure 3 makes the structure clearer. In this model, the Au layer is considered a short-circuit layer, so Z_Au is negligible.

Graphene is equivalent to a resistor, an inductor, and a capacitor. The parameters in the figure are represented as follows [41,42]:

$$\left\{\begin{array}{c}{\mathrm{Z}}_1=\mathrm{j}{\mathrm{Z}}_{\mathrm{d}}\mathrm{t}\mathrm{a}\mathrm{n}\left({\mathrm{k}}_{\mathrm{d}}{\mathrm{t}}_{\mathrm{s}}\right)\\ {\mathrm{Z}}_{\mathrm{d}}=\sqrt{{\mathsf{\mu }}_0/{\mathsf{\epsilon }}_0{\mathsf{\epsilon }}_{\mathrm{r}}}\\ {\mathrm{k}}_{\mathrm{d}}=2\mathrm{\pi }\mathrm{f}\sqrt{{\mathsf{\epsilon }}_0{\mathsf{\epsilon }}_{\mathrm{r}}{\mathsf{\mu }}_0}\end{array}\right.$$

(3)

$${\mathrm{Z}}_{\mathrm{g}}={\mathrm{R}}_{\mathrm{g}}+\mathrm{j}{\mathrm{X}}_{\mathrm{g}}={\mathrm{R}}_{\mathrm{g}}+\mathrm{j}(2\mathsf{\pi }\mathrm{f}{\mathrm{L}}_{\mathrm{g}}-{\displaystyle \frac{1}{2\mathsf{\pi }\mathrm{f}{\mathrm{C}}_{\mathrm{g}}}})$$

(4)

$$\left\{\begin{array}{c}{\mathrm{Z}}_{\mathrm{i}\mathrm{n}}={\displaystyle \frac{{\mathrm{Z}}_1·{\mathrm{Z}}_{\mathrm{g}}}{{\mathrm{Z}}_1+{\mathrm{Z}}_{\mathrm{g}}}}\\ \mathrm{\Gamma }={\displaystyle \frac{\mathrm{R}\mathrm{e}\left({\mathrm{Z}}_{\mathrm{i}\mathrm{n}}\right)-{\mathrm{Z}}_0}{\mathrm{R}\mathrm{e}\left({\mathrm{Z}}_{\mathrm{i}\mathrm{n}}\right)+{\mathrm{Z}}_0}}\\ {\mathrm{Z}}_0=\sqrt{{\displaystyle \frac{{\mathsf{\mu }}_0}{{\mathsf{\epsilon }}_0}}}=120\mathrm{\pi }\end{array}\right.$$

(5)

Calculations of Rg, Lg, and Cg for graphene can be found in Ref [43].

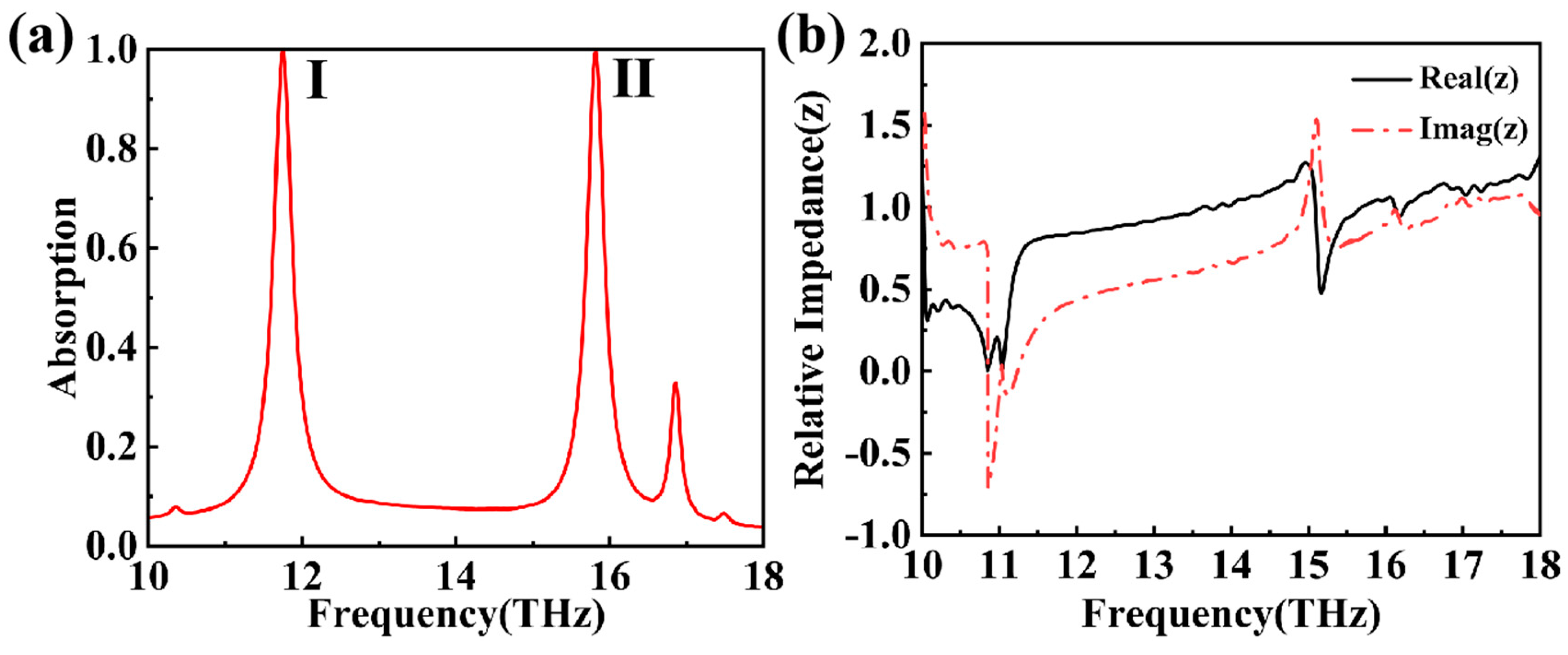
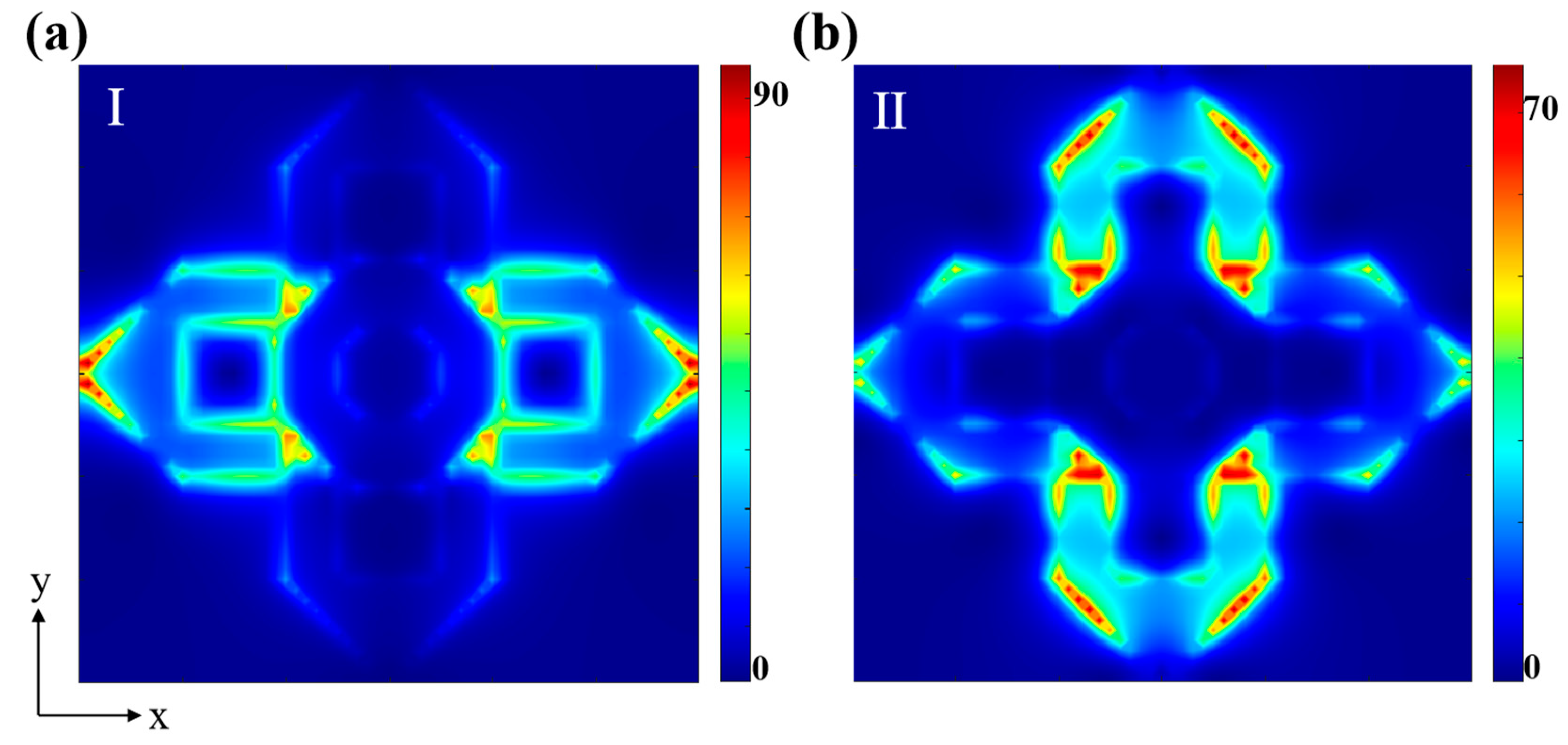
3. Outcomes and Analysis

The absorption condition of the graphene absorber model is shown in Figure 4a. It is apparent from the figure that there are two absorption peaks in the absorber in this paper. The absorptivity of the absorber is calculated by the following formula [40,44]:

$$\mathrm{A}\left(\mathsf{\omega }\right)=1-\mathrm{T}\left(\mathsf{\omega }\right)-\mathrm{R}\left(\mathsf{\omega }\right)$$

(6)

Since Au has strong reflection ability as the bottom layer, it could be considered that the T(ω) = 0. The above formula is changed to [45]:

$$\mathrm{A}\left(\mathsf{\omega }\right)=1-\mathrm{R}\left(\mathsf{\omega }\right)$$

(7)

For A(ω) to achieve perfect absorption, then R(ω) should be as small as possible. The absorber designed in this paper has two absorption peaks in the near-terahertz band, which are named Mode I and Mode II respectively; the absorption rates are 99.9947% and 99.6079% in sequence. Subsequently, the internal generation mode of the absorption peaks in this paper will be analyzed through electric field diagrams and impedance matching.

According to the impedance matching principle, it can be known that when the real part approaches 1 and the imaginary part approaches 0, the impedance of the absorber shall match that of space. At this time, the best absorption effect is achieved [46,47]. In Figure 4b, at 11.7475 THz, it can be seen that the real part of the impedance of the absorber designed in this paper is 0.88, and the imaginary part is 0.37. The fit is improved, resulting in perfect absorption at this resonance frequency. Consequently, absorptive peak Mode I emerges. At 15.8196 THz, the real part is 1.03 and the imaginary part is 0.61. However, the imaginary part exhibits poor fitting accuracy, leading to lower absorption compared to the first peak. Thus, the peak value of I is higher than that of II.

In order to further explore the internal principle of narrowband absorption, FDTD Solution is used for simulation to obtain the electric field distribution in the xoy plane at 11.7475 THz and 15.8196 THz, and the electric field intensity diagrams of the two peaks are analyzed, as illustrated in Figure 5. Figure 5a is the electric field diagram of absorptive peak Mode I. It might be known that the electric field has an uneven distribution and is mainly distributed in the horizontal direction. The electric field at the circular pore and at the tip of the triangle is stronger than that in other places. Figure 5b is the electric field diagram corresponding to absorptive peak Mode II. The field of electricity enhancement is primarily in the vertical direction, but there is also a distribution in the horizontal direction, causing large-scale coupling and resulting in energy loss [48,49]. Therefore, absorptive peak Mode II has a smaller peak value than absorptive peak Mode I. Moreover, absorptive peak Modes I and II both have absorption rates greater than 99%. One of the reasons for the ultra-high absorption is local plasmon resonance. That is to say, the electric field couples with the plasma in specific areas on the surface of graphene to generate electric dipole resonance. This resonance leads to an uneven distribution of the electric field. Subsequently, it leads to the enhancement of the local electric field and the regionalization and strengthening of the electromagnetic field, thus producing such an uneven electric field [50]. The second reason is that there is gold as the substrate at the bottom. Due to the reflection of the gold substrate, the incident ray might be reflected continuously between graphene and the gold, strengthening the uptake of light and attaining ultra-high absorption [51]. According to this explanation, a top layer of graphene absorbs incoming light of the specific frequency. At two positions where the absorber is located, the peak absorption rate of more than 99% is achieved, which can be called perfect absorption. In short, the local electric field is chiefly distributed in the segment with graphene absorber patterns. Moreover, there is almost no electric field beyond the pattern. Based on periodic conditions, the electric field allocation of graphene is periodic. This ability to locally concentrate the electric field can also localize light near graphene [52]. So far, only the reasons for the perfect absorption of the absorber at two frequencies have been explained.

In Figure 6a, (i) represents the initial design of the absorber, which is a cruciform model that appears as resonance peaks with absorption of 93.44% and 53.29%, respectively. Figure 7a clearly shows that the magnetic and electric fields are predominantly confined within the SiO₂ at 10.21 THz and 16.44 THz. Notably, no electron wave penetration into the underlying Au layer is observed, which confirms the excitation of a Fabry–Pérot resonance cavity between the graphene and gold layers [53]. It is also shown that electromagnetic waves are reflected back and forth between graphene–silica–gold, resulting in high absorption. From (i) in Figure 7b, large-scale coupling of the electric field occurs, leading to energy dissipation. Subsequently, based on (i) in Figure 6a, we cut four triangles in both the horizontal and vertical directions to obtain (ii), with absorption rates of 77.89% and 66.5%, respectively. Although there are two absorption peaks, the absorption rate is low. However, as can be seen in Figure 7b(ii), a localized EMF enhancement occurs at the tip of the triangle. We then cut the rectangle in all four directions as shown in Figure 7b(iii), which is still a centrosymmetric figure, but with only one resonance peak of higher absorptivity. From the electric field analysis in Figure 7b(iii), the weaker electric field distribution in the horizontal direction corresponds to a lower value of the absorptivity of the resonance peaks, and the stronger electric field distribution in the vertical direction thus corresponds to a higher absorptivity. Next, we considered the central square and cut it into a circle with a radius of 1.1 µm, as shown in (iv), resulting in two absorption peaks but with a lower absorption rate. From the electric field analysis in Figure 7b(iv), the electric field strength increases in the horizontal direction, but the localized enhancement of the electric field of the first resonance peak occurs only outside the circle, which is small in scope, and the distribution of the electric field in the vertical direction remains strong. Finally, we cut the circle into a ring, as shown in (v), achieving two absorption peaks with absorption rates exceeding 99%, and an average absorption rate of 99.8%. The final design not only realizes two absorption peaks, but also achieves an absorption rate of more than 99%, showing extremely high electromagnetic wave absorption efficiency. This study provides a valuable reference for designing high-performance electromagnetic wave-absorbing materials.

Next, the relaxation time and Fermi level of graphene were adjusted to investigate the impact of their changes on the absorber designed in this paper. The formula for relaxation time is as follows [54]:

$$\mathsf{\tau }={\displaystyle \frac{\mathsf{\mu }{\mathsf{\mu }}_{\mathrm{c}}}{\mathrm{e}{\mathrm{V}}_{\mathrm{F}}^2}}$$

(8)

In which $\mathsf{\mu }$ represents the graphene carrier drift mobility and ${\mathsf{\mu }}_{\mathrm{c}}$ stands for the chemical potential. The graphene relaxation time can be modulated by chemical doping of the graphene layer. As τ increases, the increase in carriers leads to a greater contribution of them to plasma oscillations. This results in an increase in the absorption rate. As can be seen in Figure 8a, the alteration in τ has little to no influence on the frequency position where the absorptive peak lies. However, it has a relatively more significant influence on its absorptivity. The absorptivity changes as the value of τ changes. When τ is equal to 0.1 ps, absorptive peak Mode I has an absorptivity of 44.9771%, whereas absorptive peak Mode II only has an absorptivity of 36.4499%. When τ = 0.6 ps, absorptive peak Mode I has an absorptivity of 92.69888%, while that of absorptive peak Mode II is 88.9752%. The absorptive peak set by the model proposed in this paper is at τ = 1.1 ps. Absorptive peak Mode I has an absorptivity of 99.9947%, while that of absorptive peak Mode II is 99.6079%. Therefore, when the electromagnetic field is coupled to graphene, the relaxation time should not be unduly short [55,56]. If it is too short, for example, τ = 0.1 ps, the absorptive peak value is very low. For absorptive peak Modes I and II, they are only 44.9771% and 36.4499%, respectively. However, if it is extremely long, a large portion of the light is bounced back and therefore the absorption rate cannot help but decrease. At τ = 1.6 ps, the absorptivity of absorptive peak Mode I declines to 95.7149%, while absorptive peak Mode II has an absorptivity of 98.2862%, It is less than that of the model proposed in this paper. τ is extremely long, while at τ = 2.1 ps, the absorptivity of absorptive peak Mode I drops to 89.5530%, whereas the absorptivity of absorptive peak Mode II is 93.7126%, showing an obvious downward trend. The relaxation time is more on the long side or more on the short side, and the absorptivity tends to show a downward tendency [57]. Therefore, in order to achieve perfect absorption, an appropriate relaxation time should be chosen. So, this paper adopts the absorptive peak model corresponding to τ = 1.1 ps.

The Fermi level, chemical doping, or external voltage can be used to adjust it; the relationship is as follows [58]:

$${\mathrm{E}}_{\mathrm{f}}={\mathrm{\hslash }\mathrm{V}}_{\mathrm{F}}\sqrt{{\displaystyle \frac{\mathsf{\pi }{\mathsf{\epsilon }}_0{\mathsf{\epsilon }}_{\mathrm{r}}{\mathrm{V}}_{\mathrm{g}}}{\mathrm{e}{\mathrm{t}}_{\mathrm{s}}}}}$$

(9)

The Fermi level can be regulated through chemical impurity incorporation or applied voltage. The applied voltage is denoted as ${\mathrm{V}}_{\mathrm{g}}$. The electron charge is represented by e. Fermi velocity is represented by ${\mathrm{V}}_{\mathrm{F}}={10}^6\mathrm{m}/\mathrm{s}$, ${\epsilon }_0$ is the permittivity of free space. ${\mathsf{\epsilon }}_{\mathrm{r}}$ is the relative permittivity of SiO₂, and the depth of SiO₂ is represented by ${\mathrm{t}}_{\mathrm{s}}$. The Fermi level of graphene can also be regulated by other means such as chemical doping [59].

It can be seen from Figure 8b that with the change in the Fermi level of graphene, the frequency and absorption rate changes of each absorptive peak model can be observed. When the Fermi level of graphene goes up, both absorptive peak models move in the direction of increasing frequency, that is, blue shift. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 0.90 eV up to 0.95 eV, the frequency of absorptive peak Mode I changes from 11.1703 THz to 11.5711 THz, with a movement distance of 0.4008 THz. The displacement of absorptive peak Mode II changes from 15.0341 THz to 15.5631 THz, with a movement distance of 0.529 THz. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 0.95 eV up to 1.00 eV, the displacement of absorptive peak Mode I changes from 11.5711 THz to 11.7475 THz, with a movement distance of 0.1764 THz. The displacement of absorptive peak Mode II moves from 15.5631 THz to 15.8196 THz, with a movement distance of 0.2565 THz. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from1.00 eV up to 1.05 eV, the displacement of absorptive peak Mode I changes from 11.7475 THz to 12.2124 THz, with a movement distance of 0.4649 THz. The displacement of absorptive peak Mode II changes from 15.8196 THz to 16.4289 THz. with a movement distance of 0.6093 THz. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 1.05 eV up to 1.10 eV, the displacement of absorptive peak mode I changes from 12.2124 THz to 12.5491 THz, with a movement distance of 0.3295 THz. The displacement of absorptive peak mode I changes from 16.4289 THz to 16.8457 THz, with a movement distance of 0.4168 THz. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 0.90 eV up to 0.95 eV, the absorptivity of absorptive peak I changes from 97.8417% to 99.5779%, and the absorptivity of absorptive peak II changes from 99.5984% to 99.6205%. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 0.95 eV up to 1.00 eV, the absorptivity of absorptive peak I changes from 99.5779% to 99.9947%. and the absorptivity of absorptive peak II changes from 99.6205% to 99.6079%. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 1.00 eV up to 1.05 eV, the absorptivity of absorptive peak I changes from 99.9947% to 98.9805%, and the absorptivity of absorptive peak II changes from 99.6079% to 99.5148%. When ${\mathrm{E}}_{\mathrm{f}}$ ranges from 1.05 eV up to 1.10 eV, the absorptivity of absorptive peak I changes from 98.9805% to 97.6016%, and the absorptivity of absorptive peak II changes from 99.5148% to 99.2080%. Without changing other conditions, we control the position and peak value of the absorptive peak by means of changing the Fermi level. Therefore, ${\mathrm{E}}_{\mathrm{f}}$ = 1.00 eV is chosen as the Fermi level selected by the model in this paper; in this way, the levels of absorption of both absorptive peak Mode I and absorptive peak Mode II can exceed 99%. Of course, we can know that the Fermi level of graphene can control and influence the frequency of the absorptive peak, while the relaxation time is mainly able to control and influence the absorptivity of the absorptive peak [60,61]. We tune the frequency and peak value of the absorptive peak by adjusting the relaxation time and Fermi level. We can precisely obtain the desired type of absorptive peak. Thus, the absorber in this paper is adjustable.

Not only that, the effect of polarization angle variation on the absorption rate needs to be considered in practical applications [62,63,64]. As shown in Figure 9a,b, we simulated the absorptivity maps of the absorber in TE mode and TM mode and present the normalized absorption spectra (from TM polarization to TE polarization) when the normal polarization angle was varied in the range from 0° to 90°. We can find that the absorption curves of the two modes completely overlap and there is no change in absorption at each polarization angle as the polarization angle is increased from 0° to 90°, which is due to the fact that the structure we designed is completely symmetric. The relationship between wavelength and absorptivity is obvious. When the polarization angle changes, the absorbance is almost unaffected, and the absorbance in the same frequency band remains almost constant. In conclusion, this absorber not only maintains high absorbance in a wide band, but also has polarization insensitivity, resulting in a wider range of applications and higher value.

For better practical applications, we next discuss the influence of the external refractive index and silicon dioxide refractive index on the absorbing device. As shown in Figure 10a, the ambient refractive index in this paper is changed from 1.00 to 1.08 at equal intervals. A blue shift takes place in the absorber model in this paper. We discuss the sensitivity of the absorber with $∆\mathrm{n}$ = 0.02 [65]:

$$\mathrm{S}={\displaystyle \frac{∆\mathrm{f}}{∆\mathrm{n}}}$$

(10)

where $∆\mathrm{f}$ is the peak movement distance. As shown in Figure 10b, we can know that S_I = 2.405 THz/RIU, and n is the environmental refractive index. S_II = 3.205 THz/RIU, where RIU stands for the refractive index unit. Mode I and Mode II have different sensitivities to environmental changes. In the case of resonance at a higher frequency, the higher the sensitivity, when it comes to the shift of the absorption peak, the larger the frequency interval. Peak I’s moving distance of frequency is further than that of peak II. This can also be clearly seen in Figure 10a. In terms of absorption rate, as the environmental refractive index rises, the absorptivity of absorption peak Mode I shows a declining tendency, with the environmental refractive index changing from 1.00 to 1.08; the maximum absorptivity of absorption peak Mode I is 99.99%, while the minimum value is 99.66%, a decrease of 0.33%. The change is relatively small. Absorptive peak Mode II shows a slightly upward and then downward trend. The maximum absorption rate of absorptive peak Mode II is 99.80%, and 99.07% is the minimum value. It has changed by 0.73%. As the environmental refractive index changes, Mode II changes more than Mode I. At the same time, we can see the differences formed by these two absorptive peak models. However, as the environmental refractive index changes, the absorptivity of the absorption model in this paper is still very high; both are above 99%. Judging from the value of S, the absorber designed in this paper is very sensitive to environmental changes. Later, a comparison will be made between the absorber designed in this paper and other absorbers. As is shown in Figure 10c, we also discuss the situation of SiO₂’s refractive index changing from 1.90 to 2.10 and the sensitive absorptive peak when ∆n’ = 0.05, where $∆\mathrm{f}$ is the peak movement distance.

As shown in Figure 10b, can know that S_I = 2.405 THz/RIU, and n is the environmental refractive index. S_II = 3.205 THz/RIU, where RIU stands for the refractive index unit. Mode I and Mode II have different sensitivities to environmental changes. In the case of resonance at a higher frequency, the higher the sensitivity, the larger the frequency interval when it comes to the shift of the absorption peak. Peak I’s moving distance of frequency is further than that of peak II. This can also be clearly seen in Figure 10a. In terms of absorption rate, as the environmental refractive index rises, the absorptivity of absorption peak Mode I shows a declining tendency. As the environmental refractive index transforms from 1.00 to 1.08, which corresponds to the red, green, blue, orange, and purple solid lines, respectively, the maximum absorptivity of absorption peak Mode I is 99.99%, while the minimum value is 99.66%, a decrease of 0.33%. The change is relatively small. Absorptive peak Mode II shows a slightly upward and then downward trend. The maximum absorption rate of absorptive peak Mode II is 99.80%, and 99.07% is the minimum value. It has changed by 0.73%. As the environmental refractive index changes, Mode II changes more than Mode I. At the same time, we can see the differences formed by these two absorptive peak models. However, as the environmental index of refraction changes, the absorptivity of the absorption model in this paper is still very high, both above 99%. Judging from the value of S, the absorber designed in this paper is very sensitive to environmental changes. Later, a comparison will be made between the absorber designed in this paper and other absorbers. As is shown in Figure 10c, we also discuss the situation of SiO₂’s refractive index changing from 1.90 to 2.10, which corresponds to the black, blue, red, green, and purple solid lines, respectively, and the sensitive absorptive peak when ∆n’ = 0.05. We can also see that through our calculation [66]:

$${\mathrm{S}}^{\prime }={\displaystyle \frac{∆{\mathrm{f}}^{\prime }}{∆{\mathrm{n}}^{\prime }}}$$

(11)

${\mathrm{S}}^{\prime }$ is the sensitivity of SiO₂. Through calculation, ${\mathrm{S}}_{\mathrm{I}}^{\prime }$ = 4.81 THz/RIU and ${\mathrm{S}}_{\mathrm{I}\mathrm{I}}^{\prime }$ = 6.412 THz/RIU. The frequency density of mode peak I and mode peak II is different. Mode II has high sensitivity and a large movement distance. Upon the change in the refractive index of SiO₂, the absorptivity of the absorption mode in this paper exceeds 98%. As the SiO₂’s refractive index increases, the change in absorptivity Mode I increases first, then decreases, and then increases again. The change in absorption maximum Mode II is continuously decreasing. Such changes also indicate the different properties of the bimodal model. The absorption peak can be manipulated by regulating the SiO₂’s index of refraction.

Figure 11 shows the changes regarding the FWHM of two absorption peaks as well as the figure of merit (FOM) in both absorption modes. The relationship between FOM and sensitivity and FWHM is as below [67]:

$$\mathrm{F}\mathrm{O}\mathrm{M}={\displaystyle \frac{\mathrm{S}}{\mathrm{F}\mathrm{W}\mathrm{H}\mathrm{M}}}$$

(12)

where full width at half maxima is FWHM and S is the sensitivity. According to the results calculated, the maximum values of FOM for Mode I and Mode II are FOM_I = 8.4803 RIU⁻¹ and FOM_II = 11.3011 RIU⁻¹.

This paper also compares the Q values in two modes. The equation is given as below [68,69]:

$$\mathrm{Q}={\displaystyle \frac{\mathrm{f}\left(\mathrm{r}\mathrm{e}\mathrm{s}\right)}{\mathrm{F}\mathrm{W}\mathrm{H}\mathrm{M}}}$$

(13)

where f(res) represents the resonance frequency and full width at half maxima is FWHM. We calculated that the maximum Q values of Mode I and Mode II are Q_I = 41.423 and Q_II = 55.781, respectively.

Finally, we compare the absorber designed in this paper with other designed absorbers. Through

Table 1. This work compared with other absorbers.

Source	Materials	Peak Count	Mean Absorption Rate	Sensitivity [THz/RIU]	FOM	Q-Factor
[25]	Graphene	2	96.05%	1.84	~	~
[70]	Graphene	2	99.5%	0.984	3.57	14.54
[71]	Graphene	2	98.41%	1.15	1.55	8.92
[72]	metal layer	1	99%	2.1	7.03	~
[73]	Graphene	3	97.93%	0.382	3.24	58.64
[74]	Graphene	1	76.17%	0.114	3.15	11.2
[75]	Graphene	3	99.53%	1.86	2.14	~
This work	Graphene	2	99.8%	3.205	11.301	48.61

, we know that the absorber in this paper has significant advantages in terms of average absorption rate, figure of merit (FOM) value, sensitivity, and Q-Factor [25,70,71,72,73,74,75]. For [70], there are two absorption peaks but its sensitivity is only 0.984 THz/RIU, which is much lower than our absorber sensitivity. For [71], its sensitivity is lower, at only 1.15 THz/RIU, the FOM value is only 1.55, and the Q-Factor is much less than 48.61. Ref. [72] has a sensitivity of 2.1 THz/RIU, but the FOM value is not as high as in this paper, and the number of peaks is not as high as in this paper. The Q-Factor in [73] is higher, and it has more peaks than in this paper, but its sensitivity is low and it lacks in sensing. In [74], absorption and sensitivity are extremely low. In [25], both sensitivity and absorbance are lower than in this paper. Ref. [75] has more absorption peaks than this paper, but its sensitivity and FOM values are lower than in this paper.

In summary, the graphene absorber designed in this paper achieves an average absorption of 99.8%, which is significantly higher than in other absorber models. Its sensitivity reaches 3.205 THz/RIU, which is also higher than in other absorbers. In addition, its FOM value is 11.301, which is higher than the maximum value of other absorbers in Table 1. The designed dual-mode perfect absorber has a number of advantages that make it promising for a wide range of applications in the fields of sensors, optoelectronic devices, etc. The absorber operates in the near-terahertz band, which provides an important support for the research in this band.

4. Conclusions

In summary, the near-terahertz tunable bimodal perfect absorber designed in this paper features a flower-like pattern on the top layer, arranged periodically with high symmetry. It is a classic three-dimensional metamaterial perfect absorber (MPA) structure and is easy to fabricate. Using impedance matching theory, the matching conditions of the absorption peaks were verified, with peak I exhibiting superior matching compared to peak II, and the corresponding peak value was also higher, with an average absorptivity of 99.8%. To discuss the principle of the dual-mode narrowband absorption designed in this paper, electric field distribution diagrams were analyzed. It was found that the electric field is mainly concentrated in the graphene-patterned area, with almost no electric field outside the pattern. Through the design evolution process, it was further concluded that the absorption peaks of the absorber model in this paper are optimized results. In the article, the effects of changing the relaxation time and Fermi level on the absorber were also explored, leading to the conclusion that the absorber designed in this paper is tunable. Next, the influence of external factors on the absorber was investigated. First, as the polarization angle of the incident light changes, the absorber designed in this paper shows no significant change, leading to the conclusion that the absorber is not affected by the polarization angle of the incident light. Additionally, by changing the refractive index, it was found that our absorber has a sensitivity of 3.205 THz/RIU, a figure of merit (FOM) of 11.301, and a Q-Factor of 48.61, making it suitable for manufacturing refractive index sensors.