Hausdorff Distance and Similarity Measures for Single-Valued Neutrosophic Sets with Application in Multi-Criteria Decision Making
Abstract
:1. Introduction
- Proposing two novel forms of Hausdorff distance for SVNSs.
- Developing some SMs for SVNSs using our newly defined Hausdorff distances.
- Construction of an MCDM method under single-valued neutrosophic environment.
- Identification/selection of best alternative among available options as well as the overall ranking of alternatives under study by using our proposed SVN-MCDM.
- Application of our proposed SVN-MCDM scheme to solve two real problems of MCDM under SVN environment.
2. Literature Review
3. Preliminaries
4. Hausdorff Distance and Similarity Measures for SVNSs Based on Hausdorff Metric
4.1. Hausdorff Distance for SVNSs
4.2. Similarity Measures for SVNSs
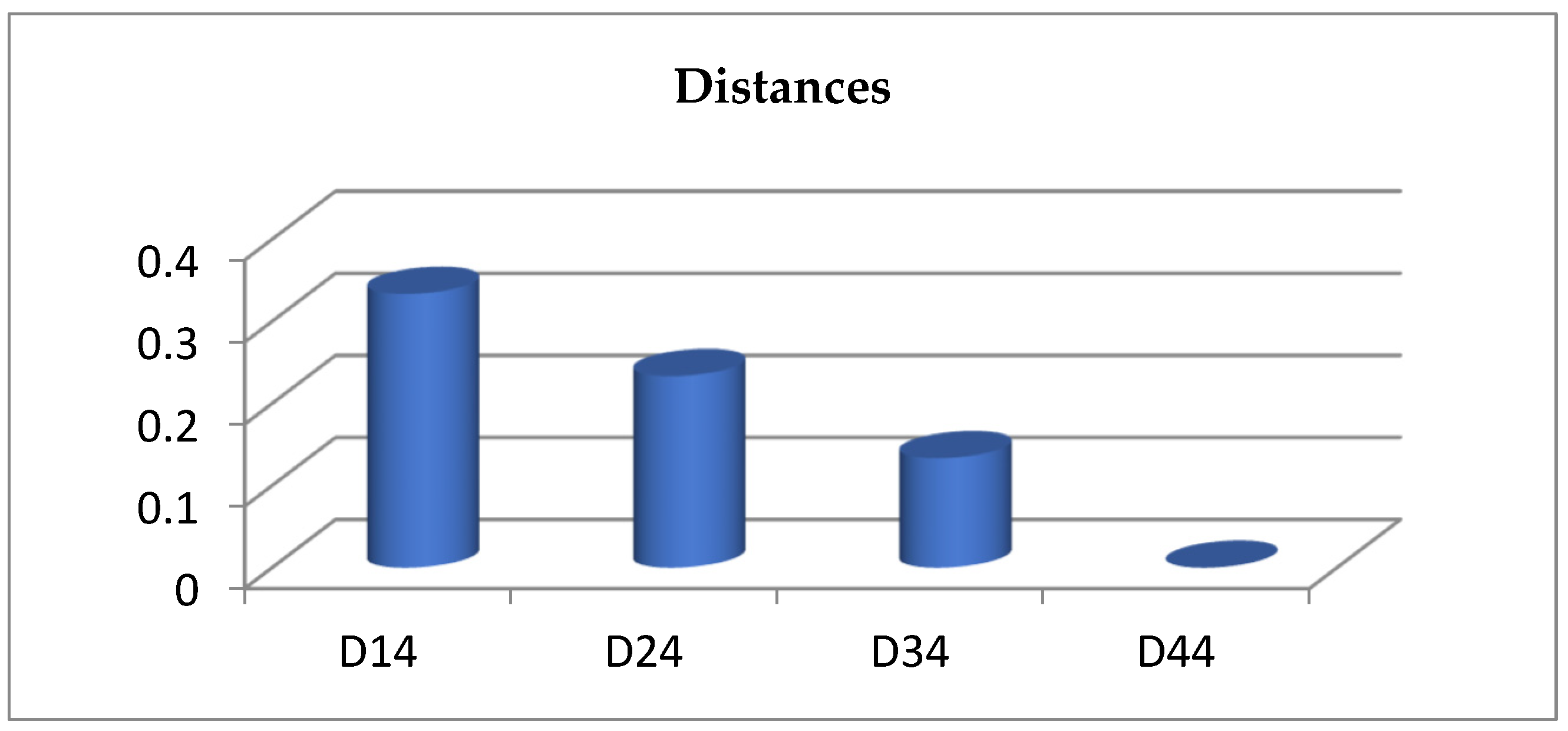
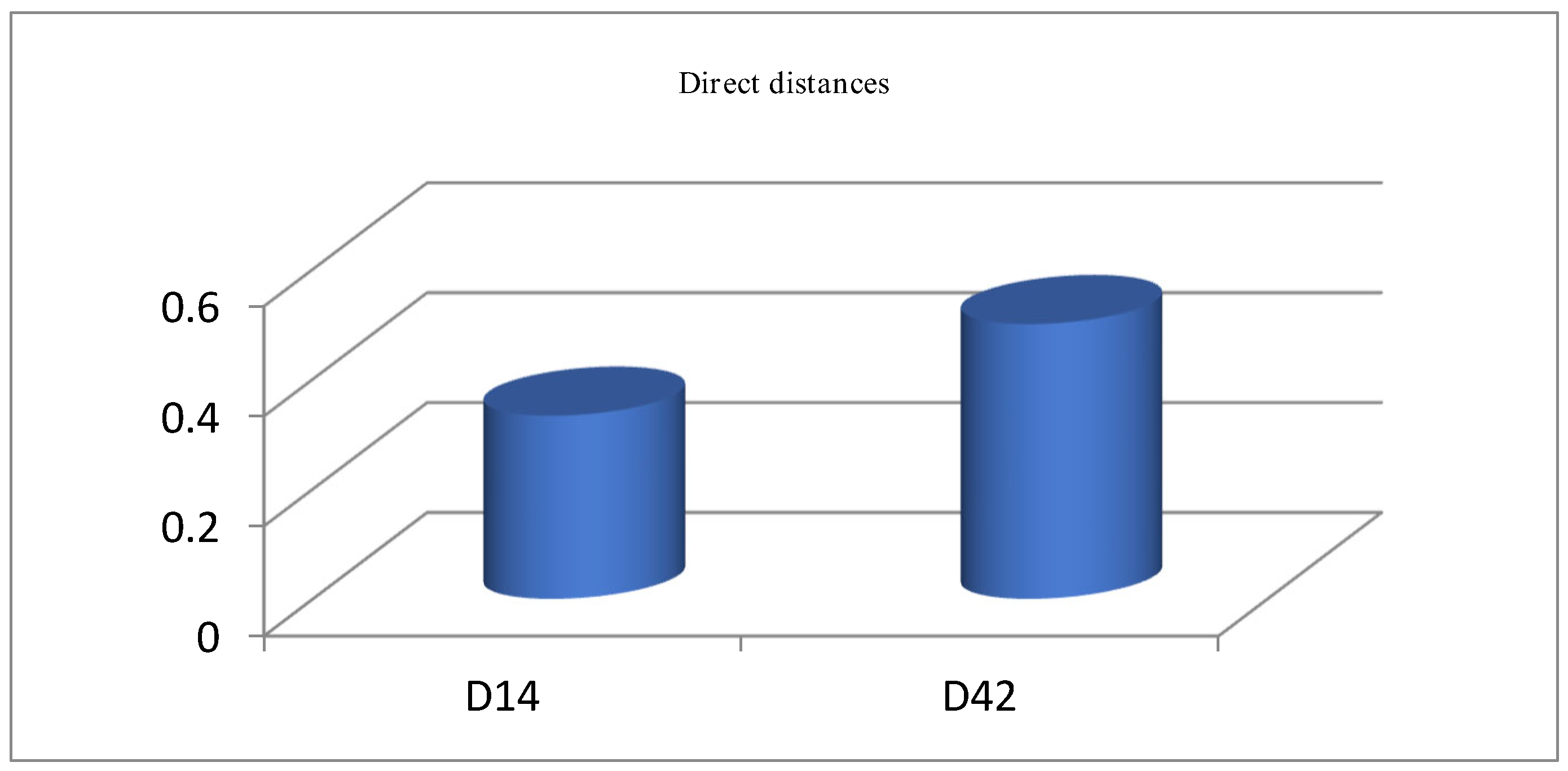
4.3. Numerical Analysis and Illustration
5. Application of the Proposed Methods in Multi-Criteria Decision Making
6. Managerial Insights and Advantages
7. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef] [Green Version]
- Chang, S.T.; Lu, K.P.; Yang, M.S. Fuzzy change-point algorithms for regression models. IEEE Trans. Fuzzy Syst. 2015, 23, 2343–2357. [Google Scholar] [CrossRef]
- Lu, K.P.; Chang, S.T.; Yang, M.S. Change-point detection for shifts in control charts using fuzzy shift change-point algorithms. Comput. Ind. Eng. 2016, 93, 12–27. [Google Scholar] [CrossRef]
- Ruspini, E.H.; Bezdek, J.C.; Keller, J.M. Fuzzy clustering: A historical perspective. IEEE Comput. Intell. Mag. 2019, 14, 45–55. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic Fuzzy Sets: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Hwang, C.M.; Yang, M.S. New construction for similarity measures between intuitionistic fuzzy sets based on lower, upper and middle fuzzy sets. Int. J. Fuzzy Syst. 2013, 15, 359–366. [Google Scholar]
- Garg, H.; Kaur, J. A Novel (R,S)-norm entropy measure of intuitionistic fuzzy sets and its applications in multi-attribute decision-making. Mathematics 2018, 6, 92. [Google Scholar] [CrossRef] [Green Version]
- Yang, M.S.; Hussian, Z.; Ali, M. Belief and plausibility measures on intuitionistic fuzzy sets with construction of belief-plausibility TOPSIS. Complexity 2020, 4, 1–12. [Google Scholar] [CrossRef]
- Smarandache, F. A Unifying Field in Logics. Neutrosophy: Neutrosophic Probability, Set and Logic; American Research Press: Rehoboth, DE, USA, 1999. [Google Scholar]
- Smarandache, F. Neutrosophic set-a generalization of the intuitionistic fuzzy set. Int. J. Pure Appl. Math. 2005, 24, 287–297. [Google Scholar]
- Wang, H.; Smarandache, F.; Zhang, Y.; Sunderraman, R.S. Single valued neutrosophic sets. Multispace Multistructure 2010, 4, 410–413. [Google Scholar]
- Huang, H.L. New distance measure of single-valued neutrosophic sets and its application. Int. J. Intell. Syst. 2016, 31, 1021–1032. [Google Scholar] [CrossRef] [Green Version]
- Sodenkamp, M.A.; Tavana, M.; Caprio, D.D. An aggregation method for solving group multi-criteria decision-making problems with single-valued neutrosophic sets. Appl. Soft Comput. 2018, 71, 715–727. [Google Scholar] [CrossRef] [Green Version]
- Chai, J.S.; Selvachandran, G.; Smarandache, F.; Gerogiannis, V.C.; Son, L.H.; Bui, Q.T.; Vo, B. New similarity measures for single-valued neutrosophic sets with applications in pattern recognition and medical diagnosis problems. Complex Intell. Syst. 2021, 7, 703–723. [Google Scholar] [CrossRef]
- Khan, M.J.; Kumam, P.; Deebani, W.; Kumam, W.; Shah, Z. Distance and similarity measures for spherical fuzzy sets and their applications in selecting mega projects. Mathematics 2020, 8, 519. [Google Scholar] [CrossRef] [Green Version]
- Saqlain, M.; Riaz, M.; Saleem, M.A.; Yang, M.S. Distance and similarity measures for neutrosophic hypersoft set (NHSS) with construction of NHSS-TOPSIS and applications. IEEE Access 2021, 9, 30803–30816. [Google Scholar] [CrossRef]
- Grzegorzewski, P. Distances between intuitionistic fuzzy sets and/or interval-valued fuzzy sets based on the Hausdorff metric. Fuzzy Sets Syst. 2004, 148, 319–328. [Google Scholar] [CrossRef]
- Nalder, J.S. Hyperspaces of Sets; Marcel Dekker: New York, NY, USA, 1978. [Google Scholar]
- Yang, M.S.; Hussian, Z. Distance and similarity measures of hesitant fuzzy sets based on Hausdorff metric with applications to multi-criteria decision making and clustering. Soft Comput. 2019, 23, 5835–5848. [Google Scholar] [CrossRef]
- Xu, D.; Xian, H.; Cui, X.; Hong, Y. A new single-valued neutrosophic distance for TOPSIS, MABAC and new similarity measure in multi-attribute decision-Making. IAENG Int. J. Appl. Math. 2020, 50, 72–79. [Google Scholar]
- Bhaumik, A.; Roy, S.K.; Weber, G.W. Multi-objective linguistic-neutrosophic matrix game and its applications to tourism management. J. Dyn. Games 2021, 8, 101–118. [Google Scholar] [CrossRef]
- Ghosh, S.; Roy, S.K.; Verdegay, J.L. Fixed-charge solid transportation problem with budget constraints based on carbon emission in neutrosophic environment. Soft Comput. 2022, 26, 11611–11625. [Google Scholar] [CrossRef]
- De, S.K.; Roy, B.; Bhattacharya, K. Solving an EPQ model with doubt fuzzy set: A robust intelligent decision-making approach. Knowl.-Based Syst. 2022, 235, 107666. [Google Scholar] [CrossRef]
- Giri, B.K.; Roy, S.K. Neutrosophic multi-objective green four-dimensional fixed-charge transportation problem. Int. J. Mach. Learn. Cybern. 2022, 13, 3089–3112. [Google Scholar] [CrossRef]
- Roy, B.; De, S.K.; Bhattacharya, K. Decision making in two-layer supply chain with doubt fuzzy set. Int. J. Syst. Sci. Oper. Logist. 2022, 1–27. [Google Scholar] [CrossRef]
- Ye, J. Another form of correlation coefficient between single valued neutrosophic sets and its multiple attribute decision-making method. Neutrosophic Sets Syst. 2013, 1, 8–12. [Google Scholar]
- Das, S.K.; Roy, S.K. Effect of variable carbon emission in a multi-objective transportation-p-facility location problem under neutrosophic environment. Comput. Ind. Eng. 2019, 132, 311–324. [Google Scholar] [CrossRef]
- Biswas, P.; Pramanik, S.; Giri, B.C. TOPSIS method for multi-attribute group decision-making under single-valued neutrosophic environment. Neural Comput. Appl. 2016, 27, 727–737. [Google Scholar] [CrossRef]
- Garg, H. A novel divergence measure and its based TOPSIS method for multi criteria decision-making under single-valued neutrosophic environment. J. Intell. Fuzzy Syst. 2019, 36, 101–115. [Google Scholar]
- Zhang, Z.; Wu, C. A novel method for single-valued neutrosophic multi-criteria decision making with incomplete weight information. Neutrosophic Sets Syst. 2014, 4, 35–49. [Google Scholar]
- Jana, C.; Pal, M. Multi-criteria decision making process based on some single-valued neutrosophic Dombi power aggregation operators. Soft Comput. 2021, 25, 5055–5072. [Google Scholar] [CrossRef]
- Xu, D.S.; Wei, C.; Wei, G.W. TODIM method for single-valued neutrosophic multiple attribute decision making. Information 2017, 8, 125. [Google Scholar] [CrossRef] [Green Version]
- Borah, G.; Dutta, P. Multi-attribute cognitive decision making via convex combination of weighted vector similarity measures for single-valued neutrosophic sets. Cogn. Comput. 2021, 13, 1019–1033. [Google Scholar] [CrossRef] [PubMed]
- Zeng, S.; Luo, D.; Zhang, C.; Li, X. A correlation-based TOPSIS method for multiple attribute decision making with single-valued neutrosophic information. Int. J. Inf. Technol. Decis. Mak. 2020, 19, 343–358. [Google Scholar] [CrossRef]
- Ye, J. Multiple attribute group decision-making method with completely unknown weights based on similarity measures under single valued neutrosophic environment. J. Intell. Fuzzy Syst. 2014, 27, 2927–2935. [Google Scholar] [CrossRef]
- Huttenlocher, D.P.; Klanderman, G.A.; Rucklidge, W.J. Comparing images using the Hausdorff distance. IEEE Trans. Pattern Anal. Mach. Intell. 1993, 15, 850–863. [Google Scholar] [CrossRef] [Green Version]
- Hussian, Z.; Yang, M.S. Distance and similarity measures of Pythagorean fuzzy sets based on the Hausdorff metric with application to fuzzy TOPSIS. Int. J. Intell. Syst. 2019, 34, 2633–2654. [Google Scholar] [CrossRef]


| Authors | MCDM | Hausdorff Distance | Multiple Comparisons (for Nearest Point) | Measures | Application | |
|---|---|---|---|---|---|---|
| 1 | Ye [27] | Yes | No | No | CC | MCDM |
| 2 | Ye [36] | Yes | No | No | SM | MCDM |
| 3 | Zang and Wu [31] | Yes | No | No | DM | TOPSIS |
| 4 | Biswas et al. [29] | Yes | No | No | SM | TOPSIS |
| 5 | Xu et al. [33] | Yes | No | No | DM | TODIM |
| 6 | Garg [30] | Yes | No | No | Div-M | TOPSIS |
| 7 | Zeng et al. [35] | Yes | No | No | CC | TOPSIS |
| 8 | Xu et al. [21] | Yes | So-called | No | SM | TOPSIS |
| 9 | Borah and Dutta [34] | Yes | No | No | Vector SMs | MCDM |
| 10 | Jana and Pal [32] | Yes | No | No | DPAO | MCDM |
| 11 | Our propose method | Yes | Yes | Yes | Hausdorff distance based SM | The proposed SVN-MCDM |
| Notations | Explanation |
|---|---|
| Hamming distance between SVNSs and | |
| Hausdorff forward direct distance between and | |
| Hausdorff backward direct distance between and | |
| Hausdorff distance between and | |
| Average based Hausdorff forward direct distance between and | |
| Average based Hausdorff backward direct distance between and | |
| Minimum distance between and | |
| Average based Hausdorff distance between and | |
| A monotonically decreasing function | |
| Similarity measure between and | |
| Similarity measure between and based on simple linear function | |
| Similarity measure between and based on rational function | |
| Similarity measure between and based on exponential function | |
| ith alternative | |
| jth criteria/attribute | |
| The evaluation value of ith alternative on the basis of the jth criteria | |
| Single-valued neutrosophic decision matrix (SVNDM) | |
| Ideal alternative (A theoretical standard for comparison) | |
| The maximum value of Truth membership degree for jth criteria among all of the alternatives | |
| The minimum value of indeterminacy degree for jth criteria among all of the alternatives | |
| The minimum value of falsity degree for jth criteria among all of the alternatives | |
| Best alternative/option | |
| Set of nominees | |
| Ideal Nominee (A theoretical standard) | |
| Set of service brands | |
| Ideal service brand (A theoretical standard) |
| Students | Criterion (Y) | |||||
|---|---|---|---|---|---|---|
| (0.93, 0, 0.07) | (0.9, 0.2, 0.3) | (0.88, 0.3, 0.1) | (0.83, 0.2, 0.4) | (0.9, 0.3, 0.1) | (0.87, 0.22, 0.34) | |
| (0.92, 0, 0.08) | (0.8, 0.3, 0.4) | (0.9, 0.3, 0.4) | (0.8, 0.35, 0.2) | (0.84, 0.4, 0.3) | (0.86, 0.3, 0.4) | |
| (0.91, 0, 0.09) | (0.85, 0.4, 0.2) | (0.8, 0.4, 0.2) | (0.82, 0.3, 0.3) | (0.9, 0.3, 0.4) | (0.88, 0.4, 0.28) | |
| SMs | |||
|---|---|---|---|
| 0.933889 | 0.875977 | 0.898796 | |
| 0.903611 | 0.82417 | 0.854633 | |
| 0.909167 | 0.833461 | 0.862637 |
| Services Brands | Criterion (C) | |||||
|---|---|---|---|---|---|---|
| (0.85, 0.24, 0.2) | (0.8, 0.28, 0.2) | (0.84, 0.4, 0.3) | (0.8, 0.2, 0.4) | (0.82, 0.2, 0.4) | (0.8, 0.2, 0.3) | |
| (0.8, 0.3, 0.2) | (0.78, 0.1, 0.3) | (0.82, 0.3, 0.15) | (0.7, 0.3, 0.4) | (0.8, 0.3, 0.1) | (0.82, 0.24, 0.4) | |
| (0.9, 0.1, 0.2) | (0.7, 0.3, 0.4) | (0.8, 0.2, 0.2) | (0.75, 0.2, 0.3) | (0.8, 0.4, 0.2) | (0.78, 0.3, 0.2) | |
| (0.88, 0.2, 0.22) | (0.84, 0.18, 0.28) | (0.9, 0.2, 0.3) | (0.8, 0.3, 0.2) | (0.85, 0.2, 0.2) | (0.9, 0.3, 0.2) | |
| (0.89, 0.1, 0.2) | (0.84, 0.1, 0.2) | (0.9, 0.2, 0.15) | (0.8, 0.2, 0.2) | (0.85, 0.2, 0.1) | (0.9, 0.2, 0.2) | |
| SMs | ||||
|---|---|---|---|---|
| 0.917222 | 0.873889 | 0.896111 | 0.932778 | |
| 0.847101 | 0.776024 | 0.811777 | 0.874024 | |
| 0.874321 | 0.812562 | 0.843899 | 0.897152 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, M.; Hussain, Z.; Yang, M.-S. Hausdorff Distance and Similarity Measures for Single-Valued Neutrosophic Sets with Application in Multi-Criteria Decision Making. Electronics 2023, 12, 201. https://doi.org/10.3390/electronics12010201
Ali M, Hussain Z, Yang M-S. Hausdorff Distance and Similarity Measures for Single-Valued Neutrosophic Sets with Application in Multi-Criteria Decision Making. Electronics. 2023; 12(1):201. https://doi.org/10.3390/electronics12010201
Chicago/Turabian StyleAli, Mehboob, Zahid Hussain, and Miin-Shen Yang. 2023. "Hausdorff Distance and Similarity Measures for Single-Valued Neutrosophic Sets with Application in Multi-Criteria Decision Making" Electronics 12, no. 1: 201. https://doi.org/10.3390/electronics12010201
APA StyleAli, M., Hussain, Z., & Yang, M.-S. (2023). Hausdorff Distance and Similarity Measures for Single-Valued Neutrosophic Sets with Application in Multi-Criteria Decision Making. Electronics, 12(1), 201. https://doi.org/10.3390/electronics12010201












