Management of Multiple-Transmitter Multiple-Receiver Wireless Power Transfer Systems Using Improved Current Distribution Control Strategy

Abstract

For high-power single-transmitter single-receiver wireless power transfer (STSRWPT) systems, the coils suffer from high voltage and current stresses. With increased power requirements, the coils become bottlenecks for power flow. To increase the power level, multiple-transmitter multiple-receiver wireless power transfer (MTMRWPT) systems with parallel circuits are developed that reduce the voltage and current stresses on the coils and improve power-handling capability. Firstly, an improved current distribution (ICD) control strategy is developed to simultaneously achieve high transfer efficiency, balanced current distribution and constant output voltage. Secondly, it is further shown that the ICD control strategy has the advantage that the currents at the transmitter coils are balanced and it reduces the control complexity simultaneously. Thirdly, an asynchronous particle swarm optimization (APSO) algorithm is applied to the ICD control strategy to verify the feasibility of the proposed control strategy. Lastly, a two-transmitter two-receiver wireless power transfer (WPT) system based on the ICD control strategy is proved to obtain an efficiency of more than 89.1% and provides the target output voltage 20 V with balanced current distribution.

1. Introduction

High-power wireless power transfer (WPT) systems are being widely used due to growing demand for fast charging technology and high-power transfer technology. High-power WPT system are applied to electric vehicles (EVs) [1,2,3], electric buses [4,5], trains [6,7] and other transportation vehicles like online electric vehicles (OLEV) [8,9,10]. However, high-power WPT systems suffer from several drawbacks that include: having large voltage and current stresses at the coils, limited magnetic field space due to application-specific coil sizes and shapes. WPT systems with multiple coils are developed for several applications. one such example is having receiver coils under the front and the rear of the car respectively that can sufficiently utilize chassis space [11]. In addition, multiple-transmitter multiple-receiver wireless power transfer (MTMRWPT) systems are applied to roadway powered electric vehicles to provide large lateral tolerance between the rail and pick-up coils [12]. Multiple transmitters based on electromagnetic cavity resonance are applied to simultaneously power multiple small receivers in a three dimensional volume of space [13].

In recent years, many studies have been carried out using various approaches to increase system efficiency. Some research [14,15] has increased system efficiency by introducing new compensation networks. A mixed-resonant coupling model is developed [14,15] to either improve the transfer efficiency or extend the transfer range, which finally achieves 85% efficiency at a distance of 10 cm. Resonators [6,16] are added for a short distance to enhance magnetic field coupling. However, this increases the distance between transmitter coil and receiver coil and causes weak magnetic coupling. Whereas, intermediate coils [17,18] are introduced to optimize the magnetic coupling. However, it is difficult to place intermediate coils between transmitter coil and receiver coil in applications like EVs. Some research [19,20,21] has chosen optimizing the magnetic coupling with high-permeability materials such as ferrites. However, the extra weight and volume make this an inappropriate solution for compact applications. Some research increased efficiency by operating converters at high frequency in the MHz range [22,23]. However, it is not suitable for high-power applications. In WPT systems, maximum efficiency is obtained when load resistances correspond to the impedance-matching condition [24,25]. However, the impedance-matching method is difficult to apply to MTMRWPT systems due to the complex mutual coupling and electric connection between coils. In [12] load resistances are separated at two equal resistances in a two-transmitter two-receiver WPT system to simplify the system, but it is only suitable for two groups of coils of similar parameters. It is a complex exercise to derive the maximum efficiency conditions in conventional MTMRWPT circuit models, there is a need for further developing mathematical models to study the MTMRWPT systems in detail.

Various topologies and control strategies have been developed to improve system efficiency. A two-transmitter and two-receiver circuit is developed with uncontrolled rectifiers at the secondary side [26]. This reduces the complexity of control, but fails to manipulate the equivalent load resistances. A buck circuit [27] is introduced to the secondary side to control the equivalent resistances However, this adds extra segments to the system and increases the overall power losses. WPT system introduces a buck-boost converter [28] to the secondary side to regulate the load resistances. When the system transfers 16 W to the load, the maximum efficiency is 78%. Moreover, a buck circuit is introduced at the primary side to manipulate the input voltage and a boost circuit to the secondary side [29] to manipulate the load. When the system supply 100 W, the obtained maximum system efficiency is 79%. Dual active bridges [30,31] are introduced to WPT system to manipulate the matching resistance so as to achieve high efficiency. The dual active bridges topology has the advantage of small conduction loss and the ability of manipulating the load resistances. Increased efficiency of 85.4% with a 468.6 W output power is obtained in [30], and 80.4% with a 18 W output power is obtained in [31] However, it is a complicated exercise for the MTMRWPT system to simultaneously achieve high efficiency and voltage regulation by removing the mutual coupling between receiver coils and separating the electrical connections at the secondary side.

Some research on single-transmitter multiple-receiver WPT (STMRWPT) systems is presented in [24,32,33,34,35,36]. A STMRWPT system is developed in [32] for portable devices which are of low-complexity receiver design. Resonant coupling systems [33] with multiple receivers are modeled with a simplified circuit model and resonant frequency splitting issue is observed in multiple receivers system. To maximize the efficiency in STMRWPT system, impedance matching theory is introduced to the STMRWPT system [24]. To increase system efficiency, metamaterials [34] are introduced to multiple receiver WPT system, where the efficiency is increased by about 20%. To provide high efficiency and target output power in a two-receiver WPT system, a control strategy based on simplified math model is proposed [35]. To meet the constant source supply, a load independent output voltage method [36] is proposed to satisfy the demands of different load devices. However, STMRWPT systems are mostly applied for portable devices which do not need accurate tuning. This hardly meets the power level demands of each receiver, and the efficiencies of the systems have to be accurately optimized. Besides, single transmitter would suffer from high current stress for high-power applications.

Some research focused on MTMRWPT systems in [12,33,37,38,39]. Cross-coupling between multiple transmitters or receivers is investigated in [37]. It reveals that the effective resonant frequency is changed because of cross-coupling and should be tuned accordingly. Moreover, the splitting frequency issues in multiple-coil WPT systems are observed in [33] and the chirp signals are used to spread and excited inductive multiple-transmitter multiple-receivers WPT system. To meet high-power demand, a new WPT topology based on LCL topology is presented to promote integration of multiple WPT applications [38]. However, it introduces extra intermediate circuits for the WPT system which cause inconvenience in field applications. MTMRWPT systems are developed in roadway-powered electric vehicles to provide higher power handling capacity and a large lateral tolerance [12]. However, the uncontrollable rectifiers are constructed at the secondary side and the receivers can not be controlled by the rectifier. The study in [39] develops a two-transmitter two-receiver WPT system to promote transmitter capacity and verifies that the same-side cross-coupling will lower system efficiency. However, the research only focuses on dual groups of coils with same parameters and multiple receivers with different parameters should be further studied. In addition, the approaches mentioned above have not solved the problems of maximum efficiency and balanced current distribution in multiple transmitters and receivers.

In this paper, an improved current distribution (ICD) control strategy is developed to address the challenges of delivering constant output voltage, improving current distribution, high-efficiency in power transfer through the MTMRWPT system. Besides, a detailed mathematical model of MTMRWPT systems is derived and analyzed, which is applicable for systems with multiple different receiver coils. An active bridge is introduced to the rectifiers to control the receivers. In this study, MTMRWPT systems are proposed to provide a higher power level and maximum utilization of space. The active bridges of transmitters are responsible for voltage regulation and current distribution, and the active bridges of receivers are responsible for resistance matching to provide high efficiency.

The organization of this paper is arranged as follows. Section 2 analyzes the mathematical model of the MTMRWPT system. Section 3 develops the ICD strategy to regulate output voltage, balance current distribution and operate under high efficiency. The asynchronous particle swarm optimization (APSO) method is introduced to the proposed control strategy to verify the proposed control strategy can reduce the control complexity in MTMRWPT systems. Simulation and experiment results are presented in Section 4. Finally, the conclusion is shown in Section 5.

2. Modeling of Multiple-Transmitter Multiple-Receiver Wireless Power Transfer (MTMRWPT) System and Best-Matching Resistances

This section describes a MTMRWPT system involving inductive coupling. For high-power single-transmitter single-receiver wireless power transfer (STSRWPT) systems, the coils suffer from high voltage and high current stresses. To solve the problem of improving the system power level, this research adopts the parallel circuits to improve the power level. Active bridge circuits are adopted to achieve both current distribution and maximum efficiency.

Figure 1a presents the schematic and equivalent circuits of the MTMRWPT system. Each coil group is composed of a transmitter coil and a receiver coil. For coil groups are placed far away from each other, the mutual inductances between each coil group are neglected. R_i1, L_i1, and C_i1 represent the resistance, inductance, and compensation capacitance of the ith transmitter coil respectively; R_i2, L_i2, and C_i2 represent the resistance, inductance, and compensation capacitance of the ith receiver coil respectively. R_o denotes the load resistance, C_o denotes the filter capacitance and U_in denotes the input DC voltage. Q_i1~Q_i8 (i = 1, 2, …, n) are the gate drive signals of the converters of the ith group. Figure 2 shows the square-wave voltages and resonant currents waveforms in the MTMRWPT system. 2β_i1 denote the conduction angle of the transmitter coils of, and ϕ_i1 denotes the phase difference between the fundamental component of the U_i1 square wave and resonant current I_i1. 2β_i2 denote the conduction angle of the receiver coils of the system, and ϕ_i2 denotes the phase difference between the fundamental component of the U_i2 square wave and resonant current I_i2.

Since it is complicated to derive the maximum efficiency by combining all the parameters mentioned above, this research analyzes the MTMRWPT system by splitting the electrical circuit into various coil groups and derives the maximum efficiency of each split group. Figure 1b shows the equivalent circuit of the split group, and Z_eqi denotes the equivalent load resistance of ith group.

In Figure 1b, U_i1 denotes the fundamental component of inverter voltage. By performing the Fourier decomposition, U_i1 is derived as:

$$U_{i1}=\frac{2\sqrt{2}}{\pi }{U}_{\mathrm{in}}\sin {\beta }_{i1}\times e^{j{\varphi }_{i1}}$$

(1)

Using the Kirchhoff voltage laws (KVLs), the equivalent mathematical model of the ith group in the MTMRWPT system can be derived as:

$$\left[\begin{array}{cc}{R}_{i1}+j\omega L_{i1}+\frac{1}{jw{C}_{i1}}& -j\omega M_i\\ -j\omega M_i& R_{i2}+j\omega L_{i2}+\frac{1}{j\omega C_{i2}}+Z_{\mathrm{eq}i}\end{array}\right]\left[\begin{array}{c}{I}_{i1}\\ I_{i2}\end{array}\right]=\left[\begin{array}{c}{U}_{i1}\\ 0\end{array}\right]$$

(2)

Supposing that the filter capacitance is large enough, the output voltage will remain unchanged, once the system achieves its steady state. U_i2 denotes the fundamental component of the inverter voltage in receiver coils is derived as:

$$U_{i2}=\frac{2\sqrt{2}}{\pi }{U}_{\mathrm{o}}\sin {\beta }_{i2}\times e^{j{\varphi }_{i2}}$$

(3)

Solving (1) and (2), the output voltage and currents are derived as follows:

$$U_{\mathrm{o}}=\frac{U_{\mathrm{in}}{\displaystyle \sum _{i=1}^n\frac{\omega M_i\sin {\beta }_{i1}\sin {\beta }_{i2}}{R_{i1}{R}_{i2}+{\omega }^2{M}_i{}^2}}}{\frac{{\pi }^2}{8R_{\mathrm{o}}}+\frac{R_{i1}{\sin }^2{\beta }_{i2}}{R_{i1}{R}_{i2}+{\omega }^2{M}_i{}^2}}$$

(4)

$$I_{i1}=\frac{\left(\pi U_{i1}{R}_{i2}+2\sqrt{2}\omega M_i{U}_{\mathrm{o}}\sin {\beta }_{i2}\right)}{\pi \left(R_{i1}{R}_{i2}+M_i{}^2{\omega }^2\right)}$$

(5)

$$I_{i2}=\frac{\left(M_i\pi U_{i1}\omega -2\sqrt{2}{R}_{i1}{U}_{\mathrm{o}}\sin {\beta }_{i2}\right)}{\pi \left(R_{i1}{R}_{i2}+M_i{}^2{\omega }^2\right)}$$

(6)

The equivalent resistance of the matching resistance segment R_eqi is derived as:

$$R_{\mathrm{eq}i}=\frac{U_{i2}}{I_{i2}}$$

(7)

The input resistance R_ini of ith group is:

$$R_{\mathrm{in}i}=R_{i1}+\frac{\omega M^2}{R_{i2}+R_{\mathrm{eq}i}}$$

(8)

Resistance R_ri (i = 1, 2,…,n) is defined as follows to describe the resistance of receiver coils of the ith group:

$$R_{\mathrm{r}i}=R_{i2}+R_{\mathrm{eq}i}$$

(9)

Supposing there is no efficiency loss in resistance matching segments, the output voltage U_o satisfies:

$${\displaystyle \sum _{i=1}^n{U}_{i2}{I}_{i2}}\cos {\phi }_{i2}=\frac{U_{\mathrm{o}}{}^2}{R_{\mathrm{o}}}$$

(10)

The system efficiency η satisfies:

$$\eta =\frac{U_{\mathrm{o}}{}^2/R_{\mathrm{o}}}{{\displaystyle \sum _{i=1}^n{U}_{i1}{I}_{i1}\cos {\phi }_{i1}}}$$

(11)

To solve the maximum efficiency of the systems, which is the best matching resistance control strategy, the β_i1 and β_i2 have to satisfy:

$$\frac{\partial \eta }{\partial {\beta }_{i1}}=0, \frac{\partial \eta }{\partial {\beta }_{i2}}=0$$

(12)

The solutions of (12) are difficult to derive by analytical calculation, it means that the best matching resistances are difficult to achieve. In this paper, a simplified solution is proposed to satisfy high-efficiency transfer demand.

3. Current Distribution and Improved Current Distribution (ICD) Control Strategy

3.1. Resistance-Matching Rules of ICD Control Strategy

Since, the system efficiency depends on the variables β_i1 and β_i2, it is difficult to directly derive the maximum efficiency by solving all the equations above. In this research, the matching resistance is adopted to achieve the maximum efficiency condition. If each group achieves its maximum efficiency, the maximum efficiency of overall system can be achieved. From [19], the matching resistance in STSRWPT system is obtained as follows:

$$R_{\mathrm{eq}i,BEST}=R_{i2}\sqrt{1+\frac{{\omega }^2{M}_i{}^2}{R_{i1}{R}_{i2}}}$$

(13)

In addition, make $R_{\mathrm{eq}i}=R_{\mathrm{eq}i,BEST}$ in each group, the system will achieve its maximum efficiency.

By solving (13), the β_i2 can be derived as:

$${\beta }_{i2, \mathrm{BEST}}=\arcsin \frac{R_{\mathrm{eq}i, BEST}{M}_i{\displaystyle \prod _{j=1, j\ne i}^n\left(A_j+R_{\mathrm{eq}j,BEST}{R}_{j1}\right)}}{2\sqrt{2R_{\mathrm{o}}}\sqrt{{\displaystyle \sum _{i=1}^n\left(R_{\mathrm{eq}i, BEST}{M}_i{}^2{\displaystyle \prod _{j=1, j\ne i}^n{\left(A_j+R_{\mathrm{eq}j,BEST}{R}_{j1}\right)}^2}\right)}}}$$

(14)

where, $A_j=R_{j1}{R}_{j2}+{\omega }^2{M}_j{}^2$.

Supposing β_i1 are set the same value, the efficiency no longer depends on the variables β_i1 and U_in according to (11) and (13). Therefore, β_i1 and U_in can be tuned to control the output voltage under the constraint condition (4), to assure the constant output voltage. β_i2 are tuned to assure the system operates under high efficiency. For a MTMRWPT system, a control strategy has to be developed to satisfy both minimum current distribution condition and maximum efficiency condition. However, when β_i1 are set the same value, the current distribution in multiple coils are unbalanced, and some coils may suffer from high current stress. To solve this problem, the ICD control strategy trades-off with a little efficiency loss to balance the current distribution in different coils. From detailed simulation studies and experimental results, this proves that the strategy achieves balancing of the current stresses and operation at high efficiency. In this control strategy, the β_i2 are tuned to assure system operate under high efficiency and β_i1 are tuned to control the current distribution and output voltage. Therefore, the system can be simplified to an optimized problem.

3.2. Currents and Voltages Distribution

Under the proposed control condition based on (14), the voltage and the current distribution are analyzed as follows:

Based on the ICD control strategy, the β_i2 at the receiver are set as β_i2best and β_i1 are swept from 0° to 90° to balance the current distribution. The variation of voltage and current at both transmitter and receiver coils are listed as follows:

(a)

The voltages U_i1 at the transmitter coils change rapidly due to the variation of β_i1. It is because the input voltage U_in remains unchanged and the β_i1 sweep from 0° to 90°, and according to (1), the voltages U_i1 at the transmitter coils change rapidly due to the variation of β_i1.

(b)

The voltages U_i2 at the receiver coils generally remain unchanged despite the variation of β_i1. It is because based on the constant output voltage condition the output voltage U_o remains unchanged, and the β_i2 has to remain unchanged based on (14) to provide high efficiency. Therefore, according to (3), the voltages U_i2 at the receiver coils generally remain unchanged.

(c)

The currents I_i1 at the transmitter coils remain unchanged generally despite the variation of β_i1. This is because, based on Figure 1b, the currents I_i2 (i = 1, 2, …, n) follow (15).

$$I_{i2}=\frac{U_{i2}-I_{i2}\left(R_{i2}+j{X}_{i2}\right)}{j\omega M_i}$$

(15)

Under full compensation condition, the jX_i2 = 0 and I_i2R_i2 are comparatively too small compared to U_i2 so that equation (15) can be replaced by (16):

$$I_{i2}\approx \frac{U_{i2}}{j\omega M_i}$$

(16)

U_i2 remain generally unchanged based on previous description (b) and hence I_i2 are unchanged accordingly.

(d)

The currents I_i2 at the receiver coils vary rapidly due to the variation of β_i1. It is because the output voltage U_o and β_i2 remain unchanged, but the equivalent resistances R_eqi of resistance matching segments vary when β_i1 vary. The current I_i2 is sensitive to the variation of equivalent resistances R_eqi, and the I_i2 vary rapidly accordingly.

For high-power level WPT systems, the unbalanced currents increase the current stress of coils. The currents I_i1 at the transmitter coils remain unchanged regardless of the variation of β_i1, the control distribution variables I_i1 and I_i2 reduce to I_i1.

3.3. ICD Control Strategy

For MTMRWPT systems, a control strategy has to be developed to satisfy the minimum current distribution condition and maximum efficiency condition. Such a problem can be simplified to an optimized question.

The target of the ICD control strategy is to assure minimum current distribution, that is

$$f_1={\displaystyle \sum _{i=1}^n\left(I_{i1}{}^2+I_{i2}{}^2\right)}$$

(17)

For the proposed control strategy, the current distribution is generally balanced in transmitter coils of MTMRWPT system. The fitness function can be simplified to (18).

$$f_2={\displaystyle \sum _{i=1}^n{I}_{i2}{}^2}$$

(18)

The constraint condition under such a control strategy is to satisfy the constant output voltage U_o according to (4).

The ICD control strategy can provide constant output voltage and balance the current distribution simultaneously. This is because in an n-transmitter n-receiver WPT system, the dimension of control variables β_i1 and β_i2 is 2n, and the dimension of controlled variables I_i1, I_i2 and U_o is (2n+1) totally. However, based on ICD control strategy, the β_i2 are set as β_{i2, BEST} to provide high efficiency, the control variables β_i1 and β_i2 are reduced to β_i1, the dimension of which is n. Moreover, according to Section 3.2 (c), since I_i1 remain unchanged despite the variation of β_i1 and are previously designed based on (16), the controlled variables I_i1, I_i2 and U_o are reduced to I_i2 and U_o, the dimension of which is (n+1). Furthermore, the current distribution focuses on the balanced current distribution which can be represented as $\frac{I_i}{I_{i+1}}=k_i$, (i = 1, 2, …, n − 1). The dimension of current ratio k_i is (n-1). Therefore, the actual controlled variables I_i1, I_i2 and U_o reduce to k_i and U_o, the dimension of which is n totally. This is equal to the dimension of actual control variables β_i1 which is n totally. Therefore, by controlling β_i1, the ICD control strategy can control output voltage and current distribution simultaneously.

Otherwise, the efficiency of the system is controlled by β_i2, and the output voltages and current distribution are cooperatively controlled by β_i1.

For two transmitters and two receivers WPT systems, current index I₁₂/I₂₂ at the receiver coil changes more rapidly than I₁₁/I₂₁ at the transmitter coil with the change of β_i1. Therefore, system control is simplified to control the current distribution in receivers, that is

$$f\min =I_{12}{}^2+I_{22}{}^2$$

(19)

Therefore, for two-transmitters two-receivers WPT systems, the ICD control strategy should satisfy the following conditions:

(a)

The β_i2 satisfy the optimal matching resistances based on (14);

(b)

The current distribution satisfies $\frac{I_{12}}{I_{22}}=1$;

(c)

The output voltage equals U_o. In this study, the control variables are β₁₂ and β₂₂, the target variables are $\frac{I_{12}}{I_{22}}$ and U_o.

3.4. Asynchronous Particle Swarm Optimization (APSO)

As an evolutionary technique, particle swarm optimization (PSO) was proposed mainly for continuous optimization problems. In this paper, an APSO algorithm [40] is applied to search for a solution to the balanced current distribution problem. β_i1 are the control variables to obtain a feasible solution of current distribution.

To illustrate the application of the APSO algorithm in tracking the optimized current distribution, first a solution vector of x_i with m particles and n dimension is determined as follows:

$$\overrightarrow{x_i}=[{\beta }_{11}, {\beta }_{21}, \dots , {\beta }_{n1}], \left(i=1, 2, \dots , m\right)$$

(20)

In this APSO algorithm, the solution vectors, which are also called particles, are scattered through the multi-dimensional search space. Generally speaking, the current distribution problem can be formulated as (17) or (18).

In multi-dimensional search space, during the (t + 1)^th transfer, the i^th coordinate component of the transfer vector of the t^th particle is manipulated according to the following equations:

$$v_{is}(t+1)=v_{is}(t)+c_1{r}_{1s}(p_{is}(t)-x_{is}(t))+c_2{r}_{2s}(p_{gs}(t)-x_{is}(t))$$

(21)

$$x_{is}(t+1)=x_{is}(t)+v_{is}(t+1)$$

(22)

where, i= 1, …, m, and m is the size of the swarm; d = 1, …, n, and n is the size of the space of a given problem. Constant output voltage is the constriction factor. c₁ and c₂ are positive constants used to control the impact of the local and global component. p_is is the best experience in each generation, and the p_gs is the best particle in the swarm. r_1s and r_2s are random attenuation factors. v_is(t) and x_is(t) are the velocity and position of the i^th particle in the d^th dimension at t^th generation. r_1s and r_2s are both the random numbers between 0 and 1.

The MTMRWPT system is constraint by the constant output voltage according to (4) and basic system constraints according to (23).

$$\{\begin{array}{l}0\le I_{i1}\\ 0\le I_{i2}\\ 0\le {\beta }_{i1}\\ {\beta }_{i1}\le 90°\end{array}$$

(23)

In n-transmitter n-receiver WPT system, there are 2n dimensions to the solution with β_i1 and β_i2 (i = 1, 2, …, n)_. The system should provide balanced current distribution and meanwhile provide constant output voltage. For β_i2 are controlled to a set system manipulating in high-efficiency condition based on (14), the control dimensions are reduced to n dimensions. The output voltage is constraint by the equality constraint condition (4), the exact solution of current distribution is reduced to (n − 1) dimensions.

4. Results

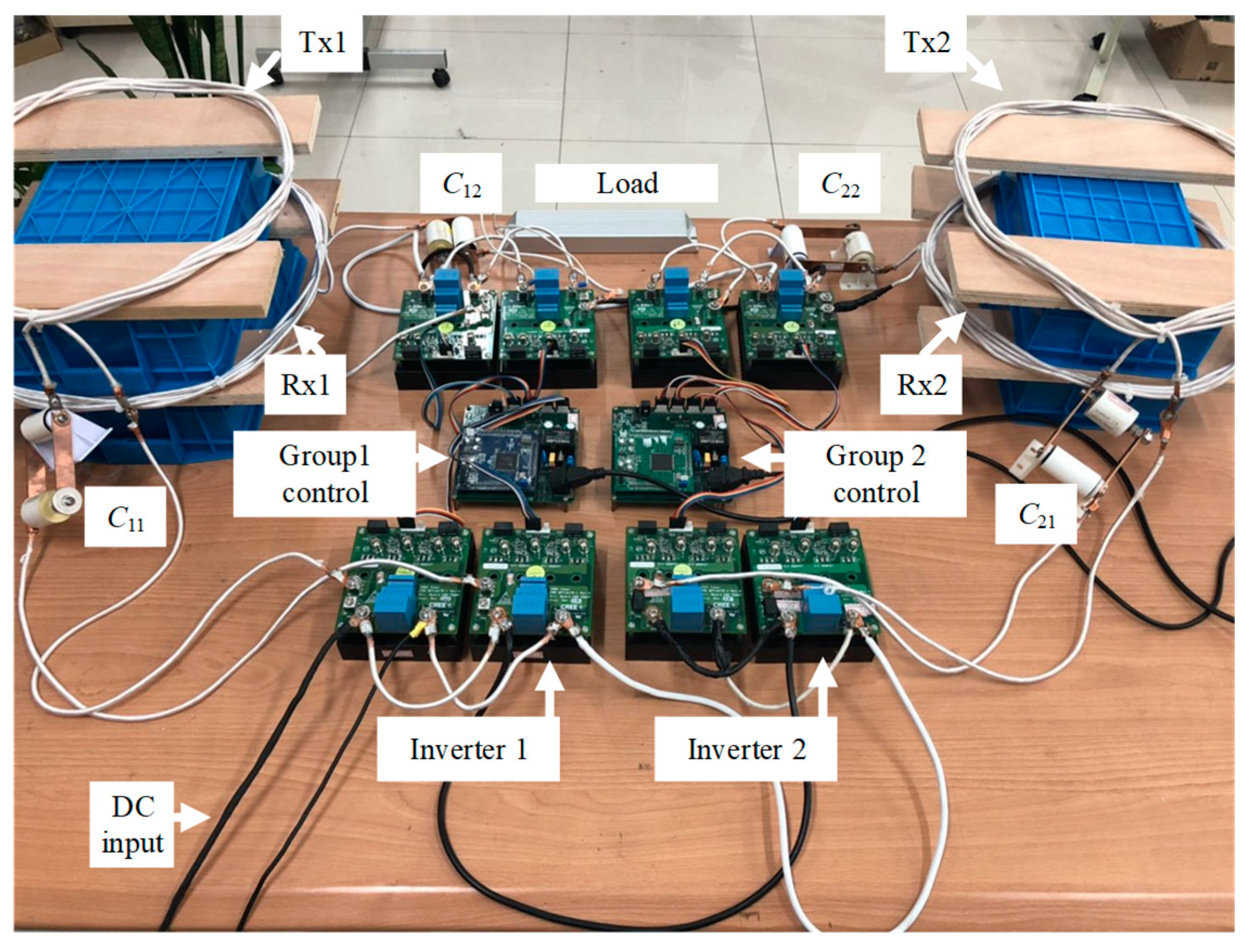
To verify the validity of the proposed MTMRWPT system, the mathematical model is calculated and simulated using MATLAB and MATLAB/Simulink platform. An experimental prototype was implemented as shown in Figure 3.

Table 1. Key parameters of the two-transmitter two-receiver WPT system.

Symbol	Quantity	Value
L11, L12, L21, L22	Inductances of coils	22.78 μH, 22.97 μH, 22.92 μH, 22.30 μH
C11, C12, C21, C22	Compensation capacitor	110 nF, 110 nF, 110 nF, 110 nF
R11, R12, R21, R22	Parasitic resistances	12.25 mΩ, 12.79 mΩ, 11.58 mΩ, 12.01 mΩ
f	Operating frequency	100 kHz
M1,M2	Mutual inductance	5μH, 5μH
φ11, φ12, φ21, φ22	Phase	0, 0, 0, 0
Uin	Input voltage	30 V

presents parameters of a two-transmitter two-receiver WPT system.

In Figure 3, Tx1 and Tx2 denote the transmitter coils, Rx1 and Rx2 denote the receiver coils. C₁₁ and C₂₁ denote the compensation capacitances at the primary side, C₂₁ and C₂₂ denote the compensation capacitances at the secondary side. The active-bridge at each coil consist of two half-bridge circuit modules KIT8020CRD8FF1217P-1. The MOSFET used in half-bridge circuit modules is SCT3030KL, the turn on resistance of which is only 30 mΩ, approximately. Group 1 and group 2 are respectively controlled by a controller consist of a digital signal processor (DSP) TMS320F28335.

Firstly, we compare output voltage and current distribution in different β_i1. In this two-transmitter two-receiver WPT system, R_o is set as 2 Ω, β₂₁ sweeps from 25° to 75° and β₁₁ is manipulated according to the constant output voltage condition (4) to provide constant output voltage. β₁₂ and β₂₂ are set according to (14) to assure system operate at high-efficiency condition. Figure 4 shows the experiment waveform of the transmitters and receivers.

According to the Section 3.3, the ICD control strategy can provide control output voltage U_o and current distribution simultaneously. In Figure 5a, the output voltage is controlled by β₁₁ and β₂₁ based on (4) and remains unchanged in general. It indicates that by controlling β₁₁ and β₂₁, the ICD control strategy can control output voltage U_o. Figure 5b illustrates under the ICD control strategy in the two-transmitter two-receiver WPT system, when the β₂₁ changes and β₁₁ is set according to (4), the voltages U₁₁ and U₂₁ of active bridges in transmitter changes rapidly between 15 V and 27 V, and the variation range of the voltages U₁₂ and U₂₂ of active bridges in receivers is smaller between 17.5 V and 20 V compared to the voltages in transmitters. Figure 5c illustrates under the ICD control strategy in two-transmitter two-receiver WPT system, when the β₂₁ changes and β₁₁ is set according to (4), the currents I₁₂ and I₂₂ of active bridges in receivers changes rapidly between 3 A and 8 A, and the variation range of the currents I₁₁ and I₂₁ of active bridges in transmitters is smaller between 5.5 A and 6 A compared to the currents at receivers. For the ICD control strategy, as analyzed in Section 3.2 (c) and (d), the currents I_i1 at the transmitter coils remain unchanged generally despite the variation of β_i1, and the currents I_i2 at the receiver coils change rapidly due to the variation of β_i1. Therefore, the current distribution in the receivers has to be balanced while the current distribution in transmitter remained unchanged despite the variation of β_i1. The constraint condition (17) is then reduced to (18), which will reduce the complexity of system control.

Figure 5d illustrates efficiencies in two-transmitter two-receiver WPT system. In Figure 5d the efficiency reaches maximum efficiency of 89.2% in general, which indicates that by controlling β₁₂ and β₂₂, efficiencies of system is well optimized, the ICD control strategy provides a solution for MTMRWPT systems to provide constant output voltage, balance the current distribution and support high-efficiency power transfer.

Secondly, this study compares the efficiency of the MTMRWPT system with a STSRWPT system. A STSRWPT system can provide higher efficiency than MTMRWPT system because of fewer components and smaller power loss, However, the coils suffer from higher current and voltage stress and restrict the power level of the WPT system. To compare the efficiency of these two systems, the load resistance of s STSRWPT system is set at 4 Ω, while the load resistance of MTMRWPT system is set at 2 Ω. This ensures the optimal β_i2 of two systems equal 85° and avoids the influence of conduction loss of inverters in receiver coils. The two systems transfer 200 W output power respectively and the input voltage of two systems is set the same value of 30 V. The coils of STSRWPT is composed of coil Tx1 and Rx1.

In experiment, the two systems provide 200 W power respectively, and the maximum efficiency of STSRWPT system is 89.8% which is a little higher than the 89.2% in the MTMRWPT system. The currents in the STSRWPT system are I₁₁ = 7.83 A, I₁₂ = 7.34 A, while the current in MTMRWPT are I₁₁ = 5.84 A, I₁₂ = 5.85 A, I₂₁ = 5.68 A, I₂₂ = 5.29 A when β₁₁ = 40°and β₂₁ = 45°. This means that the MTMRWPT system reduces the current stress in the WPT system; the voltages in the STSRWPT system are U₁₁ = 25.05 V, U₁₂ = 25.61 V, while the voltages in the MTMRWPT are U₁₁ = 19.83 V, U₁₂ = 20.68 V, U₂₁ = 19.10 V, U₂₂ = 19.10 V. It can be inferred that the MTMRWPT system reduces the voltage stress in WPT system and it is valuable in high-power transfer system to balance the current distribution and control the output voltage.

Thirdly, discussing the influence of β₁₁ and β₂₁ to system efficiency and equivalent resistances of receiver coils in two-transmitter two-receiver WPT system. Figure 6 is the calculation results based on the system parameters in Table 1. β₁₂ and β₂₂ are set based on ICD control strategy on (14), β₁₁ and β₂₁ are swept from 0° to 90° to achieve the efficiencies and input resistances and equivalent resistances of system. Figure 6a,b show the input resistances R_ini at the transmitters vs. β₁₁ and β₂₁. Figure 6c,d show that the resistances R_ri at the receiver coils vs. β₁₁ and β₂₁. Figure 6e shows the efficiency vs. β₁₁ and β₂₁.

Figure 6c,d reveals that the resistances R_ri at the receiver coils vary more rapidly than input resistances R_ini at the transmitter coils in Figure 6a,b. In Figure 6a,b, the input resistances R_in1 vary between 0.937 Ω and 5.099 Ω and R_in2 vary between 0.938 Ω and 5.099 Ω when the β₁₁ and β₂₁ vary from 10° to 90°. In Figure 6c,d, the resistances R_r1 vary between 1.855 Ω and 11.988 Ω and R_r2 varies between 1.850 Ω and 11.884 Ω when the β₁₁ and β₂₁ vary from 10° to 90°; it means the currents at the receiver coils vary more rapidly than the ones at the transmitter coils. β₁₂ and β₂₂ are set β_{12, BEST} and β_{22, BEST} at the receiver side, the reflected resistances of two receiver coils are similar and the currents at the transmitter coils are balanced, the fitness function in (17) can be simplified to (18).

In the Figure 6c,d, the equivalent resistances R_ri vary when β₁₁ and β₂₁ vary. it has the advantage that the equivalent resistances R_eqi is under control and can manipulate the equivalent resistances R_eqi of receivers to match system requirement. The resistances R_ri at the receiver coils vary more rapidly than input resistances R_ini at the transmitter coils. However, there is a wide-range stationary interval where R_ri vary slowly when β₁₁ and β₂₁ vary. The equivalent resistance R_r1 varies between 2.823 Ω and 3.726 Ω, and R_r2 varies between 2.815 Ω and 3.712 Ω when the β₁₁ and β₂₁ vary from 60° to 90°. Furthermore, when β₁₁ and β₂₁ get close to the optimal β₁₁ and β₂₁ at which the system achieves optimal efficiency, the efficiency changes slowly. As shown Figure 6e, when the β₁₁ and β₂₁ change between 60° to 90° and the efficiency changes between 92.52% and 92.56%. This means that the ICD control strategy has a wide control range of β₁₁ and β₂₁ at which system can provide high efficiency.

In Figure 6c when β₁₁ is small the equivalent resistance R_r2 increases rapidly. This is because when β₁₁ is small the output voltage U_o becomes the low base on (4), but the current I₂₂ remain unchanged based on (16). In the same way, Figure 6d reveals that when β₂₁ is small the resistance R_r1 increases rapidly. This indicates that the β₁₁ and β₂₁ should not be small to avoid rapid variation of resistances R_ri. The equivalent resistances at the receiver coils is with wider control range and it is easier for the receiver coils to be manipulated to the resistance-matching condition (13).

Fourthly, to verify the feasibility of ICD control strategy, this study compares the ICD control strategy with best resistance matching control strategies at a three-transmitter three-receiver WPT system. The simulations compare their current distribution based on the calculation method, the APSO method with fitness function f₁ and the APSO method with fitness function f₂. The ICD control strategy is based on (14) to derive the solution of β_i2, while the best resistance matching control strategy is based on (12) to derive the numerical solution of β_i2. The calculation method set β₁₁ = β₂₁ = β₃₁ to derive the numerical solutions of β_11, β_21, β_31, while APSO method achieves the solutions of β_11, β_21, β₃₁ with different fitness functions f₁ and f₂. The parameters of the system are presented in

Table 2. Key parameters of the three-transmitter three-receiver WPT system.

Symbol	Quantity	Value
L11, L12, L21, L22, L31, L32,	Inductances of coils	22.78 μH, 22.97 μH, 22.92 μH, 22.30 μH, 22.93 μH, 22.28 μH
C11, C12, C21, C22, C31, C32	Compensation capacitor	110 nF, 110 nF, 110 nF, 110 nF, 110 nF, 110 nF
R11, R12, R21, R22, R31, R32	Parasitic resistances	12.25 mΩ, 12.79 mΩ, 11.58 mΩ, 12.01 mΩ, 12.26 mΩ, 12.78 mΩ
f	Operating frequency	100 kHz
M1,M2, M3	Mutual inductance	5 μH, 5 μH, 5 μH
φ11, φ12, φ21, φ22, φ31, φ32	Phase	0, 0, 0, 0, 0, 0
Uin	Input voltage	30 V

.

The line trace in Figure 7 shows the efficiencies solutions based on best resistance matching control strategy and the solutions based on the ICD control strategy, and the histograms show the current distribution. In Figure 7, the efficiencies of best resistance matching control strategy, which are 92.788%, 92.792%, and 92.790%, respectively, are higher than the efficiencies based on the ICD control strategy which are 92.804%, 92.860%, and 92.858%, respectively. However, the current distribution is not well balanced in the best resistance-matching control strategy. This is because the input resistances are unbalanced in the best resistance-matching control strategy. Focusing on solutions based on the ICD control strategy, the current distribution is decently balanced and the efficiency is still high enough to satisfy system demands. Figure 7 shows that the efficiency based on the APSO method with fitness function f₁ and f₂ are higher than the efficiency based on the calculation method. This is because the calculation method is based on the conditions that satisfy β₁₁ = β₂₁ = β₃₁ while the β₁₁, β₂₁ and β₃₁ in APSO method are optimized for balanced current distribution.

Figure 8 shows the generation simulation results of three-transmitter three-receiver WPT system based on APSO with different fitness functions. The parameters of three-transmitter three-receiver WPT system are listed in Table 2. Figure 8a shows the simulation results of APSO with fitness function f₁ and Figure 8b shows the simulation results of APSO with fitness function f₂. The output probability versus generations based on APSO is calculated on account of (17-18). As shown in Figure 8, it is found that both the output probability based on fitness function f₁ and f₂ decreases quickly and good convergences are achieved within 15 generations. It also reveals that the current distribution in both the APSO based on the ICD control strategy with both fitness function f₁ and f₂ are balanced in Figure 7. This means the fitness function f₂ can replace the fitness function f₁ in practical applications to reduce the control complexity of balancing current distribution.

5. Conclusions

In this article, an ICD control strategy for the MTMRWPT system has been developed to provide high system efficiency, constant output voltage, and balanced current distribution. Firstly, a detailed mathematical model has been derived and analyzed, and a two-transmitter two-receiver WPT system has been implemented to verify the practicability of the ICD control strategy. Secondly, a STSRWPT system and two-transmitter two-receiver WPT system have been compared through experimental results to verify that the proposed system can reduce the voltage and current stresses with small power loss, which improves system power capacity. Thirdly, a three-transmitter three-receiver WPT system has been further simulated to prove that the ICD control strategy can applied to MTMRWPT system. And an APSO methods with different fitness functions has been simulated to verify that the ICD strategy has the advantage that the currents at the transmitter coils are balanced and it reduces the control complexity simultaneously. Lastly, it has achieved an 89.2% efficiency, 20 V target output voltage, 200 W output power and balanced current distribution at 100 kHz in a two-transmitter two-receiver WPT system.