Symmetries and the Hilbert Space of Large N Extended States †
Abstract
:1. Introduction
2. The Large N Hamiltonian
3. Translations: The Large N Soliton
4. Thermofield Double State at Large N
Normal Modes and Bulk Fields
5. 1/N Expansions and Collective Coordinates
5.1. Zero Modes and Large Operators
5.2.
5.3. as a Collective Coordinate
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. TFD State Decomposition
Appendix B. Symmetry Transformations
- M is a two-(functional)-parameter group:so that its inverse is
- . Let , then we have
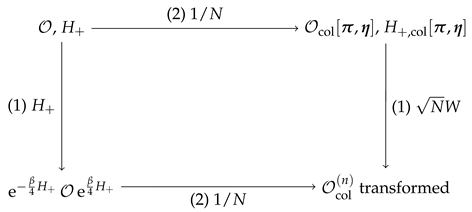
Appendix C. Constraint of Thermofield Double State
| 1 | A bit explanation of the notations: Here, with and . In the context of AdS/CFT, one also denotes and . |
| 2 | Here, inside the traces one has one bi-local field and some c-numbers, and the notation means , and similarly in the following discussions. |
| 3 | Here, to emphasize the Bogoliubov transformation of fields, we put a subscript for the fields. Comparing with the notations in [9], we have , and similarly for other bi-local oscillators. |
References
- Maldacena, J.M. Eternal black holes in anti-de Sitter. J. High Energy Phys. 2003, 2003, 021. [Google Scholar] [CrossRef]
- Leutheusser, S.; Liu, H. Causal connectability between quantum systems and the black hole interior in holographic duality. Phys. Rev. D 2023, 108, 086019. [Google Scholar] [CrossRef]
- Leutheusser, S.A.W. Emergent Times in Holographic Duality. Phys. Rev. D 2023, 108, 086020. [Google Scholar] [CrossRef]
- Witten, E. Gravity and the crossed product. J. High Energy Phys. 2022, 10, 008. [Google Scholar] [CrossRef]
- Chandrasekaran, V.; Penington, G.; Witten, E. Large N algebras and generalized entropy. J. High Energy Phys. 2023, 2023, 9. [Google Scholar] [CrossRef]
- Klebanov, I.R.; Polyakov, A.M. AdS dual of the critical O(N) vector model. Phys. Lett. B 2002, 550, 213–219. [Google Scholar] [CrossRef]
- Giombi, S.; Yin, X. Higher Spin Gauge Theory and Holography: The Three-Point Functions. J. High Energy Phys. 2010, 9, 115. [Google Scholar] [CrossRef]
- Das, S.R.; Jevicki, A. Large N collective fields and holography. Phys. Rev. D 2003, 68, 044011. [Google Scholar] [CrossRef]
- Jevicki, A.; Yoon, J. Bulk from Bi-locals in Thermo Field CFT. J. High Energy Phys. 2016, 02, 090. [Google Scholar] [CrossRef]
- Jevicki, A.; Liu, X.; Yoon, J.; Zheng, J. Dynamical Symmetry and the Thermofield State at Large N. Universe 2022, 8, 114. [Google Scholar] [CrossRef]
- Gervais, J.L.; Jevicki, A.; Sakita, B. Perturbation Expansion Around Extended Particle States in Quantum Field Theory. Phys. Rev. D 1975, 12, 1038. [Google Scholar] [CrossRef]
- de Mello Koch, R.; Kemp, G. Holography of information in AdS/CFT. J. High Energy Phys. 2022, 12, 95. [Google Scholar] [CrossRef]
- de Mello Koch, R. Microscopic entanglement wedges. J. High Energy Phys. 2023, 08, 056. [Google Scholar] [CrossRef]
- de Mello Koch, R. Gravitational dynamics from collective field theory. J. High Energy Phys. 2023, 10, 151. [Google Scholar] [CrossRef]
- Koch, R.D.M.; Jevicki, A.; Liu, X.; Mathaba, K.; Rodrigues, J.A.P. Large N optimization for multi-matrix systems. J. High Energy Phys. 2022, 1, 168. [Google Scholar] [CrossRef]
- Mathaba, K.; Mulokwe, M.; Rodrigues, J.A.P. Large N Master Field Optimization: The Quantum Mechanics of two Yang–Mills coupled Matrices. arXiv 2023, arXiv:2306.00935. [Google Scholar] [CrossRef]
- Vasiliev, M.A. Higher Spin Gauge Theories: Star Product and AdS Space; World Scientific: Singapore, 1999; pp. 533–610. [Google Scholar] [CrossRef]
- Didenko, V.E.; Skvortsov, E.D. Elements of Vasiliev theory. arXiv 2014, arXiv:1401.2975. [Google Scholar]
- de Mello Koch, R.; Jevicki, A.; Rodrigues, J.a.P.; Yoon, J. Canonical Formulation of O(N) Vector/Higher Spin Correspondence. J. Phys. A 2015, 48, 105403. [Google Scholar] [CrossRef]
- Christ, N.H.; Lee, T.D. Quantum Expansion of Soliton Solutions. Phys. Rev. D 1975, 12, 1606. [Google Scholar] [CrossRef]
- Korepin, V.E.; Faddeev, L.D. Quantization of Solitons. Theor. Math. Phys. 1975, 25, 1039–1049. [Google Scholar] [CrossRef]
- Takahasi, Y.; Umezawa, H. Thermo field dynamics. Collect. Phenom. 1975, 2, 55–80. [Google Scholar]
- Shenker, S.H.; Yin, X. Vector Models in the Singlet Sector at Finite Temperature. arXiv 2011, arXiv:1109.3519. [Google Scholar]
- Mathur, S.D. Black holes and holography. J. Phys. Conf. Ser. 2012, 405, 012005. [Google Scholar] [CrossRef]
- Marolf, D.; Wall, A.C. Eternal Black Holes and Superselection in AdS/CFT. Class. Quant. Grav. 2013, 30, 025001. [Google Scholar] [CrossRef]
- Jensen, K.; Sonner, J. Wormholes and entanglement in holography. Int. J. Mod. Phys. D 2014, 23, 1442003. [Google Scholar] [CrossRef]
- Jafferis, D.L.; Kolchmeyer, D.K. Entanglement Entropy in Jackiw-Teitelboim Gravity. arXiv 2019, arXiv:1911.10663. [Google Scholar]
- Penington, G.; Witten, E. Algebras and States in JT Gravity. arXiv 2023, arXiv:2301.07257. [Google Scholar]
- Engelhardt, N.; Folkestad, R. Canonical purification of evaporating black holes. Phys. Rev. D 2022, 105, 086010. [Google Scholar] [CrossRef]
- Dabholkar, A. Quantum Entanglement in String Theory. arXiv 2022, arXiv:2207.03624. [Google Scholar]
- Gomez, C. Cosmology as a Crossed Product. arXiv 2022, arXiv:2207.06704. [Google Scholar]
- Cottrell, W.; Freivogel, B.; Hofman, D.M.; Lokhande, S.F. How to Build the Thermofield Double State. J. High Energy Phys. 2019, 2019, 58. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jevicki, A.; Liu, X.; Zheng, J. Symmetries and the Hilbert Space of Large N Extended States. Universe 2024, 10, 99. https://doi.org/10.3390/universe10020099
Jevicki A, Liu X, Zheng J. Symmetries and the Hilbert Space of Large N Extended States. Universe. 2024; 10(2):99. https://doi.org/10.3390/universe10020099
Chicago/Turabian StyleJevicki, Antal, Xianlong Liu, and Junjie Zheng. 2024. "Symmetries and the Hilbert Space of Large N Extended States" Universe 10, no. 2: 99. https://doi.org/10.3390/universe10020099
APA StyleJevicki, A., Liu, X., & Zheng, J. (2024). Symmetries and the Hilbert Space of Large N Extended States. Universe, 10(2), 99. https://doi.org/10.3390/universe10020099







