Transport Coefficients of Relativistic Matter: A Detailed Formalism with a Gross Knowledge of Their Magnitude
Abstract
1. Introduction
- Natural units:
- Minkowski Metric:
- Partial derivative:
- The symmetric spatial rank-2 projector: , ()
- The symmetric spatial and traceless rank-4 projector:
- The symmetric spatial and traceless projection of an arbitrary tensor :
2. Relatisvistic Fluid Dynamics
- is defined as the energy density,
- is defined as ith component momentum density or the energy current density in ith direction,
- is the ith component momentum current density in jth the direction or jth component momentum current density in ith the direction.
- is defined as the particle density,
- is defined as the particle current.
- The symmetric spatial rank-2 projector ():Definition: .Properties: , , , and . In the LRF, the matrix corresponds to becomes completely spatial:Any 4-vector can be uniquely decomposed into a part parallel to and a part perpendicular to as , where the first part will have only temporal components and the second part have only spatial components in LRF.
- The spatial gradient () and temporal gradient (D):Definition: ,Properties: . In LRF , and . One can decompose in a general frame as: .
- The symmetric spatial and traceless rank-4 projector ():Definition: .Properties: , , , ,The projector projects the symmetric traceless part of a 2-rank tensor onto the direction orthogonal to , i.e., where we define trace of any tensor by the contraction .
- The Landau–Lifshitz frame (LF):
- The Eckart frame (EF):
3. Kinetic Theory
3.1. Boltzmann Equation and Balance Equation
- We will define a function , usually known as summational invariant, with the following condition:
3.2. Entropy Production and Equilibrium
- The local equilibrium distribution () obeys the condition of detailed balance, which is expressed as follows:
- The global equilibrium distribution should satisfy the Boltzmann Equation, and entropy production defined in Equation (49) should vanish.
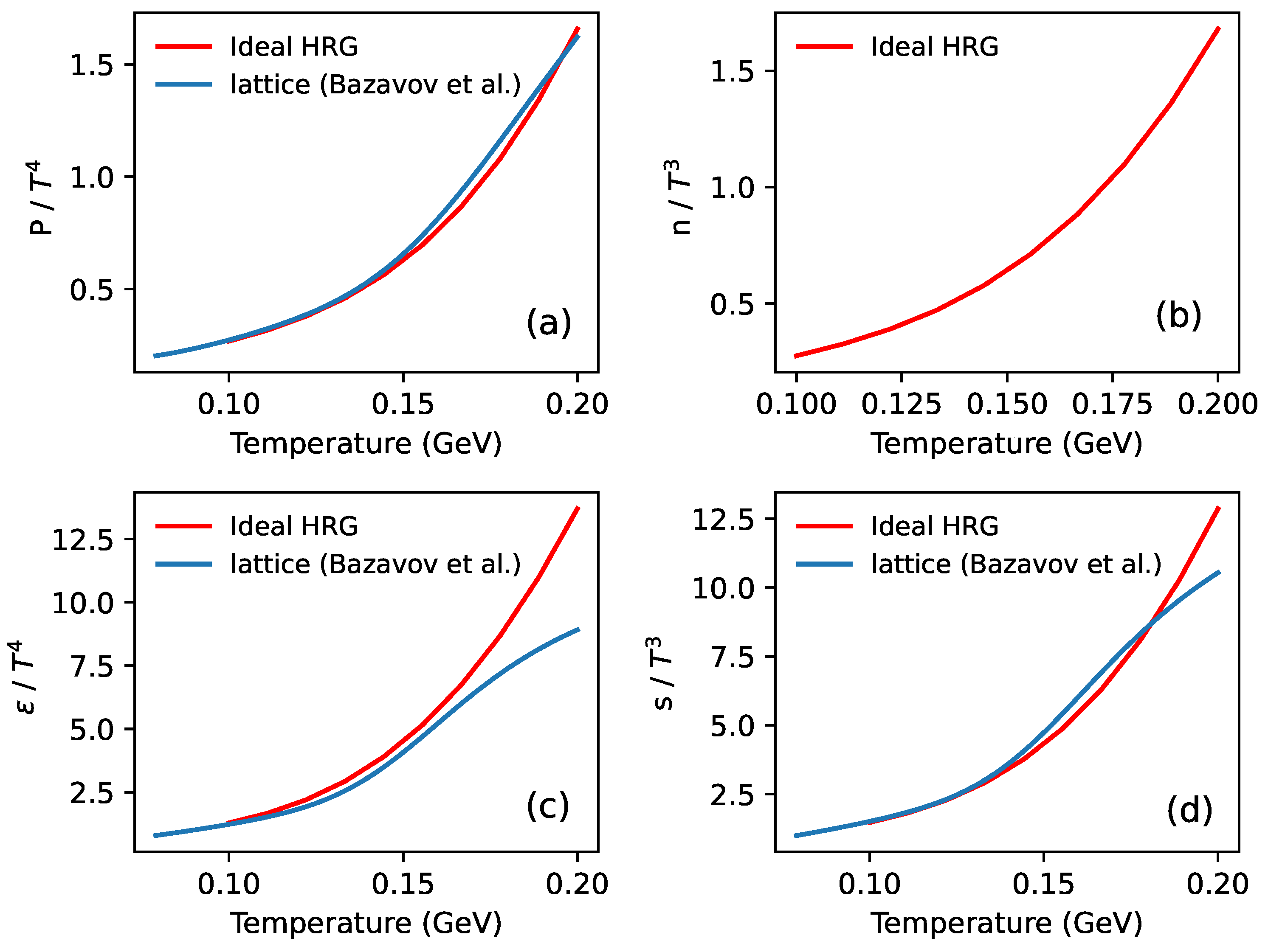
3.3. Local Equilibrium Thermodynamics
4. Transport Coefficients in Chapman–Enskog Approximation
4.1. Transport Coefficients in Chapman–Enskog Approximation for Collision Term
5. Transport Coefficients in Different Models of Relaxation Time Approximations
5.1. Anderson–Witting Model
5.2. Marle’s Model
5.3. BGK Model
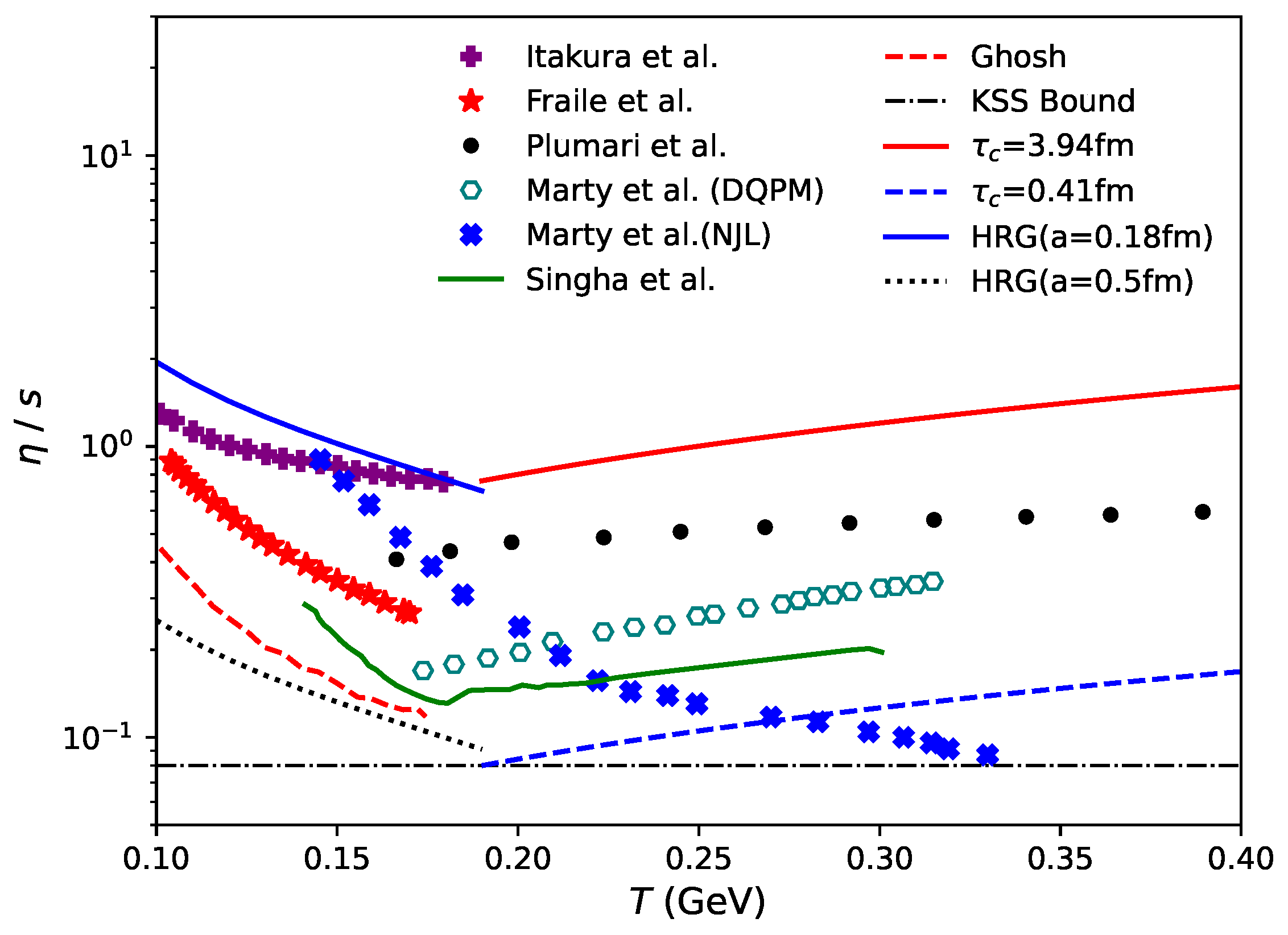
6. Numerical Values of Shear Viscosity to Entropy Density Ratio
- (1).
- Resonance type interaction:
- (2).
- Finite-size effect:
- (3).
- Effect of magnetic field:
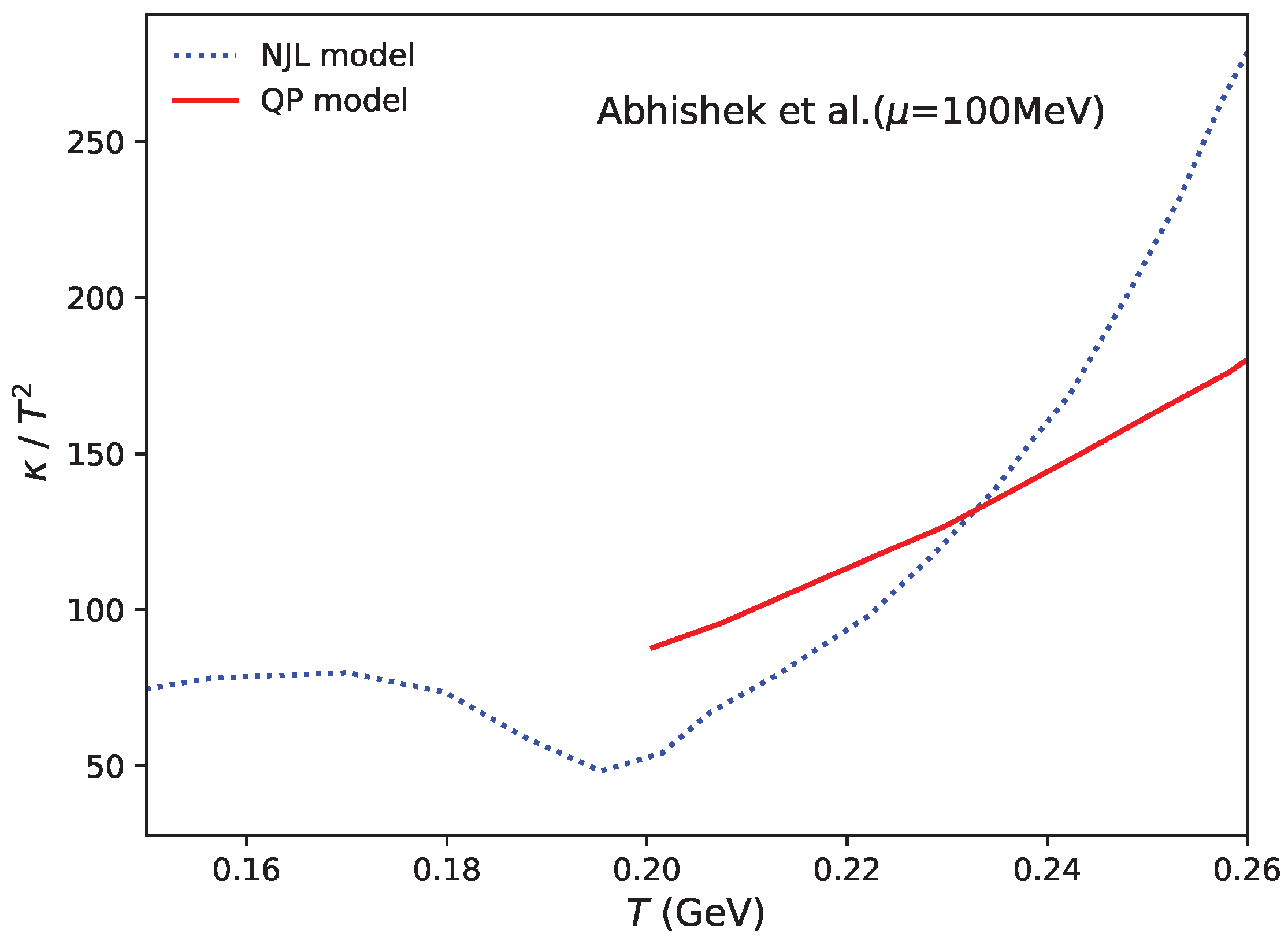
7. Discussions on Bulk Viscosity, Electrical Conductivity, and Thermal Conductivity
8. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | A Fluid can consist of multiple particle species. Even for a single species at sufficiently high energies, one may have the corresponding antispecies viz, for , the pair production can create . The stress–energy tensor and the other charge and particle flows can be described accordingly [92,230,231]. We will ignore such scenarios and stick with a single species fluid throughout this section. |
References
- Itoh, N. Hydrostatic Equilibrium of Hypothetical Quark Stars. Prog. Theor. Phys. 1970, 44, 291. [Google Scholar] [CrossRef]
- Lee, T.D.; Wick, G.C. Vacuum stability and vacuum excitation in a spin-0 field theory. Phys. Rev. D 1974, 9, 2291–2316. [Google Scholar] [CrossRef]
- Collins, J.C.; Perry, M.J. Superdense Matter: Neutrons Or Asymptotically Free Quarks? Phys. Rev. Lett. 1975, 34, 1353. [Google Scholar] [CrossRef]
- Rischke, D.H.; Bernard, S.; Maruhn, J.A. Relativistic hydrodynamics for heavy ion collisions. 1. General aspects and expansion into vacuum. Nucl. Phys. A 1995, 595, 346–382. [Google Scholar] [CrossRef]
- Rischke, D.H.; Pursun, Y.; Maruhn, J.A. Relativistic hydrodynamics for heavy ion collisions. 2. Compression of nuclear matter and the phase transition to the quark—Gluon plasma. Nucl. Phys. A 1995, 595, 383–408, Erratum in Nucl. Phys. A 1996, 596, 717. [Google Scholar] [CrossRef]
- Shuryak, E. Why does the quark gluon plasma at RHIC behave as a nearly ideal fluid? Prog. Part. Nucl. Phys. 2004, 53, 273–303. [Google Scholar] [CrossRef]
- Denicol, G.S.; Rischke, D.H. Relativistic Fluid Dynamics. In Microscopic Foundations of Relativistic Fluid Dynamics; Springer International Publishing: Cham, Switzerland, 2021; pp. 1–28. [Google Scholar] [CrossRef]
- Jameson, A. Aerodynamics. In Encyclopedia of Computational Mechanics; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2004; Chapter 11. [Google Scholar] [CrossRef]
- Mogilner, A.; Manhart, A. Intracellular Fluid Mechanics: Coupling Cytoplasmic Flow with Active Cytoskeletal Gel. Annu. Rev. Fluid Mech. 2018, 50, 347–370. [Google Scholar] [CrossRef]
- Landau, L.; Lifshitz, E. Fluid Mechanics: Volume 6; Number v. 6; Elsevier Science: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Rocha, G.S.; Wagner, D.; Denicol, G.S.; Noronha, J.; Rischke, D.H. Theories of Relativistic Dissipative Fluid Dynamics. arXiv 2023, arXiv:2311.15063. [Google Scholar] [CrossRef]
- Gavassino, L.; Disconzi, M.M.; Noronha, J. Universality Classes of Relativistic Fluid Dynamics I: Foundations. arXiv 2023, arXiv:2302.03478. [Google Scholar]
- Gavassino, L.; Disconzi, M.M.; Noronha, J. Universality Classes of Relativistic Fluid Dynamics II: Applications. arXiv 2023, arXiv:2302.05332. [Google Scholar]
- Noronha, J.; Spaliński, M.; Speranza, E. Transient Relativistic Fluid Dynamics in a General Hydrodynamic Frame. Phys. Rev. Lett. 2022, 128, 252302. [Google Scholar] [CrossRef]
- Hoult, R.E.; Kovtun, P. Causal first-order hydrodynamics from kinetic theory and holography. Phys. Rev. D 2022, 106, 066023. [Google Scholar] [CrossRef]
- Hoult, R.E.; Kovtun, P. Stable and causal relativistic Navier–Stokes equations. J. High Energy Phys. 2020, 6, 067. [Google Scholar] [CrossRef]
- Kovtun, P. First-order relativistic hydrodynamics is stable. J. High Energy Phys. 2019, 10, 034. [Google Scholar] [CrossRef]
- Eckart, C. The Thermodynamics of Irreversible Processes. III. Relativistic Theory of the Simple Fluid. Phys. Rev. 1940, 58, 919–924. [Google Scholar] [CrossRef]
- Cercignani, C.; Kremer, G.M. Thermomechanics of Relativistic Fluids. In The Relativistic Boltzmann Equation: Theory and Applications; Birkhäuser Basel: Basel, Switzerland, 2002; pp. 99–110. [Google Scholar]
- Chapman, S.; Cowling, T. The Mathematical Theory of Non-uniform Gases: An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases; Cambridge Mathematical Library, Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Israel, W. Nonstationary irreversible thermodynamics: A causal relativistic theory. Ann. Phys. 1976, 100, 310–331. [Google Scholar] [CrossRef]
- Stewart, J. On transient relativistic thermodynamics and kinetic theory. Proc. R. Soc. Lond. Math. Phys. Sci. 1977, 357, 59–75. [Google Scholar]
- Transient relativistic thermodynamics and kinetic theory. Ann. Phys. 1979, 118, 341–372. [CrossRef]
- Hiscock, W.A.; Lindblom, L. Generic instabilities in first-order dissipative relativistic fluid theories. Phys. Rev. D 1985, 31, 725–733. [Google Scholar] [CrossRef]
- Hiscock, W.A.; Lindblom, L. Stability and causality in dissipative relativistic fluids. Ann. Phys. 1983, 151, 466–496. [Google Scholar] [CrossRef]
- Hiscock, W.A.; Lindblom, L. Linear plane waves in dissipative relativistic fluids. Phys. Rev. D 1987, 35, 3723–3732. [Google Scholar] [CrossRef]
- Hiscock, W.A.; Lindblom, L. Nonlinear pathologies in relativistic heat-conducting fluid theories. Phys. Lett. A 1988, 131, 509–513. [Google Scholar] [CrossRef]
- Hiscock, W.A.; Olson, T.S. Effects of frame choice on nonlinear dynamics in relativistic heat-conducting fluid theories. Phys. Lett. A 1989, 141, 125–130. [Google Scholar] [CrossRef]
- Bemfica, F.S.; Disconzi, M.M.; Noronha, J. Causality and existence of solutions of relativistic viscous fluid dynamics with gravity. Phys. Rev. D 2018, 98, 104064. [Google Scholar] [CrossRef]
- Disconzi, M.M. On the existence of solutions and causality for relativistic viscous conformal fluids. Commun. Pure Appl. Anal. 2019, 18, 1567–1599. [Google Scholar] [CrossRef]
- Bemfica, F.S.; Bemfica, F.S.; Disconzi, M.M.; Disconzi, M.M.; Noronha, J.; Noronha, J. Nonlinear Causality of General First-Order Relativistic Viscous Hydrodynamics. Phys. Rev. D 2019, 100, 104020, Erratum in Phys. Rev. D 2022, 105, 069902. [Google Scholar] [CrossRef]
- Bemfica, F.S.; Disconzi, M.M.; Noronha, J. First-Order General-Relativistic Viscous Fluid Dynamics. Phys. Rev. X 2022, 12, 021044. [Google Scholar] [CrossRef]
- Das, A.; Florkowski, W.; Ryblewski, R. Correspondence between Israel-Stewart and first-order casual and stable hydrodynamics for the boost-invariant massive case with zero baryon density. Phys. Rev. D 2020, 102, 031501. [Google Scholar] [CrossRef]
- Biswas, R.; Mitra, S.; Roy, V. Is first-order relativistic hydrodynamics in a general frame stable and causal for arbitrary interactions? Phys. Rev. D 2022, 106, L011501. [Google Scholar] [CrossRef]
- Nordheim, L.W. On the Kinetic Method in the New Statistics and Its Application in the Electron Theory of Conductivity. Proc. R. Soc. Lond. Ser. A 1928, 119, 689–698. [Google Scholar] [CrossRef]
- Uehling, E.A.; Uhlenbeck, G.E. Transport Phenomena in Einstein-Bose and Fermi–Dirac Gases. I. Phys. Rev. 1933, 43, 552–561. [Google Scholar] [CrossRef]
- Lichnerowicz, A.; Marrot, R. Propriétés statistiques des ensembles de particules en relativité restreinte. CR Acad. Sci. Paris 1940, 210, 759–761. [Google Scholar]
- Chernikov, N. The generalized stochastic problem of particle motion. Sov. Phys. Dokl. 1957, 2, 103. [Google Scholar]
- Chernikov, N.A. The relativistic collision integral. In Soviet Phys. (Doklady); Joint Institute for Nuclear Research: Dubna, Russia, 1957; Volume 2. [Google Scholar]
- Chernikov, N.A. Flux vector and mass tensor of a relativistic ideal gas. Sov. Phys. Dokl. 1962, 7, 414. [Google Scholar]
- Chernikov, N.A. Kinetic equation for a relativistic gas in an arbitrary gravitational field. Sov. Phys. Dokl. 1962, 7, 397. [Google Scholar]
- Chernikov, N.A. Relativistic Maxwell–Boltzmann distribution and the integral form of the conservation laws. Dokl. Akad. Nauk. Russ. Acad. Sci. 1962, 144, 544–547. [Google Scholar]
- Chernikov, N.A. Derivation of the equations of relativistic hydrodynamics from the relativistic transport equation. Phys. Lett. 1963, 5, 115–117. [Google Scholar] [CrossRef]
- Chernikov, N. Equilibrium distribution of the relativistic gas. Acta Phys. Pol 1964, 26, 1069–1092. [Google Scholar]
- Chernikov, N. The relativistic gas in the gravitational field. Acta Phys. Pol 1963, 23, 629–645. [Google Scholar]
- Chernikow, N. Microscopic foundation of relativistic hydrodynamics. Acta Physiol. Polon 1965, 27. [Google Scholar]
- Tauber, G.E.; Weinberg, J. Internal state of a gravitating gas. Phys. Rev. 1961, 122, 1342. [Google Scholar] [CrossRef]
- Israel, W. Relativistic kinetic theory of a simple gas. J. Math. Phys. 1963, 4, 1163–1181. [Google Scholar] [CrossRef]
- Ehlers, J. General-Relativistc Kinetic Theory Of Gases. In Relativistic Fluid Dynamics; Cattaneo, C., Ed.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 301–388. [Google Scholar] [CrossRef]
- Hilbert, D. Begründung der kinetischen Gastheorie. Math. Ann. 1912, 72, 562–577. [Google Scholar] [CrossRef]
- Chapman, S. VI. On the law of distribution of molecular velocities, and on the theory of viscosity and thermal conduction, in a non-uniform simple monatomic gas. Philos. Trans. R. Soc. Lond. Ser. Contain. Pap. Math. Phys. Character 1916, 216, 279–348. [Google Scholar]
- Enskog, D. Kinetische Theorie der Vorgänge in Mässig Verdünnten Gasen. I. Allgemeiner Teil; Almquist & Wiksells Boktryckeri: Uppsala, Sweden, 1917. [Google Scholar]
- Grad, H. On the kinetic theory of rarefied gases. Commun. Pure Appl. Math. 1949, 2, 331–407. [Google Scholar] [CrossRef]
- Kelly, D.C. The Kinetic Theory of a Relativistic Gas; unpublished report; Miami University: Oxford, OH, USA, 1963. [Google Scholar]
- Marle, C. Sur l’établissement des équations de l’hydrodynamique des fluides relativistes dissipatifs. I.—L’équation de Boltzmann relativiste. Ann. l’institut Henri Poincaré. Sect. A Phys. Théorique 1969, 10, 67–126. [Google Scholar]
- Marle, C. Sur l’établissement des équations de l’hydrodynamique des fluides relativistes dissipatifs. II.—Méthodes de résolution approchée de l’équation de Boltzmann relativiste. Ann. l’institut Henri Poincaré. Sect. A Phys. Théorique 1969, 10, 127–194. [Google Scholar]
- Anderson, J.L. Relativistic Boltzmann Theory and the Grad Method of Moments. In Relativity; Carmeli, M., Fickler, S.I., Witten, L., Eds.; Springer US: Boston, MA, USA, 1970; pp. 109–124. [Google Scholar]
- Stewart, J.M. Non-equilibrium relativistic kinetic theory. In Non-Equilibrium Relativistic Kinetic Theory; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–113. [Google Scholar]
- De Groot, S.; van Weert, C.G.; Hermens, W.T.; Van Leeuwen, W. On relativistic kinetic gas theory: I. The second law for a gas mixture outside equilibrium. Physica 1968, 40, 257–276. [Google Scholar] [CrossRef]
- de Groot, S.; van Weert, C.; Hermens, W.; van Leeuwen, W. On relativistic kinetic gas theory: II. Reciprocal relations between transport phenomena. Physica 1969, 40, 581–593. [Google Scholar] [CrossRef]
- de Groot, S.; van Weert, C.; Hermens, W.; van Leeuwen, W. On relativistic kinetic gas theory. III: The non-relativistic limit and its range of validity. Physica 1969, 42, 309–319. [Google Scholar] [CrossRef]
- van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: IV. The linearized transport equation and its solution for a mixture of relativistic maxwellian molecules. Physica 1971, 51, 1–15. [Google Scholar] [CrossRef]
- van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: V. The coefficients of heat conductivity, diffusion and thermal diffusion for a binary mixture of relativistic Maxwellian molecules. Physica 1971, 51, 16–31. [Google Scholar] [CrossRef]
- van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: VI. The viscosity for a gas of Maxwellian molecules. Physica 1971, 51, 32–49. [Google Scholar] [CrossRef]
- Guichelaar, J.; Van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: VII. The propagation and absorption of sound. Physica 1972, 59, 97–108. [Google Scholar] [CrossRef]
- Hermens, W.; van Leeuwen, W.; van Weert, C.; de Groot, S. On relativistic kinetic gas theory: VIII. Reciprocal relations for a reactive mixture. Physica 1972, 60, 472–487. [Google Scholar] [CrossRef]
- van Leeuwen, W.; Polak, P.; de Groot, S. On relativistic kinetic gas theory: IX. Transport coefficients for systems of particles with arbitrary interaction. Physica 1973, 63, 65–94. [Google Scholar] [CrossRef]
- Polak, P.; van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: X. Transport coefficients in the intermediate relativistic regime. Values for special models. Physica 1973, 66, 455–473. [Google Scholar] [CrossRef]
- van Weert, C.; de Jagher, P.; de Groot, S. On relativistic kinetic gas theory: XI. Volume viscosity in a model consisting of particles with excited states. Physica 1973, 66, 474–483. [Google Scholar] [CrossRef]
- Guichelaar, J.; van Leeuwen, W.; de Groot, S. On relativistic kinetic gas theory: XII. A kinetic description of the dispersion and absorption of sound. Physica 1973, 68, 342–358. [Google Scholar] [CrossRef]
- Guichelaar, J. On relativistic kinetic gas theory: XIII. Sound propagation in a multi-component mixture. Physica 1974, 75, 593–602. [Google Scholar] [CrossRef]
- Van Leeuwen, W.; Kox, A.; de Groot, S. On relativistic kinetic gas theory: XIV. Transport coefficients of a binary mixture. General expressions. Phys. A Stat. Mech. Appl. 1975, 79, 233–255. [Google Scholar] [CrossRef]
- van Leeuwen, W. On relativistic kinetic gas theory: XV. The Ritz method in relativistic Boltzmann theory. Phys. A Stat. Mech. Appl. 1975, 81, 249–275. [Google Scholar] [CrossRef]
- Kox, A.; De Groot, S.; Van Leeuwen, W. On relativistic kinetic gas theory: XVI. The temperature dependence of the transport coefficients for a simple gas of hard spheres. Phys. A Stat. Mech. Appl. 1976, 84, 155–164. [Google Scholar] [CrossRef]
- Kox, A.; Van Leeuwen, W.; De Groot, S. On relativistic kinetic gas theory: XVII. Diffusion and thermal diffusion in a binary mixture of hard spheres. Phys. A Stat. Mech. Appl. 1976, 84, 165–174. [Google Scholar] [CrossRef]
- De Groot, S.; Van Weert, C.; Hermens, W.; van Leeuwen, W. Relativistic entropy law for a gas mixture outside equilibrium. Phys. Lett. A 1968, 26, 439–440. [Google Scholar] [CrossRef]
- Kox, A. On relativistic kinetic gas theory: XVIII. Diffusion in a Lorentz gas of hard spheres. Phys. A Stat. Mech. Appl. 1976, 84, 603–612. [Google Scholar] [CrossRef]
- De Groot, S.; Kox, A.; Van Leeuwen, W. On relativistic kinetic gas theory: XIX. The electron-neutrino system. Phys. A Stat. Mech. Appl. 1976, 84, 613–619. [Google Scholar] [CrossRef]
- De Groot, S.; Van Weert, C.; Hermens, W.; Van Leeuwen, W. Relativistic onsager relations in kinetic gas theory. Phys. Lett. A 1968, 26, 345–346. [Google Scholar] [CrossRef]
- Guichelaar, J.; van Leeuwen, W.; de Groot, S. Sound dispersion and absorption derived from relativistic kinetic theory. Phys. Lett. A 1973, 43, 323–324. [Google Scholar] [CrossRef]
- Kox, A.; van Leeuwen, W.; de Groot, S. Diffusion and thermal diffusion coefficients of a relativistic gas mixture. Phys. Lett. A 1974, 47, 221–223. [Google Scholar] [CrossRef]
- van Leeuwen, W.; Polak, P.; de Groot, S. Transport coefficients calculated by means of a relativistic extension of Enskog’s method. Phys. Lett. A 1971, 37, 323–324. [Google Scholar] [CrossRef]
- de Groot, S.; van Weert, C.; Hermens, W.; van Leeuwen, W. On the role of space and time symmetries in kinetic theory. Physica 1969, 43, 109–131. [Google Scholar] [CrossRef]
- van Weert, C.; van Leeuwen, W.; de Groot, S. Elements of relativistic kinetic theory. Physica 1973, 69, 441–457. [Google Scholar] [CrossRef]
- de Groot, S. Transport coefficients in special relativity. Physica 1973, 69, 12–20. [Google Scholar] [CrossRef]
- Dijkstra, J.; van Leeuwen, W. Mathematical aspects of relativistic kinetic theory. Phys. A Stat. Mech. Appl. 1978, 90, 450–486. [Google Scholar] [CrossRef]
- Van Erkelens, H.; Van Leeuwen, W. Relativistic Boltzmann-theory for a plasma: V. Reduction of the collision integrals. Phys. A Stat. Mech. Appl. 1980, 101, 205–222. [Google Scholar] [CrossRef]
- Bhatnagar, P.L.; Gross, E.P.; Krook, M. A Model for Collision Processes in Gases. I. Small Amplitude Processes in Charged and Neutral One-Component Systems. Phys. Rev. 1954, 94, 511–525. [Google Scholar] [CrossRef]
- Krook, M. Dynamics of Rarefied Gases. Phys. Rev. 1955, 99, 1896–1897. [Google Scholar] [CrossRef]
- Anderson, J.; Witting, H. A relativistic relaxation-time model for the Boltzmann equation. Physica 1974, 74, 466–488. [Google Scholar] [CrossRef]
- Jaiswal, A. Relativistic dissipative hydrodynamics from kinetic theory with relaxation time approximation. Phys. Rev. C 2013, 87, 051901. [Google Scholar] [CrossRef]
- Jaiswal, A. Relativistic third-order dissipative fluid dynamics from kinetic theory. Phys. Rev. C 2013, 88, 021903. [Google Scholar] [CrossRef]
- Jaiswal, A.; Ryblewski, R.; Strickland, M. Transport coefficients for bulk viscous evolution in the relaxation time approximation. Phys. Rev. C 2014, 90, 044908. [Google Scholar] [CrossRef]
- Florkowski, W.; Jaiswal, A.; Maksymiuk, E.; Ryblewski, R.; Strickland, M. Relativistic quantum transport coefficients for second-order viscous hydrodynamics. Phys. Rev. C 2015, 91, 054907. [Google Scholar] [CrossRef]
- Jaiswal, A.; Friman, B.; Redlich, K. Relativistic second-order dissipative hydrodynamics at finite chemical potential. Phys. Lett. B 2015, 751, 548–552. [Google Scholar] [CrossRef]
- Jaiswal, A.; Friman, B.; Redlich, K. Weak and strong coupling limits of the Boltzmann equation in the relaxation-time approximation. arXiv 2016, arXiv:1602.05424. [Google Scholar]
- Bhadury, S.; Kurian, M.; Chandra, V.; Jaiswal, A. Second order relativistic viscous hydrodynamics within an effective description of hot QCD medium. J. Phys. G 2021, 48, 105104. [Google Scholar] [CrossRef]
- Bhadury, S.; Florkowski, W.; Jaiswal, A.; Ryblewski, R. Relaxation time approximation with pair production and annihilation processes. Phys. Rev. C 2020, 102, 064910. [Google Scholar] [CrossRef]
- Florkowski, W.; Ryblewski, R. Separation of elastic and inelastic processes in the relaxation-time approximation for the collision integral. Phys. Rev. C 2016, 93, 064903. [Google Scholar] [CrossRef]
- Sarkar, S.; Adhya, S.P. Dynamically screened strongly quantized electron transport in binary neutron-star merger. Eur. Phys. J. C 2023, 83, 313. [Google Scholar] [CrossRef]
- Sarkar, S.; Sharma, R. The shear viscosity of two-flavor crystalline color superconducting quark matter. Phys. Rev. D 2017, 96, 094025. [Google Scholar] [CrossRef]
- Manuel, C.; Sarkar, S.; Tolos, L. Thermal conductivity due to phonons in the core of superfluid neutron stars. Phys. Rev. C 2014, 90, 055803. [Google Scholar] [CrossRef]
- Basu, S.; Chatterjee, S.; Chatterjee, R.; Nayak, T.K.; Nandi, B.K. Specific Heat of Matter Formed in Relativistic Nuclear Collisions. Phys. Rev. C 2016, 94, 044901. [Google Scholar] [CrossRef]
- Sarwar, G.; Chatterjee, S.; Alam, J.e. An estimate of the bulk viscosity of the hadronic medium. J. Phys. G 2017, 44, 055101. [Google Scholar] [CrossRef]
- Sarkar, S. Viscous Coefficients of a Hot Pion Gas. Adv. High Energy Phys. 2013, 2013, 627137. [Google Scholar] [CrossRef]
- Kalikotay, P.; Chaudhuri, N.; Ghosh, S.; Gangopadhyaya, U.; Sarkar, S. Viscous coefficients and thermal conductivity of a πKN gas mixture in the medium. Eur. Phys. J. A 2020, 56, 79. [Google Scholar] [CrossRef]
- Ghosh, S.; Mitra, S.; Sarkar, S. Medium effects on the electrical conductivity of a hot pion gas. Nucl. Phys. A 2018, 969, 237–253. [Google Scholar] [CrossRef]
- Gangopadhyaya, U.; Ghosh, S.; Sarkar, S.; Mitra, S. In-medium viscous coefficients of a hot hadronic gas mixture. Phys. Rev. C 2016, 94, 044914. [Google Scholar] [CrossRef]
- Mitra, S.; Gangopadhyaya, U.; Sarkar, S. Medium effects on the relaxation of dissipative flows in a hot pion gas. Phys. Rev. D 2015, 91, 094012. [Google Scholar] [CrossRef]
- Mitra, S.; Sarkar, S. Medium effects on the thermal conductivity of a hot pion gas. Phys. Rev. D 2014, 89, 054013. [Google Scholar] [CrossRef]
- Mitra, S.; Chandra, V. Transport coefficients of a hot QCD medium and their relative significance in heavy-ion collisions. Phys. Rev. D 2017, 96, 094003. [Google Scholar] [CrossRef]
- Deng, X.G.; Fang, D.Q.; Ma, Y.G. Shear viscosity of nucleonic matter. Prog. Part. Nucl. Phys. 2023, 104095, in press. [Google Scholar] [CrossRef]
- Rybczyński, M.; Wilk, G.; Włodarczyk, Z. Relaxation and correlation times of nonequilibrium multiparticle systems. Phys. Rev. D 2021, 103, 114026. [Google Scholar] [CrossRef]
- Van Erkelens, H.; Van Leeuwen, W. Relativistic Boltzmann theory for a plasma: I. The entropy production. Phys. A Stat. Mech. Appl. 1977, 89, 113–126. [Google Scholar] [CrossRef]
- van Erkelens, H.; van Leeuwen, W. Relativistic Boltzmann theory for a plasma: II. Reciprocal relations. Phys. A Stat. Mech. Appl. 1977, 89, 225–244. [Google Scholar] [CrossRef]
- van Erkelens, H.; van Leeuwen, W. Relativistic Boltzmann theory for a plasma: III. Viscous phenomena. Phys. A Stat. Mech. Appl. 1978, 90, 97–108. [Google Scholar] [CrossRef]
- van Erkelens, H.; van Leeuwen, W. Relativistic Boltzmann-theory for a plasma: IV. A variational method. Phys. A Stat. Mech. Appl. 1978, 91, 88–98. [Google Scholar] [CrossRef]
- Van Erkelens, H. Relativistic Boltzmann theory for a plasma: VI. The relativistic Landau equation. Phys. A Stat. Mech. Appl. 1981, 107, 48–70. [Google Scholar] [CrossRef]
- van Erkelens, H. Relativistic Boltzmann theory for a plasma: VII. Propagation of hydromagnetic waves. Phys. A Stat. Mech. Appl. 1982, 111, 462–490. [Google Scholar] [CrossRef]
- Van Erkelens, H. Relativistic Boltzmann theory for a plasma: VIII. Energy transport by hydromagnetic waves. Phys. A Stat. Mech. Appl. 1982, 112, 331–342. [Google Scholar] [CrossRef]
- Van Erkelens, H. Relativistic Boltzmann theory for a plasma: IX. Attenuation of hydromagnetic waves. Phys. A Stat. Mech. Appl. 1982, 116, 499–515. [Google Scholar] [CrossRef]
- van Erkelens, H.; van Leeuwen, W. Relativistic Boltzmann theory for a plasma: X. Electrical conduction of the cosmological fluid. Phys. A Stat. Mech. Appl. 1984, 123, 72–98. [Google Scholar] [CrossRef]
- Stewart, E.; Tuchin, K. Continuous evolution of electromagnetic field in heavy-ion collisions. Nucl. Phys. A 2021, 1016, 122308. [Google Scholar] [CrossRef]
- Tuchin, K. Electromagnetic field of ultrarelativistic charge in topologically random nuclear matter. Nucl. Phys. A 2020, 1001, 121907. [Google Scholar] [CrossRef]
- Stewart, E.; Tuchin, K. Magnetic field in expanding quark-gluon plasma. Phys. Rev. C 2018, 97, 044906. [Google Scholar] [CrossRef]
- Peroutka, B.; Tuchin, K. Electromagnetic fields from quantum sources in heavy-ion collisions. Nucl. Phys. A 2017, 967, 860–863. [Google Scholar] [CrossRef]
- Tuchin, K. Initial value problem for magnetic fields in heavy ion collisions. Phys. Rev. C 2016, 93, 014905. [Google Scholar] [CrossRef]
- Tuchin, K. Electromagnetic field and the chiral magnetic effect in the quark-gluon plasma. Phys. Rev. C 2015, 91, 064902. [Google Scholar] [CrossRef]
- Tuchin, K. Electromagnetic fields in high energy heavy-ion collisions. Int. J. Mod. Phys. E 2014, 23, 1430001. [Google Scholar] [CrossRef]
- Tuchin, K. Time and space dependence of the electromagnetic field in relativistic heavy-ion collisions. Phys. Rev. C 2013, 88, 024911. [Google Scholar] [CrossRef]
- Yan, L.; Huang, X.G. Dynamical evolution of a magnetic field in the preequilibrium quark-gluon plasma. Phys. Rev. D 2023, 107, 094028. [Google Scholar] [CrossRef]
- Chen, Y.; Sheng, X.L.; Ma, G.L. Electromagnetic fields from the extended Kharzeev-McLerran-Warringa model in relativistic heavy-ion collisions. Nucl. Phys. A 2021, 1011, 122199. [Google Scholar] [CrossRef]
- Sun, J.A.; Yan, L. Estimate magnetic field strength in heavy-ion collisions via the direct photon elliptic flow. arXiv 2023, arXiv:2311.03929. [Google Scholar]
- Almirante, G.; Astrakhantsev, N.; Braguta, V.; D’Elia, M.; Maio, L.; Naviglio, M.; Sanfilippo, F.; Trunin, A. Electromagnetic conductivity of quark-gluon plasma at finite baryon chemical potential and electromagnetic field. In Proceedings of the 39th International Symposium on Lattice Field Theory, Bonn, Germany, 8–13 August 2022; p. 155. [Google Scholar] [CrossRef]
- Nakamura, K.; Miyoshi, T.; Nonaka, C.; Takahashi, H.R. Charge-dependent anisotropic flow in high-energy heavy-ion collisions from a relativistic resistive magneto-hydrodynamic expansion. Phys. Rev. C 2023, 107, 034912. [Google Scholar] [CrossRef]
- K, G.K.; Kurian, M.; Chandra, V. Thermal and thermoelectric responses of hot QCD medium in time-varying magnetic fields. Phys. Rev. D 2022, 106, 034008. [Google Scholar] [CrossRef]
- Stewart, E. Magnetic Fields in the Quark-Gluon Plasma: Flow Contribution, Initial Conditions, and CP-Odd Domains. Ph.D. Thesis, Iowa State University, Ames, IA, USA, 2022. [Google Scholar]
- Gezhagn, T.; Chaubey, A.K. Electromagnetic Field Evolution in Relativistic Heavy Ion Collision and Its Effect on Flow of Particles. Front. Phys. 2022, 9, 791108. [Google Scholar] [CrossRef]
- Plumari, S.; Coci, G.; Minissale, V.; Das, S.K.; Oliva, L.; Sambataro, M.L.; Greco, V. Transport Properties of Heavy Quarks and Their Correlations to the Bulk Dynamics and the Initial Electromagnetic Field. Springer Proc. Phys. 2020, 250, 109–113. [Google Scholar] [CrossRef]
- Oliva, L.; Plumari, S.; Greco, V. Directed flow of D mesons at RHIC and LHC: Non-perturbative dynamics, longitudinal bulk matter asymmetry and electromagnetic fields. J. High Energy Phys. 2021, 05, 034. [Google Scholar] [CrossRef]
- Dubla, A.; Gürsoy, U.; Snellings, R. Charge-dependent flow as evidence of strong electromagnetic fields in heavy-ion collisions. Mod. Phys. Lett. A 2020, 35, 2050324. [Google Scholar] [CrossRef]
- Oliva, L. Electromagnetic fields and directed flow in large and small colliding systems at ultrarelativistic energies. Eur. Phys. J. A 2020, 56, 255. [Google Scholar] [CrossRef]
- Kord, A.F.; Ghaani, A.; Moghaddam, M.H. Analytical solution of magneto-hydrodynamics with acceleration effects of Bjorken expansion in heavy-ion collisions. Eur. Phys. J. Plus 2022, 137, 53. [Google Scholar] [CrossRef]
- Astrakhantsev, N.; Braguta, V.V.; D’Elia, M.; Kotov, A.Y.; Nikolaev, A.A.; Sanfilippo, F. Lattice study of the electromagnetic conductivity of the quark-gluon plasma in an external magnetic field. Phys. Rev. D 2020, 102, 054516. [Google Scholar] [CrossRef]
- Inghirami, G.; Mace, M.; Hirono, Y.; Del Zanna, L.; Kharzeev, D.E.; Bleicher, M. Magnetic fields in heavy ion collisions: Flow and charge transport. Eur. Phys. J. C 2020, 80, 293. [Google Scholar] [CrossRef]
- Gürsoy, U.; Kharzeev, D.; Marcus, E.; Rajagopal, K.; Shen, C. Charge-dependent Flow Induced by Magnetic and Electric Fields in Heavy Ion Collisions. Phys. Rev. C 2018, 98, 055201. [Google Scholar] [CrossRef]
- Roy, V.; Pu, S.; Rezzolla, L.; Rischke, D.H. Effect of intense magnetic fields on reduced-MHD evolution in √sNN = 200 GeV Au+Au collisions. Phys. Rev. C 2017, 96, 054909. [Google Scholar] [CrossRef]
- Das, S.K.; Plumari, S.; Chatterjee, S.; Alam, J.; Scardina, F.; Greco, V. Directed Flow of Charm Quarks as a Witness of the Initial Strong Magnetic Field in Ultra-Relativistic Heavy Ion Collisions. Phys. Lett. B 2017, 768, 260–264. [Google Scholar] [CrossRef]
- Huang, X.G. Electromagnetic fields and anomalous transports in heavy-ion collisions—A pedagogical review. Rept. Prog. Phys. 2016, 79, 076302. [Google Scholar] [CrossRef]
- Sahoo, B.; Pradhan, K.K.; Sahu, D.; Sahoo, R. Effect of a magnetic field on the thermodynamic properties of a high-temperature hadron resonance gas with van der Waals interactions. Phys. Rev. D 2023, 108, 074028. [Google Scholar] [CrossRef]
- Goswami, K.; Sahu, D.; Dey, J.; Sahoo, R.; Stock, R. Anisotropy of magnetized quark matter. arXiv 2023, arXiv:2310.02711. [Google Scholar]
- Pradhan, K.K.; Sahu, D.; Scaria, R.; Sahoo, R. Conductivity, diffusivity, and violation of the Wiedemann-Franz Law in a hadron resonance gas with van der Waals interactions. Phys. Rev. C 2023, 107, 014910. [Google Scholar] [CrossRef]
- Pradhan, G.S.; Sahu, D.; Deb, S.; Sahoo, R. Hadron gas in the presence of a magnetic field using non-extensive statistics: A transition from diamagnetic to paramagnetic system. J. Phys. G 2023, 50, 055104. [Google Scholar] [CrossRef]
- Singh, K.; Dey, J.; Sahoo, R. Thermal conductivity of evolving quark-gluon plasma in the presence of a time-varying magnetic field. arXiv 2023, arXiv:2307.12568. [Google Scholar] [CrossRef]
- Singh, K.; Dey, J.; Sahoo, R.; Ghosh, S. Effect of time-varying electromagnetic field on Wiedemann-Franz law in a hot hadronic matter. Phys. Rev. D 2023, 108, 094007. [Google Scholar] [CrossRef]
- Satapathy, S.; De, S.; Dey, J.; Ghosh, S. Spatial diffusion of heavy quarks in background magnetic field. arXiv 2022, arXiv:2212.08933. [Google Scholar] [CrossRef]
- Dey, J.; Bandyopadhyay, A.; Gupta, A.; Pujari, N.; Ghosh, S. Electrical conductivity of strongly magnetized dense quark matter - possibility of quantum Hall effect. Nucl. Phys. A 2023, 1034, 122654. [Google Scholar] [CrossRef]
- Dash, A.; Samanta, S.; Dey, J.; Gangopadhyaya, U.; Ghosh, S.; Roy, V. Anisotropic transport properties of a hadron resonance gas in a magnetic field. Phys. Rev. D 2020, 102, 016016. [Google Scholar] [CrossRef]
- Ghosh, S.; Bandyopadhyay, A.; Farias, R.L.S.; Dey, J.; Krein, G.a. Anisotropic electrical conductivity of magnetized hot quark matter. Phys. Rev. D 2020, 102, 114015. [Google Scholar] [CrossRef]
- Bandyopadhyay, A.; Ghosh, S.; Farias, R.L.S.; Ghosh, S. Quantum version of transport coefficients in Nambu–Jona-Lasinio model at finite temperature and strong magnetic field. Eur. Phys. J. C 2023, 83, 489. [Google Scholar] [CrossRef]
- Ghosh, R.; Bandyopadhyay, A.; Nilima, I.; Ghosh, S. Anisotropic tomography of heavy quark dissociation by using the general propagator structure in a finite magnetic field. Phys. Rev. D 2022, 106, 054010. [Google Scholar] [CrossRef]
- Nilima, I.; Bandyopadhyay, A.; Ghosh, R.; Ghosh, S. Heavy quark potential and LQCD based quark condensate at finite magnetic field. Eur. Phys. J. C 2023, 83, 30. [Google Scholar] [CrossRef]
- Satapathy, S.; Ghosh, S.; Ghosh, S. Quantum field theoretical structure of electrical conductivity of cold and dense fermionic matter in the presence of a magnetic field. Phys. Rev. D 2022, 106, 036006. [Google Scholar] [CrossRef]
- Satapathy, S.; Ghosh, S.; Ghosh, S. Kubo estimation of the electrical conductivity for a hot relativistic fluid in the presence of a magnetic field. Phys. Rev. D 2021, 104, 056030. [Google Scholar] [CrossRef]
- Banerjee, D.; Das, P.; Paul, S.; Modak, A.; Budhraja, A.; Ghosh, S.; Prasad, S.K. Effect of magnetic field on jet transport coefficient q^. Pramana 2023, 97, 206. [Google Scholar] [CrossRef]
- Ghosh, S.; Ghosh, S. One-loop Kubo estimations of the shear and bulk viscous coefficients for hot and magnetized Bosonic and Fermionic systems. Phys. Rev. D 2021, 103, 096015. [Google Scholar] [CrossRef]
- Dey, J.; Samanta, S.; Ghosh, S.; Satapathy, S. Quantum expression for the electrical conductivity of massless quark matter and of the hadron resonance gas in the presence of a magnetic field. Phys. Rev. C 2022, 106, 044914. [Google Scholar] [CrossRef]
- Rahaman, M.; Das, S.K.; Alam, J.e.; Ghosh, S. Effect of magnetic screening mass on the diffusion of heavy quarks. Int. J. Mod. Phys. E 2021, 30, 2150093. [Google Scholar] [CrossRef]
- Sebastian, J.; Thakur, L.; Mishra, H.; Haque, N. Heavy quarkonia in QGP medium in an arbitrary magnetic field. Phys. Rev. D 2023, 108, 094001. [Google Scholar] [CrossRef]
- Singh, B.; Kurian, M.; Mazumder, S.; Mishra, H.; Chandra, V.; Das, S.K. Momentum broadening of heavy quark in a magnetized thermal QCD medium. arXiv 2020, arXiv:2004.11092. [Google Scholar]
- Das, A.; Mishra, H.; Mohapatra, R.K. Magneto-Seebeck coefficient and Nernst coefficient of a hot and dense hadron gas. Phys. Rev. D 2020, 102, 014030. [Google Scholar] [CrossRef]
- Singh, B.; Mazumder, S.; Mishra, H. HQ Collisional energy loss in a magnetized medium. J. High Energy Phys. 2020, 05, 068. [Google Scholar] [CrossRef]
- Singh, B.; Mishra, H. Heavy quark transport in a viscous semi QGP. Phys. Rev. D 2020, 101, 054027. [Google Scholar] [CrossRef]
- Das, A.; Mishra, H.; Mohapatra, R.K. Transport coefficients of hot and dense hadron gas in a magnetic field: A relaxation time approach. Phys. Rev. D 2019, 100, 114004. [Google Scholar] [CrossRef]
- Das, A.; Mishra, H.; Mohapatra, R.K. Electrical conductivity and Hall conductivity of a hot and dense quark gluon plasma in a magnetic field: A quasiparticle approach. Phys. Rev. D 2020, 101, 034027. [Google Scholar] [CrossRef]
- Rath, S.; Dash, S. Nonextensive effects on the viscous properties of hot and magnetized QCD matter. arXiv 2023, arXiv:2307.12002. [Google Scholar] [CrossRef]
- Rath, S.; Dash, S. Charge and heat transport coefficients of a hot and dense QCD matter in the presence of a weak magnetic field. PoS 2023, LHCP2022, 252. [Google Scholar] [CrossRef]
- Rath, S.; Dash, S. Impact of nonextensivity on the transport coefficients of a magnetized hot and dense QCD matter. Eur. Phys. J. C 2023, 83, 867. [Google Scholar] [CrossRef]
- Shaikh, A.; Rath, S.; Dash, S.; Panda, B. Flow of charge and heat in thermal QCD within the weak magnetic field limit: A Bhatnagar-Gross-Krook model approach. Phys. Rev. D 2023, 108, 056021. [Google Scholar] [CrossRef]
- Rath, S.; Dash, S. Momentum transport properties of a hot and dense QCD matter in a weak magnetic field. Eur. Phys. J. C 2022, 82, 797. [Google Scholar] [CrossRef]
- Rath, S.; Dash, S. Effects of weak magnetic field and finite chemical potential on the transport of charge and heat in hot QCD matter. Eur. Phys. J. A 2023, 59, 25. [Google Scholar] [CrossRef]
- Rath, S.; Patra, B.K. Effect of magnetic field on the charge and thermal transport properties of hot and dense QCD matter. Eur. Phys. J. C 2020, 80, 747. [Google Scholar] [CrossRef]
- Rath, S.; Patra, B.K. Momentum and its affiliated transport coefficients for hot QCD matter in a strong magnetic field. Phys. Rev. D 2020, 102, 036011. [Google Scholar] [CrossRef]
- Rath, S.; Patra, B.K. Revisit to electrical and thermal conductivities, Lorenz and Knudsen numbers in thermal QCD in a strong magnetic field. Phys. Rev. D 2019, 100, 016009. [Google Scholar] [CrossRef]
- Rath, S.; Krishna Patra, B. Thermomagnetic properties and Bjorken expansion of hot QCD matter in a strong magnetic field. Eur. Phys. J. A 2019, 55, 220. [Google Scholar] [CrossRef]
- Rath, S.; Patra, B.K. One-loop QCD thermodynamics in a strong homogeneous and static magnetic field. J. High Energy Phys. 2017, 12, 098. [Google Scholar] [CrossRef]
- Dey, D.; Patra, B.K. Thermoelectric response of a weakly magnetized thermal QCD medium. Phys. Rev. D 2021, 104. [Google Scholar] [CrossRef]
- Dey, D.; Patra, B.K. Seebeck effect in a thermal QCD medium in the presence of strong magnetic field. Phys. Rev. D 2020, 102, 096011. [Google Scholar] [CrossRef]
- Dash, A.; Shokri, M.; Rezzolla, L.; Rischke, D.H. Charge diffusion in relativistic resistive second-order dissipative magnetohydrodynamics. Phys. Rev. D 2023, 107, 056003. [Google Scholar] [CrossRef]
- Das, A.; Dave, S.S.; Saumia, P.S.; Srivastava, A.M. Effects of magnetic field on plasma evolution in relativistic heavy-ion collisions. Phys. Rev. C 2017, 96, 034902. [Google Scholar] [CrossRef]
- Bagchi, P.; Dutta, N.; Adhya, S.P.; Chatterjee, B. Dissociation of heavy quarkonium states in a rapidly varying strong magnetic field. Mod. Phys. Lett. A 2023, 38, 2350035. [Google Scholar] [CrossRef]
- Bagchi, P.; Dutta, N.; Chatterjee, B.; Adhya, S.P. Evolution of Quarkonia States in a Rapidly Varying Strong Magnetic Field. Springer Proc. Phys. 2021, 261, 555–561. [Google Scholar] [CrossRef]
- Adhya, S.P.; Mandal, M.; Biswas, S.; Roy, P.K. Pionic dispersion relations in the presence of a weak magnetic field. Phys. Rev. D 2016, 93, 074033. [Google Scholar] [CrossRef]
- Dash, A.; Panda, A.K. Charged participants and their electromagnetic fields in an expanding fluid. Phys. Lett. B 2024, 848, 138342. [Google Scholar] [CrossRef]
- Kharzeev, D.E.; McLerran, L.D.; Warringa, H.J. The effects of topological charge change in heavy ion collisions: “Event by event and violation”. Nucl. Phys. A 2008, 803, 227–253. [Google Scholar] [CrossRef]
- Huang, X.G.; Huang, M.; Rischke, D.H.; Sedrakian, A. Anisotropic Hydrodynamics, Bulk Viscosities and R-Modes of Strange Quark Stars with Strong Magnetic Fields. Phys. Rev. D 2010, 81, 045015. [Google Scholar] [CrossRef]
- Huang, X.G.; Sedrakian, A.; Rischke, D.H. Kubo formulae for relativistic fluids in strong magnetic fields. Ann. Phys. 2011, 326, 3075–3094. [Google Scholar] [CrossRef]
- Mohanty, P.; Dash, A.; Roy, V. One particle distribution function and shear viscosity in magnetic field: A relaxation time approach. Eur. Phys. J. A 2019, 55, 35. [Google Scholar] [CrossRef]
- Denicol, G.S.; Huang, X.G.; Molnár, E.; Monteiro, G.M.; Niemi, H.; Noronha, J.; Rischke, D.H.; Wang, Q. Nonresistive dissipative magnetohydrodynamics from the Boltzmann equation in the 14-moment approximation. Phys. Rev. D 2018, 98. [Google Scholar] [CrossRef]
- Denicol, G.S.; Molnár, E.; Niemi, H.; Rischke, D.H. Resistive dissipative magnetohydrodynamics from the Boltzmann-Vlasov equation. Phys. Rev. D 2019, 99, 056017. [Google Scholar] [CrossRef]
- Panda, A.K.; Dash, A.; Biswas, R.; Roy, V. Relativistic non-resistive viscous magnetohydrodynamics from the kinetic theory: A relaxation time approach. J. High Energy Phys. 2021, 03, 216. [Google Scholar] [CrossRef]
- Panda, A.K.; Dash, A.; Biswas, R.; Roy, V. Relativistic resistive dissipative magnetohydrodynamics from the relaxation time approximation. Phys. Rev. D 2021, 104, 054004. [Google Scholar] [CrossRef]
- Dey, J.; Satapathy, S.; Murmu, P.; Ghosh, S. Shear viscosity and electrical conductivity of the relativistic fluid in the presence of a magnetic field: A massless case. Pramana 2021, 95, 125. [Google Scholar] [CrossRef]
- Biswas, R.; Dash, A.; Haque, N.; Pu, S.; Roy, V. Causality and stability in relativistic viscous non-resistive magneto-fluid dynamics. J. High Energy Phys. 2020, 10, 171. [Google Scholar] [CrossRef]
- Gangopadhyaya, U.; Roy, V. Order-by-order anisotropic transport coefficients of a magnetised fluid: A Chapman–Enskog approach. J. High Energy Phys. 2022, 9, 114. [Google Scholar] [CrossRef]
- Hernandez, J.; Kovtun, P. Relativistic magnetohydrodynamics. J. High Energy Phys. 2017, 5, 001. [Google Scholar] [CrossRef]
- Hattori, K.; Hongo, M.; Huang, X.G. New Developments in Relativistic Magnetohydrodynamics. Symmetry 2022, 14, 1851. [Google Scholar] [CrossRef]
- Danielewicz, P.; Gyulassy, M. Dissipative Phenomena in Quark Gluon Plasmas. Phys. Rev. D 1985, 31, 53–62. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, T.; Teaney, D. Nearly Perfect Fluidity: From Cold Atomic Gases to Hot Quark Gluon Plasmas. Rept. Prog. Phys. 2009, 72, 126001. [Google Scholar] [CrossRef]
- Arnold, P.; Moore, G.D.; Yaffe, L.G. Transport coefficients in high temperature gauge theories (I): Leading-log results. J. High Energy Phys. 2000, 2000, 001. [Google Scholar] [CrossRef]
- Marty, R.; Bratkovskaya, E.; Cassing, W.; Aichelin, J.; Berrehrah, H. Transport coefficients from the Nambu–Jona–Lasinio model for SU(3)f. Phys. Rev. C 2013, 88, 045204. [Google Scholar] [CrossRef]
- Sasaki, C.; Redlich, K. Transport coefficients near chiral phase transition. Nucl. Phys. A 2010, 832, 62–75. [Google Scholar] [CrossRef]
- Ghosh, S.; Peixoto, T.C.; Roy, V.; Serna, F.E.; Krein, G.a. Shear and bulk viscosities of quark matter from quark–meson fluctuations in the Nambu–Jona-Lasinio model. Phys. Rev. C 2016, 93, 045205. [Google Scholar] [CrossRef]
- Deb, P.; Kadam, G.P.; Mishra, H. Estimating transport coefficients in hot and dense quark matter. Phys. Rev. D 2016, 94, 094002. [Google Scholar] [CrossRef]
- Chakraborty, P.; Kapusta, J.I. Quasi-Particle Theory of Shear and Bulk Viscosities of Hadronic Matter. Phys. Rev. C 2011, 83, 014906. [Google Scholar] [CrossRef]
- Singha, P.; Abhishek, A.; Kadam, G.; Ghosh, S.; Mishra, H. Calculations of shear, bulk viscosities and electrical conductivity in the Polyakov-quark–meson model. J. Phys. G 2019, 46, 015201. [Google Scholar] [CrossRef]
- Lang, R.; Weise, W. Shear viscosity from Kubo formalism: NJL model study. Eur. Phys. J. A 2014, 50, 63. [Google Scholar] [CrossRef]
- Cassing, W.; Linnyk, O.; Steinert, T.; Ozvenchuk, V. Electrical Conductivity of Hot QCD Matter. Phys. Rev. Lett. 2013, 110, 182301. [Google Scholar] [CrossRef] [PubMed]
- Fernandez-Fraile, D.; Gomez Nicola, A. Transport coefficients and resonances for a meson gas in Chiral Perturbation Theory. Eur. Phys. J. C 2009, 62, 37–54. [Google Scholar] [CrossRef]
- Ghosh, S.; Krein, G.a.; Sarkar, S. Shear viscosity of a pion gas resulting from ρππ and σππ interactions. Phys. Rev. C 2014, 89, 045201. [Google Scholar] [CrossRef]
- Ghosh, S. Nucleon thermal width owing to pion-baryon loops and its contributions to shear viscosity. Phys. Rev. C 2014, 90, 025202. [Google Scholar] [CrossRef]
- Ghosh, S. Shear viscosity of pionic and nucleonic components from their different possible mesonic and baryonic thermal fluctuations. Braz. J. Phys. 2015, 45, 687–698. [Google Scholar] [CrossRef]
- Rahaman, M.; Ghosh, S.; Ghosh, S.; Sarkar, S.; Alam, J.e. Contribution of a kaon component in the viscosity and conductivity of a hadronic medium. Phys. Rev. C 2018, 97, 035201. [Google Scholar] [CrossRef]
- Ghosh, S.; Ghosh, S.; Bhattacharyya, S. Phenomenological bound on the viscosity of the hadron resonance gas. Phys. Rev. C 2018, 98, 045202. [Google Scholar] [CrossRef]
- Ghosh, S.; Samanta, S.; Ghosh, S.; Mishra, H. Viscosity calculations from Hadron Resonance Gas model: Finite size effect. Int. J. Mod. Phys. E 2019, 28, 1950036. [Google Scholar] [CrossRef]
- Noronha-Hostler, J.; Noronha, J.; Greiner, C. Transport Coefficients of Hadronic Matter near T(c). Phys. Rev. Lett. 2009, 103, 172302. [Google Scholar] [CrossRef]
- Kadam, G.P.; Mishra, H. Dissipative properties of hot and dense hadronic matter in an excluded-volume hadron resonance gas model. Phys. Rev. C 2015, 92, 035203. [Google Scholar] [CrossRef]
- Ghosh, S.; Chatterjee, S.; Mohanty, B. Bulk viscosity for pion and nucleon thermal fluctuation in the hadron resonance gas model. Phys. Rev. C 2016, 94, 045208. [Google Scholar] [CrossRef]
- Ghosh, S. Electrical conductivity of hadronic matter from different possible mesonic and baryonic loops. Phys. Rev. D 2017, 95, 036018. [Google Scholar] [CrossRef]
- Fotakis, J.A.; Molnár, E.; Niemi, H.; Greiner, C.; Rischke, D.H. Multicomponent relativistic dissipative fluid dynamics from the Boltzmann equation. Phys. Rev. D 2022, 106, 036009. [Google Scholar] [CrossRef]
- Groot, S.R.; Leeuwen, W.A.; van Weert, C.G.; Weert, C.G. Relativistic Kinetic Theory: Principles and Applications; North Holland: Amsterdam, The Netherlands, 1980. [Google Scholar]
- Andersson, N.; Comer, G.L. Relativistic fluid dynamics: Physics for many different scales. Living Rev. Relativ. 2021, 24, 3. [Google Scholar] [CrossRef]
- Dittman, R.H.; Zemansky, M.W. Heat and Thermodynamics, 7th ed.; McGraw-Hill Higher Education: Cambridge, UK, 1997. [Google Scholar]
- Jaiswal, A.; Roy, V. Relativistic Hydrodynamics in Heavy-Ion Collisions: General Aspects and Recent Developments. Adv. High Energy Phys. 2016, 2016, 9623034. [Google Scholar] [CrossRef]
- Kremer, G. An Introduction to the Boltzmann Equation and Transport Processes in Gases; Interaction of Mechanics and Mathematics, Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Cercignani, C.; Kremer, G. The Relativistic Boltzmann Equation: Theory and Applications; Progress in Mathematical Physics, Springer: Basel, Switzerland, 2012. [Google Scholar]
- De Groot, S.R. Relativistic Kinetic Theory. Principles and Applications; North Holland: Amsterdam, The Netherlands, 1980. [Google Scholar]
- Romatschke, P.; Pratt, S. Extracting the shear viscosity of a high temperature hadron gas. arXiv 2014, arXiv:1409.0010. [Google Scholar]
- Csernai, L.P.; Kapusta, J.I.; McLerran, L.D. On the Strongly-Interacting Low-Viscosity Matter Created in Relativistic Nuclear Collisions. Phys. Rev. Lett. 2006, 97, 152303. [Google Scholar] [CrossRef]
- Kapusta, J.I. Viscous Properties of Strongly Interacting Matter at High Temperature. Landolt-Bornstein 2010, 23, 563–580. [Google Scholar] [CrossRef]
- Adler, S.S.; Afanasiev, S.; Aidala, C.; Ajitanand, N.; Akiba, Y.; Alexander, J.; Amirikas, R.; Aphecetche, L.; Aronson, S.; Averbeck, R.; et al. Elliptic Flow of Identified Hadrons in A u+ A u Collisions at s N N= 200 G e V. Phys. Rev. Lett. 2003, 91, 182301. [Google Scholar] [CrossRef]
- Romatschke, P.; Romatschke, U. Viscosity Information from Relativistic Nuclear Collisions: How Perfect is the Fluid Observed at RHIC? Phys. Rev. Lett. 2007, 99, 172301. [Google Scholar] [CrossRef]
- Rose, J.B.; Torres-Rincon, J.M.; Schäfer, A.; Oliinychenko, D.R.; Petersen, H. Shear viscosity of a hadron gas and influence of resonance lifetimes on relaxation time. Phys. Rev. C 2018, 97, 055204. [Google Scholar] [CrossRef]
- Demir, N.; Bass, S.A. Shear-Viscosity to Entropy-Density Ratio of a Relativistic Hadron Gas. Phys. Rev. Lett. 2009, 102, 172302. [Google Scholar] [CrossRef]
- Meyer, H.B. A Calculation of the shear viscosity in SU(3) gluodynamics. Phys. Rev. D 2007, 76, 101701. [Google Scholar] [CrossRef]
- Quack, E.; Klevansky, S.P. Effective 1/N(c) expansion in the NJL model. Phys. Rev. C 1994, 49, 3283–3288. [Google Scholar] [CrossRef]
- Ghosh, S.; Lahiri, A.; Majumder, S.; Ray, R.; Ghosh, S.K. Shear viscosity due to Landau damping from the quark-pion interaction. Phys. Rev. C 2013, 88, 068201. [Google Scholar] [CrossRef]
- Saha, K.; Ghosh, S.; Upadhaya, S.; Maity, S. Transport coefficients in a finite volume Polyakov–Nambu–Jona-Lasinio model. Phys. Rev. D 2018, 97, 116020. [Google Scholar] [CrossRef]
- Ghosh, S.; Chatterjee, B.; Mohanty, P.; Mukharjee, A.; Mishra, H. Impact of magnetic field on shear viscosity of quark matter in Nambu–Jona-Lasinio model. Phys. Rev. D 2019, 100, 034024. [Google Scholar] [CrossRef]
- Plumari, S.; Puglisi, A.; Scardina, F.; Greco, V. Shear Viscosity of a strongly interacting system: Green-Kubo vs. Chapman–Enskog and Relaxation Time Approximation. Phys. Rev. C 2012, 86, 054902. [Google Scholar] [CrossRef]
- Bazavov, A.; Bhattacharya, T.; DeTar, C.; Ding, H.T.; Gottlieb, S.; Gupta, R.; Hegde, P.; Heller, U.M.; Karsch, F.; Laermann, E.; et al. Equation of state in (2 + 1)-flavor QCD. Phys. Rev. D 2014, 90, 094503. [Google Scholar] [CrossRef]
- Abhishek, A.; Mishra, H.; Ghosh, S. Transport coefficients in the Polyakov quark meson coupling model: A relaxation time approximation. Phys. Rev. D 2018, 97, 014005. [Google Scholar] [CrossRef]
- Bernhard, J.E.; Moreland, J.S.; Bass, S.A. Bayesian estimation of the specific shear and bulk viscosity of quark–gluon plasma. Nature Phys. 2019, 15, 1113–1117. [Google Scholar] [CrossRef]
- Moreland, J.S.; Bernhard, J.E.; Bass, S.A. Estimating nucleon substructure properties in a unified model of p-Pb and Pb-Pb collisions. Nucl. Phys. A 2019, 982, 503–506. [Google Scholar] [CrossRef]
- Eskola, K.J. Nearly perfect quark–gluon fluid. Nat. Phys. 2019, 15, 1111–1112. [Google Scholar] [CrossRef]
- Dobado, A.; Torres-Rincon, J.M. Bulk viscosity and the phase transition of the linear sigma model. Phys. Rev. D 2012, 86, 074021. [Google Scholar] [CrossRef]
- Fernandez-Fraile, D.; Gomez Nicola, A. Bulk viscosity and the conformal anomaly in the pion gas. Phys. Rev. Lett. 2009, 102, 121601. [Google Scholar] [CrossRef]
- Mitra, S.; Sarkar, S. Medium effects on the viscosities of a pion gas. Phys. Rev. D 2013, 87, 094026. [Google Scholar] [CrossRef]
- Lee, C.H.; Zahed, I. Electromagnetic radiation in hot QCD matter: Rates, electric conductivity, flavor susceptibility, and diffusion. Phys. Rev. C 2014, 90, 025204. [Google Scholar] [CrossRef]
- Puglisi, A.; Plumari, S.; Greco, V. Electric conductivity from the solution of the relativistic Boltzmann equation. Phys. Rev. D 2014, 90, 114009. [Google Scholar] [CrossRef]
- Greif, M.; Bouras, I.; Greiner, C.; Xu, Z. Electric conductivity of the quark-gluon plasma investigated using a perturbative QCD based parton cascade. Phys. Rev. D 2014, 90, 094014. [Google Scholar] [CrossRef]
- Amato, A.; Aarts, G.; Allton, C.; Giudice, P.; Hands, S.; Skullerud, J.I. Electrical Conductivity of the Quark-Gluon Plasma Across the Deconfinement Transition. Phys. Rev. Lett. 2013, 111, 172001. [Google Scholar] [CrossRef]
- Abhishek, A.; Das, A.; Kumar, D.; Mishra, H. Thermoelectric transport coefficients of quark matter. Eur. Phys. J. C 2022, 82, 71. [Google Scholar] [CrossRef]
- Dias de Deus, J.; Hirsch, A.S.; Pajares, C.; Scharenberg, R.P.; Srivastava, B.K. Transport coefficient to trace anomaly in the clustering of color sources approach. Phys. Rev. C 2016, 93, 024915. [Google Scholar] [CrossRef]
- Alvarado García, J.R.; Fierro, P.; Téllez, A.F.; Bautista, I. Viscosity of a nonequilibrium hot and dense QCD drop formed at the LHC. Phys. Rev. D 2023, 108, 114002. [Google Scholar] [CrossRef]
- Sahu, D.; Sahoo, R. Thermodynamic and transport properties of matter formed in pp, p-Pb, Xe–Xe and Pb–Pb collisions at the Large Hadron Collider using color string percolation model. J. Phys. G Nucl. Part. Phys. 2021, 48, 125104. [Google Scholar] [CrossRef]
- Kubo, R. Statistical mechanical theory of irreversible processes. 1. General theory and simple applications in magnetic and conduction problems. J. Phys. Soc. Jap. 1957, 12, 570–586. [Google Scholar] [CrossRef]
- Jeon, S. Hydrodynamic transport coefficients in relativistic scalar field theory. Phys. Rev. D 1995, 52, 3591–3642. [Google Scholar] [CrossRef]
- Ghosh, S. A real-time thermal field theoretical analysis of Kubo-type shear viscosity: Numerical understanding with simple examples. Int. J. Mod. Phys. A 2014, 29, 1450054. [Google Scholar] [CrossRef]


| Framework [Reference] | |||
|---|---|---|---|
| HTL [210] | Arnold et al. | - | 1.8 |
| LQCD [245] | Meyer | - | 0.1 |
| NJL [211] | Marty et al. | 1–0.3 | 0.3–0.08 |
| NJL [212] | Sasaki et al. | 1–0.5 | 0.5–0.55 |
| NJL [213] | Ghosh et al. | - | 0.5–0.12 |
| NJL [214] | Deb et al. | 2–0.25 | 0.25–0.5 |
| LSM [215] | Chakraborty and Kapusta | 0.87–0.55 | 0.55–0.62 |
| PQM [216] | Singha et al. | 5–0.5 | 0.3–0.08 |
| URQMD [244] | Demir and Bass | 1 | - |
| SMASH [243] | Rose et al. | 1 | - |
| Unitarization [219] | Fernandez-Fraile and Nicola | 0.8–0.3 | - |
| HFT [220,221,222,223] | Ghosh et al. | 0.4–0.1 | - |
| HFT [106] | Kalikotay et al. | 0.8–0.25 | - |
| HRG [224,225] | Ghosh et al. | 0.13–0.28 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dwibedi, A.; Padhan, N.; Chatterjee, A.; Ghosh, S. Transport Coefficients of Relativistic Matter: A Detailed Formalism with a Gross Knowledge of Their Magnitude. Universe 2024, 10, 132. https://doi.org/10.3390/universe10030132
Dwibedi A, Padhan N, Chatterjee A, Ghosh S. Transport Coefficients of Relativistic Matter: A Detailed Formalism with a Gross Knowledge of Their Magnitude. Universe. 2024; 10(3):132. https://doi.org/10.3390/universe10030132
Chicago/Turabian StyleDwibedi, Ashutosh, Nandita Padhan, Arghya Chatterjee, and Sabyasachi Ghosh. 2024. "Transport Coefficients of Relativistic Matter: A Detailed Formalism with a Gross Knowledge of Their Magnitude" Universe 10, no. 3: 132. https://doi.org/10.3390/universe10030132
APA StyleDwibedi, A., Padhan, N., Chatterjee, A., & Ghosh, S. (2024). Transport Coefficients of Relativistic Matter: A Detailed Formalism with a Gross Knowledge of Their Magnitude. Universe, 10(3), 132. https://doi.org/10.3390/universe10030132






