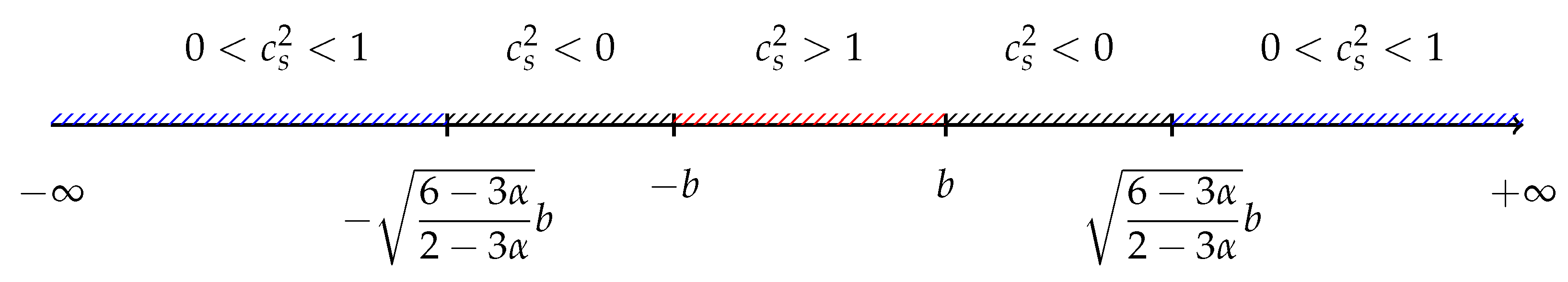Regular Friedmann Universes and Matter Transformations
Abstract
1. Introduction
2. Regular Friedmann Universes and Scalar Fields
3. Regular Friedmann Universes and Tachyons
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Robertson, H.P. Relativistic Cosmology. Rev. Mod. Phys. 1933, 5, 62–90. [Google Scholar] [CrossRef]
- Lifshitz, E.M.; Khalatnikov, I.M. Investigations in relativistic cosmology. Adv. Phys. 1963, 12, 185–249. [Google Scholar] [CrossRef]
- Penrose, R. Structure of Space-Time; W.A. Benjamin: New York, NY, USA; Amsterdam, The Netherlands, 1968. [Google Scholar]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University Press: Cambridge, MA, USA; New York, NY, USA, 1973. [Google Scholar]
- Hawking, S.W.; Penrose, R. The Singularities of gravitational collapse and cosmology. Proc. R. Soc. Lond. Ser. A 1970, 314, 529–548. [Google Scholar]
- Belinsky, V.A.; Khalatnikov, I.M.; Lifshitz, E.M. Oscillatory approach to a singular point in the relativistic cosmology. Adv. Phys. 1970, 19, 525–573. [Google Scholar] [CrossRef]
- Misner, C.W. Mixmaster universe. Phys. Rev. Lett. 1969, 22, 1071–1074. [Google Scholar] [CrossRef]
- Barrow, J.D.; Galloway, G.J.; Tipler, F.J. The closed-universe recollapse conjecture. Mon. Not. R. Astron. Soc. 1986, 223, 835–844. [Google Scholar] [CrossRef]
- Barrow, J.D. Sudden Future Singularities. Class. Quantum Gravity 2004, 21, L79–L82. [Google Scholar] [CrossRef]
- Barrow, J.D. More General Sudden Singularities. Class. Quantum Gravity 2004, 21, 5619–5622. [Google Scholar] [CrossRef]
- Barrow, J.D.; Tsagas, C.G. New Isotropic and Anisotropic Sudden Singularities. Class. Quantum Gravity 2005, 22, 1563–1571. [Google Scholar] [CrossRef]
- Gorini, V.; Kamenshchik, A.Y.; Moschella, U.; Pasquier, V. Tachyons, scalar fields and cosmology. Phys. Rev. D 2004, 69, 123512. [Google Scholar] [CrossRef]
- Shtanov, Y.; Sahni, V. Unusual cosmological singularities in brane world models. Class. Quantum Gravity 2002, 19, L101–L107. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y. Quantum cosmology and late-time singularities. Class. Quantum Gravity 2013, 30, 173001. [Google Scholar] [CrossRef]
- Fernandez-Jambrina, L.; Lazkoz, R. Geodesic behaviour of sudden future singularities. Phys. Rev. D 2004, 70, 121503. [Google Scholar] [CrossRef]
- Keresztes, Z.; Gergely, L.; Kamenshchik, A.Y.; Gorini, V.; Polarski, D. Soft singularity crossing and transformation of matter properties. Phys. Rev. D 2013, 88, 023535. [Google Scholar] [CrossRef]
- Gasperini, M.; Veneziano, G. Pre-big bang in string cosmology. Astropart. Phys. 1993, 1, 317–339. [Google Scholar] [CrossRef]
- Lidsey, J.E.; Wands, D.; Copeland, E.J. Superstring cosmology. Phys. Rep. 2000, 337, 343–492. [Google Scholar] [CrossRef]
- Gasperini, M.; Veneziano, G. The Pre-big bang scenario in string cosmology. Phys. Rep. 2003, 373, 1–212. [Google Scholar] [CrossRef]
- Khoury, J.; Ovrut, B.A.; Steinhardt, P.J.; Turok, N. The Ekpyrotic universe: Colliding branes and the origin of the hot big bang. Phys. Rev. D 2001, 64, 123522. [Google Scholar] [CrossRef]
- Khoury, J.; Ovrut, B.A.; Seiberg, N.; Steinhardt, P.J.; Turok, N. From big crunch to big bang. Phys. Rev. D 2002, 65, 086007. [Google Scholar] [CrossRef]
- Khoury, J.; Steinhardt, P.J.; Turok, N. Designing cyclic universe models. Phys. Rev. Lett. 2004, 92, 031302. [Google Scholar] [CrossRef] [PubMed]
- Kamenshchik, A.Y.; Pozdeeva, E.O.; Vernov, S.Y.; Tronconi, A.; Venturi, G. Transformations between Jordan and Einstein frames: Bounces, antigravity, and crossing singularities. Phys. Rev. D 2016, 94, 063510. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Pozdeeva, E.O.; Vernov, S.Y.; Starobinsky, A.A.; Tronconi, A.; Venturi, G. Induced gravity and minimally and conformally coupled scalar fields in Bianchi-I cosmological models. Phys. Rev. D 2017, 97, 023536. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Pozdeeva, E.O.; Starobinsky, A.A.; Tronconi, A.; Vardanyan, T.; Venturi, G.; Vernov, S.Y. Duality between static spherically or hyperbolically symmetric solutions and cosmological solutions in scalar-tensor gravity. Phys. Rev. D 2018, 98, 124028. [Google Scholar] [CrossRef]
- Bars, I.; Chen, P.J.; Steinhardt, P.J.; Turok, N. Antigravity and the Big Crunch/Big Bang Transition. Phys. Lett. B 2012, 715, 278–281. [Google Scholar] [CrossRef]
- Bars, I.; Steinhardt, P.J.; Turok, N. Sailing through the big crunch-big bang transition. Phys. Rev. D 2014, 89, 061302. [Google Scholar] [CrossRef]
- Wetterich, C. Variable gravity Universe. Phys. Rev. D 2014, 89, 024005. [Google Scholar] [CrossRef]
- Wetterich, C. Eternal Universe. Phys. Rev. D 2014, 90, 043520. [Google Scholar] [CrossRef]
- Creminelli, P.; Nicolis, A.; Trincherini, E. Galilean Genesis: An Alternative to inflation. JCAP 2010, 1011, 021. [Google Scholar] [CrossRef]
- Easson, D.; Sawicki, I.; Vikman, A. G-Bounce. JCAP 2011, 11, 021. [Google Scholar] [CrossRef]
- Volkova, V.A.; Mironov, S.A.; Rubakov, V.A. Cosmological Scenarios with Bounce and Genesis in Horndeski Theory and Beyond. JETP 2019, 129, 553–565. [Google Scholar] [CrossRef]
- Bardeen, J.M. Non-singular general-relativistic gravitational collapse. In Proceedings of the International Conference GR5, Tbilisi, Georgia, 9–16 September 1968; p. 174. [Google Scholar]
- Spallucci, E.; Smailagic, A. Regular black holes from from semi-classical down to Planckian size. Int. J. Mod. Phys. D 2017, 26, 1730013. [Google Scholar] [CrossRef]
- Sebastiani, L.; Zerbini, S. Some remarks on non-singular spherically symmetric space-times. Astronomy 2022, 1, 99–125. [Google Scholar] [CrossRef]
- Simpson, A.; Visser, M. Black bounce to traversable wormhole. JCAP 2019, 2, 042. [Google Scholar] [CrossRef]
- Simpson, A.; Martin-Moruno, P.; Visser, M. Vaidya spacetimes, black-bounces, and traversable wormholes. Class. Quantum Gravity 2019, 36, 145007. [Google Scholar] [CrossRef]
- Franzin, E.; Liberati, S.; Mazza, J.; Simpson, A.; Visser, M. Charged black-bounce spacetimes. JCAP 2021, 7, 036. [Google Scholar] [CrossRef]
- Simpson, A.; Visser, M. The eye of the storm: A regular Kerr black hole. JCAP 2022, 3, 011. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Walia, R.K. Field sources for Simpson-Visser spacetimes. Phys. Rev. D 2022, 105, 044039. [Google Scholar] [CrossRef]
- Synge, J.L. Relativity: The General Theory; North-Holland Company: Amsterdam, The Netherlands, 1960. [Google Scholar]
- Ellis, G.F.R.; Garfinkle, D. The Synge G-Method: Cosmology, wormholes, firewalls, geometry. arXiv 2023, arXiv:2311.06881. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Fabris, J.C. Regular phantom black holes. Phys. Rev. Lett. 2006, 96, 251101. [Google Scholar] [CrossRef] [PubMed]
- Chataignier, L.; Kamenshchik, A.Y.; Tronconi, A.; Venturi, G. Regular black holes, universes without singularities, and phantom-scalar field transitions. Phys. Rev. D 2023, 107, 023508. [Google Scholar] [CrossRef]
- Kamenshchik, A.; Petriakova, P. Newman-Janis algorithm’s application to regular black hole models. Phys. Rev. D 2023, 107, 124020. [Google Scholar] [CrossRef]
- Bronnikov, K.A. Black bounces, wormholes, and partly phantom scalar fields. Phys. Rev. D 2022, 106, 064029. [Google Scholar] [CrossRef]
- Fisher, I.Z. Scalar mesostatic field with regard for gravitational effects. Zh. Eksp. Teor. Fiz. 1948, 18, 636–640. [Google Scholar]
- Bergmann, O.; Leipnik, R. Space-Time Structure of a Static Spherically Symmetric Scalar Field. Phys. Rev. 1957, 107, 1157–1161. [Google Scholar] [CrossRef]
- Buchdahl, H.A. Reciprocal Static Metrics and Scalar Fields in the General Theory of Relativity. Phys. Rev. 1959, 115, 1325–1328. [Google Scholar] [CrossRef]
- Janis, A.I.; Newman, E.T.; Winicour, J. Reality of the Schwarzschild Singularity. Phys. Rev. Lett. 1968, 20, 878–880. [Google Scholar] [CrossRef]
- Janis, A.I.; Robinson, D.C.; Winicour, J. Comments on Einstein scalar solutions. Phys. Rev. 1969, 186, 1729–1731. [Google Scholar] [CrossRef]
- Wyman, M. Static Spherically Symmetric Scalar Fields in General Relativity. Phys. Rev. D 1981, 24, 839–841. [Google Scholar] [CrossRef]
- Agnese, A.G.; La Camera, M. Gravitation without black holes. Phys. Rev. D 1985, 31, 1280–1286. [Google Scholar] [CrossRef]
- Xanthopoulos, B.C.; Zannias, T. Einstein Gravity Coupled to a Massless Scalar Field in Arbitrary Space-time Dimensions. Phys. Rev. D 1989, 40, 2564–2567. [Google Scholar] [CrossRef]
- Gaudin, M.; Gorini, V.; Kamenshchik, A.; Moschella, U.; Pasquier, V. Gravity of a static massless scalar field and a limiting Schwarzschild-like geometry. Int. J. Mod. Phys. D 2006, 15, 1387–1406. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Cannata, F.; Kamenshchik, A.Y. Smooth dynamical crossing of the phantom divide line of a scalar field in simple cosmological models. Phys. Rev. D 2005, 72, 043531. [Google Scholar] [CrossRef]
- Cannata, F.; Kamenshchik, A.Y. Networks of cosmological histories, crossing of the phantom divide line and potentials with cusps. Int. J. Mod. Phys. D 2007, 16, 1683–1704. [Google Scholar] [CrossRef]
- Sen, A. Rolling tachyon. JHEP 2002, 4, 048. [Google Scholar] [CrossRef]
- Padmanabhan, T. Accelerated expansion of the universe driven by tachyonic matter. Phys. Rev. D 2002, 66, 021301. [Google Scholar] [CrossRef]
- Feinstein, A. Power law inflation from the rolling tachyon. Phys. Rev. D 2002, 66, 063511. [Google Scholar] [CrossRef]
- Frolov, A.V.; Kofman, L.; Starobinsky, A.A. Prospects and problems of tachyon matter cosmology. Phys. Lett. B 2002, 545, 8–16. [Google Scholar] [CrossRef]
- Gibbons, G.W. Cosmological evolution of the rolling tachyon. Phys. Lett. B 2002, 537, 1–4. [Google Scholar] [CrossRef]
- Feinberg, G. Possibility of faster-than-light particles. Phys. Rev. 1967, 159, 1089–1105. [Google Scholar] [CrossRef]
- Born, M.; Infeld, L. Foundations of the new field theory. Proc. Roy. Soc. Lond. A 1934, 144, 425–451. [Google Scholar] [CrossRef]
- Ketov, S.V. Many faces of Born-Infeld theory. arXiv 2001, arXiv:hep-th/0108189. [Google Scholar]
- Ketov, S.V. A Manifestly N = 2 supersymmetric Born-Infeld action. Mod. Phys. Lett. A 1999, 14, 501–510. [Google Scholar] [CrossRef]
- Ketov, S.V. N = 1 and N = 2 supersymmetric non-Abelian Born-Infeld actions from superspace. Phys. Lett. B 2000, 491, 207–213. [Google Scholar] [CrossRef]
- Seiberg, N.; Witten, E. String theory and noncommutative geometry. JHEP 1999, 9, 032. [Google Scholar] [CrossRef]
- Jimenez, J.B.; Heisenberg, L.; Olmo, G.J.; Rubiera-Garcia, D. Born-Infeld inspired modifications of gravity. Phys. Rep. 2018, 727, 1–129. [Google Scholar] [CrossRef]
- Parker, L.; Fulling, S.A. Quantized matter fields and the avoidance of singularities in general relativity. Phys. Rev. D 1973, 7, 2357–2374. [Google Scholar] [CrossRef]
- Starobinskii, A.A. On a nonsingular isotropic cosmological model. Sov. Astron. Lett. 1978, 4, 82–84. [Google Scholar]
- Hawking, S.W. Quantum Cosmology. Presented at the Les Houches Summer School on Theoretical Physics: Relativity, Groups and Topology, Les Houches, France, 27 June–4 August 1983; pp. 333–379. [Google Scholar]
- Page, D.N. A Fractal Set of Perpetually Bounces Universes? Class. Quantum Gravity 1984, 1, 417–427. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Grishchuk, L.P.; Zeldovich, Y.B.; Khalatnikov, I.M. Inflationary stages in cosmological models with a scalar field. Sov. Phys. JETP 1985, 62, 195–203. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Khalatnikov, I.M. On the generality of inflationary solutions in cosmological models with a scalar field. Sov. Phys. JETP 1987, 66, 441–449. [Google Scholar]
- Belinsky, V.A.; Ishihara, H.; Khalatnikov, I.M.; Sato, H. On the degree of generality of inflation in Friedmann cosmological models with a massive scalar field. Prog. Theor. Phys. 1988, 79, 676–684. [Google Scholar] [CrossRef][Green Version]
- Kamenshchik, A.Y.; Khalatnikov, I.M.; Toporensky, A.V. Simplest cosmological model with the scalar field. Int. J. Mod. Phys. D 1997, 6, 673–692. [Google Scholar] [CrossRef]
- Vikman, A. Can dark energy evolve to the phantom? Phys. Rev. D 2005, 71, 023515. [Google Scholar] [CrossRef]
- Yurov, A. Phantom scalar fields result in inflation rather than Big Rip. arXiv 2003, arXiv:astro-ph/0305019. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Moschella, U.; Pasquier, V. An alternative to quintessence. Phys. Lett. B 2001, 511, 265–268. [Google Scholar] [CrossRef]
- Fabris, J.C.; Goncalves, S.V.B.; de Souza, P.E. Density perturbations in an Universe dominated by the Chaplygin gas. Gen. Relativ. Gravit. 2002, 34, 53–63. [Google Scholar] [CrossRef]
- Bilic, N.; Tupper, G.B.; Viollier, R.D. Unification of Dark Matter and Dark Energy: The Inhomogeneous Chaplygin Gas. Phys. Lett. B 2002, 535, 17–21. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Generalized Chaplygin gas, accelerated expansion and dark energy matter unification. Phys. Rev. D 2002, 66, 043507. [Google Scholar] [CrossRef]
- Carter, B. Duality Relation Between Charged Elastic Strings And Superconducting Cosmic Strings. Phys. Lett. B 1989, 224, 61–66. [Google Scholar] [CrossRef]
- Vilenkin, A. Effect of Small Scale Structure on the Dynamics of Cosmic Strings. Phys. Rev. D 1990, 41, 3038–3040. [Google Scholar] [CrossRef]
- Misner, C.W. Absolute zero of time. Phys. Rev. 1969, 186, 1328–1333. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kamenshchik, A.; Petriakova, P. Regular Friedmann Universes and Matter Transformations. Universe 2024, 10, 137. https://doi.org/10.3390/universe10030137
Kamenshchik A, Petriakova P. Regular Friedmann Universes and Matter Transformations. Universe. 2024; 10(3):137. https://doi.org/10.3390/universe10030137
Chicago/Turabian StyleKamenshchik, Alexander, and Polina Petriakova. 2024. "Regular Friedmann Universes and Matter Transformations" Universe 10, no. 3: 137. https://doi.org/10.3390/universe10030137
APA StyleKamenshchik, A., & Petriakova, P. (2024). Regular Friedmann Universes and Matter Transformations. Universe, 10(3), 137. https://doi.org/10.3390/universe10030137