Improved Statistical Analysis for the Neutrinoless Double-Beta Decay Matrix Element of 136Xe
Abstract
:1. Introduction
2. The Statistical Model
3. Results of the Statistical Analysis
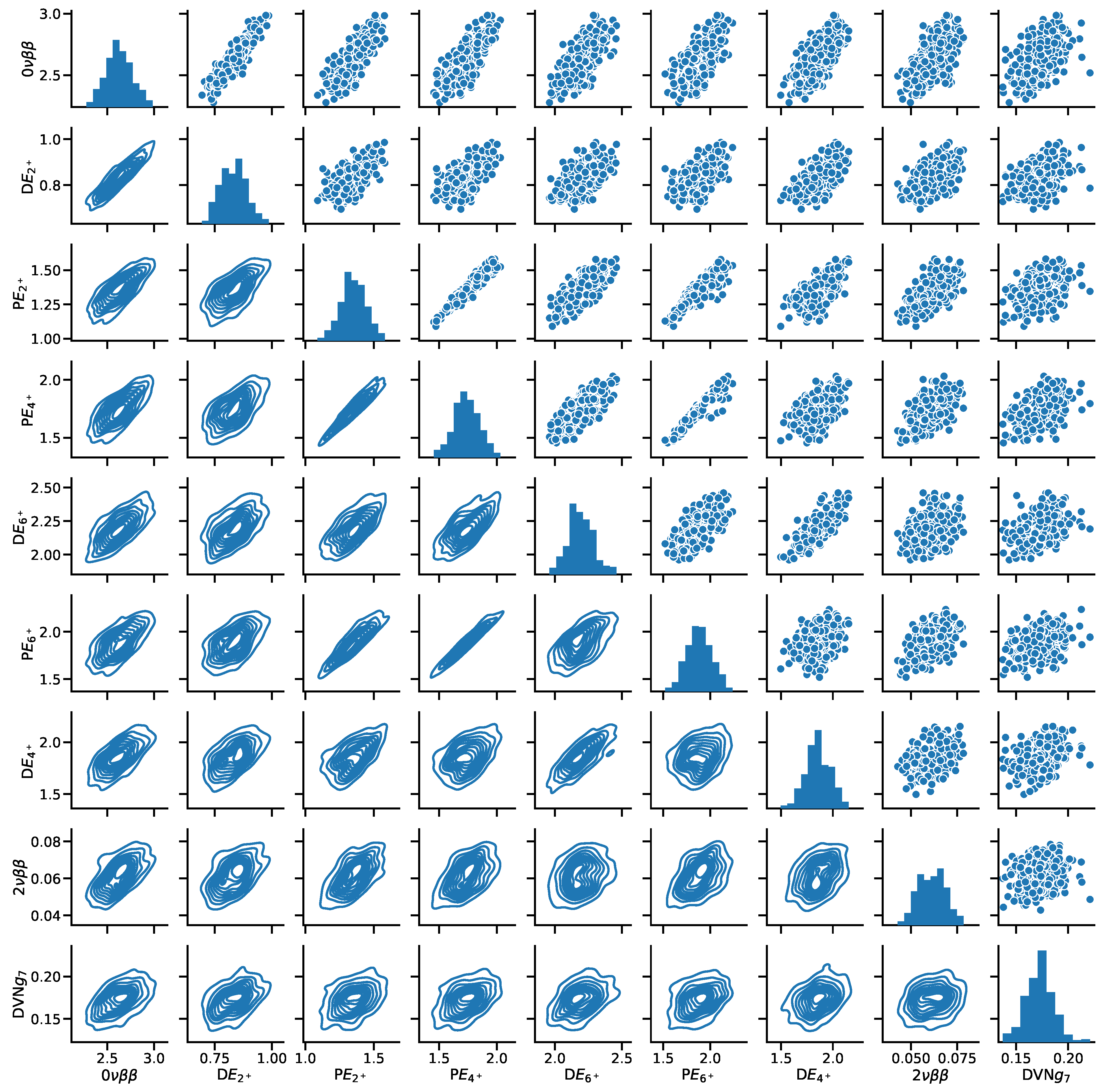
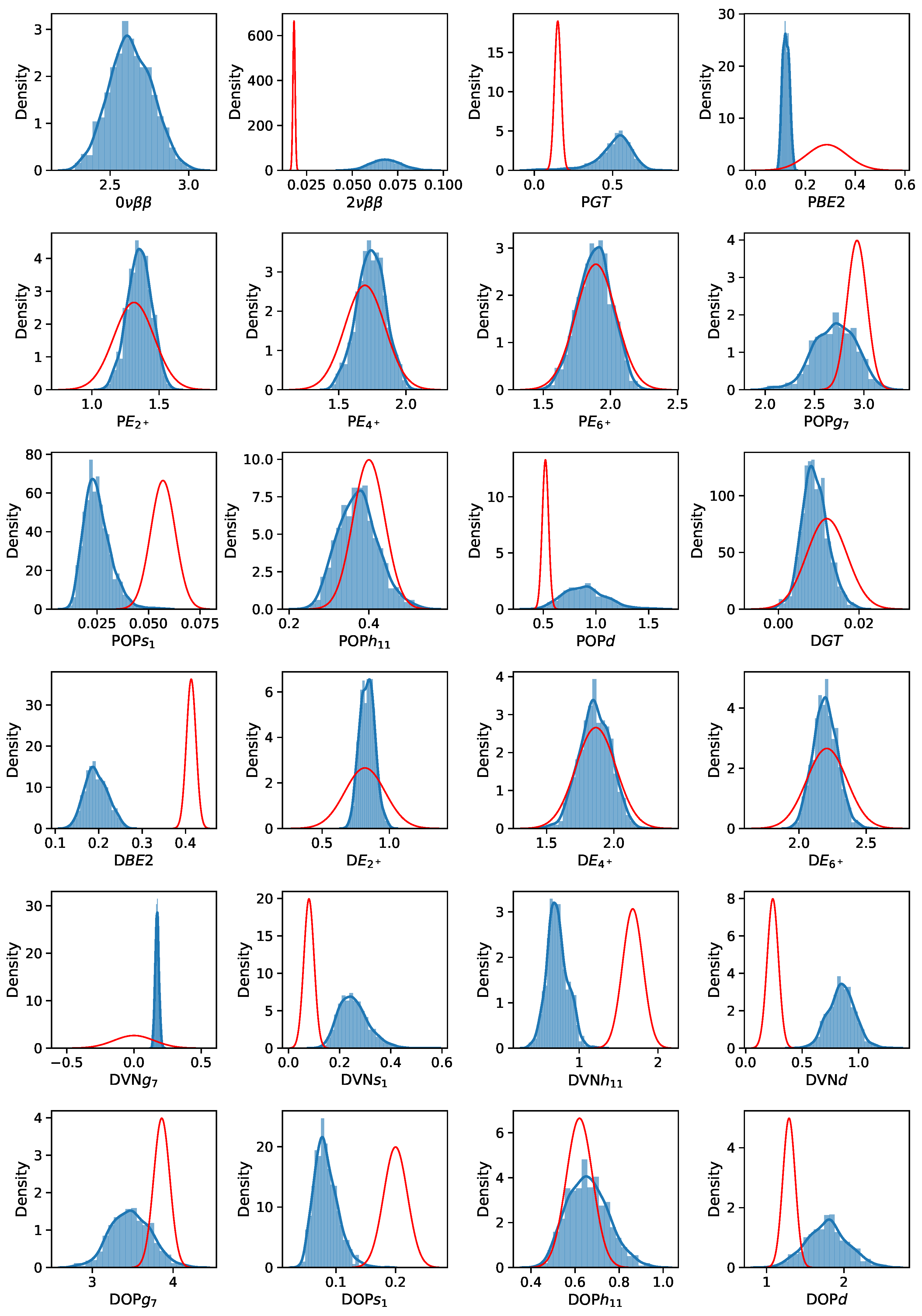
3.1. Description of Experimental Data and Statistical Results
3.2. The Bayesian Model Averaging
4. Conclusions and Outlook
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BSM | Beyond the Standard Model |
| LNV | Lepton Number Violation |
| PSF | Phase Space Factors |
| NME | Nuclear Matrix Element(s) |
| DBD | Double Beta Decay |
| pn-QRPA | proton-neutron Quasiparticle Random Phase Approximation |
| IBA | Interacting Boson Approximation |
| TBME | Two-Body Matrix Elements |
| KDE | Kernel Distribution Estimate |
| Probability Distribution Function | |
| SVD (svd) | name of nuclear effective Hamiltonian |
| GCN5082 (gcn) | name of nuclear effective Hamiltonian |
| jj55t (j5t) | name of nuclear effective Hamiltonian |
| jj55 | nuclear valence space |
References
- Agostini, M.; Benato, G.; Detwiler, J.A.; Menéndez, J.; Vissani, F. Toward the discovery of matter creation with neutrinoless ββ decay. Rev. Mod. Phys. 2023, 95, 025002. [Google Scholar] [CrossRef]
- Schechter, J.; Valle, J.W.F. Neutrinoless double-beta decay in SU(2)XU(1) theories. Phys. Rev. D 1982, 25, 2951. [Google Scholar] [CrossRef]
- Hirsch, M.; Kovalenko, S.; Schmidt, I. Extended Black box theorem for lepton number and flavor violating processes. Phys. Lett. B 2006, 642, 106. [Google Scholar] [CrossRef]
- Avignone, F.T., III; Elliott, S.R.; Engel, J. Double beta decay, Majorana neutrinos, and neutrino mass. Rev. Mod. Phys. 2008, 80, 481. [Google Scholar] [CrossRef]
- Vergados, J.D.; Ejiri, H.; Simkovic, F. Theory of neutrinoless double-beta decay. Rep. Prog. Phys. 2012, 75, 106301. [Google Scholar] [CrossRef] [PubMed]
- Bödeker, D.; Buchmüller, W. Baryogenesis from the weak scale to the grand unification scale. Rev. Mod. Phys. 2021, 93, 035004. [Google Scholar] [CrossRef]
- Barabash, A. Precise Half-Life Values for Two-Neutrino Double-beta Decay: 2020 Review. Universe 2020, 6, 159. [Google Scholar] [CrossRef]
- Doi, M.; Kotani, T.; Nishiura, H.; Takasugi, E. Double beta decay. Prog. Theor. Exp. Phys. 1983, 69, 602. [Google Scholar] [CrossRef]
- Doi, M.; Kotani, T.; Takasugi, E. Double-beta decay and Majorana neutrino. Prog. Theor. Phys. Suppl. 1985, 83, 1. [Google Scholar] [CrossRef]
- Suhonen, J.; Civitarese, O. Weak-interaction and nuclear-structure aspects of nuclear double beta decay. Phys. Rep. 1998, 300, 123. [Google Scholar] [CrossRef]
- Kotila, J.; Iachello, F. Phase-space factors for double-beta decay. Phys. Rev. C 2012, 85, 034316. [Google Scholar] [CrossRef]
- Stoica, S.; Mirea, M. New calculations for phase space factors involved in double-beta decay. Phys. Rev. C 2013, 88, 037303. [Google Scholar] [CrossRef]
- Mirea, M.; Pahomi, T.; Stoica, S. Phase space factors for double beta decay: An up-date. Rom. Rep. Phys. 2015, 67, 872. [Google Scholar]
- Caurier, E.; Poves, A.; Zuker, A.P. A full 0ℏω description of the 2νββ decay of 48Ca. Phys. Lett. B 1990, 252, 13. [Google Scholar] [CrossRef]
- Caurier, E.; Nowacki, F.; Poves, A.; Retamosa, J. Shell Model Studies of the Double Beta Decays of 76Ge, 82Se, and 136Xe. Phys. Rev. Lett. 1996, 77, 1954. [Google Scholar] [CrossRef] [PubMed]
- Caurier, E.; Martinez-Pinedo, G.; Nowacki, F.; Poves, A.; Zuker, A.P. The shell model as a unified view of nuclear structure. Rev. Mod. Phys. 2005, 77, 427. [Google Scholar] [CrossRef]
- Horoi, M.; Stoica, S.; Brown, B.A. Shell-model calculations of two-neutrino double-beta decay rates of 48Ca with the GXPF1A interaction. Phys. Rev. C 2007, 75, 034303. [Google Scholar] [CrossRef]
- Horoi, M.; Stoica, S. Shell model analysis of the neutrinoless double-beta decay of Ca-48. Phys. Rev. C 2010, 81, 024321. [Google Scholar] [CrossRef]
- Horoi, M. Shell model analysis of competing contributions to the double-beta decay of Ca-48. Phys. Rev. C 2013, 87, 014320. [Google Scholar] [CrossRef]
- Horoi, M.; Brown, B.A. Shell-Model Analysis of the Xe-136 Double Beta Decay Nuclear Matrix Elements. Phys. Rev. Lett. 2013, 110, 222502. [Google Scholar] [CrossRef]
- Sen’kov, R.A.; Horoi, M. Accurate shell-model nuclear matrix elements for neutrinoless double-beta decay. Phys. Rev. C 2014, 90, 051301(R). [Google Scholar] [CrossRef]
- Neacsu, A.; Horoi, M. Shell model studies of the 130Te neutrinoless double-beta decay. Phys. Rev. C 2015, 91, 024309. [Google Scholar] [CrossRef]
- Horoi, M.; Neacsu, A. Shell model predictions for 124Sn double-β decay. Phys. Rev. C 2016, 93, 024308. [Google Scholar] [CrossRef]
- Horoi, M.; Neacsu, A. Shell model study of using an effective field theory for disentangling several contributions to neutrinoless double-beta decay. Phys. Rev. C 2018, 98, 035502. [Google Scholar] [CrossRef]
- Simkovic, F.; Pantis, G.; Vergados, J.D.; Faessler, A. Additional nucleon current contributions to neutrinoless double-beta decay. Phys. Rev. C 1999, 60, 055502. [Google Scholar] [CrossRef]
- Stoica, S.; Klapdor-Kleingrothaus, H. Critical view on double-beta decay matrix elements within Quasi Random Phase Approximation-based methods. Nucl. Phys. A 2001, 694, 269. [Google Scholar] [CrossRef]
- Rodin, V.; Faessler, A.; Simkovic, F.; Vogel, P. Assessment of uncertainties in QRPA 0 nu beta beta-decay nuclear matrix elements. Nucl. Phys. A 2006, 766, 107–131. [Google Scholar] [CrossRef]
- Kortelainen, M.; Suhonen, J. Improved short-range correlations and 0 nu beta beta nuclear matrix elements of Ge-76 and Se-82. Phys. Rev. C 2007, 75, 051303(R). [Google Scholar] [CrossRef]
- Faessler, A.; Rodin, V.; Simkovic, F. Nuclear matrix elements for neutrinoless double-beta decay and double-electron capture. J. Phys. G 2012, 39, 124006. [Google Scholar] [CrossRef]
- Simkovic, F.; Rodin, V.; Faessler, A.; Vogel, P. 0νββ and 2νββ nuclear matrix elements, quasiparticle random-phase approximation, and isospin symmetry restoration. Phys. Rev. C 2013, 87, 045501. [Google Scholar] [CrossRef]
- Barea, J.; Iachello, F. Neutrinoless double-beta decay in the microscopic interacting boson model. Phys. Rev. C 2009, 79, 044301. [Google Scholar] [CrossRef]
- Barea, J.; Kotila, J.; Iachello, F. Nuclear matrix elements for double-beta decay. Phys. Rev. C 2013, 87, 014315. [Google Scholar] [CrossRef]
- Rodriguez, T.R.; Martinez-Pinedo, G. Energy Density Functional Study of Nuclear Matrix Elements for Neutrinoless beta beta Decay. Phys. Rev. Lett. 2010, 105, 252503. [Google Scholar] [CrossRef] [PubMed]
- Rath, P.K.; Chandra, R.; Chaturvedi, K.; Lohani, P.; Raina, P.K.; Hirsch, J.G. Neutrinoless beta beta decay transition matrix elements within mechanisms involving light Majorana neutrinos, classical Majorons, and sterile neutrinos. Phys. Rev. C 2013, 88, 064322. [Google Scholar] [CrossRef]
- Novario, S.; Gysbers, P.; Engel, J.; Hagen, G.; Jansen, G.R.; Morris, T.D.; Navrátil, P.; Papenbrock, T.; Quaglioni, S. Coupled-Cluster Calculations of Neutrinoless Double-β Decay in 48Ca. Phys. Rev. Lett. 2021, 126, 182502. [Google Scholar] [CrossRef]
- Yao, J.M.; Bally, B.; Engel, J.; Wirth, R.; Rodríguez, T.R.; Hergert, H. Ab Initio Treatment of Collective Correlations and the Neutrinoless Double Beta Decay of 48Ca. Phys. Rev. Lett. 2020, 124, 232501. [Google Scholar] [CrossRef]
- Belley, A.; Payne, C.G.; Stroberg, S.R.; Miyagi, T.; Holt, J.D. Ab Initio Neutrinoless Double-Beta Decay Matrix Elements for 48Ca, 76Ge, and 82Se. Phys. Rev. Lett. 2021, 126, 042502. [Google Scholar] [CrossRef]
- Cirigliano, V.; Davoudi, Z.; Engel, J.; Furnstahl, R.J.; Hagen, G.; Heinz, U.; Hergert, H.; Horoi, M.; Johnson, C.W.; Lovato, A.; et al. Towards precise and accurate calculations of neutrinoless double-beta decay. J. Phys. G Nucl. Part. Phys. 2022, 49, 120502. [Google Scholar] [CrossRef]
- Horoi, M.; Neacsu, A.; Stoica, S. Statistical analysis for the neutrinoless double-β-decay matrix element of 48Ca. Phys. Rev. C 2022, 106, 054302. [Google Scholar] [CrossRef]
- Horoi, M.; Neacsu, A.; Stoica, S. Predicting the neutrinoless double-β-decay matrix element of 136Xe using a statistical approach. Phys. Rev. C 2023, 107, 045501. [Google Scholar] [CrossRef]
- Kejzlar, V.; Neufcourt, L.; Nazarewicz, W.; Reinhard, P.G. Statistical aspects of nuclear mass models. J. Phys. G Nucl. Part. Phys. 2020, 47, 094001. [Google Scholar] [CrossRef]
- Qi, C.; Xu, Z.X. Monopole-optimized effective interaction for tin isotopes. Phys. Rev. C 2012, 86, 044323. [Google Scholar] [CrossRef]
- Caurier, E.; Nowacki, F.; Poves, A.; Sieja, K. Collectivity in the light xenon isotopes: A shell model study. Phys. Rev. C 2010, 82, 064304. [Google Scholar] [CrossRef]
- Adhikari, G.; Kharusi, S.A.; Angelico, E.; Anton, G.; Arnquist, I.J.; Badhrees, I.; Bane, J.; Belov, V.; Bernard, E.P.; Bhatta, T.; et al. nEXO: Neutrinoless double beta decay search beyond 1028 year half-life sensitivity. J. Phys. G Nucl. Part. Phys. 2021, 49, 015104. [Google Scholar] [CrossRef]
- Kamland-Zen Collaboration. Kamland-Zen Collaboration. First Search for the Majorana Nature of Neutrinos in the Inverted Mass Ordering Region with KamLAND-Zen. arXiv 2022. [Google Scholar] [CrossRef]
- Si, L.; Cheng, Z.; Abdukerim, A.; Bo, Z.; Chen, W.; Chen, X.; Chen, Y.; Cheng, C.; Cheng, Y.; Cui, X.; et al. Determination of Double Beta Decay Half-Life of 136Xe with the PandaX-4T Natural Xenon Detector. Research 2022, 2022. [Google Scholar] [CrossRef] [PubMed]
- Aprile, E.; Abe, K.; Agostini, F.; Ahmed Maouloud, S.; Alfonsi, M.; Althueser, L.; Andrieu, B.; Angelino, E.; Angevaare, J.R.; Antochi, V.C.; et al. Double-weak decays of 124Xe and 136Xe in the XENON1T and XENONnT experiments. Phys. Rev. C 2022, 106, 024328. [Google Scholar] [CrossRef]
- Agostini, F.; Maouloud, S.E.M.A.; Althueser, L.; Amaro, F.; Antunovic, B.; Aprile, E.; Baudis, L.; Baur, D.; Biondi, Y.; Bismark, A.; et al. Sensitivity of the DARWIN observatory to the neutrinoless double beta decay of 136Xe. Eur. Phys. J. C 2020, 80, 808. [Google Scholar] [CrossRef]
- Märkisch, B.; Mest, H.; Saul, H.; Wang, X.; Abele, H.; Dubbers, D.; Klopf, M.; Petoukhov, A.; Roick, C.; Soldner, T.; et al. Measurement of the Weak Axial-Vector Coupling Constant in the Decay of Free Neutrons Using a Pulsed Cold Neutron Beam. Phys. Rev. Lett. 2019, 122, 242501. [Google Scholar] [CrossRef]
- Horoi, M. Double Beta Decay: A Shell Model Approach. Physics 2022, 4, 1135–1149. [Google Scholar] [CrossRef]
- Coraggio, L.; De Angelis, L.; Fukui, T.; Gargano, A.; Itaco, N.; Nowacki, F. Renormalization of the Gamow-Teller operator within the realistic shell model. Phys. Rev. C 2019, 100, 014316. [Google Scholar] [CrossRef]
- Stroberg, S.R. Beta Decay in Medium-Mass Nuclei with the In-Medium Similarity Renormalization Group. Particles 2021, 4, 521–535. [Google Scholar] [CrossRef]
- Gysbers, P.; Hagen, G.; Holt, J.D.; Jansen, G.R.; Morris, T.D.; Navrátil, P.; Papenbrock, T.; Quaglioni, S.; Schwenk, A.; Stroberg, S.R.; et al. Discrepancy between experimental and theoretical β-decay rates resolved from first principles. Nat. Phys. 2019, 15, 428–431. [Google Scholar] [CrossRef]
- Sen’kov, R.A.; Horoi, M. Neutrinoless double-beta decay of Ca-48 in the shell model: Closure versus nonclosure approximation. Phys. Rev. C 2013, 88, 064312. [Google Scholar] [CrossRef]
- Sen’kov, R.A.; Horoi, M.; Brown, B.A. Neutrinoless double-beta decay of Se-82 in the shell model: Beyond the closure approximation. Phys. Rev. C 2014, 89, 054304. [Google Scholar] [CrossRef]
- Sen’kov, R.A.; Horoi, M. Shell-model calculation of neutrinoless double-β decay of 76Ge. Phys. Rev. C 2016, 93, 044334. [Google Scholar] [CrossRef]
- Gimeno, P.; Jokiniemi, L.; Kotila, J.; Ramalho, M.; Suhonen, J. Ordinary Muon Capture on 136Ba: Comparative Study Using the Shell Model and pnQRPA. Universe 2023, 9, 270. [Google Scholar] [CrossRef]
- Rosenblatt, M. Remarks on Some Nonparametric Estimates of a Density Function. Ann. Math. Stat. 1956, 27, 832–837. [Google Scholar] [CrossRef]
- Silverman, B. Density Estimation for Statistics and Data Analysis; Chapman and Hall: London, UK, 1986. [Google Scholar]
- Horoi, M.; Neacsu, A. Analysis of mechanisms that could contribute to neutrinoless double-beta decay. Phys. Rev. D 2016, 93, 113014. [Google Scholar] [CrossRef]
- Ahmed, F.; Horoi, M. Interference effects for 0νββ decay in the left-right symmetric model. Phys. Rev. C 2020, 101, 035504. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Horoi, M. Improved Statistical Analysis for the Neutrinoless Double-Beta Decay Matrix Element of 136Xe. Universe 2024, 10, 252. https://doi.org/10.3390/universe10060252
Horoi M. Improved Statistical Analysis for the Neutrinoless Double-Beta Decay Matrix Element of 136Xe. Universe. 2024; 10(6):252. https://doi.org/10.3390/universe10060252
Chicago/Turabian StyleHoroi, Mihai. 2024. "Improved Statistical Analysis for the Neutrinoless Double-Beta Decay Matrix Element of 136Xe" Universe 10, no. 6: 252. https://doi.org/10.3390/universe10060252
APA StyleHoroi, M. (2024). Improved Statistical Analysis for the Neutrinoless Double-Beta Decay Matrix Element of 136Xe. Universe, 10(6), 252. https://doi.org/10.3390/universe10060252





