Quantum Gravity on the Computer: Impressions of a Workshop
Abstract
:1. Approaches, Observables and Numerical Methods
1.1. Introduction to Various Approaches to Quantum Gravity
1.2. Subtleties in Defining a Theory (on the Computer)
1.2.1. Relating to the Continuum
1.2.2. Approximations and Simplifications
1.3. Observables
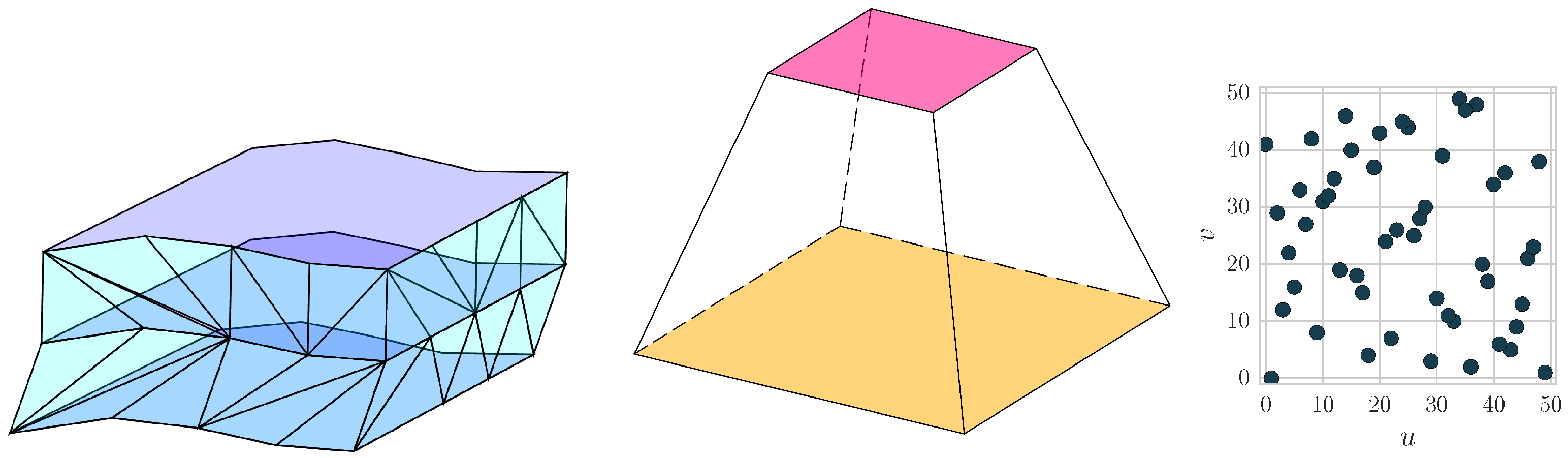
1.3.1. Non-Locality in Quantum Gravity
1.3.2. Summing over Topology
1.3.3. Quantum Cosmology
1.3.4. Measuring Dimension
1.3.5. Other Observables
1.4. Numerical Methods in Quantum Gravity
1.4.1. The Chimera Algorithm
1.4.2. Transfer Matrix Approach
1.4.3. Markov Chain Monte Carlo Simulations
1.4.4. Parallel Rejection
1.4.5. Adopting Methods from Other Fields
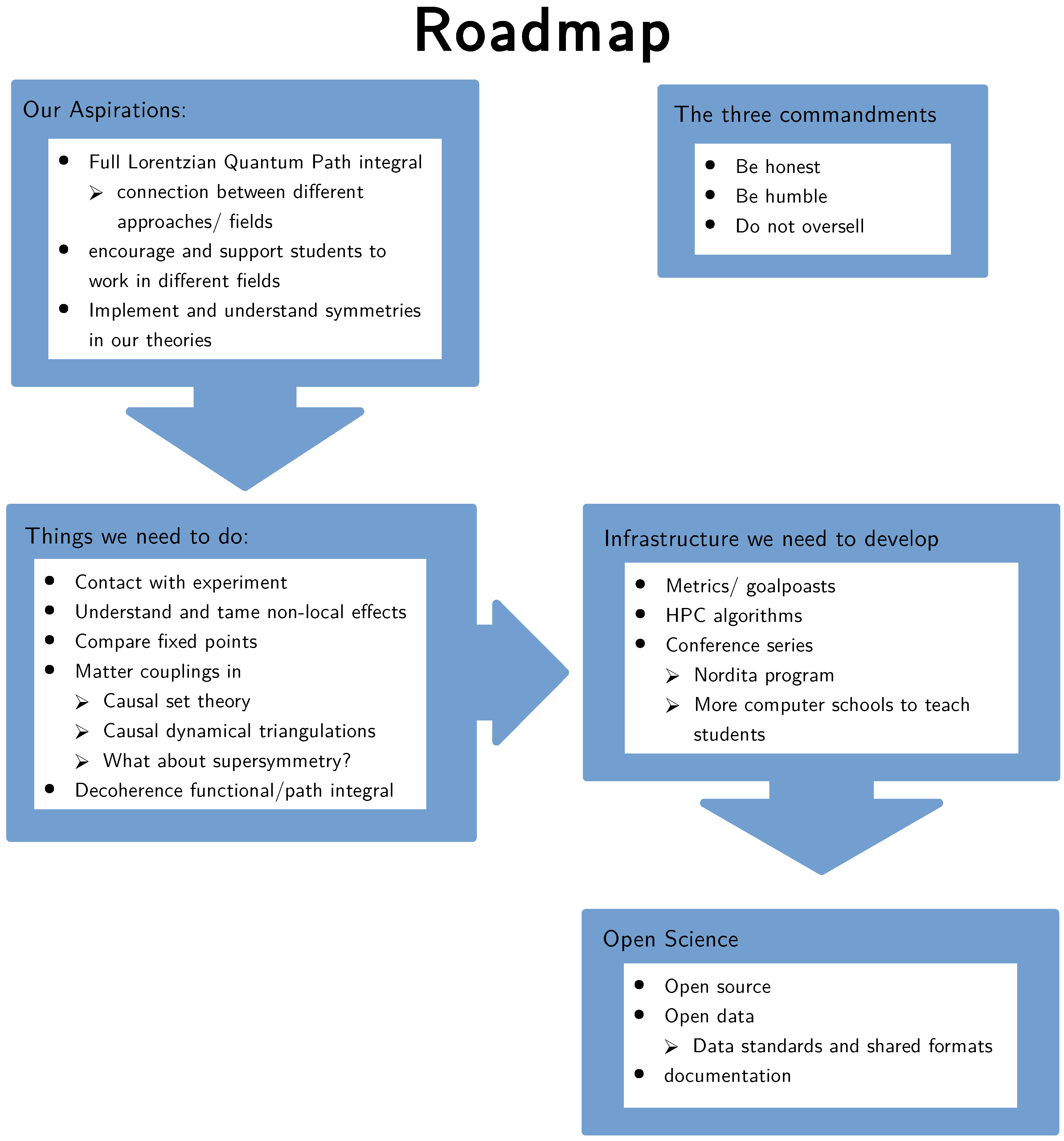
2. Roadmap
2.1. Open Science
2.2. Future
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ambjorn, J.; Loll, R. Non-perturbative Lorentzian Quantum Gravity, Causality and Topology Change. Nucl. Phys. B 1998, 536, 407–434. [Google Scholar] [CrossRef]
- Ambjørn, J.; Görlich, A.; Jurkiewicz, J.; Loll, R. Nonperturbative quantum gravity. Phys. Rep. 2012, 519, 127–210. [Google Scholar] [CrossRef] [Green Version]
- Bombelli, L.; Lee, J.; Meyer, D.; Sorkin, R. Space-Time as a Causal Set. Phys. Rev. Lett. 1987, 59, 521–524. [Google Scholar] [CrossRef] [PubMed]
- Sorkin, R.D. Causal sets: Discrete gravity. In Lectures on Quantum Gravity; Springer: Boston, MA, USA, 2005; pp. 305–327. [Google Scholar]
- Freidel, L. Group field theory: An Overview. Int. J. Theor. Phys. 2005, 44, 1769–1783. [Google Scholar] [CrossRef]
- Oriti, D. Group field theory as the microscopic description of the quantum spacetime fluid: A New perspective on the continuum in quantum gravity. arXiv, 2007; arXiv:0710.3276. [Google Scholar]
- Rivasseau, V. Quantum Gravity and Renormalization: The Tensor Track. AIP Conf. Proc. 2011, 1444, 18–29. [Google Scholar]
- Rivasseau, V. Random Tensors and Quantum Gravity. SIGMA 2016, 12, 69. [Google Scholar] [CrossRef]
- Gurau, R. Invitation to Random Tensors. SIGMA 2016, 12, 94. [Google Scholar] [CrossRef]
- Rovelli, C. Quantum Gravity; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Thiemann, T. Modern Canonical Quantum General Relativity; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Connes, A. Noncommutative Geometry; Academic Press: Cambridge, MA, USA, 1994. [Google Scholar]
- Perez, A. The Spin Foam Approach to Quantum Gravity. Living Rev. Relativ. 2013, 16, 3. [Google Scholar] [CrossRef] [PubMed]
- Rovelli, C.; Vidotto, F. Covariant Loop Quantum Gravity; Cambridge University Press: Cambrige, UK, 2014. [Google Scholar]
- Ashtekar, A.; Pawlowski, T.; Singh, P. Quantum Nature of the Big Bang. Phys. Rev. Lett. 2006, 96, 141301. [Google Scholar] [CrossRef]
- Pretorius, F. Evolution of Binary Black-Hole Spacetimes. Phys. Rev. Lett. 2005, 95, 121101. [Google Scholar] [CrossRef]
- Dürr, S.; Fodor, Z.; Frison, J.; Hoelbling, C.; Hoffmann, R.; Katz, S.D.; Krieg, S.; Kurth, T.; Lellouch, L.; Lippert, T.; et al. Ab Initio Determination of Light Hadron Masses. Science 2008, 322, 1224–1227. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Henson, J.; Rideout, D.P.; Sorkin, R.D.; Surya, S. Onset of the Asymptotic Regime for Finite Orders. Exp. Math. 2015, 26, 253–266. [Google Scholar] [CrossRef]
- Zwane, N.; Afshordi, N.; Sorkin, R.D. Cosmological tests of Everpresent Λ. Class. Quant. Grav. 2018, 35, 194002. [Google Scholar] [CrossRef]
- Dittrich, B. The continuum limit of loop quantum gravity—A framework for solving the theory. In Loop Quantum Gravity: The First 30 Years; Ashtekar, A., Pullin, J., Eds.; World Scientific: Singapore, 2017; pp. 153–179. [Google Scholar]
- Bahr, B.; Steinhaus, S. Numerical evidence for a phase transition in 4d spin foam quantum gravity. Phys. Rev. Lett. 2016, 117, 141302. [Google Scholar] [CrossRef] [PubMed]
- Donà, P.; Fanizza, M.; Sarno, G.; Speziale, S. SU(2) graph invariants, Regge actions and polytopes. Class. Quant. Grav. 2018, 35, 045011. [Google Scholar] [CrossRef] [Green Version]
- Available online: nordita.org/qg2018 (accessed on 18 January 2019).
- Regge, T. General Relativity without Coordinates. Nuovo Cim. 1961, 19, 558–571. [Google Scholar] [CrossRef]
- Di Francesco, P.; Ginsparg, P.H.; Zinn-Justin, J. 2-D Gravity and random matrices. Phys. Rep. 1995, 254, 1–133. [Google Scholar] [CrossRef]
- Gielen, S.; Oriti, D.; Sindoni, L. Cosmology from Group Field Theory Formalism for Quantum Gravity. Phys. Rev. Lett. 2013, 111, 031301. [Google Scholar] [CrossRef]
- Oriti, D. Group Field Theory and Loop Quantum Gravity; Extended Draft Version of a Contribution to the Volume: ‘Loop Quantum Gravity’; Ashtekar, A., Pullin, J., Eds.; World Scientific: Singapore, 2014. [Google Scholar]
- Carrozza, S. Flowing in Group Field Theory Space: A Review. SIGMA 2016, 12, 70. [Google Scholar] [CrossRef]
- Rovelli, C.; Smerlak, M. In quantum gravity, summing is refining. Class. Quant. Grav. 2012, 29, 055004. [Google Scholar] [CrossRef] [Green Version]
- Bahr, B. On background-independent renormalization of spin foam models. Class. Quant. Grav. 2017, 34, 075001. [Google Scholar] [CrossRef] [Green Version]
- Alesci, E.; Thiemann, T.; Zipfel, A. Linking covariant and canonical LQG: New solutions to the Euclidean Scalar Constraint. Phys. Rev. D 2012, 86, 024017. [Google Scholar] [CrossRef]
- Thiemann, T.; Zipfel, A. Linking covariant and canonical LQG II: Spin foam projector. Class. Quant. Grav. 2014, 31, 125008. [Google Scholar] [CrossRef]
- Eichhorn, A.; Surya, S.; Versteegen, F. Induced Spatial Geometry from Causal Structure. arXiv, 2018; arXiv:1809.06192. [Google Scholar]
- Glaser, L.; Surya, S. Towards a Definition of Locality in a Manifoldlike Causal Set. Phys. Rev. D 2013, 88, 124026. [Google Scholar] [CrossRef]
- Henson, J. Constructing an interval of Minkowski space from a causal set. Class. Quant. Grav. 2006, 23, L29–L35. [Google Scholar] [CrossRef]
- Zwiebach, B. A First Course in String Theory; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Polchinski, J. String Theory. Vol. 1: An Introduction to the Bosonic String. In Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Ambjorn, J.; Makeenko, Y. String theory as a Lilliputian world. Phys. Lett. B 2016, 756, 142–146. [Google Scholar] [CrossRef] [Green Version]
- Barrett, J.W.; Glaser, L. Monte Carlo simulations of random non-commutative geometries. J. Phys. A 2016, 49, 245001. [Google Scholar] [CrossRef] [Green Version]
- Glaser, L. Scaling behaviour in random non-commutative geometries. J. Phys. A 2017, 50, 275201. [Google Scholar] [CrossRef] [Green Version]
- Reuter, M.; Saueressig, F. Quantum Einstein Gravity. New J. Phys. 2012, 14, 055022. [Google Scholar] [CrossRef]
- Weinberg, S. Ultraviolet Divergences in Quantum Theories of Gravitation. In General Relativity: An Einstein Centenary Survey; Cambridge University Press: Cambridge, UK, 1980; pp. 790–831. [Google Scholar]
- Wetterich, C. Exact evolution equation for the effective potential. Phys. Lett. B 1993, 301, 90–94. [Google Scholar] [CrossRef] [Green Version]
- Gupta, R. Introduction to lattice QCD: Course. In Proceedings of the 68th Session Summer School in Theoretical Physics “Probing the Standard Model of Particle Interactions”, Les Houches, France, 28 July–5 September 1997; pp. 83–219. [Google Scholar]
- Gross, D.J.; Wilczek, F. Ultraviolet Behavior of Nonabelian Gauge Theories. Phys. Rev. Lett. 1973, 30, 1343–1346. [Google Scholar] [CrossRef]
- Wilson, K.G.; Kogut, J.B. The Renormalization group and the epsilon expansion. Phys. Rep. 1974, 12, 75–200. [Google Scholar] [CrossRef]
- Dittrich, B.; Mizera, S.; Steinhaus, S. Decorated tensor network renormalization for lattice gauge theories and spin foam models. New J. Phys. 2016, 18, 053009. [Google Scholar] [CrossRef] [Green Version]
- Dittrich, B.; Steinhaus, S. Time evolution as refining, coarse graining and entangling. New J. Phys. 2014, 16, 123041. [Google Scholar] [CrossRef] [Green Version]
- Knorr, B.; Saueressig, F. Towards reconstructing the quantum effective action of gravity. Phys. Rev. Lett. 2018, 121, 161304. [Google Scholar] [CrossRef] [PubMed]
- Tanabashi, M.; et al.; Particle Data Group Review of Particle Physics. Phys. Rev. D 2018, 98, 030001. [Google Scholar] [CrossRef]
- Levin, M.; Nave, C.P. Tensor renormalization group approach to 2D classical lattice models. Phys. Rev. Lett. 2007, 99, 120601. [Google Scholar] [CrossRef] [PubMed]
- Ambjorn, J.; Correia, J.; Kristjansen, C.; Loll, R. On the relation between Euclidean and Lorentzian 2D quantum gravity. Phys. Lett. B 2000, 475, 24–32. [Google Scholar] [CrossRef]
- Jordan, S.; Loll, R. De Sitter universe from causal dynamical triangulations without preferred foliation. Phys. Rev. D 2013, 88, 044055. [Google Scholar] [CrossRef]
- Jordan, S.; Loll, R. Causal Dynamical Triangulations without preferred foliation. Phys. Lett. B 2013, 724, 155–159. [Google Scholar] [CrossRef] [Green Version]
- Laiho, J.; Bassler, S.; Coumbe, D.; Du, D.; Neelakanta, J.T. Lattice Quantum Gravity and Asymptotic Safety. Phys. Rev. D 2017, 96, 064015. [Google Scholar] [CrossRef]
- Dona, P.; Sarno, G. Numerical methods for EPRL spin foam transition amplitudes and Lorentzian recoupling theory. Gen. Relativ. Grav. 2018, 50, 127. [Google Scholar] [CrossRef]
- Dittrich, B.; Martín-Benito, M.; Schnetter, E. Coarse graining of spin net models: Dynamics of intertwiners. New J. Phys. 2013, 15, 103004. [Google Scholar] [CrossRef]
- Dittrich, B.; Martin-Benito, M.; Steinhaus, S. Quantum group spin nets: Refinement limit and relation to spin foams. Phys. Rev. D 2014, 90, 024058. [Google Scholar] [CrossRef]
- Dittrich, B.; Schnetter, E.; Seth, C.J.; Steinhaus, S. Coarse graining flow of spin foam intertwiners. Phys. Rev. D 2016, 94, 124050. [Google Scholar] [CrossRef] [Green Version]
- Delcamp, C.; Dittrich, B. Towards a phase diagram for spin foams. Class. Quant. Grav. 2017, 34, 225006. [Google Scholar] [CrossRef] [Green Version]
- Bahr, B.; Steinhaus, S. Investigation of the Spinfoam Path integral with Quantum Cuboid Intertwiners. Phys. Rev. D 2016, 93, 104029. [Google Scholar] [CrossRef]
- Bahr, B.; Kloser, S.; Rabuffo, G. Towards a Cosmological subsector of Spin Foam Quantum Gravity. Phys. Rev. D 2017, 96, 086009. [Google Scholar] [CrossRef]
- Bahr, B.; Steinhaus, S. Hypercuboidal renormalization in spin foam quantum gravity. Phys. Rev. D 2017, 95, 126006. [Google Scholar] [CrossRef] [Green Version]
- Steinhaus, S.; Thürigen, J. Emergence of Spacetime in a restricted Spin-foam model. Phys. Rev. D 2018, 98, 026013. [Google Scholar] [CrossRef]
- Bahr, B.; Rabuffo, G.; Steinhaus, S. Renormalization in symmetry restricted spin foam models with curvature. arXiv, 2018; arXiv:1804.00023. [Google Scholar]
- Kleitman, D.J.; Rothschild, B.L. Asymptotic enumeration of partial orders on a finite set. Trans. Am. Math. Soc. 1975, 205, 205–220. [Google Scholar] [CrossRef]
- Donnelly, W.; Freidel, L. Local subsystems in gauge theory and gravity. J. High Energy Phys. 2016, 2016, 102. [Google Scholar] [CrossRef]
- Dowker, F.; Glaser, L. Causal set d’Alembertians for various dimensions. Class. Quant. Grav. 2013, 30, 195016. [Google Scholar] [CrossRef]
- Glaser, L. A closed form expression for the causal set d’Alembertian. Class. Quant. Grav. 2014, 31, 095007. [Google Scholar] [CrossRef] [Green Version]
- Aslanbeigi, S.; Saravani, M.; Sorkin, R.D. Generalized causal set d’Alembertians. J. High Energy Phys. 2014, 2014, 24. [Google Scholar] [CrossRef]
- Sorkin, R.D. Does Locality Fail at Intermediate Length-Scales. In Approaches to Quantum Gravity; Oriti, D., Ed.; Cambridge University Press: Cambridge, UK, 2007; pp. 26–43. [Google Scholar]
- Belenchia, A.; Benincasa, D.M.T.; Liberati, S. Nonlocal scalar quantum field theory from causal sets. J. High Energy Phys. 2015, 2015, 36. [Google Scholar] [CrossRef] [Green Version]
- Belenchia, A.; Benincasa, D.M.; Martín-Martínez, E.; Saravani, M. Low energy signatures of nonlocal field theories. Phys. Rev. D 2016, 94, 061902. [Google Scholar] [CrossRef] [Green Version]
- Belenchia, A.; Benincasa, D.M.T.; Liberati, S.; Marin, F.; Marino, F.; Ortolan, A. Tests of Quantum Gravity induced non-locality via opto-mechanical quantum oscillators. Phys. Rev. Lett. 2016, 116, 161303. [Google Scholar] [CrossRef]
- Philpott, L.; Dowker, F.; Sorkin, R.D. Energy-momentum diffusion from spacetime discreteness. Phys. Rev. D 2009, 79, 124047. [Google Scholar] [CrossRef]
- Ambjørn, J.; Durhuus, B. Quantum Geometry: A Statistical Field Theory Approach; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Cooperman, J.H.; Miller, J.M. A first look at transition amplitudes in (2 + 1)-dimensional causal dynamical triangulations. Class. Quant. Grav. 2014, 31, 035012. [Google Scholar] [CrossRef] [Green Version]
- Linde, A.D. Inflationary Cosmology; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Agullo, I.; Morris, N.A. Detailed analysis of the predictions of loop quantum cosmology for the primordial power spectra. Phys. Rev. D 2015, 92, 124040. [Google Scholar] [CrossRef]
- Fleischhack, C. Kinematical Foundations of Loop Quantum Cosmology. In Proceedings of the Conference on Quantum Mathematical Physics: A Bridge between Mathematics and Physics, Regensburg, Germany, 29 September–2 October 2014; pp. 201–232. [Google Scholar]
- Hanusch, M. Projective Structures in Loop Quantum Cosmology. J. Math. Anal. Appl. 2015, 428, 1005–1034. [Google Scholar] [CrossRef]
- Beetle, C.; Engle, J.S.; Hogan, M.E.; Mendonça, P. Diffeomorphism invariant cosmological sector in loop quantum gravity. Class. Quant. Grav. 2017, 34, 225009. [Google Scholar] [CrossRef] [Green Version]
- Assanioussi, M.; Dapor, A.; Liegener, K.; Pawłowski, T. Emergent de Sitter Epoch of the Quantum Cosmos from Loop Quantum Cosmology. Phys. Rev. Lett. 2018, 121, 081303. [Google Scholar] [CrossRef] [PubMed]
- Dapor, A.; Liegener, K. Cosmological Effective Hamiltonian from full Loop Quantum Gravity Dynamics. Phys. Lett. B 2018, 785, 506–510. [Google Scholar] [CrossRef]
- Alesci, E.; Cianfrani, F. Quantum Reduced Loop Gravity and the foundation of Loop Quantum Cosmology. Int. J. Mod. Phys. D 2016, 25, 1642005. [Google Scholar] [CrossRef]
- Bodendorfer, N.; Lewandowski, J.; Świeżewski, J. General relativity in the radial gauge: Reduced phase space and canonical structure. Phys. Rev. D 2015, 92, 084041. [Google Scholar] [CrossRef]
- Bodendorfer, N.; Zipfel, A. On the relation between reduced quantisation and quantum reduction for spherical symmetry in loop quantum gravity. Class. Quant. Grav. 2016, 33, 155014. [Google Scholar] [CrossRef] [Green Version]
- Ambjorn, J.; Jurkiewicz, J.; Loll, R. Emergence of a 4-D world from causal quantum gravity. Phys. Rev. Lett. 2004, 93, 131301. [Google Scholar] [CrossRef]
- Ambjørn, J.; Jurkiewicz, J.; Loll, R. The Spectral Dimension of the Universe is Scale Dependent. Phys. Rev. Lett. 2005, 95, 171301. [Google Scholar] [CrossRef] [PubMed]
- Ambjørn, J.; Gizbert-Studnicki, J.; Görlich, A.; Grosvenor, K.; Jurkiewicz, J. Four-dimensional CDT with toroidal topology. Nucl. Phys. B 2017, 922, 226–246. [Google Scholar] [CrossRef]
- Glaser, L.; Loll, R. CDT and cosmology. C. R. Phys. 2017, 18, 265–274. [Google Scholar] [CrossRef] [Green Version]
- Gielen, S.; Sindoni, L. Quantum Cosmology from Group Field Theory Condensates: A Review. SIGMA 2016, 12, 82. [Google Scholar] [CrossRef]
- Oriti, D. The universe as a quantum gravity condensate. C. R. Phys. 2017, 18, 235–245. [Google Scholar] [CrossRef] [Green Version]
- Oriti, D.; Sindoni, L.; Wilson-Ewing, E. Bouncing cosmologies from quantum gravity condensates. Class. Quant. Grav. 2017, 34, 04LT01. [Google Scholar] [CrossRef]
- Glaser, L.; Surya, S. The Hartle–Hawking wave function in 2D causal set quantum gravity. Class. Quant. Grav. 2016, 33, 065003. [Google Scholar] [CrossRef] [Green Version]
- Schleicher, D. Hausdorff Dimension, Its Properties, and Its Surprises. Am. Math. Mon. 2007, 114, 509–528. [Google Scholar] [CrossRef] [Green Version]
- Benedetti, D.; Henson, J. Spectral geometry as a probe of quantum spacetime. Phys. Rev. D 2009, 80, 124036. [Google Scholar] [CrossRef]
- Calcagni, G.; Eichhorn, A.; Saueressig, F. Probing the quantum nature of spacetime by diffusion. Phys. Rev. D 2013, 87, 124028. [Google Scholar] [CrossRef]
- Horava, P. Spectral Dimension of the Universe in Quantum Gravity at a Lifshitz Point. Phys. Rev. Lett. 2009, 102, 161301. [Google Scholar] [CrossRef] [PubMed]
- Eichhorn, A.; Mizera, S. Spectral dimension in causal set quantum gravity. Class. Quant. Grav. 2014, 31, 125007. [Google Scholar] [CrossRef] [Green Version]
- Carlip, S. Dimensional reduction in causal set gravity. Class. Quant. Grav. 2015, 32, 232001. [Google Scholar] [CrossRef] [Green Version]
- Modesto, L. Fractal Structure of Loop Quantum Gravity. Class. Quant. Grav. 2009, 26, 242002. [Google Scholar] [CrossRef]
- Calcagni, G.; Oriti, D.; Thürigen, J. Dimensional flow in discrete quantum geometries. Phys. Rev. D 2015, 91, 084047. [Google Scholar] [CrossRef]
- Alkofer, N.; Saueressig, F.; Zanusso, O. Spectral dimensions from the spectral action. Phys. Rev. D 2015, 91, 025025. [Google Scholar] [CrossRef]
- Barrett, J.W.; Druce, P.J.; Glaser, L. Spectral estimators for finite non-commutative geometries. Unpublished work. 2019. [Google Scholar]
- Amelino-Camelia, G.; Brighenti, F.; Gubitosi, G.; Santos, G. Thermal dimension of quantum spacetime. Phys. Lett. B 2017, 767, 48–52. [Google Scholar] [CrossRef]
- Kotecha, I.; Oriti, D. Statistical Equilibrium in Quantum Gravity: Gibbs states in Group Field Theory. New J. Phys. 2018, 20, 073009. [Google Scholar] [CrossRef]
- Klitgaard, N.; Loll, R. Introducing Quantum Ricci Curvature. Phys. Rev. D 2018, 97, 046008. [Google Scholar] [CrossRef]
- Klitgaard, N.; Loll, R. Implementing quantum Ricci curvature. Phys. Rev. D 2018, 97, 106017. [Google Scholar] [CrossRef] [Green Version]
- Maldacena, J.M. The Large N limit of superconformal field theories and supergravity. Int. J. Theor. Phys. 1999, 38, 1113–1133. [Google Scholar] [CrossRef]
- Dittrich, B.; Goeller, C.; Livine, E.R.; Riello, A. Quasi-local holographic dualities in non-perturbative 3d quantum gravity I—Convergence of multiple approaches and examples of Ponzano–Regge statistical duals. Nucl. Phys. B 2019, 938, 807–877. [Google Scholar] [CrossRef]
- Dittrich, B.; Goeller, C.; Livine, E.R.; Riello, A. Quasi-local holographic dualities in non-perturbative 3d quantum gravity II—From coherent quantum boundaries to BMS3 characters. Nucl. Phys. B 2019, 938, 878–934. [Google Scholar] [CrossRef]
- Bonzom, V.; Dittrich, B. 3D holography: From discretum to continuum. J. High Energy Phys. 2016, 2016, 208. [Google Scholar] [CrossRef]
- Barnich, G.; Gonzalez, H.A.; Maloney, A.; Oblak, B. One-loop partition function of three-dimensional flat gravity. J. High Energy Phys. 2015, 2015, 178. [Google Scholar] [CrossRef]
- Oblak, B. Characters of the BMS Group in Three Dimensions. Commun. Math. Phys. 2015, 340, 413–432. [Google Scholar] [CrossRef] [Green Version]
- Han, M.; Hung, L.Y. Loop Quantum Gravity, Exact Holographic Mapping, and Holographic Entanglement Entropy. Phys. Rev. D 2017, 95, 024011. [Google Scholar] [CrossRef]
- Chirco, G.; Oriti, D.; Zhang, M. Group field theory and tensor networks: Towards a Ryu–Takayanagi formula in full quantum gravity. Class. Quant. Grav. 2018, 35, 115011. [Google Scholar] [CrossRef]
- Diener, P.; Gupt, B.; Singh, P. Chimera: A hybrid approach to numerical loop quantum cosmology. Class. Quant. Grav. 2014, 31, 025013. [Google Scholar] [CrossRef]
- Ambjørn, J.; Gizbert-Studnicki, J.; Görlich, A.; Jurkiewicz, J. The effective action in 4-dim CDT. The transfer matrix approach. J. High Energy Phys. 2014, 2014, 34. [Google Scholar] [CrossRef]
- Pachner, U. P.L. Homeomorphic Manifolds are Equivalent by Elementary Shellings. Eur. J. Comb. 1991, 12, 129–145. [Google Scholar] [CrossRef]
- Neal, R.M. MCMC using Hamiltonian dynamics. arXiv, 2012; arXiv:1206.1901. [Google Scholar]
- Newman, M.E.J.; Barkema, G.T. Monte Carlo Methods in Statistical Physics; Clarendon Press: Wotton-under-Edge, UK, 1999. [Google Scholar]
- Meyer, D.A. The Dimension of Causal Sets. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1988. [Google Scholar]
| 1 | This number is estimated by assuming that if extra dimensions of this size can not be experimentally excluded it gives a conservative upper limit on the scale at which quantum gravity would appear. |
| 2 | More precisely, the operator is the inverse d’Alembertian squared sandwiched by two Ricci scalars. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Glaser, L.; Steinhaus, S. Quantum Gravity on the Computer: Impressions of a Workshop. Universe 2019, 5, 35. https://doi.org/10.3390/universe5010035
Glaser L, Steinhaus S. Quantum Gravity on the Computer: Impressions of a Workshop. Universe. 2019; 5(1):35. https://doi.org/10.3390/universe5010035
Chicago/Turabian StyleGlaser, Lisa, and Sebastian Steinhaus. 2019. "Quantum Gravity on the Computer: Impressions of a Workshop" Universe 5, no. 1: 35. https://doi.org/10.3390/universe5010035
APA StyleGlaser, L., & Steinhaus, S. (2019). Quantum Gravity on the Computer: Impressions of a Workshop. Universe, 5(1), 35. https://doi.org/10.3390/universe5010035




