Implications of Quantum Gravity for Dark Matter Searches with Atom Interferometers
Abstract
:Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cronin, A.D.; Schmiedmayer, J.; Pritchard, D.E. Optics and interferometry with atoms and molecules. Rev. Mod. Phys. 2009, 81, 1051–1129. [Google Scholar] [CrossRef]
- Adamson, P.; Chattopadhyay, S.; Coleman, J.; Graham, P.; Geer, S.; Harnik, R.; Hahn, S.; Hogan, J.; Kasevich, M.; Kovachy, T.; et al. PROPOSAL: P-1101 Matter-Wave Atomic Gradiometer Interferometric Sensor (MAGIS-100); FERMILAB-TM-2700-PPD; Fermi National Accelerator Lab. (FNAL): Batavia, IL, USA, 17 December 2018. [CrossRef]
- Abe, M.; Adamson, P.; Borcean, M.; Bortoletto, D.; Bridges, K.; Carman, S.P.; Chattopadhyay, S.; Coleman, J.; Curfman, N.M.; DeRose, K.; et al. Matter-wave Atomic Gradiometer Interferometric Sensor (MAGIS-100). Quantum Sci. Technol. 2021, 6, 44003. [Google Scholar] [CrossRef]
- Canuel, B.; Bertoldi, A.; Amand, L.; di Borgo, E.P.; Fang, B.; Geiger, R.; Gillot, J.; Henry, S.; Hinderer, J.; Holleville, D.; et al. Exploring gravity with the MIGA large scale atom interferometer. Sci. Rep. 2018, 8, 14064. [Google Scholar] [CrossRef] [PubMed]
- Canuel, B.; Abend, S.; Amaro-Seoane, P.; Badaracco, F.; Beaufils, Q.; Bertoldi, A.; Bongs, K.; Bouyer, P.; Braxmaier, C.; Chaibi, W.; et al. ELGAR—A European Laboratory for Gravitation and Atom-interferometric Research. Class. Quant. Grav. 2020, 37, 225017. [Google Scholar] [CrossRef]
- Zhan, M.S.; Wang, J.; Ni, W.T.; Gao, D.F.; Wang, G.; He, L.X.; Li, R.B.; Zhou, L.; Chen, X.; Zhong, J.Q.; et al. ZAIGA: Zhaoshan Long-baseline Atom Interferometer Gravitation Antenna. Int. J. Mod. Phys. D 2019, 29, 1940005. [Google Scholar] [CrossRef] [Green Version]
- Badurina, L.; Bentine, E.; Blas, D.; Bongs, K.; Bortoletto, D.; Bowcock, T.; Bridges, K.; Bowden, W.; Buchmueller, O.; Burrage, C.; et al. AION: An Atom Interferometer Observatory and Network. J. Cosmol. Astropart. Phys. 2020, 5, 11. [Google Scholar] [CrossRef]
- Badurina, L.; Buchmueller, O.; Ellis, J.; Lewicki, M.; McCabe, C.; Vaskonen, V. Prospective Sensitivities of Atom Interferometers to Gravitational Waves and Ultralight Dark Matter. arXiv 2021, arXiv:2108.02468. [Google Scholar] [CrossRef]
- El-Neaj, Y.A.; Alpigiani, C.; Amairi-Pyka, S.; Araújo, H.; Balaž, A.; Bassi, A.; Zupan, J. AEDGE: Atomic Experiment for Dark Matter and Gravity Exploration in Space. EPJ Quant. Technol. 2020, 7, 6. [Google Scholar] [CrossRef] [Green Version]
- Stadnik, Y.V.; Flambaum, V.V. Searching for dark matter and variation of fundamental constants with laser and maser interferometry. Phys. Rev. Lett. 2015, 114, 161301. [Google Scholar] [CrossRef] [Green Version]
- Arvanitaki, A.; Graham, P.W.; Hogan, J.M.; Rajendran, S.; Tilburg, K.V. Search for light scalar dark matter with atomic gravitational wave detectors. Phys. Rev. D 2018, 97, 075020. [Google Scholar] [CrossRef] [Green Version]
- Stadnik, Y.; Flambaum, V. Searching for dark matter with atomic clocks and laser interferometry. In Proceedings of the 2016 European Frequency and Time Forum (EFTF), York, UK, 4–7 April 2016. [Google Scholar] [CrossRef]
- Calmet, X. Hidden Sector and Gravity. Phys. Lett. B 2020, 801, 135152. [Google Scholar] [CrossRef]
- Perry, M.J. Tp Inversion in Quantum Gravity. Phys. Rev. D 1979, 19, 1720. [Google Scholar] [CrossRef]
- Gilbert, G. Wormhole induced proton decay. Nucl. Phys. B 1989, 328, 159–170. [Google Scholar] [CrossRef]
- Chen, Z.; Kobakhidze, A. Coloured gravitational instantons, the strong CP problem and the companion axion solution. arXiv 2021, arXiv:2108.05549. [Google Scholar]
- Calmet, X. On Searches for Gravitational Dark Matter with Quantum Sensors. Eur. Phys. J. Plus 2019, 134, 503. [Google Scholar] [CrossRef]
- Calmet, X.; Kuipers, F. Bounds on very weakly interacting ultra light scalar and pseudoscalar dark matter from quantum gravity. Eur. Phys. J. C 2020, 80, 781. [Google Scholar] [CrossRef]
- Calmet, X.; Kuipers, F. Theoretical bounds on dark matter masses. Phys. Lett. B 2021, 814, 136068. [Google Scholar] [CrossRef]
- Calmet, X.; Kuipers, F. Implications of quantum gravity for dark matter. Int. J. Mod. Phys. D 2021, 30, 2142004. [Google Scholar] [CrossRef]
- Ellis, J.R.; Gaillard, M.K. Fermion Masses and Higgs Representations in SU(5). Phys. Lett. B 1979, 88, 315–319. [Google Scholar] [CrossRef] [Green Version]
- Hill, C.T. Are There Significant Gravitational Corrections to the Unification Scale? Phys. Lett. B 1984, 135, 47–51. [Google Scholar] [CrossRef]
- Shafi, Q.; Wetterich, C. Modification of GUT Predictions in the Presence of Spontaneous Compactification. Phys. Rev. Lett. 1984, 52, 875. [Google Scholar] [CrossRef]
- Vayonakis, A. Planck Scale corrections to gauge coupling unification. Phys. Lett. B 1993, 307, 318–322. [Google Scholar] [CrossRef]
- Holman, R.; Hsu, S.D.H.; Kolb, E.W.; Watkins, R.; Widrow, L.M. Cosmological texture is incompatible with Planck scale physics. Phys. Rev. Lett. 1992, 69, 1489–1492. [Google Scholar] [CrossRef] [PubMed]
- Holman, R.; Hsu, S.D.H.; Kephart, T.W.; Kolb, E.W.; Watkins, R.; Widrow, L.M. Solutions to the strong CP problem in a world with gravity. Phys. Lett. B 1992, 282, 132–136. [Google Scholar] [CrossRef] [Green Version]
- Barr, S.M.; Seckel, D. Planck scale corrections to axion models. Phys. Rev. D 1992, 46, 539–549. [Google Scholar] [CrossRef]
- Ghigna, S.; Lusignoli, M.; Roncadelli, M. Instability of the invisible axion. Phys. Lett. B 1992, 283, 278–281. [Google Scholar] [CrossRef]
- Kamionkowski, M.; March-Russell, J. Planck scale physics and the Peccei-Quinn mechanism. Phys. Lett. B 1992, 282, 137–141. [Google Scholar] [CrossRef] [Green Version]
- Calmet, X.; Majee, S.K. Effective Theory for Dark Matter and a New Force in the Dark Matter Sector. Phys. Lett. B 2009, 679, 267–269. [Google Scholar] [CrossRef] [Green Version]
- Calmet, X.; Sanz, V. Excursion into Quantum Gravity via Inflation. Phys. Lett. B 2014, 737, 12–15. [Google Scholar] [CrossRef] [Green Version]
- Kallosh, R.; Linde, A.D.; Linde, D.A.; Susskind, L. Gravity and global symmetries. Phys. Rev. D 1995, 52, 912–935. [Google Scholar] [CrossRef] [Green Version]
- Kapner, D.J.; Cook, T.S.; Adelberger, E.G.; Gundlach, J.H.; Heckel, B.R.; Hoyle, C.D.; Swanson, H.E. Tests of the gravitational inverse-square law below the dark-energy length scale. Phys. Rev. Lett. 2007, 98, 21101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hoyle, C.D.; Kapner, D.J.; Heckel, B.R.; Adelberger, E.G.; Gundlach, J.H.; Schmidt, U.; Swanson, H.E. Sub-millimeter tests of the gravitational inverse-square law. Phys. Rev. D 2004, 70, 42004. [Google Scholar] [CrossRef] [Green Version]
- Adelberger, E.G.; Heckel, B.R.; Hoedl, S.A.; Hoyle, C.D.; Kapner, D.J.; Upadhye, A. Particle Physics Implications of a Recent Test of the Gravitational Inverse Sqaure Law. Phys. Rev. Lett. 2007, 98, 131104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lee, J.G.; Adelberger, E.G.; Cook, T.S.; Fleischer, S.M.; Heckel, B.R. New Test of the Gravitational 1/r2 Law at Separations down to 52 μm. Phys. Rev. Lett. 2020, 124, 101101. [Google Scholar] [CrossRef] [Green Version]
- Arkani-Hamed, N.; Dimopoulos, S.; Dvali, G.R. The Hierarchy problem and new dimensions at a millimeter. Phys. Lett. B 1998, 429, 263–272. [Google Scholar] [CrossRef] [Green Version]
- Antoniadis, I.; Arkani-Hamed, N.; Dimopoulos, S.; Dvali, G.R. New dimensions at a millimeter to a Fermi and superstrings at a TeV. Phys. Lett. B 1998, 436, 257–263. [Google Scholar] [CrossRef] [Green Version]
- Randall, L.; Sundrum, R. A Large mass hierarchy from a small extra dimension. Phys. Rev. Lett. 1999, 83, 3370–3373. [Google Scholar] [CrossRef] [Green Version]
- Calmet, X.; Hsu, S.D.H.; Reeb, D. Quantum gravity at a TeV and the renormalization of Newton’s constant. Phys. Rev. D 2008, 77, 125015. [Google Scholar] [CrossRef] [Green Version]
- Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Ambrogi, F.; Asilar, E.; Bergauer, T.; Novaes, S.F. Search for black holes and sphalerons in high-multiplicity final states in proton-proton collisions at = 13 TeV. J. High Energy Phys. 2018, 11, 42. [Google Scholar] [CrossRef] [Green Version]
- Touboul, P.; Métris, G.; Rodrigues, M.; André, Y.; Baghi, Q.; Bergé, J.; Visser, P. Space test of the Equivalence Principle: First results of the MICROSCOPE mission. Class. Quant. Grav. 2019, 36, 225006. [Google Scholar] [CrossRef] [Green Version]
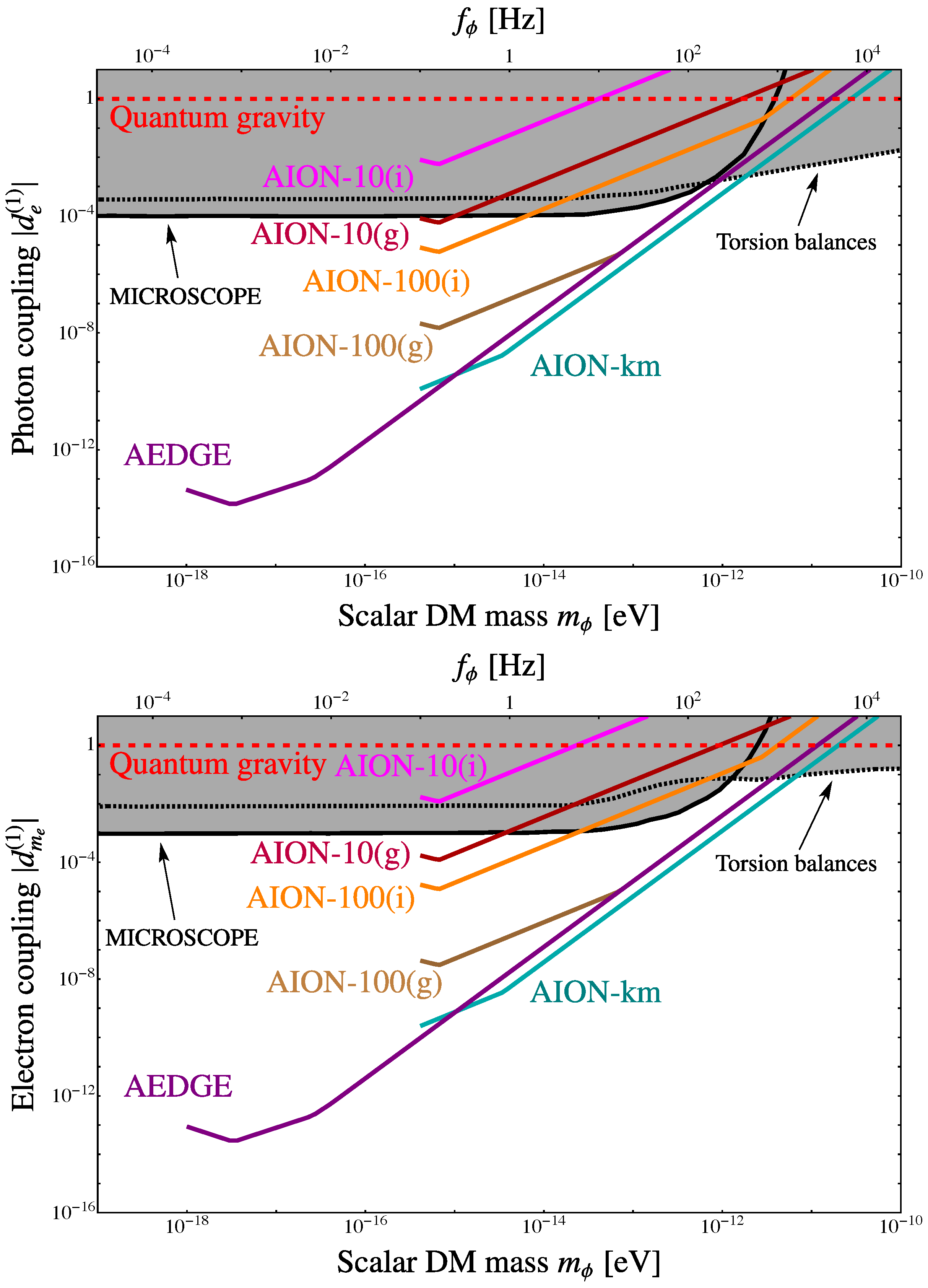
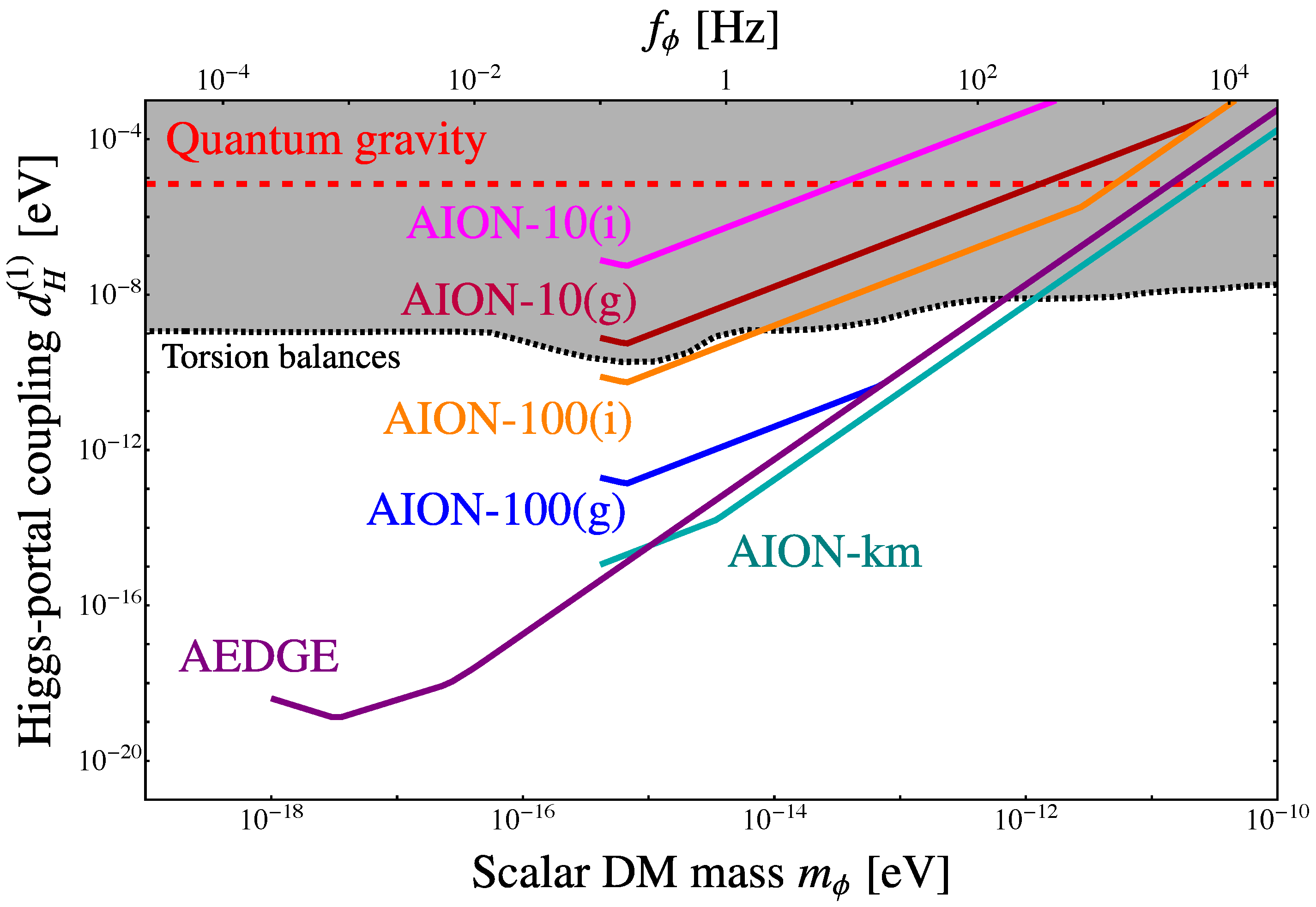
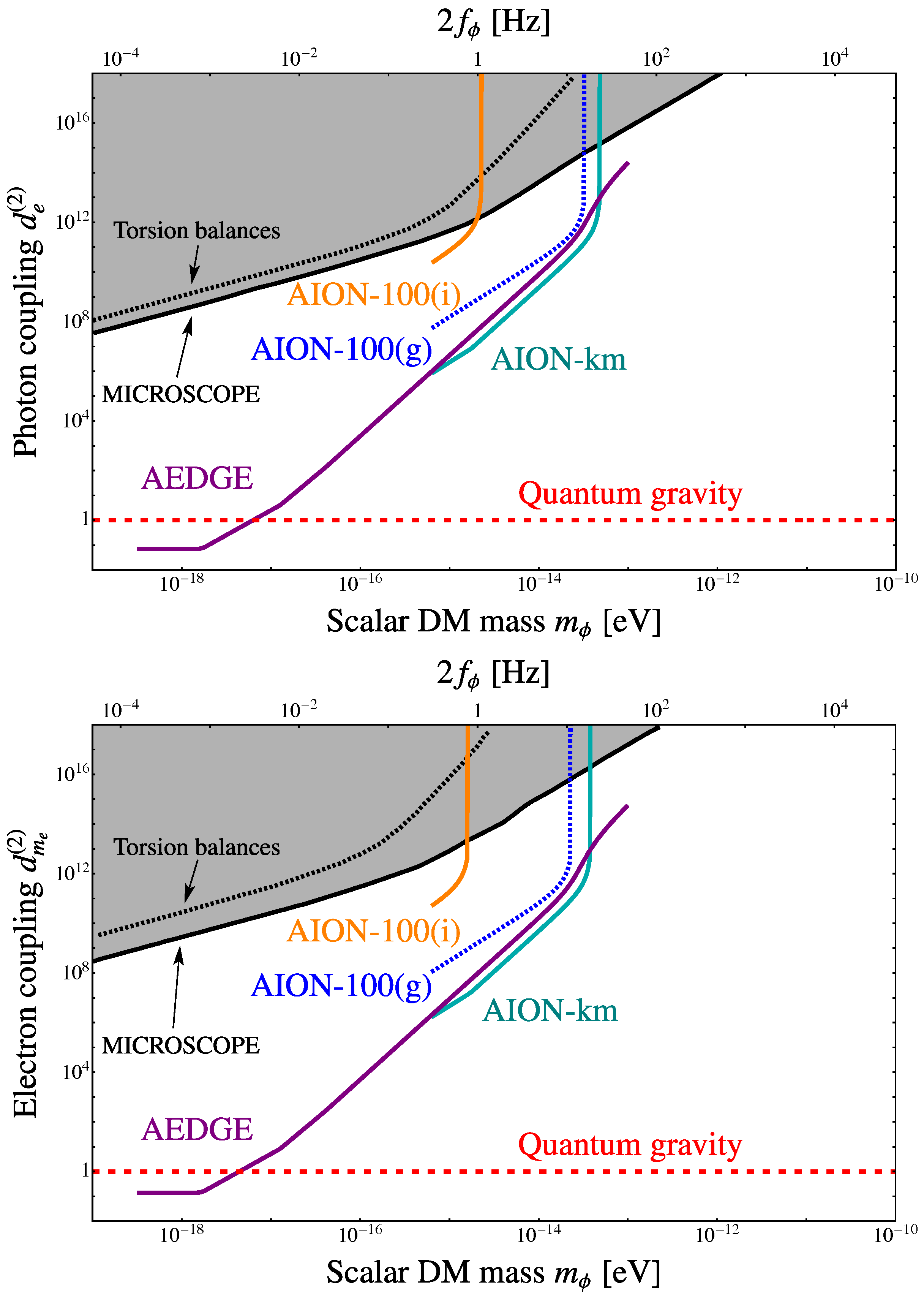
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Calmet, X.; Sherrill, N. Implications of Quantum Gravity for Dark Matter Searches with Atom Interferometers. Universe 2022, 8, 103. https://doi.org/10.3390/universe8020103
Calmet X, Sherrill N. Implications of Quantum Gravity for Dark Matter Searches with Atom Interferometers. Universe. 2022; 8(2):103. https://doi.org/10.3390/universe8020103
Chicago/Turabian StyleCalmet, Xavier, and Nathaniel Sherrill. 2022. "Implications of Quantum Gravity for Dark Matter Searches with Atom Interferometers" Universe 8, no. 2: 103. https://doi.org/10.3390/universe8020103
APA StyleCalmet, X., & Sherrill, N. (2022). Implications of Quantum Gravity for Dark Matter Searches with Atom Interferometers. Universe, 8(2), 103. https://doi.org/10.3390/universe8020103






