Thermal Quantum Correlations in Two Gravitational Cat States
Abstract
1. Introduction
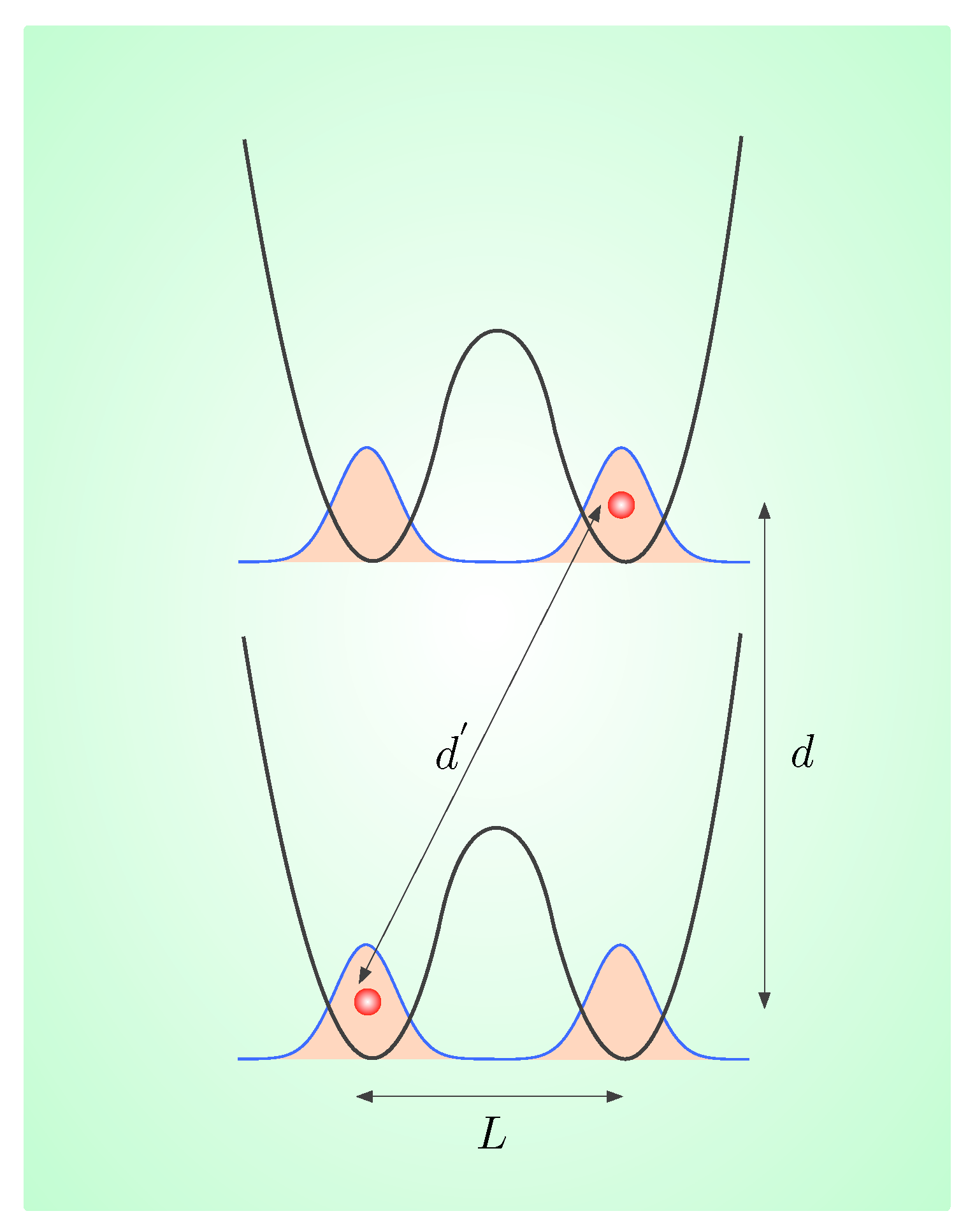
2. The Model
Thermal Density Operator
3. Thermal Quantum Correlations
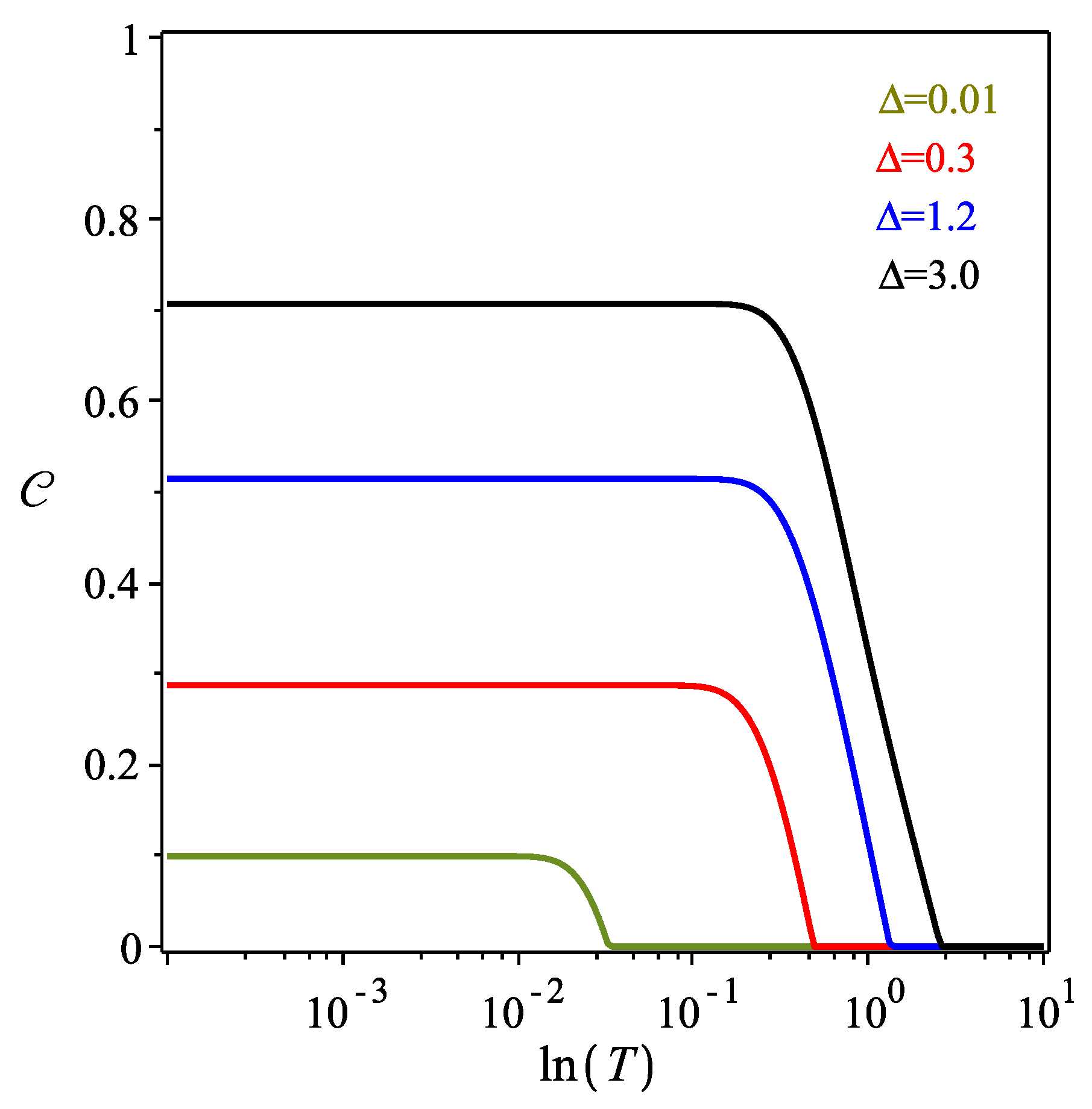
3.1. Thermal Entanglement
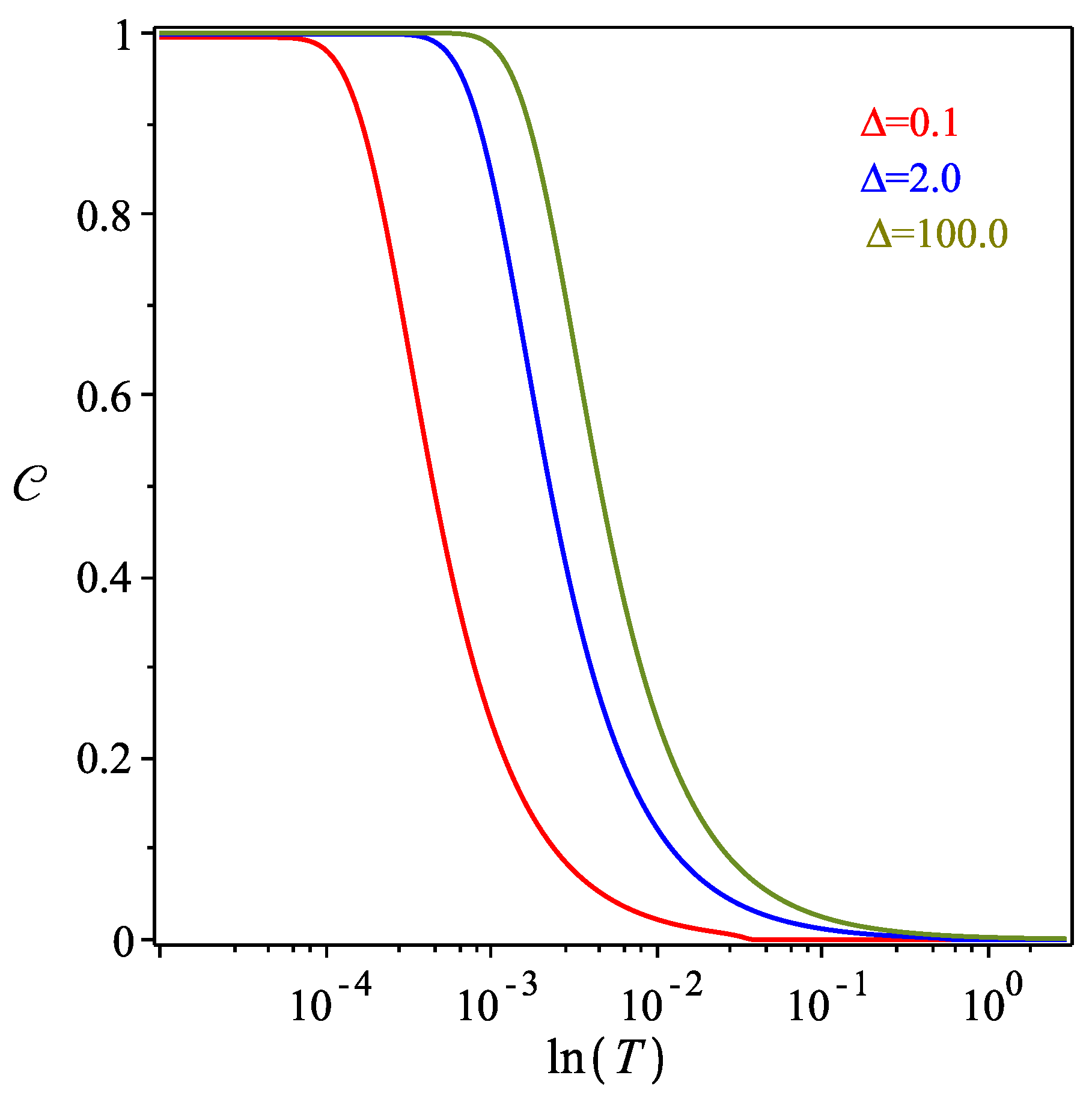
3.2. Quantum Coherence
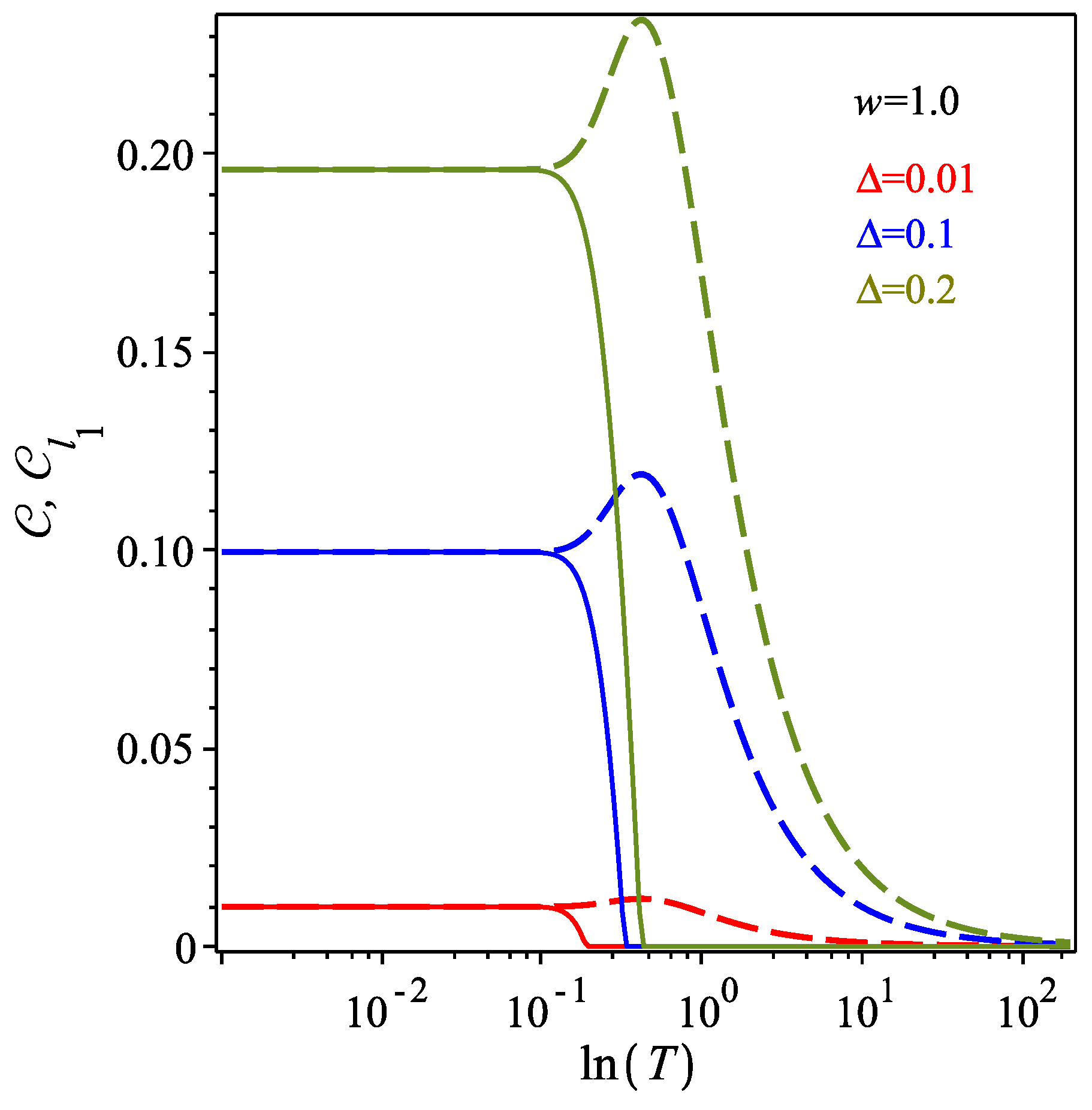
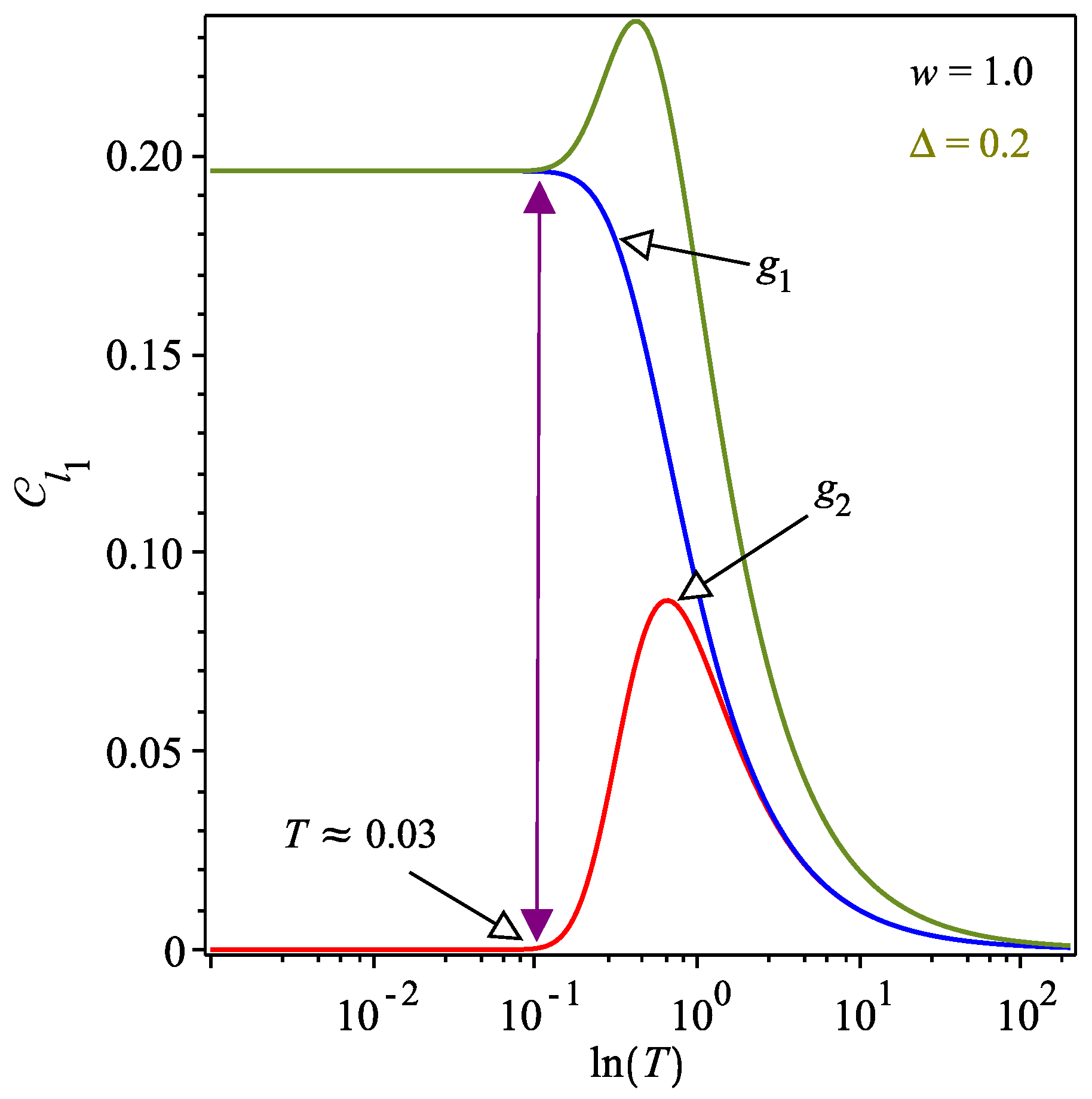
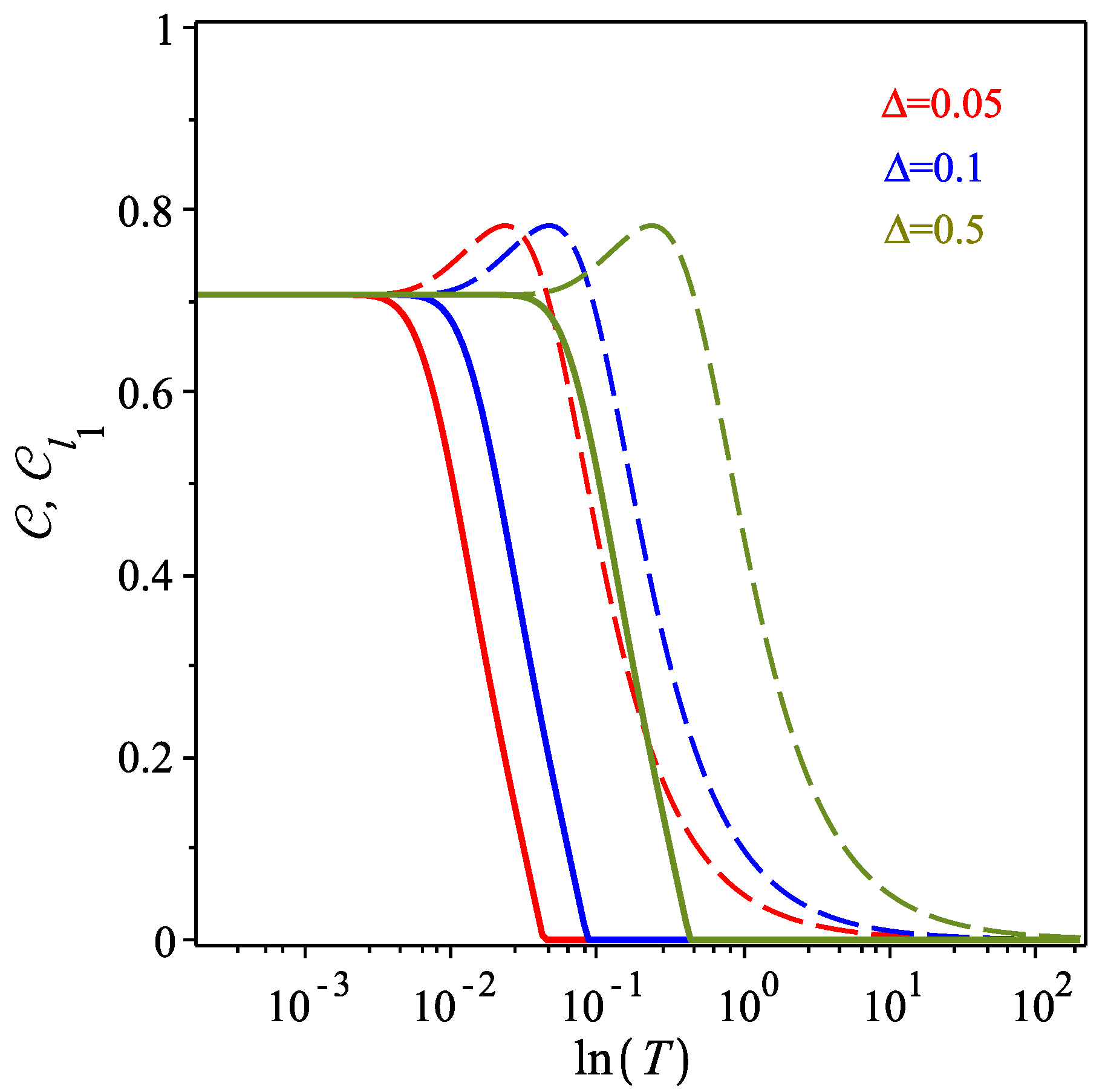
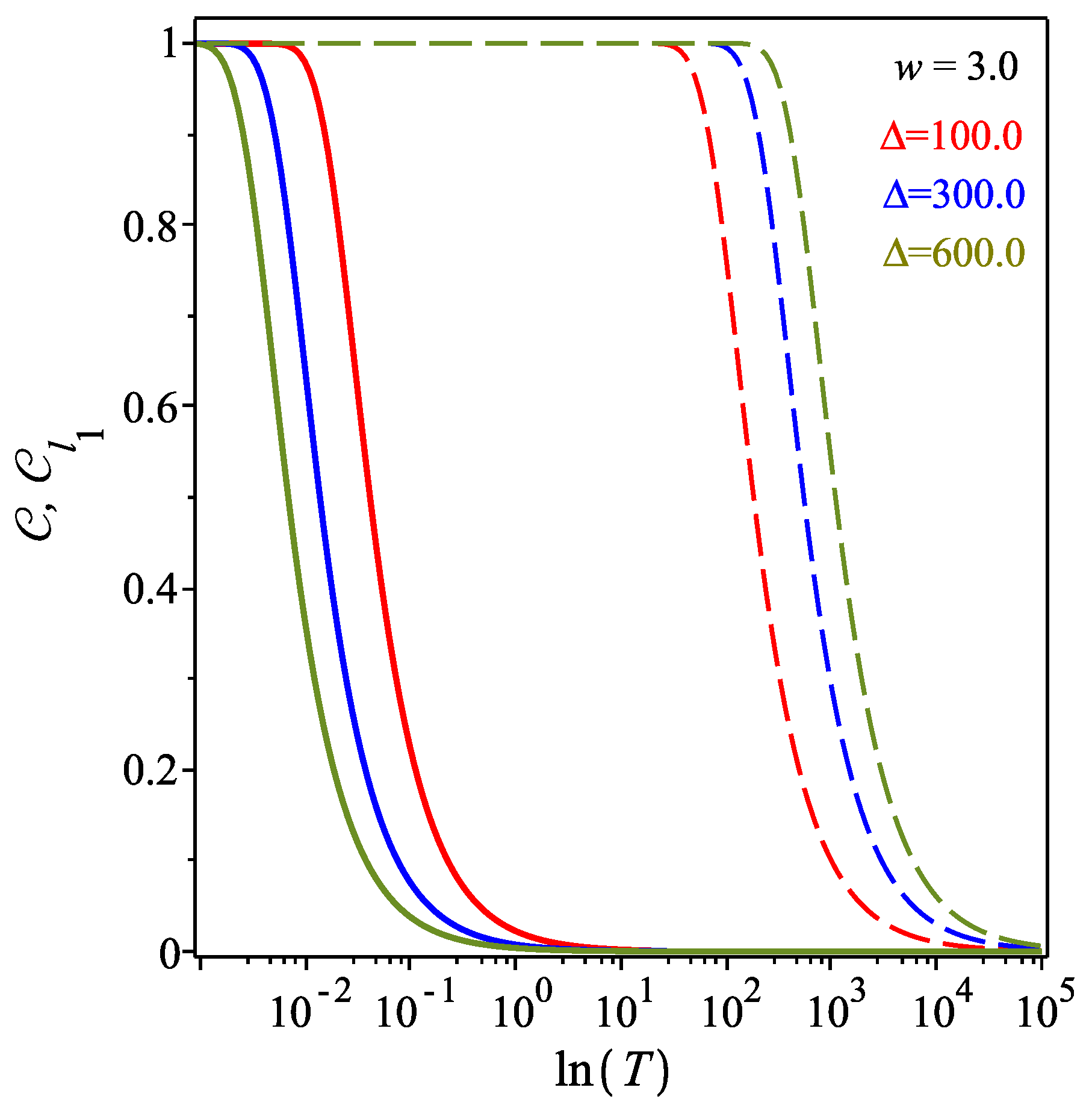
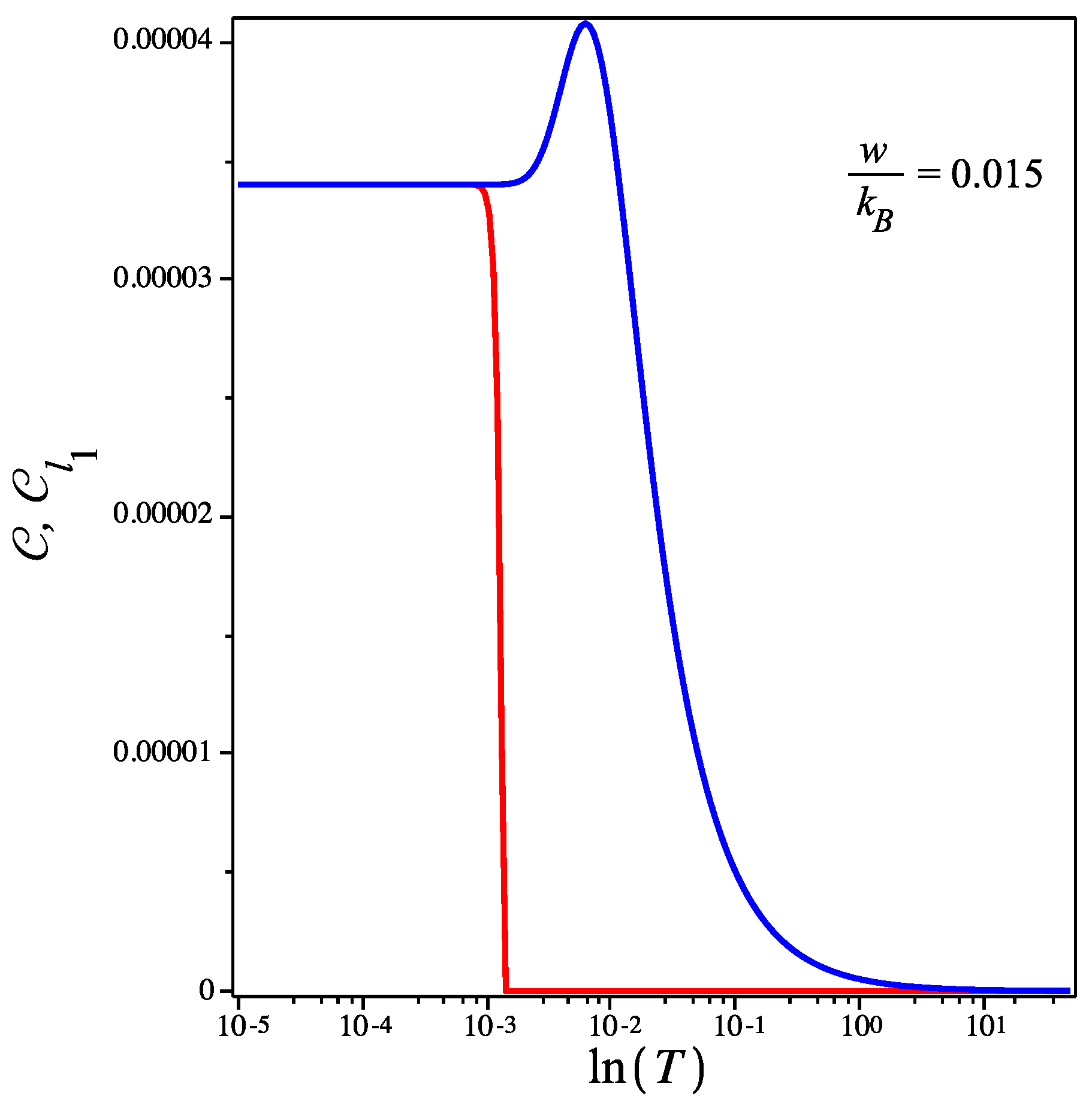
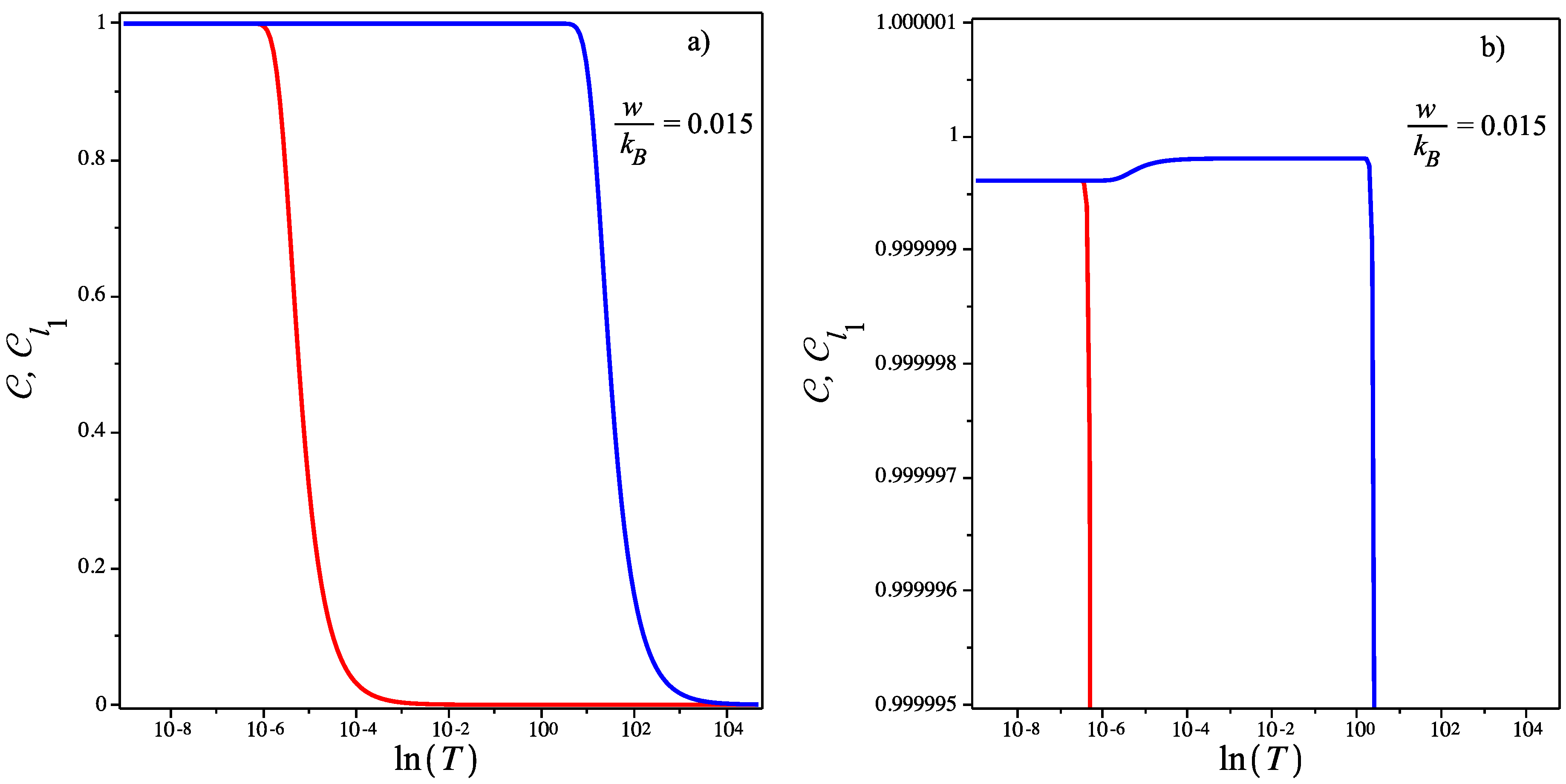
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
| 1 | We assume . |
References
- Kiefer, C. Quantum Gravity, 2nd ed.; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Rovelli, C. Loop quantum gravity. Living Rev. Rel. 1998, 1, 1–75. [Google Scholar] [CrossRef] [PubMed]
- Mukhi, S. String theory: A perspective over the last 25 years. Class. Quant. Grav. 2011, 28, 153001–153033. [Google Scholar] [CrossRef]
- Ambjorn, J.; Jurkiewicz, J.; Loll, R. Causal Dynamical Triangulations and the Quest for Quantum Gravity. arXiv 2010, arXiv:1004.0352. [Google Scholar]
- Amelino-Camelia, G. Quantum-Spacetime Phenomenology. Living Rev. Rel. 2013, 16, 5–141. [Google Scholar]
- Addazi, A.; Alvarez-Muniz, J.; Batista, R.A.; Amelino-Camelia, G.; Antonelli, V.; Arzano, M.; Asorey, M.; Atteia, J.L.; Bahamonde, S.; Bajardi, F.; et al. Quantum gravity phenomenology at the dawn of the multi-messenger era—A review. Prog. Part. Nucl. Phys. 2022, 125, 103948–104066. [Google Scholar]
- Acciari, V.A. [MAGIC, Armenian Consortium: ICRANet-Armenia at NAS RA, A. Alikhanyan National Laboratory and Finnish MAGIC Consortium: Finnish Centre of Astronomy with ESO], Bounds on Lorentz invariance violation from MAGIC observation of GRB 190114C. Phys. Rev. Lett. 2020, 125, 021301–021307. [Google Scholar] [CrossRef]
- Addazi, A.; Marciano, A.; Yunes, N. Can we probe Planckian corrections at the horizon scale with gravitational waves? Phys. Rev. Lett. 2019, 122, 081301–081306. [Google Scholar] [CrossRef]
- Arzano, M.; Kowalski-Glikman, J.; Wislicki, W. A bound on Planck-scale deformations of CPT from muon lifetime. Phys. Lett. B 2019, 794, 41–44. [Google Scholar] [CrossRef]
- Lobo, I.P.; Pfeifer, C. Reaching the Planck scale with muon lifetime measurements. Phys. Rev. D 2021, 103, 106025–106032. [Google Scholar] [CrossRef]
- Carney, D.; Stamp, P.C.E.; Taylor, J.M. Tabletop experiments for quantum gravity: A user’s manual. Class. Quant. Grav. 2019, 36, 034001–034034. [Google Scholar] [CrossRef]
- Addazi, A.; Belli, P.; Bernabei, R.; Marciano, A. Testing noncommutative spacetimes and violations of the Pauli Exclusion Principle through underground experiments. Chin. Phys. C 2018, 42, 094001–094008. [Google Scholar] [CrossRef]
- Marletto, C.; Vedral, V. Why we need to quantise everything, including gravity. NPJ Quantum Inf. 2017, 3, 29–33. [Google Scholar] [CrossRef]
- Marletto, C.; Vedral, V. Gravitationally-induced entanglement between two massive particles is sufficient evidence of quantum effects in gravity. Phys. Rev. Lett. 2017, 119, 240402–240406. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Morley, G.W.; Ulbricht, H.; Toroš, M.; Paternostro, M.; Geraci, A.; Barker, P.; Kim, M.S.; Milburn, G. Spin Entanglement Witness for Quantum Gravity. Phys. Rev. Lett. 2017, 119, 240401–240406. [Google Scholar] [CrossRef]
- Rovelli, C. Considerations on Quantum Gravity Phenomenology. Universe 2021, 7, 439–443. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Schut, M.; Toroš, M. Two mechanisms for quantum natured gravitons to entangle masses. Phys. Rev. D 2022, 105, 106028–106040. [Google Scholar] [CrossRef]
- Christodoulou, M.; Rovelli, C. On the possibility of laboratory evidence for quantum superposition of geometries. Phys. Lett. B 2019, 792, 64–68. [Google Scholar] [CrossRef]
- Christodoulou, M.; Rovelli, C. On the possibility of experimental detection of the discreteness of time. Front. Phys. 2020, 8, 207–211. [Google Scholar] [CrossRef]
- Hall, M.J.W.; Reginatto, M. On two recent proposals for witnessing nonclassical gravity. J. Phys. A 2018, 51, 085303–085317. [Google Scholar] [CrossRef]
- Reginatto, M.; Hall, M.J.W. Entanglement of quantum fields via classical gravity. arXiv 2018, arXiv:1809.04989. [Google Scholar]
- Anastopoulos, C.; Hu, B.L. Quantum Superposition of Two Gravitational Cat States. Class. Quant. Grav. 2020, 37, 235012–235035. [Google Scholar] [CrossRef]
- Rijavec, S.; Carlesso, M.; Bassi, A.; Vedral, V.; Marletto, C. Decoherence effects in non-classicality tests of gravity. New J. Phys. 2021, 23, 043040–043054. [Google Scholar] [CrossRef]
- Di Bartolomeo, G.; Carlesso, M.; Bassi, A. Gravity as a classical channel and its dissipative generalization. Phys. Rev. D 2021, 104, 104027–104037. [Google Scholar] [CrossRef]
- Nguyen, H.C.; Bernards, F. Entanglement dynamics of two mesoscopic objects with gravitational interaction. Eur. Phys. J. D 2020, 74, 69–73. [Google Scholar] [CrossRef]
- Miki, D.; Matsumura, A.; Yamamoto, K. Entanglement and decoherence of massive particles due to gravity. Phys. Rev. D 2021, 103, 026017–026028. [Google Scholar] [CrossRef]
- Qvarfort, S.; Bose, S.; Serafini, A. Mesoscopic entanglement through central–potential interactions. J. Phys. B 2020, 53, 235501–235508. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Crepeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef]
- Bennett, C.H.; Bernstein, H.J.; Popescu, S.; Schumacher, B. Concentrating partial entanglement by local operations. Phys. Rev. A 1996, 53, 2046–2052. [Google Scholar] [CrossRef]
- Amico, L.; Fazio, R.; Osterloh, A.; Vedral, V. Entanglement in many-body systems. Rev. Mod. Phys. 2008, 80, 517–576. [Google Scholar] [CrossRef]
- Li, K.; Li, Y.; Han, M.; Lu, S.; Zhou, J.; Ruan, D.; Long, G.; Wan, Y.; Lu, D.; Zeng, B.; et al. Quantum Spacetime on a Quantum Simulator. Commun. Phys. 2019, 2, 122–127. [Google Scholar] [CrossRef]
- Czelusta, G.; Mielczarek, J. Quantum simulations of a qubit of space. Phys. Rev. D 2021, 103, 046001–046017. [Google Scholar] [CrossRef]
- Streltsov, A.; Adesso, G.; Plenio, M.B. Quantum Coherence as a Resource. Rev. Mod. Phys. 2017, 89, 041003–041036. [Google Scholar] [CrossRef]
- Baumgratz, T.; Cramer, M.; Plenio, M.B. Quantifying Coherence. Phys. Rev. Lett. 2014, 113, 140401–140405. [Google Scholar] [CrossRef]
- Fröwis, F.; Dür, W. Stable macroscopic quantum superpositions. Phys. Rev. Lett. 2011, 106, 110402–110405. [Google Scholar] [CrossRef]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum-enhanced measurements: Beating the standard quantum limit. Science 2004, 306, 1330–1336. [Google Scholar] [CrossRef] [PubMed]
- Santos, J.P.; Céleri, L.C.; Landi, G.T.; Paternostro, M. The role of quantum coherence in non-equilibrium entropy production. Nat. Quant. Inf. 2019, 5, 23–29. [Google Scholar] [CrossRef]
- Lostaglio, M.; Jennings, D.; Rudolph, T. Description of quantum coherence in thermodynamic processes requires constraints beyond free energy. Nat. Commun. 2015, 6, 6383–6391. [Google Scholar] [CrossRef] [PubMed]
- Petruzziello, L.; Illuminati, F. Quantum gravitational decoherence from fluctuating minimal length and deformation parameter at the Planck scale. Nat. Commun. 2021, 12, 4449–4450. [Google Scholar] [CrossRef]
- Arzano, M.; D’Esposito, V.; Gubitosi, G. Fundamental decoherence from quantum spacetime. arXiv 2022, arXiv:2208.14119. [Google Scholar]
- Glauber, R.J. Coherent and incoherent states of the radiation field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Sudarshan, E.C.G. Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams. Phys. Rev. Lett. 1963, 10, 277–279. [Google Scholar] [CrossRef]
- Streltsov, A.; Singh, U.; Dhar, H.S.; Bera, M.N.; Adesso, G. Measuring Quantum Coherence with Entanglement. Phys. Rev. Lett. 2015, 115, 020403–020408. [Google Scholar] [CrossRef] [PubMed]
- Kammerlander, P.; Anders, J. Coherence and measurement in quantum thermodynamics. Sci. Rep. 2016, 6, 22174–22180. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Wang, X.; Zeng, Q.; Wang, J. Conditional Entropy Production and Quantum Fluctuation Theorem of Dissipative Information: Theory and Experiments. PRX Quantum 2022, 3, 030315. [Google Scholar] [CrossRef]
- Filgueiras, C.; Rojas, O.; Rojas, M. Thermal Entanglement and Correlated Coherence in Two Coupled Double Quantum Dots Systems. Ann. Der Phys. 2020, 532, 2000207–2000213. [Google Scholar] [CrossRef]
- Berrada, K. Quantum coherence in quantum dot systems. Phys. E 2020, 116, 113784–113788. [Google Scholar] [CrossRef]
- Sha, Y.-T.; Wang, Y.; Sun, Z.-H.; Hou, X.-W. Thermal quantum coherence and correlation in extended XY spin chain. Ann. Phys. 2018, 392, 229–241. [Google Scholar] [CrossRef]
- Freitas, M.; Filgueiras, C.; Rojas, M. The effects of an impurity in an Ising-XXZ diamond chain on thermal entanglement, on quantum coherence and on quantum teleportation. Ann. Der Phys. 2019, 531, 1900261–1900270. [Google Scholar] [CrossRef]
- Arian zad, H.; Rojas, M. Quantum coherence, quantum Fisher information and teleportation in the Ising-Heisenberg spin chain model of a heterotrimetallic Fe-Mn-Cu coordination polymer with magnetic impurity. Phys. Low-Dimens. Syst. Nanostruct. 2021, 126, 114455. [Google Scholar] [CrossRef]
- Ullah, K.; Köse, E.; Yagan, R.; Onbasli, M.C.; Müstecaplioglu, O.E. Steady state entanglement of distant nitrogen-vacancy centers in a coherent thermal magnon bath. Phys. Rev. Res. 2022, 4, 023221–023243. [Google Scholar] [CrossRef]
- Shi, H.-L.; Ding, S.; Wan, Q.-K.; Wang, X.-H.; Yang, W.-L. Entanglement, Coherence, and Extractable Work in Quantum Batteries. Phys. Rev. Lett. 2022, 129, 130602–130607. [Google Scholar] [CrossRef]
- Ahnefeld, F.; Theurer, T.; Egloff, D.; Matera, J.M.; Plenio, M.B. Coherence as a Resource for Shor’s Algorithm. Phys. Rev. Lett. 2022, 129, 120501–120507. [Google Scholar] [CrossRef] [PubMed]
- Sk, R.; Panigrahi, P.K. Protecting quantum coherence and entanglement in a correlated environment. Phys. A 2022, 596, 127129–140441. [Google Scholar] [CrossRef]
- Schlosshauer, M. Decoherence and the Quantum to Classical Transition, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Breuer, H.-P.; Petruccione, F. The Theory of Open Quantum Systems, 1st ed.; Oxford University Press: New York, NY, USA, 2003. [Google Scholar]
- Carlesso, M.; Bassi, A.; Paternostro, M.; Ulbricht, H. Testing the gravitational field generated by a quantum superposition. New J. Phys. 2019, 21, 093052–093060. [Google Scholar] [CrossRef]
- Wootters, W.K. Entanglement of Formation of an Arbitrary State of Two Qubits. Phys. Rev. Lett. 1998, 80, 2245–2248. [Google Scholar] [CrossRef]
- Hill, S.; Wootters, W.K. Entanglement of a Pair of Quantum Bits. Phys. Rev. Lett. 1997, 78, 5022–5025. [Google Scholar] [CrossRef]
- Tan, K.C.; Kwon, H.; Park, C.-Y.; Jeong, H. Unified view of quantum correlations and quantum coherence. Phys. Rev. A 2016, 94, 022329–022336. [Google Scholar] [CrossRef]
- Krisnanda, T.; Tham, G.Y.; Paternostro, M.; Paterek, P. Observable quantum entanglement due to gravity. npj Quantum Inf. 2020, 6, 12–17. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rojas, M.; Lobo, I.P. Thermal Quantum Correlations in Two Gravitational Cat States. Universe 2023, 9, 71. https://doi.org/10.3390/universe9020071
Rojas M, Lobo IP. Thermal Quantum Correlations in Two Gravitational Cat States. Universe. 2023; 9(2):71. https://doi.org/10.3390/universe9020071
Chicago/Turabian StyleRojas, Moises, and Iarley P. Lobo. 2023. "Thermal Quantum Correlations in Two Gravitational Cat States" Universe 9, no. 2: 71. https://doi.org/10.3390/universe9020071
APA StyleRojas, M., & Lobo, I. P. (2023). Thermal Quantum Correlations in Two Gravitational Cat States. Universe, 9(2), 71. https://doi.org/10.3390/universe9020071






