Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism
Abstract
:1. Introduction
2. Relativistic Stars in General Relativity
3. Vanishing Complexity Factor Formalism
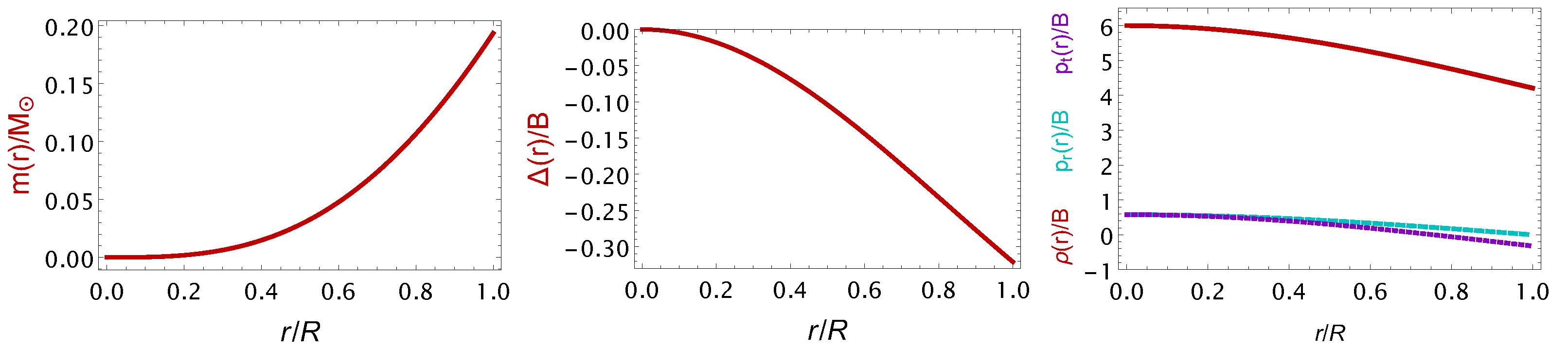
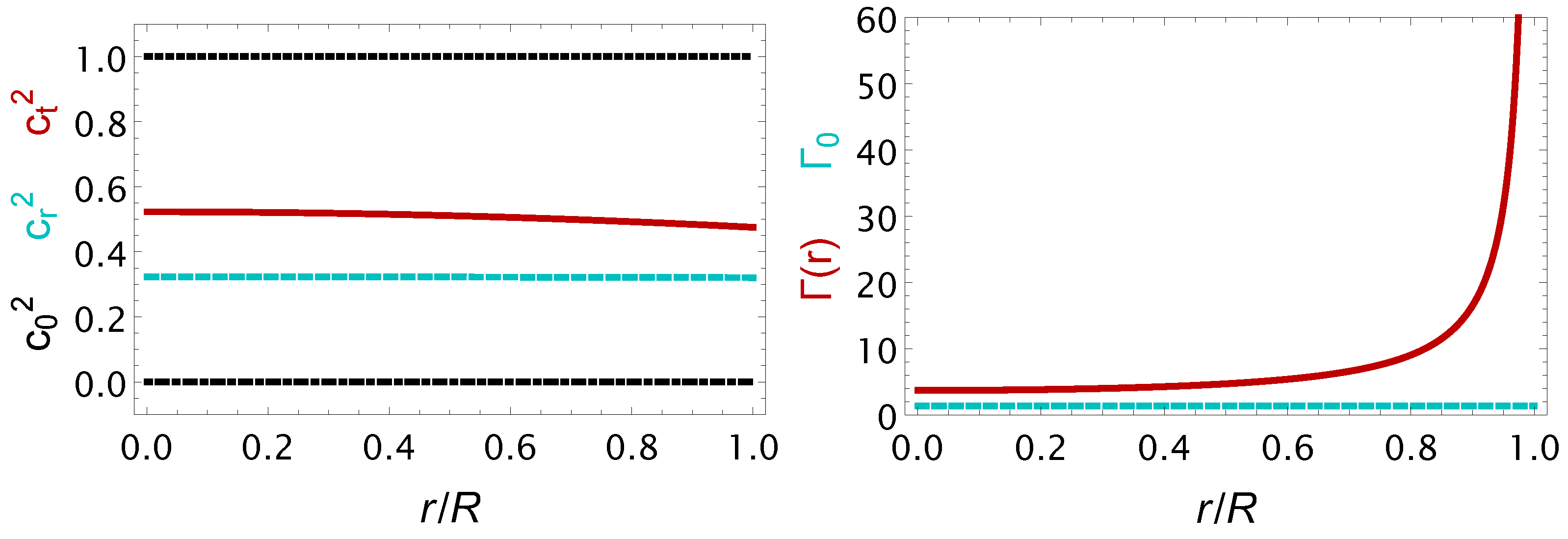
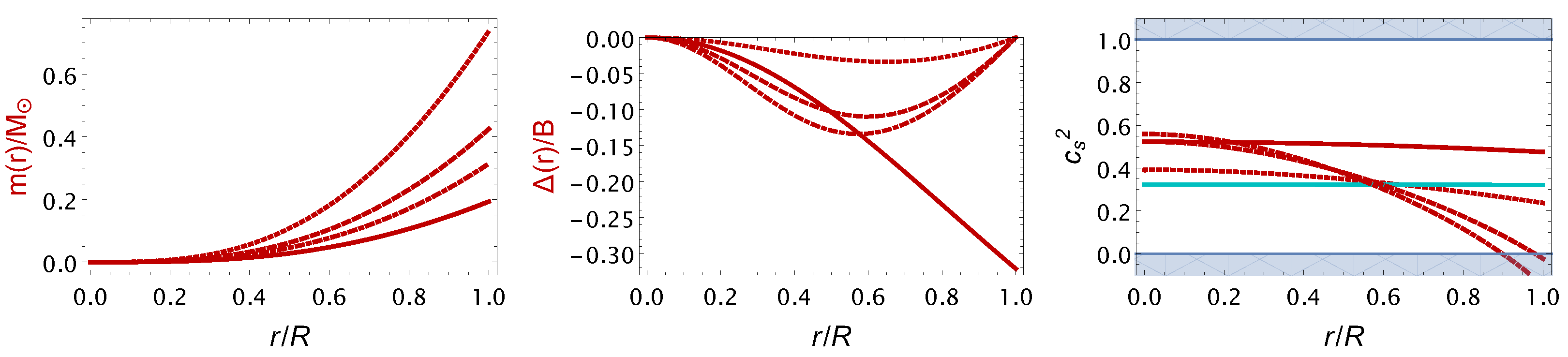
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs, and Neutron Stars: The Physics of Compact Objects; John Wiley & Sons: Hoboken, NJ, USA, 1983. [Google Scholar]
- Psaltis, D. Probes and Tests of Strong-Field Gravity with Observations in the Electromagnetic Spectrum. Living Rev. Rel. 2008, 11, 9. [Google Scholar] [CrossRef] [Green Version]
- Lorimer, D.R. Binary and Millisecond Pulsars. Living Rev. Rel. 2008, 11, 8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zorotovic, M.; Schreiber, M.R. Cataclysmic variable evolution and the white dwarf mass problem: A Review. Adv. Space Res. 2020, 66, 1080–1089. [Google Scholar] [CrossRef] [Green Version]
- Ruiter, A.J. Type Ia supernova sub-classes and progenitor origin. IAU Symp. 2020, 357, 1–15. [Google Scholar] [CrossRef]
- Burrows, A.; Vartanyan, D. Core-collapse supernova explosion theory. Nature 2021, 589, 29–39. [Google Scholar] [CrossRef]
- Einstein, A. The Field Equations of Gravitation. Sitzungsber. Preuss. Akad. Wiss. Berl. (Math. Phys.) 1915, 1915, 844–847. [Google Scholar]
- Mösta, P.; Ott, C.D.; Radice, D.; Roberts, L.F.; Schnetter, E.; Haas, R. A large-scale dynamo and magnetoturbulence in rapidly rotating core-collapse supernovae. Nature 2015, 528, 376–379. [Google Scholar] [CrossRef] [Green Version]
- Guilet, J.; Müller, E. Numerical simulations of the magnetorotational instability in protoneutron stars—I. Influence of buoyancy. Mon. Not. R. Astron. Soc. 2015, 450, 2153–2171. [Google Scholar] [CrossRef]
- Kondratyev, V.N. R-Process with Magnetized Nuclei at Dynamo-Explosive Supernovae and Neutron Star Mergers. Universe 2021, 7, 487. [Google Scholar] [CrossRef]
- Alcock, C.; Farhi, E.; Olinto, A. Strange stars. Astrophys. J. 1986, 310, 261–272. [Google Scholar] [CrossRef]
- Alcock, C.; Olinto, A. Exotic Phases of Hadronic Matter and their Astrophysical Application. Ann. Rev. Nucl. Part. Sci. 1988, 38, 161–184. [Google Scholar] [CrossRef]
- Madsen, J. Physics and astrophysics of strange quark matter. Lect. Notes Phys. 1999, 516, 162–203. [Google Scholar] [CrossRef] [Green Version]
- Weber, F. Strange quark matter and compact stars. Prog. Part. Nucl. Phys. 2005, 54, 193–288. [Google Scholar] [CrossRef] [Green Version]
- Yue, Y.L.; Cui, X.H.; Xu, R.X. Is psr b0943+10 a low-mass quark star? Astrophys. J. Lett. 2006, 649, L95–L98. [Google Scholar] [CrossRef] [Green Version]
- Leahy, D.; Ouyed, R. Supernova SN2006gy as a first ever Quark Nova? Mon. Not. R. Astron. Soc. 2008, 387, 1193. [Google Scholar] [CrossRef] [Green Version]
- Witten, E. Cosmic Separation of Phases. Phys. Rev. D 1984, 30, 272–285. [Google Scholar] [CrossRef]
- Farhi, E.; Jaffe, R.L. Strange Matter. Phys. Rev. D 1984, 30, 2379. [Google Scholar] [CrossRef]
- Henderson, J.A.; Page, D. RX J1856.5-3754 as a possible Strange Star candidate. Astrophys. Space Sci. 2007, 308, 513–517. [Google Scholar] [CrossRef] [Green Version]
- Li, A.; Peng, G.X.; Lu, J.F. Strange star candidates revised within a quark model with chiral mass scaling. Res. Astron. Astrophys. 2011, 11, 482–490. [Google Scholar] [CrossRef]
- Aziz, A.; Ray, S.; Rahaman, F.; Khlopov, M.; Guha, B.K. Constraining values of bag constant for strange star candidates. Int. J. Mod. Phys. D 2019, 28, 1941006. [Google Scholar] [CrossRef] [Green Version]
- Benic, S.; Blaschke, D.; Alvarez-Castillo, D.E.; Fischer, T.; Typel, S. A new quark-hadron hybrid equation of state for astrophysics—I. High-mass twin compact stars. Astron. Astrophys. 2015, 577, A40. [Google Scholar] [CrossRef] [Green Version]
- Yazdizadeh, T.; Bordbar, G.; Panah, B.E. The structure of hybrid neutron star in Einstein-λ gravity. Phys. Dark Universe 2022, 35, 100982. [Google Scholar] [CrossRef]
- Eslam Panah, B.; Yazdizadeh, T.; Bordbar, G.H. Contraction of cold neutron star due to in the presence a quark core. Eur. Phys. J. C 2019, 79, 815. [Google Scholar] [CrossRef]
- Jaikumar, P.; Reddy, S.; Steiner, A.W. The Strange star surface: A Crust with nuggets. Phys. Rev. Lett. 2006, 96, 041101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ofek, E.O.; Cameron, P.; Kasliwal, M.; Gal-Yam, A.; Rau, A.; Kulkarni, S.; Frail, D.; Chandra, P.; Cenko, S.; Soderberg, A.; et al. SN 2006gy: An extremely luminous supernova in the early-type galaxy NGC 1260. Astrophys. J. Lett. 2007, 659, L13–L16. [Google Scholar] [CrossRef]
- Ouyed, R.; Leahy, D.; Jaikumar, P. Predictions for signatures of the quark-nova in superluminous supernovae. arXiv 2009, arXiv:0911.5424. [Google Scholar]
- Mukhopadhyay, P.; Schaffner-Bielich, J. Quark stars admixed with dark matter. Phys. Rev. D 2016, 93, 083009. [Google Scholar] [CrossRef] [Green Version]
- Panotopoulos, G.; Lopes, I. Radial oscillations of strange quark stars admixed with fermionic dark matter. Phys. Rev. D 2018, 98, 083001. [Google Scholar] [CrossRef]
- Ruderman, M. Pulsars: Structure and dynamics. Ann. Rev. Astron. Astrophys. 1972, 10, 427–476. [Google Scholar] [CrossRef]
- Bowers, R.L.; Liang, E.P.T. Anisotropic Spheres in General Relativity. Astrophys. J. 1974, 188, 657–665. [Google Scholar] [CrossRef]
- Weber, F. Pulsars as Astrophysical Laboratories for Nuclear and Particle Physics; Routledge: New York, NY, USA, 2017. [Google Scholar]
- Bordbar, G.H.; Karami, M. Anisotropic magnetized neutron star. Eur. Phys. J. C 2022, 82, 74. [Google Scholar] [CrossRef]
- Sokolov, A.I. Universal effective coupling constants for the generalized Heisenberg model. Fiz. Tverd. Tela 1998, 40, 1284. [Google Scholar] [CrossRef] [Green Version]
- Sawyer, R.F. Condensed pi- phase in neutron star matter. Phys. Rev. Lett. 1972, 29, 382–385. [Google Scholar] [CrossRef]
- Herrera, L.; Santos, N.O. Local anisotropy in self-gravitating systems. Phys. Rep. 1997, 286, 53–130. [Google Scholar] [CrossRef]
- Barreto, W.; Rojas, S. An Equation of State for Radiating Dissipative Spheres in General Relativity. Astrophys. Space Sci. 1992, 193, 201–215. [Google Scholar] [CrossRef]
- Letelier, P.S. Anisotropic fluids with two-perfect-fluid components. Phys. Rev. D 1980, 22, 807–813. [Google Scholar] [CrossRef]
- Kippenhahn, R.; Weigert, A.; Weiss, A. Stellar Structure and Evolution; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 9783642303043. [Google Scholar] [CrossRef]
- Mak, M.K.; Harko, T. An exact anisotropic quark star model. Chin. J. Astron. Astrophys. 2002, 2, 248–259. [Google Scholar] [CrossRef]
- Deb, D.; Chowdhury, S.R.; Ray, S.; Rahaman, F.; Guha, B.K. Relativistic model for anisotropic strange stars. Ann. Phys. 2017, 387, 239–252. [Google Scholar] [CrossRef] [Green Version]
- Deb, D.; Roy Chowdhury, S.; Ray, S.; Rahaman, F. A New Model for Strange Stars. Gen. Rel. Grav. 2018, 50, 112. [Google Scholar] [CrossRef] [Green Version]
- Gabbanelli, L.; Rincón, A.; Rubio, C. Gravitational decoupled anisotropies in compact stars. Eur. Phys. J. C 2018, 78, 370. [Google Scholar] [CrossRef]
- Ovalle, J. Decoupling gravitational sources in general relativity: From perfect to anisotropic fluids. Phys. Rev. D 2017, 95, 104019. [Google Scholar] [CrossRef] [Green Version]
- Ovalle, J.; Casadio, R.; da Rocha, R.; Sotomayor, A. Anisotropic solutions by gravitational decoupling. Eur. Phys. J. C 2018, 78, 122. [Google Scholar] [CrossRef] [Green Version]
- Sharif, M.; Butt, I.I. Complexity Factor for Charged Spherical System. Eur. Phys. J. C 2018, 78, 688. [Google Scholar] [CrossRef]
- Sharif, M.; Butt, I.I. Complexity factor for static cylindrical system. Eur. Phys. J. C 2018, 78, 850. [Google Scholar] [CrossRef]
- Abbas, G.; Nazar, H. Complexity Factor For Anisotropic Source in Non-minimal Coupling Metric f(R) Gravity. Eur. Phys. J. C 2018, 78, 957. [Google Scholar] [CrossRef]
- Herrera, L.; Di Prisco, A.; Ospino, J. Complexity factors for axially symmetric static sources. Phys. Rev. D 2019, 99, 044049. [Google Scholar] [CrossRef] [Green Version]
- Prasad, A.K.; Kumar, J. Anisotropic relativistic fluid spheres with a linear equation of state. New Astron. 2022, 95, 101815. [Google Scholar] [CrossRef]
- Herrera, L. New definition of complexity for self-gravitating fluid distributions: The spherically symmetric, static case. Phys. Rev. D 2018, 97, 044010. [Google Scholar] [CrossRef] [Green Version]
- Sanudo, J.; Pacheco, A.F. Complexity and white-dwarf structure. Phys. Lett. A 2009, 373, 807–810. [Google Scholar] [CrossRef] [Green Version]
- Arias, C.; Contreras, E.; Fuenmayor, E.; Ramos, A. Anisotropic star models in the context of vanishing complexity. Ann. Phys. 2022, 436, 168671. [Google Scholar] [CrossRef]
- Andrade, J.; Contreras, E. Stellar models with like-Tolman IV complexity factor. Eur. Phys. J. C 2021, 81, 889. [Google Scholar] [CrossRef]
- Gomez-Lobo, A.G.P. Dynamical laws of superenergy in General Relativity. Class. Quant. Grav. 2008, 25, 015006. [Google Scholar] [CrossRef]
- Herrera, L.; Ospino, J.; Di Prisco, A.; Fuenmayor, E.; Troconis, O. Structure and evolution of self-gravitating objects and the orthogonal splitting of the Riemann tensor. Phys. Rev. D 2009, 79, 064025. [Google Scholar] [CrossRef] [Green Version]
- Panotopoulos, G.; Lopes, I. Millisecond pulsars modeled as strange quark stars admixed with condensed dark matter. Int. J. Mod. Phys. D 2018, 27, 1850093. [Google Scholar] [CrossRef] [Green Version]
- Moraes, P.H.R.S.; Panotopoulos, G.; Lopes, I. Anisotropic Dark Matter Stars. Phys. Rev. D 2021, 103, 084023. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A. Electrically charged strange quark stars with a non-linear equation-of-state. Eur. Phys. J. C 2019, 79, 524. [Google Scholar] [CrossRef]
- Lopes, I.; Panotopoulos, G.; Rincón, A. Anisotropic strange quark stars with a non-linear equation-of-state. Eur. Phys. J. Plus 2019, 134, 454. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A. Relativistic strange quark stars in Lovelock gravity. Eur. Phys. J. Plus 2019, 134, 472. [Google Scholar] [CrossRef] [Green Version]
- Abellán, G.; Rincon, A.; Fuenmayor, E.; Contreras, E. Beyond classical anisotropy and a new look to relativistic stars: A gravitational decoupling approach. arXiv 2020, arXiv:2001.07961. [Google Scholar]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Interior solutions of relativistic stars in the scale-dependent scenario. Eur. Phys. J. C 2020, 80, 318. [Google Scholar] [CrossRef]
- Bhar, P.; Tello-Ortiz, F.; Rincón, A.; Gomez-Leyton, Y. Study on anisotropic stars in the framework of Rastall gravity. Astrophys. Space Sci. 2020, 365, 145. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Radial oscillations and tidal Love numbers of dark energy stars. Eur. Phys. J. Plus 2020, 135, 856. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Interior solutions of relativistic stars with anisotropic matter in scale-dependent gravity. Eur. Phys. J. C 2021, 81, 63. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Slowly rotating dark energy stars. Phys. Dark Univ. 2021, 34, 100885. [Google Scholar] [CrossRef]
- Becerra-Vergara, E.A.; Mojica, S.; Lora-Clavijo, F.D.; Cruz-Osorio, A. Anisotropic Quark Stars with an Interacting Quark Equation of State. Phys. Rev. D 2019, 100, 103006. [Google Scholar] [CrossRef] [Green Version]
- Panotopoulos, G.; Tangphati, T.; Banerjee, A. Electrically charged compact stars with an interacting quark equation of state. Chin. J. Phys. 2022, 77, 1682–1690. [Google Scholar] [CrossRef]
- Beringer, J.; Arguin, J.F.; Barnett, R.M.; Copic, K.; Dahl, O.; Groom, D.E.; Lin, C.J.; Lys, J.; Murayama, H.; Wohl, C.G.; et al. Review of Particle Physics (RPP). Phys. Rev. D 2012, 86, 010001. [Google Scholar] [CrossRef] [Green Version]
- Fiorella Burgio, G.; Fantina, A.F. Nuclear Equation of state for Compact Stars and Supernovae. Astrophys. Space Sci. Libr. 2018, 457, 255–335. [Google Scholar] [CrossRef] [Green Version]
- Blaschke, D.; Chamel, N. Phases of dense matter in compact stars. Astrophys. Space Sci. Libr. 2018, 457, 337–400. [Google Scholar] [CrossRef] [Green Version]
- Silva, H.O.; Macedo, C.F.B.; Berti, E.; Crispino, L.C.B. Slowly rotating anisotropic neutron stars in general relativity and scalar–tensor theory. Class. Quant. Grav. 2015, 32, 145008. [Google Scholar] [CrossRef] [Green Version]
- Folomeev, V.; Dzhunushaliev, V. Magnetic fields in anisotropic relativistic stars. Phys. Rev. D 2015, 91, 044040. [Google Scholar] [CrossRef] [Green Version]
- Cattoen, C.; Faber, T.; Visser, M. Gravastars must have anisotropic pressures. Class. Quant. Grav. 2005, 22, 4189–4202. [Google Scholar] [CrossRef] [Green Version]
- Horvat, D.; Ilijic, S.; Marunovic, A. Radial pulsations and stability of anisotropic stars with quasi-local equation of state. Class. Quant. Grav. 2011, 28, 025009. [Google Scholar] [CrossRef]
- Arbañil, J.D.V.; Panotopoulos, G. Tidal deformability and radial oscillations of anisotropic polytropic spheres. Phys. Rev. D 2022, 105, 024008. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rincón, Á.; Panotopoulos, G.; Lopes, I. Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe 2023, 9, 72. https://doi.org/10.3390/universe9020072
Rincón Á, Panotopoulos G, Lopes I. Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe. 2023; 9(2):72. https://doi.org/10.3390/universe9020072
Chicago/Turabian StyleRincón, Ángel, Grigoris Panotopoulos, and Ilídio Lopes. 2023. "Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism" Universe 9, no. 2: 72. https://doi.org/10.3390/universe9020072
APA StyleRincón, Á., Panotopoulos, G., & Lopes, I. (2023). Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe, 9(2), 72. https://doi.org/10.3390/universe9020072







