Recurrent Interpopulation Selection in Popcorn: From Heterosis to Genetic Gains
Abstract
1. Introduction
2. Results
2.1. Genetic and Environmental Variability
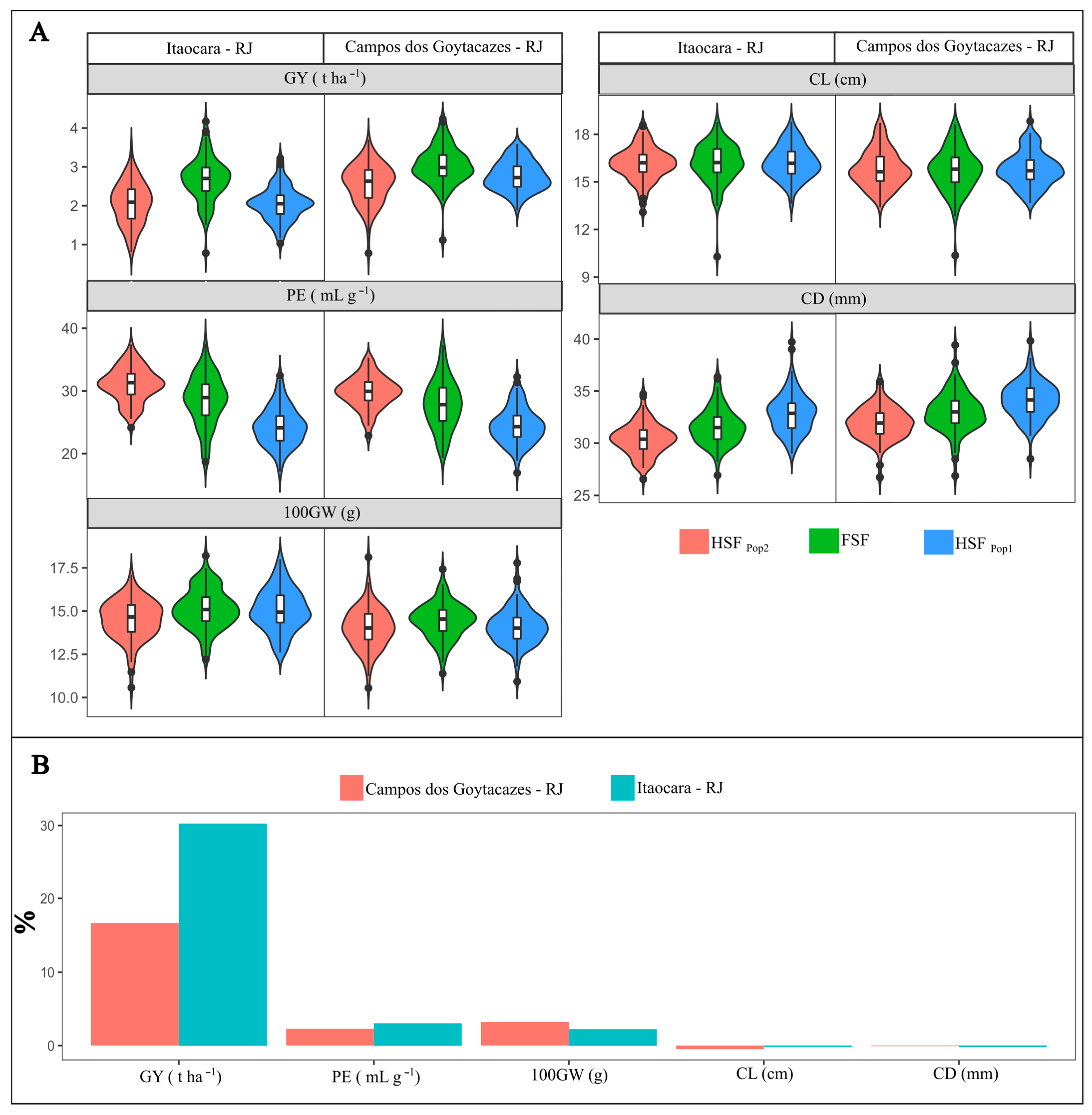
2.2. Distribution of Families, Means and Heteroses
2.3. Genetic Components: The Key to Selection of Popcorn
2.4. Expected Selection Gains
3. Discussion
3.1. Genetic and Environmental Variability
3.2. Distribution of Families, Means and Heteroses
3.3. Genetic Components: The Key to Selection in Popcorn
3.4. Expected Selection Gains
4. Materials and Methods
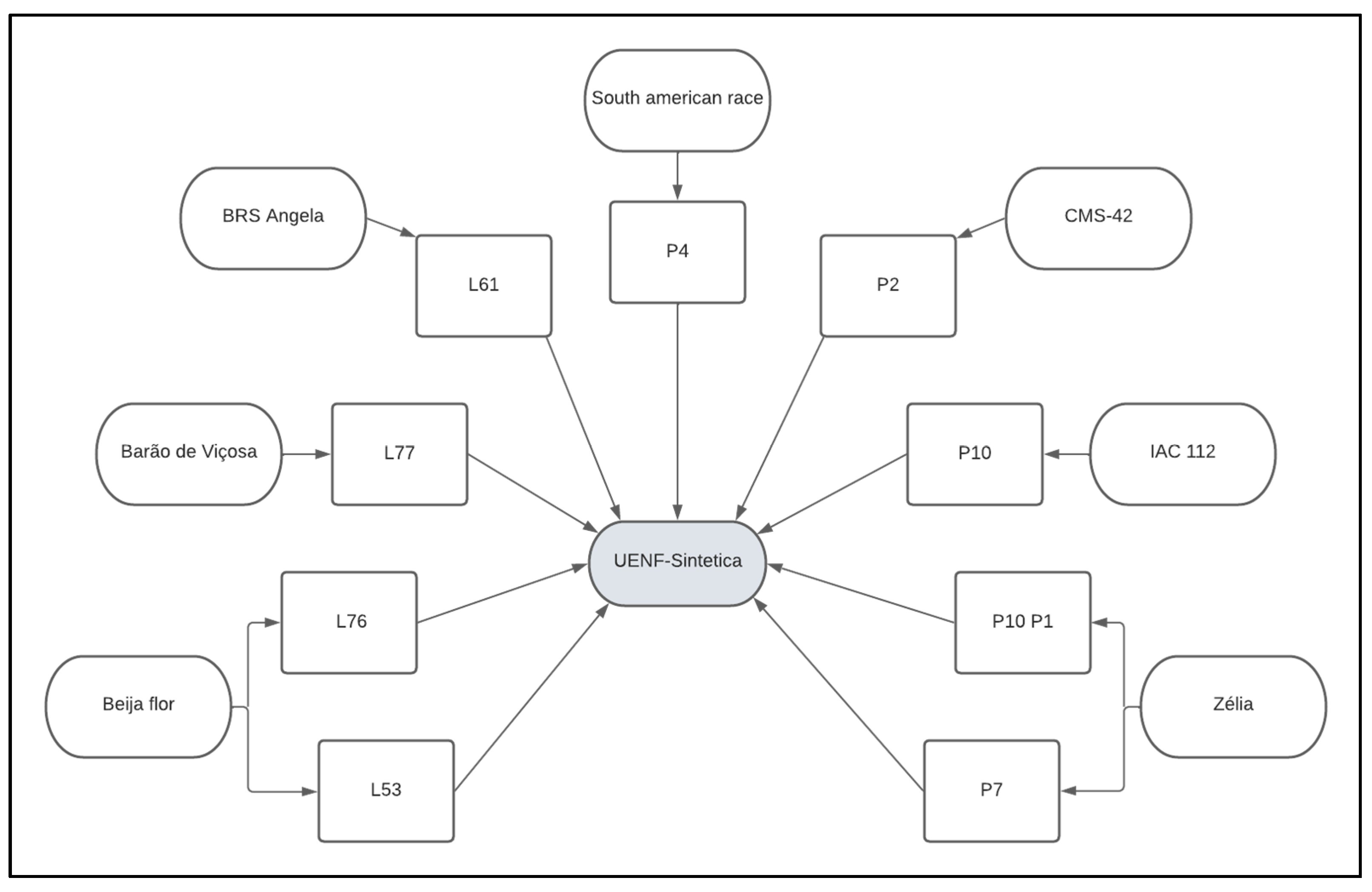
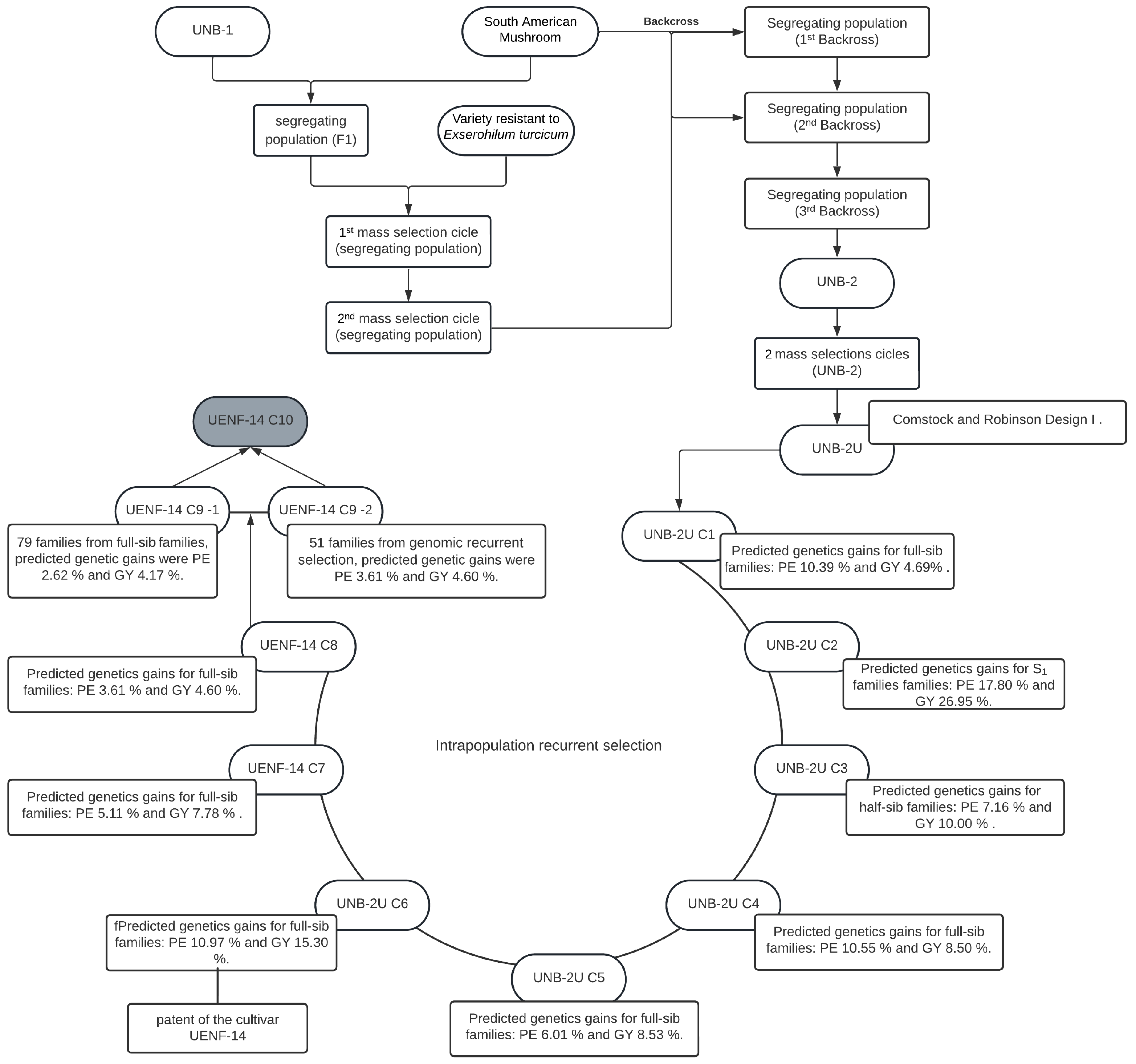
4.1. Genetic Lines
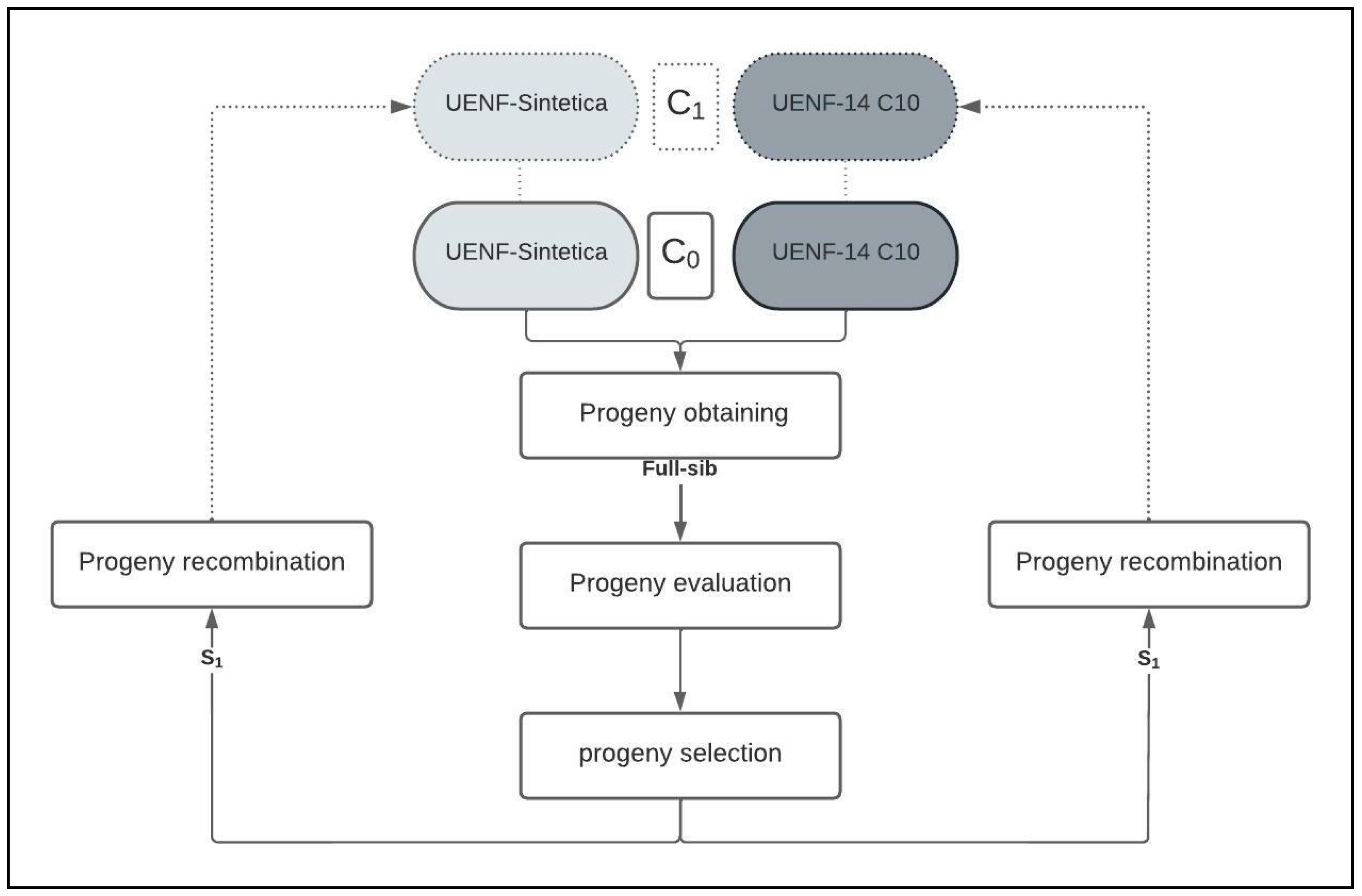
4.2. Obtaining Intrapopulation and Interpopulation Families
4.3. Evaluation of Families and Experimental Design
4.4. Evaluated Characteristics
4.5. Statistical Analyses
4.6. Selection Strategy
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pinho, R.G.V.; Brugnera, A.; Pacheco, C.A.P.; Gomes, M.d.S. Stability of Popcorn Cultivars To Different Environments in the State of Minas Gerais. Rev. Bras. Milho Sorgo 2003, 2, 53–61. [Google Scholar] [CrossRef]
- Pereira Filho, I.A.; Borghi, E. Milho-pipoca é um Novo Atrativo Para o Produtor? Available online: https://revistacampoenegocios.com.br/milho-pipoca-e-um-novo-atrativo-para-o-produtor (accessed on 11 May 2022).
- Paterniani, M.E.A.G.Z.; Fachini, C.; Rodrigues, C.S. Inovation and specialty maize breeding for market niches in the state of são paulo. Rev. Bras. Milho Sorgo 2020, 19, 19. [Google Scholar] [CrossRef]
- do Amaral Júnior, A.T.; Gonçalves, L.S.A.; de Paiva Freitas Jánior, S.; Candido, L.S.; Vittorazzi, C.; Pena, G.F.; Ribeiro, R.M.; da Conceião Silva, T.R.; Pereira, M.G.; Scapim, C.A.; et al. UENF 14: A new popcorn cultivar. Crop Breed. Appl. Biotechnol. 2013, 13, 218–220. [Google Scholar] [CrossRef]
- Guimarães, A.G.; Amaral Júnior, A.T.; Lima, V.J.; Leite, J.T.; Scapim, C.A.; Vivas, M. Genetic gains and selection advances of the uenf-14 popcorn population. Rev. Caatinga 2018, 31, 271–278. [Google Scholar] [CrossRef]
- Castro, C.R.; Scapim, C.A.; Pinto, R.J.B.; Ruffato, S.; Zeffa, D.M.; Ivamoto, S.T.; Freiria, G.H.; Gonçalves, L.S.A. Adaptability and stability analysis of new popcorn simple hybrids evaluated using additive main effects and multiplicative interaction Bayesian approaches. Bragantia 2022, 81. [Google Scholar] [CrossRef]
- Larish, L.L.B.; Brewbaker, J.L. Diallel analyses of temperate and tropical popcorns (Zea mays L.). Maydica 1999, 44, 279–284. [Google Scholar]
- Pereira, M.G.; Amaral Júnior, A.T. Estimation of Genetic Components in Popcorn Based on the Nested Design. Crop Breed. Appl. Biotechnol. 2001, 1, 3–10. [Google Scholar] [CrossRef]
- Daros, M.; Teixeira do Amaral, A., Jr.; Gonzaga Pereira, M.; Santana Santos, F.; Paula Cândido Gabriel, A.; Alberto Scapim, C.; de Paiva Freitas, S., Jr.; Silvério, L. Recurrent selection in inbred popcorn families. Sci. Agric. 2004, 61, 609–614. [Google Scholar] [CrossRef]
- Freitas Júnior, S.D.P.; do Amaral Júnior, A.T.; Pereira, M.G.; Cruz, C.D.; Scapim, C.A. Capacidade combinatória em milho-pipoca por meio de dialelo circulante. Pesqui. Agropecu. Bras. 2006, 41, 1599–1607. [Google Scholar] [CrossRef]
- Rangel, R.M.; do Amaral Júnior, A.T.; Gonçalves, L.S.A.; Freitas Júnior, S.d.P.; Candido, L.S. Análise biométrica de ganhos por seleção em população de milho pipoca de quinto ciclo de seleção recorrente. Rev. Cienc. Agron. 2011, 42, 473–481. [Google Scholar] [CrossRef]
- Cabral, P.D.S.; do Amaral Júnior, A.T.; Freitas, I.L.d.J.; Ribeiro, R.M.; Silva, T.R.d.C. Relação causa e efeito de caracteres quantitativos sobre a capacidade popcorn. Rev. Cienc. Agron. 2016, 47, 108–117. [Google Scholar] [CrossRef]
- Pacheco, C.A.P.; Gama, E.E.G.E.; Guimarães, P.E.d.O.; dos Santos, M.X.; Ferreira, A.d.S. Estimativas de parâmetros genéticos nas populações CMS-42 e CMS-43 de Milho-Pipoca. Pesq. Agropec. Bras. 1998, 33, 1995–2001. [Google Scholar]
- Vieira, R.A.; Scapim, C.A.; Moterle, L.M.; Tessmann, D.J.; do Amaral Junior, A.T.; Azeredo, L.S. The breeding possibilities and genetic parameters of maize resistance’ to foliar diseases. Euphytica 2012, 185, 325–336. [Google Scholar] [CrossRef]
- De Lima, V.J.; De Paiva, S.; Junior, F.; De Souza, Y.P.; Secifram, C. Genetic gain capitalization in the first cycle of recurrent selection in popcorn at Ceará’s Cariri. Rev. Bras. Ciênc. Agrár. 2018, 13, 1–7. [Google Scholar] [CrossRef]
- Babo, R.; Nair, S.K.; Kumar, A.; Rao, H.S.; Verma, P.; Gahalain, A.; Singh, I.S.; Gupta, H.S. Mapping QTLs for popping ability in a popcorn × flint corn cross. Theor. Appl. Genet. 2006, 112, 1392–1399. [Google Scholar] [CrossRef]
- Li, Y.L.; Dong, Y.B.; Niu, S.Z.; Cui, D.Q. QTL for popping characteristics in popcorn. Plant Breed. 2007, 126, 509–514. [Google Scholar] [CrossRef]
- Coan, M.M.D.; Barth, R.J.P.; Kuki, M.C.; Júnior, A.T.A.; Figueiredo, A.S.T.; Scapim, C.A.; Warburton, M. Inheritance Study for Popping Expansion in Popcorn vs. Flint Corn Genotypes. Agron. Appl. Genet. Resour. 2019, 111, 1–10. [Google Scholar] [CrossRef]
- Santos, J.S.; de Souza, Y.P.; Vivas, M.; Amaral Junior, A.T.; Filho, J.E.d.A.; Mafra, G.S.; Viana, A.P.; Gravina, G.d.A.; Ferreira, F.R.A. Genetic merit of popcorn lines and hybrids for multiple foliar diseases and agronomic properties. Funct. Plant Breed. J. 2020, 2, 32–47. [Google Scholar]
- Guimarães, A.G.; do Amaral Júnior, A.T.; Pena, G.F.; de Almeida Filho, J.E.; Pereira, M.G.; Santos, P.H.A.D. Genec gains in the popcorn population uenf-14: Developing the ninth generation of intrapopulation recurrent selectionton. Rev. Caatinga 2019, 32, 625–633. [Google Scholar] [CrossRef]
- Freitas, I.L.J.; Amaral Júnior, A.T.; Freitas , S.P., Jr.; Cabral, P.D.S.; Ribeiro, R.M.; Gonçalves, L.S.A. Genetic gains in the UENF-14 popcorn population with recurrent selection. Genet. Mol. Res. 2014, 13, 518–527. [Google Scholar] [CrossRef]
- Souza Junior, R.C.L. Melhoramento de espécies alógamas. In Recursos Genéticos e Melhoramento de Plantas; Fundação mT Rondonópolis: Rondonópolis, Brazil, 2001. [Google Scholar]
- Heinz, R.; Mota, L.H.d.S.; Gonçalves, M.C.; Viegas Neto, A.L.; Carlesso, A. Seleção de progênies de meio-irmãos de milho para eficiência no uso de nitrogênio. Revsita Ciênc. Agron. 2012, 43, 731–739. [Google Scholar] [CrossRef]
- Viana, J.M.S.; De Lima, R.O.; Mundim, G.B.; Condé, A.B.T.; Vilarionho, A.A. Relative efficiency of the genotypic value and combining ability effects on reciprocal recurrent selection. Theor. Appl. Genet. 2013, 126, 889–899. [Google Scholar] [CrossRef] [PubMed]
- Hallauer, A.R.; Carena, M.J.; Miranda Filho, J.D. Testers and combining ability. In Quantitative Genetics in Maize Breeding; Springer: New York, NY, USA, 2010; pp. 383–423. [Google Scholar]
- Silva, T.D.C.R.; do Amaral Júnior, A.T.; Gonçalves, L.S.A.; Candido, L.S.; Vittorazzi, C.; Scapim, C.A. Agronomic performance of popcorn genotypes in Northern and Northwestern Rio de Janeiro State. Acta Sci. Agron. 2013, 35, 57–63. [Google Scholar] [CrossRef]
- Faria, V.R.; Viana, J.M.S.; Mundim, G.B.; Silva, A.d.C.e; Câmara, T.M.M. Adaptabilidade e estabilidade de populações de milho-pipoca relacionadas por ciclos de seleção. Pesqui. Agropecu. Bras. 2010, 45, 1396–1403. [Google Scholar] [CrossRef]
- Dofing, S.M.; D’Croz-Mason, N.; Thomas-Compton, M.A. Inheritance of Expansion Volume and Yield in Two Popcorn × Dent Corn Crosses. Crop Sci. 1991, 31, 715–718. [Google Scholar] [CrossRef]
- Lu, H.-J.; Bernardo, R.; Ohm, H. Mapping QTL for popping expansion volume in popcorn with simple sequence repeat markers. Theor. Appl. Genet. 2003, 106, 423–427. [Google Scholar] [CrossRef]
- Ribeiro, R.M.; Júnior, A.T.A.; Gonçalves, L.S.A.; Candido, L.S. Genetic progress in the UNB-2U population of popcorn under recurrent selection in Rio de Janeiro, Brazil. Genet. Mol. Res. 2012, 11, 1417–1423. [Google Scholar] [CrossRef]
- Oliveira Júnior, J.F.; de Gois, G.; de Bodas Terassi, P.M.; da Silva Junior, C.A.; Blanco, C.J.C.; Sobral, B.S.; Gasparini, K.A.C. Drought severity based on the SPI index and its relation to the ENSO and PDO climatic variability modes in the regions North and Northwest of the State of Rio de Janeiro—Brazil. Atmos. Res. 2018, 212, 91–105. [Google Scholar] [CrossRef]
- Cruz, C.D.; Regazzi, A.J.; Carneiro, P.C. Aplicados, Modelos Biométricos Genético, ao Melhoramento, 3rd ed.; UFV: Viçosa, Brazil, 2012. [Google Scholar]
- Vilela, F.O.; do Amaral, A.T.; Pereira, M.G.; Scapim, C.A.; Viana, A.P.; Freitas, S.d.P. Effect of recurrent selection on the genetic variability of the UNB-2U popcorn population using RAPD markers. Acta Sci. Agron. 2008, 30, 25–30. [Google Scholar] [CrossRef]
- Carvalho, H.W.L.; Leal, M.L.S.; Cardoso, M.J.; Santos, M.X.; Tabosa, J.N.; Santos, D.M.; Lira, M.A. Adaptabilidade e Estabilidade de Híbridos de Milho em Diferentes Condições Ambientais do Nordeste Brasileiro. Rev. Bras. Milho Sorgo 2002, 1, 75–82. [Google Scholar] [CrossRef]
- Borém, A.; Miranda, G.V. Fritsche-Neto Melhoramento de Plantas; Oficina de Textos: São Paulo, Brazil, 2021. [Google Scholar]
- Daros, M.; do Amaral Junior, A.T.; Pereira, M.G. Genetic gain for grain yield and popping expansion in full-sib recurrent selection in popcorn. Crop Breed. Appl. Biotechnol. 2002, 2, 339–344. [Google Scholar] [CrossRef]
- Santos, F.S.; do Amaral Júnior, A.; Freitas Júnior, S.d.P.F.; Rangel, R.M.; Pereira, M.G. Predição de ganhos genéticos por índices de seleção na população de milho-pipoca unb-2u sob seleção recorrente. Bragantia 2007, 3, 389–396. [Google Scholar] [CrossRef]
- Freitas Júnior, S.D.P.; do Amaral Júnior, A.T.; Rangel, R.M.; Viana, A.P. Predição de ganhos genéticos na população de milho pipoca UNB-2U sob seleção recorrente utilizando-se diferentes índices de seleção. Semin. Ciênc. Agrár. 2009, 30, 803. [Google Scholar] [CrossRef]
- Oliveira, E.J.D.; Santos, V.D.S.; Lima, D.S.D.; Machado, M.D.; Lucena, R.S.; Motta, T.B.N. Estimativas de correlações genotípicas e fenotípicas em germoplasma de maracujazeiro. Bragantia 2011, 70, 255–261. [Google Scholar] [CrossRef]
- Schwantes, I.A.; do Amaral Júnior, A.T.; de Almeida Filho, J.E.; Vivas, M.; Silva Cabral, P.D.; Gonçalves Guimarães, A.; de Lima e Silva, F.H.; Araújo Diniz Santos, P.H.; Gonzaga Pereira, M.; Pio Viana, A.; et al. Genomic selection helps accelerate popcorn population breeding. Crop Sci. 2020, 60, 1373–1385. [Google Scholar] [CrossRef]
- Scapim, C.A.; Carvalho, C.G.P.; Cruz, C.D. Uma proposta de classificação dos coeficientes de variação para a cultura do milho. Pesqui. Agropecu. Bras. 1995, 30, 683–686. [Google Scholar]
- Fritsche-Neto, R.; Vieira, R.A.; Scapim, C.A.; Miranda, G.V.; Rezende, L.M. Updating the ranking of the coefficients of variation from maize experiments. Acta Sci. Agron. 2012, 34, 99–101. [Google Scholar] [CrossRef]
- Falconer, D.S. Introduction to Quantitative; Pearson: London, UK, 1996. [Google Scholar]
- Possatto Júnior, O.; José Barth Pinto, R.; Santos Rossi, E.; Carlos Kuki, M.; Augusto Bengasi Bertagna, F.; Henrique Araújo Diniz Santos, P.; Alberto Scapim, C. Evidence of additive inheritance of popping expansion in popcorn. Funct. Plant Breed. J. 2021, 3, 51–59. [Google Scholar] [CrossRef]
- de Lima, V.J.; do Amaral Júnior, A.T.; Kamphorst, S.H.; Bispo, R.B.; Leite, J.T.; Santos, T.d.O.; Schmitt, K.F.M.; Chaves, M.M.; de Oliveira, U.A.; Santos, P.H.A.D.; et al. Combined Dominance and Additive Gene Effects in Trait Inheritance of Drought-Stressed and Full Irrigated Popcorn. Agronomy 2019, 9, 782. [Google Scholar] [CrossRef]
- de Oliveira, G.H.F. Capacidade Combinatória e Correlação em Populações de Milho-Pipoca. Ph.D. Thesis, Universidade Estadual Paulista, Jaboticabal, Brazil, 2016. [Google Scholar]
- de Oliveira, G.H.F.; Murray, S.C.; Cunha Júnior, L.C.; de Lima, K.M.G.; Morais, C.d.L.M.d.; Teixeira, G.H.d.A.; Môro, G.V. Estimation and classification of popping expansion capacity in popcorn breeding programs using NIR spectroscopy. J. Cereal Sci. 2020, 91, 102861. [Google Scholar] [CrossRef]
- Sawazaki, E. Parâmetros Genéticos em Milho Pipoca (Zea mays L.); Escola Superior de Agricultura Luiz de Queiroz—USP: Piracicaba, Brazil, 1996. [Google Scholar]
- Araus, J.L.; Sánchez, C.; Cabrera-Bosquet, L. Is heterosis in maize mediated through better water use? New Phytol. 2010, 187, 392–406. [Google Scholar] [CrossRef] [PubMed]
- Cairns, J.E.; Sanchez, C.; Vargas, M.; Ordoñez, R.; Araus, J.L. Dissecting Maize Productivity: Ideotypes Associated with Grain Yield under Drought Stress and Well-watered Conditions. J. Integr. Plant Biol. 2012, 54, 1007–1020. [Google Scholar] [CrossRef] [PubMed]
- AdebAyo, M.A.; Menkir, A.; HeArne, S.; Kolawole, A.O. Gene action controlling normalized difference vegetation index in crosses of elite maize (Zea mays L.) inbred lines. Cereal Res. Commun. 2017, 45, 675–686. [Google Scholar] [CrossRef]
- Tollenaar, M.; Lee, E.A. Physiological dissection of grain yield in maize by examining genetic improvement and heterosis Producing high amylose starches in cereal endosperm View project. Maydica 2006, 51, 399–408. [Google Scholar]
- Xiao, Y.; Jiang, S.; Cheng, Q.; Wang, X.; Yan, J.; Zhang, R.; Qiao, F.; Ma, C.; Luo, J.; Li, W.; et al. The genetic mechanism of heterosis utilization in maize improvement. Genome Biol. 2021, 22, 148. [Google Scholar] [CrossRef]
- Kamphorst, S.H.; do Amaral Junior, A.T.; de Lima, V.J.; Carena, M.J.; Azeredo, V.C.; Mafra, G.S.; Santos, P.H.A.D.; Leite, J.T.; Schmitt, K.F.M.; dos Santos Junior, D.R.; et al. Driving Sustainable Popcorn Breeding for Drought Tolerance in Brazil. Front. Plant Sci. 2021, 12, 1942. [Google Scholar] [CrossRef]
- Labroo, M.R.; Studer, A.J.; Rutkoski, J.E. Heterosis and Hybrid Crop Breeding: A Multidisciplinary Review. Front. Genet. 2021, 12, 234. [Google Scholar] [CrossRef]
- Blum, A. Effective use of water (EUW) and not water-use efficiency (WUE) is the target of crop yield improvement under drought stress. Field Crop. Res. 2009, 112, 119–123. [Google Scholar] [CrossRef]
- Schwantes, I.A.; do Amaral Júnior, A.T.; Gerhardt, I.F.S.; Vivas, M.; de Lima e Silva, F.H.; Kamphorst, S.H. Diallel analysis of resistance to Fusarium ear rot in Brazilian popcorn genotypes. Trop. Plant Pathol. 2017, 42, 70–75. [Google Scholar] [CrossRef]
- Arnhold, E.; Soriano Viana, J.M.; Mora, F.; Vieira Miranda, G.; Silva, R.G. Inbreeding depression and genetic components for popping expansion and other traits in Brazilian populations of popcorn 1. Cienc. Investig. Agrar. 2010, 37, 125–132. [Google Scholar] [CrossRef]
- Scapim, C.A.; Braccini, A.d.L.; Pinto, R.J.B.; Amaral Júnior, A.T.; Rodovalho, M.d.A.; da Silva, R.M.; Moterle, L.M. Componentes genéticos de médias e depressão por endogamia em populações de milho-pipoca. Ciênc. Rural. 2006, 36, 36–41. [Google Scholar] [CrossRef]
- Cruz, C.D. GENES—A software package for analysis in experimental statistics and quantitative genetics. Acta Sci. Agron. 2013, 35, 271–276. [Google Scholar] [CrossRef]
- Cruz, C.D.; Regazzi, A.J.; Carneiro, P.C.S. Modelos Biométricos Aplicados ao Melhoramento Genético—Vol. II; UFV: Vicosa, Brazil, 2014; ISBN 8572691510. [Google Scholar]
- Mulamba, N.N.; Mock, J.J. Improvement of yield potential of the Eto Blanco maize (Zea mays L.) population by breeding for plant traits. Egypt. J. Genet. Cytol. 1978, 7, 40–51. [Google Scholar] [CrossRef]
- Santos, J.S.; Amaral Júnior, A.T.; Vivas, M.; Mafra, G.S.; Pena, G.F.; Silva, F.H.L.; Guimarães, A.G. Genetic control and combining ability of agronomic attributes and northern leaf blight-related attributes in popcorn. Genet. Mol. Res. 2017, 16, 1–11. [Google Scholar] [CrossRef]
- Mafra, G.S.; do Amaral Junior, A.T.; Vivas, M.; dos Santos, J.S.; Silva, F.H.d.L.E.; Guimarães, A.G.; Pena, G.F. The combining ability of popcorn S7 lines for Puccinia polysora resistance purposes. Bragantia 2018, 77, 519–526. [Google Scholar] [CrossRef]
- Daros, M.; do Amaral Júnior, A.T.; Pereira, M.G.; Santos, F.S.; Scapim, C.A.; Freitas Júnior, S.d.P.; Daher, R.F.; Ávila, M.R. Correlações entre caracteres agronômicos em dois ciclos de seleção recorrente em milho-pipoca. Ciênc. Rural 2004, 34, 1389–1394. [Google Scholar] [CrossRef]
- Sawazaki, E. A cultura do milho-pipoca no Brasil. O Agron. 2001, 53, 11–13. [Google Scholar]
- Cruz, C.D.; Castoldi, F. Decomposição da interação genótipo × ambientes em partes simples e complexa. Ceres 1991, 38, 422–430. [Google Scholar]
| Mean Squares | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| DF | GY | PE | 100 GW | EL | ED | ||||||
| Genotype (g) | 323 | 1.34 | ** | 90.28 | ** | 5.68 | ** | 5.75 | ** | 15.63 | ** |
| Group | 3 | 34.39 | ** | 3548.98 | ** | 29.66 | ** | 1.39 | * | 473.47 | ** |
| FSF | 99 | 1.17 | ** | 72.94 | ** | 4.54 | ** | 7.60 | ** | 12.70 | ** |
| HSFPop1 | 99 | 0.59 | ** | 39.21 | ** | 5.08 | ** | 4.25 | ** | 13.34 | ** |
| HSFPop2 | 99 | 1.27 | ** | 23.63 | ** | 5.37 | ** | 4.39 | ** | 7.53 | ** |
| Control | 23 | 1.22 | ** | 220.55 | ** | 11.36 | ** | 10.65 | ** | 13.19 | ** |
| Environment | 1 | 3.92 | ** | 126.13 | ** | 1787.39 | ** | 0.01 | ** | 433.53 | ** |
| G × E | 323 | 0.40 | ** | 12.27 | ** | 3.02 | ** | 2.83 | ** | 4.08 | ** |
| Group × E | 3 | 3.14 | ** | 87.00 | ** | 7.01 | ** | 0.58 | ns | 10.50 | ** |
| FSF × E | 99 | 0.43 | ** | 11.44 | ** | 2.93 | ** | 2.92 | ** | 3.73 | ** |
| HSFPop1 × E | 99 | 0.37 | ** | 8.84 | ns | 3.11 | ** | 2.64 | ** | 4.34 | ** |
| HSFPop2 × E | 99 | 0.33 | * | 13.89 | ** | 3.18 | ** | 2.95 | ** | 4.31 | ** |
| Control × E | 23 | 0.28 | ns | 13.93 | ** | 1.74 | ** | 2.96 | ** | 2.59 | ** |
| Residue | 1190 | 0.36 | 7.31 | 0.75 | 0.51 | 1.60 | |||||
| CVe (%) | 24.03 | 9.62 | 6.21 | 4.43 | 3.31 | ||||||
| Mean | 2.30 | 28.09 | 13.95 | 16.17 | 31.13 | ||||||
| Simple Interact. (%) | 36.58 | 54.76 | 0.14 | 16.79 | 38.80 | ||||||
| Complex Interact. (%) | 63.42 | 45.24 | 99.86 | 83.21 | 61.20 | ||||||
| Mean Squares | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| DF | GY | PE | 100 GW | EL | ED | |||||||
| Itaocara | Repetitions | 2 | 2.88 | 26.92 | 1.58 | 1.39 | 0.65 | |||||
| Block/repet. (aj) | 51 | 0.73 | 7.76 | 1.01 | 0.61 | 1.21 | ||||||
| Treat. (adjust.) | 323 | 1.03 | ** | 59.33 | ** | 4.37 | ** | 3.83 | ** | 11.59 | ** | |
| Group | 3 | 26.37 | ** | 2353.82 | ** | 23.48 | ** | 1.35 | * | 310.85 | ** | |
| FSF | 99 | 0.94 | ** | 47.15 | ** | 3.9 | ** | 4.68 | ** | 8.7 | ** | |
| HSFPop1 | 99 | 0.55 | ** | 27.54 | ** | 4.42 | ** | 3.24 | ** | 11.14 | ** | |
| HSFPop2 | 99 | 0.86 | ** | 21.5 | ** | 3.81 | ** | 3.22 | ** | 6.85 | ** | |
| Control | 23 | 0.86 | ** | 112.16 | ** | 6.1 | ** | 5.59 | ** | 7.32 | ** | |
| Error | 595 | 0.36 | 7.08 | 0.82 | 0.51 | 1.04 | ||||||
| CVe | 26.76 | 9.56 | 6.09 | 4.41 | 3.23 | |||||||
| Mean | 2.25 | 27.84 | 14.91 | 16.18 | 31.60 | |||||||
| Campos dos Goytacazes | Repetitions | 2 | 4.07 | 2.97 | 1.1 | 0.73 | ||||||
| Block/repet. (aj) | 51 | 0.49 | 10.33 | 1.07 | 0.57 | 0.99 | ||||||
| Treat. (adjust.) | 323 | 0.71 | ** | 43.23 | ** | 4.33 | ** | 4.75 | ** | 8.11 | ** | |
| Group | 3 | 11.15 | ** | 1282.15 | ** | 13.19 | ** | 0.62 | ns | 173.11 | ** | |
| FSF | 99 | 0.66 | ** | 37.23 | ** | 3.57 | ** | 5.84 | ** | 7.73 | ** | |
| HSFPop1 | 99 | 0.41 | ** | 20.5 | ** | 3.78 | ** | 3.64 | ** | 6.53 | ** | |
| HSFPop2 | 99 | 0.75 | ** | 16.03 | ** | 4.74 | ** | 4.13 | ** | 4.99 | ** | |
| Control | 23 | 0.64 | ** | 122.32 | ** | 7 | ** | 8.02 | ** | 8.45 | ** | |
| Error | 595 | 0.25 | 7.54 | 0.68 | 0.52 | 1.09 | ||||||
| CVe (%) | 21.23 | 9.69 | 6.33 | 4.45 | 3.4 | |||||||
| Mean | 2.34 | 28.35 | 12.99 | 16.17 | 30.66 | |||||||
| Itaocara | Campos dos Goytacazes | |||||
|---|---|---|---|---|---|---|
| FSF | HSFPop1 | HSFPop2 | FSF | HSFPop1 | HSFPop2 | |
| Mean Grain Yield (GY) | ||||||
| 0.19 | 0.06 | 0.16 | 0.14 | 0.05 | 0.17 | |
| (%) | 61.28 | 34.26 | 57.45 | 62.73 | 39.35 | 66.75 |
| (%) | 16.34 | 12.21 | 19.67 | 14.36 | 9.96 | 19.13 |
| (%) | 0.61 | 0.46 | 0.74 | 0.68 | 0.47 | 0.90 |
| 0.25 | 0.66 | 0.21 | 0.66 | |||
| Popping Expansion (PE) | ||||||
| 13.35 | 6.82 | 4.80 | 9.90 | 4.32 | 2.83 | |
| (%) | 84.98 | 74.28 | 67.05 | 79.75 | 63.22 | 52.96 |
| (%) | 12.81 | 10.73 | 7.06 | 10.88 | 8.04 | 5.48 |
| (%) | 1.34 | 1.12 | 0.74 | 1.12 | 0.83 | 0.57 |
| 27.28 | 19.22 | 17.28 | 11.32 | |||
| Mean 100-grain Weight (100 GW) | ||||||
| 1.03 | 1.20 | 0.99 | 0.96 | 1.03 | 1.36 | |
| (%) | 78.89 | 81.35 | 78.35 | 81.05 | 82.10 | 85.75 |
| (%) | 6.70 | 7.26 | 6.87 | 7.39 | 7.87 | 9.08 |
| (%) | 1.10 | 1.19 | 1.13 | 1.17 | 1.24 | 1.43 |
| 4.79 | 3.98 | 4.13 | 5.42 | |||
| Mean Ear Length (EL) | ||||||
| 1.39 | 0.91 | 0.90 | 1.78 | 1.04 | 1.20 | |
| (%) | 89.15 | 84.35 | 84.23 | 91.13 | 85.77 | 87.43 |
| (%) | 7.29 | 5.88 | 5.89 | 8.26 | 6.30 | 6.76 |
| (%) | 1.66 | 1.33 | 1.34 | 1.86 | 1.42 | 1.52 |
| 3.65 | 3.62 | 4.17 | 4.81 | |||
| Mean Ear Diameter (ED) | ||||||
| 2.55 | 3.37 | 1.94 | 2.21 | 1.81 | 1.30 | |
| (%) | 88.06 | 90.68 | 84.84 | 85.91 | 83.31 | 78.18 |
| (%) | 5.06 | 5.58 | 4.58 | 4.86 | 4.26 | 3.84 |
| (%) | 1.57 | 1.73 | 1.42 | 1.43 | 1.25 | 1.13 |
| 13.47 | 7.75 | 7.25 | 5.20 | |||
| Characteristics | Estimated Selection Gains (%) | |||||
|---|---|---|---|---|---|---|
| SDg | CVg | Iv | h2 | AW | ||
| Itaocara | GY | 1.58 | 0.04 | 4.38 | 4.50 | 8.72 |
| PE | 10.04 | 2.01 | 0.46 | −0.98 | 8.71 | |
| 100 GW | −1.24 | −0.06 | 2.26 | 3.01 | −0.96 | |
| EL | 1.97 | 0.32 | 5.30 | 4.93 | 3.27 | |
| ED | −0.94 | −0.14 | 2.35 | 2.53 | −0.39 | |
| 3.48 | 0.21 | 5.14 | 6.26 | 8.55 | ||
| Campos dos Goytacazes | GY | 8.69 | 1.79 | 1.65 | 1.32 | 9.68 |
| PE | 0.36 | 0.32 | 2.87 | 2.70 | −0.57 | |
| 100 GW | 4.19 | 0.88 | 5.04 | 5.09 | 4.26 | |
| EL | −0.56 | −0.03 | 2.21 | 2.30 | −1.13 | |
| ED | ||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos Junior, D.R.d.; Amaral Junior, A.T.d.; Lima, V.J.d.; Leite, J.T.; Bispo, R.B.; Azeredo, V.C.; Almeida Filho, J.E.d.; Kamphorst, S.H.; Viana, F.N.; Ribeiro, R.M.; et al. Recurrent Interpopulation Selection in Popcorn: From Heterosis to Genetic Gains. Plants 2023, 12, 1056. https://doi.org/10.3390/plants12051056
Santos Junior DRd, Amaral Junior ATd, Lima VJd, Leite JT, Bispo RB, Azeredo VC, Almeida Filho JEd, Kamphorst SH, Viana FN, Ribeiro RM, et al. Recurrent Interpopulation Selection in Popcorn: From Heterosis to Genetic Gains. Plants. 2023; 12(5):1056. https://doi.org/10.3390/plants12051056
Chicago/Turabian StyleSantos Junior, Divino Rosa dos, Antônio Teixeira do Amaral Junior, Valter Jário de Lima, Jhean Torres Leite, Rosimeire Barboza Bispo, Valdinei Cruz Azeredo, Janeo Eustáquio de Almeida Filho, Samuel Henrique Kamphorst, Flávia Nicácio Viana, Rodrigo Moreira Ribeiro, and et al. 2023. "Recurrent Interpopulation Selection in Popcorn: From Heterosis to Genetic Gains" Plants 12, no. 5: 1056. https://doi.org/10.3390/plants12051056
APA StyleSantos Junior, D. R. d., Amaral Junior, A. T. d., Lima, V. J. d., Leite, J. T., Bispo, R. B., Azeredo, V. C., Almeida Filho, J. E. d., Kamphorst, S. H., Viana, F. N., Ribeiro, R. M., Viana, A. P., & Gravina, G. d. A. (2023). Recurrent Interpopulation Selection in Popcorn: From Heterosis to Genetic Gains. Plants, 12(5), 1056. https://doi.org/10.3390/plants12051056














