Investigating the Influence of Infrared Drying Method on Linden (Tilia platyphyllos Scop.) Leaves: Kinetics, Color, Projected Area, Modeling, Total Phenolic, and Flavonoid Content
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
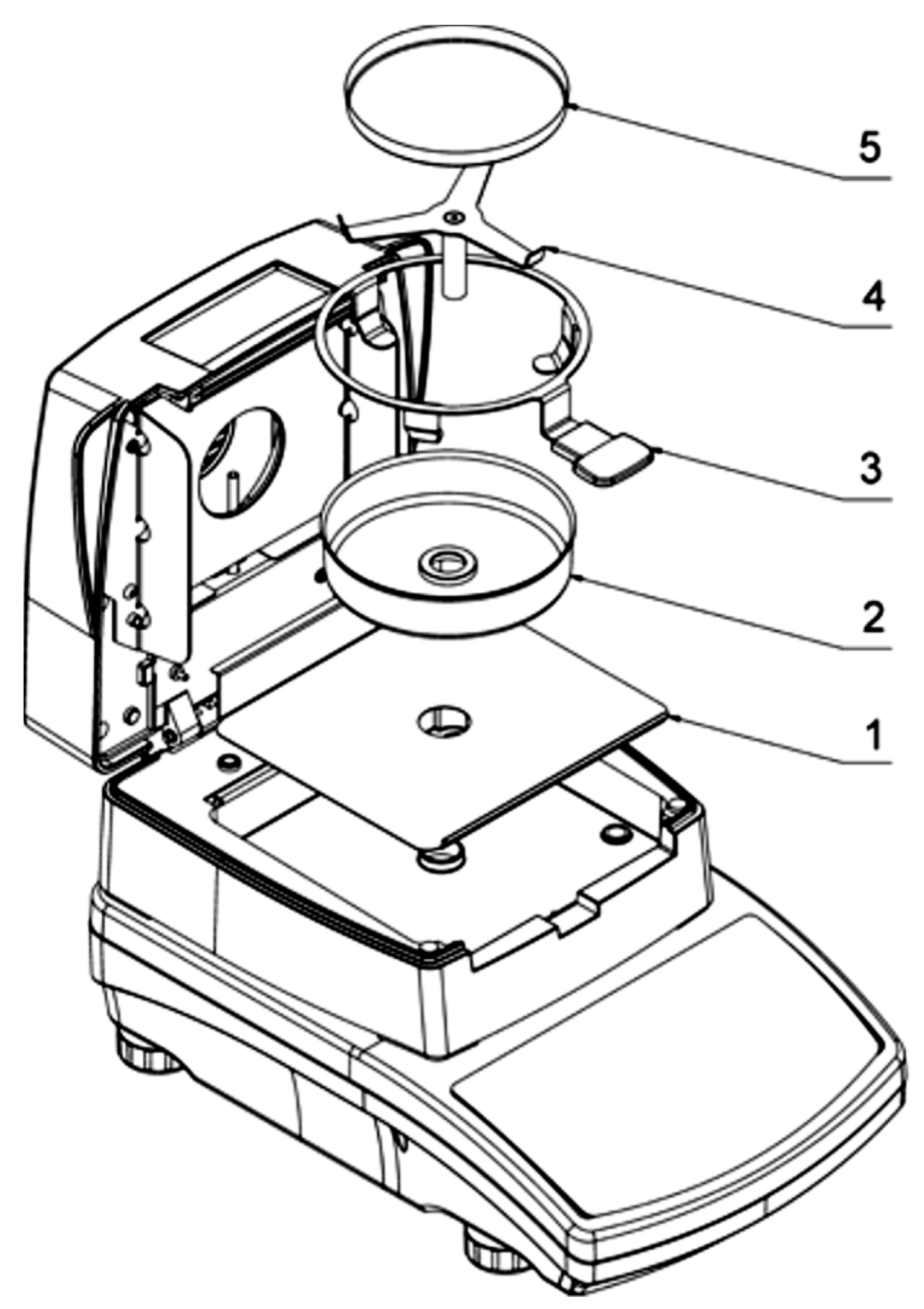
2.2. Experimental Drying Device
2.3. Color Measurements
2.4. Image Analysis to Projected Area
2.5. Mathematical Modeling of Thin Layer Drying Curves
2.6. The Effective Moisture Diffusivity and Activation Energy
2.7. Extracts Preparation
2.8. Total Phenolic Content (TPC) Assay
2.9. Total Flavonoids Assay (TFC)
2.10. Statistical Analysis
3. Results and Discussion
3.1. Moisture Contents
3.2. Color
3.3. Projected Area (PA)
3.4. Modeling of Drying Curves
3.5. Effective Moisture Diffusivity and Activation Energy
3.6. Total Phenolic Content (TPC) and Flavonoids (TFC)
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Ekor, M. The growing use of herbal medicines: Issues relating to adverse reactions and challenges in monitoring safety. Front. Pharmacol. 2014, 4, 1–10. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Certo, G.; Costa, R.; D’Angelo, V.; Russo, M.; Albergamo, A.; Dugo, G.; Germanò, M.P. Anti-angiogenic activity and phytochemical screening of fruit fractions from Vitex agnus castus. Nat. Prod. Res. 2017, 31, 2850–2856. [Google Scholar] [CrossRef] [PubMed]
- Ertekin, C.; Heybeli, N. Thin-layer infrared drying of mint leaves. J. Food Process. Preserv. 2014, 38, 1480–1490. [Google Scholar] [CrossRef]
- Wissam, Z.; Nour, A.A.; Bushra, J.; Zein, N.; Saleh, D. Extracting and studying the antioxidant capacity of polyphenols in dry linden leaves (Tilia cordata). J. Pharmacogn. Phytochem. 2017, 6, 258–262. [Google Scholar]
- Rodríguez, N.C.; Trujano, M.E.G.; Hernández, E.A.; García, M.R.; Sampieri, A.; Urrutia, E.C.; Aparicio, L.C. Anticonvulsant and antioxidant effects of Tilia americana var. mexicana and flavonoids constituents in the pentylenetetrazole-induced seizures. Oxid. Med. Cell. Longev. 2014, 2014, 10. [Google Scholar]
- Rababah, T.M.; Al-u’datt, M.; Alhamad, M.; Al-Mahasneh, M.; Ereifej, K.; Andrade, J.; Altarifi, B.; Almajwal, A.; Yang, W. Effects of drying process on total phenolics, antioxidant activity and flavonoid contents of common Mediterranean herbs. Int. J. Agric. Biol. Eng. 2015, 8, 145–150. [Google Scholar]
- Delnavazi, M.R.; Shahabi, M.; Yassa, N. Flavonoids from the leaves of Iranian Linden; Tilia rubra subsp. Caucasica. Res. J. Pharmacogn. 2015, 2, 17–22. [Google Scholar]
- Tuttu, G.; Ursavaş, S.; Soyler, R. Harvest amounts and ethnobotanical uses of the linden flowers in Turkey. Anatol. J. Forest Res. 2017, 3, 60–66. [Google Scholar]
- Lao, Y.; Zhanga, M.; Chitrakar, B.; Bhandari, B.; Fan, D. Efficient plant foods processing based on infrared heating. Food Rev. Int. 2019, 35, 640–663. [Google Scholar] [CrossRef]
- Khaled, A.Y.; Kabutey, A.; Selvi, K.Ç.; Mizera, C.; Hrabe, P.; Herak, D. Application of computational intelligence in describing the drying kinetics of persimmon fruit (Diospyros kaki) during vacuum and hot air drying process. Processes 2020, 8, 544. [Google Scholar] [CrossRef]
- Soysal, Y.; Ayhan, Z.; Esturk, O.; Arikan, M.F. Intermittent microwave–convective drying of red pepper: Drying kinetics, physical (colour and texture) and sensory quality. Biosyst. Eng. 2009, 103, 455–463. [Google Scholar] [CrossRef]
- Łechtanska, J.M.; Szadzinska, J.; Kowalski, S.J. Microwave- and infrared-assisted convective drying of green pepper: Quality and energy considerations. Chem. Eng. Process. 2015, 98, 155–164. [Google Scholar] [CrossRef]
- Pawar, S.B.; Pratape, V.M. Fundamentals of infrared heating and its application in drying of food materials: A review. J. Food Process. Eng. 2017, 40, e12308. [Google Scholar] [CrossRef]
- Guiné, R. The drying of foods and its effect on the physical-chemical, sensorial and nutritional properties. Int. J. Food Eng. 2018, 4, 93–100. [Google Scholar] [CrossRef]
- Orikasa, T.; Ono, N.; Watanabe, T.; Ando, Y.; Shiina, T.; Koide, S. Impact of blanching pretreatment on the drying rate and energy consumption during far-infrared drying of Paprika (Capsicum annuum L.). Food Qual. Saf. 2018, 2, 97–103. [Google Scholar] [CrossRef] [Green Version]
- Aboud, S.A.; Altemimi, A.B.; Al-Hilpy, A.R.S.; Chen, L.Y.; Cacciola, F. A comprehensive review on infrared heating applications in food processing. Molecules 2019, 24, 4125. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deng, L.Z.; Yang, X.H.; Mujumdar, A.S.; Zhao, J.H.; Wang, D.; Zhang, Q.; Wang, J.; Gao, Z.J.; Xiao, H.W. Red pepper (Capsicum annuum L.) drying: Effects of different drying methods on drying kinetics, physicochemical properties, antioxidant capacity, and microstructure. Dry Technol. 2018, 36, 893–907. [Google Scholar] [CrossRef]
- Rahmawati, L.; Saputra, D.; Sahim, K.; Priyanto, G. Effect of infrared radiation on chemical and physical properties on duku’s peel. Potravin. Slovak J. Food Sci. 2018, 12, 744–755. [Google Scholar]
- Riadh, M.H.; Ahmad, S.A.B.; Marhaban, M.H.; Soh, A.C. Infrared heating in food drying: An overview. Dry Technol. 2015, 33, 322–335. [Google Scholar] [CrossRef]
- Soysal, Y.; Keskin, M.; Arslan, A.; Sekerli, Y.E. Infrared drying characteristics of pepper at different maturity stages. In Proceedings of the International Conference on Energy Research, Alanya, Turkey, 1–2 November 2018; pp. 293–304. [Google Scholar]
- Sharma, G.P.; Verma, R.C.; Pathare, P.B. Thin-layer infrared radiation drying of onion slices. J. Food Eng. 2005, 67, 361–366. [Google Scholar] [CrossRef]
- Adak, N.; Heybeli, N.; Ertekin, C. Infrared drying of strawberry. Food Chem. 2017, 219, 109–116. [Google Scholar] [CrossRef] [PubMed]
- Doymaz, I. Infrared drying of kiwifruit slices. Int. J. Green Energy 2018, 15, 622–628. [Google Scholar] [CrossRef]
- Igathinathane, C.; Pordesimo, L.O.; Batchelor, W.D. Major orthogonal dimensions measurement of food grains by machine vision using ImageJ. Food Res. Int. 2009, 42, 76–84. [Google Scholar] [CrossRef]
- Juneau, K.J.; Tarasoff, C.S. Leaf area and water content changes after permanent and temporary storage. PLoS ONE 2012, 7, e42604. [Google Scholar] [CrossRef] [Green Version]
- Easlon, H.M.; Bloom, A.J. Easy leaf area: Automated digital image analysis for rapid and accurate measurement of leaf area. Appl. Plant Sci. 2014, 2, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.C.; Fon, D.S.; Fang, W.; Sokhansanj, S. Development of a visual method to test the range of applicability of thin layer drying equations using MATLAB tools. Dry Technol. 2004, 22, 1921–1948. [Google Scholar] [CrossRef]
- Dziki, D.; Kowalska, E.H.; Dziki, U.G.; Mis, A.; Rozylo, R.; Krzysiak, Z.; Hasson, W. Drying kinetics, grinding characteristics and physicochemical properties of broccoli sprouts. Processes 2019, 8, 97. [Google Scholar] [CrossRef] [Green Version]
- Nadian, M.H.; Shahin, R.; Seyed, S.M.; Soleiman, H.; Majid, K. Determination of shrinkage and shape change of apple slices during drying. In Proceedings of the 7th Asia-Pacific Drying Conference, Tianjin, China, 18–20 September 2011. [Google Scholar]
- Nahimana, H.; Zhang, M. Shrinkage and color change during microwave vacuum drying of carrot. Dry Technol. 2011, 29, 836–847. [Google Scholar] [CrossRef]
- Ertekin, C.; Yaldiz, O. Drying of eggplant and selection of a suitable thin layer drying model. J. Food Eng. 2004, 63, 349–359. [Google Scholar] [CrossRef]
- Diamente, L.M.; Munro, P.A. Mathematical modeling of the thin layer solar drying of sweet potato slices. Solar Energy 1993, 51, 271–276. [Google Scholar] [CrossRef]
- Saçılık, K.; Keskin, R.; Eliçin, A.K. Mathematical modeling of solar tunnel drying of thin layer organic tomato. J. Food Eng. 2006, 73, 231–238. [Google Scholar] [CrossRef]
- Midilli, A.; Küçük, H.; Yapar, Z. A new model for single-layer drying. Dry Technol. 2002, 20, 1503–1513. [Google Scholar] [CrossRef]
- Wang, C.Y.; Singh, R.P. A Single Layer Drying Equation For Rough Rice; ASAE Paper No. 78-3001; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1978. [Google Scholar]
- Jain, D.; Pathare, P.B. Selection and evaluation of thin layer drying models for infrared radiative and convective drying of onion slices. Biosyst. Eng. 2004, 89, 289–296. [Google Scholar] [CrossRef]
- Verma, L.R.; Bucklin, R.A.; Endan, J.B.; Wratten, F.T. Effects of drying air parameters on rice drying models. ASAE 1985, 28, 296–301. [Google Scholar] [CrossRef]
- Naghavi, Z.; Moheb, A.; Ziaei-Rad, S. Numerical simulation of rough rice drying in a deep-bed dryer using non-equilibrium model. Energy Convers. Manag. 2010, 51, 258–264. [Google Scholar] [CrossRef]
- Soysal, Y.; Oztekin, S.; Eren, Ö. Microwave drying of parsley: Modelling, kinetics, and energy aspects. Biosyst. Eng. 2006, 93, 403–413. [Google Scholar] [CrossRef]
- Mghazli, S.; Ouhammou, M.; Hidar, N.; Lahnine, L.; Idlimam, A.; Mahrouz, M. Drying characteristics and kinetics solar drying of Moroccan rosemary leaves. Renew. Energy 2017, 108, 303–310. [Google Scholar] [CrossRef]
- Crank, J. Mathematics of Diffusion, 2nd ed.; Oxford University Press: London, UK, 1975; p. 414. [Google Scholar]
- Simal, S.; Femenia, A.; Garau, M.C.; Rossello, C. Use of exponential, Page’s and diffusional models to simulate the drying kinetics of kiwi fruit. J. Food Eng. 2005, 66, 323–328. [Google Scholar] [CrossRef]
- Kara, C.; Doymaz, I. Effective moisture diffusivity determination and mathematical modelling of drying curves of apple pomace. Heat Mass Transf. 2015, 51, 983–989. [Google Scholar] [CrossRef]
- Nguyen, T.V.L.; Nguyen, M.D.; Nguyen, D.C.; Bach, L.G.; Lam, T.D. Model for Thin Layer Drying of Lemongrass (Cymbopogon citratus) by Hot Air. Processes 2019, 7, 21. [Google Scholar] [CrossRef] [Green Version]
- Singleton, V.L.; Rossi, J.A. Colorimetry of total phenolics with phosphomolybdic-phosphotungstic acid reagents. Am. J. Enol. Viticult. 1965, 16, 144–153. [Google Scholar]
- Gao, H.; Cheng, N.; Zhou, J.; Wang, B.N.; Deng, J.J.; Cao, W. Antioxidant activities and phenolic compounds of date plum persimmon (Diospyros lotus L.) fruits. J. Food Sci. Technol. 2014, 51, 950–956. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Önder, H. Nonparametric statistical methods used in biological experiments yöntemler. BSJ Eng. Sci. 2018, 1, 1–6. [Google Scholar]
- Genç, S.; Soysal, M.I. Parametric and nonparametric post hoc tests. BSJ Eng. Sci. 2018, 1, 18–27. [Google Scholar]
- Menges, H.O.; Ertekin, C. Thin layer drying model for treated and untreated Stanley plums. Energy Convers. Manag. 2006, 47, 2337–2348. [Google Scholar] [CrossRef]
- Goyal, R.K.; Kingsly, A.R.P.; Manikantan, M.R.; Ilyas, S.M. Mathematical modelling of thin layer drying kinetics of plum in a tunnel dryer. J. Food Eng. 2007, 79, 176–180. [Google Scholar] [CrossRef]
- Tasirin, S.M.; Puspasari, I.; Lun, A.W.; Chai, P.V.; Lee, W.T. Drying of kaffir lime leaves in a fluidized bed dryer with inertparticles: Kinetics and quality determination. Ind. Crop. Prod. 2014, 6, 193–201. [Google Scholar] [CrossRef]
- Therdthai, N.; Zhou, W. Characterization of microwave vacuum drying and hot air drying of mint leaves (Mentha cordifolia Opiz ex Fresen). J. Food Eng. 2009, 91, 482–489. [Google Scholar] [CrossRef]
- Li, B.; Lecourt, J.; Bishop, G. Advances in non-destructive early assessment of fruit ripeness towards defining optimal time of harvest and yield prediction—A Review. Plants 2018, 7, 2–20. [Google Scholar]
- Zielinska, M.; Markowski, M. Color characteristics of carrots: Effect of drying and rehydration. Int. J. Food Prop. 2011, 15, 450–466. [Google Scholar] [CrossRef]
- Torrez, V.; Jørgensen, P.M.; Zanne, A.E. Specific leaf area: A predictive model using dried samples. Aust. J. Bot. 2013, 61, 350–357. [Google Scholar] [CrossRef]
- Doymaz, I. Drying kinetics, rehydration and colour characteristics of convective hot-air drying of carrot slices. Heat Mass Transfer. 2017, 53, 5–35. [Google Scholar] [CrossRef]
- Doymaz, I. Thin-layer drying behaviour of mint leaves. J. Food Eng. 2006, 74, 370–375. [Google Scholar] [CrossRef]
- Park, K.J.; Vohnikova, Z.; Brod, F.P.R. Evaluation of drying parameters and desorption isotherms of garden mint leaves (Mentha crispa L.). J. Food Eng. 2002, 51, 193–199. [Google Scholar] [CrossRef]
- Onwude, D.I.; Hashim, N.; Janius, R.B.; Nawi, N.; Abdan, K. Evaluation of a suitable thin layer model for drying of pumpkin under forced air convection. Int. Food Res. J. 2016, 23, 1173–1181. [Google Scholar]
- Hii, C.L.; Law, C.L.; Cloke, M. Modeling using a new thin layer drying model and product quality of cocoa. J. Food Eng. 2009, 90, 191–198. [Google Scholar] [CrossRef]
- Zogzas, N.P.; Maroulis, Z.B.; Kouris, D.M. Moisture diffusivity data compilation in foodstuffs. Dry Technol. 1996, 14, 2225–2253. [Google Scholar] [CrossRef]
- Darvishi, H.; Asl, A.R.; Asghari, A.; Azadbakht, M.; Najafi, G.; Khodaei, J. Study of the drying kinetics of pepper. J. Saudi Soc. Agri. Sci. 2014, 13, 130–138. [Google Scholar] [CrossRef] [Green Version]
- Abraham, D.G.; Arolilo, A.P.; Rosimeire, M.R.; Cala, S.S.L.; Amanda, C.F. The air drying behaviour of osmotically dehydrated jackfruit. In Proceedings of the 14th International Drying Symposium, Sao Paulo, Brazil, 22–25 August 2004. [Google Scholar]
- Guine, R.; Lopes, P.; Joao, B.M.; Ferreira, D. Effect of ripening stage on the solar drying kinetics and properties of S. bartolomeu pears. Int. J. Acad. Res. 2009, 1, 46–52. [Google Scholar]
- Madan, A.; Pare, A.; Nanje Gowda, N.A. Mathematical modelling of thin-layer drying process of bamboo (Bambusa bambos) shoots at varying temperature. Res. Rev. J. Bot. 2014, 3, 1–9. [Google Scholar]
- Darvishi, H.; Khoshtaghaza, M.H.; Najafi, G.; Nargesi, F. Mathematical modeling of green pepper drying in microwave-convective dryer. J. Agri. Sci. Technol. 2013, 15, 457–465. [Google Scholar]
- Doymaz, I. Convective ait drying characteristics of thin layer carrots. J. Food Eng. 2004, 61, 359–364. [Google Scholar] [CrossRef]
- Turhan, M.; Turhan, K.N.; Sahbaz, F. Drying kinetics of red pepper. J. Food Proc. Preserv. 1997, 21, 209–223. [Google Scholar] [CrossRef]
- Önder, H.; Cebeci, Z. A Review on the permutation tests. Biostat. Biom. Open Access J. 2017, 3, 1–3. [Google Scholar] [CrossRef]
- López, J.; Uribe, E.; Vega-Gálvez, A.; Miranda, M.; Vergara, J.; Gonzalez, E.; Di Scala, K. Effect of air temperature on drying kinetics, vitamin C, antioxidant activity, total phenolic content, non-enzymatic browning and firmness of blueberries variety O’Neil. Food Bioprocess Technol. 2010, 3, 772–777. [Google Scholar] [CrossRef]
- Felipe, C.; da Silva, C.; Favaro-Trindade, S.M.; de Alencar, M.T.; Julio, C.B. Physicochemical properties, antioxidant activity and stability of spray-dried propolis. Kinetics, mineral content and colour characteristics of rosemary leave. Energy Convers. Manag. 2010, 49, 1258–1264. [Google Scholar]
- Złotek, U.; Dziki, U.G.; Dziki, D.; Swieca, M.; Nowak, R.; Martinez, E. Influence of drying temperature on phenolic acids composition and antioxidant activity of sprouts and leaves of white and red quinoa. Hindawi J. Chem. 2019, 2019, 1–8. [Google Scholar] [CrossRef]
- Capecka, E.; Mareczek, A.; Leja, M. Antioxidant activity of fresh and dry herbs of some Lamiaceae species. Food Chem. 2005, 93, 223–226. [Google Scholar] [CrossRef]
- Shan, F.; Tang, H.; Wang, Y.; Guo, M. Study on flavonoids from the Peony leaves by ultrasonic extraction. Adv. Mater. Res. 2011, 236–238, 2250–2254. [Google Scholar] [CrossRef]
- Roshanak, S.; Rahimmalek, M.; Goli, S.A.H. Evaluation of seven different drying treatments in respect to total flavonoid, phenolic, vitamin C content, chlorophyll, antioxidant activity and color of green tea (Camellia sinensis or C. assamica) leaves. J. Food Sci. Technol. 2015, 53, 721–729. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Azad, M.O.K.; Piao, J.P.; Park, C.H.; Cho, D.H. Far infrared irradiation enhances nutraceutical compounds and antioxidant properties in Angelica gigas nakai powder. Antioxidants 2018, 7, 189. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fernandez, N.F.; Casas, M.P.; Munoz, M.J.G.; Gonzalez, H.D. Water Extraction of Bioactive Compounds: From Plants to Drug Devolopment, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 421–439. [Google Scholar]
- Olsson, M.E.; Gustavsson, K.E.; Vagen, I.M. Quercetin and isorhamnetin in sweet and red cultivars of onion (Allium cepa L.) at harvest, after field curing, heat treatment, and storage. J. Agric. Food Chem. 2010, 58, 2323–2330. [Google Scholar] [CrossRef] [PubMed]






| Model Name | Model Equation | References | |
|---|---|---|---|
| 1 | Newton | [31] | |
| 2 | Page | [32] | |
| 3 | Henderson and Pabis | [31] | |
| 4 | Logarithmic | [33] | |
| 5 | Midilli et al. | [34] | |
| 6 | Wang and Singh | [35] | |
| 7 | Logistic | [36] | |
| 8 | Two term | [36] | |
| 9 | Verma et al. | [37] | |
| 10 | Two term exponential | [38] | |
| 11 | Diffusion approximation | [39] |
| L* | a* | b* | C | H | ΔE | |
|---|---|---|---|---|---|---|
| Fresh | 38.98 ± 1.05 b | 1.03 ± 0.11 b | 28.81 ± 0.53 a | 28.83 ± 0.54 a | 88.81 ± 0.61 a | - |
| IR-50 °C | 38.86 ± 0.66 b | −1.84 ± 0.02 c | 29.03 ± 0.86 a | 29.09 ± 0.86 a | −86.36 ± 0.14 c | 3.617 ± 0.20 |
| IR-60 °C | 42.36 ± 0.63 a | 1.22 ± 0.06 b | 30.56 ± 0.35 a | 30.59 ± 0.35 a | 88.34 ± 0.66 a | 4.11 ± 1.32 |
| IR-70 °C | 40.95 ± 1.11 ab | 3.33 ± 0.10 a | 29.63 ± 1.18 a | 29.81 ± 1.17 a | 83.4 ± 0.93 b | 3.81 ± 0.54 |
| p-value | 0.047 | <0.001 | 0.455 | 0.447 | <0.001 | 0.917 |
| Projection Area (cm2) | |
|---|---|
| Fresh | 81.00 ± 0.00 a |
| 50 °C | 79.38 ± 0.14 b |
| 60 °C | 78.26 ± 0.09 c |
| 70 °C | 75.93 ± 0.14 d |
| p-value | <0.001 |
| Temperature °C | Statistical Model and Its Constants | ||||||
|---|---|---|---|---|---|---|---|
| Midilli et al. Model | |||||||
| R2 | SEE | RSS | k | n | a | b | |
| 50 | 0.9999 | 0.0025 | 0.0001 | 0.0413 | 1.0933 | 0.9998 | 0.0003 |
| 60 | 0.9992 | 0.0102 | 0.0008 | 0.0716 | 1.1305 | 0.9984 | 0.0009 |
| 70 | 0.9984 | 0.0152 | 0.0014 | 0.1300 | 1.1340 | 0.9914 | 0.0013 |
| Verma et al. model | |||||||
| 50 | 0.9991 | 0.0095 | 0.0011 | −0.1453 | - | 6.64 × 10−7 | 0.0534 |
| 60 | 0.9978 | 0.0159 | 0.0023 | −0.0567 | - | 0.0020 | 0.0963 |
| 70 | 0.9999 | 0.0041 | 0.0001 | −0.0121 | - | 0.0267 | 0.1814 |
| Diffusion approximation model | |||||||
| 50 | 0.9994 | 0.008 | 2.4640 | 2.1067 | - | −0.0385 | 0.0262 |
| 60 | 0.9936 | 0.0269 | 0.0065 | 1.4003 | - | −0.0229 | 0.0682 |
| 70 | 0.9999 | 0.0041 | 0.0001 | 0.1814 | - | 0.9733 | −0.067 |
| Page model | |||||||
| 50 | 0.9992 | 0.009 | 0.0011 | 0.0472 | 1.0381 | - | - |
| 60 | 0.9935 | 0.0257 | 0.0066 | 0.0955 | 0.9915 | - | - |
| 70 | 0.9931 | 0.0269 | 0.0058 | 0.2584 | 0.7815 | - | - |
| Drying Temperature (°C) | Deff (m2s−1) | D0 (m2s−1) | Ea (kJ/mol) |
|---|---|---|---|
| 50 | 4.13 × 10−12 | 1.746 × 10−09 | 16.339 |
| 60 | 4.47 × 10−12 | ||
| 70 | 5.89 × 10−12 |
| Temperature (°C) | TPC (mg/g, DW) | TFC (mg/g, DW) |
|---|---|---|
| Fresh | 127.73 ± 0.76 b | 0.567 ± 0.015 b |
| 50 | 95.184 ± 0.47 a | 2.790 ± 0.150 a |
| 60 | 99.756 ± 0.63 a | 2.631 ± 0.084 a |
| 70 | 98.929 ± 0.43 a | 2.583 ± 0.145 a |
| Significance | <0.001 | <0.001 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Selvi, K.Ç. Investigating the Influence of Infrared Drying Method on Linden (Tilia platyphyllos Scop.) Leaves: Kinetics, Color, Projected Area, Modeling, Total Phenolic, and Flavonoid Content. Plants 2020, 9, 916. https://doi.org/10.3390/plants9070916
Selvi KÇ. Investigating the Influence of Infrared Drying Method on Linden (Tilia platyphyllos Scop.) Leaves: Kinetics, Color, Projected Area, Modeling, Total Phenolic, and Flavonoid Content. Plants. 2020; 9(7):916. https://doi.org/10.3390/plants9070916
Chicago/Turabian StyleSelvi, Kemal Çağatay. 2020. "Investigating the Influence of Infrared Drying Method on Linden (Tilia platyphyllos Scop.) Leaves: Kinetics, Color, Projected Area, Modeling, Total Phenolic, and Flavonoid Content" Plants 9, no. 7: 916. https://doi.org/10.3390/plants9070916
APA StyleSelvi, K. Ç. (2020). Investigating the Influence of Infrared Drying Method on Linden (Tilia platyphyllos Scop.) Leaves: Kinetics, Color, Projected Area, Modeling, Total Phenolic, and Flavonoid Content. Plants, 9(7), 916. https://doi.org/10.3390/plants9070916




