A No-Chatter Single-Input Finite-Time PID Sliding Mode Control Technique for Stabilization of a Class of 4D Chaotic Fractional-Order Laser Systems
Abstract
:1. Introduction
- Formulating a robust and stable sliding surface that suits the specific control context.
- Constructing control laws designed to quell chaotic trajectories within Fractional-Order (FO) Systems, thereby maintaining their adherence to the designated sliding surface.
- The majority of these approaches involve multi-input controller strategies, which can be challenging to implement in practical scenarios where operating multi-input controllers is complex.
- The operation of SMC controllers often leads to undesirable chattering phenomena.
- These control schemes predominantly rely on utilizing their constituent functions in their analysis and design.
- A significant portion of these studies have adopted a simplistic system definition, disregarding factors such as model uncertainties, external distributions, and input saturations.
- Fractional-Order Lyapunov Stability Theory: Our approach utilizes fractional-order Lyapunov stability theory, which is a mathematical framework adapted for systems with fractional-order dynamics. Traditional Lyapunov stability theory is designed for integer-order systems, while fractional-order Lyapunov stability theory extends its applicability to systems with fractional-order derivatives. By incorporating this theory, we can design controllers that account for the unique characteristics of fractional-order chaotic systems.
- Finite-Time Control Objective: The primary focus of our technique is to achieve finite-time control and stabilization. This means that we aim to drive the system to a desired state within a specified time frame. Traditional control strategies often target asymptotic or steady-state stability, whereas our approach emphasizes achieving stability within a finite time, which is particularly relevant in real-world applications with timing constraints.
- PID SMC Integration: Our technique integrates the PID (Proportional-Integral-Derivative) controller with a sliding mode control. The PID component provides a robust baseline control strategy, while the sliding mode control component allows for rapid adjustment and robustness to disturbances. This combination is essential for achieving the desired finite-time stabilization.
- Chatter Mitigation: One of the key distinctions of our approach is its effectiveness in mitigating chatter, which is a common issue in sliding mode controls. Chatter refers to high-frequency oscillations in the control signal, which can be undesirable and potentially damaging in practical systems. Our technique is designed to significantly reduce or eliminate chatter, which is a substantial improvement over traditional sliding mode control methods.
- Tailored for Chaotic Fractional-Order Systems: Our control technique is specifically tailored for 4D chaotic fractional-order laser systems. Chaotic systems exhibit complex, unpredictable behavior, and fractional-order dynamics introduce additional complexities. By addressing these challenges with our approach, we provide a control strategy that is uniquely suited to the characteristics of the targeted systems.
2. Preliminary Concepts
3. The Problem Formulation and Design of the Finite-Time PID Sliding Mode Control
3.1. Problem Statement
- (i)
- Complex Dynamics: Chaotic systems, such as laser systems, exhibit highly complex and unpredictable behavior. These systems are often characterized by their sensitivity to initial conditions and the presence of irregular, aperiodic oscillations. Understanding and controlling such complex dynamics is a significant challenge in the field of control theory and engineering.
- (ii)
- Fractional-Order Dynamics: Fractional-order differential equations are used to model systems with memory and hereditary properties, which are common in various real-world applications, including laser systems. The use of fractional-order dynamics provides a more accurate representation of the laser system’s behavior compared to that of traditional integer-order models.
- (iii)
- Four-Dimensional Space: In the context of 4D fractional-order laser systems, the “4D” refers to the four state variables or dimensions that describe the system’s behavior. These dimensions could represent different aspects of the laser system’s operation, such as power, intensity, wavelength, and time.
- (iv)
- Significance in Research Objectives: The significance of focusing on this specific group of laser systems in our research is twofold:
- (a)
- Practical Relevance: Many laser-based applications, including laser communication and precision manufacturing, involve 4D laser systems. Therefore, understanding and controlling the chaotic behavior in these systems is directly relevant to practical engineering applications.
- (b)
- Complexity and Challenge: The complexity of 4D chaotic fractional-order laser systems presents a formidable challenge in control theory. By addressing this class of systems, our research aims to contribute to the development of control strategies that can handle highly complex, non-integer-order dynamics and chaotic behavior.
3.2. Design of the Finite-Time PID SMC
- Practical Relevance: Chaotic behavior is often observed in real-world systems, including laser systems. Achieving stability and control in such systems is crucial for various applications, such as laser-based communication and precision instrumentation.
- Chatter Reduction: Chattering is a significant issue in sliding mode control. Traditional methods may not be effective in dealing with it. Our motivation lies in developing a control technique that can effectively reduce or eliminate chattering, which is a persistent problem in the field.
- Fractional-Order Dynamics: Fractional-order systems are a relatively new and evolving field of study. Motivated by the increasing realization of the fractional-order dynamics in physical systems, we aim to contribute to the understanding of how to control and stabilize these systems, which is still an emerging area of research.
- Finite-Time Control: Finite-time control is of interest in situations where there is a need for a response within a specific time frame. This research addresses the requirement for finite-time stabilization, which is relevant in applications with strict timing constraints.
- Chatter Reduction: Our proposed technique focuses on mitigating chattering, which is a challenging problem in sliding mode control. By achieving no-chatter control, we contribute to the improvement of control strategies for fractional-order chaotic systems.
- Finite-Time Stabilization: The introduction of a finite-time PID sliding mode control technique adds to the toolbox of control strategies for fractional-order chaotic systems, offering more versatility in addressing specific timing constraints.
- Application to Real-World Systems: By applying this technique to a class of 4D fractional-order laser systems, we bridge the gap between the theoretical developments and practical applications. This research contributes to the understanding of how fractional-order control can be applied in real-world scenarios.
4. Simulations
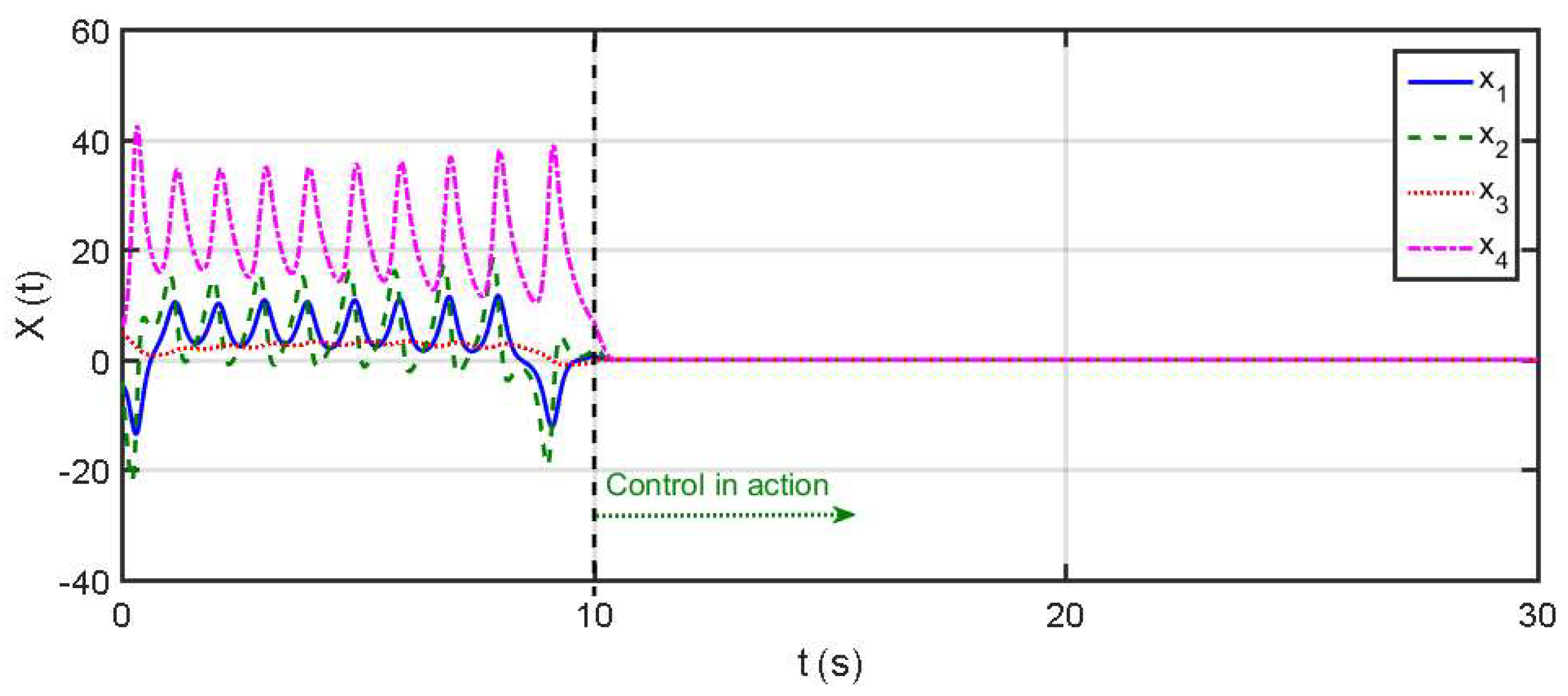
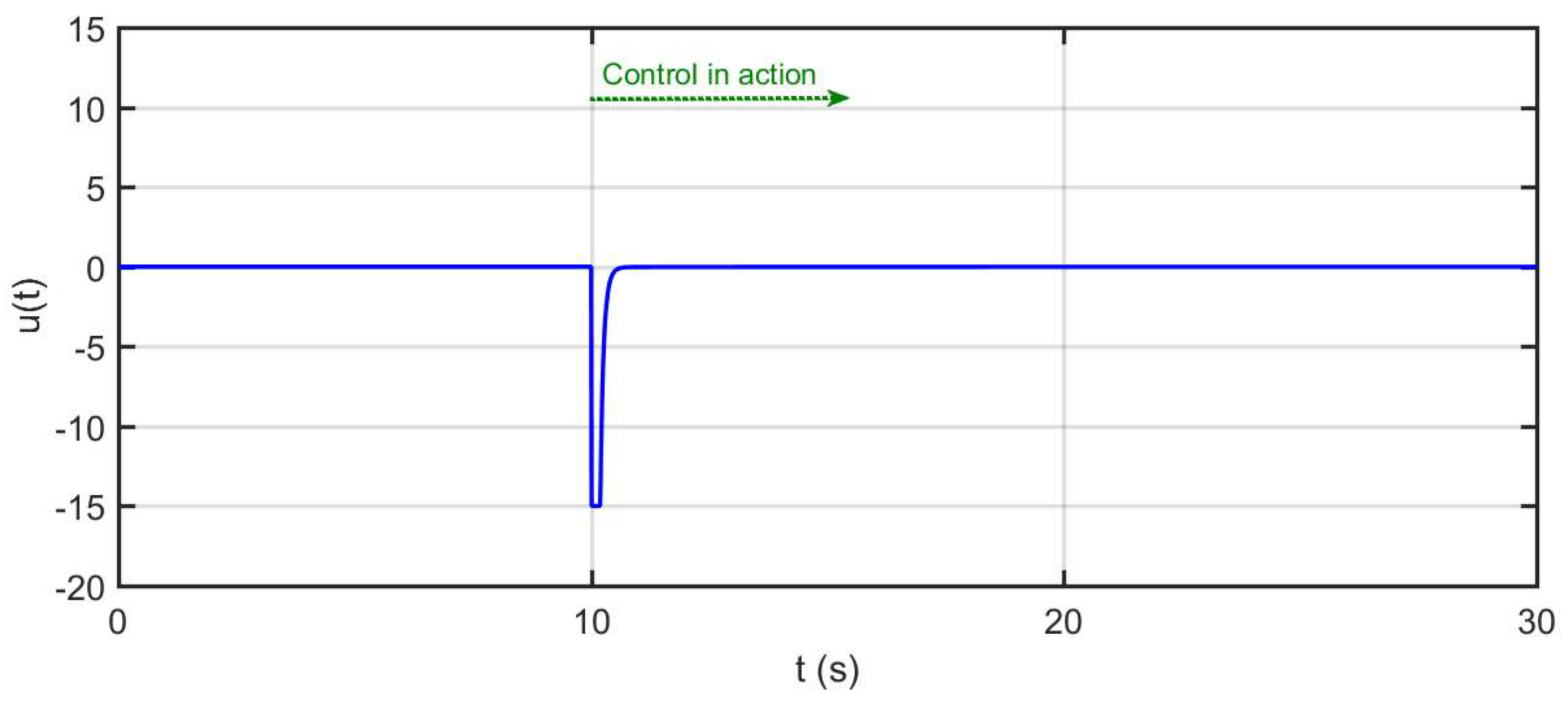
4.1. Scenario 1
4.2. Scenario 2
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Taheri, M.; Chen, Y.; Zhang, C.; Berardehi, Z.R.; Roohi, M.; Khooban, M.H. A finite-time sliding mode control technique for synchronization chaotic fractional-order laser systems with application on encryption of color images. Optik 2023, 285, 170948. [Google Scholar] [CrossRef]
- Colet, P.; Roy, R. Digital communication with synchronized chaotic lasers. Opt. Lett. 1994, 19, 2056–2058. [Google Scholar] [CrossRef] [PubMed]
- Trujillo, J.J.; Scalas, E.; Diethelm, K.; Baleanu, D. Fractional Calculus: Models and Numerical Methods; World Scientific: Singapore, 2016; Volume 5. [Google Scholar]
- Delavari, H.; Heydarinejad, H.; Baleanu, D. Adaptive fractional-order blood glucose regulator based on high-order sliding mode observer. IET Syst. Biol. 2019, 13, 43–54. [Google Scholar] [CrossRef]
- Roohi, M.; Zhang, C.; Chen, Y. Adaptive model-free synchronization of different fractional-order neural networks with an application in cryptography. Nonlinear Dyn. 2020, 100, 3979–4001. [Google Scholar] [CrossRef]
- Roohi, M.; Zhang, C.; Taheri, M.; Basse-O’Connor, A. Synchronization of Fractional-Order Delayed Neural Networks Using Dynamic-Free Adaptive Sliding Mode Control. Fractal Fract. 2023, 7, 682. [Google Scholar] [CrossRef]
- Saad, K. Comparing the Caputo, Caputo-Fabrizio and Atangana-Baleanu derivative with fractional order: Fractional cubic isothermal auto-catalytic chemical system. Eur. Phys. J. Plus 2018, 133, 94. [Google Scholar] [CrossRef]
- Yousefpour, A.; Jahanshahi, H.; Munoz-Pacheco, J.M.; Bekiros, S.; Wei, Z. A fractional-order hyper-chaotic economic system with transient chaos. Chaos Solitons Fractals 2020, 130, 109400. [Google Scholar] [CrossRef]
- Song, L.; Xu, S.; Yang, J. Dynamical models of happiness with fractional order. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 616–628. [Google Scholar] [CrossRef]
- Alikhanov, A.A.; Asl, M.S.; Huang, C.; Khibiev, A. A second-order difference scheme for the nonlinear time-fractional diffusion-wave equation with generalized memory kernel in the presence of time delay. J. Comput. Appl. Math. 2023, 438, 115515. [Google Scholar] [CrossRef]
- Roohi, M.; Khooban, M.-H.; Esfahani, Z.; Aghababa, M.P.; Dragicevic, T. A switching sliding mode control technique for chaos suppression of fractional-order complex systems. Trans. Inst. Meas. Control 2019, 41, 2932–2946. [Google Scholar] [CrossRef]
- Rasooli Berardehi, Z.; Zhang, C.; Taheri, M.; Roohi, M.; Khooban, M.H. Implementation of T-S fuzzy approach for the synchronization and stabilization of non-integer-order complex systems with input saturation at a guaranteed cost. Trans. Inst. Meas. Control 2023, 45, 2536–2553. [Google Scholar] [CrossRef]
- Rasooli Berardehi, Z.; Zhang, C.; Taheri, M.; Roohi, M.; Khooban, M.H. A Fuzzy Control Strategy to Synchronize Fractional-Order Nonlinear Systems Including Input Saturation. Int. J. Intell. Syst. 2023, 2013, 1550256. [Google Scholar] [CrossRef]
- Al-Dhaifallah, M. Fuzzy fractional-order PID control for heat exchanger. Alex. Eng. J. 2023, 63, 11–16. [Google Scholar] [CrossRef]
- Alsubaie, H.; Yousefpour, A.; Alotaibi, A.; Alotaibi, N.D.; Jahanshahi, H. Stabilization of Nonlinear Vibration of a Fractional-Order Arch MEMS Resonator Using a New Disturbance-Observer-Based Finite-Time Sliding Mode Control. Mathematics 2023, 11, 978. [Google Scholar] [CrossRef]
- Roohi, M.; Aghababa, M.P.; Haghighi, A.R. Switching adaptive controllers to control fractional-order complex systems with unknown structure and input nonlinearities. Complexity 2015, 21, 211–223. [Google Scholar] [CrossRef]
- Rabiei, K.; Razzaghi, M. An approach to solve fractional optimal control problems via fractional-order Boubaker wavelets. J. Vib. Control 2023, 29, 1806–1819. [Google Scholar] [CrossRef]
- Xie, Y.; Zhang, X.; Meng, W.; Zheng, S.; Jiang, L.; Meng, J.; Wang, S. Coupled fractional-order sliding mode control and obstacle avoidance of a four-wheeled steerable mobile robot. ISA Trans. 2021, 108, 282–294. [Google Scholar] [CrossRef]
- Mathiyalagan, K.; Sangeetha, G. Second-order sliding mode control for nonlinear fractional-order systems. Appl. Math. Comput. 2020, 383, 125264. [Google Scholar] [CrossRef]
- Wang, J.; Shao, C.; Chen, Y.-Q. Fractional order sliding mode control via disturbance observer for a class of fractional order systems with mismatched disturbance. Mechatronics 2018, 53, 8–19. [Google Scholar] [CrossRef]
- Balamash, A.; Bettayeb, M.; Djennoune, S.; Al-Saggaf, U.; Moinuddin, M. Fixed-time terminal synergetic observer for synchronization of fractional-order chaotic systems. Chaos Interdiscip. J. Nonlinear Sci. 2020, 30, 073124. [Google Scholar] [CrossRef]
- Li, G.; Zhang, X.; Yang, H. Complexity analysis and synchronization control of fractional-order Jafari-Sprott chaotic system. IEEE Access 2020, 8, 53360–53373. [Google Scholar] [CrossRef]
- Modiri, A.; Mobayen, S. Adaptive terminal sliding mode control scheme for synchronization of fractional-order uncertain chaotic systems. ISA Trans. 2020, 105, 33–50. [Google Scholar] [CrossRef]
- Song, X.; Song, S.; Balsera, I.T.; Liu, L.; Zhang, L. Synchronization of two fractional-order chaotic systems via nonsingular terminal fuzzy sliding mode control. J. Control Sci. Eng. 2017, 2017, 9562818. [Google Scholar] [CrossRef]
- Sun, Z. Synchronization of fractional-order chaotic systems with non-identical orders, unknown parameters and disturbances via sliding mode control. Chin. J. Phys. 2018, 56, 2553–2559. [Google Scholar] [CrossRef]
- Shao, K.; Xu, Z.; Wang, T. Robust finite-time sliding mode synchronization of fractional-order hyper-chaotic systems based on adaptive neural network and disturbances observer. Int. J. Dyn. Control 2021, 9, 541–549. [Google Scholar] [CrossRef]
- Sabzalian, M.H.; Mohammadzadeh, A.; Lin, S.; Zhang, W. Robust fuzzy control for fractional-order systems with estimated fraction-order. Nonlinear Dyn. 2019, 98, 2375–2385. [Google Scholar] [CrossRef]
- Babu, N.R.; Kalpana, M.; Balasubramaniam, P. A novel audio encryption approach via finite-time synchronization of fractional order hyperchaotic system. Multimed. Tools Appl. 2021, 80, 18043–18067. [Google Scholar] [CrossRef]
- Zhang, L.; Zhong, J.; Lu, J. Intermittent control for finite-time synchronization of fractional-order complex networks. Neural Netw. 2021, 144, 11–20. [Google Scholar] [CrossRef]
- Haghighi, A.; Ziaratban, R. A non-integer sliding mode controller to stabilize fractional-order nonlinear systems. Adv. Differ. Equ. 2020, 2020, 503. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier Science: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Li, C.; Deng, W. Remarks on fractional derivatives. Appl. Math. Comput. 2007, 187, 777–784. [Google Scholar] [CrossRef]
- Wang, H.; Han, Z.-Z.; Xie, Q.-Y.; Zhang, W. Finite-time chaos control via nonsingular terminal sliding mode control. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 2728–2733. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag–Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef]
- Natiq, H.; Said, M.R.M.; Al-Saidi, N.M.G.; Kilicman, A. Dynamics and Complexity of a New 4D Chaotic Laser System. Entropy 2019, 21, 34. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Mou, J.; Ma, C.; Cao, Y. Dynamic analysis of an improper fractional-order laser chaotic system and its image encryption application. Opt. Lasers Eng. 2020, 129, 106031. [Google Scholar] [CrossRef]
- Curran, P.; Chua, L. Absolute stability theory and the synchronization problem. Int. J. Bifurc. Chaos 1997, 7, 1375–1382. [Google Scholar] [CrossRef]
- Fradkov, A.L.; Evans, R.J. Control of chaos: Methods and applications in engineering. Annu. Rev. Control 2005, 29, 33–56. [Google Scholar] [CrossRef]
- Diethelm, K.; Ford, N.J.; Freed, A.D. A Predictor-Corrector Approach for the Numerical Solution of Fractional Differential Equations. Nonlinear Dyn. 2002, 29, 3–22. [Google Scholar] [CrossRef]
- Asl, M.S.; Javidi, M. An improved PC scheme for nonlinear fractional differential equations: Error and stability analysis. J. Comput. Appl. Math. 2017, 324, 101–117. [Google Scholar] [CrossRef]
- Asl, M.S.; Javidi, M.; Yan, Y. High order algorithms for numerical solution of fractional differential equations. Adv. Differ. Equ. 2021, 2021, 111. [Google Scholar] [CrossRef]
- Tu Vu, V.; Pham, T.L.; Dao, P.N. Disturbance observer-based adaptive reinforcement learning for perturbed uncertain surface vessels. ISA Trans. 2022, 130, 277–292. [Google Scholar] [CrossRef]
- Yang, J.; Li, T.; Liu, C.; Li, S.; Chen, W.H. Nonlinearity Estimator-Based Control of A Class of Uncertain Nonlinear Systems. IEEE Trans. Autom. Control 2020, 65, 2230–2236. [Google Scholar] [CrossRef]





| Parameters | b | c | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Scenario 1 | 0.7 | 2.5 | 2.3 | 1.6 | 0.8 | 3.2 | 3.4 | 5.5 | 1.6 | 0.4 | 0.4 | 4.5 | 2.5 | 3.8 | 5.4 | 0.9 |
| Scenario 2 | 0.6 | 1.9 | 2.5 | 2.6 | 0.85 | 4.1 | 3.1 | 5.1 | 2 | 0.4 | 0.4 | 4.5 | 2.5 | 4.2 | 5 | 0.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roohi, M.; Mirzajani, S.; Basse-O’Connor, A. A No-Chatter Single-Input Finite-Time PID Sliding Mode Control Technique for Stabilization of a Class of 4D Chaotic Fractional-Order Laser Systems. Mathematics 2023, 11, 4463. https://doi.org/10.3390/math11214463
Roohi M, Mirzajani S, Basse-O’Connor A. A No-Chatter Single-Input Finite-Time PID Sliding Mode Control Technique for Stabilization of a Class of 4D Chaotic Fractional-Order Laser Systems. Mathematics. 2023; 11(21):4463. https://doi.org/10.3390/math11214463
Chicago/Turabian StyleRoohi, Majid, Saeed Mirzajani, and Andreas Basse-O’Connor. 2023. "A No-Chatter Single-Input Finite-Time PID Sliding Mode Control Technique for Stabilization of a Class of 4D Chaotic Fractional-Order Laser Systems" Mathematics 11, no. 21: 4463. https://doi.org/10.3390/math11214463
APA StyleRoohi, M., Mirzajani, S., & Basse-O’Connor, A. (2023). A No-Chatter Single-Input Finite-Time PID Sliding Mode Control Technique for Stabilization of a Class of 4D Chaotic Fractional-Order Laser Systems. Mathematics, 11(21), 4463. https://doi.org/10.3390/math11214463









