A Numerical Analysis of the Non-Uniform Layered Ground Vibration Caused by a Moving Railway Load Using an Efficient Frequency–Wave-Number Method
Abstract
1. Introduction
2. Solution Method and Verification
2.1. Basic Frequency–Wave-Number Method for Uniform Soil and Program Verification
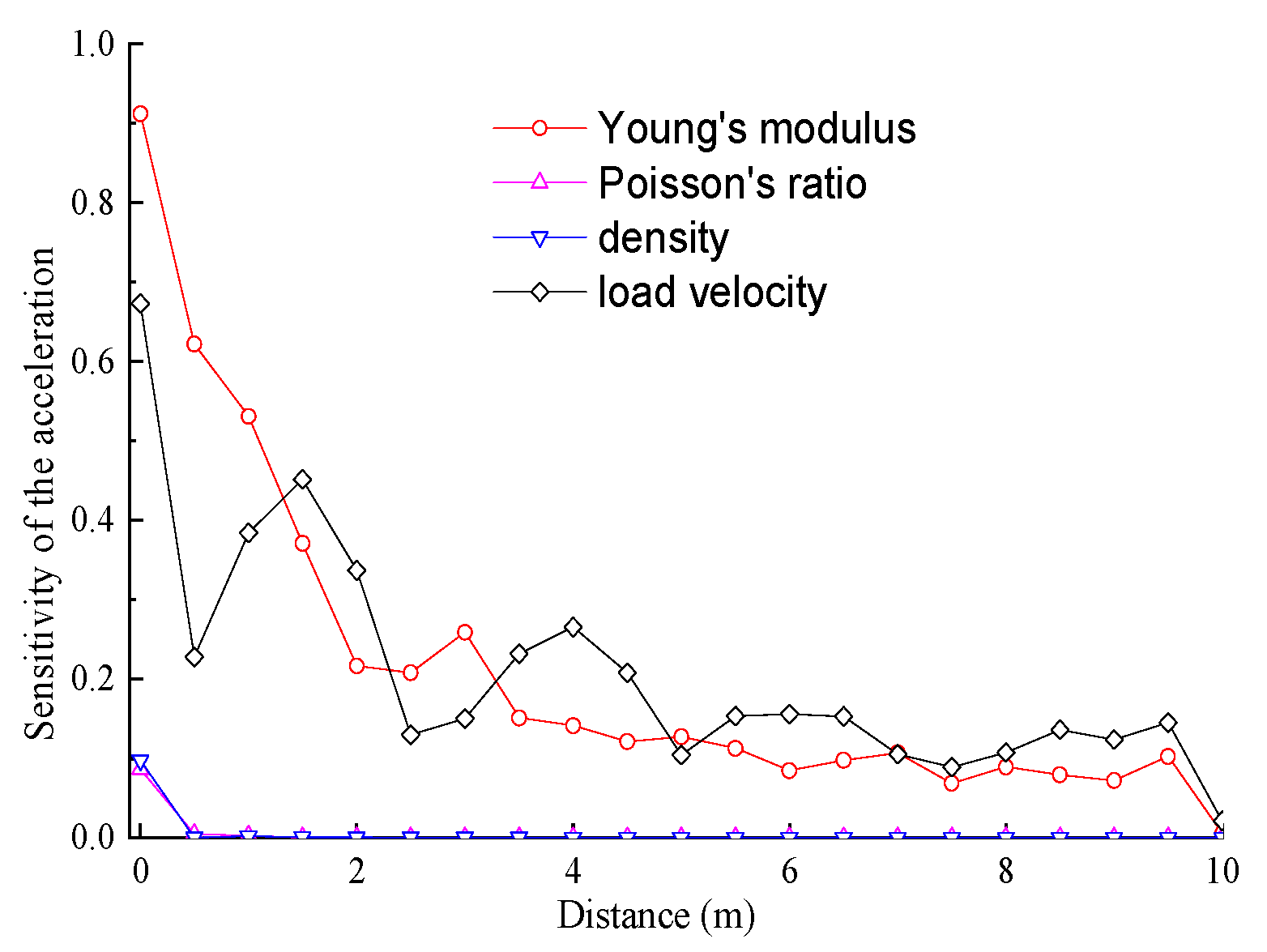
2.2. Finding the Key Factors Affecting the Foundation Vibrations Caused by a Moving Load
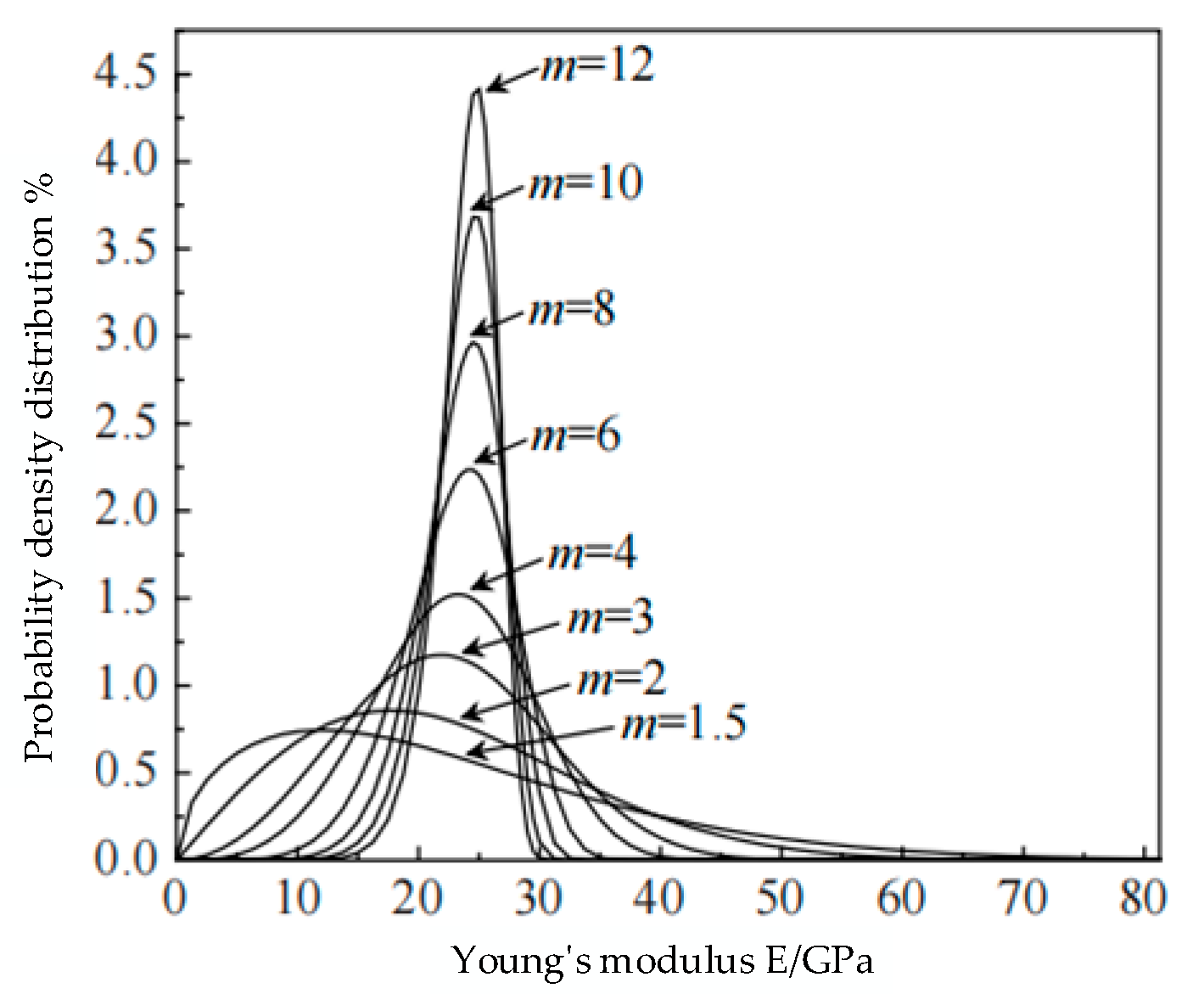
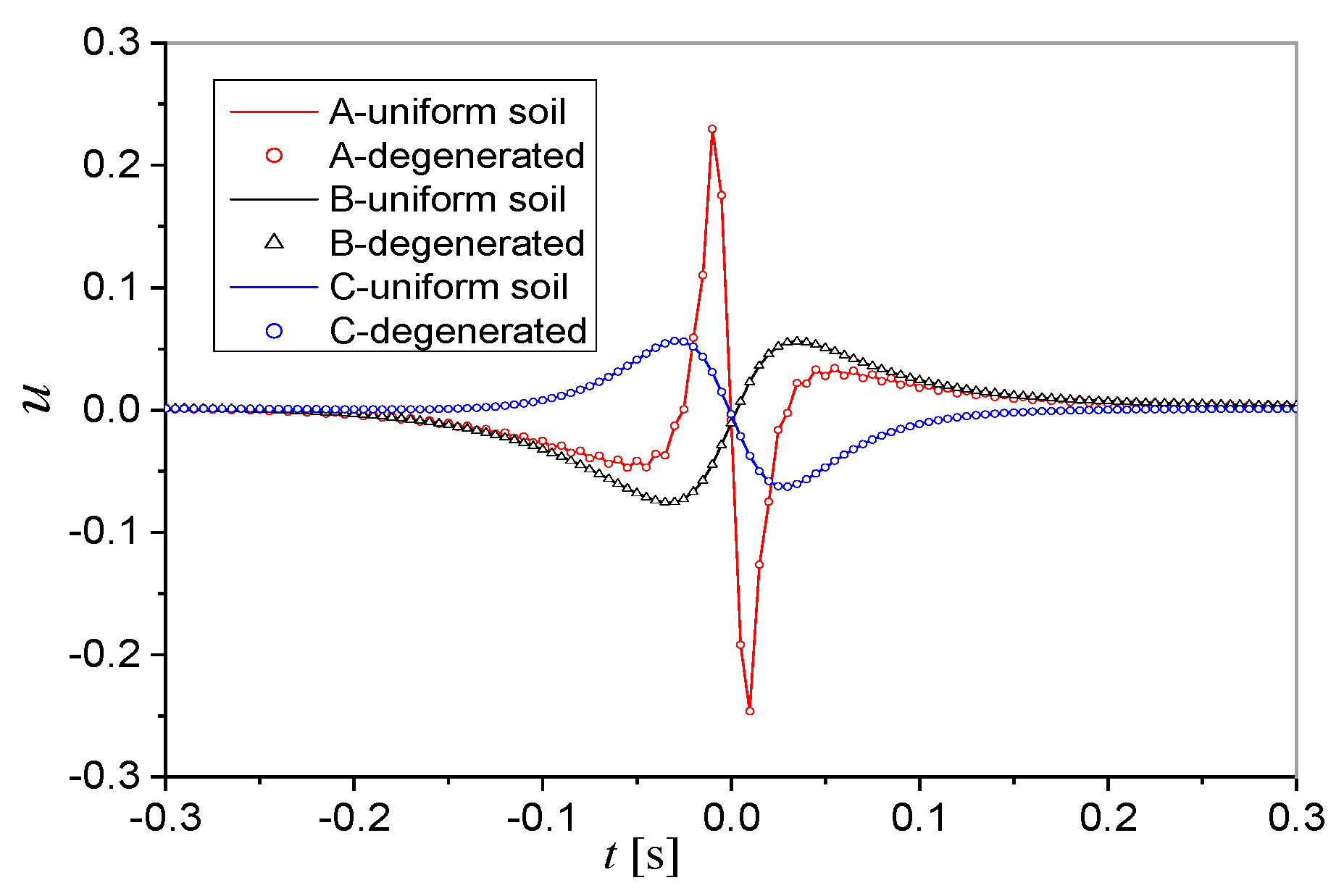
2.3. Random Model Considering Soil Spatial Variability and Program Verification
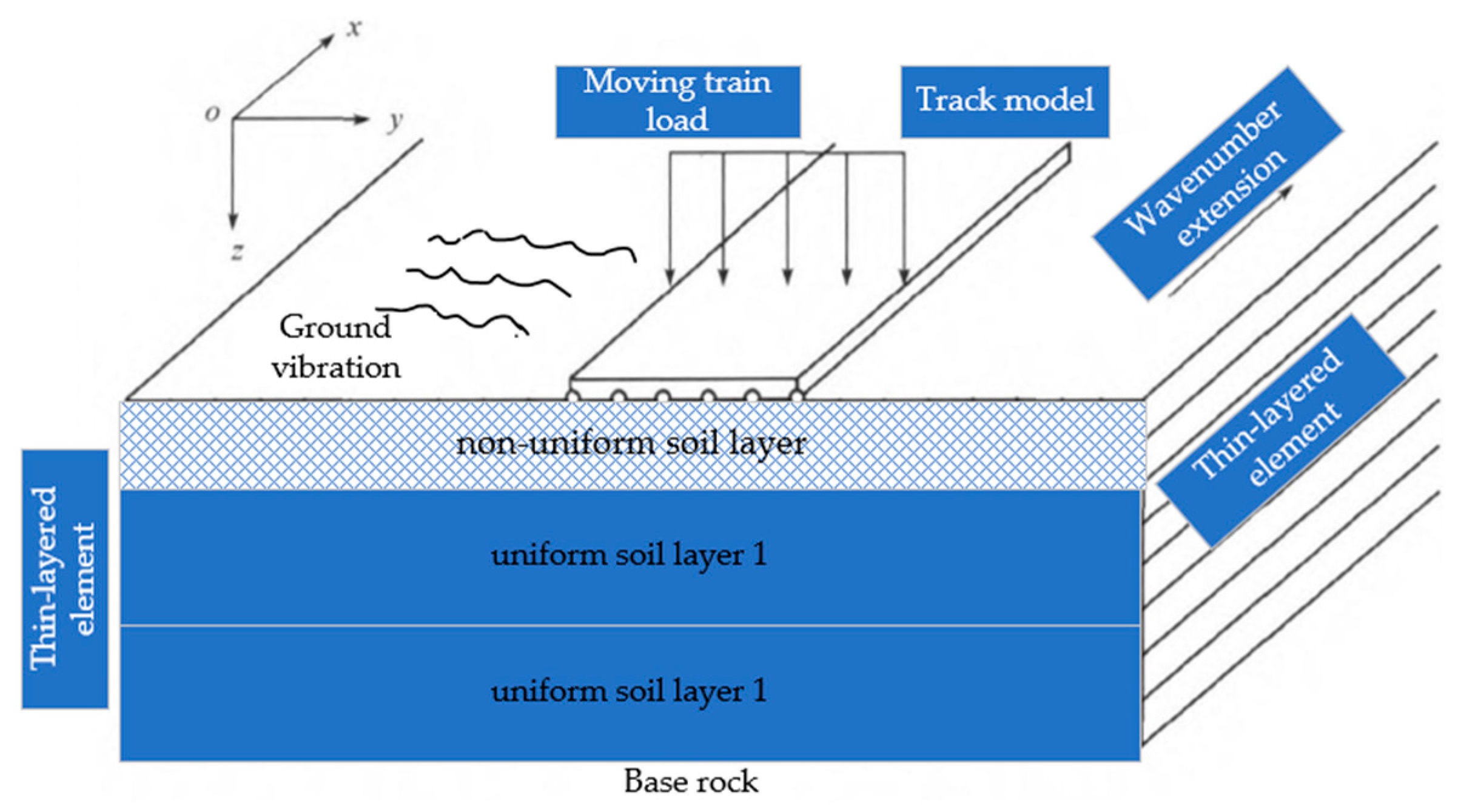
3. Analysis Model of Track and Non-Uniform Layered Ground
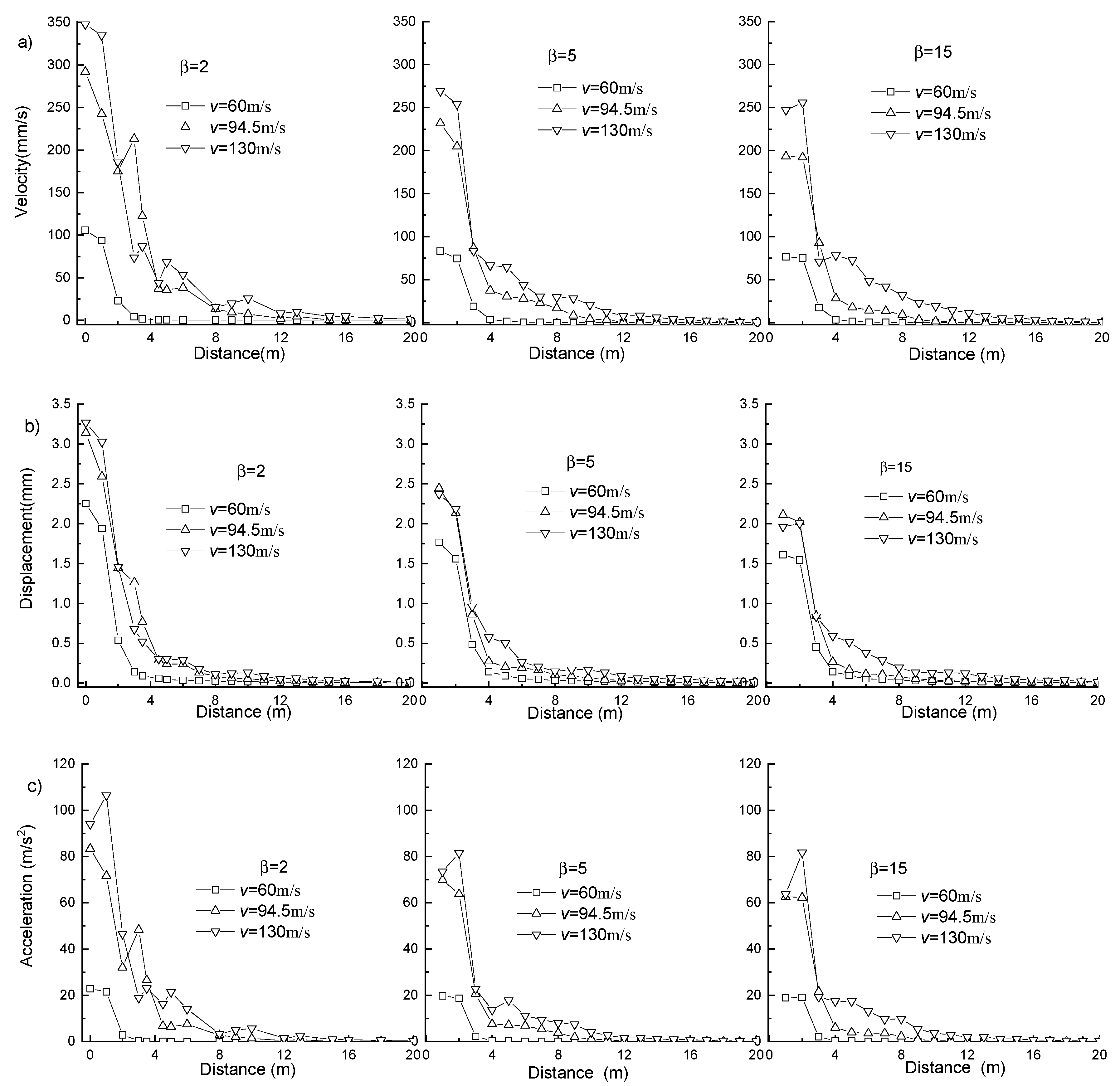
4. Numerical Results of the Ground Vibration at Different Train Speeds and Homogeneity
5. Conclusions and Further Discussion
- (1)
- The heterogeneity of Young’s modulus and the train speed have enormous implications for the ground vibration, while Poisson’s ratio and the soil density have negligible effects.
- (2)
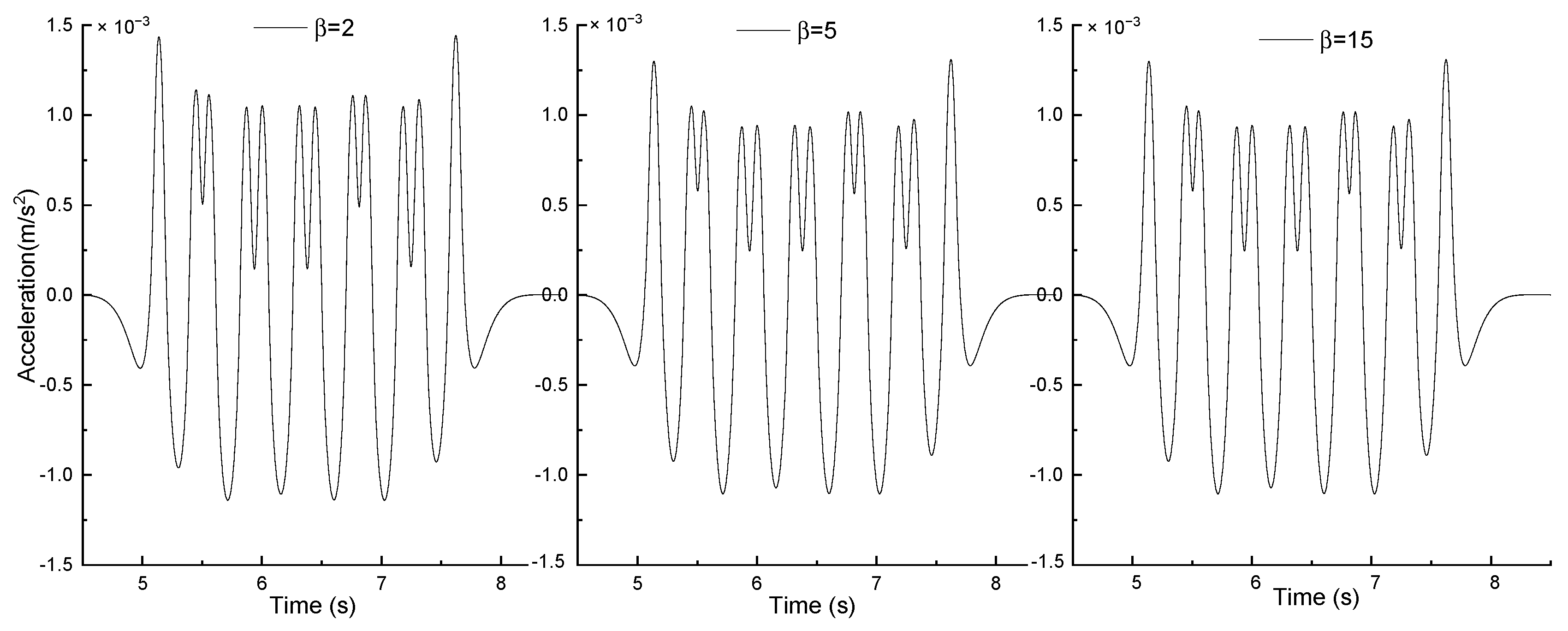
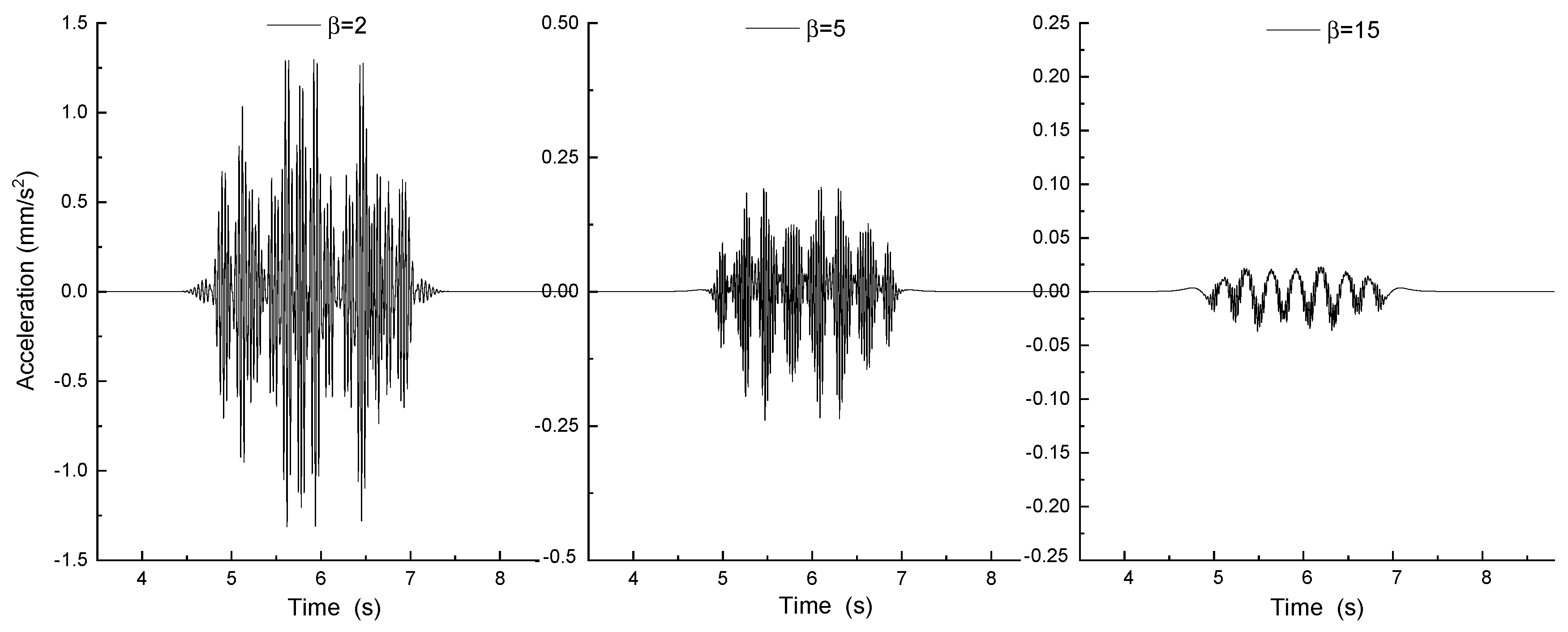
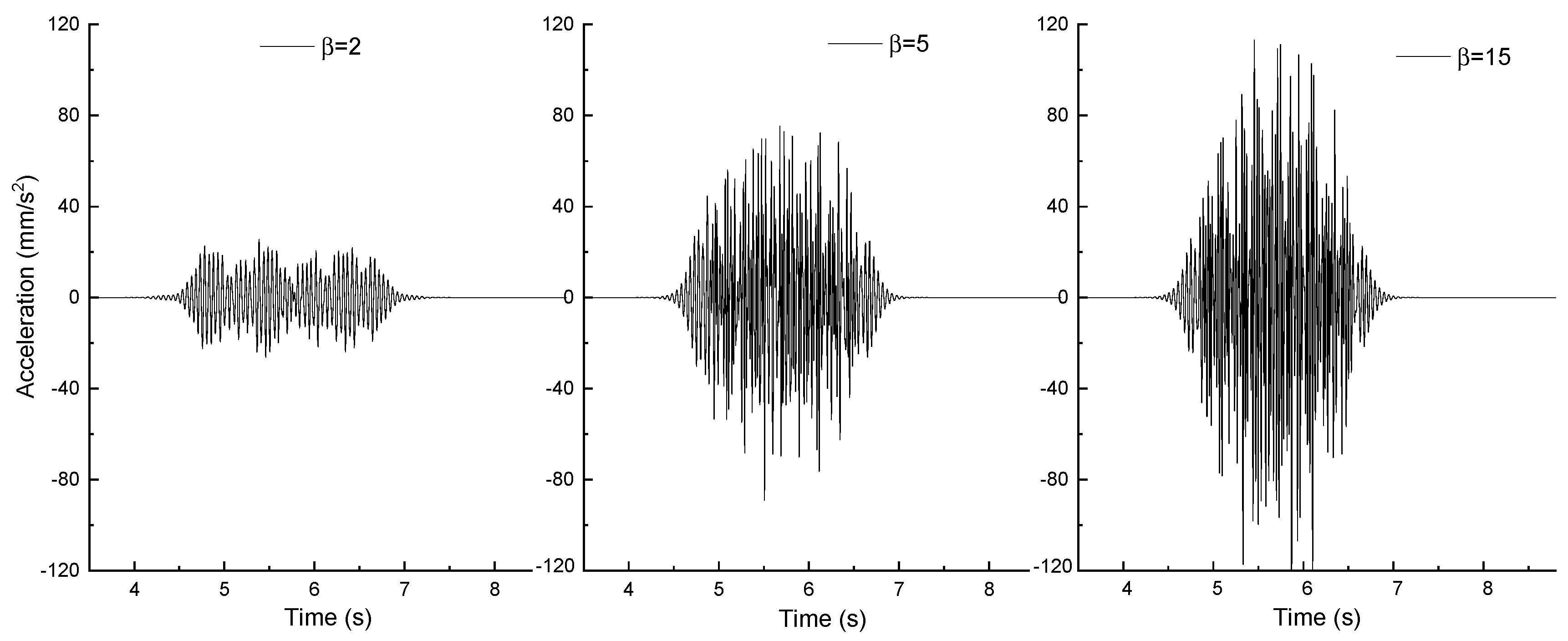
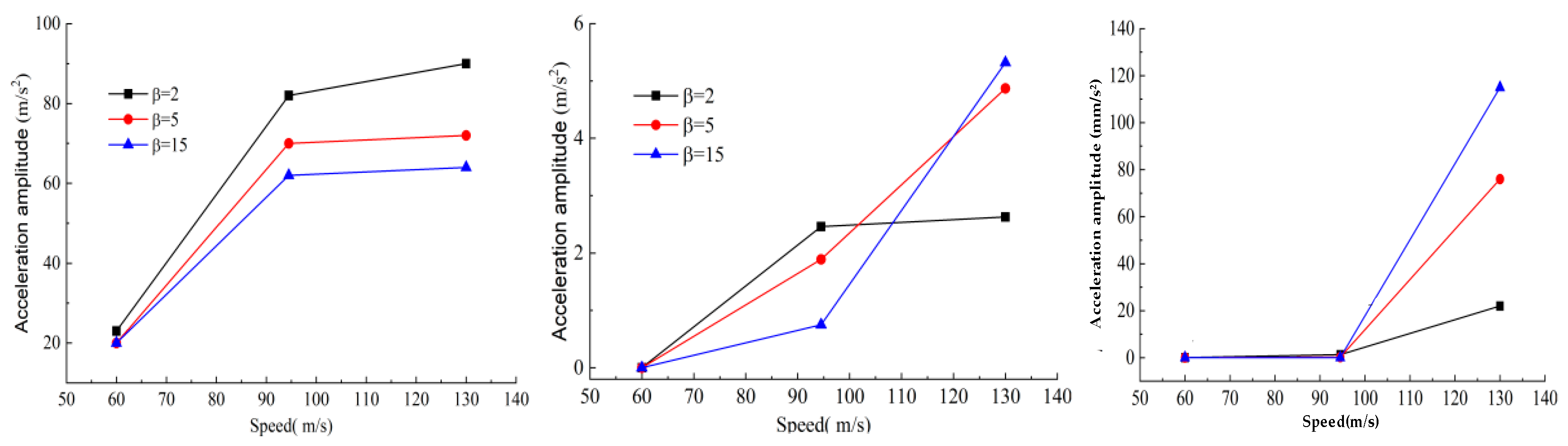
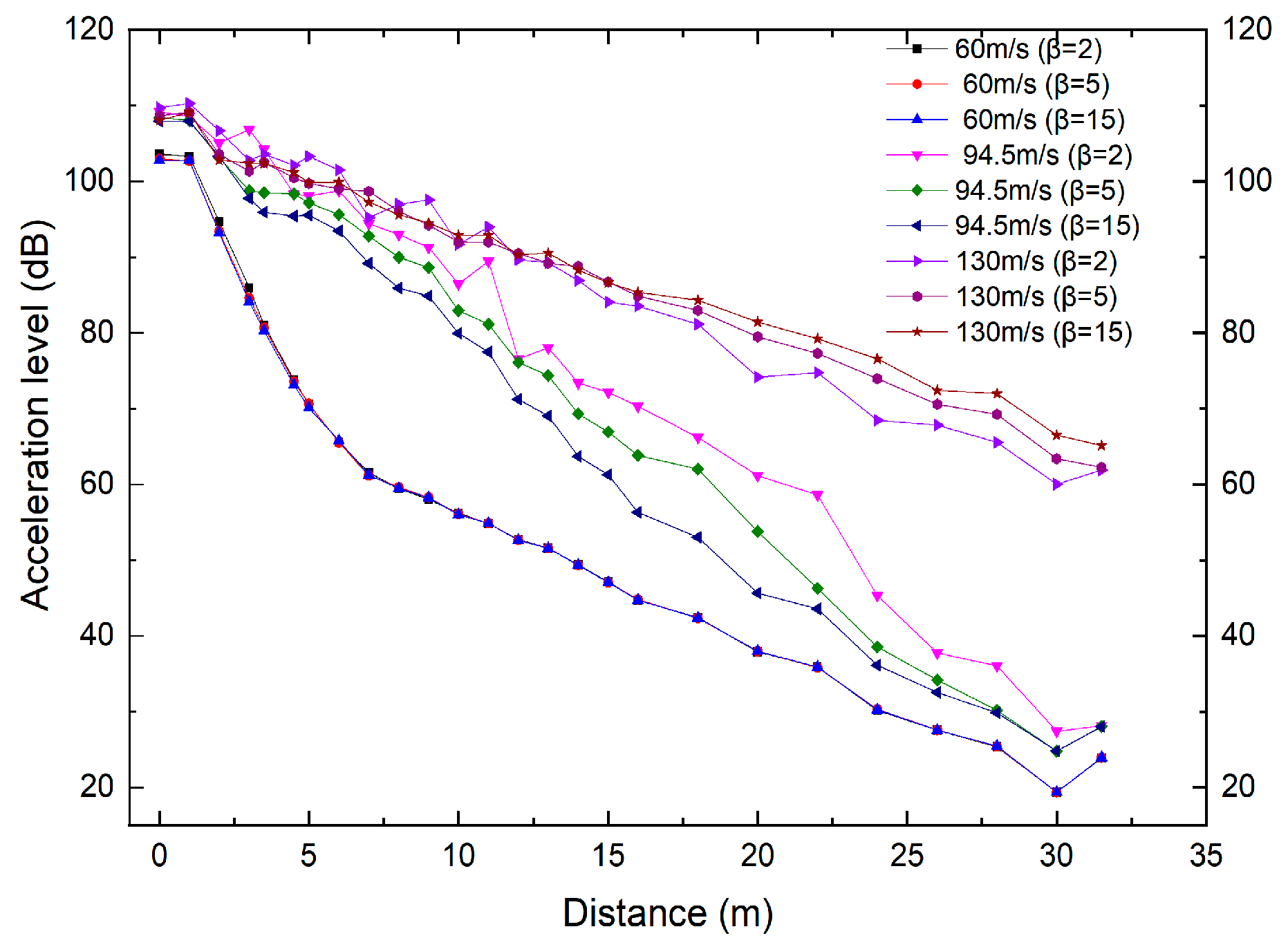
- The upward acceleration is approximately equal to the downward one at a far distance from the track center. The superficial soil homogeneity significantly influences the ground vibration acceleration at 8 m and 28 m, and the impact pattern has a strong correlation with the train speed and the location. As the homogeneity coefficient increases, the peak acceleration continuously decreases in the transonic case, while it gradually increases in the supersonic case. To reduce the far-field acceleration, we can take measures to increase the soil homogeneity in the transonic case, while in the supersonic case, we should take steps to decrease the soil homogeneity.
- (3)
- The dominant frequency remains almost unchanged with varying β, while it shifts significantly with the train speed. The influence range of heterogeneity increases with increasing train speed. A local rebound in attenuation will occur in the supersonic case, and the maximum acceleration occurs at the outer edges of the track. The weak superficial soil can reduce the far-field acceleration level in the supersonic case.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gao, G.Y.; Yao, S.F.; Sun, Y.M. Unsaturated ground vibration induced by high-speed train loads based on 2.5D finite element method. J. Tongji Univ. 2019, 47, 957–966. (In Chinese) [Google Scholar]
- Beskou, N.D. Theodorakopoulos DD. Dynamic effects of moving loads on road pavements: A review. Soil. Dyn. Earthq. Eng. 2011, 31, 547–567. [Google Scholar] [CrossRef]
- Barros, F.C.P.D.; Luco, J.E. Response of a layered viscoelastic half-space to a moving point load. Wave Motion 1994, 19, 189–210. [Google Scholar] [CrossRef]
- Jin, B.; Yue, Z.Q.; Tham, L.G. Stresses and excess pore pressure induced in saturated poroelastic half space by moving line load. Soil. Dyn. Earthq. Eng. 2004, 24–42. [Google Scholar]
- Chen, Y.; Beskou, N.D.; Qian, J. Dynamic response of an elastic plate on a cross-anisotropic poroelastic half-plane to a load moving on its surface. Soil. Dyn. Earthq. Eng. 2018, 107, 292–302. [Google Scholar] [CrossRef]
- Cai, Y.Q.; Sun, H.L.; Xu, C.J. Steady state responses of poroelastic half-space soil medium to a moving rectangular load. Int. J. Solids Struct. 2007, 44, 7183–7196. [Google Scholar] [CrossRef]
- Gao, G.Y.; Yao, S.F.; Yang, C.B. Ground vibration induced by moving train loads on unsaturated soil using 2.5D FEM. J. Harbin Inst. Technol. 2019, 51, 95–103. (In Chinese) [Google Scholar]
- Eason, G. The stresses produced in a semi-infinite solid by a moving surface force. Int. J. Eng. Sci. 1965, 2, 581–609. [Google Scholar] [CrossRef]
- Lu, Z.; Hu, Z.; Yao, H.L.; Liu, J.; Zhan, Y.X. An analytical method for evaluating highway embankment responses with consideration of dynamic wheel-pavement interactions. Soil. Dyn. Earthq. Eng. 2016, 83, 135–147. [Google Scholar] [CrossRef]
- dos Santos, N.C.; Barbosa, J.; Calçada, R.; Delgado, R. Track-ground vibrations induced by railway traffic: Experimental validation of a 3D numerical model. Soil. Dyn. Earthq. Eng. 2017, 97, 324–344. [Google Scholar] [CrossRef]
- Lefeuve-Mesgouez, G.; Mesgouez, A. Three-dimensional dynamic response of a porous multilayered ground under moving loads of various distributions. Adv. Eng. Softw. 2012, 46, 75–84. [Google Scholar] [CrossRef]
- Dieterman, H.A.; Metrikine, A. Steady-state displacements of a beam on an elastic half-space due to a uniformly moving constant load. Eur. J. Mech. A/Solids 1997, 16, 295–306. [Google Scholar]
- Sheng, X.; Jones, C.J.C.; Petyt, M. Ground vibration generated by a load moving along a railway track. J. Sound. Vib. 1999, 228, 129–156. [Google Scholar] [CrossRef]
- Lefeuve-Mesgouez, G.; Le Houédec, D.; Peplow, A.T. Ground vibration in the vicinity of a high-speed moving harmonic strip load. J. Sound. Vib. 2000, 231, 1289–1309. [Google Scholar] [CrossRef]
- Hwang, R.N.; Lysmer, J.J. Response of buried structures to traveling waves. J. Geotech. Eng. Div. 1981, 107, 183–200. [Google Scholar] [CrossRef]
- Ba, Z.N.; Liu, Y.; Liang, J.W. Near-fault broadband seismograms synthesis in a stratified transversely isotropic half-space using a semi-analytical frequency-wavenumber method. Eng. Anal. Bound. Elem. 2023, 146, 1–16. [Google Scholar] [CrossRef]
- Yang, Y.B.; Hung, H.H. A 2.5D finite/infinite element approach for modeling visco-elastic bodies subjected to moving loads. Int. J. Numer. Methods Eng. 2001, 51, 1317–1336. [Google Scholar] [CrossRef]
- Yang, Y.B.; Hung, H.H.; Chang, D.W. Train-induced wave propagation in multi-layered soils using finite/infinite element simulation. Soil. Dyn. Earthq. Eng. 2003, 23, 263–278. [Google Scholar] [CrossRef]
- Takemiya, H.; Bian, X.C. Substructure simulation of inhomogeneous track and layered ground dynamic interaction under train passage. J. Eng. Mech. Div. 2005, 131, 699–711. [Google Scholar] [CrossRef]
- Bian, X.C.; Chen, Y.M.; Hu, T. Numerical simulation of high-speed train induced ground vibrations using 2.5D finite element approach. Sci. China Phys. Mech. Astron. 2008, 51, 632–650. [Google Scholar] [CrossRef]
- Bian, X.C.; Jiang, H.; Chang, C.; Hu, J.; Chen, Y. Track and ground vibrations generated by high-speed train running on ballastless railway with excitation of vertical track irregularities. Soil. Dyn. Earthq. Eng. 2015, 76, 29–43. [Google Scholar] [CrossRef]
- Gao, G.Y.; Yao, S.F.; Yang, J.; Chen, J. Investigating ground vibration induced by moving train loads on unsaturated ground using 2.5D FEM. Soil. Dyn. Earthq. Eng. 2019, 124, 72–85. [Google Scholar] [CrossRef]
- Wang, R.; Hu, Z.P. Current situation and prospects of 2.5D finite element method for the analysis of dynamic response of railwaysubgrade. Rock. Soil. Mech. 2024, 45, 284–301. [Google Scholar]
- Phoon, K.K.; Kulhawy, F.H. Evaluation of geotechnical property variability. Can. Geotech. J. 1999, 36, 625–639. [Google Scholar] [CrossRef]
- Zhang, L.L. Reliability Theory of Geotechnical Engineering; Tongji University Press: Shanghai, China, 2011. (In Chinese) [Google Scholar]
- Papadopoulos, M.; Francois, S.; Degrande, G.; Lombaert, G. The influence of uncertain local subsoil conditions on the response of buildings to ground vibration. J. Sound. Vib. 2018, 418, 200–220. [Google Scholar] [CrossRef]
- Hu, H.; Huang, Y. PDEM-based stochastic seismic response analysis of sites with spatially variable soil properties. Soil. Dyn. Earthq. Eng. 2019, 125, 105736. [Google Scholar] [CrossRef]
- Wang, Y.; He, J.; Shu, S.; Zhang, H.; Wu, Y. Seismic responses of rectangular tunnels in liquefiable soil considering spatial variability of soil properties. Soil. Dyn. Earthq. Eng. 2022, 162, 1074–1089. [Google Scholar] [CrossRef]
- Alamanis, N.; Dakoulas, P. Effects of spatial variability of soil properties and ground motion characteristics on permanent displacements of slopes. Soil. Dyn. Earthq. Eng. 2022, 161, 1–22. [Google Scholar] [CrossRef]
- Deng, E.; Liu, X.Y.; Ni, Y.-Q.; Wang, Y.-W.; Zhao, C.-Y. A coupling analysis method of foundation soil dynamic responses induced by metro train based on PDEM and stochastic field theory. Comput. Geotech. 2023, 154, 1051–1080. [Google Scholar] [CrossRef]
- Wang, M.X.; Wu, Q.; Li, D.Q.; Du, W. Numerical-based seismic displacement hazard analysis for earth slopes considering spatially variable soils. Soil. Dyn. Earthq. Eng. 2023, 171, 1067–1079. [Google Scholar] [CrossRef]
- Coelho, B.Z.; Nuttall, J.; Noordam, A.; Dijkstra, J. The impact of soil variability on uncertainty in predictions of induced vibrations. Soil. Dyn. Earthq. Eng. 2023, 169, 1055–1078. [Google Scholar]
- Gao, G.Y.; Chen, G.Q.; Zhang, B. Analysis of active isolation of vertical non uniform fundamental wave barrier under train load. Vib. Shock. 2013, 32, 57–62. (In Chinese) [Google Scholar]
- Zhou, F.X.; Cao, Y.C.; Zhao, W.G. Dynamic response of non-homogeneous foundation to rectangular moving loads. J. Lanzhou Univ. Technol. 2015, 41, 122–126. (In Chinese) [Google Scholar]
- Zhou, F.X.; Cao, Y.C.; Zhao, W.G. Dynamic response analysis of non-uniform foundation under moving loads. Geotech. Mech. 2015, 36, 2027–2033. (In Chinese) [Google Scholar]
- Ma, Q.; Zhou, F.X. Dynamic response of gradient non-uniform soil under moving loads. J. Nat. Disasters 2018, 27, 61–67. [Google Scholar]
- Shi, L.W.; Ma, Q.; Shu, J.H. Dynamic responses of graded non-homogeneous unsaturated soils under a strip load. Chin. J. Theor. Appl. Mech. 2022, 54, 2008–2018. [Google Scholar]
- Hou, H.; Yang, J.; Cheng, L. Simulation of Viscoelastic Artificial Boundary Based on Thin Layer Element. J. Yangtze River Sci. Res. Inst. 2020, 37, 164–169. (In Chinese) [Google Scholar]
- Connolly, D.; Kouroussis, G.; Giannopoulos, A.; Verlinden, O.; Woodward, P.; Forde, M. Assessment of railway vibrations using an efficient scoping model. Soil. Dyn. Earthq. Eng. 2014, 58, 37–47. [Google Scholar] [CrossRef]
- Feng, Q.S.; Cheng, G. Dynamic Characteristics Analysis of Ballastless Track Stiffness in Frequency Domain by Using Wavenumber Finite Element Method. J. China Railw. Soc. 2017, 39, 102–107. (In Chinese) [Google Scholar]
- Deng, P.H.; Liu, Q.S.; Lu, H.F. FDEM numerical study on the mechanical characteristics and failure behavior of heterogeneous rock based on the Weibull distribution of mechanical parameters. Comput. Geotech. 2023, 154, 105–138. [Google Scholar] [CrossRef]
- He, J.F. Analyses of Ground Dynamic Responses and Settlement by 2.5D FEM for Moving Train; Tongji University Press: Shanghai, China, 2010. (In Chinese) [Google Scholar]
- Costa, P.A.; Colaço, A.; Calçada, R.; Cardoso, A.S. Critical speed of railway tracks: Detailed and simplified approaches. Transp. Geotech. 2015, 2, 30–46. [Google Scholar] [CrossRef]
- Zhai, W.M.; Han, H.Y. Research on ground vibration caused by high-speed trains running on soft soil foundation lines. Sci. China Ser. E 2012, 42, 1148–1156. (In Chinese) [Google Scholar]
- Yao, S.; Yue, L.; Xie, W.; Zheng, S.; Tang, S.; Liu, J.; Wang, W. Investigating the Influence of Non-Uniform Characteristics of Layered Foundation on Ground Vibration Using an Efficient 2.5D Random Finite Element Method. Mathematics 2024, 12, 1488. [Google Scholar] [CrossRef]
- Liang, R.; Liu, W.; Ma, M.; Liu, W.N. An efficient model for predicting the train-induced ground-borne vibration and uncertainty quantification based on Bayesian neural network. J. Sound. Vib. 2020, 495, 908–922. [Google Scholar] [CrossRef]
- Temimi, H.; Kurkcu, H. An accurate asymptotic approximation and precise numerical solution of highly sensitive Troesch’s problem. Appl. Math. Comput. 2014, 235, 253–260. [Google Scholar] [CrossRef]
- Djehiche, B.; N’zi, M.; Owo, J.-M. Stochastic viscosity solutions for SPDEs with continuous coefficients. J. Math. Anal. Appl. 2011, 384, 63–69. [Google Scholar] [CrossRef]
- Gao, G.Y.; Yao, S.F.; Sun, Y.M. 2.5D Finite Element analysis of unsaturated ground vibration induced by High Speed Rail Load. Earth-Quake Eng. Eng. Vib. 2019, 33, 234–245. [Google Scholar]
- Gao, G.Y.; Yao, S.F.; Cui, Y.J. Zoning of confined aquifers inrush and quicksand in Shanghai region. Nat. Hazards 2018, 91, 1341–1363. [Google Scholar] [CrossRef]
- Yao, S.F.; Zhang, Z.N.; Ge, X.R. Correlation between fracture energy and geometrical characteristic of mesostructure of marble. Rock. Soil. Mech. 2016, 37, 2341–2346. [Google Scholar]
- Meng, Z.; Wang, Y.; Zheng, S.; Wang, X.; Liu, D.; Zhang, J.; Shao, Y. Abnormal monitoring data detection based on matrix manipulation and the cuckoo search algorithm. Mathematics 2024, 12, 1345. [Google Scholar] [CrossRef]
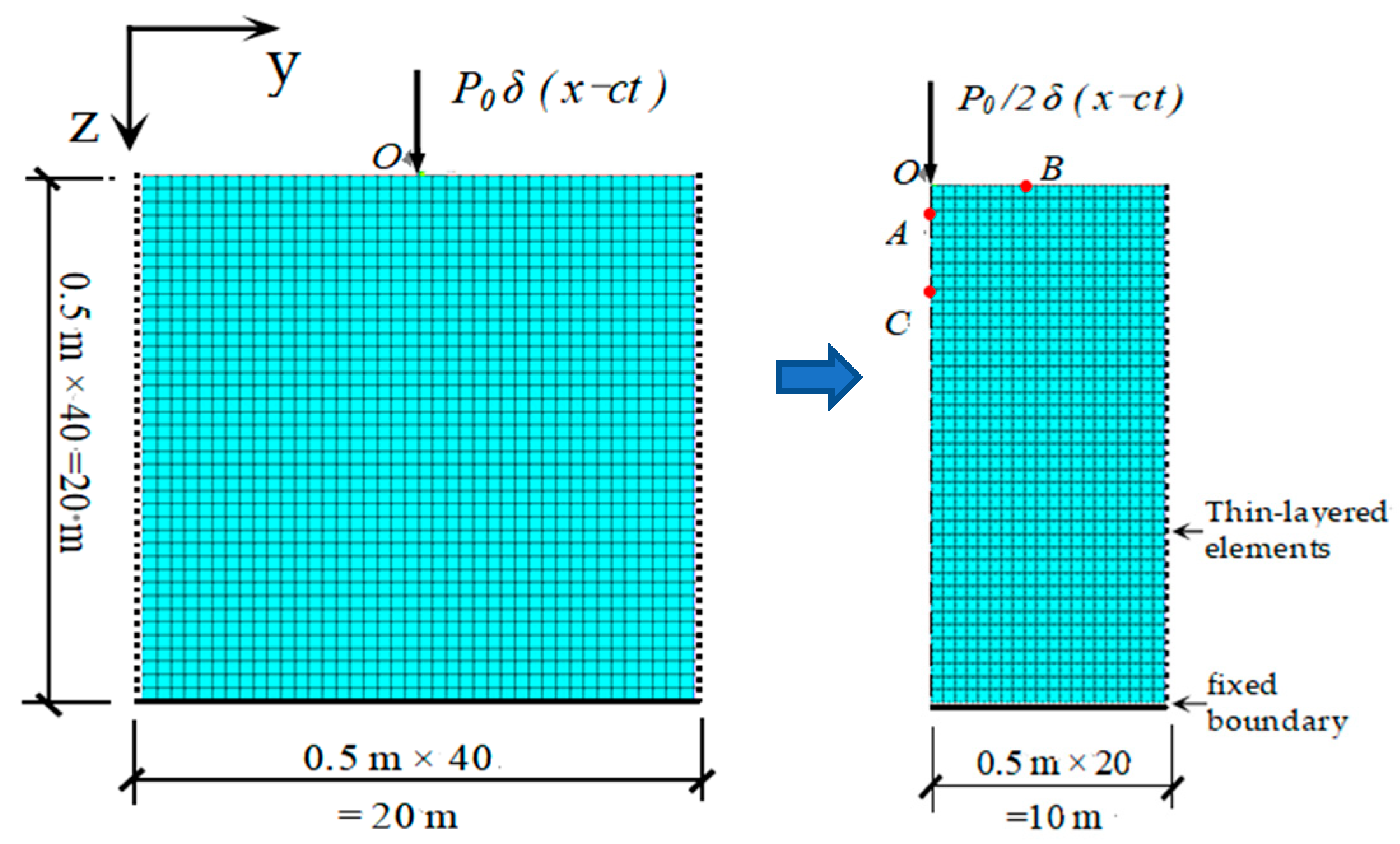
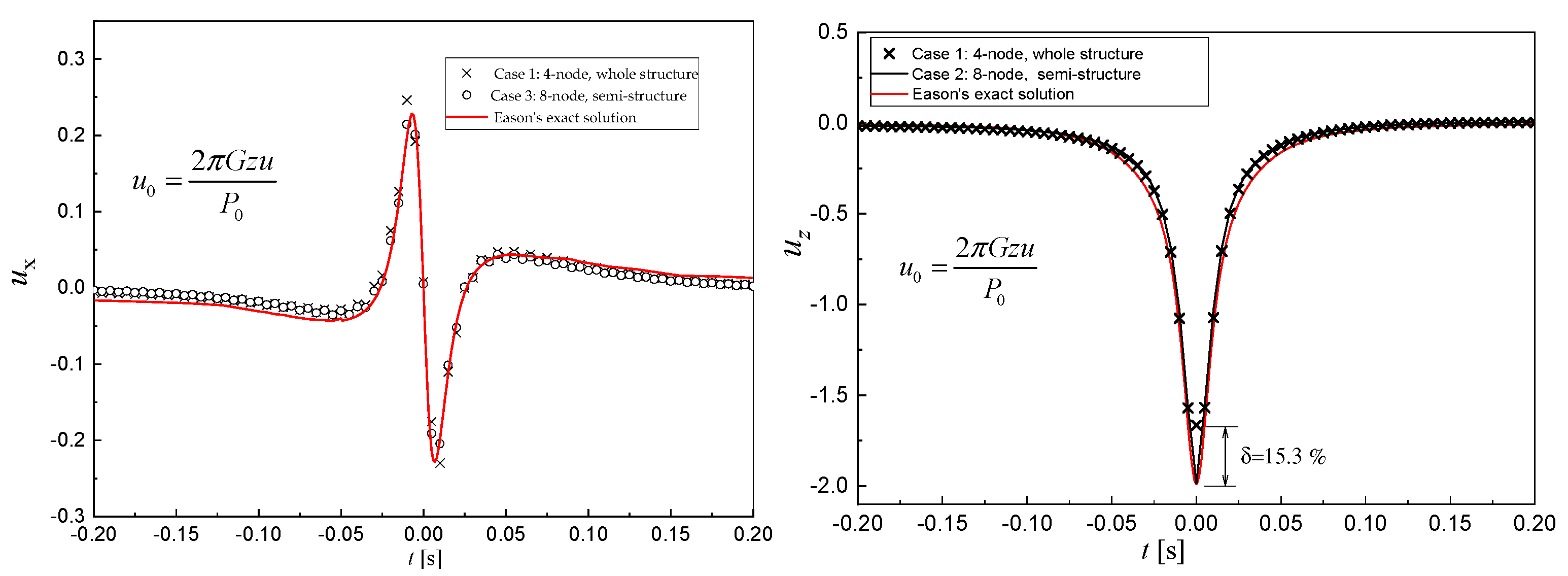
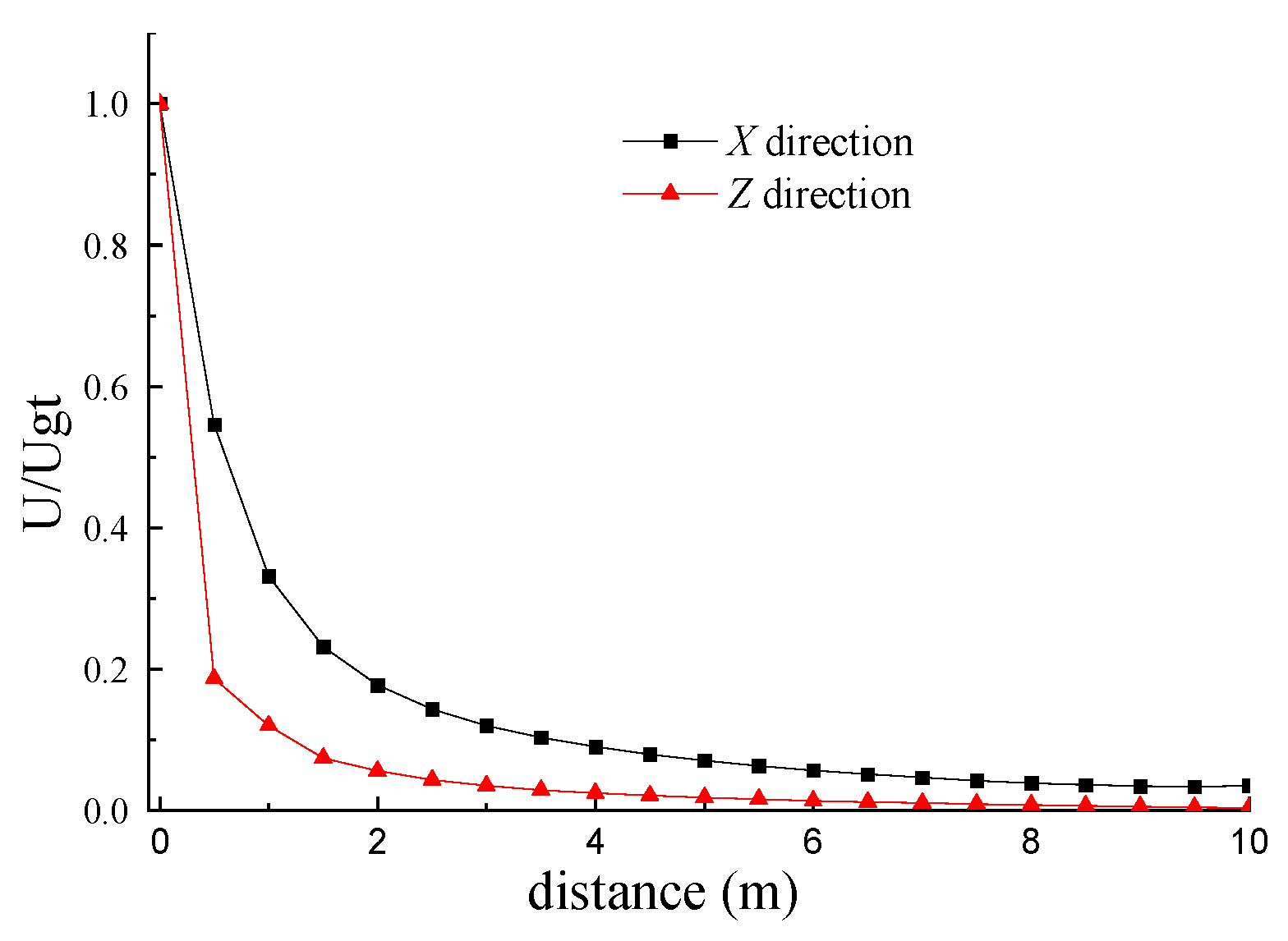
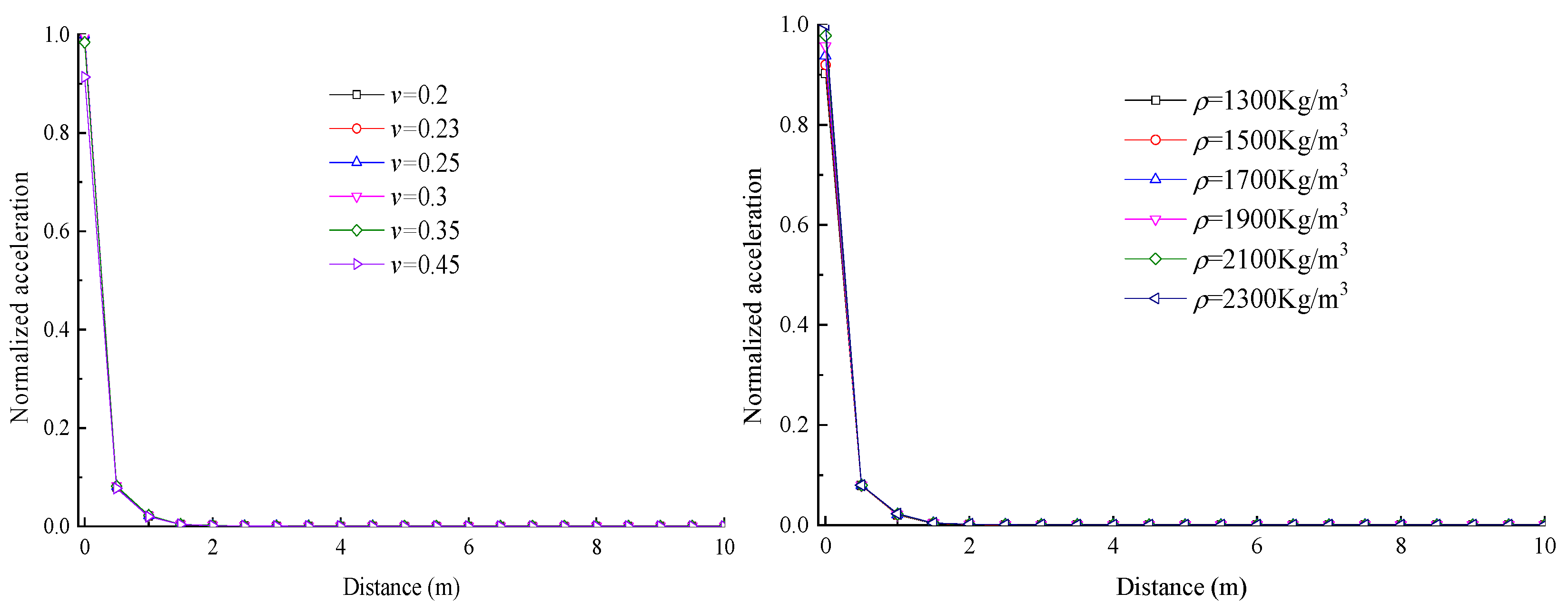





| Parameters | Young’s Modulus (MPa) | Poisson’s Ratio | Density (kg/m3) | Load Velocity (m/s) |
|---|---|---|---|---|
| Benchmark value | 50 | 0.25 | 2000 | 100.0 |
| Variation range | [20, 120] | [0.20, 0.45] | [1300, 2300] | [55.6, 164.0] |
| Young’s Modulus (MPa) | Poisson’s Ratio | Density (kg/m3) | Load Velocity (m/s) |
|---|---|---|---|
| 20 | 0.20 | 1300 | 55.6 |
| 25 | 0.23 | 1500 | 83.3 |
| 40 | 0.25 | 1700 | 97.2 |
| 60 | 0.30 | 1900 | 111.1 |
| 100 | 0.35 | 2100 | 125.0 |
| 120 | 0.45 | 2300 | 164.0 |
| Soil Layer No. | Thickness (m) | Shear Wave Velocity (m/s) | Density (kg/m3) | Poisson’s Ratio | Damping Coefficient | Mean Value of Elastic Modulus (107 pa) | Homogeneity Coefficient β | Shear Modulus (107 pa) |
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 95 | 1500 | 0.35 | 0.05 | 3.66 | 2, 5, 15 | 1.35 |
| 2 | 2 | 150 | 1700 | 0.30 | 0.05 | 9.95 | 200 | 3.83 |
| 3 | 26 | 280 | 1800 | 0.25 | 0.05 | 35.28 | 200 | 14.11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, S.; Xie, W.; Geng, J.; Xu, X.; Zheng, S. A Numerical Analysis of the Non-Uniform Layered Ground Vibration Caused by a Moving Railway Load Using an Efficient Frequency–Wave-Number Method. Mathematics 2024, 12, 1750. https://doi.org/10.3390/math12111750
Yao S, Xie W, Geng J, Xu X, Zheng S. A Numerical Analysis of the Non-Uniform Layered Ground Vibration Caused by a Moving Railway Load Using an Efficient Frequency–Wave-Number Method. Mathematics. 2024; 12(11):1750. https://doi.org/10.3390/math12111750
Chicago/Turabian StyleYao, Shaofeng, Wei Xie, Jianlong Geng, Xiaolu Xu, and Sen Zheng. 2024. "A Numerical Analysis of the Non-Uniform Layered Ground Vibration Caused by a Moving Railway Load Using an Efficient Frequency–Wave-Number Method" Mathematics 12, no. 11: 1750. https://doi.org/10.3390/math12111750
APA StyleYao, S., Xie, W., Geng, J., Xu, X., & Zheng, S. (2024). A Numerical Analysis of the Non-Uniform Layered Ground Vibration Caused by a Moving Railway Load Using an Efficient Frequency–Wave-Number Method. Mathematics, 12(11), 1750. https://doi.org/10.3390/math12111750






