Light-Fueled Self-Propulsion of Liquid Crystal Elastomer-Engined Automobiles in Zero-Energy Modes
Abstract
:1. Introduction
2. Model and Formulation
2.1. Dynamics of the Self-Propulsion LCE Turntable-Engined Automobile
2.2. Photothermally Responsive LCE Rope Model
2.3. Nondimensionalization and Solution Method
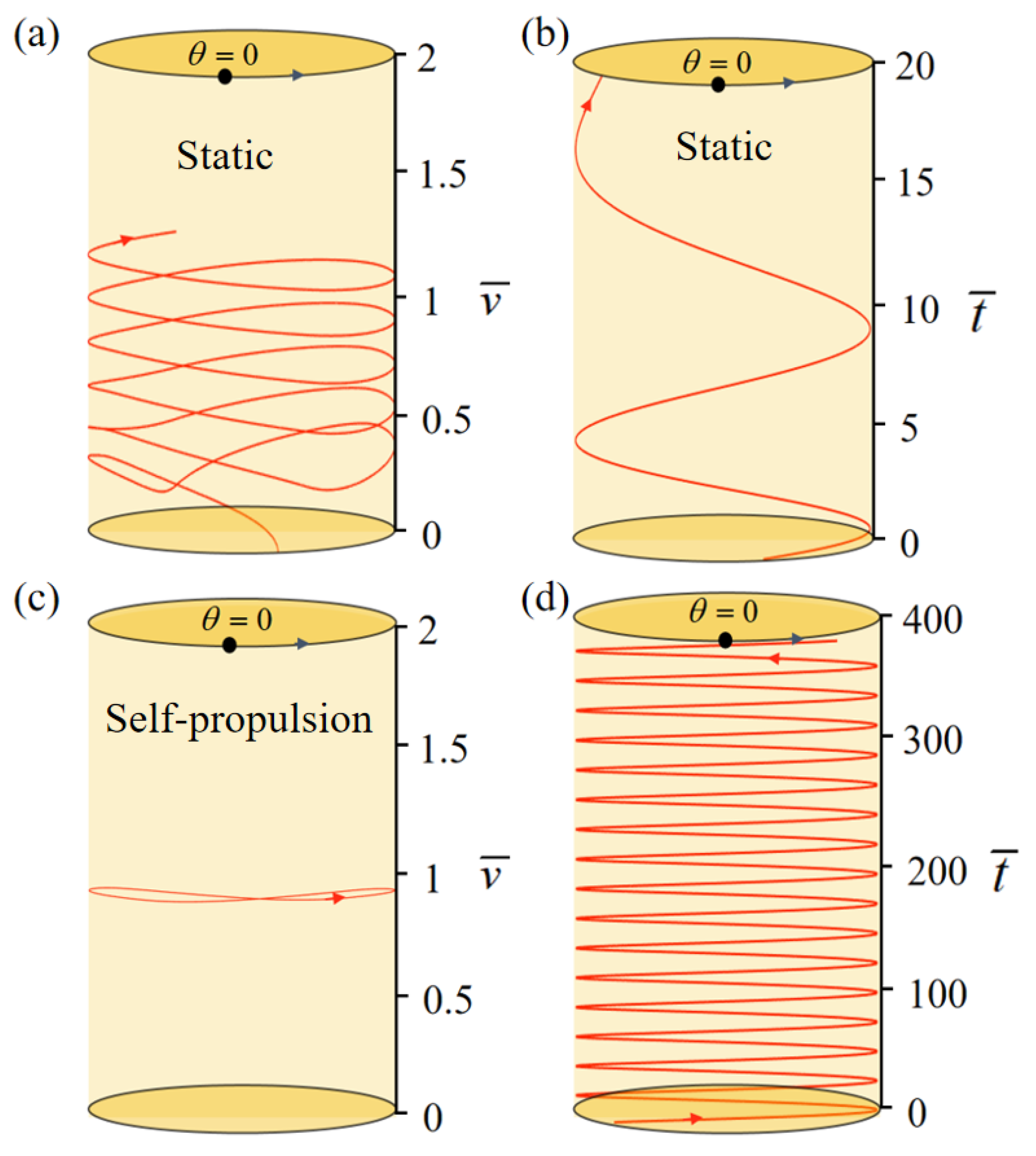
3. Two Motion Patterns and Mechanism of Self-Excited Motion
3.1. Two Motion Patterns
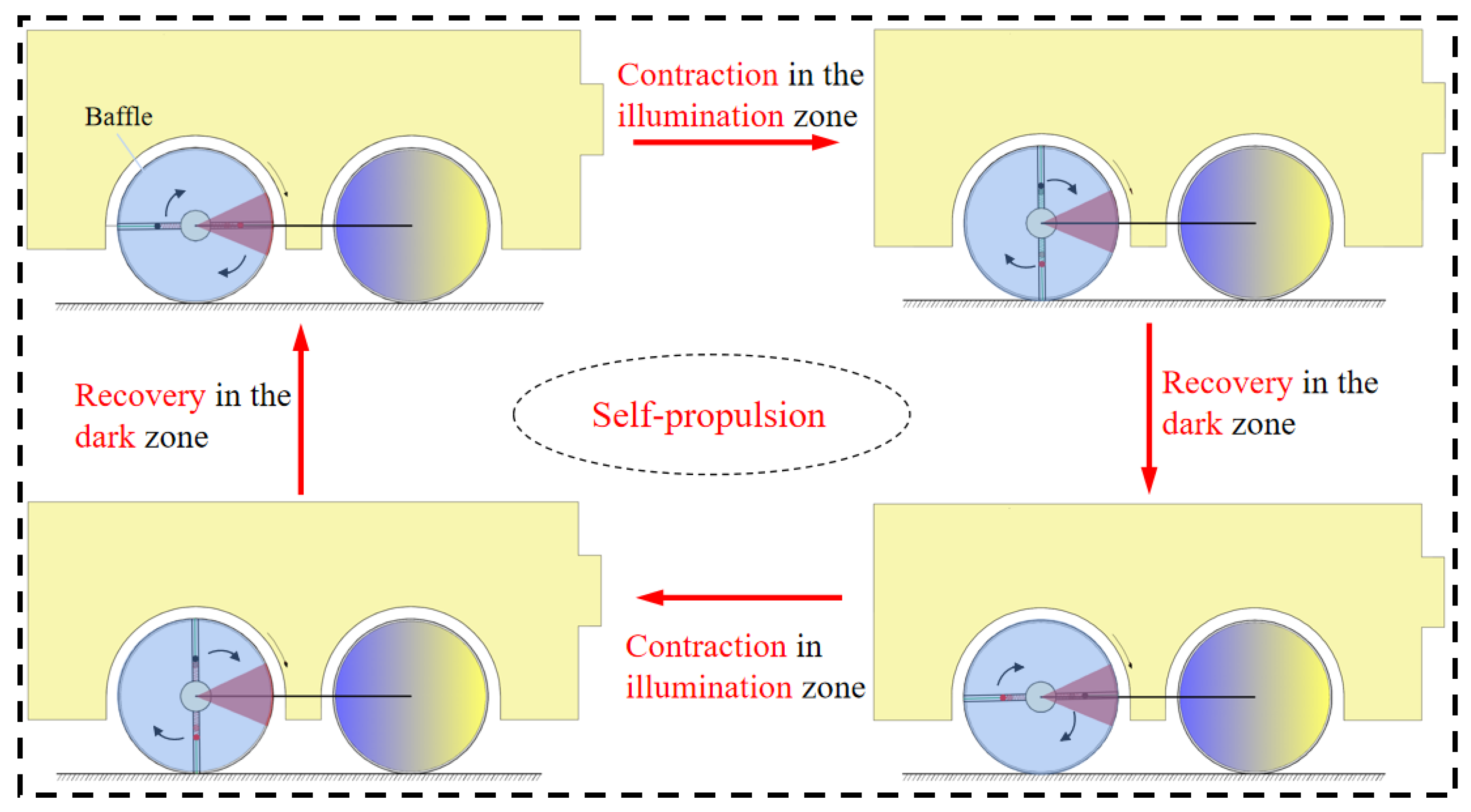
3.2. Mechanism of Self-Propulsion
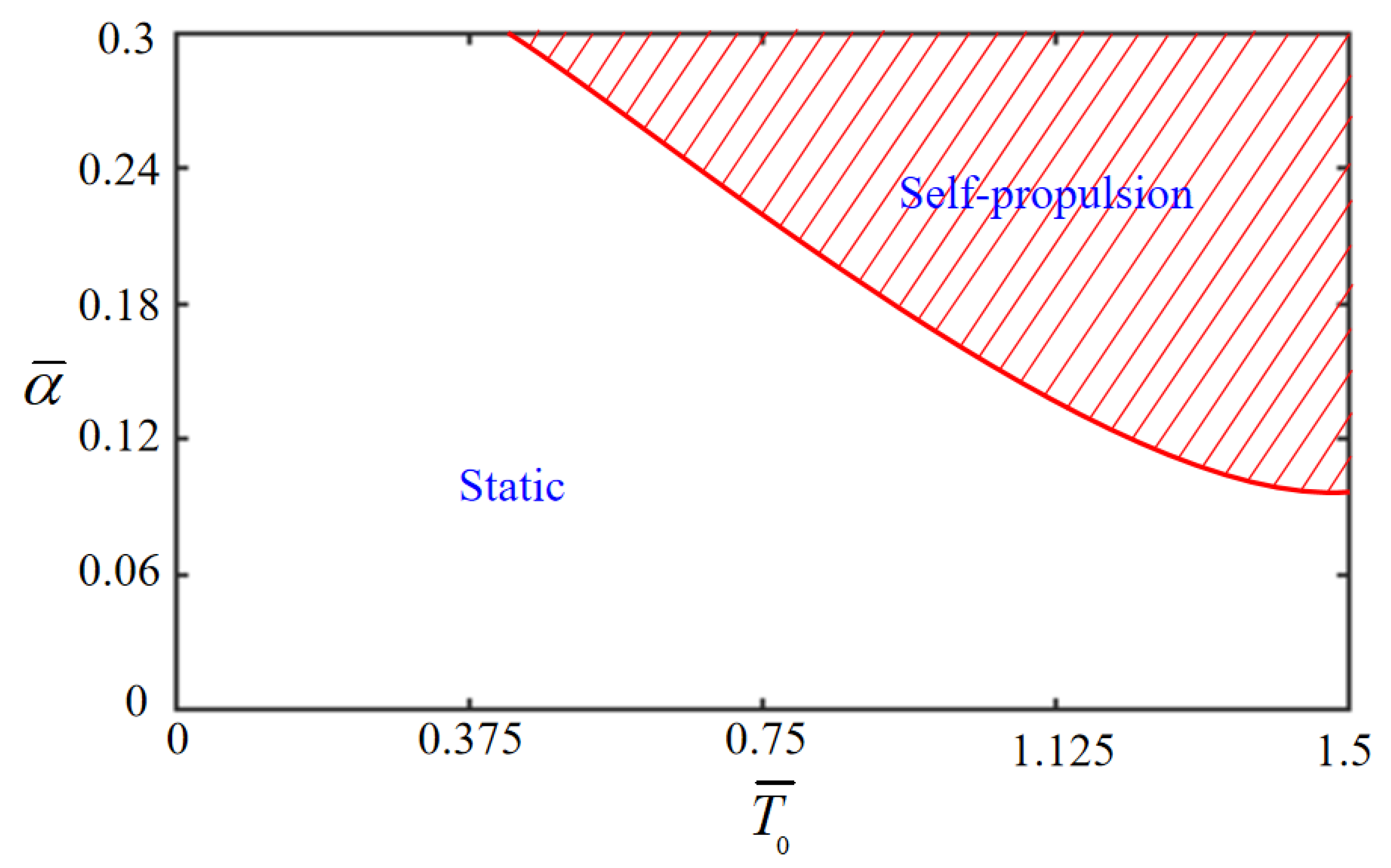
3.3. Critical Condition for the Self-Propulsion
4. Parametric Study
4.1. Effect of Gravitational Acceleration
4.2. Effect of Limit Temperature
4.3. Effect of Thermal Contraction Coefficient
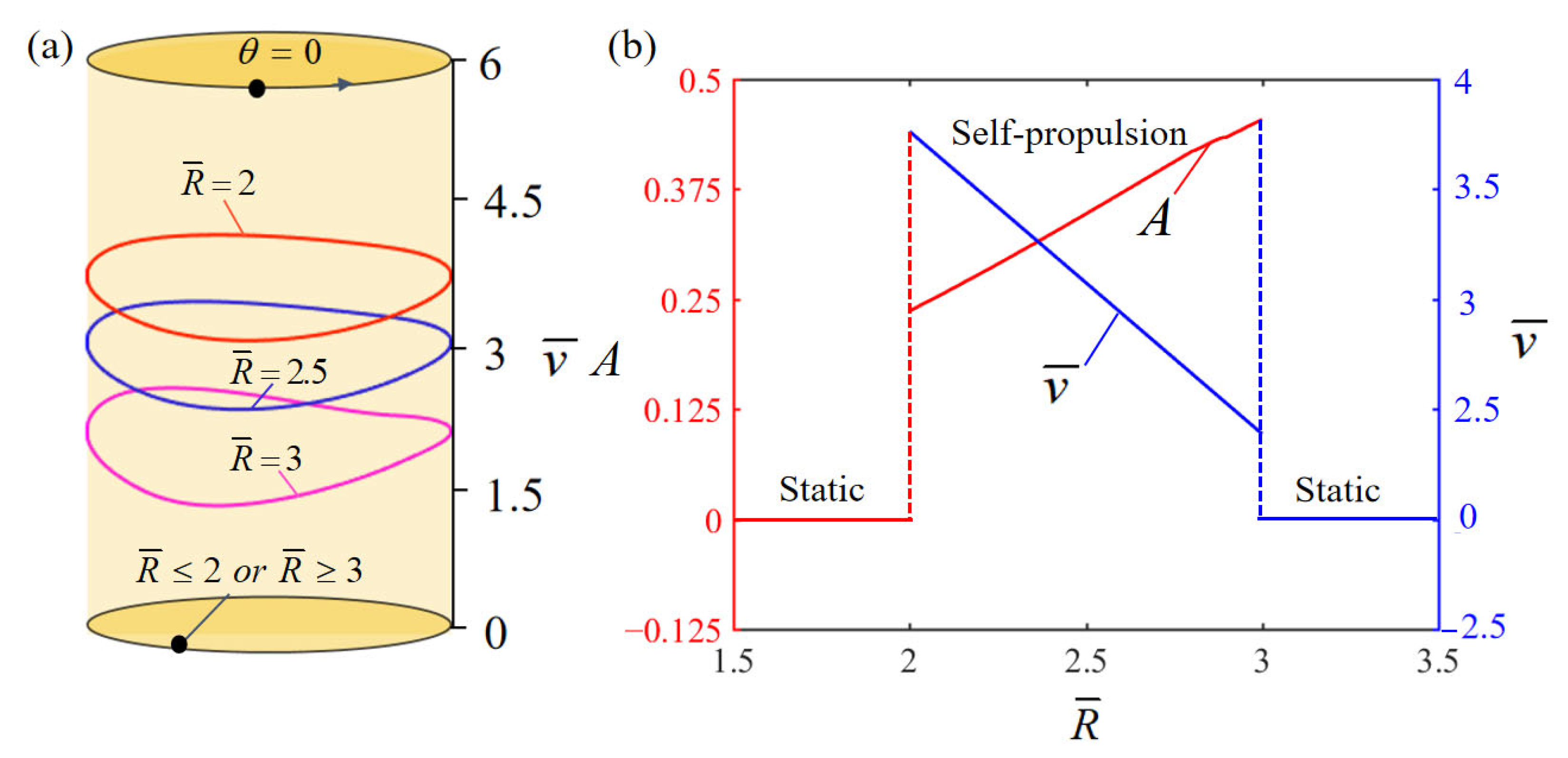
4.4. Effect of Turntable Radius
4.5. Effect of Damping Factor
4.6. Effect of Rolling Resistance Coefficient
4.7. Effect of Elastic Stiffness of LCE Rope
4.8. Effect of Elastic Stiffness of Spring
4.9. Effect of Illumination Zone Angle
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ding, W.J. Self-Excited Vibration; Tsing–Hua University Press: Beijing, China, 2009. [Google Scholar]
- Liu, Z.; Qi, M.; Zhu, Y.; Huang, D.; Zhang, X.; Lin, L.; Yan, X. Mechanical response of the isolated cantilever with a floating potential in steady electrostatic field. Int. J. Mech. Sci. 2019, 161, 105066. [Google Scholar] [CrossRef]
- Charroyer, L.; Chiello, O.; Sinou, J.J. Self−excited vibrations of a nonsmooth contact dynamical system with planar friction based on the shooting method. Int. J. Mech. Sci. 2018, 144, 90–101. [Google Scholar] [CrossRef]
- Hu, W.; Lum, G.Z.; Mastrangeli, M.; Sitti, M. Small−scale soft−bodied robot with multimodal locomotion. Nature 2018, 554, 81–85. [Google Scholar] [CrossRef] [PubMed]
- Iliuk, I.; Balthazar, J.M.; Tusset, A.M.; Piqueira, J.R.; Pontes, D.B.R.; Felix, J.L.; Bueno, A.M. Application of passive control to energy harvester efficiency using a nonideal portal frame structural support system. J. Intell. Mater. Syst. Struct. 2014, 25, 417–429. [Google Scholar] [CrossRef]
- Korner, K.; Kuenstler, A.S.; Hayward, R.C.; Audoly, B.; Bhattacharya, K. A nonlinear beam model of photomotile structures. Proc. Natl. Acad. Sci. USA 2020, 117, 9762–9770. [Google Scholar] [CrossRef] [PubMed]
- Martella, D.; Nocentini, S.C.; Parmeggiani, C.; Wiersma, D.S. Self−regulating capabilities in photonic robotics. Adv. Mater. Technol. 2019, 4, 1800571. [Google Scholar] [CrossRef]
- Sangwan, V.; Taneja, A.; Mukherjee, S. Design of a robust self−excited biped walking mechanism. Mech. Theory 2004, 39, 1385–1397. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. Piezoelectric Energy Harvesting; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Rosso, M. Intentional and Inherent Nonlinearities in Piezoelectric Energy Harvesting; Springer Nature: Berlin/Heidelberg, Germany, 2024. [Google Scholar]
- Briand, D.; Yeatman, E.; Roundy, S. Micro Energy Harvesting; John Wiley & Sons: Hoboken, NJ, USA, 2015; ISBN 978-3-527-31902-2. [Google Scholar]
- Mohsen, S.; Henry, A.S.; Steven, R.A. A review of energy harvesting using piezoelectric materials: State-of-the-art a decade later (2008–2018). Smart Mater. Struct. 2019, 28, 113001. [Google Scholar]
- Lin, Y.-L.; Kyung, C.-M.; Hiroto, Y.; Liu, Y. Smart Sensors and Systems; Springer Nature Switzerland AG: Cham, Switzerland, 2020. [Google Scholar]
- Ambaye, G.; Boldsaikhan, E.; Krishnan, K. Soft Robot Design, Manufacturing, and Operation Challenges: A Review. J. Manuf. Mater. Process. 2024, 8, 79. [Google Scholar] [CrossRef]
- Yang, Z. Advanced MEMS/NEMS Fabrication and Sensors; Springer: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Alberto, C.; Raffaele, A.; Claudia, C.; Attilio, F.; Aldo, G. Stefano Mariani, Mechanics of Microsystems; Wiley: Hoboken, NJ, USA, 2018; ISBN 9781119053835. [Google Scholar]
- Yoshida, R. Self−oscillating gels driven by the Belousov−Zhabotinsky reaction as novel smart materials. Adv. Mater. 2010, 22, 3463–3483. [Google Scholar] [CrossRef]
- Yashin, V.V.; Balazs, A.C. Pattern formation and shape changes in self−oscillating polymer gels. Science 2006, 314, 798–801. [Google Scholar] [CrossRef]
- Boissonade, J.; Kepper, P.D. Multiple types of spatio−temporal oscillations induced by differential diffusion in the Landolt reaction. Phys. Chem. Chem. Phys. 2011, 13, 4132–4137. [Google Scholar] [CrossRef] [PubMed]
- He, Q.G.; Wang, Z.J.; Wang, Y.; Wang, Z.J.; Li, C.H.; Annapooranan, R.; Zeng, J.; Chen, R.K.; Cai, S. Electrospun liquid crystal elastomer microfiber actuator. Sci. Robot. 2021, 6, eabi9704. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Zhang, C.; Chen, B.; Wang, Z.; Xu, Y.; Xiao, R. Bioinspired design of stimuli-responsive artificial muscles with multiple actuation modes. Smart Mater. Struct. 2023, 32, 085023. [Google Scholar] [CrossRef]
- Park, S.; Oh, Y.; Moon, J.; Chung, H. Recent Trends in Continuum Modeling of Liquid Crystal Networks: A Mini−Review. Polymers 2023, 15, 1904. [Google Scholar] [CrossRef]
- Hu, Z.; Li, Y.; Lv, J. Phototunable self−oscillating system driven by a self−winding fiber actuator. Nat. Commun. 2021, 12, 3211. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Chi, Y.; Hong, Y.; Li, Y.; Yang, S.; Yin, J. Twisting for soft intelligent autonomous robot in unstructured environments. Proc. Natl. Acad. Sci. USA 2022, 119, e2200265119. [Google Scholar] [CrossRef] [PubMed]
- Graeber, G.; Regulagadda, K.; Hodel, P.; Küttel, C.; Landolf, D.; Schutzius, T.; Poulikakos, D. Leidenfrost droplet trampolining. Nat. Commun. 2021, 12, 1727. [Google Scholar] [CrossRef]
- Chakrabarti, A.; Choi, G.P.T.; Mahadevan, L. Self−Excited Motions of Volatile Drops on Swellable Sheets. Phys. Rev. Lett. 2020, 124, 258002. [Google Scholar] [CrossRef]
- Lv, X.; Yu, M.; Wang, W.; Yu, H. Photothermal pneumatic wheel with high loadbearing capacity. Compos. Commun. 2021, 24, 100651. [Google Scholar] [CrossRef]
- Tang, R.; Liu, Z.; Xu, D.; Liu, J.; Yu, L.; Yu, H. Optical pendulum generator based on photomechanical liquid−crystalline actuators. ACS Appl. Mater. Interfaces 2015, 7, 8393–8397. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Lou, J.; Zhang, B.; Dai, Y.; Li, K. Stability analysis of a liquid crystal elastomer self-oscillator under a linear temperature field. Appl. Math. Mech. (Engl. Ed.) 2024, 45, 337–354. [Google Scholar] [CrossRef]
- Lahikainen, M.; Zeng, H.; Priimagi, A. Reconfigurable photoactuator through synergistic use of photochemical and photo thermal effects. Nat. Commun. 2018, 9, 4148. [Google Scholar] [CrossRef]
- Kim, Y.; Berg, J.; Crosby, A.J. Autonomous snapping and jumping polymer gels. Nat. Mater. 2021, 20, 1695–1701. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Dai, Y.; Li, K.; Xu, P. Theoretical study of chaotic jumping of liquid crystal elastomer ball under periodic illumination. Nonlinear Dyn. 2024, 112, 5349–5360. [Google Scholar] [CrossRef]
- Xu, P.; Chen, Y.; Wu, H.; Dai, Y.; Li, K. Chaotic motion behaviors of liquid crystal elastomer pendulum under periodic illumination. Results Phys. 2024, 56, 107332. [Google Scholar] [CrossRef]
- Liu, J.; Shi, F.; Song, W.; Dai, Y.; Li, K. Modeling of self-oscillating flexible circuits based on liquid crystal elastomers. Int. J. Mech. Sci. 2024, 270, 109099. [Google Scholar] [CrossRef]
- Wu, H.; Zhao, C.; Dai, Y.; Li, K. Light-fueled self-fluttering aircraft with a liquid crystal elastomer-based engine. Commun. Nonlinear Sci. Numer. Simul. 2024, 133, 107942. [Google Scholar] [CrossRef]
- Yu, Y.; Hu, H.; Dai, Y.; Li, K. Modeling the light-powered self-rotation of a liquid crystal elastomer fiber-based engine. Phys. Rev. E 2024, 109, 034701. [Google Scholar] [CrossRef]
- Wu, H.; Zhao, C.; Dai, Y.; Li, K. Modeling of a light-fueled self-paddling boat with a liquid crystal elastomer-based motor. Phys. Rev. E 2024, 109, 044705. [Google Scholar] [CrossRef]
- Qiu, Y.; Chen, J.; Dai, Y.; Zhou, L.; Yu, Y.; Li, K. Mathematical Modeling of the Displacement of a Light-Fuel Self-Moving Automobile with an On-Board Liquid Crystal Elastomer Propulsion Device. Mathematics 2024, 12, 1322. [Google Scholar] [CrossRef]
- Cunha, M.; Peeketi, A.R.; Ramgopal, A.; Annabattula, R.K.; Schenning, A. Light−driven continual oscillatory rocking of a polymer film. Chem. Open 2020, 9, 1149–1152. [Google Scholar]
- Cheng, Y.; Lu, H.; Lee, X.; Zeng, H.; Priimagi, A. Kirigami−based light−induced shape−morphing and locomotion. Adv. Mater. 2019, 32, 1906233. [Google Scholar] [CrossRef]
- Gelebart, A.H.; Mulder, D.J.; Varga, M.; Konya, A.; Vantomme, G.; Meijer, E.W.; Selinger, R.S.; Broer, D.J. Making waves in a photoactive polymer film. Nature 2017, 546, 632–636. [Google Scholar] [CrossRef] [PubMed]
- Warner, M.; Terentjev, E.M. Liquid Crystal Elastomers; Oxford University Press: London, UK, 2007. [Google Scholar]
- Corbett, D.; Warner, M. Linear and nonlinear photoinduced deformations of can−tilevers. Phys. Rev. Lett. 2007, 99, 174302. [Google Scholar] [CrossRef] [PubMed]
- Qiu, Y.; Wu, H.; Dai, Y.; Li, K. Behavior prediction and inverse design for self-rotating skipping ropes based on random forest and neural network. Mathematics 2024, 12, 1019. [Google Scholar] [CrossRef]
- Wu, H.; Zhang, B.; Li, K. Synchronous behaviors of three coupled liquid crystal elastomer-based spring oscillators under linear temperature fields. Phys. Rev. E 2024, 109, 024701. [Google Scholar] [CrossRef]
- Wu, H.; Lou, J.; Dai, Y.; Zhang, B.; Li, K. Bifurcation analysis in liquid crystal elastomer spring self-oscillators under linear light fields. Chaos Solitons Fractals 2024, 181, 114587. [Google Scholar] [CrossRef]
- Chen, B.; Liu, C.; Xu, Z.; Wang, Z.; Xiao, R. Modeling the thermo-responsive behaviors of polydomain and monodomain nematic liquid crystal elastomers. Mech. Mater. 2024, 188, 104838. [Google Scholar] [CrossRef]
- Bisoyi, H.K.; Urbas, A.M.; Li, Q. Soft materials driven by photo thermal effect and their applications. Adv. Opt. Mater. 2018, 6, 1800458. [Google Scholar] [CrossRef]
- Yu, Y.; Li, L.; Liu, E.; Han, X.; Wang, J.; Xie, Y.; Lu, C. Light−driven core−shell fiber actuator based on carbon nanotubes/liquid crystal elastomer for artificial muscle and phototropic locomotion. Carbon 2022, 187, 97–107. [Google Scholar] [CrossRef]
- Sun, J.; Wang, Y.; Liao, W.; Yang, Z. Ultrafast, High-Contractile Electrothermal-Driven Liquid Crystal Elastomer Fibers towards Artificial Muscles. Small 2021, 17, 2103700. [Google Scholar] [CrossRef] [PubMed]
- Lu, D.; Wang, L.; Chen, B.; Xu, Z.; Wang, Z.; Xiao, R. Shape memory behaviors of 3D printed liquid crystal elastomers. Soft Sci. 2023, 3, 4. [Google Scholar]
- Wang, L.; Wei, Z.; Xu, Z.; Yu, Q.; Wu, Z.L.; Wang, Z.; Qian, J.; Xiao, R. Shape Morphing of 3D Printed Liquid Crystal Elastomer Structures with Precuts. ACS Appl. Polym. Mater. 2023, 5, 7477–7484. [Google Scholar] [CrossRef]
- Wang, Y.; Yin, R.; Jin, L.; Liu, M.; Gao, Y.; Raney, J.; Yang, S. 3D-Printed Photoresponsive Liquid Crystal Elastomer Composites for Free-Form Actuation. Adv. Funct. Mater. 2023, 33, 2210614. [Google Scholar] [CrossRef]
- He, Q.; Wang, Z.; Wang, Y.; Minori, A.; Tolley, M.T.; Cai, S. Electrically controlled liquid crystal elastomer–based soft tubular actuator with multimodal actuation. Sci. Adv. 2019, 5, eaax5746. [Google Scholar] [CrossRef] [PubMed]
- Liao, B.; Zang, H.; Chen, M.; Wang, Y.; Lang, X.; Zhu, N.; Yang, Z.; Yi, Y. Soft rod−climbing robot inspired by winding locomotion of snake. Soft Robot. 2020, 7, 500–511. [Google Scholar] [CrossRef] [PubMed]
- Haberl, J.M.; Sanchez-Ferrer, A.; Mihut, A.M.; Dietsch, H.; Hirt, A.M.; Mezzenga, R. Liquid−crystalline elastomer−nanoparticle hybrids with reversible switch of magnetic memory. Adv. Mater. 2013, 25, 1787–1791. [Google Scholar] [CrossRef]
- Li, M.H.; Keller, P.; Li, B.; Wang, X.; Brunet, M. Light−driven side−on nematic elastomer actuators. Adv. Mater. 2003, 15, 569–572. [Google Scholar] [CrossRef]
- Qian, X.; Chen, Q.; Yang, Y.; Xu, Y.; Li, Z.; Wang, Z.; Wu, Y.; Wei, Y.; Ji, Y. Untethered recyclable tubular actuators with versatile locomotion for soft continuum robots. Adv. Mater. 2018, 30, 1801103. [Google Scholar] [CrossRef]
- Wei, Z.; Tianle, S.; Yuntong, D.; Kai, L.; Zhao, J. Light-powered self-propelled trolley with a liquid crystal elastomer pendulum motor. Int. J. Solids Struct. 2023, 285, 112500. [Google Scholar]
- Strogatz, S.H. Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry, and Engineering; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Pacejka, H. Tire and Vehicle Dynamics; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Atanackovic, T.M.; Guran, A. Hooke’s law. In Theory of Elasticity for Scientists and Engineers; Springer: Berlin/Heidelberg, Germany, 2000; pp. 85–111. [Google Scholar]
- Camacho-Lopez, M.; Finkelmann, H.; Palffy-Muhoray, P.; Shelley, M. Fast liquid-crystal elastomer swims into the dark. Nat. Mater. 2004, 3, 307. [Google Scholar] [CrossRef] [PubMed]
- Hogan, P.M.; Tajbakhsh, A.R.; Terentjev, E.M. UV manipulation of order and macroscopic shape in nematic elastomers. Phys. Rev. 2002, 65, 041720. [Google Scholar] [CrossRef] [PubMed]
- Baumann, A.; Sánchez-Ferrer, A.; Jacomine, L.; Martinoty, P.; Houerou, V.; Ziebert, F.; Kulić, I. Motorizing fibers with geometric zero-energy modes. Nat. Mater. 2018, 17, 523. [Google Scholar] [CrossRef] [PubMed]







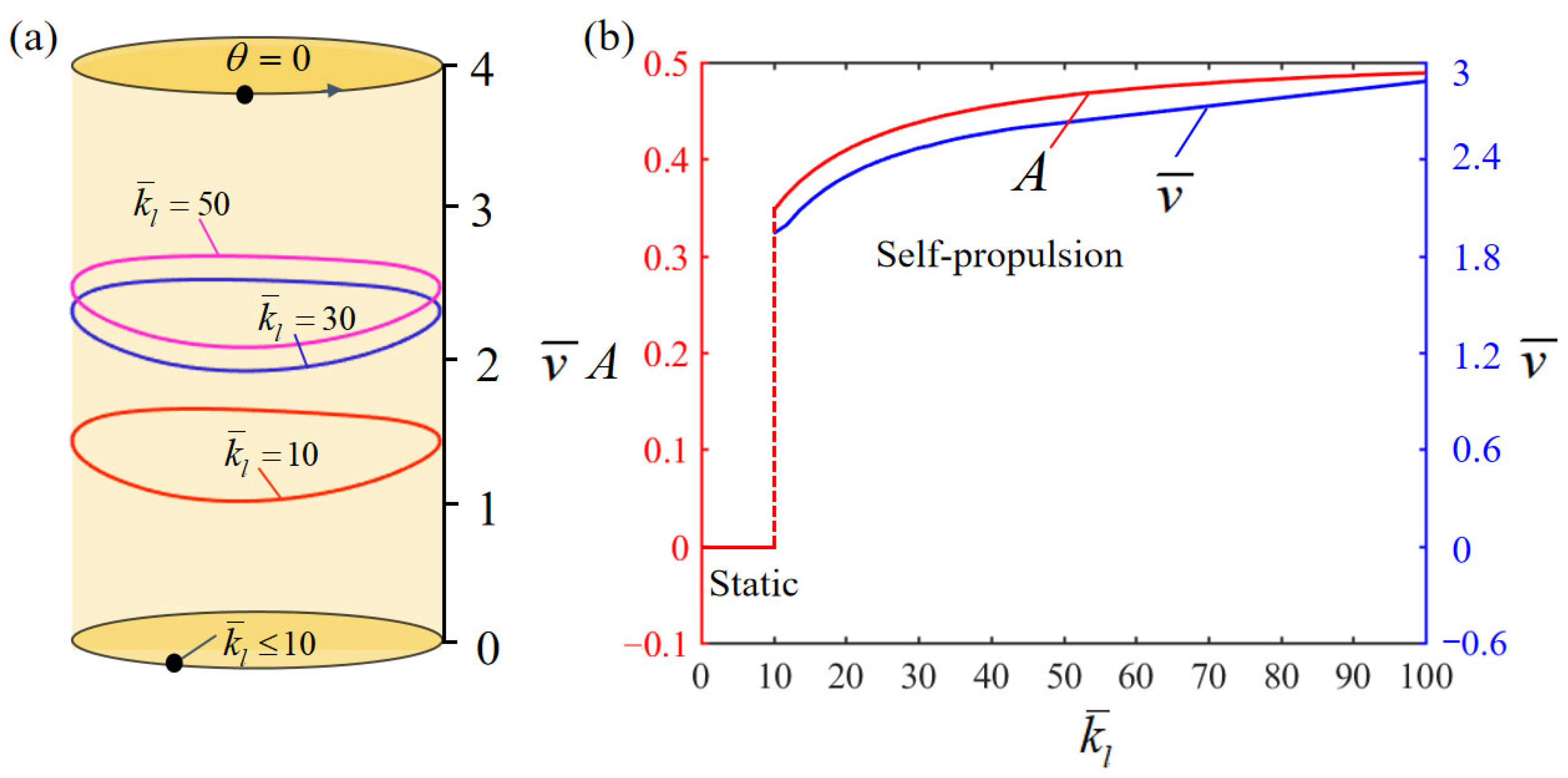

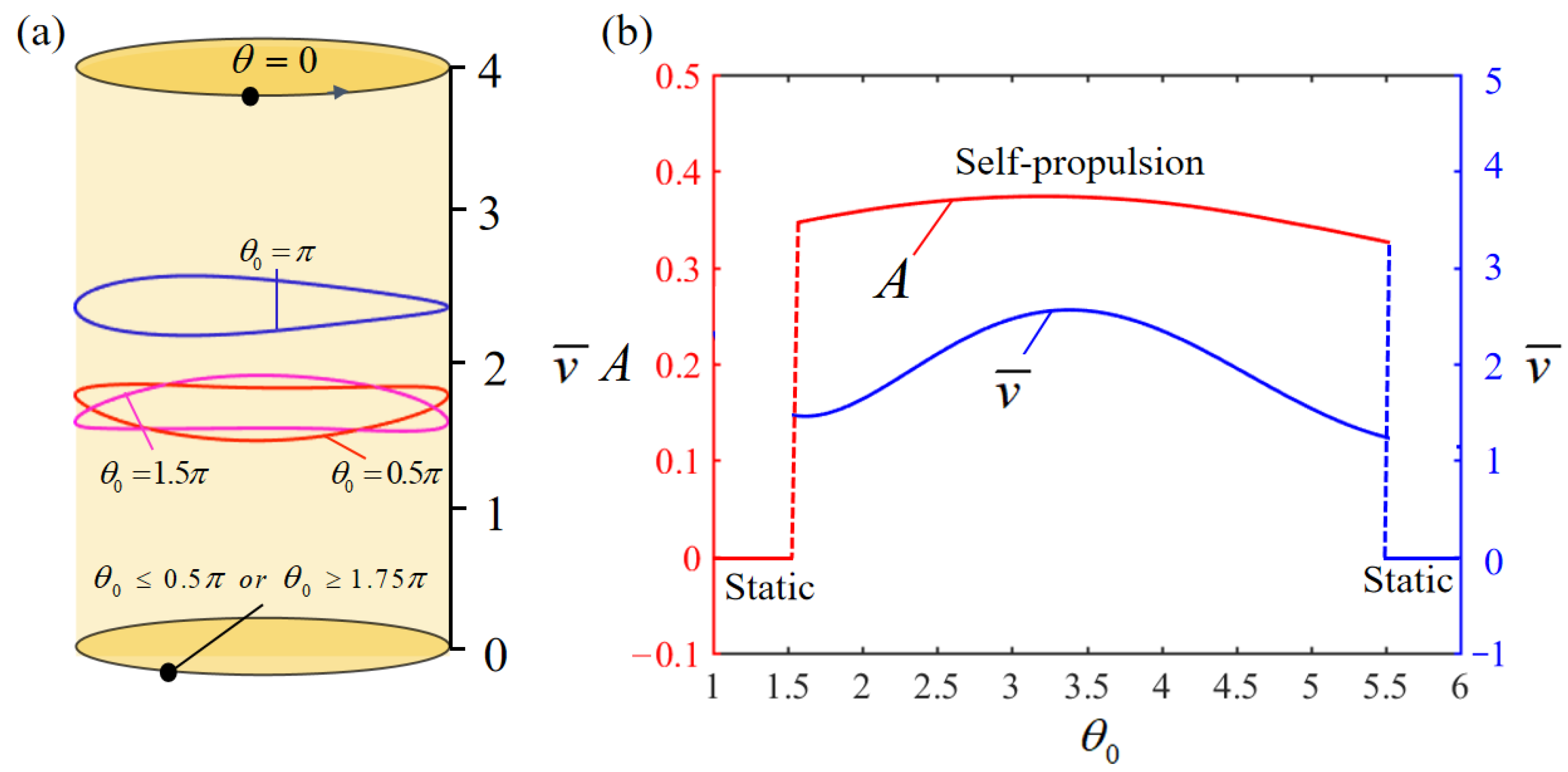
| Parameter | Definition | Value | Unit |
|---|---|---|---|
| Thermal contraction coefficient of LCE rope [20,63] | 0–0.35 | / | |
| Gravitational acceleration | 10 | ||
| Damping factor | 0.001~0.01 | ||
| Illumination zone angle | / | ||
| Initial angular velocity | 0.4~2 | ||
| Elastic stiffness of spring | 0.005~50 | ||
| Elastic stiffness of LCE rope | 0.005~50 | ||
| Distance from mass ball to turntable center | 0.04~0.08 | ||
| Initial length of spring | 0.01~0.02 | ||
| Initial length of LCE rope | 0.02 | ||
| Thermal characteristic time [64] | 0.001~0.1 | ||
| J | Moment of inertia of wheels | 0~1 | mgmm2 |
| Rolling resistance coefficient | 0~0.004 | m | |
| R | Wheel radius | 0.04~0.06 | m |
| Heat flux [64] | 0~0.02 | ||
| K | Heat transfer coefficient [65] | 1 |
| Parameter | |||||
| Value | 1~1.5 | 1~10 | 0.01~0.2 | 0.1~0.35 | 10~100 |
| Parameter | |||||
| Value | 1~50 | 2~3 | 0~0.03 |
| Dimensionless Parameter | Mass Ball Amplitude A | |
|---|---|---|
| Increases with increasing | Decreases and then stabilizes with increasing | |
| Increases with increasing | Increases with increasing | |
| Increases with increasing | Increases with increasing | |
| Decreases with increasing | Increases with increasing | |
| Decreases with increasing | Remains constant with increasing | |
| Decreases with increasing | Remains constant with increasing | |
| Increases slightly with increasing | Increases slightly with increasing | |
| Decreases with increasing | Decreases with increasing | |
| Increases and then decreases with increasing | Increases and then decreases with increasing |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, Z.; Dai, Y.; Liu, J.; Li, K. Light-Fueled Self-Propulsion of Liquid Crystal Elastomer-Engined Automobiles in Zero-Energy Modes. Mathematics 2024, 12, 2109. https://doi.org/10.3390/math12132109
Yuan Z, Dai Y, Liu J, Li K. Light-Fueled Self-Propulsion of Liquid Crystal Elastomer-Engined Automobiles in Zero-Energy Modes. Mathematics. 2024; 12(13):2109. https://doi.org/10.3390/math12132109
Chicago/Turabian StyleYuan, Zongsong, Yuntong Dai, Junxiu Liu, and Kai Li. 2024. "Light-Fueled Self-Propulsion of Liquid Crystal Elastomer-Engined Automobiles in Zero-Energy Modes" Mathematics 12, no. 13: 2109. https://doi.org/10.3390/math12132109










