Arm Angle Tracking Control with Pole Balancing Using Equivalent Input Disturbance Rejection for a Rotational Inverted Pendulum
Abstract
:1. Introduction
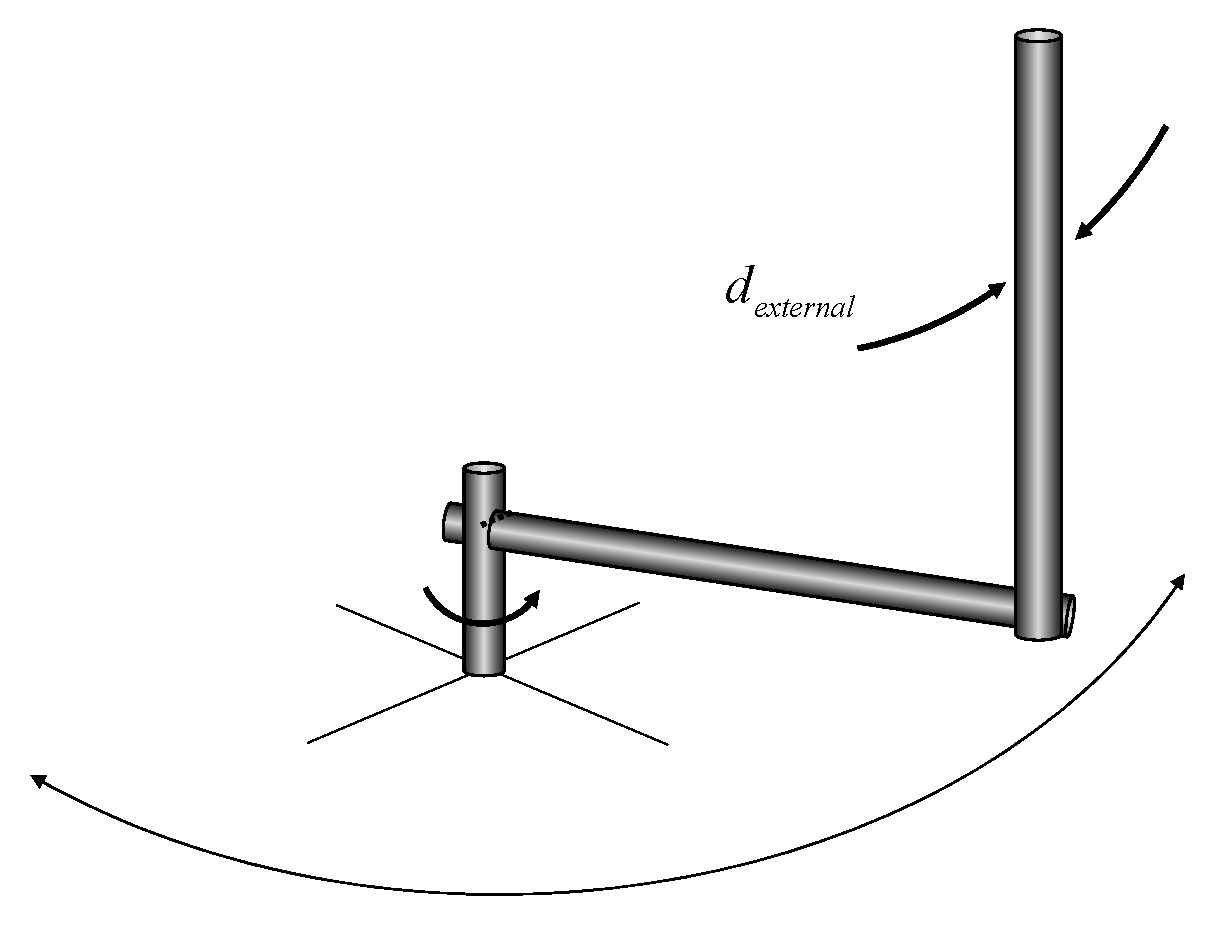
2. System Modeling
3. EID Estimator Design
4. LQR Based Tracking Controller Design
5. Experimental Results
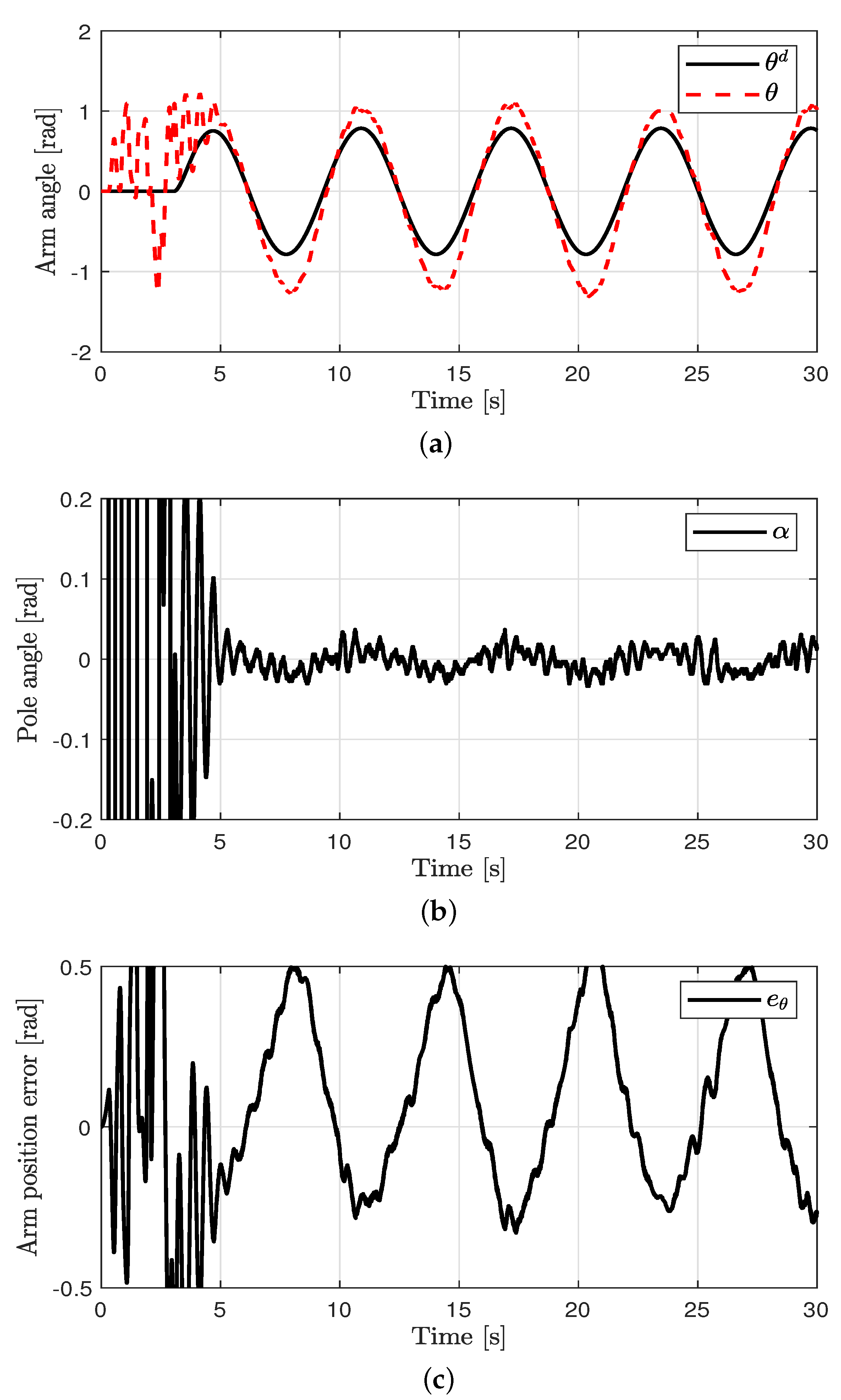
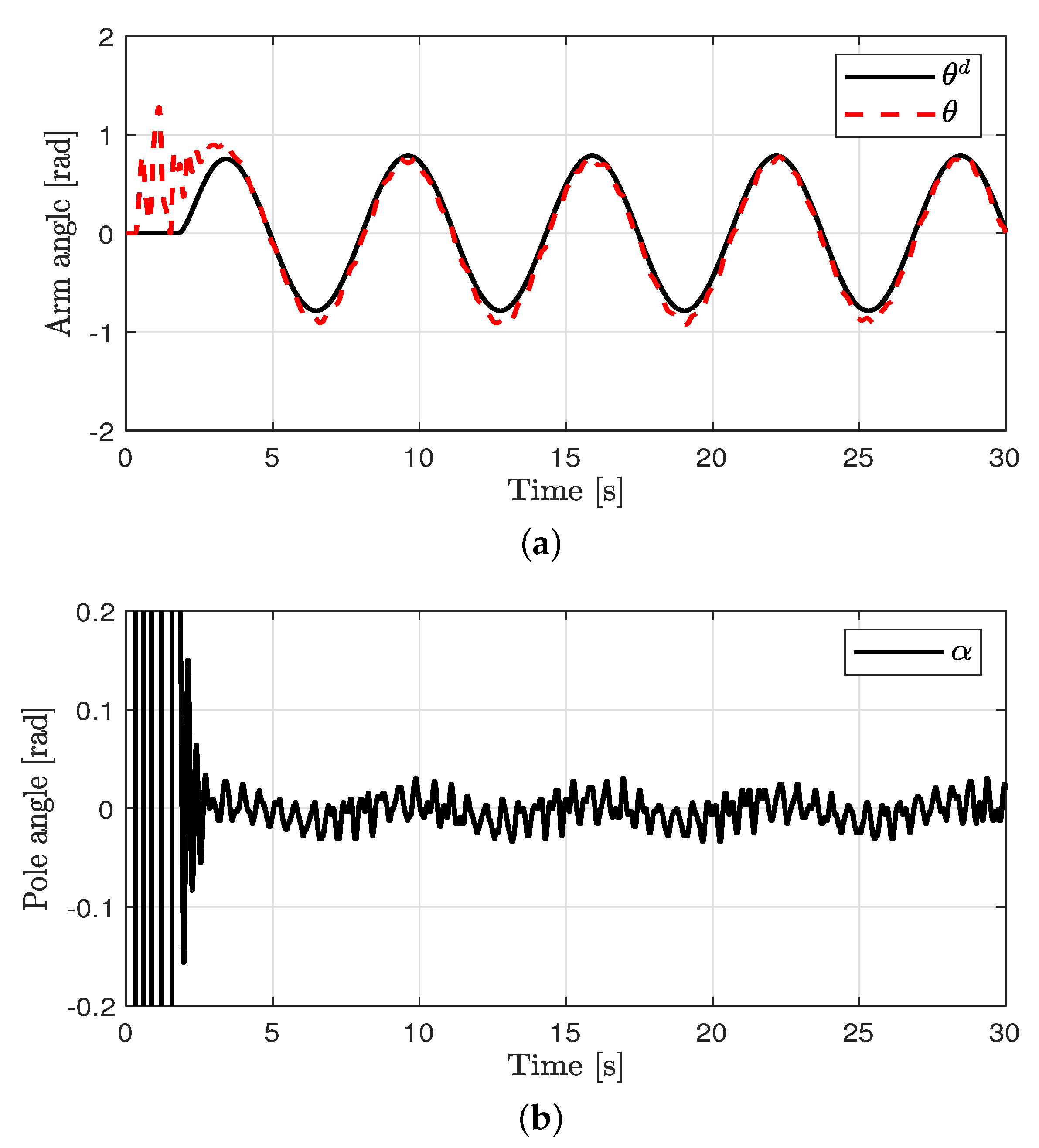
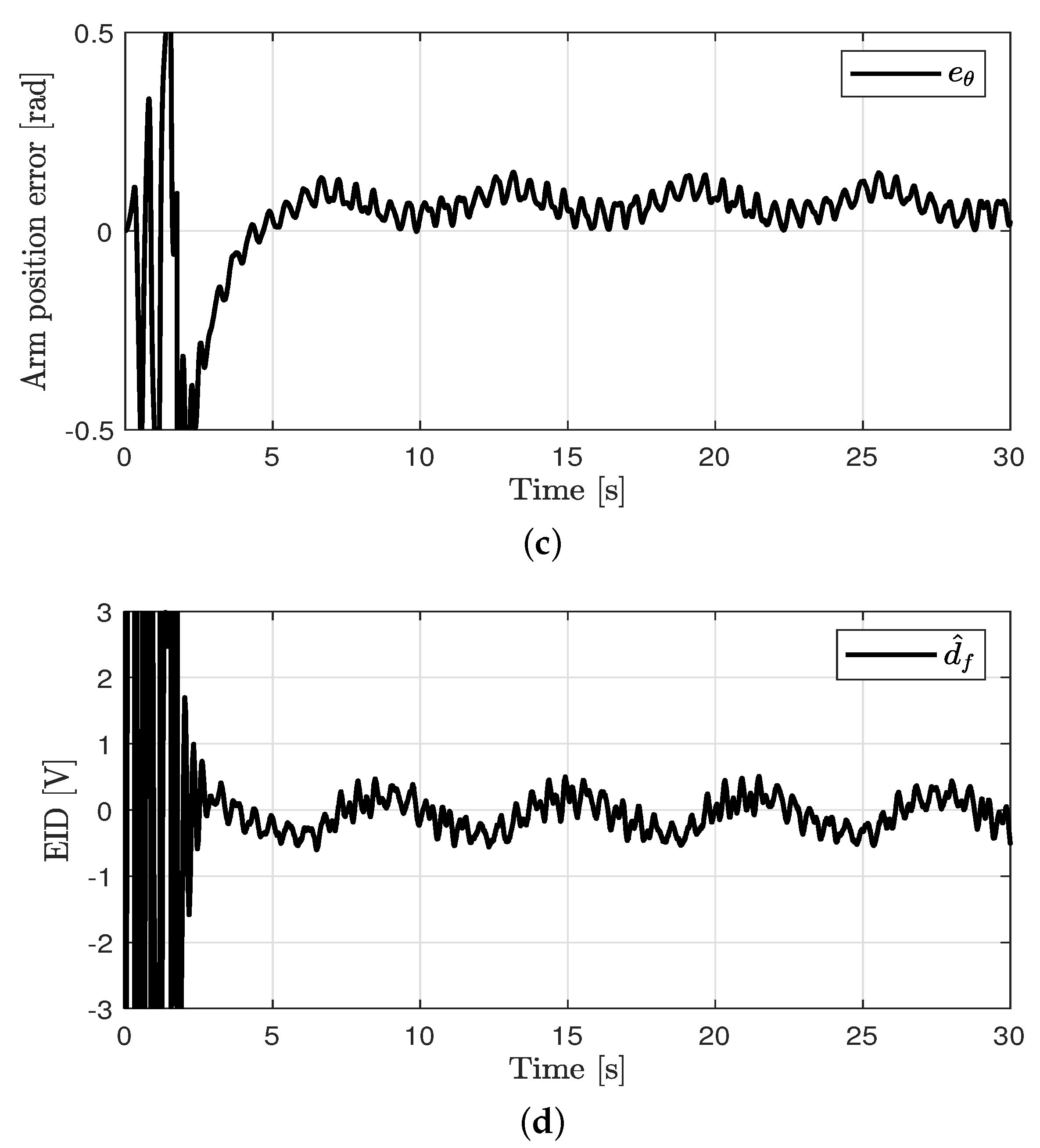
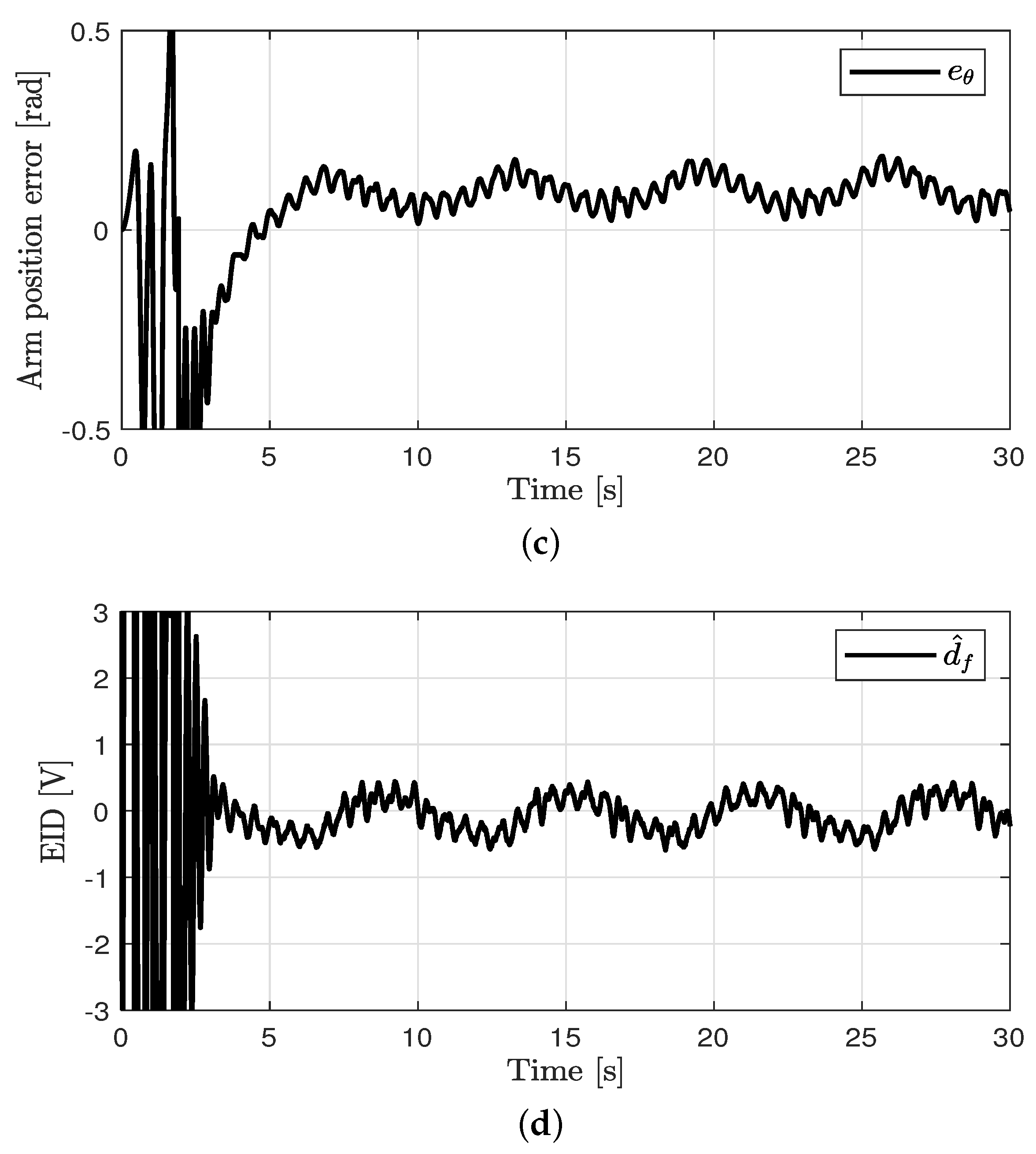
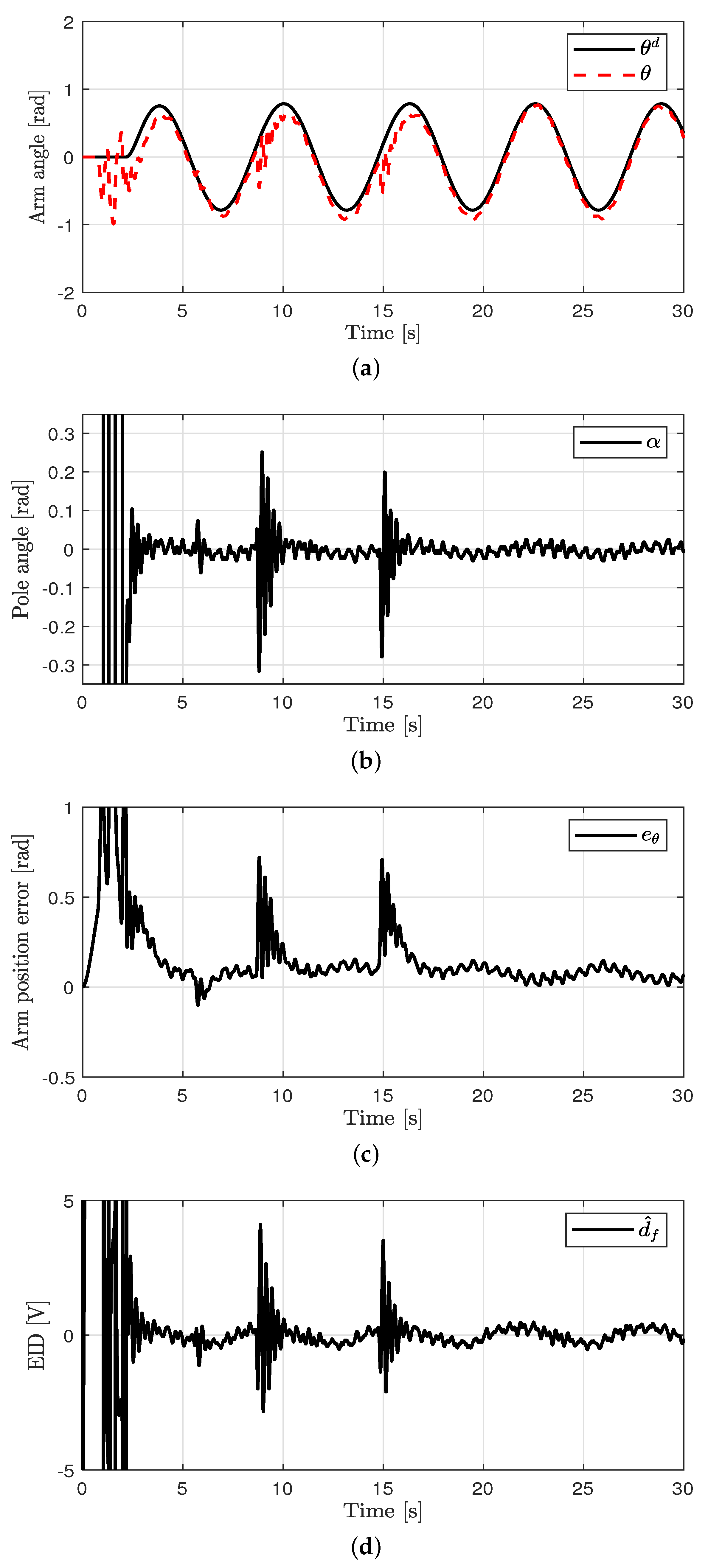
5.1. Performances of Arm Angle Tracking Control and Pole Balancing
- Case 1: Conventional proportional-derivative (PD) controller,
- Case 2: Proposed method without EID compensation,
- Case 3: Proposed method with EID compensation, .
- Case 3: Proposed method with EID compensation under the parameter uncertainties (at most, ±20%), .
5.2. Robustness against External Disturbance
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Spong, M.W. Underactuated mechanical systems. In Control Problems in Robotics and Automation; Springer: Berlin/Heidelberg, Germany, 1998; pp. 135–150. [Google Scholar]
- Awtar, S.; King, N.; Allen, T.; Bang, I.; Hagan, M.; Skidmore, D.; Craig, K. Inverted pendulum systems: Rotary and arm-driven-a mechatronic system design case study. Mechatronics 2002, 12, 357–370. [Google Scholar] [CrossRef]
- Furuta, K.; Yamakita, M.; Kobayashi, S. Swing up control of inverted pendulum. Proc. Int. Conf. Ind. Electron. Control Instrum. 1991, 206, 263–269. [Google Scholar]
- Mathew, N.; Rao, J.; Koteswara, K.; Sivakumaran, N. Swing up and stabilization control of a rotary inverted pendulum. IFAC Proc. Vol. 2013, 46, 654–659. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Zheng, X. Swing-up and stabilization control design for an underactuated rotary inverted pendulum system: Theory and experiments. IEEE Trans. Ind. Electron. 2018, 65, 7229–7238. [Google Scholar] [CrossRef]
- Chen, T.; Shan, J.; Wen, H. Distributed adaptive attitude control for networked underactuated flexible spacecraft. IEEE Trans. Aerosp. Electron. Syst. 2018, 55, 215–225. [Google Scholar] [CrossRef]
- Chevallereau, C.; Grizzle, J.W.; Shih, C.L. Asymptotically stable walking of a five-link underactuated 3-D bipedal robot. IEEE Trans. Robot. 2009, 25, 37–50. [Google Scholar] [CrossRef] [Green Version]
- Chignoli, M.; Wensing, P.M. Variational-based optimal control of underactuated balancing for dynamic quadrupeds. IEEE Access 2000, 8, 49785–49797. [Google Scholar] [CrossRef]
- Ashrafiuon, H.; Nersesov, S.; Clayton, G. Trajectory tracking control of planar underactuated vehicles. IEEE Trans. Automat. Control 2017, 62, 1959–1965. [Google Scholar] [CrossRef]
- Tu, F.; Ge, S.S.; Choo, Y.S.; Hang, C.C. Adaptive dynamic positioning control for accommodation vessels with multiple constraints. IET Control Theory Appl. 2017, 11, 329–340. [Google Scholar] [CrossRef]
- Huang, X.; Yan, Y. Saturated backstepping control of underactuated spacecraft hovering for formation flights. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 1988–2000. [Google Scholar] [CrossRef]
- Tao, Q.; Wang, J.; Xu, Z.; Lin, T.X.; Yuan, Y.; Zhang, F. Swing-Reducing Flight Control System for an Underactuated Indoor Miniature Autonomous Blimp. IEEE/ASME Trans. Mechatron. 2021. [Google Scholar] [CrossRef]
- Johnson, M.A.; Moradi, M.H. PID Control; Springer: London, UK, 2005. [Google Scholar]
- Hazem, Z.B.; Fotuhi, M.J.; Bingül, Z. Development of a fuzzy-LQR and fuzzy-LQG stability control for a double link rotary inverted pendulum. J. Franklin Inst. 2020, 357, 10529–10556. [Google Scholar] [CrossRef]
- Saleem, O.; Mahmood-Ul-Hasan, K. Indirect adaptive state-feedback control of rotary inverted pendulum using self-mutating hyperbolic-functions for online cost variation. IEEE Access 2020, 8, 91236–91247. [Google Scholar] [CrossRef]
- Eini, R.; Abdelwahed, S. Indirect Adaptive fuzzy Controller Design for a Rotational Inverted Pendulum. In Proceedings of the 2018 Annual American Control Conference, Milwaukee, WI, USA, 27–29 June 2018; pp. 1677–1682. [Google Scholar]
- Park, M.-S.; Chwa, D. Swing-up and stabilization control of inverted-pendulum systems via coupled sliding-mode control method. IEEE Trans. Ind. Electron. 2009, 56, 3541–3555. [Google Scholar] [CrossRef]
- Nguyen, N.P.; Oh, H.; Kim, Y.; Moon, J.; Yang, J.; Chen, W.H. Fuzzy-based super-twisting sliding mode stabilization control for under-actuated rotary inverted pendulum systems. IEEE Access 2020, 8, 185079–185092. [Google Scholar] [CrossRef]
- Kang, L.; Hongbo, G.; Haibo, J.; Zhengyuan, H. Adaptive sliding mode based disturbance attenuation tracking control for wheeled mobile robots. Int. J. Control Automat. Syst. 2020, 18, 1288–1298. [Google Scholar]
- Kang, L.; Haibo, J.; Yinuo, Z. Extended state observer based adaptive sliding mode tracking control of wheeled mobile robot with input saturation and uncertainties. Proc. Ins. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 5460–5476. [Google Scholar]
- Rudra, S.; Ranjit, K.B. Robust adaptive backstepping control of inverted pendulum on cart system. Int. J. Control Automat. 2012, 5, 13–26. [Google Scholar]
- Rahmani, R.; Mobayen, S.; Fekih, A.; Ro, J.-S. Robust passivity cascade technique-based control using RBFN approximators for the stabilization of a cart inverted pendulum. Mathematics 2021, 9, 1229. [Google Scholar] [CrossRef]
- Won, D.; Kim, W.; Shin, D.; Chung, C.C. High gain disturbance observer based backstepping control with output tracking error constraint for electro-hydraulic systems. IEEE Trans. Control Syst. Technol. 2015, 23, 787–795. [Google Scholar] [CrossRef]
- Kim, W.; Suh, S. Optimal disturbance observer design for high tracking performance in motion control systems. Mathematics 2020, 8, 1633. [Google Scholar] [CrossRef]
- Jin-Hua, S.; Mingxing, F.; Yasuhiro, O.; Hiroshi, H.; Min, W. Improving disturbance-rejection performance based on an equivalent-input-disturbance approach. IEEE Trans. Ind. Electron. 2008, 55, 380–389. [Google Scholar] [CrossRef]
- Wang, J.J. Simulation studies of inverted pendulum based on PID controllers. Simul. Model. Pract. Theory 2011, 19, 440–449. [Google Scholar] [CrossRef]
- Quanser User Mannual. Available online: https://quanserinc.box.com/shared/static/jewhkc82kbgng0la81mx74dilriv4dw0.zip (accessed on 28 August 2021).
- Åström, K.J.; Furuta, K. Swinging up a pendulum by energy control. Automatica 2000, 36, 287–295. [Google Scholar] [CrossRef] [Green Version]
- Kang, L.; Rujing, W.; Xiaodong, W.; Xingxian, W. Anti-saturation adaptive finite-time neural network based fault-tolerant tracking control for a quadrotor UAV with external disturbances. Aerosp. Sci. Technol. 2021, 115, 106790. [Google Scholar]
- Kang, L.; Rujing, W. Antisaturation command filtered backstepping control based disturbance rejection for a quadarotor UAV. IEEE Trans. Circuits Syst. II Express Briefs 2021. [Google Scholar] [CrossRef]
- Kang, L.; Xingxian, W.; Rujing, W.; Guowei, S.; Xiaodong, W. Antisaturation finite-time attitude tracking control based observer for a quadrotor. IEEE Trans. Circuits Syst. II Express Briefs 2020, 68, 2047–2051. [Google Scholar]





| Symbol | Description | Value |
|---|---|---|
| DC motor torque constant | 0.042 N·m/A | |
| R | Terminal resistance | 8.4 |
| Rotor inertia | 4.0 × 10 kg·m | |
| Rotary arm mass | 0.095 kg | |
| Rotary arm length | 0.085 m | |
| Pendulum mass | 0.024 kg | |
| Pendulum length | 0.129 m | |
| Rotary arm inertia | 5.72 × 10 kg·m | |
| Pendulum inertia | 5.72 × 10 kg·m | |
| g | Gravitational acceleration | 9.81 m/s |
| Case | ASE |
|---|---|
| Case 1 | 0.0817 |
| Case 2 | 0.0122 |
| Case 3 | 0.061 |
| Case 4 | 0.062 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, H.; Gil, J.; You, S.; Gui, Y.; Kim, W. Arm Angle Tracking Control with Pole Balancing Using Equivalent Input Disturbance Rejection for a Rotational Inverted Pendulum. Mathematics 2021, 9, 2745. https://doi.org/10.3390/math9212745
Lee H, Gil J, You S, Gui Y, Kim W. Arm Angle Tracking Control with Pole Balancing Using Equivalent Input Disturbance Rejection for a Rotational Inverted Pendulum. Mathematics. 2021; 9(21):2745. https://doi.org/10.3390/math9212745
Chicago/Turabian StyleLee, Hojin, Jeonghwan Gil, Sesun You, Yonghao Gui, and Wonhee Kim. 2021. "Arm Angle Tracking Control with Pole Balancing Using Equivalent Input Disturbance Rejection for a Rotational Inverted Pendulum" Mathematics 9, no. 21: 2745. https://doi.org/10.3390/math9212745
APA StyleLee, H., Gil, J., You, S., Gui, Y., & Kim, W. (2021). Arm Angle Tracking Control with Pole Balancing Using Equivalent Input Disturbance Rejection for a Rotational Inverted Pendulum. Mathematics, 9(21), 2745. https://doi.org/10.3390/math9212745








