An Algebraic Approach for Identification of Rotordynamic Parameters in Bearings with Linearized Force Coefficients
Abstract
:1. Introduction
2. Materials and Methods
2.1. Mathematical Model of the Rotor-Bearing System
2.2. Operation Velocity of the Rotor-Bearing System
- is the excitation frequency at the ramp beginning;
- is the change ratio with respect to time of the excitation frequency;
- t is the time.
2.3. Mathematical Model for Bearing Rotordynamic Parameters Identification
2.3.1. Algebraic Identifier with Constant Operation Velocity
2.3.2. Algebraic Identifier with Variable Operation Velocity
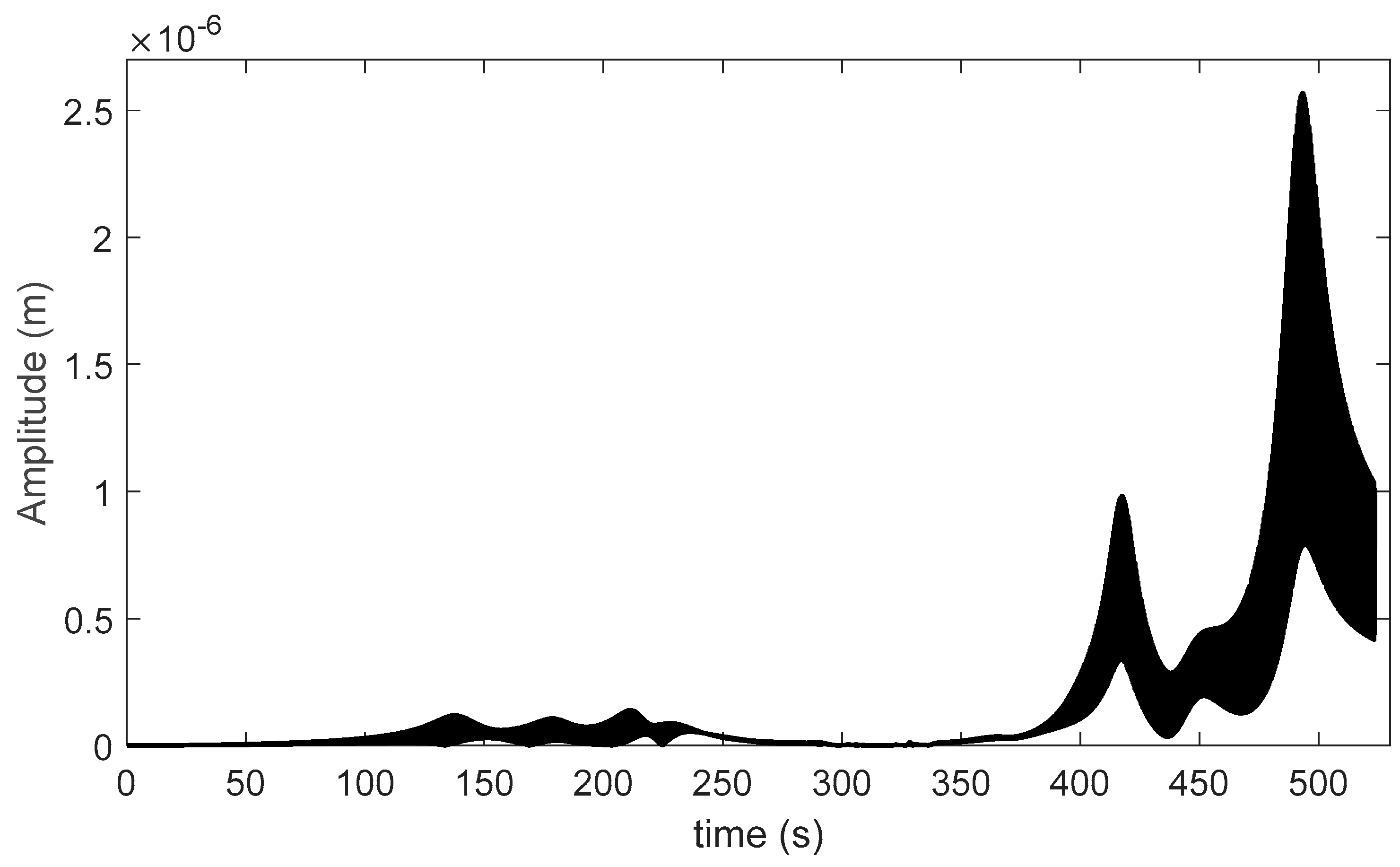
3. Results
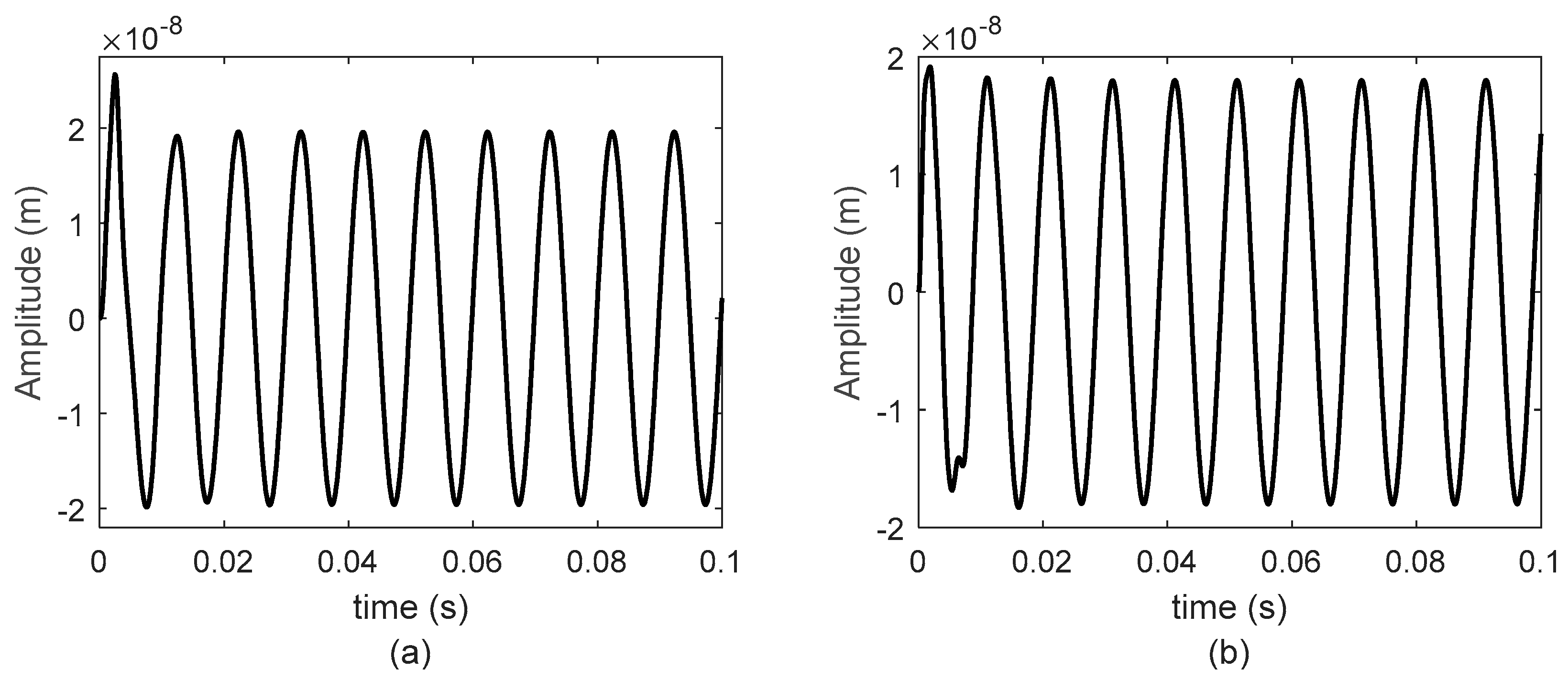
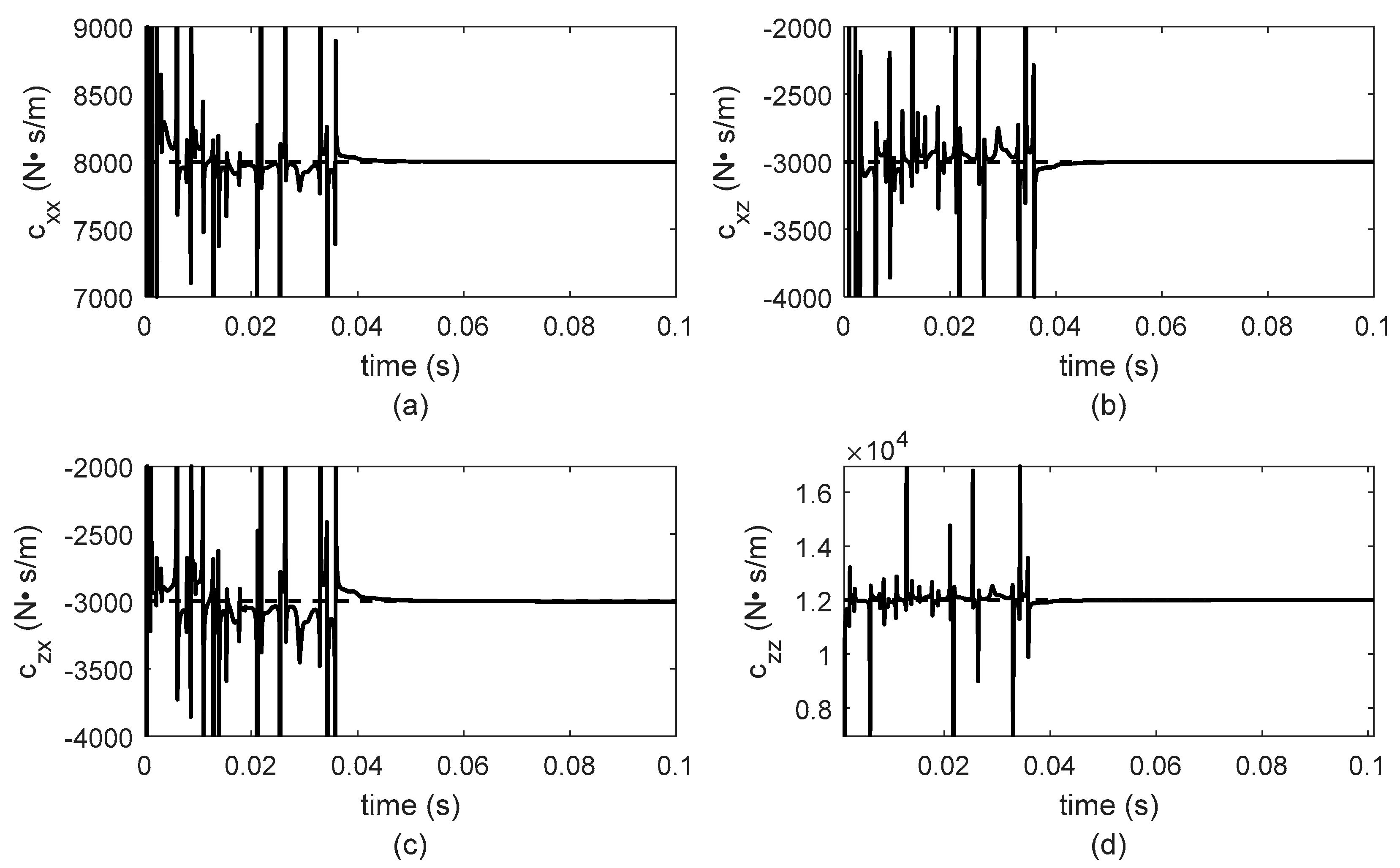
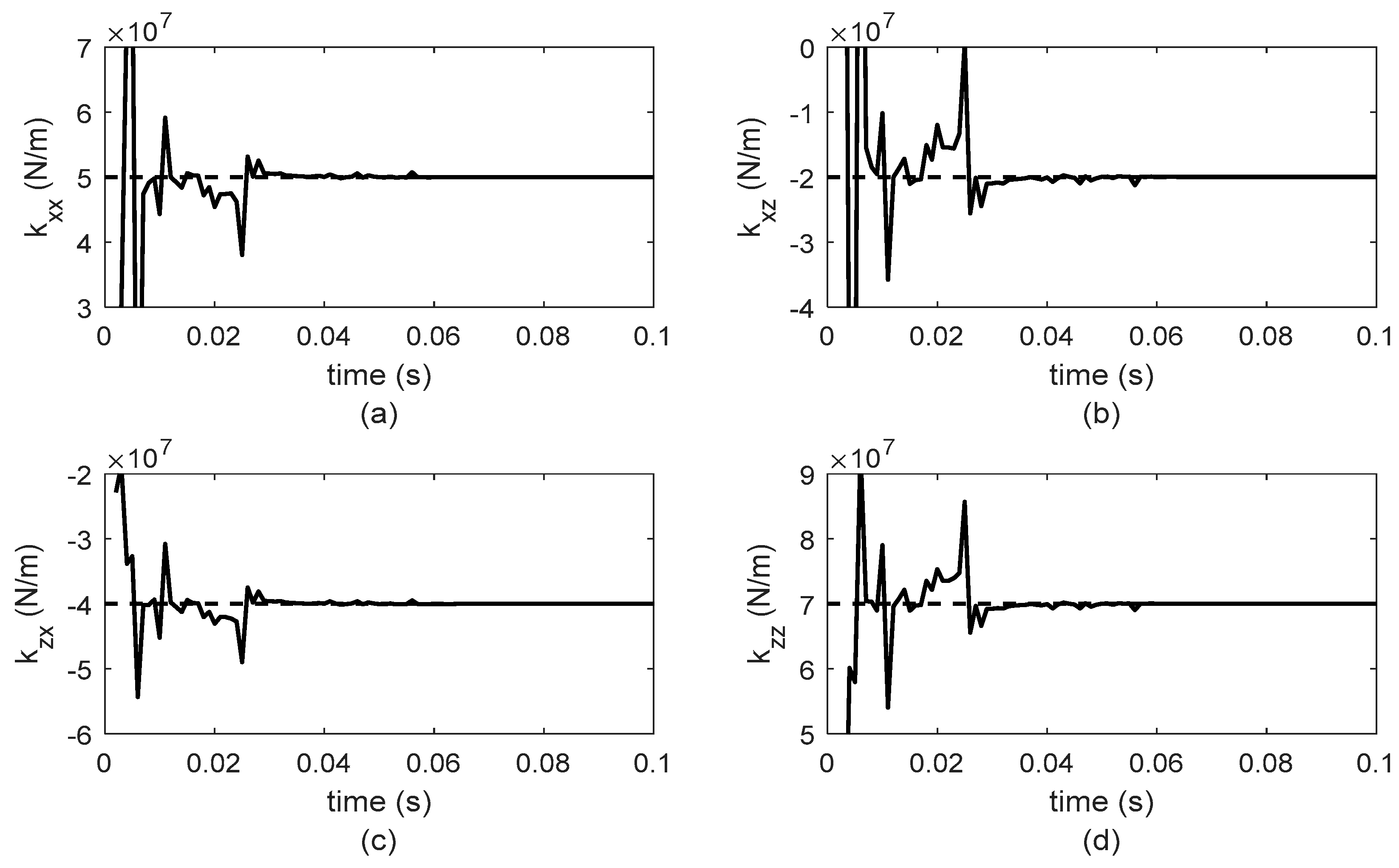
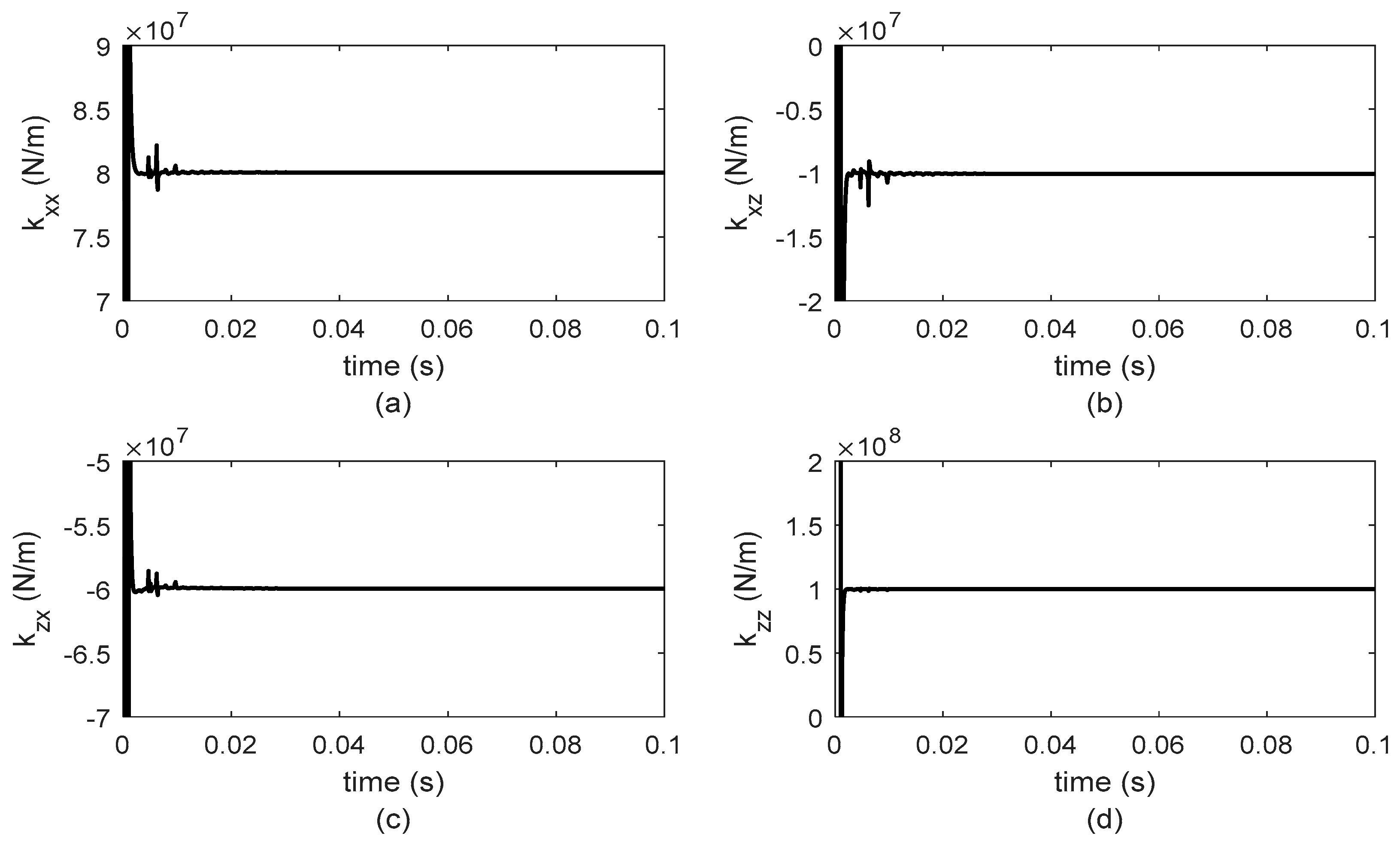
3.1. Algebraic Parameter Identification with Constant System Velocity
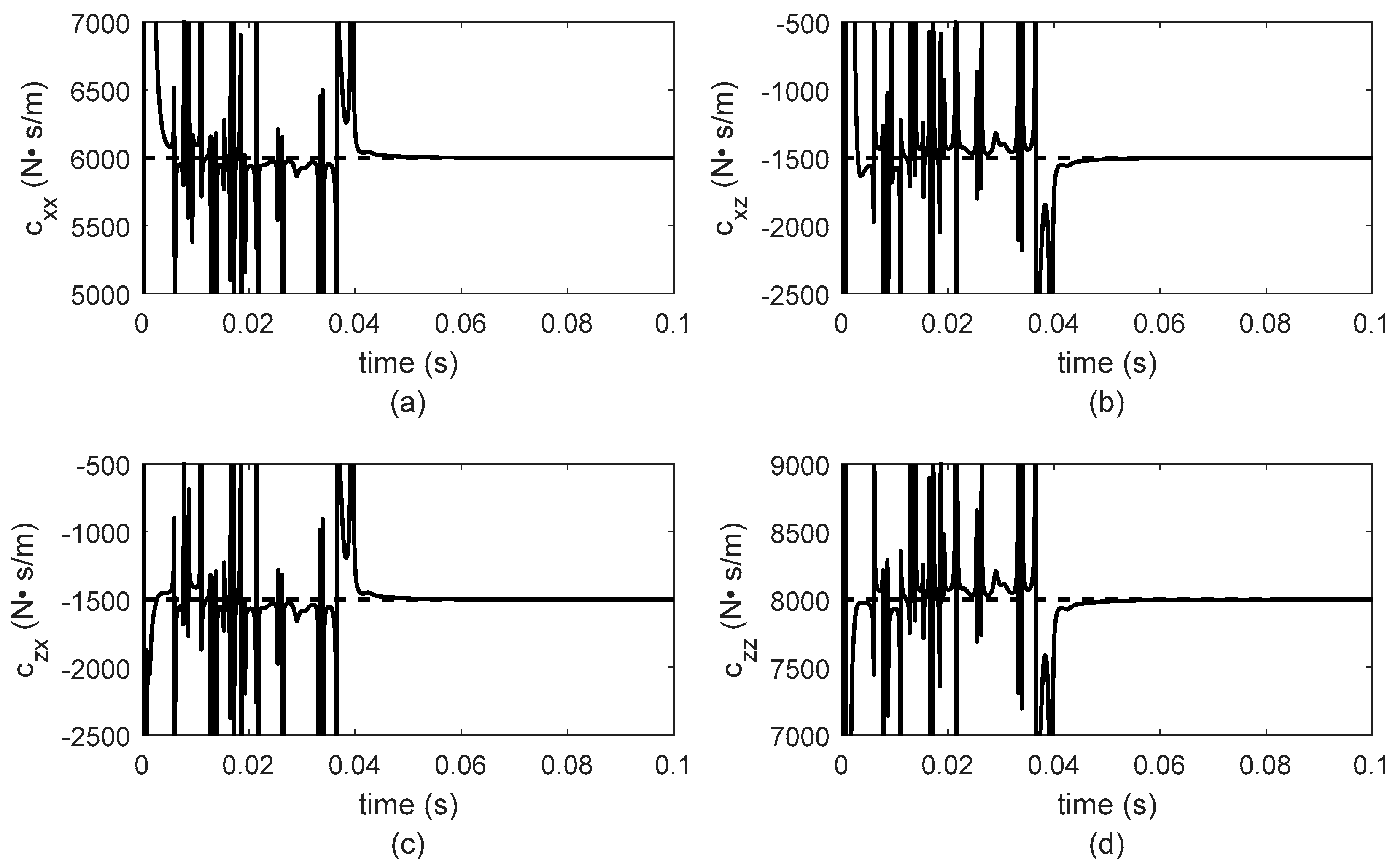
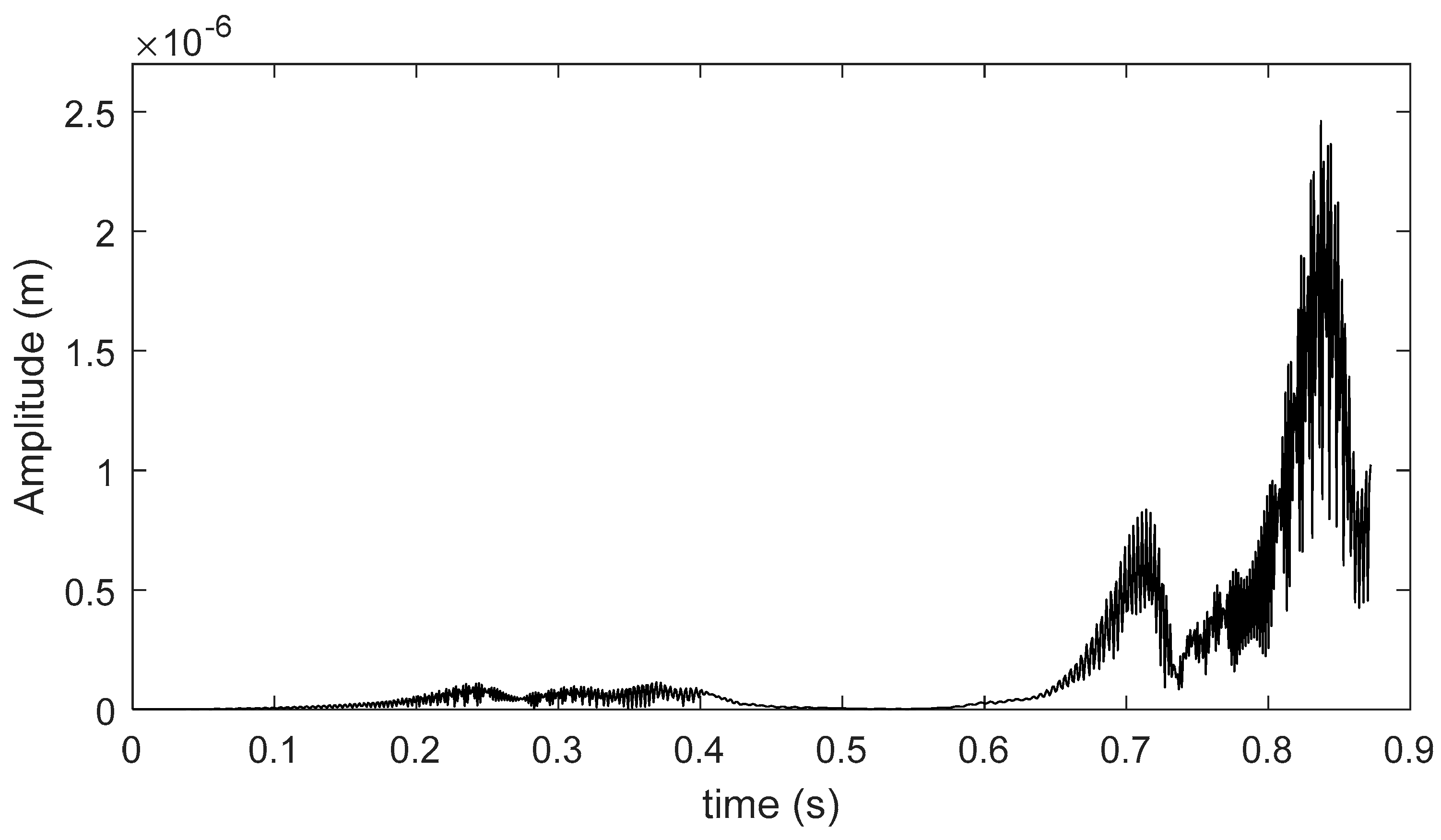
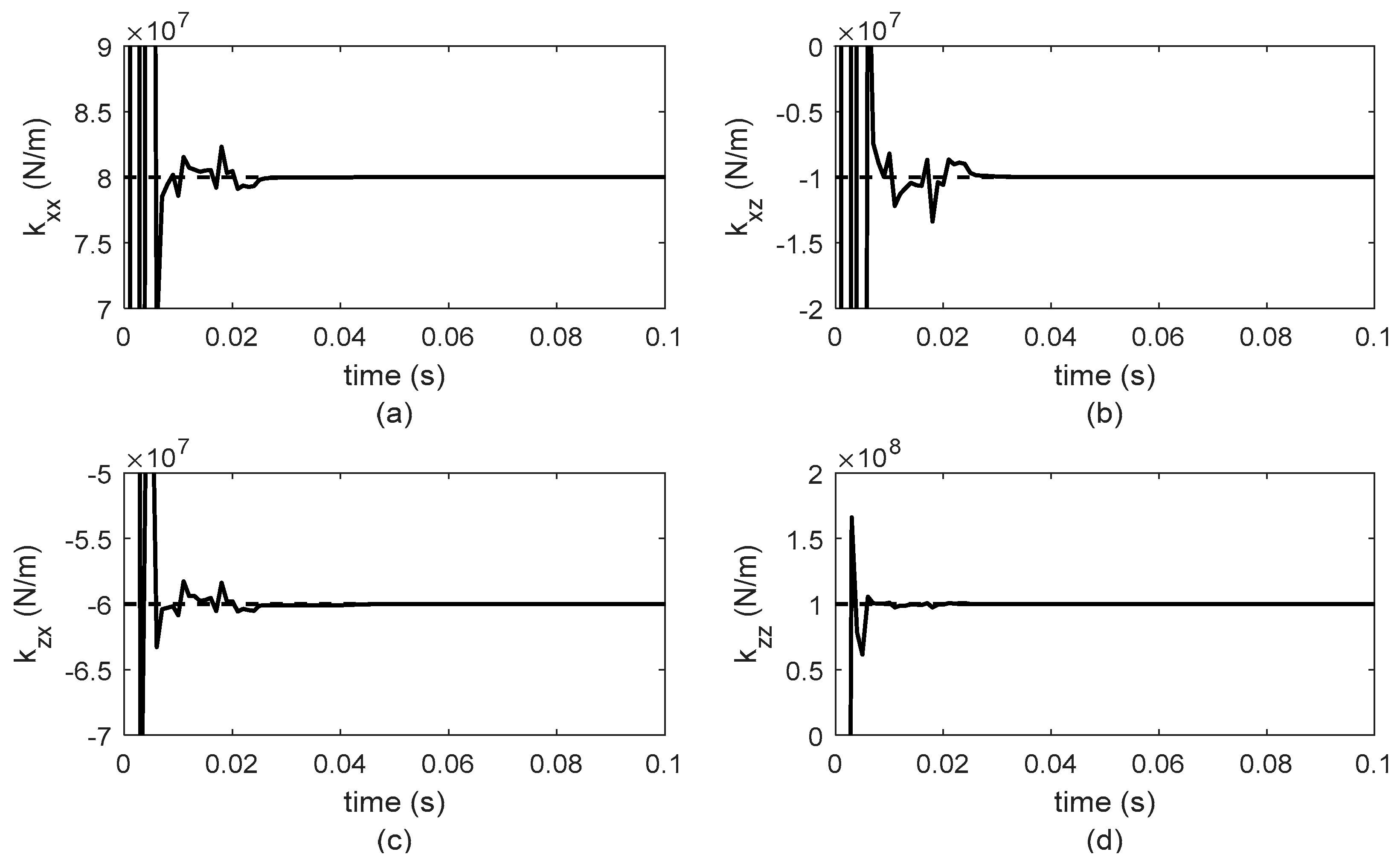
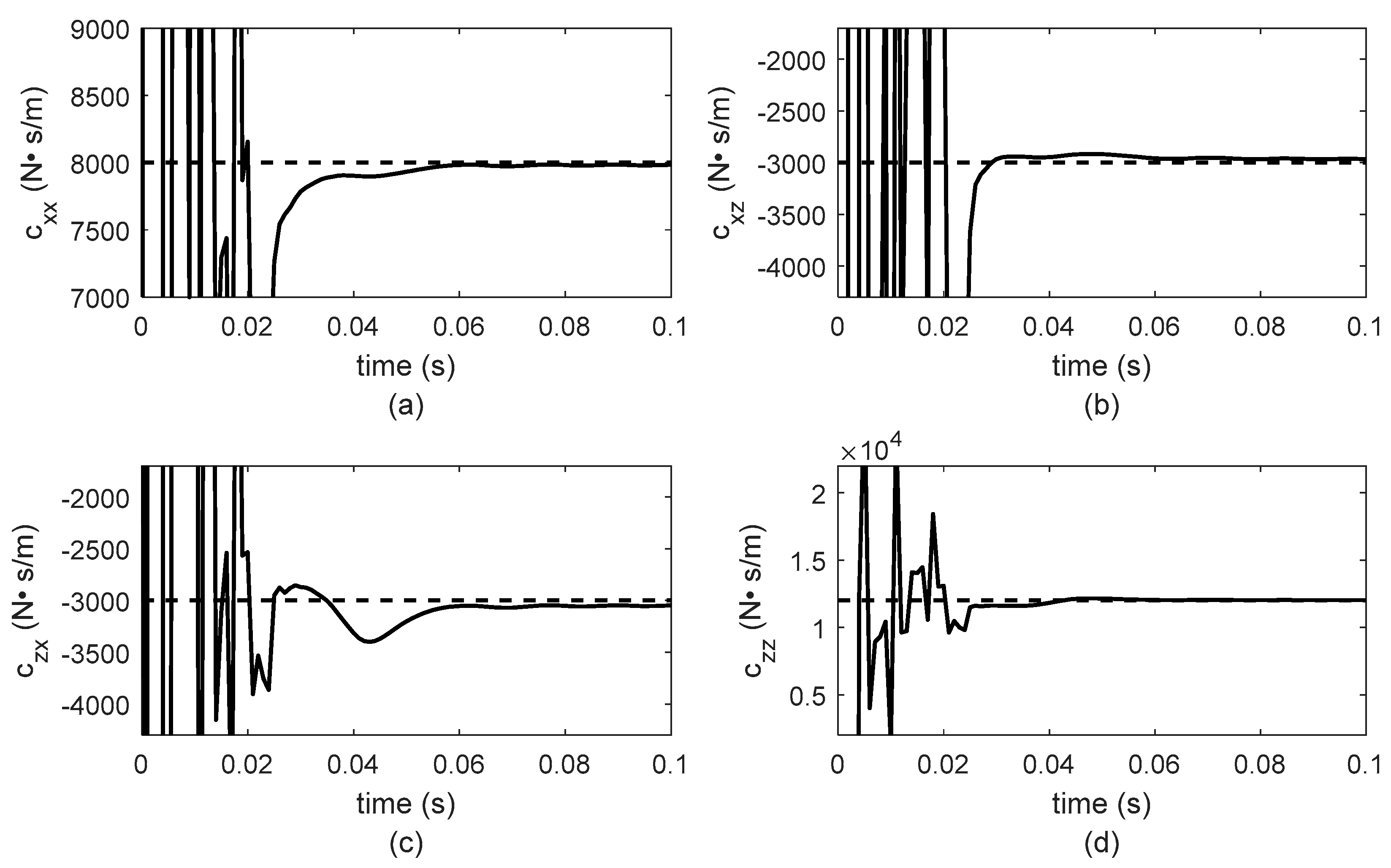
3.2. Algebraic Parameter Identification with Variable System Velocity
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Koutromanos, I. Fundamentals of Finite Element Analysis: Linear Finite Element Analysis, 1st ed.; John Wiley & Sons: New York, NY, USA, 2018. [Google Scholar]
- Mendoza-Larios, J.G.; Barredo, E.; Colín, J.; Blanco-Ortega, A.; Arias-Montiel, M.; Mayén, J. Computational platform for the analysis and simulation of rotor-bearing systems of multiple degrees of freedom. Rev. Int. Metod. Numer. 2020, 36, 1–10. [Google Scholar]
- Shen, Z.; Chouvion, B.; Thouverez, F.; Beley, A. Enhanced 3D solid finite element formulation for rotor dynamics simulation. Finite Elem. Anal. Des. 2021, 195, 103584. [Google Scholar] [CrossRef]
- Tiwari, R. Rotor System: Analysis and Identification, 1st ed.; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Matsushita, O.; Tanaka, M.; Kobayashi, M.; Keogh, P.; Kanki, H. Vibrations of Rotating Machinery. Volume 2. Advanced Rotordynamics: Applications of Analysis, Troubleshooting and Diagnosis, 1st ed.; Springer Japan KK: Tokyo, Japan, 2019. [Google Scholar]
- Breńkacz, L. Bearing Dynamic Coefficients in Rotordynamics. Computation Methods and Practical Applications, 1st ed.; Wiley-ASME: New York, NY, USA, 2021. [Google Scholar]
- Narendiranath Babu, T.; Manvel Raj, T.; Lakshmanan, T. A review on application of dynamic parameters of journal bearing for vibration and condition monitoring. J. Mech. 2015, 31, 391–416. [Google Scholar] [CrossRef]
- Tiwari, R.; Chougale, A. Identification of bearing dynamic parameters and unbalance state in a flexible rotor system fully levitated on active magnetic bearings. Mechatronics 2014, 24, 274–286. [Google Scholar] [CrossRef]
- Xu, Y.; Zhou, J.; Jin, C.; Guo, Q. Identification of the dynamic parameters of active magnetic bearings based on the transfer matrix model updating method. J. Mech. Sci. Technol. 2016, 30, 2971–2979. [Google Scholar] [CrossRef]
- Mao, W.; Han, X.; Liu, G.; Liu, J. Bearing dynamic parameters identification of a flexible rotor-bearing system based on transfer matrix method. Inverse Probl. Sci. Eng. 2016, 24, 372–392. [Google Scholar] [CrossRef]
- Yao, J.; Liu, L.; Yang, F.; Scarpa, F.; Gao, J. Identification and optimization of unbalance parameters in rotor-bearing systems. J. Sound Vib. 2018, 431, 54–69. [Google Scholar] [CrossRef] [Green Version]
- Colín-Ocampo, J.; Guitérrez-Wing, E.S.; Ramírez-Moroyoqui, F.J.; Abúndez-Pliego, A.; Blanco-Ortega, A.; Mayén, J. A novel methodology for the angular position identification of the unbalance force on asymmetric rotors by response polar plot analysis. Mech. Syst. Signal Process. 2017, 95, 172–186. [Google Scholar] [CrossRef]
- Mao, W.; Li, J.; Huang, Z.; Liu, J. Bearing dynamic parameters identification for sliding bearing-rotor system with uncertainty. Inverse Probl. Sci. Eng. 2018, 26, 1094–1108. [Google Scholar] [CrossRef]
- Chen, C.; Jing, J.; Cong, J.; Dai, Z. Identification of dynamic coefficients in circular journals bearings from unbalance response and complementary equations. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2019, 233, 1016–1028. [Google Scholar] [CrossRef]
- Rajasekhara, R.M.; Srinivas, J. An optimized bearing parameter identification approach from vibration response spectra. J. Vibroeng. 2019, 21, 1519–1532. [Google Scholar]
- Wang, A.; Yao, W.; He, K.; Meng, G.; Cheng, X.; Yang, J. Analytical modelling and numerical experiment for simultaneous identification of unbalance and rolling-bearing coefficients of the continuous single-disc and single-span rotor-bearing system with Rayleigh beam model. Mech. Syst. Signal Process. 2019, 116, 322–346. [Google Scholar] [CrossRef]
- Wang, X.; Li, H.; Meng, G. Rotordynamic coefficients of a controllable magnetorheological fluid lubricated floating ring bearing. Tribol. Int. 2017, 114, 1–14. [Google Scholar] [CrossRef]
- Kang, Y.; Shi, Z.; Zhang, H.; Zhen, D.; Gu, F. A novel method for the dynamic coefficients identification of journal bearings using Kalman filter. Sensors 2020, 20, 565. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, Y.; Yang, R.; Sugita, N.; Mao, J.; Shinshi, T. Identification of bearing dynamic parameters and unbalanced forces in a flexible rotor system supported by oil-film bearings and active magnetic devices. Actuators 2021, 10, 216. [Google Scholar] [CrossRef]
- Taherkhani, Z.; Ahmadian, H. Stochastic model updating of rotor support parameters using Bayesian approach. Mech. Syst. Signal Process. 2021, 158, 107702. [Google Scholar] [CrossRef]
- Brito, G.C., Jr.; Machado, R.D.; Neto, A.C.; Kimura, L.Y. A method for the experimental estimation of direct and cross-coupled dynamic coefficients of tilting-pad journal bearings of vertical hydro-generators. Struct. Health Monit. 2021, 1–17. [Google Scholar] [CrossRef]
- San Andrés, L.; Jeung, S.H. Orbit-model force coefficients for fluid film bearings: A step beyond linearization. ASME J. Eng. Gas Turbines Power 2015, 132, 022502. [Google Scholar]
- Yang, L.H.; Wang, W.M.; Zhao, S.Q.; Sun, Y.H.; Yu, L. A new nonlinear dynamic analysis method of rotor system supported by oil-film journal bearings. Appl. Math. Model. 2014, 38, 5239–5255. [Google Scholar] [CrossRef]
- Dyk, S.; Smolík, L.; Rendl, J. Predictive capability of various linearization approaches for floating-ring bearings in nonlinear dynamics of turbochargers. Mech. Mach. Theory 2020, 149, 103843. [Google Scholar] [CrossRef]
- Nelles, O. Nonlinear System Identification. From Classical Approaches to Neural Networks, Fuzzy Models, and Gaussian Processes, 1st ed.; Springer Nature: Cham, Switzerland, 2020. [Google Scholar]
- Tangirala, A.K. Principles of System Identification: Theory and Practice, 1st ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Aster, R.C.; Borchers, B.; Thurber, C.H. Parameter Estimation and Inverse Problems, 3rd ed.; Elsevier: Cambridge, MA, USA, 2019. [Google Scholar]
- Arias-Montiel, M.; Beltrán-Carbajal, F.; Silva-Navarro, G. On-line algebraic identification of eccentricity parameters in active rotor-bearing systems. Int. J. Mech. Sci. 2014, 85, 152–159. [Google Scholar] [CrossRef]
- Sira-Ramírez, H.; García-Rodríguez, C.; Cortés-Romero, J.; Luviano-Juárez, A. Algebraic Identification and Estimation Methods in Feedback Control Systems, 1st ed.; John Wiley & Sons: West Sussex, UK, 2014. [Google Scholar]
- Trujillo-Franco, L.G.; Abundis-Fong, H.F.; Campos-Amezcua, R.; Gomez-Martinez, R.; Martinez-Perez, A.I.; Campos-Amezcua, A. Single output and algebraic modal parameters identification of a wind turbine blade: Experimental results. Appl. Sci. 2021, 11, 3016. [Google Scholar] [CrossRef]
- Silva-Navarro, G.; Beltrán-Carbajal, F.; Trujillo-Franco, L.G.; Peza-Solís, J.F.; García-Pérez, O.A. Online estimation techniques for natural and excitation frequencies on MDOF vibrating mechanical systems. Actuators 2021, 10, 41. [Google Scholar] [CrossRef]
- Trujillo-Franco, L.G.; Silva-Navarro, G.; Beltrán-Carbajal, F.; Campos-Mercado, E.; Abundis-Fong, H.F. On-line modal parameter identification applied to linear and nonlinear vibration absorbers. Actuators 2020, 9, 119. [Google Scholar] [CrossRef]
- Beltrán-Carbajal, F.; Silva-Navarro, G. Generalized nonlinear stiffness identification on controlled mechanical vibrating systems. Asian J. Control 2019, 21, 1281–1292. [Google Scholar] [CrossRef]
- Beltran-Carbajal, F.; Silva-Navarro, G.; Trujillo-Franco, L.G. On-line parametric estimation of damped multiple frequency oscillations. Electr. Power Syst. Res. 2018, 154, 423–432. [Google Scholar] [CrossRef]
- Beltrán-Carbajal, F.; Silva-Navarro, G.; Trujillo-Franco, L.G. A sequential algebraic parametric identification approach for nonlinear vibrating mechanical systems. Asian J. Control 2017, 19, 1–11. [Google Scholar] [CrossRef]
- Mendoza-Larios, J.G.; Colín-Ocampo, J.; Blanco-Ortega, A.; Abúndez-Pliego, A.; Gutiérrez-Wing, E.S. Automatic balancing of a rotor-bearing system: On-line algebraic identifier for a rotordynamic balancing system. Rev. Ibearoam. Autom. Inform. Ind. 2016, 13, 281–292. [Google Scholar]
- Colín-Ocampo, J.; Mendoza-Larios, J.G.; Blanco-Ortega, A.; Abúndez-Pliego, A.; Gutiérrez-Wing, E.S. Unbalance determination in rotor-bearing systems at constant velocity: Algebraic identification method. Ing. Mec. Tecnol. Des. 2016, 5, 385–394. (In Spanish) [Google Scholar]
- Beltrán-Carbajal, F.; Silva-Navarro, G. On the algebraic parameter identification of vibrating mechanical systems. Int. J. Mech. Sci. 2015, 92, 178–186. [Google Scholar] [CrossRef]
- Beltrán-Carbajal, F.; Silva-Navarro, G.; Arias-Montiel, M. Active unbalance control of rotor systems using on-line algebraic identification methods. Asian J. Control 2013, 15, 1627–1637. [Google Scholar] [CrossRef]
- Lalanne, M.; Ferraris, G. Rotordynamics Prediction in Engineering, 2nd ed.; John Wiley & Sons: West Sussex, UK, 1998. [Google Scholar]
- Kharab, A.; Guenther, R.B. An Introduction to Numerical Methods. A MATLAB Approach; CRC Pess: Boca Raton, FL, USA, 2019. [Google Scholar]
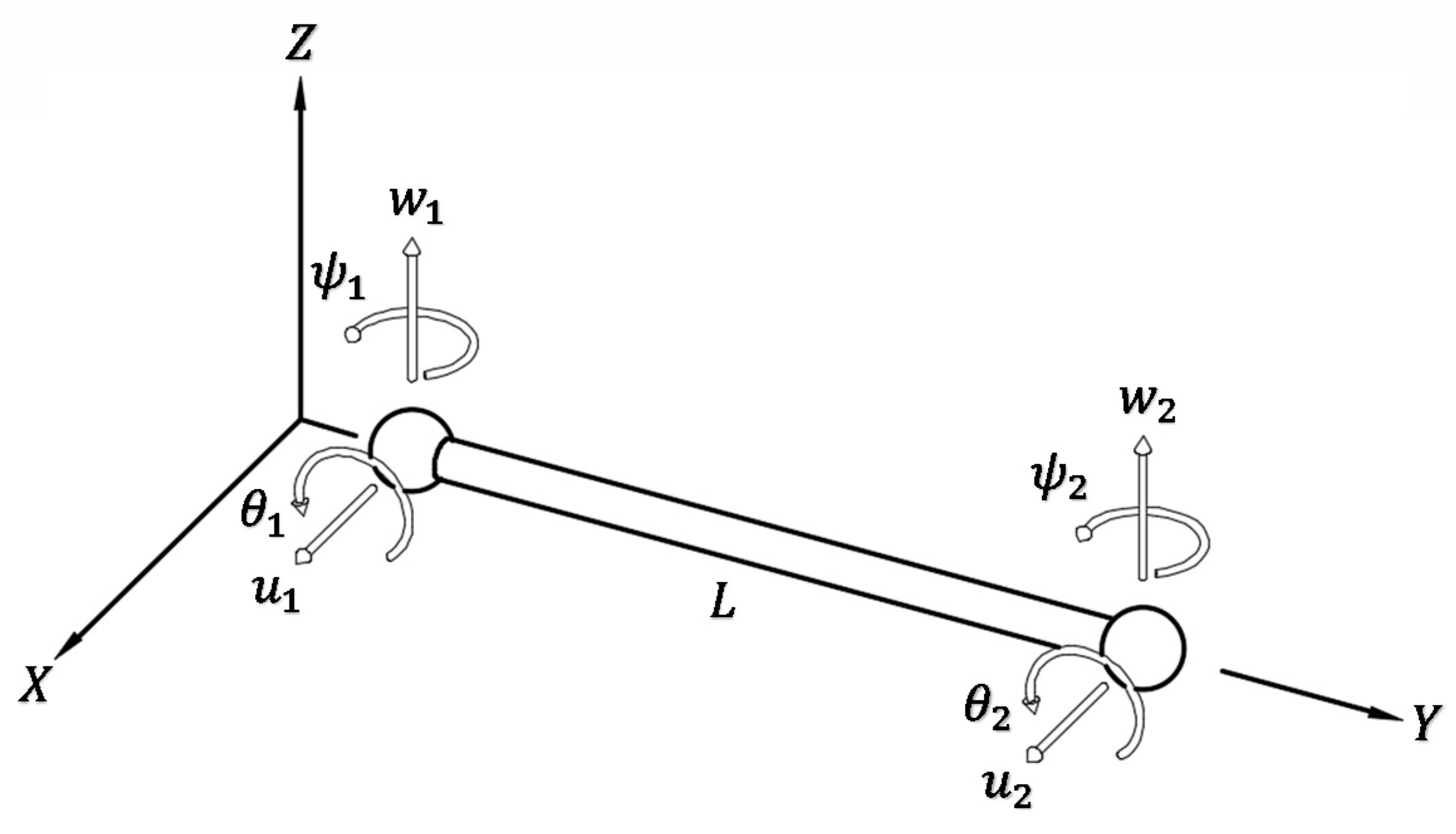
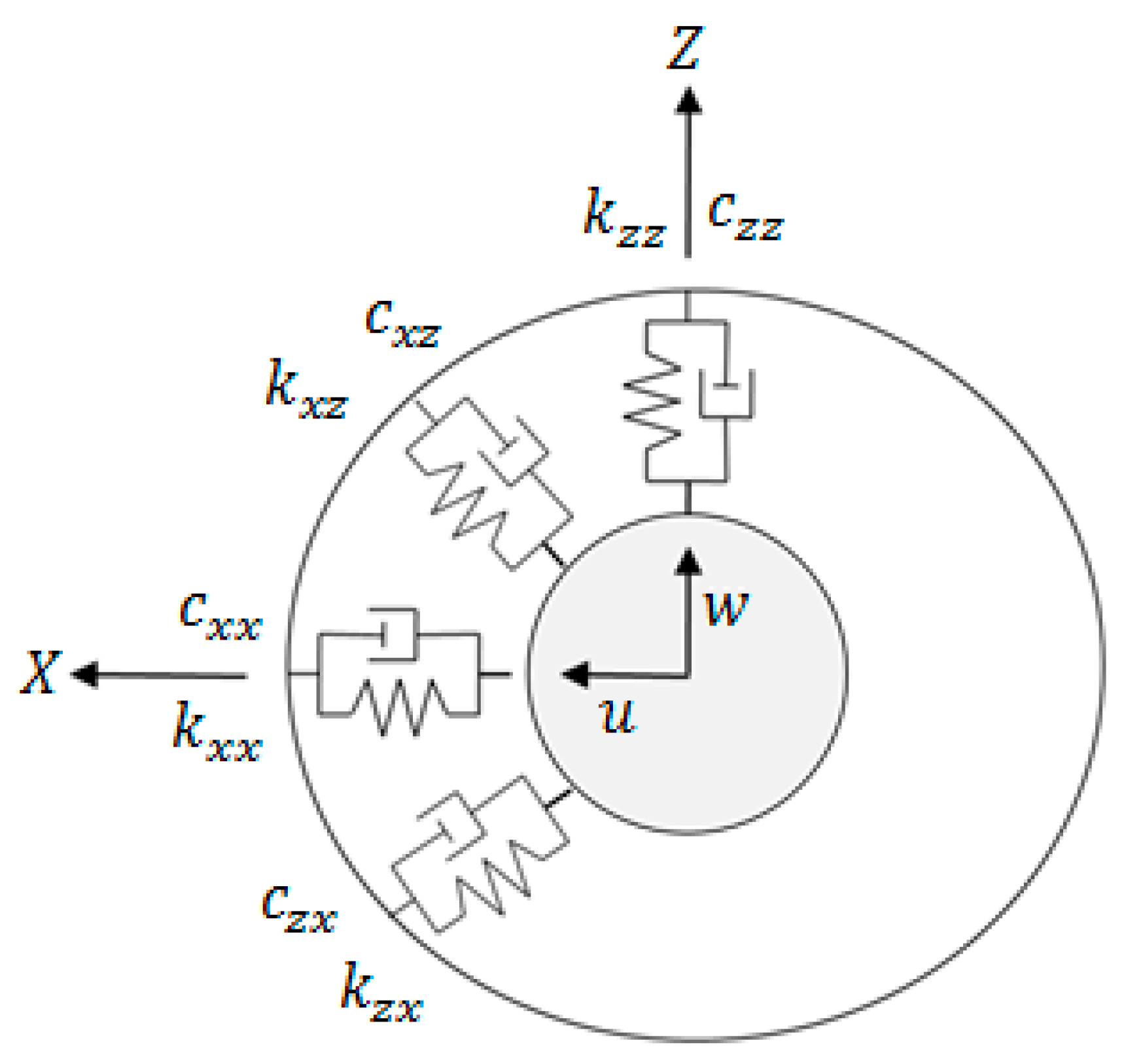
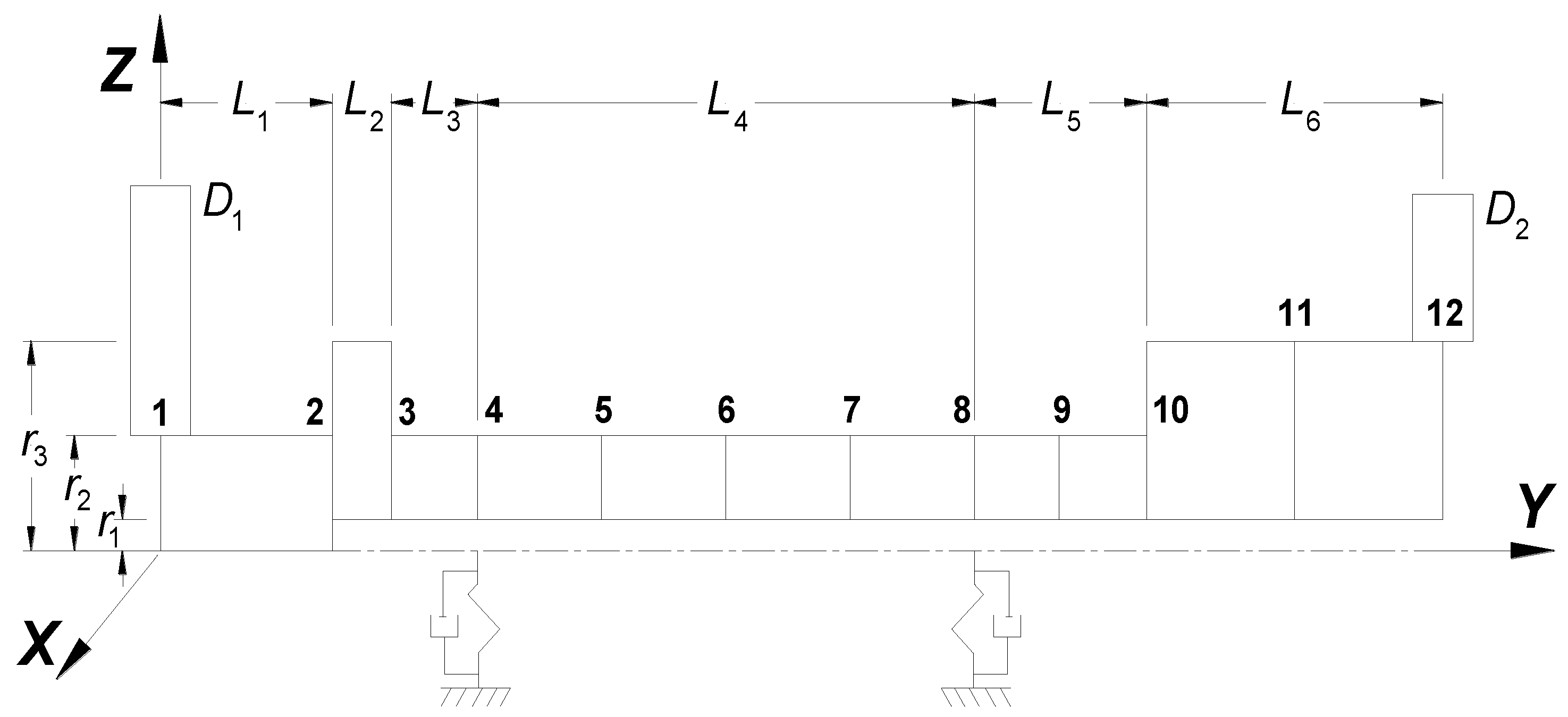
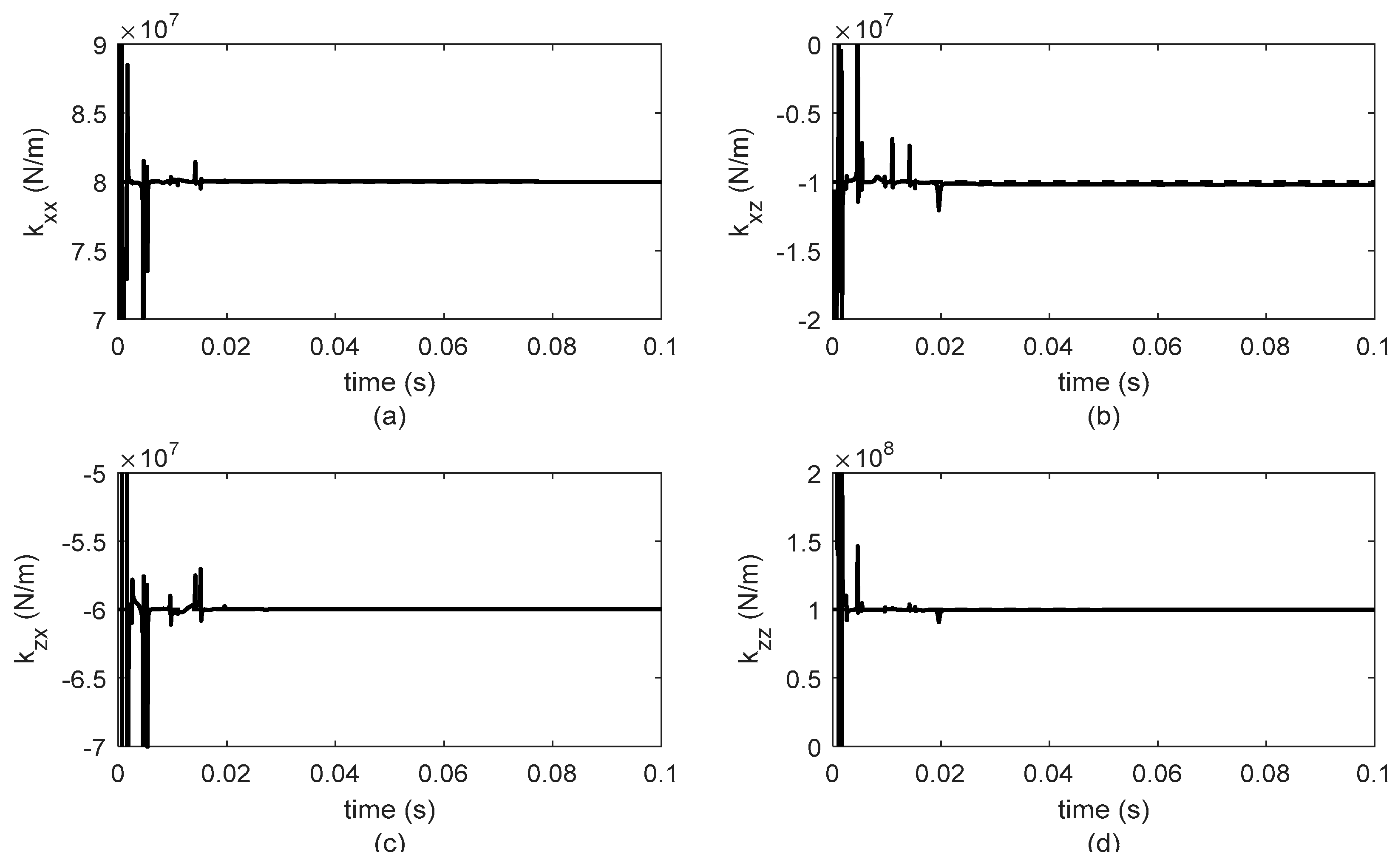
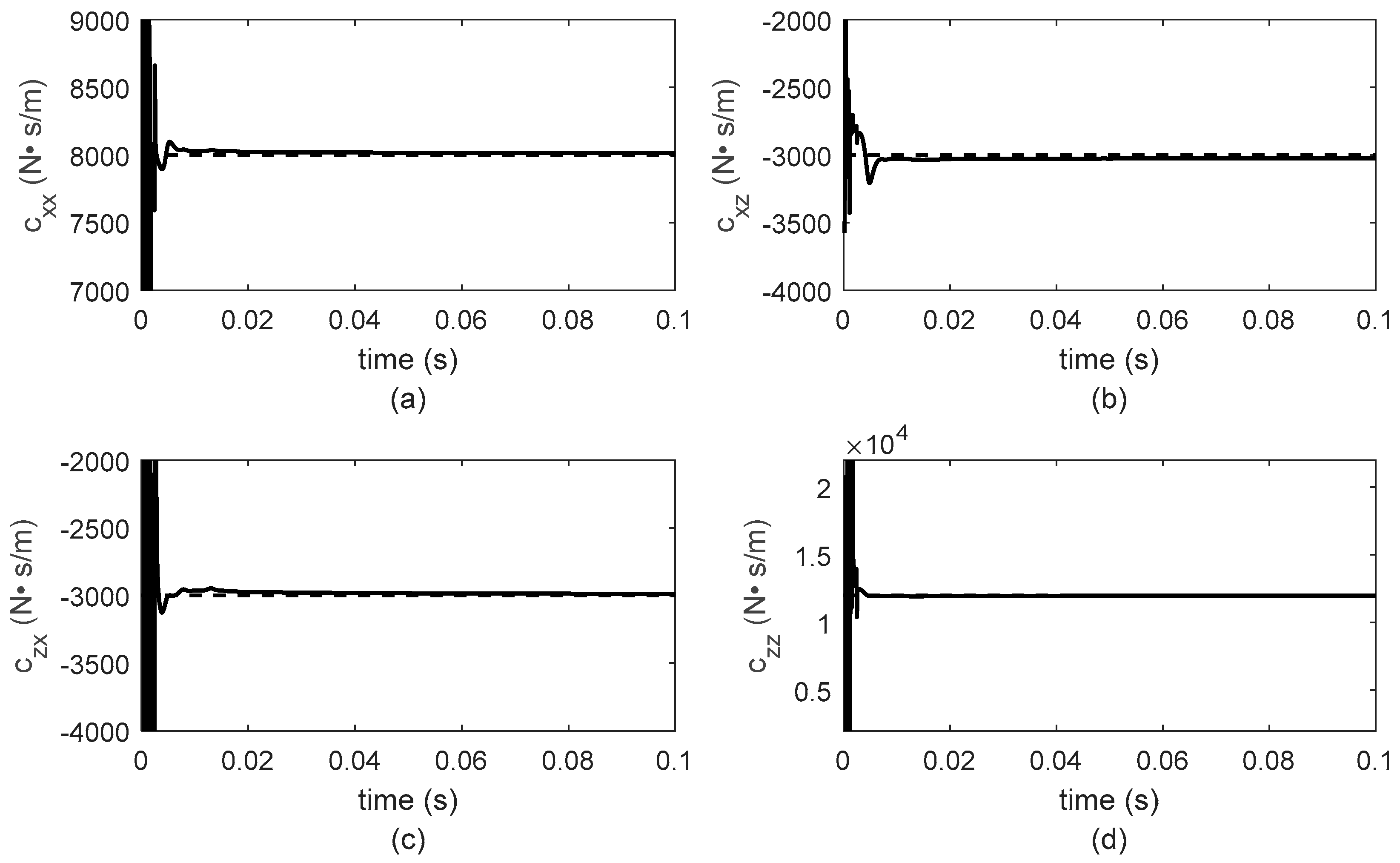
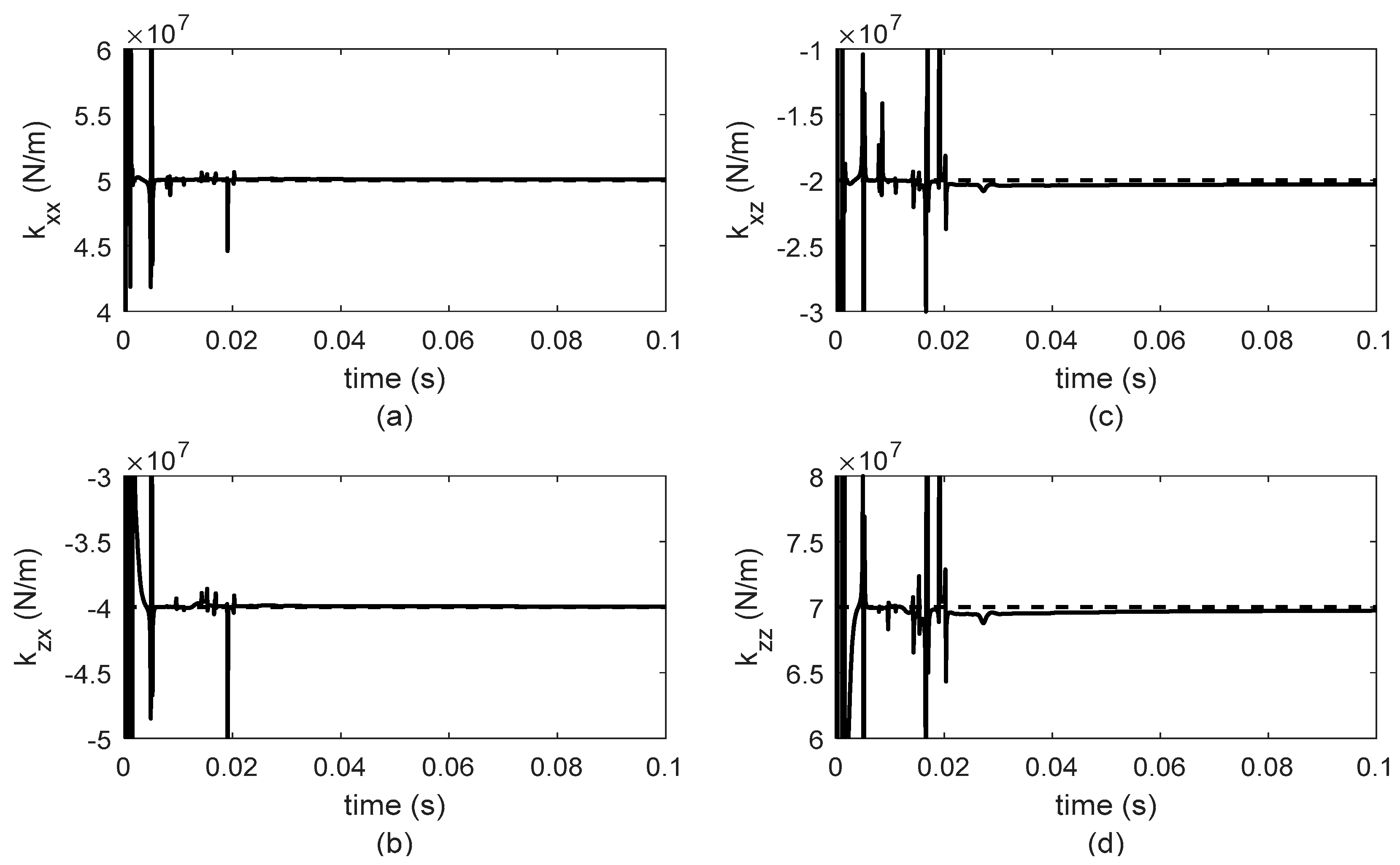
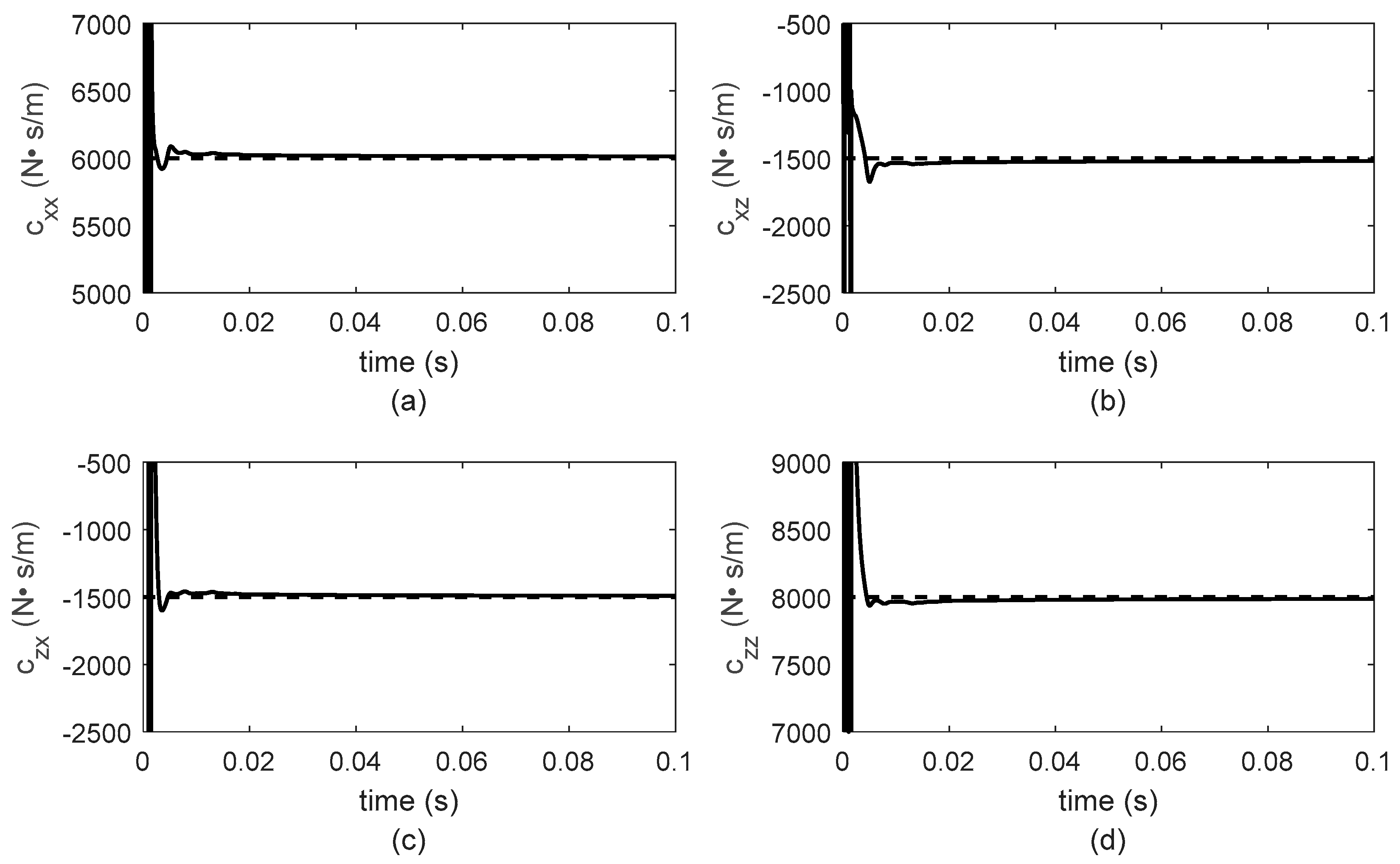



| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Modulus of elasticity | |||
| Density | |||
| Poisson ratio | |||
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| mass | mass | ||
| moment of inertia | moment of inertia | ||
| polar moment of inertia | polar moment of inertia | ||
| mass unbalance | mass unbalance |
| Parameter | Bearing 1 (Node 4) | Bearing 2 (Node 8) |
|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mendoza-Larios, J.G.; Barredo, E.; Arias-Montiel, M.; Baltazar-Tadeo, L.A.; Landa-Damas, S.J.; Tapia-Herrera, R.; Colín-Ocampo, J. An Algebraic Approach for Identification of Rotordynamic Parameters in Bearings with Linearized Force Coefficients. Mathematics 2021, 9, 2747. https://doi.org/10.3390/math9212747
Mendoza-Larios JG, Barredo E, Arias-Montiel M, Baltazar-Tadeo LA, Landa-Damas SJ, Tapia-Herrera R, Colín-Ocampo J. An Algebraic Approach for Identification of Rotordynamic Parameters in Bearings with Linearized Force Coefficients. Mathematics. 2021; 9(21):2747. https://doi.org/10.3390/math9212747
Chicago/Turabian StyleMendoza-Larios, José Gabriel, Eduardo Barredo, Manuel Arias-Montiel, Luis Alberto Baltazar-Tadeo, Saulo Jesús Landa-Damas, Ricardo Tapia-Herrera, and Jorge Colín-Ocampo. 2021. "An Algebraic Approach for Identification of Rotordynamic Parameters in Bearings with Linearized Force Coefficients" Mathematics 9, no. 21: 2747. https://doi.org/10.3390/math9212747
APA StyleMendoza-Larios, J. G., Barredo, E., Arias-Montiel, M., Baltazar-Tadeo, L. A., Landa-Damas, S. J., Tapia-Herrera, R., & Colín-Ocampo, J. (2021). An Algebraic Approach for Identification of Rotordynamic Parameters in Bearings with Linearized Force Coefficients. Mathematics, 9(21), 2747. https://doi.org/10.3390/math9212747







