Study on Purging Strategy of Polymer Electrolyte Membrane Fuel Cell under Different Operation Conditions
Abstract
:1. Introduction
2. Materials and Methods
- (1)
- Ideal gas law is assumed for gas mixtures in low pressure environment.
- (2)
- Laminar and incompressible flow was considered for the gaseous phase.
- (3)
- Ignoring the influence of gravity in micro-channels.
- (4)
- The performance of electrode materials remains stable.
3. Results and Discussion
3.1. Grid Independency
3.2. Output Characteristics of PEMFC under Subzero Temperatures Conditions
3.2.1. Electrochemical Characteristics of PEMFC under Different Initial Conditions
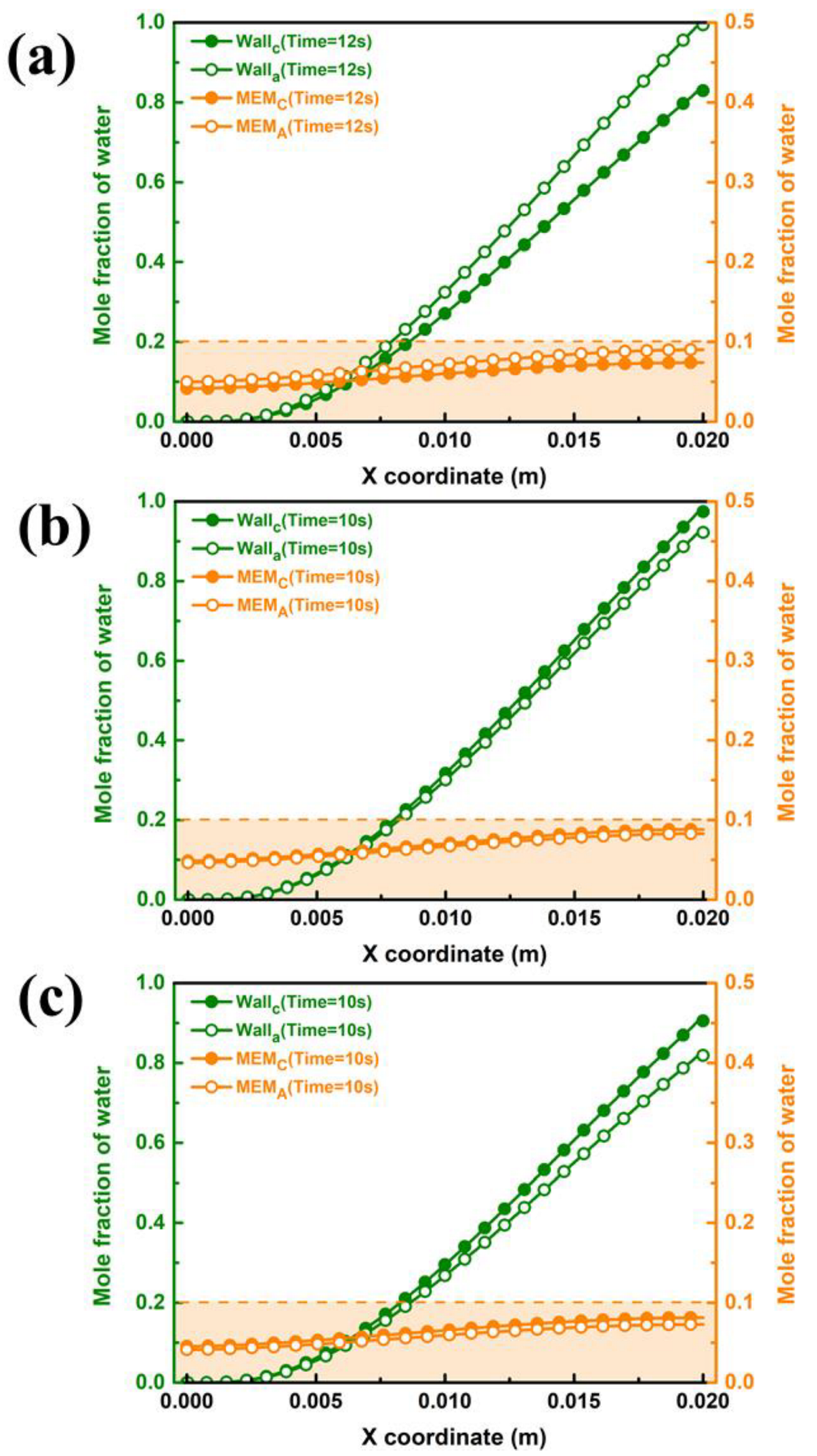
3.2.2. Water Distribution under Different Initial Conditions
3.3. Purging Strategy under Different Initial Distribution Conditions
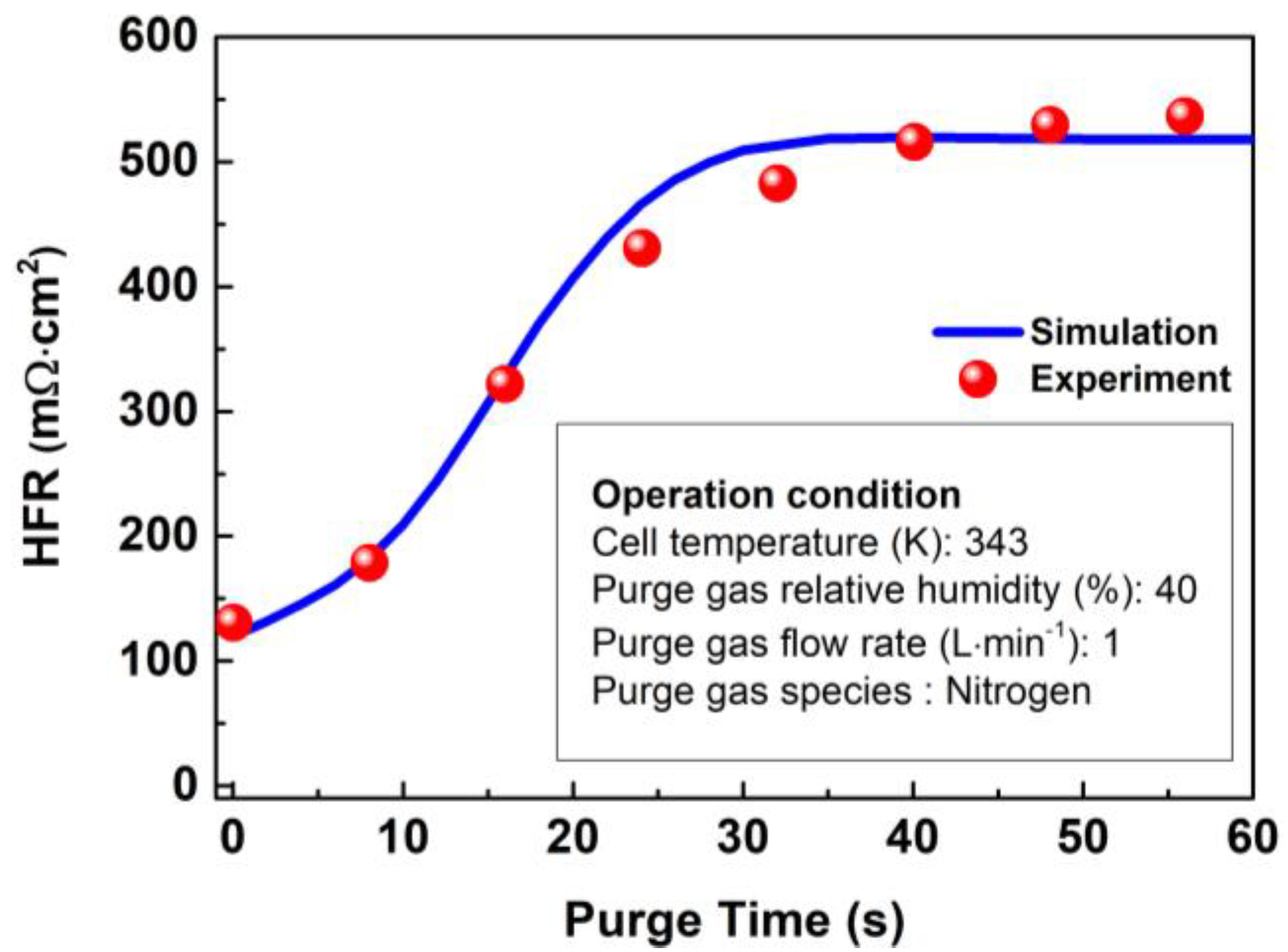
3.3.1. Model Validation
3.3.2. Characteristics of the Dryness Process in GC of Different Cases
3.3.3. Characteristics of the Dryness Process in GC of Different Cases
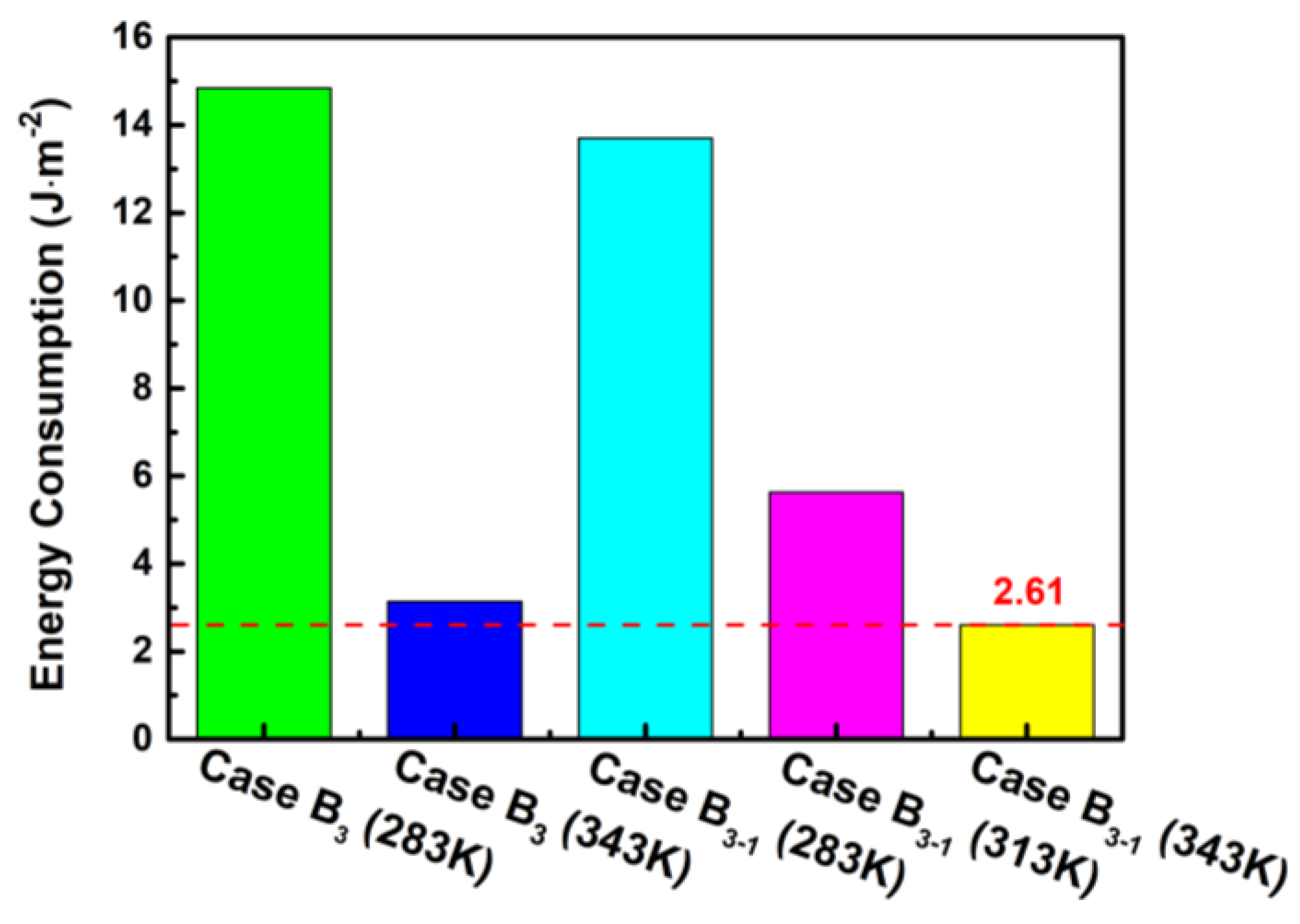
3.4. Analysis of Energy Consumption under Different Purge Conditions
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area, m2 |
| c | molar concentration, mol m−3 |
| D | diffusion coefficient, m2 s−1 |
| F | Faraday’s constant, C mol−1 |
| h | heat transfer coefficient, W m−2 K−1 |
| j | reference exchange current density, A m−2 |
| I | cell current density, A m−2 |
| k | thermal conductivity, W m−1 K−1 |
| M | molar mass, kg mol−1 |
| p | pressure, Pa |
| R | universal gas constant, 8.314 J mol−1 K−1 |
| s | liquid water saturation |
| S | source term of governing equations |
| t | time, s |
| T | temperature, K |
| J | molar flow, mol m−2 s−1 |
| u | velocity vector, m s−1 |
| x, y, z | coordinate, m |
| i | species |
| Abbreviations | |
| sat | saturated |
| ref | reference |
| eff | effective |
| eq | equilibrium |
| cl | catalytic layer |
| gc | gas channel |
| gdl | gas diffusion layer |
| pem | proton exchange membrane |
| ohm | ohmic heat |
| EW | equivalent weight, kg mol−1 |
| l−v | liquid to vapour |
| cl→gdl | catalytic layer to gas diffusion layer |
| PEMFC | proton exchange membrane fuel cell |
| Greek Symbols | |
| transfer coefficient | |
| porosity | |
| stoichiometric flow ratio | |
| overpotential, V | |
| membrane water content | |
| concentration index | |
| viscosity, kg m−1 s−1 | |
| density, kg m−3 | |
| electrical conductivity, S m−1 | |
| thickness, m | |
| resistance coefficient, kg−1 m4 | |
| volume fraction of polymer | |
| potential, V | |
| Superscripts | |
| a | anode |
| c | cathode |
| in | inlet |
| ext | external |
References
- Sun, C.Y.; Zhang, H. Review of the development of first-generation redox flow batteries: Iron-chromium system. ChemSusChem 2022, 15, e202101798. [Google Scholar] [CrossRef] [PubMed]
- Priya, K.; Sathishkumar, K.; Rajasekar, N. A comprehensive review on parameter estimation techniques for Proton Exchange Membrane fuel cell modeling. Renew. Sustain. Energ. Rev. 2018, 93, 121–144. [Google Scholar] [CrossRef]
- Lu, X.Q.; Qu, Y.; Wang, Y.D.; Qin, C.; Liu, G. A comprehensive review on hybrid power system for PEMFC-HEV: Issues and strategies. Energy Convers. Manag. 2018, 171, 1273–1291. [Google Scholar] [CrossRef]
- Lei, L.; He, P.; He, P.; Tao, W.Q. A comparative study: The effect of current loading modes on the cold start-up process of PEMFC stack. Energy Convers. Manag. 2022, 251, 114991. [Google Scholar] [CrossRef]
- Wang, Y.; Sauer, D.U.; Koehne, S.; Ersoez, A. Dynamic modeling of high temperature PEM fuel cell start-up process. Int. J. Hydrogen Energy 2022, 251, 114991. [Google Scholar] [CrossRef]
- Kamal, R.; Chan, S.H. Sensitivity analysis of anode overpotential during start-up process of a high temperature proton exchange membrane fuel cell. Electrochim. Acta 2015, 176, 965–975. [Google Scholar] [CrossRef]
- Rajalakshmi, N.; Pandiyan, S.; Dhathathreyan, K.S. Design and development of modular fuel cell stacks for various applications. Int. J. Hydrogen Energy 2008, 33, 449–454. [Google Scholar] [CrossRef]
- Zhao, D.D.; Xia, L.; Dang, H.B.; Wu, Z.Z.; Li, H.Y. Design and control of air supply system for PEMFC UAV based on dynamic decoupling strategy. Energy Convers. Manag. 2022, 253, 115159. [Google Scholar] [CrossRef]
- Hu, D.H.; Wang, Y.T.; Li, J.W.; Yang, Q.Q.; Wang, J. Investigation of optimal operating temperature for the PEMFC and its tracking control for energy saving in vehicle applications. Energy Convers. Manag. 2021, 249, 114842. [Google Scholar] [CrossRef]
- Lee, K.; Han, G.; Cho, S.; Bae, J. Pressurized diesel fuel processing using hydrogen peroxide for the fuel cell power unit in low-oxygen environments. J. Power Sources 2018, 380, 37–45. [Google Scholar] [CrossRef]
- Han, G.; Kwon, Y.; Kim, J.B.; Lee, S.; Bae, J.; Cho, E.; Lee, B.J.; Cho, S.; Park, J. Development of a high-energy-density portable/mobile hydrogen energy storage system incorporating an electrolyzer, a metal hydride and a fuel cell. Appl. Energy 2020, 259, 114175. [Google Scholar] [CrossRef]
- Chaparro, A.M.; Ferreira-Aparicio, P.; Folgado, M.A.; Hubscher, R.; Lange, C.; Weber, N. Thermal neutron radiography of a passive proton exchange membrane fuel cell for portable hydrogen energy systems. J. Power Sources 2020, 480, 228668. [Google Scholar] [CrossRef]
- Lin, R.; Weng, Y.M.; Lin, X.W.; Xiong, F. Rapid cold start of proton exchange membrane fuel cells by the printed circuit board technology. Int. J. Hydrogen Energy 2014, 39, 18369–18378. [Google Scholar] [CrossRef]
- Jiao, K.; Li, X.G. Water transport in polymer electrolyte membrane fuel cells. Prog. Energy Combust. 2011, 37, 221–291. [Google Scholar] [CrossRef]
- Liao, Z.H.; Wei, L.; Dafalla, A.M.; Suo, Z.B.; Jiang, F.M. Numerical study of subfreezing temperature cold start of proton exchange membrane fuel cells with zigzag-channeled flow field. Int. J. Heat. Mass. Tran. 2021, 165, 120733. [Google Scholar] [CrossRef]
- Niu, H.P.; Ji, C.W.; Wang, S.F.; Shi, M.Y.; Zhang, H.L.; Liang, C. Analysis of the cold start behavior of a polymer electrolyte membrane fuel cell in constant power start-up mode. Int. J. Energy Res. 2021, 45, 19245–19264. [Google Scholar] [CrossRef]
- Lin, R.; Zhong, D.; Lan, S.B.; Guo, R.; Ma, Y.Y.; Cai, X. Experimental validation for enhancement of PEMFC cold start performance: Based on the optimization of micro porous layer. Appl. Energy 2021, 300, 117306. [Google Scholar] [CrossRef]
- Huo, S.; Li, L.C.; Shi, W.Y.; Wang, R.F.; Lu, B.B.; Yin, Y.; Zhu, C.Y.; Wang, Y.; Jiao, K.; Hou, Z.J. Characteristics of cold start behavior of PEM fuel cell with metal foam as cathode flow field under subfreezing temperature. Int. J. Green Energy 2021, 18, 1129–1146. [Google Scholar] [CrossRef]
- Rios, G.M.; Schirmer, J.; Gentner, C.; Kallo, J. Efficient thermal management strategies for cold starts of a proton exchange membrane fuel cell system. Appl. Energy 2020, 279, 115813. [Google Scholar] [CrossRef]
- Himanen, O.; Hottinen, T.; Tuurala, S. Operation of a planar free-breathing PEMFC in a dead-end mode. Electorchem. Commun. 2007, 9, 891–894. [Google Scholar] [CrossRef]
- Liu, Z.Y.; Chen, J.; Liu, H.; Yan, C.Z.; Hou, Y.; He, Q.G.; Zhang, J.J.; Hissel, D. Anode purge management for hydrogen utilization and stack durability improvement of PEM fuel cell systems. Appl. Energy 2020, 275, 115110. [Google Scholar] [CrossRef]
- Lin, Y.F.; Chen, Y.S. Experimental study on the optimal purge duration of a proton exchange membrane fuel cell with a dead-ended anode. J. Power Sources 2017, 340, 176–182. [Google Scholar] [CrossRef]
- Cannio, M.; Righi, S.; Santangelo, P.E.; Romagnoli, M.; Pedicini, R.; Carbone, A.; Gatto, I. Smart catalyst deposition by 3D printing for Polymer Electrolyte Membrane Fuel Cell manufacturing. Renew. Energy 2021, 163, 414–422. [Google Scholar] [CrossRef]
- Luo, Y.; Jiao, K. Cold start of proton exchange membrane fuel cell. Prog. Energy Combust. Sci. 2018, 64, 29–61. [Google Scholar] [CrossRef]
- Yang, W.W.; Zhao, T.S.; Chen, R.; Xu, C. An approach for determining the liquid water distribution in a liquid-feed direct methanol fuel cell. J. Power Sources 2009, 190, 216–222. [Google Scholar] [CrossRef]
- He, Y.L.; Miao, Z.; Zhao, T.S.; Yang, W.W. Numerical study of the effect of the GDL structure on water crossover in a direct methanol fuel cell. Int. J. Hydrogen Energy 2012, 37, 4422–4438. [Google Scholar] [CrossRef]
- Kang, S. Quasi-three dimensional dynamic modeling of a proton exchange membrane fuel cell with consideration of two-phase water transport through a gas diffusion layer. Energy 2015, 90, 1388–1400. [Google Scholar] [CrossRef]
- Chen, X.; Xu, J.H.; Liu, Q.; Chen, Y.; Wang, X.D.; Li, W.B.; Ding, Y.J.; Wan, Z.M. Active disturbance rejection control strategy applied to cathode humidity control in PEMFC system. Energy Convers. Manag. 2021, 224, 113389. [Google Scholar] [CrossRef]
- Liu, S.H.; Chen, T.; Zhang, C.; Xie, Y. Study on the performance of proton exchange membrane fuel cell (PEMFC) with dead-ended anode in gravity environment. Appl. Energy 2020, 261, 114454. [Google Scholar] [CrossRef]
- Xie, Q.Z.; Zheng, M.G. CFD Simulation and Performance Investigation on a Novel Bionic Spider-Web-Type Flow Field for PEM Fuel Cells. Processes 2021, 9, 1526. [Google Scholar] [CrossRef]
- Yoo, B.; Lim, K.; Salihi, H.; Ju, H. A parametric study on the performance requirements of key fuel cell components for the realization of high-power automotive fuel cells. Int. J. Heat. Mass. Tran. 2022, 186, 122477. [Google Scholar] [CrossRef]
- Han, C.L.; Jiang, T.; Shang, K.; Xu, B.; Chen, Z.Q. Heat and mass transfer performance of proton exchange membrane fuel cells with electrode of anisotropic thermal conductivity. Int. J. Heat. Mass. Tran. 2022, 182, 121957. [Google Scholar] [CrossRef]
- Han, C.L.; Chen, Z.Q. Study on electrochemical and mass transfer coupling characteristics of proton exchange membrane (PEM) fuel cell based on a fin-like electrode surface. Int. J. Hydrogen Energy 2018, 43, 8026–8039. [Google Scholar] [CrossRef]
- Son, L.D.; Um, S.; Kim, Y.B. Effect of stacking pressure on the performance of polymer electrolyte membrane fuel cell with various channel types. Energy Convers. Manag. 2021, 232, 113803. [Google Scholar] [CrossRef]
- Yang, C.; Wan, Z.M.; Chen, X.; Kong, X.Z.; Zhang, J.; Huang, T.M.; Wang, X.D. Geometry optimization of a novel M-like flow field in a proton exchange membrane fuel cell. Energy Convers. Manag. 2021, 228, 113651. [Google Scholar] [CrossRef]
- Mu, Y.T.; He, P.; Ding, J.; Tao, W.Q. Modeling of the operation conditions on the gas purging performance of polymer electrolyte membrane fuel cells. Int. J. Hydrogen Energy 2017, 42, 11788–11802. [Google Scholar] [CrossRef]
- Wang, B.W.; Deng, H.; Jiao, K. Purge strategy optimization of proton exchange membrane fuel cell with anode recirculation. Appl. Energy 2018, 225, 1–13. [Google Scholar] [CrossRef]
- Shen, J.; Zeng, L.; Liu, Z.; Liu, W. Performance investigation of PEMFC with rectangle blockages in Gas Channel based on field synergy principle. Heat. Mass. Transfer. 2019, 55, 811–822. [Google Scholar] [CrossRef]
- Tajiri, K.; Wang, C.Y.; Tabuchi, Y. Water removal from a PEFC during gas purge. Electrochim. Acta 2008, 53, 6337–6343. [Google Scholar] [CrossRef]
- Sun, C.Y.; Negro, E.; Nale, A.; Pagot, G.; Vezzu, K.; Zawodzinski, T.A.; Meda, L.; Gambaro, C.; Di Noto, V. An efficient barrier toward vanadium crossover in redox flow batteries: The bilayer [Nafion/(WO3)(x)] hybrid inorganic-organic membrane. Electrochim. Acta 2021, 378, 138133. [Google Scholar] [CrossRef]
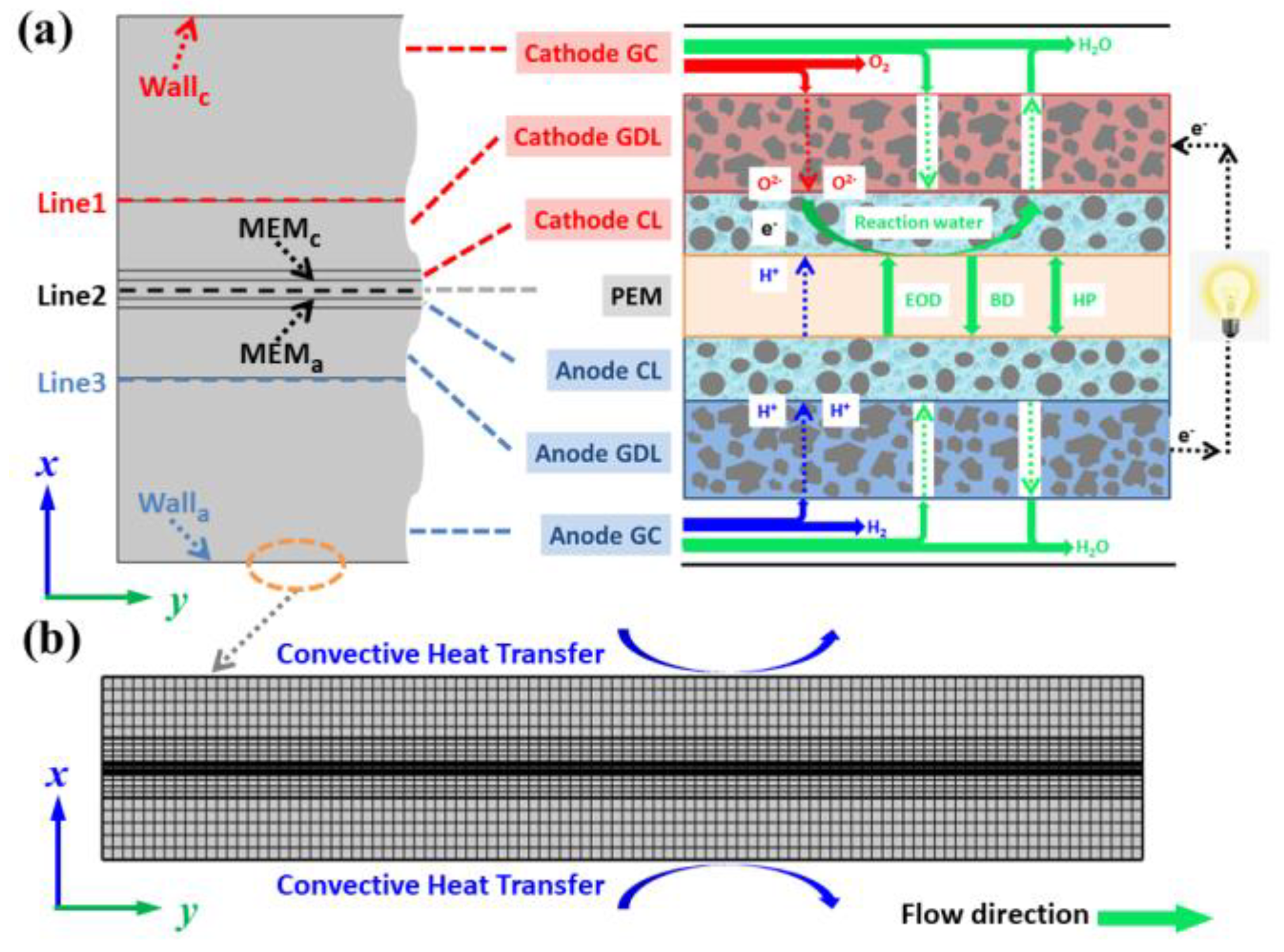

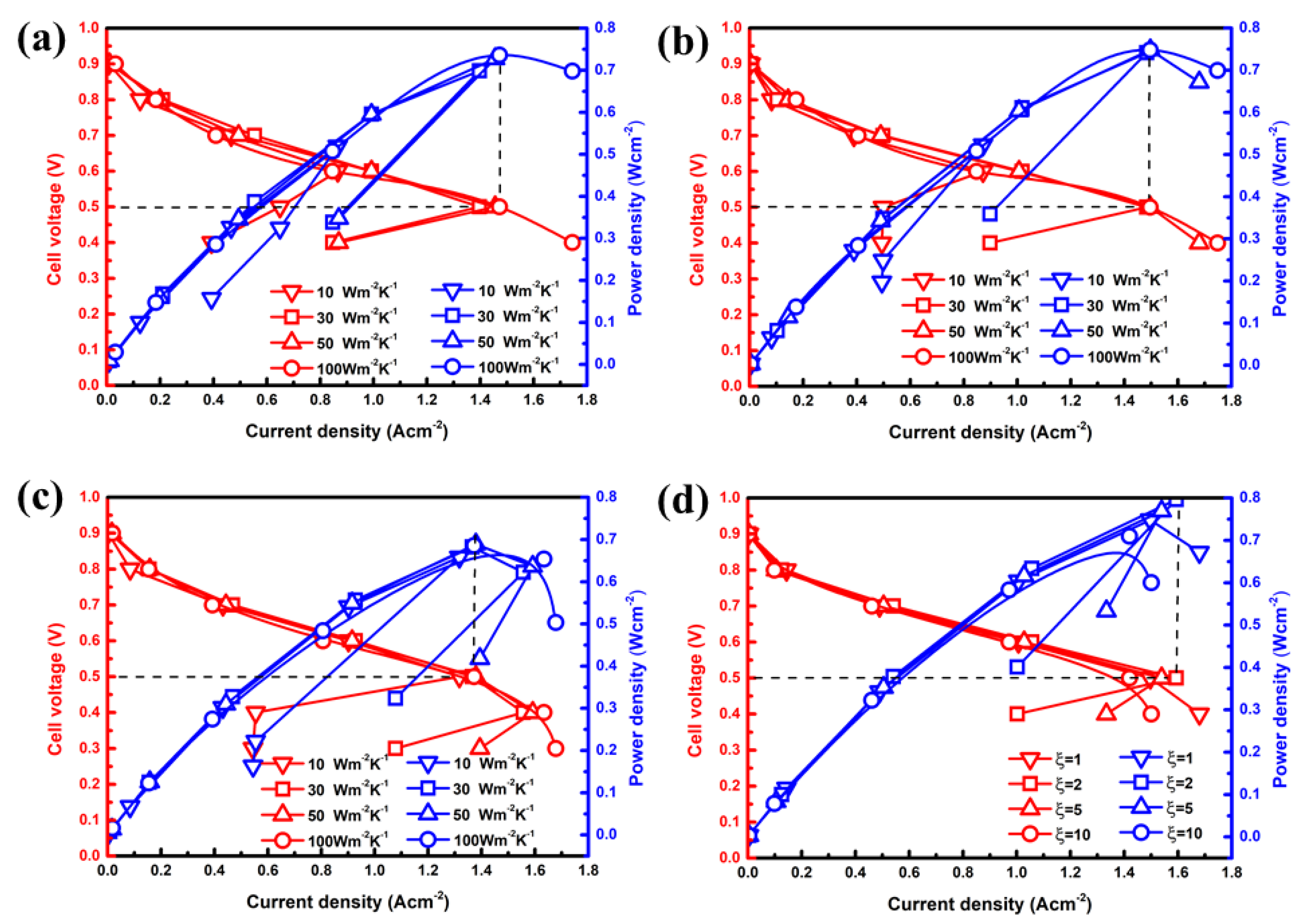
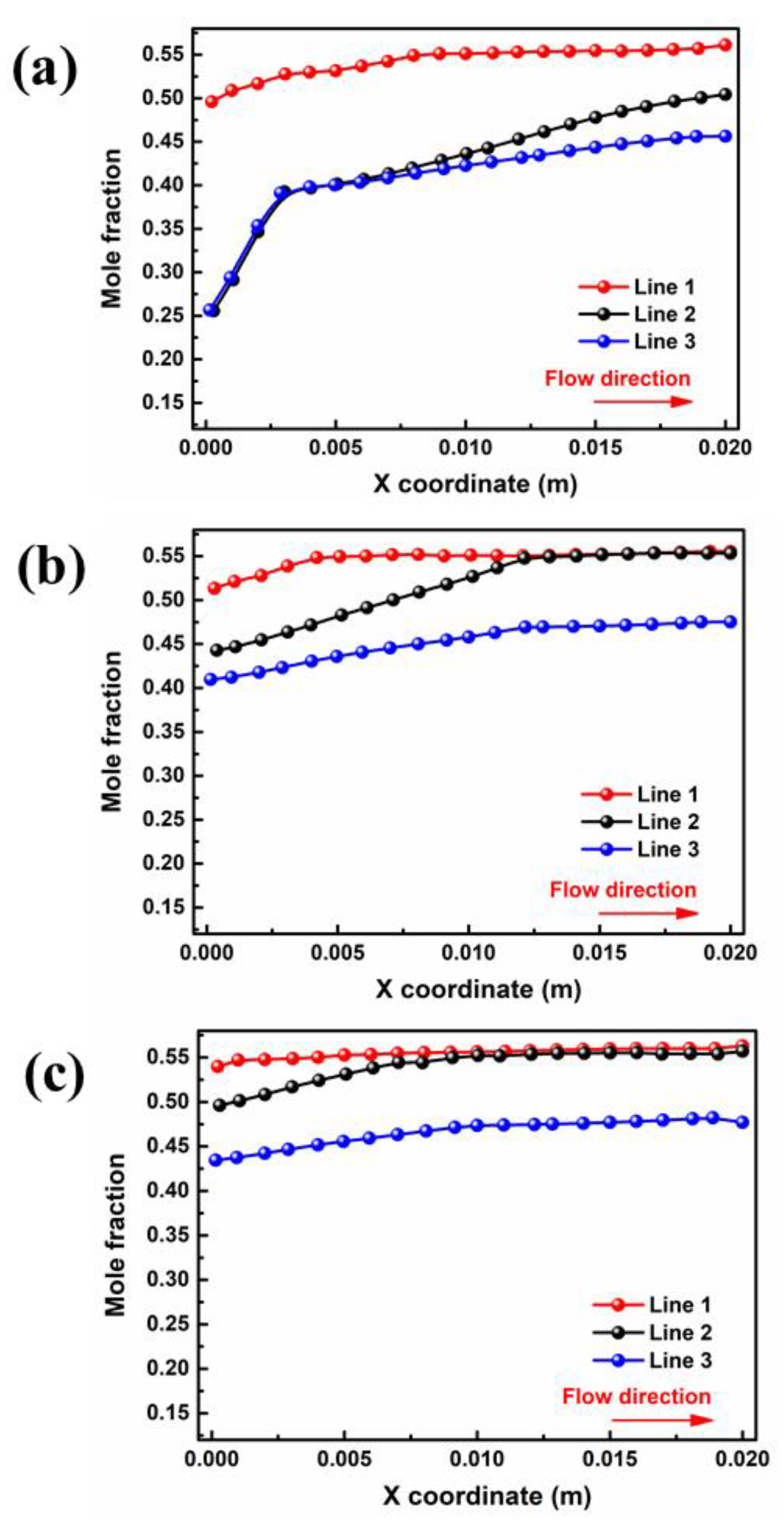
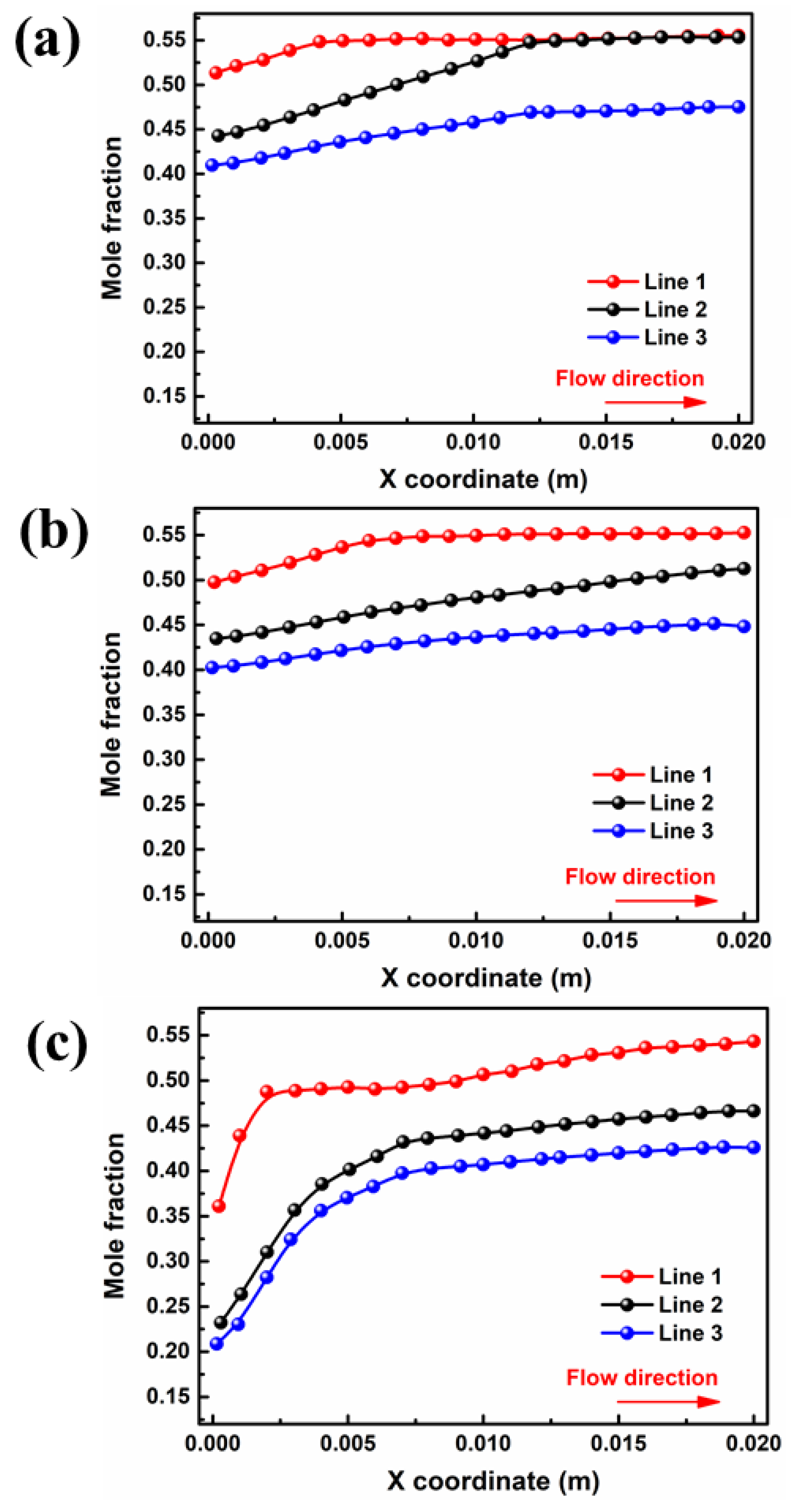


| Component | H (mm) | L (mm) |
|---|---|---|
| GC | 1 | 20 |
| GDL | 0.38 | 20 |
| CL | 0.05 | 20 |
| PEM | 0.1 | 20 |
| Parameter | Value | Ref. |
|---|---|---|
| 0.743 | [32] | |
| 0.257 | [32] | |
| 0.228 | [32] | |
| 0.749 | [32] | |
| 0.023 | [32] | |
| 101,325 | [32] | |
| 0.5 | [35] | |
| 1 | [36] | |
| 0.95 | [36] | |
| 222 | [32] | |
| 10,000 | [32] | |
| 41; 41 | [37] | |
| 2000; 0.00001 | [37] |
| h (W m−2K−1) | 10 | 30 | 50 | 100 | |
|---|---|---|---|---|---|
| 20/1 | Case A1 | Case A2 | Case A3 | Case A4 | |
| 60/1 | Case B1 | Case B2 | Case B3 | Case B4 | |
| 100/1 | Case C1 | Case C2 | Case C3 | Case C4 | |
| 60/2 | - | - | Case B3-1 | - | |
| 60/5 | - | - | Case B3-2 | - | |
| 60/10 | - | - | Case B3-3 | - | |
| Operating Voltage | Current Density under Different Grid Numbers (A cm−2) | |||
|---|---|---|---|---|
| 38,456 | 48,306 | 57,944 | 65,342 | |
| 0.8 V | 0.13 | 0.14 | 0.14 | 0.14 |
| 0.6 V | 0.96 | 0.99 | 1.01 | 1.01 |
| 0.4 V | 1.65 | 1.67 | 1.68 | 1.68 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, S.; Tian, A.; Han, C. Study on Purging Strategy of Polymer Electrolyte Membrane Fuel Cell under Different Operation Conditions. Processes 2023, 11, 290. https://doi.org/10.3390/pr11010290
Chen S, Tian A, Han C. Study on Purging Strategy of Polymer Electrolyte Membrane Fuel Cell under Different Operation Conditions. Processes. 2023; 11(1):290. https://doi.org/10.3390/pr11010290
Chicago/Turabian StyleChen, Shengpeng, Aina Tian, and Chaoling Han. 2023. "Study on Purging Strategy of Polymer Electrolyte Membrane Fuel Cell under Different Operation Conditions" Processes 11, no. 1: 290. https://doi.org/10.3390/pr11010290
APA StyleChen, S., Tian, A., & Han, C. (2023). Study on Purging Strategy of Polymer Electrolyte Membrane Fuel Cell under Different Operation Conditions. Processes, 11(1), 290. https://doi.org/10.3390/pr11010290






