Correlations for Total Entropy Generation and Bejan Number for Free Convective Heat Transfer of an Eco-Friendly Nanofluid in a Rectangular Enclosure under Uniform Magnetic Field
Abstract
:1. Introduction
2. Problem Description
2.1. Governing Equations and Boundary Condition
2.2. N-F Properties Equations
2.3. Numerical Procedure
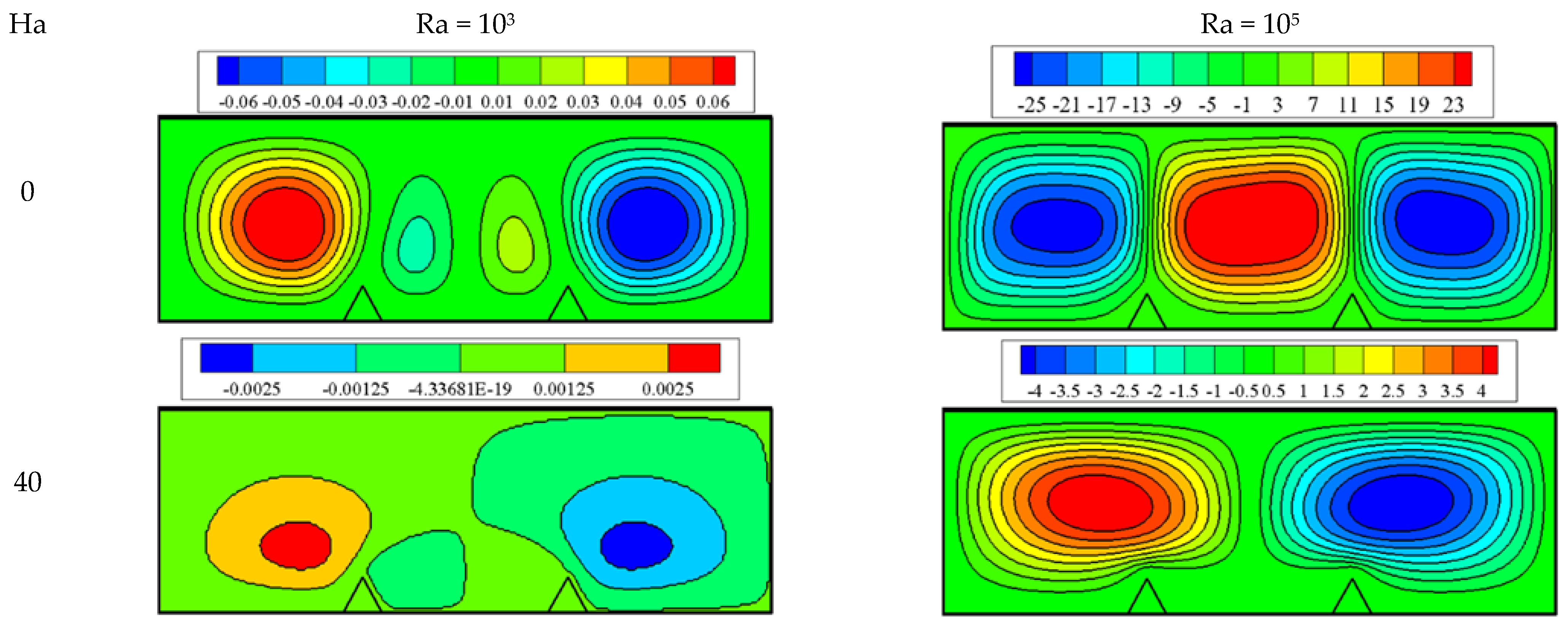
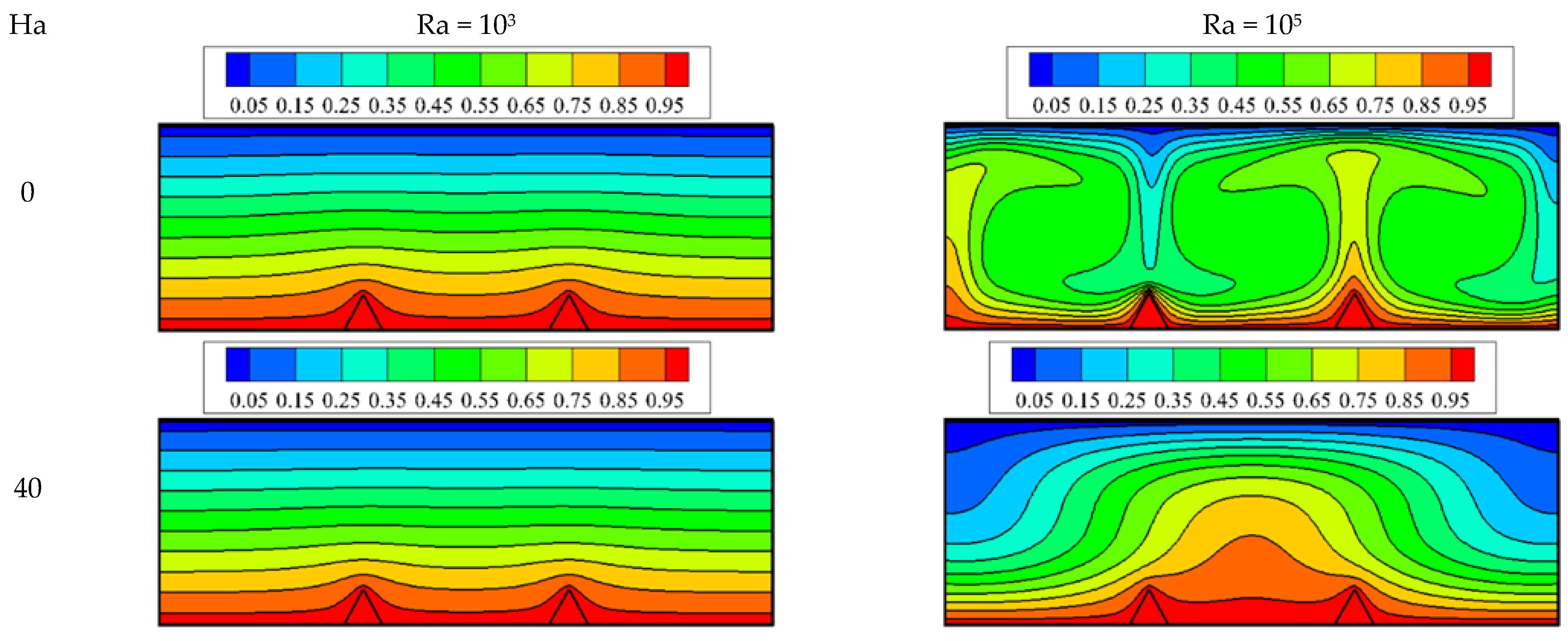
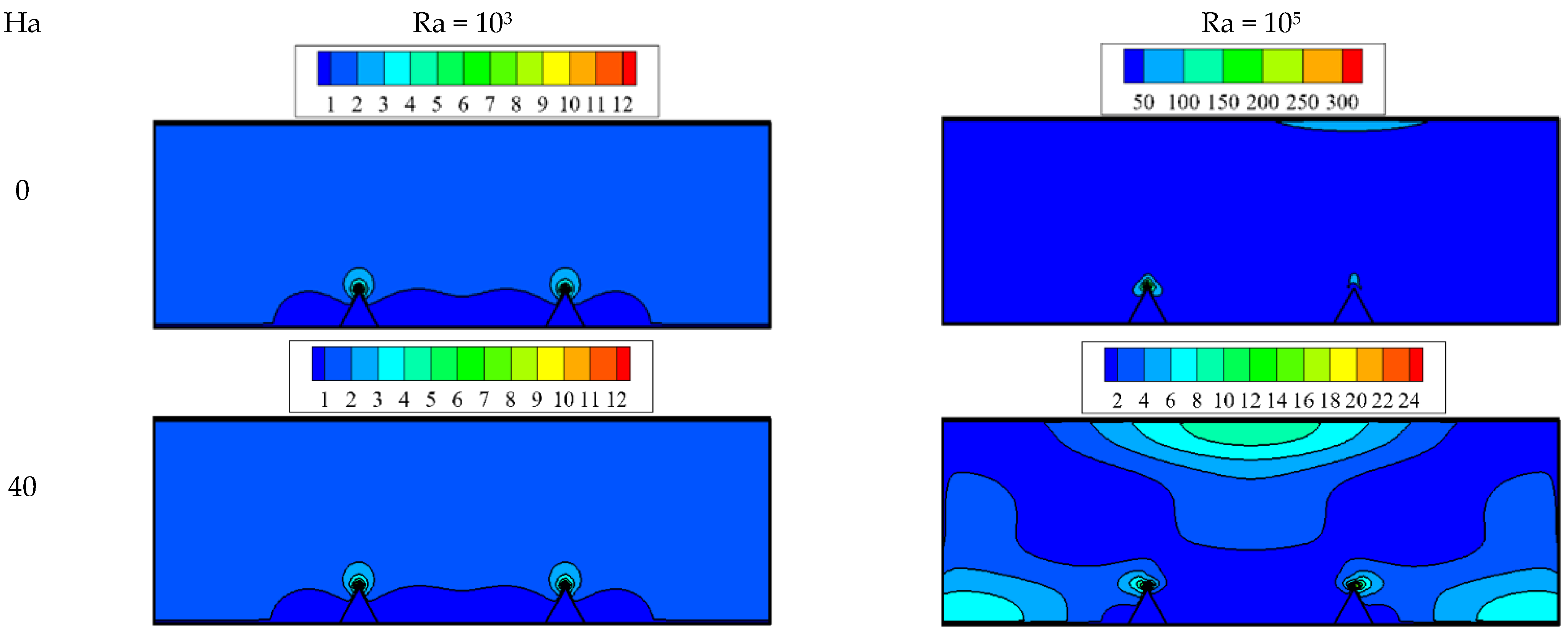
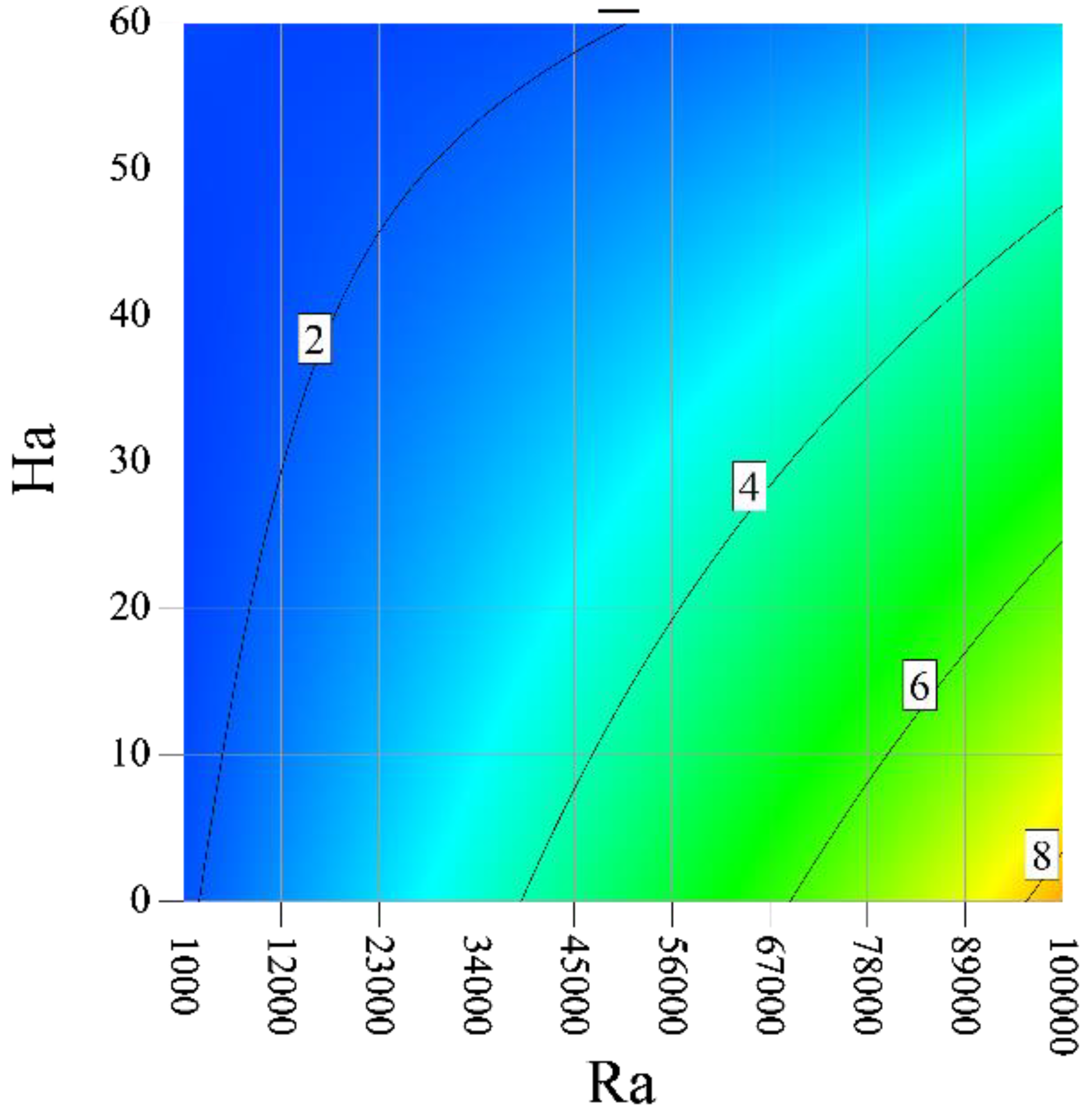
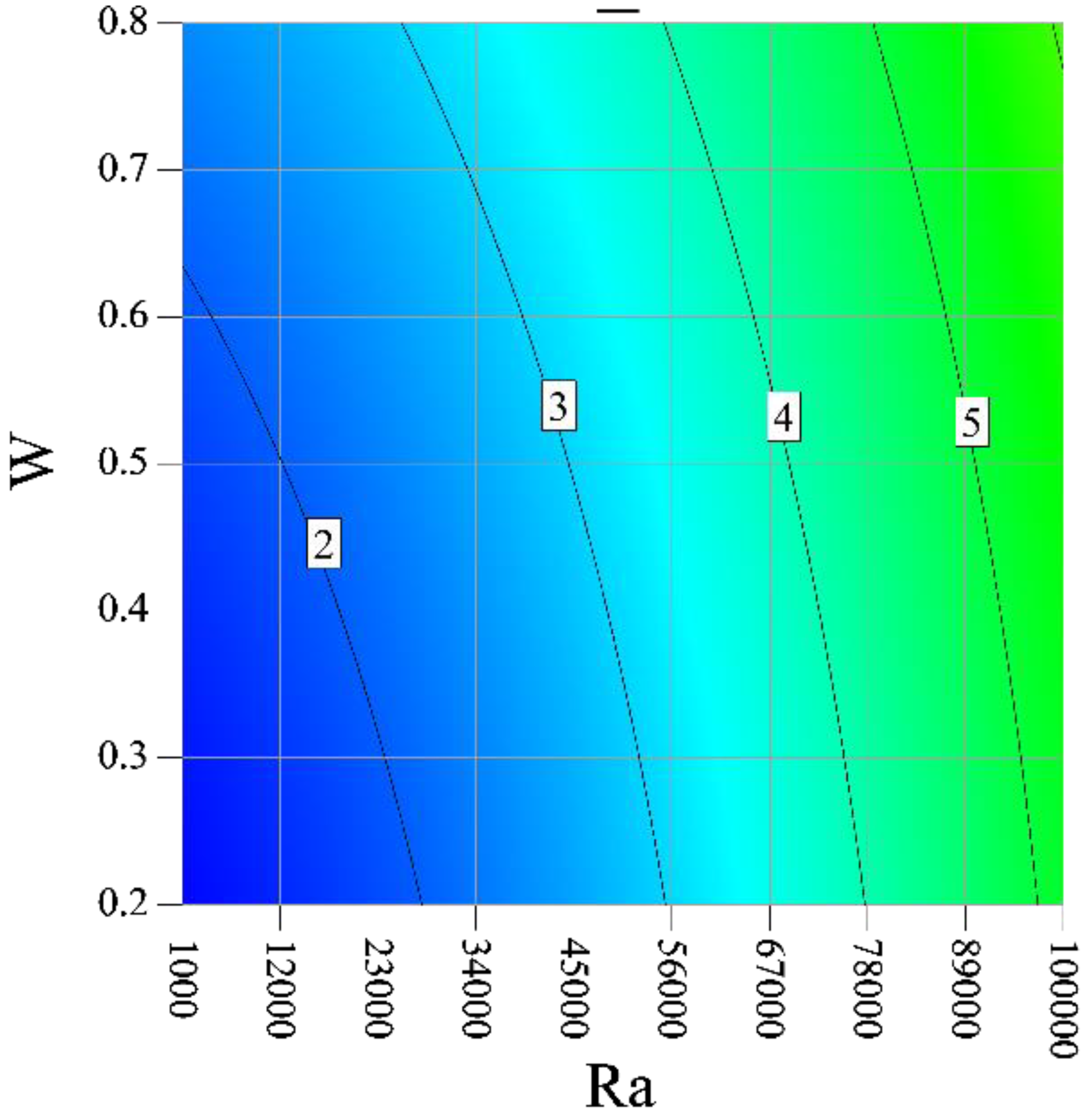
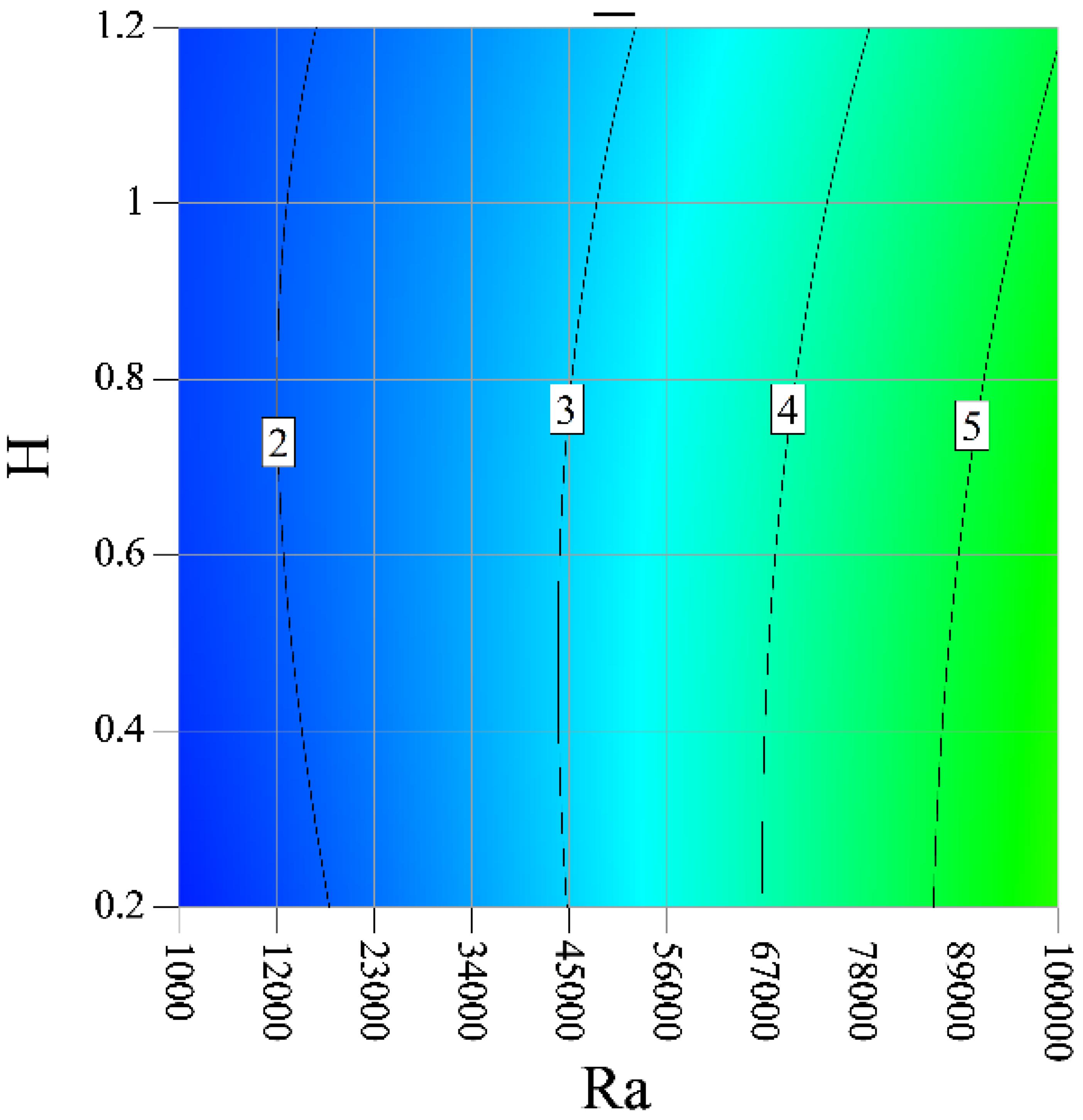
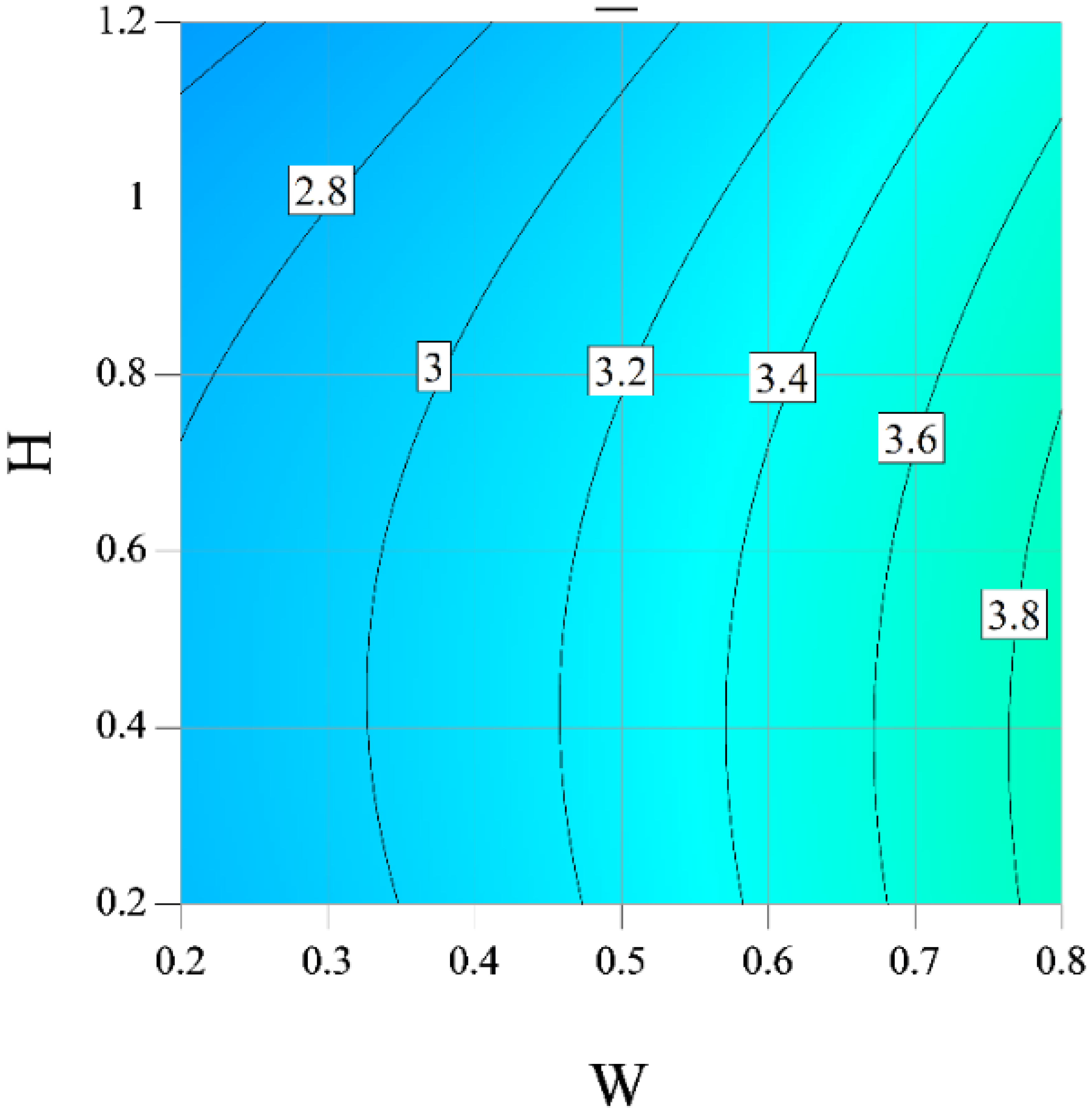
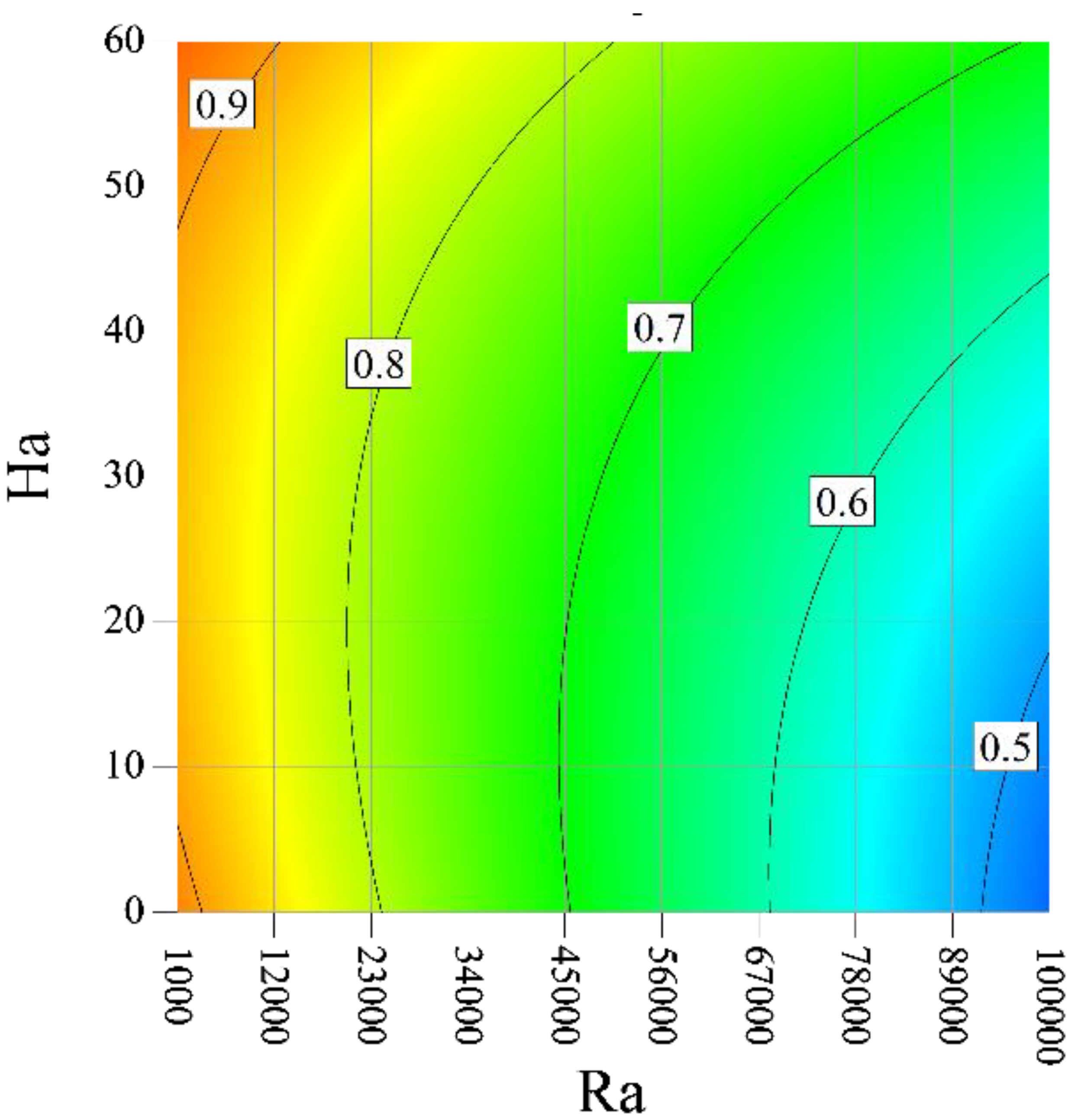
3. Results and Discussion
4. Conclusions
- The addition of silver eco-friendly N-Ps to a mixture of water and E-G enhances the amount of EGN, so that adding 0.3% of N-F enhances the amount of EGN by 3.8%;
- An increment in the Ra enhances ENG and reduces the Be. The ENG has a decreasing trend with the Ha;
- An enhancement in the blade height causes the amount of EGN to enhance. An increment in the blade height results in a reduction in the Be at the low Ra, but the Be is first enhanced and reduced at high Ra;
- The increase of Ha at high Ra leads to an increase in the Be, but at low Ras, the Be first has a decreasing trend and then an increasing trend;
- Enhancing the fin length in strong convections decreases the ENG. An increment in the fin length results in a reduction in the Be.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| B0 | Magnetic Field Strength |
| Be | Bejan number |
| Cp | |
| g | Gravitational acceleration [m/s2] |
| h | |
| H | Obstacle non-dimensional length |
| Ha | Hartmann number |
| k | |
| l | Enclosure length [m] |
| L | Enclosure non-dimensional length |
| Nusselt number | |
| Non-dimensional | |
| P | |
| Pr | |
| Ra | entropy generation [W/K] |
| S_T | Temperature [K] |
| T | |
| U,V | Obstacle non-dimensional height |
| W | |
| X,Y | |
| Greek Symbols | |
| Thermal expansion (1/K) | |
| σ | Electrical conductivity |
| α | Thermal diffusivity |
| φ | Solid volume fraction |
| Λ | Irreversibility distribution ratio |
| θ | Temperature |
| μ | Dynamic viscosity |
| ρ | Density |
| Subscripts | |
| f | Pure fluid |
| g | Generation |
| nf | Nanofluid |
| m | Average |
| p | Nanoparticle |
Appendix A
| Grid | S_T |
|---|---|
| 120 × 360 | 8.98 |
| 130 × 390 | 8.68 |
| 140 × 420 | 8.43 |
| 150 × 450 | 8.33 |
| 160 × 480 | 8.33 |

References
- Li, X.; Feng, Y.; Liu, B.; Yi, D.; Yang, X.; Zhang, W.; Chen, G.; Liu, Y.; Bai, P. Influence of NbC particles on microstructure and mechanical properties of AlCoCrFeNi high-entropy alloy coatings prepared by laser cladding. J. Alloys Compd. 2019, 788, 485–494. [Google Scholar] [CrossRef]
- Wang, H.; Wang, M.; Tang, Y. A novel zinc-ion hybrid supercapacitor for long-life and low-cost energy storage applications. Energy Storage Mater. 2018, 13, 1–7. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, Y.; Zhang, F.; Lee, C.-S. A Novel Aluminum–Graphite Dual-Ion Battery. Adv. Energy Mater. 2016, 6, 1502588. [Google Scholar] [CrossRef] [Green Version]
- Fan, Z.; Ji, P.-p.; Zhang, J.; Segets, D.; Chen, D.-R.; Chen, S.-C. Wavelet neural network modeling for the retention efficiency of sub-15 nm nanoparticles in ultrafiltration under small particle to pore diameter ratio. J. Membr. Sci. 2021, 635, 119503. [Google Scholar] [CrossRef]
- Cui, X.; Li, C.; Ding, W.; Chen, Y.; Mao, C.; Xu, X.; Liu, B.; Wang, D.; Li, H.N.; Zhang, Y.; et al. Minimum quantity lubrication machining of aeronautical materials using carbon group nanolubricant: From mechanisms to application. Chin. J. Aeronaut. 2021. [Google Scholar] [CrossRef]
- Yan, S.-R.; Aghakhani, S.; Karimipour, A. Influence of a membrane on nanofluid heat transfer and irreversibilities inside a cavity with two constant-temperature semicircular sources on the lower wall: Applicable to solar collectors. Phys. Scr. 2020, 95, 085702. [Google Scholar] [CrossRef]
- Eshgarf, H.; Kalbasi, R.; Maleki, A.; Shadloo, M.S.; Karimipour, A. A review on the properties, preparation, models and stability of hybrid nanofluids to optimize energy consumption. J. Therm. Anal. Calorim. 2021, 144, 1959–1983. [Google Scholar] [CrossRef]
- Ghalandari, M.; Maleki, A.; Haghighi, A.; Shadloo, M.S.; Nazari, M.A.; Tlili, I. Applications of nanofluids containing carbon nanotubes in solar energy systems: A review. J. Mol. Liq. 2020, 313, 113476. [Google Scholar] [CrossRef]
- Komeilibirjandi, A.; Raffiee, A.H.; Maleki, A.; Nazari, M.A.; Shadloo, M.S. Thermal conductivity prediction of nanofluids containing CuO nanoparticles by using correlation and artificial neural network. J. Therm. Anal. Calorim. 2020, 139, 2679–2689. [Google Scholar] [CrossRef]
- Wang, N.; Maleki, A.; Nazari, M.A.; Tlili, I.; Shadloo, M.S. Thermal Conductivity Modeling of Nanofluids Contain MgO Particles by Employing Different Approaches. Symmetry 2020, 12, 206. [Google Scholar] [CrossRef] [Green Version]
- Afrand, M.; Pordanjani, A.H.; Aghakhani, S.; Oztop, H.F.; Abu-Hamdeh, N. Free convection and entropy generation of a nanofluid in a tilted triangular cavity exposed to a magnetic field with sinusoidal wall temperature distribution considering radiation effects. Int. Commun. Heat Mass Transf. 2020, 112, 104507. [Google Scholar] [CrossRef]
- Shahsavani, E.; Afrand, M.; Kalbasi, R. Using experimental data to estimate the heat transfer and pressure drop of non-Newtonian nanofluid flow through a circular tube: Applicable for use in heat exchangers. Appl. Therm. Eng. 2018, 129, 1573–1581. [Google Scholar] [CrossRef]
- Ghodsinezhad, H.; Sharifpur, M.; Meyer, J.P. Experimental investigation on cavity flow natural convection of Al2O3—water nanofluids. Int. Commun. Heat Mass Transf. 2016, 76, 316–324. [Google Scholar] [CrossRef]
- Sharifpur, M.; Solomon, A.B.; Ottermann, T.L.; Meyer, J.P. Optimum concentration of nanofluids for heat transfer enhancement under cavity flow natural convection with TiO2–Water. Int. Commun. Heat Mass Transf. 2018, 98, 297–303. [Google Scholar] [CrossRef]
- Solomon, A.B.; van Rooyen, J.; Rencken, M.; Sharifpur, M.; Meyer, J.P. Experimental study on the influence of the aspect ratio of square cavity on natural convection heat transfer with Al2O3/Water nanofluids. Int. Commun. Heat Mass Transf. 2017, 88, 254–261. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S. Natural convection heat transfer in an inclined enclosure filled with a water-CuO nanofluid. Numer. Heat Transf. Part A Appl. 2009, 55, 807–823. [Google Scholar] [CrossRef]
- Cheng, F.; Betts, J.W.; Kelly, S.M.; Schaller, J.; Heinze, T. Synthesis and antibacterial effects of aqueous colloidal solutions of silver nanoparticles using aminocellulose as a combined reducing and capping reagent. Green Chem. 2013, 15, 989–998. [Google Scholar] [CrossRef]
- Mashayekhi, R.; Khodabandeh, E.; Bahiraei, M.; Bahrami, L.; Toghraie, D.; Akbari, O.A. Application of a novel conical strip insert to improve the efficacy of water–Ag nanofluid for utilization in thermal systems: A two-phase simulation. Energy Convers. Manag. 2017, 151, 573–586. [Google Scholar] [CrossRef]
- Essa, F.A.; Elsheikh, A.H.; Algazzar, A.A.; Sathyamurthy, R.; Ali, M.K.A.; Elaziz, M.A.; Salman, K.H. Eco-friendly coffee-based colloid for performance augmentation of solar stills. Process. Saf. Environ. Prot. 2020, 136, 259–267. [Google Scholar] [CrossRef]
- Kosinska, A.; Balakin, B.V.; Kosinski, P. Use of biodegradable colloids and carbon black nanofluids for solar energy applications. AIP Adv. 2021, 11, 055214. [Google Scholar] [CrossRef]
- Kalimuthu, K.; Cha, B.S.; Kim, S.; Park, K.S. Eco-friendly synthesis and biomedical applications of gold nanoparticles: A review. Microchem. J. 2020, 152, 104296. [Google Scholar] [CrossRef]
- Aghakhani, S.; Ghasemi, B.; Pordanjani, A.H.; Wongwises, S.; Afrand, M. Effect of replacing nanofluid instead of water on heat transfer in a channel with extended surfaces under a magnetic field. Int. J. Numer. Methods Heat Fluid Flow 2019, 24, 1249–1271. [Google Scholar] [CrossRef]
- Afrand, M.; Farahat, S.; Nezhad, A.H.; Sheikhzadeh, G.A.; Sarhaddi, F. Numerical Simulation of Electrically Conducting Fluid Flow And Free Convective Heat Transfer In An Annulus On Applying A Magnetic Field. Heat Transf. Res. 2014, 45, 749–766. [Google Scholar] [CrossRef]
- Afrand, M.; Farahat, S.; Nezhad, A.H.; Sheikhzadeh, G.A.; Sarhaddi, F. 3-D numerical investigation of natural convection in a tilted cylindrical annulus containing molten potassium and controlling it using various magnetic fields. Int. J. Appl. Electromagn. Mech. 2014, 46, 809–821. [Google Scholar] [CrossRef]
- Tian, M.-W.; Rostami, S.; Aghakhani, S.; Goldanlou, A.S.; Qi, C. A techno-economic investigation of 2D and 3D configurations of fins and their effects on heat sink efficiency of MHD hybrid nanofluid with slip and non-slip flow. Int. J. Mech. Sci. 2021, 189, 105975. [Google Scholar] [CrossRef]
- Giwa, S.O.; Sharifpur, M.; Meyer, J.P. Effects of uniform magnetic induction on heat transfer performance of aqueous hybrid ferrofluid in a rectangular cavity. Appl. Therm. Eng. 2020, 170, 115004. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Hayat, T.; Muhammad, T.; Alsaedi, A. MHD forced convection flow of nanofluid in a porous cavity with hot elliptic obstacle by means of Lattice Boltzmann method. Int. J. Mech. Sci. 2018, 135, 532–540. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Mejri, I.; Abbassi, M.A.; Omri, A. Lattice Boltzmann simulation of MHD natural convection in a nanofluid-filled cavity with linear temperature distribution. Powder Technol. 2014, 256, 257–271. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S.; Raisi, A. Magnetic field effect on natural convection in a nanofluid-filled square enclosure. Int. J. Therm. Sci. 2011, 50, 1748–1756. [Google Scholar] [CrossRef]
- Hu, Y.; Qing, J.x.; Liu, Z.H.; Conrad, Z.J.; Cao, J.N.; Zhang, X.P. Hovering efficiency optimization of the ducted propeller with weight penalty taken into account. Aerosp. Sci. Technol. 2021, 117, 106937. [Google Scholar] [CrossRef]
- Li, X.; Dong, Z.-Q.; Yu, P.; Wang, L.-P.; Niu, X.-D.; Yamaguchi, H.; Li, D.-C. Effect of self-assembly on fluorescence in magnetic multiphase flows and its application on the novel detection for COVID-19. Phys. Fluids 2021, 33, 042004. [Google Scholar] [CrossRef]
- Jiang, C.; Xiang, L.; Miao, S.; Shi, L.; Xie, D.; Yan, J.; Zheng, Z.; Zhang, X.; Tang, Y. Flexible Interface Design for Stress Regulation of a Silicon Anode toward Highly Stable Dual-Ion Batteries. Adv. Mater. 2020, 32, 1908470. [Google Scholar] [CrossRef]
- Lu, Z.-Q.; Zhao, L.; Ding, H.; Chen, L.-Q. A dual-functional metamaterial for integrated vibration isolation and energy harvesting. J. Sound Vib. 2021, 509, 116251. [Google Scholar] [CrossRef]
- Pordanjani, A.H.; Aghakhani, S.; Afrand, M.; Sharifpur, M.; Meyer, J.P.; Xu, H.; Ali, H.M.; Karimi, N.; Cheraghian, G. Nanofluids: Physical phenomena, applications in thermal systems and the environment effects—A critical review. J. Clean. Prod. 2021, 320, 128573. [Google Scholar] [CrossRef]
- Dutta, S.; Biswas, A.K.; Pati, S. Natural convection heat transfer and entropy generation inside porous quadrantal enclosure with nonisothermal heating at the bottom wall. Numer. Heat Transf. Part A Appl. 2018, 73, 222–240. [Google Scholar] [CrossRef]
- Kefayati, G.R.; Tang, H. Double-diffusive laminar natural convection and entropy generation of Carreau fluid in a heated enclosure with an inner circular cold cylinder (Part II: Entropy generation). Int. J. Heat Mass Transf. 2018, 120, 683–713. [Google Scholar] [CrossRef]
- Sadeghi, M.S.; Dogonchi, A.S.; Ghodrat, M.; Chamkha, A.J.; Alhumade, H.; Karimi, N. Natural convection of CuO-water nanofluid in a conventional oil/water separator cavity: Application to combined-cycle power plants. J. Taiwan Inst. Chem. Eng. 2021, 124, 307–319. [Google Scholar] [CrossRef]
- Shaw, S.; Nayak, M.K.; Dogonchi, A.S.; Chamkha, A.J.; Elmasry, Y.; Alsulami, R. Hydrothermal and entropy generation analyses of magneto-cross nanoliquid under rectified Fourier viewpoint: A robust approach to industrial applications. Case Stud. Therm. Eng. 2021, 26, 100974. [Google Scholar] [CrossRef]
- Salari, M.; Rezvani, A.; Mohammadtabar, A.; Mohammadtabar, M. Numerical Study of Entropy Generation for Natural Convection in Rectangular Cavity with Circular Corners. Heat Transf. Eng. 2015, 36, 186–199. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Entropy generation of nanofluid in presence of magnetic field using Lattice Boltzmann Method. Phys. A Stat. Mech. Its Appl. 2015, 417, 273–286. [Google Scholar] [CrossRef]
- Shojaeian, M.; Koşar, A. Convective heat transfer and entropy generation analysis on Newtonian and non-Newtonian fluid flows between parallel-plates under slip boundary conditions. Int. J. Heat Mass Transf. 2014, 70, 664–673. [Google Scholar] [CrossRef]
- Mahian, O.; Mahmud, S.; Wongwises, S. Entropy generation between two rotating cylinders with magnetohydrodynamic flow using nanofluids. Thermophys. Heat Transf. 2013, 27, 161–169. [Google Scholar] [CrossRef]
- Pordanjani, A.H.; Aghakhani, S. Numerical Investigation of Natural Convection and Irreversibilities between Two Inclined Concentric Cylinders in Presence of Uniform Magnetic Field and Radiation. Heat Transf. Eng. 2021, 1–21. [Google Scholar] [CrossRef]
- Aghakhani, S.; Pordanjani, A.H.; Afrand, M.; Sharifpur, M.; Meyer, J.P. Natural convective heat transfer and entropy generation of alumina/water nanofluid in a tilted enclosure with an elliptic constant temperature: Applying magnetic field and radiation effects. Int. J. Mech. Sci. 2020, 174, 105470. [Google Scholar] [CrossRef]
- Khodadadi, H.; Aghakhani, S.; Majd, H.; Kalbasi, R.; Wongwises, S.; Afrand, M. A comprehensive review on rheological behavior of mono and hybrid nanofluids: Effective parameters and predictive correlations. Int. J. Heat Mass Transf. 2018, 127, 997–1012. [Google Scholar] [CrossRef]
- Pak, B.C.; Cho, Y.I. Hydrodynamic and heat transfer study of dispersed fluids with submicron metallic oxide particles. Exp. Heat Transf. 1998, 11, 151–170. [Google Scholar] [CrossRef]
- Bhanvase, B.A.; Sarode, M.R.; Putterwar, L.A.; Abdullah, A.K.; Deosarkar, M.P.; Sonawane, S.H. Intensification of convective heat transfer in water/ethylene glycol based nanofluids containing TiO2 nanoparticles. Chem. Eng. Process. Process. Intensif. 2014, 82, 123–131. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Hormozi, F. Intensification of forced convection heat transfer using biological nanofluid in a double-pipe heat exchanger. Exp. Therm. Fluid Sci. 2015, 66, 279–289. [Google Scholar] [CrossRef]
- Natsuki, J.; Natsuki, T.; Hashimoto, Y. A review of silver nanoparticles: Synthesis methods, properties and applications. Int. J. Mater. Sci. Appl. 2015, 4, 325–332. [Google Scholar] [CrossRef]
- Natsuki, T.; Natsuki, J. One-step synthesis of silver nanoparticles using low molecular weight compounds at room temperature. Int. J. Mater. Eng. Technol. 2015, 13, 109. [Google Scholar] [CrossRef]
- Żyła, G.; Fal, J. Viscosity, thermal and electrical conductivity of silicon dioxide–ethylene glycol transparent nanofluids: An experimental studies. Thermochim. Acta 2017, 650, 106–117. [Google Scholar] [CrossRef]
- Cheraghian, G. Improved heavy oil recovery by nanofluid surfactant flooding-an experimental study. In Proceedings of the 78th EAGE Conference and Exhibition 2016, Vienna, Austria, 30 May–2 June 2016. [Google Scholar] [CrossRef]
- Cheraghian, G.; Nezhad, S.S.K.; Bazgir, S. Improvement of thermal stability of polyacryl amide solution used as a nano-fluid in enhanced oil recovery process by nanoclay. Int. J. Nanosci. Nanotechnol. 2015, 11, 201–208. [Google Scholar]
- Pirmohammadi, M.; Ghassemi, M. Effect of magnetic field on convection heat transfer inside a tilted square enclosure. Int. Commun. Heat Mass Transf. 2009, 36, 776–780. [Google Scholar] [CrossRef]




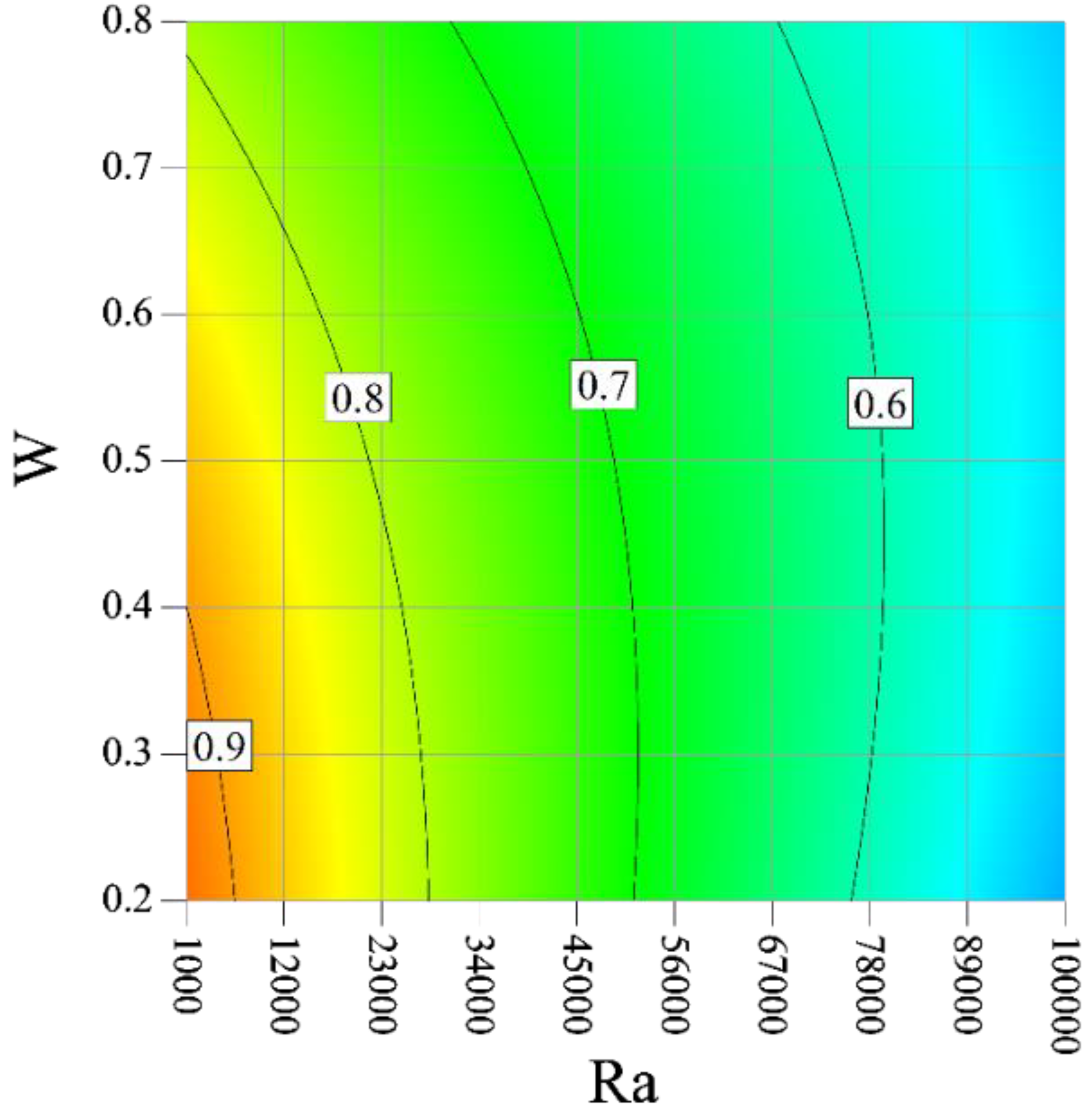
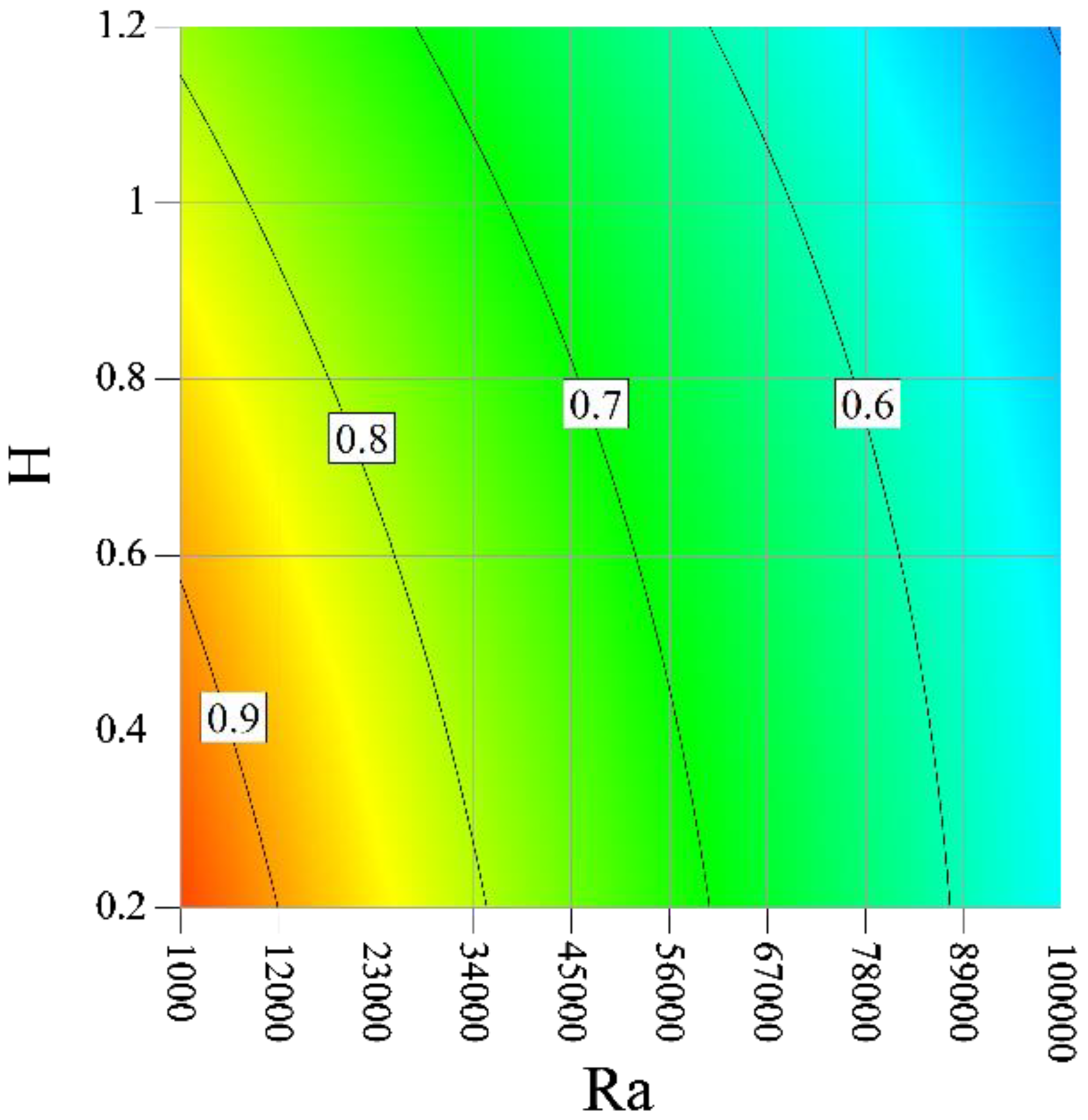
| 9 | ||||||
|---|---|---|---|---|---|---|
| E-G | 2430.8 | 0.2532 | 1088 | 0.0141 | 9.2 × 10−5 | - |
| Water | 4179 | 0.613 | 997.1 | 0.001 | 0.05 | - |
| Ag | 235 | 429 | 10,500 | - | 1.6 × 10−4 | 40 |
| S_T | ||
|---|---|---|
| 0 | 8.02 | 0 |
| 0.1 | 8.02 | 1.7 |
| 0.2 | 8.02 | 2.8 |
| 0.3 | 8.02 | 3.8 |
| 0.4 | 8.02 | 4.4 |
| 0.5 | 8.02 | 6.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khetib, Y.; Abo-Dief, H.M.; Alanazi, A.K.; Cheraghian, G.; Sajadi, S.M.; Sharifpur, M. Correlations for Total Entropy Generation and Bejan Number for Free Convective Heat Transfer of an Eco-Friendly Nanofluid in a Rectangular Enclosure under Uniform Magnetic Field. Processes 2021, 9, 1930. https://doi.org/10.3390/pr9111930
Khetib Y, Abo-Dief HM, Alanazi AK, Cheraghian G, Sajadi SM, Sharifpur M. Correlations for Total Entropy Generation and Bejan Number for Free Convective Heat Transfer of an Eco-Friendly Nanofluid in a Rectangular Enclosure under Uniform Magnetic Field. Processes. 2021; 9(11):1930. https://doi.org/10.3390/pr9111930
Chicago/Turabian StyleKhetib, Yacine, Hala M. Abo-Dief, Abdullah K. Alanazi, Goshtasp Cheraghian, S. Mohammad Sajadi, and Mohsen Sharifpur. 2021. "Correlations for Total Entropy Generation and Bejan Number for Free Convective Heat Transfer of an Eco-Friendly Nanofluid in a Rectangular Enclosure under Uniform Magnetic Field" Processes 9, no. 11: 1930. https://doi.org/10.3390/pr9111930
APA StyleKhetib, Y., Abo-Dief, H. M., Alanazi, A. K., Cheraghian, G., Sajadi, S. M., & Sharifpur, M. (2021). Correlations for Total Entropy Generation and Bejan Number for Free Convective Heat Transfer of an Eco-Friendly Nanofluid in a Rectangular Enclosure under Uniform Magnetic Field. Processes, 9(11), 1930. https://doi.org/10.3390/pr9111930








