Modeling Transient Flow in CO2 Injection Wells by Considering the Phase Change
Abstract
:1. Introduction
2. Transient Method
2.1. Establishment of the Model
- Mass conservation
- Momentum conservation
- Energy conservation
2.2. Calculation of Key Parameters
2.2.1. Properties of CO2
2.2.2. Heat Transfer
2.2.3. Heat Transfer
2.3. Initial and Boundary Conditions
2.3.1. Initial Conditions
2.3.2. Boundary Conditions
2.4. Solution Method
3. Validation of the Model
4. Results and Discussion
4.1. Case Study—A Fracturing Well
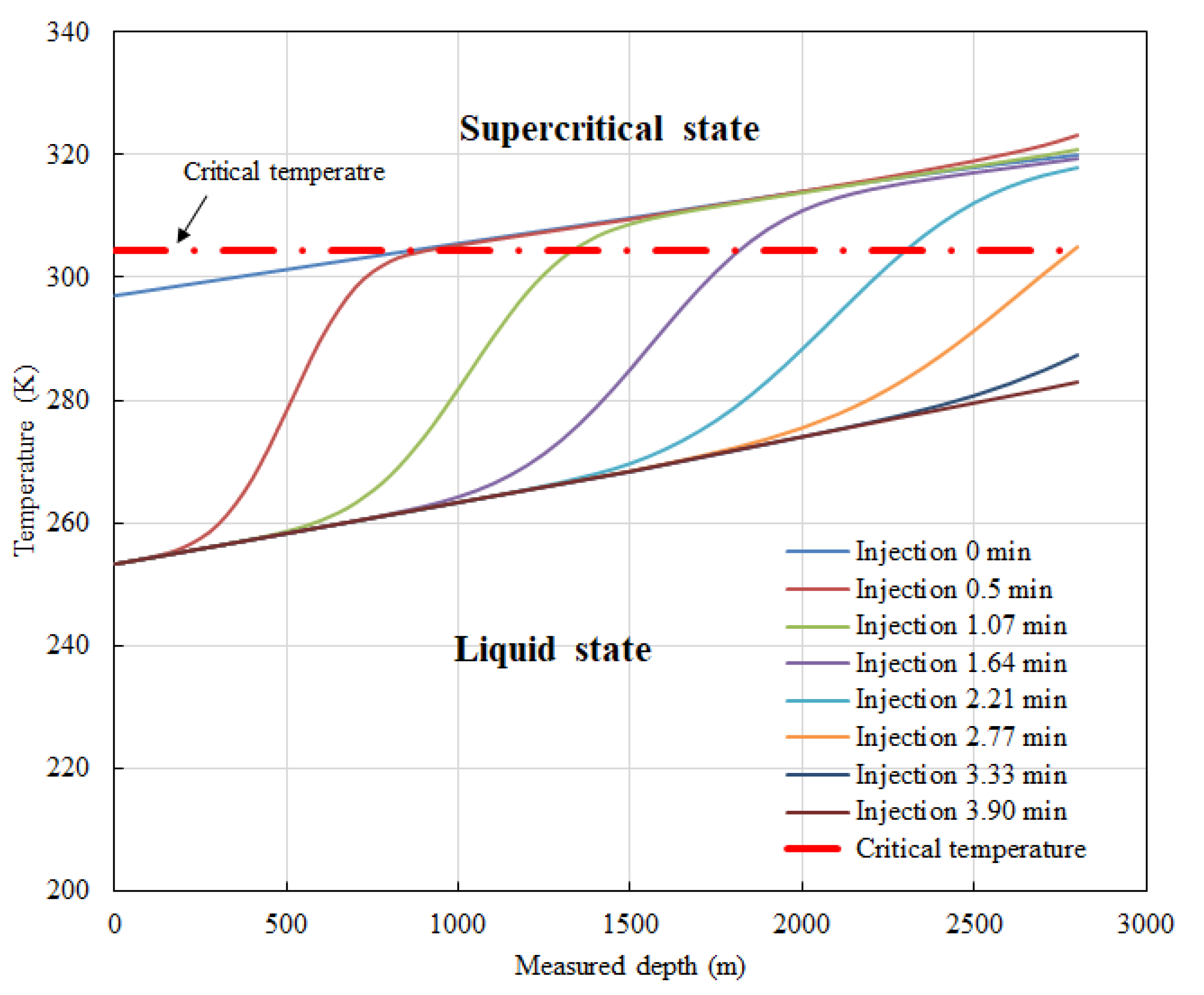
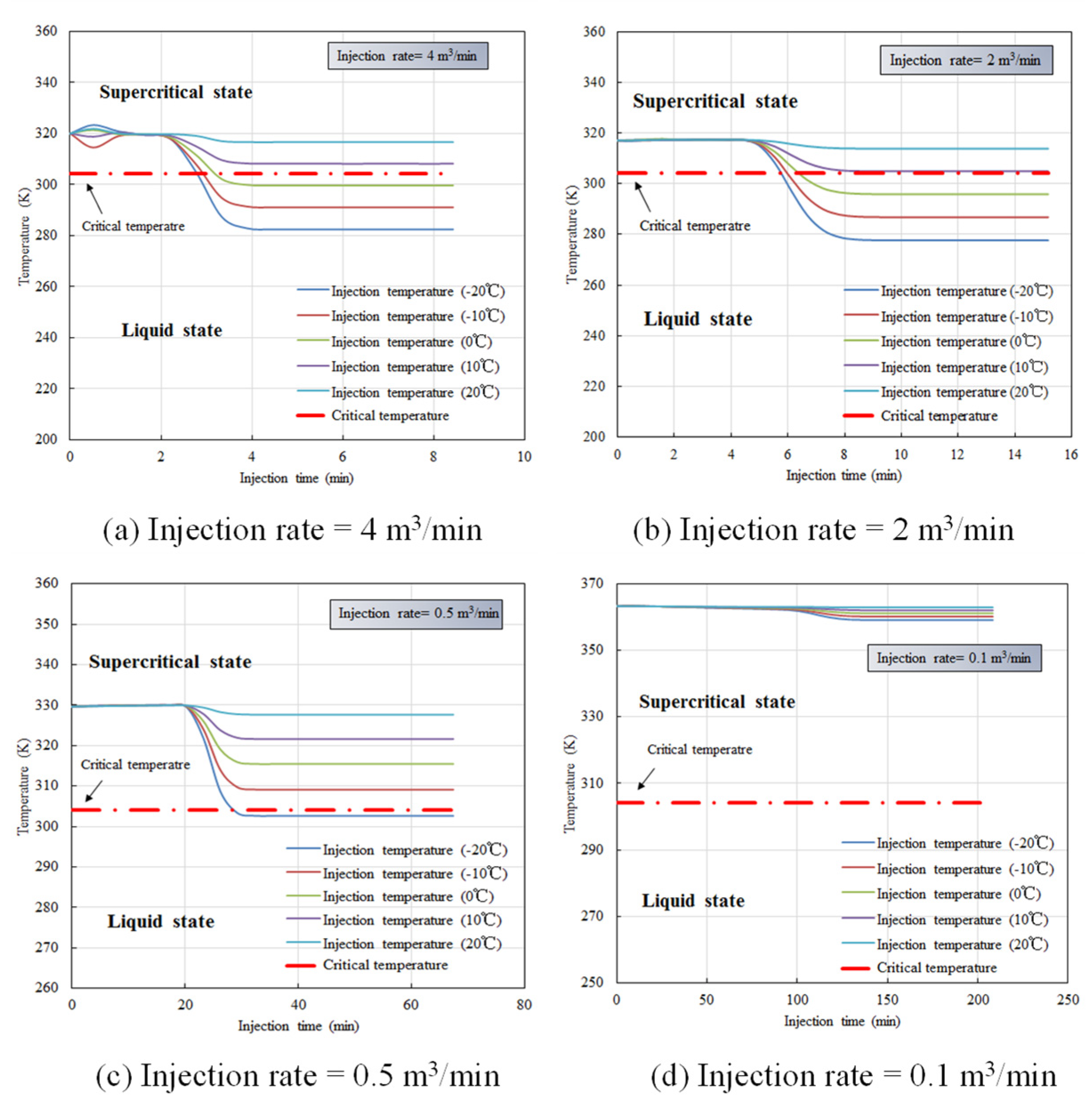
4.2. Control of the Phase State
4.3. The Impact of Density Variability on the Flow
4.3.1. Criteria of Flow Compressibility
4.3.2. Deviations between Incompressible and Compressible Flow
5. Conclusions and Suggestions
- A transient prediction model of CO2 injection wells was developed, which can simulate the temperature and pressure distributions by the finite difference method. The model was validated using field data.
- The phase state distribution was primarily determined by the wellbore temperature. The phase transition between the liquid and supercritical state may occur during the injection period.
- The supercritical state of CO2 can be achieved by reducing the injection rate or increasing the injection temperature. For fracturing wells with high injection rates, increasing the injection temperature is possible for the supercritical state. For CO2 EOR wells with small injection rates, the supercritical state is easily achieved by sufficient heat exchange with the formation.
- When the injection rate is small, the compressibility of CO2 can be ignored. However, if the injection rate is high, the variability of CO2 density cannot be neglected as it could lead to significant errors in pressure profiles.
- In fracturing wells, the CO2 is injected into the formation fractures at the well bottom. Therefore, the influence of the fractures on the CO2 flow should be studied in the future.
- In some cases, the CO2 may be injected with water to form CO2 foam. The behavior of the two-phase flow of CO2 and water in the wellbore warrants further exploration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of the Governing Equations
References
- Espie, A.A. A New Dawn for CO2 EOR. In Proceedings of the International Petroleum Technology Conference, Doha, Qatar, 21–23 November 2005. [Google Scholar]
- Gray, L.; Goodyear, S. Overcoming the CO2 Supply Challenge for CO2 EOR. In Proceedings of the Abu Dhabi International Petroleum Exhibition and Conference, Abu Dhabi, United Arab Emirates, 10–13 November 2014. [Google Scholar]
- Li, S.B.; Zhang, D.X. How Effective Is Carbon Dioxide as an Alternative Fracturing Fluid? SPE J. 2019, 24, 857–876. [Google Scholar] [CrossRef]
- Luk, S.; Apshkrum, M. Economic Optimization of Liquid CO2 Fracturing. In Proceedings of the Gas Technology Conference, Calgary, AB, Canada, 28 April 1998. [Google Scholar]
- Wang, J.T.; Sun, B.J.; Li, H.; Wang, X.; Wang, Z.Y.; Sun, X.H. Phase state control model of supercritical CO2 fracturing by temperature control. Int. J. Heat Mass Transf. 2018, 118, 1012–1021. [Google Scholar] [CrossRef]
- Meng, S.W.; Liu, H.; Xu, J.G.; Duan, T.W.; Yang, Q.H.; Yao, Z.X. The Evolution and Control of Fluid Phase During Liquid CO2 Fracturing. In Proceedings of the SPE Asia Pacific Hydraulic Fracturing Conference, Beijing, China, 24–26 August 2016. [Google Scholar]
- Li, X.J.; Li, G.S.; Sepehrnoori, K.; Wang, H.Z.; Liu, Q.L.; Zhang, H.Y.; Chen, Z.M. Estimation and Analysis of Carbon Dioxide Friction Loss in Wellbore during Liquid/Supercritical Carbon Dioxide Fracturing. SPE Prod. Oper. 2019, 34, 244–259. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Sun, B.J.; Wang, J.T.; Hou, L. Experimental study on the friction coefficient of supercritical carbon dioxide in pipes. Int. J. Greenh. Gas Control 2014, 25, 151–161. [Google Scholar] [CrossRef]
- Hasan, A.R.; Kabir, C.S. Wellbore heat-transfer modeling and applications. J. Pet. Sci. Eng. 2012, 86–87, 127–136. [Google Scholar] [CrossRef]
- Hasan, A.R.; Kabir, C.S.; Wang, X. A robust steady-state model for flowing-fluid temperature in complex wells. SPE Prod. Oper. 2009, 24, 269–276. [Google Scholar] [CrossRef]
- Raymond, L.R. Temperature Distribution in a Circulating Drilling Fluid. J. Pet. Technol. 1969, 21, 333–341. [Google Scholar] [CrossRef]
- Eickmeier, J.R.; Ersoy, D.; Ramey, H.J. Wellbore Temperatures and Heat Losses during Production or Injection Operations. J. Can. Pet. Technol. 1970, 9, 115–121. [Google Scholar] [CrossRef]
- Haas, C.; Macherhammer, M.G.; Klopcic, N.; Trattner, A. Capabilities and Limitations of 3D-CFD Simulation of Anode Flow Fields of High-Pressure PEM Water Electrolysis. Processes 2021, 9, 968. [Google Scholar] [CrossRef]
- Cranshaw, M.B.; Bolling, J.D. A Numerical Model of the Non-Isothermal Flow of Carbon Dioxide in Wellbores. In Proceedings of the SPE California Regional Meeting, San Francisco, CA, USA, 24–26 March 1982. [Google Scholar]
- Zhang, Y.; Tang, R.X. CO2 wellbore pressure and temperature distribution. Offshore Oil 2007, 2, 59–64, 108. [Google Scholar]
- Yasunami, T. Numerical Temperature Prediction System in Injection Tubing, Bottom Hole and Reservoir Condition for Supercritical CO2 Injection into Deep Coal Seams. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 21–24 September 2008. [Google Scholar]
- Paterson, L.; Ennis-King, J.P.; Sharma, S. Observations of Thermal and Pressure Transients in Carbon Dioxide Wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 19–22 September 2010. [Google Scholar]
- Lu, M.; Connell, L.D. The transient behaviour of CO2 flow with phase transition in injection wells during geological storage-Application to a case study. J. Pet. Sci. Eng. 2014, 147, 7–18. [Google Scholar] [CrossRef]
- Sun, B.J.; Sun, X.H.; Wang, Z.Y.; Wang, J.T.; Liu, S.J.; Xia, Q.; Cai, D.J. Flow behavior analysis during supercritical CO2 drilling: Consideration of varying thermo-physical properties of CO2 in wellbore. J. China Univ. Pet. (Edit. Nat. Sci.) 2016, 40, 88–95. [Google Scholar]
- Song, W.Q.; Ni, H.J.; Wang, R.H.; Sun, B.J.; Shen, Z.H. Pressure transmission in the tubing of supercritical carbon dioxide fracturing. J. CO2 Util. 2017, 21, 467–472. [Google Scholar] [CrossRef]
- Yi, L.P.; Li, X.G.; Yang, Z.Z.; Sun, J. Coupled calculation model for transient temperature and pressure of carbon dioxide injection well. Int. J. Heat Mass Transf. 2018, 121, 680–690. [Google Scholar] [CrossRef]
- Guo, J.C.; Zeng, J. A coupling model for wellbore transient temperature and pressure of fracturing with supercritical carbon dioxide. Acta Pet. Sin. 2015, 36, 203–209. [Google Scholar]
- Gong, Q.; Xu, Z.G.; Wang, M.Q.; Qin, J. Numerical investigation on wellbore temperature and pressure during carbon dioxide fracturing. Appl. Therm. Eng. 2019, 157, 113675. [Google Scholar] [CrossRef]
- Wang, J.T.; Sun, B.J.; Chen, W.Q.; Xu, J.C.; Wang, Z.Y. Calculation model of unsteady temperature–pressure fields in wellbores and fractures of supercritical CO2 fracturing. Fuel 2019, 253, 1168–1183. [Google Scholar] [CrossRef]
- Lyu, X.R.; Zhang, S.C.; Ma, X.F.; Wang, F.; Mou, J.Y. Numerical study of non-isothermal flow and wellbore heat transfer characteristics in CO2 fracturing. Energy 2018, 156, 555–568. [Google Scholar] [CrossRef]
- Lyu, X.R.; Zhang, S.C.; Ma, X.F.; Wang, F.; Mou, J.Y. Numerical investigation of wellbore temperature and pressure fields in CO2 fracturing. Appl. Therm. Eng. 2018, 132, 760–768. [Google Scholar] [CrossRef]
- Li, X.G.; Yi, L.P.; Yang, Z.Z.; Chen, Y.T.; Sun, J. Coupling model for calculation of transient temperature and pressure during coiled tubing drilling with supercritical carbon dioxide. Int. J. Heat Mass Transf. 2018, 125, 400–412. [Google Scholar] [CrossRef]
- Chen, N.H. An Explicit Equation for Friction Factor in Pipe. Ind. Eng. Chem. Fundam. 1979, 18, 296–297. [Google Scholar] [CrossRef]
- Landau, L.D. Fluid Mechanics, 2nd ed.; Pergamon Press: Oxford, UK, 1987; pp. 17–21. [Google Scholar]


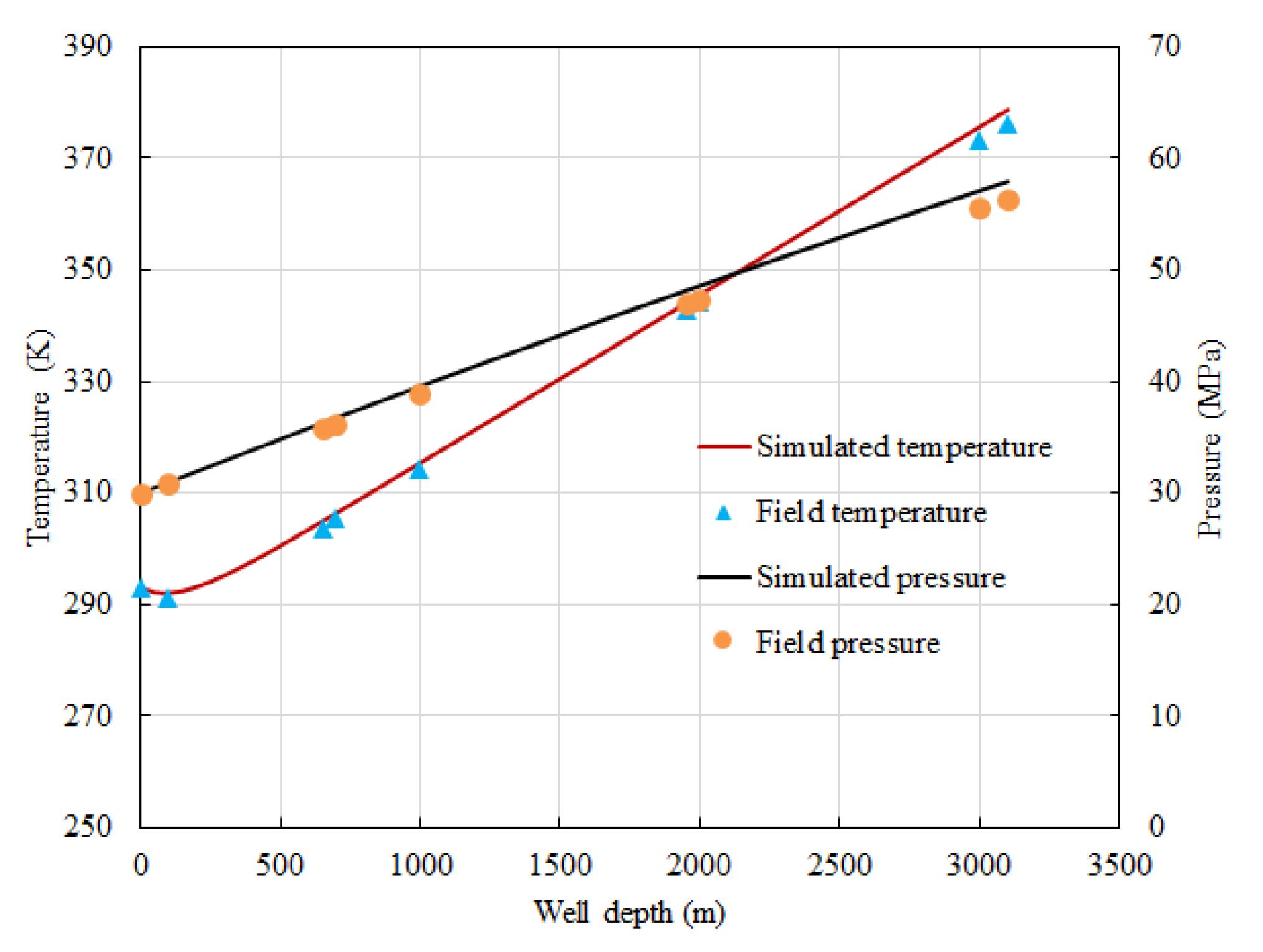


| Temperature | Pressure | ||||
|---|---|---|---|---|---|
| Measured | Simulated | Relative | Measured | Simulated | Relative |
| data (°C) | data (°C) | errors (%) | data (MPa) | data (MPa) | errors (%) |
| 101.00 | 101.90 | 0.89 | 52.02 | 52.51 | 0.94 |
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Measured depth (m) | 2800 | Wellbore diameter (mm) | 444 |
| Tubing external diameter (mm) | 89 | Tubing wall (mm) | 6.5 |
| Surface casing external diameter (mm) | 339.7 | Surface casing wall (mm) | 9.65 |
| Surface casing depth (m) | 63 | Cement outside surface casing | Surface |
| Technical casing external diameter (mm) | 244.47 | Technical casing wall (mm) | 10.03 |
| Technical casing depth (m) | 969 | Cement outside technical casing | Surface |
| Production casing external diameter (mm) | 139.7 | Production casing wall (mm) | 9.17 |
| Production casing depth (m) | 2775 | Cement outside production casing (m) | 1424 |
| Geothermal gradient (K/100m) | 2.95 | Surface temperature (K) | 24 |
| Injection rate (m3/min) | 4 | Injection pressure (MPa) | 80 |
| Injection temperature (K) | −20 | Injection time (hour) | 1 |
| String thermal conductivity (J/(m∙s∙K)) | 53 | Annular fluid thermal conductivity (J/(m∙s∙K)) | 0.557 |
| Cement thermal conductivity (J/(m∙s∙K)) | 0.627 | Formation thermal conductivity (J/(m∙s∙K)) | 1.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wan, N.-H.; Wang, L.-S.; Hou, L.-T.; Wu, Q.-L.; Xu, J.-Y. Modeling Transient Flow in CO2 Injection Wells by Considering the Phase Change. Processes 2021, 9, 2164. https://doi.org/10.3390/pr9122164
Wan N-H, Wang L-S, Hou L-T, Wu Q-L, Xu J-Y. Modeling Transient Flow in CO2 Injection Wells by Considering the Phase Change. Processes. 2021; 9(12):2164. https://doi.org/10.3390/pr9122164
Chicago/Turabian StyleWan, Nian-Hui, Li-Song Wang, Lin-Tong Hou, Qi-Lin Wu, and Jing-Yu Xu. 2021. "Modeling Transient Flow in CO2 Injection Wells by Considering the Phase Change" Processes 9, no. 12: 2164. https://doi.org/10.3390/pr9122164
APA StyleWan, N.-H., Wang, L.-S., Hou, L.-T., Wu, Q.-L., & Xu, J.-Y. (2021). Modeling Transient Flow in CO2 Injection Wells by Considering the Phase Change. Processes, 9(12), 2164. https://doi.org/10.3390/pr9122164






