Numerical Analysis of the Spontaneous Combustion Accidents of Oil Storage Tanks Containing Sulfur

Abstract

In order to study various influencing factors of spontaneous combustion accidents of sulfur-containing oil storage tanks, this paper constructed a two-dimensional model of the storage tank wall by COMSOL Multiphysics software. The proposed model takes temperature change trend as an observation index to explore the heat transfer process of the tank wall. By fitting the function curve of heat release with oxidation reaction temperature of sulfur corrosion products and comparing with constant heat sources, it is found that heat source intensity will affect the temperature growth trend of tank spontaneous combustion. In addition, the air convection heat transfer coefficient represents the interference degree of external environment to the spontaneous combustion heating process, and the wall heating rate decreases with the increase of air convection heat transfer coefficient. The different heat release rates in varying oxidation stages will lead to distinct temperature growth trends. The larger the air convection heat transfer coefficient, the greater the temperature difference between the inner and outer walls of the tank, which is not conducive to the detection of the abnormal temperature of the heat source. The difference of thermal insulation and thermal conductivity of tank materials also affects the wall heat transfer, so the material properties should be considered comprehensively in actual production. The research results can provide a research basis and a theoretical basis for monitoring, prevention, and control of spontaneous combustion of sour oil storage tanks.

1. Introduction

With the rapid development of the petroleum industry, the demand for crude oil processing is increasing, but at the same time, fire and explosion accidents caused by corrosion and spontaneous combustion of large sulfur-containing oil storage tanks have occurred from time to time, resulting in serious consequences. There have been many fire and explosion accidents in China National Petroleum Corporation due to self-heating of oil tank rust [1]. According to statistics, there were 83 fire and explosion accidents in oil tanks from 1962 to 2013, which caused 445 deaths, accompanied by huge property losses and serious damage to the ecological environment [2].

For storage tanks that store oil products for a long time, the rust and a small amount of hydrogen sulfide in the gas phase space will react to produce sulfur corrosion products, a mixture of rust, mackinawite (FeS), greigite (Fe₃S₄), and pyrite (FeS₂), which will cause corrosion to the wall surface [3]. Many scholars first studied the oxidation process and self-heating tendency of sulfur corrosion products [4,5,6], analyzed the oxidation kinetics of iron sulfide [7,8], then put their focus on the influences of various factors on the spontaneous combustion of sulfur-containing oil storage tanks. The effects of temperature, moisture, and oxygen volume fraction on the pyrophoricity were studied by Robert Walker et al. [9,10,11]. Asaki [12] proved that there are differences in the oxidation reaction of ferrous sulfide with different microscopic forms. Somot [13] proposed that hydrogen sulfide is easy to combust in the environment of ferric sulfide. Mellor [14] and Baltrus [15] showed that the necessary condition for the spontaneous combustion relative humidity. Furthermore, the influences of other factors on the oxidation of sulfurized rust also were investigated. Li [16] studied the influence of the atmosphere gas. Dou [17] showed that different water concentrations had different effects on the spontaneous combustion tendency of iron sulfide. The effect of heating rate on the pyrophoric risk of sulfide minerals was demonstrated by Iliyas [18] and Dou [19]. Man [20] found that the smaller the particle size of ferric sulfide, the easier it is to ignite. Zhao [21] studied the effects of flow rate of hydrogen sulfide, environmental temperature, and setting time on the quantities and types of iron sulfides. The effects of the existence of water, oil, and monocrystal sulfur were discussed by Li [22]. Mebarki [23] selected five governing parameters to assess the oxidation self-heating hazard of sulfurized rust, for particular ambient conditions in crude oil tanks, including water content, mass of sulfurized rust, operating temperature, air flow rate, and oxygen concentration in the respiratory or safety valve.

In summary, many scholars focused on the spontaneous combustion tendency of sulfur corrosion products when analyzing the influencing factors of tank spontaneous combustion. However, accidents of spontaneous combustion involve many other factors such as human, material, environment, and management [24], while few scholars have studied these and they have not paid attention to the change of temperature field of storage tank under various influencing factors.

In order to find out the influence of different factors on spontaneous combustion accidents and provide a theoretical basis for the prevention and monitoring, this paper studies the heat transfer process on the tank wall with temperature as the observation index. The variation of the storage tank wall temperature throughout the oxidation exothermic process is explored by fitting a number function equation for the variation of the sulfur corrosion product exotherm with temperature. In addition, by changing a variety of external factors, the trend of temperature change of the storage tank under different influencing factors is explored. Since the oxidation and spontaneous combustion process in the storage tank is a spatial heat transfer problem, the heat transfer problem is essentially a transient process, and it is difficult to obtain accurate temperature field distribution results by ordinary analysis methods. Therefore, this paper adopts the finite element thermal analysis method and uses COMSOL Multiphysics to conduct numerical simulation to investigate the change of temperature field on the wall of storage tanks.

2. Materials and Methods

Finite element thermal analysis was adopted for numerical simulation and a two-dimensional geometric model was used to construct the calculation. Parameters were set before calculation, including materials, governing equations, boundary conditions, and initial conditions.

2.1. Geometric Model and Material Parameters

2.1.1. Geometric Model

In general, it is difficult to monitor the temperature of oil and sulfur corrosion products from the outer wall of the storage tank due to the temperature barrier effect of the tank wall. Therefore, this study started from the inside to investigate the temperature change of the tank wall during the oxidation exothermic reaction of sulfur corrosion products. In order to facilitate the numerical calculation, the physical model was simplified to a two-dimensional plane model, and the cross section of the tank body closest to the corrosion product layer was taken as the research surface. The wall thickness of the tank was set to be 20 mm, and the sulfur corrosion product was attached to the inner wall with a thickness of 5 mm. The geometric model is shown in Figure 1.

2.1.2. Material Parameters

Sulfur corrosion products are mainly a mixture of rust, FeS, FeS₂, and Fe₃S₄. Anticorrosion materials are usually selected for the wall of storage tanks. At present, epoxy glass flake is mostly used in China. The related thermodynamic parameters are shown in

Table 1. Thermal analysis parameters of basic structural materials.

Materials	Density (kg·m−3)	Specific Heat Capacity (J·kg−1·°C−1)	Thermal Conductivity (W·m−1·°C−1)
Sulfur corrosion products	4860	650	0.5
Tank wall (epoxy glass flake)	3100	1581	0.2

[25,26].

2.2. Governing Equations

According to the theory of heat transfer, the differential equations of heat transfer process between the same object or different objects can be summarized [27,28,29]. Combined with the second law of thermodynamics, it can be known that the temperature difference is the reason of heat transfer from high temperature to low temperature, and the heat transfer modes are heat conduction, heat convection, and heat radiation, respectively. In this study, the heat transfer process of the tank wall was explored and modeled based on the following assumptions [27]:

• Heat transfer modes are heat conduction and heat convection, ignoring heat radiation.
• Air is a continuous medium.
• The physical parameters of air and solid substances are constant and do not change with temperature.
• Air is an incompressible fluid.
• Air is a Newtonian fluid, following the Newtonian formula.
• Ignoring heat dissipation caused by viscous dissipation.

2.2.1. Heat Conduction Energy Equation

Sulfur corrosion products are regarded as heat sources because of the exothermic heat of oxidation. In the case of an internal heat source, the heat transfer process on the tank wall can be essentially simplified to a two-dimensional space heat transfer process. Assuming that the wall of the storage tank is homogeneous and isotropic, the two-dimensional thermal conductivity differential equation is given by:

$$\lambda \left(\frac{{\partial }^{2}T}{\partial x^2}+\frac{{\partial }^{2}T}{\partial y^2}\right)+q_v=\rho c_p\frac{\partial T}{\partial \tau }\left(\tau >0\right)$$

(1)

where:

• λ—thermal conductivity (W·m⁻¹·°C⁻¹), constant;
• c_p—specific heat capacity of the tank wall (J·kg⁻¹·°C⁻¹);
• ρ—the surface density of the storage tank (kg·m⁻³);
• x, y—two-dimensional coordinate axis (-);
• T—the temperature of the tank wall (°C);
• τ—heat transfer time (s);
• q_v—the internal heat source (W·m⁻³).

2.2.2. Convective Heat Transfer Energy Equation

There is a convective heat exchange process between the tank wall contacting with the external environment and air, and the energy equation is given as follows:

$$\lambda \left(\frac{{\partial }^{2}T}{\partial x^2}+\frac{{\partial }^{2}T}{\partial y^2}\right)+q_v=\rho c_p\left(\frac{\partial T}{\partial \tau }+u\frac{\partial T}{\partial x}+v\frac{\partial T}{\partial y}\right)\left(\tau >0\right)$$

(2)

where:

• u—air velocity in x direction (m·s⁻¹);
• v—air velocity in y direction (m·s⁻¹).

2.3. Boundary Conditions and Initial Conditions

For the heat transfer process on the tank wall, the boundary conditions are as the following three types:

$$t_w=f\left(x,y,z,\tau \right)$$

(3)

$$-\lambda {\left(\frac{\partial t}{\partial n}\right)}_w=0$$

(4)

$$-\lambda {\left(\frac{\partial t}{\partial n}\right)}_w=h\left(t_w-t_f\right)$$

(5)

where:

• t_w—the temperature of the outer wall of the storage tank (°C);
• t_f—the air temperature (°C);
• ∂t/∂n—the rate of temperature change in the normal direction of an isothermal surface (-);
• h—air convection heat transfer coefficient (W·m⁻²·°C⁻¹).

The initial temperature of the inner wall was 20 °C, the outer wall was set as the third boundary condition, and the other walls were set as adiabatic.

2.4. Meshing

The two-dimensional structure was divided into structured grids for use in numerical simulation by the COMSOL Multiphysics software. The mesh size has a great influence on the accuracy of numerical simulation, so it is necessary to do a mesh independence test. Three meshes with different units of elements, 5086, 1542, and 752, were generated. Figure 2 shows the temperature of outer wall of the tank with the time under these three meshes. It can be found that the temperature curves are stable and have no significant difference with all three meshes. But with the increase of mesh units, the temperature curve becomes closer. Considering calculation time and accuracy, the mesh with 1542 units was more suitable, and it was chosen for the numerical simulation, which is shown in Figure 3. According to the grid quality results, the average element quality is 0.927, which meets the grid accuracy requirements. The specific parameters are shown in

Table 2. Grid information statistics.

Physical Quantities	Value
Mesh units	1542
Average element quality	0.927
Mesh nodes	822

.

3. Results and Discussion

The results were analyzed and discussed after the simulation operation. The effects of critical influencing factors affecting the heat transfer on the tank wall were discussed in detail. These factors include the intensity of the heat source, air convective heat transfer coefficients, and wall materials.

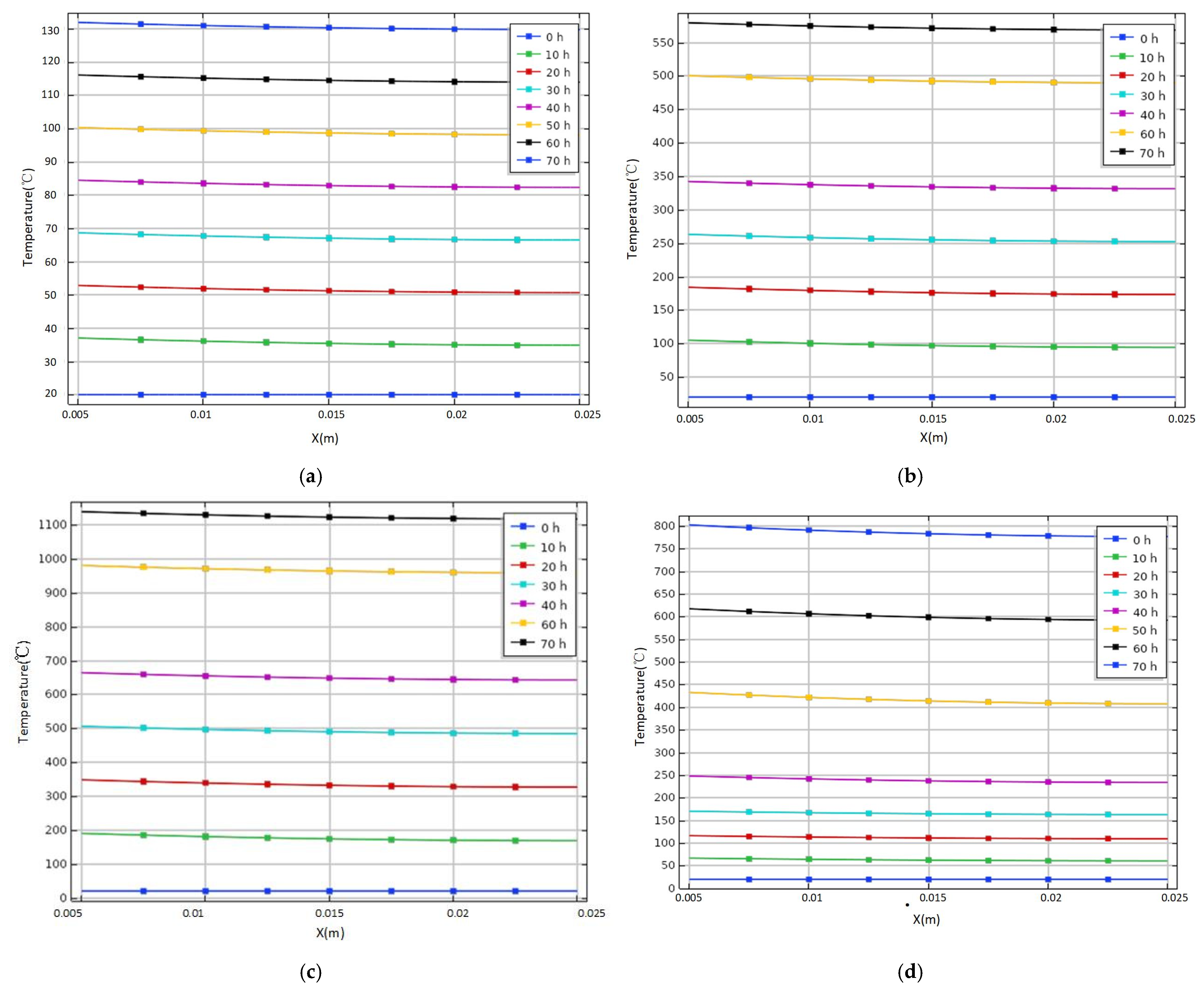
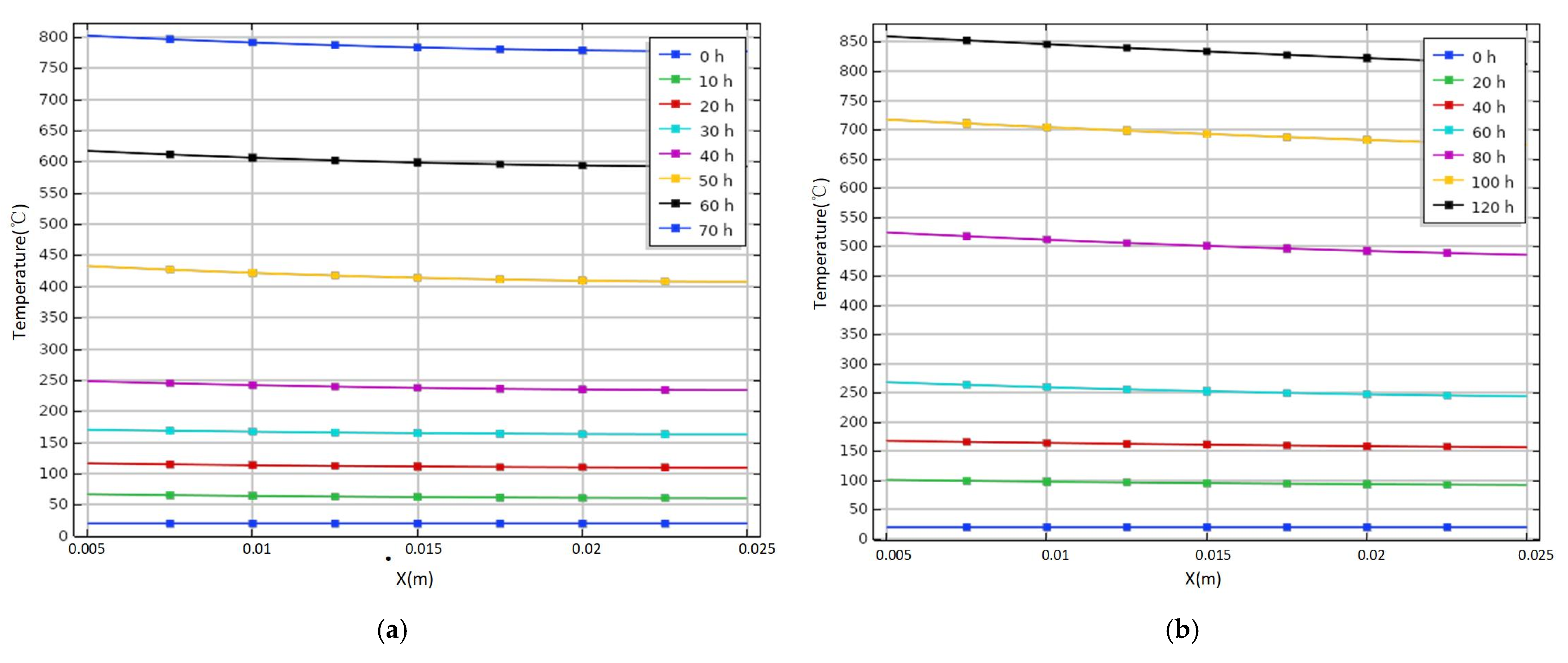
3.1. Variation of Temperature of Tank under Different Heat Source Intensity

Sulfur corrosion products contact with oxygen in the air and generate an oxidation exothermic reaction to release heat. Therefore, sulfur corrosion products attached to the inner wall surface were defined as a heat source, Q represents the amount of heat, and W represents the unit.

The sulfur corrosion products were used as a constant heat source firstly in order to clarify the influence of different heat release on the spontaneous combustion of the storage tank, and the exothermic heat Q was set to 1 W, 5 W, and 10 W, respectively, for simulation calculation. However, according to relevant research [30], the heat release of sulfur corrosion products is not constant in the actual heat release process, so it is necessary to define the function equation of heat source intensity in order to be more realistic. From the literature, it is found that the exothermic heat Q of sulfur corrosion products changes with the temperature T of the oxidation exothermic reaction [30]. Therefore, the Origin software was used to find out the function of the exothermic heat Q with the temperature T by importing experimental data for numerical fitting. For convenience of understanding, the heat release is denoted as Q₁, and the temperature of oxidation exothermic reaction is denoted as T. The self-heating process of sulfur corrosion products is divided into three stages. The first stage is the initial oxidation stage, and the temperature is between 0 °C and 400 °C. The function of the heat Q₁ changing with the temperature T of oxidation exothermic reaction can be described by

$$Q_1=2.16-2.03\times {10}^{-3}\times T+1.18\times {10}^{-5}\times T^2$$

(6)

The second stage is the intermediate oxidation stage, and the temperature is between 400 °C and 500 °C. The intensity of the oxidation exothermic reaction enhances gradually at this stage, and the heat release increases significantly. The function of the heat release Q₁ with the temperature T is given as follows:

$$Q_1=1.13\times {10}^{-15}{e}^{\frac{T}{14.15}}+3.3$$

(7)

The third stage is the deep oxidation stage, and the temperature is between 500 °C and 700 °C. The oxidation exothermic reaction is the most intense at this stage, and the heat release continues to increase. The function of the heat release Q₁ changing with the temperature T is expressed by

$$\begin{array}{c}{Q}_1=5966.13-26.80\times \left(T+130\right)+0.04\times {\left(T+130\right)}^2\\ +1.98\times {10}^{-5}\times {\left(T+130\right)}^3+5.5\end{array}$$

(8)

Numerical simulation was carried out by changing the intensity of the heat source in COMSOL Multiphysics, which makes statistics and analysis of the data after the operation. The analysis involved taking a section from the wall of the storage tank, observing the heat transfer process from sulfur corrosion products (heat source) to the outer wall of the storage tank, and recording the temperature rise within 70 h, as shown in Figure 4.

Figure 4 shows the difference of temperature rising trend between the constant heat source and the variable heat source. When Q is equal to 1 W, 5 W, and 10 W, the heating rate is uniform and the temperature span is the basically the same every 10 h. However, the temperature growth rate is significantly different when Q is equal to Q₁, and it rises slowly before 40 h, and increases rapidly every 10 h after 40 h. The analysis reason is that the oxidation exothermic reaction of sulfur corrosion products is not constant, and different oxidation exothermic stages lead to different temperature increasing trends. It can be judged that it is in the initial stage of oxidation exothermic reaction before 40 h. The internal temperature of the storage tank rises slowly at this stage, so it is easier to control the spontaneous combustion trend. After 40 h, the temperature rises rapidly, and it can be determined that it is in the accelerated period of oxidation exothermic reaction. In this period, the risk of spontaneous combustion of the storage tank is much higher than before. It can be seen from the simulation results that the difference of heating rate is related to the oxidation stage, which is consistent with the experimental results in the literature [26,31].

The temperature rising trend of the outer wall surface of the storage tank under different heat source intensities can be obtained by extracting the temperature data of the outer wall surface, as shown in Figure 5. It can be seen that there is an obvious inflection point on the curve when Q is equal to Q₁, which proves that the heat source intensity will affect the temperature field of inside tank due to the uneven change of oxidation process with time. It can be inferred that different heating rates in varying oxidation stages have distinct effects on the spontaneous combustion of the storage tank. In order to fit reality, Q₁ was used as the heat source intensity input in subsequent simulation settings.

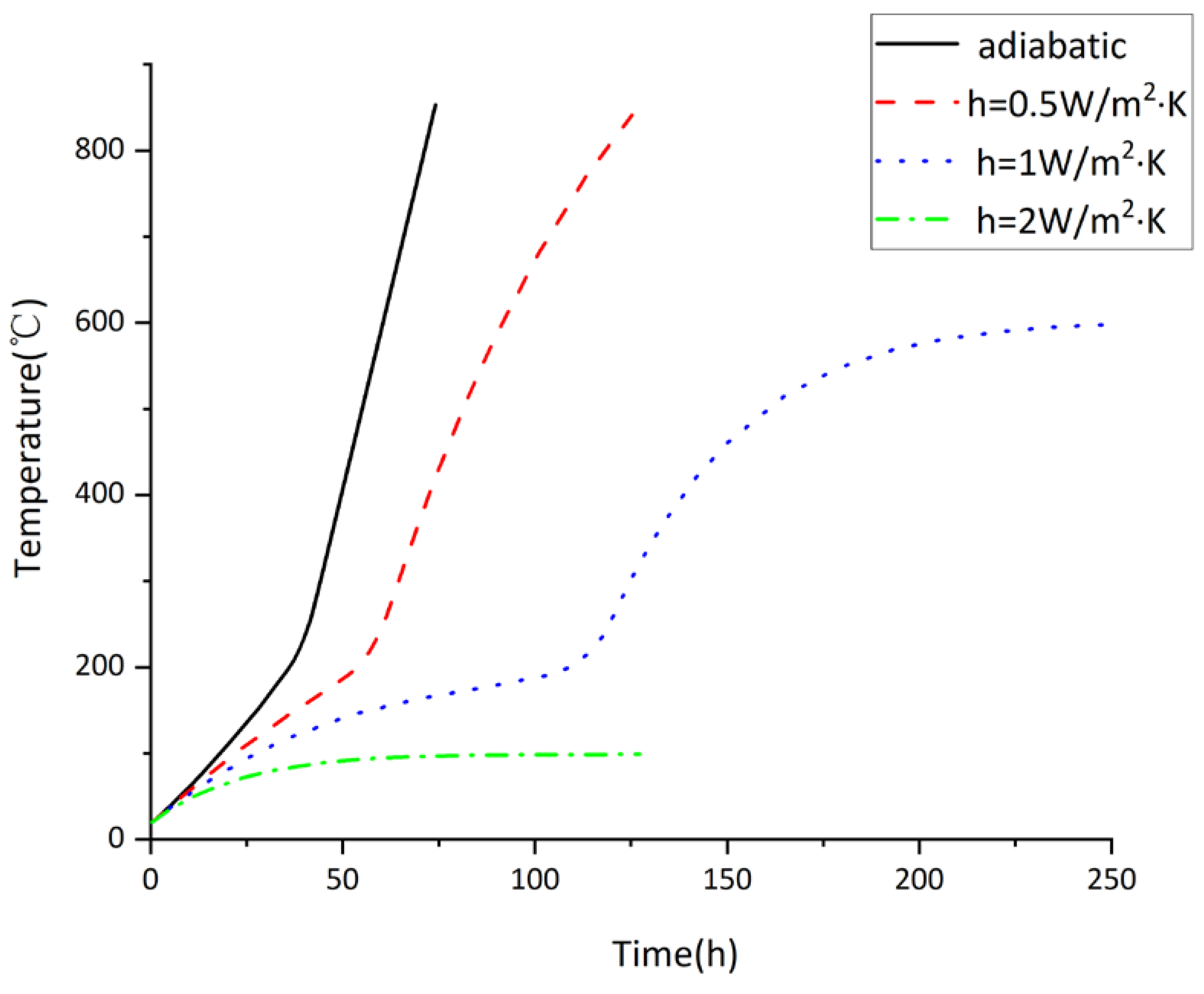
3.2. Variation of Temperature of Tank under Different Air Convective Heat Transfer Coefficients

The boundary condition was set as adiabatic, the temperature changes within 72 h were documented, and the tendency of temperature change of the tank wall was recorded every 10 h as shown in Figure 6a. Assuming that there is heat exchange between outside wall of the storage tank and the air, the heat transfer of the tank wall under different air convection heat transfer rates was explored by changing the air convection heat transfer coefficient h. The temperature increasing rate decreases when h is equal to 0.5 W·m⁻²·°C⁻¹, so analyzing the temperature changes within 120 h and the wall temperature changes every 20 h is required, as shown in Figure 6b. The temperature rising trend slows down further when h is 1 W·m⁻²·°C⁻¹, so the temperature changes within 240 h and the wall temperature changes once every 24 h, shown in Figure 6c. Because the temperature increases very slowly after 120 h when h is 2 W·m⁻²·°C⁻¹, it is logical to record the temperature changes within 120 h and the wall temperature changes every 20 h, as shown in the Figure 6d.

Figure 6a depicts that the temperature rise of the tank wall goes through two stages under the adiabatic condition. The temperature rises slowly from 0 to 250 °C within 40 h, which is the initial stage of exothermic oxidation reaction of sulfur corrosion products. There is a large temperature span from 40 h to 70 h and the temperature ascends promptly to about 800 °C, which is the accelerated period of oxidation exothermic reaction. The difference between the heat source temperature and the wall temperature is very small at this stage, therefore if the temperature continues to rise without external intervention, it is possible to cause spontaneous combustion accidents.

The external environment will affect the temperature field of the storage tank when heat transfer exists between the outer wall of the tank and the air. When the h is equal to 0.5 W·m⁻²·°C⁻¹, Figure 6b shows that the temperature takes 60 h to rise to 250 °C and 120 h to reach 800 °C, in comparison with Figure 6a. The temperature rises slowly from 0 to 60 h, which is the initial stage of oxidation exothermic reaction, and increases sharply from 60 h to 120 h, which is the accelerated stage of oxidation heat release.

The external environment interferes more strongly with the internal heat transfer process when h is 1 W·m⁻²·°C⁻¹. The temperature rises slowly to 650 °C within 96 h, which indicates that it is the early stage of oxidation exothermic reaction. The span of temperature rise increases gradually from 96 h to 192 h, which is judged as the oxidation acceleration period. It is difficult to continue rising for the internal temperature after 192 h because of the interference of convective heat transfer of external air. Therefore, the heat transfer coefficient has a certain influence on the spontaneous combustion accidents of storage tanks.

The influence of external environment on internal heat transfer is more obvious when h is equal to 2 W·m⁻²·°C⁻¹. It is hard to continue heating up when the temperature of the storage tank rises to 115 °C. The heating time is concentrated from 0 to 60 h, and the temperature difference between the heat source and the outer wall surface is large. The analysis reason is that the internal heat source continues to release heat, while the convection heat transfer between the outer wall surface and the air continues to dissipate heat, resulting in a large temperature difference between the inner and outer walls of the storage tank.

Figure 7 shows the temperature rising trend of the outer wall surface of the storage tank under different values of h. The temperature of the outer wall of the storage tank rises rapidly in the adiabatic environment (without external interference), leading oil products to reach the ignition point and combustion temperature in a short time, which may cause spontaneous combustion easily. With the increase of air convective heat transfer coefficient, the external interference on temperature field enhances, which makes the wall temperature rise more and more slowly. The outer wall temperature tends to be flat after 200 h when h is 1 W·m⁻²·°C⁻¹, and the temperature stops increasing until about 150 °C when h is 2 W·m⁻²·°C⁻¹. Hu [32] pointed out that the greater the air convection coefficient is, the faster the heat transfer on the outer surface of the storage tank is, and the lower the outer surface temperature is. This is consistent with the simulation results. Thus, the disturbance of the external environment will affect the spontaneous combustion of the storage tank, hinder the heat transfer of the heat source, delay the time when the oil reaches the spontaneous combustion point, and even make it hard to reach the spontaneous combustion point. Therefore, the air convection heat transfer coefficient has a strong influence on the spontaneous combustion of the storage tank.

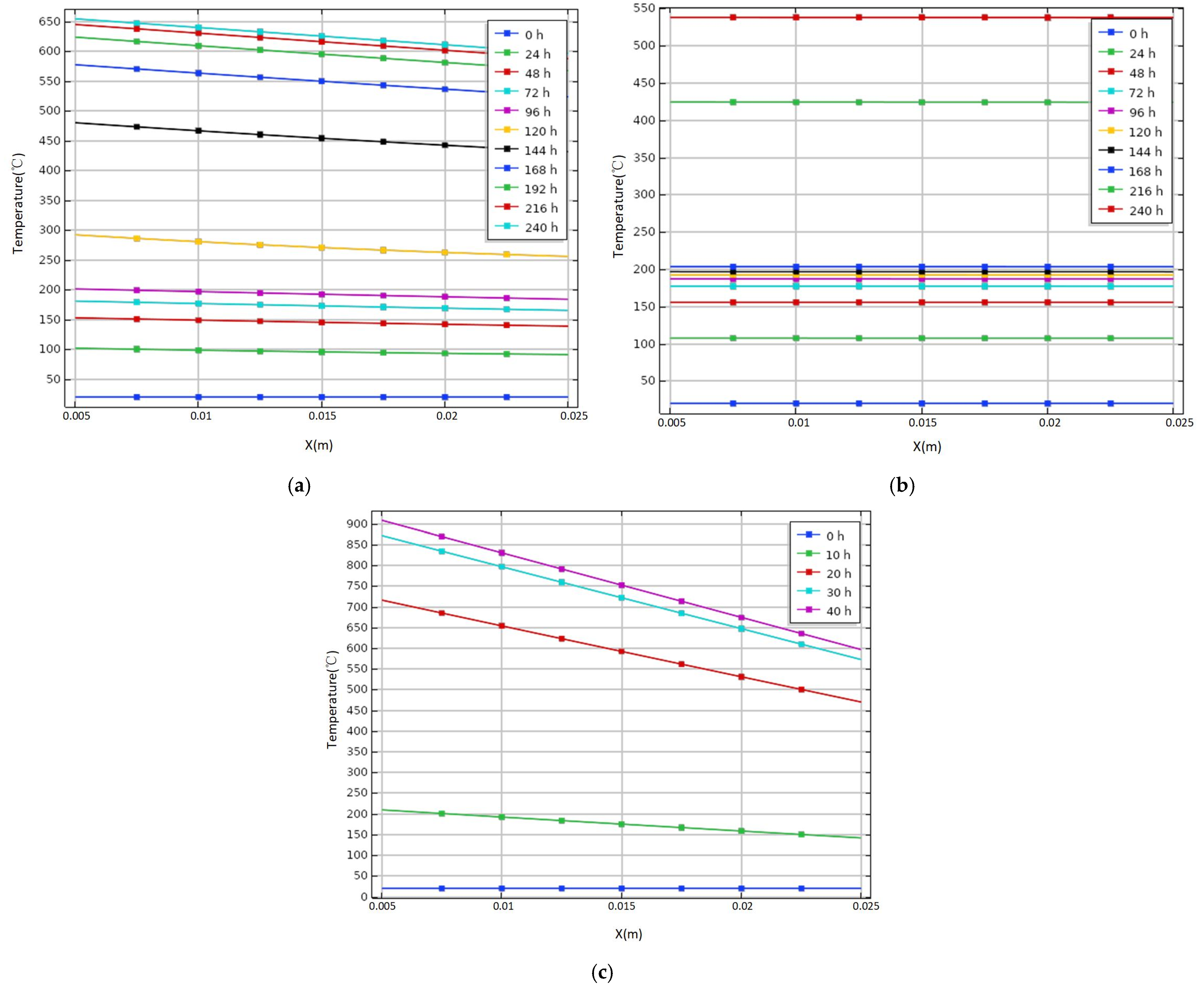
3.3. Variation of Temperature of Tank under Different Wall Materials

Yang [33] proposed that the difference of wall materials has a certain influence on spontaneous combustion accidents. The effect of the material was confirmed in the accident investigation [34]. The heat preservation and heat dissipation of wall materials affect the heat transfer process, resulting in different times when the heat source temperature is transferred to the outer wall. If the thermal insulation performance is too strong, the internal heat source will keep heating up, which makes it difficult to detect from the outside and take corresponding measures, resulting in temperature out of control. If the oil continues to heat up after reaching the ignition point, it is very likely to cause a fire. Thus, the appropriate material of storage tanks plays an important role in fire and disaster prevention.

In order to reduce corrosion, materials with strong anticorrosion ability are usually added to the wall material of the storage tanks, so the general anticorrosion material epoxy glass flake (material 1) was selected for analysis. According to the literature [35], 12MnNiVR steel plate produced in China and SPV490Q steel plate imported from Japan are used as wall materials, whose components are mainly low-carbon steel with 1% carbon content, so low-carbon steel was selected for analysis of material 2. According to the standard SH3010-2000 “technical specification for thermal insulation of petrochemical equipment and pipelines”, rock wool was selected as the wall thermal insulation material for finding out the function of the thermal insulation performance. The relevant thermodynamic parameters are shown in

Table 3. Parameters related to thermal analysis of different materials on tank wall.

Material Number	Wall Materials	Density (kg·m−3)	Specific Heat Capacity (J·kg−1·°C−1)	Thermal Conductivity (W·m−1·°C−1)
1	epoxy glass flake	3100	1581	0.2
2	low-carbon steel	7790	470	43.2
3	rock wool	120	750	0.037

.

The temperature changes in the heat transfer process of the storage tank when h is equal to 1 W·m⁻²·°C⁻¹ are shown in Figure 8, and the Materials 1, 2, and 3 correspond to (a), (b), and (c), respectively.

It can be seen from Figure 8a that the tank temperature rises rapidly after 120 h and reaches 650 °C after 240 h when material 1 (epoxy glass flake) is used as the wall material. Figure 8b shows that the temperature increases steadily to 200 °C within 168 h, rises rapidly after 168 h, and presents a dramatic growth every 24 h by using material 2 (low-carbon steel) as the wall material. After 240 h, the temperature of outer wall reaches about 530 °C. It can be analyzed from Figure 8c that the heat transfer process is more rapid by using material 3 (rock wool) as wall material, and the temperature increases greatly every 10 h, resulting in the outer wall surface reaching about 500 °C in 20 h and about 600 °C in 40 h. In addition, it can be seen from the inclination of the straight line that the temperature difference between the inner wall and the outer wall is the largest when rock wool is used as the wall material (when the temperature of the inner wall rises to 900 °C after 40 h, the temperature of the outer wall is only 600 °C), indicating that its thermal conductivity is the worst. Judging from this, if rock wool is simply used as the material of the storage tank, it is not conducive to the monitoring of spontaneous combustion and the corresponding emergency measures taken by the staff, which leads to the occurrence of spontaneous combustion accidents of the storage tank.

The temperature variation curve of the outer wall surface of each tank material with time is shown in Figure 9. There are obvious inflection points when the wall materials are epoxy glass flake and low-carbon steel. It can be inferred that the sulfur corrosion products are in the initial stage of oxidation exothermic reaction for the slow heating rate before the inflection point and it is in the accelerated period of oxidation exothermic reaction with rapid heating rate. If the corresponding measures are not taken in time during the accelerated period, it may easily lead to spontaneous combustion of the storage tank, causing serious accident consequences. Thus, it is easy to detect the process of oxidation exothermic reaction and targeted monitoring by using these two materials. Significantly, the heat transfer process is rapid and the temperature ascending velocity of the outer wall is too fast when rock wool is used as the material of the storage tank. Otherwise, the temperature difference between the inner wall and the outer wall is too large because of its strong thermal insulation performance and weak thermal conductivity, which leads to the outer wall surface temperature not being noticed when the internal temperature has reached the ignition point of the oil. In conclusion, it is not conducive to the prevention of spontaneous combustion to use material 3 compared with the first two.

4. Conclusions

In this paper, a variation of temperature field analysis model for spontaneous combustion accidents of sulfur-containing oil storage tanks is developed by COMSOL Multiphysics. The variation of temperature field of tank wall in the process of heat transfer under different influencing factors was analyzed. The conclusions are drawn as follows:

• The sulfur corrosion products do not release heat as a constant heat source. The oxidation exothermic reaction stage can be determined by the heat transfer on the wall when the heat release changes with time. The rising trend of temperature of the storage tank is different under various exothermic intensities, which has discrepant effects on the spontaneous combustion of the storage tank. Therefore, the different stages and corresponding exothermic trends of sulfur corrosion products should be considered in the actual process.
• The disturbance of external environment is expressed by air convection heat transfer coefficient h, and the heat transfer on the tank wall changes with different values of h. The temperature rises fastest under the adiabatic condition compared with other cases. With the increase of h, the heating rate slows down gradually. Due to the continuous heat exchange between the external circumstance and the wall, it is difficult for the temperature of the outer wall to continue ascending. Thus, it can be inferred that the air convection heat transfer coefficient will affect the temperature field of tank spontaneous combustion. The heat exchange between the external circumstance and the wall increases with the increment of the value of h, making it difficult for the temperature of spontaneous combustion to continue to rise, which can play a certain role in heat dissipation and prevention of heat accumulation. However, if the internal heat source continues to heat up, the temperature difference between the external wall temperature and the internal heat source is too large to detect in time, delaying the early warning time and missing the abnormal internal high temperature. Therefore, the appropriate air convection heat transfer coefficient has a great influence on the prevention and control of tank spontaneous combustion accidents.
• The temperature change of tank spontaneous combustion will be affected by the heat preservation and heat dissipation of wall materials. The wall heat transfer rate is slow when epoxy glass flake and low-carbon steel are used as storage tank materials. The former temperature rises slowly within 120 h, and the latter rises slowly within 168 h, so it is easy to prevent and control spontaneous combustion in these two periods. The heat transfer process on the wall is very speedy when rock wool is used as the material of the storage tank, so the outer wall can reach a high temperature of 600 °C in 40 h. Moreover, it is adverse to the detection of internal abnormal conditions because of the large temperature difference between inside and outside of the tank wall, so it is necessary to consider the difference of temperature conduction between different materials in temperature monitoring.